The Role of the Indian Ocean Basin-Wide and El Niño–Southern Oscillation Modes in Interannual Rainfall Variability over South America during Austral Summer
Abstract
1. Introduction
2. Data and Methods
3. Results
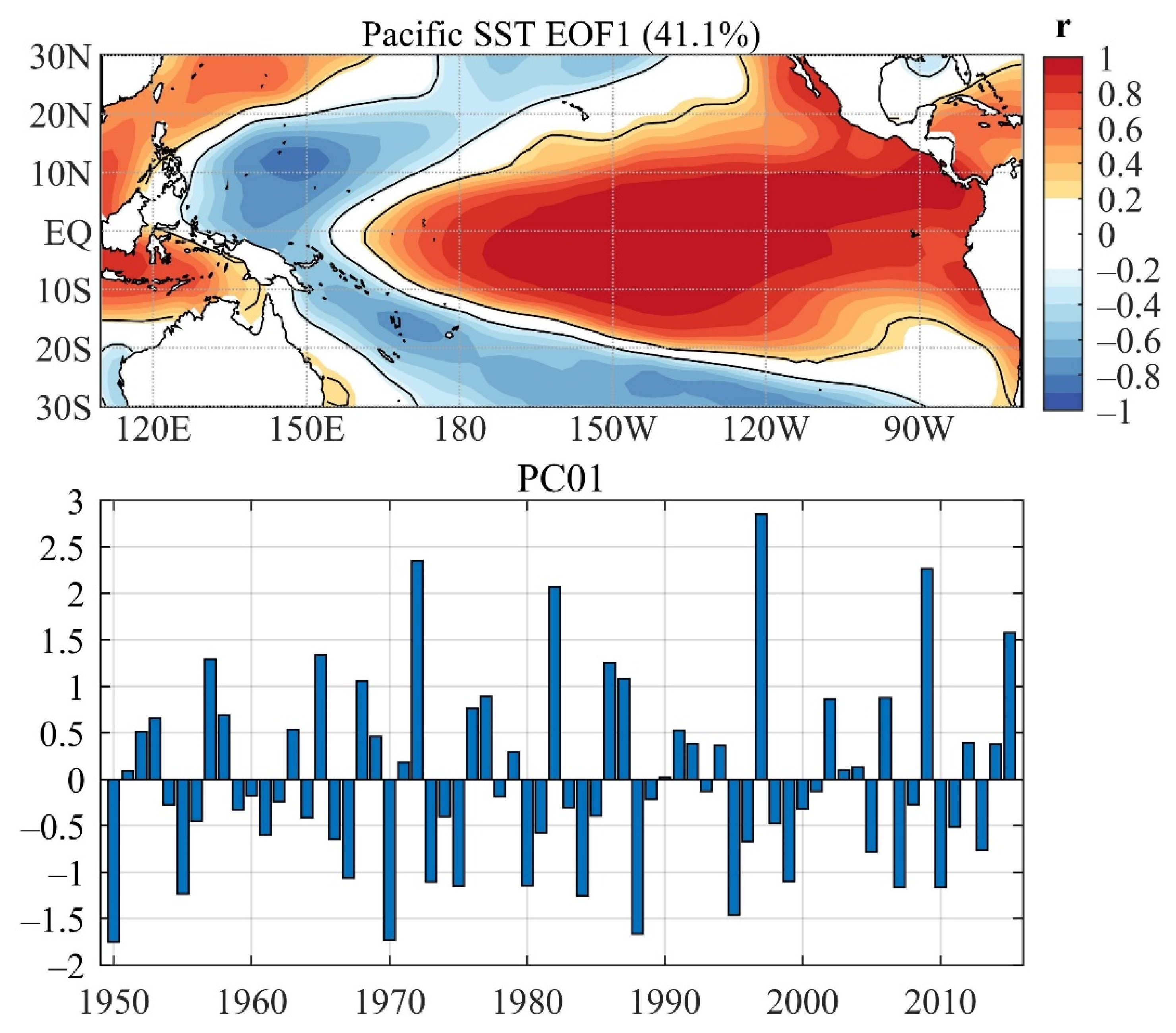
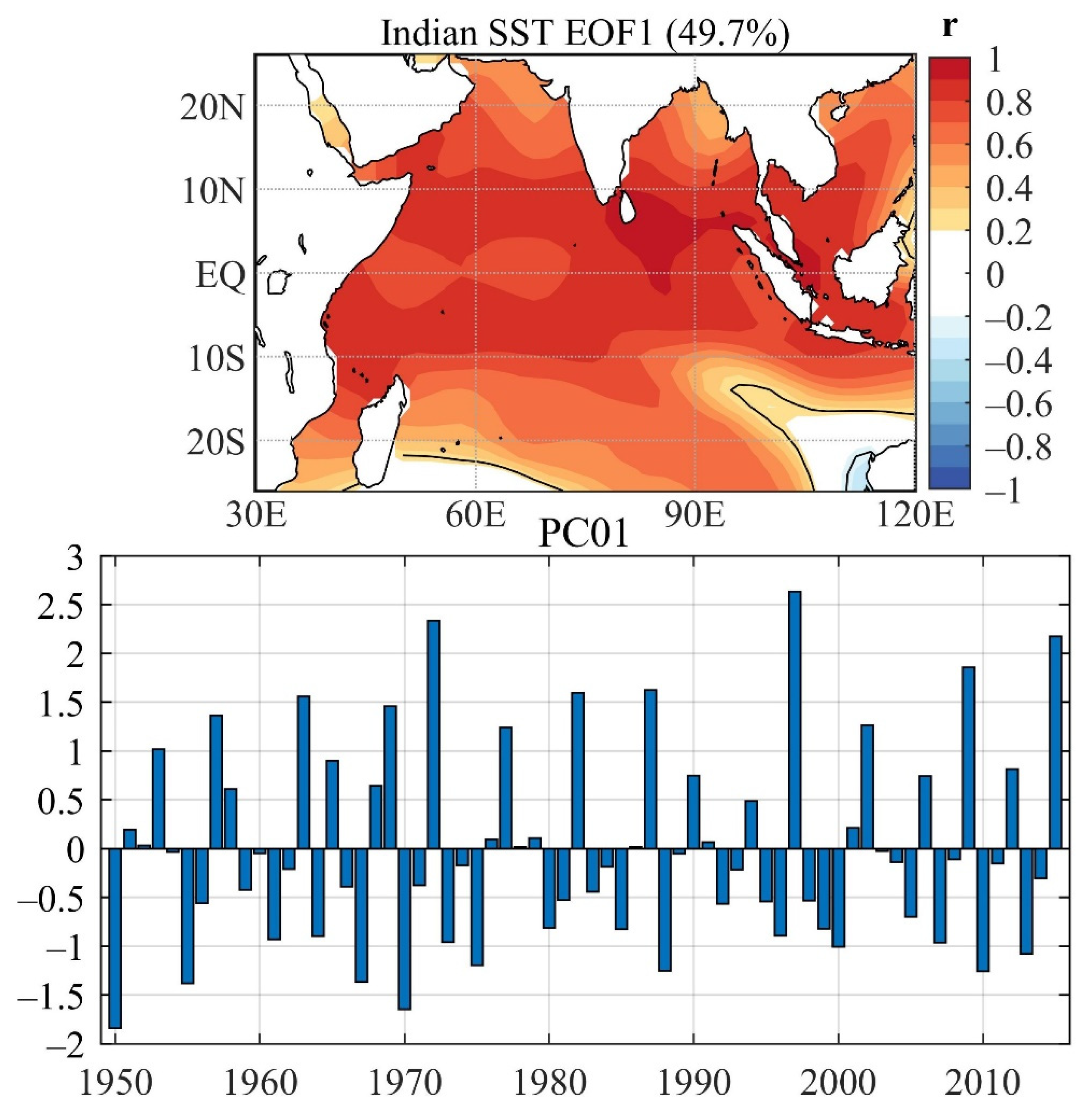
3.1. EOF Modes of the SST Anomalies in the Tropical Indian and Pacific Oceans
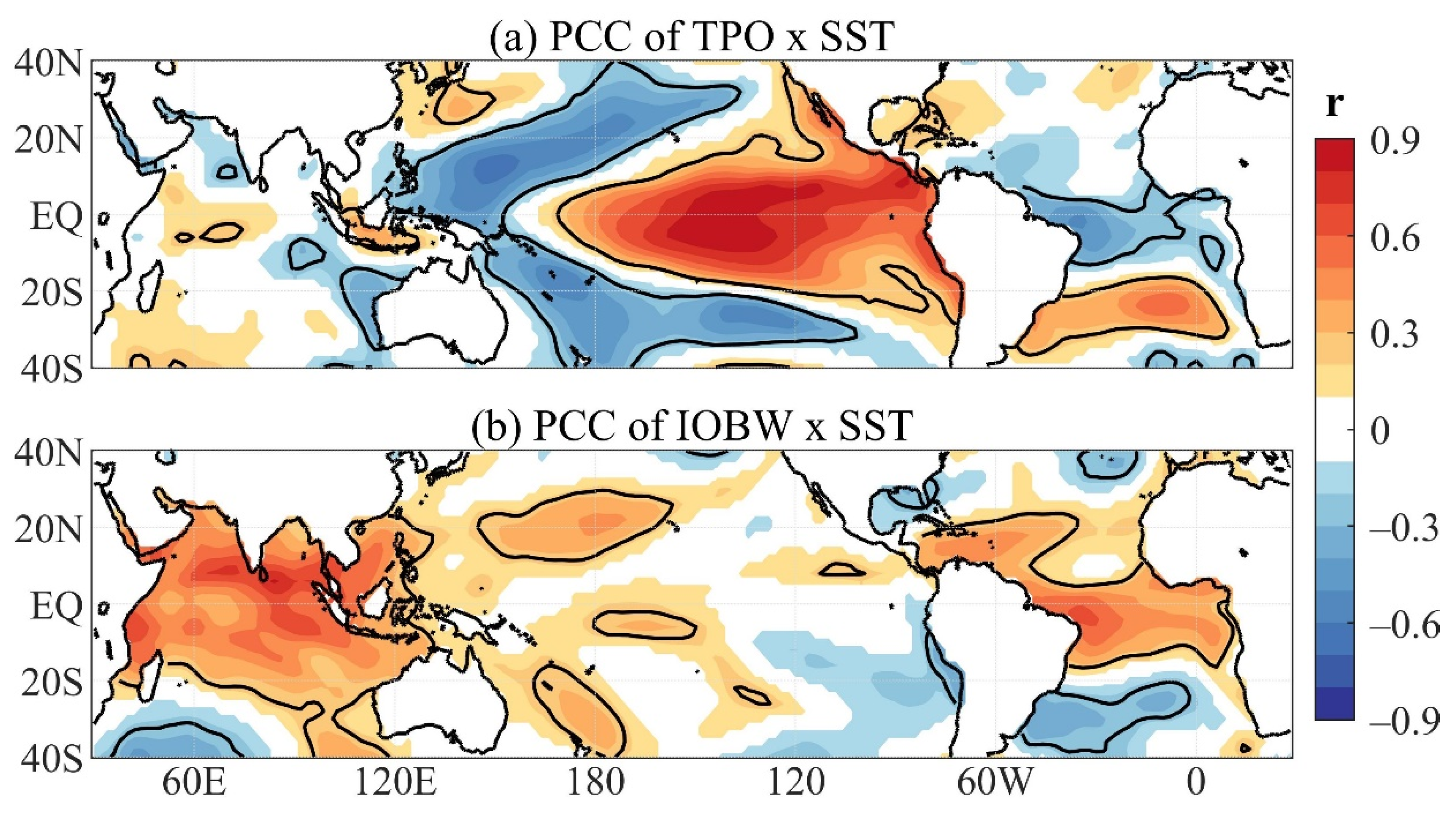
3.2. SST Anomaly Patterns
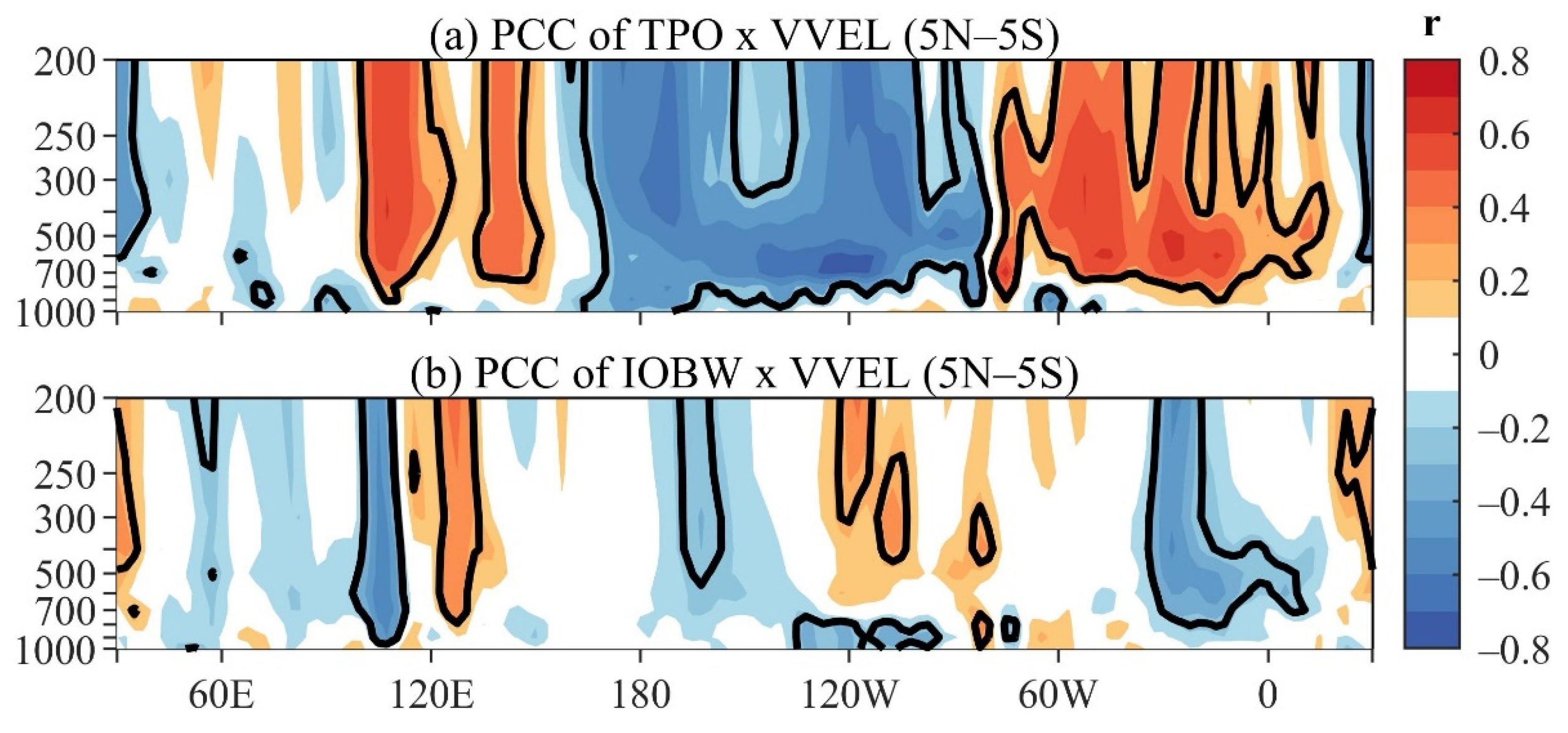
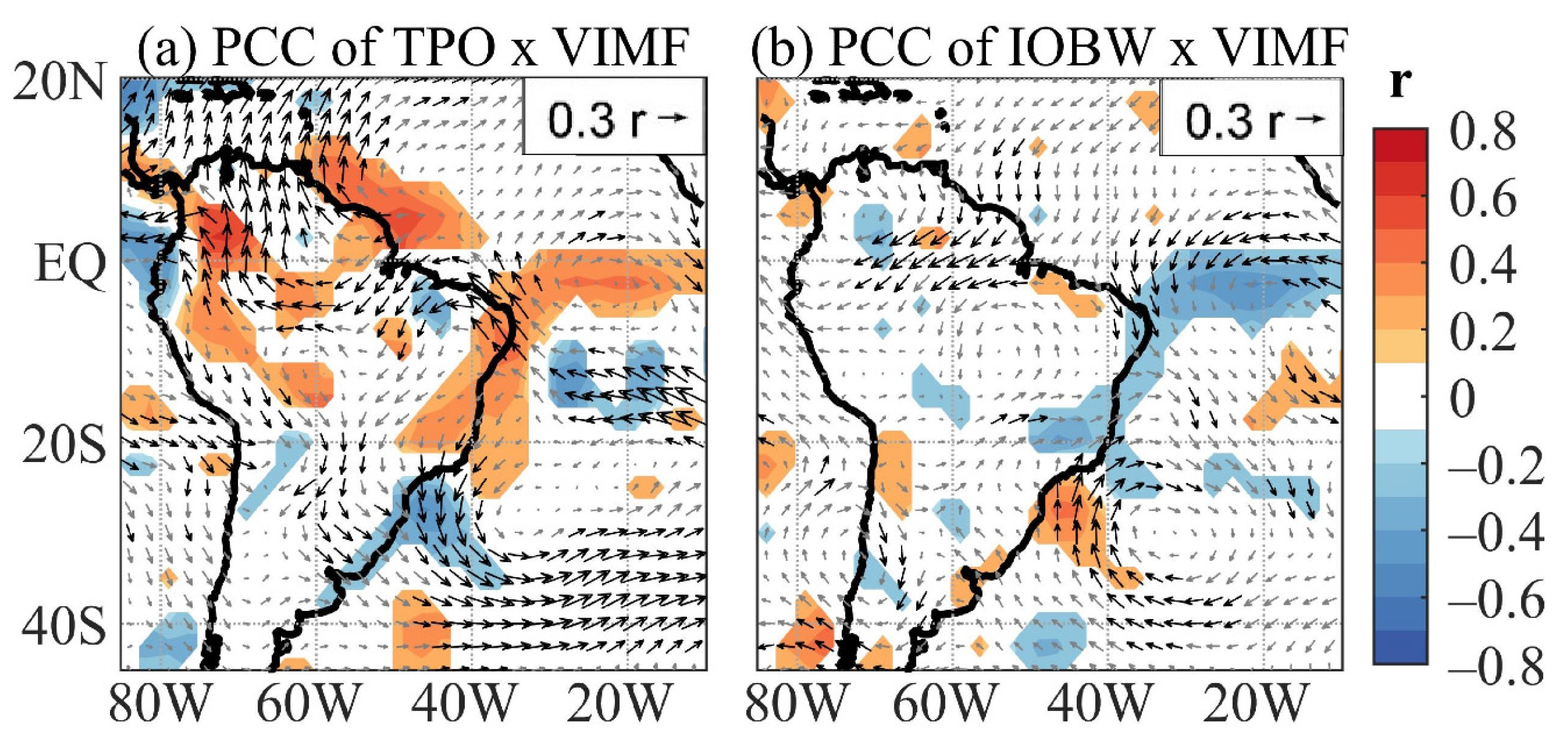
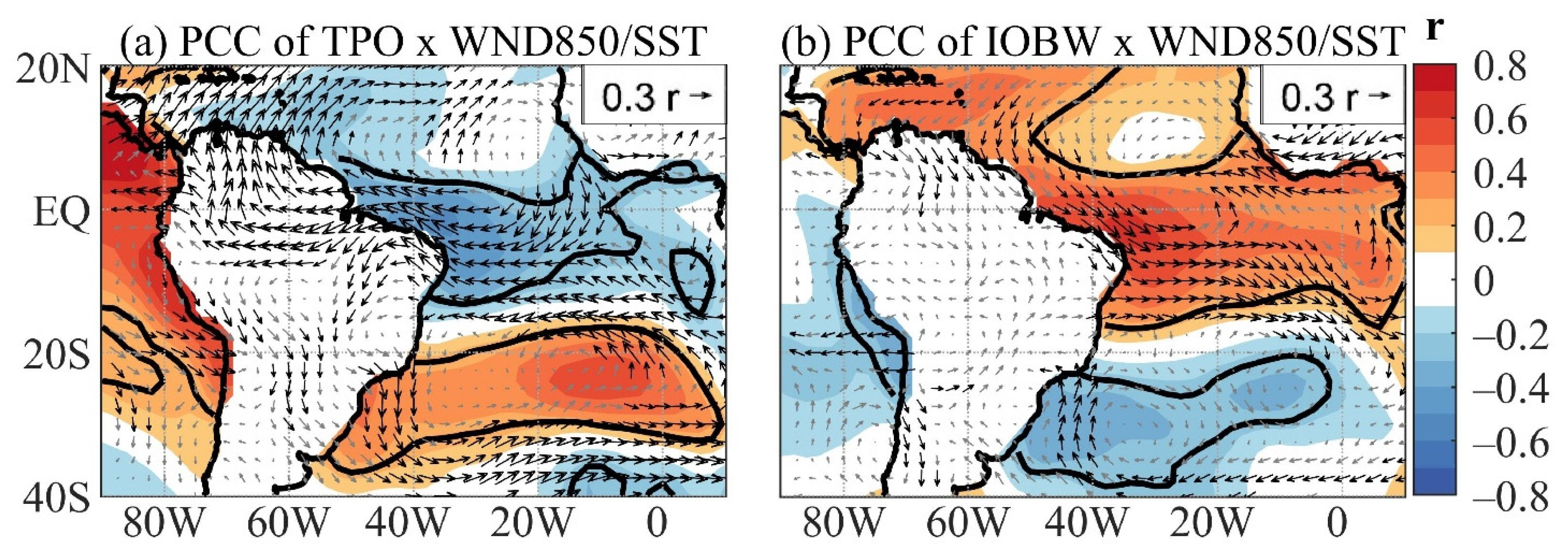
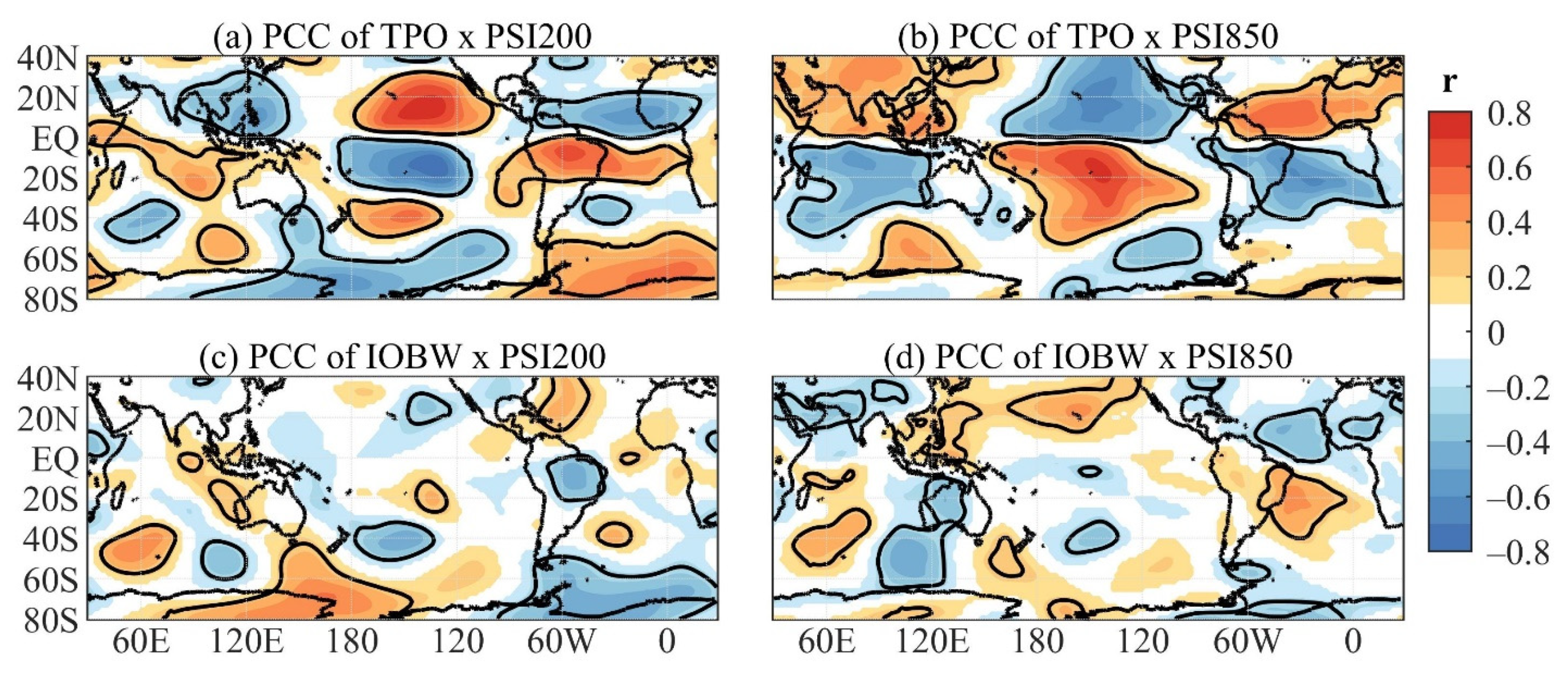
3.3. Atmospheric Circulation and Rainfall Anomaly Patterns
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, J.; Lau, K.M. Principal modes of interannual and decadal variability of summer rainfall over South America. Int. J. Climatol. 2001, 21, 1623–1644. [Google Scholar] [CrossRef]
- Grimm, A.M.; Ambrizzi, T. Teleconnections into South America from the Tropics and Extratropics on Interannual and Intraseasonal Timescales. In Past Climate Variability in South America and Surrounding Regions. Developments in Paleoenvironmental Research; Vimeux, F., Sylvestre, F.K.M., Eds.; Springer: Dordrecht, The Netherlands, 2009; pp. 159–191. ISBN 9789048126729. [Google Scholar]
- Mo, K.C.; Higgins, R.W. The Pacific-South American modes and tropical convection during the Southern Hemisphere winter. Mon. Weather Rev. 1998, 126, 1581–1596. [Google Scholar] [CrossRef]
- Paegle, J.N.; Mo, K.C. Linkages between summer rainfall variability over South America and sea surface temperature anomalies. J. Clim. 2002, 15, 1389–1407. [Google Scholar] [CrossRef]
- Ropelewski, C.F.; Halpert, M.S. Global and Regional scale precipitation patterns associated with the El Niño-Southern Oscillation. Mon. Weather Rev. 1987, 115, 1606–1626. [Google Scholar] [CrossRef]
- Kousky, V.E.; Kagano, M.T.; Cavalcanti, I.F.A. A review of the Southern Oscillation: Oceanic-atmospheric circulation changes and related rainfall anomalies. Tellus A 1984, 36A, 490–504. [Google Scholar] [CrossRef]
- Kayano, M.T.; Rao, V.B.; Moura, A.D. Tropical circulations and the associated rainfall anomalies during two contrasting years. J. Climatol. 1988, 8, 477–488. [Google Scholar] [CrossRef]
- Grimm, A.M.; Ferraz, S.E.T.; Gomes, J. Precipitation Anomalies in Southern Brazil Associated with El Niño and La Niña Events. J. Clim. 1998, 11, 2863–2880. [Google Scholar] [CrossRef]
- Grimm, A.M. The El Niño Impact on the Summer Monsoon in Brazil: Regional Processes versus Remote Influences. J. Clim. 2003, 16, 263–280. [Google Scholar] [CrossRef]
- Grimm, A.M.; Pal, J.S.; Giorgi, F. Connection between spring conditions and peak summer monsoon rainfall in South America: Role of soil moisture, surface temperature, and topography in eastern Brazil. J. Clim. 2007, 20, 5929–5945. [Google Scholar] [CrossRef]
- Kousky, V.E.; Ropelewski, C.F. Extremes in the Southern Oscillation and their Relationship to Precipitation Anomalies with Emphasis on the South American Region. Rev. Bras. Meteorol. 1989, 4, 351–363. [Google Scholar]
- Cai, W.; McPhaden, M.J.; Grimm, A.M.; Rodrigues, R.R.; Taschetto, A.S.; Garreaud, R.D.; Dewitte, B.; Poveda, G.; Ham, Y.-G.; Santoso, A.; et al. Climate impacts of the El Niño–Southern Oscillation on South America. Nat. Rev. Earth Environ. 2020, 1, 215–231. [Google Scholar] [CrossRef]
- Wang, C. Three-ocean interactions and climate variability: A review and perspective. Clim. Dyn. 2019, 53, 5119–5136. [Google Scholar] [CrossRef]
- Chambers, D.P.; Tapley, B.D.; Stewart, R.H. Anomalous warming in the Indian Ocean coincident with El Niño. J. Geophys. Res. Ocean 1999, 104, 3035–3047. [Google Scholar] [CrossRef]
- Annamalai, H.; Xie, S.P.; McCreary, J.P.; Murtugudde, R. Impact of Indian Ocean Sea surface temperature on developing El Niño. J. Clim. 2005, 18, 302–320. [Google Scholar] [CrossRef]
- Xie, S.P.; Hu, K.; Hafner, J.; Tokinaga, H.; Du, Y.; Huang, G.; Sampe, T. Indian Ocean capacitor effect on Indo-Western Pacific climate during the summer following El Niño. J. Clim. 2009, 22, 730–747. [Google Scholar] [CrossRef]
- Taschetto, A.S.; Ambrizzi, T. Can Indian Ocean SST anomalies influence South American rainfall? Clim. Dyn. 2012, 38, 1615–1628. [Google Scholar] [CrossRef]
- Klein, S.A.; Soden, B.J.; Lau, N.-C. Remote Sea Surface Temperature Variations during ENSO: Evidence for a Tropical Atmospheric Bridge. J. Clim. 1999, 12, 917–932. [Google Scholar] [CrossRef]
- Deser, C.; Alexander, M.A.; Xie, S.P.; Phillips, A.S. Sea surface temperature variability: Patterns and mechanisms. Ann. Rev. Mar. Sci. 2010, 2, 115–143. [Google Scholar] [CrossRef] [PubMed]
- Lau, N.C.; Nath, M.J. Atmosphere-ocean variations in the Indo-Pacific sector during ENSO episodes. J. Clim. 2003, 16, 3–20. [Google Scholar] [CrossRef]
- Saji, N.H.; Goswani, B.N.; Vinayachandran, P.N.; Yamagat, T. A dipole mode in the tropical Indian Ocean. Nature 1999, 401, 360–363. [Google Scholar] [CrossRef] [PubMed]
- Webster, P.J.; Moore, A.M.; Loschnigg, J.P.; Leben, R.R. Coupled ocean-atmosphere dynamics in the Indian Ocean during 1997–1998. Nature 1999, 401, 356–360. [Google Scholar] [CrossRef]
- Ashok, K.; Guan, Z.; Yamagata, T. Impact of the Indian Ocean dipole on the relationship between the Indian monsoon rainfall and ENSO. Geophys. Res. Lett. 2001, 28, 4499–4502. [Google Scholar] [CrossRef]
- Clark, C.O.; Webster, P.J.; Cole, J.E. Interdecadal variability of the relationship between the Indian Ocean zonal mode and East African coastal rainfall anomalies. J. Clim. 2003, 16, 548–554. [Google Scholar] [CrossRef]
- Cai, W.; Cowan, T.; Raupach, M. Positive Indian Ocean Dipole events precondition southeast Australia bushfires. Geophys. Res. Lett. 2009, 36, 1–6. [Google Scholar] [CrossRef]
- Saji, N.H.; Ambrizzi, T.; Ferraz, S.E.T. Indian Ocean Dipole mode events and austral surface air temperature anomalies. Dyn. Atmos. Ocean 2005, 39, 87–101. [Google Scholar] [CrossRef]
- Chan, S.C.; Behera, S.K.; Yamagata, T. Indian Ocean Dipole influence on South American rainfall. Geophys. Res. Lett. 2008, 35, 10–14. [Google Scholar] [CrossRef]
- Drumond, A.R.d.M.; Ambrizzi, T. The role of the South Indian and Pacific oceans in South American monsoon variability. Theor. Appl. Climatol. 2008, 94, 125–137. [Google Scholar] [CrossRef]
- González, M.H.; Skansi, M.M.; Losano, F. A statistical study of seasonal winter rainfall prediction in the Comahue region (Argentina). Atmosfera 2010, 23, 277–294. [Google Scholar]
- González, M.H.; Vera, C.S. On the interannual wintertime rainfall variability in the Southern Andes. Int. J. Climatol. 2010, 30, 643–657. [Google Scholar] [CrossRef]
- Berman, A.L.; Silvestri, G.; Compagnucci, R.; Velasco Herrera, V. Oceanic influence on southernmost South American precipitation. Atmosfera 2012, 25, 217–233. [Google Scholar]
- Han, W.; Vialard, J.; McPhaden, M.J.; Lee, T.; Masumoto, Y.; Feng, M.; De Ruijter, W.P.M. Indian ocean decadal variability: A review. Bull. Am. Meteorol. Soc. 2014, 95, 1679–1703. [Google Scholar] [CrossRef]
- Cai, W.; Wu, L.; Lengaigne, M.; Li, T.; McGregor, S.; Kug, J.S.; Yu, J.Y.; Stuecker, M.F.; Santoso, A.; Li, X.; et al. Pantropical climate interactions. Science 2019, 363, eaav4236. [Google Scholar] [CrossRef]
- Alory, G.; Wijffels, S.; Meyers, G. Observed temperature trends in the Indian Ocean over 1960–1999 and associated mechanisms. Geophys. Res. Lett. 2007, 34, 2–7. [Google Scholar] [CrossRef]
- Du, Y.; Xie, S.P. Role of atmospheric adjustments in the tropical Indian Ocean warming during the 20th century in climate models. Geophys. Res. Lett. 2008, 35, 2–6. [Google Scholar] [CrossRef]
- Dong, L.; Zhou, T.; Wu, B. Indian Ocean warming during 1958–2004 simulated by a climate system model and its mechanism. Clim. Dyn. 2014, 42, 203–217. [Google Scholar] [CrossRef]
- Roxy, M.K.; Ritika, K.; Terray, P.; Masson, S. The curious case of Indian Ocean warming. J. Clim. 2014, 27, 8501–8509. [Google Scholar] [CrossRef]
- Rao, V.B.; Hada, K. Characteristics of rainfall over Brazil: Annual variations and connections with the Southern Oscillation. Theor. Appl. Climatol. 1990, 42, 81–91. [Google Scholar] [CrossRef]
- Rasmusson, E.M.; Arkin, P.A. Interannual climate variability associated with the El Niño/ Southern Oscillation. Elsevier Oceanogr. Ser. 1985, 40, 697–725. [Google Scholar]
- Huang, B.; Thorne, P.W.; Banzon, V.F.; Boyer, T.; Chepurin, G.; Lawrimore, J.H.; Menne, M.J.; Smith, T.M.; Vose, R.S.; Zhang, H.M. Extended reconstructed Sea surface temperature, Version 5 (ERSSTv5): Upgrades, validations, and intercomparisons. J. Clim. 2017, 30, 8179–8205. [Google Scholar] [CrossRef]
- National Oceanic and Atmospheric Administration; Extended Reconstructed Sea Surface Temperature (NOAA/ERSST) SST Data. Available online: https://www.esrl.noaa.gov/psd/data/gridded/ (accessed on 1 March 2018).
- Schneider, U.; Becker, A.; Finger, P.; Meyer-Christoffer, A.; Ziese, M.; Rudolf, B. GPCC’s new land surface precipitation climatology based on quality-controlled in situ data and its role in quantifying the global water cycle. Theor. Appl. Climatol. 2014, 115, 15–40. [Google Scholar] [CrossRef]
- Global Precipitation Climatology Centre (GPCC) Monthly Gridded Precipitation Data. Available online: https://www.esrl.noaa.gov/psd/data/gridded/data.gpcc.html (accessed on 3 May 2019).
- Kalnay, E.; Kanamitsu, M.; Kistler, R.; Collins, W.; Deaven, D.; Gandin, L.; Iredell, M.; Saha, S.; White, G.; Woollen, J.; et al. The NCEP/ NCAR reanalysis project. Bull. Amer. Meteor. Soc. 1996, 77, 437–471. [Google Scholar] [CrossRef]
- National Center for Environmental Prediction; National Center for Atmospheric Research (NCEP/NCAR) Reanalysis-I Project Dataset. Available online: https://psl.noaa.gov/data/gridded/ (accessed on 3 September 2020).
- Peixoto, J.P.; Oort, A.H. Physics of Climate; MIT Press: San Diego, CA, USA, 1992; ISBN 7287064. [Google Scholar]
- Torrence, C.; Compo, G.P. A Practical Guide to Wavelet Analysis Christopher. Bull. Am. Meteorol. Soc. 1998, 97, 61–78. [Google Scholar] [CrossRef]
- North, G.L.; Bell, T.L.; Chalan, R.F. Sampling Errors in the Estimtion of Empirical Othogonal Functions. Mon. Weather Rev. 1982, 110, 699–706. [Google Scholar] [CrossRef]
- Panofsky, H.G.; Brier, G.W. Some Applications of Statistics to Meteorology; Earth and Mineral Sciences Continuing Education, College of Earth and Mineral Sciences, Ed.; Pennsylvania State University: Pennsylvania, PA, USA, 1968. [Google Scholar]
- Rasmusson, E.M.; Wallace, J.M. Meteorological Aspects of the El Niño/Southern Oscillation. Science 1983, 222, 1195–1202. [Google Scholar] [CrossRef]
- An, S.I.; Jin, F.F. Nonlinearity and asymmetry of ENSO. J. Clim. 2004, 17, 2399–2412. [Google Scholar] [CrossRef]
- Bombardi, R.J.; Carvalho, L.M.V. The South Atlantic dipole and variations in the characteristics of the South American Monsoon in the WCRP-CMIP3 multi-model simulations. Clim. Dyn. 2011, 36, 2091–2102. [Google Scholar] [CrossRef][Green Version]
- Kayano, M.T.; Andreoli, R.V.; Ferreira de Souza, R.A. Relations between ENSO and the South Atlantic SST modes and their effects on the South American rainfall. Int. J. Climatol. 2013, 33, 2008–2023. [Google Scholar] [CrossRef]
- Matsuno, T. Quasi-Geostrophic Motions in the Equatorial Area. J. Meteorol. Soc. Japan. Ser. II 1966, 44, 25–43. [Google Scholar] [CrossRef]
- Gill, A.E. Some simple solutions for heat-induced tropical circulation. Q. J. R. Meteorol. Soc. 1980, 106, 447–462. [Google Scholar] [CrossRef]
- Hoskins, B.J.; Ambrizzi, T. Rossby wave propagation on a realistic longitudinally varying flow. J. Atmos. Sci. 1993, 50, 1661–1671. [Google Scholar] [CrossRef]
- Abid, M.A.; Ashfaq, M.; Kucharski, F.; Evans, K.J.; Almazroui, M. Tropical Indian Ocean mediates ENSO influence over central Southwest Asia during the Wet Season. Geophys Res Lett. 2020, 47, e2020GL089308. [Google Scholar] [CrossRef]
- Abid, M.A.; Kucharski, F.; Molteni, F.; Kang, I.S.; Tompkins, A.M.; Almazroui, M. Separating the Indian and Pacific Ocean impacts on the Euro-Atlantic response to ENSO and its transition from early to late winter. J. Clim. 2021, 34, 1531–1548. [Google Scholar] [CrossRef]
- Liao, H.; Wang, C. Sea Surface temperature Anomalies in the Western Indian Ocean as a Trigger for Atlantic Niño Events. Geophys. Res Lett. 2021, 48, e2021GL092489. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kayano, M.T.; Andreoli, R.V.; Cerón, W.L.; Souza, R.A.F. The Role of the Indian Ocean Basin-Wide and El Niño–Southern Oscillation Modes in Interannual Rainfall Variability over South America during Austral Summer. Atmosphere 2021, 12, 1094. https://doi.org/10.3390/atmos12091094
Kayano MT, Andreoli RV, Cerón WL, Souza RAF. The Role of the Indian Ocean Basin-Wide and El Niño–Southern Oscillation Modes in Interannual Rainfall Variability over South America during Austral Summer. Atmosphere. 2021; 12(9):1094. https://doi.org/10.3390/atmos12091094
Chicago/Turabian StyleKayano, Mary T., Rita V. Andreoli, Wilmar L. Cerón, and Rodrigo A. F. Souza. 2021. "The Role of the Indian Ocean Basin-Wide and El Niño–Southern Oscillation Modes in Interannual Rainfall Variability over South America during Austral Summer" Atmosphere 12, no. 9: 1094. https://doi.org/10.3390/atmos12091094
APA StyleKayano, M. T., Andreoli, R. V., Cerón, W. L., & Souza, R. A. F. (2021). The Role of the Indian Ocean Basin-Wide and El Niño–Southern Oscillation Modes in Interannual Rainfall Variability over South America during Austral Summer. Atmosphere, 12(9), 1094. https://doi.org/10.3390/atmos12091094





