Analysis of Rainfall Erosivity Trends 1980–2018 in a Complex Terrain Region (Abruzzo, Central Italy) from Rain Gauges and Gridded Datasets
Abstract
1. Introduction
2. Materials and Methods
2.1. Rainfall Data
2.2. Calculation of the Indices MFI and PCI, and of the USLE Erosivity Factor R
3. Results
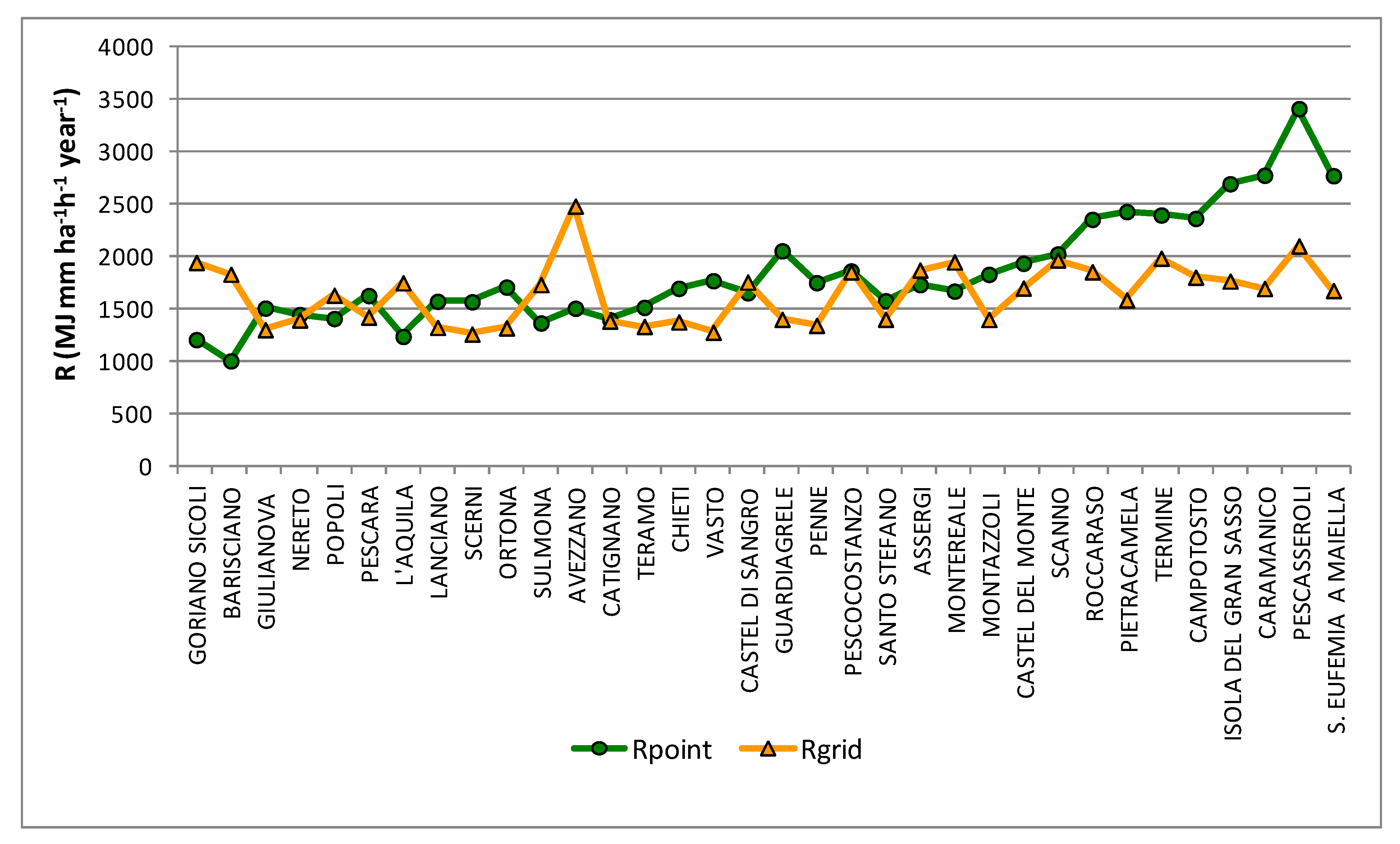
3.1. Relationships between Rainfall Erosivity Factor R and MFI, PCI, and SI
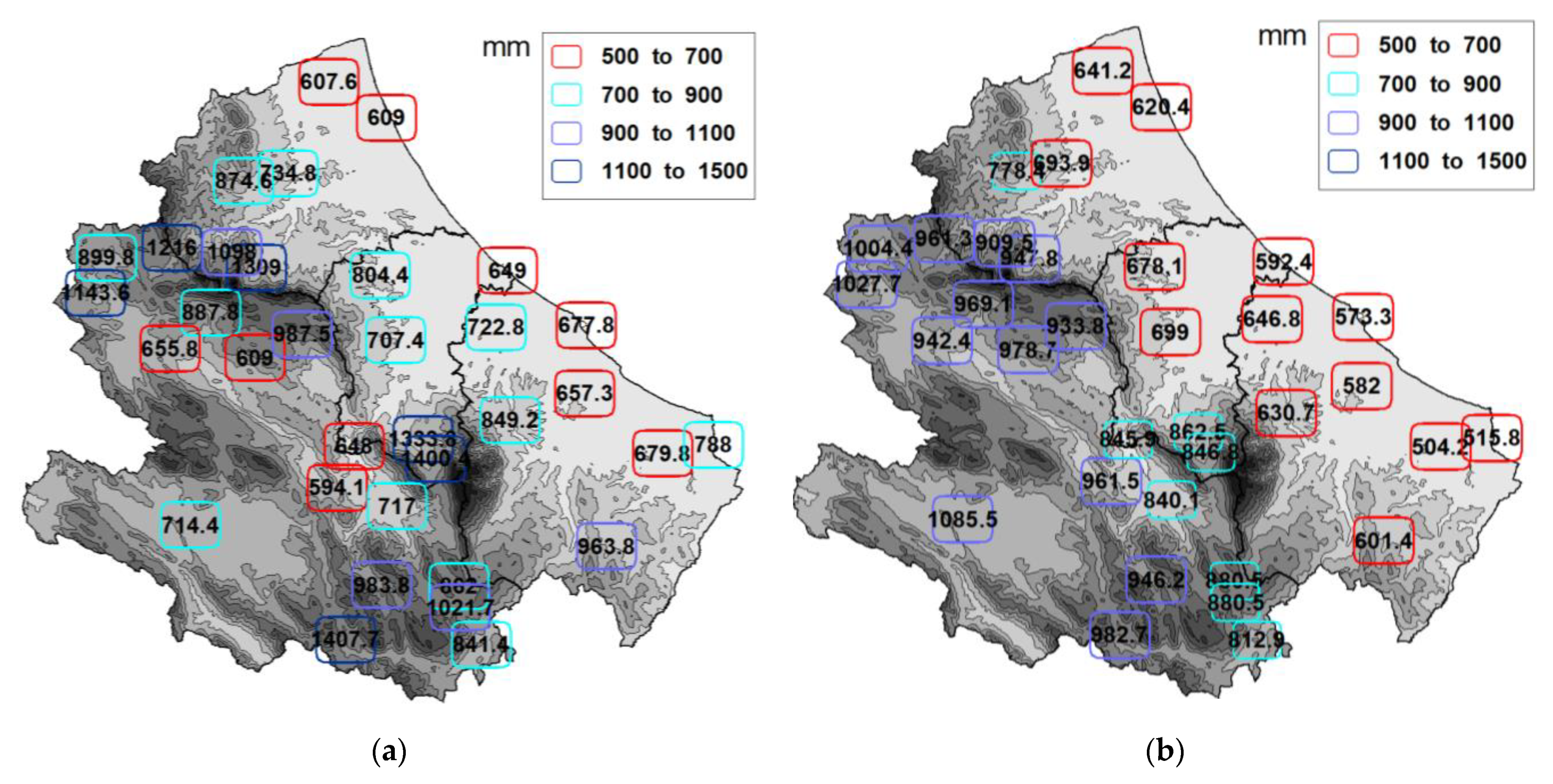
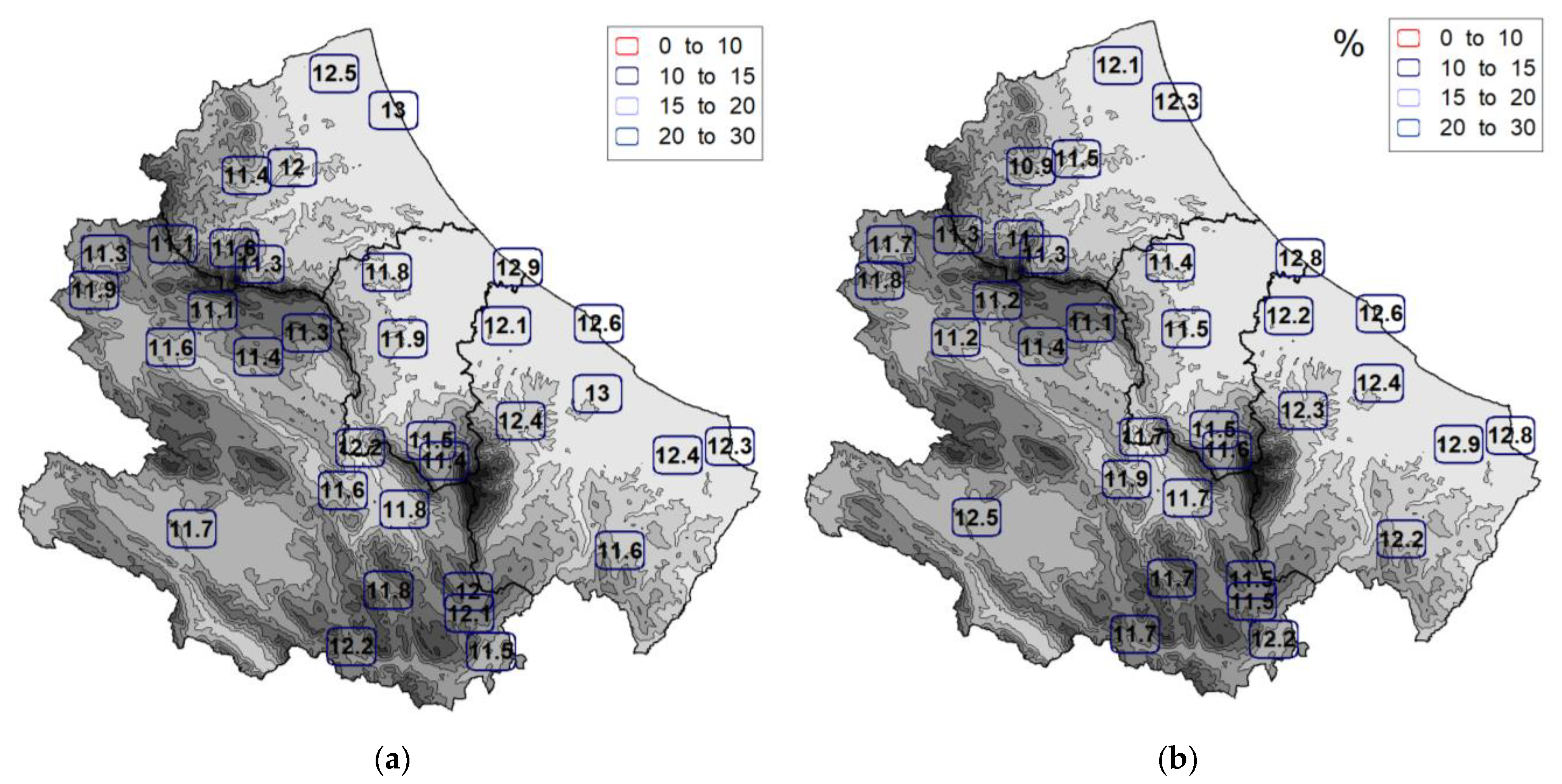
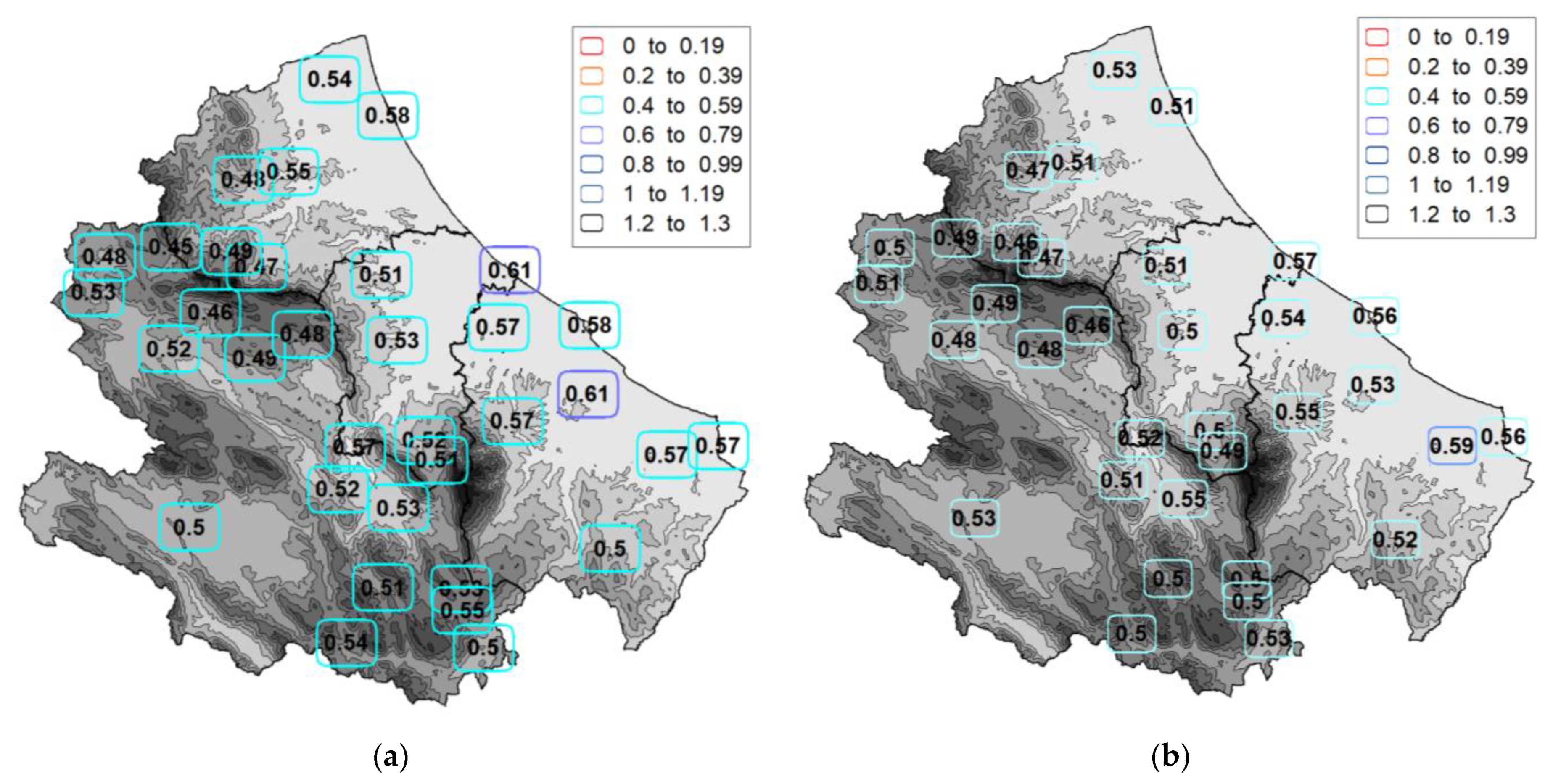
3.2. Spatial Variability of MFI, PCI, and SI
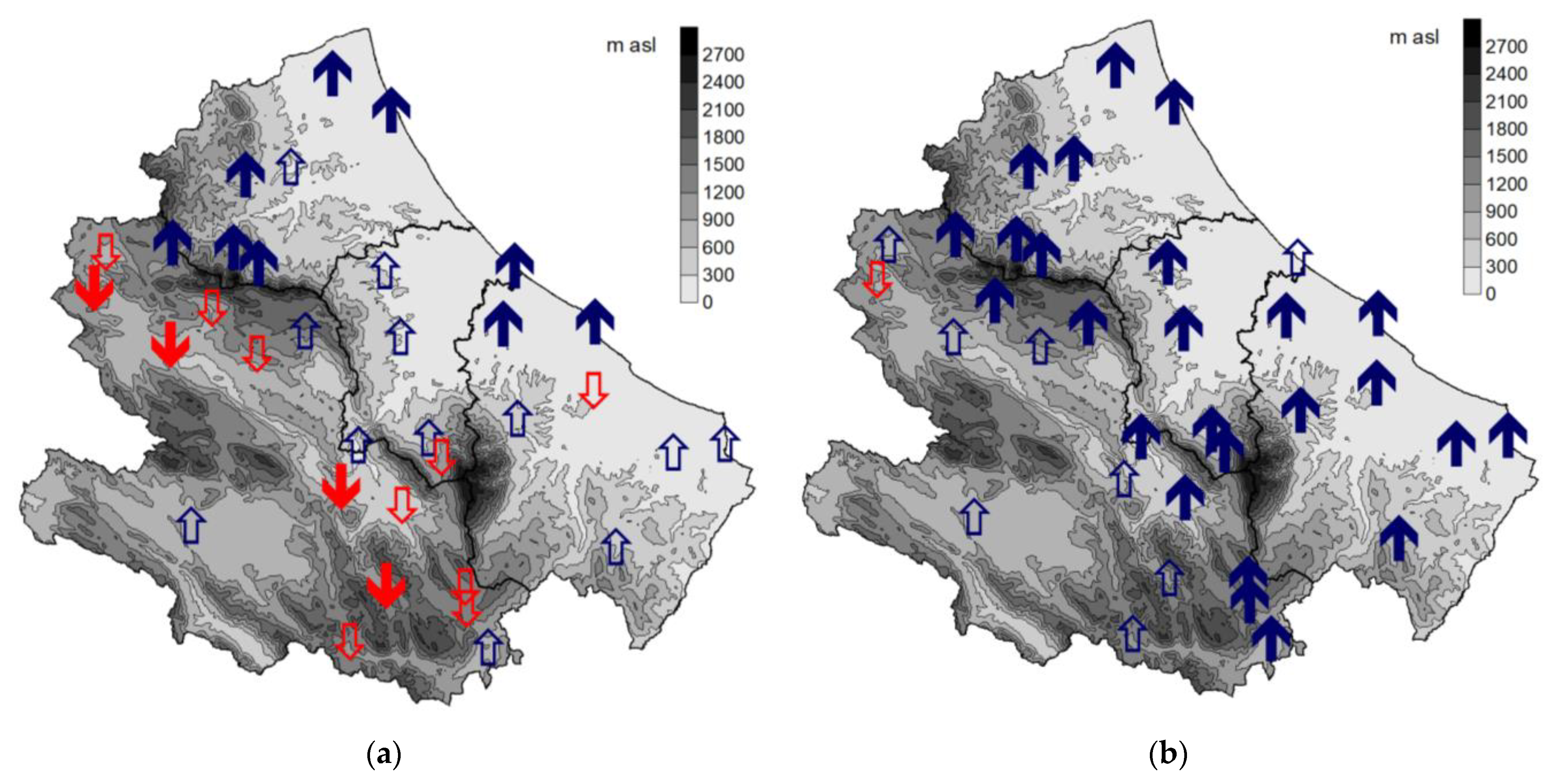
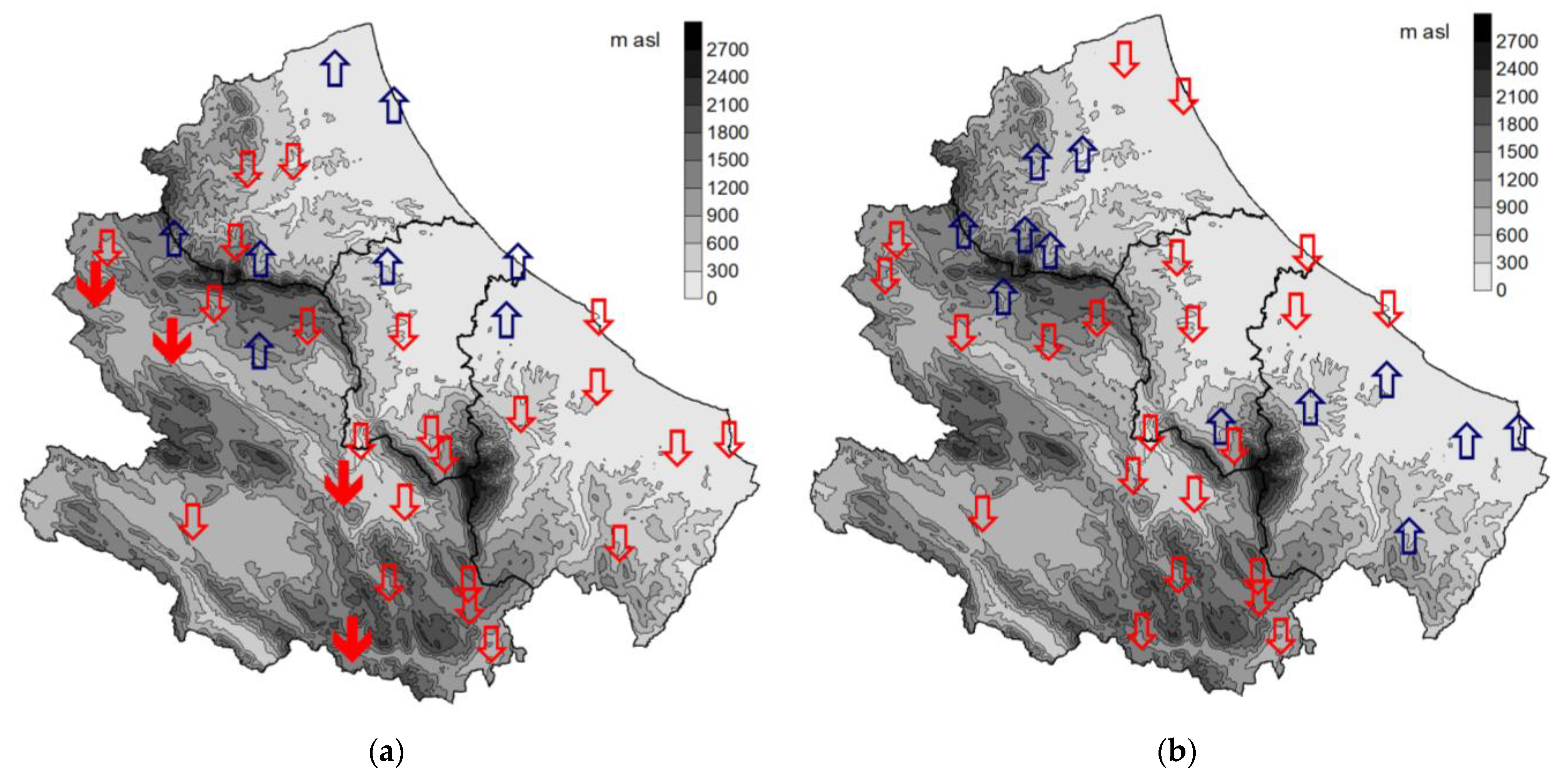
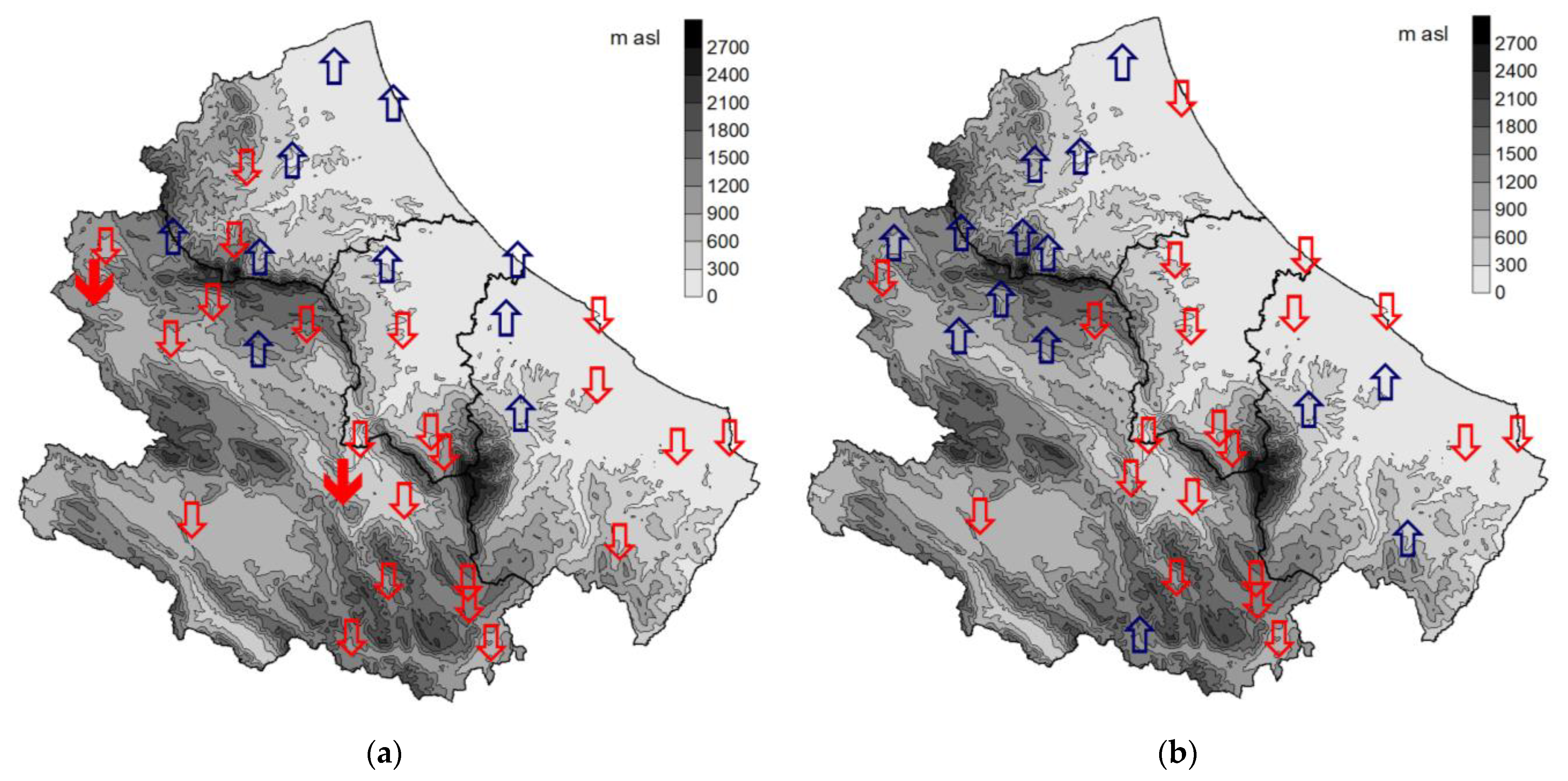
3.3. Trend Analysis of MFI, PCI, and SI
4. Discussion and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Mann–Kendall Nonparametric Trend Test
Appendix A.2. Sen Slope
References
- Dvořák, J.; Novák, L.P. Developments in Soil Science; Elsevier: Amsterdam, The Netherlands, 1994; Volume 23, p. 13. ISBN 978-0-444-98792-1. [Google Scholar]
- Wischmeier, W.H.; Smith, D.D. Predicting Rainfall Erosion Losses: A Guide to Conservation Planning [USA]. Department of Agriculture Handbook (USA). 1978. Available online: https://naldc.nal.usda.gov/download/CAT79706928/PDF (accessed on 20 May 2021).
- Panagos, P.; Ballabio, C.; Borrelli, P.; Meusburger, K.; Klik, A.; Rousseva, S.; Tadić, M.P.; Michaelides, S.; Hrabalíková, M.; Olsen, P.; et al. Rainfall erosivity in Europe. Sci. Total Environ. 2015, 511, 801–814. [Google Scholar] [CrossRef] [PubMed]
- Renard, K.G.; Agricultural Research Service, W.; Foster, G.R.; Weesies, G.A.; McCool, D.K.; Yoder, D.C. Predicting Soil Erosion by Water: A Guide to Conservation Planning with the Revised Universal Soil Loss Equation (RUSLE); USDA: Washington, DC, USA, 1997.
- Yin, S.; Nearing, M.A.; Borrelli, P.; Xue, X. Rainfall Erosivity: An Overview of Methodologies and Applications. Vadose Zone J. 2017, 16, 1–6. [Google Scholar] [CrossRef]
- Arnoldus, H.M.J. An approximation of the rainfall factor in the Universal Soil Loss Equation. Approx. Rainfall Factor Univers. Soil Loss Equ. 1980, 6, 127–132. [Google Scholar]
- Oliver, J.E. Monthly Precipitation Distribution: A Comparative Index. Prof. Geogr. 1980, 32, 300–309. [Google Scholar] [CrossRef]
- Walsh, R.P.D.; Lawler, D.M. Rainfall Seasonality: Description, Spatial Patterns and Change through Time. Weather 1981, 36, 201–208. [Google Scholar] [CrossRef]
- Abd Elbasit, M.A.M.; Huang, J.; Ojha, C.; Yasuda, H.; Adam, E.O. Spatiotemporal Changes of Rainfall Erosivity in Loess Plateau, China. ISRN Soil Sci. 2013, 2013, 1–8. [Google Scholar] [CrossRef]
- Bessaklia, H.; Ghenim, A.N.; Megnounif, A.; Martin-Vide, J. Spatial variability of concentration and aggressiveness of precipitation in North-East of Algeria. J. Water Land Dev. 2018, 36, 3–15. [Google Scholar] [CrossRef]
- Gregori, E.; Andrenelli, M.C.; Zorn, G. Assessment and classification of climatic aggressiveness with regard to slope instability phenomena connected to hydrological and morphological processes. J. Hydrol. 2006, 329, 489–499. [Google Scholar] [CrossRef]
- Renard, K.G.; Freimund, J.R. Using monthly precipitation data to estimate the R-factor in the revised USLE. J. Hydrol. 1994, 157, 287–306. [Google Scholar] [CrossRef]
- Yu, B.; Rosewell, C.J. Technical Notes: A Robust Estimator of the R-factor for the Universal Soil Loss Equation. Trans. ASAE 1996, 39, 559–561. [Google Scholar] [CrossRef]
- Taguas, E.V.; Carpintero, E.; Ayuso, J.L. Assessing land degradation risk through the long-term analysis of erosivity: A case study in Southern Spain. Land Degrad. Dev. 2013, 24, 179–187. [Google Scholar] [CrossRef]
- Hernando, D.; Romana, M.G. Estimating the rainfall erosivity factor from monthly precipitation data in the Madrid Region (Spain). J. Hydrol. Hydromech. 2015, 63, 55–62. [Google Scholar] [CrossRef]
- Di Lena, B.; Antenucci, F.; Vergni, L.; Mariani, L. Analysis of the Climatic Aggressiveness of Rainfall in the Abruzzo Region. Ital. J. Agrometeorol. 2013, 1, 33–44. [Google Scholar]
- Märker, M.; Angeli, L.; Bottai, L.; Costantini, R.; Ferrari, R.; Innocenti, L.; Siciliano, G. Assessment of land degradation susceptibility by scenario analysis: A case study in Southern Tuscany, Italy. Geomorphology 2008, 93, 120–129. [Google Scholar] [CrossRef]
- Ferro, V.; Giordano, G.; Iovino, M. Isoerosivity and erosion risk map for Sicily. Hydrol. Sci. J. 1991, 36, 549–564. [Google Scholar] [CrossRef]
- Capolongo, D.; Diodato, N.; Mannaerts, C.M.; Piccarreta, M.; Strobl, R.O. Analyzing temporal changes in climate erosivity using a simplified rainfall erosivity model in Basilicata (southern Italy). J. Hydrol. 2008, 356, 119–130. [Google Scholar] [CrossRef]
- De Santos Loureiro, N.; de Azevedo Coutinho, M. A new procedure to estimate the RUSLE EI30 index, based on monthly rainfall data and applied to the Algarve region, Portugal. J. Hydrol. 2001, 250, 12–18. [Google Scholar] [CrossRef]
- Nunes, A.N.; Lourenço, L.; Vieira, A.; Bento-Gonçalves, A. Precipitation and Erosivity in Southern Portugal: Seasonal Variability and Trends (1950–2008): Seasonal variability and trends (1950–2008). Land Degrad. Dev. 2016, 27, 211–222. [Google Scholar] [CrossRef]
- De Luis, M.; González-Hidalgo, J.C.; Longares, L.A. Is rainfall erosivity increasing in the Mediterranean Iberian Peninsula? Land Degrad. Dev. 2010, 21, 139–144. [Google Scholar] [CrossRef]
- García-Marín, A.P.; Ayuso-Muñoz, J.L.; Cantero, F.N.; Ayuso-Ruiz, J.L. Spatial and Trend Analyses of Rainfall Seasonality and Erosivity in the West of Andalusia (Period 1945–2005). Soil Sci. 2017, 182, 146–158. [Google Scholar] [CrossRef]
- Lukić, T.; Lukić, A.; Basarin, B.; Ponjiger, T.M.; Blagojević, D.; Mesaroš, M.; Milanović, M.; Gavrilov, M.; Pavić, D.; Zorn, M.; et al. Rainfall erosivity and extreme precipitation in the Pannonian basin. Open Geosci. 2019, 11, 664–681. [Google Scholar] [CrossRef]
- Elagib, N.A. Changing rainfall, seasonality and erosivity in the hyper-arid zone of Sudan. Land Degrad. Dev. 2011, 22, 505–512. [Google Scholar] [CrossRef]
- Valdés-Pineda, R.; Pizarro, R.; Valdés, J.B.; Carrasco, J.F.; García-Chevesich, P.; Olivares, C. Spatio-temporal trends of precipitation, its aggressiveness and concentration, along the Pacific coast of South America (36–49° S). Hydrol. Sci. J. 2016, 61, 2110–2132. [Google Scholar] [CrossRef]
- AL-Shamarti, H.K.A. The Variation of Annual Precipitation and Precipitation Concentration Index of Iraq. J. Appl. Phys. 2016, 8, 36–44. [Google Scholar] [CrossRef]
- Ghenim, A.N.; Megnounif, A. Spatial distribution and temporal trends in daily and monthly rainfall concentration indices in Kebir-Rhumel watershed. LARHYSS J. 2016, 26, 85–97. [Google Scholar]
- Zhang, K.; Yao, Y.; Qian, X.; Wang, J. Various characteristics of precipitation concentration index and its cause analysis in China between 1960 and 2016. Int. J. Climatol. 2019, 39, 4648–4658. [Google Scholar] [CrossRef]
- D’Asaro, F.; D’Agostino, L.; Bagarello, V. Assessing changes in rainfall erosivity in Sicily during the twentieth century. Hydrol. Process. 2007, 21, 2862–2871. [Google Scholar] [CrossRef]
- Capra, A.; Porto, P.; La Spada, C. Long-term variation of rainfall erosivity in Calabria (Southern Italy). Theor. Appl. Climatol. 2017, 128, 141–158. [Google Scholar] [CrossRef]
- Lu, H.; Yu, B. Spatial and seasonal distribution of rainfall erosivity in Australia. Soil Res. 2002, 40, 887. [Google Scholar] [CrossRef]
- Vrieling, A.; Hoedjes, J.C.B.; van der Velde, M. Towards large-scale monitoring of soil erosion in Africa: Accounting for the dynamics of rainfall erosivity. Glob. Planet. Chang. 2014, 115, 33–43. [Google Scholar] [CrossRef]
- Zhu, Z.; Yu, B. Validation of Rainfall Erosivity Estimators for Mainland China. Trans. ASABE 2015, 61–71. [Google Scholar]
- Bezak, N.; Ballabio, C.; Mikoš, M.; Petan, S.; Borrelli, P.; Panagos, P. Reconstruction of past rainfall erosivity and trend detection based on the REDES database and reanalysis rainfall. J. Hydrol. 2020, 590, 125372. [Google Scholar] [CrossRef]
- Wang, M.; Yin, S.; Yue, T.; Yu, B.; Wang, W. Rainfall Erosivity Estimation Using Gridded Daily Precipitation Datasets. Hydrol. Earth Syst. Sci. Discuss. 2020, 1–30. [Google Scholar] [CrossRef]
- Curci, G.; Guijarro, J.A.; Di Antonio, L.; Di Bacco, M.; Di Lena, B.; Scorzini, A.R. Building a local climate reference dataset: Application to the Abruzzo region (Central Italy), 1930–2019. Int. J. Climatol. 2021, joc.7081. [Google Scholar] [CrossRef]
- Cornes, R.C.; van der Schrier, G.; van den Besselaar, E.J.M.; Jones, P.D. An Ensemble Version of the E-OBS Temperature and Precipitation Data Sets. J. Geophys. Res. Atmos. 2018, 123, 9391–9409. [Google Scholar] [CrossRef]
- Vergni, L.; Todisco, F.; Di Lena, B.; Mannocchi, F. Effect of the North Atlantic Oscillation on winter daily rainfall and runoff in the Abruzzo region (Central Italy). Stoch. Environ. Res. Risk Assess 2016, 30, 1901–1915. [Google Scholar] [CrossRef]
- Guijarro, J.A. Climatol: Climate Tools (Series Homogenization and Derived Products); R-Package. 2019. Available online: https://CRAN.R-project.org/package=climatol (accessed on 20 May 2021).
- Vergni, L.; Di Lena, B.; Todisco, F.; Mannocchi, F. Uncertainty in drought monitoring by the Standardized Precipitation Index: The case study of the Abruzzo region (central Italy). Theor. Appl. Climatol. 2017, 128, 13–26. [Google Scholar] [CrossRef]
- CORINE Soil Erosion Risk and Important Land Resources-in the Southern Regions of the European Community-European Environment Agency. Available online: https://www.eea.europa.eu/publications/COR0-soil (accessed on 13 April 2021).
- Sumner, G.; Homar, V.; Ramis, C. Precipitation seasonality in eastern and southern coastal Spain. Int. J. Climatol. 2001, 21, 219–247. [Google Scholar] [CrossRef]
- Wischmeier, W.H. A Rainfall Erosion Index for a Universal Soil-Loss Equation. Soil Sci. Soc. Am. J. 1959, 23, 246–249. [Google Scholar] [CrossRef]
- Nearing, M.A.; Yin, S.; Borrelli, P.; Polyakov, V.O. Rainfall erosivity: An historical review. Catena 2017, 157, 357–362. [Google Scholar] [CrossRef]
- Di Lena, B.; Vergni, L.; Antenucci, F.; Todisco, F.; Mannocchi, F. Analysis of drought in the region of Abruzzo (Central Italy) by the Standardized Precipitation Index. Theor. Appl. Climatol. 2014, 115, 41–52. [Google Scholar] [CrossRef]
- Panagos, P.; Meusburger, K.; Ballabio, C.; Borrelli, P.; Alewell, C. Soil erodibility in Europe: A high-resolution dataset based on LUCAS. Sci. Total Environ. 2014, 479–480, 189–200. [Google Scholar] [CrossRef] [PubMed]
- Bosco, C.; de Rigo, D.; Dewitte, O.; Poesen, J.; Panagos, P. Modelling soil erosion at European scale: Towards harmonization and reproducibility. Nat. Hazards Earth Syst. Sci. 2015, 15, 225–245. [Google Scholar] [CrossRef]
- Mann, H.B. Nonparametric Tests against Trend. Econometrica 1945, 13, 245–259. [Google Scholar] [CrossRef]
- Kendall, M.G. Rank Correlation Measures; Charles Griffin: London, UK, 1975. [Google Scholar]
- Theil, H. A Rank-Invariant Method of Linear and Polynomial Regression Analysis. In Henri Theil’s Contributions to Economics and Econometrics: Econometric Theory and Methodology; Advanced Studies in Theoretical and Applied Econometrics; Raj, B., Koerts, J., Eds.; Springer: Dordrecht, The Netherlands, 1992; pp. 345–381. ISBN 978-94-011-2546-8. [Google Scholar]
- Sen, P.K. Estimates of the Regression Coefficient Based on Kendall’s Tau. J. Am. Stat. Assoc. 1968, 63, 1379–1389. [Google Scholar] [CrossRef]
- Bronaugh, D.; Consortium, A.W. For the P.C.I. zyp: Zhang + Yue-Pilon Trends Package. 2019. Available online: https://CRAN.R-project.org/package=zyp (accessed on 21 May 2021).
- McLeod, A.I. Kendall: Kendall Rank Correlation and Mann-Kendall Trend Test. 2011. Available online: https://CRAN.R-project.org/package=Kendall (accessed on 21 May 2021).



| Index | Class | Description |
|---|---|---|
| MFI | <60 | Very low |
| 60–90 | Low | |
| 90–120 | Moderate | |
| 120–160 | High | |
| >160 | Very high | |
| PCI | <10% | Uniform distribution |
| 10–15% | Moderate distribution | |
| 15–20% | Irregular distribution | |
| >20% | Strongly irregular distribution | |
| SI | <0.19 | Precipitation spread throughout the year |
| 0.20–0.39 | Precipitation spread throughout the year, but with a definite wetter season | |
| 0.40–0.59 | Rather seasonal with a short drier season | |
| 0.60–0.79 | Seasonal | |
| 0.80–0.99 | Markedly seasonal with a long dry season | |
| 1.00–1.19 | Most precipitation in 3 months | |
| >1.20 | Extreme seasonality, with almost all precipitation in 1–2 months |
| Point Dataset | |||||
|---|---|---|---|---|---|
| Low | Moderate | High | Very High | ||
| Grid Dataset | Low | 8 | 6 | 0 | 0 |
| Moderate | 5 | 6 | 7 | 0 | |
| High | 1 | 0 | 0 | 1 | |
| Very High | 0 | 0 | 0 | 0 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Lena, B.; Curci, G.; Vergni, L. Analysis of Rainfall Erosivity Trends 1980–2018 in a Complex Terrain Region (Abruzzo, Central Italy) from Rain Gauges and Gridded Datasets. Atmosphere 2021, 12, 657. https://doi.org/10.3390/atmos12060657
Di Lena B, Curci G, Vergni L. Analysis of Rainfall Erosivity Trends 1980–2018 in a Complex Terrain Region (Abruzzo, Central Italy) from Rain Gauges and Gridded Datasets. Atmosphere. 2021; 12(6):657. https://doi.org/10.3390/atmos12060657
Chicago/Turabian StyleDi Lena, Bruno, Gabriele Curci, and Lorenzo Vergni. 2021. "Analysis of Rainfall Erosivity Trends 1980–2018 in a Complex Terrain Region (Abruzzo, Central Italy) from Rain Gauges and Gridded Datasets" Atmosphere 12, no. 6: 657. https://doi.org/10.3390/atmos12060657
APA StyleDi Lena, B., Curci, G., & Vergni, L. (2021). Analysis of Rainfall Erosivity Trends 1980–2018 in a Complex Terrain Region (Abruzzo, Central Italy) from Rain Gauges and Gridded Datasets. Atmosphere, 12(6), 657. https://doi.org/10.3390/atmos12060657







