A Novel Composite Coding Method for Incoherent Scatter Radar
Abstract
1. Complementary Code, Alternating Code and Composite Code
1.1. Complementary Code
1.2. Alternating Code
1.3. Composite Code
2. Performance of the Composite Code
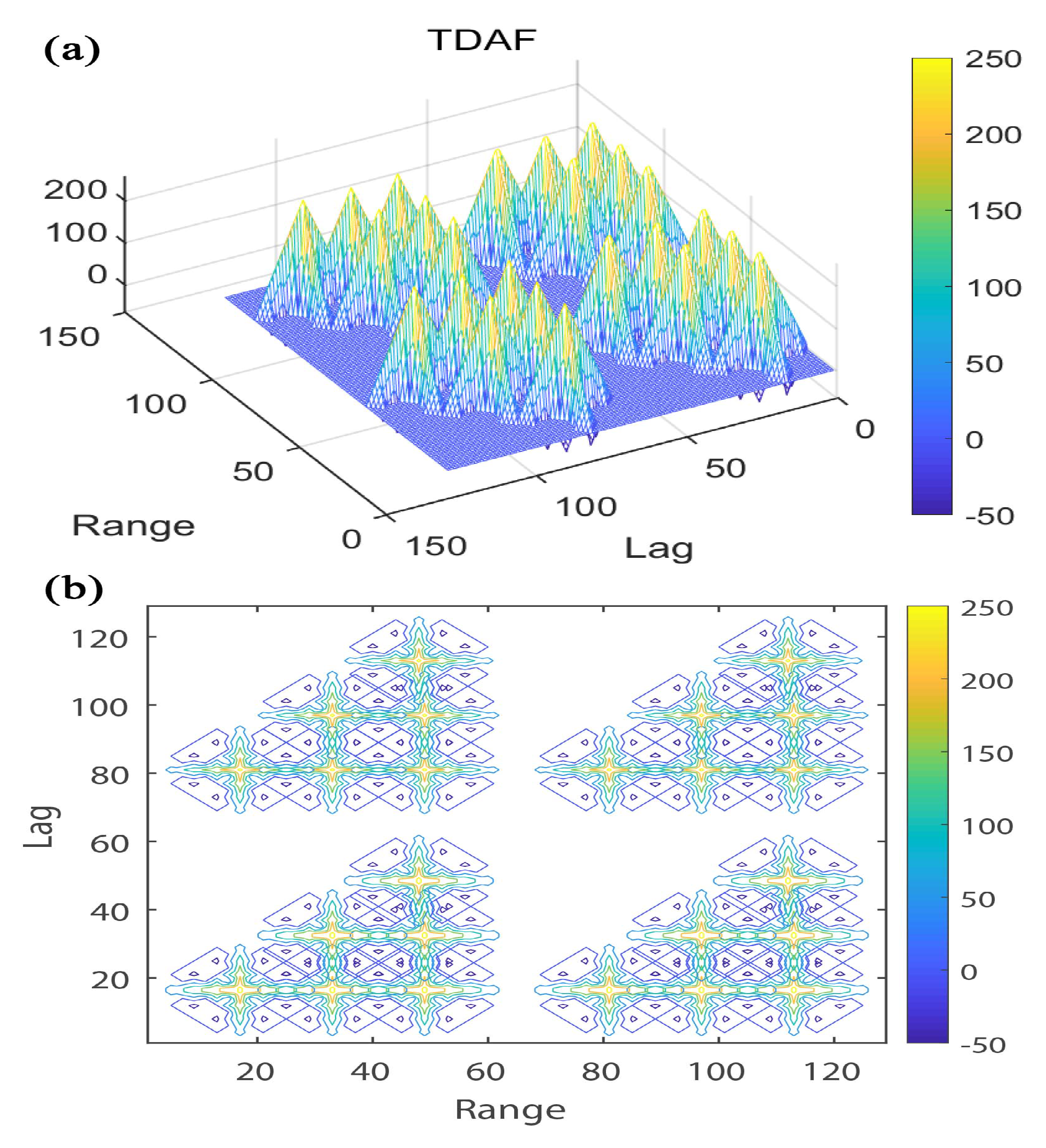
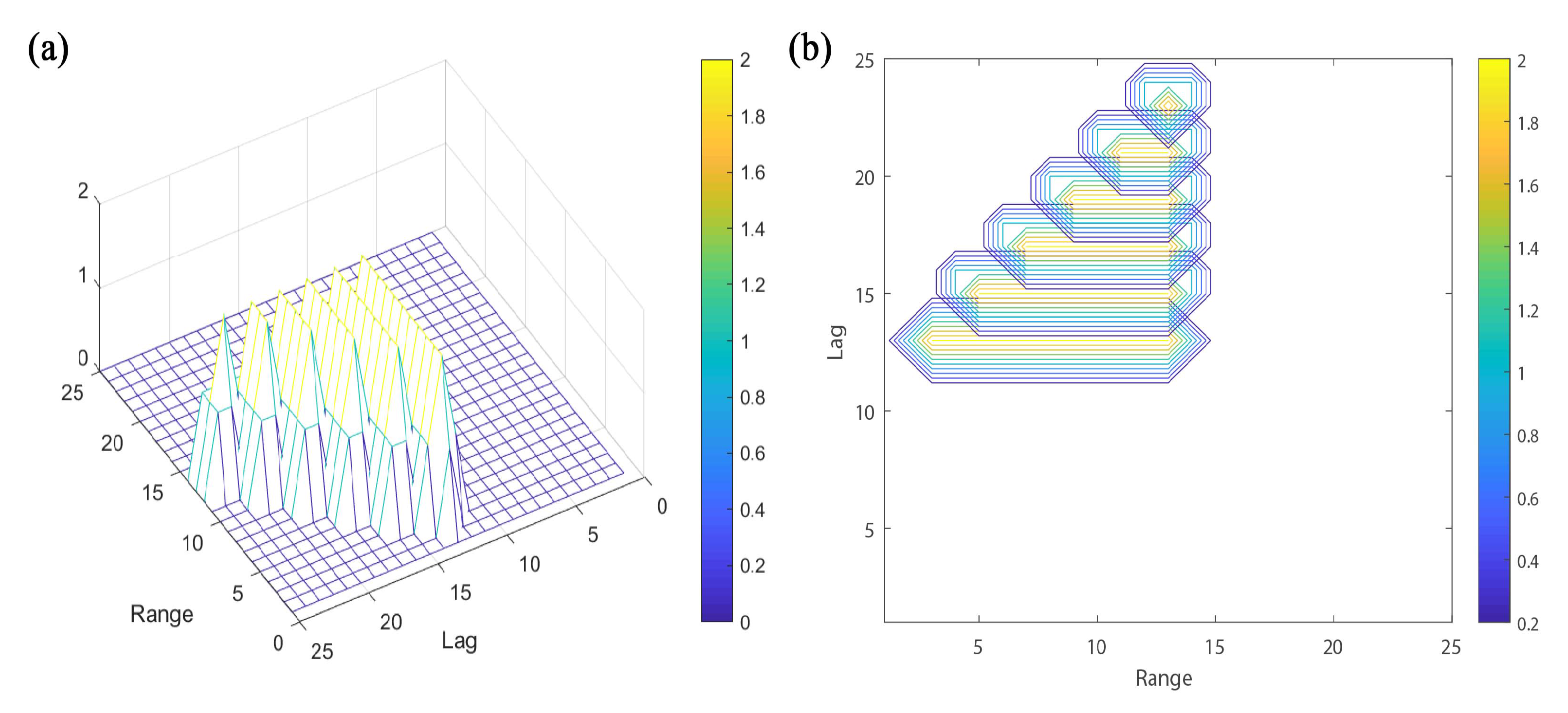
2.1. Two-Dimensional Ambiguity Function
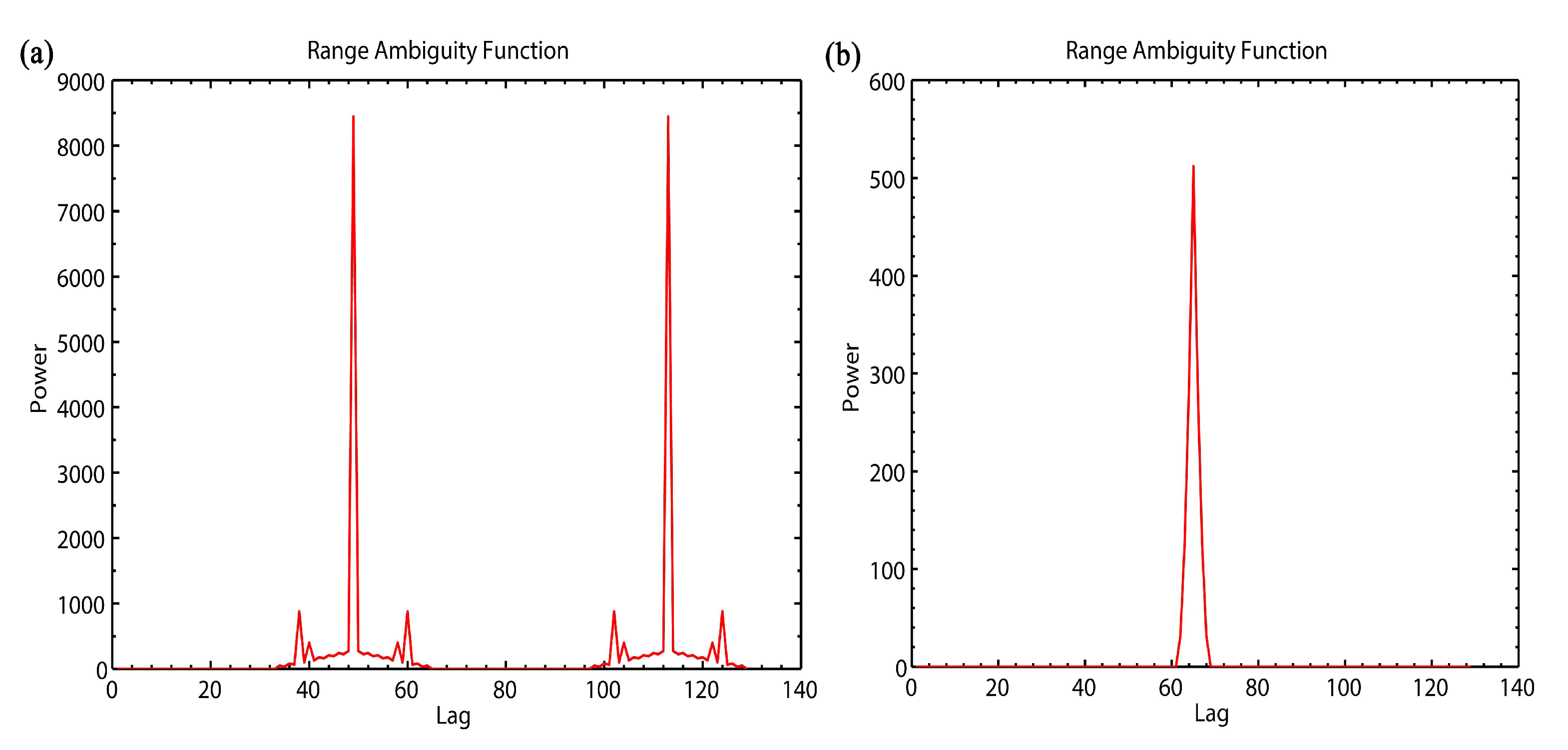
2.2. Range Ambiguity Function
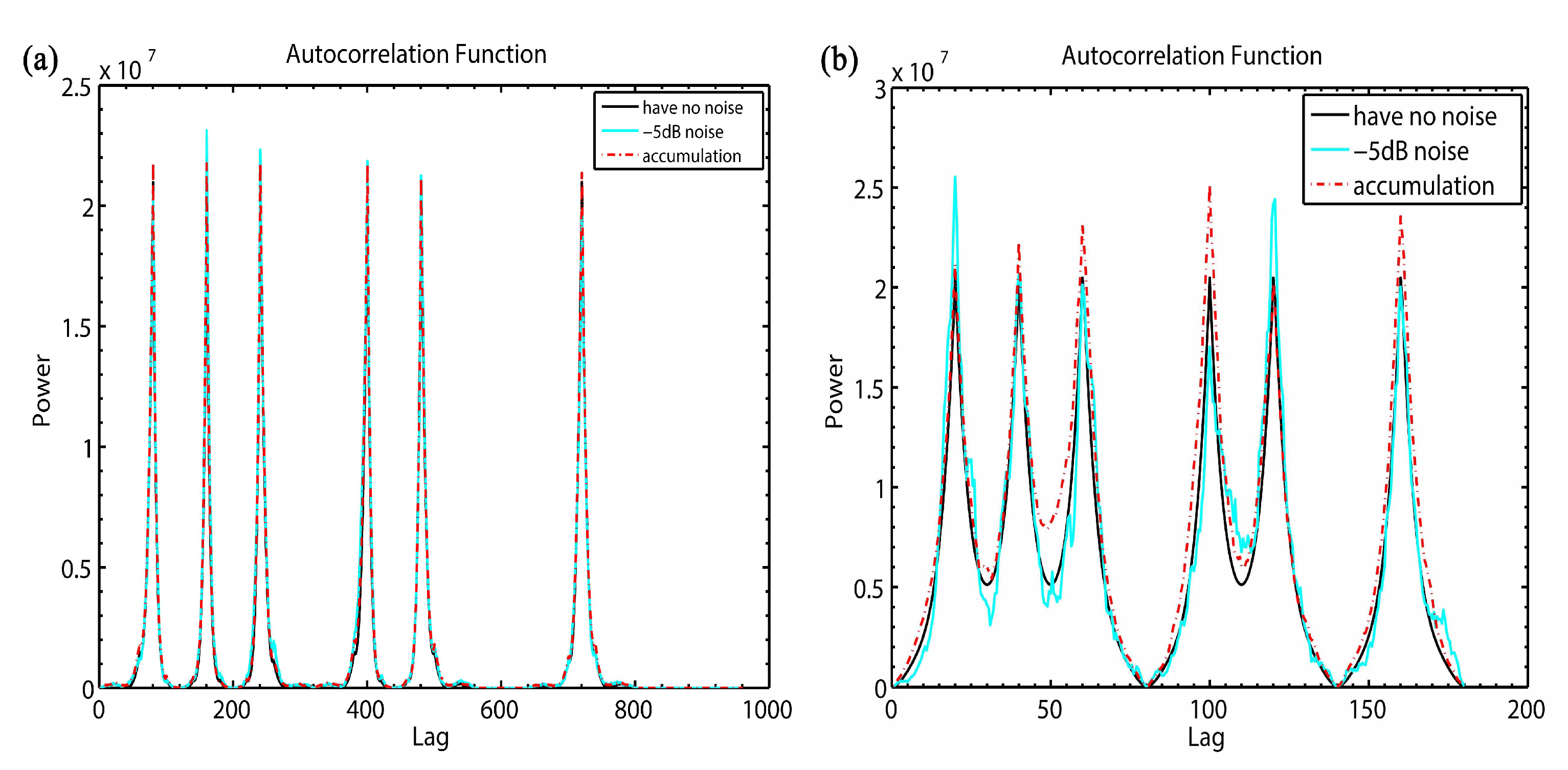
2.3. Anti-Noise Performance
3. Summary and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gordon, W.E. Incoherent scattering of radio waves by free electrons with applications to space exploration by radar. Proc. Ire 1958, 46, 1824–1829. [Google Scholar] [CrossRef]
- Li, L.; Lu, Y.; Wang, Y.; Ji, H.; Ding, Z. A modified parameter model-based spectrum estimation method for incoherent scatter radar. In Proceedings of the 2017 2nd International Conference on Image, Vision and Computing (ICIVC), Chengdu, China, 2–4 June 2017. [Google Scholar]
- Hagfors, T. Incoherent scatter radar observations of the plasma line with a chirped pulse system. Radio Sci. 1982, 17, 727–734. [Google Scholar] [CrossRef]
- Nygrén, T.; Jalonen, L.; Huuskonen, A. A new method of measuring the ion-neutral collision frequency using incoherent scatter radar. Planet. Space Sci. 1987, 35, 337–343. [Google Scholar] [CrossRef]
- Farley, D.T. Multiple-Pulse Incoherent-Scatter Correlation Function Measurements. Radio Sci. 1972, 7, 661–666. [Google Scholar] [CrossRef]
- Hysell, D.L.; Larsen, M.F.; Zhou, Q.H. Common volume coherent and incoherent scatter radar observations of mid-latitude sporadic E-layers and QP echoes. Ann. Geophys. 2004, 22, 3277–3290. [Google Scholar] [CrossRef]
- Lehtinen, M.S. Ingemar Häggström A new modulation principle for incoherent scatter measurements. Radio Sci. 1987, 22, 625–634. [Google Scholar] [CrossRef]
- Sulzer, M.P. A new type of alternating code for incoherent scatter measurements. Radio Sci. 1993, 28, 995–1001. [Google Scholar] [CrossRef]
- Nygrén, T.; Markkanen, M. Long alternating codes 1. Search byplaying dominoes. Radio Sci. 1997, 32, 1–8. [Google Scholar] [CrossRef]
- Lehtinen, M.S.; Huuskonen, A.; Markkanen, M. Randomization ofalternating codes: Improving incoherent scatter measurements byreducing correlations of gated autocorrelation function estimates. Radio Sci. 1997, 32, 2271–2282. [Google Scholar] [CrossRef]
- Häggström, I. Incoherent Scatter Studies of Upper Atmmosphere Dynamics and Coding Techniques. Ph.D. Thesis, Swedish Institute of Space Physics, Kiruna, Sweden, 1990. [Google Scholar]
- Yang, G.; Duan, P.; Jiang, C.; Liu, T.; Lan, T.; Zhao, Z.; Shi, S.; Xu, C. Application of biphase complete complementary code for ionospheric sounding. Sensors 2018, 18, 2811. [Google Scholar] [CrossRef] [PubMed]
- Damtie, B.; Nygrén, T.; Lehtinen, M.S.; Huuskonen, A. High resolution observation of sporadic-E layers within the polar cap ionosphere using a new incoherent scatter radar experiment. Ann. Geophys. 2002, 20, 1429–1438. [Google Scholar] [CrossRef][Green Version]
- Lehtinen, M.; Markkanen, J.; Vaananen, A.; Huuskonen, A.; Damtie, B.; Nygrén, T.; Rahkola, J. A new incoherent scatter technique in the EISCAT Svalbard Radar. Radio Sci. 2002, 37, 1050. [Google Scholar] [CrossRef]
- Guo, Y.; Ming, Y.; Kai, Y.; Deng, X. Simulation and Performance Evaluation of Three Types of ISR Coding Systems. IEEE Geosci. Remote Sens. Lett. 2018, 15, 1–4. [Google Scholar] [CrossRef]
- Golay, M. Multi-silt spectrometry. J. Opt. Soc. Am. 1949, 39, 437–444. [Google Scholar] [CrossRef] [PubMed]
- Golay, M. Complementary series. IRE Trans. Inf. Theory 1961, 7, 82–87. [Google Scholar] [CrossRef]
- Markkanen, M.; Nygrén, T. A 64-bit strong alternating code discovered. Radio Sci. 1996, 31, 241–243. [Google Scholar] [CrossRef]
- Li, L.; Chen, Y.; Ji, H.; Ding, Z.; Jin, W. Research on signal modulation and processing for incoherent scatter radar. In Proceedings of the 2015 10th International Conference on Information, Communications and Signal Processing (ICICS), Singapore, 2–4 December 2015; pp. 1–4. [Google Scholar]
- Lehtinen, M.; Huuskonen, A. General incoherent scatter analysis and GUISDAP. J. Atmos. Terr. Phys. 1996, 58, 435–452. [Google Scholar] [CrossRef]
- Trunk, V. Range Resolution of Targets Using Automatic Detectors. IEEE Trans. Aerosp. Electron. Syst. 2007, 14, 750–755. [Google Scholar] [CrossRef]
- Foreman, T.L.; Wilson, S.G. Multiple pulse detection in distributed rayleigh clutter. In Proceedings of the Record of the IEEE 2000 International Radar Conference [Cat. NO. 00CH37037], Alexandria, VA, USA, 12 May 2000; pp. 637–642. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.; Yuan, K.; Yao, M.; Xiong, J. A Novel Composite Coding Method for Incoherent Scatter Radar. Atmosphere 2021, 12, 1518. https://doi.org/10.3390/atmos12111518
Chen H, Yuan K, Yao M, Xiong J. A Novel Composite Coding Method for Incoherent Scatter Radar. Atmosphere. 2021; 12(11):1518. https://doi.org/10.3390/atmos12111518
Chicago/Turabian StyleChen, Haoyi, Kai Yuan, Ming Yao, and Jiawei Xiong. 2021. "A Novel Composite Coding Method for Incoherent Scatter Radar" Atmosphere 12, no. 11: 1518. https://doi.org/10.3390/atmos12111518
APA StyleChen, H., Yuan, K., Yao, M., & Xiong, J. (2021). A Novel Composite Coding Method for Incoherent Scatter Radar. Atmosphere, 12(11), 1518. https://doi.org/10.3390/atmos12111518




