Droplet Fate in a Cough Puff
Abstract
1. Introduction
2. Methodology
− v(0) (B/C) [ M(t) (1 + A/C)− 1]/(1 + A/C)
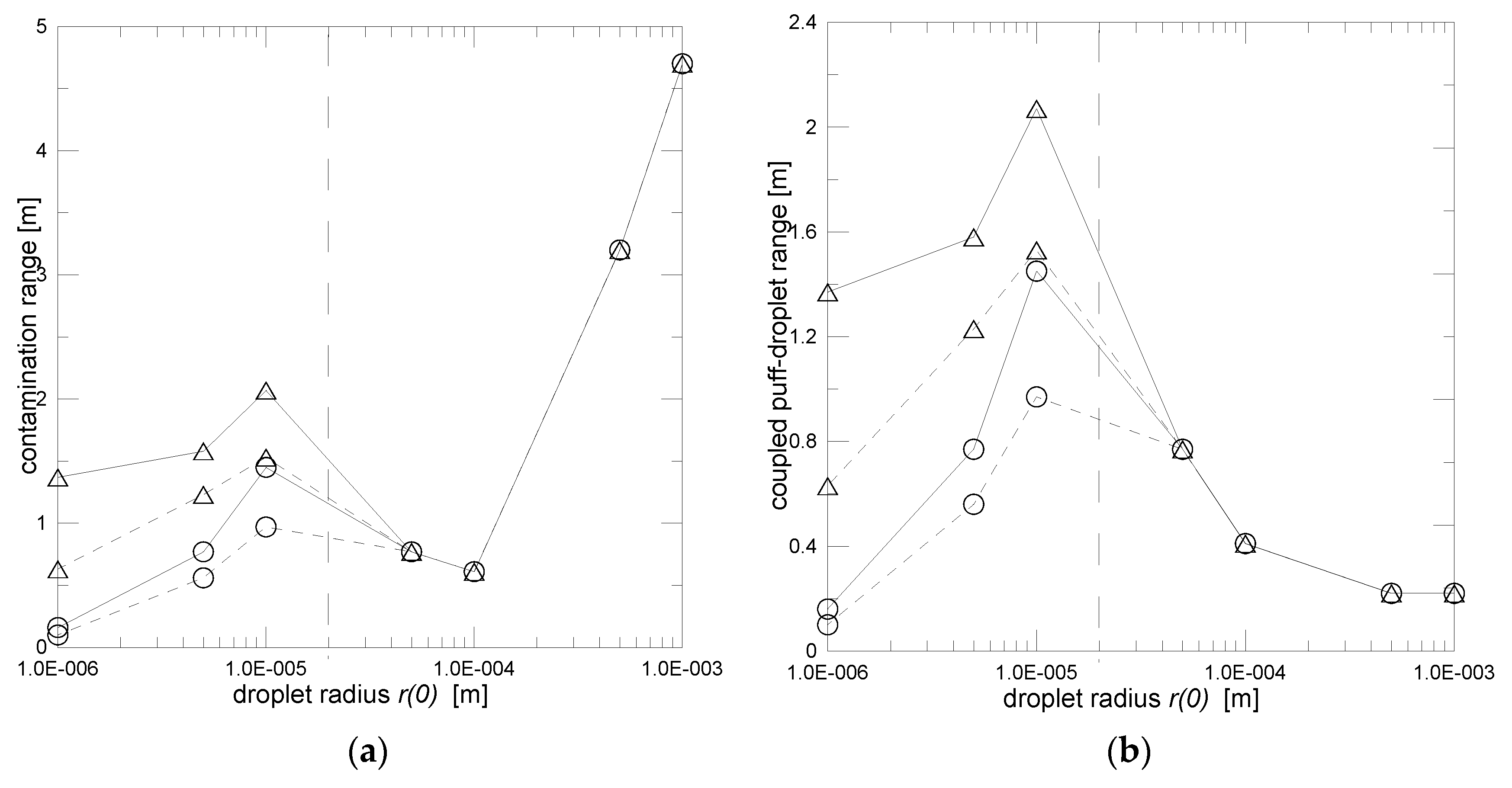
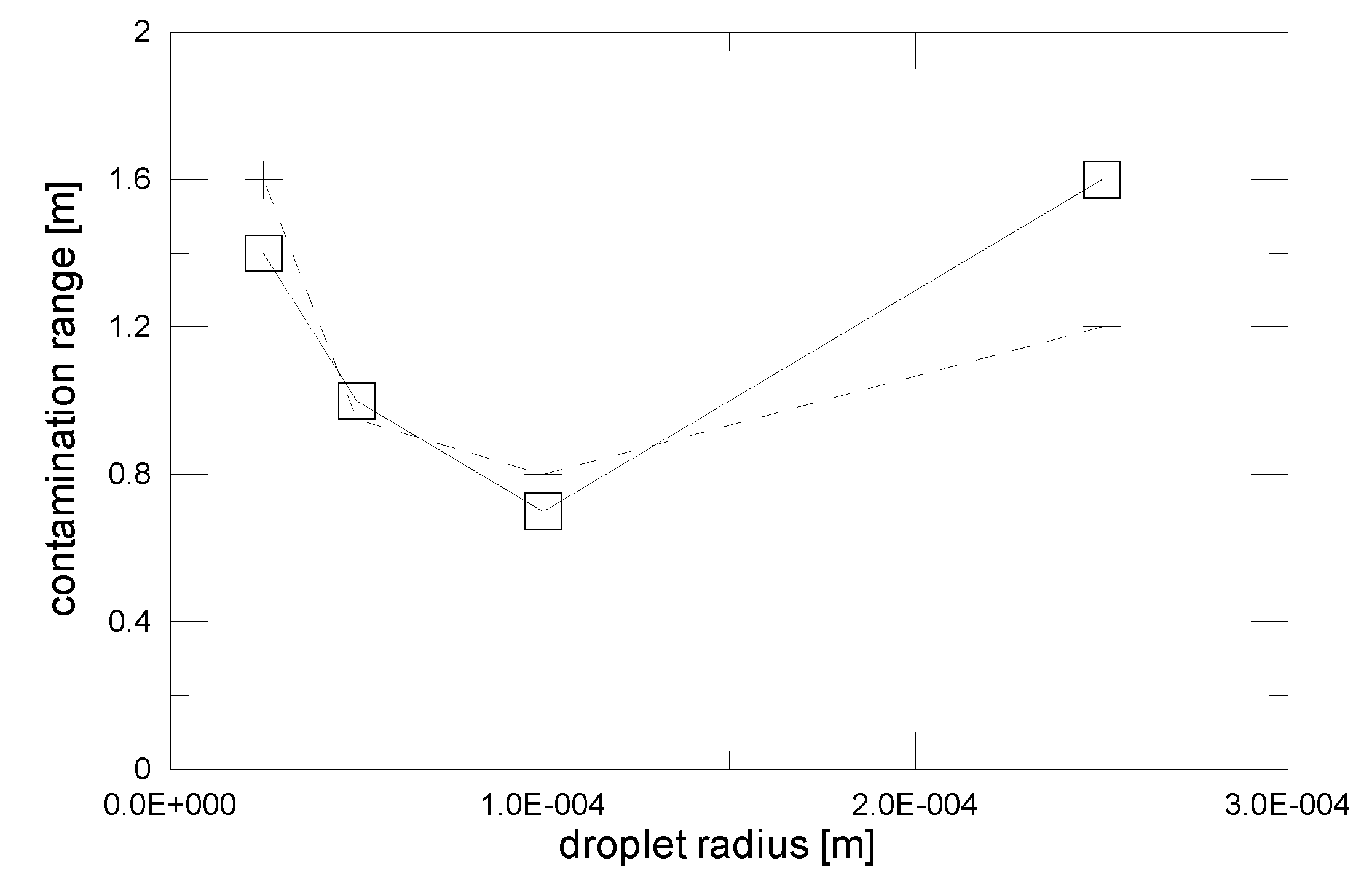
3. Results
4. Discussion and Conclusions
Funding
Conflicts of Interest
References
- Asadi, S.; Bouvier, N.; Wexler, A.S.; Ristenpart, W.D. The coronavirus pandemic and aerosols: Does COVID-19 transmit via expiratory particles? Aerosol. Sci. Technol. 2020, 54, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Blocken, B.; Malizia, F.; van Druenen, T.; Marchal, T. Towards Aerodynamically Equivalent COVID-19 1.5 m Social Distancing for Walking and Running. 2020. Available online: http://www.urbanphysics.net/Social%20Distancing%20v20_White_Paper.pdf (accessed on 10 May 2020).
- Wells, W.F. On airborne infection. Study II. Droplet and droplet nuclei. Am. J. Hyg. 1934, 20, 611–618. [Google Scholar]
- Bourouiba, L.; Dehandshoewercker, E.; Bush, J.W.M. Violent expiratory events: On coughing and sneezing. J. Fluid. Mech. 2014, 745, 537–563. [Google Scholar] [CrossRef]
- Bourouiba, L. Turbulent Gas Clouds and Respiratory Pathogen Emissions: Potential Implications for Reducing Transmission of COVID-19. JAMA 2020, 323, 1837–1838. [Google Scholar] [CrossRef] [PubMed]
- Carleton, T.; Meng, K.C. Causal Empirical Estimates Suggest COVID-19 Transmission Rates Are Highly Seasonal. Available online: https://www.medrxiv.org/content/10.1101/2020.03.26.20044420v1 (accessed on 10 May 2020). [CrossRef]
- Bukhari, Q.; Jameel, Y. Will Coronavirus Pandemic Diminish by Summer? Available online: https://www.ssrn.com/abstract=3556998 (accessed on 10 May 2020). [CrossRef]
- Kampf, G.; Todt, D.; Pfaender, S.; Steinmann, E. Persistence of coronaviruses on inanimate surfaces and their inactivation with biocidal agents. J. Hosp. Infect. 2020, 104, 246–251. [Google Scholar] [CrossRef] [PubMed]
- Contini, D.; Costabile, F. Does air pollution influence COVID-19 outbreaks? Atmosphere 2020, 11, 377. [Google Scholar] [CrossRef]
- Monteith, J.L.; Unsworth, M. Principles of Environmental Physics, 2nd ed.; Arnold, E., Ed.; Edward Arnold Publishers Ltd.: London, UK, 1990. [Google Scholar]
- Houze, R.A. Cloud Dynamics; Academic Press: London, UK, 1993. [Google Scholar]
- Weil, J.C. Plume Rise. In Lectues on Air Pollution Modelling; Wenkatram, A., Wyngaard, J.C., Eds.; American Meteorological Society: Boston, MA, USA, 1988. [Google Scholar]
- Bush, J.W.M.; Thurber, B.A.; Blanchette, F. Particle clouds in homogeneous and stratified environments. J. Fluid Mech. 2003, 489, 29–54. [Google Scholar] [CrossRef]
- Gupta, J.K.; Lin, C.H.; Chen, Q. Flow dynamics and characterization of a cough. Indoor Air 2009, 19, 517–525. [Google Scholar] [CrossRef] [PubMed]
- Asadi, S.; Wexler, A.S.; Cappa, C.D.; Barreda, S.; Bouvier, N.M.; Ristenpart, W.D. Aerosol emission and superemission during human speech increase with voice loudness. Sci. Rep. 2019, 9, 2348. [Google Scholar] [CrossRef] [PubMed]
- Shimmei, K.; Nakamura, T.; Ng, C.F.S.; Hashizume, M.; Murakami, Y.; Maruyama, A.; Misaki, T.; Okabe, N.; Nishiwaki, Y. Association between seasonal influenza and absolute humidity: Time-series analysis with daily surveilance data in Japan. Sci. Rep. 2020, 10, 7764. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Tang, K.; Feng, K.; Lin, X.; Lv, W.; Chen, K.; Wang, F. High Temperature and High Humidity Reduce the Transmission of COVID-19. Available online: https://www.ssrn.com/abstract=3551767 (accessed on 10 May 2020). [CrossRef]
- Casanova, L.M.; Jeon, S.; Rutala, W.A.; Weber, D.J.; Sobsey, M.D. Effects of Air Temperature and Relative Humidity on Coronavirus Survival on Surfaces. Appl. Environ. Microbiol. 2010, 76, 2712–2717. [Google Scholar] [CrossRef] [PubMed]
- Tellier, R.; Li, Y.; Cowling, B.J.; Tang, J.W. Recognition of aerosol transmission of infectious agents: A commentary. BMC Infect. Dis. 2019, 19, 101. [Google Scholar] [CrossRef] [PubMed]
- Lewis, D. Is the Coronavirus Airborne? The Experts can’t agree. Nature 2020, 580, 175. [Google Scholar] [CrossRef] [PubMed]
| r (0) (m) | t (s) | xm (m) | r (m) | z (m) | Vx (ms−1) | Zp (m) |
|---|---|---|---|---|---|---|
| 1 × 10−3 C | 0.73 (0.02) | 4.70 (0.22) | 10 (10) × 10−4 | 0 (2.0) | 0.43 (4.99) | 0.15 (0.07) |
| 1 × 10−3 W | 0.73 (0.02) | 4.70 (0.22) | 10 (10) × 10−4 | 0 (2.0) | 0.43 (4.99) | 0.15 (0.07) |
| 5 × 10−4 C | 0.84 (0.02) | 3.20 (0.22) | 5 (5) × 10−4 | 0 (2.0) | 0.39 (4.99) | 0.15 (0.07) |
| 5 ×10−4 W | 0.84 (0.02) | 3.20 (0.22) | 5 (5) × 10−4 | 0 (2.0) | 0.39 (4.99) | 0.15 (0.07) |
| 1 × 10−4 C | 1.88 (0.04) | 0.61 (0.41) | 10 (10) × 10−5 | 0 (1.99) | 0.20 (3.44) | 0.18 (0.08) |
| 1 × 10−4 W | 1.88 (0.04) | 0.61 (0.41) | 9.8 (10) × 10−5 | 0 (1.99) | 0.20 (3.44) | 0.18 (0.08) |
| 5 × 10−5 C | 6.7 (0.12) | 0.77 (0.77) | 4.8 (5.0) ×10−5 | 0 (1.98) | 0.07 (1.67) | 0.24 (0.10) |
| 5 × 10−5 W | 6.7 (0.12) | 0.77 (0.77) | 3.2 (5.0) × 10−5 | 0 (1.98) | 0.07 (1.67) | 0.24 (0.10) |
| 1 × 10−5 C | 5.9 | 2.07 | 0 [1.9 × 10−6] | 1.98 | 0.08 | 0.23 |
| 1 × 10−5 W | 0.54 | 0.97 | 0 [8.0 × 10−7] | 1.99 | 0.55 | 0.14 |
| 5 × 10−6 C | 2.98 | 1.58 | 0 [9.0 × 10−7] | 2.0 | 0.14 | 0.2 |
| 5 × 10−6 W | 0.16 | 0.56 | 0 [<1 × 10−8] | 2.0 | 1.38 | 0.11 |
| 1 × 10−6 C | 1.78 | 1.37 | 0 [1.0 × 10−7] | 2.0 | 0.21 | 0.18 |
| 1 × 10−6 W | 0.013 | 0.1 | 0 [1.5 × 10−7] | 2.0 | 6.0 | 0.07 |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martano, P. Droplet Fate in a Cough Puff. Atmosphere 2020, 11, 841. https://doi.org/10.3390/atmos11080841
Martano P. Droplet Fate in a Cough Puff. Atmosphere. 2020; 11(8):841. https://doi.org/10.3390/atmos11080841
Chicago/Turabian StyleMartano, Paolo. 2020. "Droplet Fate in a Cough Puff" Atmosphere 11, no. 8: 841. https://doi.org/10.3390/atmos11080841
APA StyleMartano, P. (2020). Droplet Fate in a Cough Puff. Atmosphere, 11(8), 841. https://doi.org/10.3390/atmos11080841





