Assessment of Regional Climate Model Simulations of the Katabatic Boundary Layer Structure over Greenland
Abstract
1. Introduction
2. Experimental Data and Model Description
2.1. The KABEG Experiment
2.2. The CCLM Model
3. Results
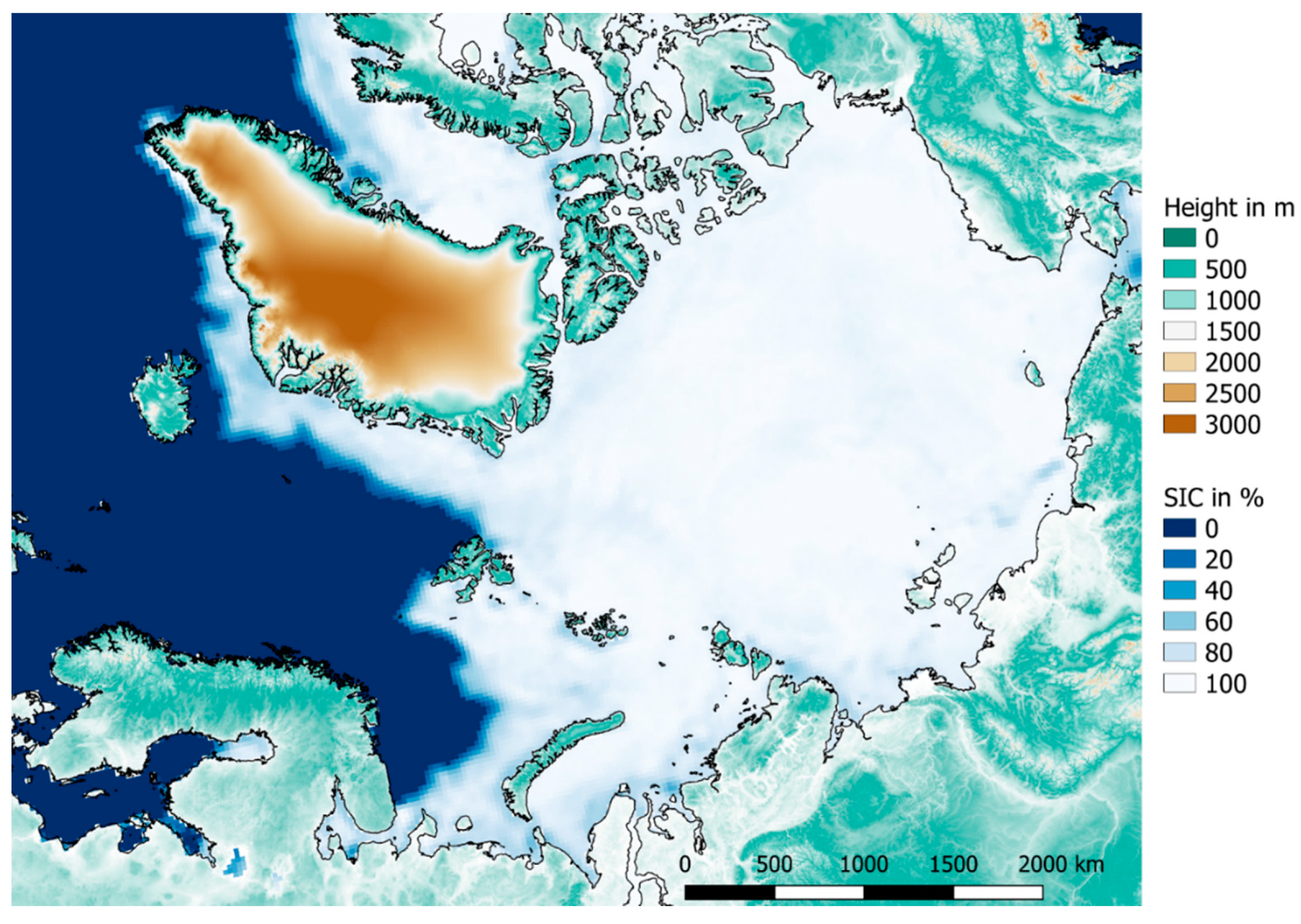
3.1. CCLM Simulations for Greenland
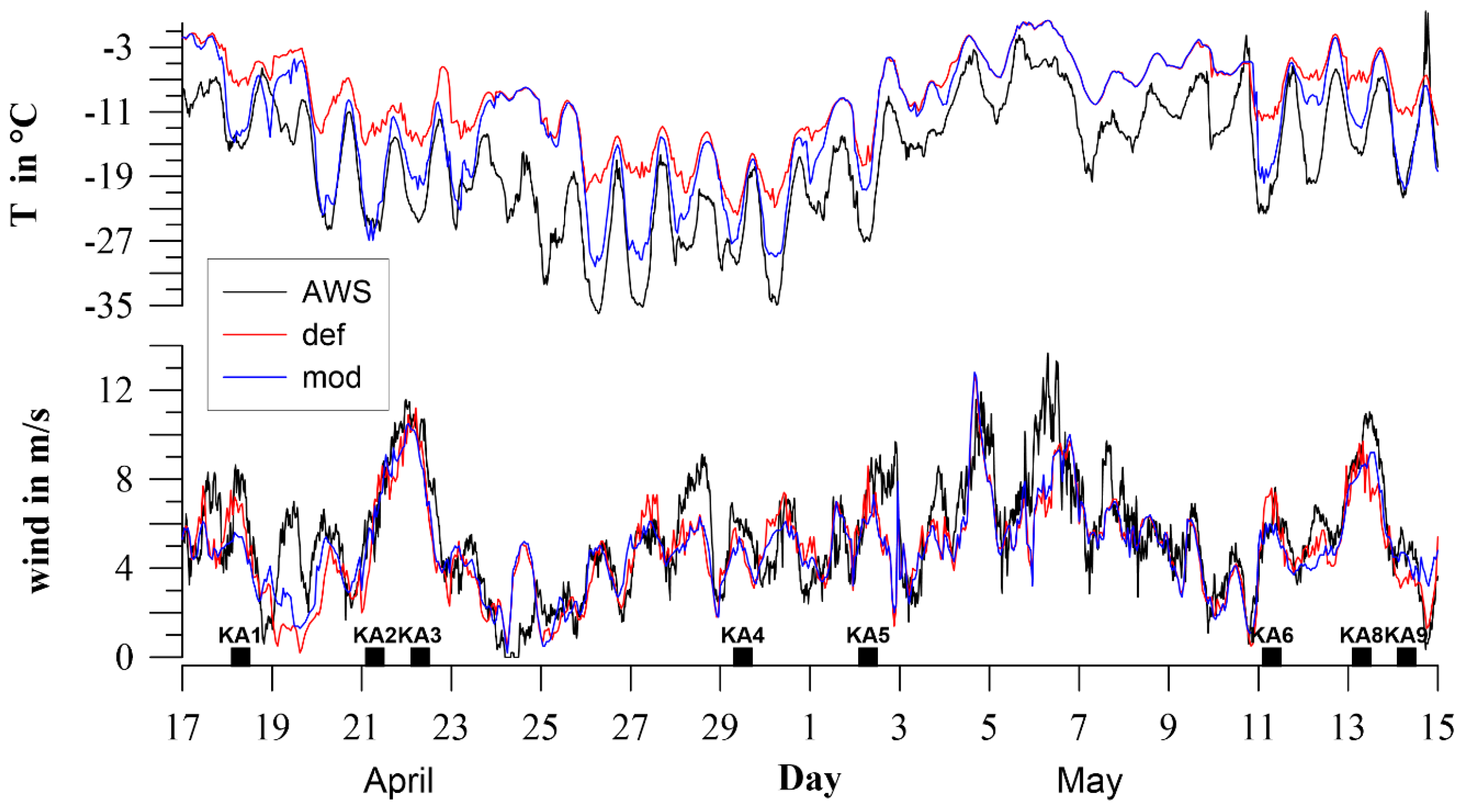
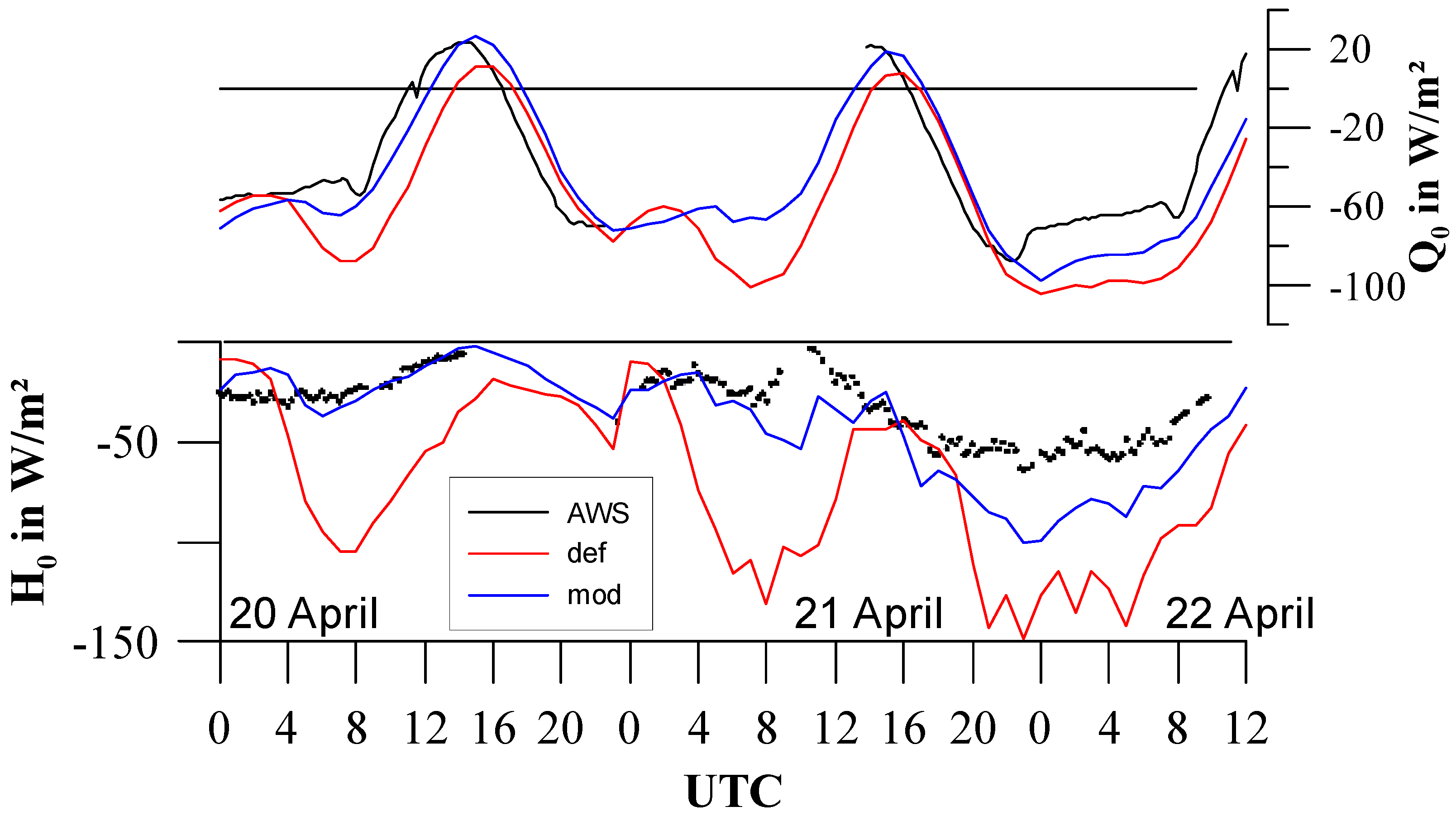
3.2. Comparison with KABEG AWS Data
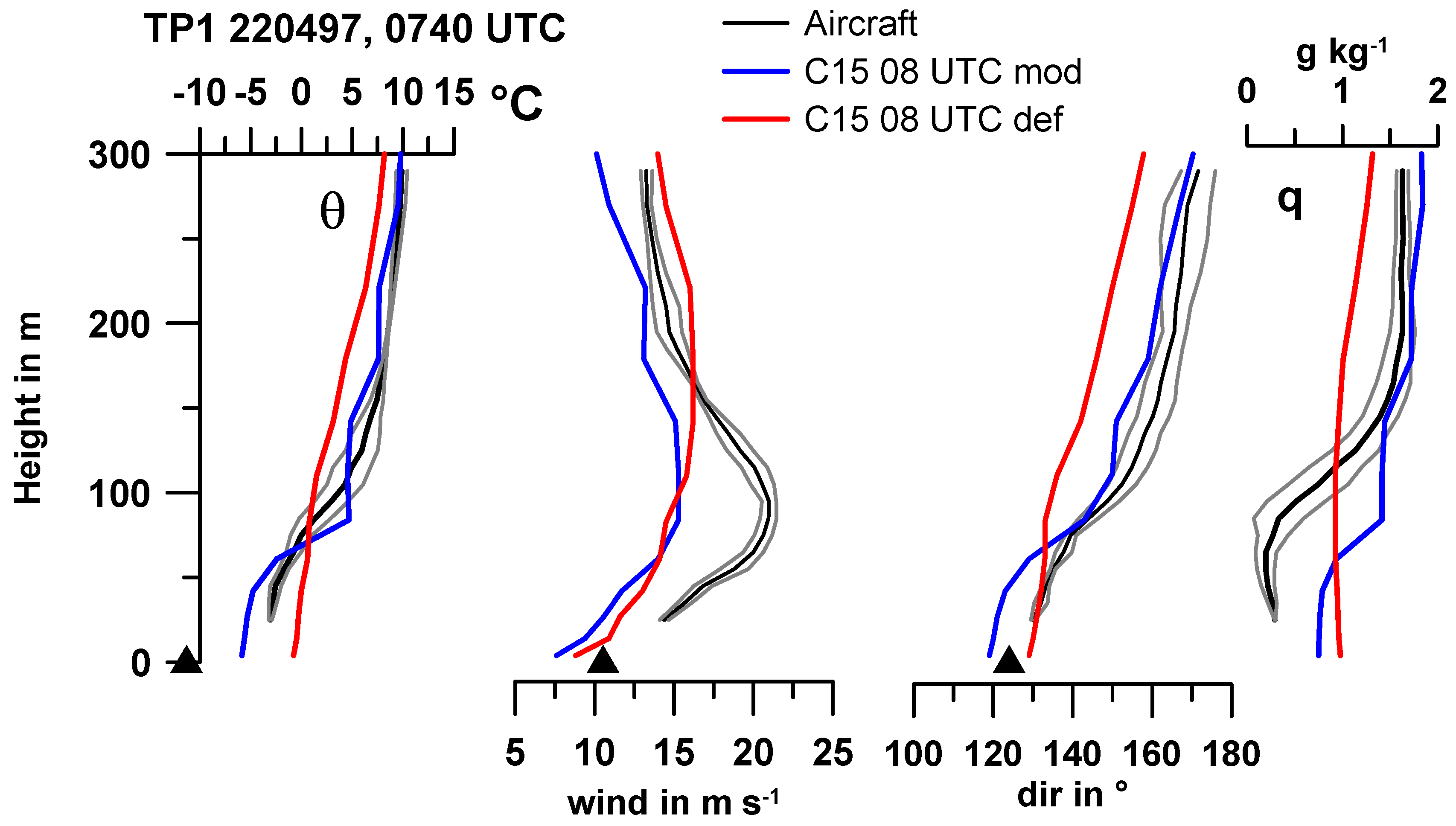
3.3. Comparison with KABEG Aircraft Profiles
3.3.1. Case Study
3.3.2. ABL Statistics
4. Discussion
5. Conclusions
- The modified parameterization (mod) yielded slightly higher katabatic winds near the surface for the monthly means of April and May 1997. The near-surface temperature in the katabatic wind regions was slightly colder.
- Comparison with an AWS for April and May 1997 showed much better simulation of the daily course of the temperature and the sensible heat flux for the mod run.
- Comparison with aircraft profiles showed that the mod run yielded more realistic profiles, particularly a better representation of the LLJ.
Funding
Acknowledgments
Conflicts of Interest
Data
Appendix A

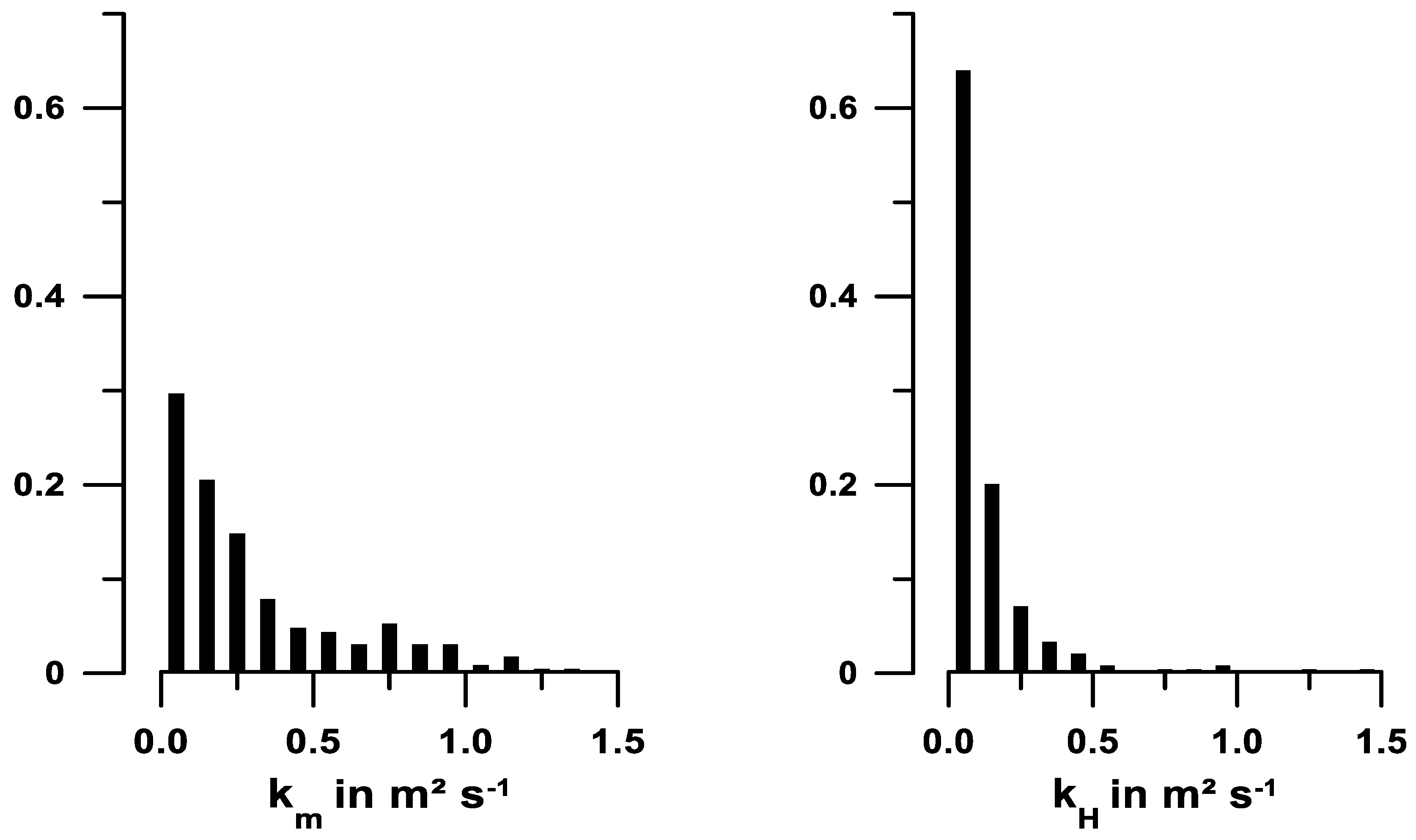
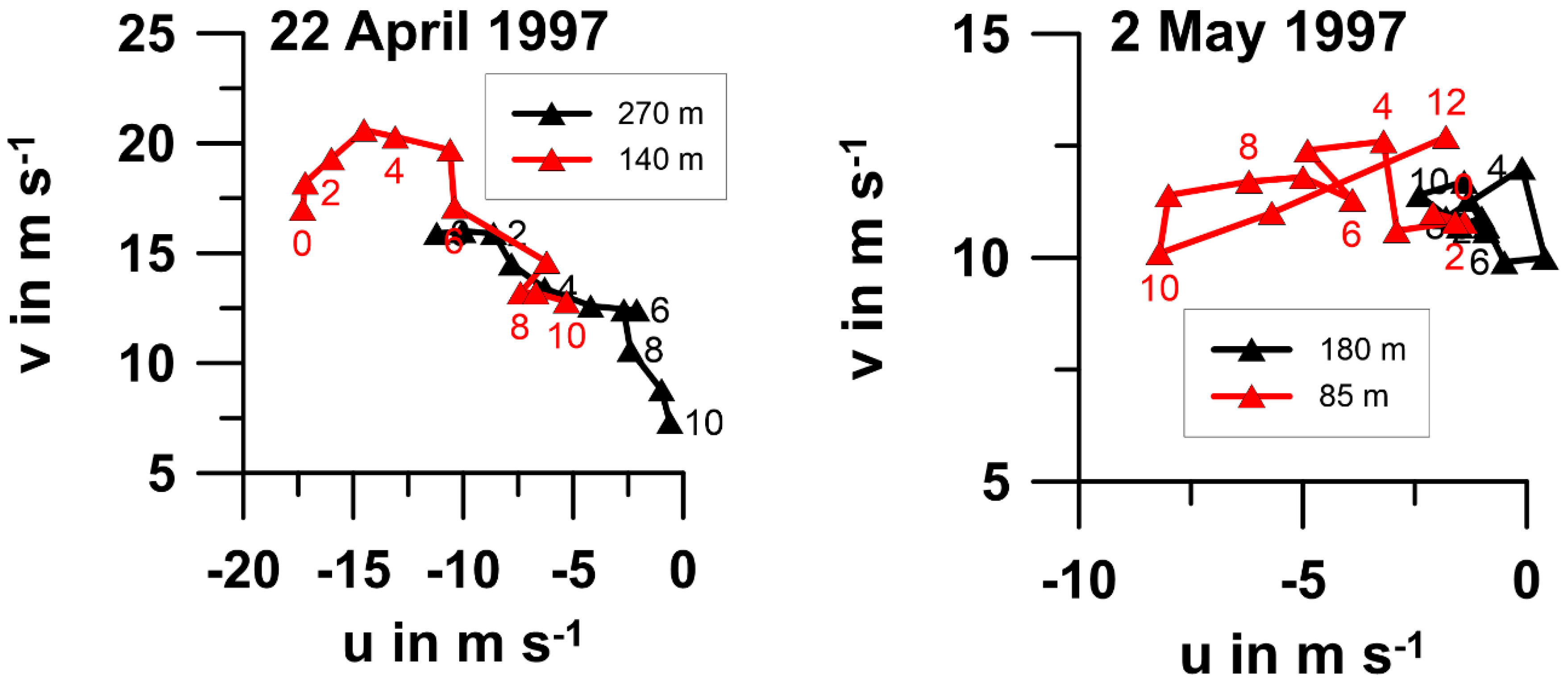
References
- Rasmussen, L. Greenland Winds and Satellite Imagery. Vejret Dan. Meteorol. Soc. 1989, 32–37. [Google Scholar]
- Heinemann, G.; Klein, T. Modelling and observations of the katabatic flow dynamics over Greenland. Tellus A Dyn. Meteorol. Oceanogr. 2002, 54, 542–554. [Google Scholar] [CrossRef]
- Van den Broeke, M.R.; Duynkerke, P.G.; Henneken, E.A.C. Heat, momentum and moisture budgets of the katabatic layer over the melting zone of the west Greenland ice sheet in summer. Bound. Layer Meteorol. 1994, 71, 393–413. [Google Scholar] [CrossRef]
- Oerlemans, J.; Vugts, H.F. A Meteorological Experiment in the Melting Zone of the Greenland Ice Sheet. Bull. Am. Meteor. Soc. 1993, 74, 355–365. [Google Scholar] [CrossRef]
- Heinemann, G. The KABEG’97 field experiment: An aircraft-based study of katabatic wind dynamics over the Greenland ice sheet. Bound. Layer Meteorol. 1999, 93, 75–116. [Google Scholar] [CrossRef]
- Bromwich, D.H.; Cassano, J.J.; Klein, T.; Heinemann, G.; Hines, K.M.; Steffen, K.; Box, J.E. Mesoscale Modeling of Katabatic Winds over Greenland with the Polar MM5*. Mon. Weather Rev. 2001, 129, 2290–2309. [Google Scholar] [CrossRef]
- Klein, T.; Heinemann, G.; Gross, P. Simulation of the katabatic flow near the Greenland ice margin using a high-resolution nonhydrostatic model. Meteorol. Z. 2001, 10, 331–339. [Google Scholar] [CrossRef]
- Klein, T.; Heinemann, G.; Bromwich, D.H.; Cassano, J.J.; Hines, K.M. Mesoscale modeling of katabatic winds over Greenland and comparisons with AWS and aircraft data. Meteorol. Atmos. Phys. 2001, 78, 115–132. [Google Scholar] [CrossRef][Green Version]
- Sedlar, J.; Tjernström, M.; Rinke, A.; Orr, A.; Cassano, J.; Fettweis, X.; Heinemann, G.; Seefeldt, M.; Solomon, A.; Matthes, H.; et al. Confronting Arctic troposphere, clouds, and surface energy budget representations in regional climate models with observations. J. Geophys. Res. 2020. [Google Scholar] [CrossRef]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.A.; Balsamo, G.; Bauer, P.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Bromwich, D.H.; Wilson, A.B.; Bai, L.; Liu, Z.; Barlage, M.; Shih, C.-F.; Maldonado, S.; Hines, K.M.; Wang, S.-H.; Woollen, J.; et al. The Arctic System Reanalysis, Version 2. Bull. Am. Meteor. Soc. 2018, 99, 805–828. [Google Scholar] [CrossRef]
- Gutjahr, O.; Heinemann, G. A model-based comparison of extreme winds in the Arctic and around Greenland. Int. J. Clim. 2018, 38, 5272–5292. [Google Scholar] [CrossRef]
- Ettema, J.; van den Broeke, M.R.; van Meijgaard, E.; van de Berg, W.J.; Box, J.E.; Steffen, K. Climate of the Greenland ice sheet using a high-resolution climate model–Part 1: Evaluation. Cryosphere 2010, 4, 511–527. [Google Scholar] [CrossRef]
- Heinemann, G. Aircraft-Based Measurements of Turbulence Structures in The Katabatic Flow Over Greenland. Bound. Layer Meteorol. 2002, 103, 49–81. [Google Scholar] [CrossRef]
- Heinemann, G. Local Similarity Properties of the Continuously Turbulent Stable Boundary Layer over Greenland. Bound. Layer Meteorol. 2004, 112, 283–305. [Google Scholar] [CrossRef]
- Drüe, C.; Heinemann, G. Airborne Investigation of Arctic Boundary-Layer Fronts over the Marginal Ice Zone of the Davis Strait. Bound. Layer Meteorol. 2001, 101, 261–292. [Google Scholar] [CrossRef]
- Steffen, K.; Box, J.E.; Abdalati, W. Greenland Climate Network: GC-Net. US Army Cold Reg. Reatt. Eng. 1996, 98–103. [Google Scholar]
- Rockel, B.; Will, A.; Hense, A. The Regional Climate Model COSMO-CLM (CCLM). Meteorol. Z. 2008, 17, 347–348. [Google Scholar] [CrossRef]
- Akperov, M.; Rinke, A.; Mokhov, I.I.; Matthes, H.; Semenov, V.A.; Adakudlu, M.; Cassano, J.; Christensen, J.H.; Dembitskaya, M.A.; Dethloff, K.; et al. Cyclone Activity in the Arctic From an Ensemble of Regional Climate Models (Arctic CORDEX). J. Geophys. Res. 2018, 123, 2537–2554. [Google Scholar] [CrossRef]
- Hersbach, H.; de Rosnay, P.; Bell, B.; Schepers, D.; Simmons, A.; Soci, C.; Abdalla, S.; Alonso-Balmaseda, M.; Balsamo, G.; Bechtold, P.; et al. Operational Global Reanalysis: Progress, Future Directions and Synergies with NWP; European Centre for Medium Range Weather Forecasts: Reading, UK, 2018. [Google Scholar]
- OSI SAF. Global Sea Ice Concentration Climate Data Record v2.0-Multimission; EUMETSAT: Darmstadt, Germany, 2017. [Google Scholar]
- Hastings, D.A.; Dunbar, P.K. Global Land One-kilometer Base Elevation (GLOBE) Digital Elevation Model, Documentation. Natl. Ocean. Atmos. Adm. 1999, 325, 80305–83328. [Google Scholar]
- Zentek, R. COSMO Documentation (Archived Version from 2019, Uploaded with Permission of the DWD). 2019. Available online: https://zenodo.org/record/3339384 (accessed on 13 May 2020).
- Gutjahr, O.; Heinemann, G.; Preußer, A.; Willmes, S.; Drüe, C. Quantification of ice production in Laptev Sea polynyas and its sensitivity to thin-ice parameterizations in a regional climate model. Cryosphere 2016, 10, 2999–3019. [Google Scholar] [CrossRef]
- Kohnemann, S.H.E.; Heinemann, G.; Bromwich, D.H.; Gutjahr, O. Extreme Warming in the Kara Sea and Barents Sea during the Winter Period 2000–16. J. Clim. 2017, 30, 8913–8927. [Google Scholar] [CrossRef]
- Zentek, R.; Heinemann, G. Verification of the regional atmospheric model CCLM v5.0 with conventional data and lidar measurements in Antarctica. Geosci. Model Dev. 2020, 13, 1809–1825. [Google Scholar] [CrossRef]
- Buzzi, M.; Rotach, M.W.; Raschendorfer, M.; Holtslag, A.A.M. Evaluation of the COSMO-SC turbulence scheme in a shear-driven stable boundary layer. Meteorol. Z. 2011, 20, 335–350. [Google Scholar] [CrossRef]
- Louis, J.-F. A parametric model of vertical eddy fluxes in the atmosphere. Bound. Layer Meteorol. 1979, 17, 187–202. [Google Scholar] [CrossRef]
- Mellor, G.L.; Yamada, T. A Hierarchy of Turbulence Closure Models for Planetary Boundary Layers. J. Atmos. Sci. 1974, 31, 1791–1806. [Google Scholar] [CrossRef]
- Cerenzia, I.; Tampieri, F.; Tesini, S. Diagnosis of Turbulence Schema in Stable Atmospheric Conditions and Sensitivity Tests. COSMO Newsl. 2014, 14, 1–11. [Google Scholar]
- Hebbinghaus, H.; Heinemann, G. LM simulations of the Greenland boundary layer, comparison with local measurements and SNOWPACK simulations of drifting snow. Cold Reg. Sci. Technol. 2006, 46, 36–51. [Google Scholar] [CrossRef]
- Souverijns, N.; Gossart, A.; Demuzere, M.; Lenaerts, J.T.M.; Medley, B.; Gorodetskaya, I.V.; Vanden Broucke, S.; van Lipzig, N.P.M. A New Regional Climate Model for POLAR-CORDEX: Evaluation of a 30-Year Hindcast with COSMO-CLM 2 Over Antarctica. J. Geophys. Res. 2019, 124, 1405–1427. [Google Scholar] [CrossRef]
- Heinemann, G.; Falk, U. Surface Winds and Energy Fluxes Near the Greenland Ice Margin under Conditions of Katabatic Winds. Polarforschung 2002, 71, 15–31. [Google Scholar]
- Renfrew, I.A.; Anderson, P.S. Profiles of katabatic flow in summer and winter over Coats Land, Antarctica. Q. J. R. Meteorol. Soc. 2006, 132, 779–802. [Google Scholar] [CrossRef]
- Vignon, E.; Hourdin, F.; Genthon, C.; Gallée, H.; Bazile, E.; Lefebvre, M.-P.; Madeleine, J.-B.; van de Wiel, B.J.H. Antarctic boundary layer parametrization in a general circulation model: 1-D simulations facing summer observations at Dome C. J. Geophys. Res. 2017, 122, 6818–6843. [Google Scholar] [CrossRef]
- Box, J.E.; Rinke, A. Evaluation of Greenland Ice Sheet Surface Climate in the HIRHAM Regional Climate Model Using Automatic Weather Station Data. J. Clim. 2003, 16, 1302–1319. [Google Scholar] [CrossRef]
- Stiperski, I.; Holtslag, A.A.M.; Lehner, M.; Hoch, S.W.; Whiteman, C.D. On the turbulence structure of deep katabatic flows on a gentle mesoscale slope. Q. J. R. Meteorol. Soc. 2020, 146, 1206–1231. [Google Scholar] [CrossRef]
- Grachev, A.A.; Leo, L.S.; Di Sabatino, S.; Fernando, H.J.S.; Pardyjak, E.R.; Fairall, C.W. Structure of Turbulence in Katabatic Flows Below and Above the Wind-Speed Maximum. Bound. Layer Meteorol. 2016, 159, 469–494. [Google Scholar] [CrossRef]
- Rinke, A.; Dethloff, K.; Cassano, J.J.; Christensen, J.H.; Curry, J.A.; Du, P.; Girard, E.; Haugen, J.-E.; Jacob, D.; Jones, C.G.; et al. Evaluation of an ensemble of Arctic regional climate models: Spatiotemporal fields during the SHEBA year. Clim. Dyn. 2006, 26, 459–472. [Google Scholar] [CrossRef]
- Drüe, C.; Heinemann, G. Characteristics of intermittent turbulence in the upper stable boundary layer over Greenland. Bound. Layer Meteorol. 2007, 124, 361–381. [Google Scholar] [CrossRef]

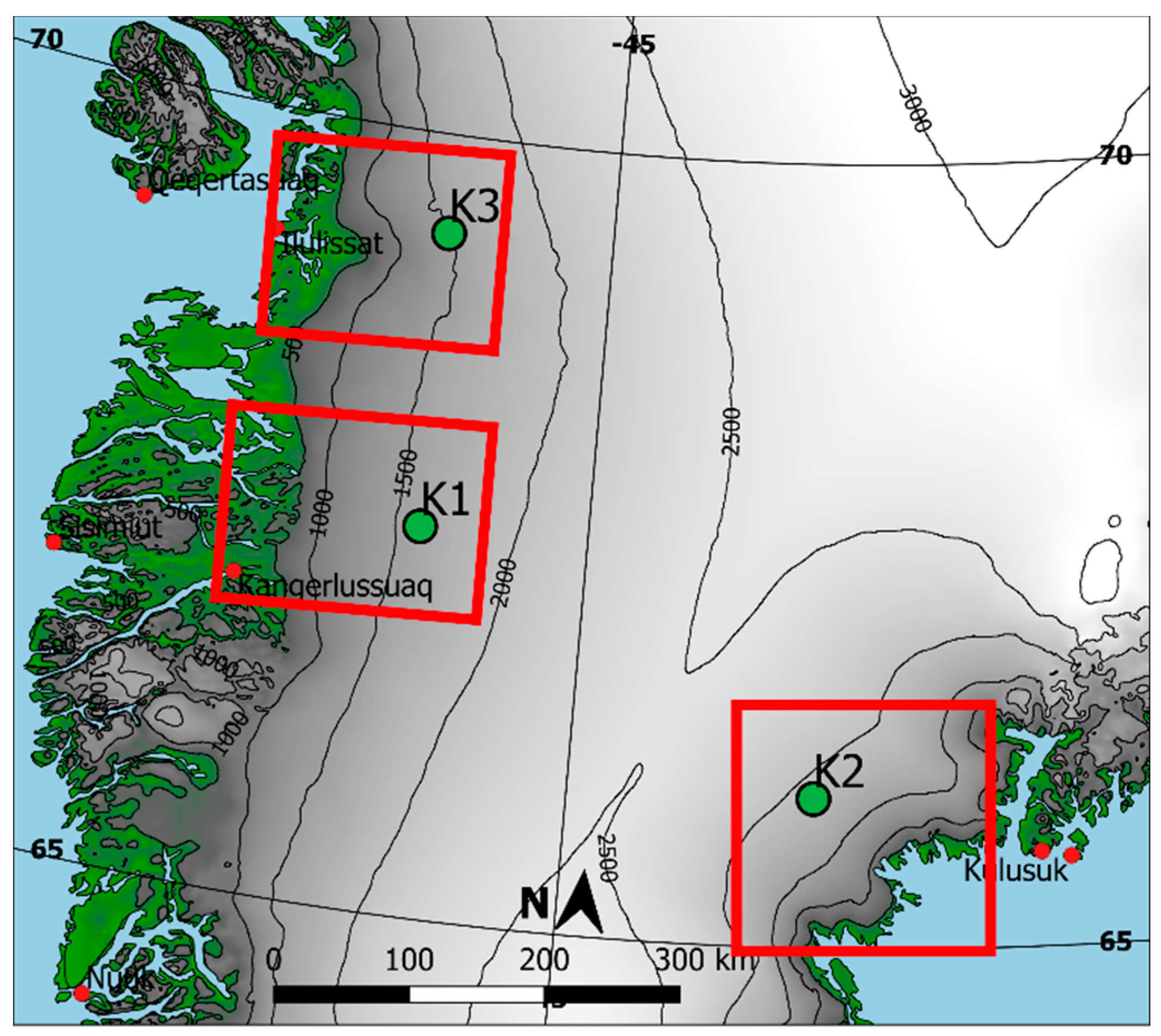
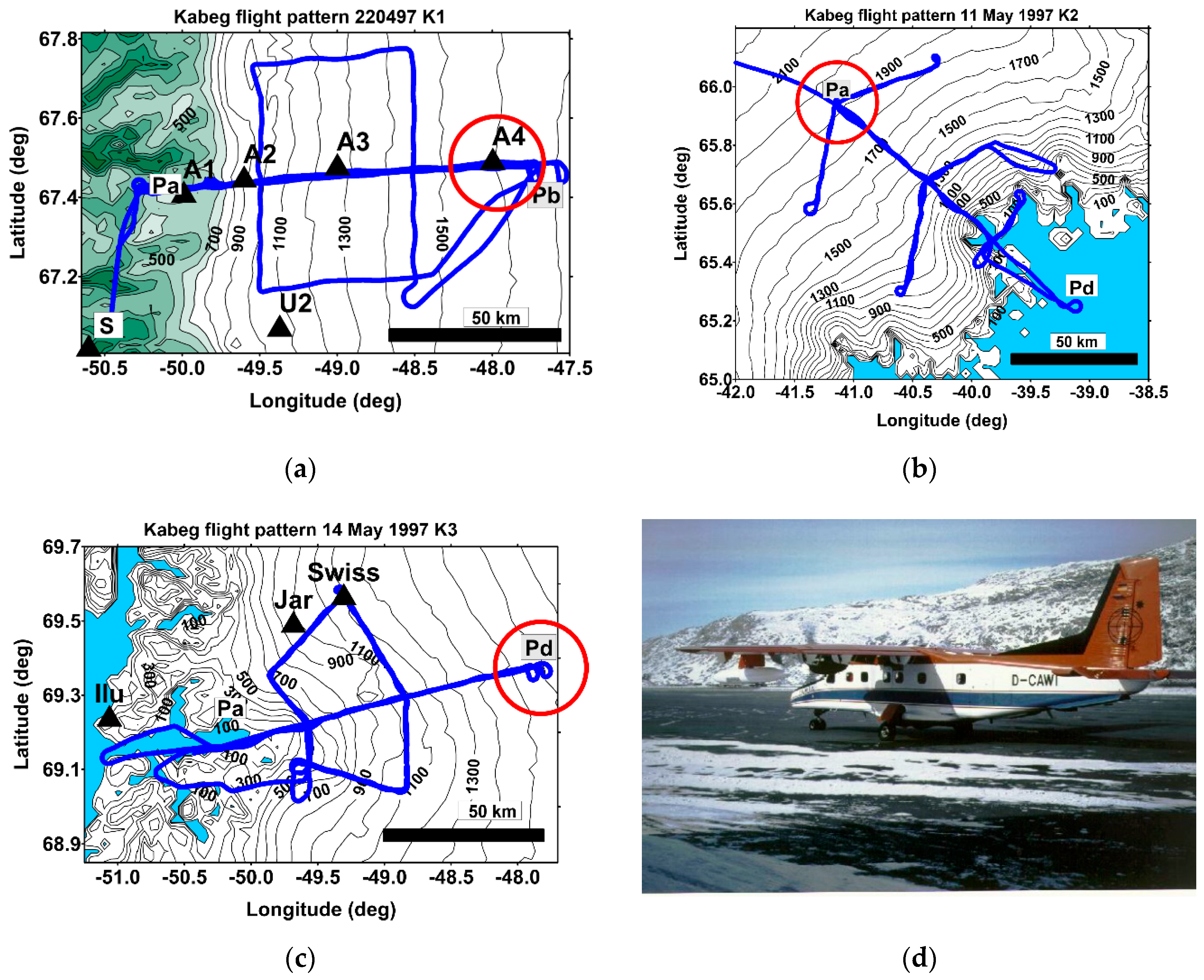


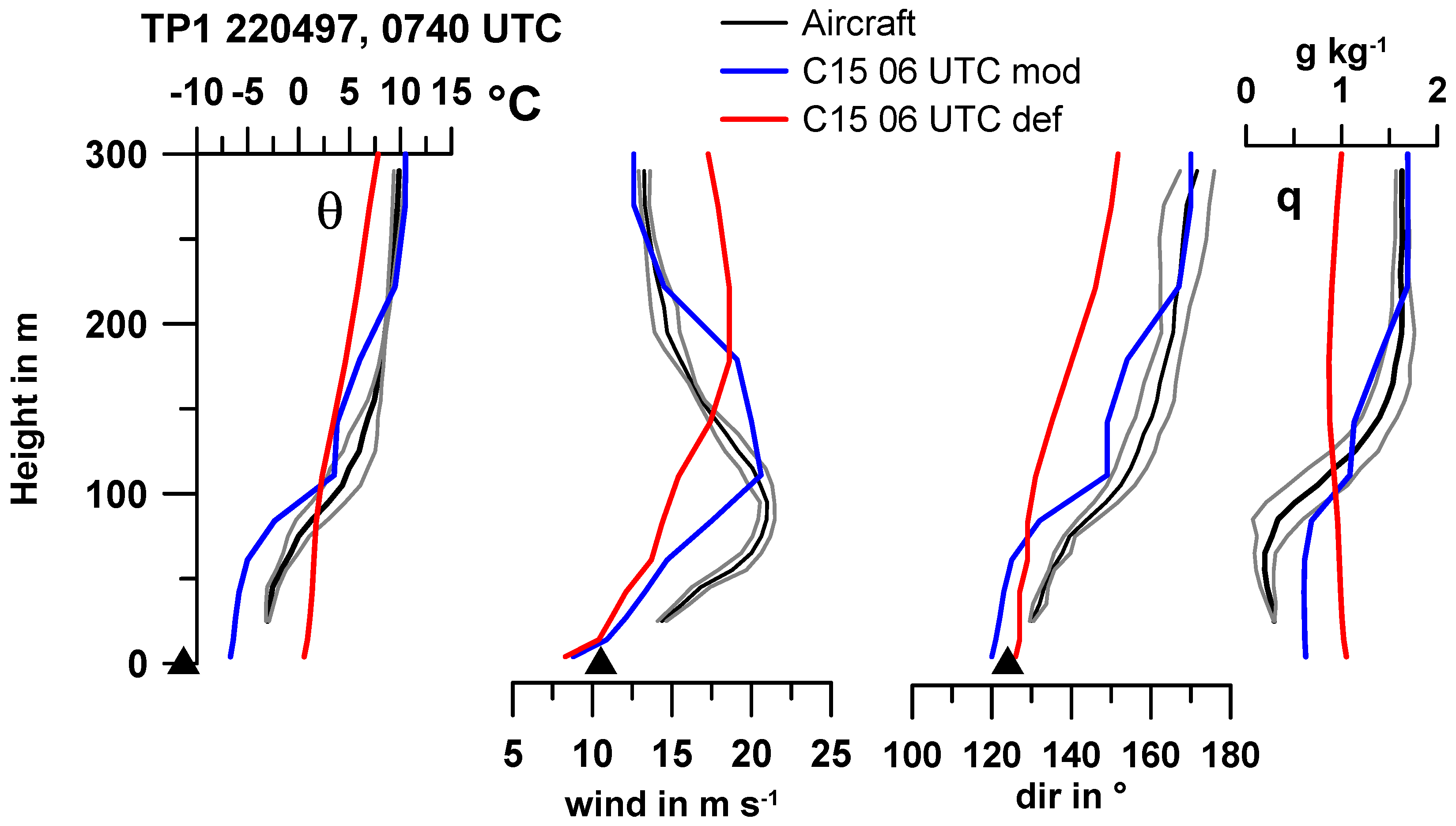
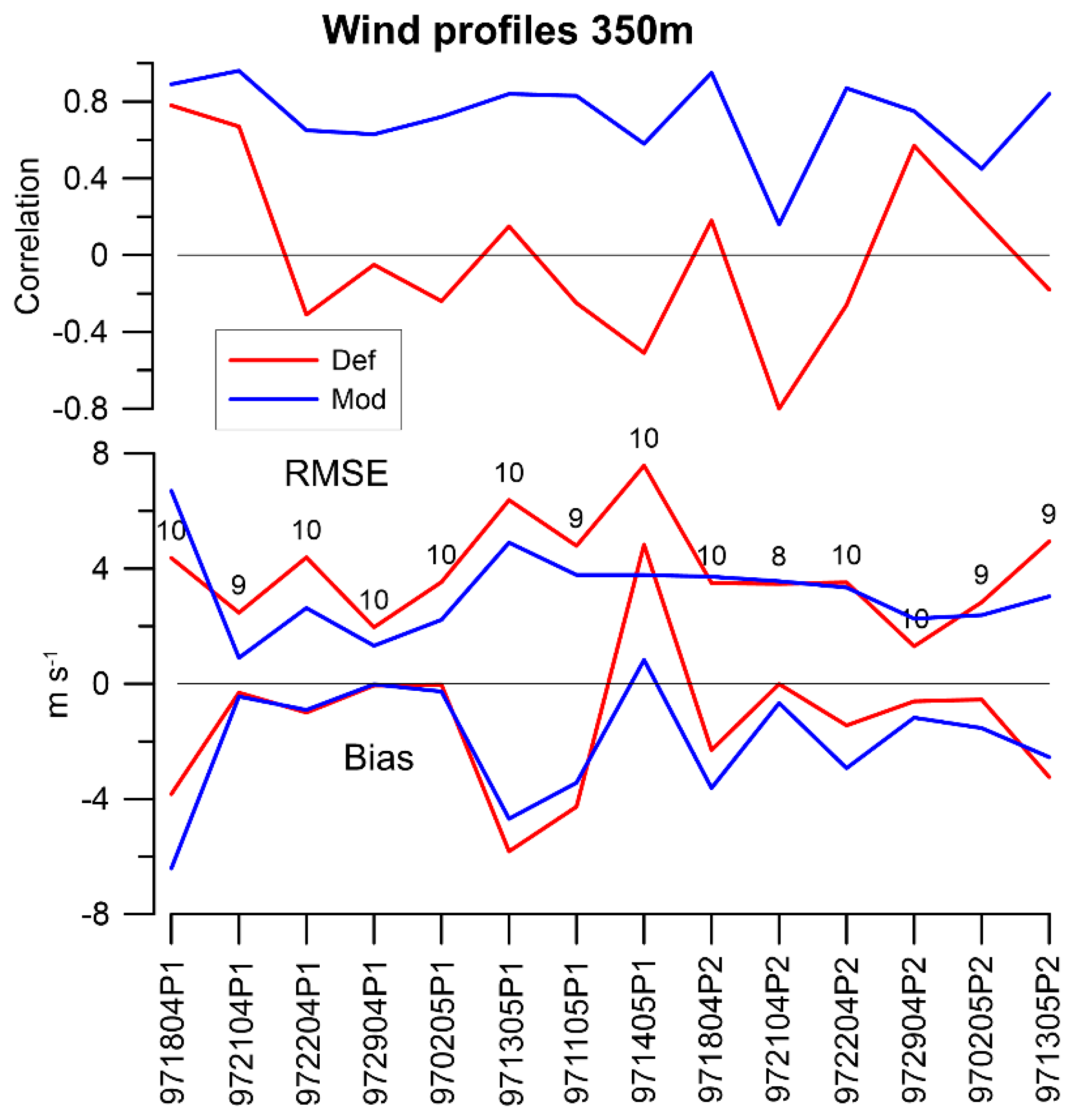
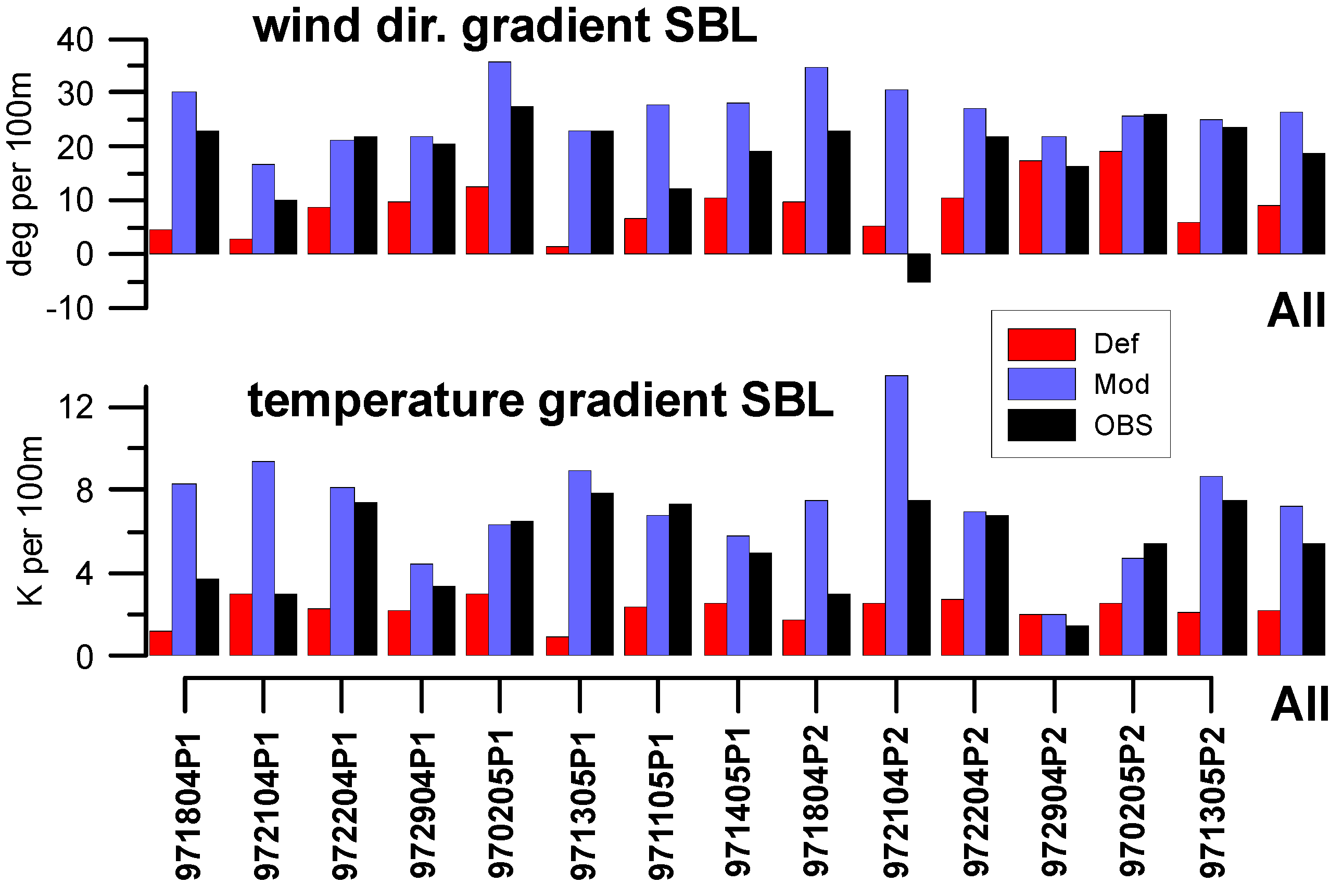

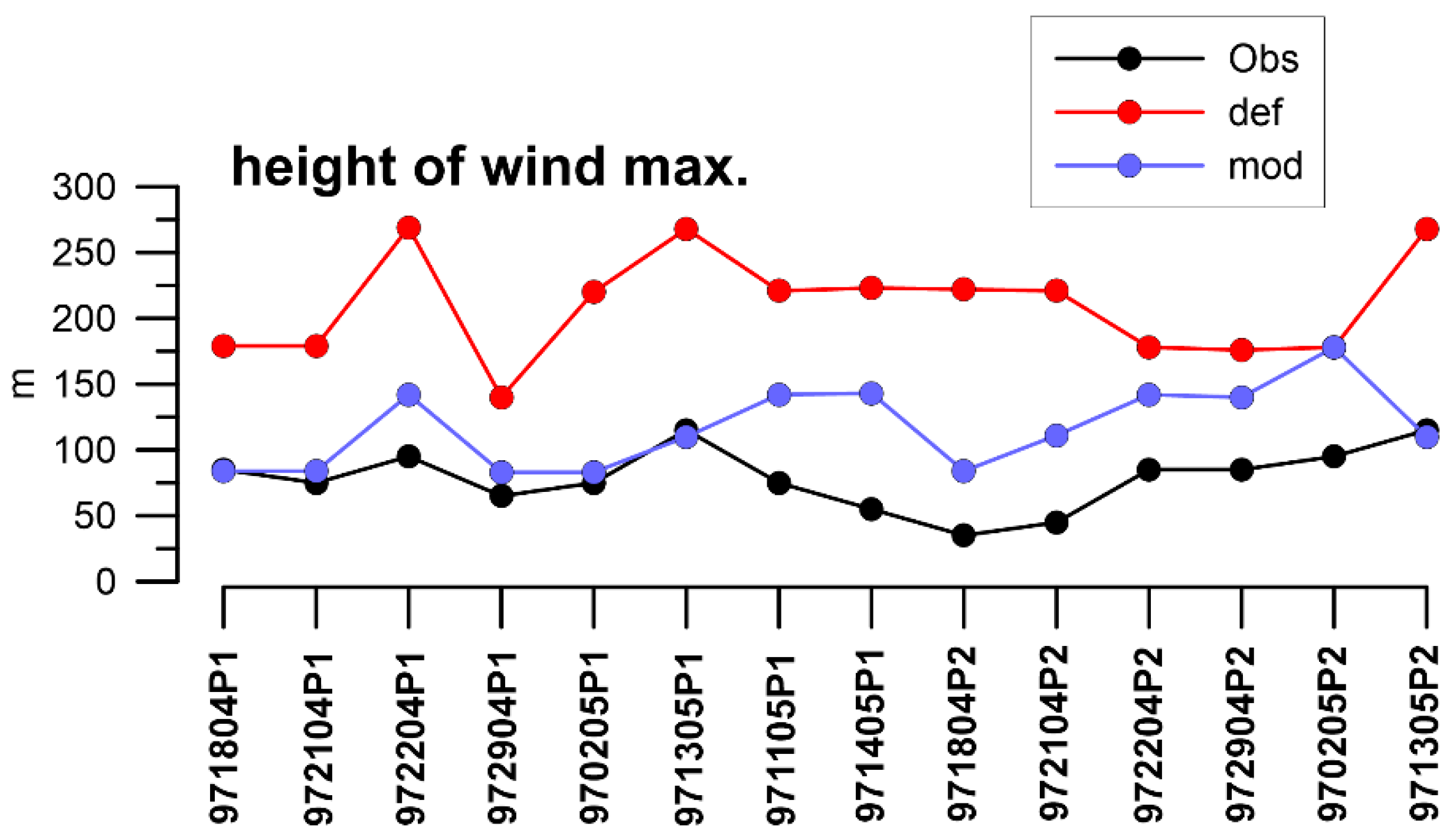
| Date Time of Flight | Flight, Area | Profile | Time for the Comparison CCLM/Aircraft | Wind Max Height/Strength | Location for Comparison, Lat/Long. |
|---|---|---|---|---|---|
| 18 April 1997 07:00–11:45 UTC | KA1, K1 | P1 P2 | 05:00/07:30 UTC 09:00/11:00 UTC | 85 m/15.4 ms−1 35 m/11.9 ms−1 | A4, 67.485/−47.800 |
| 21 April 1997 06:30–11:50 UTC | KA2, K1 | P1 P2 | 09:00/06:50 UTC 10:00/10:00 UTC | 75 m/13.6 ms−1 45 m/17.0 ms−1 | A4, 67.485/−47.800 |
| 22 April 1997 07:05–12:10 UTC | KA3, K1 | P1 P2 | 06:00/07:40 UTC 10:00/11:00 UTC | 95 m/21.0 ms−1 85 m/16.3 ms−1 | A4, 67.485/−47.800 |
| 29 April 1997 10:20–15:40 UTC | KA4, K1 | P1 P2 | 10:00/10:30 UTC 13:00/13:00 UTC | 65 m/10.1 ms−1 85 m/9.5 ms−1 | A4, 67.485/−47.800 |
| 2 May 1997 06:05–11:50 UTC | KA5 K1 | P1 P2 | 05:00/06:10 UTC 10:00/09:40 UTC | 75 m/14.4 ms−1 95 m/15.7 ms−1 | A4, 67.485/−47.800 |
| 11 May 1997 06:35–11:30 UTC | KA6, K2 | P1 | 08:00/06:00 UTC | 75 m/18.7 ms−1 | Pa, 65.947/−41.153 |
| 13 May 1997 06:00–12:05 UTC | KA8, K1 | P1 P2 | 08:00/07:50 UTC 09:00/08:40 UTC | 115 m/22.3 ms−1 115 m/20.6 ms−1 | A4, 67.485/−47.800 |
| 14 May 1997 06:00–11:35 UTC | KA9, K3 | P1 | 08:00/06:50 | 55 m/15.7 ms−1 | Pd, 69.367/−48.000 |
| Quantity, Simulation | Bias | RMSE | Corr. | AA 1 | Diff. AA (Sim–AWS) |
|---|---|---|---|---|---|
| Temp, def | 7.1 K | 7.9 K | 0.89 | 7.8 K | −5.0 K |
| Temp, mod | 4.6 K | 5.6 K | 0.88 | 12.5 K | −0.3 K |
| Wind, def | −0.5 m/s | 1.7 m/s | 0.76 | - | - |
| Wind, mod | −0.5 m/s | 1.6 m/s | 0.79 | - | - |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Heinemann, G. Assessment of Regional Climate Model Simulations of the Katabatic Boundary Layer Structure over Greenland. Atmosphere 2020, 11, 571. https://doi.org/10.3390/atmos11060571
Heinemann G. Assessment of Regional Climate Model Simulations of the Katabatic Boundary Layer Structure over Greenland. Atmosphere. 2020; 11(6):571. https://doi.org/10.3390/atmos11060571
Chicago/Turabian StyleHeinemann, Günther. 2020. "Assessment of Regional Climate Model Simulations of the Katabatic Boundary Layer Structure over Greenland" Atmosphere 11, no. 6: 571. https://doi.org/10.3390/atmos11060571
APA StyleHeinemann, G. (2020). Assessment of Regional Climate Model Simulations of the Katabatic Boundary Layer Structure over Greenland. Atmosphere, 11(6), 571. https://doi.org/10.3390/atmos11060571





