Contribution of Phoretic and Electrostatic Effects to the Collection Efficiency of Submicron Aerosol Particles by Raindrops
Abstract
1. Introduction and Theoretical Background
2. The Experimental Setup
2.1. The Drop Generator
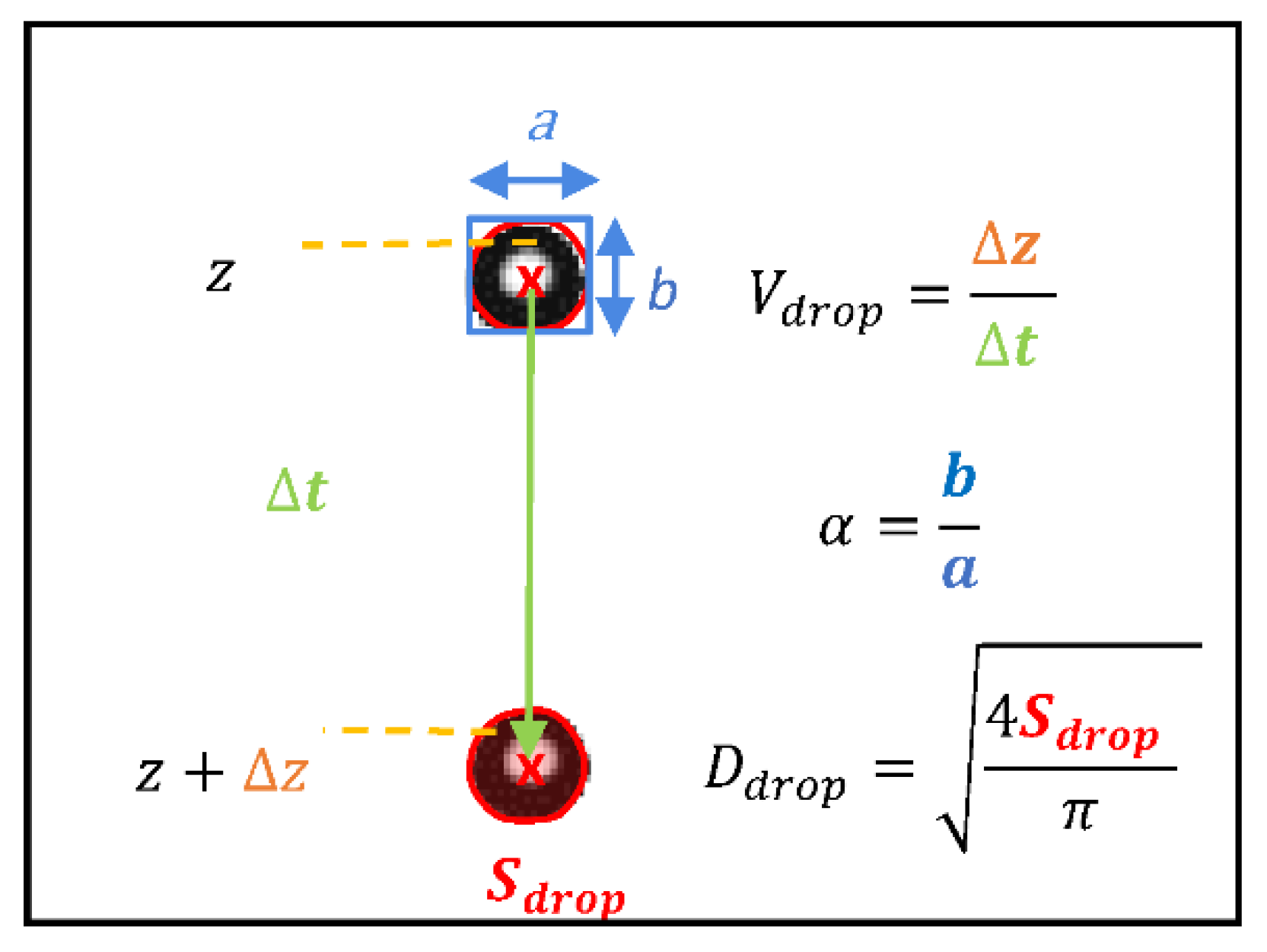
2.2. The Freefall Shaft
2.3. The Aerosol Chamber
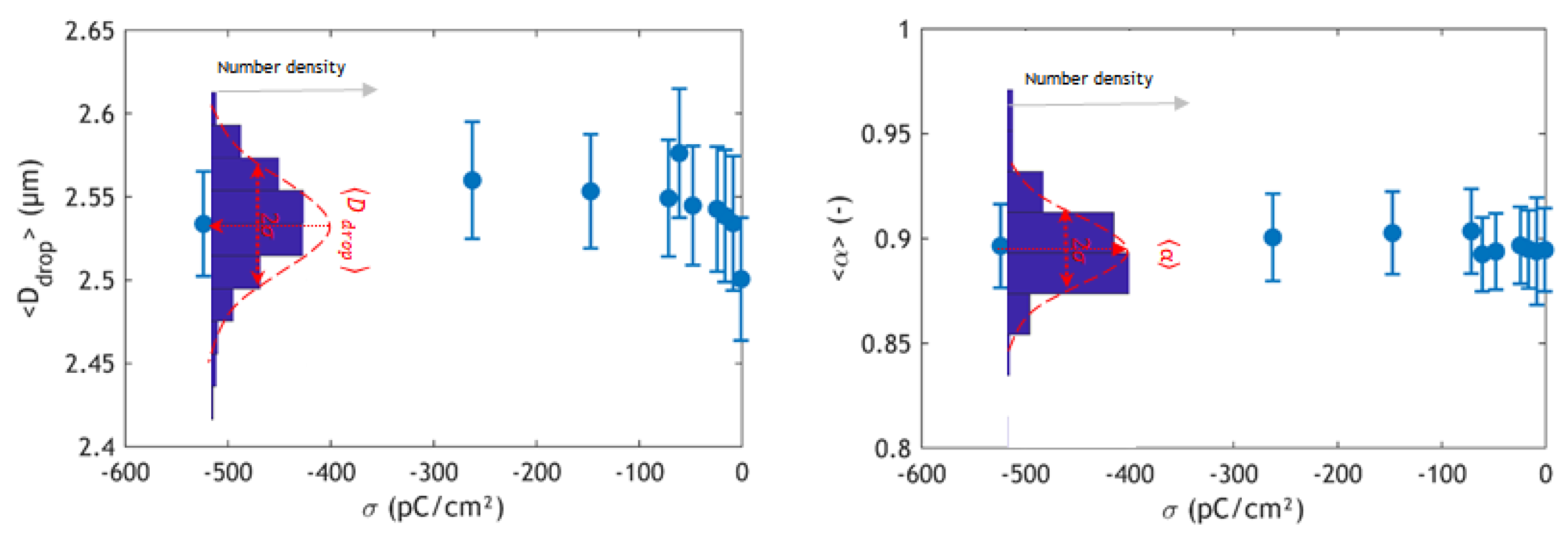
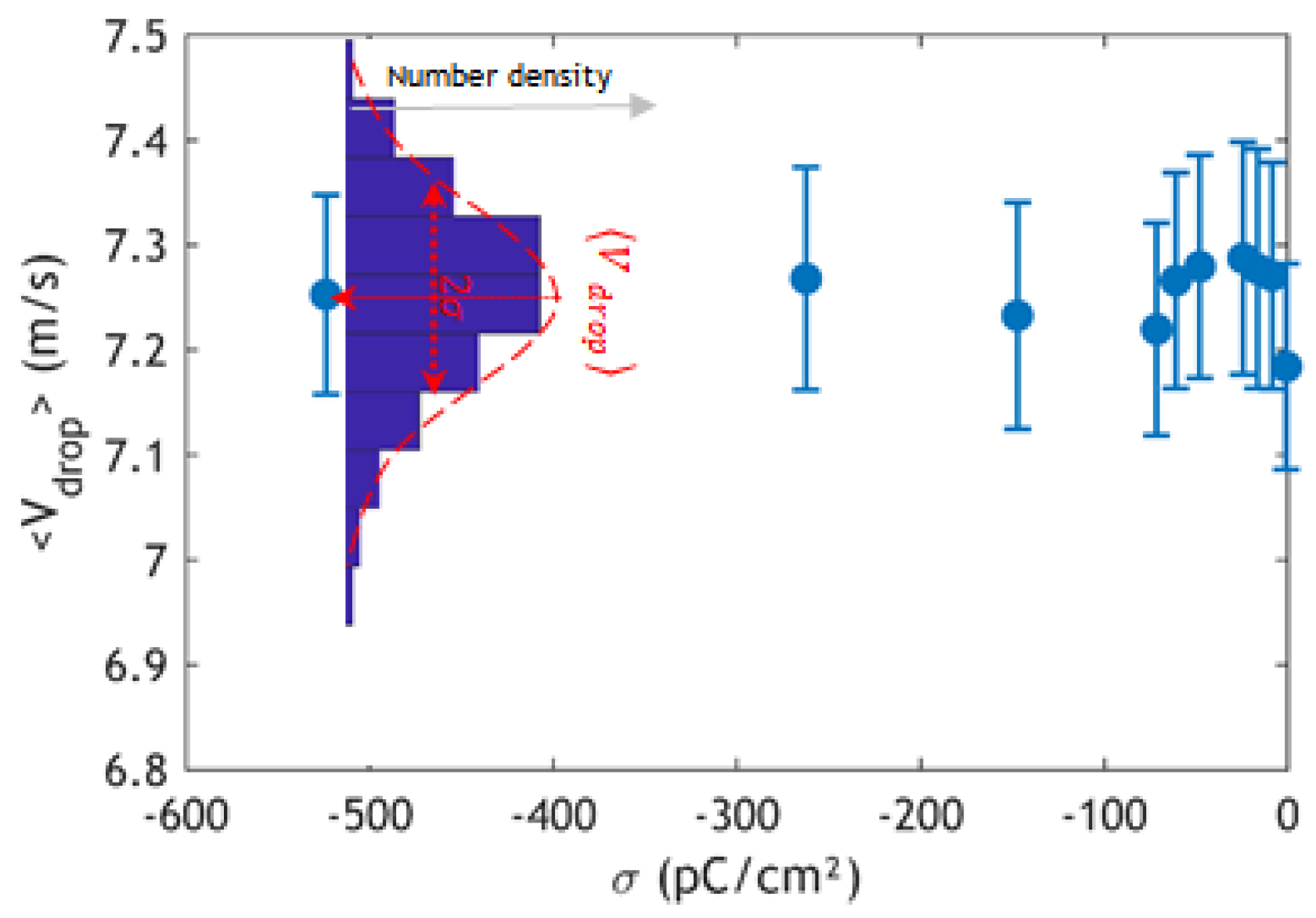
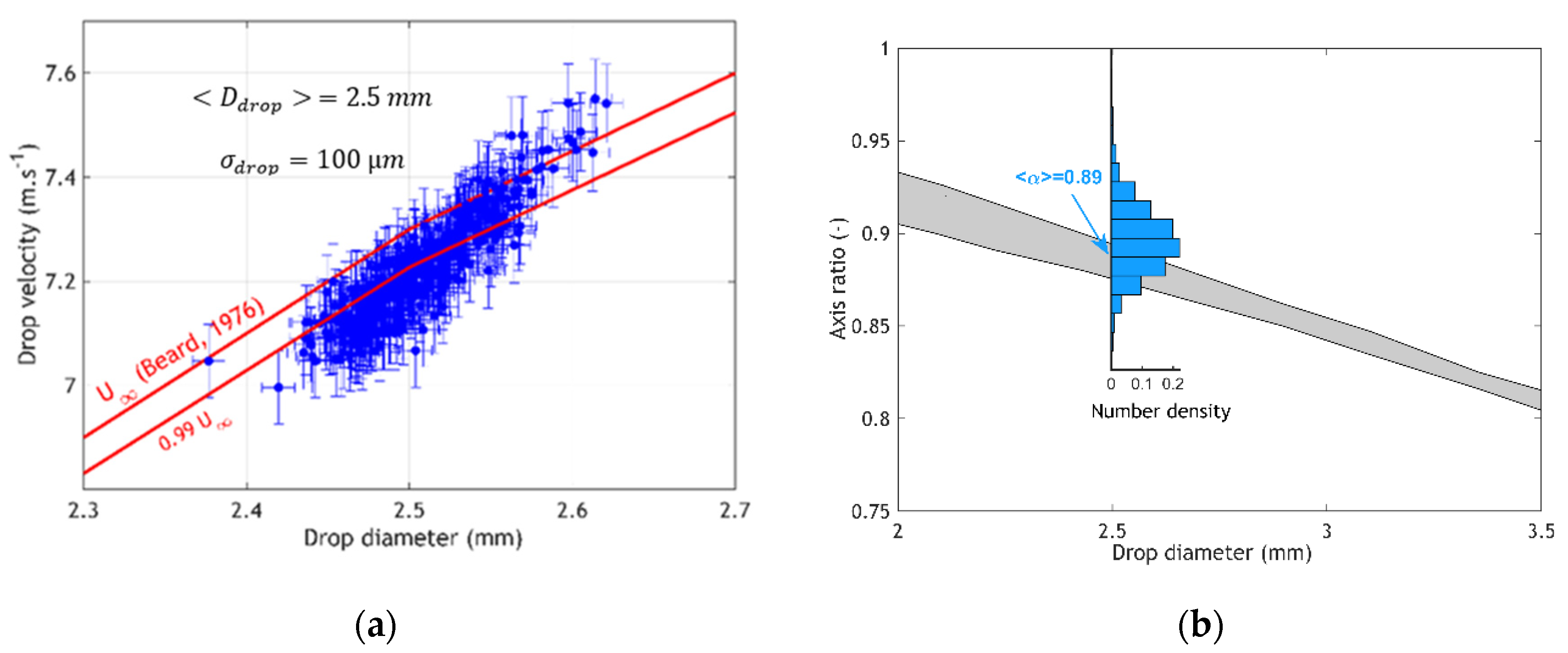
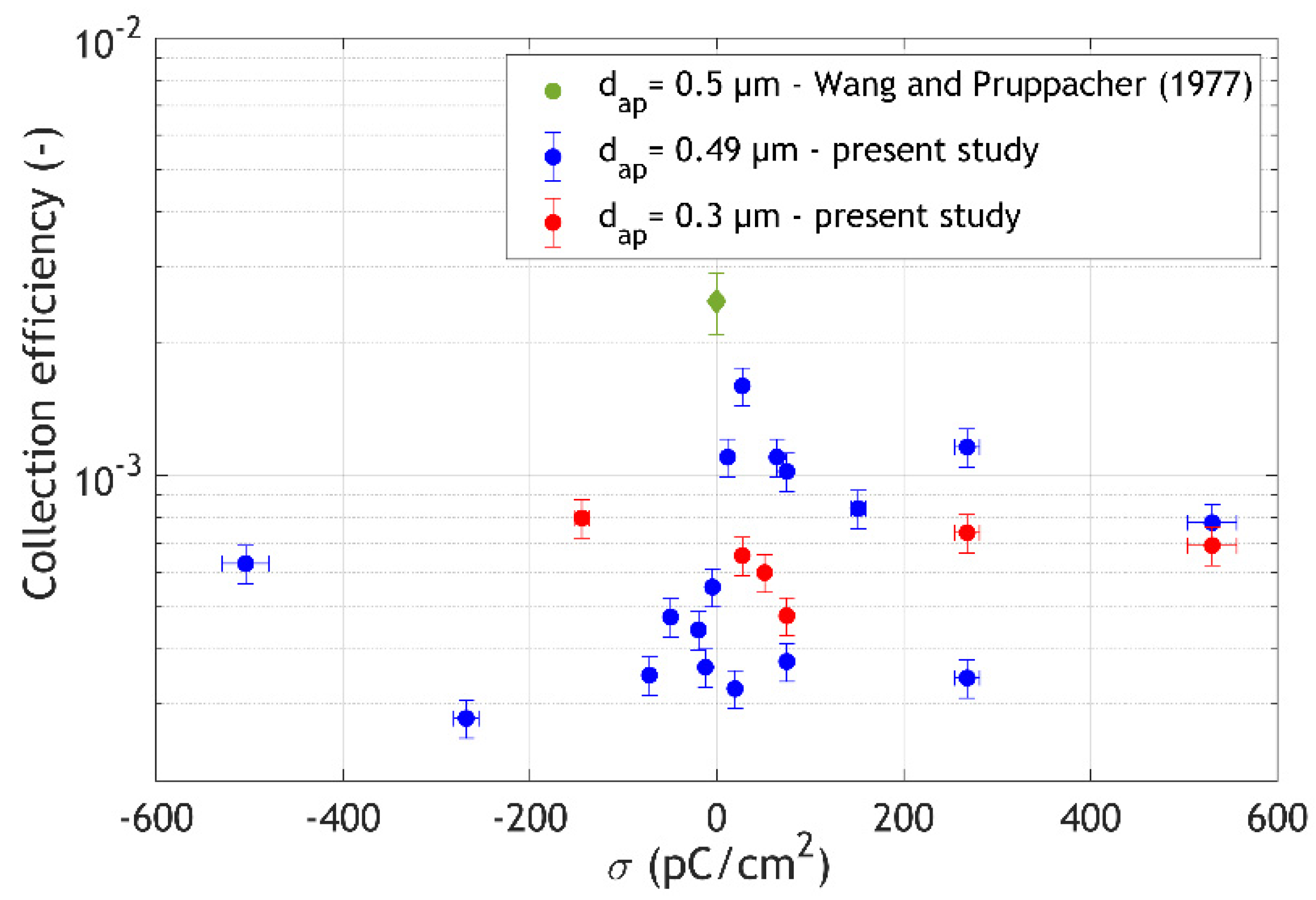
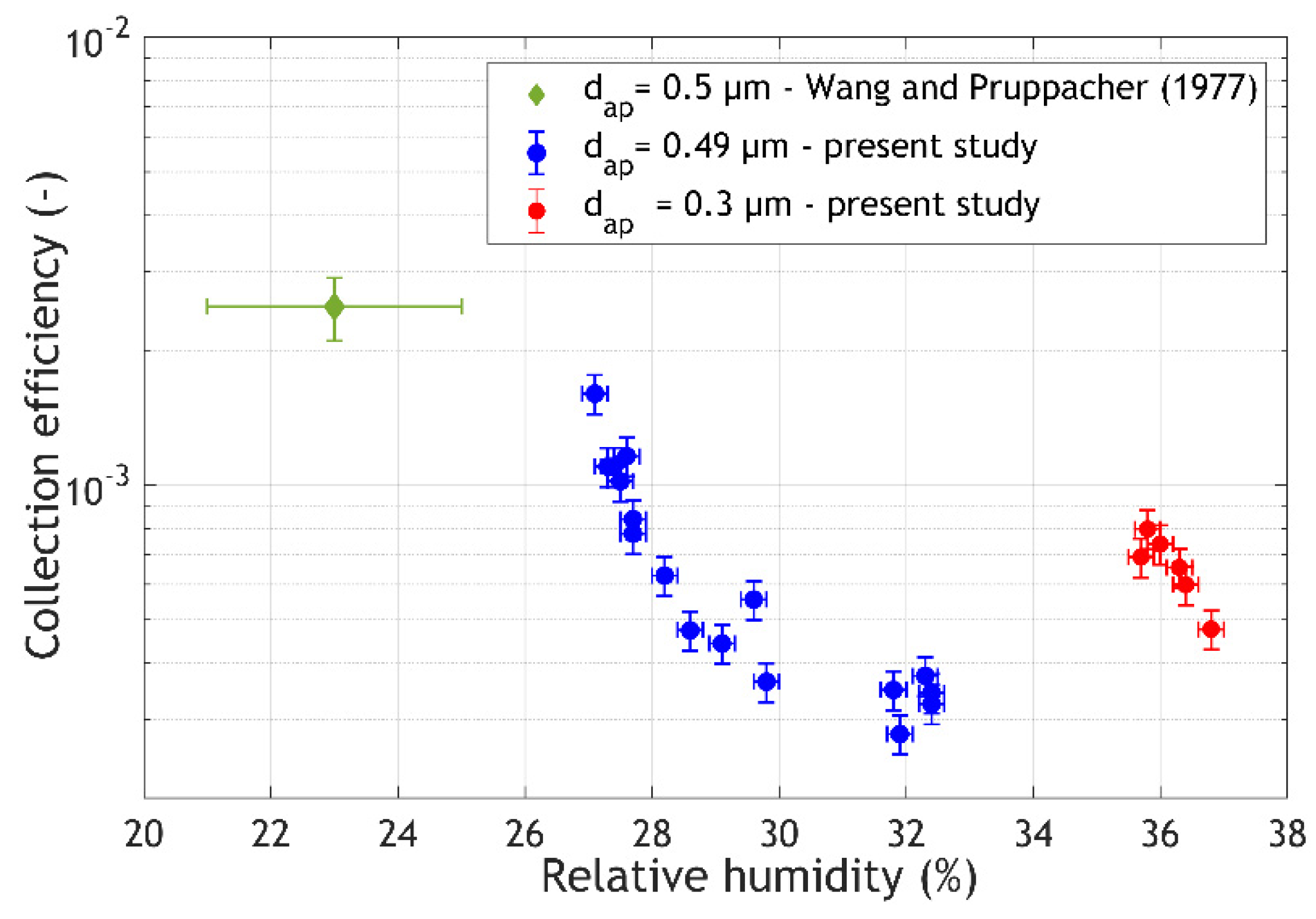
3. Results and Discussion
- ▪
- the size and charge of the aerosol particles,
- ▪
- the size and charge of the drops, and
- ▪
- the relative humidity in the aerosol chamber.
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Jaenicke, R. Tropospheric aerosols. In International Geophysics; Academic Press: Cambridge, MA, USA, 1993; Volume 54, pp. 1–31. [Google Scholar]
- Dockery, D.W.; Schwartz, J.; Spengler, J.D. Air pollution and daily mortality: Associations with particulates and acid aerosols. Environ. Res. 1992, 59, 362–373. [Google Scholar] [CrossRef]
- Bristow, C.S.; Hudson-Edwards, K.A.; Chappell, A. Fertilizing the Amazon and equatorial Atlantic with West African dust. Geophys. Res. Lett. 2010, 37. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Klett, J.D. Microstructure of atmospheric clouds and precipitation. In Microphysics of Clouds and Precipitation (Section 12.4); Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Twomey, S. Pollution and the planetary albedo. Atmos. Environ. (1967) 1974, 8, 1251–1256. [Google Scholar] [CrossRef]
- Hegerl, G.C.; Zwiers, F.W.; Braconnot, P.; Gillett, N.P.; Luo, Y.; Marengo Orsini, J.A.; Nicholls, N.; Penner, J.E.; Stott, P.A. Understanding and Attributing Climate Change; Cambridge University Press: Cambridge, UK, 2007. [Google Scholar]
- Baklanov, A.; Sørensen, J. Parameterisation of radionuclide deposition in atmospheric long-range transport modelling. Phys. Chem. Earth Part B Hydrol. Oceans Atmos. 2001, 26, 787–799. [Google Scholar] [CrossRef]
- Mathieu, A.; Kajino, M.; Korsakissok, I.; Périllat, R.; Quélo, D.; Querel, A.; Saunier, O.; Sekiyama, T.T.; Igarashi, Y.; Didier, D. Fukushima Daiichi–derived radionuclides in the atmosphere, transport and deposition in Japan: A review. Appl. Geochem. 2018, 91, 122–139. [Google Scholar] [CrossRef]
- Flossmann, A.I. Interaction of Aerosol Particles and Clouds. J. Atmos. Sci. 1998, 55, 879–887. [Google Scholar] [CrossRef]
- Laguionie, P.; Roupsard, P.; Maro, D.; Solier, L.; Rozet, M.; Hébert, D.; Connan, O. Simultaneous quantification of the contributions of dry, washout and rainout deposition to the total deposition of particle-bound 7 Be and 210 Pb on an urban catchment area on a monthly scale. J. Aerosol Sci. 2014, 77, 67–84. [Google Scholar] [CrossRef]
- Depee, A.; Lemaitre, P.; Gelain, T.; Mathieu, A.; Monier, M.; Flossmann, A. Theoretical study of aerosol particle electroscavenging by clouds. J. Aerosol Sci. 2019, 135, 1–20. [Google Scholar] [CrossRef]
- Jaenicke, R. Physical aspects of the atmospheric aerosol. In Chemistry of the Unpolluted and Polluted Troposphere; Springer: Dordrecht, The Netherlands, 1982; pp. 341–373. [Google Scholar]
- Whitby, K.T. The physical characteristics of sulfur aerosols. Atmos. Environ. (1967) 1978, 12, 135–159. [Google Scholar] [CrossRef]
- Slinn, W.G. Precipitation Scavenging: Some Problems, Approximate Solutions and Suggestions for Future Research; No. BNWL-SA-5062; Battelle Pacific Northwest Labs.: Richland, WA, USA, 1974. [Google Scholar]
- Slinn, W. Some approximations for the wet and dry removal of particles and gases from the atmosphere. Water Air Soil Pollut. 1977, 7, 513–543. [Google Scholar] [CrossRef]
- Wang, P.K.; Pruppacher, H.R. An Experimental Determination of the Efficiency with Which Aerosol Particles are Collected by Water Drops in Subsaturated Air. J. Atmos. Sci. 1977, 34, 1664–1669. [Google Scholar] [CrossRef]
- Beard, K.V. Experimental and numerical collision efficiencies for submicron particles scavenged by small raindrops. J. Atmos. Sci. 1974, 31, 1595–1603. [Google Scholar] [CrossRef][Green Version]
- Grover, S.N.; Pruppacher, H.R.; Hamielec, A.E. A Numerical Determination of the Efficiency with Which Spherical Aerosol Particles Collide with Spherical Water Drops Due to Inertial Impaction and Phoretic and Electrical Forces. J. Atmos. Sci. 1977, 34, 1655–1663. [Google Scholar] [CrossRef]
- Greenfield, S.M. Rain Scavenging of Radioactive Particulate Matter from The Atmosphere. J. Meteorol. 1957, 14, 115–125. [Google Scholar] [CrossRef]
- Santachiara, G.; Prodi, F.; Belosi, F. A Review of Termo- and Diffusio-Phoresis in the Atmospheric Aerosol Scavenging Process. Part 1: Drop Scavenging. Atmos. Clim. Sci. 2012, 2, 148–158. [Google Scholar] [CrossRef]
- Dépée, A.; Lemaitre, P.; Gelain, T.; Monier, M.; Flossmann, A. Laboratory study of the collection efficiency of submicron aerosol particles by cloud droplets. Part I—Influence of relative humidity. Atmos. Chem. Phys. Discuss. 2020. in review. [Google Scholar] [CrossRef]
- Lai, K.Y.; Dayan, N.; Kerker, M. Scavenging of aerosol particles by a falling water drop. J. Atmos. Sci. 1978, 35, 674–682. [Google Scholar] [CrossRef]
- Barlow, A.K.; Latham, J. A laboratory study of the scavenging of sub-micron aerosol by charged raindrops. Q. J. R. Meteorol. Soc. 1983, 109, 763–770. [Google Scholar] [CrossRef]
- Pranesha, T.S.; Kamra, A.K. Scavenging of aerosol particles by large water drops: Neutral case. J. Geophys. Res. Space Phys. 1996, 101, 23373–23380. [Google Scholar] [CrossRef]
- Tinsley, B.A.; Rohrbaugh, R.P.; Hei, M.; Beard, K.V. Effects of image charges on the scavenging of aerosol particles by cloud droplets and on droplet charging and possible ice nucleation processes. J. Atmos. Sci. 2000, 57, 2118–2134. [Google Scholar] [CrossRef]
- Dépée, A.; Lemaitre, P.; Gelain, T.; Monier, M.; Flossmann, A. Laboratory study of the collection efficiency of submicron aerosol particles by cloud droplets. Part II—Influence of electric charges. Atmos. Chem. Phys. Discuss. 2020. in review. [Google Scholar] [CrossRef]
- Grover, S.N.; Beard, K.V. A Numerical Determination of the Efficiency with which Electrically Charged Cloud Drops and Small Raindrops Collide with Electrically Charged Spherical Particles of Various Densities. J. Atmos. Sci. 1975, 32, 2156–2165. [Google Scholar] [CrossRef]
- Cherrier, G.; Belut, E.; Gerardin, F.; Tanière, A.; Rimbert, N. Aerosol particles scavenging by a droplet: Microphysical modeling in the Greenfield gap. Atmos. Environ. 2017, 166, 519–530. [Google Scholar] [CrossRef]
- Quérel, A.; Lemaitre, P.; Monier, M.; Porcheron, E.; Flossmann, A.I.; Hervo, M. An experiment to measure raindrop collection efficiencies: Influence of rear capture. Atmos. Meas. Tech. 2014, 7, 1321–1330. [Google Scholar] [CrossRef]
- Kerker, M.; Hampl, V. Scavenging of Aerosol Particles by a Failing Water Drop and Calculation of Washout Coefficients. J. Atmos. Sci. 1974, 31, 1368–1376. [Google Scholar] [CrossRef]
- Vohl, O.; Mitra, S.; Wurzler, S.; Diehl, K.; Pruppacher, H. Collision efficiencies empirically determined from laboratory investigations of collisional growth of small raindrops in a laminar flow field. Atmos. Res. 2007, 85, 120–125. [Google Scholar] [CrossRef]
- Lemaitre, P.; Querel, A.; Monier, M.; Menard, T.; Porcheron, E.; Flossmann, A.I. Experimental evidence of the rear capture of aerosol particles by raindrops. Atmos. Chem. Phys. Discuss. 2017, 17, 4159–4176. [Google Scholar] [CrossRef]
- Ladino, L.A.; Stetzer, O.; Hattendorf, B.; Günther, D.; Croft, B.; Lohmann, U. Experimental Study of Collection Efficiencies between Submicron Aerosols and Cloud Droplets. J. Atmos. Sci. 2011, 68, 1853–1864. [Google Scholar] [CrossRef]
- Ardon-Dryer, K.; Huang, Y.-W.; Cziczo, D.J. Laboratory studies of collection efficiency of sub-micrometer aerosol particles by cloud droplets on a single-droplet basis. Atmos. Chem. Phys. Discuss. 2015, 15, 9159–9171. [Google Scholar] [CrossRef]
- Szakáll, M.; Diehl, K.; Mitra, S.K.; Borrmann, S. A Wind Tunnel Study on the Shape, Oscillation, and Internal Circulation of Large Raindrops with Sizes between 2.5 and 7.5 mm. J. Atmos. Sci. 2009, 66, 755–765. [Google Scholar] [CrossRef]
- Bringi, V.N.; Chandrasekar, V.; Hubbert, J.; Gorgucci, E.; Randeu, W.L.; Schoenhuber, M. Raindrop Size Distribution in Different Climatic Regimes from Disdrometer and Dual-Polarized Radar Analysis. J. Atmos. Sci. 2003, 60, 354–365. [Google Scholar] [CrossRef]
- Volken, M.; Schumann, T. A Critical review of below-cloud aerosol scavenging results on Mt. Rigi. Water Air Soil Pollut. 1993, 68, 15–28. [Google Scholar] [CrossRef]
- Laakso, L.; Grönholm, T.; Rannik, Ü.; Kosmale, M.; Fiedler, V.; Vehkamäki, H.; Kulmala, M. Ultrafine particle scavenging coefficients calculated from 6 years field measurements. Atmos. Environ. 2003, 37, 3605–3613. [Google Scholar] [CrossRef]
- Chate, D.; Pranesha, T. Field studies of scavenging of aerosols by rain events. J. Aerosol Sci. 2004, 35, 695–706. [Google Scholar] [CrossRef]
- Depuydt, G. Etude Expérimentale in situ du Potentiel de Lessivage de L’Aérosol Atmosphérique par les Precipitations. Ph.D. Dissertation, Institut National Polytechnique de Toulouse, Toulouse, France, 2013. [Google Scholar]
- Querel, A.; Monier, M.; Flossmann, A.I.; Lemaitre, P.; Porcheron, E. The importance of new collection efficiency values including the effect of rear capture for the below-cloud scavenging of aerosol particles. Atmos. Res. 2014, 142, 57–66. [Google Scholar] [CrossRef]
- Sow, M.; Lemaitre, P. The effect of electrostatic charges on the removal of radioactive aerosols in the atmosphere by raindrops. J. Phys. Conf. Ser. 2015, 646, 012011. [Google Scholar] [CrossRef]
- Sow, M.; Lemaitre, P. Influence of electric charges on the washout efficiency of atmospheric aerosols by raindrops. Ann. Nucl. Energy 2016, 93, 107–113. [Google Scholar] [CrossRef]
- Takahashi, T. Measurement of electric charge of cloud droplets, drizzle, and raindrops. Rev. Geophys. 1973, 11, 903. [Google Scholar] [CrossRef]
- Adam, J.R.; Semonin, R.G. Collection Efficiency of Raindrops for Submicron Particulates; No. CONF-700601; Illinois State Water Survey: Urbana, IL, USA, 1971. [Google Scholar]
- Stefan, J. Über die Verdampfung aus einem kreisförmig oder elliptisch begrenzten Becken. Ann. Phys. 1882, 253, 550–560. [Google Scholar]
- Bowman, C.T. Course Notes on Combustion; Stanford University Course Reference Material for ME 371: Fundamentals of Combustion; Stanford University: Stanford, CA, USA, 2004. [Google Scholar]
- Schmitt, K.H.; Waldmann, L. Untersuchungen an Schwebstoffteilchen in diffundierenden Gasen. Z. Nat. A 1960, 15, 843–851. [Google Scholar] [CrossRef]
- LeClair, B.P.; Hamielec, A.E.; Pruppacher, H.R.; Hall, W.D. A Theoretical and Experimental Study of the Internal Circulation in Water Drops Falling at Terminal Velocity in Air. J. Atmos. Sci. 1972, 29, 728–740. [Google Scholar] [CrossRef]
- Adrian, R.J. Particle-Imaging Techniques for Experimental Fluid Mechanics. Annu. Rev. Fluid Mech. 1991, 23, 261–304. [Google Scholar] [CrossRef]
- Wang, P.K.; Pruppacher, H.R. Acceleration to Terminal Velocity of Cloud and Raindrops. J. Appl. Meteorol. 1977, 16, 275–280. [Google Scholar] [CrossRef]
- Beard, K.V. Terminal Velocity Adjustment for Cloud and Precipitation Drops Aloft. J. Atmos. Sci. 1977, 34, 1293–1298. [Google Scholar] [CrossRef]
- Szakáll, M.; Mitra, S.K.; Diehl, K.; Borrmann, S. Shapes and oscillations of falling raindrops—A review. Atmos. Res. 2010, 97, 416–425. [Google Scholar] [CrossRef]
- Zrnic, D.S.; Doviak, R.; Mahapatra, P.R. The effect of charge and electric field on the shape of raindrops. Radio Sci. 1984, 19, 75–80. [Google Scholar] [CrossRef]
- Andsager, K.; Beard, K.V.; Laird, N.F. Laboratory Measurements of Axis Ratios for Large Raindrops. J. Atmos. Sci. 1999, 56, 2673–2683. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Beard, K.V. A wind tunnel investigation of the internal circulation and shape of water drops falling at terminal velocity in air. Q. J. R. Meteorol. Soc. 1970, 96, 247–256. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Pitter, R.L. A semi-empirical determination of the shape of cloud and rain drops. J. Atmos. Sci. 1971, 28, 86–94. [Google Scholar]
- Green, A.W. An Approximation for the Shapes of Large Raindrops. J. Appl. Meteorol. 1975, 14, 1578–1583. [Google Scholar] [CrossRef]
- Beard, K.V.; Chuang, C. A New Model for the Equilibrium Shape of Raindrops. J. Atmos. Sci. 1987, 44, 1509–1524. [Google Scholar] [CrossRef]
- Mocho, V. Pollution Microbienne, Particulaire et Gazeuse d’un Espace Protégé par une ou Plusieurs Arrières de Confinement Dynamique. Ph.D. Thesis, University of Paris-Val-de-Marne, Paris, France, 1996. [Google Scholar]
- Glover, W.; Chan, H.-K. Electrostatic charge characterization of pharmaceutical aerosols using electrical low-pressure impaction (ELPI). J. Aerosol Sci. 2004, 35, 755–764. [Google Scholar] [CrossRef]
- Vehring, R.; Foss, W.R.; Lechuga-Ballesteros, D. Particle formation in spray drying. J. Aerosol Sci. 2007, 38, 728–746. [Google Scholar] [CrossRef]
- Hardy, D.; Walker, J.; Lemaitre, P.; Reid, J. High-Time Resolution Measurements of Droplet Evaporation Kinetics and Particle Crystallisation. Available online: https://eac2020.de/2020/07/31/id350-high-time-resolution-measurements-of-droplet-evaporation-kinetics-and-particle-crystallisation/ (accessed on 22 September 2020).
- Davis, M.H. Electrostatic Field and Force on a Dielectric Sphere near a Conducting Plane—A Note on the Application of Electrostatic Theory to Water Droplets. Am. J. Phys. 1969, 37, 26. [Google Scholar] [CrossRef]
- Kulkarni, P.; Baron, P.A.; Willeke, K. (Eds.) Aerosol Measurement: Principles, Techniques, and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Michel, R.; Maitrot, M.; Madru, R. Proprietes electriques du chlorure d’argent monocristallin et charge d’espace. J. Phys. Chem. Solids 1973, 34, 1039–1050. [Google Scholar] [CrossRef]
- Mansour, S.; Yahia, I.S.; Sakr, G.; Mansour, S.A. Electrical conductivity and dielectric relaxation behavior of fluorescein sodium salt (FSS). Solid State Commun. 2010, 150, 1386–1391. [Google Scholar] [CrossRef]
- Wang, P.K.; Grover, S.N.; Pruppacher, H.R. On the Effect of Electric Charges on the Scavenging of Aerosol Particles by Clouds and Small Raindrops. J. Atmos. Sci. 1978, 35, 1735–1743. [Google Scholar] [CrossRef]
- Young, K.C. The Role of Contact Nucleation in Ice Phase Initiation in Clouds. J. Atmos. Sci. 1974, 31, 768–776. [Google Scholar] [CrossRef]
- Slinn, W.G.N.; Hales, J.M. A Reevaluation of the Role of Thermophoresis as a Mechanism of In- and Below-Cloud Scavenging. J. Atmos. Sci. 1971, 28, 1465–1471. [Google Scholar] [CrossRef]
- Davenport, H.; Peters†, L.K. Field studies of atmospheric particulate concentration changes during precipitation. Atmos. Environ. (1967) 1978, 12, 997–1008. [Google Scholar] [CrossRef]
- Adrian, L.; Adrian, R.J.; Westerweel, J. Particle Image Velocimetry (No. 30); Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Lindken, R.; Merzkirch, W. A novel PIV technique for measurements in multiphase flows and its application to two-phase bubbly flows. Exp. Fluids 2002, 33, 814–825. [Google Scholar] [CrossRef]
- Wieneke, B.; Pfeiffer, K. Adaptive PIV with variable interrogation window size and shape. In Proceedings of the 5th International Symposium on Applications of Laser Techniques to Fluid Mechanics Lisbon, Lisbon, Portugal, 5–8 July 2010. [Google Scholar]
- Saylor, J.R.; Jones, B.K. The existence of vortices in the wakes of simulated raindrops. Phys. Fluids 2005, 17, 031706. [Google Scholar] [CrossRef]
- Menard, T.; Tanguy, S.; Berlemont, A. Coupling level set/VOF/ghost fluid methods: Validation and application to 3D simulation of the primary break-up of a liquid jet. Int. J. Multiph. Flow 2007, 33, 510–524. [Google Scholar] [CrossRef]
- Osher, S.; Fedkiw, R.; Piechor, K. Level Set Methods and Dynamic Implicit Surfaces. Appl. Mech. Rev. 2004, 57, B15. [Google Scholar] [CrossRef]
- Fedkiw, R.; Aslam, T.; Merriman, B.; Osher, S. A Non-oscillatory Eulerian Approach to Interfaces in Multimaterial Flows (the Ghost Fluid Method). J. Comput. Phys. 1999, 152, 457–492. [Google Scholar] [CrossRef]
- Sussman, M.; Puckett, E.G. A Coupled Level Set and Volume-of-Fluid Method for Computing 3D and Axisymmetric Incompressible Two-Phase Flows. J. Comput. Phys. 2000, 162, 301–337. [Google Scholar] [CrossRef]
- Tanguy, S.; Ménard, T.; Berlemont, A. A Level Set Method for vaporizing two-phase flows. J. Comput. Phys. 2007, 221, 837–853. [Google Scholar] [CrossRef]



| (µm) | (µm) | σ (pC.cm−2) | (e) | RH (%) | E (-) |
|---|---|---|---|---|---|
| 0.62 ± 0.02 | (−5 ± 0.3) × 102 | 0 ± 0.1 | 28.2 ± 0.2 | (6.28 ± 0.6) × 10−4 | |
| (−2.7 ± 0.1) × 102 | 31.9 ± 0.2 | (2.78 ± 0.2) × 10−4 | |||
| (−7.2 ± 0.4) × 101 | 31.8 ± 0.2 | (3.49 ± 0.4) × 10−4 | |||
| (−4.94 ± 0.2) × 101 | 28.6 ± 0.2 | (4.74 ± 0.4) × 10−4 | |||
| (−1.95 ± 0.1) × 101 | 29.1 ± 0.2 | (4.43 ± 0.4) × 10−4 | |||
| (−1.20 ± 0.1) × 101 | 29.8 ± 0.2 | (3.64 ± 0.4) × 10−4 | |||
| −4.52 | 29.6 ± 0.2 | (5.55 ± 0.6) × 10−4 | |||
| (1.17 ± 0.06) × 101 | 27.3 ± 0.2 | (1.10 ± 0.1) × 10−3 | |||
| 0.49 | (2 ± 0.1) × 101 | 32.4 ± 0.2 | (3.25 ± 0.2) × 10−4 | ||
| (2.75 ± 0.1) × 101 | 27.1 ± 0.2 | (1.60 ± 0.2) × 10−3 | |||
| (6.44 ± 0.3) × 101 | 27.4 ± 0.2 | (1.10 ± 0.1) × 10−3 | |||
| (7.50 ± 0.4) × 101 | 27.5 ± 0.2 | (1.02 ± 0.1) × 10−3 | |||
| (7.50 ± 0.4) × 101 | 32.3 ± 0.2 | (3.75 ± 0.2) × 10−4 | |||
| (1.51 ± 0.1) × 102 | 27.7 ± 0.2 | (8.39 ± 0.8) × 10−4 | |||
| (2.67 ± 0.1) × 102 | 27.6 ± 0.2 | (1.16 ± 0.7) × 10−3 | |||
| (2.67 ± 0.1) × 102 | 32.4 ± 0.2 | (3.44 ± 0.4) × 10−4 | |||
| (5.31 ± 0.3) × 102 | 27.7 ± 0.2 | (7.79 ± 0.7) × 10−4 | |||
| 0.42 ± 0.02 | 0.3 | (−1.44 ± 0.07) × 102 | 35.8 ± 0.2 | (7.98 ± 0.7) × 10−4 | |
| (2.75 ± 0.1) × 102 | 0 ± 0.1 | 36.3 ± 0.2 | (6.55 ± 0.6) × 10−4 | ||
| (5.12 ± 0.2) × 101 | 36.4 ± 0.2 | (5.99 ± 0.6) × 10−4 | |||
| (7.49 ± 0.4) × 101 | 36.8 ± 0.2 | (4.77 ± 0.4) × 10−4 | |||
| (2.67 ± 0.1) × 102 | 36.0 ± 0.2 | (7.39 ± 0.7) × 10−4 | |||
| (5.31 ± 0.3) × 102 | 35.7 ± 0.2 | (6.91 ± 0.6) × 10−4 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lemaitre, P.; Sow, M.; Quérel, A.; Dépée, A.; Monier, M.; Menard, T.; Flossmann, A. Contribution of Phoretic and Electrostatic Effects to the Collection Efficiency of Submicron Aerosol Particles by Raindrops. Atmosphere 2020, 11, 1028. https://doi.org/10.3390/atmos11101028
Lemaitre P, Sow M, Quérel A, Dépée A, Monier M, Menard T, Flossmann A. Contribution of Phoretic and Electrostatic Effects to the Collection Efficiency of Submicron Aerosol Particles by Raindrops. Atmosphere. 2020; 11(10):1028. https://doi.org/10.3390/atmos11101028
Chicago/Turabian StyleLemaitre, Pascal, Mamadou Sow, Arnaud Quérel, Alexis Dépée, Marie Monier, Thibaut Menard, and Andrea Flossmann. 2020. "Contribution of Phoretic and Electrostatic Effects to the Collection Efficiency of Submicron Aerosol Particles by Raindrops" Atmosphere 11, no. 10: 1028. https://doi.org/10.3390/atmos11101028
APA StyleLemaitre, P., Sow, M., Quérel, A., Dépée, A., Monier, M., Menard, T., & Flossmann, A. (2020). Contribution of Phoretic and Electrostatic Effects to the Collection Efficiency of Submicron Aerosol Particles by Raindrops. Atmosphere, 11(10), 1028. https://doi.org/10.3390/atmos11101028







