Modelling the Optical Properties of Soot Particles under Various Aging Conditions
Abstract
1. Introduction
2. Methods
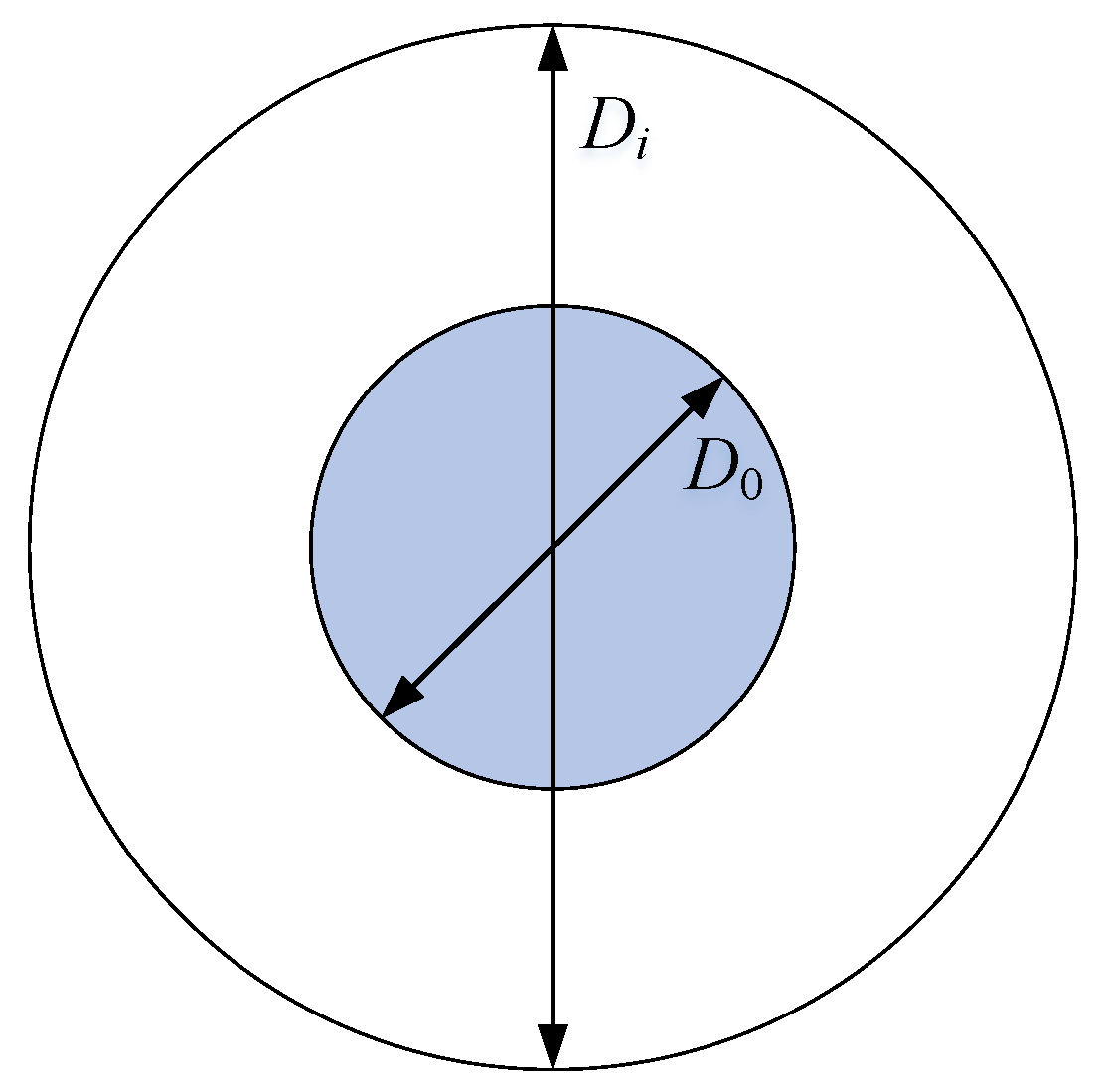
2.1. The Core-Shell Model
2.2. The Derivation of Absorption Exponent β
3. Results and Discussion
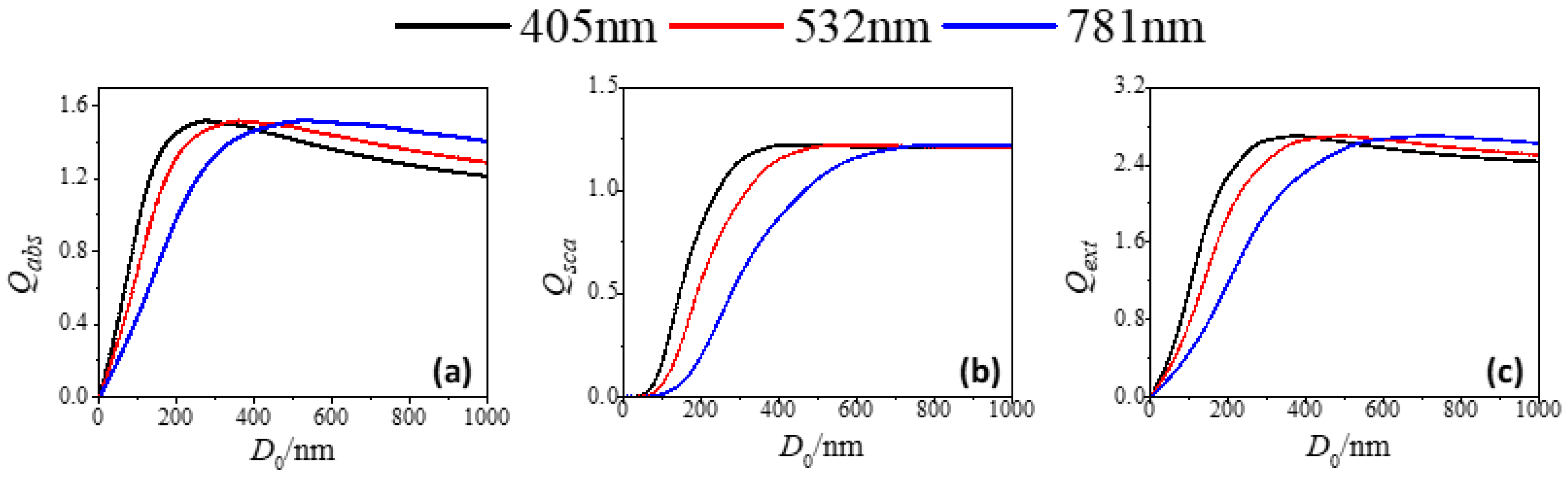
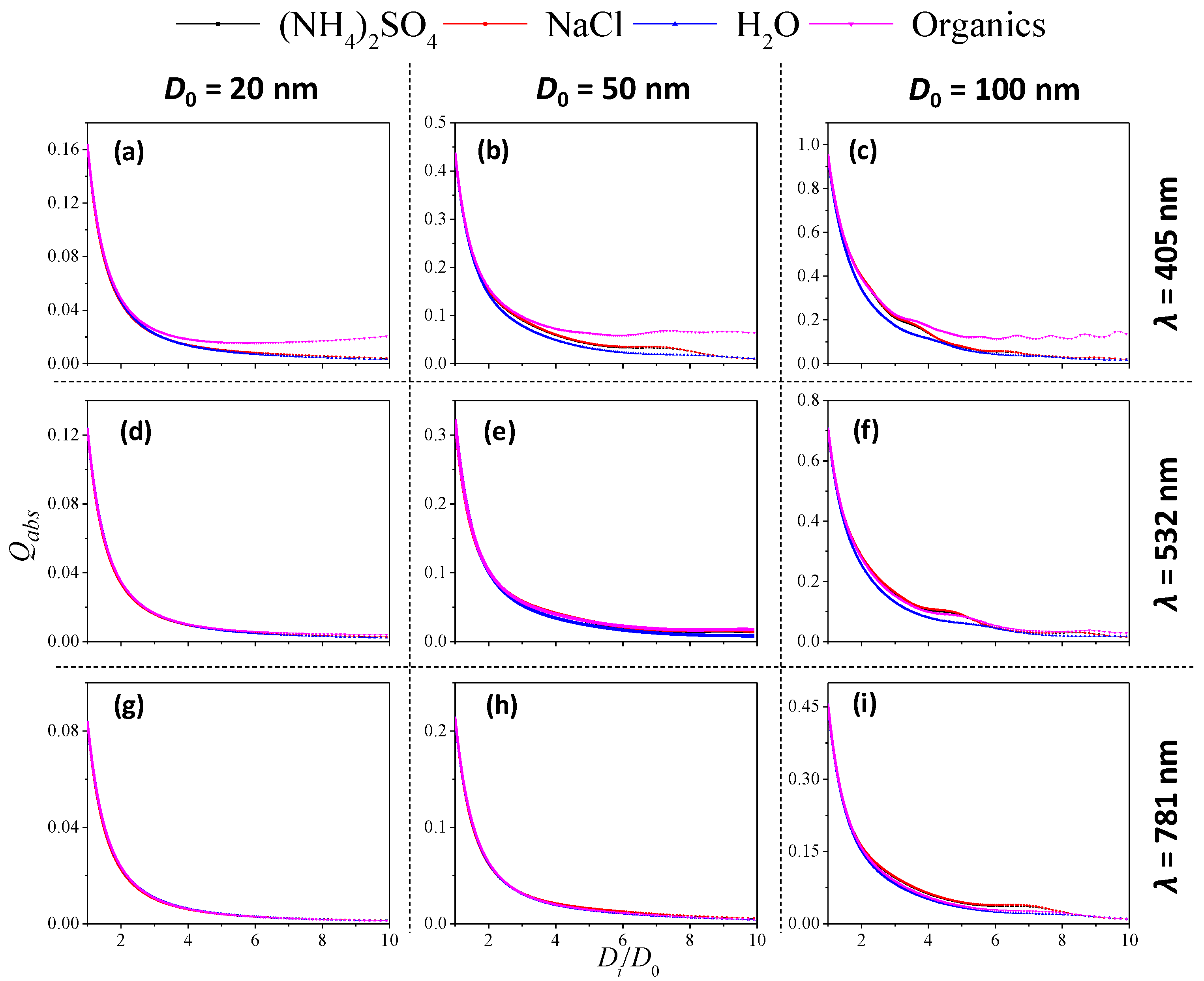
3.1. Absorption Efficiency Qabs
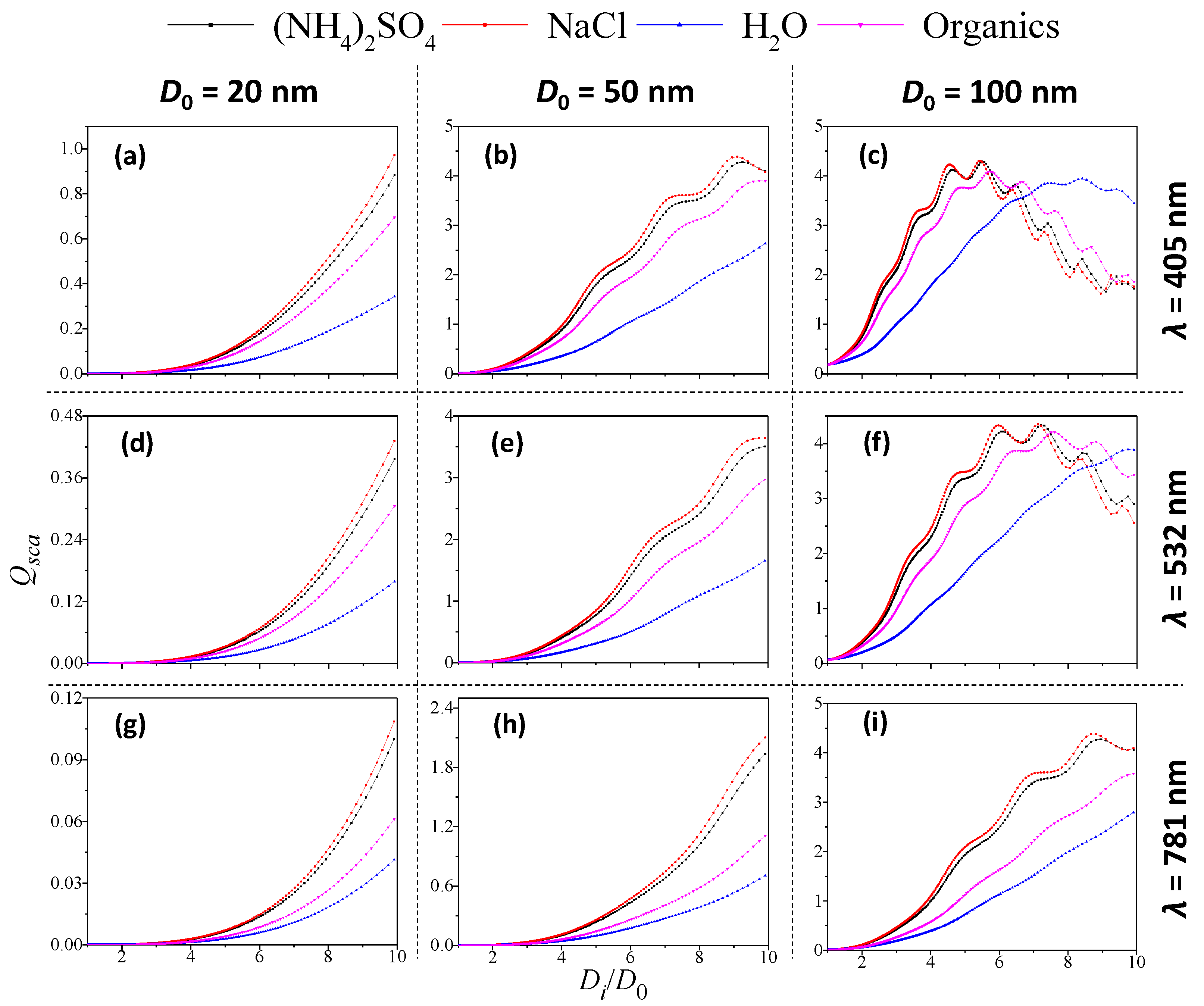
3.2. Scattering Efficiency Qsca and Extinction Efficiency Qext
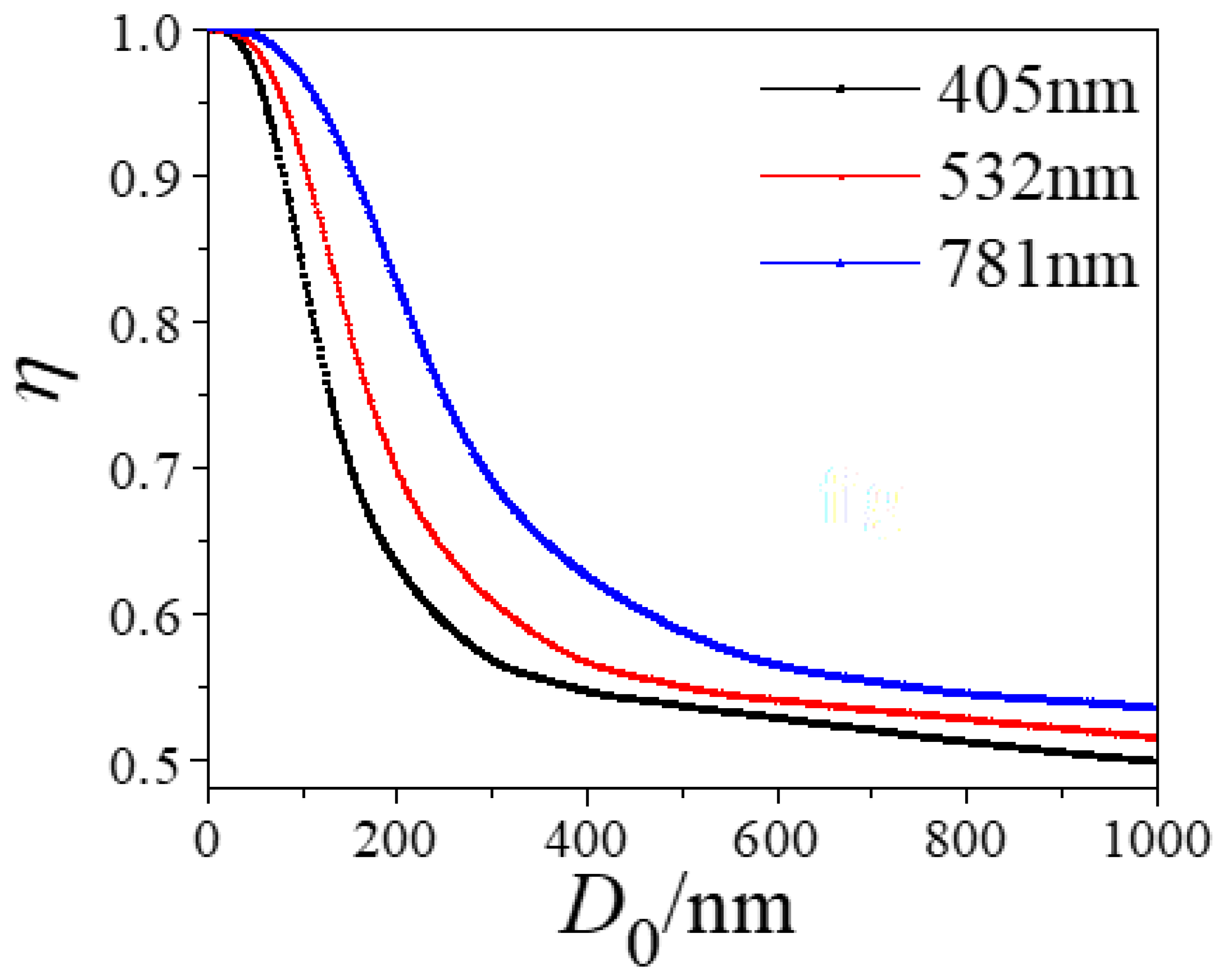
3.3. Absorption Proportion η of Soot Particles
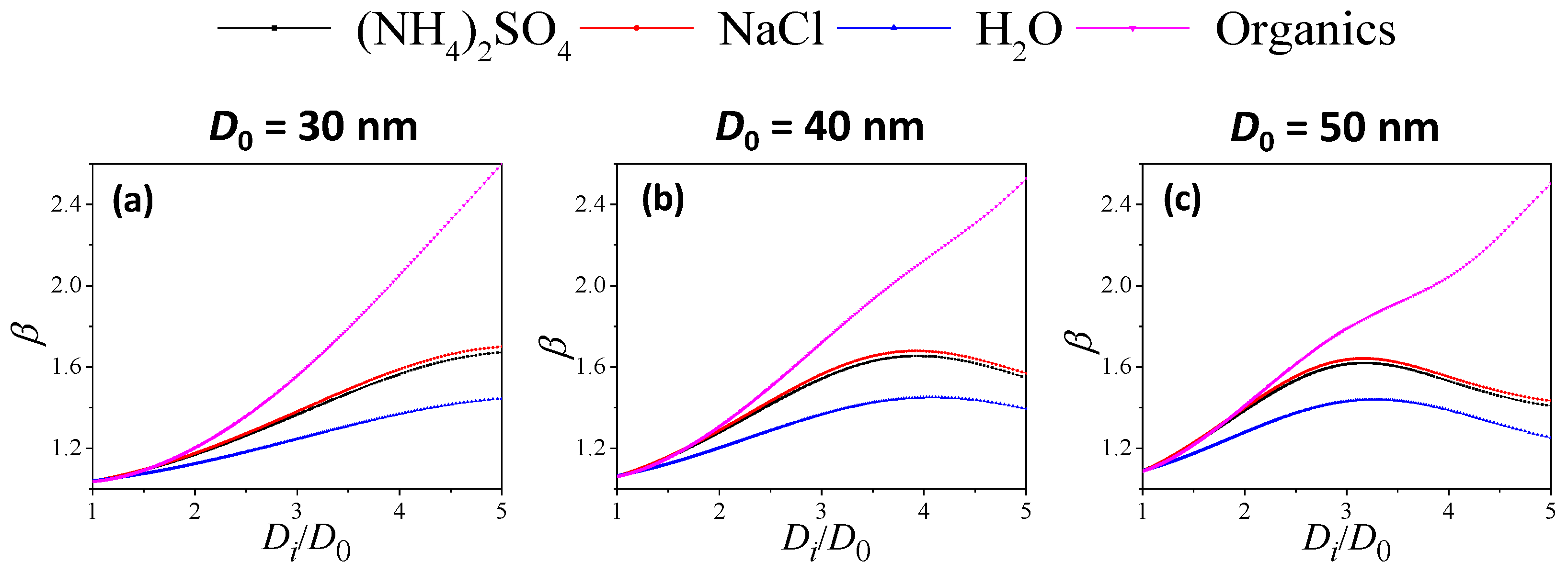
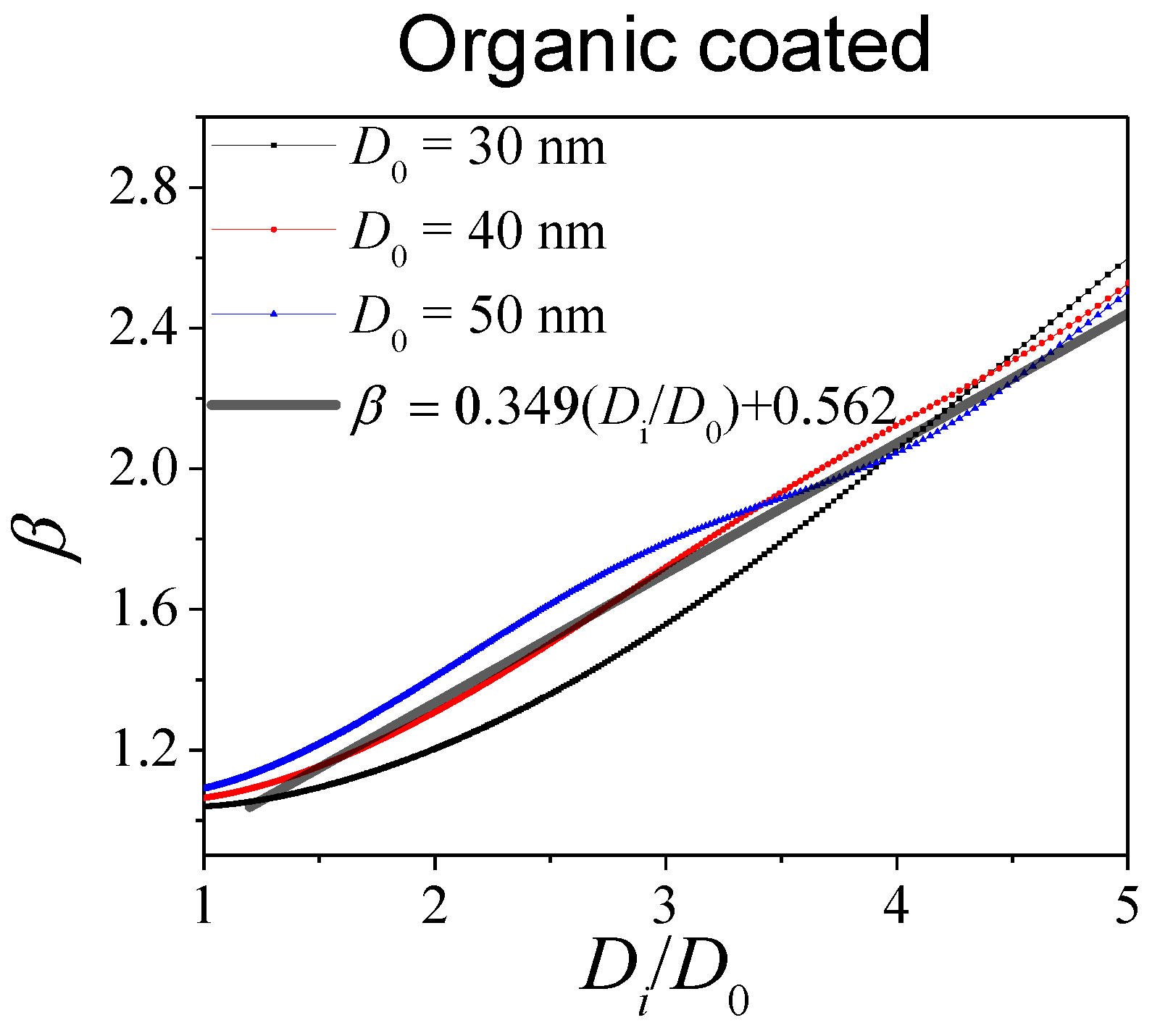
3.4. Absorption Exponent β
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Guo, S.; Hu, M.; Lin, Y.; Gomez-Hernandez, M.; Zamora, M.L.; Peng, J.; Collins, D.R.; Zhang, R. OH-initiated oxidation of m-xylene on black carbon aging. Environ. Sci. Technol. 2016, 50, 8605–8612. [Google Scholar] [CrossRef] [PubMed]
- Schnitzler, E.G.; Dutt, A.; Charbonneau, A.M.; Olfert, J.S.; Jäger, W. Soot aggregate restructuring due to coatings of secondary organic aerosol derived from aromatic precursors. Environ. Sci. Technol. 2014, 48, 14309–14316. [Google Scholar] [CrossRef] [PubMed]
- Schwarz, J.P.; Spackman, J.R.; Fahey, D.W.; Gao, R.S.; Lohmann, U.; Stier, P.; Watts, L.A.; Thomson, D.S.; Lack, D.A.; Pfister, L. Coatings and their enhancement of black carbon light absorption in the tropical atmosphere. J. Geophys. Res. Atmos. 2008, 113. [Google Scholar] [CrossRef]
- Peng, J.; Hu, M.; Guo, S.; Du, Z.; Zheng, J.; Shang, D.; Levy Zamora, M.; Zeng, L.; Shao, M.; Wu, Y.; et al. Markedly enhanced absorption and direct radiative forcing of black carbon under polluted urban environments. Proc. Natl. Acad. Sci. USA 2016, 113, 4266–4271. [Google Scholar] [CrossRef] [PubMed]
- Cui, X.; Wang, X.; Yang, L.; Chen, B.; Chen, J.; Andersson, A.; Gustafsson, Ö. Radiative absorption enhancement from coatings on black carbon aerosols. Sci. Total Environ. 2016, 551, 51–56. [Google Scholar] [CrossRef]
- Wang, Q.; Huang, R.; Cao, J.; Han, Y.; Wang, G.; Li, G.; Wang, Y.; Dai, W.; Zhang, R.; Zhou, Y. Mixing state of black carbon aerosol in a heavily polluted urban area of China: Implications for light absorption enhancement. Aerosol Sci. Technol. 2014, 48, 689–697. [Google Scholar] [CrossRef]
- Bond, T.C.; Bergstrom, R.W. Light absorption by carbonaceous particles: An investigative review. Aerosol Sci. Technol. 2006, 40, 27–67. [Google Scholar] [CrossRef]
- Lack, D.A.; Langridge, J.M.; Bahreini, R.; Cappa, C.D.; Middlebrook, A.M.; Schwarz, J.P. Brown carbon and internal mixing in biomass burning particles. Proc. Natl. Acad. Sci. USA 2012, 109, 14802–14807. [Google Scholar] [CrossRef]
- Cheng, T.; Gu, X.; Wu, Y.; Chen, H. Effects of atmospheric water on the optical properties of soot aerosols with different mixing states. J. Quant. Spectrosc. Radiat. Transf. 2014, 147, 196–206. [Google Scholar] [CrossRef]
- Doner, N.; Liu, F.; Yon, J. Impact of necking and overlapping on radiative properties of coated soot aggregates. Aerosol Sci. Technol. 2017, 51, 532–542. [Google Scholar] [CrossRef]
- Bond, T.C.; Doherty, S.J.; Fahey, D.W.; Forster, P.M.; Berntsen, T.; DeAngelo, B.J.; Flanner, M.G.; Ghan, S.; Kärcher, B.; Koch, D.; et al. Bounding the role of black carbon in the climate system: A scientific assessment. J. Geophys. Res. Atmos. 2013, 118, 5380–5552. [Google Scholar] [CrossRef]
- Li, W.; Shao, L.; Zhang, D.; Ro, C.; Hu, M.; Bi, X.; Geng, H.; Matsuki, A.; Niu, H.; Chen, J. A review of single aerosol particle studies in the atmosphere of East Asia: Morphology, mixing state, source, and heterogeneous reactions. J. Clean. Prod. 2016, 112, 1330–1349. [Google Scholar] [CrossRef]
- Shiraiwa, M.; Kondo, Y.; Iwamoto, T.; Kita, K. Amplification of Light Absorption of Black Carbon by Organic Coating. Aerosol Sci. Technol. 2010, 44, 46–54. [Google Scholar] [CrossRef]
- Abo Riziq, A.; Trainic, M.; Erlick, C.; Segre, E.; Rudich, Y. Extinction efficiencies of coated absorbing aerosols measured by cavity ring down aerosol spectrometry. Atmos. Chem. Phys. 2008, 8, 1823–1833. [Google Scholar] [CrossRef]
- Liu, F.; Yon, J.; Bescond, A. On the radiative properties of soot aggregates—Part 2: Effects of coating. J. Quant. Spectrosc. Radiat. Transf. 2016, 172, 134–145. [Google Scholar] [CrossRef]
- Bauer, S.E.; Menon, S.; Koch, D.; Bond, T.C.; Tsigaridis, K. A global modeling study on carbonaceous aerosol microphysical characteristics and radiative effects. Atmos. Chem. Phys. 2010, 10, 7439–7456. [Google Scholar] [CrossRef]
- Riemer, N.; Vogel, H.; Vogel, B. Soot aging time scales in polluted regions during day and night. Atmos. Chem. Phys. 2004, 4, 1885–1893. [Google Scholar] [CrossRef]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Mätzler, C. MATLAB Functions for Mie Scattering and Absorption, Version 2. IAP Res. Rep. 2002, 8, 9. [Google Scholar]
- Liu, C.; Panetta, R.L.; Yang, P. The Influence of Water Coating on the Optical Scattering Properties of Fractal Soot Aggregates. Aerosol Sci. Technol. 2012, 46, 31–43. [Google Scholar] [CrossRef]
- Massabò, D.; Caponi, L.; Bernardoni, V.; Bove, M.C.; Brotto, P.; Calzolai, G.; Cassola, F.; Chiari, M.; Fedi, M.E.; Fermo, P. Multi-wavelength optical determination of black and brown carbon in atmospheric aerosols. Atmos. Environ. 2015, 108, 1–12. [Google Scholar] [CrossRef]
- Guo, X.; Nakayama, T.; Yamada, H.; Inomata, S.; Tonokura, K.; Matsumi, Y. Measurement of the light absorbing properties of diesel exhaust particles using a three-wavelength photoacoustic spectrometer. Atmos. Environ. 2014, 94, 428–437. [Google Scholar] [CrossRef]
- Nakayama, T.; Sato, K.; Matsumi, Y.; Imamura, T.; Yamazaki, A.; Uchiyama, A. Wavelength and NOx dependent complex refractive index of SOAs generated from the photooxidation of toluene. Atmos. Chem. Phys. 2013, 13, 531–545. [Google Scholar] [CrossRef]
- Adachi, K.; Buseck, P.R. Internally mixed soot, sulfates, and organic matter in aerosol particles from Mexico City. Atmos. Chem. Phys. 2008, 8, 6469–6481. [Google Scholar] [CrossRef]
- Adachi, K.; Zaizen, Y.; Kajino, M.; Igarashi, Y. Mixing state of regionally transported soot particles and the coating effect on their size and shape at a mountain site in Japan. J. Geophys. Res. Atmos. 2014, 119, 5386–5396. [Google Scholar] [CrossRef]
- Li, J.; Anderson, J.; Buseck, P. TEM study of aerosol particles from clean and polluted marine boundary layers over the North Atlantic. J. Geophys. Res. 2003, 108. [Google Scholar] [CrossRef]
- Scarnato, B.V.; Vahidinia, S.; Richard, D.T.; Kirchstetter, T.W. Effects of internal mixing and aggregate morphology on optical properties of black carbon using a discrete dipole approximation model. Atmos. Chem. Phys. 2013, 13, 5089–5101. [Google Scholar] [CrossRef]
- Andreae, M.O.; Gelencsér, A. Black carbon or brown carbon? The nature of light-absorbing carbonaceous aerosols. Atmos. Chem. Phys. 2006, 6, 3131–3148. [Google Scholar] [CrossRef]
- Alexander, D.T.; Crozier, P.A.; Anderson, J.R. Brown carbon spheres in East Asian outflow and their optical properties. Science 2008, 321, 833–836. [Google Scholar] [CrossRef]
- Smyth, K.C.; Shaddix, C.R. The elusive history of m ~= 1.57 − 0.56i for the refractive index of soot. Combust. Flame 1996, 107, 314–320. [Google Scholar] [CrossRef]
- Chen, L.; Jiang, Y.; Sun, Y.; Gao, X.; Cen, K. Analysis of Absorption and Scattering Properties of Water Host Haze Droplet with Insoluble Solid Inclusion. Chin. J. Lasers 2015, 234–241. [Google Scholar] [CrossRef]
- Lang-Yona, N.; Abo-Riziq, A.; Erlick, C.; Segre, E.; Trainic, M.; Rudich, Y. Interaction of internally mixed aerosols with light. Phys. Chem. Chem. Phys. 2010, 12, 21–31. [Google Scholar] [CrossRef] [PubMed]
- Bulatov, V.; Fisher, M.; Schechter, I. Aerosol analysis by cavity-ring-down laser spectroscopy. Anal. Chim. Acta 2002, 466, 1–9. [Google Scholar] [CrossRef]
- Kirchstetter, T.W.; Novakov, T.; Hobbs, P.V. Evidence that the spectral dependence of light absorption by aerosols is affected by organic carbon. J. Geophys. Res. Atmos. 2004, 109. [Google Scholar] [CrossRef]
- Schnaiter, M.; Linke, C.; Möhler, O.; Naumann, K.H.; Saathoff, H.; Wagner, R.; Schurath, U.; Wehner, B. Absorption amplification of black carbon internally mixed with secondary organic aerosol. J. Geophys. Res. Atmos. 2005, 110. [Google Scholar] [CrossRef]
- Liu, D.; Whitehead, J.; Alfarra, M.R.; Reyes-Villegas, E.; Spracklen, D.V.; Reddington, C.L.; Kong, S.; Williams, P.I.; Ting, Y.; Haslett, S. Black-carbon absorption enhancement in the atmosphere determined by particle mixing state. Nat. Geosci. 2017, 10, 184. [Google Scholar] [CrossRef]
- Li, K.; Chen, L.; Han, K.; Lv, B.; Bao, K.; Wu, X.; Gao, X.; Cen, K. Smog chamber study on aging of combustion soot in isoprene/SO2/NOx system: Changes of mass, size, effective density, morphology and mixing state. Atmos. Res. 2017, 184, 139–148. [Google Scholar] [CrossRef]



| Wavelength λ/nm | Reference | |||
|---|---|---|---|---|
| 405 | 532 | 781 | ||
| Soot | 1.57–0.56i | 1.57–0.56i | 1.57–0.56i | [30] |
| Water | 1.33–0i | 1.33–0i | 1.33–0i | [31] |
| (NH4)2SO4 | 1.521–0i | 1.521–0i | 1.521–0i | [32] |
| NaCl | 1.54427–0i | 1.54427–0i | 1.54427–0i | [33] |
| Organics | 1.467–0.003i | 1.457–0.0004i | 1.403–0i | [23] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, K.; Yan, M.; Shen, J.; Zhang, X.; Geng, C.; Yang, W.; Azzi, M. Modelling the Optical Properties of Soot Particles under Various Aging Conditions. Atmosphere 2020, 11, 86. https://doi.org/10.3390/atmos11010086
Li K, Yan M, Shen J, Zhang X, Geng C, Yang W, Azzi M. Modelling the Optical Properties of Soot Particles under Various Aging Conditions. Atmosphere. 2020; 11(1):86. https://doi.org/10.3390/atmos11010086
Chicago/Turabian StyleLi, Kangwei, Mingming Yan, Jiandong Shen, Xin Zhang, Chunmei Geng, Wen Yang, and Merched Azzi. 2020. "Modelling the Optical Properties of Soot Particles under Various Aging Conditions" Atmosphere 11, no. 1: 86. https://doi.org/10.3390/atmos11010086
APA StyleLi, K., Yan, M., Shen, J., Zhang, X., Geng, C., Yang, W., & Azzi, M. (2020). Modelling the Optical Properties of Soot Particles under Various Aging Conditions. Atmosphere, 11(1), 86. https://doi.org/10.3390/atmos11010086






