Model of Daytime Oxygen Emissions in the Mesopause Region and Above: A Review and New Results
Abstract
1. Introduction
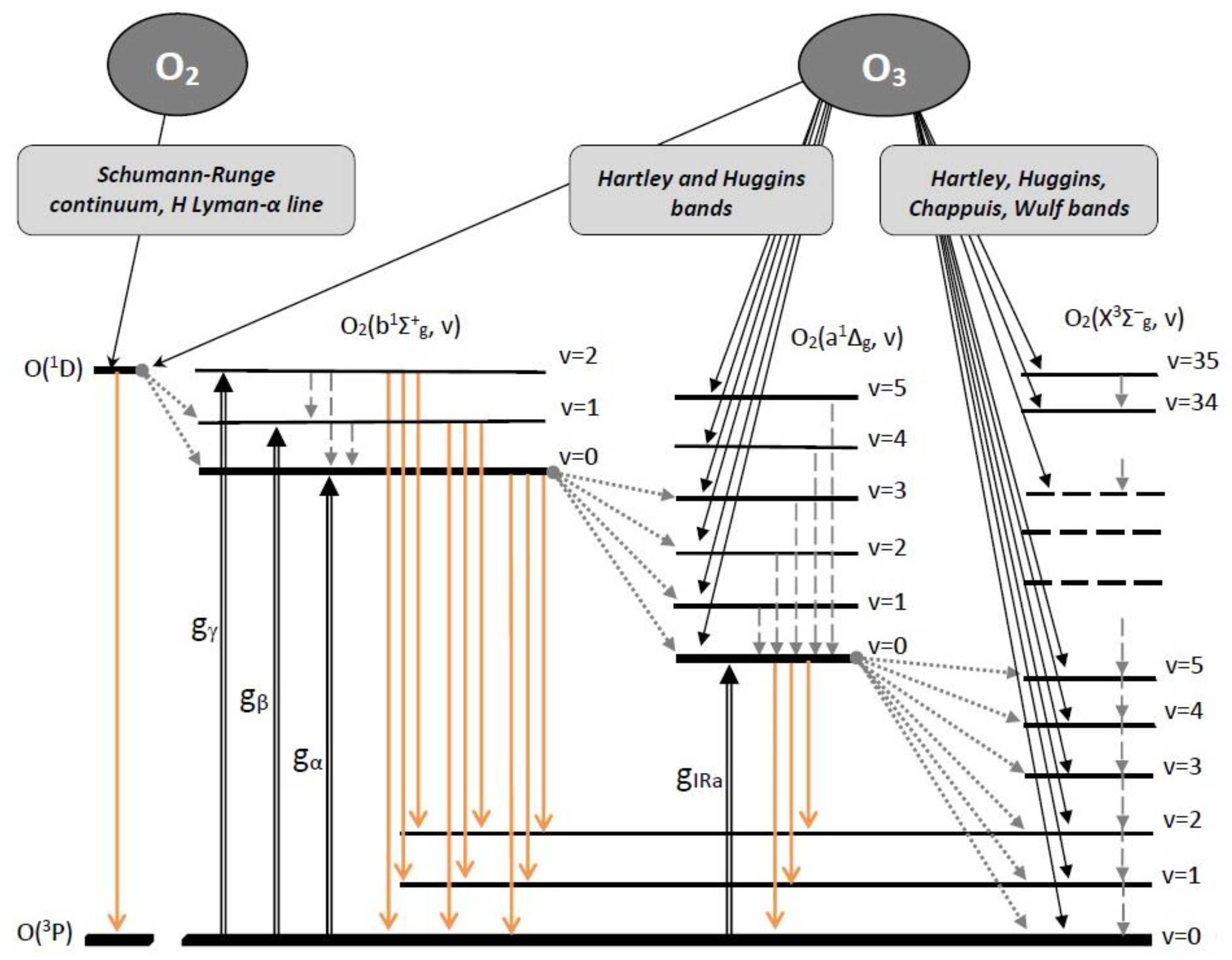
- The main problem is related to the design of the MSZ model. The bottom line is that this model is intended only to explain the altitude profiles of the volume emission rates of atmospheric (0, 0) band and IR atmospheric (0, 0) band. Although, simultaneously with the O2(a1Δg, v = 0) and O2(b1Σ+g, v = 0) levels, the electronic–vibrational levels of oxygen molecules with v ≥ 1 are populated. Emission transitions from the O2(a1Δg, v ≥ 0) and O2(b1Σ+g, v ≥ 0) are observed in the atmospheric glow and clearly correlate with each other [12,22,23,24]. Note that emission bands of an oxygen molecule with much higher levels of vibrational excitation O2(b1Σ+g, v′ = 0–15) are also observed in the atmospheric glow [25].
- The next problem arises from the first. Measured VERs and spectral features of the O2 atmospheric (Atm) (0–0) band are widely used for solving applied tasks such as estimating altitude profiles of kinetic temperature, pressure, aerosol content, cloud height etc. In last decades, it has become technically possible to measure VER from electronically–vibrationally excited levels of O2 molecule with sufficient spectral resolution, e.g., in the O2 Atm (1–1), O2 Atm (2–2), O2 Atm (2–1), O2 IR Atm (1–0) bands and others. Airglow in these bands have been used for remote sensing. However, the main feature of the MSZ model is that it does not consider vibrational excitations at all.
- A significant uncertainty of ozone altitude profiles retrieved from emission in 1.27 μm channel has been found using the MSZ model. As shown based on the analysis of nine satellite experiments [26], values of ozone concentration in the mesosphere and lower thermosphere (MLT) retrieved via the MSZ model are 20%–70% higher than the same obtained by other methods.
- Currently, there is only one rocket experiment (METEORS) which conducted simultaneous measurements of VERs altitude profiles in Atm (0–0) and IR Atm (0–0) bands [27]. However, ozone altitude profiles retrieved from VERs in Atm and IR Atm bands based on experimental data and using the MSZ model didn’t match, although, naturally, they should be identical.
- The most important issue of modern photochemistry is related with a role of O2(X3Σg−, v = 1) level [28] which perhaps is a key component in a quasi-resonant energy exchange with H2O(010) level. Radiance from the H2O(010) level forms the 6.3 μm band in water vapor. Naturally, energy transfer from the top electronically–vibrationally excited levels of oxygen molecule O2(a1Δg, v) and O2(b1Σ+g, v) should be completed by energy transfer between vibrational levels of ground state of oxygen molecule. The aforementioned energy transfer includes several intermediate steps:
- (i)
- O2(b1Σ+g, v ≥ 1) → O2(b1Σ+g, v = 0);
- (ii)
- O2(b1Σ+g, v = 0) → O2(a1Δg, v ≤ 3);
- (iii)
- O2(a1Δg, v ≥ 1) → O2(a1Δg, v = 0);
- (iv)
- O2(a1Δg, v = 0) → O2(X3Σg−, v ≤ 5);
- (v)
- O2(X3Σg−, v) + N2(X1Σg+, v′ = 0) → O2(X3Σg−, v − 2) + N2(X1Σg+, v′ = 1);
- (vi)
- O2(X3Σg−, v > 1) → O2(X3Σg−, v = 1);
- (vii)
- O2(X3Σg−, v = 1) ↔ H2O(010).
2. Formation of Excited Oxygen Components in the Daytime MLT
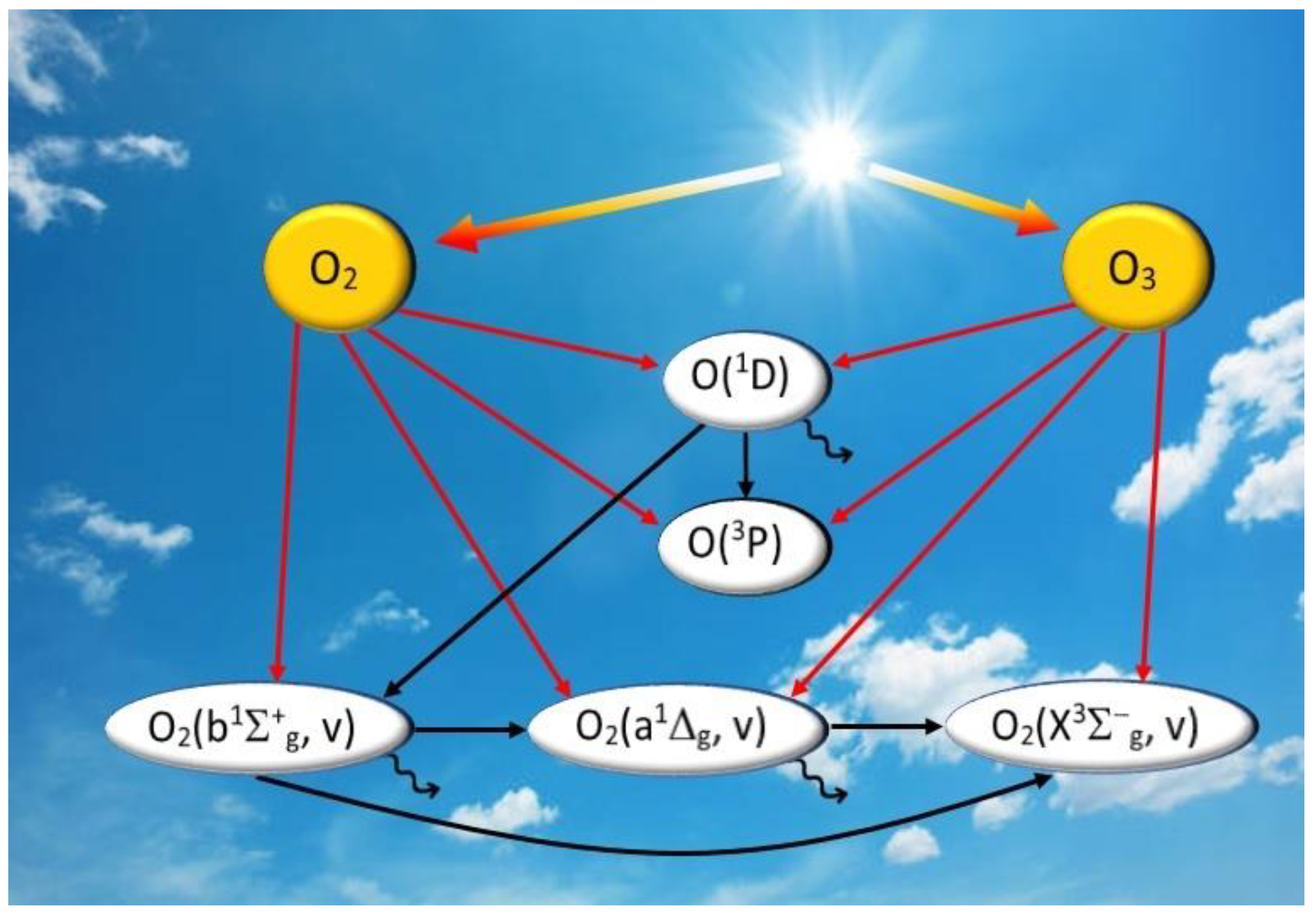
2.1. O3 Photodissociation and Its Products
- (i)
- singlet channel O3 + hv (λ = 200–320 nm) → O2(a1Δg, v = 0–5) + O(1D) in Hartley band;
- (ii)
- triplet channel O3 + hv (λ = 200–900 nm) → O2(X3Σ−g, v = 0–35) + O(3P) in Hartley, Huggins, Chappuis and Wulf bands.
2.2. Energy Transfer in Collisional Reactions
2.3. Emission Transitions
2.4. Another Mechanism of O2(b1Σ+g, v = 0) Excitation
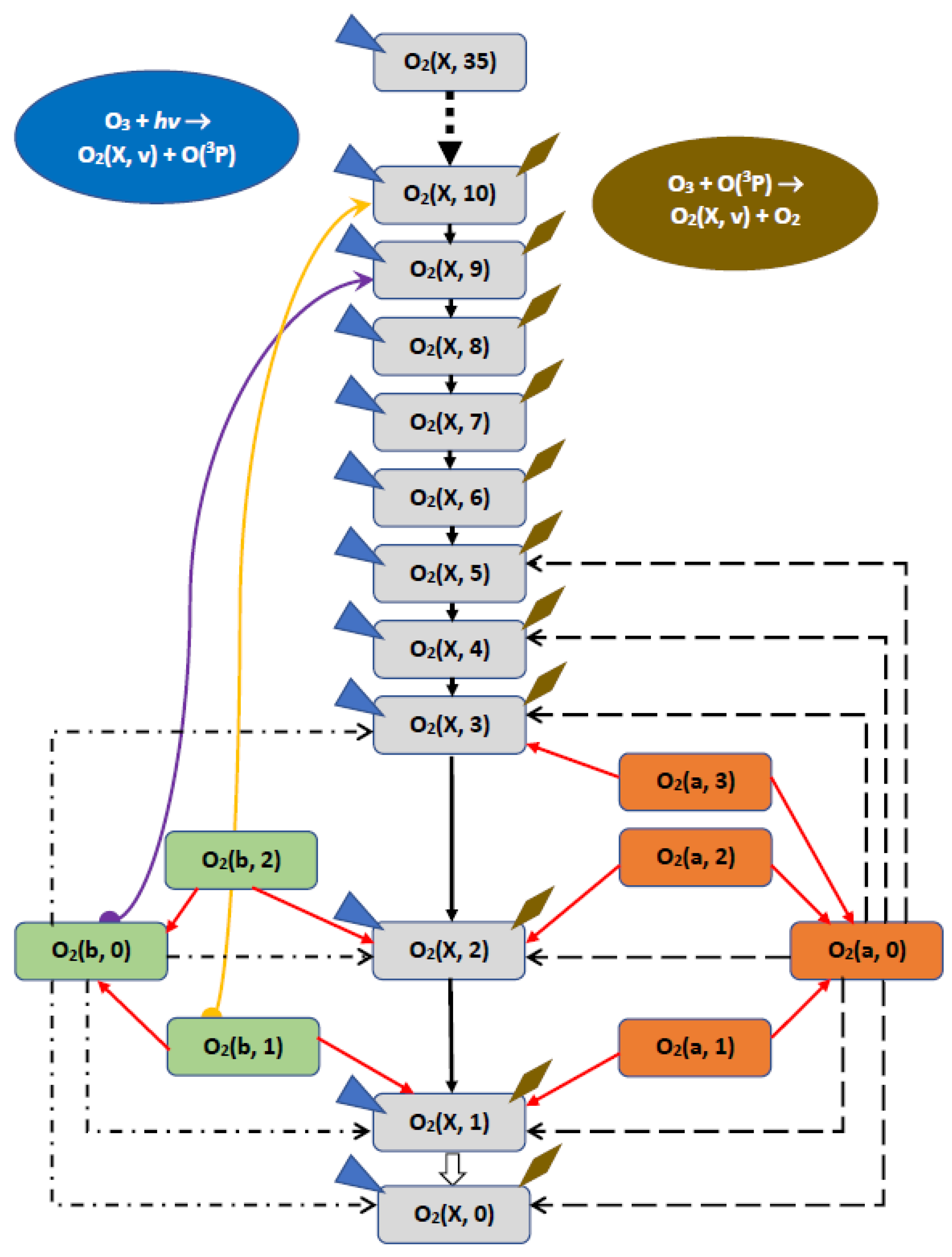
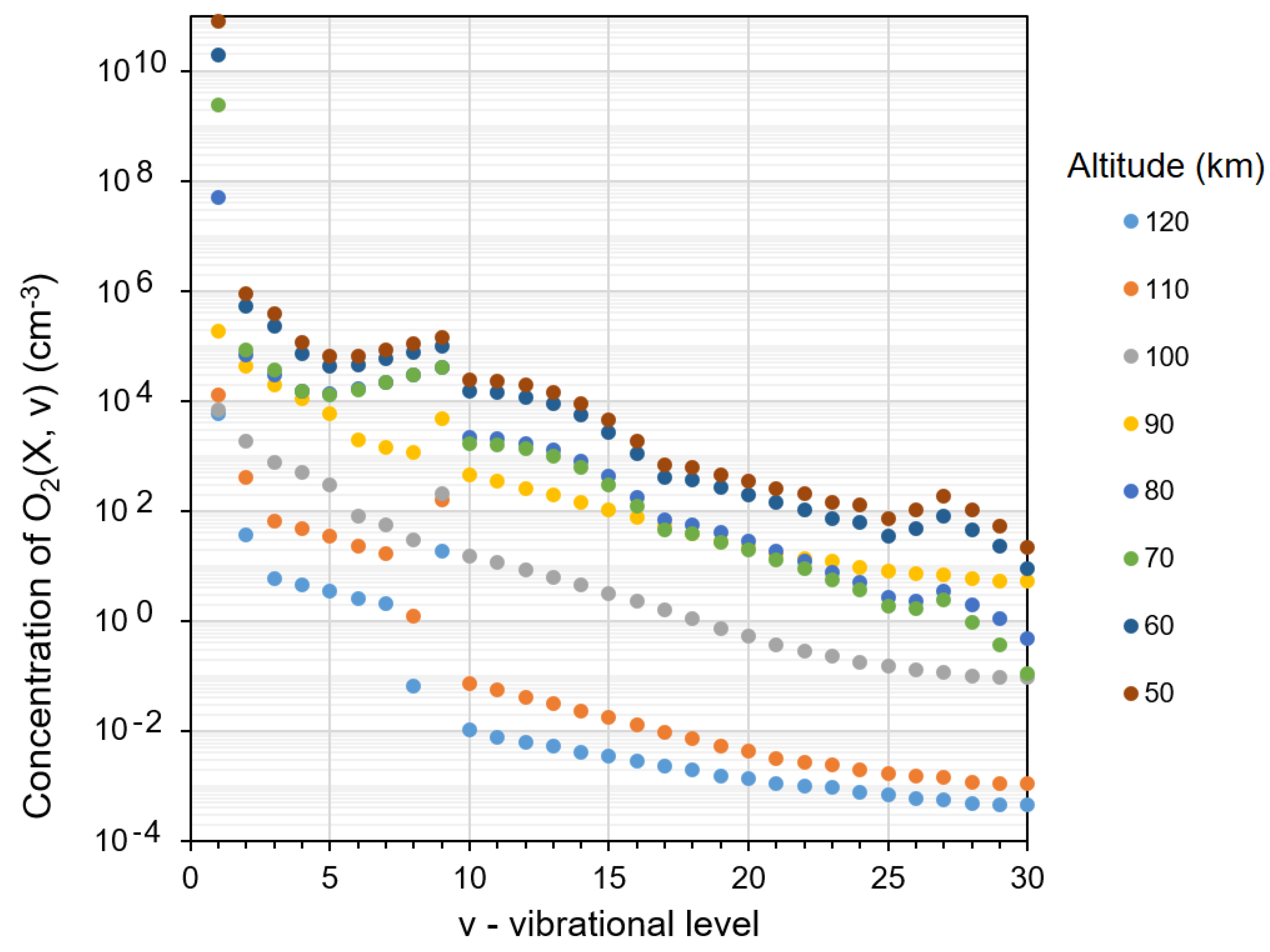
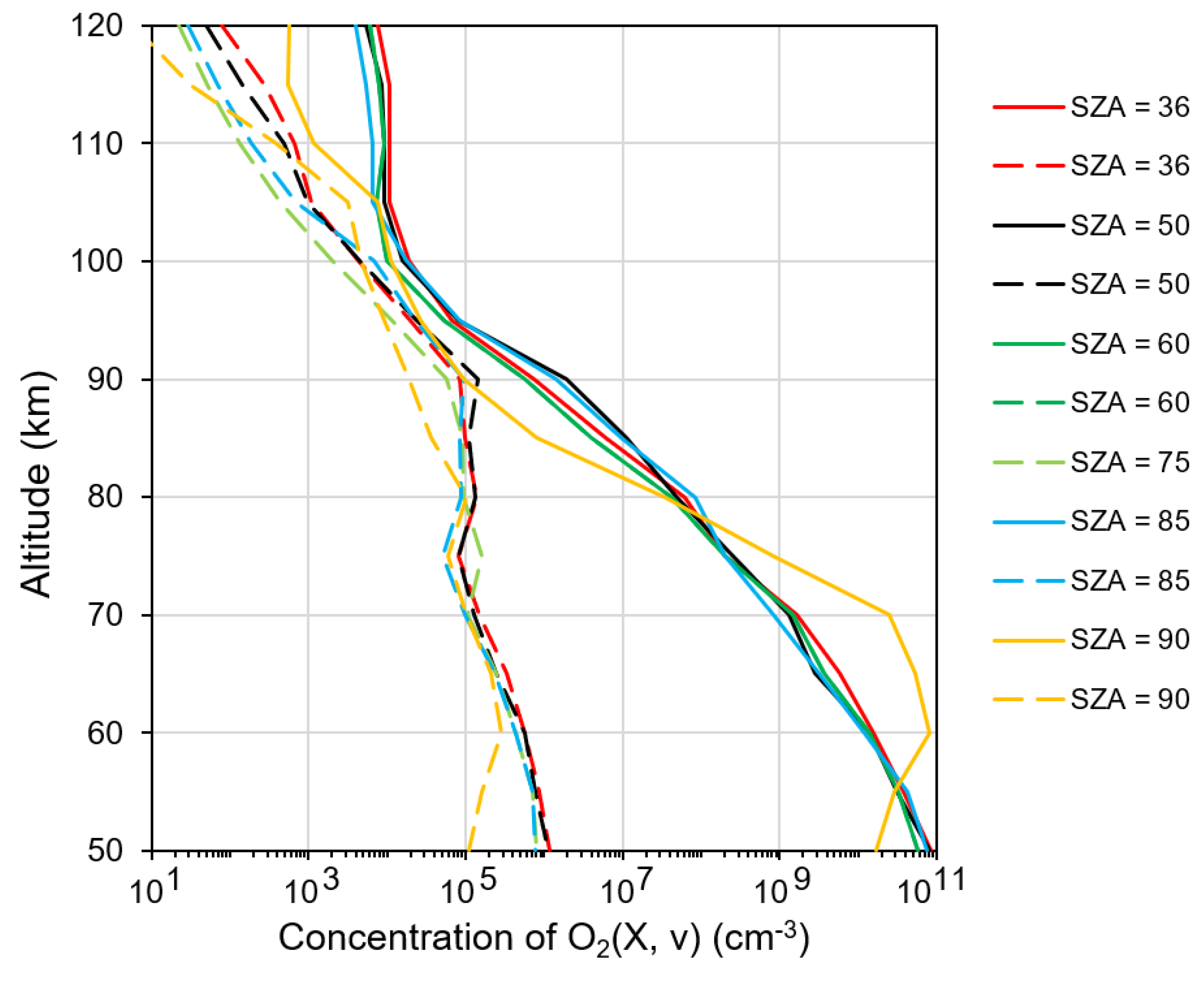
2.5. Kinetics of O2(X3Σ−g, v) in MLT
- (1)
- Direct population of O2(X3Σ¯g, v = 1–35) as a result of ozone photolysis (in the triplet channel) in the Hartley, Huggins, Chappuis, and Wulf bands. Moreover, the photodissociation rate substantially depends on the wavelength of solar radiation, as can be seen from Figure 2. The methodology for calculating rates of the photodissociation processes which sequentially takes into account threshold values of the excitation for each vibrational level is described in detail in [33,46].
- (2)
- (3)
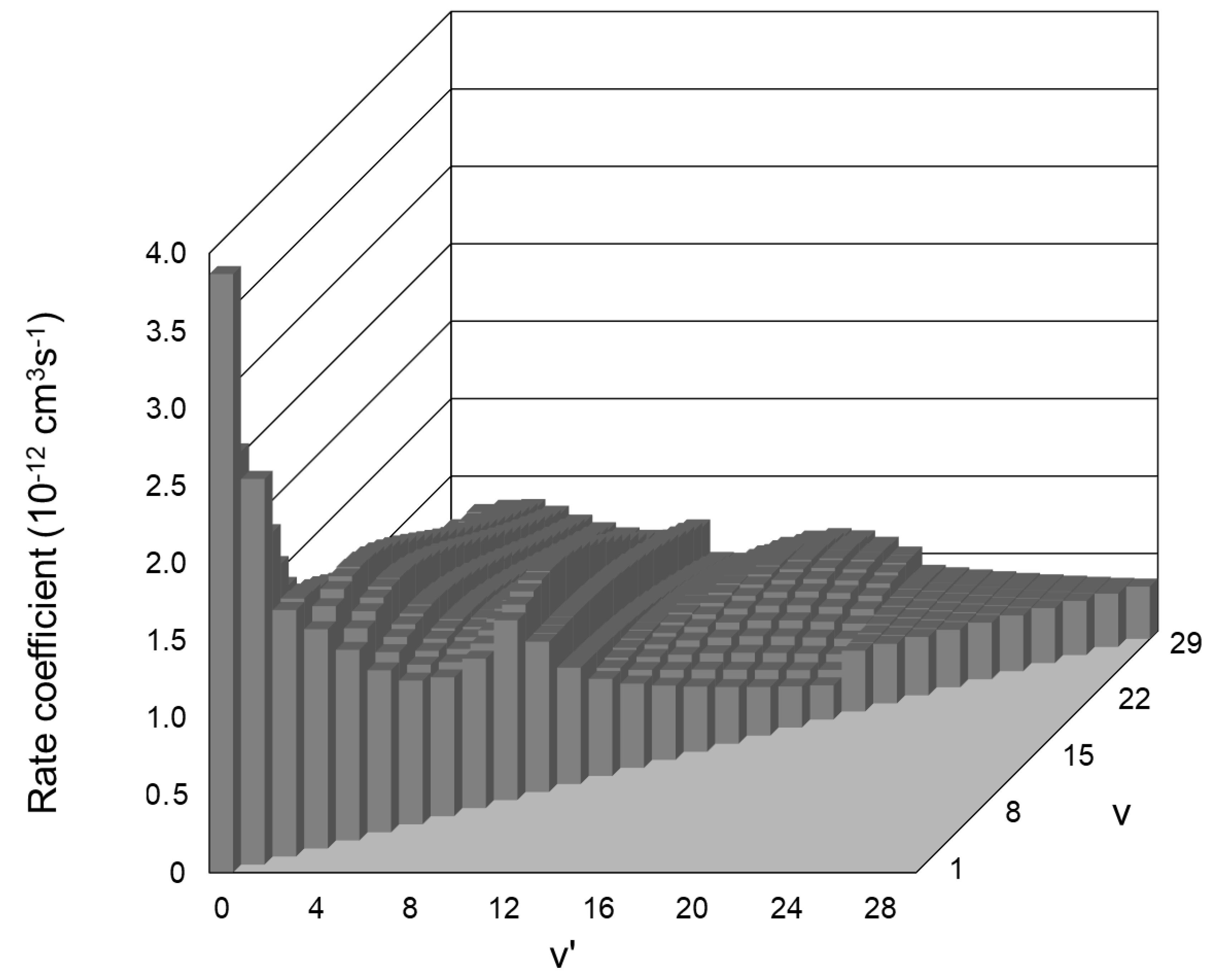
- Cascade population of each O2(X3Σ¯g, v) level due to transitions from all overlying (with respect to it) levels as a result of reaction (14). The rate coefficients of all cascade transitions were calculated by [62] and visualized by us in Figure 6. The term which takes into account the contribution of mentioned cascade transitions in the kinetic equation for O2(X3Σ−g, v) isThus, we have to consider 464 cascade transitions to describe the population of the O2(X3Σ−g, v = 1) level only.
- (4)
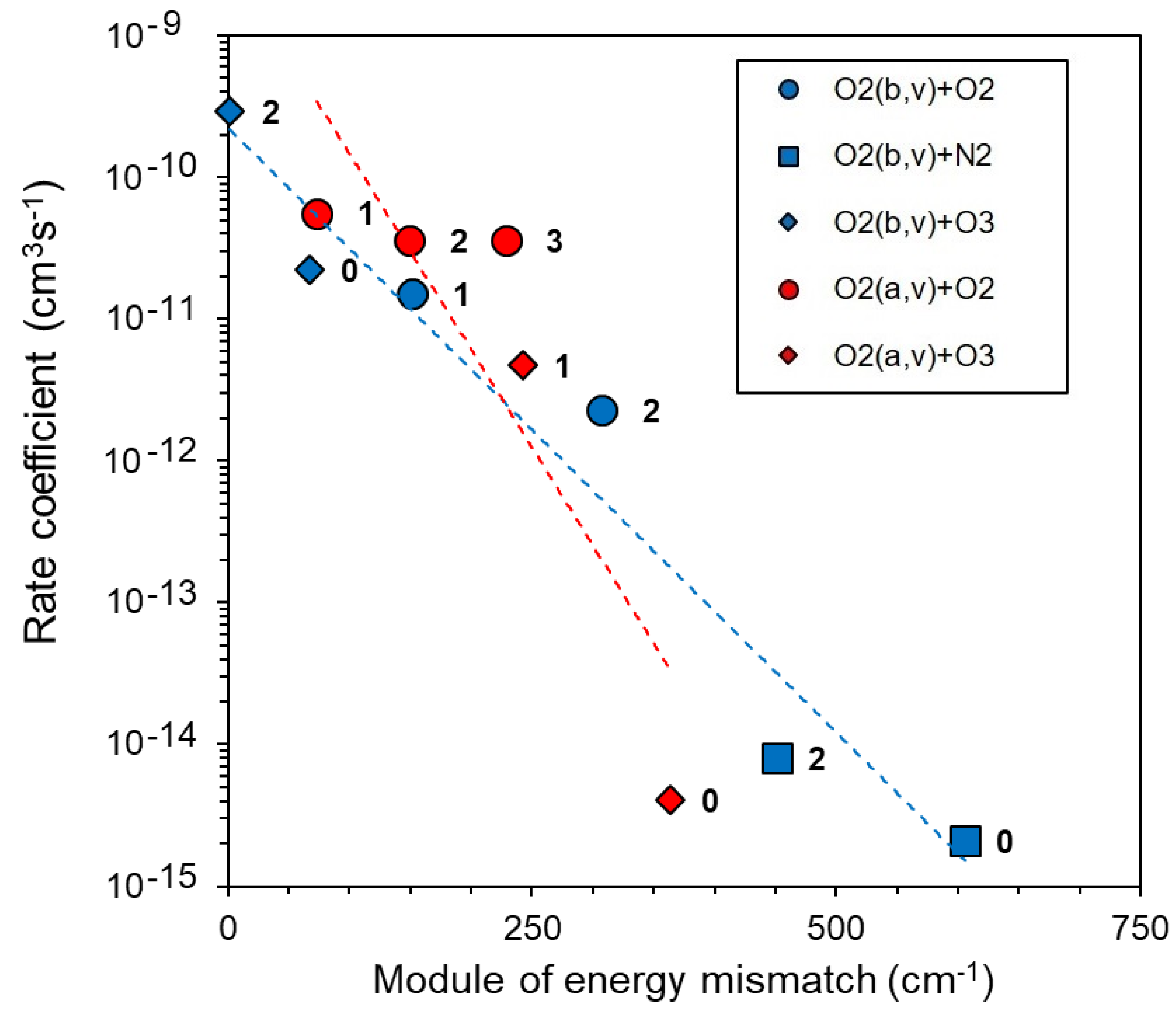
- Energy transfer from O2(b1Σ+g, v ≤ 2) and O2(a1Δg, v ≤ 5) levels as a result of fast reactions (9), (10).
- (5)
- The processes of (V–V) and (V–T) vibrational relaxation in collisions with O2 and N2 (see Section 2.2).
3. Kinetics of O2 and O3 Photolysis Products in MLT. The Modern Model
4. Applications
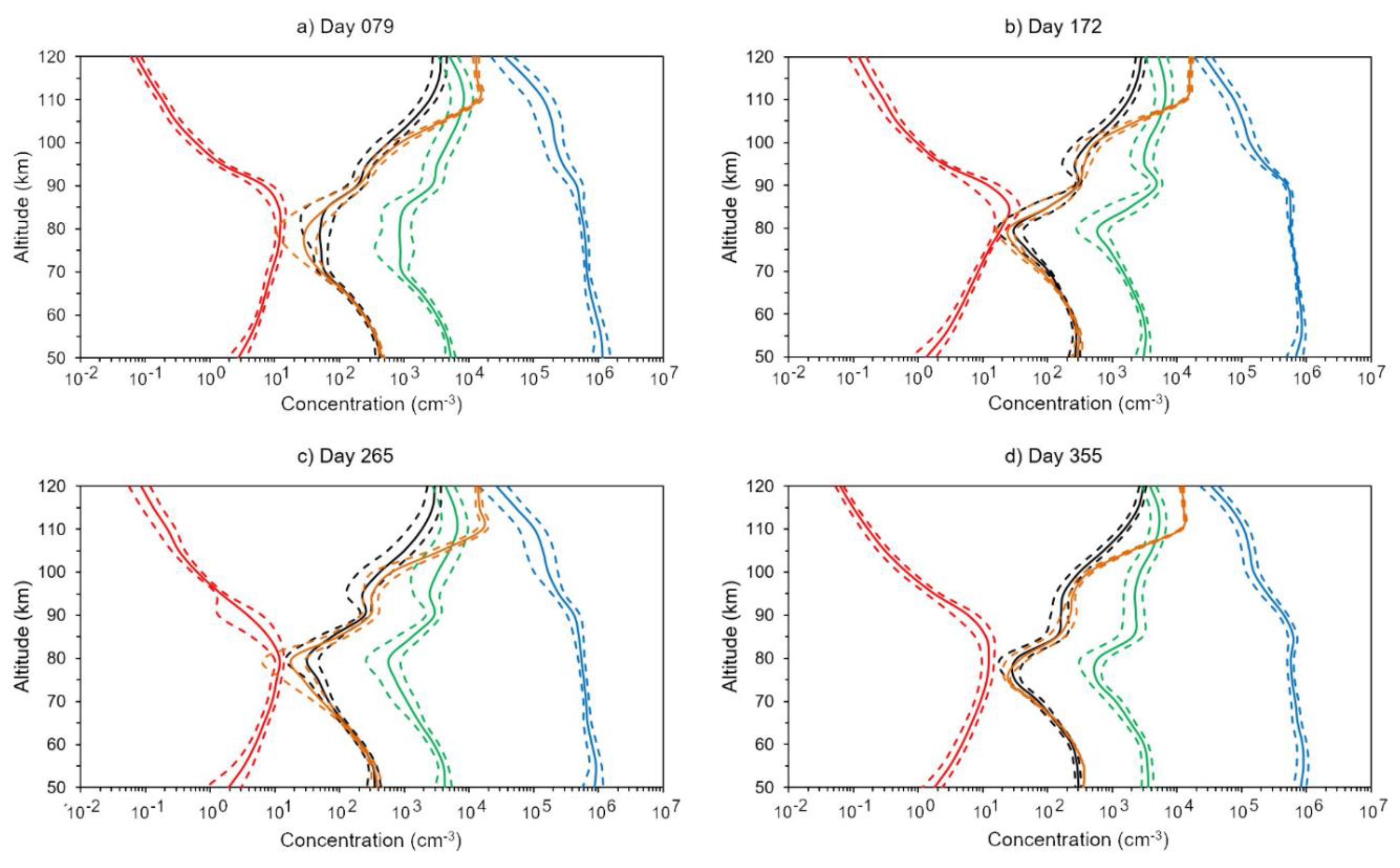
4.1. Forward Problem
4.2. Inverse Problem
5. Conclusions
- (a)
- The study presents contemporary insights to the daytime oxygen emissions in the mesopause region and above. We consider this altitude region, since in the mesosphere and lower thermosphere, intense energy transfer occurs between electronically vibrationally excited singlet levels of the oxygen molecule. In Section 2.2, we showed that a significant part of these reactions has high rates due to quasi-resonant effects during energy transfer.
- (b)
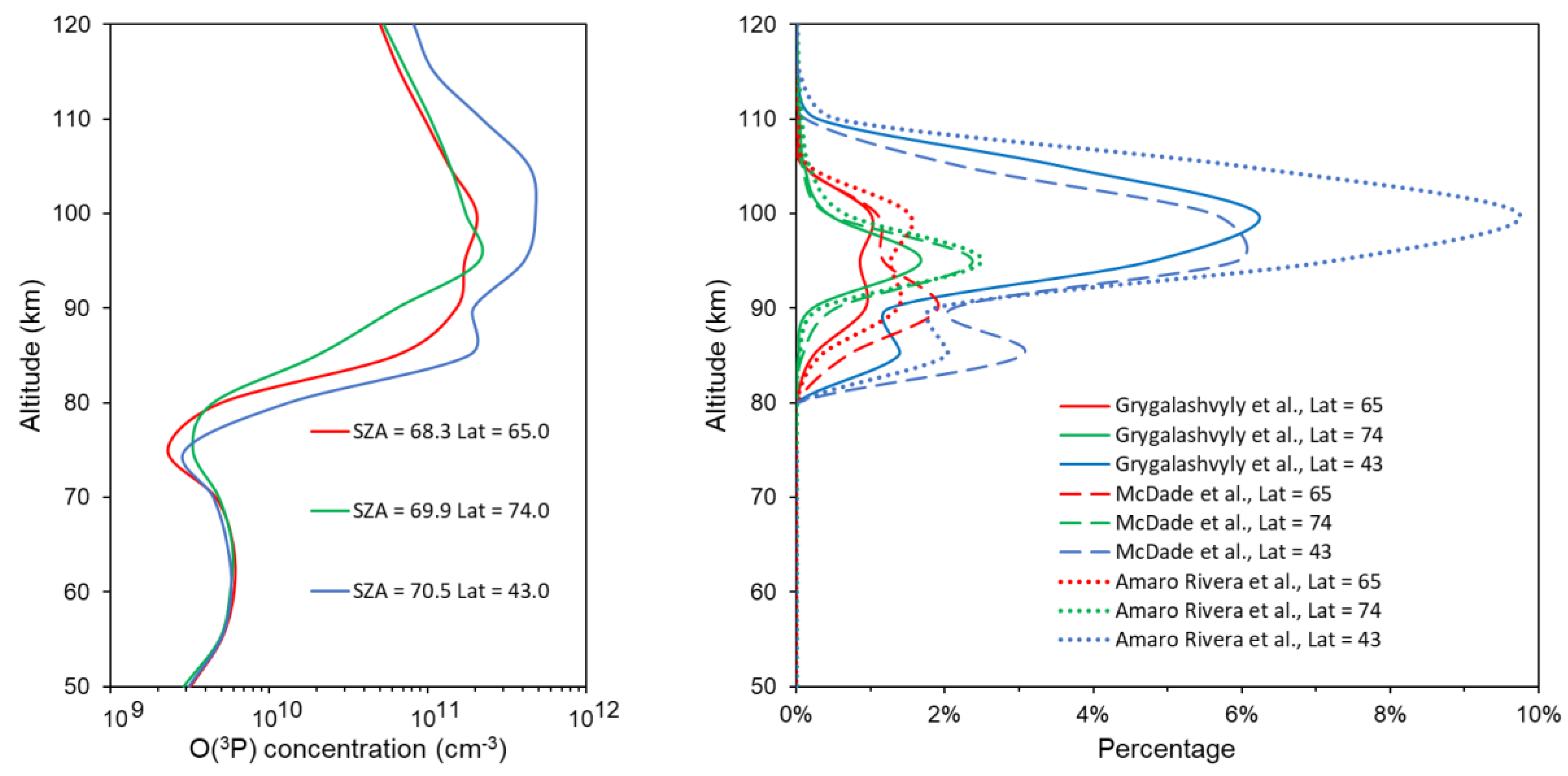
- Above the mesopause, special attention should be given to both the profile of atomic oxygen itself and to processes with its participation, since rate coefficients of reactions involving O(3P) have the greatest error today. Below the mesopause region where the role of atomic oxygen is insignificant, considering the electronic–vibrational kinetics of the O2 and O3 photolysis products solves the issues of the MSZ model associated with an overstatement of the retrieved ozone concentration in the mesosphere (see Introduction).
- (c)
- In the presented new version of the YM2011 model, we first considered the additional channels for the formation of O(1D) atoms during the photolysis of ozone in the Huggins band, as well as the energy transfer from O2(b1Σ+g, v = 0, 1) to O2(X3Σ−g, v = 9 and 10). Taking into account the energy transfer from vibrationally excited singlet levels of O2 molecule to vibrationally excited levels of the ground electronic state allows us to construct a complete model of the altitude distribution of O2(X3Σg−, v = 1–35) in the MLT region (Section 2.5). Currently, there is only one kinetics model of photolysis products that considers an energy transfer between 44 electronically–vibrationally excited levels of molecular oxygen and excited oxygen atom, includes collected for many years database of reaction rate coefficients and recently calculated Einstein coefficients, namely, the YM2011 model.
- (d)
- Special attention is given to the association of oxygen atoms in the triple reaction (Section 2.4) It has been shown that for different sets of fitting coefficients its contribution to O2(b1Σ+g, v) and O2(a1Δg, v) population is neglectable in daytime conditions.
- (e)
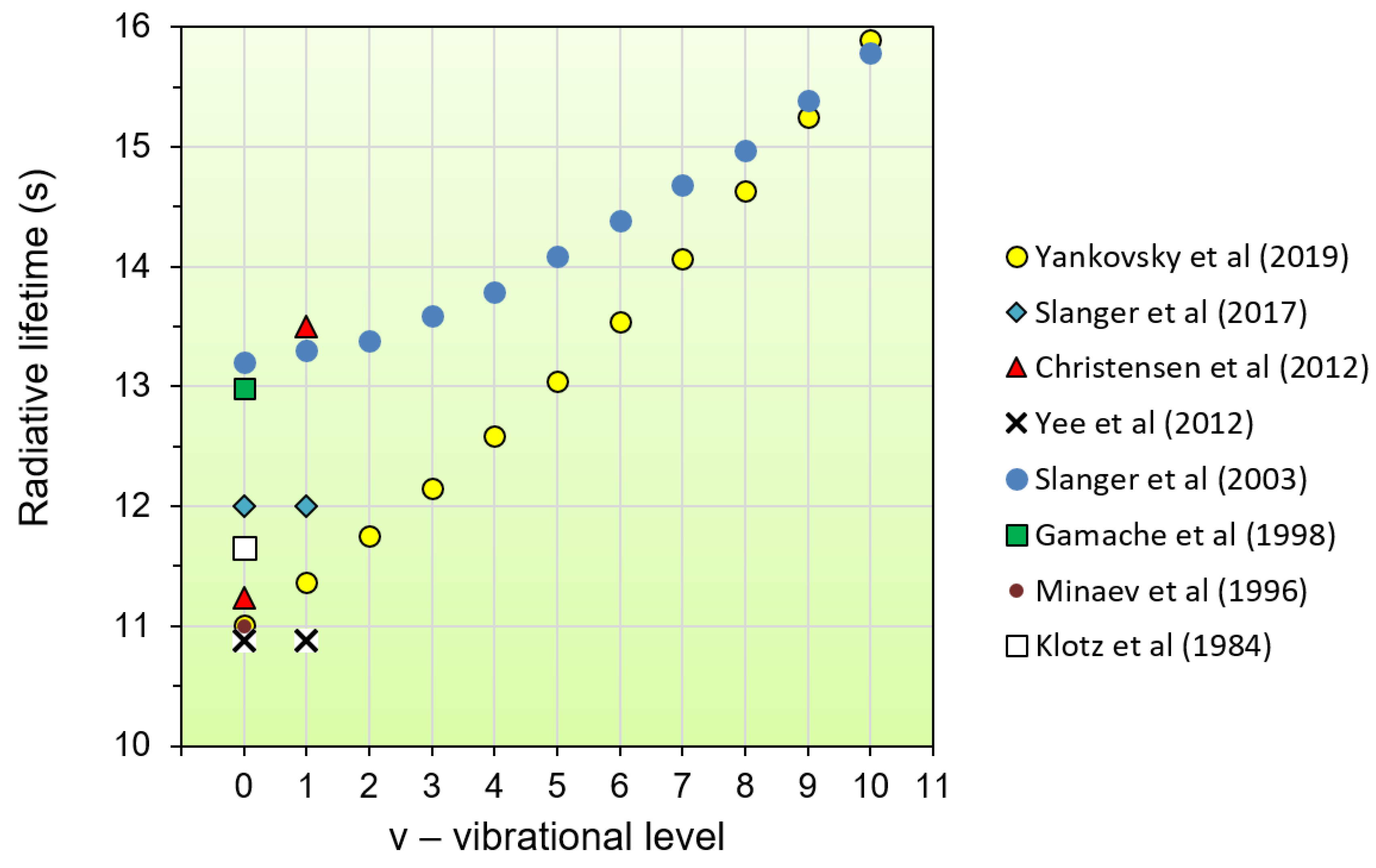
- For the first time, new estimates of the radiative lifetimes of electronically–vibrationally excited oxygen molecules O2(b1Σ+g, v = 0–10) are presented. These estimates are relevant due to the fact that in the thermosphere, as the height increases, the role of radiation quenching becomes dominant compared to the collisional deactivation (see Section 2.3).
- (f)
- The model YM2011 allows us to solve both forward and inverse problems. By the forward problem, we mean the calculation of altitude profiles of concentration of excited oxygen components in the MLT region (see Section 4.1). This is especially important for those components whose observations have not yet been implemented. By the inverse photochemical problem, we understand the retrieval of concentrations of non-radiating small atmospheric components that are in the main unexcited state (for example, O(3P), O3, CO2) based on the observation of altitude profiles of the emitting singlet levels of oxygen atoms and molecules (see Section 4.2).
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Yankovsky, V.A.; Martyshenko, K.V.; Manuilova, R.O.; Feofilov, A.G. Oxygen dayglow emissions as proxies for atomic oxygen and ozone in the mesosphere and lower thermosphere. J. Mol. Spectrosc. 2016, 327, 209–232. [Google Scholar] [CrossRef]
- Yankovsky, V.; Vorobeva, E.; Manuilova, R. New techniques for retrieving the [O(3P)], [O3] and [CO2] altitude profiles from dayglow oxygen emissions: Uncertainty analysis by the Monte Carlo method. Adv. Space Res. 2019, 64, 1948–1967. [Google Scholar] [CrossRef]
- Degen, V. Nightglow emission rates in the O2 Herzberg bands. J. Geophys. Res. 1977, 82, 2437–2438. [Google Scholar] [CrossRef]
- Llewellyn, E.J.; Solheim, B.H. The excitation of the infrared atmospheric oxygen bands in the nightglow. Planet. Space Sci. 1978, 26, 533–538. [Google Scholar] [CrossRef]
- Klais, O.; Laufer, A.H.; Kurylo, M.J. Atmospheric quenching of vibrationally excited O2(a1Δg, v). J. Chem. Phys. 1980, 73, 2696–2699. [Google Scholar] [CrossRef]
- Greer, R.G.H.; Llewellyn, E.J.; Solheim, B.H.; Witt, G. The excitation of the O2(b1Σg+) in the nightglow. Planet. Space Sci. 1981, 29, 383–389. [Google Scholar] [CrossRef]
- Harris, R.D.; Adams, G.W. Where does the O(1D) energy go? J. Geophys. Res. 1983, 88, 4918–4928. [Google Scholar] [CrossRef]
- Thomas, R.J.; Young, R.A. Measurement of atomic oxygen and related airglows in the lower thermosphere. J. Geophys. Res. 1981, 86, 7389–7393. [Google Scholar] [CrossRef]
- Shimazaki, T. The photochemical time constants of minor constituents and their families in the middle atmosphere. J. Atmos. Terr. Phys. 1984, 46, 173–191. [Google Scholar] [CrossRef]
- McDade, I.C.; Llewellyn, E.J. The excitation of O(1S) and O2 bands in the nightglow: a brief review and preview. Can. J. Phys. 1986, 12, 1626–1630. [Google Scholar] [CrossRef]
- Clancy, R.T.; Rusch, D.W.; Thomas, R.J.; Allen, M.; Eckman, R.S. Model ozone photochemistry on the basic of solar mesosphere explorer mesospheric observations. J. Geophys. Res. 1987, 92, 3067–3080. [Google Scholar] [CrossRef]
- Slanger, T.G.; Llewellyn, E.J.; McDade, I.C.; Witt, G. Comment on The O2 atmospheric dayglow in the thermosphere by M. R. Torr, B.Y. Welsh, and D. G. Torr. J. Geophys. Res. 1987, 92, 7753–7755. [Google Scholar] [CrossRef]
- Evans, W.F.J.; McDade, I.C.; Yuen, J.; Llewellyn, E.J. A rocket measurement of the O2 Infrared Atmospheric (0-0) band emission in the dayglow and a determination of the mesospheric ozone and atomic oxygen densities. Can. J. Phys. 1988, 66, 941–946. [Google Scholar] [CrossRef]
- Batista, P.P.; Takahashi, H.; Sahai, Y.; Llewellyn, E.J. Mesospheric ozone concentration at an equatorial location from the 1.27 μm O2 airglow emissions. J. Geophys. Res. 1996, 101, 7917–7921. [Google Scholar] [CrossRef]
- McDade, I.C.; Murtagh, D.P.; Greer, R.G.H.; Dickinson, P.H.G.; Witt, G.; Stegman, J.; Llewellyn, E.J.; Thomas, L.; Jenkins, D.B. ETON 2: quenching parameters for the proposed precursor of O2(b1Σg+) and O(1S) in the terrestrial nightglow. Planet. Space Sci. 1986, 34, 789–800. [Google Scholar] [CrossRef]
- Murtagh, D.P.; McDade, I.C.; Greer, R.G.H.; Stegman, J.; Witt, G.; Llewellyn, E.J. ETON 4: an experimental investigation of the altitude dependence of the O2(A) vibrational populations in the nightglow. Planet. Space Sci. 1986, 34, 811–817. [Google Scholar] [CrossRef]
- Greer, R.G.H.; Murtagh, D.P.; McDade, I.C.; Dickinson, P.H.G.; Thomas, L.; Jenkins, D.B.; Stegman, J.; Llewellyn, E.J.; Witt, G.; Mackinnon, D.J.; et al. ETON 1: A database pertinent to the study of energy transfer in the oxygen nightglow. Planet. Space Sci. 1986, 34, 771–788. [Google Scholar] [CrossRef]
- Grygalashvyly, M.; Eberhart, M.; Hedin, J.; Strelnikov, B.; Lübken, F.-J.; Rapp, M.; Löhle, S.; Fasoulas, S.; Khaplanov, M.; Gumbel, J.; et al. Atmospheric band fitting coefficients derived from a self-consistent rocket-borne experiment. Atmos. Chem. Phys. 2019, 19, 1207–1220. [Google Scholar] [CrossRef]
- Amaro-Rivera, Y.; Huang, T.-Y.; Urbina, J.; Vargas, F. Empirical values of branching ratios in the three-body recombination reaction for O(1S) and O2(0,0) airglow chemistry. Adv. Space Res. 2018, 62, 2679–2691. [Google Scholar] [CrossRef]
- Lednyts’kyy, O.; von Savigny, C.; Sinnhuber, M.; Iwagami, N.; Mlynczak, M. Multiple Airglow Chemistry approach for atomic oxygen retrievals on the basis of in situ nightglow emissions. J. Atmos. Sol. Terr. 2019, 194, 105096. [Google Scholar] [CrossRef]
- Mlynczak, M.G.; Solomon, S.C.; Zaras, D.S. An updated model for O2(a1Δg) concentrations in the mesosphere and lower mesosphere and implications for remote sensing of ozone at 1.27 μm. J. Geophys. Res. 1993, 98, 18639–18648. [Google Scholar] [CrossRef]
- Torr, M.T.; Torr, D.G. A Preliminary Spectroscopic Assessment of the Spacelab 1/Shuttle Optical Environment. J. Geophys. Res. 1985, 90, 1683–1690. [Google Scholar] [CrossRef]
- Torr, M.R.; Owens, J.K.; Torr, D.G. Reply [to “Comment on ‘The O2 Atmospheric dayglow in the thermosphere’ by M. R. Torr, B.Y. Welsh, and D. G. Torr”]. J. Geophys. Res. 1987, 92, 7756–7760. [Google Scholar] [CrossRef]
- Yee, J.-H.; DeMajistre, R.; Morgan, F. The O2(b1Σg+) dayglow emissions: application to middle and upper atmosphere remote sensing. Can. J. Phys. 2012, 90, 769–784. [Google Scholar] [CrossRef]
- Slanger, T.G.; Copeland, R.A. Energetic Oxygen in the Upper Atmosphere and the Laboratory. Chem. Rev. 2003, 103, 4731–4766. [Google Scholar] [CrossRef] [PubMed]
- Smith, A.K.; Harvey, V.L.; Mlynczak, M.G.; Funke, B.; Garcia-Comas, M.; Kaufmann, M.; Kyrölä, E.; López-Puertas, M.; McDade, I.; Randall, C.E.; et al. Satellite observations of ozone in the upper mesosphere. J. Geophys. Res. 2013, 118, 5803–5821. [Google Scholar] [CrossRef]
- Mlynczak, M.G.; Morgan, F.; Yee, J.-H.; Espy, P.; Murtagh, D.; Marshall, B.T.; Schmidlin, F. Simultaneous measurements of the O2(a1Δg) and O2(b1Σg+) airglows and ozone in the daytime mesosphere. Geophys. Res. Lett. 2001, 28, 999–1002. [Google Scholar] [CrossRef]
- López-Puertas, M.; Taylor, F.W. Non-LTE Radiative Transfer in the Atmosphere; World Scientific Pub.: Singapore, 2001; Volume 3, 487p. [Google Scholar]
- Park, H.; Slanger, T.G. O2(X, v = 8–22) 300K quenching rate coefficients for O2 and N2, and O2(X) vibrational distribution from 248 nm O3 photodissociation. J. Chem. Phys. 1994, 100, 287–300. [Google Scholar] [CrossRef]
- Yankovsky, V.A.; Manuilova, R.O. New self-consistent model of daytime emissions of O2(a1Δg, v ≥ 0) and O2(b1Σg+, v = 0, 1, 2) in the middle atmosphere. Retrieval of vertical ozone profile from the measured intensity profiles of these emissions. Atmos. Ocean. Opt. 2003, 16, 536–540. [Google Scholar]
- Yankovsky, V.A.; Manuilova, R.O.; Kuleshova, V.A. Heating of the middle atmosphere as a result of quenching of the products of O2 and O3 photodissociation. SPIE Proc. Int. Soc. Opt. Eng. 2004, 5743, 34–40. [Google Scholar] [CrossRef]
- Yankovsky, V.A.; Manuilova, R.O. Model of daytime emissions of electronically-vibrationally excited products of O3 and O2 photolysis: application to ozone retrieval. Ann. Geophys. 2006, 24, 2823–2839. [Google Scholar] [CrossRef][Green Version]
- Yankovsky, V.A.; Babaev, A.S. Photolysis of O3 at Hartley, Chappuis, Huggins, and Wulf Bands in the Middle Atmosphere: Vibrational Kinetics of Oxygen Molecules O2(X3Σ−g, v ≤ 35). Atmos. Ocean. Opt. 2011, 24, 6–16. [Google Scholar] [CrossRef]
- Yankovsky, V.A.; Manuilova, R.O.; Babaev, A.S.; Feofilov, A.G.; Kutepov, A.A. Model of electronic-vibrational kinetics of the O3 and O2 photolysis products in the middle atmosphere: applications to water vapor retrievals from SABER/TIMED 6.3 μm radiance measurements. Int. J. Remote Sens. 2011, 32, 3065–3078. [Google Scholar] [CrossRef]
- Yankovsky, V.A.; Manuilova, R.O. Possibility of simultaneous [O3] and [CO2] altitude distribution retrievals from the daytime emissions of electronically-vibrationally excited molecular oxygen in the mesosphere. J. Atmos. Sol. Terr. Phys. 2018, 179, 22–33. [Google Scholar] [CrossRef]
- Bucholtz, A.; Skinner, W.R.; Abreu, V.J.; Hays, P.B. The dayglow of the O2 atmospheric band system. Planet. Space Sci. 1986, 34, 1031–1035. [Google Scholar] [CrossRef]
- Mlynczak, M.G.; Marshall, B.T. A reexamination of the role of solar heating in the O2 atmospheric and infrared atmospheric bands. Geophys. Res. Lett. 1996, 23, 657–660. [Google Scholar] [CrossRef]
- Witt, G.; Stegman, J.; Murtagh, D.P.; McDade, I.C.; Greer, R.G.H.; Dickinson, P.H.G.; Jenkins, D.B. Collisional energy transfer and the excitation of O2(b1Σg+) in the atmosphere. J. Photochem. 1984, 25, 365–378. [Google Scholar] [CrossRef]
- Torr, M.T.; Torr, D.G. The role of metastable species in the thermosphere. Rev. Geophys. 1982, 20, 91–114. [Google Scholar] [CrossRef]
- Svanberg, M.; Pettersson, J.B.C.; Murtagh, D. Ozone photodissociation in the Hartley band: A statistical description of the ground state decomposition channel O2(X3Σ−g) + O(3P). J. Chem. Phys. 1995, 102, 8887–8896. [Google Scholar] [CrossRef]
- Sparks, R.K.; Carlson, L.R.; Snobatake, K.; Kowalczyk, M.L.; Lee, Y.T. Ozone photolysis: A determination of the electronic and vibrational state distributions of primary products. J. Chem. Phys. 1980, 72, 1401–1402. [Google Scholar] [CrossRef]
- Valentini, J.J.; Gerrity, D.P.; Phillips, D.L.; Nieh, J.-C.; Tabor, K.D. CARS spectroscopy of O2(a1Δg) from the Hartley band photodissociation of O3: Dynamics of the dissociation. J. Chem. Phys. 1987, 86, 6745–6756. [Google Scholar] [CrossRef]
- Thelen, M.-A.; Gejo, T.; Harrison, J.A.; Huber, J.R. Photodissociation of ozone in the Hartley band: Fluctuation of the vibrational state distribution in the O2(a1Δg) fragment. J. Chem. Phys. 1995, 103, 7946–7955. [Google Scholar] [CrossRef]
- Dylewski, S.M.; Geiser, J.D.; Houston, P.L. The energy distribution, angular distribution, and alignment of the O(1D2) fragment from the photodissociation of ozone between 235 and 305 nm. J. Chem. Phys. 2001, 115, 7460–7473. [Google Scholar] [CrossRef]
- Yankovsky, V.A.; Kuleshova, V.A. Ozone photodissociation at excitation within the Hartley absorption band. Analytical description of quantum yields O2(a1Δg, v = 0–3) depending on the wavelength. Atmos. Ocean. Opt. 2006, 19, 514–518. [Google Scholar]
- Yankovsky, V.A.; Kuleshova, V.A.; Manuilova, R.O.; Semenov, A.O. Retrieval of total ozone in the mesosphere with a new model of electronic-vibrational kinetics of O2 and O3 photolysis products. Izvestiya Atmos. Ocean. Phys. 2007, 43, 514–525. [Google Scholar] [CrossRef]
- Martyshenko, K.V.; Yankovsky, V.A. IR Band of O2 at 1.27 μm as the Tracer of O3 in the Mesosphere and Lower Thermosphere: Correction of the Method. Geomagn. Aeron. 2017, 57, 229–241. [Google Scholar] [CrossRef]
- Klatt, M.; Smith, I.W.M.; Symonds, A.C.; Tuckett, R.P.; Ward, G.N. State-specific rate constants for the relaxation of O2(X3Σ−g, v = 8–11) in collisions with O2, N2, NO2, CO2, N2O and He. J. Chem. Soc. Faraday Trans. 1996, 92, 193–199. [Google Scholar] [CrossRef]
- Hernandez, R.; Toumi, R.; Clary, D.C. State-selected vibrational relaxation rates for highly vibrationally excited oxygen molecules. J. Chem. Phys. 1995, 102, 9544–9556. [Google Scholar] [CrossRef]
- Watanabe, S.; Usuda, S.; Fujii, H.; Hatano, H.; Tokue, I.; Yamasaki, K. Vibrational relaxation of O2(X3Σ−g, v = 9–13) by collisions with O2. Phys. Chem. Chem. Phys. 2007, 9, 4407–4413. [Google Scholar] [CrossRef]
- Kalogerakis, K.S.; Copeland, R.A.; Slanger, T.G. Vibrational energy transfer in O2(X3Σ−g, v = 2, 3) + O2 collisions at 330K. J. Chem. Phys. 2005, 123, 044309. [Google Scholar] [CrossRef]
- Mack, J.A.; Mikulesky, F.; Wodtke, A.M. Resonant vibration-vibration energy transfer between highly vibrationally excited O2(X, v = 15–26) and CO2, N2O, N2, and O3. J. Chem. Phys. 1996, 105, 4105–4116. [Google Scholar] [CrossRef]
- Rogaski, C.A.; Price, J.M.; Mack, J.A.; Wodtke, A.M. Laboratory evidence for a possible non-LTE mechanism of stratospheric ozone formation. Geophys. Res. Lett. 1993, 20, 2885–2888. [Google Scholar] [CrossRef]
- Price, J.M.; Mack, J.A.; Rogaski, C.A.; Wodtke, A.M. Vibrational-state-specific self-relaxation rate constant. Measurements of highly vibrationally excited O2(v = 19–28). Chem. Phys. 1993, 175, 83–98. [Google Scholar] [CrossRef]
- Coletti, C.; Billing, G.D. Vibrational energy transfer in molecular oxygen collisions. Chem. Phys. Lett. 2002, 356, 14–22. [Google Scholar] [CrossRef]
- Bloemink, H.I.; Copeland, R.A.; Slanger, T.G. Collisional removal of vibrationally excited O2(b1Σg+) by O2, N2, and CO2. J. Chem. Phys. 1998, 109, 4237–4245. [Google Scholar] [CrossRef]
- Hwang, E.S.; Bergman, A.; Copeland, R.A.; Slanger, T.G. Temperature dependence of the collisional removal of O2(b1Σg+, v = 1 and 2) at 110–260 K, and atmospheric applications. J. Chem. Phys. 1999, 110, 18–24. [Google Scholar] [CrossRef]
- Kalogerakis, K.S.; Copeland, R.A.; Slanger, T.G. Collisional removal of O2(b1Σg+, v = 2, 3). J. Chem. Phys. 2002, 116, 4877–4885. [Google Scholar] [CrossRef]
- Pejakovic, D.A.; Campbell, Z.; Kalogerakis, K.S.; Copeland, R.A.; Slanger, T.G. Collisional relaxation of O2(X3Σ−g, v = 1) and O2(a1Δg, v = 1) by atmospherically relevant species. J. Chem. Phys. 2011, 135, 094309. [Google Scholar] [CrossRef]
- Burkholder, J.B.; Abbatt, J.P.D.; Huie, R.E.; Kurylo, M.J.; Wilmouth, D.M.; Sander, S.P.; Barker, J.R.; Kolb, C.E.; Orkin, V.L.; Wine, P.H. Chemical Kinetics and Photochemical Data for Use in Atmospheric Studies: Evaluation Number 18; California Institute of Technology: Pasadena, CA, USA, 2015. [Google Scholar]
- Yankovsky, V.A. Electron-vibrational relaxation of O2(b1Σg+, v = 1, 2) molecules in collision with ozone, oxygen molecules and atoms. Russ. J. Phys. Chem. 1991, 10, 291–306. [Google Scholar]
- Esposito, F.; Armehise, I.; Capitta, G.; Capitelli, M. O–O2 state-to-state vibrational-relaxation and dissociation rates based on quasiclassical calculations. Chem. Phys. 2008, 351, 91–98. [Google Scholar] [CrossRef]
- Dayou, F.; Hernandez, M.I.; Campos-Martinez, J.; Hernandez-Lamoneda, R. Nonadiabatic couplings in the collisional removal of O2(b1Σg+, v) by O2. J. Chem. Phys. 2010, 132, 044313. [Google Scholar] [CrossRef] [PubMed]
- Klingshirn, H.; Maier, M. Quenching of the 1Σg+ state in liquid isotopes. J. Chem. Phys. 1985, 82, 714–719. [Google Scholar] [CrossRef]
- Pejakovic, D.A.; Wouters, E.R.; Phillips, K.E.; Slanger, T.G.; Copeland, R.A.; Kalogerakis, K.S. Collisional removal of O2(b1Σg+, v =1) by O2 at thermospheric temperatures. J. Geophys. Res. 2005, 110A, A03308. [Google Scholar] [CrossRef]
- Torbin, A.P.; Pershin, A.A.; Mebel, A.M.; Zagidullin, M.V.; Heaven, M.C.; Azyazov, V.N. Collisional relaxation of O2(a1Δg, v = 1, 2, 3) by CO2. Chem. Phys. Lett. 2017, 691, 456–461. [Google Scholar] [CrossRef]
- Wild, E.; Klingshirn, H.; Maier, M. Relaxation of the a1Δg state on pure liquid oxygen and in liquid mixtures of (16)O2 and (18)O2. J. Photochem. 1984, 25, 134–143. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Dalgarno, A.; Billing, G.D. Multiquantum vibrational transitions in O2(v≥25) + O2(v=0) collisions. Chem. Phys. Lett. 1998, 288, 657–662. [Google Scholar] [CrossRef]
- Billing, G.D. VV and VT rates in N2–O2 collisions. Chem. Phys. 1994, 179, 463–467. [Google Scholar] [CrossRef]
- Sharma, R.D.; Welsh, J.A. Vibrational energy transfer in O2(v = 2–8)–O2(v = 0) collisions. J. Chem. Phys. 2009, 130, 194306. [Google Scholar] [CrossRef]
- Yu, S.; Drouin, B.J.; Miller, C.E. High resolution spectral analysis of oxygen. IV. Energy levels, partition sums, band constants, RKR potentials, Franck-Condon factors involving the X3Σ−g, a1Δg and b1Σg+ states. J. Chem. Phys. 2014, 141, 174302. [Google Scholar] [CrossRef]
- Nicholls, R.W. Franck-Condon Factors to High Vibrational Quantum Numbers V: O2 Band Systems. J. Res. Natl. Bur. Stand. Sect. Phys. Chem. 1965, 69A, 369–373. [Google Scholar] [CrossRef]
- Krupenie, P.H. The spectrum of molecular oxygen. J. Phys. Chem. Ref. Data 1972, 1, 423–521. [Google Scholar] [CrossRef]
- Minaev, B.F. Magnetic phosphorescence of molecular oxygen. A study of the b–X transition probability using multiconfiguration response theory. Chem. Phys. 1996, 208, 299–311. [Google Scholar] [CrossRef]
- Brown, L.R.; Plymate, C. Experimental line parameters of oxygen A band at 760 nm. J. Mol. Spectrosc. 2000, 199, 166–179. [Google Scholar] [CrossRef] [PubMed]
- Gamache, R.R.; Goldman, A. Einstein A coefficient, integrated band intensity, and population factors: application to the a1Δg–X3Σ−g (0, 0) O2 band. J. Quantative Spectrosc. Radiat. Transf. 2001, 69, 389–401. [Google Scholar] [CrossRef]
- Goldman, A.; Stephen, T.V.; Rothman, L.S.; Giver, L.P.; Mandin, J.-Y.; Gamache, R.R.; Rinsland, C.P.; Murcray, F.J. The 1-μm CO2 bands and the O2(0-1) X3Σg−–a1Δg and (1-0) X3Σg−–b1Σg+ bands in the Earth atmosphere. J. Quantative Spectrosc. Radiat. Transf. 2003, 82, 197–205. [Google Scholar] [CrossRef]
- Gordon, I.E.; Rothman, L.S.; Toon, G.C. Revision of spectral parameters for the B- and g-bands of oxygen and their validation against atmospheric spectra. J. Quantative Spectrosc. Radiat. Transf. 2011, 112, 2310–2322. [Google Scholar] [CrossRef]
- Long, D.A.; Hodges, J.T. On spectroscopic models of the O2 A-band and their impact upon atmospheric retrievals. J. Geophys. Res. 2012, 117, D12309. [Google Scholar] [CrossRef]
- Naus, H.; Ubachs, W. The b1Σg+–X3Σg− (3, 0) band of (16)O2 and (18)O2. J. Mol. Spectrosc. 1999, 193, 442–445. [Google Scholar] [CrossRef][Green Version]
- Rothman, L.S.; Rinsland, C.P.; Goldman, A.; Massie, S.T.; Edwards, D.P.; Flaud, J.M.; Schroeder, J. The HITRAN molecular spectroscopic database and hawks (HITRAN atmospheric workstation): 1996 Edition. J. Quantative Spectrosc. Radiat. Transf. 2010, 60, 665–710. [Google Scholar] [CrossRef]
- Kuznetsova, L.A.; Kuzmenko, N.E.; Kuziakov, I.I.; Plastinin, I.A. Probabilities of Optical Transitions of Diatomic Molecules; Soviet Phys. Uspekhi. ed.; NAUKA: Moscow, Russia, 1980; 320p. (In Russian) [Google Scholar]
- Kassi, S.; Romanini, D.; Campargue, A.; Bussery-Honvault, B. Very high sensitivity CW-cavity ring down spectroscopy: Application to the a1Δg(0)–X3Σ−g(1) O2 band near 1.58 μm. Chem. Phys. Lett. 2005, 409, 281–287. [Google Scholar] [CrossRef]
- Gamache, R.R.; Goldman, A.; Rothman, L.S. Improved spectral parameters for the three most abundant isotopomers of the oxygen molecule. J. Quantative Spectrosc. Radiat. Transf. 1998, 59, 495–509. [Google Scholar] [CrossRef]
- Christensen, A.B.; Yee, J.-H.; Bishop, R.L.; Budzien, S.A.; Hecht, J.H.; Sivjee, G.; Stephan, A.W. Observations of molecular oxygen Atmospheric band emission in the thermosphere using the near infrared spectrometer on the ISS/RAIDS experiment. J. Geophys. Res. 2012, 117, A04315. [Google Scholar] [CrossRef]
- Slanger, T.G.; Pejakovic, D.A.; Kostko, O.; Matsiev, D.; Kalogerakis, K.S. Atmospheric dayglow diagnostics involving the O2(b–X) Atmospheric band emission: Global Oxygen and Temperature (GOAT) mapping. J. Geophys. Res. Space Phys. 2017, 122, 3640–3649. [Google Scholar] [CrossRef]
- Klotz, R.; Marian, C.M.; Peyrimhoff, S.D.; Hess, B.A.; Buenker, R.J. Calculation of spin-forbidden radiative transitions using correlated wavefunctions: lifetimes of b, a states in O2, S2 and SO. Chem. Phys. 1984, 89, 223–236. [Google Scholar] [CrossRef]
- Slanger, T.G.; Black, G. O(1S) in the lower thermosphere—Chapman vs. Barth. Planet. Space Sci. 1977, 25, 79–88. [Google Scholar] [CrossRef]
- Wu, K.; Fu, D.; Feng, Y.; Li, J.; Hao, X.; Li, F. Simulation and application of the emission line O19P18 of O2(a1Δg) dayglow near 1.27 μm for wind observations from limb-viewing satellites. Opt. Express 2018, 26, 16984–16999. [Google Scholar] [CrossRef]
- Atkinson, R.; Baulch, D.L.; Cox, R.A.; Crowley, J.N.; Hampson, R.F.; Hynes, R.G.; Jenkin, M.E.; Rossi, M.J.; Troe, J. Evaluated kinetic and photochemical data for atmospheric chemistry: Volume I–gas phase reactions of Ox, HOx, NOx and SOx species. Atmos. Chem. Phys. 2004, 4, 1461–1738. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Billing, G.D. Quantum-classical reaction path study of the reaction O(3P) + O3(1A1) → 2 O2(X3Σ−g). J. Chem. Phys. 1996, 104, 9482–9494. [Google Scholar] [CrossRef]
- SABER—Sounding of the Atmosphere Using Broadband Emission Radiometry. Available online: http://saber.gats-inc.com/data.php (accessed on 19 January 2020).
- SORCE. Available online: http://science.nasa.gov/missions/sorce/ (accessed on 19 January 2020).
- He, W.; Wu, K.; Feng, Y.; Fu, D.; Chen, Z.; Li, F. Radiative Transfer Characteristics of the O2 Infrared Atmospheric Band in Limb-Viewing Geometry. Remote Sens. 2019, 11, 2702. [Google Scholar] [CrossRef]

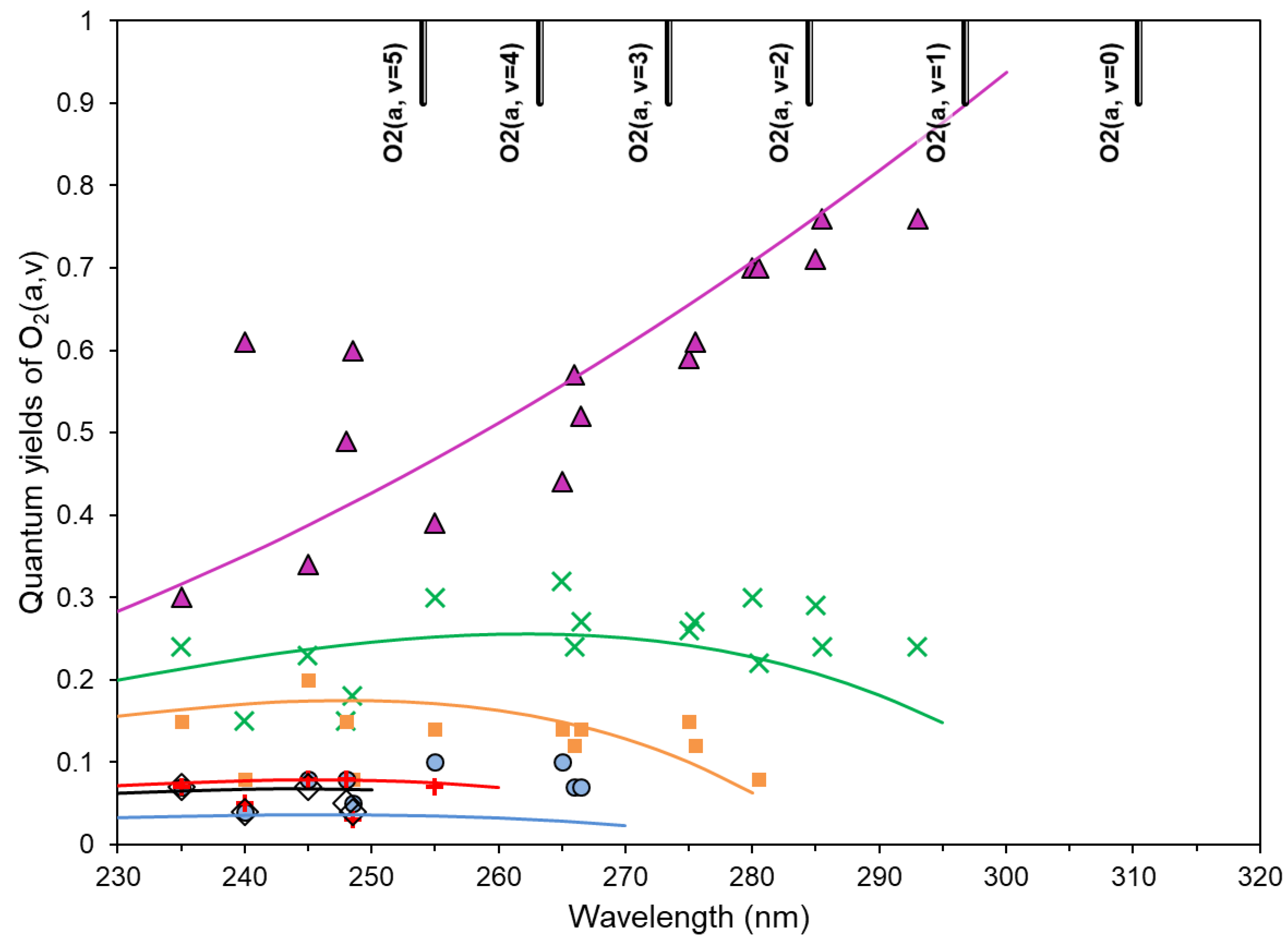

| Products of O3 Photodissociation in Hartley Band | O2(a1Δg, v) | |||||
|---|---|---|---|---|---|---|
| v = 0 | v = 1 | v = 2 | v = 3 | v = 4 | v = 5 | |
| Threshold wavelength, nm | 310 | 296 | 284 | 273 | 263 | 254 |
| Threshold value of x | 0.937 | 0.689 | 0.576 | 0.483 | 0.407 | 0.339 |
| Cv | 1.068 | 1.233 | 0.564 | 0.375 | 0.377 | 0.473 |
| No. | Reaction | Rate Coefficient, cm3 s−1 [2] | Supposed Products of Reaction | Energy Mismatch, cm−1 |
|---|---|---|---|---|
| 1 | O2(b1Σg+, v = 2) + O(3P) → | 1.07 × 10−11 | O2 + O(1D) | 37.6 |
| 2 | O2(b1Σg+, v = 1) + O(3P) → | 4.5 × 10−12 | O2(X3Σg−, v = 10) + O(3P) | 2.3 |
| 3 | O2(b1Σg+, v = 0) + O(3P) → | 8 × 10−14 | O2(a1Δg, v = 0) + O(3P) | 5238.0 |
| 4 | O2(a1Δg, v = 0) + O(3P) → | <3 × 10−16 | O2 + O(3P) | 7883.0 |
| Transition. | v′ = 0 | v′ = 1 | v′ = 2 | v′ = 3 | ||||
|---|---|---|---|---|---|---|---|---|
| v″ = 0 | v″ = 1 | v″ = 0 | v″ = 1 | v″ = 0 | v″ = 1 | v″ = 0 | v″ = 1 | |
| O2 Atm band (v′-v″) | 8.93 × 10−2 | 4.67 × 10−3 | 7.20 × 10−3 | 7.01 × 10−2 | 2.69 × 10−4 | 6.73 × 10−6 | ||
| O2 Noxon band (v′-v″) | 1.20 × 10−3 | |||||||
| O2 IR Atm band (v′-v″) | 2.26 × 10−4 | 2.81 × 10−6 | ||||||
| Details of Calculation. | Transitions | Reference |
|---|---|---|
| Morse potential | O2(b, v′ ≤ 3 ↔ X, v″ ≤ 3) | [72] |
| O2(b, v′ ≤ 3 ↔ a, v″ ≤ 5) | ||
| O2(a, v′ ≤ 5 ↔ X, v″ ≤ 6) | ||
| RKR potential | O2(b, v′ ≤ 3 ↔ X, v″ ≤ 8) | [73] |
| O2(a, v′ ≤ 3 ↔ X, v″ ≤ 6) | ||
| RKR potential | O2(b, v′ ≤ 4 ↔ X, v″ ≤ 4) | [23] |
| Transition moments from [74] | O2(b, v′ ≤ 10 ↔ X, v″ ≤ 9) | [25] |
| RKR potential | O2(b, v′ ≤ 10 ↔ X, v″ ≤ 35) | [71] |
| O2(b, v′ ≤ 10 ↔ a, v″ ≤ 15) | ||
| O2(a, v′ ≤ 10 ↔ X, v″ ≤ 35) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yankovsky, V.; Vorobeva, E. Model of Daytime Oxygen Emissions in the Mesopause Region and Above: A Review and New Results. Atmosphere 2020, 11, 116. https://doi.org/10.3390/atmos11010116
Yankovsky V, Vorobeva E. Model of Daytime Oxygen Emissions in the Mesopause Region and Above: A Review and New Results. Atmosphere. 2020; 11(1):116. https://doi.org/10.3390/atmos11010116
Chicago/Turabian StyleYankovsky, Valentine, and Ekaterina Vorobeva. 2020. "Model of Daytime Oxygen Emissions in the Mesopause Region and Above: A Review and New Results" Atmosphere 11, no. 1: 116. https://doi.org/10.3390/atmos11010116
APA StyleYankovsky, V., & Vorobeva, E. (2020). Model of Daytime Oxygen Emissions in the Mesopause Region and Above: A Review and New Results. Atmosphere, 11(1), 116. https://doi.org/10.3390/atmos11010116





