Evolution of Turbulence in the Kelvin–Helmholtz Instability in the Terrestrial Magnetopause
Abstract
1. Introduction
2. The Data: Geotail and THEMIS Magnetopause Crossing
3. Analysis
3.1. Magnetic Field Spectral Properties
3.2. Intermittency
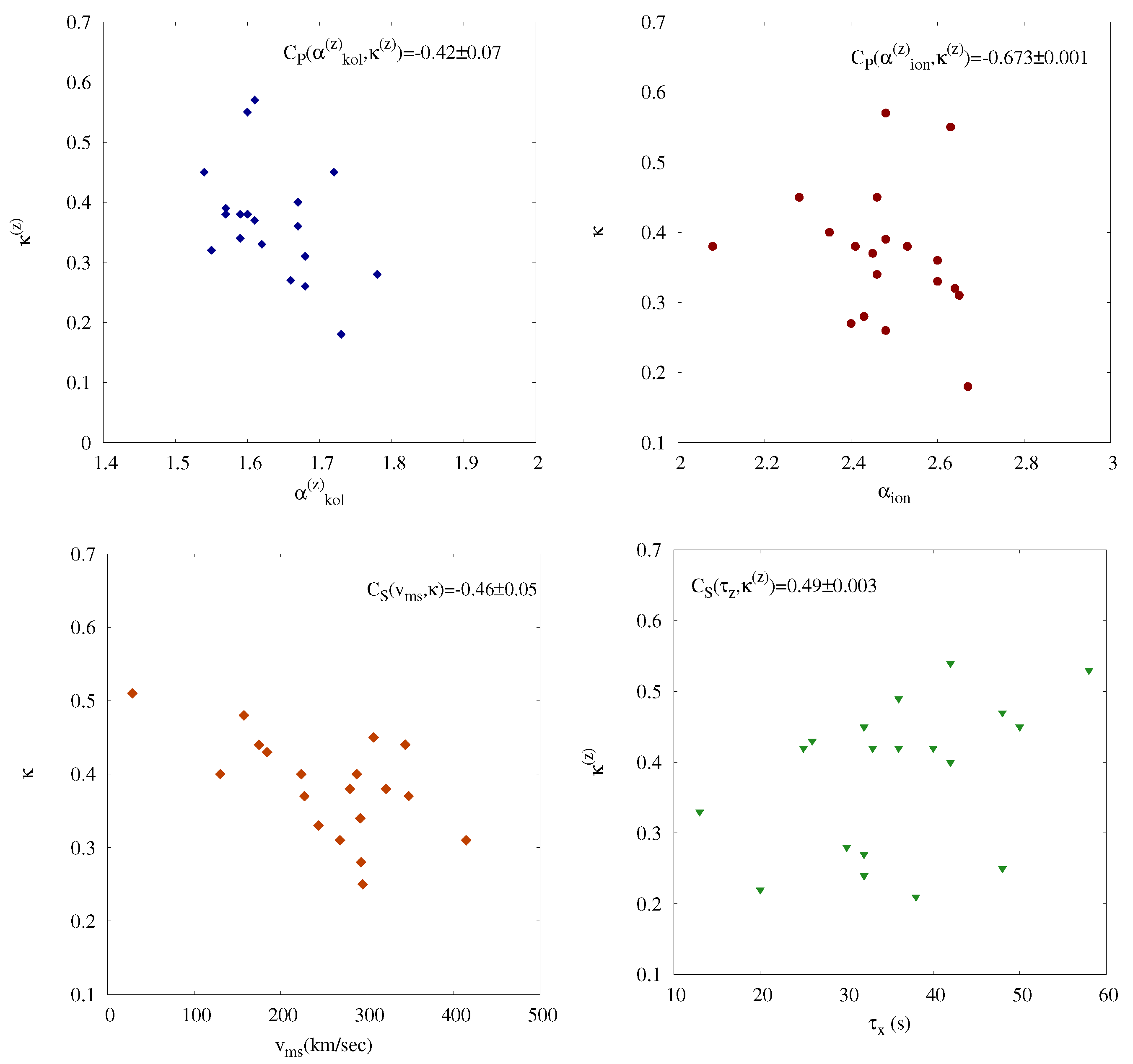
4. Evolution of the Turbulence along the Flank Magnetopause
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Additional Information about Daily Conditions of the Upstream Solar Wind
| Geotail Mission | |||||||
| Event | Interval (UT) | (nT) | , , (nT) | (km/s) | , , (km/s) | (1/cm3) | (K) |
| S | 1995-03-24 0600-0800 | 9.7 | 1.4; −4.5; 8.3 | 332 | −332; 12.3; −6,3 | 18.1 | 18,018 |
| O | 1997-01-10 2050-2400 | 13.7 | −2.5; −11.8; 5.7 | 414 | −414; 3.4; −13.9 | 20.6 | 11,343 |
| P | 1997-01-11 0400-0500 | 22.2 | −7.5; −3.8; 20.3 | 454 | −453; −19.0; 16.0 | 14.1 | 116,348 |
| Q | 1997-02-12 1430-1600 | 3.5 | −0.7; −0.3; 3.3 | 382 | −382; −4.1; −2.5 | 5.4 | 22,156 |
| T | 1998-04-13 0315-0430 | 8.8 | 4.5; −2.9; 6.7 | 390 | −390; −5.5; 4.8 | 3.8 | 23,776 |
| L | 1998-08-01 0530-0730 | 9.7 | −2.6; 7.8; 8.9 | 404 | −404; −7.9; −8.2 | 8.3 | 35,023 |
| D | 1998-12-27 1800-2100 | 5.2 | −1.8; 6.2; 4.7 | 371 | −370; −10.1; −29.3 | 4.4 | 34,764 |
| N | 1999-02-15 1445-1515 | 8.2 | −2.0; 3.9; 5.2 | 600 | −599; 25.5; −8.0 | 3.7 | 187,717 |
| M | 1999-07-20 0630-0730 | 6.3 | −2.1; 0.2; 5.9 | 303 | −303; 3.2; −5.0 | 15.4 | 25,486 |
| E | 2000-11-01 1030-1200 | 6.6 | 4.5; −0.2; 4.5 | 443 | −443; −8.7; −11.6 | 6.2 | 86,515 |
| H | 2001-01-25 1330-1630 | 3.4 | 1.5; −1.7; 1.8 | 372 | −372; 10.4; −13.1 | 4.5 | 10,762 |
| B | 2001-11-16 1900-2000 | 8.9 | −3.6; 5.4; 2.9 | 379 | −378; 1.6; −14.5 | 4.0 | 59,474 |
| C | 2001-12-07 2000-2130 | 5.4 | 2.3; −2.0; 3.4 | 462 | −461; 19.3; 9.1 | 3.1 | 101,406 |
| E2 | 2002-03-25 0530-0900 | 17.0 | 5.7; −13.1; 9.0 | 444 | −443; 6.5; 17.9 | 5.7 | 37,541 |
| F | 2002-03-25 1000-1300 | 14.8 | 5.2; −11.0; 8.2 | 435 | −435; −9.2; 12.1 | 6.7 | 20,890 |
| A | 2002-10-15 2100-2300 | 14.9 | 2.7; 8.6; 5.5 | 532 | −525; 49.8; −61.1 | 4.1 | 812,629 |
| R | 2003-07-17 0330-0500 | 3.9 | 2.7; −1.3; 1.8 | 632 | −632; 11.5; 13.5 | 2.6 | 190,142 |
| THEMIS Mission | |||||||
| TH1 | 2008-11-06 0850-0920 | 2.7 | 2.1; −0.8; 1.5 | 288 | −288; −8.7; 3.1 | 6.5 | 14,542 |
| TH2 | 2008-11-18 0720-0730 | 3.4 | -0.9; −1.1; 3.1 | 347 | −346; −15.1; 9.9 | 4.5 | 31,423 |
References
- Pucci, F.; Malara, F.; Perri, S.; Zimbardo, G.; Sorriso-Valvo, L.; Valentini, F. Energetic particle transport in the presence of magnetic turbulence: influence of spectral extension and intermittency. Mon. Not. R. Astron. Soc. 2016, 459, 3395–3406. [Google Scholar] [CrossRef]
- Zimbardo, G.; Greco, A.; Veltri, P.; Voros, Z.; Taktakishvili, A.L. Magnetic turbulence in and around the Earth’s magnetosphere. Astrophys. Space Sci. Trans. 2008, 4, 35–40. [Google Scholar] [CrossRef][Green Version]
- Mitchell, D.G.; Kutchko, F.; Williams, D.J.; Eastman, T.E.; Frank, L.A.; Russell, C.T. An extended study of the low-latitude boundary layer on the dawn and dusk flanks of the magnetosphere. J. Geophys. Res. 1987, 92, 7394–7404. [Google Scholar] [CrossRef]
- Bavassano Cattaneo, M.B.; Marcucci, M.F.; Bogdanova, Y.V.; Réme, H.; Dandouras, I.; Kistler, L.M.; Lucek, E. Global reconnection topology as inferred from plasma observations inside Kelvin–Helmholtz vortices. Ann. Geophys. 2010, 28, 893–906. [Google Scholar] [CrossRef]
- Johnson, J.R.; Wing, S.; Delamere, P.A. Kelvin Helmholtz Instability in Planetary Magnetospheres. Space Sci. Rev. 2014, 184, 1–31. [Google Scholar] [CrossRef]
- Hasegawa, H. Comment on "Evolution of Kelvin–Helmholtz activity on the dusk flank magnetopause" by Foullon et al. J. Geophys. Res. 2009, 114, A03205. [Google Scholar] [CrossRef]
- Sckopke, N.; Paschmann, G.; Haerendel, G.; Sonnerup, B.U.; Bame, S.J.; Forbes, T.G.; Hones, E.W.; Russell, C.T. Structure of the low-latitude boundary layer. J. Geophys. Res. 1981, 86, 2099–2110. [Google Scholar] [CrossRef]
- Sonnerup, B. Theory of the low-latitude boundary layer. J. Geophys. Res. 1980, 85, 2017–2026. [Google Scholar] [CrossRef]
- Lui, A.T.Y.; Venkatesan, D.; Murphree, J.S. Auroral bright spots on the dayside oval. J. Geophys. Res. 1989, 94, 5515. [Google Scholar] [CrossRef]
- Yamamoto, T. A linear analysis of the hybrid Kelvin-Helmholtz/Rayleigh-Taylor instability in an electrostatic magnetosphere-ionosphere coupling system. J. Geophys. Res. 2008, 113, A06206. [Google Scholar] [CrossRef]
- Chen, S.H.; Kivelson, M.G. On nonsinusoidal waves at the Earth’s magnetopause. Geophys. Res. Lett. 1993, 20, 2699–2702. [Google Scholar] [CrossRef]
- Fairfield, D.H.; Otto, A.; Mukai, T.; Kokubun, S.; Lepping, R.P.; Steinberg, J.T.; Lazarus, A.J.; Yamamoto, T. Geotail observations of the Kelvin–Helmholtz instability at the equatorial magnetotail boundary for parallel northward fields. J. Geophys. Res. 2000, 105, 21159–21173. [Google Scholar] [CrossRef]
- Kivelson, M.G.; Chen, S.H. The Magnetopause: Surface Waves and Instabilities and Their Possible Dynamical Consequences; Geophys. Monogr. Ser., 90; Song, P., Sonnerup, B.U.Ö., Thomsen, M.F., Eds.; American Geophysicsl Union: Washington, DC, USA, 1995; Volume 20, pp. 257–268. [Google Scholar]
- Fujimoto, M.; Tonooka, T.; Mukai, T. Vortex-Like Fluctuations in the Magnetotail Flanks and Their Possible Roles in Plasma Transport; Geophys. Monogr. Ser. 133; Newell, P.T., Onsager, T., Eds.; American Geophysicsl Union: Washington, DC, USA, 2003; pp. 241–251. [Google Scholar]
- Hasegawa, H.; Fujimoto, M.; Takagi, K.; Saito, Y.; Mukai, T.; Réme, H. Single-spacecraft detection of rolled-up Kelvin–Helmholtz vortices at the flank magnetopause. J. Geophys. Res. 2006, 111, A09203. [Google Scholar]
- Terasawa, T.; Fujimoto, M.; Mukai, T.; Shinohara, I.; Saito, Y.; Yamamoto, T.; Machida, S.; Kokubun, S.; Lazarus, A.J.; Steinberg, J.T.; et al. Solar wind control of density and temperature in the near-Earth plasma sheet: Wind/Geotail collaboration. Geophys. Res. Lett. 1997, 24, 935–938. [Google Scholar] [CrossRef]
- Wing, S.; Newell, P.T. 2D plasma sheet ion density and temperature profiles for northward and southward IMF. Geophys. Res. Lett. 2002, 29, 9. [Google Scholar] [CrossRef]
- Sundberg, T.; Boardsen, S.A.; Slavin, J.A.; Anderson, B.J.; Korth, H.; Zurbuchen, T.H.; Raines, J.M.; Solomon, S.C. MESSENGER orbital observations of large-amplitude Kelvin-Helmholtz waves at Mercury’s magnetopause. J. Geophys. Res. 2012, 117, A04216. [Google Scholar] [CrossRef]
- Nakamura, T.K.M.; Hasegawa, H.; Daughton, W.; Eriksson, S.; Li, W.Y.; Nakamura, R. Turbulent mass transfer caused by vortex induced reconnection in collisionless magnetospheric plasmas. Nat. Commun. 2017, 8, 1582. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.H.; Kivelson, M.G.; Gosling, J.T.; Walker, R.J.; Lazarus, A.J. Anomalous aspects of magnetosheath flow and of the shape and oscillations of the magnetopause during an interval of strongly northward interplanetary magnetic field. J. Geophys. Res. 1993, 98, 5727–5742. [Google Scholar] [CrossRef]
- Fairfield, D.H.; Kuznetsova, M.M.; Mukai, T.; Nagai, T.; Gombosi, T.I.; Ridley, A.J. Waves on the dusk flank boundary layer during very northward interplanetary magnetic field conditions: Observations and simulation. J. Geophys. Res. 2007, 112, A08206. [Google Scholar] [CrossRef]
- Farrugia, C.J.; Gratton, F.T.; Torbert, R.B. Viscous-type processes in the solar wind-magnetosphere interaction. Space Sci. Rev. 2001, 95, 443–456. [Google Scholar]
- Hasegawa, H.; Fujimoto, M.; Phan, T.D.; Réme, H.; Balogh, A.; Dunlop, M.W.; Hashimoto, C.; Tandokoro, R. Transport of solar wind into Earth’s magnetosphere through rolled-up Kelvin–Helmholtz vortices. Nature 2004, 430, 755–758. [Google Scholar] [CrossRef] [PubMed]
- Kokubun, S.; Yamamoto, T.; Acuna, M.H.; Hayashi, K.; Shiokawa, K.; Kawano, H. The GEOTAIL magnetic field experiment. J. Geomag. Geo-Electr. 1994, 46, 7–21. [Google Scholar] [CrossRef]
- Otto, A.; Fairfield, D.H. Kelvin-Helmholtz instability at the magnetotail boundary: MHD simulation and comparison with Geotail observations. J. Geophys. Res. 2000, 105, 17521. [Google Scholar] [CrossRef]
- Foullon, C.; Farrugia, C.J.; Fazakerley, A.N.; Owen, C.J.; Gratton, F.T.; Torbert, R.B. Evolution of Kelvin-Helmholtz activity on the dusk flank magnetopause. J. Geophys. Res. 2008, 113, A11203. [Google Scholar] [CrossRef]
- Fairfield, D.H.; Farrugia, C.J.; Mukai, T.; Nagai, T.; Federov, A. Motion of the dusk flank boundary layer caused by solar wind pressure changes and the Kelvin-Helmholtz instability: 10–11 January 1997. J. Geophys. Res. 2003, 108, 1460–1471. [Google Scholar] [CrossRef]
- Fujimoto, M.; Terasawa, T.; Mukai, T. The Low-Latitude Boundary Layer in the Tail-Flanks; Geophysical Monograph 105; Nishida, A., Baker, D.N., Cowley, S.W.H., Eds.; American Geophysical Union: Washington, DC, USA, 1998; pp. 33–44. [Google Scholar]
- Stenuit, H.; Fujimoto, M.; Fuselier, S.A.; Sauvaud, J.A.; Wing, S.; Fedorov, A.; Budnik, E.; Savin, S.P.; Trattner, K.J.; Angelopoulos, V.; et al. Multispacecraft study on the dynamics of the dusk-flankmagnetosphere under northward IMF: 10–11 January 1997. J. Geophys. Res. 2002, 107, 1333. [Google Scholar] [CrossRef]
- Lin, D.; Wang, C.; Li, W.; Tang, B.; Guo, X.; Peng, Z. Properties of Kelvin–Helmholtz waves at the magnetopause under northward interplanetary magnetic field: Statistical study. J. Geophys. Res. 2014, 119, 7485–7494. [Google Scholar] [CrossRef]
- Takagi, K.; Hashimoto, C.; Hasegawa, H.; Fujimoto, M.; TanDokoro, R. Kelvin-Helmholtz instability in a magnetotail flank-like geometry: Three-dimensional MHD simulations. J. Geophys. Res. 2006, 111, A08202. [Google Scholar] [CrossRef]
- Taylor, G.I. The Spectrum of Turbulence. Proc. R. Soc. Lond. A 1938, 164, 476–490. [Google Scholar] [CrossRef]
- Klein, K.G.; Howes, G.G.; TenBarge, J.M. The violation of the Taylor hypothesis in measurements of solar wind turbulence. Astrophys. J. Lett. 2014, 790, L20. [Google Scholar] [CrossRef]
- Fredricks, R.W.; Coroniti, F.V. Ambiguities in the deduction of rest frame fluctuation spectra from spectra computed in moving frames. J. Geophys. Res. 1976, 81, 5591–5595. [Google Scholar] [CrossRef]
- Stawarz, J.E.; Eriksson, S.; Wilder, F.D.; Ergun, R.E.; Schwartz, S.J.; Pouquet, A.; Burch, J.L.; Giles, B.L.; Khotyaintsev, Y.; Contel, O.L.; et al. Observations of turbulence in a Kelvin–Helmholtz event on 8 September 2015 by the Magnetospheric Multiscale mission. J. Geophys. Res. 2016, 121, 11021–11034. [Google Scholar] [CrossRef]
- Chaston, C.C.; Wilber, M.; Mozer, F.S.; Fujimoto, M.; Goldstein, M.L.; Acuna, M.; Réme, H.; Fazakerley, A. Mode Conversion and Anomalous Transport in Kelvin–Helmholtz Vortices and Kinetic Alfvén Waves at the Earth’s Magnetopause. Phys. Rev. Lett. 2007, 99, 175004. [Google Scholar] [CrossRef] [PubMed]
- Chaston, C.C.; Bonnell, J.W.; Clausen, L.; Angelopoulos, V. Energy transport by kinetic-scale electromagnetic waves in fast plasma sheet flows. J. Geophys. Res. 2012, 117, A09202. [Google Scholar] [CrossRef]
- Hapgood, M.A. Space physics coordinate transformations-A user guide. Planet Space Sci. 1992, 40, 711–717. [Google Scholar] [CrossRef]
- Russell, C.T. Geophysical Coordinate Transformations. Cosmic Electrodyn. 1971, 2, 184–196. [Google Scholar]
- Pope, S. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Gutynska, O.; Šafránková, J.; Němeček, Z. Correlation length of magnetosheath fluctuations: Cluster statistics. Ann. Geophys. 2008, 26, 2503–2513. [Google Scholar] [CrossRef][Green Version]
- Leamon, R.J.; Smith, C.W.; Ness, N.F.; Matthaeus, W.H.; Wong, H.K. Observational constraints on the dynamics of the interplanetary magnetic field dissipation range. J. Geophys. Res. 1998, 103, 4775–4787. [Google Scholar] [CrossRef]
- Sahraoui, F.; Belmont, G.; Rezeau, L.; Cornilleau-Wehrlin, N.; Pinçon, J.L.; Balogh, A. Anisotropic Turbulent Spectra in the Terrestrial Magnetosheath as Seen by the Cluster Spacecraft. Phys. Rev. Lett. 2006, 96, 075002. [Google Scholar] [CrossRef]
- Frisch, U. Turbulence. The Legacy of A. N. Kolmogorov; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Sorriso-Valvo, L.; Carbone, V.; Veltri, P.; Consolini, G.; Bruno, R. Intermittency in the solar wind turbulence through probability distribution functions of fluctuations. Geophys. Res. Lett. 1999, 26, 1801–1804. [Google Scholar] [CrossRef]
- Bruno, R.; Carbone, V. The Solar Wind as a Turbulence Laboratory. Living Rev. Sol. Phys. 2005, 2, 4. [Google Scholar] [CrossRef]
- Sreenivasan, K.R.; Antonia, R.A. The phenomenology of small-scale turbulence. Ann. Rev. Fluid Mech. 1997, 29, 435–472. [Google Scholar] [CrossRef]
- Anselmet, F.; Gagne, Y.; Hopfinger, E.J.; Antonia, R.A. High-order velocity structure functions in turbulent shear flows. J. Fluid Mech. 1984, 140, 63–89. [Google Scholar] [CrossRef]
- Chhiber, R.; Chasapis, A.; Bandyopadhyay, R.; Parashar, T.N.; Matthaeus, W.H.; Maruca, B.A.; Moore, T.E.; Burch, J.L.; Torbert, R.B.; Russell, C.T.; et al. Higher-order turbulence statistics in the Earth’s magnetosheath and the solar wind using Magnetospheric Multiscale observations. J. Geophys. Res. 2018, 123, 9941–9954. [Google Scholar] [CrossRef]
- Book, D.L.; Sibeck, D.G. Plasma transport through the magnetopause turbulent interchange processes. J. Geophys. Res. 1995, 100, 9567–9573. [Google Scholar] [CrossRef]
- Labelle, J.; Treumann, R.A. Plasma waves at the dayside magnetopause. Space Sci. Rev. 1988, 47, 175–202. [Google Scholar] [CrossRef]
- Bavassano Cattaneo, M.B.; Marcucci, M.F.; Retinò, A.; Pallocchia, G.; Réme, H.; Dandouras, I.; Kistler, L.M.; Klecker, B.; Carlson, C.W.; Korth, A.; et al. Kinetic signatures during a quasi-continuous lobe reconnection event: Cluster Ion Spectrometer (CIS) observations. J. Geophys. Res. 2006, 111, A09212. [Google Scholar] [CrossRef]
- Frey, H.U.; Phan, T.D.; Fuselier, S.A.; Mende, S.B. Continuous magnetic reconnection at Earth’s magnetopause. Nature 2003, 426, 533–537. [Google Scholar] [CrossRef]
- Phan, T.D.; Dunlop, M.W.; Paschmann, G.; Klecker, B.; Bosqued, J.M.; Réme, H.; Balogh, A.; Twitty, C.; Mozer, F.S.; Carlson, C.W.; et al. Cluster observations of continuous reconnection at the magnetopause under steady interplanetary magnetic field conditions. Ann. Geophys. 2004, 22, 2355–2367. [Google Scholar] [CrossRef]
- Retinò, A.; Bavassano Cattaneo, M.B.; Marcucci, M.F.; Vaivads, A.; André, M.; Khotyaintsev, Y.; Phan, T.; Pallocchia, G.; Réme, H.; Möbius, E.; et al. Cluster multispacecraft observations at the high-latitude duskside magnetopause: Implications for continuous and component magnetic reconnection. Ann. Geophys. 2005, 23, 461–473. [Google Scholar]
- Song, P.; Russell, C.T. A model of the formation of the low-latitude boundary layer. J. Geophys. Res. 1992, 97, 1411. [Google Scholar] [CrossRef]
- Breuillard, H.; Yordanova, E.; Vaivads, A.; Alexandrova, O. The effects of kinetic instabilities on small-scale turbulence in Earth’s magnetosheath. Astrophys. J. 2016, 829, 54–60. [Google Scholar] [CrossRef]
- Zimbardo, G.; Greco, A.; Sorriso-Valvo, L.; Perri, S.; Vöros, Z.; Aburjania, G.; Chargazia, K.; Alexandrova, O. Magnetic turbulence in the geospace environment. Space Sci. Rev. 2010, 156, 89–134. [Google Scholar] [CrossRef]
- Alexandrova, O.; Chen, C.H.K.; Sorriso-Valvo, L.; Horbury, T.S.; Bale, S.D. Solar Wind Turbulence and the Role of Ion Instabilities. Space Sci. Rev. 2013, 178, 101–139. [Google Scholar] [CrossRef]
- Rossi, C.; Califano, F.; Retinò, A.; Sorriso-Valvo, L.; Henri, P.; Servidio, S.; Valentini, F.; Chasapis, A.; Rezeau, L. Two-fluid numerical simulations of turbulence inside Kelvin–Helmholtz vortices: Intermittency and reconnecting current sheets. Phys. Plasmas 2015, 22, 122303. [Google Scholar] [CrossRef]

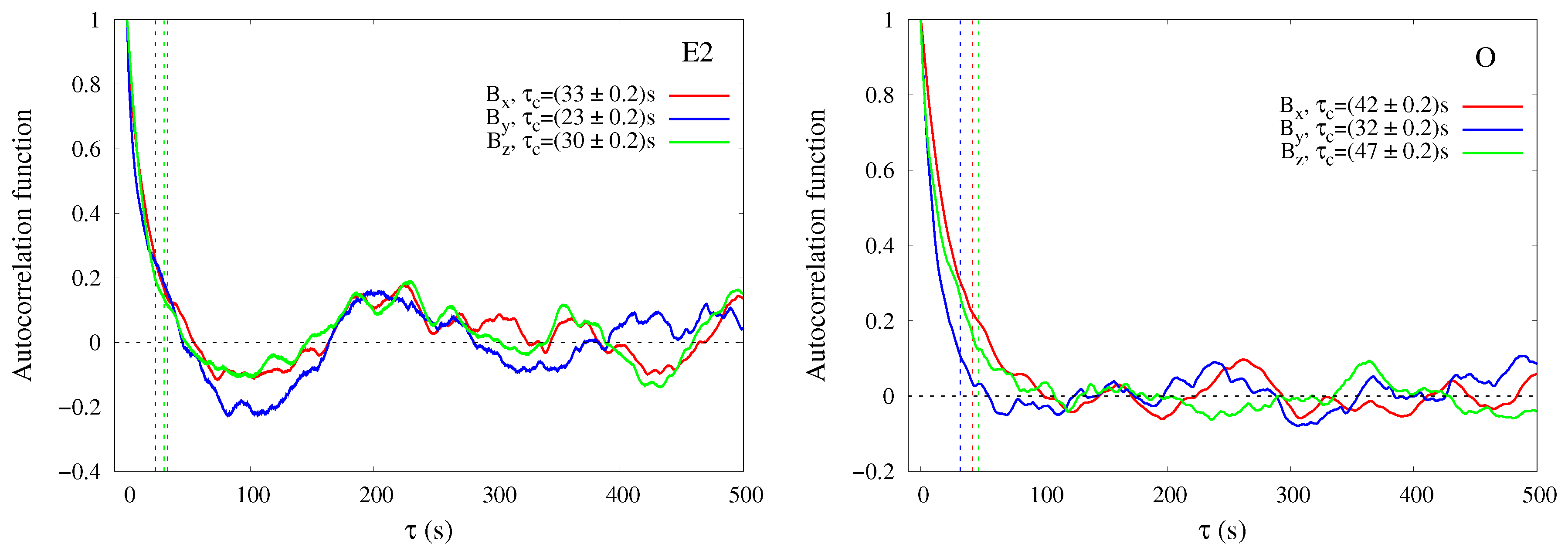
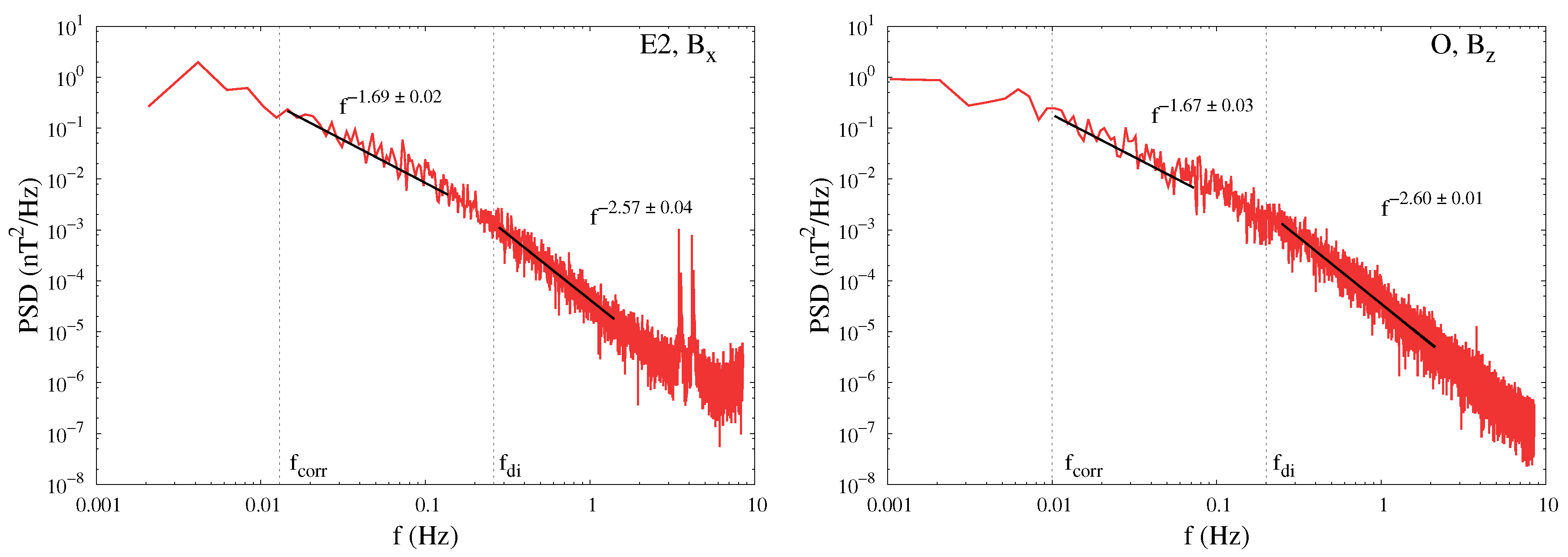
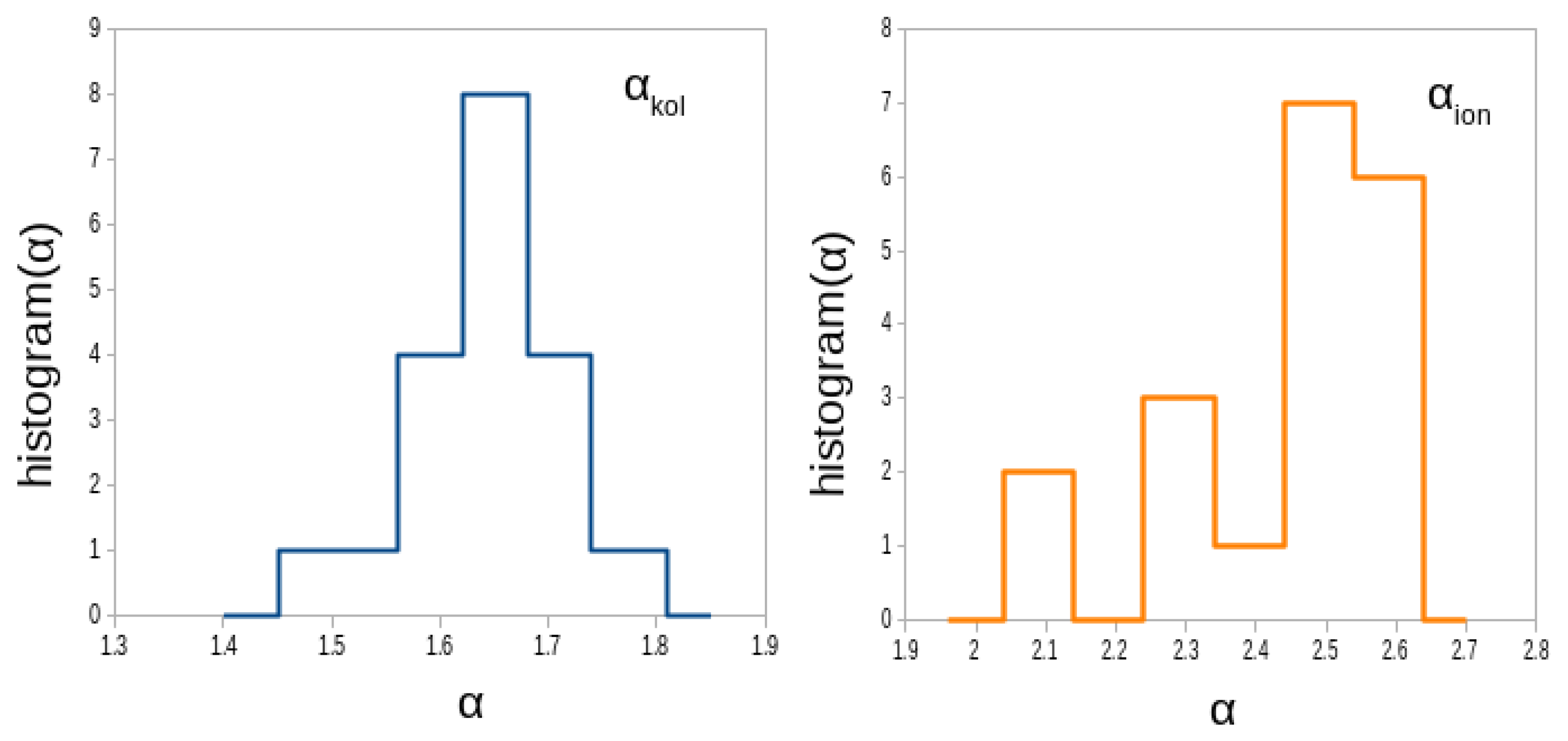


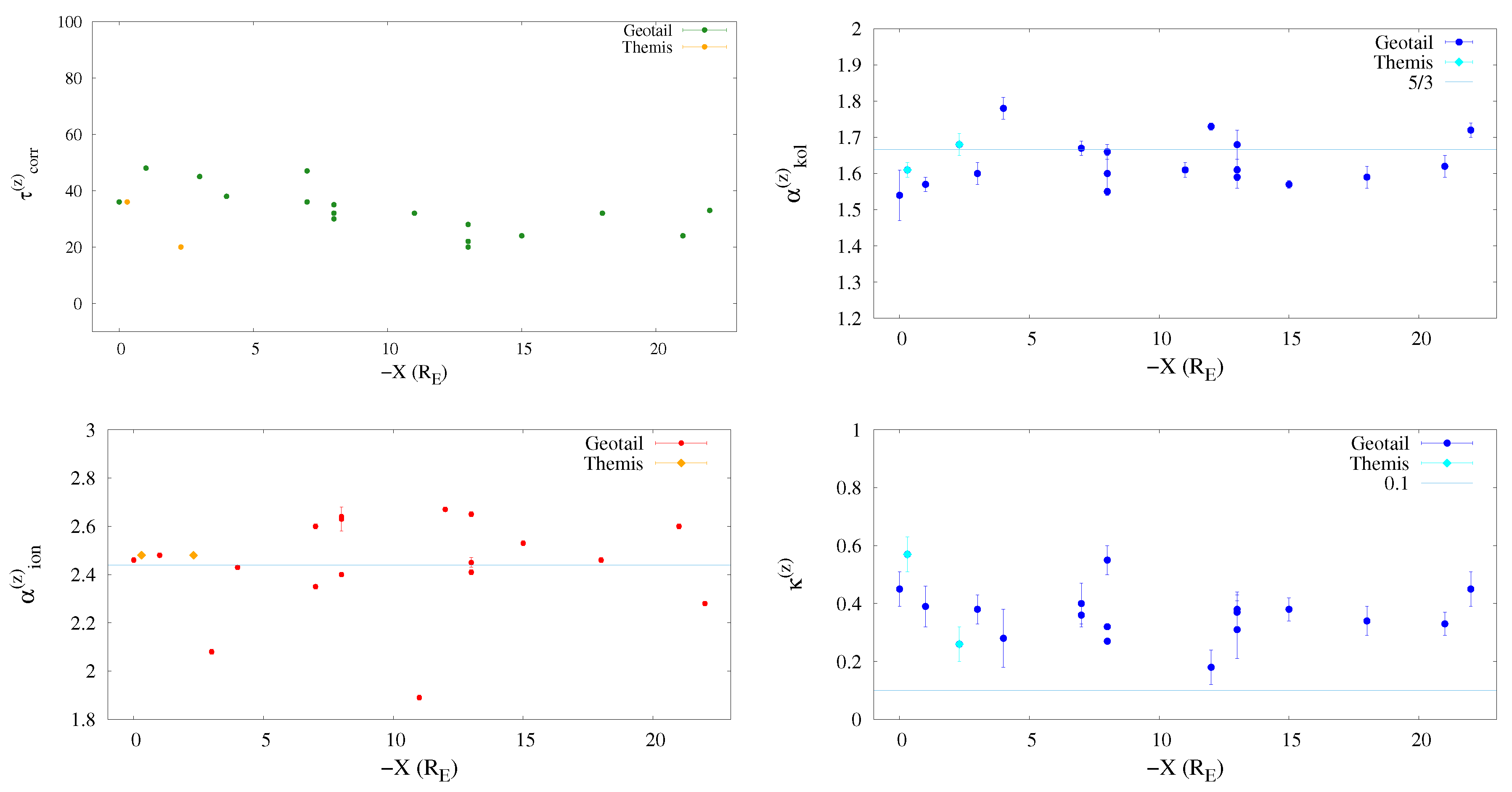
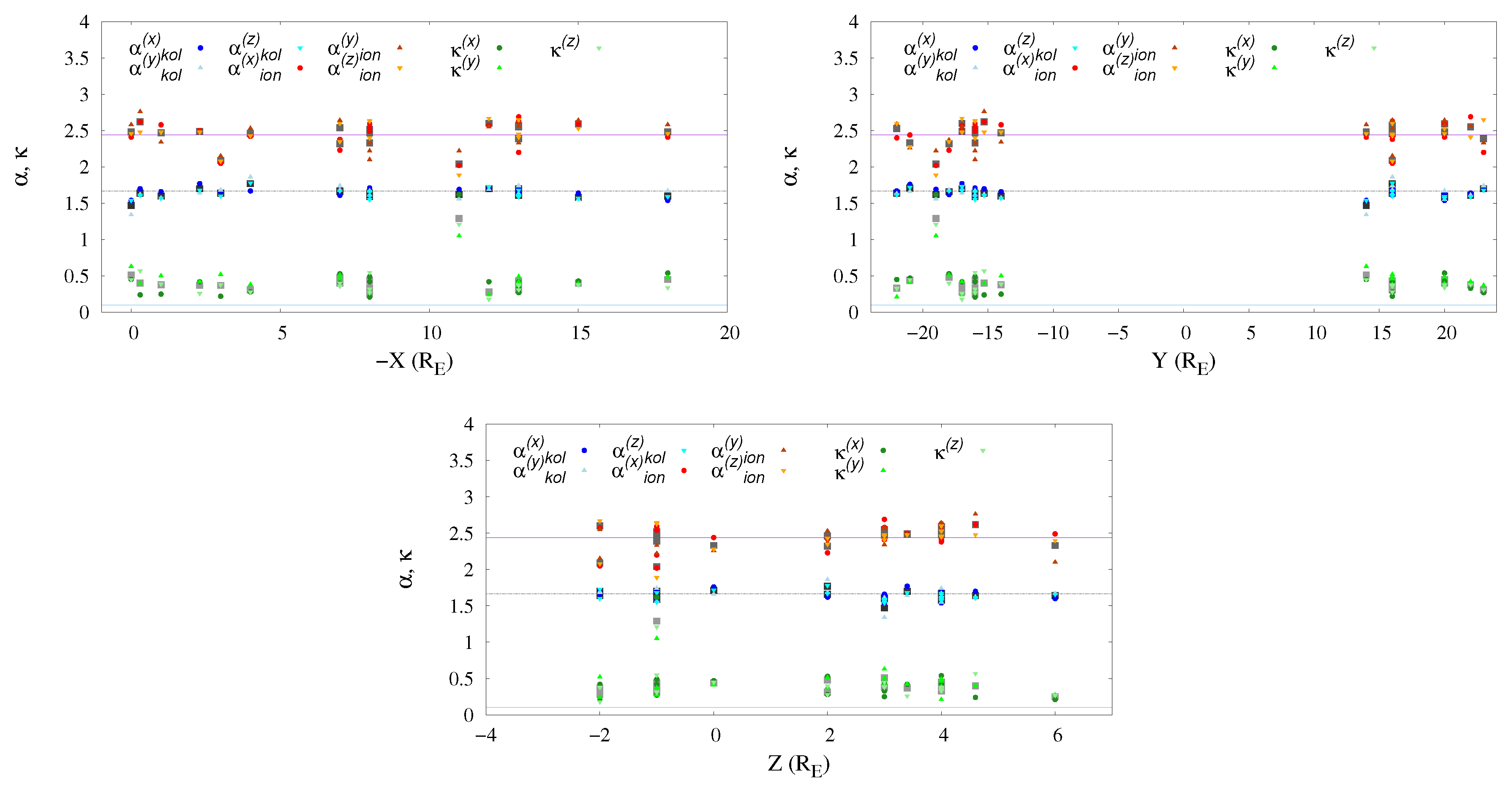

| GEOTAIL MISSION | |||||||
| Event | Interval | GSM Position | IMF Condition | Mixing Status | Period | ||
| S | 1995-03-24 0600-0800 | (−15, 20, 4) | ES1 NBZ2 | Mixed | 2–3 | 227 | 5.3 |
| O | 1997-01-10 2050-2400 | (−7, 16, 4) | ND3 NBZ after N-t4 | Weakly mixed | 2–3 | 169 | 4.0 |
| P | 1997-01-11 0400-0500 | (−13, 16, 4) | ES NBZ | Mixed | ∼ 3 | 219 | 6.2 |
| Q | 1997-02-12 1430-1600 | (−13, 22, 3) | ES NBZ | Mixed | ∼ 2 | 281 | 5.3 |
| T | 1998-04-13 0315-0430 | (−18, 20, 4) | ES NBZ | Mixed | 2–3 | 312 | 7.3 |
| L | 1998-08-01 0530-0730 | ( 0, 14, −3) | ES NBZ | Mixed | ∼ 3 | 92 | 2.6 |
| D | 1998-12-27 1800-2100 | (−21, −22, −4) | ES NBZ | Mixed | 3–4 | 247 | 8.1 |
| N | 1999-02-15 1445-1515 | ( −4, 16, 2) | NBZ after N-t | Mixed | ∼ 2 | 279 | 5.3 |
| M | 1999-07-20 0630-0730 | ( −3, 16, −2) | ES NBZ | Mixed | 2–3 | 230 | 5.4 |
| E | 2000-11-01 1030-1200 | ( −8, −16, 6) | ES NBZ | Mixed | 2-3 | 298 | 7.0 |
| H | 2001-01-25 1330-1630 | (−22, −21, 0) | ES NBZ | Mixed | ∼ 5 | 182 | 8.6 |
| B | 2001-11-16 1900-2000 | (−7, −18, 2) | Extended NBZ (often ND) | Weakly mixed | 2–3 | 183 | 4.3 |
| C | 2001-12-07 2000-2130 | (−11, −19, −1) | SNBZ5 after N-t | Weakly mixed | ∼ 3 | 257 | 7.3 |
| E2 | 2002-03-25 0530-0900 | (−12, −17, −2) | ES NBZ | Mixed | 2–3 | 299 | 7.0 |
| F | 2002-03-25 1000-1300 | (−8, −16, −1) | ES NBZ | Mixed | 2–3 | 299, 347 | 7.0, 8.2 |
| A | 2002-10-15 2100-2300 | (−1, −14, 3) | Extended NBZ | Mixed | 2–3 | 332 | 7.8 |
| R | 2003-07-17 0330-0500 | (−13, 23, −1) | ND NBZ after N-t | Weakly mixed | ∼ 2 | 419 | 7.9 |
| THEMIS MISSION | |||||||
| TH1 | 2008-11-06 0850-0920 | (−0.3, −15.3, 4.6) | ES NBZ | Mixed | ∼ 2 | 288 | 3.3 |
| TH2 | 2008-11-18 0720-0730 | (−2.3, −17, 3.4) | ES NBZ | Mixed | ∼ 3 | 348 | 3.5 |
| (km/s) | (km/s) | (deg) | |
|---|---|---|---|
| A | 547 | 332 | 104,34 |
| B | 315 | 183 | 80,83 |
| C | 345 | 257 | 117,32 |
| D | 204 | 247 | 68,41 |
| E | 268 | 298 | 99,1 |
| E2 | 400 | 299 | 60,69 |
| F | 344 | 347 | 76,15 |
| H | 182 | 182 | 65,2 |
| L | 286 | 92 | 72,58 |
| M | 200 | 230 | 92,84 |
| N | 234 | 279 | 85,41 |
| O | 232 | 169 | 71,98 |
| P | 268 | 219 | 64,57 |
| Q | 181 | 281 | 90,8 |
| R | 260 | 419 | 110,54 |
| S | 173 | 227 | 99,84 |
| T | 456 | 312 | 55,97 |
| TH1 | 219 | 288 | 72,76 |
| TH2 | 307 | 348 | 139,23 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Mare, F.; Sorriso-Valvo, L.; Retinò, A.; Malara, F.; Hasegawa, H. Evolution of Turbulence in the Kelvin–Helmholtz Instability in the Terrestrial Magnetopause. Atmosphere 2019, 10, 561. https://doi.org/10.3390/atmos10090561
Di Mare F, Sorriso-Valvo L, Retinò A, Malara F, Hasegawa H. Evolution of Turbulence in the Kelvin–Helmholtz Instability in the Terrestrial Magnetopause. Atmosphere. 2019; 10(9):561. https://doi.org/10.3390/atmos10090561
Chicago/Turabian StyleDi Mare, Francesca, Luca Sorriso-Valvo, Alessandro Retinò, Francesco Malara, and Hiroshi Hasegawa. 2019. "Evolution of Turbulence in the Kelvin–Helmholtz Instability in the Terrestrial Magnetopause" Atmosphere 10, no. 9: 561. https://doi.org/10.3390/atmos10090561
APA StyleDi Mare, F., Sorriso-Valvo, L., Retinò, A., Malara, F., & Hasegawa, H. (2019). Evolution of Turbulence in the Kelvin–Helmholtz Instability in the Terrestrial Magnetopause. Atmosphere, 10(9), 561. https://doi.org/10.3390/atmos10090561







