The Role of Background Wind and Moisture in the Atmospheric Response to Oceanic Eddies During Winter in the Kuroshio Extension Region
Abstract
1. Introduction
2. Model Configuration
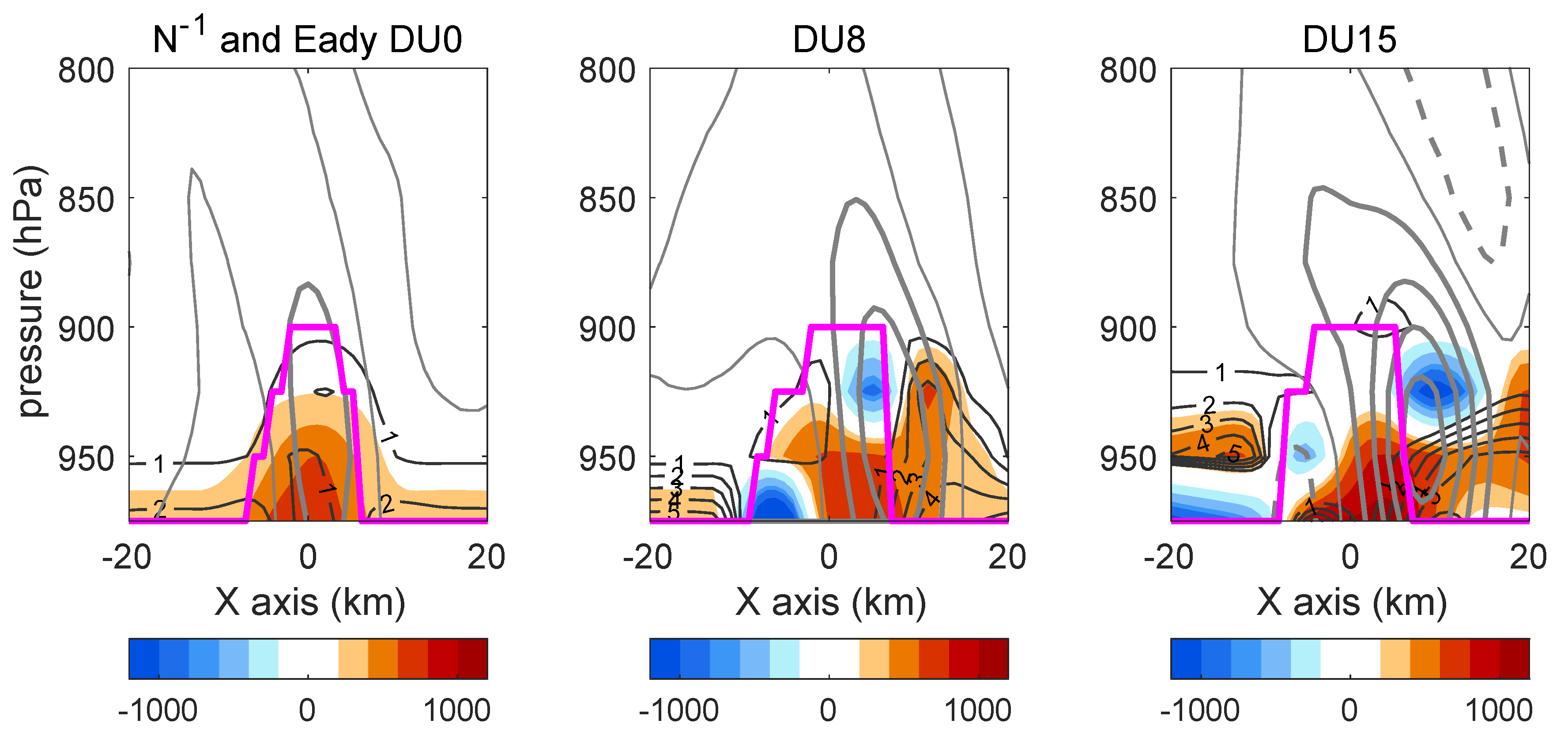
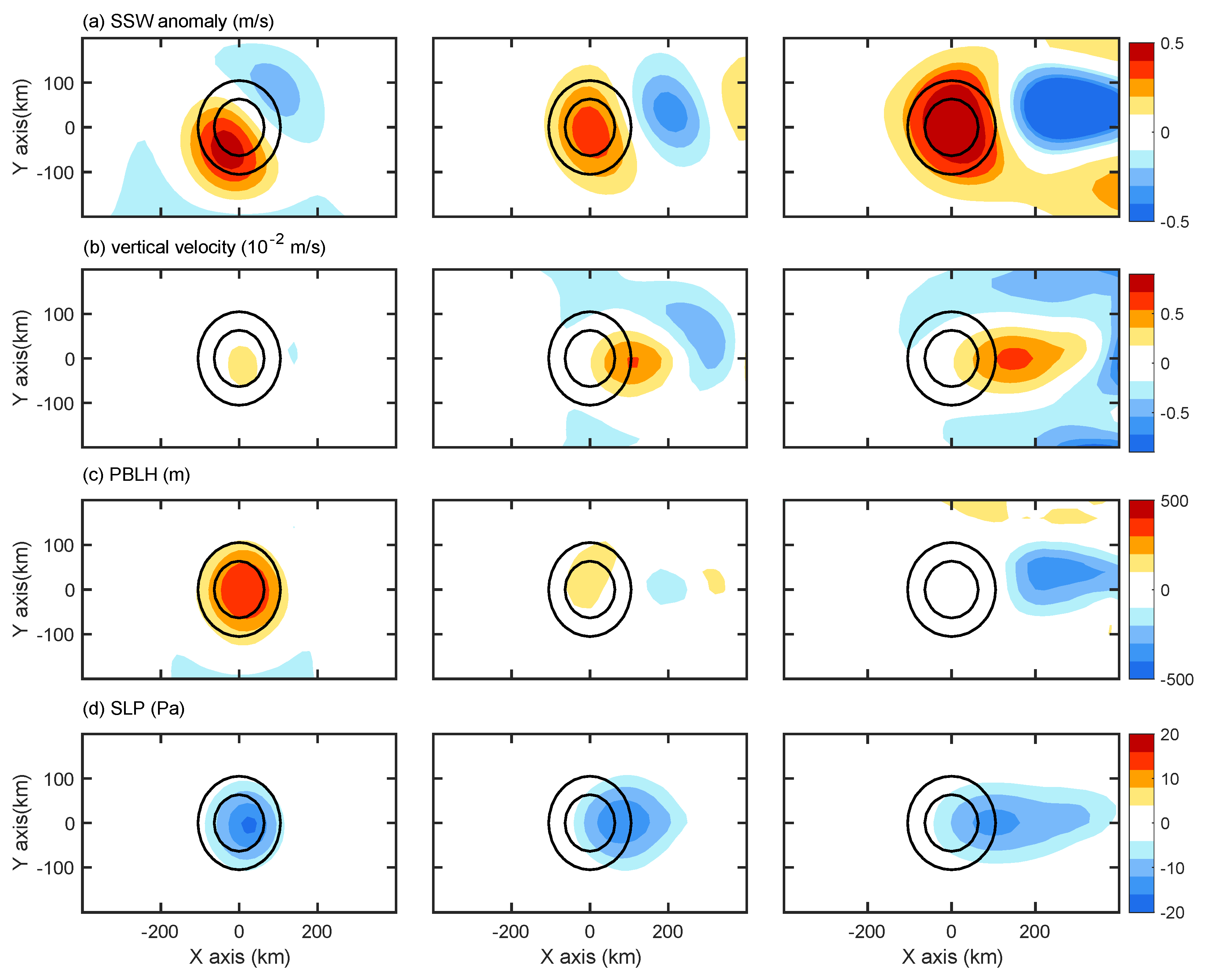
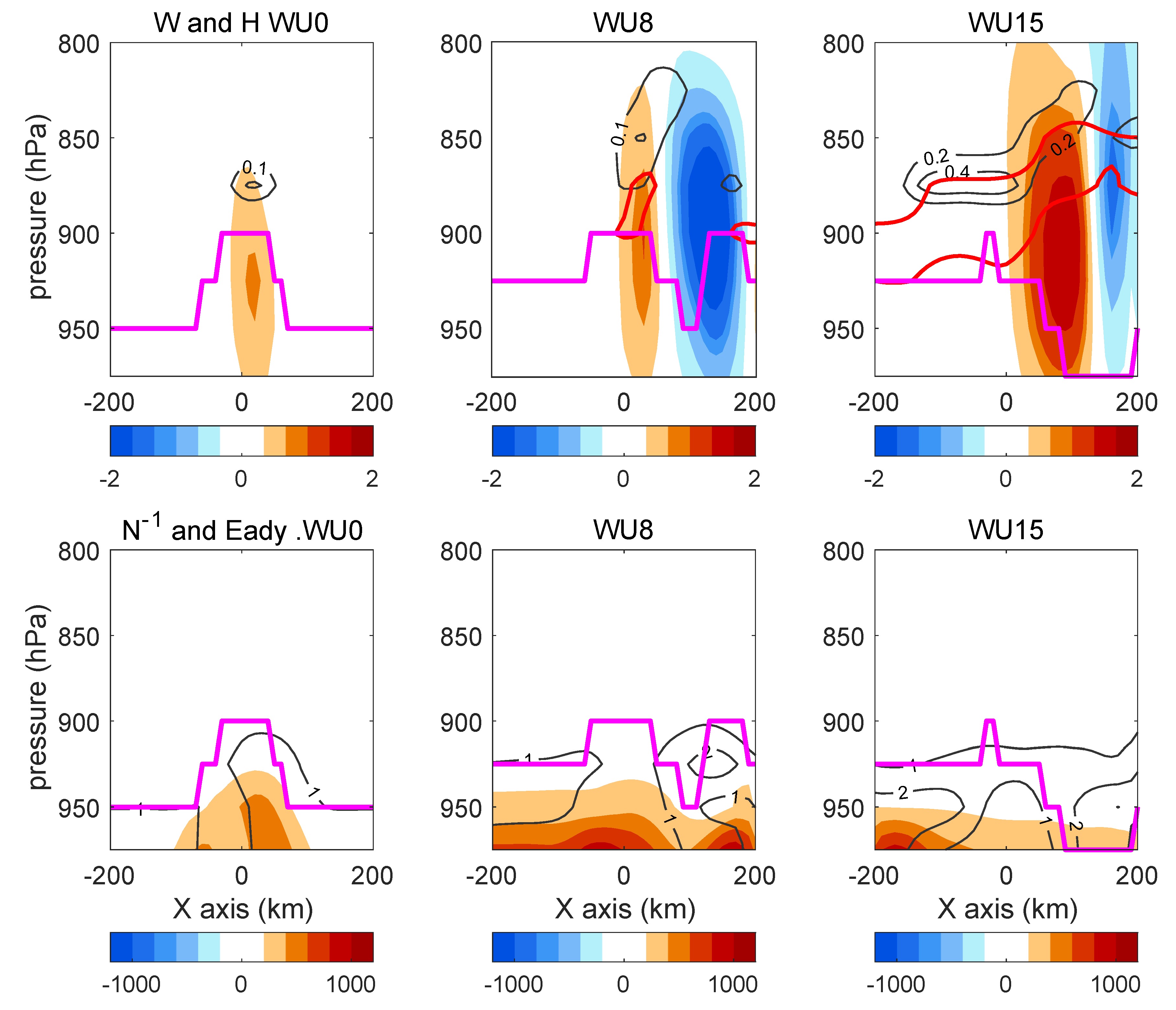
3. Effect of Background Wind
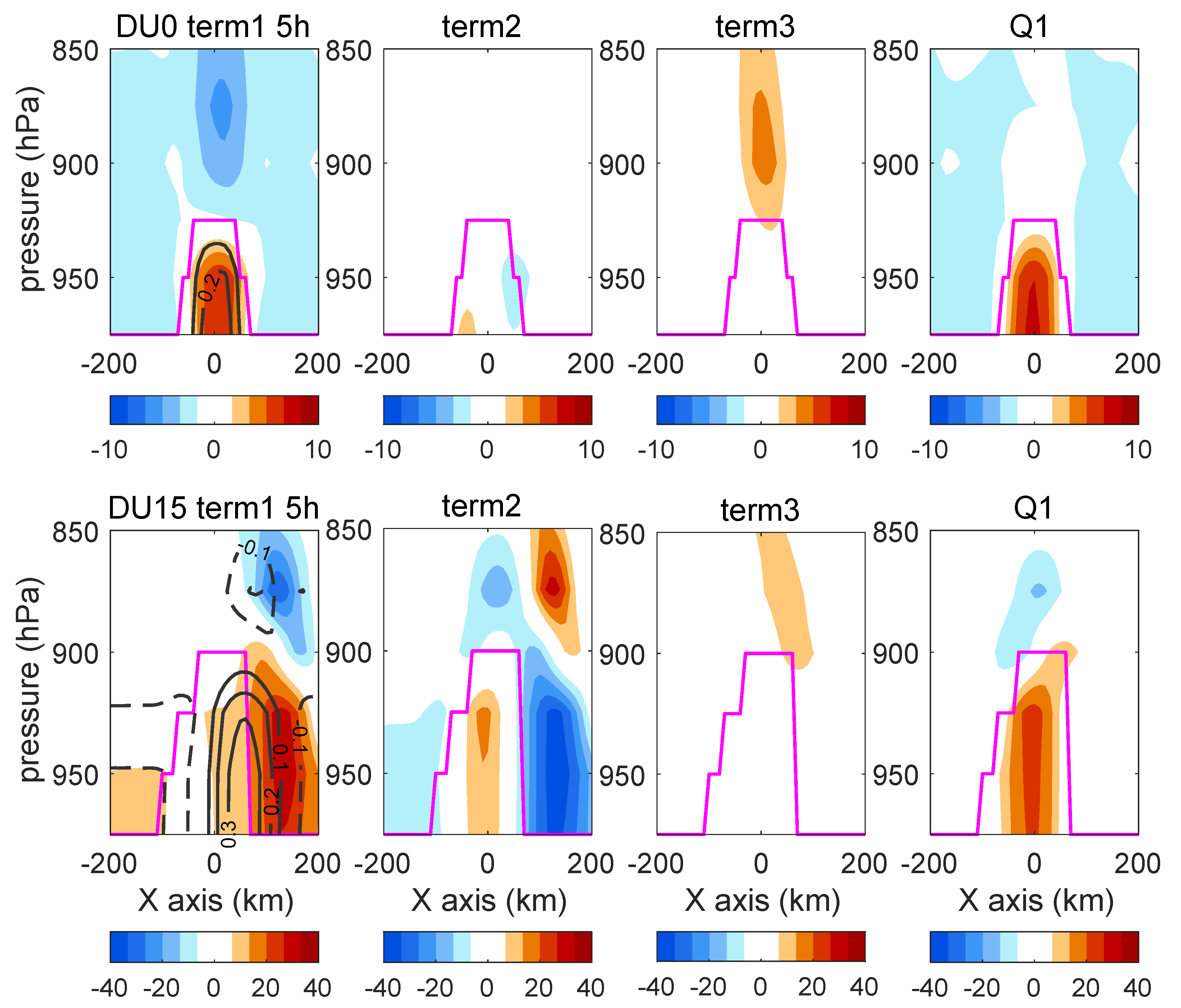
4. Effects of Moisture and Diabatic Heating
4.1. Comparison with the Dry Run
4.2. Vertical Transport of Moisture
4.3. Suppressed Vertical Mixing in the Wet Run
5. Conclusions and Discussions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Park, K.; Cornillon, P.; Codiga, D. Modification of surface winds near ocean fronts: Effects of Gulf Stream rings on scatterometer (QuikSCAT, NSCAT) wind observations. J. Geophys. Res. 2006, 111, c03021. [Google Scholar] [CrossRef]
- Small, R.J.; deSzoeke, S.P.; Xie, S.P.; O’Neill, L.; Seo, H.; Song, Q.; Cornillon, P.; Spall, M.; Minobe, S. Air-sea interaction over ocean fronts and eddies. Dyn. Atmos. Oceans 2008, 45, 274–319. [Google Scholar] [CrossRef]
- Chelton, D.B.; Xie, S.P. Coupled ocean–atmosphere interaction at oceanic mesoscales. Oceanography 2010, 23, 52–69. [Google Scholar] [CrossRef]
- Frenger, I.; Gruber, N.; Knutti, R.; Münnich, M. Imprint of Southern Ocean eddies on winds, clouds and rainfall. Nat. Geosci. 2013, 6, 608–612. [Google Scholar] [CrossRef]
- Ma, J.; Xu, H.; Dong, C.; Lin, P.; Liu, Y. Atmospheric responses to oceanic eddies in the Kuroshio Extension region. J. Geophys. Res. 2015, 120, 6313–6330. [Google Scholar] [CrossRef]
- Sugimoto, S.; Aono, K.; Fukui, S. Local atmospheric response to warm mesoscale ocean eddies in the Kuroshio–Oyashio Confluence region. Sci. Rep. 2017, 7, 11871. [Google Scholar] [CrossRef] [PubMed]
- Chen, L.J.; Jia, Y.L.; Liu, Q.Y. Oceanic eddy-driven atmospheric secondary circulation in the winter Kuroshio Extension region. J. Oceanogr. 2017, 73, 295–307. [Google Scholar] [CrossRef]
- Wallace, J.M.; Mitchell, T.P.; Deser, C. The influence of sea surface temperature on surface wind in the eastern equatorial Pacific: Seasonal and interannual variability. J. Clim. 1989, 2, 1492–1499. [Google Scholar] [CrossRef]
- Xie, S.P. Satellite observations of cool ocean-atmosphere interaction. Bull. Am. Meteorol. Soc. 2004, 85, 195–208. [Google Scholar] [CrossRef]
- Kilpatrick, T.; Schneider, N.; Qiu, B. Atmospheric response to a midlatitude SST front: Alongfront winds. J. Atmos. Sci. 2016, 73, 3489–3509. [Google Scholar] [CrossRef]
- Schneider, N.; Qiu, B. The atmospheric response to weak sea surface temperature fronts. J. Atmos. Sci. 2015, 72, 3356–3377. [Google Scholar] [CrossRef]
- Spall, M.A. Midlatitude wind stress-sea surface temperature coupling in the vicinity of oceanic fronts. J. Clim. 2007, 20, 3785–3801. [Google Scholar] [CrossRef]
- Lindzen, R.S.; Nigam, S. On the role of sea surface temperature gradients in forcing lowlevel winds and convergence in the tropics. J. Atmos. Sci. 1987, 44, 2418–2436. [Google Scholar] [CrossRef]
- Lambaerts, J.; Lapeyre, G.; Plougonven, R.; Klein, P. Atmospheric response to sea surface temperature mesoscale structures. J. Geophys. Res. Atmos. 2013, 118, 9611–9621. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, W.T. Observational evidence of frontal-scale atmospheric responses to Kuroshio Extension variability. J. Clim. 2015, 28, 9459–9472. [Google Scholar] [CrossRef]
- Shan, H.; Dong, C. Atmospheric responses to oceanic mesoscale eddies based on an idealized model. Int. J. Climatol. 2019, 39, 1665–1683. [Google Scholar] [CrossRef]
- Ma, X.H.; Chang, P.; Saravanan, R.; Montuoro, R.; Hsies, J.; Wu, D.X.; Lin, X.P.; Wu, L.X.; Zhao, J. Distant Influence of Kuroshio Eddies on North Pacific Weather Patterns? Sci. Rep. 2015, 5, 17785. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.H.; Chang, P.; Saravanan, R.; Montuoro, R.; Nakamura, H.; Wu, D.X.; Lin, X.P.; Wu, L.X. Importance of Resolving Kuroshio Front and Eddy Influence in Simulating North Pacific Storm Track. J. Clim. 2017, 30, 1861–1880. [Google Scholar] [CrossRef]
- Skamarock, W.; Klemp, J.B.; Dudhia, J.; Gill, D.O.; Barker, D.M.; Huang, X.Y.; Wang, W.; Powers, J.G. A Description of the Advanced Research WRF Version 3, 3rd ed.; National Center for Atmospheric Research: Boulder, CO, USA, 2008; p. 88. [Google Scholar]
- Hong, S.Y.; Dudhia, J.; Chen, S.H. A Revised Approach to Ice Microphysical Processes for the Bulk Parameterization of Clouds and Precipitation. Mon. Weather Rev. 2004, 132, 103–120. [Google Scholar] [CrossRef]
- Hong, S.Y.; Lim, J.O.J. The WRF Single-Moment 6-Class Microphysics Scheme (WSM6). Asia Pac. J. Atmos. Sci. 2006, 42, 129–151. [Google Scholar]
- Kain, J.S. The Kain-Fritsch convective parameterization: An update. J. Appl. Meteorol. 2004, 43, 170–181. [Google Scholar] [CrossRef]
- Hong, S.Y.; Noh, Y.; Dudhia, J. A new vertical diffusion package with an explicit treatment of entrainment processes. Mon. Weather Rev. 2006, 134, 2318–2341. [Google Scholar] [CrossRef]
- Mlawer, E.J.; Taubman, S.J.; Brown, P.D.; Iacono, M.J.; Clough, S.A. Radiative transfer for inhomogeneous atmosphere: RRTM, a validated correlated-k model for the longwave. J. Geophys. Res. 1997, 102, 16663–16682. [Google Scholar] [CrossRef]
- Dudhia, J. Numerical study of convection observed during the winter monsoon experiment using a mesoscale two-dimensional model. J. Atmos. Sci. 1989, 46, 3077–3107. [Google Scholar] [CrossRef]
- Yanai, M.; Esbensen, S.; Chu, J.H. Determination of bulk properties of tropical cloud clusters from large-scale heat and moisture budgets. J. Atmos. Sci. 1973, 30, 611–627. [Google Scholar] [CrossRef]
- Yanai, M.; Tomita, T. Seasonal and interannual variability of atmospheric heat sources and moisture sinks as determined from NCEP-NCAR reanalysis. J. Clim. 1998, 11, 463–482. [Google Scholar] [CrossRef]
- Kobashi, F.; Xie, S.P.; Iwasaka, N.; Sakamoto, T.T. Deep atmospheric response to the North Pacific oceanic subtropical front in spring. J. Clim. 2008, 21, 5960–5975. [Google Scholar] [CrossRef]
- Shaman, J.; Samelson, R.; Skyllingstad, E. Air-sea fluxes over the Gulf Stream region: Atmospheric controls and trends. J. Clim. 2010, 23, 2651–2670. [Google Scholar] [CrossRef]
- Song, Q.; Cornillon, P.; Hara, T. Surface wind response to oceanic fronts. J. Geophys. Res. 2006, 111, C12006. [Google Scholar] [CrossRef]
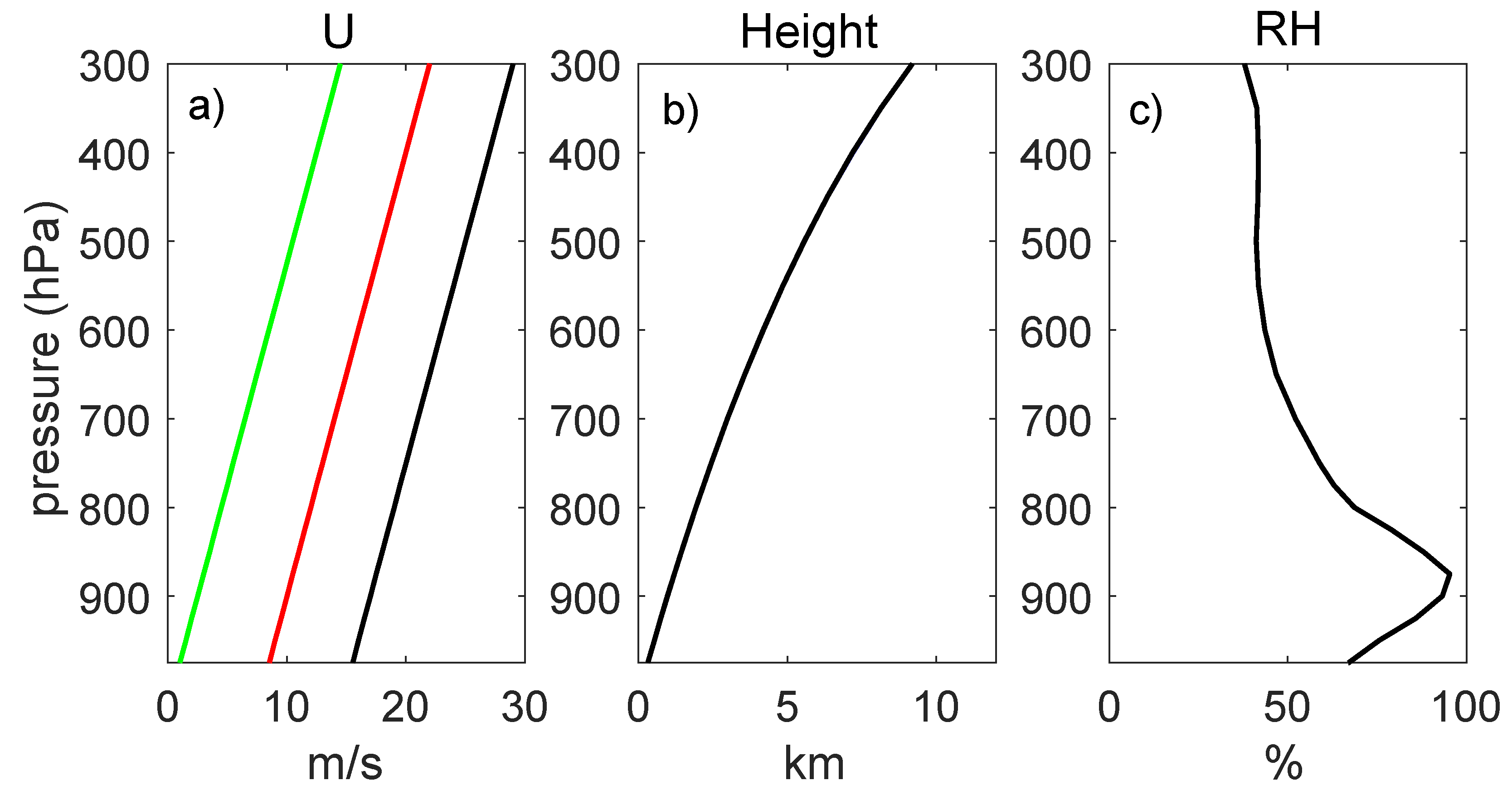
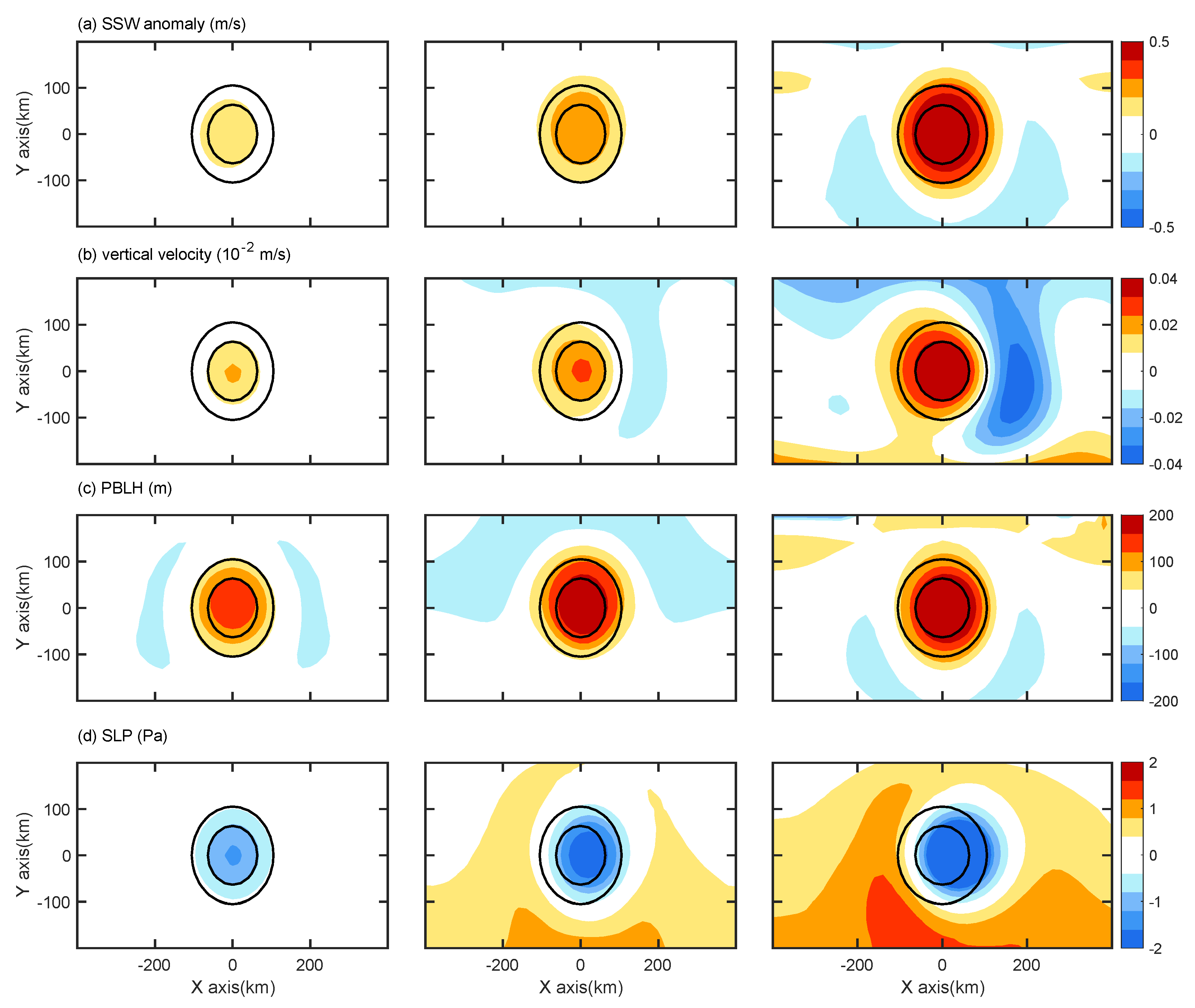
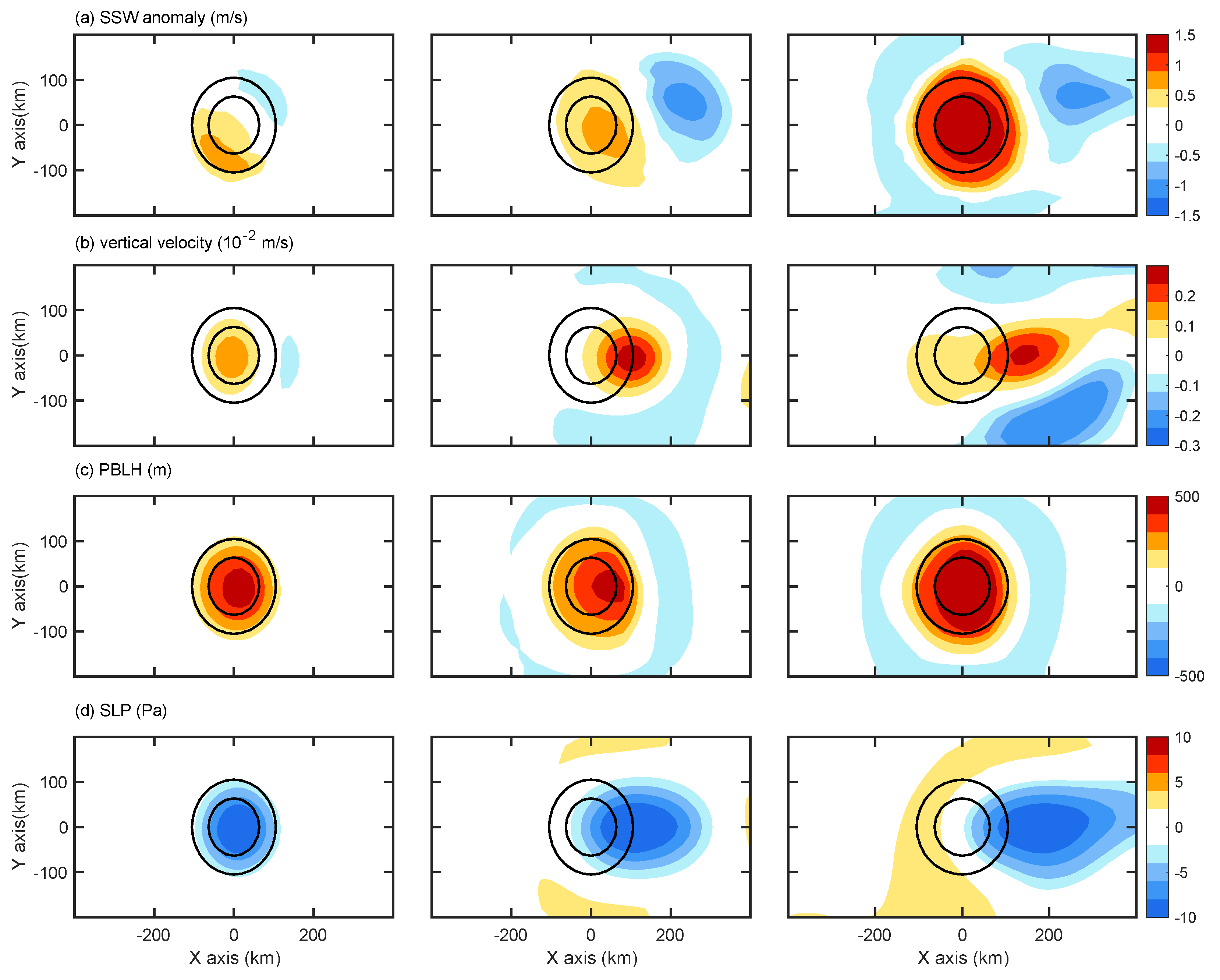
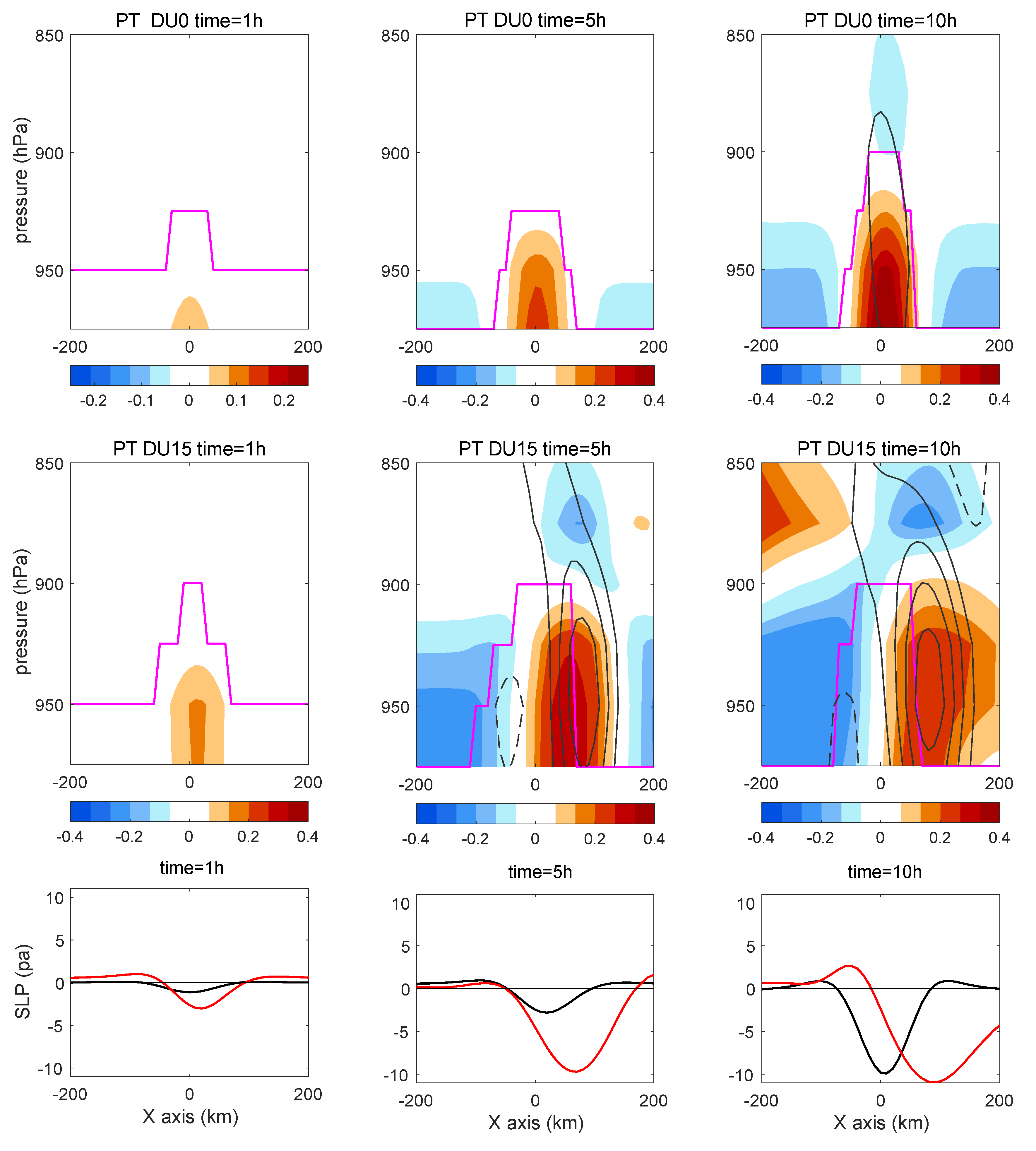
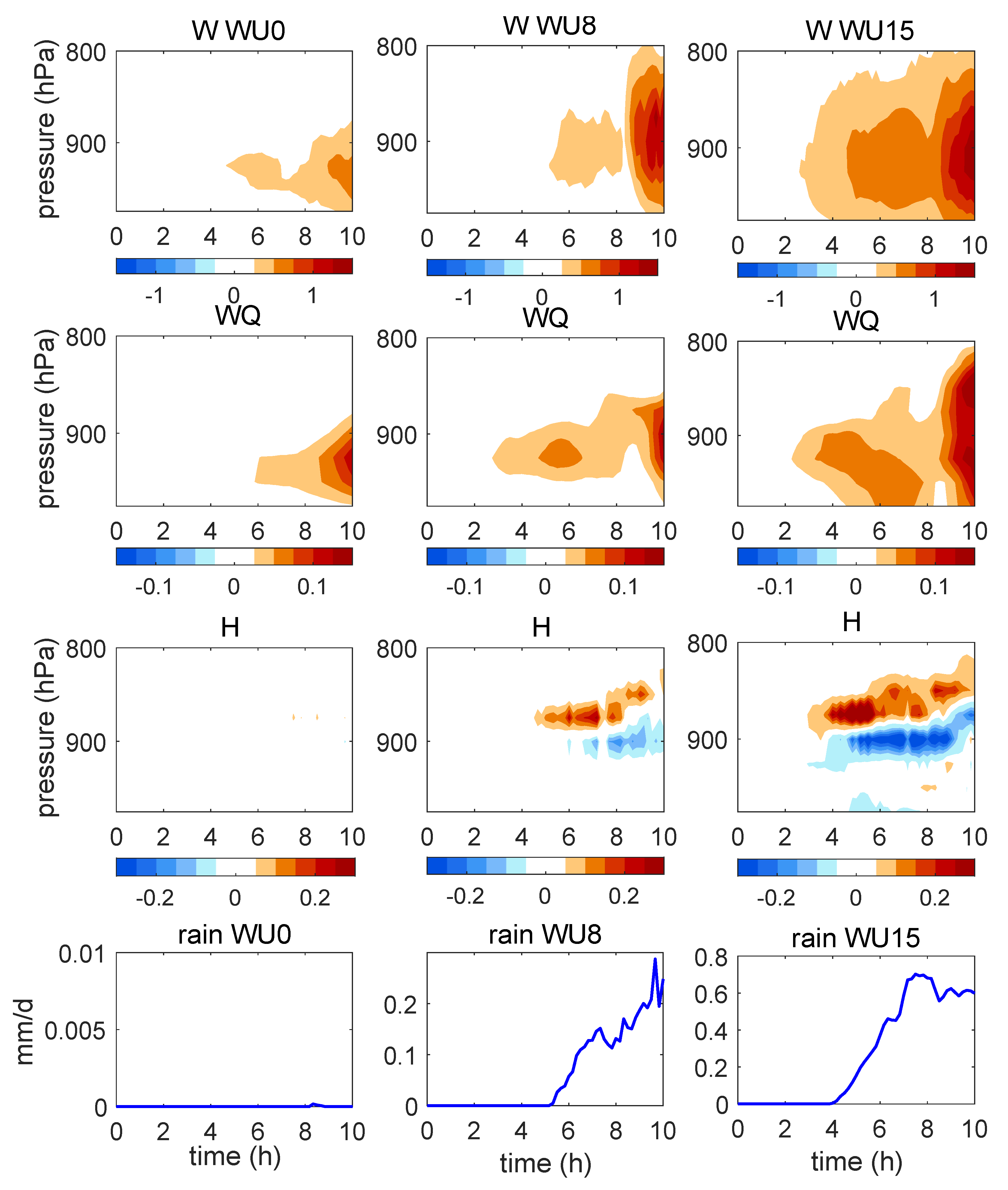
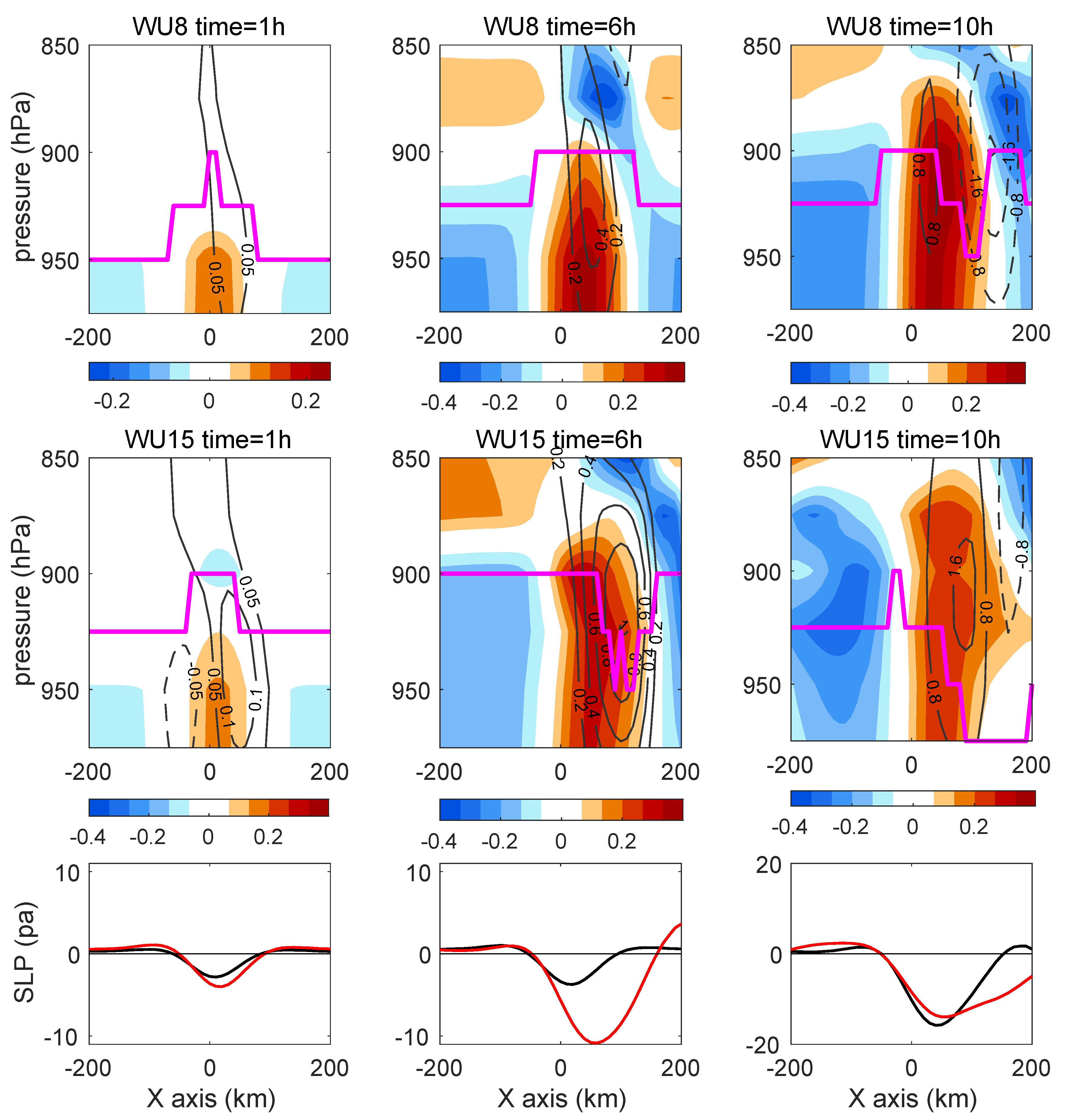
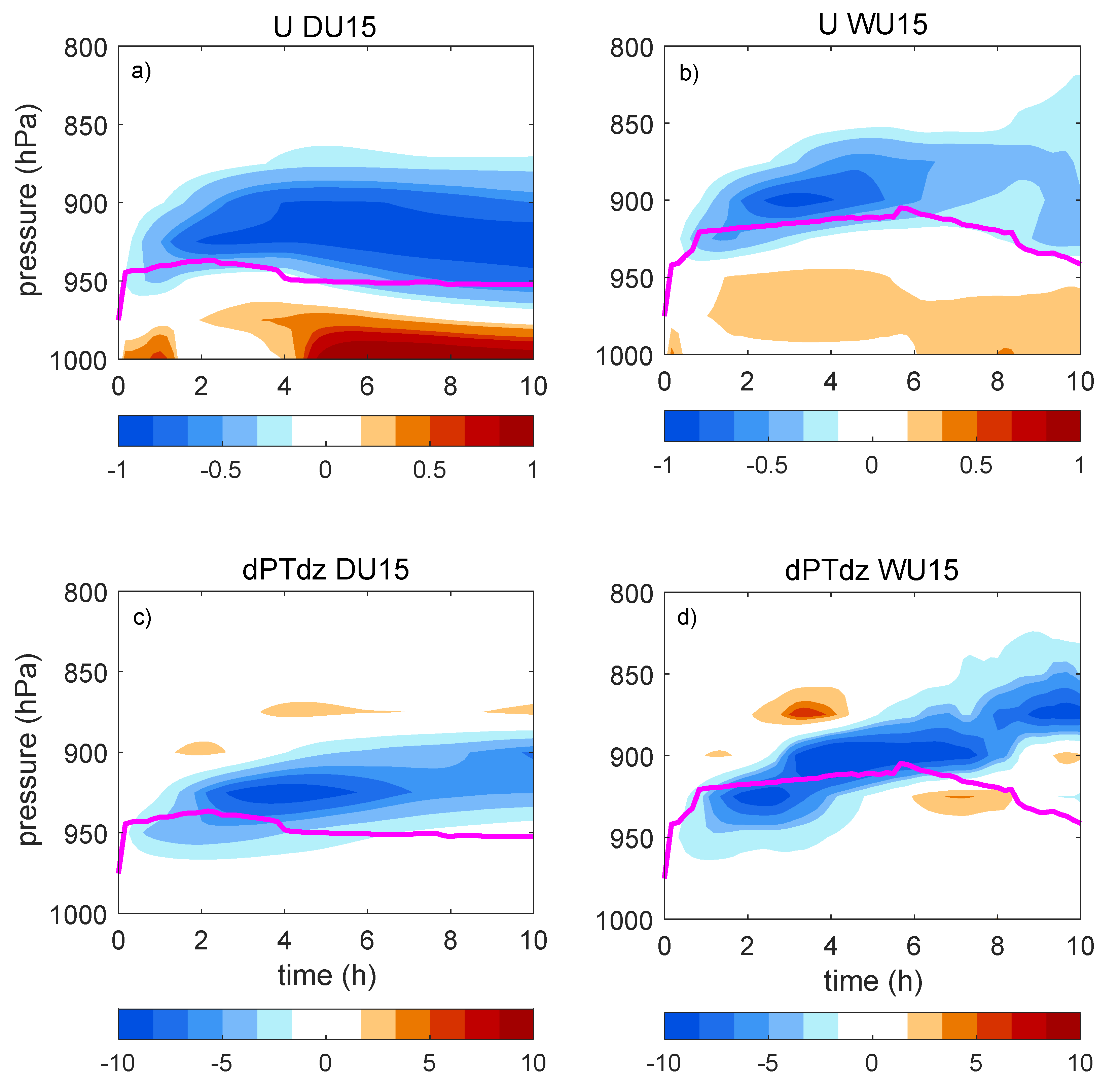
| Dry EXPs | Wet EXPs | Background Wind Speed (m/s) |
|---|---|---|
| DU0 | WU0 | 0.5 |
| DU8 | WU8 | 8 |
| DU15 | WU15 | 15 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, Y.; Chen, L.; Liu, Q.; Yang, X.; Wu, Y. The Role of Background Wind and Moisture in the Atmospheric Response to Oceanic Eddies During Winter in the Kuroshio Extension Region. Atmosphere 2019, 10, 527. https://doi.org/10.3390/atmos10090527
Jia Y, Chen L, Liu Q, Yang X, Wu Y. The Role of Background Wind and Moisture in the Atmospheric Response to Oceanic Eddies During Winter in the Kuroshio Extension Region. Atmosphere. 2019; 10(9):527. https://doi.org/10.3390/atmos10090527
Chicago/Turabian StyleJia, Yinglai, Longjing Chen, Qinyu Liu, Xiaohui Yang, and Yifei Wu. 2019. "The Role of Background Wind and Moisture in the Atmospheric Response to Oceanic Eddies During Winter in the Kuroshio Extension Region" Atmosphere 10, no. 9: 527. https://doi.org/10.3390/atmos10090527
APA StyleJia, Y., Chen, L., Liu, Q., Yang, X., & Wu, Y. (2019). The Role of Background Wind and Moisture in the Atmospheric Response to Oceanic Eddies During Winter in the Kuroshio Extension Region. Atmosphere, 10(9), 527. https://doi.org/10.3390/atmos10090527




