Influence of the Heights of Low-Level Jets on Power and Aerodynamic Loads of a Horizontal Axis Wind Turbine Rotor
Abstract
1. Introduction
2. Inflow Wind Fields
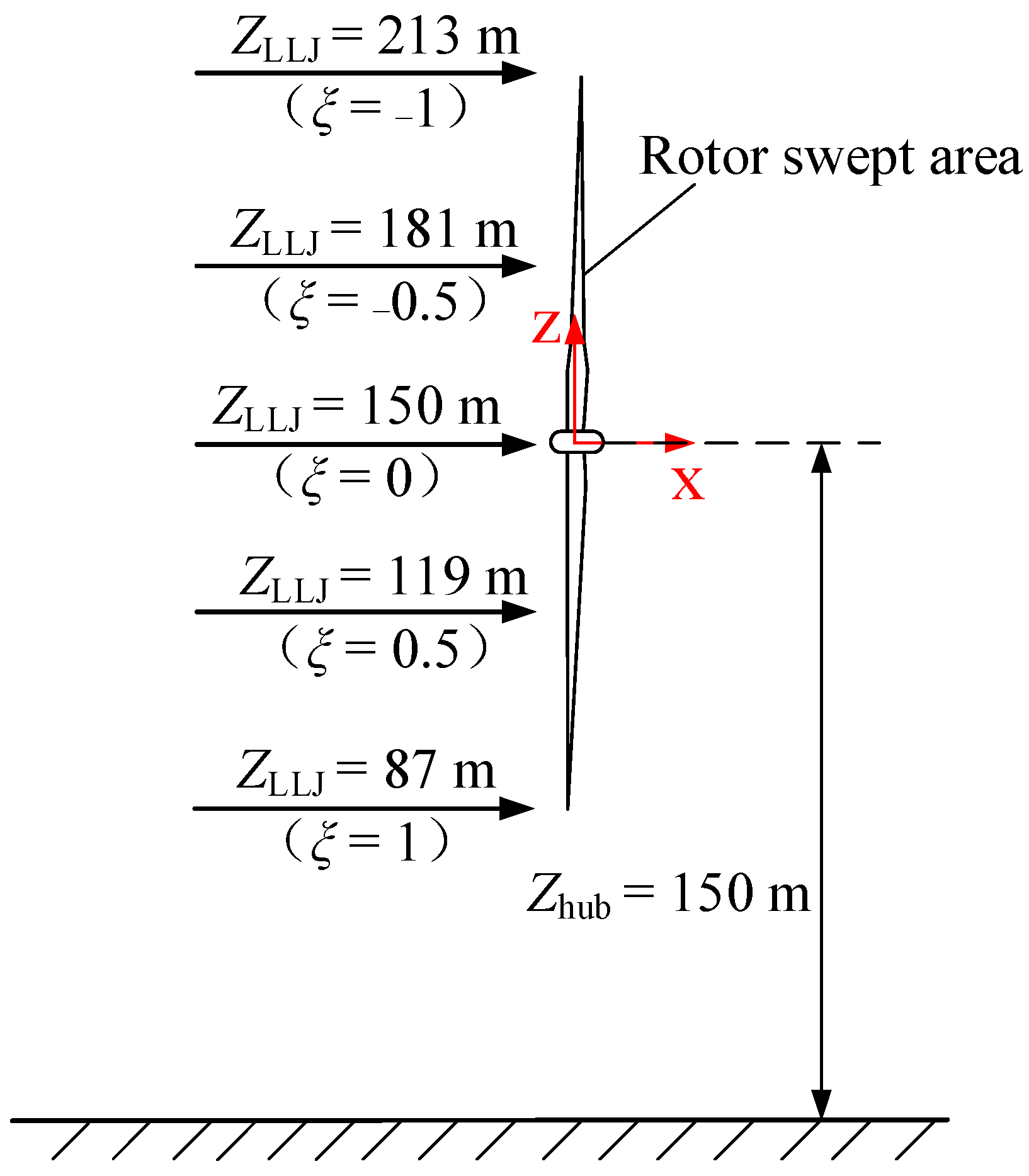
2.1. GP_LLJ Spectral Model
2.2. IEC Von Karman Isotropic Spectral Model
2.3. Simulation of Fluctuating Wind Fields
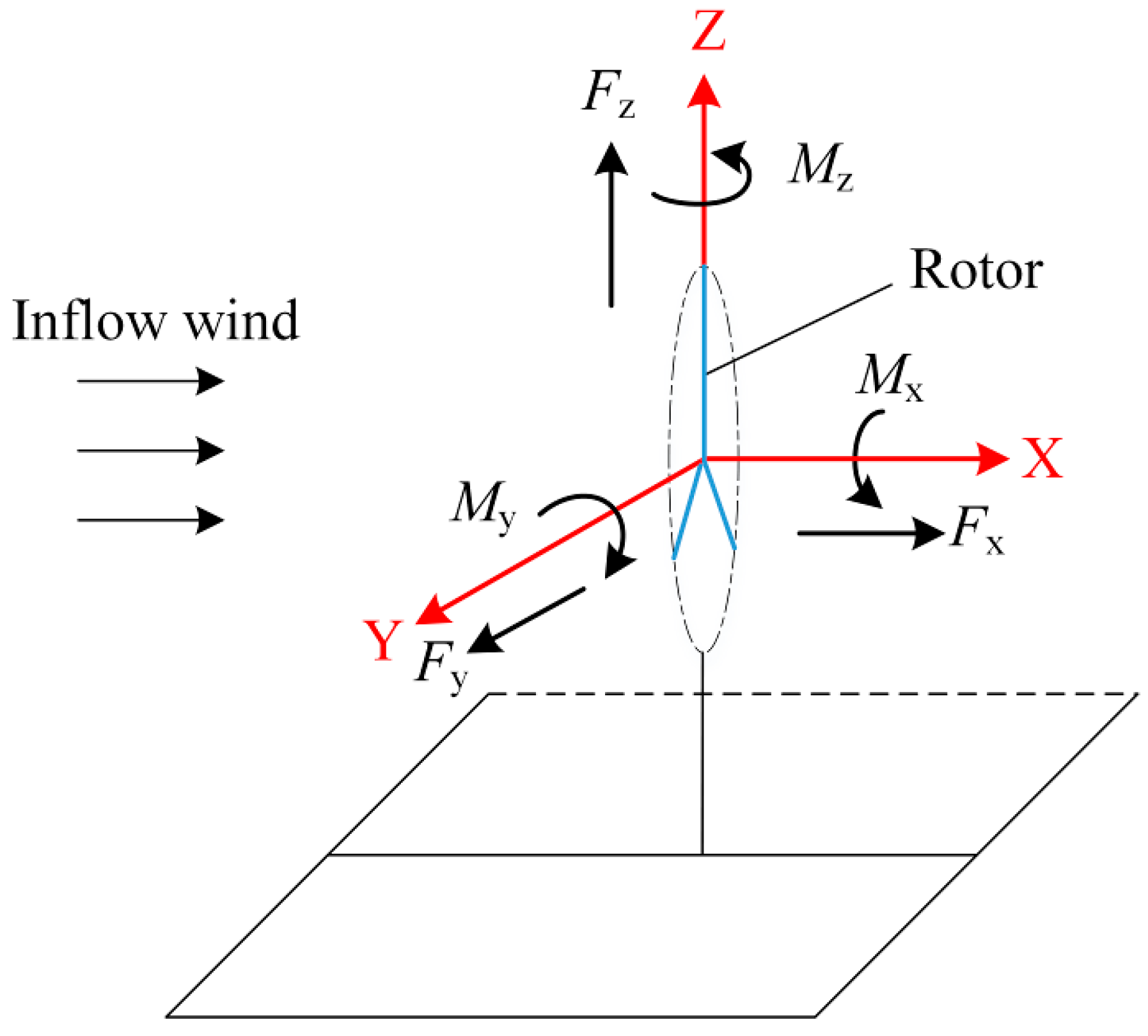
3. Wind Turbine Model and FAST
4. Validation of Calculation Model and Method in FAST
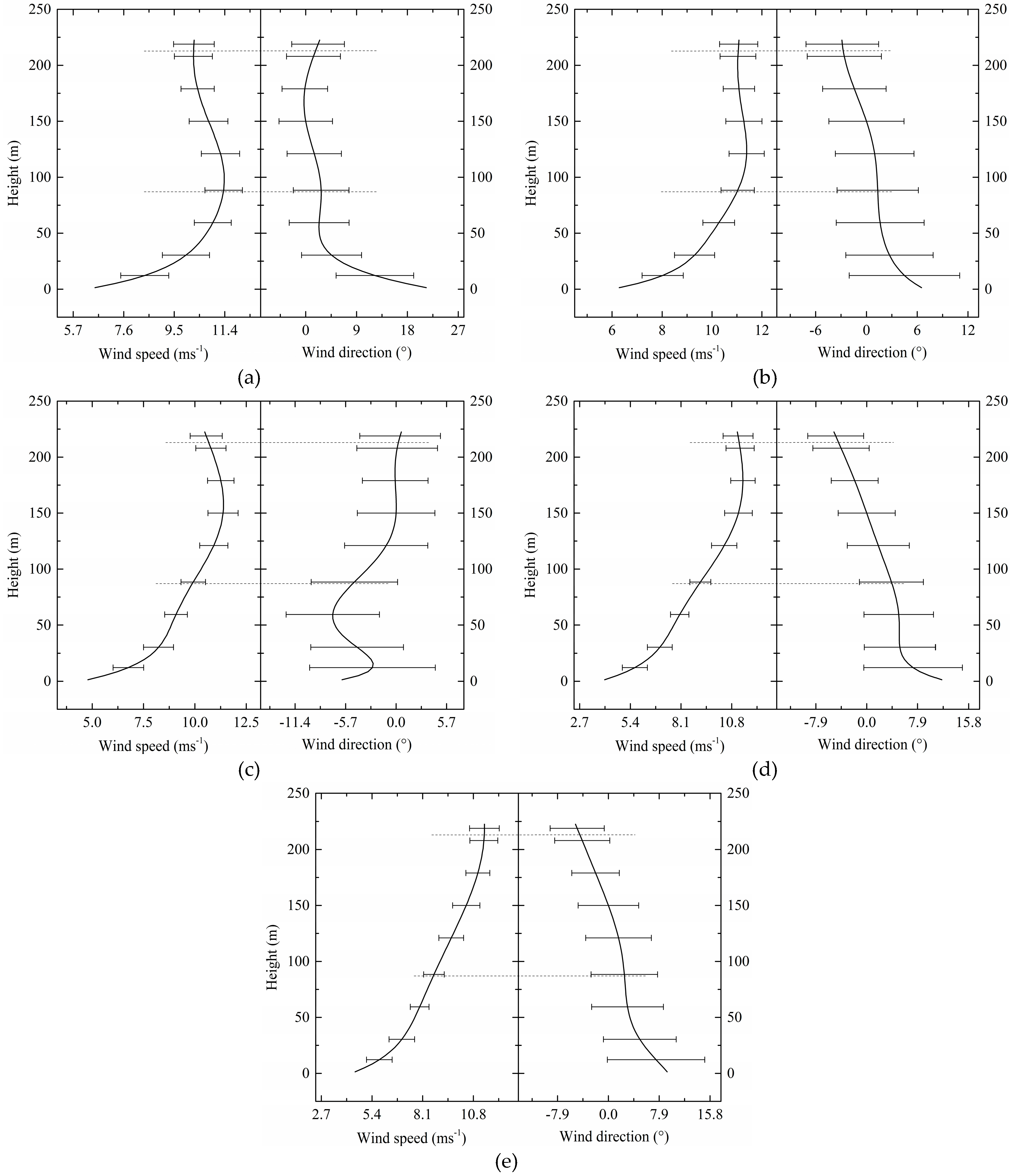
5. Case Introduction
6. Results and Discussion
6.1. Rotor Power
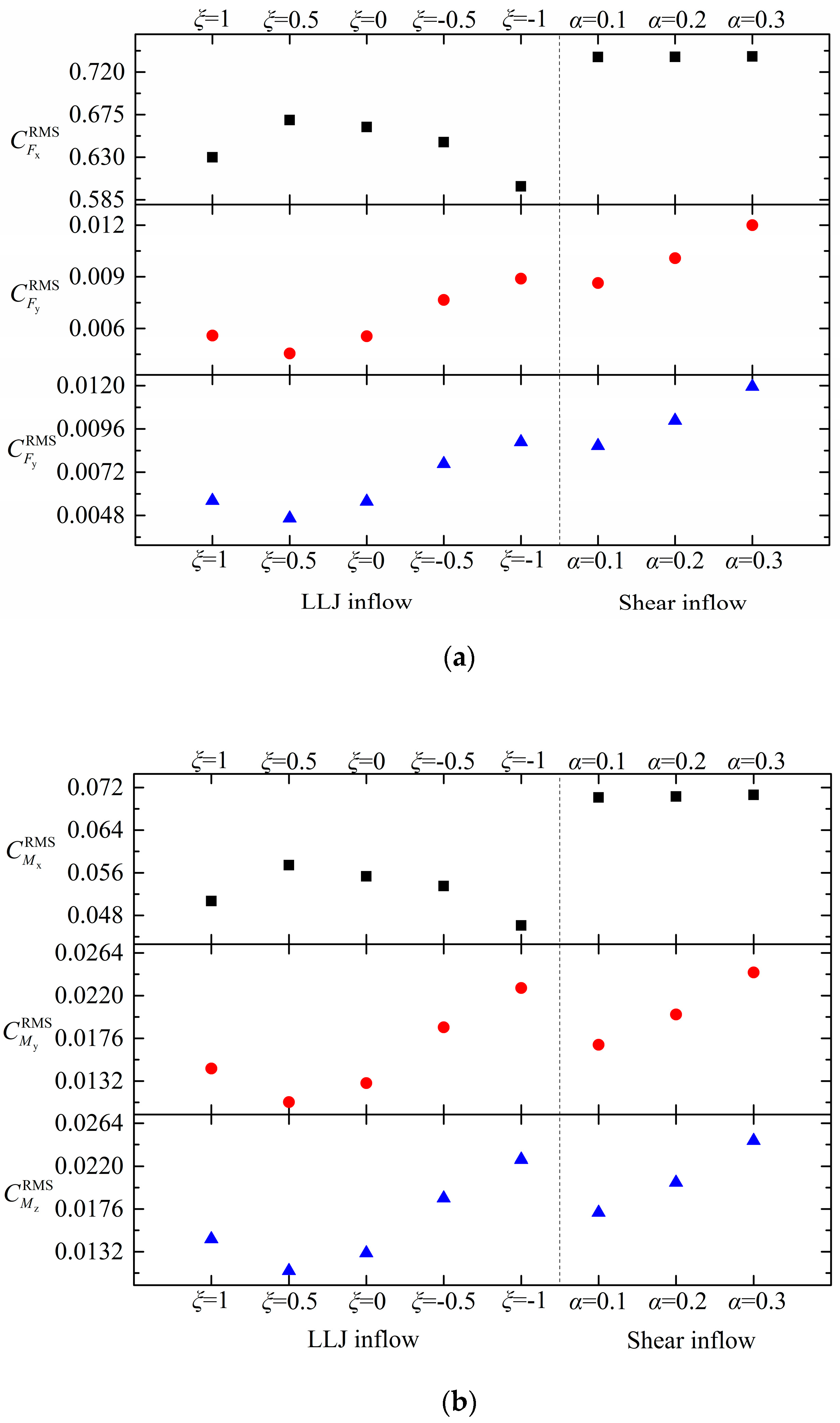
6.2. Aerodynamic Loads
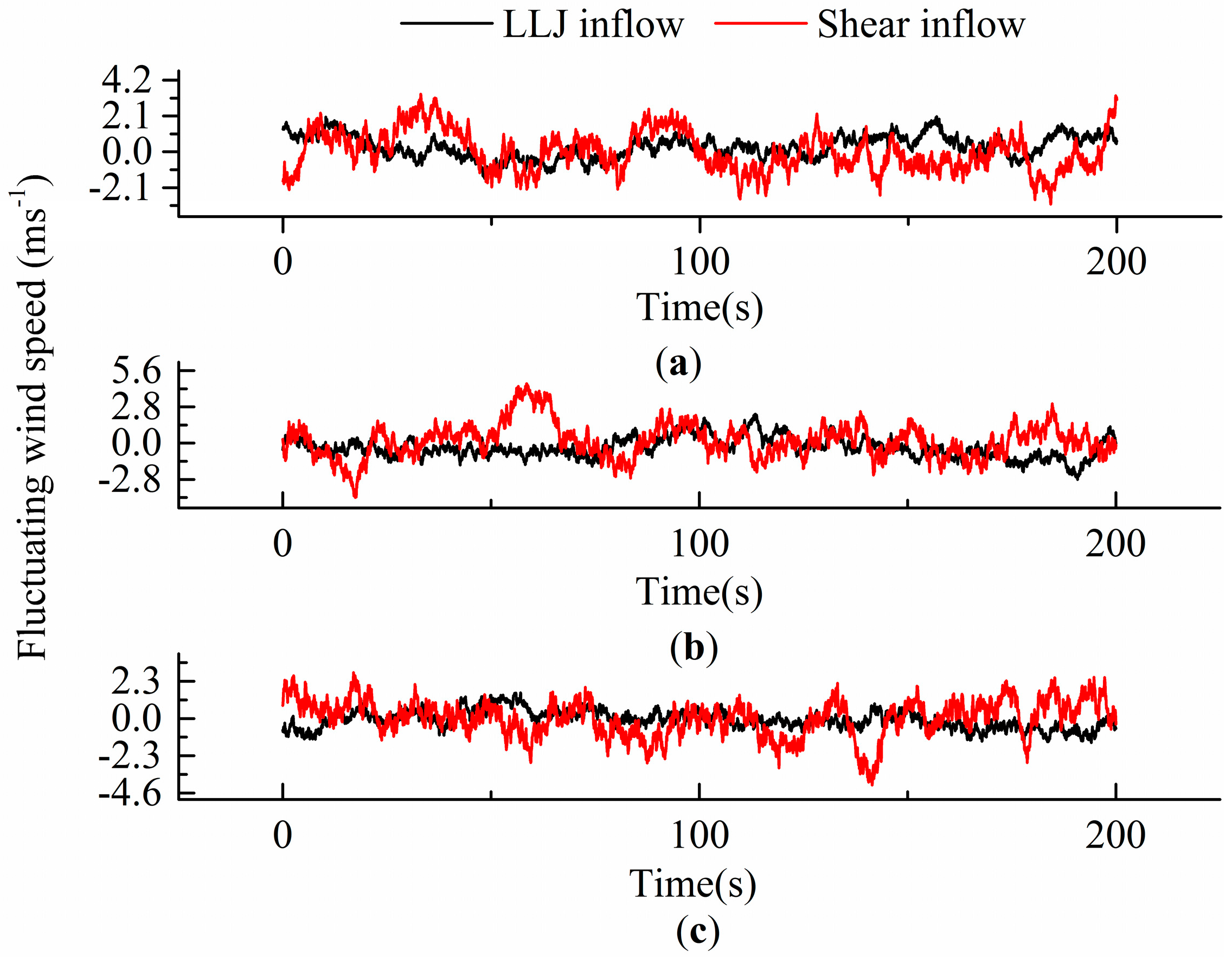
6.3. Spectrum Analysis
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Emeis, S. Wind speed and shear associated with low-level jets over Northern Germany. Meteorol. Z. 2014, 23, 295–304. [Google Scholar] [CrossRef]
- Shu, Z.R.; Li, Q.S.; He, Y.C.; Chan, P.W. Investigation of low-level jet characteristics based on wind profiler observations. J. Wind Eng. Ind. Aerodyn. 2018, 174, 369–381. [Google Scholar] [CrossRef]
- Baas, P.; Bosveld, F.C.; Klein Baltink, H.; Holtslag, A.A.M. A climatology of nocturnal low-level jets at Cabauw. J. Appl. Meteorol. Clim. 2009, 48, 1627–1642. [Google Scholar] [CrossRef]
- Ferro Duarte, H.; Leclerc, M.Y.; Zhang, G.; Durden, D.; Kurzeja, R.; Parker, M.; Werth, D. Impact of Nocturnal Low-Level Jets on Near-Surface Turbulence Kinetic Energy. Bound. Layer Meteorol. 2015, 156, 349–370. [Google Scholar] [CrossRef]
- Kelley, N.; Shirazi, M.; Jager, D.; Wilde, S.; Patton, E.G.; Sullivan, P. Lamar Low-Level Jet Project Interim Report; Technical Report. NREL/TP-500-34593; National Renewable Energy Laboratory: Golden, CO, USA, 2004.
- Lampert, A.; Bernalte Jimenez, B.; Gross, G.; Wulff, D.; Kenull, T. One-year observations of the wind distribution and low-level jet occurrence at Braunschweig, North German Plain. Wind Energy 2015, 19, 1807–1817. [Google Scholar] [CrossRef]
- Gutierrez, W.; Araya, G.; Kiliyanpilakkil, V.P.; Ruiz-Columbie, A.; Tutkun, M.; Castillo, L. Structural impact assessment of low level jets over wind turbines. J. Renew. Sustain. Energy 2016, 8, 23308. [Google Scholar] [CrossRef]
- Wilczak, J.; Finley, C.; Freedman, J.; Cline, J.; Bianco, L.; Olson, J.; Djalalova, I.; Sheridan, L.; Ahlstrom, M.; Manobianco, J.; et al. The wind forecast improvement project (WFIP): A public-private partnership addressing wind energy forecast needs. Bull. Am. Meteorol. Soc. 2015, 96, 1699–1718. [Google Scholar] [CrossRef]
- Banta, R.M.; Pichugina, Y.L.; Brewer, W.A. Turbulent Velocity-Variance Profiles in the Stable Boundary Layer Generated by a Nocturnal Low-Level Jet. J. Atmos. Sci. 2006, 63, 2700–2719. [Google Scholar] [CrossRef]
- Debnath, M.C. Influence of Atmospheric Boundary Layer on Turbulence in Wind Turbine Wake. Ph.D. Thesis, University of Texas, San Antonio, TX, USA, 2014. [Google Scholar]
- Banta, R.M. Stable-boundary-layer regimes from the perspective of the low-level jet. Acta Geophys. 2008, 56, 58–87. [Google Scholar] [CrossRef]
- Storm, B.; Dudhia, J.; Basu, S.; Swift, A.; Giammanco, I. Evaluation of the weather research and forecasting model on forecasting low-level jets: Implications for wind energy. Wind Energy 2009, 12, 81–90. [Google Scholar] [CrossRef]
- Wharton, S.; Simpson, M.; Osuna, J.; Newman, J.; Miller, W. Role of Surface Energy Exchange for Simulating Wind Turbine Inflow: A Case Study in the Southern Great Plains, USA. Atmosphere 2015, 6, 21–49. [Google Scholar] [CrossRef]
- Jonkman, B.J.; Buhl, M.L., Jr. TurbSim User’s Guide; Technical Report. NREL/TP-500-39797; National Renewable Energy Laboratory: Golden, CO, USA, 2006.
- Lundquist, J.K.; Mirocha, J.D. Interaction of nocturnal low-level jets with urban geometries as seen in Joint Urban 2003 data. J. Appl. Meteorol. Clim. 2008, 47, 44–58. [Google Scholar] [CrossRef]
- Chaudhari, A.; Agafonova, O.; Hellsten, A.; Sorvari, J. Numerical Study of the Impact of Atmospheric Stratification on a Wind-Turbine Performance. J. Phys. Conf. Ser. 2017, 854, 12007. [Google Scholar] [CrossRef]
- Helmis, C.; Sgouros, G.; Wang, Q. On the Vertical Structure and Spectral Characteristics of the Marine Low-Level Jet. Atmos. Res. 2015, 152, 74–81. [Google Scholar] [CrossRef]
- Højstrup, J. Velocity Spectra in the Unstable Planetary Boundary Layer. J. Atm. Sci. 1982, 39, 2239–2248. [Google Scholar] [CrossRef]
- Olesen, H.; Larsen, S.; Højstrup, J. Modeling velocity spectra in the lower part of the planetary boundary layer. Bound.-Layer Meteorol. 1984, 29, 285–312. [Google Scholar] [CrossRef]
- NWTC Information Portal (TurbSim). Last Modified 14 June 2016. Available online: https://nwtc.nrel.gov/TurbSim (accessed on 31 January 2019).
- Kelley, N.D. Turbulence-Turbine Interaction: The Basis for the Development of the TurbSim Stochastic Simulator; Technical Report. NREL/TP-5000-52353; National Renewable Energy Laboratory: Golden, CO, USA, 2011.
- Emeis, S. Wind Energy Meteorology, 2nd ed.; Springer: New York, NY, USA, 2013; p. 34. ISBN 978-3-642-30523-8. [Google Scholar]
- Jonkman, J.; Butterfield, S.; Musial, W.; Scott, G. Definition of a 5-MW Reference Wind Turbine for Offshore System Development; Technical Report. NREL/TP-500-38060; National Renewable Energy Laboratory: Golden, CO, USA, 2009.
- Jonkman, J.; Buhl, M.L.J. FAST User’s Guide; Technical Report. NREL/EL-500-38230; National Renewable Energy Laboratory: Golden, CO, USA, 2005.
- Leishman, J.G.; Beddoes, T.S. A Semi-Empirical Model for Dynamic Stall. J. Am. Helicopter Soc. 1989, 34, 3–17. [Google Scholar] [CrossRef]
- Choukulkar, A.; Pichugina, Y.; Clack, C.; Calhoun, R.; Banta, R.; Brewer, A.; Hardesty, M. A new formulation for rotor equivalent wind speed for wind resource assessment and wind power forecasting. Wind Energy 2016, 19, 1439–1452. [Google Scholar] [CrossRef]
- Zhou, N.; Chen, J.; Adams, D.E.; Fleeter, S. Influence of inflow conditions on turbine loading and wake structures predicted by large eddy simulations using exact geometry. Wind Energy 2015, 19, 803–824. [Google Scholar] [CrossRef]
- Churchfield, M.; Lee, S.; Michalakes, J.; Moriarty, P.J. A numerical study of the effects of atmospheric and wake turbulence on wind turbine dynamics. J. Turbul. 2012, 13, 1–32. [Google Scholar] [CrossRef]







| Parameter | Value |
|---|---|
| Simulation time (s) | 200 |
| Time step (s) | 0.05 |
| Average gradient Richardson number | 0.02 |
| Friction velocity (m/s) | 0.55 |
| Surface roughness length (m) | 0.005 |
| Hub height (m) | 150 |
| Maximum wind speed (m/s) | 11.4 |
| LLJ height (m) | 87, 119, 150, 181, 213 |
| Parameter | Value |
|---|---|
| Simulation time (s) | 200 |
| Time step (s) | 0.05 |
| Turbulence length scale (m) | 73.5 |
| Turbulence intensity (%) | 10 |
| Hub height (m) | 150 |
| Average hub-height wind speed (m/s) | 11.4 |
| Wind shear exponent | 0.1, 0.2, 0.3 |
| Parameters | Value |
|---|---|
| Rotor, hub diameter (m) | 126, 3 |
| Shaft tilt, precone (°) | 5, 2.5 |
| Airfoil distribution | NACA series airfoils, DU series airfoils |
| Blade number | 3 |
| Cut-in, rated, cut-out wind speed (m/s) | 3, 11.4, 25 |
| Cut-in, rated rotor speed (r/min) | 6.9, 12.1 |
| Wind Speed (m/s) | 5 | 7 | 9 | 11.4 | 15 | 20 | 25 |
| Pitch angle (°) | 0 | 0 | 0 | 0 | 10.45 | 17.47 | 23.47 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Yang, C.; Li, S. Influence of the Heights of Low-Level Jets on Power and Aerodynamic Loads of a Horizontal Axis Wind Turbine Rotor. Atmosphere 2019, 10, 132. https://doi.org/10.3390/atmos10030132
Zhang X, Yang C, Li S. Influence of the Heights of Low-Level Jets on Power and Aerodynamic Loads of a Horizontal Axis Wind Turbine Rotor. Atmosphere. 2019; 10(3):132. https://doi.org/10.3390/atmos10030132
Chicago/Turabian StyleZhang, Xuyao, Congxin Yang, and Shoutu Li. 2019. "Influence of the Heights of Low-Level Jets on Power and Aerodynamic Loads of a Horizontal Axis Wind Turbine Rotor" Atmosphere 10, no. 3: 132. https://doi.org/10.3390/atmos10030132
APA StyleZhang, X., Yang, C., & Li, S. (2019). Influence of the Heights of Low-Level Jets on Power and Aerodynamic Loads of a Horizontal Axis Wind Turbine Rotor. Atmosphere, 10(3), 132. https://doi.org/10.3390/atmos10030132





