Simulation of Flax Threshing Process by Different Forms of Threshing Drums in Combined Harvesting
Abstract
1. Introduction
2. Materials and Methods
2.1. Analysis of Threshing Devices and Intercropping Processes
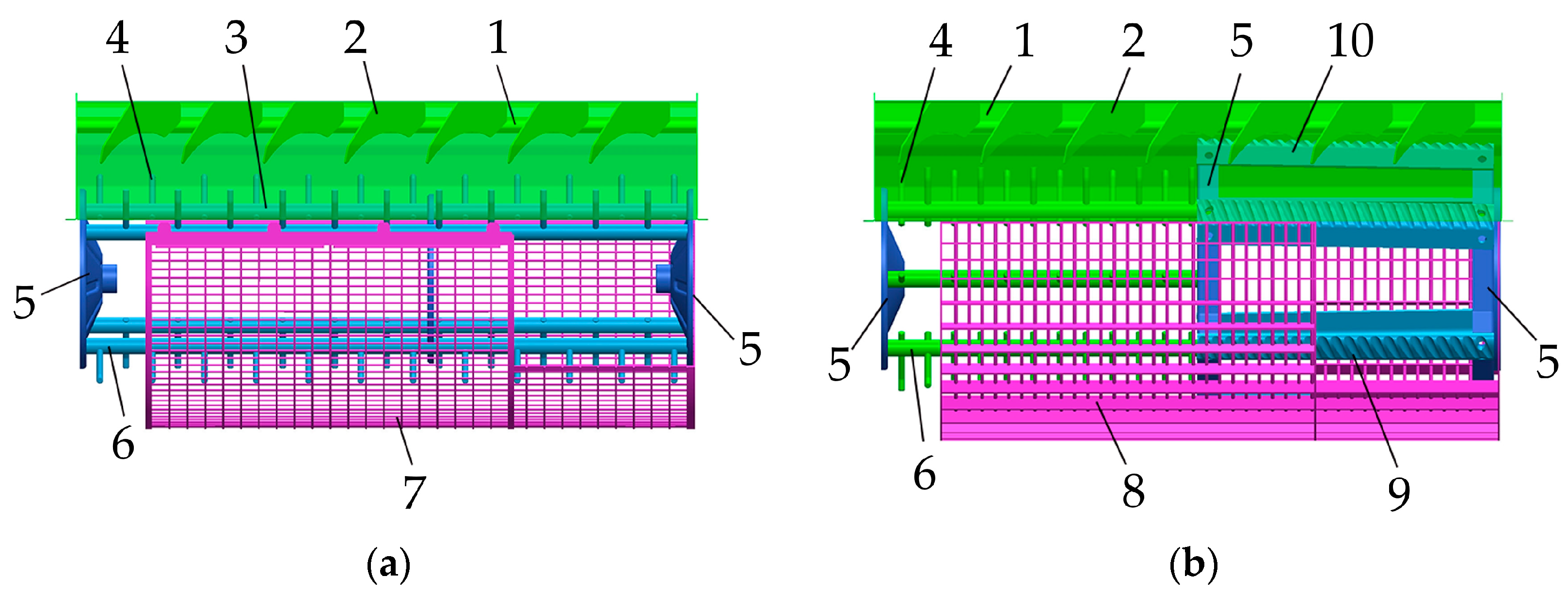
2.1.1. Threshing Device
2.1.2. Interaction Theory Analysis
2.2. Joint DEM-FEA Simulation Modeling and Setup
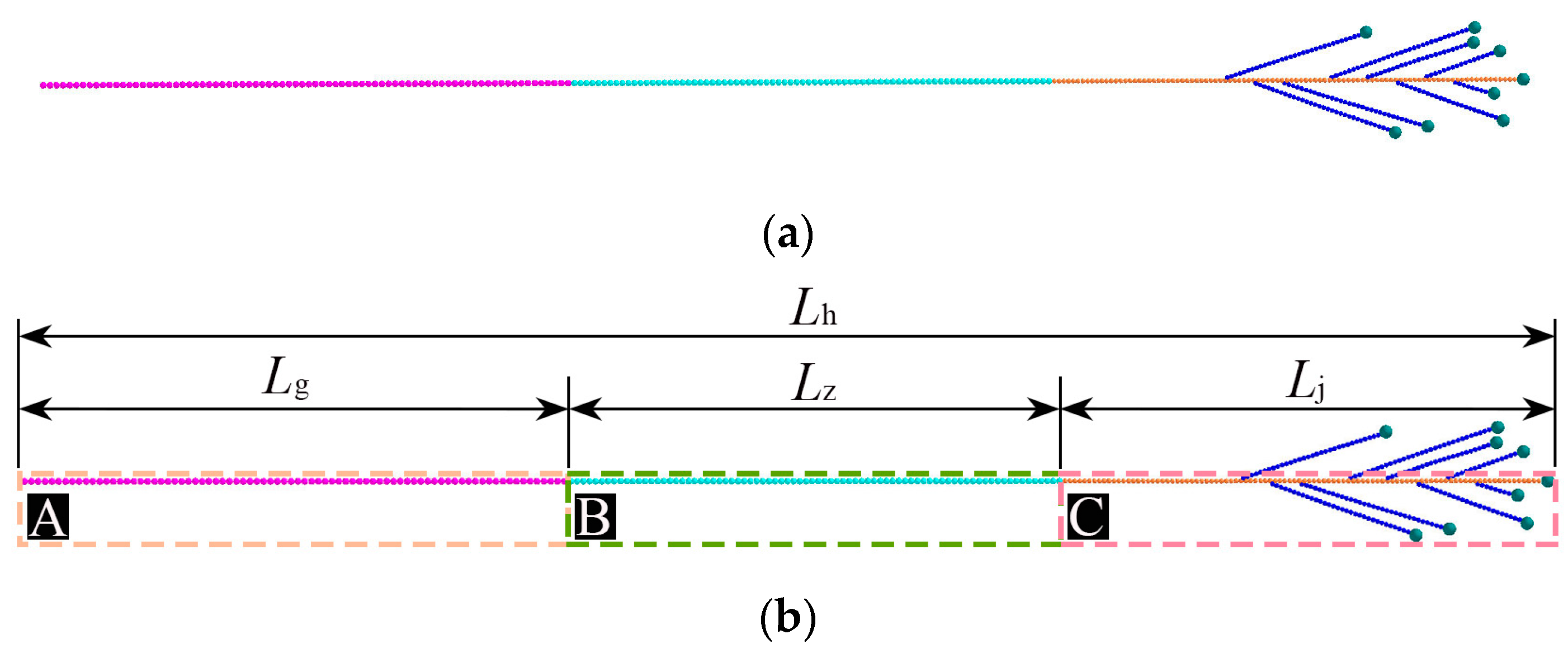
2.2.1. DEM Modeling of Flax Plants
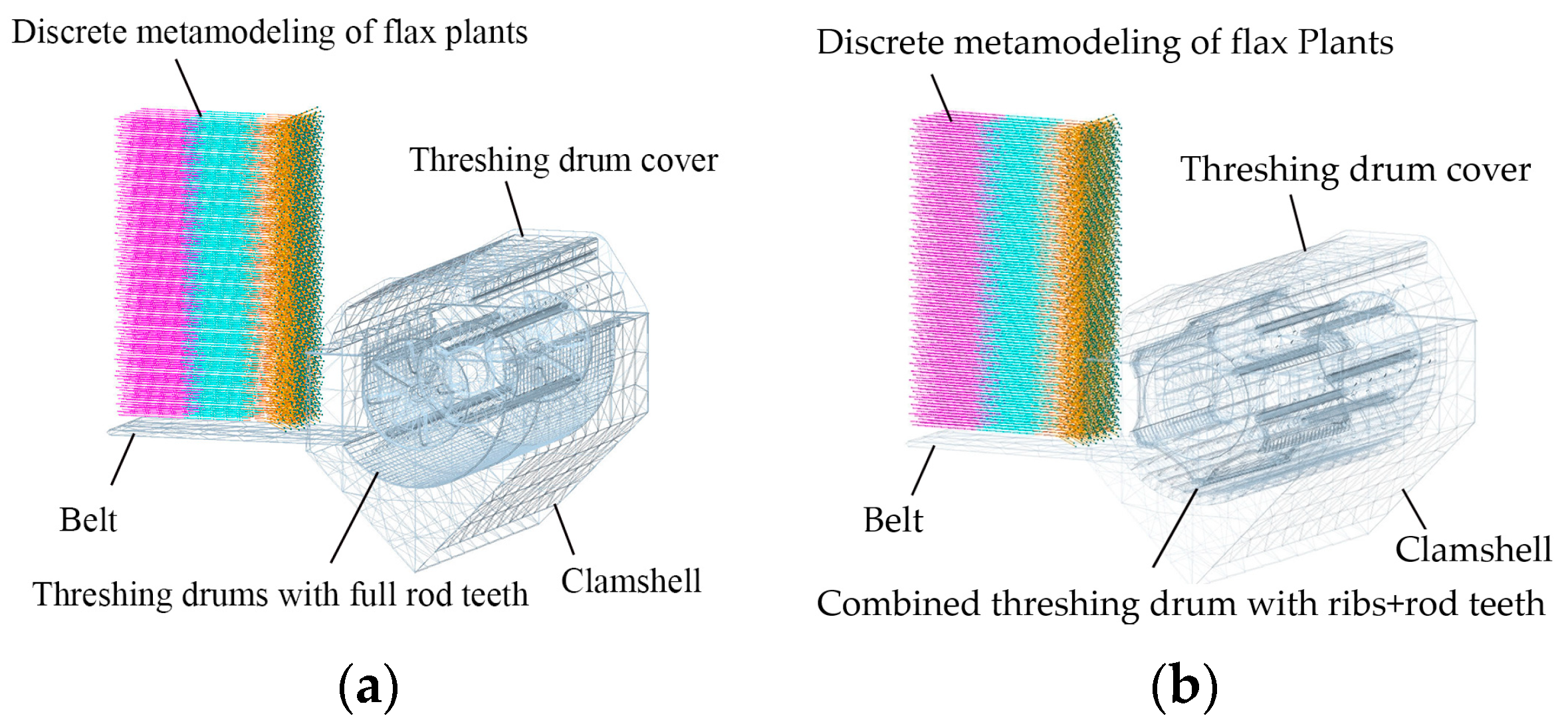
2.2.2. Threshing Drum FEA Modeling
2.2.3. Parameter Settings
3. Results
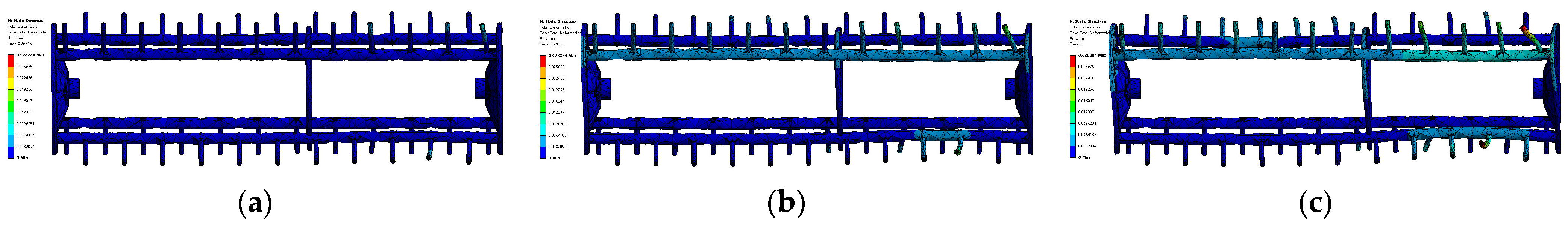
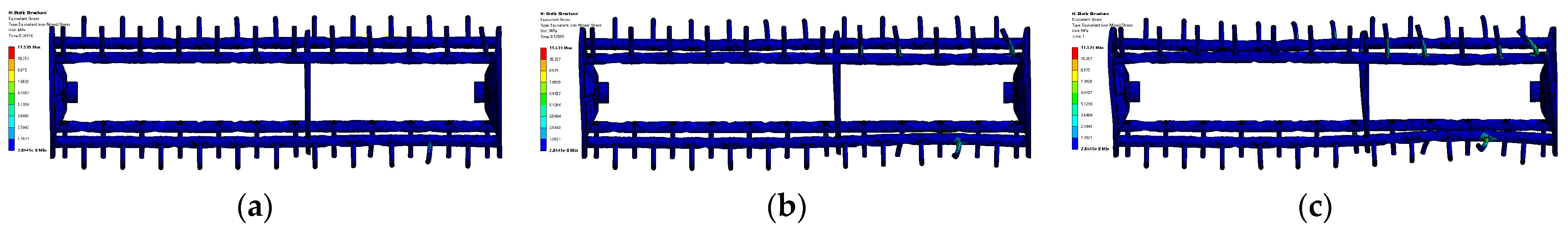
3.1. DEM-FEA Simulation Results Analysis
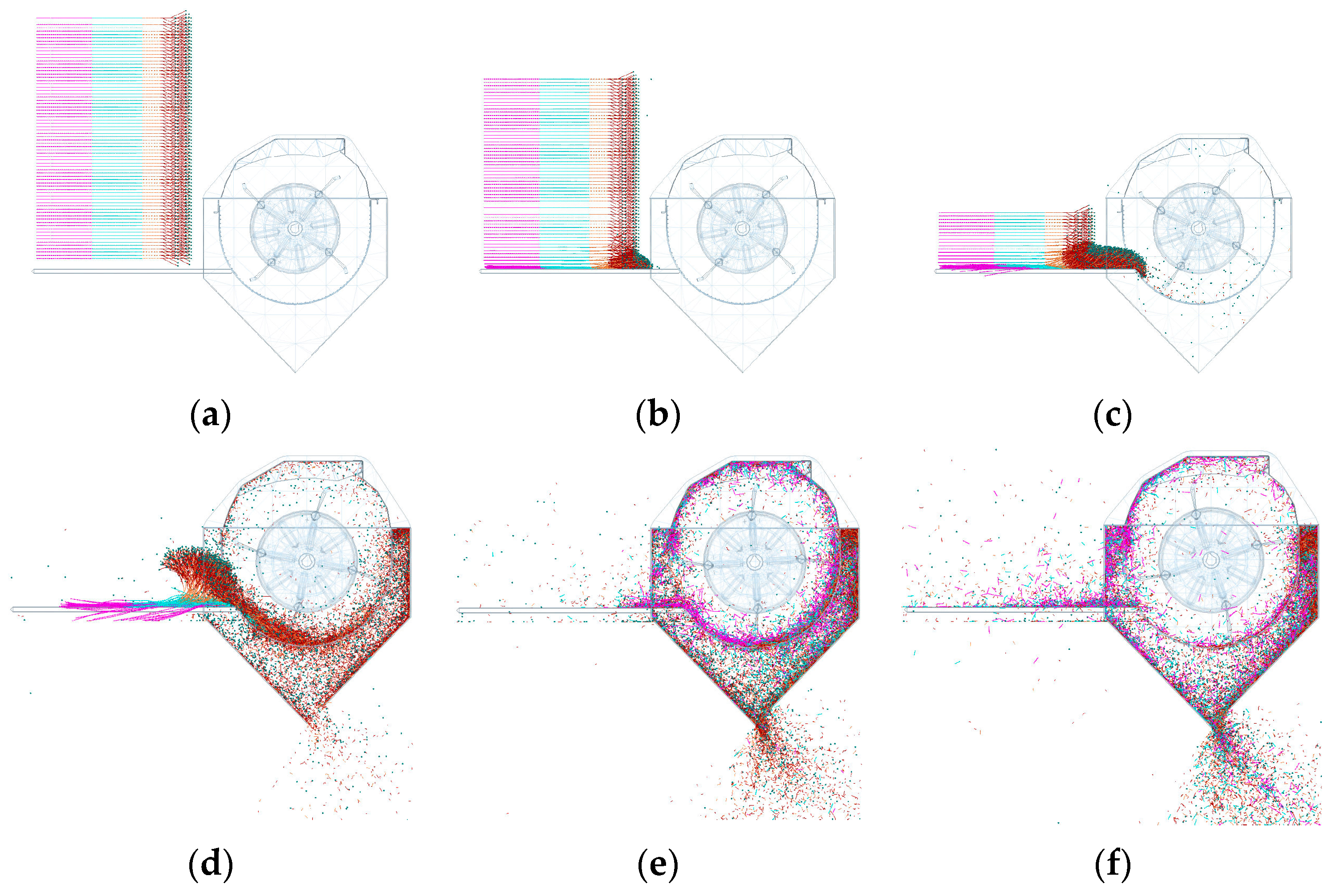
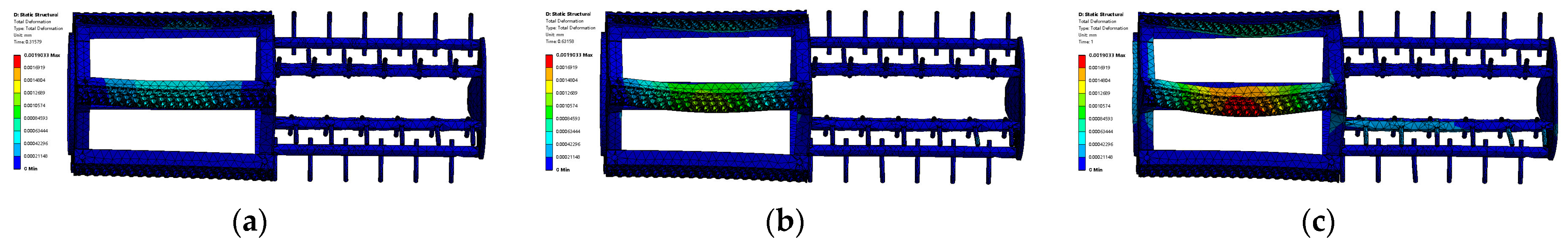
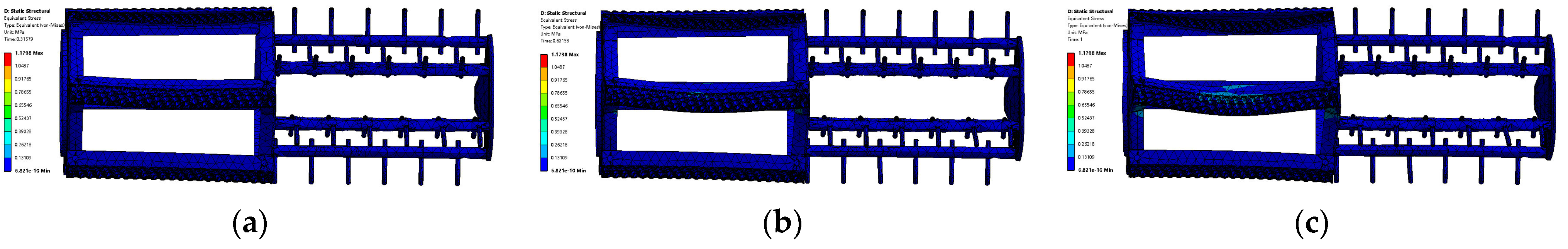
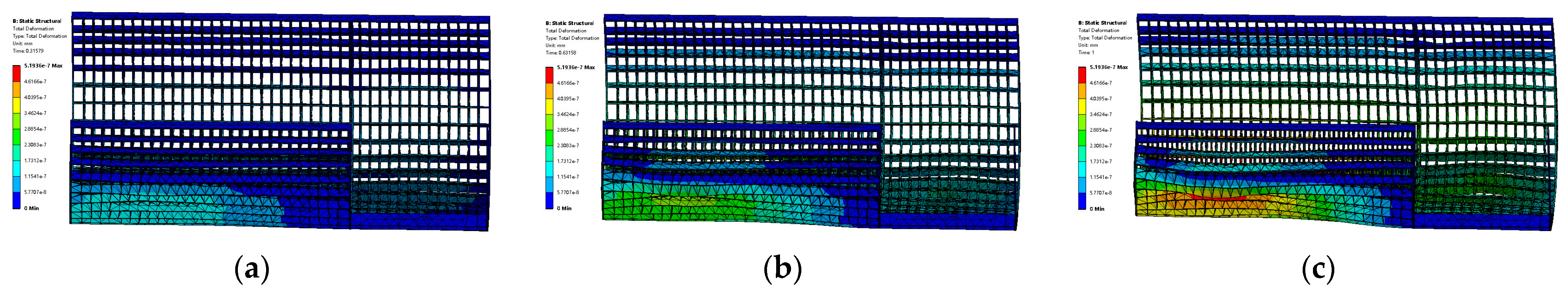
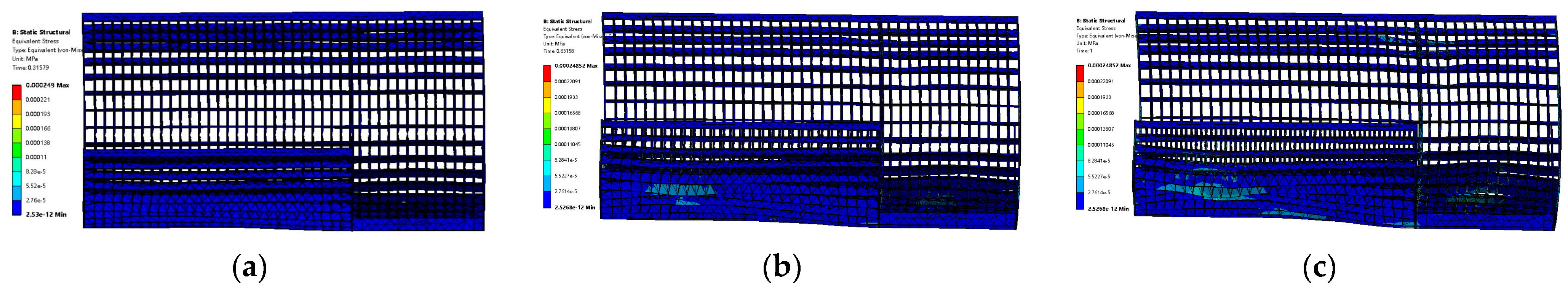
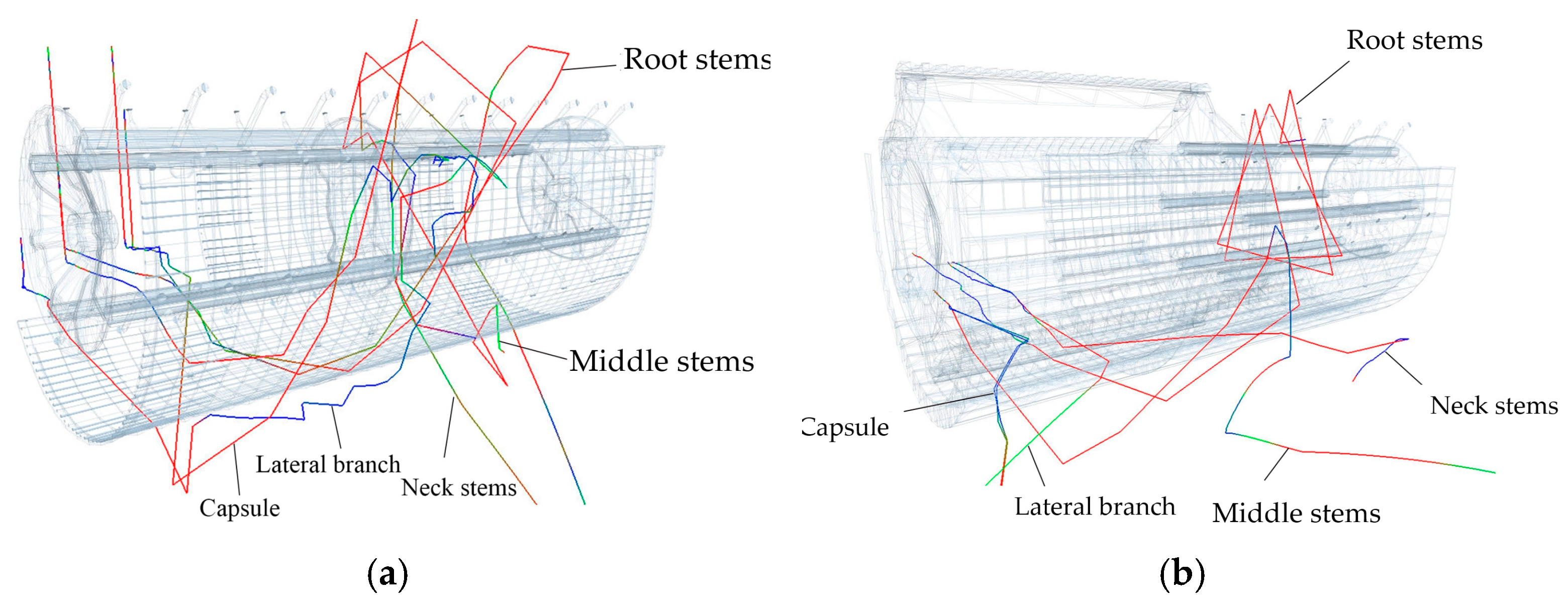
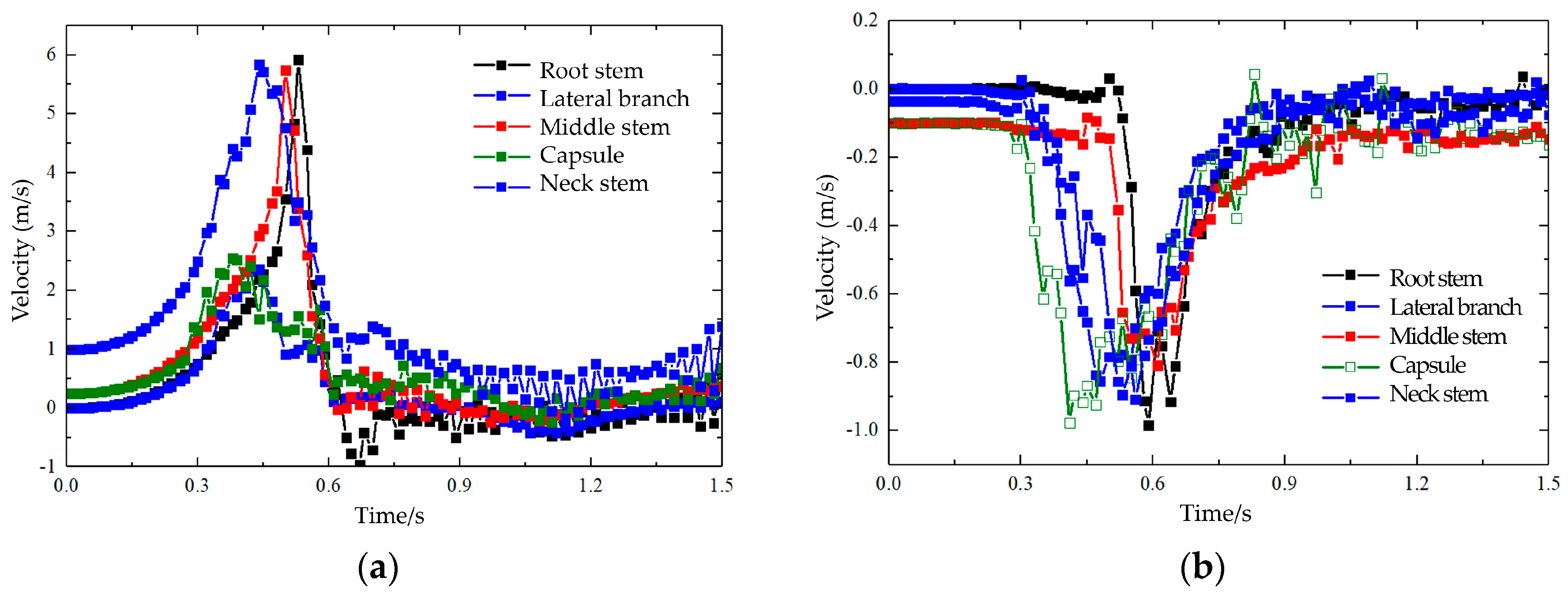
3.1.1. Analysis of T1 Model Simulation Results
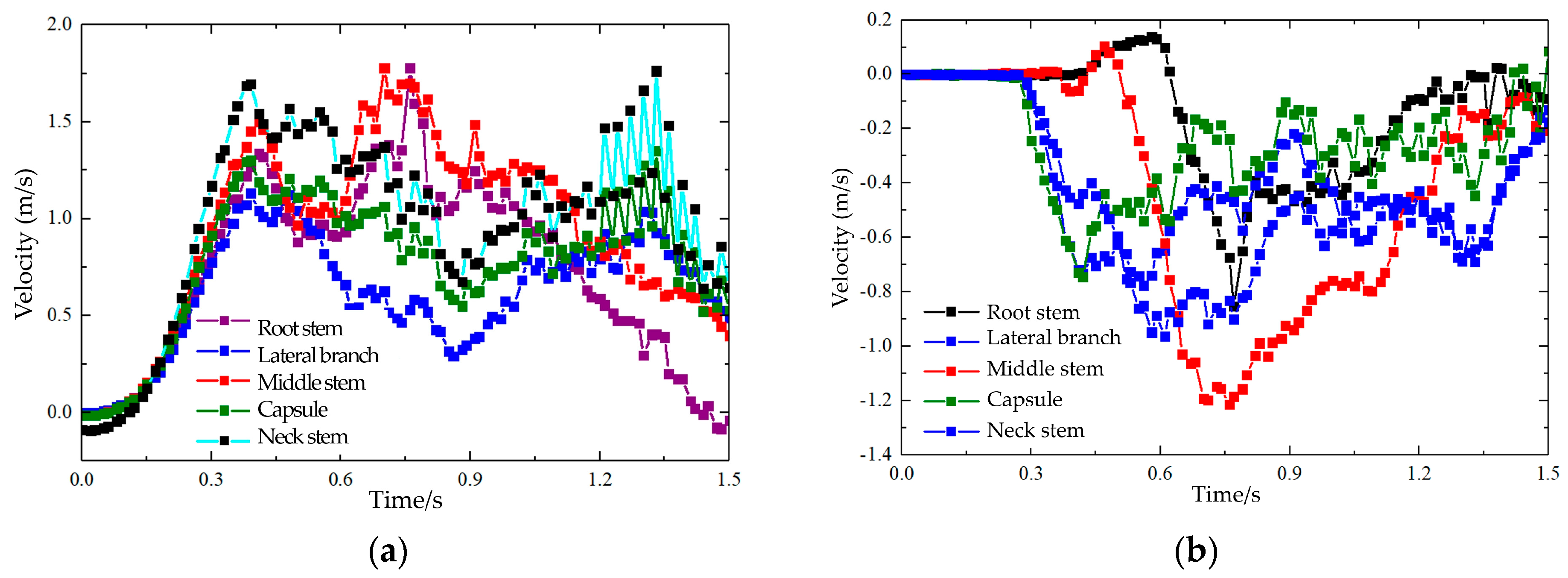
3.1.2. Analysis of T2 Model Simulation Results
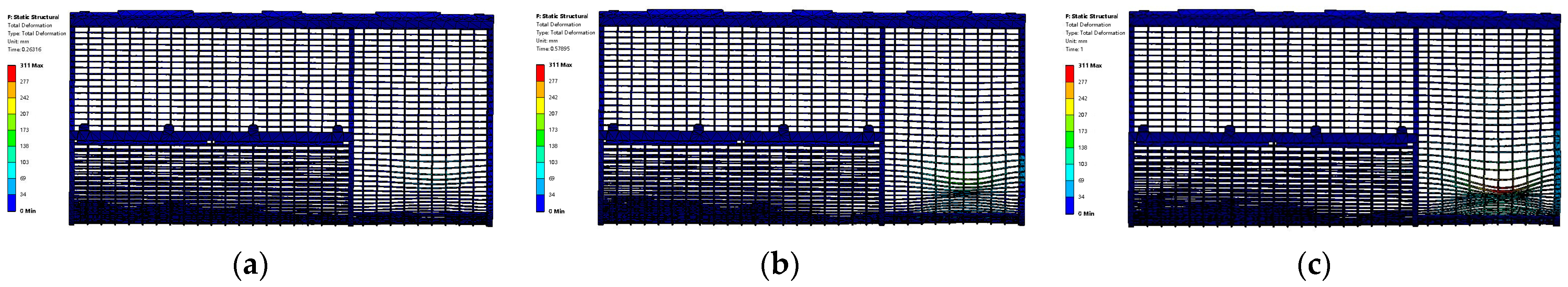
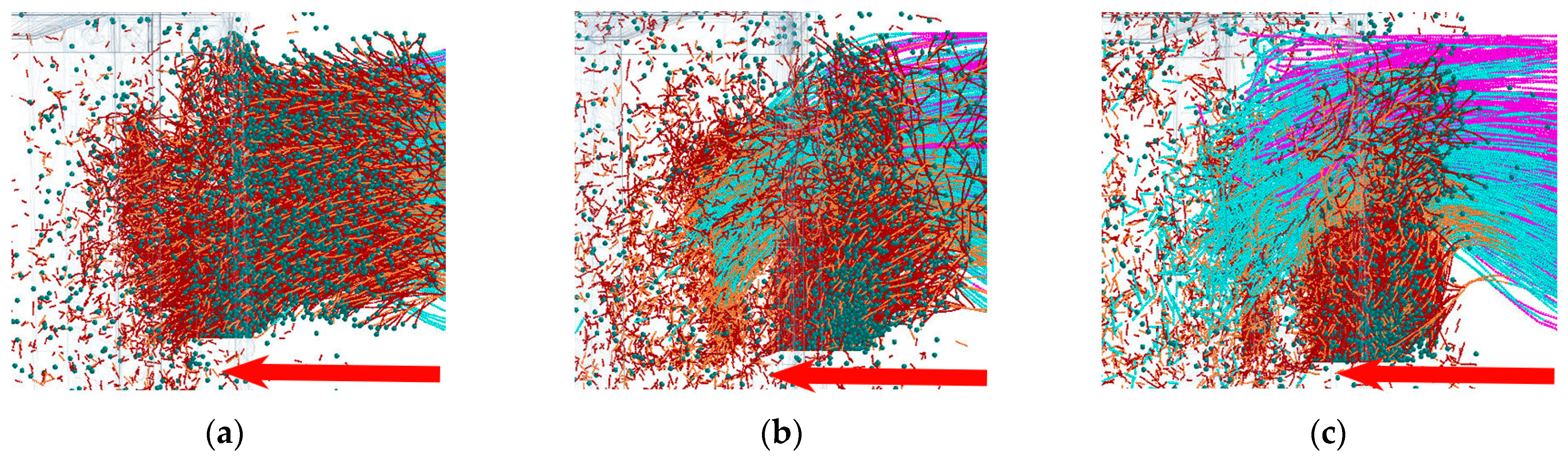
3.2. Analysis of Flax Plant Movement
3.3. Mechanism of Flax Plant Threshing
4. Field Trial
4.1. Test Materials
4.2. Test Method
4.3. Test Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Čeh, B.; Štraus, S.; Hladnik, A.; Kušar, A. Impact of Linseed Variety, Location and Production Year on Seed Yield, Oil Content and Its Composition. Agronomy 2020, 10, 1770. [Google Scholar] [CrossRef]
- Rasheed, A.; He, P.; Long, Z.; Gillani, S.F.A.; Wang, Z.; Morsy, K.; Hashem, M.; Jie, Y. Cadmium (Cd) Tolerance and Phytoremediation Potential in Fiber Crops: Research Updates and Future Breeding Efforts. Agronomy 2024, 14, 2713. [Google Scholar] [CrossRef]
- Shi, R.; Dai, F.; Liu, X.; Zhao, W.; Qu, J.; Zhang, F.; Qing, D. Design and experiments of crawler-type hilly and mountainous flax combine harvester. Trans. Chin. Soc. Agric. Eng. 2021, 37, 59–67. [Google Scholar]
- Liang, Z.; Qin, Y.; Su, Z. Establishment of a Feeding Rate Prediction Model for Combine Harvesters. Agriculture 2024, 14, 589. [Google Scholar] [CrossRef]
- Wang, X.; Xie, F.; Ren, S.; Wang, X.; Zhang, Z. A mathematical model and test of the horizontal axial flow threshing separation device. Hunan Agric. Univ. (Nat. Sci.) 2020, 46, 480–487. [Google Scholar]
- Hussain, S.; Lei, X.; Wu, H.; Li, H.; Song, H.; Zheng, D.; Ghafoor, A. Optimizing the design of a multi-stage tangential roller threshing unit using CFD modeling and experimental studies. Comput. Electron. Agric. 2024, 226, 109400. [Google Scholar] [CrossRef]
- Liang, Z.; Million, E.W. Development of cleaning systems for combine harvesters: A. review. Biosyst. Eng. 2023, 236, 79–102. [Google Scholar] [CrossRef]
- Liu, Y.; Li, Y.; Dong, Y.; Huang, M.; Zhang, T.; Cheng, J. Development of a variable-diameter threshing drum for rice combine harvester using MBD-DEM coupling simulation. Comput. Electron. Agric. 2022, 196, 106859. [Google Scholar] [CrossRef]
- Sudajan, S.; Salokhe, V.M.; Triratanasirichai, K. PM—Power and machinery: Effect of type of drum, drum speed and feed rate on sunflower threshing. Biosyst. Eng. 2002, 83, 413–421. [Google Scholar] [CrossRef]
- Liu, W.; Zeng, S.; Chen, X. Design and experiment of spiral step cleaning device for ratooning rice based on CFD-DEM coupling. Comput. Electron. Agric. 2024, 224, 109207. [Google Scholar] [CrossRef]
- Wang, Q.; Mao, H.; Li, Q. Modelling and simulation of the grain threshing process based on the discrete element method. Comput. Electron. Agric. 2020, 178, 105790. [Google Scholar] [CrossRef]
- Wu, J.; Tang, Q.; Mu, S.; Yang, X.; Jiang, L.; Hu, Z. Design and test of self-leveling system for cleaning screen of grain combine harvester. Agriculture 2023, 13, 377. [Google Scholar] [CrossRef]
- Que, K.; Tang, Z.; Wang, T.; Su, Z.; Ding, Z. Effects of Unbalanced Incentives on Threshing Drum Stability during Rice Threshing. Agriculture 2024, 14, 777. [Google Scholar] [CrossRef]
- Xu, P.; Dai, F.; Shi, R.; Zhao, W.; Song, X.; Zhao, Q.; Zhao, Y. Contact parameter calibration for flax threshing materials using machine learning and the Brazil nut effect. Powder Technol. 2024, 446, 120190. [Google Scholar] [CrossRef]
- Dai, F.; Xu, P.; Yuan, Z.; Shi, R.; Zhao, Y.; Song, X.; Zhao, W. Simulation and Optimization Experiment: Working Process of a Cleaning Device for Flax Combine Harvester. Agriculture 2023, 13, 2123. [Google Scholar] [CrossRef]
- Santiago, A.A.; Vargas, J.; Pérez, L.; Corona-García, C.; Alfonso, I. Mathematical and finite element analysis estimations of the reinforcement effect on Young’s modulus of polymer membrane/montmorillonite clay nanocomposites. Polym. Compos. 2021, 42, 2112–2121. [Google Scholar] [CrossRef]
- Foulk, J.A.; Akin, D.E.; Dodd, R.B. Processability of flax plant stems into functional bast fibers. Compos. Interfaces 2008, 15, 147–168. [Google Scholar] [CrossRef]
- Bu, L.; Chen, C.; Hu, G.; Zhou, J.; Adilet, S.; Chen, J. Investigating the dynamic behavior of an apple branch-stem-fruit model using experimental and simulation analysis. Comput. Electron. Agric. 2021, 186, 106224. [Google Scholar] [CrossRef]
- Zhang, D.; Yi, S.; Zhang, J.; Bao, Y. Establishment of millet threshing and separating model and optimization of harvester parameters. Alex. Eng. J. 2022, 61, 11251–11265. [Google Scholar] [CrossRef]
- Kunaev, V.; Bazarov, B.; Tavshanov, I.; Kadyrov, A.; Abdugaliyeva, G.; Kydyrbayeva, S. Evaluation of the effect of enrichment of slag aggregate for pavement subbase by grains density on its physical and mechanical characteristics. Results Eng. 2023, 18, 101181. [Google Scholar] [CrossRef]
- Feng, X.; Wang, L.; Bi, S.; Wang, B.; Ma, Z.; Gao, Y. Effects of Threshing Devices, Maize Varieties and Moisture Content of Grains on the Percentage of Maize Grains Broken in Harvesting. Agronomy 2023, 13, 1615. [Google Scholar] [CrossRef]
- Dai, F.; Song, X.; Guo, W.; Zhao, W.; Zhang, F.; Zhang, S. Simulation and test on separating cleaning process of flax threshing material based on gas-solid coupling theory. Int. J. Agric. Biol. Eng. 2020, 13, 73–81. [Google Scholar] [CrossRef]
- Xiao, F.; Luo, M.; Huang, F.; Zhou, M.; An, J.; Kuang, S.; Yu, A. CFD–DEM investigation of gas-solid flow and wall erosion of vortex elbows conveying coarse particles. Powder Technol. 2023, 424, 118524. [Google Scholar] [CrossRef]
- Xu, L.; Li, Y.; Zheng, M.; Ma, Z.; Zhao, Z.; Wang, C. Theoretical analysis and finite element simulation of a rice kernel obliquely impacted by a threshing tooth. Biosyst. Eng. 2013, 114, 146–156. [Google Scholar]
- Pérez, L.; Mercado, R.; Alfonso, I. Young’s modulus estimation for CNT reinforced metallic foams obtained using different space holder particles. Compos. Struct. 2017, 168, 26–32. [Google Scholar] [CrossRef]
- Tang, Z.; Zhang, H.; Wang, X.; Gu, X.; Zhang, B.; Liu, S. Rice threshing state prediction of threshing cylinder undergoing unbalanced harmonic response. Comput. Electron. Agric. 2023, 204, 107547. [Google Scholar] [CrossRef]
- Dudarev, I. A review of fiber flax harvesting: Conditions, technologies, processes and machines. J. Nat. Fibers 2022, 19, 4496–4508. [Google Scholar] [CrossRef]





| Projects | Parameters | Value | |
|---|---|---|---|
| Intrinsic parameter | Root Stems | Poisson’s ratio | 0.082 |
| Shear modulus/Mpa | 1316.19 | ||
| Density/(kg/m3) | 410.9 | ||
| Normal stiffness/(N/m3) | 1.2 × 109 | ||
| Tangential stiffness/(N/m3) | 6 × 108 | ||
| Normal critical stress/MPa | 13.4 | ||
| Tangential critical stress/MPa | 13.04 | ||
| Middle Stems | Poisson’s ratio | 0.085 | |
| Shear modulus/Mpa | 2956.99 | ||
| Density/(kg/m3) | 485.1 | ||
| Normal stiffness/(N/m3) | 1.3 × 109 | ||
| Tangential stiffness/(N/m3) | 6.5 × 108 | ||
| Normal critical stress/MPa | 5.6 | ||
| Tangential critical stress/MPa | 7.78 | ||
| Neck Stems | Poisson’s ratio | 0.089 | |
| Shear modulus/Mpa | 2146.32 | ||
| Density/(kg/m3) | 465.6 | ||
| Normal stiffness/(N/m3) | 0.9 × 109 | ||
| Tangential stiffness/(N/m3) | 4.5 × 108 | ||
| Normal critical stress/MPa | 1 | ||
| Tangential critical stress/MPa | 4.67 | ||
| Contact parameter | Threshing devices | Shear modulus/Mpa | 7.9 × 104 |
| Density/(kg/m3) | 7800 | ||
| Stems-stems | Coefficient of recovery | 0.3 | |
| Static Friction Factor | 0.508 | ||
| Dynamic friction factor | 0.033 | ||
| Stems-threshing units | Coefficient of recovery | 0.33 | |
| Static Friction Factor | 0.28 | ||
| Dynamic friction factor | 0.14 | ||
| Test Indexes | Total Loss Rate/% | Impurity Rate/% | Work Efficiency/(hm2/h) |
|---|---|---|---|
| Standard values | ≤5 | ≤5 | - |
| T1 model operator | 3.32 | 3.57 | 0.09 |
| T2 model operator | 2.29 | 3.39 | 0.22 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, R.; Chang, L.; Zhao, W.; Dai, F.; Liang, Z. Simulation of Flax Threshing Process by Different Forms of Threshing Drums in Combined Harvesting. Agronomy 2025, 15, 36. https://doi.org/10.3390/agronomy15010036
Shi R, Chang L, Zhao W, Dai F, Liang Z. Simulation of Flax Threshing Process by Different Forms of Threshing Drums in Combined Harvesting. Agronomy. 2025; 15(1):36. https://doi.org/10.3390/agronomy15010036
Chicago/Turabian StyleShi, Ruijie, Leilei Chang, Wuyun Zhao, Fei Dai, and Zhenwei Liang. 2025. "Simulation of Flax Threshing Process by Different Forms of Threshing Drums in Combined Harvesting" Agronomy 15, no. 1: 36. https://doi.org/10.3390/agronomy15010036
APA StyleShi, R., Chang, L., Zhao, W., Dai, F., & Liang, Z. (2025). Simulation of Flax Threshing Process by Different Forms of Threshing Drums in Combined Harvesting. Agronomy, 15(1), 36. https://doi.org/10.3390/agronomy15010036








