Experimental Study on the Application of “Dry Sowing and Wet Emergence” Drip Irrigation Technology with One Film, Three Tubes, and Three Rows
Abstract
1. Introduction
2. Materials and Methods
2.1. Overview of the Study Area
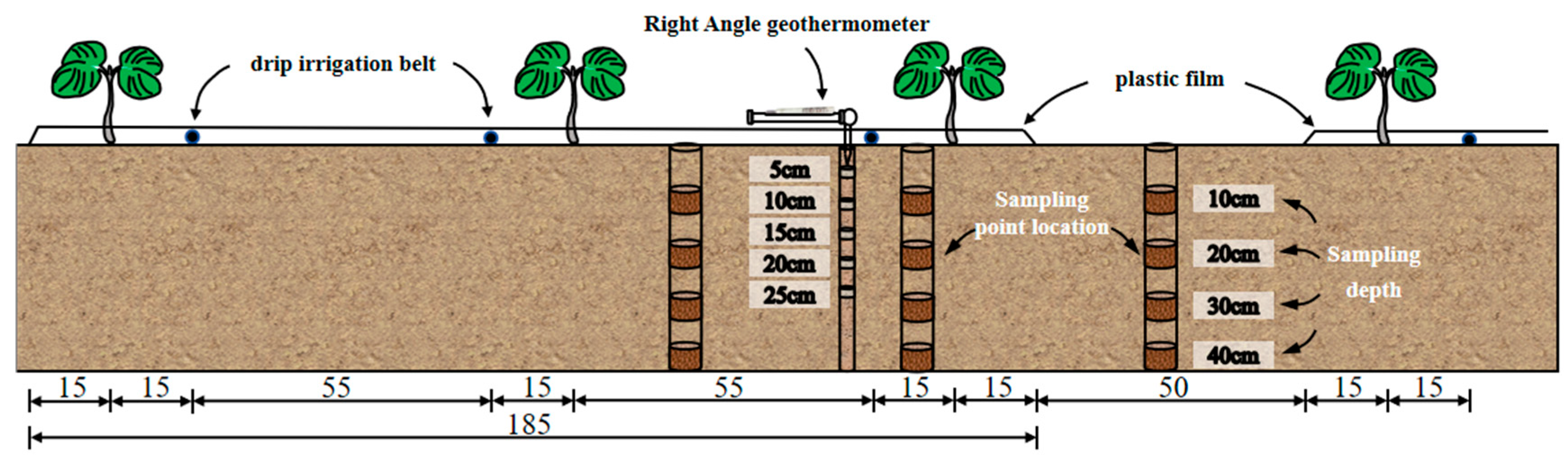
2.2. Experimental Design
2.3. Measurement Indicators
2.3.1. Soil Water Content
2.3.2. Soil Salt Content
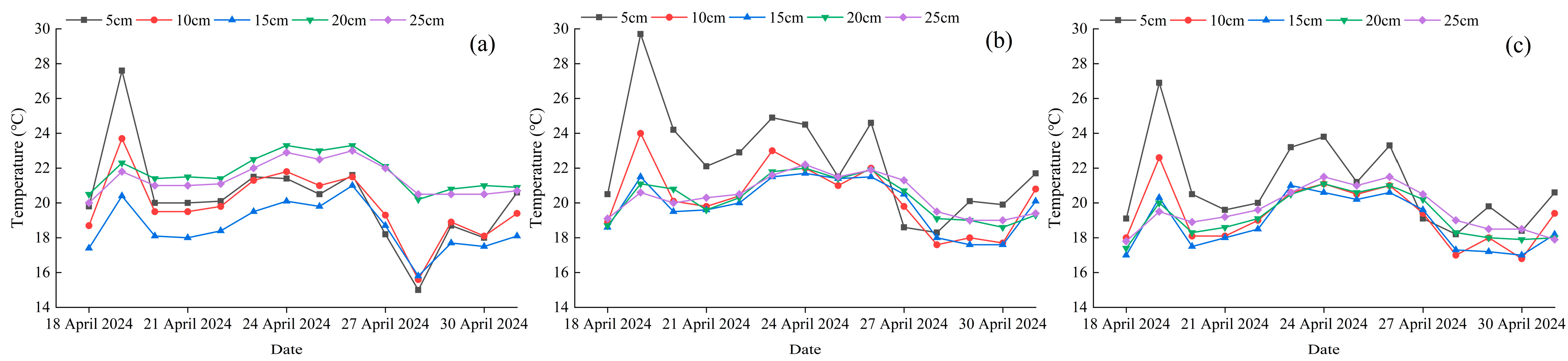
2.3.3. Soil Temperature
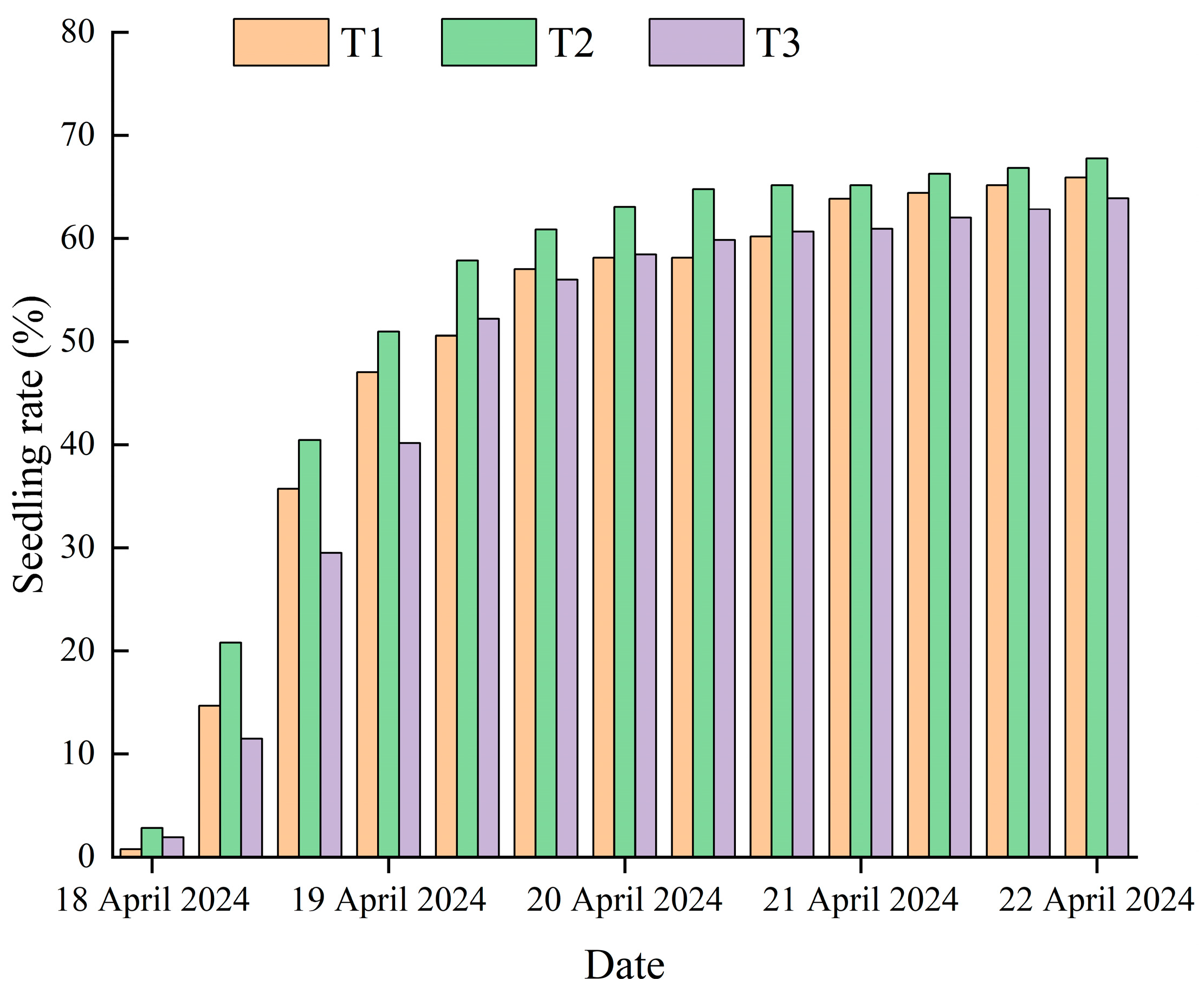
2.3.4. Characteristics of Cotton Emergence
2.4. Model Simulation
2.4.1. Cotton Emergence Process—Logistic Modeling
2.4.2. HYDRUS-2D Model
- 1.
- Soil water transport equation
- 2.
- Soil solute transport equation
- 3.
- Basic equation of soil evapotranspiration
- 4.
- Initial and boundary conditions for soil water movement
- 5.
- Initial and boundary conditions for soil salt transport
- 6.
- Model mesh discretization
2.5. Evaluation of Model Results
2.6. Data Processing
3. Result and Analysis
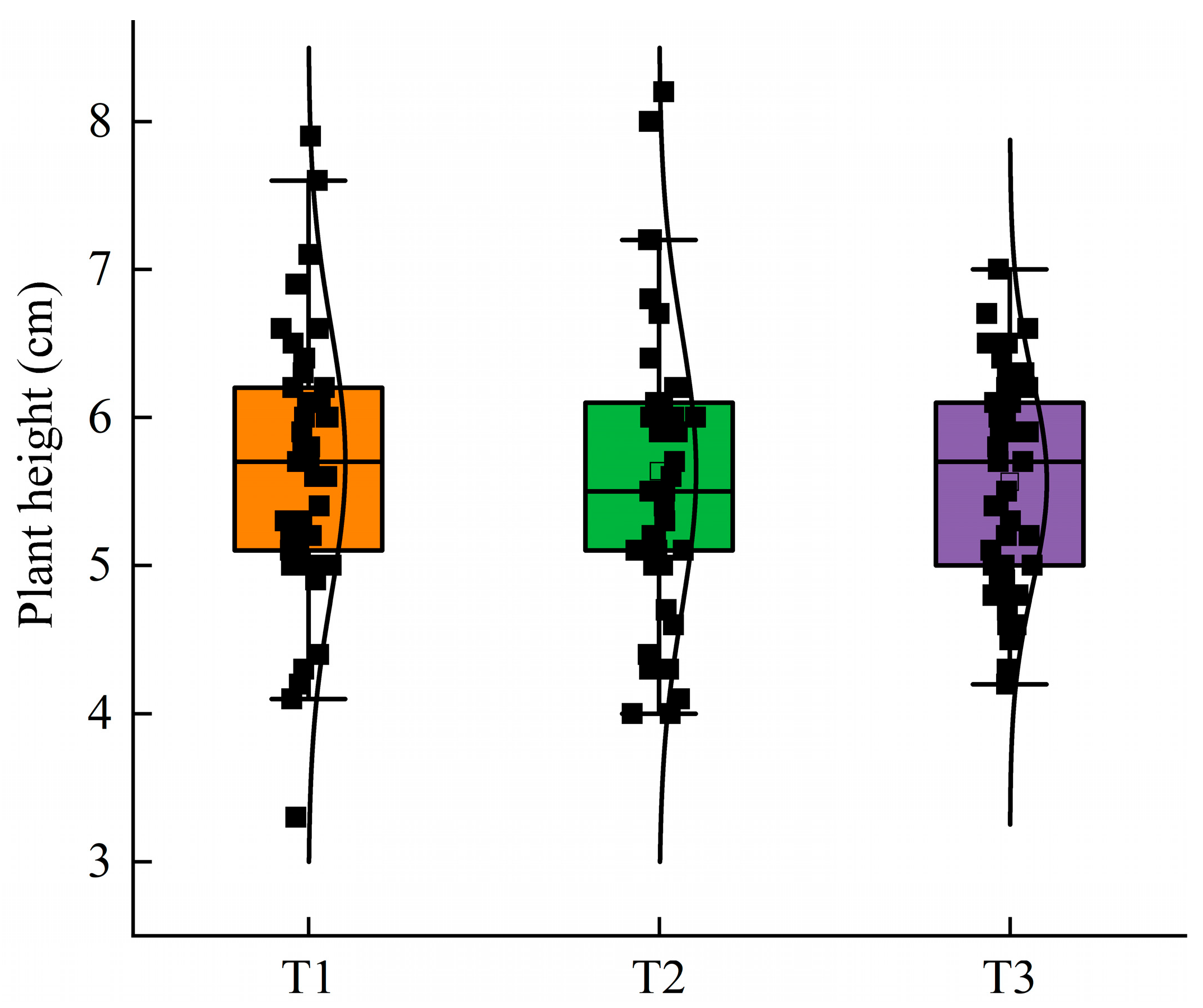
3.1. Effects of Different Water Amounts on Soil Water, Heat, and Salt Conditions and Seedling Growth
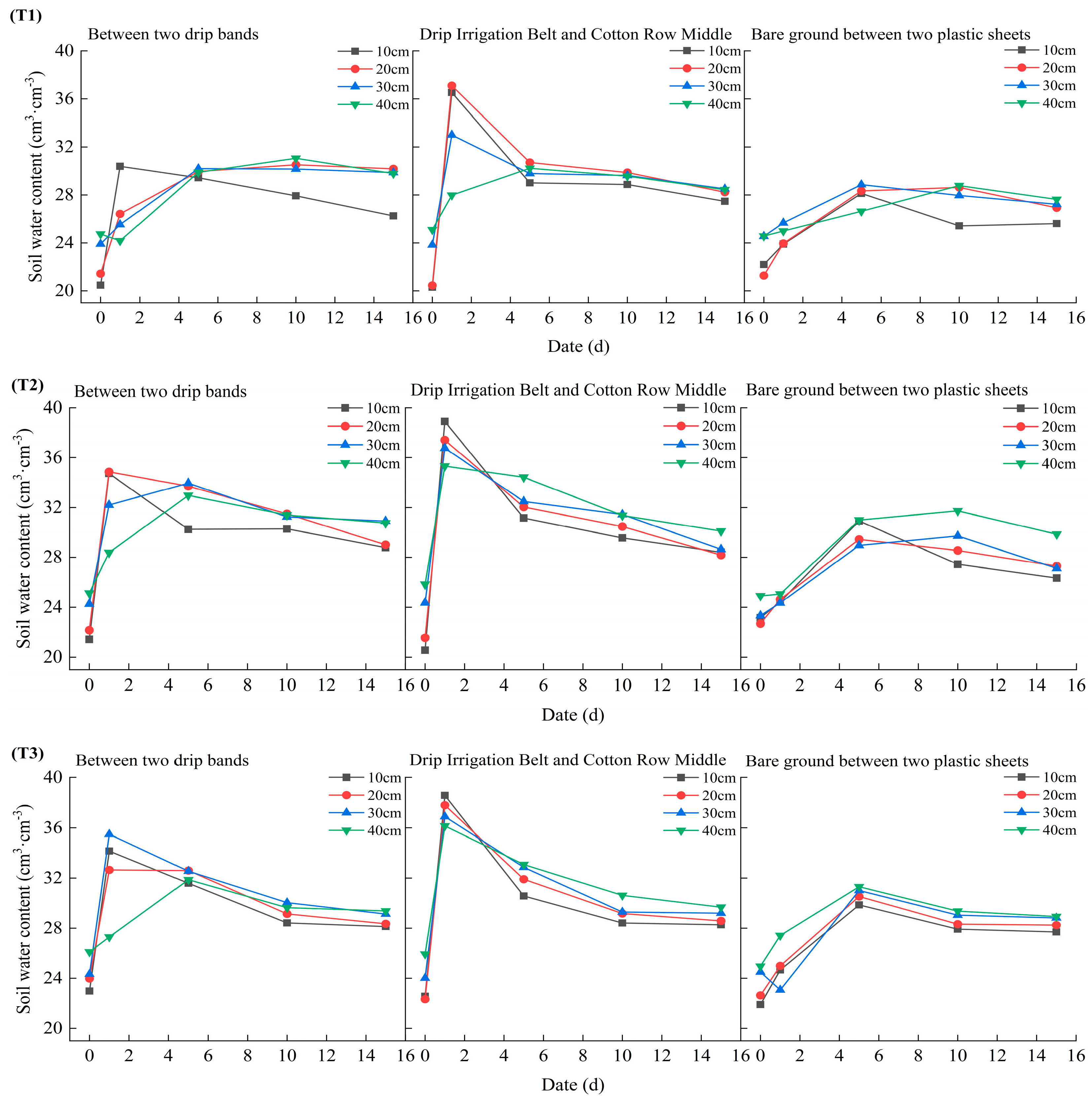
3.1.1. Characteristics of Soil Water Distribution
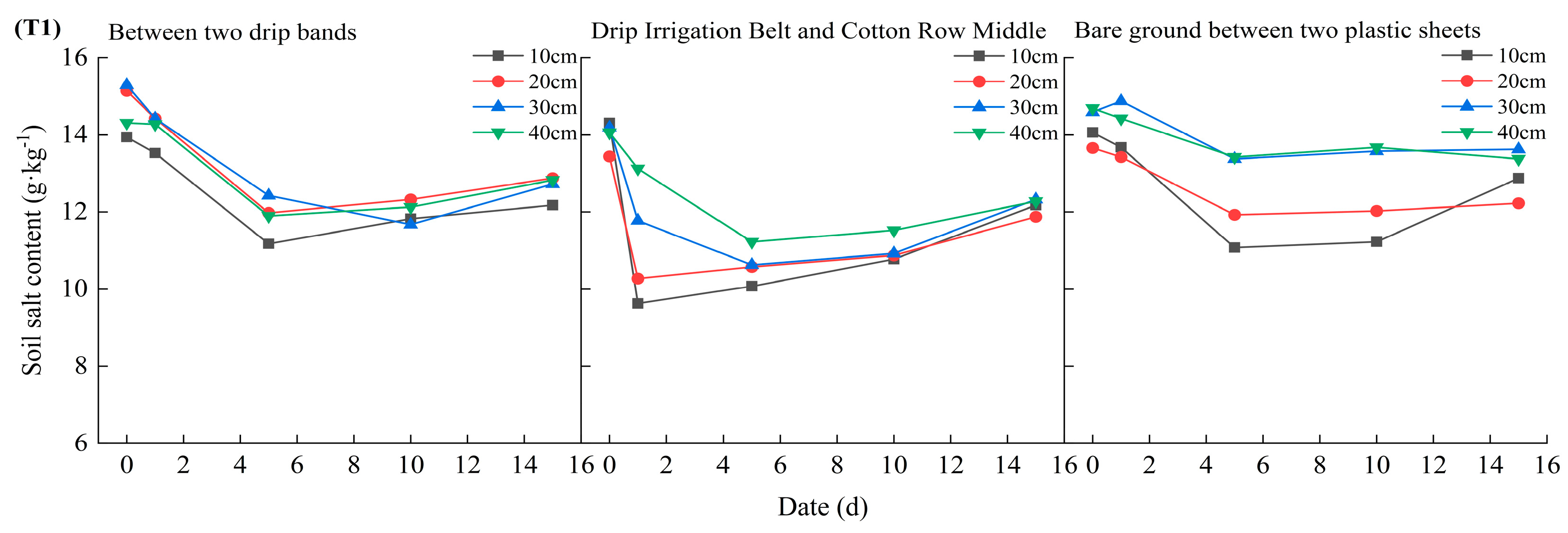
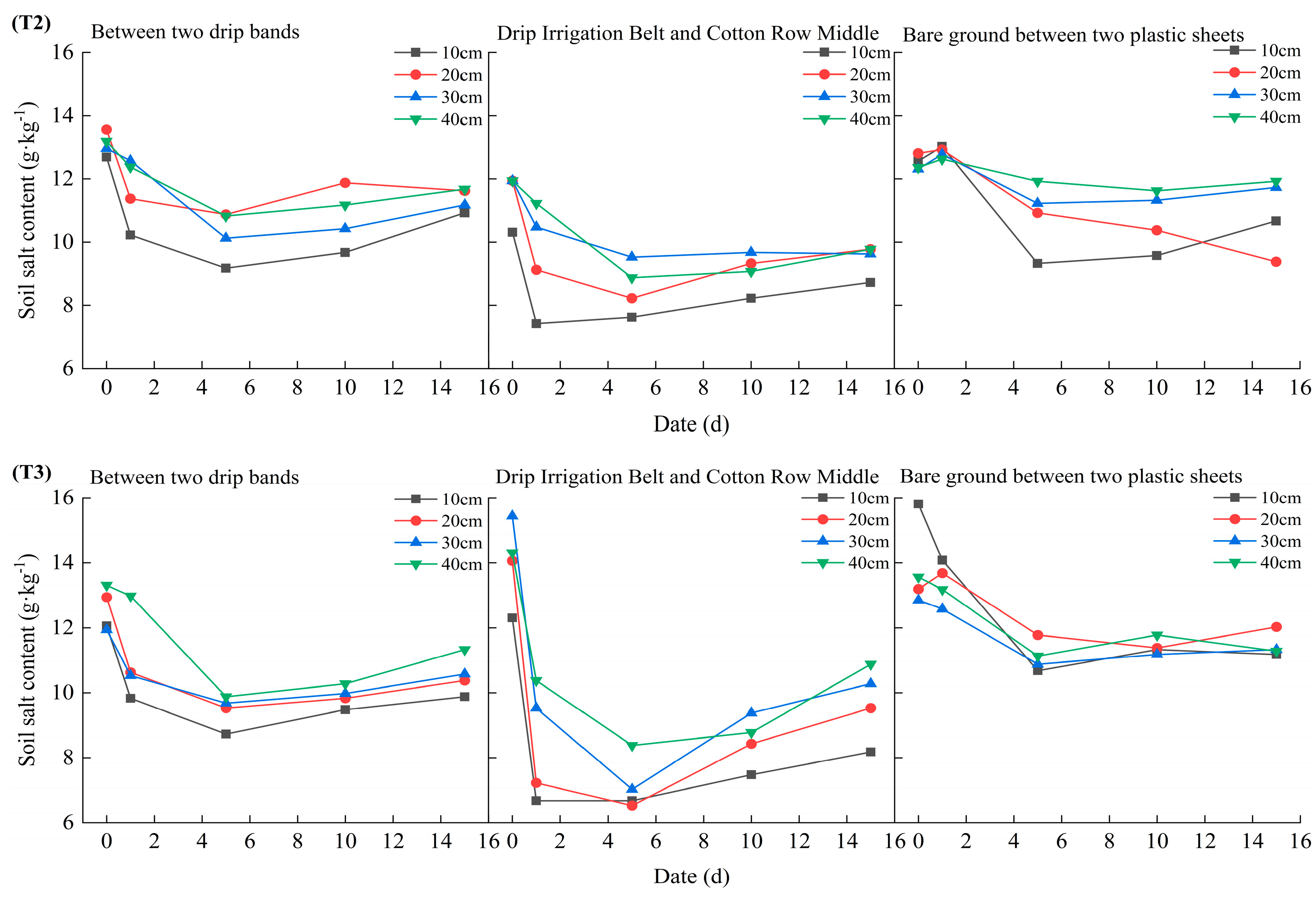
3.1.2. Characteristics of Soil Salt Distribution
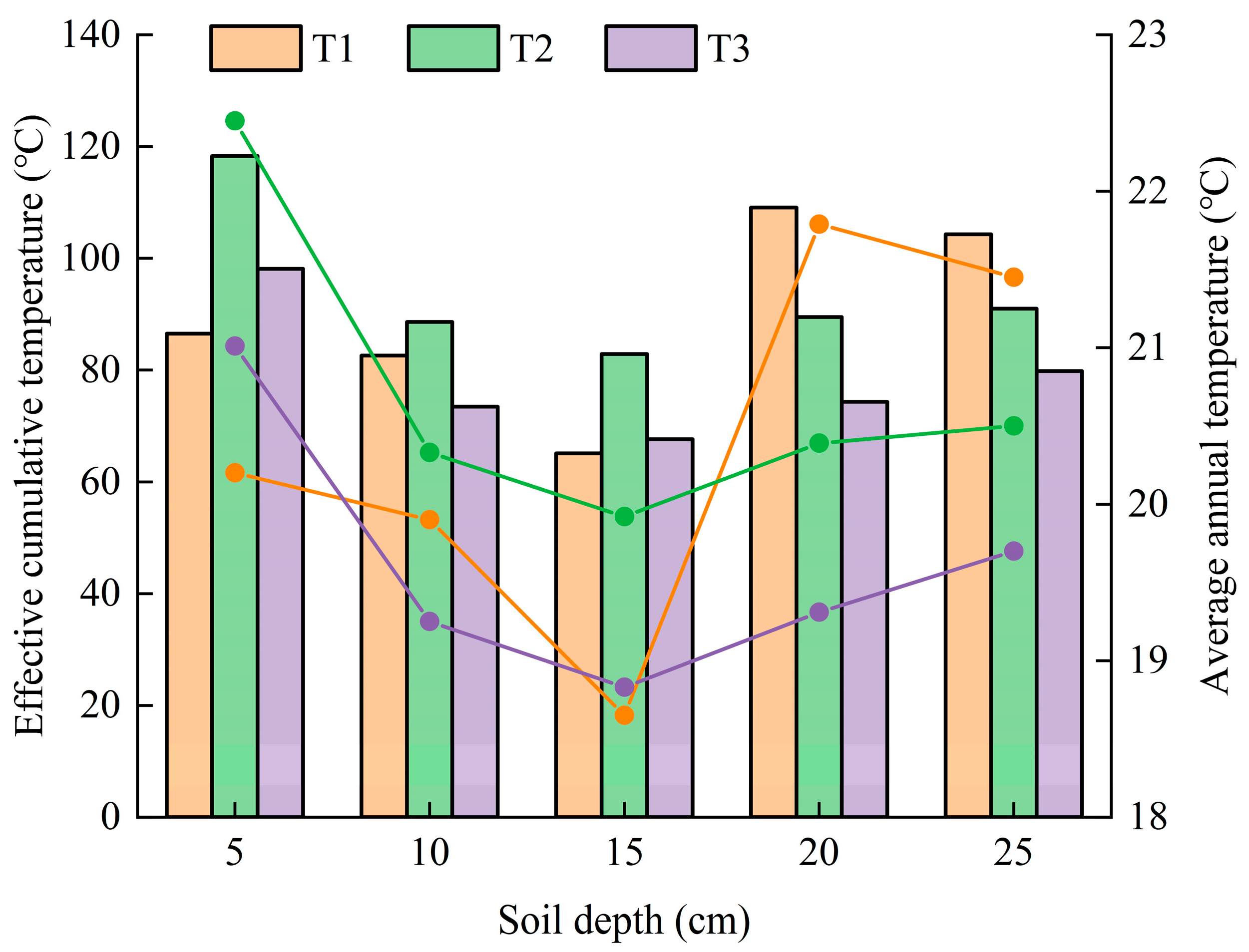
3.1.3. Characteristics of Soil Temperature Changes
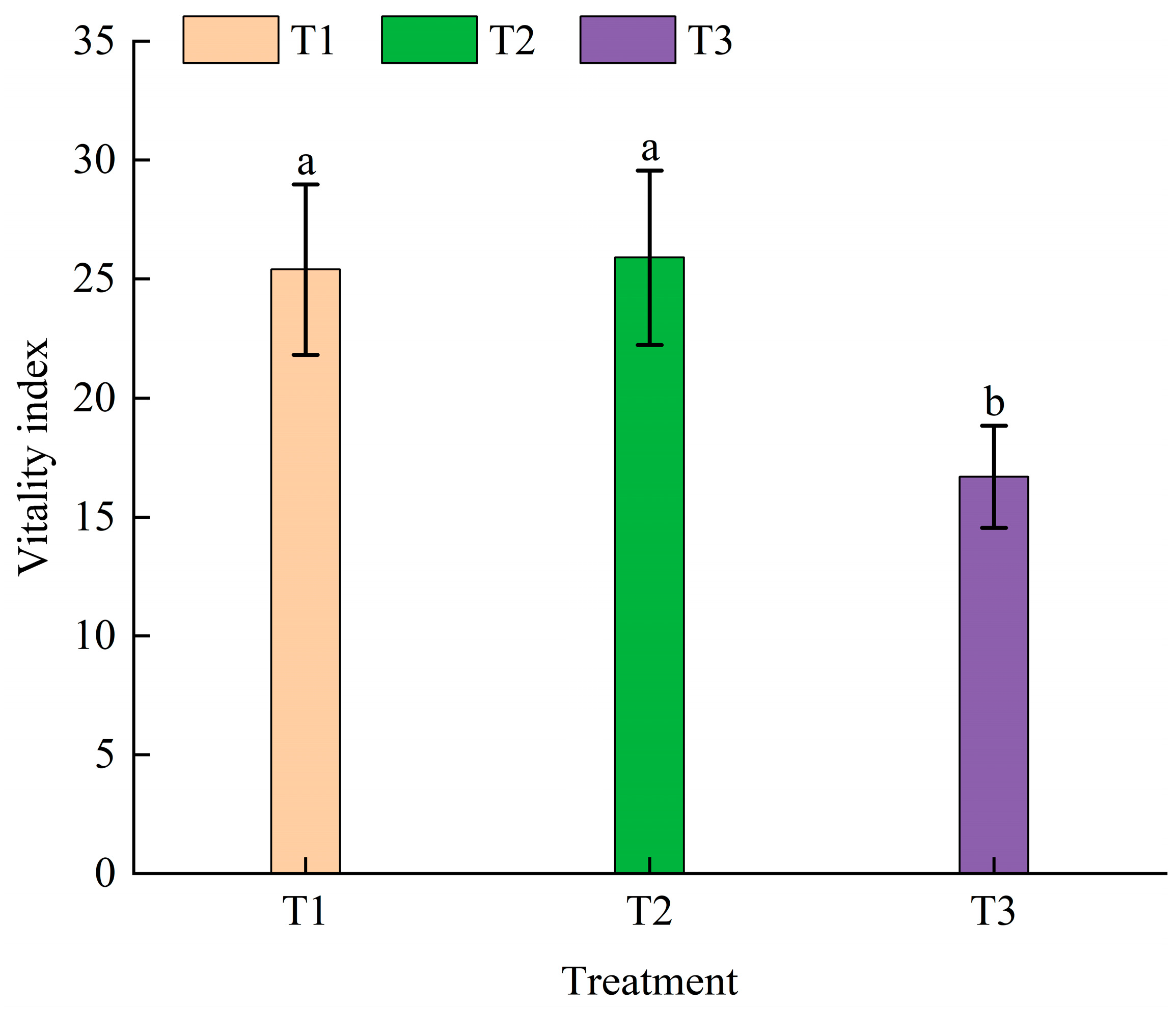
3.1.4. Analysis of Cotton Emergence Situation
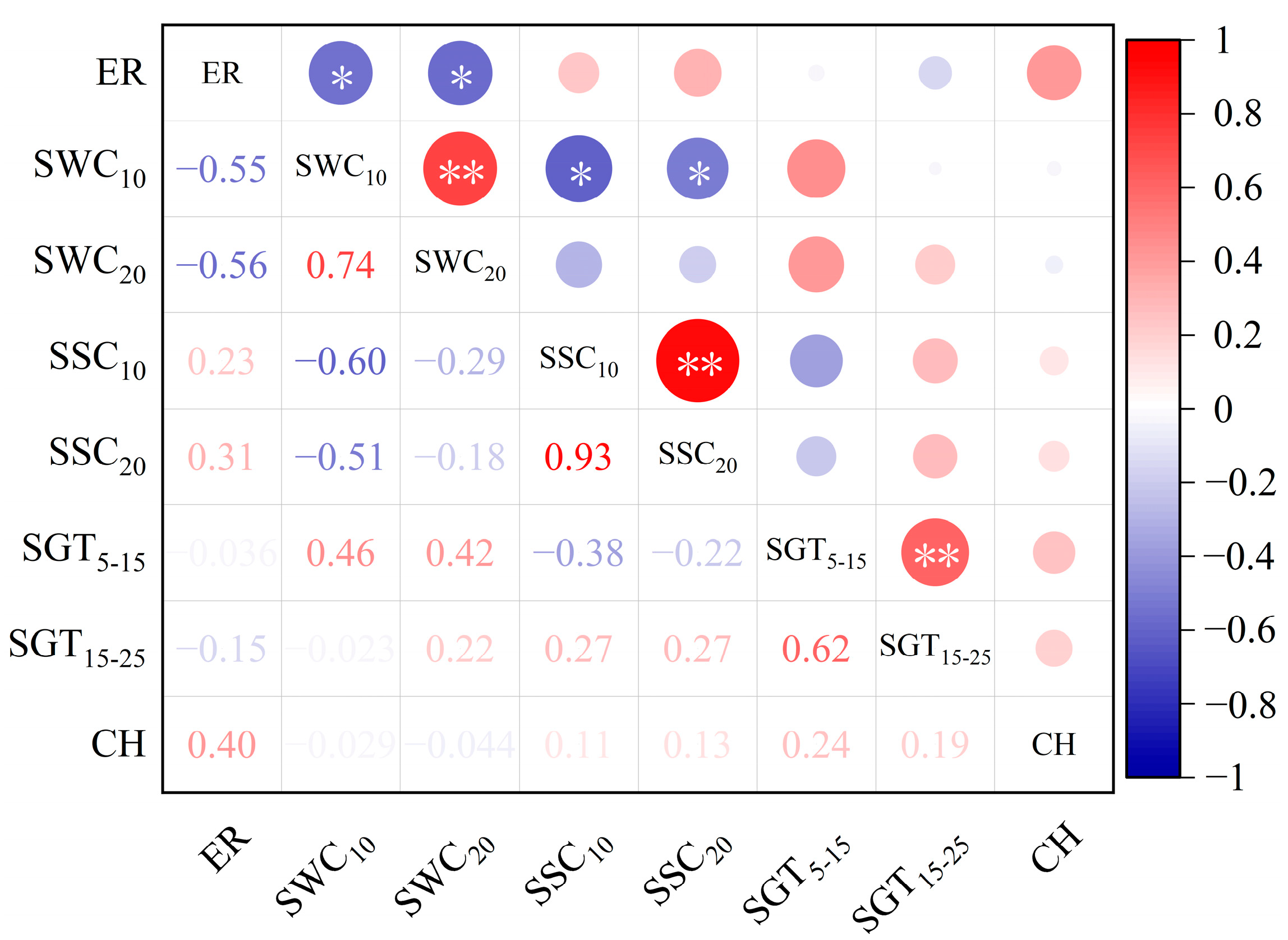
3.1.5. Analysis of Factors Affecting Cotton Emergence Rate
3.2. HYDRUS-2D Model Calibration and Validation
3.2.1. Determination of Model Hydraulic Parameters
3.2.2. Model Calibration and Validation
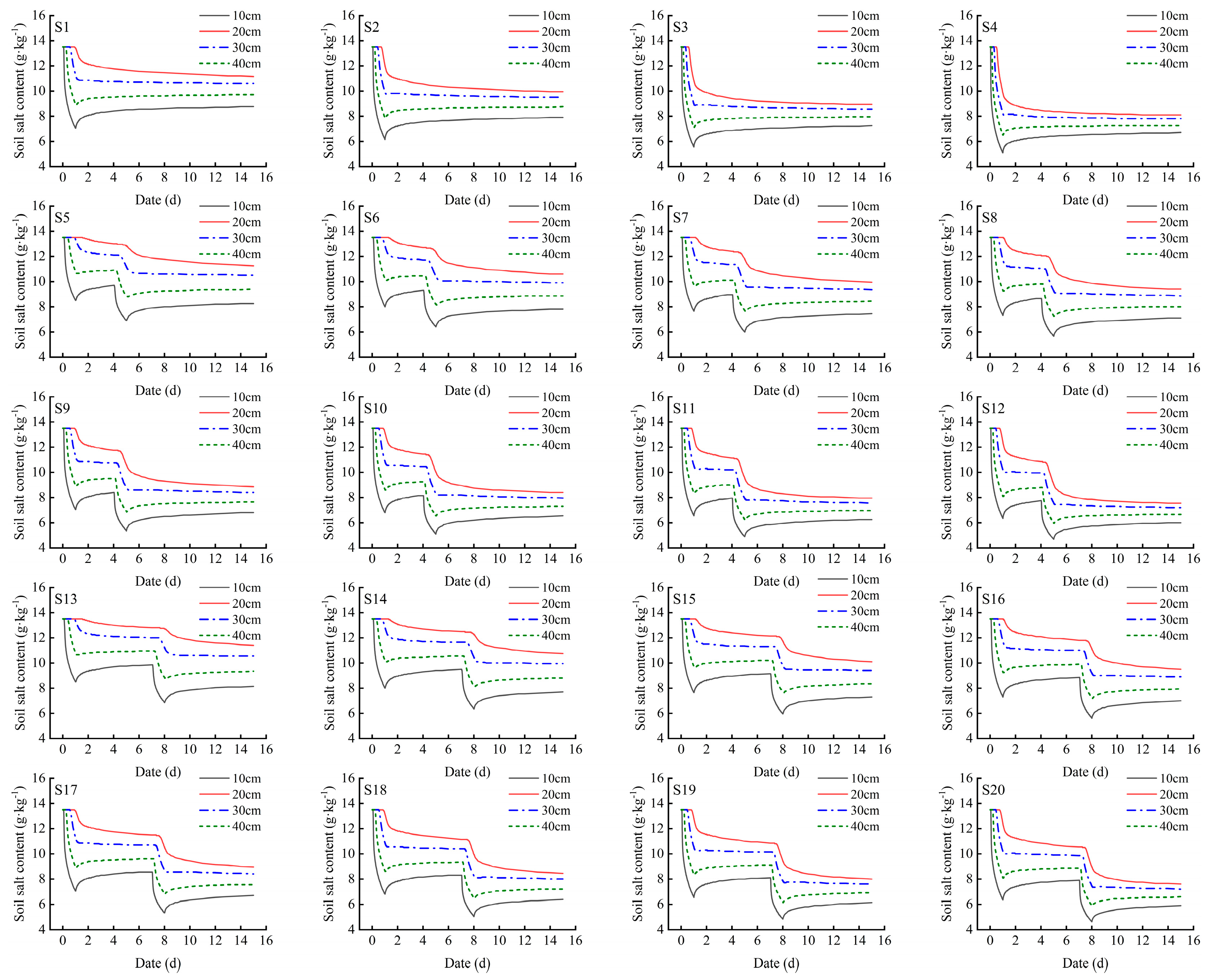
3.3. Simulation of Soil Water and Salt Transport Processes under Different Scenarios
3.3.1. Model Scenario Simulation Settings
3.3.2. Simulation of Soil Water Transport Processes
3.3.3. Simulation of Soil Salt Transport Process
3.4. Optimization of Drip Irrigation Regime for Cotton with “Dry Sowing and Wet Emergence”
4. Discussion
4.1. The Effect of Soil Water, Heat, and Salt on Cotton Emergence Rate under Different Water Amounts
4.2. Simulation of Soil Water and Salt Distribution Patterns Based on the HYDRUS-2D Model
4.3. Optimization and Effect Analysis of “Dry Sowing and Wet Emergence” Irrigation Regime for Cotton
5. Conclusions
- (1)
- The average water content of the soil in the 0–40 cm soil layer within the membrane showed a trend of increasing and then decreasing over time, while the average water content of the soil in the 0–40 cm soil layer in the bare ground between the membranes showed a gradual increase over time; the average salt content of the soil in the 0–40 cm layer within the membrane and in the bare ground between the membranes showed a general trend of decreasing and then increasing over time.
- (2)
- The seedling vigor index of the T2 treatment was 25.9, and the highest cotton seedling emergence rate was 67.79%. The seedling vigor index of the T3 treatment was only 16.7, and the lowest cotton seedling emergence rate was 63.93%. Therefore, the appropriate reduction in irrigation amount can moderately improve the seedling emergence rate and seedling emergence index of cotton seeds.
- (3)
- In the 20 scenario simulations, under the same irrigation amount, the average soil water content in the 10–40 cm soil layer was as follows: irrigation interval of 7 days > irrigation interval of 4 days > irrigation interval of 14 days. The average soil salt content in the 10–40 cm soil layer was as follows: irrigation interval of 14 days > irrigation interval of 7 days > irrigation interval of 4 days.
- (4)
- By establishing the regression equations of the seedling emergence rate with the soil water content and soil salt content under the planting pattern of one film, three tubes, and three rows, it is recommended to choose the irrigation regime of “dry sowing and wet emergence” for cotton with medium–low water (300–450 m3/hm2) at 14-day intervals, or medium–low water (300–375 m3/hm2) at 7-day intervals, to achieve a higher seedling emergence rate.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Jans, Y.; von Bloh, W.; Schaphoff, S.; Müller, C. Global cotton production under climate change-Implications for yield and water consumption. Hydrol. Earth Syst. Sci. 2021, 25, 2027–2044. [Google Scholar] [CrossRef]
- Meng, Z.; Ran, Y.Q. Current issues and countermeasures for the high-quality development of Xinjiang’s cotton industry. South China Agric. 2023, 17, 240–243+247. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Zhang, W.T.; Tian, C.Y.; Mai, W.X. Application, Problems and Solution Strategies of “Dry Sowing and Wet Emergence” Planting Technology of Cotton in Southern Xinjian. Chin. Agric. Sci. Bull. 2024, 40, 62–65. [Google Scholar]
- Xiao, C.; Li, M.; Fan, J.L.; Zhang, F.C.; Li, Y.; Cheng, H.L.; Li, Y.P.; Hou, X.H.; Chen, J.Q. Salt leaching with brackish water during growing season improves cotton growth and productivity, water use efficiency and soil sustainability in southern Xinjiang. Water 2021, 13, 2602. [Google Scholar] [CrossRef]
- Chen, X.L.; Sun, C.M.; Liu, P. The practice of cotton “sowing drily and emerging wet” technology in Korla, Xinjiang. China Cotton 2021, 48, 41–42+45. [Google Scholar]
- Wang, J.S.; Wang, Y. Effect of Dry Seeding and Wet Budding of Cotton with Drip Irrigation under plastic film. J. Tarim Univ. 2006, 1, 77–78. [Google Scholar] [CrossRef]
- Chen, S.S.; Pei, D.; Wang, Z.H.; Zhang, X.Y.; Chen, S.Y.; Sun, H.Y. Influence of irrigation modes on water consumption and yield of cotton with drip irrigation under plastic mulch in North China Plain. Agric. Res. Arid Areas 2005, 6, 30–35. [Google Scholar] [CrossRef]
- Li, L.; Dong, H.L.; Ma, Y.Z.; Li, P.C.; Li, C.M.; Zhang, N.; Wan, S.M.; Xu, W.X. Effects of Row Spacing Patterns on the Growth, Yield and Quality of Machine-picked Cotton. Xinjiang Agric. Sci. 2020, 57, 713–721. [Google Scholar]
- Zheng, M.; Bai, Y.G.; Zhang, J.H.; Liu, H.B.; Wang, B.; Xiao, J.; Ding, Y.; Han, Z.Y. Effects of irrigation quantity and irrigation frequency of dry sowing and wet seedling on soil compaction, water salt distribution and seedling emergence in cotton fields. Agric. Res. Arid Areas 2022, 40, 100–107. [Google Scholar] [CrossRef]
- Shao, J.J.; Li, P.C.; Zheng, C.S.; Sun, M.; Feng, W.N.; Zhang, X.L.; Dong, H.L. Effects of salt stress on emergence rate and development of cotton seedlings under dry-sowing and wet-emergence planting mode. Cotton Sci. 2023, 35, 288–301. [Google Scholar] [CrossRef]
- Dong, X.; Chai, Z.P.; Bai, Y.G.; Liu, H.B.; Ding, Y.; Xiao, J. Effect of Different “Dry Broadcast and Wet Surface” Planting Method on Soil Water, Heat, Salt and Yield in Cotton Fields in Southern Xinjiang. Water Sav. Irrig. 2024, 2, 33–40. [Google Scholar] [CrossRef]
- Ding, Y.; Zhang, J.H.; Bai, Y.G.; Zhao, J.H.; Zheng, M.; Liu, H.B.; Xiao, J.; Han, Z.Y. Study on the effects of different water treatments on the emergence rate of cotton. Xinjiang Agric. Sci. 2023, 60, 2380–2389. [Google Scholar] [CrossRef]
- Zhang, M.Z.; Li, Q.H.; Shi, X.L. Soil Science and Agronomy; China Water & Power Press: Beijing, China, 2007. [Google Scholar]
- Du, M.Q.; Wu, R.J.; Yang, M.F. Comparative Study of Drying Weighing Method and TDR Method for Observing Soil Moisture. Technol. Soil Water Conserv. 2018, 4, 7–9. [Google Scholar] [CrossRef]
- Chen, W.; Li, M.; Li, Q. The Influence of Winter Irrigation Amount on the Characteristics of Water and Salt Distribution and WUE in Different Saline-Alkali Farmlands in Northwest China. Sustainability 2023, 15, 15428. [Google Scholar] [CrossRef]
- Xu, H.X.; Yang, W.H.; Wang, Y.Q.; Zhou, D.Y.; Xia, J.Y.; Feng, X.A. Effect of temperature on seed vigor of cotton. China Cotton 2004, 2, 15–16. [Google Scholar] [CrossRef]
- Yi, G.; Quan, J.W.; Kang, W. Spring irrigation with magnetized water affects soil water-salt distribution, emergence, growth, and photosynthetic characteristics of cotton seedlings in Southern Xinjiang, China. BMC Plant Biol. 2023, 23, 174. [Google Scholar] [CrossRef]
- Nasr, H.M.; Selles, F. Seedling emergence as influenced by aggregate size, bulk density, and penetration resistance of the seedbed. Soil Till. Res. 1995, 34, 61–76. [Google Scholar] [CrossRef]
- Simunek, J.; Sejna, M.; van, G. The Hydrus-2D Software Package for Simulating Two-Dimensional Movement of Water, Heat, and Multiple Solutes in Variably Saturated Media; Version 2.0, IGWMC-TPS-53; International Ground Water Modeling Center, Colorado School of Mines: Golden, CA, USA, 1999; p. 251. [Google Scholar]
- Li, Z.R.; Hu, D.; You, G.D. Experiment and simulation on salt removing of drip irrigation under the film in cotton field. J. Shihezi Univ. 2018, 36, 376–384. [Google Scholar] [CrossRef]
- Wang, S.X.; Dong, X.G.; Wu, B.; Yang, P.N. Numerical simulation and control mode of soil water and salt movement in arid salinization region. Trans. Chin. Soc. Agric. Eng. 2012, 28, 142–148. [Google Scholar] [CrossRef]
- Wu, M.H.; Wang, Y. Characteristics and influencing factors of crop water demand and yield in Shache irrigation area of Xin-jiang. Chin. J. Ecol. 2023, 42, 1858–1868. [Google Scholar] [CrossRef]
- Simunek, J.; Sejna, M.; van Genuchten, T. HYDRUS-2D. 1999. Available online: https://www.pc-progress.com/en/Default.aspx?hydrus-2d (accessed on 5 September 2024).
- Van Genuchten, M.T. Closed-form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Phogat, V.; Pitt, T.; Stevens, R.M.; Cox, J.W.; Šimůnek, J.; Petrie, P.R. Assessing the role of rainfall redirection techniques for arresting the land degradation under drip irrigated grapevines. J. Hydrol. 2020, 587, 125000. [Google Scholar] [CrossRef]
- Xu, M.; Li, J.L.; Ye, F.M.; Zhu, H.; Wang, Z.S. Effects of Temperature and Humidity on Cotton Seed Germination. J. Agric. 2022, 12, 10. [Google Scholar]
- Du, X.J.; Yan, B.W.; Xu, K.; Wang, S.Y.; Gao, Z.D.; Ren, X.Q.; Hu, S.W.; Yun, W.J. Research Progress on Water-salt Transport Theories and Models in Saline-alkali Soil. Chin. J. Soil Sci. 2021, 52, 713–721. [Google Scholar] [CrossRef]
- Zhang, W.; Lv, X.; Li, L.H.; Liu, J.G.; Sun, Z.J.; Zhang, X.W.; Yang, Z.P. Salt transfer law for cotton field with drip irrigation under the plastic mulch in Xinjiang Region. Trans. CSAE 2008, 24, 15–19. [Google Scholar]
- Zhang, X.M.; Guo, K.; Xie, Z.X.; Fen, X.H.; Liu, X.J. Effect of frozen saline water irrigation in winter on soil salt and water dynamics, germination and yield of cotton in coastal soils. Chin. J. Eco-Agric. 2012, 20, 1310–1314. [Google Scholar] [CrossRef]
- Yu, Q.; Zhang, H.L.; Chai, Q.; Li, Z.J.; Tang, S.J. Effects of different soil moistures on emergence and root of Kentucky bluegrass. Pratacultural Sci. 2013, 30, 1338–1343. [Google Scholar]
- Cao, H.X.; Kang, S.Z.; He, H. Effects of evaporation and irrigation frequency on soil water distribution. Trans. Chin. Soc. Agric. Eng. 2003, 6, 1–4. [Google Scholar] [CrossRef]
- Bai, X.E.; Ai, Y.D.; Yang, H.S.; Ge, Y.X.; Xing, Z.; Hu, X.T.; Zhou, S.W. Effects of Different Initial Water Content and Dripper Discharge on Wetted Body Characteristic of Drip Irrigation. J. Irrig. Drain. 2019, 38, 73–78. [Google Scholar] [CrossRef]
- Wu, X. Characteristics of Salt and Water Movement and Numerical Simulation in Greenhouse Soil under Different Irrigation Frequencies. Sichuan Agricultural University. 2014. Available online: https://kns.cnki.net/kcms2/article/abstract?v=QenloEQs_R8N3SMS8ribVPkoDzm2RACZNQnYjmuvZemPk8Is55XboPbrNiJOwXQSPJCmVuBsZ-CXmmTrkxIEg4Yc41qP55fygRghzB8JxlHjQHlMcgn78u7UsfHnDdEpVNkfs8r7mEJknpiwTlTao7X085fhSNo34cr0cRtNkVM198Kft7IGD4ZXcufRgwQ1IHqgXfIxmCU=&uniplatform=NZKPT&language=CHS (accessed on 5 September 2024).
- Liu, T.; Wu, X.; Zheng, Z.C.; Li, Y.X. Effects of Irrigation Frequency on Water and Salt Movement in Greenhouse Soil. J. Ecol. Rural Environ. 2016, 32, 622–631. [Google Scholar] [CrossRef]
- Tan, J.L.; Wang, X.N.; Tian, J.C.; Su, X.L. Effect of gravel-sand mulching on movements of soil water and salts under different amounts of brackish water. Trans. Chin. Soc. Agric. Eng. 2018, 34, 100–108. [Google Scholar] [CrossRef]
- Hu, Q.J.; Zheng, M. Study on the Convelation Between Soil Environment of “Dry Sowing and Wet Germination” and Cotton Emergence Rate in Southern Xinjiang. Heilongjiang Agric. Sci. 2023, 10, 6–12. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, J.Z.; Li, B.Z.; Wang, Z.H.; Li, W.H. Response characteristics and numerical simulation of soil water and salt dynamics in different salinized cotton seedling stage to submembrane drip irrigation. J. Shihezi Univ. 2022, 40, 204–212. [Google Scholar] [CrossRef]
- Wang, F.; Lv, D.S.; Wang, Z.H.; Zhang, J.Z.; Liu, J.; Wen, Y.; Qin, C.; Hu, G.R. Effects of water-salt coupling on seeding emergence rate and seeding growth of cotton under drip irrigation. Water Sav. Irrig. 2023, 3, 91–97. [Google Scholar] [CrossRef]
- Zhong, Z.B.; He, S.; Zhang, W.H.; Zhou, J.W.; Zhen, G.Y.; Ma, J.Y.; Cheng, H.; Shi, C.; Zhang, X. Study on cotton formation and water salt transportation under salinization. Xinjiang Agric. Sci. 2023, 60, 2390–2395. [Google Scholar] [CrossRef]





| Soil Depth (cm) | Bulk Density (g/cm3) | Particle Mass Fraction (%) | Saturated Water Content (cm3·cm3) | Field Water Holding Capacity (cm3·cm3) | ||
|---|---|---|---|---|---|---|
| Sand (2–0.05 mm) | Silt (0.05–0.002 mm) | Clay (<0.002 mm) | ||||
| 0–10 | 1.46 | 71.16 | 26.10 | 2.79 | 44.01 | 33.01 |
| 10–20 | 1.46 | 73.80 | 23.65 | 2.55 | 43.30 | 32.91 |
| 20–30 | 1.47 | 73.47 | 24.00 | 2.53 | 40.09 | 30.87 |
| 30–40 | 1.47 | 74.35 | 23.22 | 2.43 | 40.47 | 30.35 |
| Treatment | Logistic Equation | R2 | RMSE | MAE |
|---|---|---|---|---|
| T1 | Y = 65.93/(1 + 16.872e−0.867t) | 0.962 | 4.30% | 3.67% |
| T2 | Y = 67.79/(1 + 19.323e−1.027t) | 0.981 | 3.05% | 2.61% |
| T3 | Y = 63.93/(1 + 24.747e−0.931t) | 0.987 | 2.54% | 2.18% |
| Soil Depth (cm) | Soil Texture | Bulk Density (g/cm3) | θr (cm3/cm3) | θs (cm3/cm3) | α (cm) | n | Ks | L |
|---|---|---|---|---|---|---|---|---|
| 0~10 | Sandy loam | 1.46 | 0.092 | 0.455 | 0.037 | 1.525 | 58.60 | 0.5 |
| 10~20 | Sandy loam | 1.46 | 0.083 | 0.449 | 0.045 | 1.553 | 64.29 | 0.5 |
| 20~30 | Sandy loam | 1.47 | 0.080 | 0.419 | 0.045 | 1.577 | 66.46 | 0.5 |
| 30~40 | Sandy loam | 1.47 | 0.078 | 0.418 | 0.045 | 1.600 | 63.84 | 0.5 |
| Parameters | Evaluation Indicators | Model Calibration (T2 Treatment) | |||
|---|---|---|---|---|---|
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil water content | R2 | 0.90 | 0.90 | 0.90 | 0.86 |
| RMSE (cm3·cm−3) | 1.89 | 1.96 | 1.94 | 2.37 | |
| MAE (cm3·cm−3) | 1.71 | 1.83 | 1.71 | 1.99 | |
| Parameters | Evaluation indicators | Model validation (T1 treatment) | |||
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil water content | R2 | 0.87 | 0.87 | 0.92 | 0.89 |
| RMSE (cm3·cm−3) | 1.70 | 1.99 | 2.12 | 2.10 | |
| MAE (cm3·cm−3) | 1.41 | 1.64 | 1.95 | 1.92 | |
| Parameters | Evaluation indicators | Model validation (T3 treatment) | |||
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil water content | R2 | 0.87 | 0.87 | 0.92 | 0.89 |
| RMSE (cm3·cm−3) | 1.70 | 1.99 | 2.12 | 2.10 | |
| MAE (cm3·cm−3) | 1.41 | 1.64 | 1.95 | 1.92 | |
| Parameters | Evaluation Indicators | Model Calibration (T2 Treatment) | |||
|---|---|---|---|---|---|
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil salt content | R2 | 0.86 | 0.83 | 0.85 | 0.79 |
| RMSE (g/kg) | 1.30 | 1.51 | 1.24 | 1.07 | |
| MAE (g/kg) | 1.21 | 1.40 | 1.13 | 0.92 | |
| Parameters | Evaluation indicators | Model validation (T1 treatment) | |||
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil salt content | R2 | 0.80 | 0.92 | 0.86 | 0.81 |
| RMSE (g/kg) | 1.33 | 1.01 | 0.64 | 0.67 | |
| MAE (g/kg) | 1.23 | 0.87 | 0.54 | 0.65 | |
| Parameters | Evaluation indicators | Model validation (T3 treatment) | |||
| 0–10 cm | 10–20 cm | 20–30 cm | 30–40 cm | ||
| Soil salt content | R2 | 0.88 | 0.89 | 0.83 | 0.79 |
| RMSE (g/kg) | 1.17 | 1.04 | 1.00 | 0.95 | |
| MAE (g/kg) | 1.10 | 0.89 | 0.90 | 0.72 | |
| Treatment | Single Irrigation Amount (m3/hm2) | Irrigation Frequency (times) | Irrigation Interval (day) | Total Irrigation Amount (m3/hm2) |
|---|---|---|---|---|
| S1 | 300 | 1 | 14 | 300 |
| S2 | 450 | 1 | 14 | 450 |
| S3 | 600 | 1 | 14 | 600 |
| S4 | 750 | 1 | 14 | 750 |
| S5 | 150 | 2 | 4 | 300 |
| S6 | 187.5 | 2 | 4 | 375 |
| S7 | 225 | 2 | 4 | 450 |
| S8 | 262.5 | 2 | 4 | 525 |
| S9 | 300 | 2 | 4 | 600 |
| S10 | 337.5 | 2 | 4 | 675 |
| S11 | 375 | 2 | 4 | 750 |
| S12 | 412.5 | 2 | 4 | 825 |
| S13 | 150 | 2 | 7 | 300 |
| S14 | 187.5 | 2 | 7 | 375 |
| S15 | 225 | 2 | 7 | 450 |
| S16 | 262.5 | 2 | 7 | 525 |
| S17 | 300 | 2 | 7 | 600 |
| S18 | 337.5 | 2 | 7 | 675 |
| S19 | 375 | 2 | 7 | 750 |
| S20 | 412.5 | 2 | 7 | 825 |
| Treatment | Emergence Rate y1 (%) | Emergence Rate y2 (%) | Emergence Rate y (%) | Treatment | Emergence Rate y1 (%) | Emergence Rate y2 (%) | Emergence Rate y (%) |
|---|---|---|---|---|---|---|---|
| S1 | 72.94 | 64.12 | 70.85 | S11 | 32.96 | 26.09 | 31.33 |
| S2 | 71.08 | 56.29 | 67.57 | S12 | 30.48 | 21.40 | 28.33 |
| S3 | 69.71 | 48.38 | 64.65 | S13 | 71.20 | 63.75 | 69.43 |
| S4 | 68.98 | 40.34 | 62.19 | S14 | 69.48 | 60.31 | 67.31 |
| S5 | 57.61 | 56.07 | 57.24 | S15 | 67.79 | 56.40 | 65.09 |
| S6 | 52.01 | 50.48 | 51.65 | S16 | 66.16 | 52.85 | 63.01 |
| S7 | 46.86 | 45.31 | 46.49 | S17 | 64.63 | 49.18 | 60.97 |
| S8 | 42.60 | 40.03 | 41.99 | S18 | 63.43 | 45.60 | 59.20 |
| S9 | 38.96 | 35.11 | 38.05 | S19 | 62.24 | 41.59 | 57.34 |
| S10 | 35.72 | 30.72 | 34.54 | S20 | 61.20 | 38.10 | 55.72 |
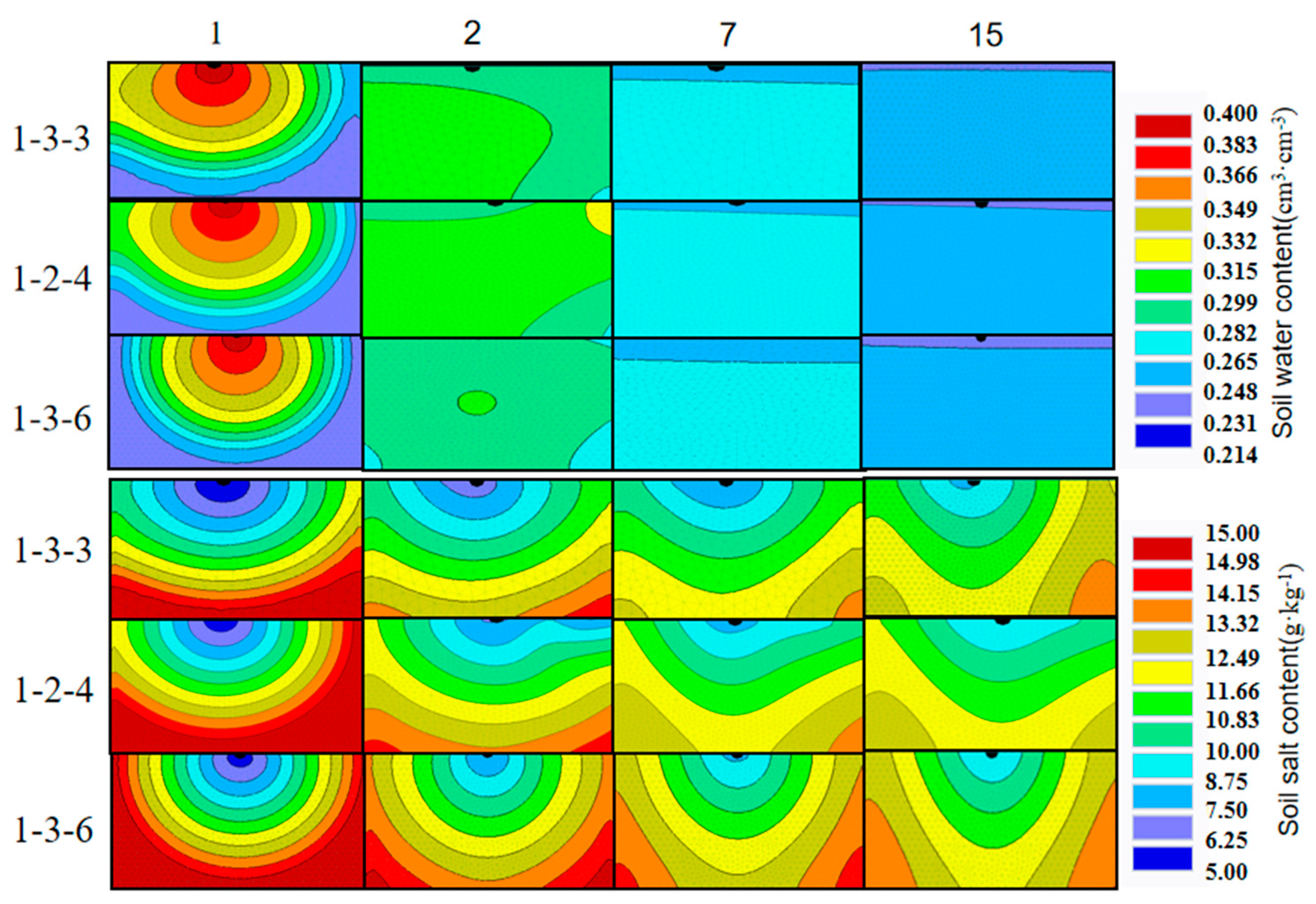
| Planting Patterns | Treatment | Emergence Rate y1 (%) | Emergence Rate y2 (%) | Emergence Rate y (%) |
|---|---|---|---|---|
| 1 film 3 tubes 3 rows | S2 | 71.08 | 56.26 | 67.57 |
| 1 film 2 tubes 4 rows | S2 | 69.80 | 54.73 | 66.23 |
| 1 film 3 tubes 6 rows | S2 | 70.14 | 54.50 | 66.44 |
| 1 film 3 tubes 3 rows | S13 | 71.20 | 63.75 | 69.43 |
| 1 film 2 tubes 4 rows | S13 | 69.93 | 61.56 | 67.95 |
| 1 film 3 tubes 6 rows | S13 | 69.97 | 61.39 | 67.94 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, H.; Wang, C. Experimental Study on the Application of “Dry Sowing and Wet Emergence” Drip Irrigation Technology with One Film, Three Tubes, and Three Rows. Agronomy 2024, 14, 2406. https://doi.org/10.3390/agronomy14102406
Wang H, Wang C. Experimental Study on the Application of “Dry Sowing and Wet Emergence” Drip Irrigation Technology with One Film, Three Tubes, and Three Rows. Agronomy. 2024; 14(10):2406. https://doi.org/10.3390/agronomy14102406
Chicago/Turabian StyleWang, Hongxin, and Chunxia Wang. 2024. "Experimental Study on the Application of “Dry Sowing and Wet Emergence” Drip Irrigation Technology with One Film, Three Tubes, and Three Rows" Agronomy 14, no. 10: 2406. https://doi.org/10.3390/agronomy14102406
APA StyleWang, H., & Wang, C. (2024). Experimental Study on the Application of “Dry Sowing and Wet Emergence” Drip Irrigation Technology with One Film, Three Tubes, and Three Rows. Agronomy, 14(10), 2406. https://doi.org/10.3390/agronomy14102406





