Vibration Response of Walnuts under Vibration Harvesting
Abstract
:1. Introduction
2. Theoretical Model of the Vibration Response of Walnuts
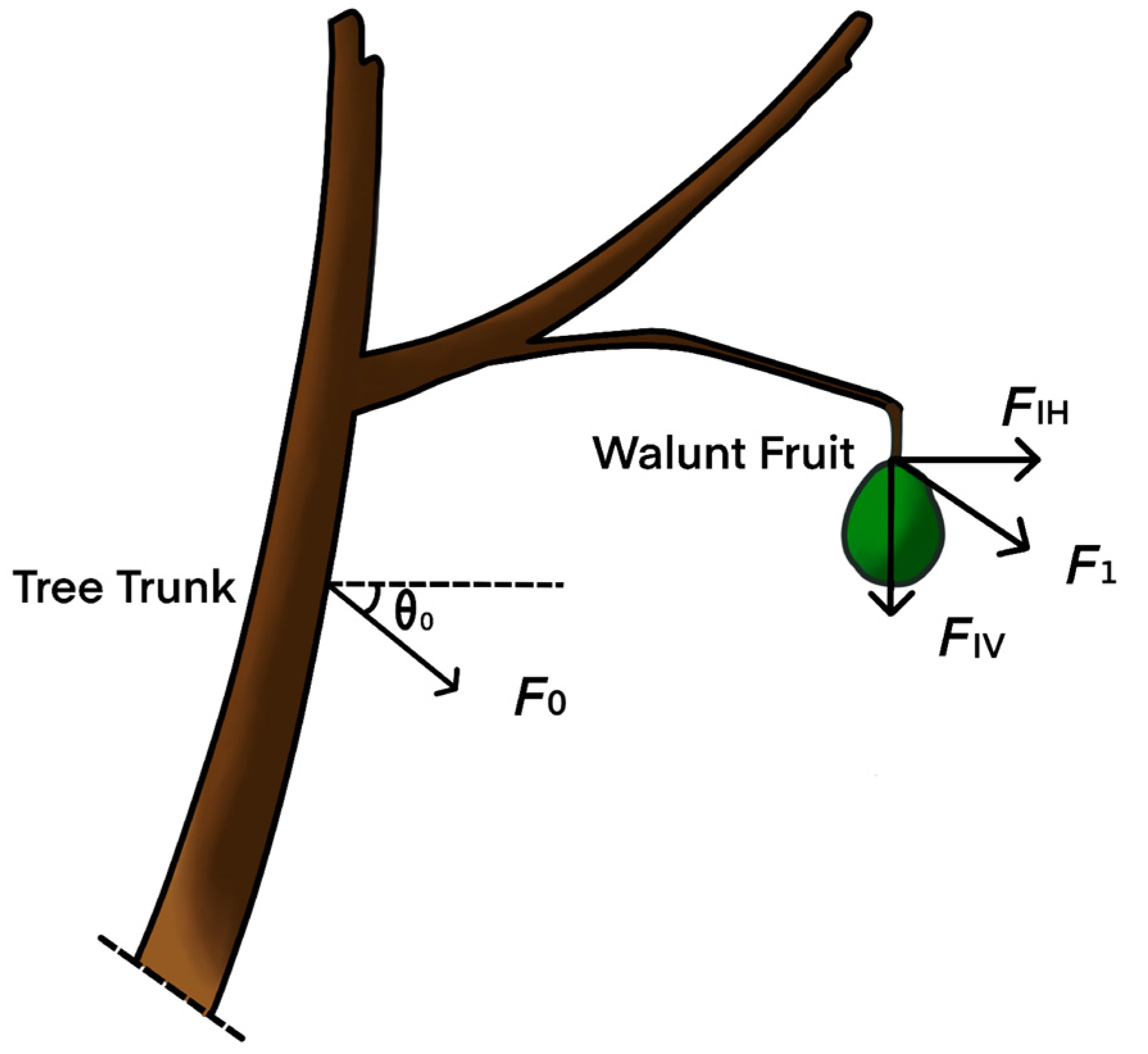
2.1. Force Analysis
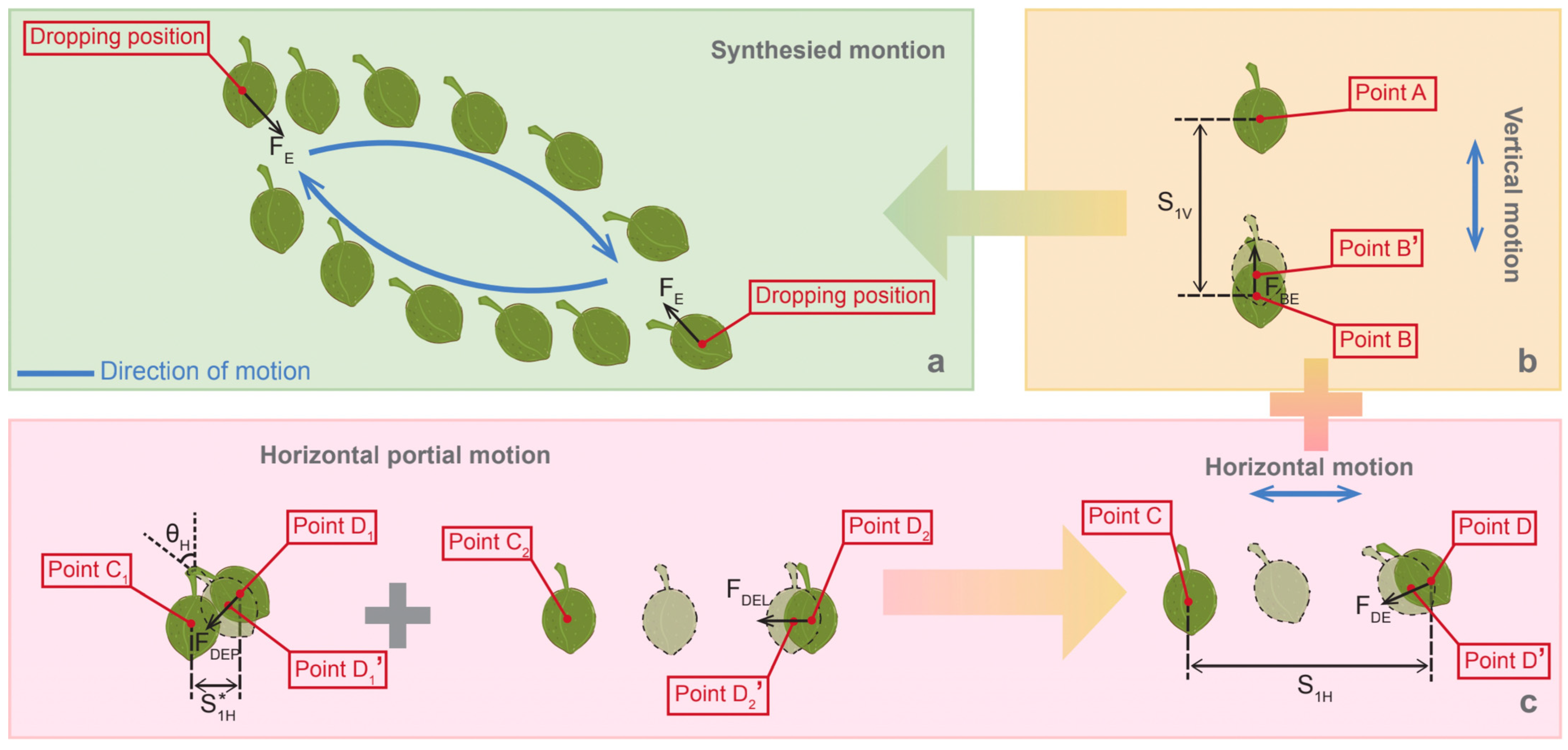
2.2. Vibration Response in the Vertical Direction
2.3. Vibration Response in the Horizontal Direction
2.4. Synthesized Motion of the Vibration Response
3. Experimental Setup
4. Results and Discussion
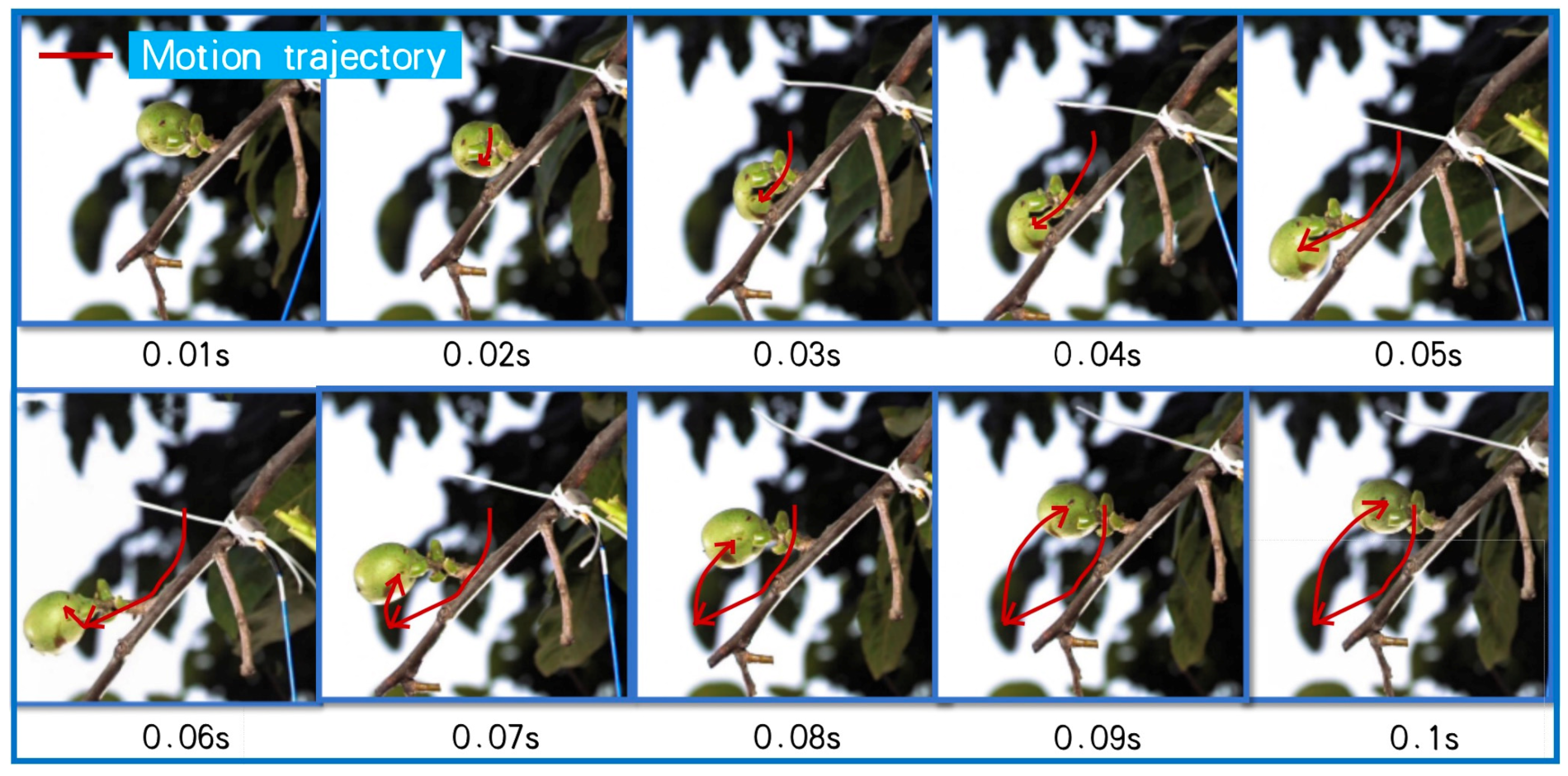
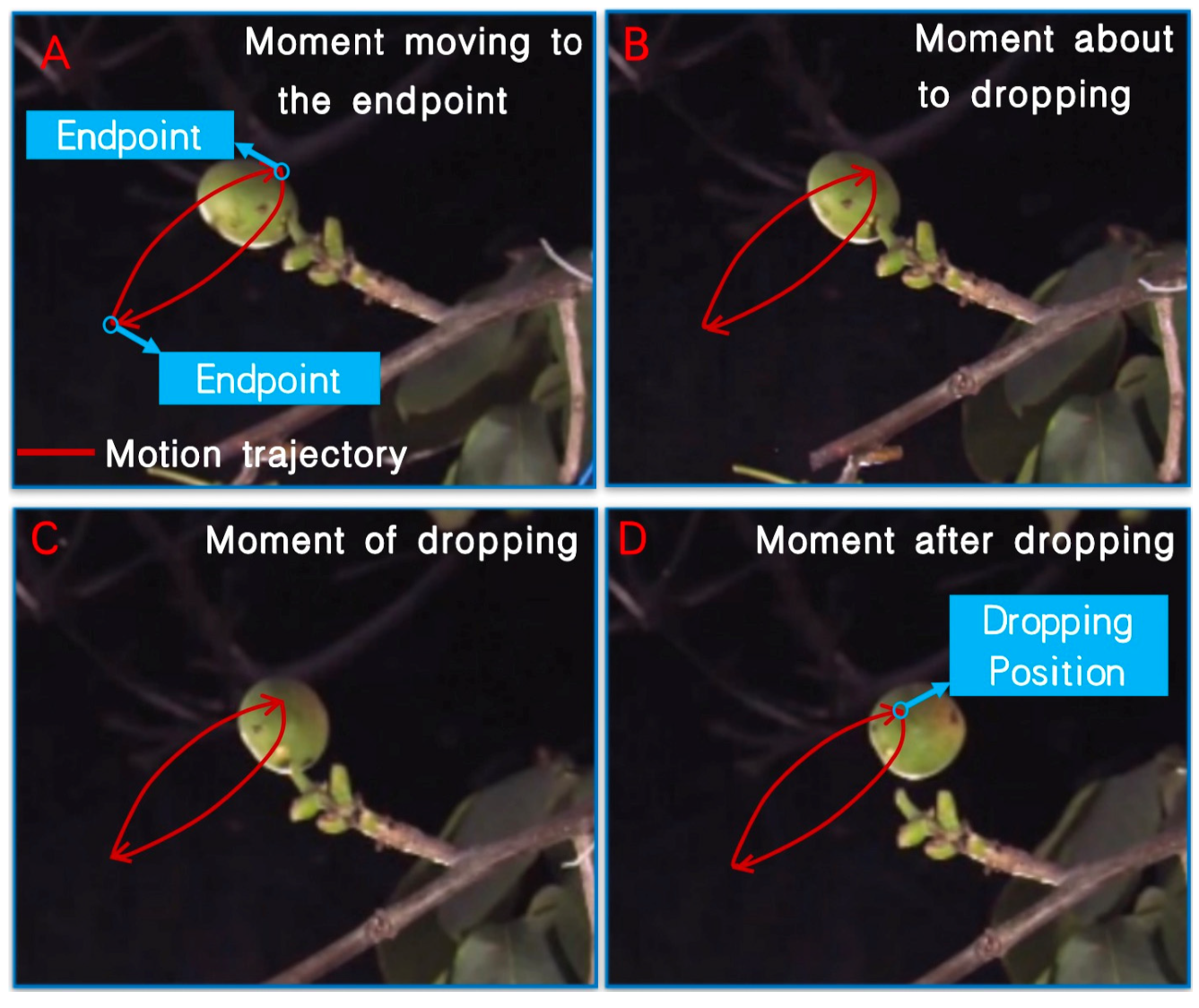
4.1. Motion Trajectory and Dropping Position of Walnuts in Vibration Harvesting
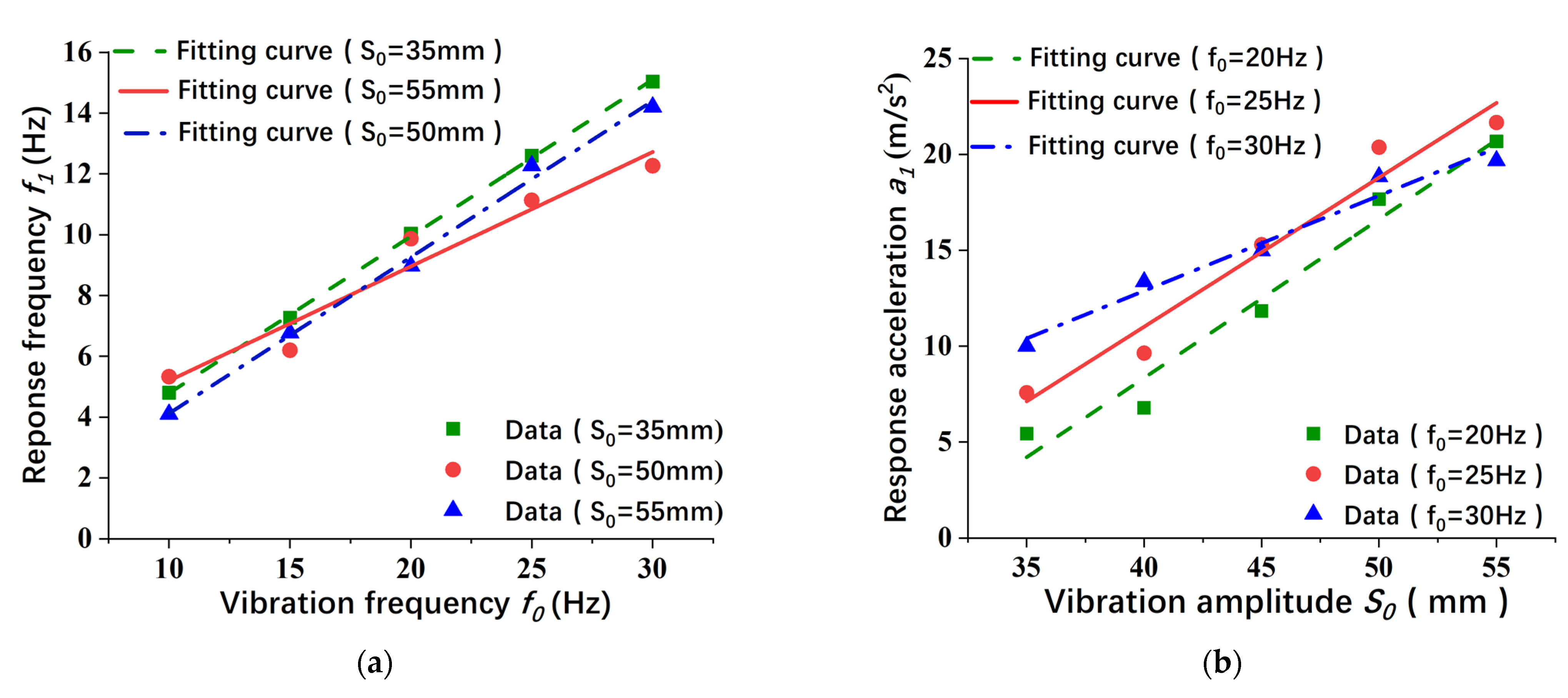
4.2. Relationship between the Detachment Force and the Vibration Frequency and Amplitude
5. Conclusions
- Theoretical and experimental results both showed that the motion trajectory of the walnuts during vibration harvesting is similar to an ellipse, and the dropping positions are at the two end points of the trajectory.
- For vibration harvesting of walnuts under actual harvesting conditions, the response amplitude of the walnuts is proportional to the vibration amplitude of the device. Additionally, the response frequency of the walnuts is proportional to the vibration frequency of the device, with the attenuation rate being approximately 50%.
- Using the theoretical model and extending the experiment, the equation for the relationship between the detachment force and the vibration response and amplitude was obtained, which shows the detachment force is proportional to the vibration amplitude and the square of the vibration frequency. This relationship further reflects that in order to achieve better vibration response and improve the fruit harvesting rate, vibration frequency and vibration amplitude should be considered comprehensively.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Notation | |
| Weight of walnut fruits (kg) | |
| Angle of with respect to the horizontal direction (deg) | |
| Maximum angle of the horizontal pendulum (deg) | |
| Vibration force at the input end (N) | |
| Excitation force at the fruits (N) | |
| Component of in the vertical direction (N) | |
| Component of in the horizontal direction (N) | |
| Maximum resultant external force of synthesized motion (N) | |
| Resultant external force in the horizontal direction (N) | |
| Resultant external force in the vertical direction (N) | |
| Resultant external force at point B (N) | |
| Resultant external force at point D (N) | |
| Centripetal force of the horizontal pendulum (N) | |
| Resultant external force of the pendulum at point D (N) | |
| Resultant external force of linear motion with varying acceleration at point D (N) | |
| Vector in the same direction as | |
| Vector in the same direction as | |
| Vector in the same direction as | |
| Ratio of to | |
| Vibration amplitude (mm) | |
| Response amplitude at the fruits (mm) | |
| Component of in the vertical direction (mm) | |
| Component of in the horizontal direction (mm) | |
| Displacement of the horizontal pendulum (mm) | |
| Decay rate of | |
| Decay rate of | |
| Decay rate of | |
| Ratio of to | |
| Vibration frequency (Hz) | |
| Vibration response frequency of fruits (Hz) | |
| Velocity at point B’ (m/s) | |
| Velocity of horizontal pendulum at D’ point (m/s) | |
| Component of in horizontal direction (m/s) | |
| Component of in the vertical direction (m/s) | |
| Velocity of horizontal linear motion with varying acceleration at point D’ (m/s) | |
| Time at which decreases to zero | |
| Time at which or decreases to zero | |
| Ratio of in the half motion cycle | |
| Ratio of in the half motion cycle | |
References
- Thomson, G.; McCaskill, M.; Goodwin, I.; Kearney, G.; Lolicato, S. Potential impacts of rising global temperatures on Australia’s pome fruit industry and adaptation strategies. N. Zeal. J. Crop Hort. 2014, 42, 21–30. [Google Scholar] [CrossRef]
- Webb, B.K.; Hood, C.E.; Jenkins, W.H.; Veal, C.D. Development of an over-the-row peach harvester. Trans. ASAE 1973, 16, 450–455. [Google Scholar] [CrossRef]
- Churchill, D.B.; Sumner, H.R. A new system for raking and picking up oranges. Trans. ASAE 1977, 20, 617–620. [Google Scholar] [CrossRef]
- Churchill, D.B.; Hedden, S.L. A double-sided rake-pickup machine for citrus. Trans. ASAE 1983, 26, 1034–1036. [Google Scholar] [CrossRef]
- Sanders, K.F. Orange harvesting systems review. Biosyst. Eng. 2005, 90, 115–125. [Google Scholar] [CrossRef]
- Hedden, S.L.; Churchill, D.B.; Whitney, J.D. Trunk shakers for citrus harvesting—Part II: Tree growth, fruit yield and removal. Appl. Eng. Agric. 1988, 4, 102–106. [Google Scholar] [CrossRef]
- Du, X.; Chen, D.; Zhang, Q.; Scharf, P.A.; Whiting, M.D. Dynamic responses of sweet cherry trees under vibratory excitations. Biosyst. Eng. 2012, 111, 305–314. [Google Scholar] [CrossRef]
- Erdoǧan, D.; Güner, M.; Dursun, E.; Gezer, İ. Mechanical harvesting of apricots. Biosyst. Eng. 2003, 85, 19–28. [Google Scholar] [CrossRef]
- Zhang, W.; Yao, W.; Xv, X. Study of picking method for carya cathayensis. Appl. Mech. Mater. 2011, 1366, 71–78. [Google Scholar]
- Du, X.; Wu, C.; He, L.; Tong, J. Dynamic characteristics of dwarf Chinese hickory trees under impact excitations for mechanical fruit harvesting. Int. J. Agric. Biol. Eng. 2015, 8, 17–25. [Google Scholar]
- Bentaher, H.; Haddar, M.; Fakhfakh, T.; Mâalej, A. Finite elements modeling of olive tree mechanical harvesting using different shakers. Trees 2013, 27, 1537–1545. [Google Scholar] [CrossRef]
- Tinoco, H.A.; Ocampo, D.A.; Peña, F.M.; Sanz-Uribe, J.R. Finite element modal analysis of the fruit-peduncle of Coffea arabica L. var. Colombia estimating its geometrical and mechanical properties. Comput. Electron. Agric. 2014, 108, 17–27. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, L.; Zhang, A.; Hang, X. Finite element explicit dynamics simulation of motion and shedding of jujube fruits under forced vibration. Comput. Electron. Agric. 2022, 198, 107009. [Google Scholar] [CrossRef]
- Castro-García, S.; Blanco-Roldán, G.L.; Gil-Ribes, J.A.; Agüera-Vega, J. Dynamic analysis of olive trees in intensive orchards under forced vibration. Trees 2008, 22, 795–802. [Google Scholar] [CrossRef]
- Zhou, J.; He, L.; Zhang, Q.; Karkee, M. Effect of excitation position of a handheld shaker on fruit removal efficiency and damage in mechanical harvesting of sweet cherry. Biosyst. Eng. 2014, 125, 36–44. [Google Scholar] [CrossRef]
- He, L.; Liu, X.; Du, X.; Wu, C. In-situ identification of shaking frequency for adaptive vibratory fruit harvesting. Comput. Electron. Agric. 2020, 170, 105245. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, L.; Liu, G.; Xuan, Y.; Zhou, H.; Jiang, H. Frequency response curves and dynamic characteristics of a ginkgo tree in different growth periods. Trans. ASABE 2020, 63, 1673–1684. [Google Scholar] [CrossRef]
- He, L.; Zhou, J.; Du, X.; Chen, D.; Zhang, Q.; Karkee, M. Energy efficacy analysis of a mechanical shaker in sweet cherry harvesting. Biosyst. Eng. 2013, 116, 309–315. [Google Scholar] [CrossRef]
- Leone, A.; Romaniello, R.; Tamborrino, A.; Catalano, P.; Peri, G. Identification of vibration frequency, acceleration, and duration for efficient olive harvesting Using a Trunk Shaker. Trans. ASABE 2015, 58, 19–26. [Google Scholar]
- Castro-García, S.; Blanco-Roldán, G.L.; Gil-Ribes, J.A. Frequency response of Pinus Pinea, L. for selective cone harvesting by vibration. Trees 2011, 25, 801–808. [Google Scholar] [CrossRef]
- Castro-Garcia, S.; Sola-Guirado, R.R.; Gil-Ribes, J.A. Vibration analysis of the fruit detachment process in late-season ‘Valencia’ orange with canopy shaker technology. Biosyst. Eng. 2018, 170, 130–137. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, L.; Xuan, Y.; Xu, Y.; Liu, G. Shedding frequency and motion of jujube fruits in various excitation modes. Trans. ASABE 2020, 63, 881–889. [Google Scholar] [CrossRef]
- He, L.; Zhang, X.; Ye, Y.; Karkee, M.; Zhang, Q. Effect of shaking location and duration on mechanical harvesting of fresh market apples. Appl. Eng. Agric. 2019, 35, 175–183. [Google Scholar] [CrossRef]
- Coelho, A.L.F.; Santos, F.L.; Pinto, F.A.C.; Queiroz, D.M. Detachment efficiency of fruits from coffee plants subjected to mechanical vibrations. Pesq. Agrop. Trop. 2015, 45, 406–412. [Google Scholar] [CrossRef]
- Savary, S.K.J.U.; Ehsani, R.; Schueller, J.K.; Rajaraman, B.P. Simulation study of citrus tree canopy motion during harvesting using a canopy shaker. Trans. ASABE 2010, 53, 1373–1381. [Google Scholar] [CrossRef]
- Tsatsarelis, C.A. Vibratory olive harvesting: The response of the fruit-stem system to fruit removing actions. J. Agric. Eng. Res. 1987, 38, 77–90. [Google Scholar] [CrossRef]
- Castro-Garcia, S.; Blanco-Roldán, G.L.; Ferguson, L.; González-Sánchez, E.J.; Gil-Ribes, J.A. Frequency response of late-season ‘Valencia’ orange to selective harvesting by vibration for juice industry. Biosyst. Eng. 2017, 155, 77–83. [Google Scholar] [CrossRef]
- Tombesi, S.; Poni, S.; Palliotti, A.; Farinelli, D. Mechanical vibration transmission and harvesting effectiveness is affected by the presence of branch suckers in olive trees. Biosyst. Eng. 2017, 158, 1–9. [Google Scholar] [CrossRef]
- Zhou, J.; He, L.; Karkee, M.; Zhang, Q. Analysis of shaking-induced cherry fruit motion and damage. Biosyst. Eng. 2016, 144, 105–114. [Google Scholar] [CrossRef]
- Wei, J.; Yang, G.; Yan, H.; Jing, B.; Yu, Y. Rigid-flexible coupling simulation and experimental vibration analysis of pistachio tree for optimal mechanized harvesting efficiency. Mech. Adv. Mater. Struct. 2021, 28, 2360–2369. [Google Scholar] [CrossRef]
- Ortiz, C.; Torregrosa, A.; Castro-García, S. Citrus fruit movement assessment related to fruit damage during harvesting with an experimental low-frequency–high-amplitude device. Agronomy 2022, 12, 1337. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Xu, D.; Cao, J. Vibration Response of Walnuts under Vibration Harvesting. Agronomy 2023, 13, 461. https://doi.org/10.3390/agronomy13020461
Liu C, Xu D, Cao J. Vibration Response of Walnuts under Vibration Harvesting. Agronomy. 2023; 13(2):461. https://doi.org/10.3390/agronomy13020461
Chicago/Turabian StyleLiu, Changyi, Daochun Xu, and Jiale Cao. 2023. "Vibration Response of Walnuts under Vibration Harvesting" Agronomy 13, no. 2: 461. https://doi.org/10.3390/agronomy13020461
APA StyleLiu, C., Xu, D., & Cao, J. (2023). Vibration Response of Walnuts under Vibration Harvesting. Agronomy, 13(2), 461. https://doi.org/10.3390/agronomy13020461




