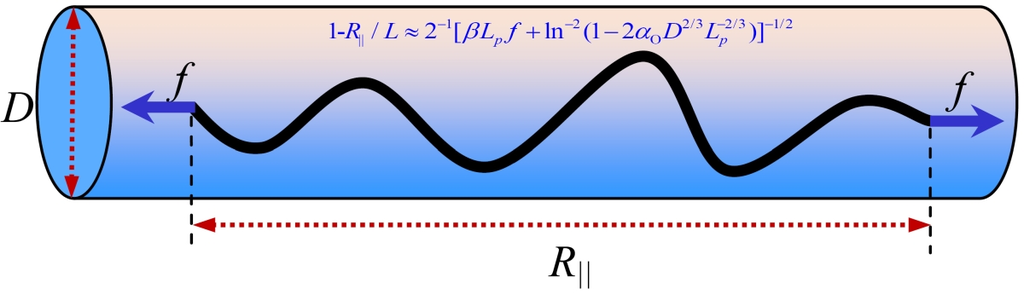
Stretching a Semiflexible Polymer in a Tube
Abstract
:1. Introduction
2. Materials and Methods
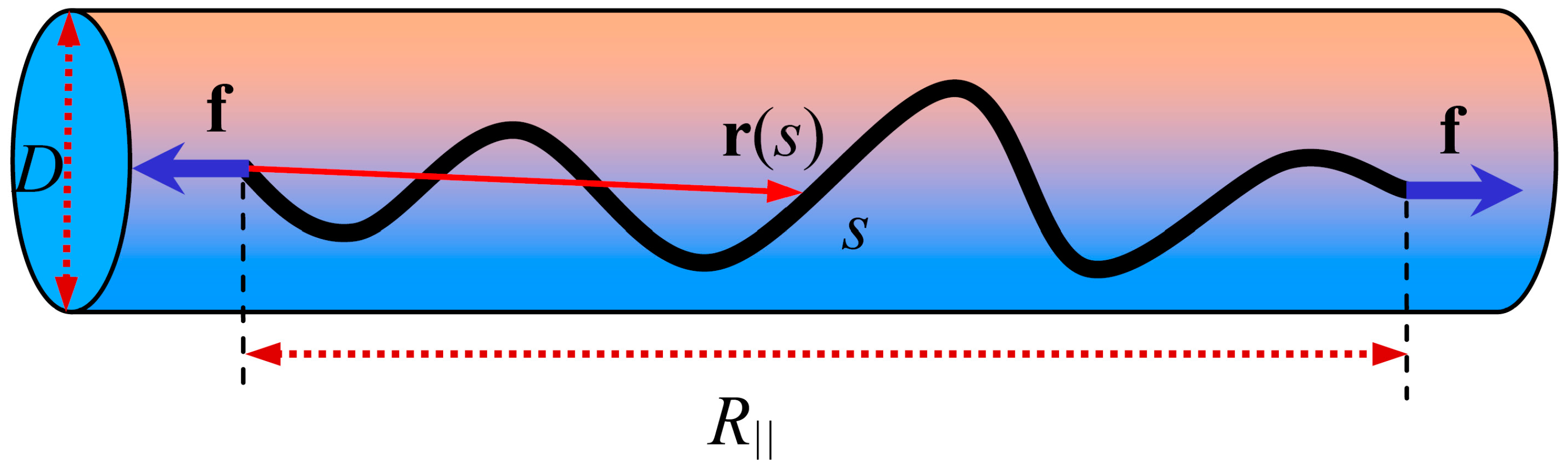
2.1. Model
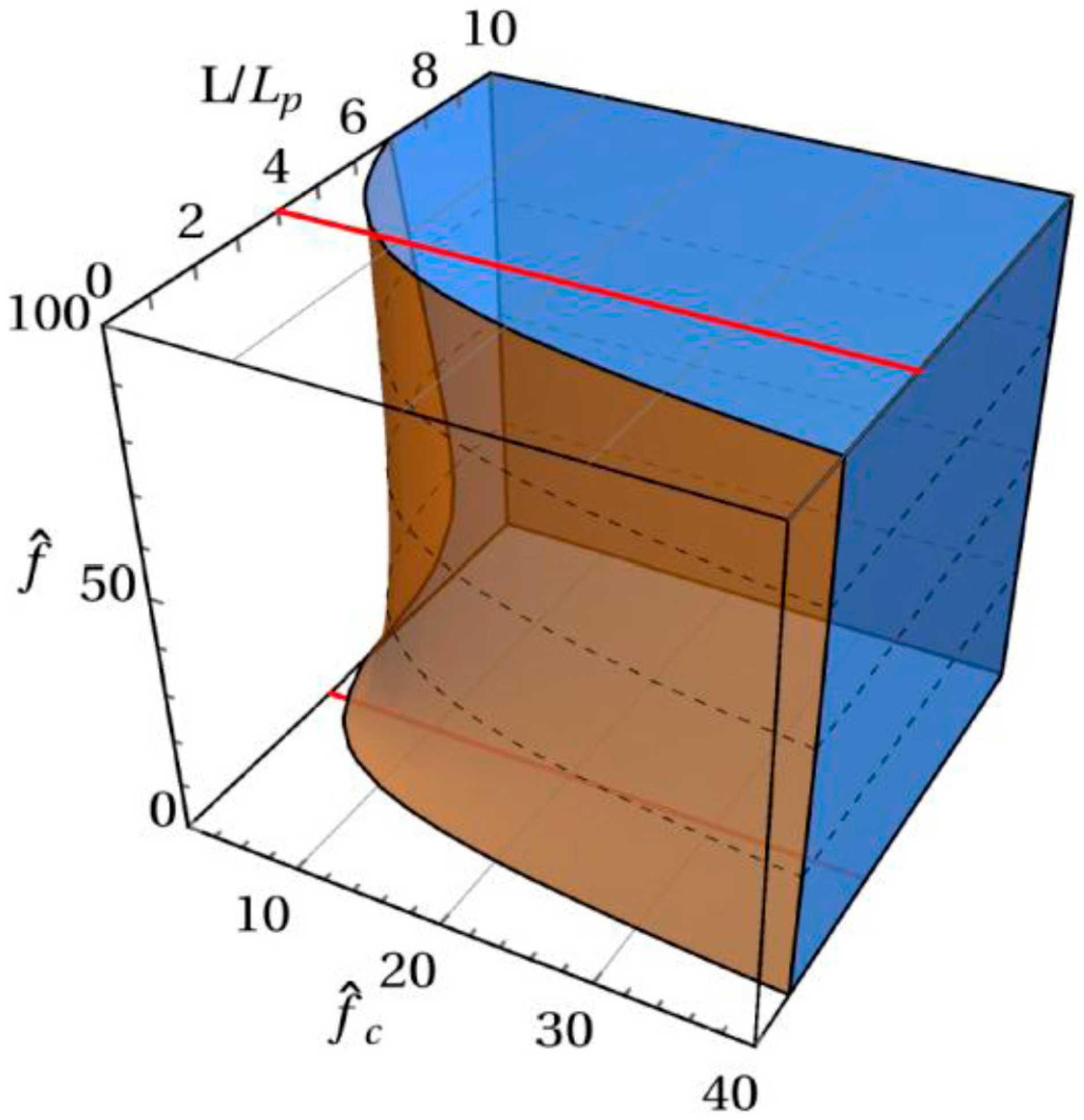
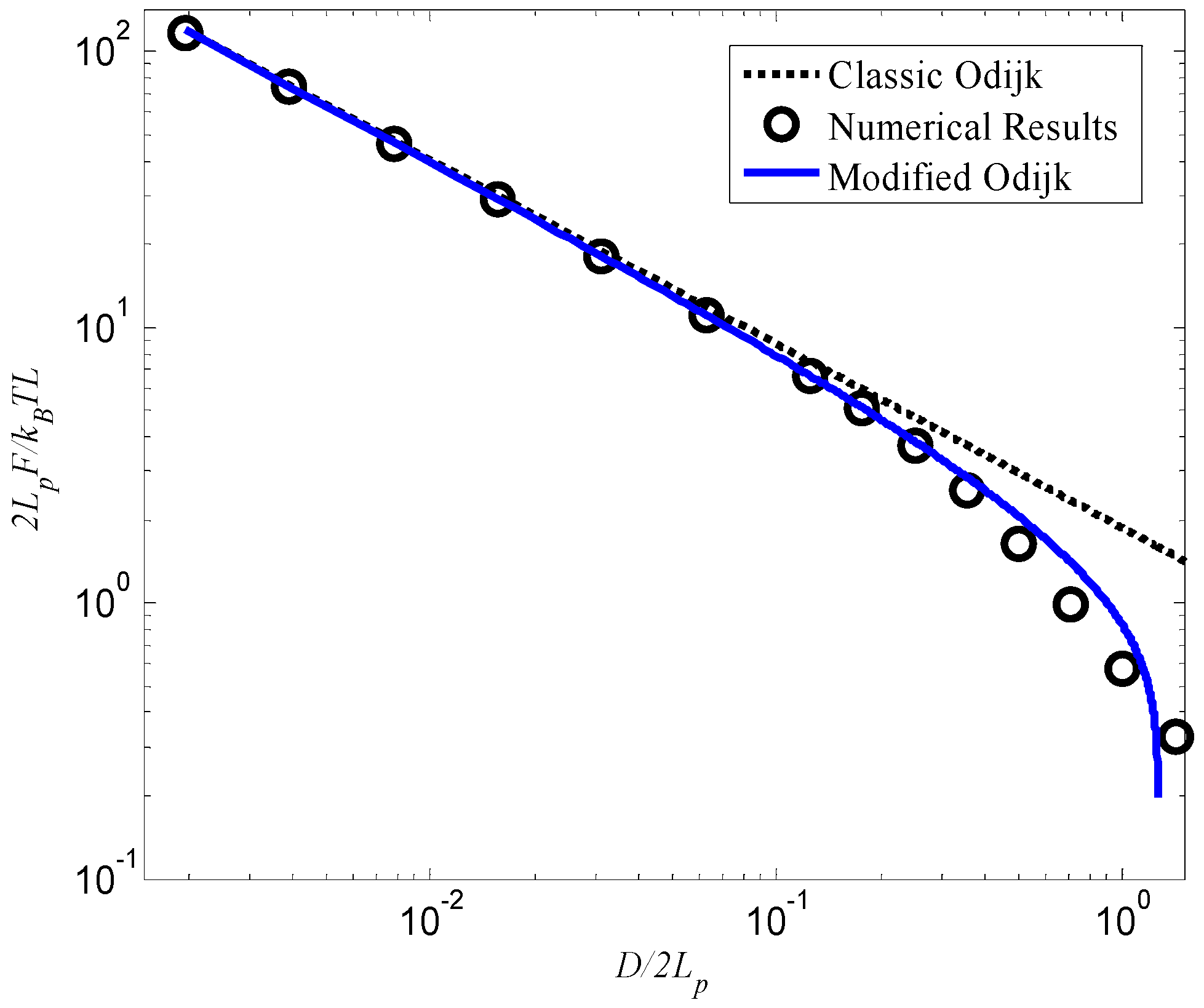
2.2. Modified Odijk Length and Effective Stretching Force Due to Confinement
2.3. Brownian Dynamics Simulations
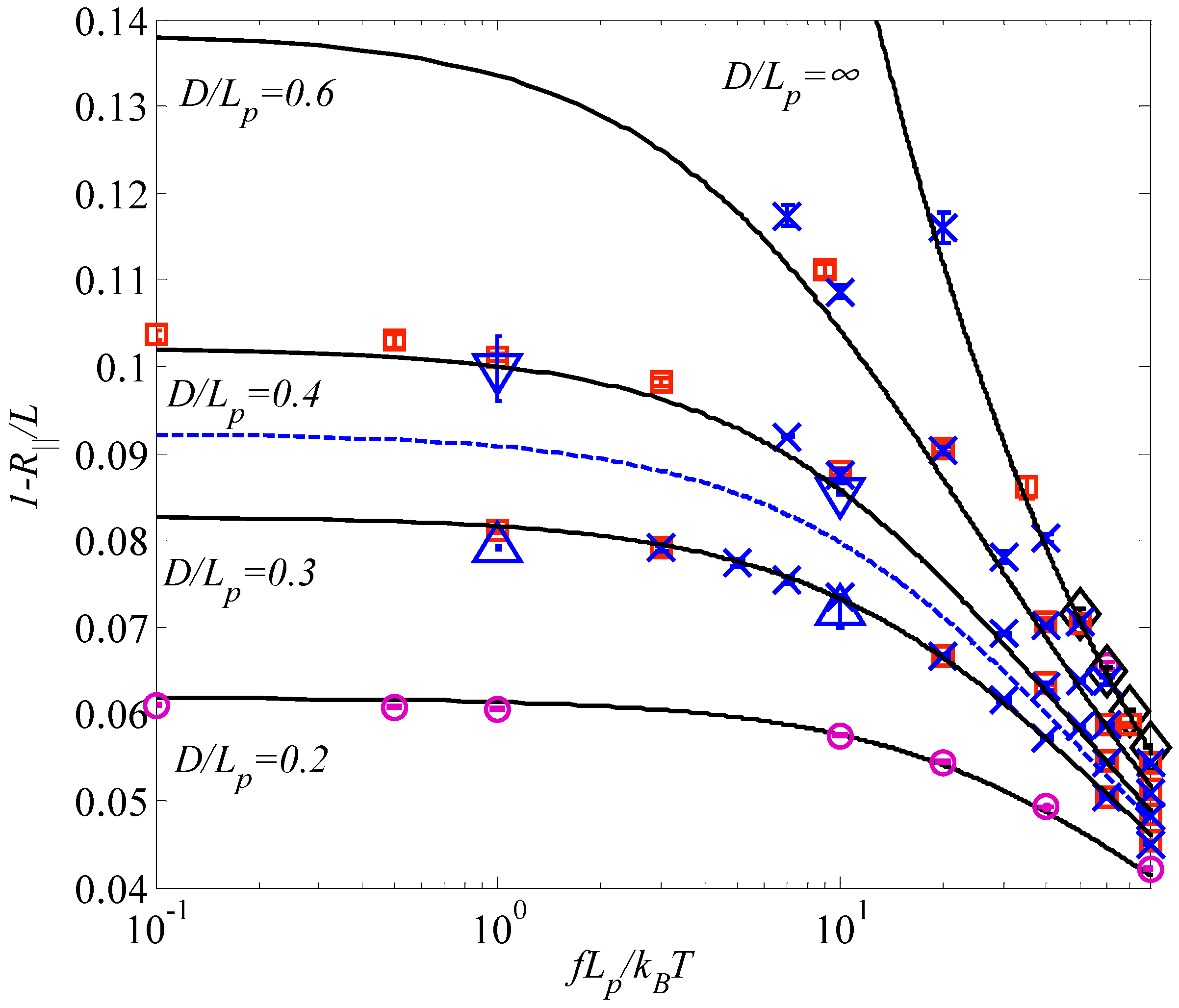
3. Discussion
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Levy, S.L.; Craighead, H.G. DNA manipulation, sorting, and mapping in nanouidic systems. Chem. Soc. Rev. 2010, 39, 1133–1152. [Google Scholar] [CrossRef] [PubMed]
- Strychalski, E.A.; Lau, H.W.; Archer, L.A. Nonequilibrium separation of short DNA using nanoslit arrays. J. Appl. Phys. 2009, 106, 024915. [Google Scholar] [CrossRef] [PubMed]
- Jun, S.; Mulder, B. Entropy-driven spatial organization of highly confined polymers: Lessons for the bacterial chromosome. Proc. Natl. Acad. Sci. USA 2006, 103, 12388–12393. [Google Scholar] [CrossRef] [PubMed]
- Kster, S.; Pfohl, T. An in vitro model system for cytoskeletal confinement. Cell Motil. Cytoskelet. 2009, 66, 771–776. [Google Scholar] [CrossRef] [PubMed]
- Reisner, W.; Larsen, N.B.; Silahtaroglu, A.; Kristensen, A.; Tommerup, N.; Tegenfeldt, J.O.; Flyvbjerg, H. Single-molecule denaturation mapping of DNA in nanofluidic channels. Proc. Natl. Acad. Sci. USA 2010, 107, 13294–13299. [Google Scholar] [CrossRef] [PubMed]
- Reisner, W.; Pedersen, J.N.; Austin, R.H. DNA confinement in nanochannels: Physics and biological applications. Rep. Prog. Phys. 2012, 75, 106601. [Google Scholar] [CrossRef] [PubMed]
- Jo, K.; Dhingra, D.M.; Odijk, T.; de Pablo, J.J.; Graham, M.D.; Runnheim, R.; Forrest, D.; Schwartz, D.C. A single-molecule barcoding system using nanoslits for DNA analysis. Proc. Natl. Acad. Sci. USA 2007, 104, 2673–2678. [Google Scholar] [CrossRef] [PubMed]
- Odijk, T. The statistics and dynamics of confined or entangled stiff polymers. Macromolecules 1983, 16, 1340–1344. [Google Scholar] [CrossRef]
- Wang, J.; Gao, H. Brownian dynamics simulations of charged semiflexible polymers confined to curved surfaces. J. Mech. Behav. Biomed. Mater. 2011, 4, 174–179. [Google Scholar] [CrossRef] [PubMed]
- De Gennes, P.G. Scaling Concepts in Polymer Physics, 1st ed.; Cornell University Press: Ithaca, NY, USA, 1979. [Google Scholar]
- Dai, L.; Ng, S.Y.; Doyle, P.S.; van der Maarel, J.R.C. Conformation model of back-folding and looping of a single DNA molecule confined inside a nanochannel. ACS Macro Lett. 2012, 1, 1046–1050. [Google Scholar] [CrossRef]
- Marko, J.F.; Siggia, E.D. Stretching DNA. Macromolecules 1995, 28, 8759–8770. [Google Scholar] [CrossRef]
- Kierfeld, J.; Niamploy, O.; Sa-Yakanit, V.; Lipowsky, R. Stretching of semiflexible polymers with elastic bonds. Eur. Phys. J. E 2004, 14, 17–34. [Google Scholar] [CrossRef] [PubMed]
- Kratky, O.; Porod, G. Röntgenuntersuchung gelöster Fadenmoleküle. Recl. Trav. Chim. Pays-Bas 1949, 68, 1106–1122. [Google Scholar] [CrossRef]
- Chen, J.Z.Y. Theory of wormlike chains in confinement. Prog. Polym. Sci. 2016, 54–55, 3–46. [Google Scholar] [CrossRef]
- Chen, J.Z.Y. Free Energy and Extension of a Wormlike Chain in Tube Confinement. Macromolecules 2013, 46, 9837–9844. [Google Scholar] [CrossRef]
- Odijk, T. Physics of tightly curved semiflexible polymer chains. Macromolecules 1993, 26, 6897–6902. [Google Scholar] [CrossRef]
- Burkhardt, T.W. Free energy of a semiflexible polymer in a tube and statistics of a randomly-accelerated particle. J. Phys. A Math. Gen. 1997, 30, L167–L172. [Google Scholar] [CrossRef]
- Wang, J.; Gao, H.J. A generalized bead-rod model for Brownian dynamics simulations of wormlike chains under strong confinement. J. Chem. Phys. 2005, 123, 084906. [Google Scholar] [CrossRef] [PubMed]
- Burkhardt, T.W.; Yang, Y.; Gompper, G. Fluctuations of a long, semiflexible polymer in a narrow channel. Phys. Rev. E 2010, 82, 1003–1009. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Burkhardt, T.W.; Gompper, G. Free energy and extension of a semiflexible polymer in cylindrical confining geometries. Phys. Rev. E 2007, 76, 55–86. [Google Scholar] [CrossRef] [PubMed]
- Odijk, T. DNA confined in nanochannels: Hairpin tightening by entropic depletion. J. Chem. Phys. 2006, 125, 261–288. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Gao, H. Stretching a stiff polymer in a tube. J. Mater. Sci. 2007, 42, 8838–8843. [Google Scholar] [CrossRef]
- Levi, P.; Mecke, K. Radial distribution function for semiflexible polymers confined in microchannels. Europhys. Lett. 2007, 78, 38001. [Google Scholar] [CrossRef]
- Burkhardt, T.W. Harmonically confined, semiflexible polymer in a channel: Response to a stretching force and spatial distribution of the endpoints. J. Stat. Phys. 2011, 145, 1472–1484. [Google Scholar] [CrossRef]
- Su, T.; Das, S.K.; Xiao, M.; Purohit, P.K. transition between two regimes describing internal fluctuation of DNA in a nanochannel. PLoS ONE 2011, 6, 1089–1105. [Google Scholar] [CrossRef] [PubMed]
- Burkhardt, T.W. Free energy of a semiflexible polymer confined along an axis. J. Phys. A Math. Gen. 1995, 28, L629–L635. [Google Scholar] [CrossRef]
- Yamakawa, H. Modern Theory of Polymer Solutions; Harper and Row: New York, NY, USA, 1971. [Google Scholar]

© 2016 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, R.; Wang, J. Stretching a Semiflexible Polymer in a Tube. Polymers 2016, 8, 328. https://doi.org/10.3390/polym8090328
Li R, Wang J. Stretching a Semiflexible Polymer in a Tube. Polymers. 2016; 8(9):328. https://doi.org/10.3390/polym8090328
Chicago/Turabian StyleLi, Runhua, and Jizeng Wang. 2016. "Stretching a Semiflexible Polymer in a Tube" Polymers 8, no. 9: 328. https://doi.org/10.3390/polym8090328
APA StyleLi, R., & Wang, J. (2016). Stretching a Semiflexible Polymer in a Tube. Polymers, 8(9), 328. https://doi.org/10.3390/polym8090328