Enhancing the Strength of 3D-Printed Polymer Exoprosthetic Socket by Localized Non-Planar Continuous Carbon Fiber Reinforcement
Abstract
1. Introduction
- (i)
- Investigation into optimal reinforcement schemes by determination of the optimal spacing interval for carbon rod integration in rectangular polymer specimens through three-point bending tests.
- (ii)
- Finite element modeling of the prosthetic socket with carbon rods positioned in critical stress zones identified during the first phase.
- (iii)
- (iv)
- Manufacturing of a cylindrical prototype with localized carbon fiber reinforcement employing advantages of multi-axial 3D-printing.
2. Materials and Methods
2.1. Materials
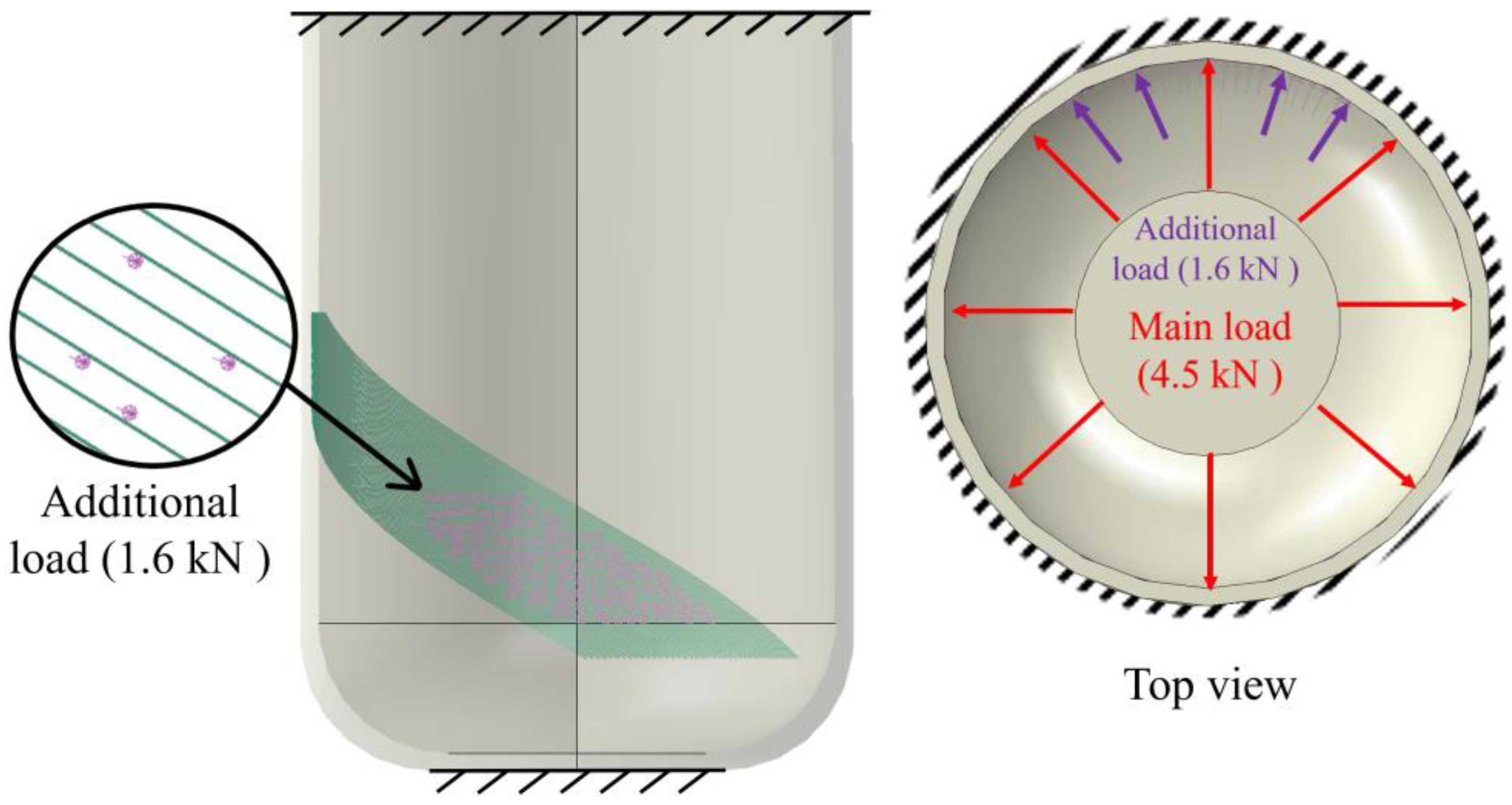
2.2. Modeling and Numerical Simulation
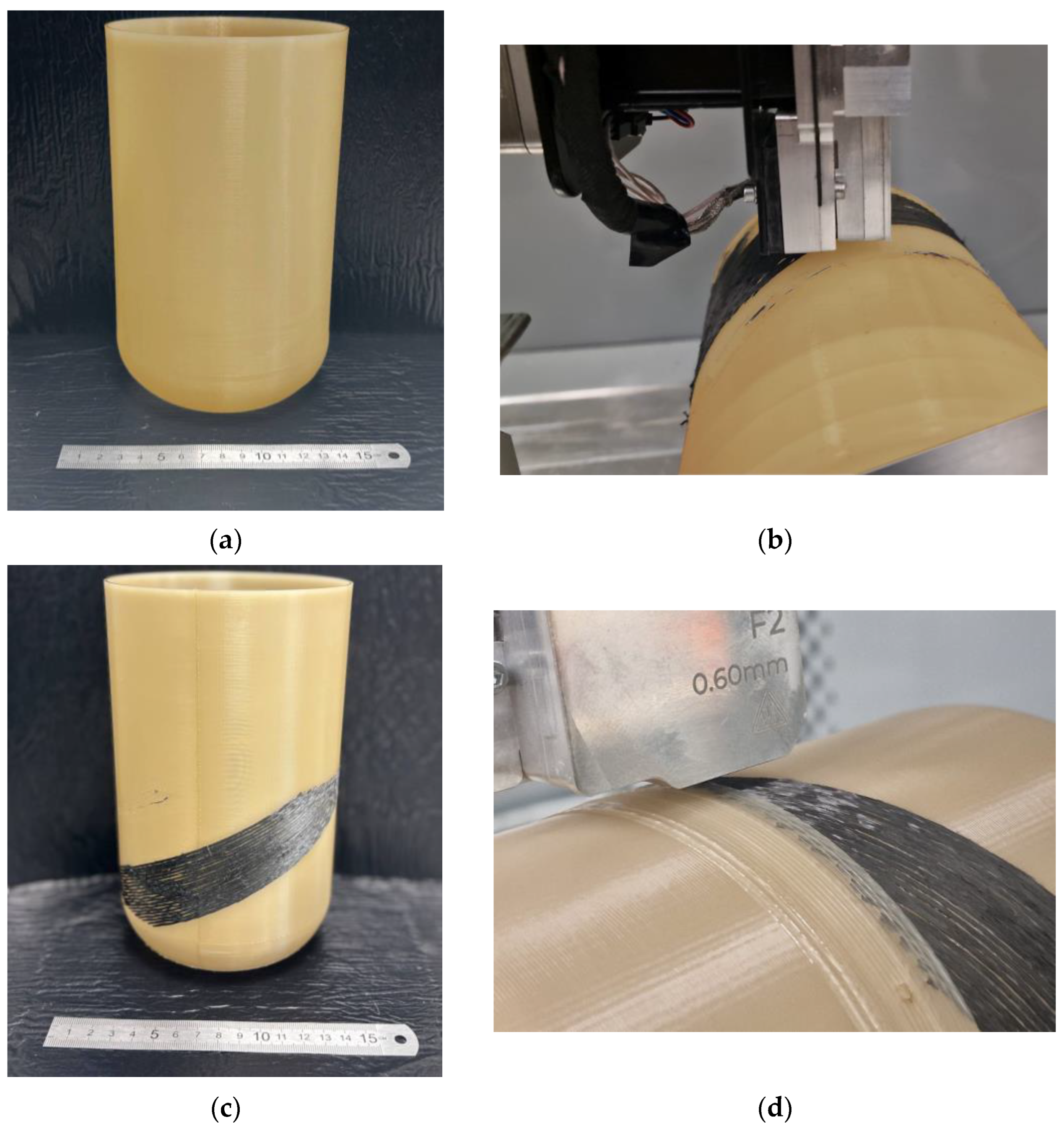
2.3. Multi-Axial Additive Manufacturing and Non-Planar Reinforcement
3. Results
3.1. Optimal Arrangement of Carbon Rods
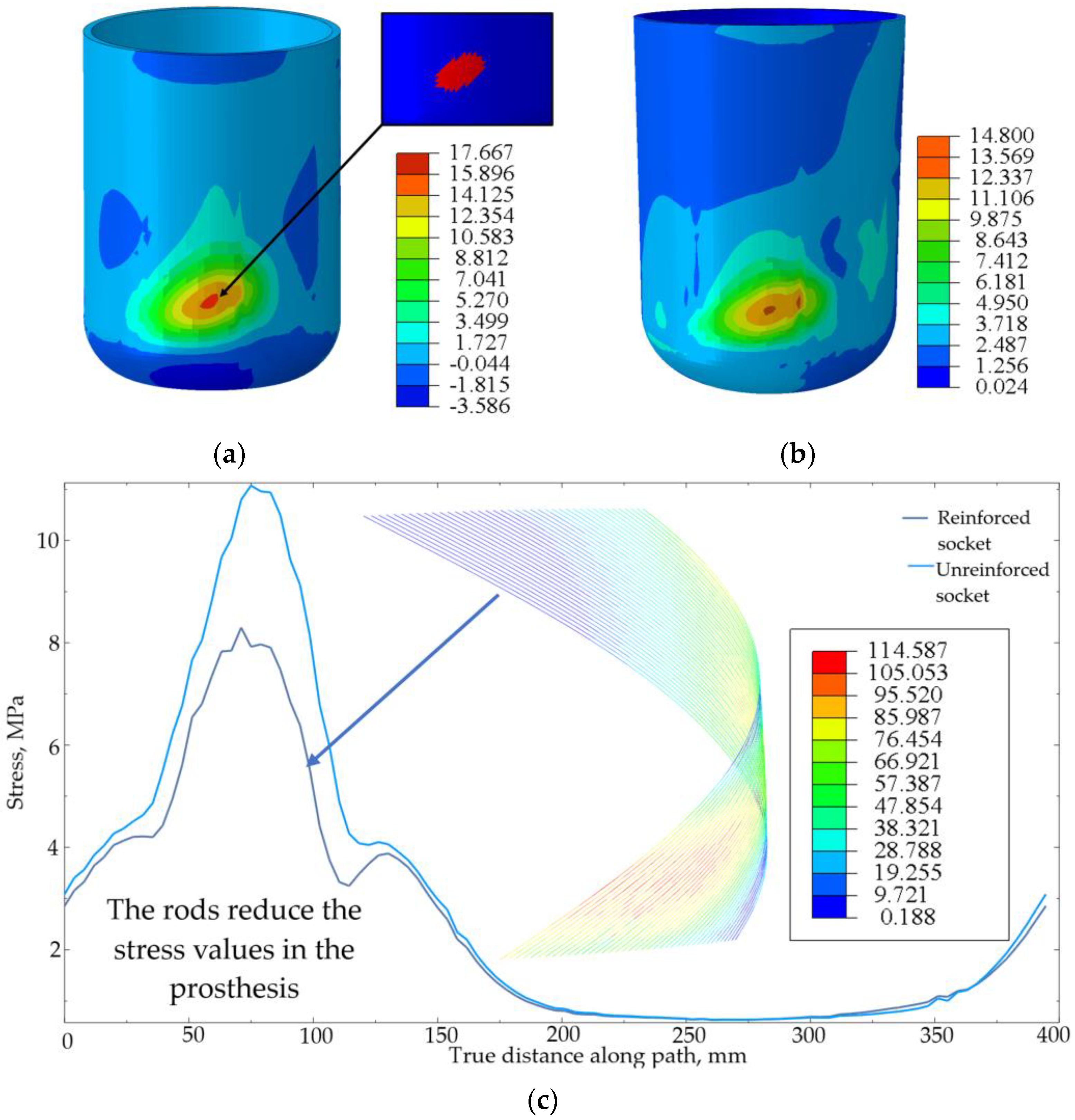
- The presence of reinforcing elements reduced the stress levels throughout the volume of the polymer matrix and prevented crack initiation on the surface of the sample.
- At a distance of 1.9 mm between the centers of the rods, the strength criterion had a value less than 1, leading to failure of the polymer matrix between the rods.
- The optimal distance between centers of the rods was determined to be 1.5 mm, which provides a safety margin according to the strength criterion.
- The effect of reinforcement was observed in a 4 mm thick plate only when the rods were positioned close to the points of load application.
3.2. Numerical Investigation of Damage Accumulation Process
3.3. Manufacturing of Socket Prototype
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Subih, M.; Arifin, N.; Al-Fakih, E.A. Pressure Reduction Management System in Three-Dimensional-Printed Transtibial Prosthetic Socket during Stance Phase. J. Prosthetics Orthot. 2024, 36, 42–48. [Google Scholar] [CrossRef]
- Wang, Y.; Tan, Q.; Pu, F.; Boone, D.; Zhang, M. A Review of the Application of Additive Manufacturing in Prosthetic and Orthotic Clinics from a Biomechanical Perspective. Engineering 2020, 6, 1258–1266. [Google Scholar] [CrossRef]
- Marinopoulos, T.; Li, S.; Silberschmidt, V.V. Structural Integrity of 3D-Printed Prosthetic Sockets: Experimental Study For Paediatric Applications. J. Mater. Res. Technol. 2023, 24, 2734–2742. [Google Scholar] [CrossRef]
- Sun, C.; Tang, L.; Liu, T.; Wang, L.; Tian, X.; Liu, C.; Li, D. A Shape-Performance Synergistic Strategy for Design and Additive Manufacturing of Continuous Fiber Reinforced Transfemoral Prosthetic Socket. Compos. Part B Eng. 2024, 281, 111518. [Google Scholar] [CrossRef]
- Kim, S.; Yalla, S.V.; Shetty, S.; Rosenblatt, N.J. Structural Integrity of custom-Designed Additive Manufactured Prosthetic Sockets Compared to Traditional Sockets. Results Mater. 2024, 21, 100549. [Google Scholar] [CrossRef]
- Sengeh, D.M.; Herr, H. A Variable-Impedance Prosthetic Socket for a Transtibial Amputee Designed from Magnetic Resonance Imaging Data. JPO J. Prosthetics Orthot. 2013, 25, 129–137. [Google Scholar] [CrossRef]
- Gholizadeh, H.; Abu Osman, N.; Kamyab, M.; Eshraghi, A.; Abas, W.W.; Azam, M. Transtibial Prosthetic Socket Pistoning: Static Evaluation of Seal-In® X5 and Dermo® Liner Using Motion Analysis System. Clin. Biomech. 2012, 27, 34–39. [Google Scholar] [CrossRef]
- Baars, E.C.T.; Geertzen, J.H.B. Literature Review of the Possible Advantages of Silicon Liner Socket Use in Trans-Tibial Prostheses. Prosthetics Orthot. Int. 2005, 29, 27–37. [Google Scholar] [CrossRef]
- Ning, F.; Cong, W.; Qiu, J.; Wei, J.; Wang, S. Additive Manufacturing of Carbon Fiber Reinforced Thermoplastic Composites Using Fused Deposition Modeling. Compos. Part B Eng. 2015, 80, 369–378. [Google Scholar] [CrossRef]
- Goh, G.D.; Dikshit, V.; Nagalingam, A.P.; Goh, G.L.; Agarwala, S.; Sing, S.L.; Wei, J.; Yeong, W.Y. Characterization of Mechanical Properties and Fracture Mode of Additively Manufactured Carbon Fiber and Glass Fiber Reinforced Thermoplastics. Mater. Des. 2018, 137, 79–89. [Google Scholar] [CrossRef]
- Liao, G.; Li, Z.; Cheng, Y.; Xu, D.; Zhu, D.; Jiang, S.; Guo, J.; Chen, X.; Xu, G.; Zhu, Y. Properties of Oriented Carbon Fiber/Polyamide 12 Composite Parts Fabricated by Fused Deposition Modeling. Mater. Des. 2018, 139, 283–292. [Google Scholar] [CrossRef]
- Manzoor, F.; Golbang, A.; Jindal, S.; Dixon, D.; McIlhagger, A.; Harkin-Jones, E.; Crawford, D.; Mancuso, E. 3D Printed PEEK/HA Composites for Bone Tissue Engineering Applications: Effect of Material Formulation on Mechanical Performance and Bioactive Potential. J. Mech. Behav. Biomed. Mater. 2021, 121, 104601. [Google Scholar] [CrossRef] [PubMed]
- Dou, H.; Cheng, Y.; Ye, W.; Zhang, D.; Li, J.; Miao, Z.; Rudykh, S. Effect of Process Parameters on Tensile Mechanical Properties of 3D Printing Continuous Carbon Fiber-Reinforced PLA Composites. Materials 2020, 13, 3850. [Google Scholar] [CrossRef]
- Lobov, E.; Dobrydneva, A.; Vindokurov, I.; Tashkinov, M. Effect of Short Carbon Fiber Reinforcement on Mechanical Properties of 3D-Printed Acrylonitrile Butadiene Styrene. Polymers 2023, 15, 2011. [Google Scholar] [CrossRef]
- Ramlee, M.H.; Ammarullah, M.I.; Sukri, N.S.M.; Hassan, N.S.F.; Baharuddin, M.H.; Kadir, M.R.A. Investigation on Three-Dimensional Printed Prosthetics Leg Sockets Coated with Different Reinforcement Materials: Analysis on Mechanical Strength and Microstructural. Sci. Rep. 2024, 14, 6842. [Google Scholar] [CrossRef]
- Hofstätter, T.; Pedersen, D.B.; Tosello, G.; Hansen, H.N. State-of-the-Art of Fiber-Reinforced Polymers in Additive Manufacturing Technologies. J. Reinf. Plast. Compos. 2017, 36, 1061–1073. [Google Scholar] [CrossRef]
- Parandoush, P.; Lin, D. A Review on Additive Manufacturing of Polymer-Fiber Composites. Compos. Struct. 2017, 182, 36–53. [Google Scholar] [CrossRef]
- Wang, X.; Jiang, M.; Zhou, Z.W.; Gou, J.H.; Hui, D. 3D Printing of Polymer Matrix Composites: A Review and Prospective. Compos. Part B Eng. 2017, 110, 442–458. [Google Scholar] [CrossRef]
- Uhlmann, E.; Sammler, F.; Richarz, S.; Reucher, G.; Hufschmied, R.; Frank, A.; Stawiszynski, B.; Protz, F. Machining of Carbon and Glass Fibre Reinforced Composites. Procedia CIRP 2016, 46, 63–66. [Google Scholar] [CrossRef]
- Feng, Q.; Cong, W.L.; Pei, Z.J.; Ren, C.Z. Rotary Ultrasonic Machining of Carbon Fiber-Reinforced Polymer: Feasibility Study. Mach. Sci. Technol. 2012, 16, 380–398. [Google Scholar] [CrossRef]
- Stegmann, J.; Lund, E. Discrete Material Optimization of General Composite Shell Structures. Int. J. Numer. Methods Eng. 2005, 62, 2009–2027. [Google Scholar] [CrossRef]
- Tashkinov, M.A. Methods of Stochastic Mechanics for Characterization of Deformation in Randomly Reinforced Composite Materials. In Engineering Materials; Springer: Cham, Switzerland, 2015. [Google Scholar]
- Tashkinov, M.A.; Vil’deman, V.E.; Mikhailova, N.V. Method of Successive Approximations in a Stochastic Boundary-Value Problem in the Elasticity Theory of Structurally Heterogeneous Media. Compos. Mech. Comput. Appl. Int. J. 2011, 2, 21–37. [Google Scholar] [CrossRef]
- Talreja, R. Assessment of the Fundamentals of Failure Theories for Composite Materials. Compos. Sci. Technol. 2014, 105, 190–201. [Google Scholar] [CrossRef]
- Távara, L.; Moreno, L.; Paloma, E.; Mantič, V. Accurate Modelling of Instabilities Caused by Multi-Site Interface-Crack Onset and Propagation in Composites Using the Sequentially Linear Analysis and Abaqus. Compos. Struct. 2019, 225, 110993. [Google Scholar] [CrossRef]
- Jafaripour, M.; Taheri-Behrooz, F. Creep Behavior Modeling of Polymeric Composites Using Schapery Model Based on Micro-macromechanical Approaches. Eur. J. Mech.-A/Solids 2020, 81, 103963. [Google Scholar] [CrossRef]
- Arruda, M.; Almeida-Fernandes, L.; Castro, L.; Correia, J. Tsai–Wu Based Orthotropic Damage Model. Compos. Part C Open Access 2021, 4, 100122. [Google Scholar] [CrossRef]
- Kathrotiya, D.; Yusuf, A.; Bhagchandani, R.K.; Gupta, S. A Study for the Development of Prosthetic Foot by Additive Manufacturing. J. Braz. Soc. Mech. Sci. Eng. 2023, 45, 187. [Google Scholar] [CrossRef]
- ISO 10993-1:2018; Biological Evaluation of Medical Devices—Part 1: Evaluation and Testing within a Risk Management Process. International Organization for Standardization: Geneva, Switzerland, 2018.
- ISO 527-1:2012; Plastics—Determination of Tensile Properties—Part 1: General Principles. Second edition; International Organization for Standardization: Geneva, Switzerland, 2012.
- Pepeliaev, A.; Lobov, E.; Vindokurov, I.; Tashkinov, M. Comparison of Mechanical Properties of 3D-Printed ABS, PA12 and PET-G Reinforced with Short Fiber. Procedia Struct. Integr. 2024, 61, 224–231. [Google Scholar] [CrossRef]
- Hambli, R. Multiscale Prediction of Crack Density and Crack Length Accumulation in Trabecular Bone Based on Neural Networks and Finite Element Simulation. Int. J. Numer. Methods Biomed. Eng. 2010, 27, 461–475. [Google Scholar] [CrossRef]
- Dolgikh, D.; Tashkinov, M.; Sudoplatova, D.; Silberschmidt, V.V. Introducing Microarchitecture into 3D-Printed Prosthesis Socket: Pressure Distribution and Mechanical Performance. Med. Eng. Phys. 2023, 122, 104075. [Google Scholar] [CrossRef]
- Dolgikh, D.A.; Tashkinov, M.A.; Silberschmidt, V.V. Pressure Redistribution in Additively Manufactured Composite Prosthesis by Architecture Control. Multiscale Multidiscip. Model. Exp. Des. 2023, 7, 1565–1580. [Google Scholar] [CrossRef]
- Mazars, J.; Pijaudier-Cabot, G. From Damage to Fracture Mechanics and Conversely a Combined Approach. In Proceedings of the Proceedings of Engineering Mechanics, Boulder, CO, USA, 21–24 May 1995. [Google Scholar]
- Chacón, J.M.; Caminero, M.A.; Núñez, P.J.; García-Plaza, E.; García-Moreno, I.; Reverte, J.M. Additive Manufacturing of Continuous Fibre Reinforced Thermoplastic Composites Using Fused Deposition Modelling: Effect of Process Parameters on Mechanical Properties. Compos. Sci. Technol. 2019, 181, 107688. [Google Scholar] [CrossRef]
- Jiao, W.; Chen, L.; Xie, J.; Yang, Z.; Fang, J.; Chen, L. Effect of Weaving Structures on the Geometry Variations and Mechanical Properties of 3D LTL Woven Composites. Compos. Struct. 2020, 252, 112756. [Google Scholar] [CrossRef]
- Zhang, J.; Huang, S.; Gu, B.; Sun, B. Fiber Architecture Effect on Tensile and Compressive Damage Mechanisms of 3D Angle-Interlock Woven Composites. Compos. Commun. 2023, 40, 101582. [Google Scholar] [CrossRef]
- Wielhorski, Y.; Mendoza, A.; Rubino, M.; Roux, S. Numerical Modeling of 3D Woven Composite Reinforcements: A Review. Compos. Part A Appl. Sci. Manuf. 2022, 154, 106729. [Google Scholar] [CrossRef]

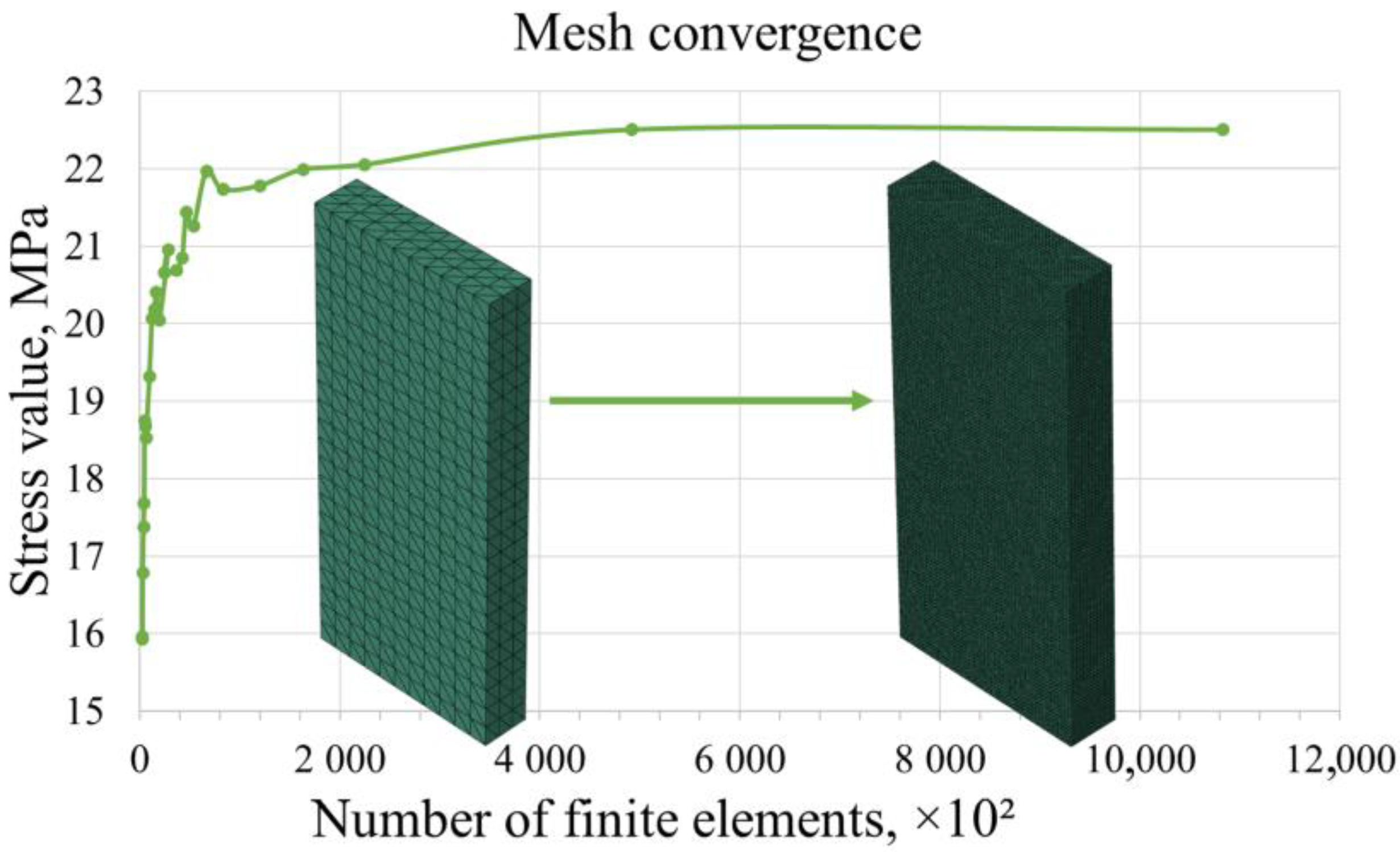
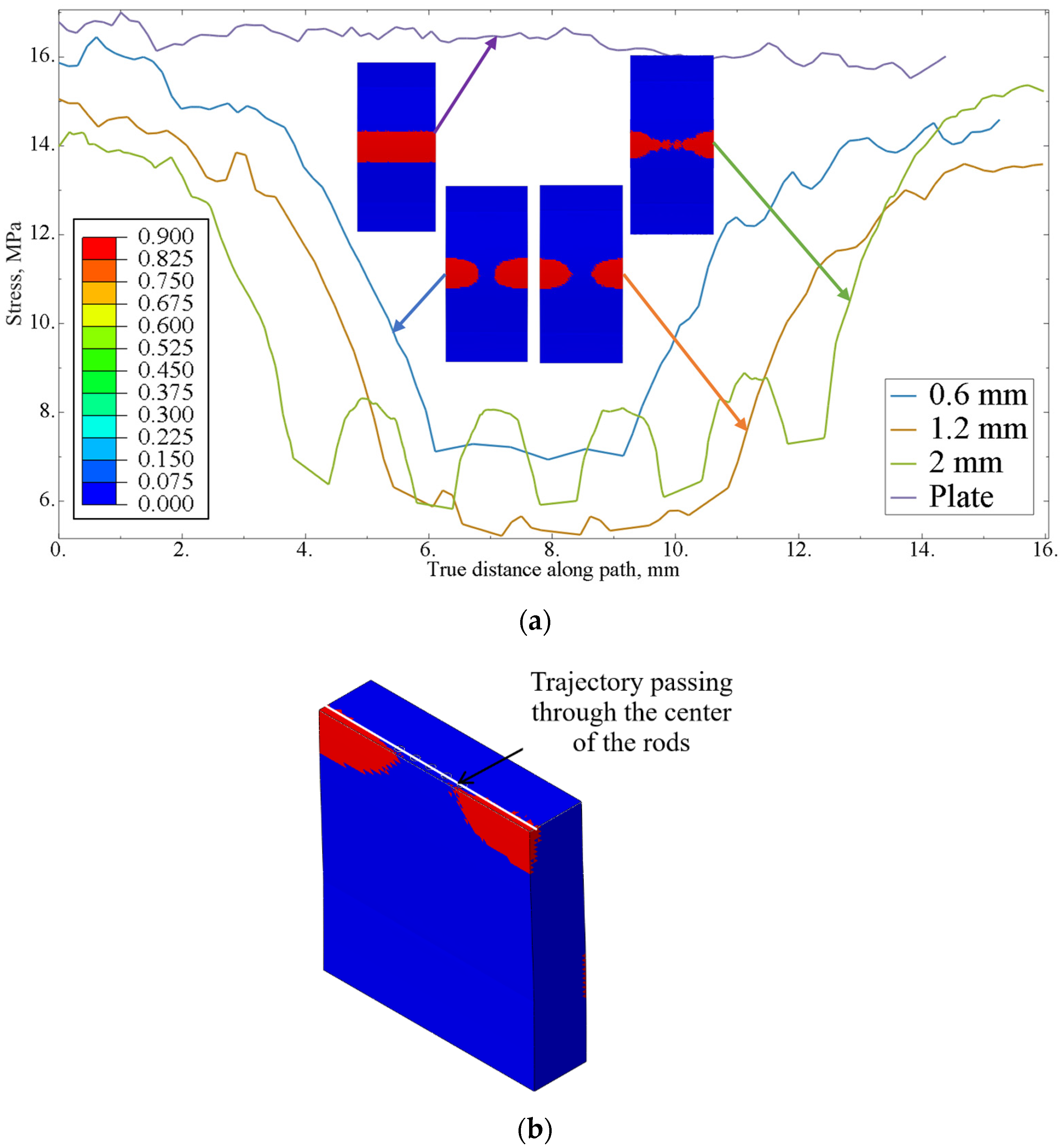

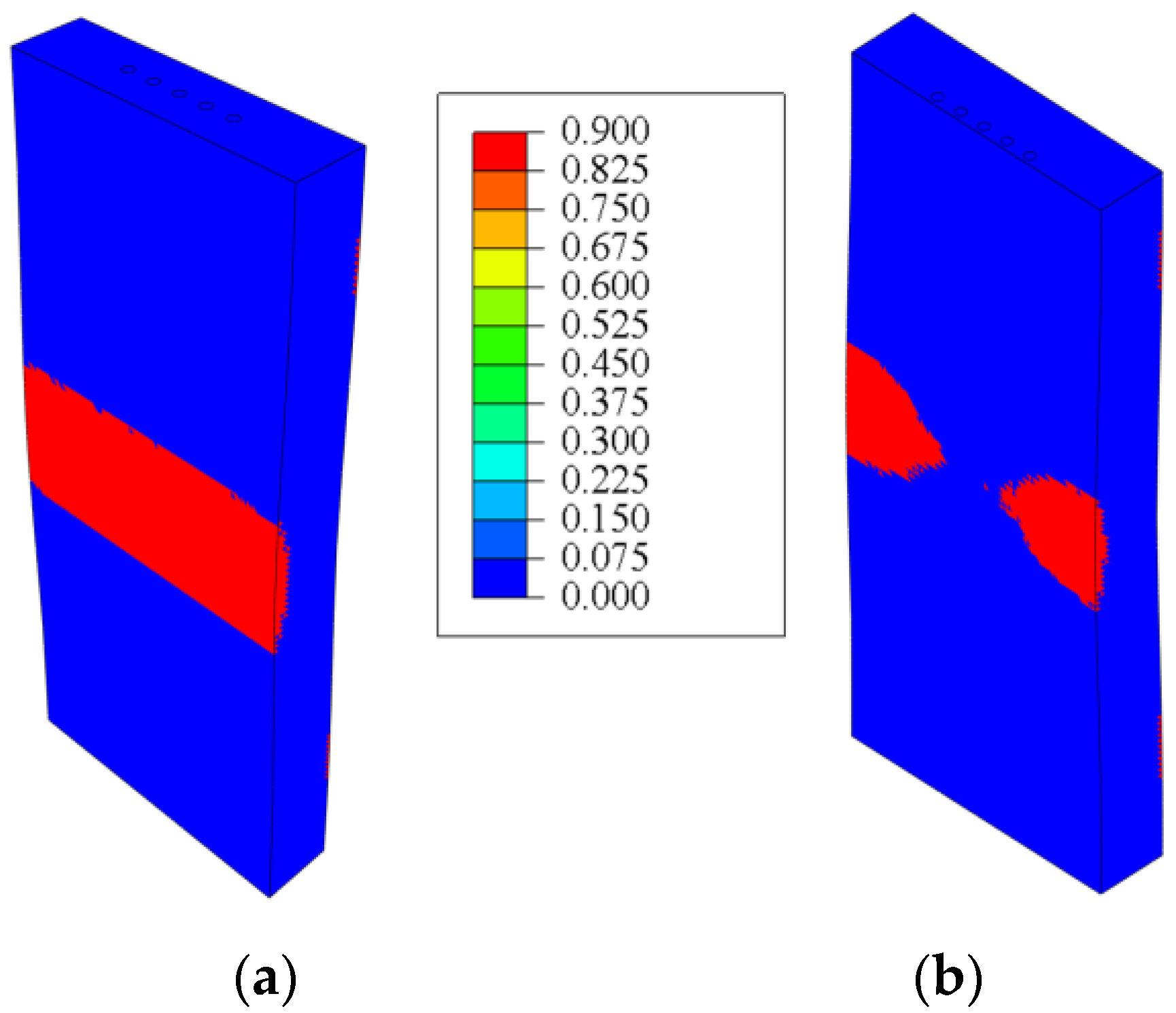


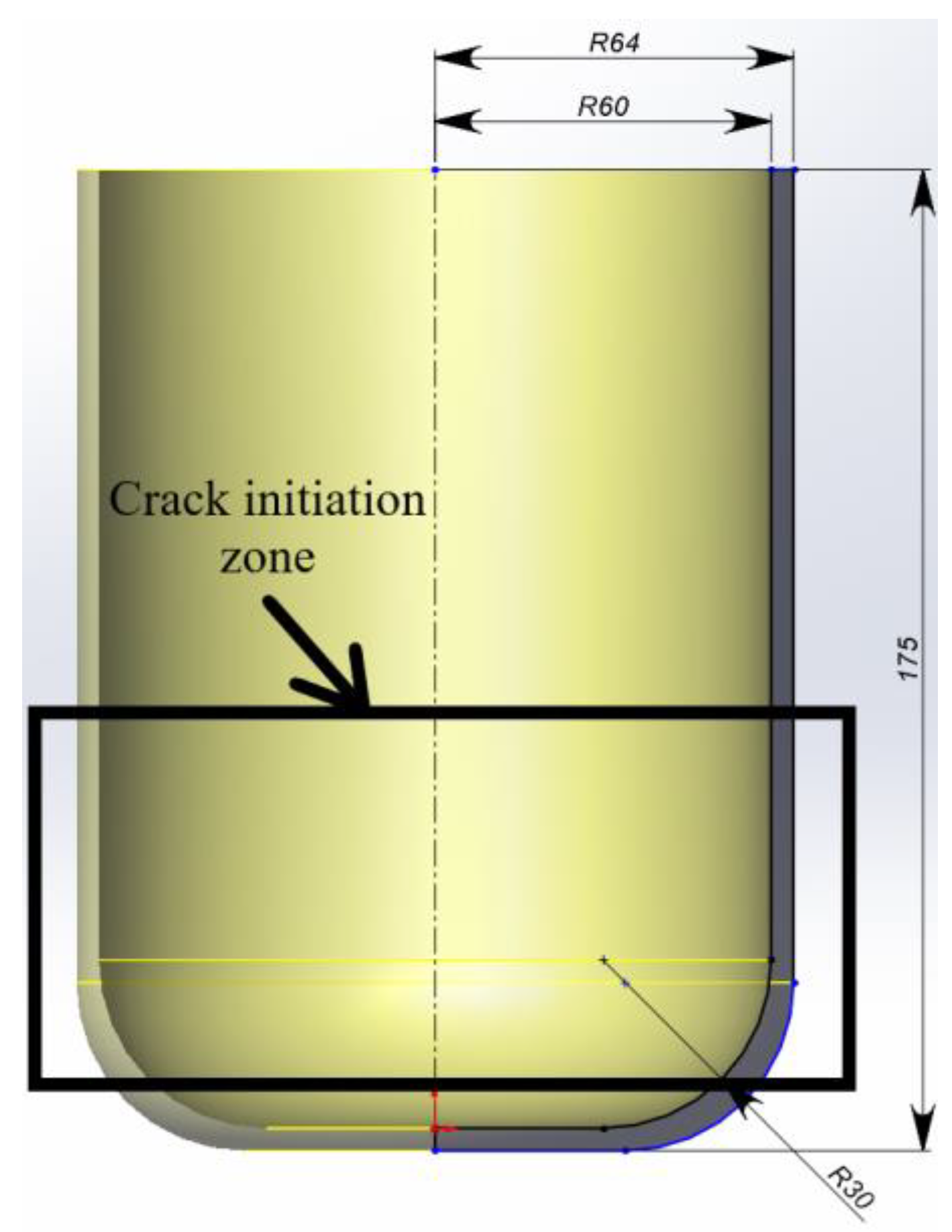





| Material | Elastic Modulus E, MPa | , MPa | Density (g/cm3) | Source | |
|---|---|---|---|---|---|
| Carbon rod | 60 000 | 0.35 | 520 | 1.24 | From manufacturer |
| PA12 | 1445.15 ± 111.18 | 0.3 | 15.47 ± 1.40 | 1.02 | [31] |
| Distance Between Centers of Rods, mm | Maximum Normal Stresses in Rods, MPa | Maximum Normal Stresses in Polymer on the Outer Surface of Samples, MPa | , Rods | , Polymer |
|---|---|---|---|---|
| 0.6 | 385.04 | 10.92 | 1.35 | 1.42 |
| 0.7 | 401.91 | 11.70 | 1.29 | 1.32 |
| 0.8 | 415.64 | 12.15 | 1.25 | 1.27 |
| 0.9 | 423.23 | 12.40 | 1.23 | 1.25 |
| 1 | 439.89 | 13.46 | 1.18 | 1.15 |
| 1.1 | 448.30 | 13.52 | 1.16 | 1.14 |
| 1.2 | 446.90 | 13.62 | 1.16 | 1.14 |
| 1.3 | 451.20 | 13.26 | 1.15 | 1.17 |
| 1.4 | 460.18 | 13.72 | 1.13 | 1.13 |
| 1.5 | 461.71 | 13.89 | 1.13 | 1.11 |
| 1.6 | 467.45 | 14.73 | 1.11 | 1.05 |
| 1.7 | 474.58 | 15.08 | 1.09 | 1.03 |
| 1.8 | 477.56 | 15.17 | 1.09 | 1.02 |
| 1.9 | 485.59 | 15.53 | 1.07 | 0.99 |
| 2 | 512.61 | 16.70 | 1.01 | 0.92 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dolgikh, D.; Lobov, E.; Bezukladnikov, I.; Shalimov, A.; Tashkinov, M. Enhancing the Strength of 3D-Printed Polymer Exoprosthetic Socket by Localized Non-Planar Continuous Carbon Fiber Reinforcement. Polymers 2025, 17, 1097. https://doi.org/10.3390/polym17081097
Dolgikh D, Lobov E, Bezukladnikov I, Shalimov A, Tashkinov M. Enhancing the Strength of 3D-Printed Polymer Exoprosthetic Socket by Localized Non-Planar Continuous Carbon Fiber Reinforcement. Polymers. 2025; 17(8):1097. https://doi.org/10.3390/polym17081097
Chicago/Turabian StyleDolgikh, Daria, Evgeniy Lobov, Igor Bezukladnikov, Aleksandr Shalimov, and Mikhail Tashkinov. 2025. "Enhancing the Strength of 3D-Printed Polymer Exoprosthetic Socket by Localized Non-Planar Continuous Carbon Fiber Reinforcement" Polymers 17, no. 8: 1097. https://doi.org/10.3390/polym17081097
APA StyleDolgikh, D., Lobov, E., Bezukladnikov, I., Shalimov, A., & Tashkinov, M. (2025). Enhancing the Strength of 3D-Printed Polymer Exoprosthetic Socket by Localized Non-Planar Continuous Carbon Fiber Reinforcement. Polymers, 17(8), 1097. https://doi.org/10.3390/polym17081097






