Enhancing Mechanical Properties of Chitosan–Silica Aerogels with Tricalcium Phosphate Nanoparticles: A Molecular Dynamics Study for Bone Tissue Engineering
Abstract
1. Introduction
2. Simulation Approach
2.1. MD Simulation
2.2. Details of Simulation
2.3. Limitations
3. Results and Discussion
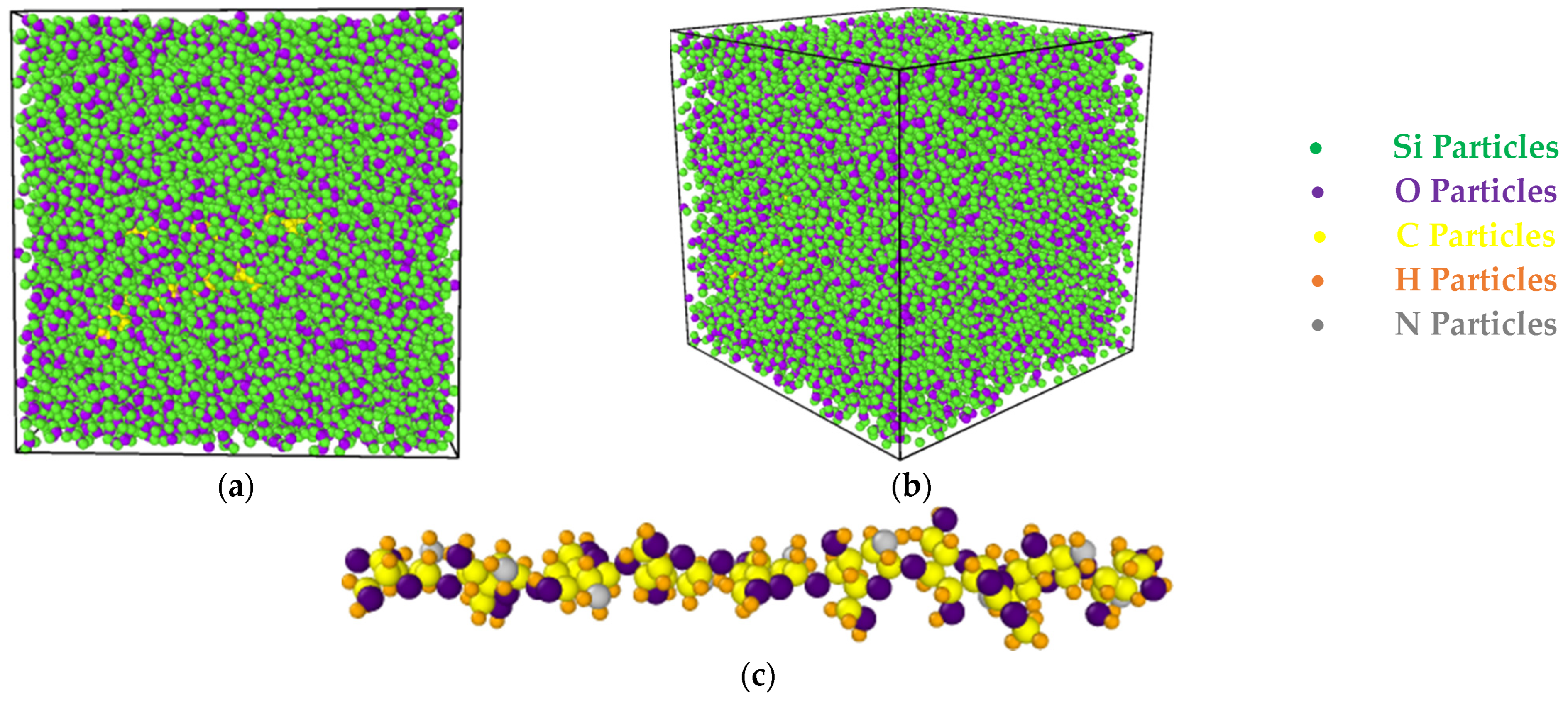
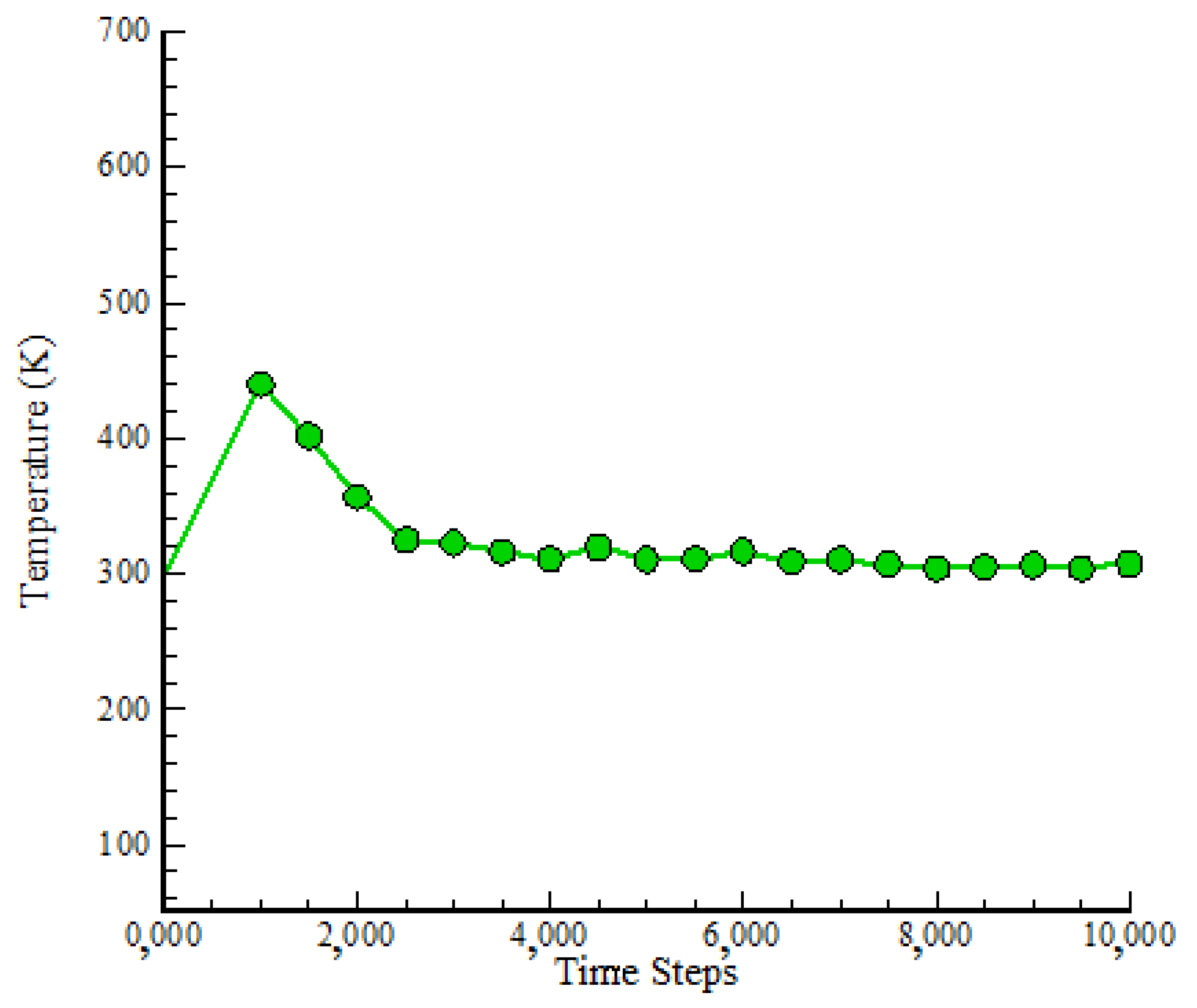
3.1. The Results of the Atomic Structure Balancing Step
3.2. RDF
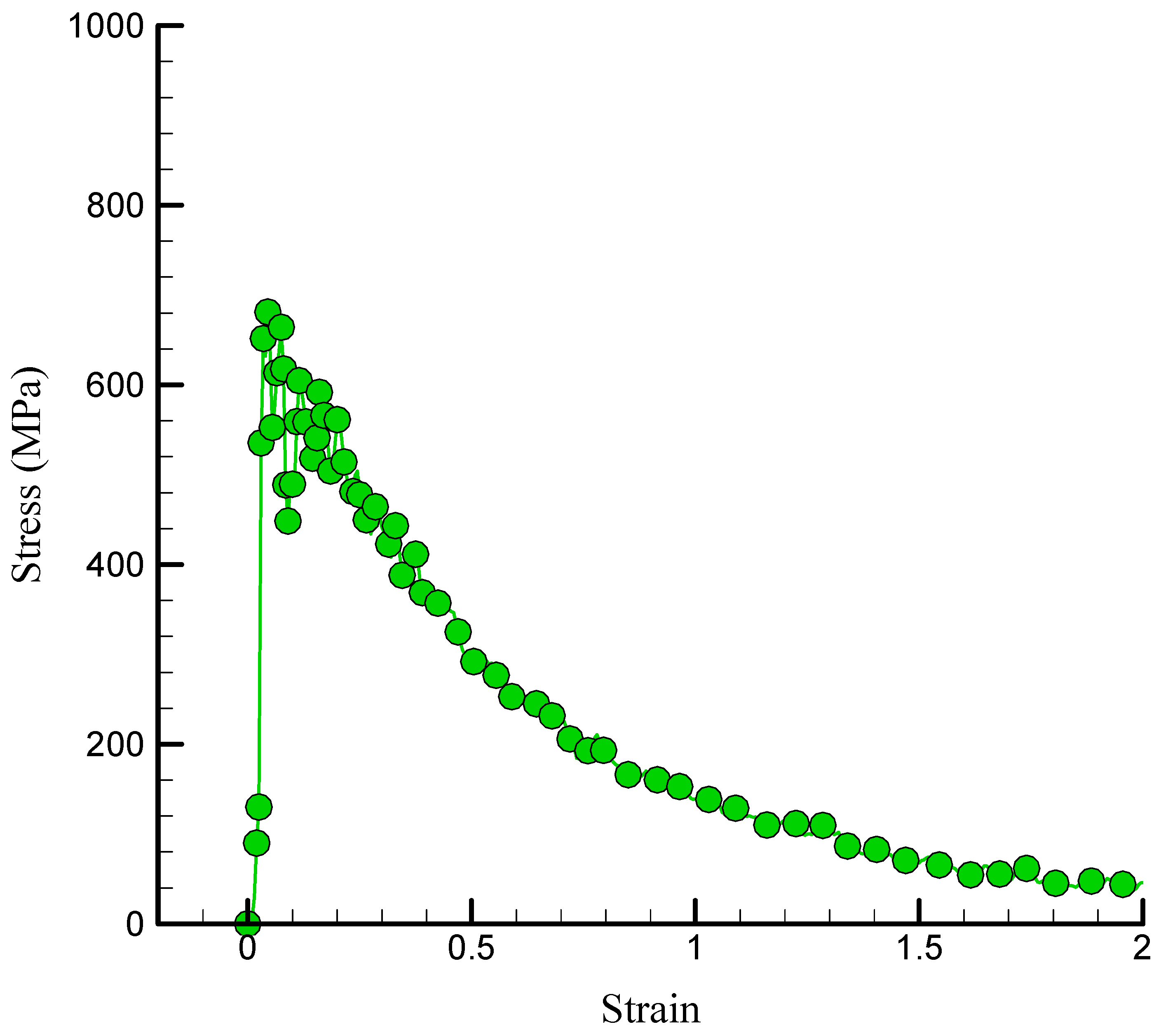
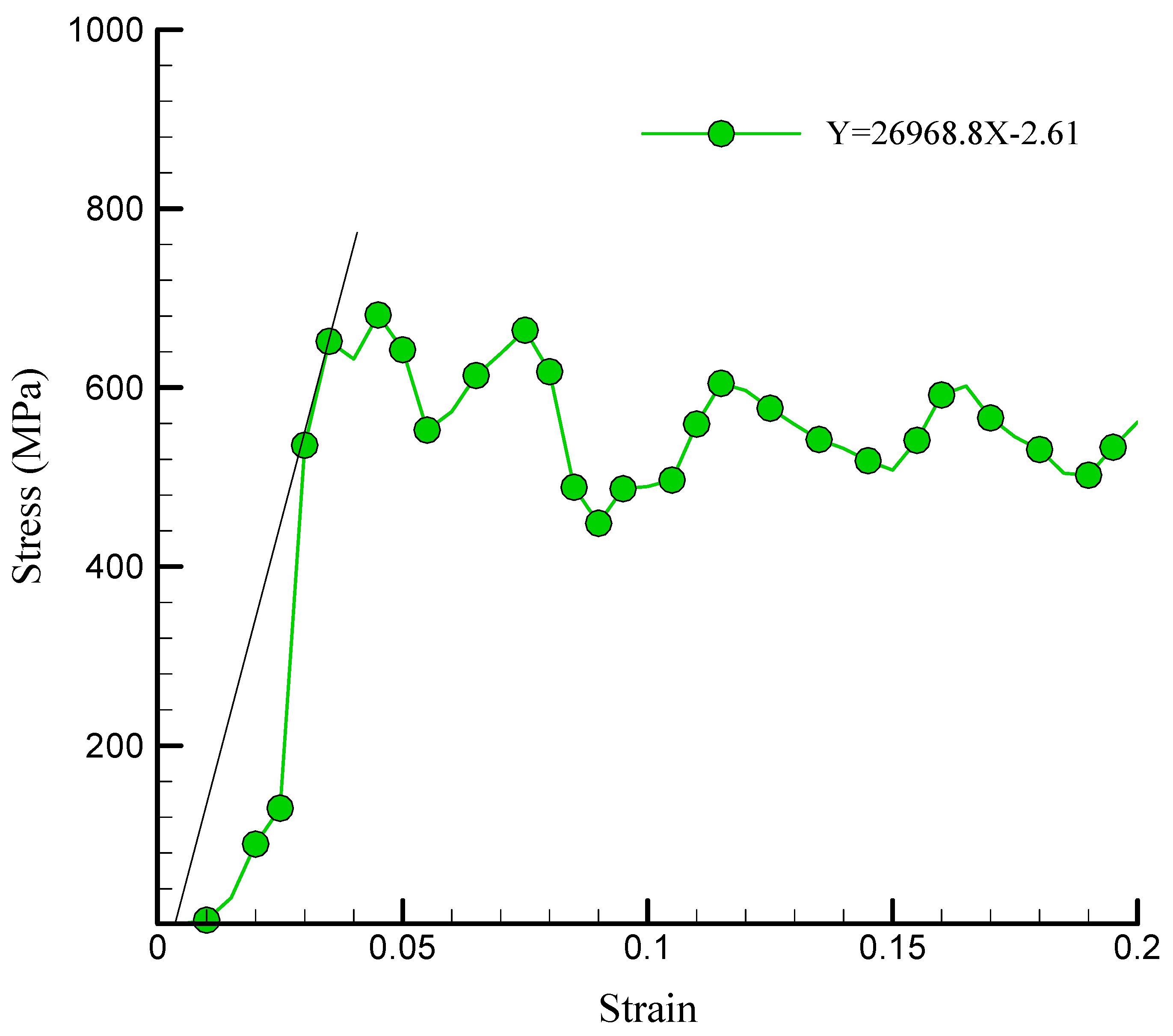
3.3. The Results Related to the Mechanical Attributes of the Modeled NC
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviation
| Nanocomposites | NCs |
| Tricalcium phosphate | TCP |
| Poly-3-hydroxybutyrate | PHB |
| Temperature | Temp |
| Amorphous Calcium Phosphate | ACP |
| Nanoparticles | NPs |
| Hydroxyapatite | HA |
| Molecular Dynamics | MD |
| Potential Energy | PE |
| Ultimate Strength | US |
| Young’s Modulus | YM |
| Radial Distribution Function | RDF |
References
- Fu, S.; Sun, Z.; Huang, P.; Li, Y.; Hu, N. Some basic aspects of polymer nanocomposites: A critical review. Nano Mater. Sci. 2019, 1, 2–30. [Google Scholar] [CrossRef]
- Okpala, C.C. The benefits and applications of nanocomposites. Int. J. Adv. Eng. Technol. 2014, 12, 18. [Google Scholar]
- Suryanarayana, C.; Al-Aqeeli, N. Mechanically alloyed nanocomposites. Prog. Mater. Sci. 2013, 58, 383–502. [Google Scholar] [CrossRef]
- Wetzel, B.; Haupert, F.; Zhang, M.Q. Epoxy nanocomposites with high mechanical and tribological performance. Compos. Sci. Technol. 2003, 63, 2055–2067. [Google Scholar] [CrossRef]
- Bagherifard, A.; Joneidi Yekta, H.; Akbari Aghdam, H.; Motififard, M.; Sanatizadeh, E.; Ghadiri Nejad, M.; Esmaeili, S.; Saber-Samandari, S.; Sheikhbahaei, E.; Khandan, A. Improvement in osseointegration of tricalcium phosphate-zircon for orthopedic applications: An in vitro and in vivo evaluation. Med. Biol. Eng. Comput. 2020, 58, 1681–1693. [Google Scholar] [CrossRef] [PubMed]
- Keikhaei, S.; Mohammadalizadeh, Z.; Karbasi, S.; Salimi, A. Evaluation of the effects of β-tricalcium phosphate on physical, mechanical and biological properties of Poly (3-hydroxybutyrate)/chitosan electrospun scaffold for cartilage tissue engineering applications. Mater. Technol. 2019, 34, 615–625. [Google Scholar] [CrossRef]
- Sprio, S.; Guicciardi, S.; Dapporto, M.; Melandri, C.; Tampieri, A. Synthesis and mechanical behavior of β-tricalcium phosphate/titania composites addressed to regeneration of long bone segments. J. Mech. Behav. Biomed. Mater. 2013, 17, 1–10. [Google Scholar] [CrossRef]
- Hou, X.; Zhang, L.; Zhou, Z.; Luo, X.; Wang, T.; Zhao, X.; Lu, B.; Chen, F.; Zheng, L. Calcium phosphate-based biomaterials for bone repair. J. Funct. Biomater. 2022, 13, 187. [Google Scholar] [CrossRef]
- Almulhim, K.S.; Syed, M.R.; Alqahtani, N.; Alamoudi, M.; Khan, M.; Ahmed, S.Z.; Khan, A.S. Bioactive inorganic materials for dental applications: A narrative review. Materials 2022, 15, 6864. [Google Scholar] [CrossRef]
- Ong, K.L.; Yun, B.M.; White, J.B. New biomaterials for orthopedic implants. Orthop. Res. Rev. 2015, 7, 107–130. [Google Scholar] [CrossRef]
- Zerankeshi, M.M.; Mofakhami, S.; Salahinejad, E. 3D porous HA/TCP composite scaffolds for bone tissue engineering. Ceram. Int. 2022, 48, 22647–22663. [Google Scholar] [CrossRef]
- Shuai, C.; Yu, L.; Feng, P.; Gao, C.; Peng, S. Interfacial reinforcement in bioceramic/biopolymer composite bone scaffold: The role of coupling agent. Colloids Surf. B Biointerfaces 2020, 193, 111083. [Google Scholar] [CrossRef] [PubMed]
- Mirjalili, F.; Mohammadi, H.; Azimi, M.; Hafezi, M.; Osman, N.A.A. Synthesis and characterization of β-TCP/CNT nanocomposite: Morphology, microstructure and in vitro bioactivity. Ceram. Int. 2017, 43, 7573–7580. [Google Scholar] [CrossRef]
- Huang, Q.; Liang, Z.; Li, J.; Bai, Y.; He, J.; Lin, Z. Size dependence of particulate calcium phosphate fillers in dental resin composites. ACS Omega 2021, 6, 35057–35066. [Google Scholar] [CrossRef] [PubMed]
- Bakhori, N.M.; Ismail, Z.; Hassan, M.Z.; Dolah, R. Emerging trends in nanotechnology: Aerogel-based materials for biomedical applications. Nanomaterials 2023, 13, 1063. [Google Scholar] [CrossRef]
- Salama, A. Recent progress in preparation and applications of chitosan/calcium phosphate composite materials. Int. J. Biol. Macromol. 2021, 178, 240–252. [Google Scholar] [CrossRef]
- Meti, P.; Mahadik, D.; Lee, K.-Y.; Wang, Q.; Kanamori, K.; Gong, Y.-D.; Park, H.-H. Overview of organic–inorganic hybrid silica aerogels: Progress and perspectives. Mater. Des. 2022, 222, 111091. [Google Scholar] [CrossRef]
- Maleki, H.; Durães, L.; García-González, C.A.; Del Gaudio, P.; Portugal, A.; Mahmoudi, M. Synthesis and biomedical applications of aerogels: Possibilities and challenges. Adv. Colloid Interface Sci. 2016, 236, 1–27. [Google Scholar] [CrossRef]
- Fourie, J.; Taute, F.; du Preez, L.; De Beer, D. Chitosan composite biomaterials for bone tissue engineering—A review. Regen. Eng. Transl. Med. 2022, 8, 1–21. [Google Scholar] [CrossRef]
- Kołodziejska, M.; Jankowska, K.; Klak, M.; Wszoła, M. Chitosan as an underrated polymer in modern tissue engineering. Nanomaterials 2021, 11, 3019. [Google Scholar] [CrossRef]
- Pérez-Moreno, A.; Piñero, M.; Fernández-Montesinos, R.; Pinaglia-Tobaruela, G.; Reyes-Peces, M.V.; Mesa-Díaz, M.d.M.; Vilches-Pérez, J.I.; Esquivias, L.; de la Rosa-Fox, N.; Salido, M. Chitosan-Silica Hybrid Biomaterials for Bone Tissue Engineering: A Comparative Study of Xerogels and Aerogels. Gels 2023, 9, 383. [Google Scholar] [CrossRef] [PubMed]
- Shahi, S.; Karbasi, S. Evaluation of physical and mechanical properties of B-tri-calcium phosphate/poly-3-hydroxybutyrate nanocomposite scaffold for bone tissue engineering application. Sci. Iran. 2017, 24, 1654–1668. [Google Scholar] [CrossRef][Green Version]
- Pérez-Moreno, A.; Reyes-Peces, M.V.; Vilches-Pérez, J.I.; Fernández-Montesinos, R.; Pinaglia-Tobaruela, G.; Salido, M.; De la Rosa-Fox, N.; Piñero, M. Effect of washing treatment on the textural properties and bioactivity of silica/chitosan/TCP xerogels for bone regeneration. Int. J. Mol. Sci. 2021, 22, 8321. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Ei-Ghannam, A. Effect of processing parameters on the microstructure and mechanical behavior of silica-calcium phosphate nanocomposite. J. Mater. Sci. Mater. Med. 2010, 21, 2087–2094. [Google Scholar] [CrossRef]
- Hu, X.; Zhang, W.; Hou, D. Synthesis, microstructure and mechanical properties of tricalcium phosphate–hydroxyapatite (TCP/HA) composite ceramic. Ceram. Int. 2020, 46, 9810–9816. [Google Scholar] [CrossRef]
- Available online: https://www.researchgate.net/publication/319911837_Nanostructured_bioactive_silicachitosanTCP_aerogels_for_tissue_engineering (accessed on 29 January 2024).
- Alder, B.J.; Wainwright, T.E. Studies in molecular dynamics. I. General method. J. Chem. Phys. 1959, 31, 459–466. [Google Scholar] [CrossRef]
- Rapaport, D.C. The Art of Molecular Dynamics Simulation; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Spreiter, Q.; Walter, M. Classical molecular dynamics simulation with the Velocity Verlet algorithm at strong external magnetic fields. J. Comput. Phys. 1999, 152, 102–119. [Google Scholar] [CrossRef]
- Hairer, E.; Lubich, C.; Wanner, G. Geometric numerical integration illustrated by the Störmer–Verlet method. Acta Numer. 2003, 12, 399–450. [Google Scholar] [CrossRef]
- Solel, E.; Ruth, M.; Schreiner, P.R. London dispersion helps refine steric A-values: Dispersion energy donor scales. J. Am. Chem. Soc. 2021, 143, 20837–20848. [Google Scholar] [CrossRef]
- Nguyen, N.C.; Peraire, J.; Cockburn, B. Hybridizable discontinuous Galerkin methods for the time-harmonic Maxwell’s equations. J. Comput. Phys. 2011, 230, 7151–7175. [Google Scholar] [CrossRef]
- Rappé, A.K.; Casewit, C.J.; Colwell, K.; Goddard, W.A., III; Skiff, W.M. UFF, a full periodic table force field for molecular mechanics and molecular dynamics simulations. J. Am. Chem. Soc. 1992, 114, 10024–10035. [Google Scholar] [CrossRef]
- Mayo, S.L.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Available online: https://docs.lammps.org/pair_tersoff.html (accessed on 29 January 2024).
- Patil, S.P.; Rege, A.; Sagardas; Itskov, M.; Markert, B. Mechanics of nanostructured porous silica aerogel resulting from molecular dynamics simulations. J. Phys. Chem. B 2017, 121, 5660–5668. [Google Scholar] [CrossRef] [PubMed]
- Hanwell, M.D.; Curtis, D.E.; Lonie, D.C.; Vandermeersch, T.; Zurek, E.; Hutchison, G.R. Avogadro: An advanced semantic chemical editor, visualization, and analysis platform. J. Cheminform. 2012, 4, 17. [Google Scholar] [CrossRef]
- Martínez, L.; Andrade, R.; Birgin, E.G.; Martínez, J.M. PACKMOL: A package for building initial configurations for molecular dynamics simulations. J. Comput. Chem. 2009, 30, 2157–2164. [Google Scholar] [CrossRef] [PubMed]
- Gonçalves, W.; Morthomas, J.; Chantrenne, P.; Perez, M.; Foray, G.; Martin, C.L. Elasticity and strength of silica aerogels: A molecular dynamics study on large volumes. Acta Mater. 2018, 145, 165–174. [Google Scholar] [CrossRef]
- Qiang, L.; Li, Z.; Zhao, T.; Zhong, S.; Wang, H.; Cui, X. Atomic-scale interactions of the interface between chitosan and Fe3O4. Colloids Surf. A Physicochem. Eng. Asp. 2013, 419, 125–132. [Google Scholar] [CrossRef]
- Murillo, J.S.R.; Bachlechner, M.E.; Campo, F.A.; Barbero, E.J. Structure and mechanical properties of silica aerogels and xerogels modeled by molecular dynamics simulation. J. Non-Cryst. Solids 2010, 356, 1325–1331. [Google Scholar] [CrossRef]
- Alasvandian, S.; Shahgholi, M.; Karimipour, A. Investigating the effects of chitosan atomic ratio and drug type on mechanical properties of silica aerogel/chitosan nanocomposites using molecular dynamics approach. J. Mol. Liq. 2024, 401, 124639. [Google Scholar] [CrossRef]
- Takeshita, S.; Zhao, S.; Malfait, W.J.; Koebel, M.M. Chemistry of chitosan aerogels: Three-dimensional pore control for tailored applications. Angew. Chem. Int. Ed. 2021, 60, 9828–9851. [Google Scholar] [CrossRef]
- Karamikamkar, S.; Yalcintas, E.P.; Haghniaz, R.; de Barros, N.R.; Mecwan, M.; Nasiri, R.; Davoodi, E.; Nasrollahi, F.; Erdem, A.; Kang, H. Aerogel-based biomaterials for biomedical applications: From fabrication methods to disease-targeting applications. Adv. Sci. 2023, 10, 2204681. [Google Scholar] [CrossRef] [PubMed]
- Ashraf, M.A.; Peng, W.; Zare, Y.; Rhee, K.Y. Effects of size and aggregation/agglomeration of nanoparticles on the interfacial/interphase properties and tensile strength of polymer nanocomposites. Nanoscale Res. Lett. 2018, 13, 214. [Google Scholar] [CrossRef] [PubMed]
- Mohammed, U.; Sun, C. Effect of Nanoparticle Dispersion on Mechanical Behavior of Polymer Nanocomposites. In Proceedings of the 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Palm Springs, CA, USA, 4–7 May 2009. [Google Scholar]
- Wang, Z.; Lv, Q.; Chen, S.; Li, C.; Sun, S.; Hu, S. Effect of interfacial bonding on interphase properties in SiO2/epoxy nanocomposite: A molecular dynamics simulation study. ACS Appl. Mater. Interfaces 2016, 8, 7499–7508. [Google Scholar] [CrossRef] [PubMed]






| Particle Type | ε (kcal/mol) | σ (Å) |
|---|---|---|
| C | 0.105 | 3.851 |
| F | 3.0124 | 2.7329 |
| O | 0.06 | 3.50 |
| H | 0.044 | 2.886 |
| N | 0.415 | 3.995 |
| Si | 0.0043 | 3.69 |
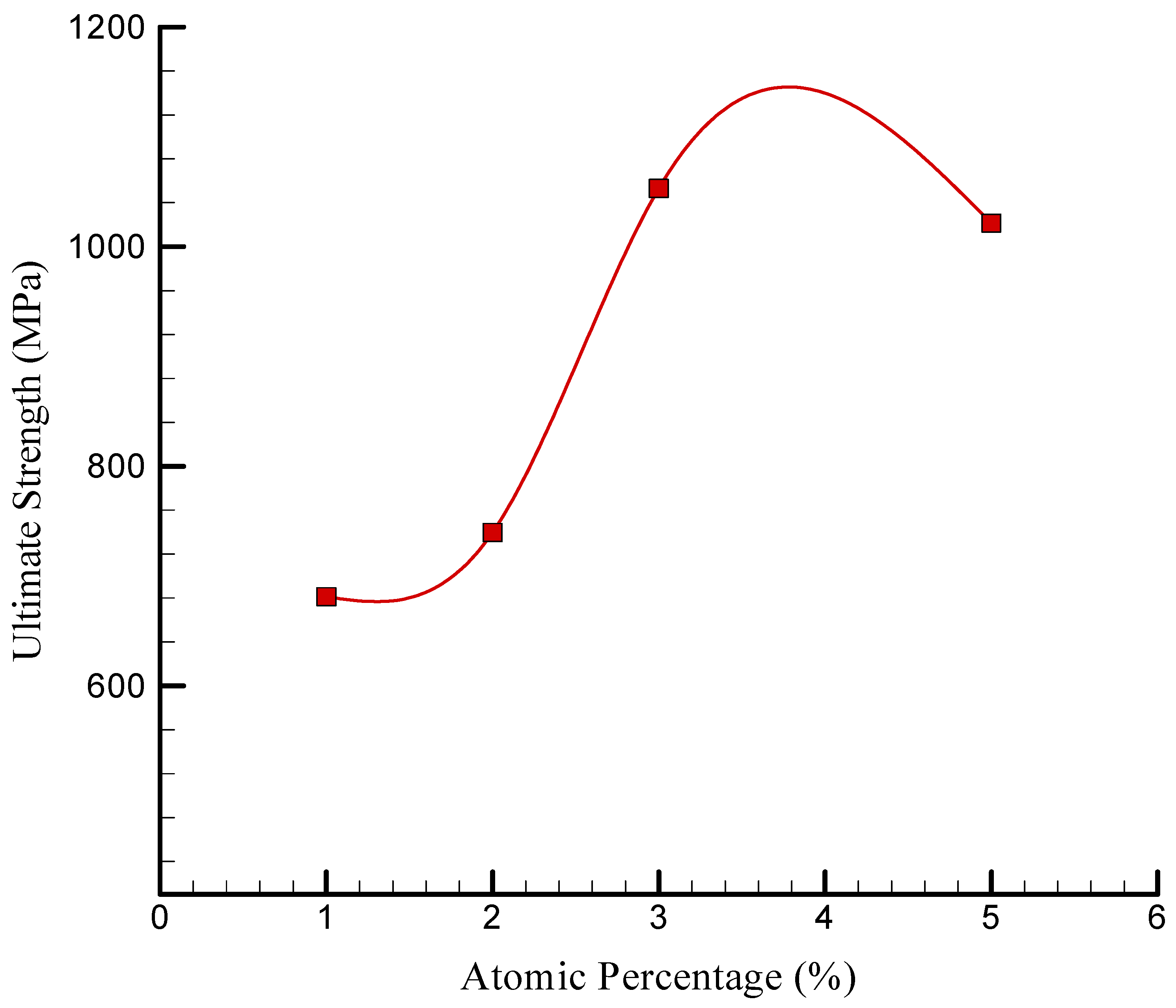
| Atomic Percentage of TCP (%) | US (MPa) | YM (GPa) |
|---|---|---|
| 1 | 681.145 (±3) | 26.968 (±1.02) |
| 2 | 739.742 (±5) | 29.932 (±2.1) |
| 3 | 1053.183 (±2) | 43.468 (±0.89) |
| 5 | 1021.418 (±4) | 42.008 (±1.25) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Attaeyan, A.; Shahgholi, M.; Karimipour, A. Enhancing Mechanical Properties of Chitosan–Silica Aerogels with Tricalcium Phosphate Nanoparticles: A Molecular Dynamics Study for Bone Tissue Engineering. Polymers 2025, 17, 755. https://doi.org/10.3390/polym17060755
Attaeyan A, Shahgholi M, Karimipour A. Enhancing Mechanical Properties of Chitosan–Silica Aerogels with Tricalcium Phosphate Nanoparticles: A Molecular Dynamics Study for Bone Tissue Engineering. Polymers. 2025; 17(6):755. https://doi.org/10.3390/polym17060755
Chicago/Turabian StyleAttaeyan, Ali, Mohamad Shahgholi, and Arash Karimipour. 2025. "Enhancing Mechanical Properties of Chitosan–Silica Aerogels with Tricalcium Phosphate Nanoparticles: A Molecular Dynamics Study for Bone Tissue Engineering" Polymers 17, no. 6: 755. https://doi.org/10.3390/polym17060755
APA StyleAttaeyan, A., Shahgholi, M., & Karimipour, A. (2025). Enhancing Mechanical Properties of Chitosan–Silica Aerogels with Tricalcium Phosphate Nanoparticles: A Molecular Dynamics Study for Bone Tissue Engineering. Polymers, 17(6), 755. https://doi.org/10.3390/polym17060755







