Model Prediction and Experimental Validation of Transverse Permeability of Large-Tow Carbon Fiber Composites
Abstract
1. Introduction
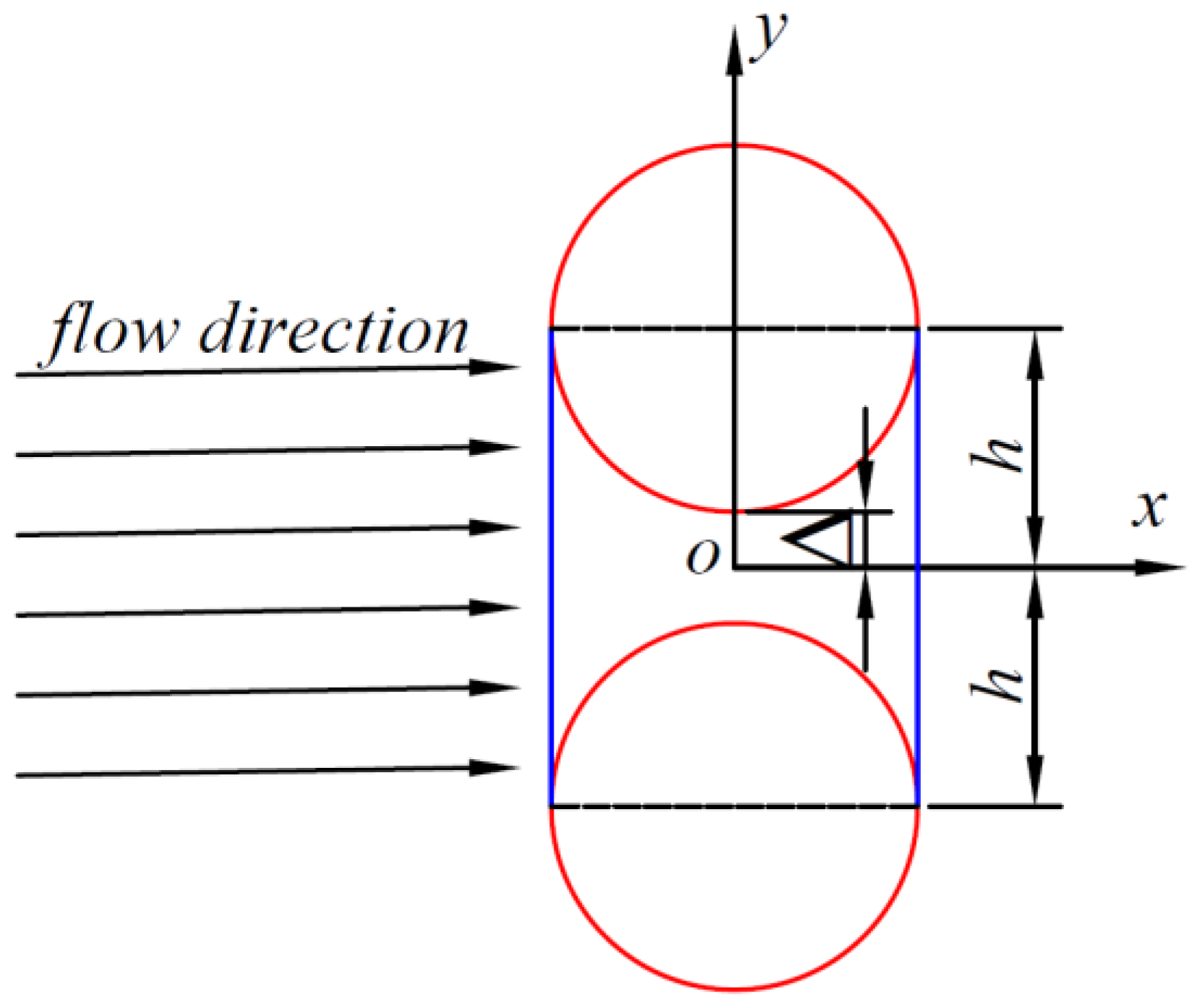
Model Development Based on Monofilament Gap
2. Theoretical Horizontal Permeability
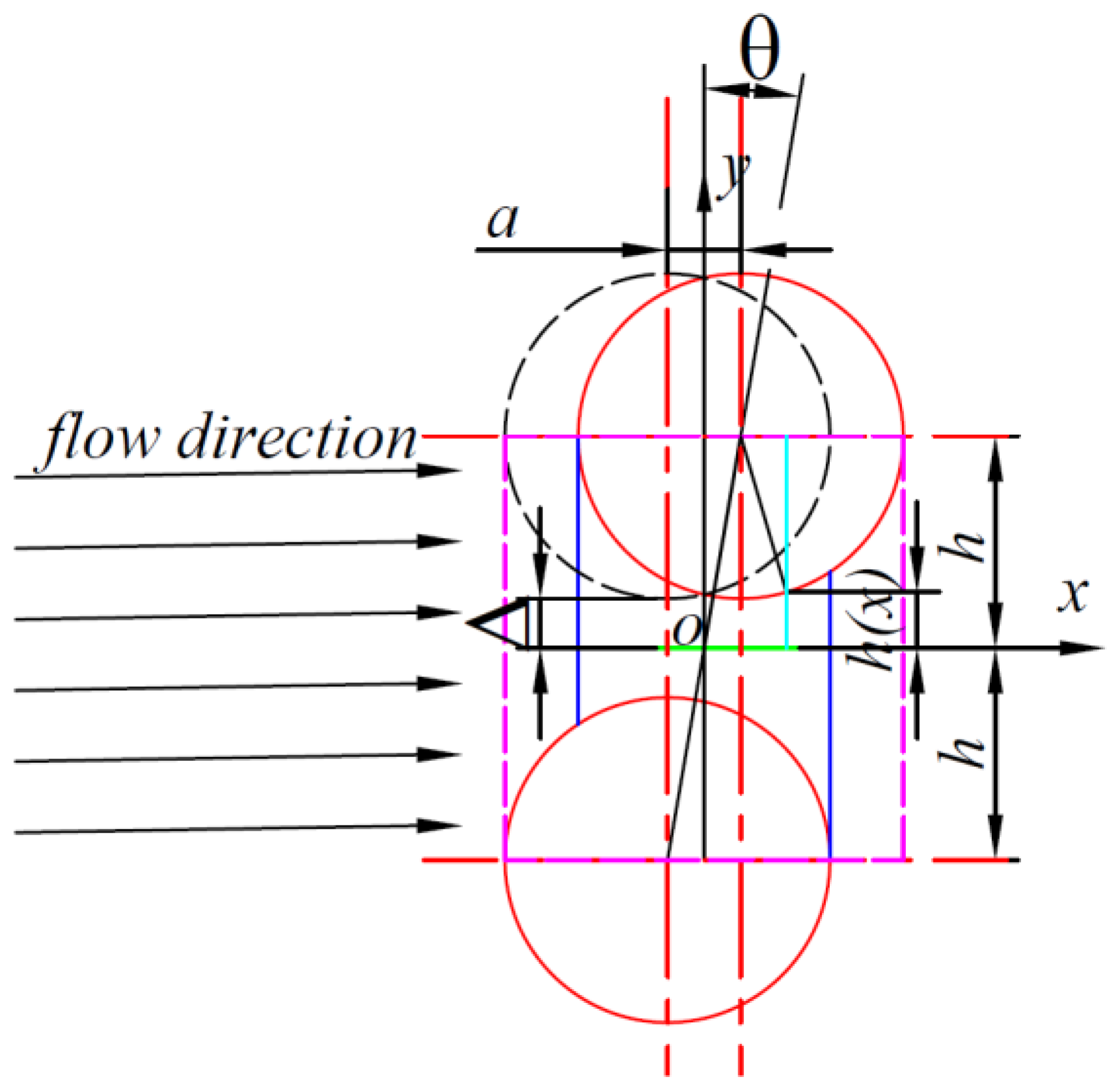
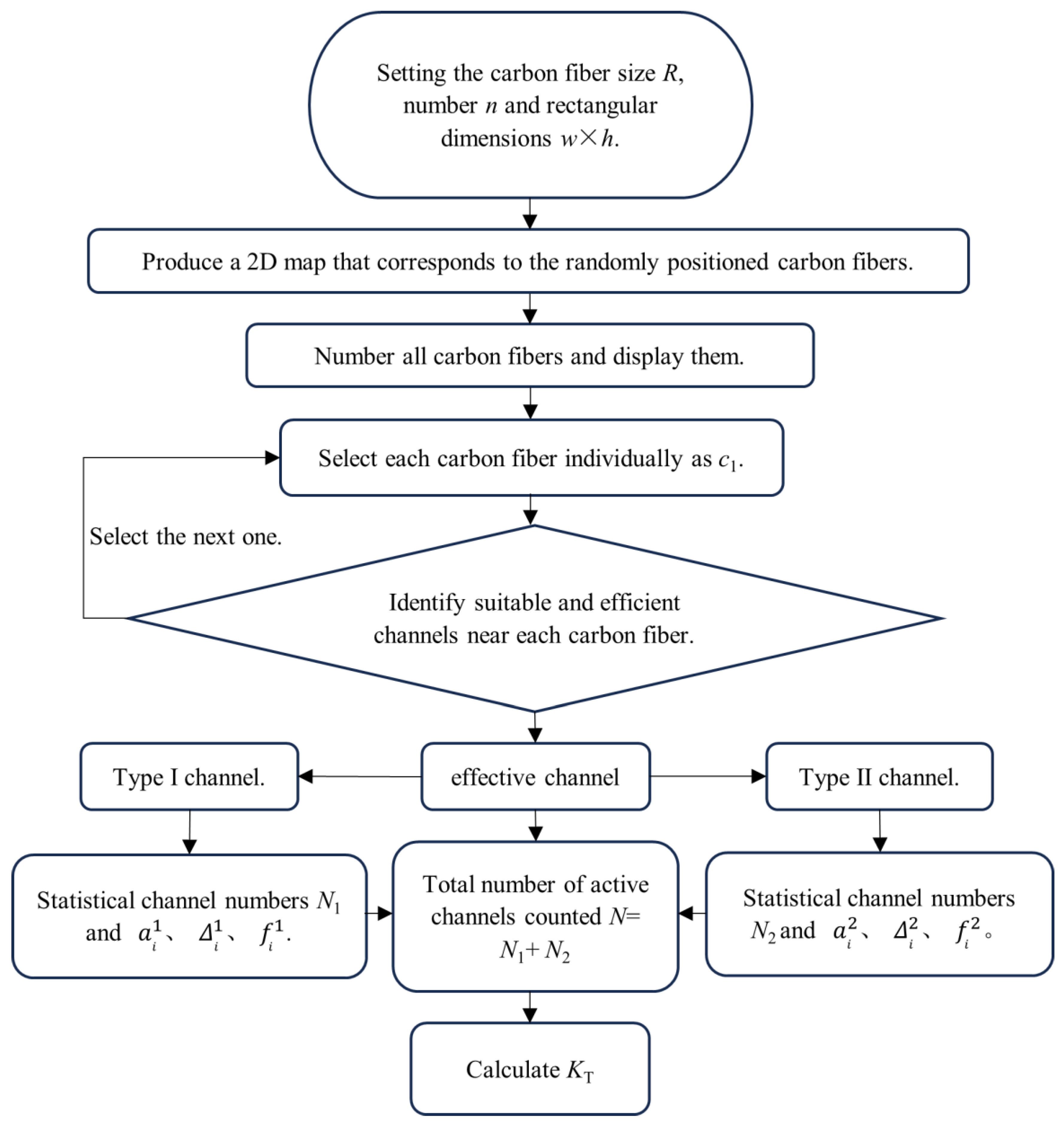
3. Carbon Fiber Large-Tow Transverse Permeability Modeling
3.1. Model Development Based on Monofilament Gap
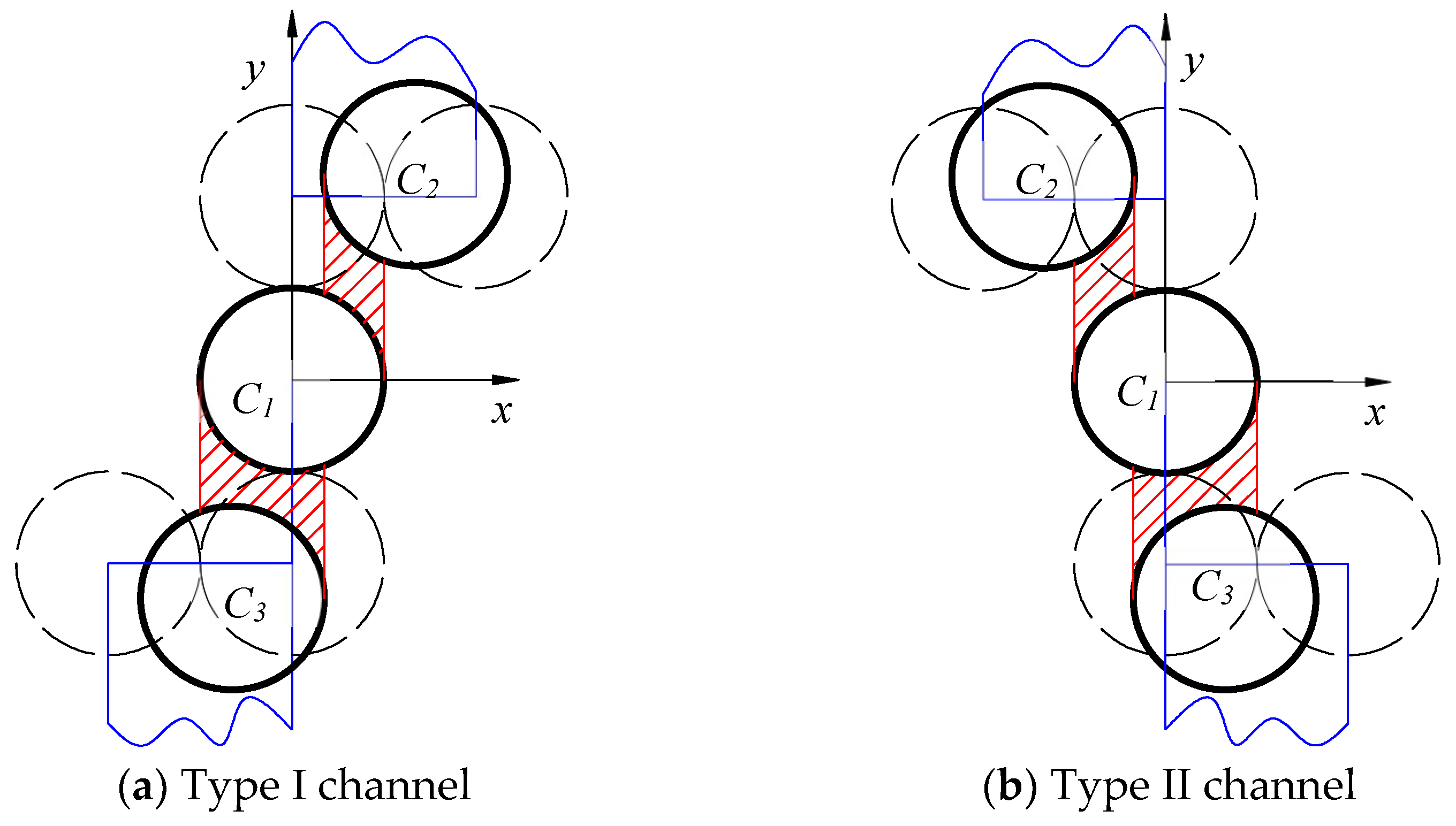
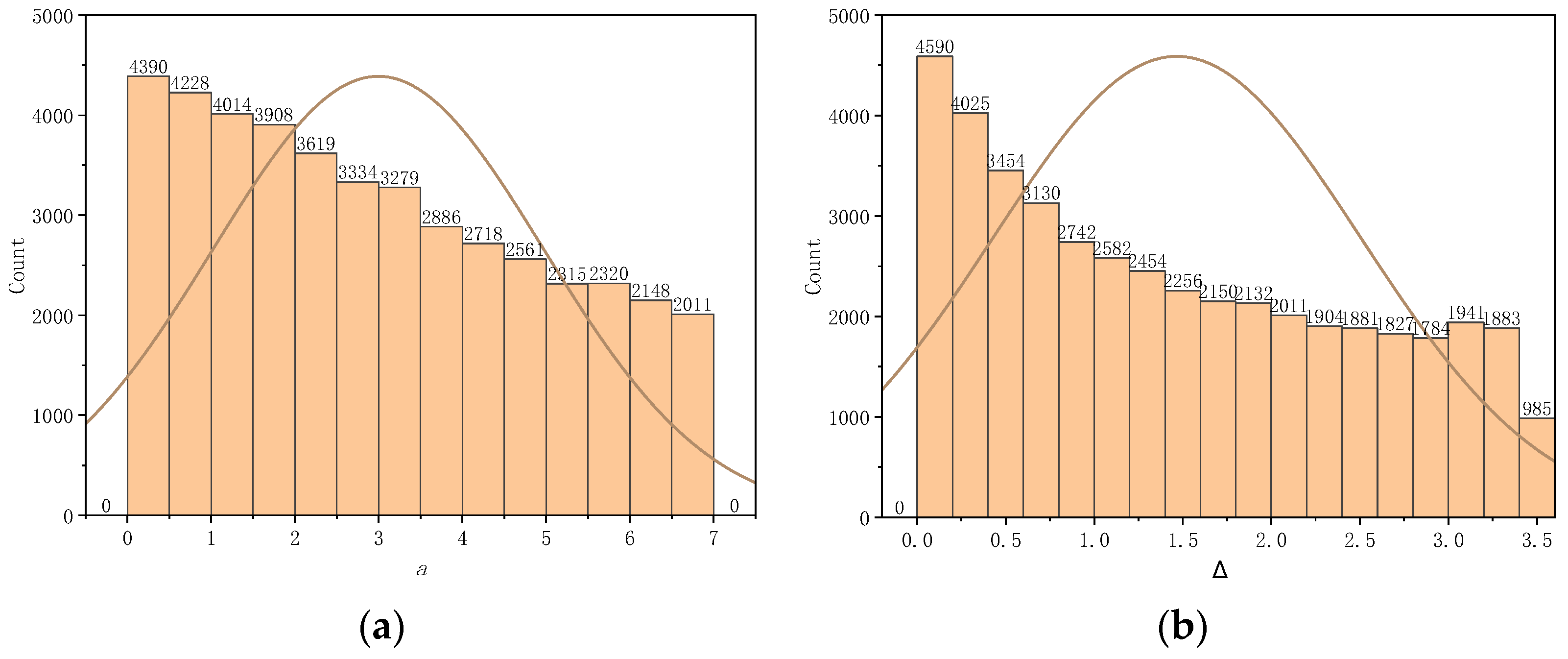
3.2. Large Tow Stochastic Model
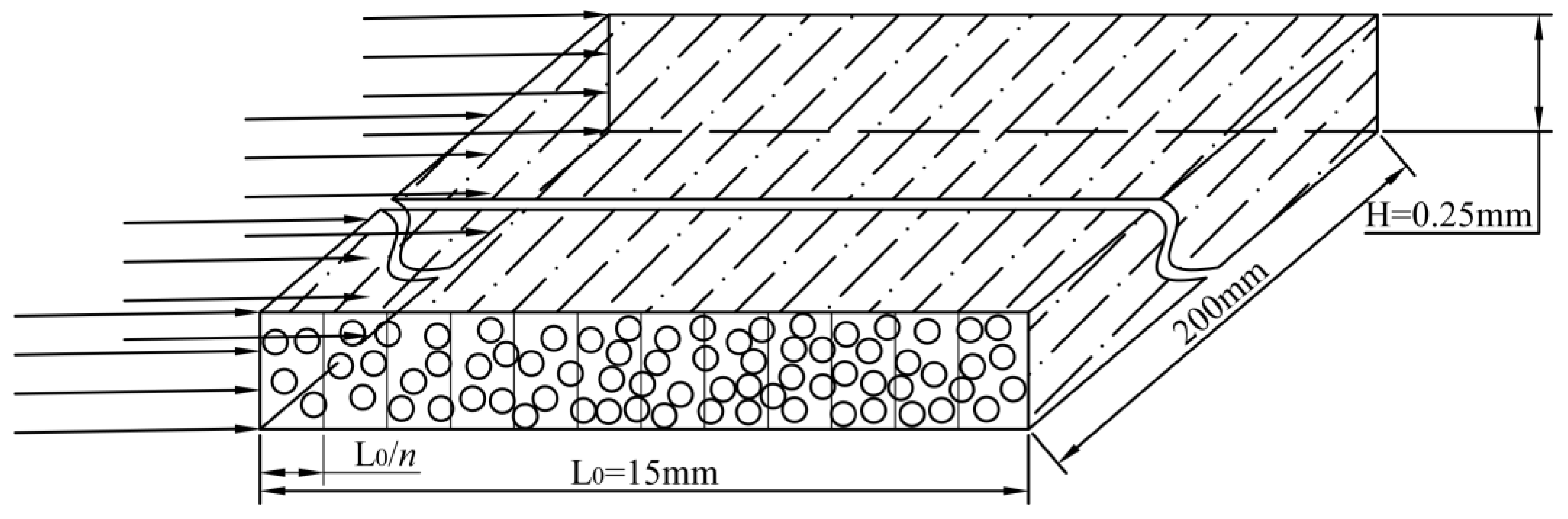
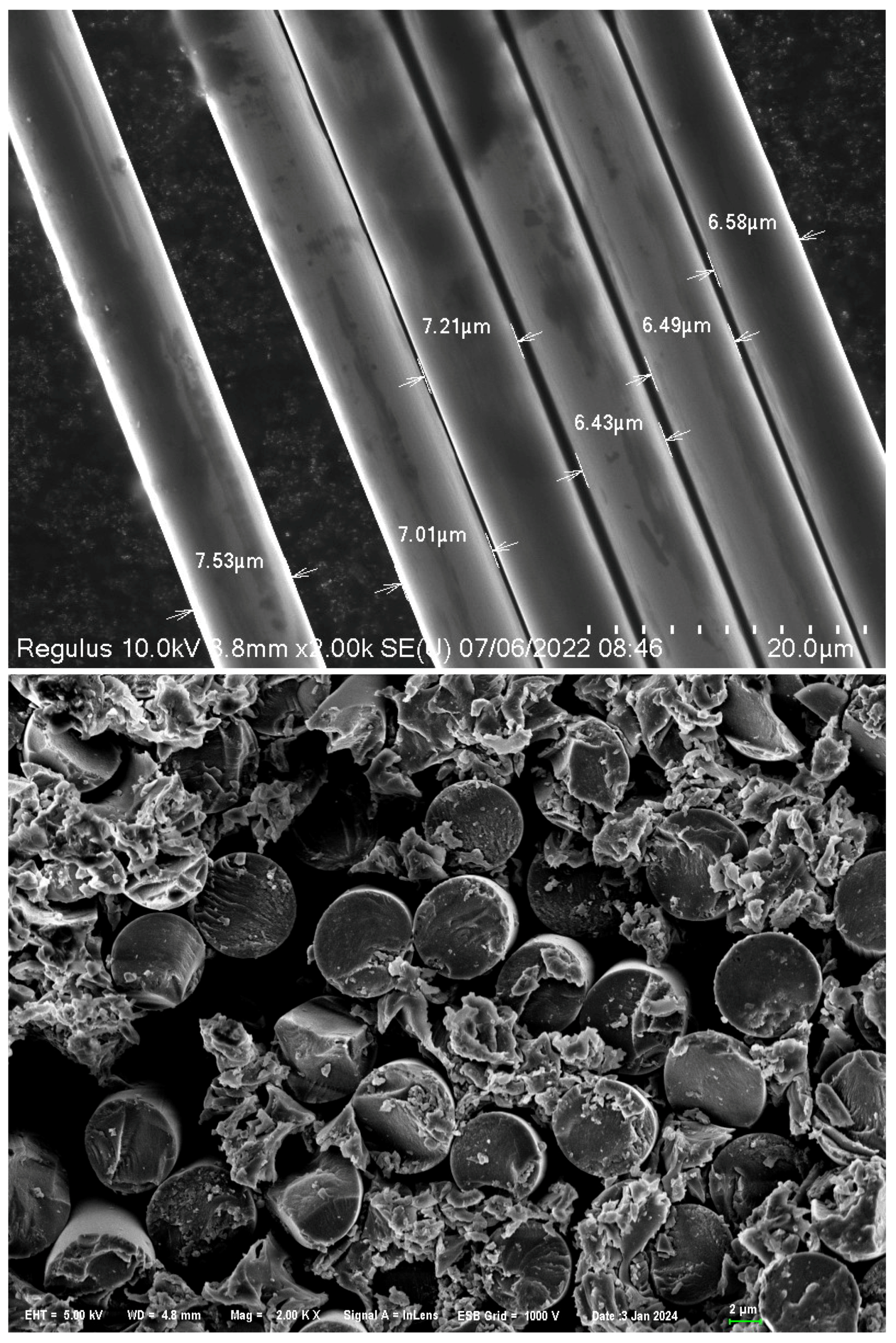
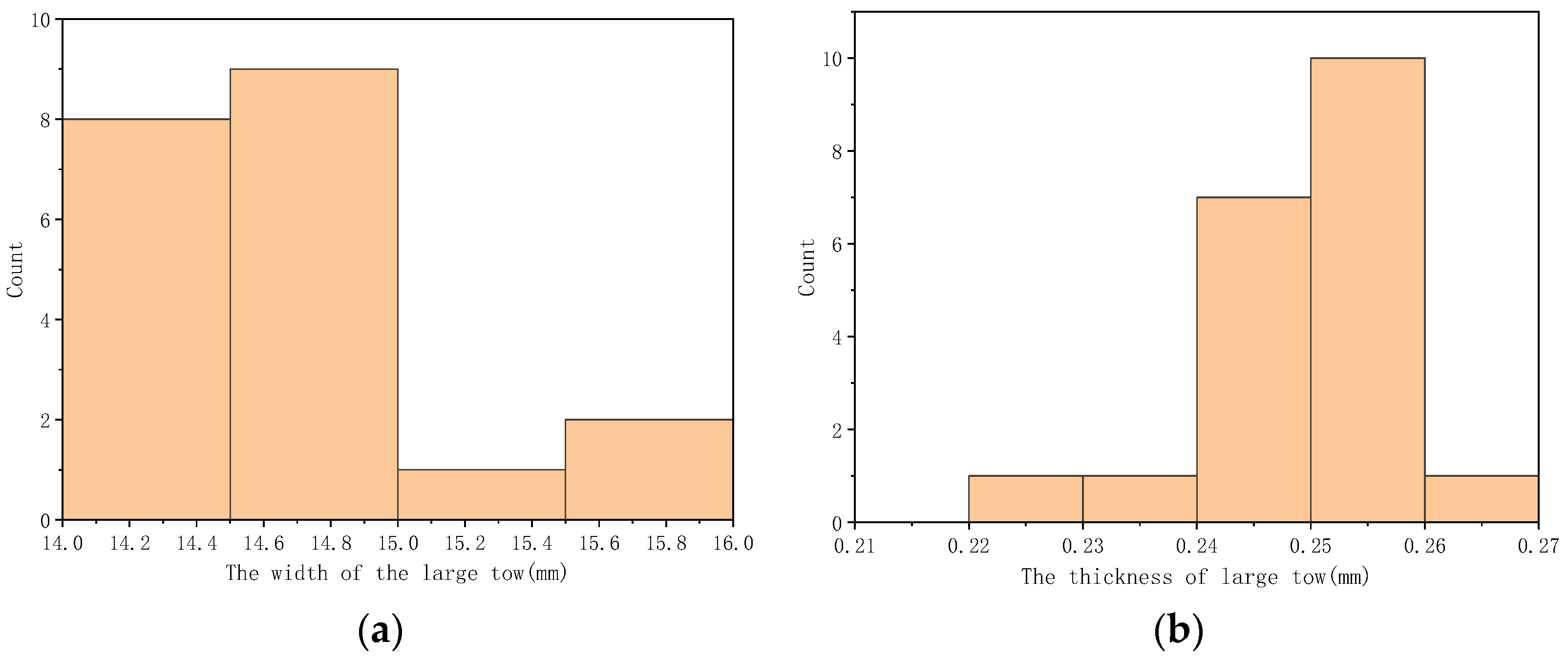
4. Experimental Section
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Dai, D.; Lan, X.; Wang, Z. Hierarchical carbon fiber reinforced SiC/C aerogels with efficient electromagnetic wave absorption properties. Compos. Part B Eng. 2023, 248, 110376. [Google Scholar] [CrossRef]
- Hong, H.; Bae, K.J.; Jung, H.; Oh, Y.; You, N.-H.; Lee, J.-C.; Yu, J. Preparation and characterization of carbon fiber reinforced plastics (CFRPs) incorporating through-plane-stitched carbon fibers. Compos. Struct. 2022, 284, 115198. [Google Scholar] [CrossRef]
- Qu, C.-B.; Wu, T.; Huang, G.-W.; Li, N.; Li, M.; Ma, J.-L.; Liu, Y.; Xiao, H.-M. Improving cryogenic mechanical properties of carbon fiber reinforced composites based on epoxy resin toughened by hydroxyl-terminated polyurethane. Compos. Part B Eng. 2021, 210, 108569. [Google Scholar] [CrossRef]
- Yao, T.-T.; Zhang, X.-F.; Zhang, W.-S.; Liu, Y.-T.; Liu, Q.; Wu, G.-P. Controlled attachment of polycarbonate nanoparticles on carbon fibers for increased resin impregnation and interfacial adhesion in carbon fiber composites. Compos. Part B Eng. 2021, 224, 109218. [Google Scholar] [CrossRef]
- Shirolkar, N.; Maffe, A.; DiLoreto, E.; Gulgunje, P.; Gupta, K.; Park, J.G.; Kirmani, M.H.; Liang, R.; Kumar, S. Continuous small diameter carbon fibers. Carbon 2023, 201, 1193–1199. [Google Scholar] [CrossRef]
- Khan, H.; Kaur, J.; Naebe, M.; Hutchinson, S.; Varley, R.J. Continuous, pilot-scale production of carbon fiber from a textile grade PAN polymer. Mater. Today Commun. 2022, 31, 103231. [Google Scholar] [CrossRef]
- Hiremath, N.; Young, S.; Ghossein, H.; Penumadu, D.; Vaidya, U.; Theodore, M. Low cost textile-grade carbon-fiber epoxy com-posites for automotive and wind energy applications. Compos. Part B Eng. 2020, 198, 108156. [Google Scholar] [CrossRef]
- Song, Y.; Liu, C.; Li, H.; Xu, K.; Geng, H.; Wu, H.; Zu, L.; Jia, X.; Ge, L.; Yang, X. Optimizing dual-scale wettability of epoxy resin on large-tow carbon fiber via tension-driven capillary wicking. Compos. Part B Eng. 2023, 264, 110936. [Google Scholar] [CrossRef]
- Sun, T.; Zhang, X.; Qiu, B.; Luo, Y.; Ling, Y.; Chen, Y.; Xu, Z.; Liang, M.; Zou, H. Controllable construction of gradient modulus intermediate layer on high strength and high modulus carbon fibers to enhance interfacial properties of epoxy composites by efficient electrochemical grafting. Compos. Part B Eng. 2022, 247, 110279. [Google Scholar] [CrossRef]
- Kumar, B.S.; Chandrakant, J.S.; Di, B.Y. Experimental and microscopic investigation on mechanical performance of textile spread-tow thin ply composites. Fibers Polym. 2019, 20, 1036–1045. [Google Scholar]
- Wang, J.; Anthony, D.B.; Fuentes, C.A.; De Luca, H.G.; Zhang, D.; Bismarck, A.; Van Vuure, A.W.; Shaffer, M.S.; Seveno, D. Wettability of carbon nanotube-grafted carbon fibers and their interfacial properties in polypropylene thermoplastic composite. Compos. Part A Appl. Sci. Manuf. 2022, 159, 106993. [Google Scholar] [CrossRef]
- Lim, S.H.; On, S.Y.; Kim, H.; Bang, Y.H.; Kim, S.S. Resin impregnation and interfacial adhesion behaviors in carbon fiber/epoxy composites: Effects of polymer slip and normalized surface free energy with respect to the sizing agents. Compos. Part A Appl. Sci. Manuf. 2021, 146, 106424. [Google Scholar] [CrossRef]
- Endruweit, A.; Long, A.C. Influence of stochastic variations in the fibre spacing on the permeability of bi-directional textile fabrics. Compos. Part A Appl. Sci. Manuf. 2006, 37, 679–694. [Google Scholar] [CrossRef]
- Bechtold, G.; Ye, L. Influence of fibre distribution on the transverse flow permeability in fibre bundles. Compos. Sci. Technol. 2003, 63, 2069–2079. [Google Scholar] [CrossRef]
- Sangani, A.S.; Yao, C. Transport processes in random arrays of cylinders. II. Viscous flow. Phys. Fluids 1988, 31, 2435–2444. [Google Scholar] [CrossRef]
- Cai, Z.; Berdichevsky, A.L. Numerical simulation on the permeability variations of a fiber assembly. Polym. Compos. 1993, 14, 529–539. [Google Scholar] [CrossRef]
- Lundström, T.S.; Gebart, B.R. Effect of perturbation of fiber architecture on permeability inside fiber tows. J. Compos. Mater. 1995, 29, 424–443. [Google Scholar] [CrossRef]
- Chen, X.; Papathanasiou, T. Micro-scale modeling of axial flow through unidirectional disordered fiber arrays. Compos. Sci. Technol. 2007, 67, 1286–1293. [Google Scholar] [CrossRef]
- Chen, X.; Papathanasiou, T.D. The transverse permeability of disordered fiber arrays: A statistical correlation in terms of the mean nearest interfiber spacing. Transp. Porous Media 2008, 71, 233–251. [Google Scholar] [CrossRef]
- Huan, T.; Pillai, K.M. Fast liquid composite molding simulation of unsaturated flow in dual-scale fiber mats using the imbibition characteristics of a fabric-based unit cell. Polym. Compos. 2010, 31, 1790–1807. [Google Scholar]
- Parnas, R.S.; Salem, A.J.; Sadiq, T.A.; Wang, H.-P.; Advani, S.G. The interaction between micro- and macro-scopic flow in RTM preforms. Compos. Struct. 1994, 27, 93–107. [Google Scholar] [CrossRef]
- Binétruy, C.; Hilaire, B.; Pabiot, J. The interactions between flows occurring inside and outside fabric tows during rtm. Compos. Sci. Technol. 1997, 57, 587–596. [Google Scholar] [CrossRef]
- Binetruy, C.; Hilaire, B.; Pabiot, J. Tow impregnation model and void formation mechanisms during RTM. J. Compos. Mater. 1998, 32, 223–245. [Google Scholar] [CrossRef]
- Chan, A.W.; Morgan, R.J. Tow impregnation during resin transfer molding of bi-directional nonwoven fabrics. Polym. Compos. 1993, 14, 335–340. [Google Scholar] [CrossRef]
- Pillai, K.M.; Advani, S.G. Numerical simulation of unsaturated flow in woven fiber preforms during the resin transfer molding process. Polym. Compos. 1998, 19, 71–80. [Google Scholar] [CrossRef]
- Pillai, K.M.; Advani, S.G. A model for unsaturated flow in woven fiber preforms during mold filling in resin transfer molding. J. Compos. Mater. 1998, 32, 1753–1783. [Google Scholar] [CrossRef]
- Simacek, P.; Advani, S.G. A numerical model to predict fiber tow saturation during liquid composite molding. Compos. Sci. Technol. 2003, 63, 1725–1736. [Google Scholar] [CrossRef]
- Wang, Y.; Grove, S. Modelling microscopic flow in woven fabric reinforcements and its application in dual-scale resin infusion modelling. Compos. Part A Appl. Sci. Manuf. 2008, 39, 843–855. [Google Scholar] [CrossRef]
- Endruweit, A.; Gommer, F.; Long, A. Stochastic analysis of fibre volume fraction and permeability in fibre bundles with random filament arrangement. Compos. Part A Appl. Sci. Manuf. 2013, 49, 109–118. [Google Scholar] [CrossRef]
- Godbole, M.G.; Purandare, R.; Harshe, R.; Hood, A.; Gururaja, S.; Joshi, M.; Advani, S. Influence of filament distribution on transverse tow permeability: Model predictions and experimental validation. Compos. Part A Appl. Sci. Manuf. 2019, 118, 150–161. [Google Scholar] [CrossRef]
- Gebart, B. Permeability of Unidirectional Reinforcements for RTM. J. Compos. Mater. 1992, 26, 1100–1133. [Google Scholar] [CrossRef]



| Number of Carbon Fibers | Number of Type I Channels | Number of Type II Channels | Active Channels Count | Horizontal Permeability |
|---|---|---|---|---|
| 48 (15 μm × 250 μm) | 29 | 29 | 58 | 3.3745 × 10−12 |
| 480 (150 μm × 250 μm) | 321 | 325 | 646 | 2.5912 × 10−12 |
| 4800 (1500 μm × 250 μm) | 2097 | 3141 | 6238 | 2.5002 × 10−12 |
| 48,000 (15,000 μm × 250 μm) | 31,611 | 31,624 | 63,235 | 2.0971 × 10−12 |
| Trial No. | Inlet Length, h (mm) | Inlet Time, t (s) | Viscosity, μ (cp) | Porosity, ϕ | Pressure Difference, P (Pa) | Permeability, (m2) |
|---|---|---|---|---|---|---|
| 1 | 10 | 330 | 1040 | 0.508 | 64,000 | |
| 2 | 15 | 520 | 1040 | 0.508 | 64,000 | |
| 3 | 18 | 650 | 1040 | 0.508 | 64,000 | |
| 4 | 20 | 700 | 1040 | 0.508 | 64,000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Y.; Zhang, Q.; Rao, J.; Liu, D. Model Prediction and Experimental Validation of Transverse Permeability of Large-Tow Carbon Fiber Composites. Polymers 2024, 16, 1266. https://doi.org/10.3390/polym16091266
Feng Y, Zhang Q, Rao J, Liu D. Model Prediction and Experimental Validation of Transverse Permeability of Large-Tow Carbon Fiber Composites. Polymers. 2024; 16(9):1266. https://doi.org/10.3390/polym16091266
Chicago/Turabian StyleFeng, Yu, Qiaoxin Zhang, Jun Rao, and Dong Liu. 2024. "Model Prediction and Experimental Validation of Transverse Permeability of Large-Tow Carbon Fiber Composites" Polymers 16, no. 9: 1266. https://doi.org/10.3390/polym16091266
APA StyleFeng, Y., Zhang, Q., Rao, J., & Liu, D. (2024). Model Prediction and Experimental Validation of Transverse Permeability of Large-Tow Carbon Fiber Composites. Polymers, 16(9), 1266. https://doi.org/10.3390/polym16091266





