Influence of Failure-Load Prediction in Composite Single-Lap Joints with Brittle and Ductile Adhesives Using Different Progressive-Damage Techniques
Abstract
1. Introduction
2. Methodology
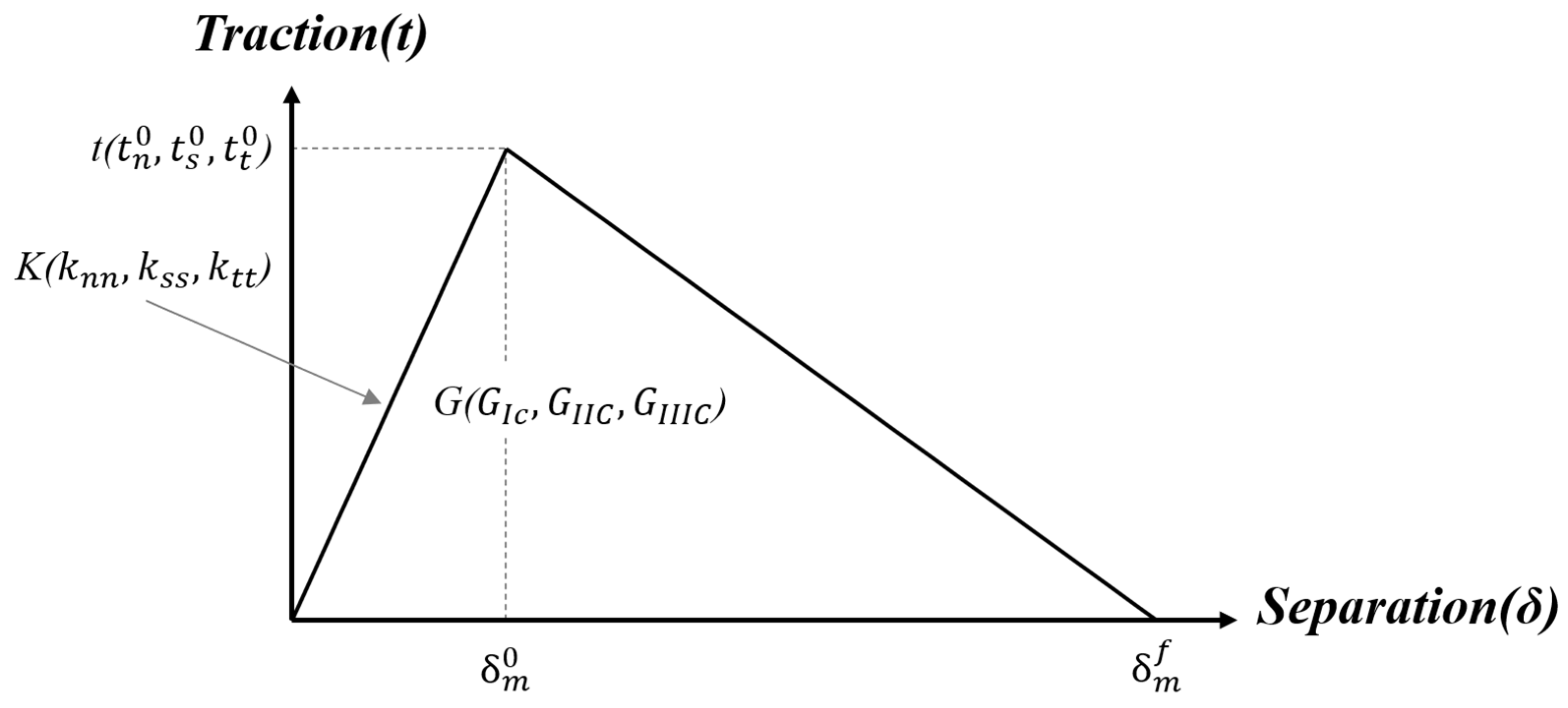
2.1. Cohesive Zone Model (CZM)
2.2. Virtual Crack Closure Technique (VCCT)
3. Numerical Model
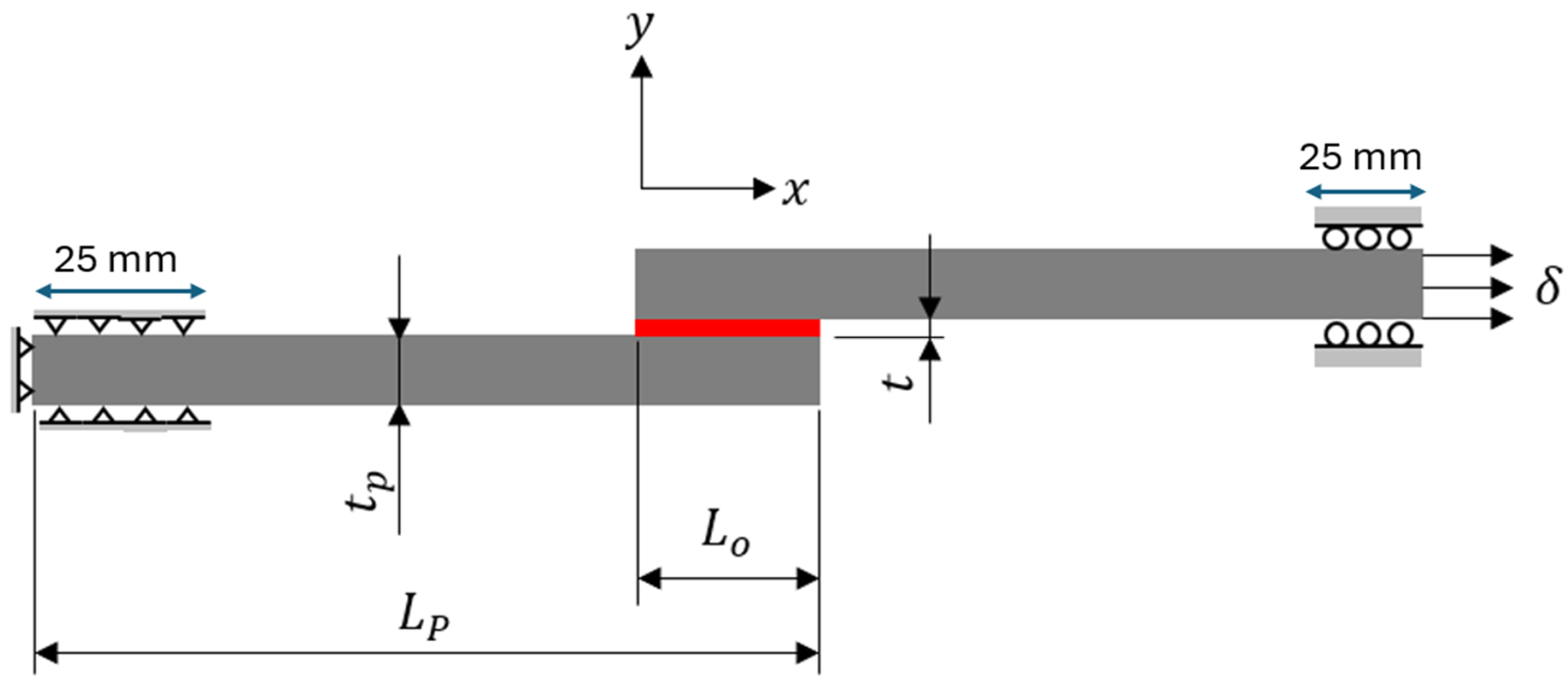
3.1. FE Model of Composite Single Lap Joint
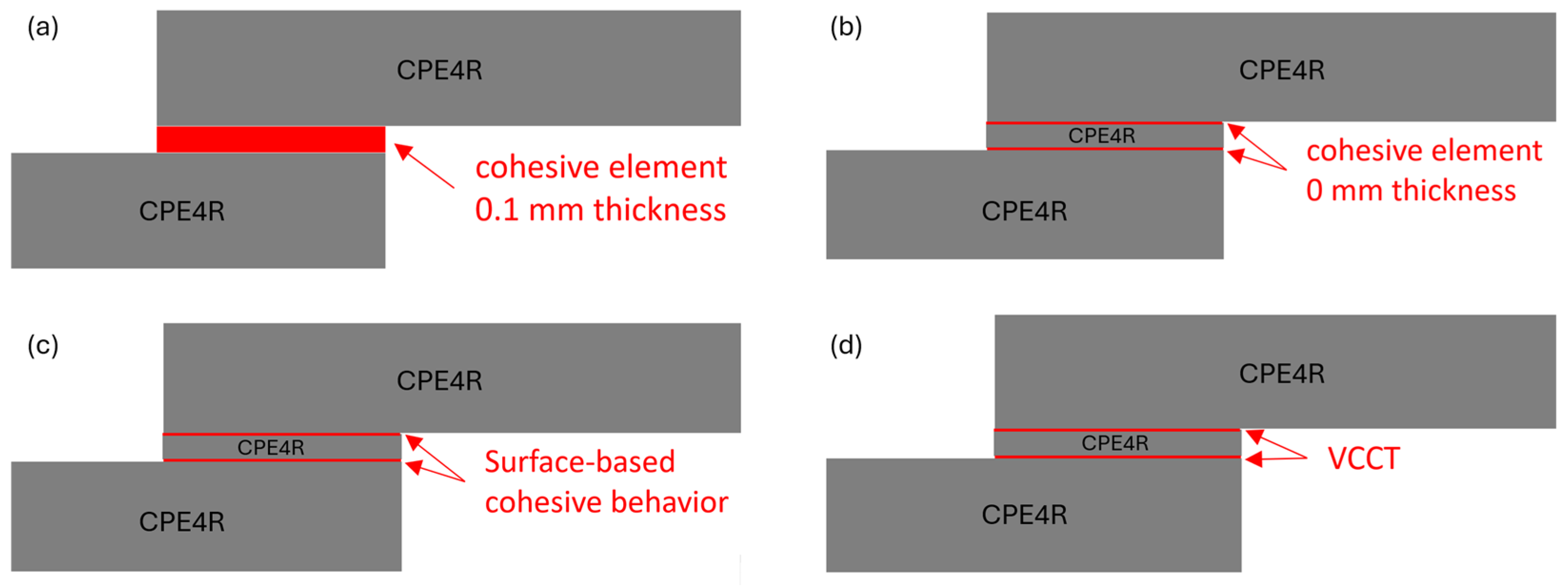
3.2. Cohesive Element in Abaqus
3.3. Surface-Based Cohesive Behavior in Abaqus
3.4. VCCT in Abaqus
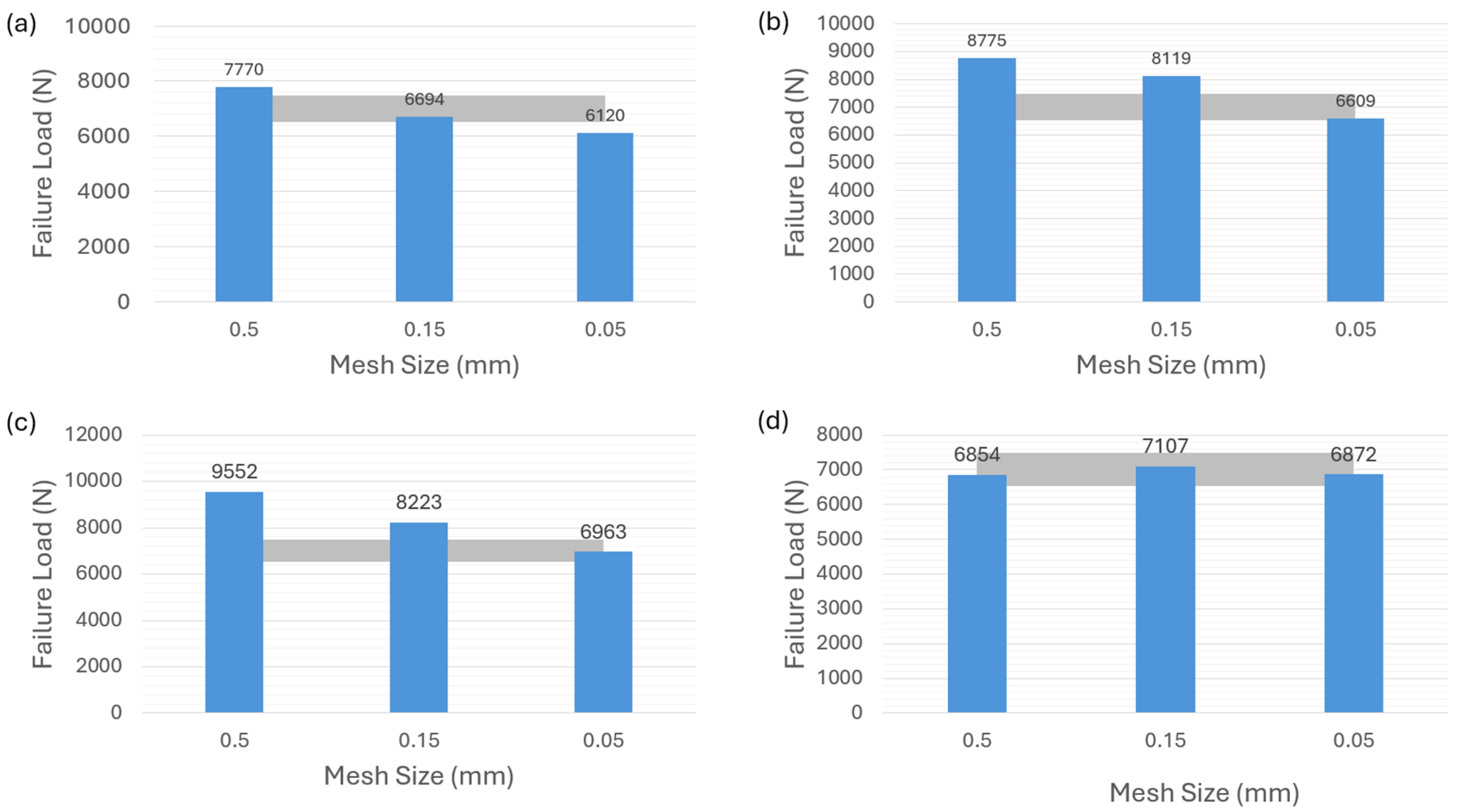
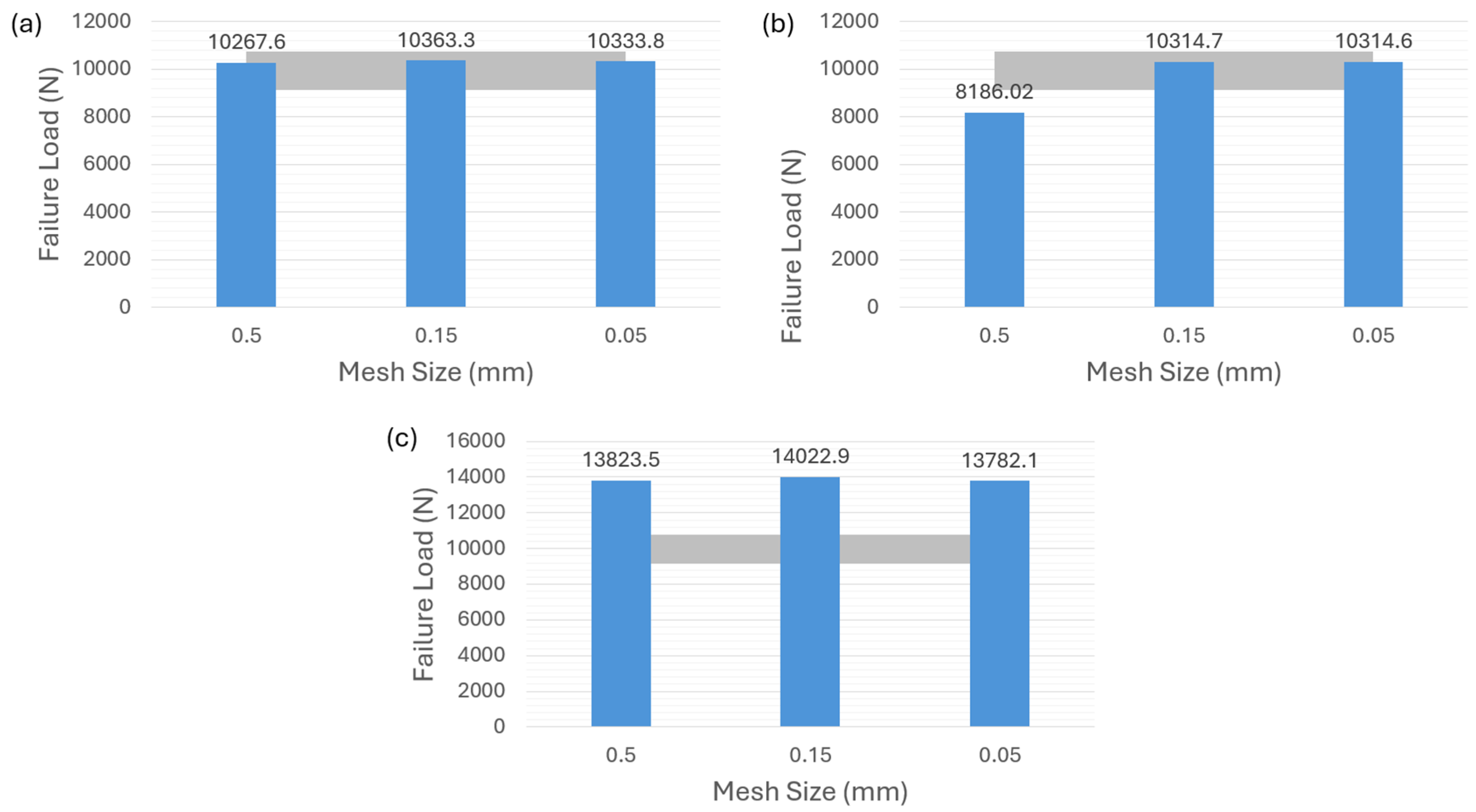
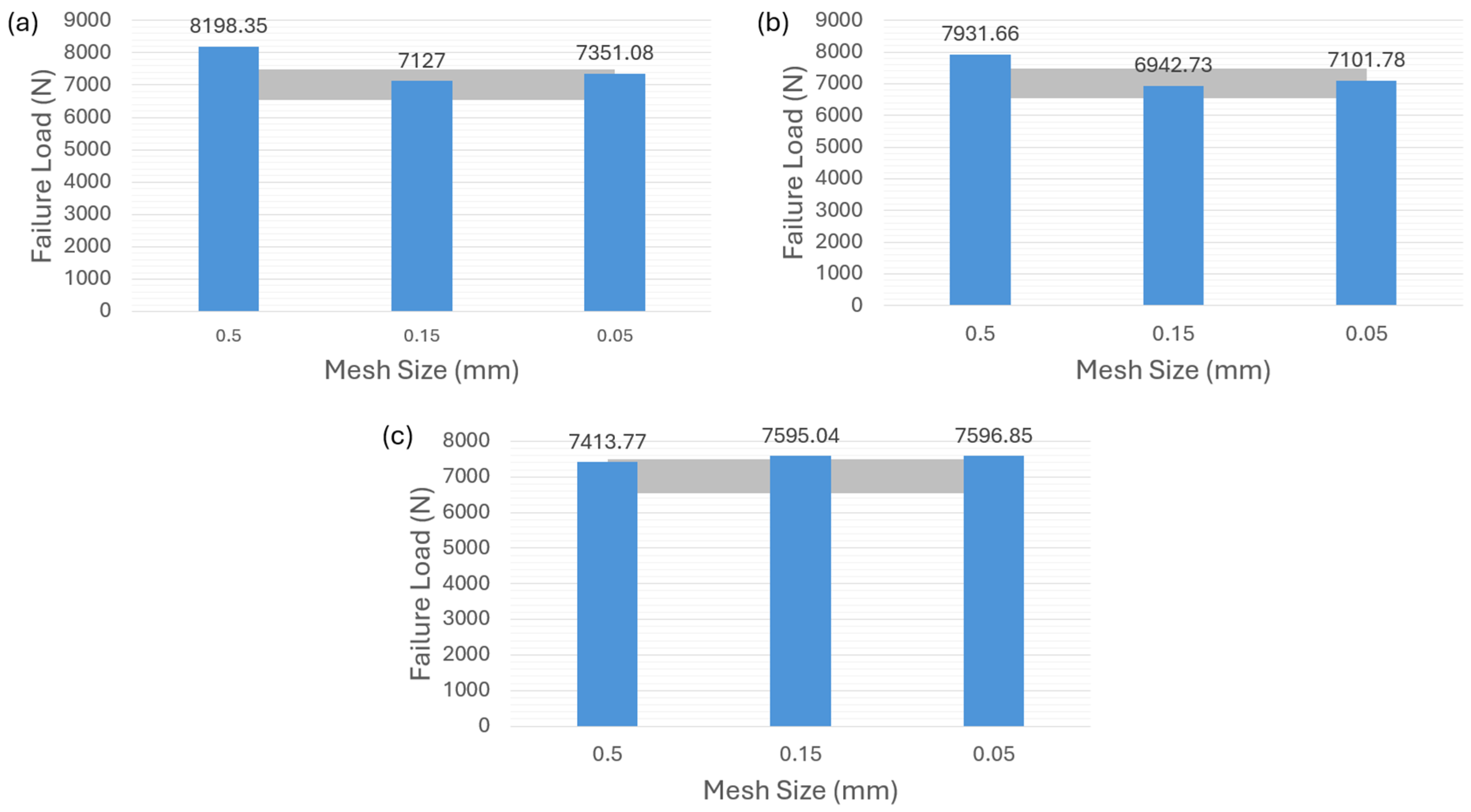
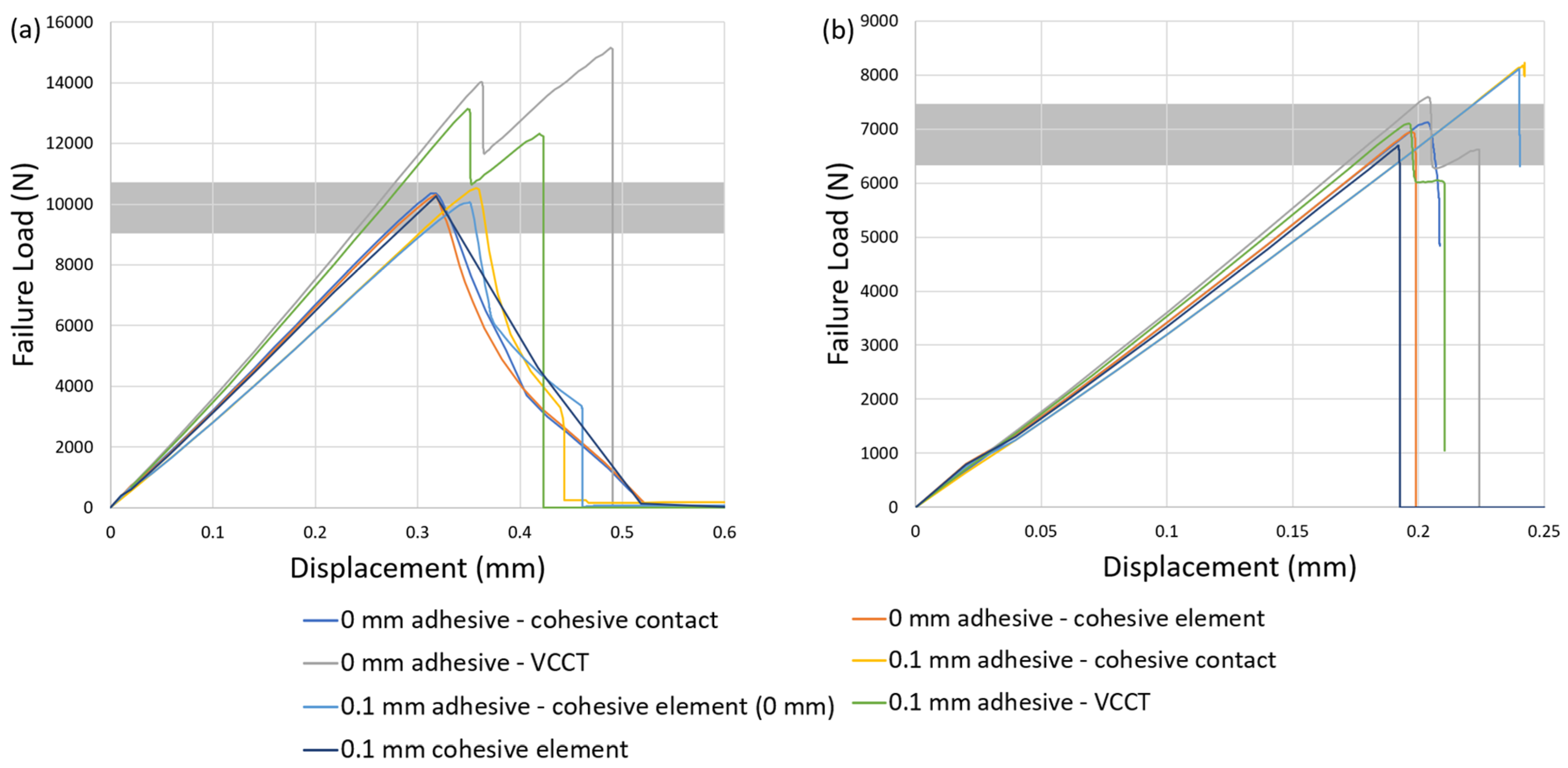
4. Result and Discussion
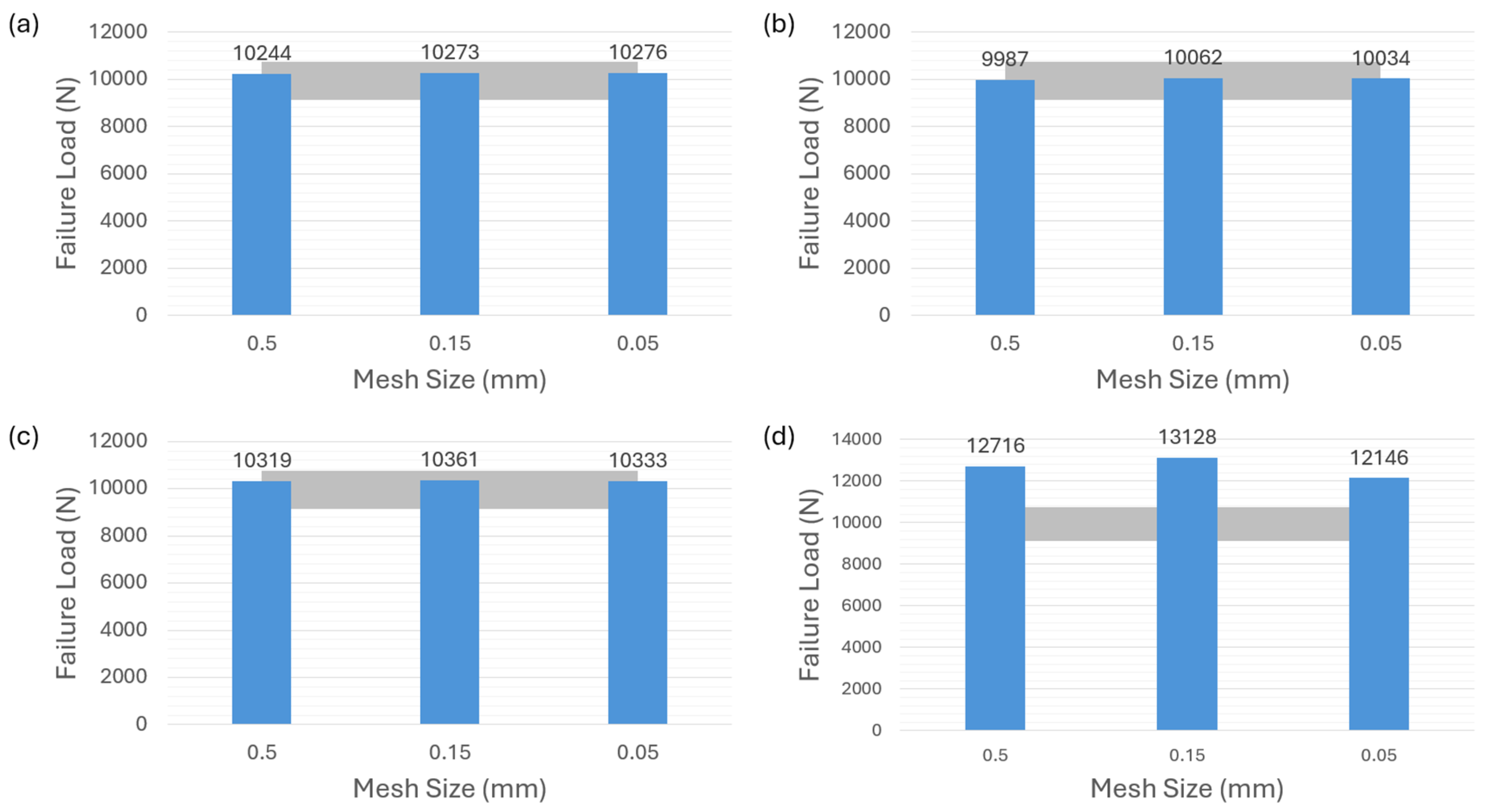
4.1. Modeling Strategies for Nonzero Thickness of Adhesive
4.2. Modeling Strategies for Zero Thickness of Adhesive
4.3. Comparisons
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Banea, M.D.; da Silva, L.F.M. Adhesively bonded joints in composite materials: An overview. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2009, 223, 1–18. [Google Scholar] [CrossRef]
- Budhe, S.; Banea, M.D.; de Barros, S.; da Silva, L.F.M. An updated review of adhesively bonded joints in composite materials. Int. J. Adhes. Adhes. 2017, 72, 30–42. [Google Scholar] [CrossRef]
- da Silva, L.F.M.; Neves, P.J.C.D.; Adams, R.D.; Spelt, J.K. Analytical models of adhesively bonded joints—Part I: Literature survey. Int. J. Adhes. Adhes. 2009, 29, 319–330. [Google Scholar] [CrossRef]
- da Silva, L.F.M.; Neves, P.J.C.D.; Adams, R.D.; Wang, A.; Spelt, J.K. Analytical models of adhesively bonded joints—Part II: Comparative study. Int. J. Adhes. Adhes. 2009, 29, 331–341. [Google Scholar] [CrossRef]
- Yousefsani, S.A.; Tahani, M. Accurate determination of stress distributions in adhesively bonded homogeneous and heterogeneous double-lap joints. Eur. J. Mech. A/Solids 2013, 39, 197–208. [Google Scholar] [CrossRef]
- Icardi, U.; Sola, F. Analysis of bonded joints with laminated adherends by a variable kinematics layerwise model. Int. J. Adhes. Adhes. 2014, 50, 244–254. [Google Scholar] [CrossRef]
- Wu, X.-F.; Zhao, Y. Stress-function variational method for interfacial stress analysis of adhesively bonded joints. Int. J. Solids Struct. 2013, 50, 4305–4319. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, C. Three-parameter, elastic foundation model for analysis of adhesively bonded joints. Int. J. Adhes. Adhes. 2009, 29, 495–502. [Google Scholar] [CrossRef]
- Yousefsani, S.A.; Tahani, M. Analytical solutions for adhesively bonded composite single-lap joints under mechanical loadings using full layerwise theory. Int. J. Adhes. Adhes. 2013, 43, 32–41. [Google Scholar] [CrossRef]
- Anyfantis, K.N.; Tsouvalis, N.G. A 3D ductile constitutive mixed-mode model of cohesive elements for the finite element analysis of adhesive joints. J. Adhes. Sci. Technol. 2013, 27, 1146–1178. [Google Scholar] [CrossRef]
- Gunnion, A.J.; Herszberg, I. Parametric study of scarf joints in composite structures. Compos. Struct. 2006, 75, 364–376. [Google Scholar] [CrossRef]
- Harris, J.A.; Adams, R.A. Strength prediction of bonded single lap joints by non-linear finite element methods. Int. J. Adhes. Adhes. 1984, 4, 65–78. [Google Scholar] [CrossRef]
- Liu, P.; Cheng, X.; Wang, S.; Liu, S.; Cheng, Y. Numerical analysis of bearing failure in countersunk composite joints using 3D explicit simulation method. Compos. Struct. 2016, 138, 30–39. [Google Scholar] [CrossRef]
- Luo, H.; Yan, Y.; Zhang, T.; Liang, Z. Progressive failure and experimental study of adhesively bonded composite single-lap joints subjected to axial tensile loads. J. Adhes. Sci. Technol. 2016, 30, 894–914. [Google Scholar] [CrossRef]
- Pickett, A.K.; Hollaway, L. The analysis of elastic-plastic adhesive stress in bonded lap joints in FRP structures. Compos. Struct. 1985, 4, 135–160. [Google Scholar] [CrossRef]
- Sadeghi, M.Z.; Gabener, A.; Zimmermann, J.; Saravana, K.; Weiland, J.; Reisgen, U.; Schroeder, K.U. Failure load prediction of adhesively bonded single lap joints by using various FEM techniques. Int. J. Adhes. Adhes. 2020, 97, 102493. [Google Scholar] [CrossRef]
- Ramalho, L.D.C.; Campilho, R.D.S.G.; Belinha, J.; da Silva, L.F.M. Static strength prediction of adhesive joints: A review. Int. J. Adhes. Adhes. 2020, 96, 102451. [Google Scholar] [CrossRef]
- He, X. A review of finite element analysis of adhesively bonded joints. Int. J. Adhes. Adhes. 2011, 31, 248–264. [Google Scholar] [CrossRef]
- Adluru, H.K.; Hoos, K.H.; Iarve, E.V.; Ratcliffe, J.G. Delamination initiation and migration modeling in clamped tapered laminated beam specimens under static loading. Compos. Part A Appl. Sci. Manuf. 2019, 118, 202–212. [Google Scholar] [CrossRef]
- Tay, T.E.; Liu, G.; Tan, V.B.C.; Sun, X.S.; Pham, D.C. Progressive failure analysis of composites. J. Compos. Mater. 2008, 42, 1921–1966. [Google Scholar] [CrossRef]
- Maimí, P.; Camanho, P.P.; Mayugo, J.A.; Dávila, C.G. A continuum damage model for composite laminates: Part I—Constitutive model. Mech. Mater. 2007, 39, 897–908. [Google Scholar] [CrossRef]
- Maimí, P.; Camanho, P.P.; Mayugo, J.A.; Dávila, C.G. A continuum damage model for composite laminates: Part II—Computational implementation and validation. Mech. Mater. 2007, 39, 909–919. [Google Scholar] [CrossRef]
- Iarve, E.V.; Gurvich, M.R.; Mollenhauer, D.H.; Rose, C.A.; Dávila, C.G. Mesh-independent matrix cracking and delamination modeling in laminated composites. Int. J. Numer. Methods Eng. 2011, 88, 749–773. [Google Scholar] [CrossRef]
- Liang, Y.-J.; McQuien, J.S.; Iarve, E.V. Implementation of the regularized extended finite element method in Abaqus framework for fracture modeling in laminated composites. Eng. Fract. Mech. 2020, 230, 106989. [Google Scholar] [CrossRef]
- Liang, Y.-J.; Iarve, E.V. Fracture Analysis of Clamped Tapered Beam Specimen under Static Loading by Regularized Extended Finite Element Method in Abaqus Implementation. Appl. Compos. Mater. 2021, 28, 2047–2060. [Google Scholar] [CrossRef]
- Liang, Y.-J.; Dávila, C.G.; Iarve, E.V. A reduced-input cohesive zone model with regularized extended finite element method for fatigue analysis of laminated composites in Abaqus. Compos. Struct. 2021, 275, 114494. [Google Scholar] [CrossRef]
- Barenblatt, G.I. The Mathematical Theory of Equilibrium Cracks in Brittle Fracture. Adv. Appl. Mech. 1962, 7, 55–129. [Google Scholar] [CrossRef]
- Dugdale, D.S. Yielding of steel sheets containing slits. J. Mech. Phys. Solids 1960, 8, 100–104. [Google Scholar] [CrossRef]
- Camacho, G.T.; Ortiz, M. Computational modelling of impact damage in brittle materials. Int. J. Solids Struct. 1996, 33, 2899–2938. [Google Scholar] [CrossRef]
- Needleman, A. An analysis of decohesion along an imperfect interface. Int. J. Fract. 1990, 42, 21–40. [Google Scholar] [CrossRef]
- Needleman, A. A Continuum Model for Void Nucleation by Inclusion Debonding. J. Appl. Mech. 1987, 54, 525–531. [Google Scholar] [CrossRef]
- Tvergaard, V.; Hutchinson, J.W. The relation between crack growth resistance and fracture process parameters in elastic-plastic solids. J. Mech. Phys. Solids 1992, 40, 1377–1397. [Google Scholar] [CrossRef]
- Campilho, R.D.S.G.; Banea, M.D.; Pinto, A.M.G.; da Silva, L.F.M.; de Jesus, A.M.P. Strength prediction of single- and double-lap joints by standard and extended finite element modelling. Int. J. Adhes. Adhes. 2011, 31, 363–372. [Google Scholar] [CrossRef]
- Kaiser, I.; Tan, C.; Tan, K.T. Bio-inspired patterned adhesive single-lap joints for CFRP and titanium. Compos. B Eng. 2021, 224, 109182. [Google Scholar] [CrossRef]
- Li, S.; Liu, W.; Sun, W.; Hou, S. Effects of adherend notching on the bonding performance of composite single-lap joints. Eng. Fract. Mech. 2023, 281, 109141. [Google Scholar] [CrossRef]
- Krueger, R. Virtual crack closure technique: History, approach, and applications. Appl. Mech. Rev. 2004, 57, 109–143. [Google Scholar] [CrossRef]
- Krueger, R. The virtual crack closure technique for modeling interlaminar failure and delamination in advanced composite materials. In Numerical Modelling of Failure in Advanced Composite Materials; Elsevier: Amsterdam, The Netherlands, 2015; pp. 3–53. [Google Scholar] [CrossRef]
- Jokinen, J.; Wallin, M.; Saarela, O. Applicability of VCCT in mode I loading of yielding adhesively bonded joints—A case study. Int. J. Adhes. Adhes. 2015, 62, 85–91. [Google Scholar] [CrossRef]
- Eder, M.A.; Bitsche, R.D. Fracture analysis of adhesive joints in wind turbine blades. Wind Energy 2015, 18, 1007–1022. [Google Scholar] [CrossRef]
- Shokrieh, M.M.; Rajabpour-Shirazi, H.; Heidari-Rarani, M.; Haghpanahi, M. Simulation of mode I delamination propagation in multidirectional composites with R-curve effects using VCCT method. Comput. Mater. Sci. 2012, 65, 66–73. [Google Scholar] [CrossRef]
- Simulia, D. Abaqus 6.17 Documentation; DS SIMULIA Corp.: Johnston, RI, USA, 2017. [Google Scholar]
- Irwin, G.R. Analysis of Stresses and Strains Near the End of a Crack Traversing a Plate. J. Appl. Mech. 1957, 24, 361–364. [Google Scholar] [CrossRef]


| E11 (MPa) | E22, E33 (MPa) | G12, G13 (MPa) | G23 (MPa) | v12, v13 | v23 |
|---|---|---|---|---|---|
| 109,000 | 8819 | 4315 | 3200 | 0.342 | 0.38 |
| Properties | Araldite® AV138 | Araldite® 2015 |
|---|---|---|
| 4.89 | 1.85 | |
| 1.56 | 0.56 | |
| 39.45 | 21.63 | |
| 30.2 | 17.9 | |
| 0.2 | 0.43 | |
| 0.38 | 4.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chuang, Y.-C.; Su, C.-S.; Liang, Y.-J. Influence of Failure-Load Prediction in Composite Single-Lap Joints with Brittle and Ductile Adhesives Using Different Progressive-Damage Techniques. Polymers 2024, 16, 964. https://doi.org/10.3390/polym16070964
Chuang Y-C, Su C-S, Liang Y-J. Influence of Failure-Load Prediction in Composite Single-Lap Joints with Brittle and Ductile Adhesives Using Different Progressive-Damage Techniques. Polymers. 2024; 16(7):964. https://doi.org/10.3390/polym16070964
Chicago/Turabian StyleChuang, Yung-Cheng, Cong-Sheng Su, and Yu-Jui Liang. 2024. "Influence of Failure-Load Prediction in Composite Single-Lap Joints with Brittle and Ductile Adhesives Using Different Progressive-Damage Techniques" Polymers 16, no. 7: 964. https://doi.org/10.3390/polym16070964
APA StyleChuang, Y.-C., Su, C.-S., & Liang, Y.-J. (2024). Influence of Failure-Load Prediction in Composite Single-Lap Joints with Brittle and Ductile Adhesives Using Different Progressive-Damage Techniques. Polymers, 16(7), 964. https://doi.org/10.3390/polym16070964







