Ferroelectric Properties of Polymer–Semiconductor Hybrid Material or Composite under Optical Excitation
Abstract
1. Introduction
2. Materials and Methods
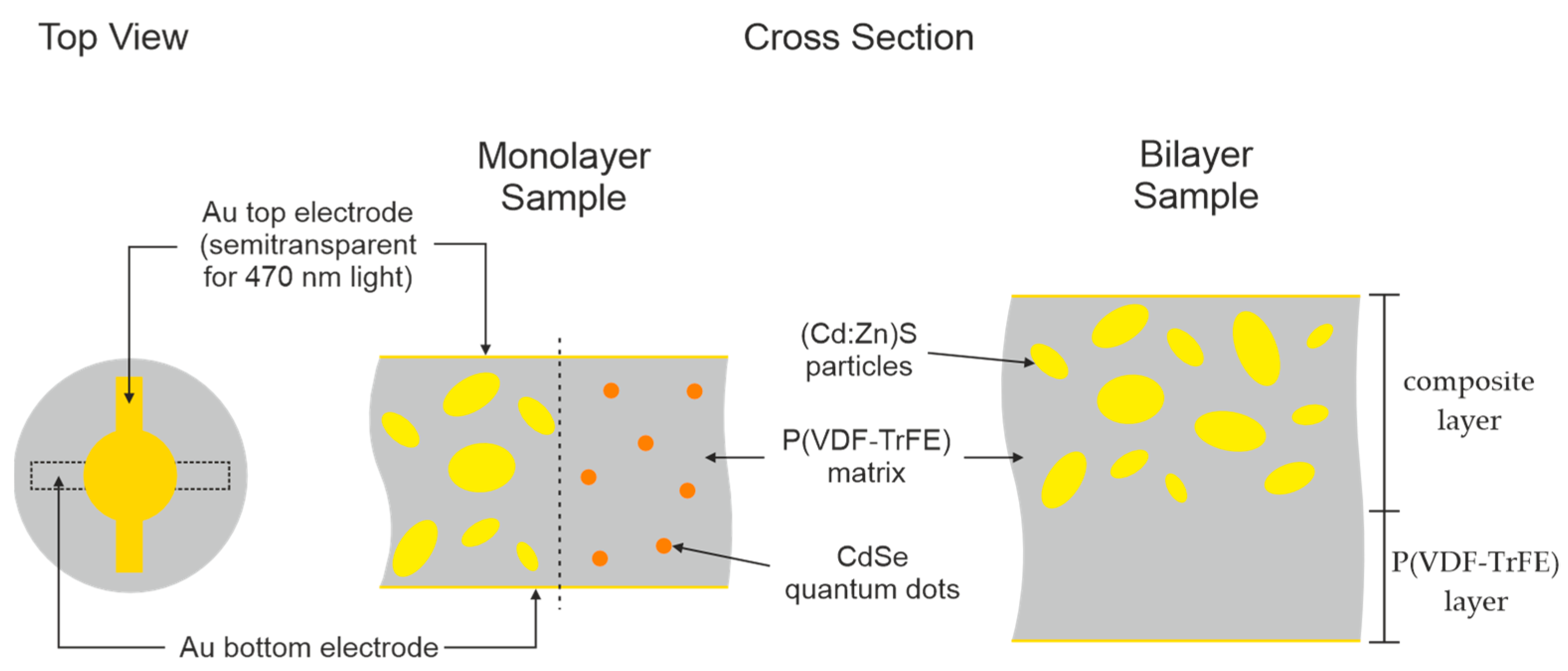
2.1. Sample Preparation
2.2. Microstructure and Optical Characterization
2.3. Electrical and Ferroelectric Properties
3. Results
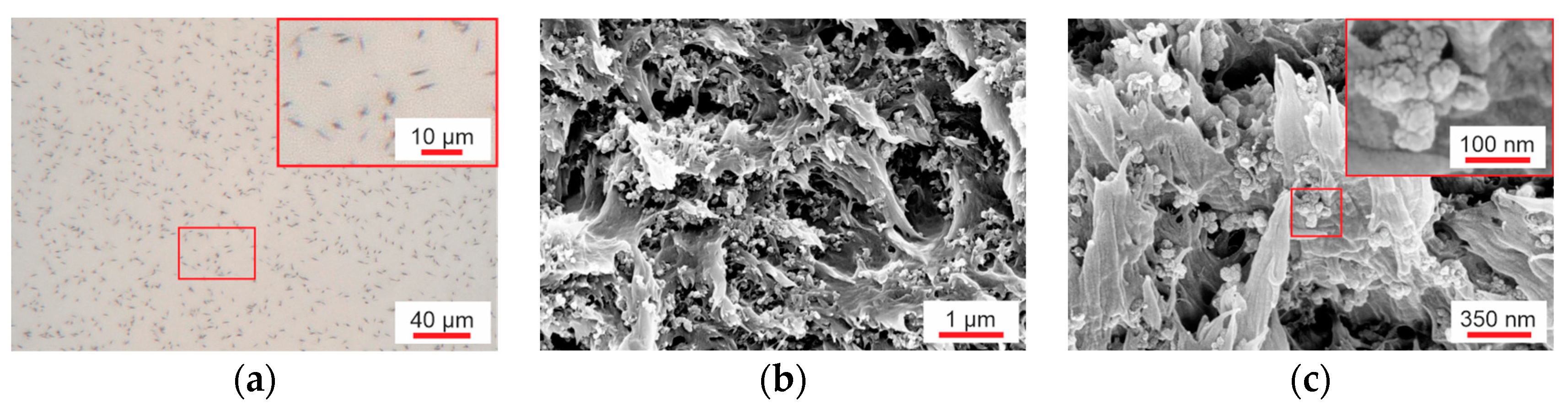
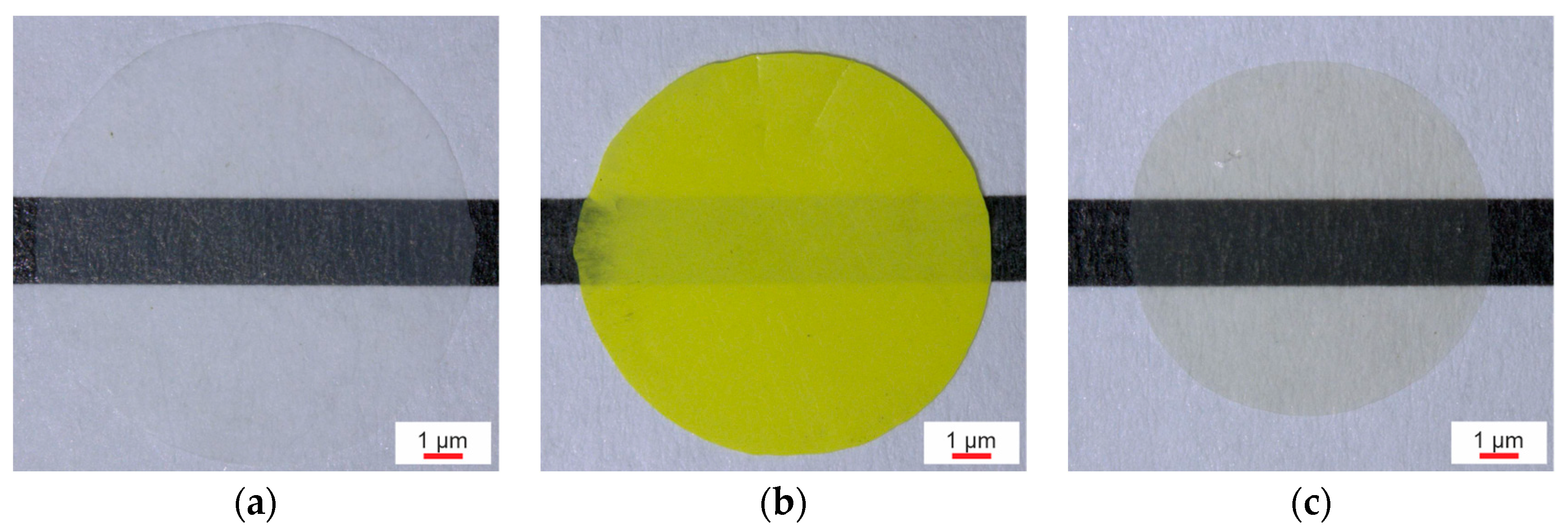
3.1. Composite Morphology
3.2. Electrical Properties
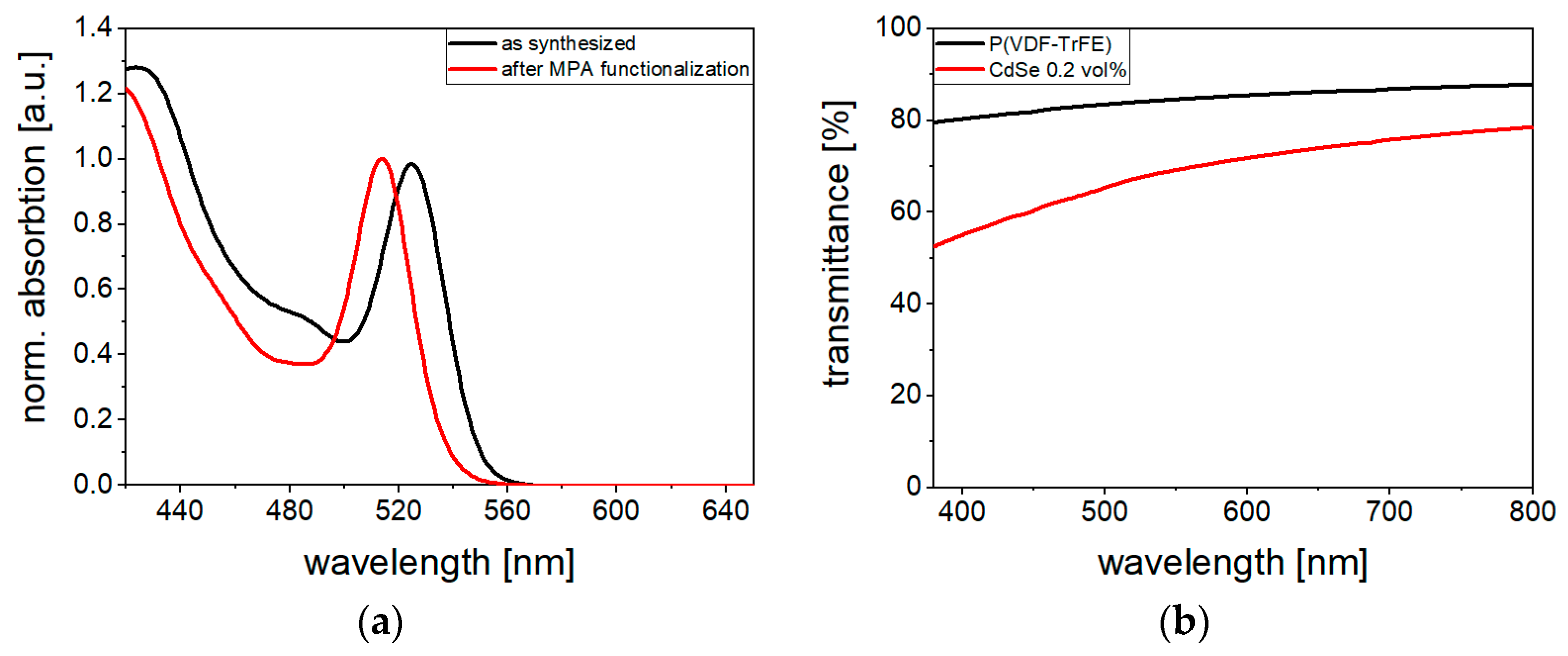
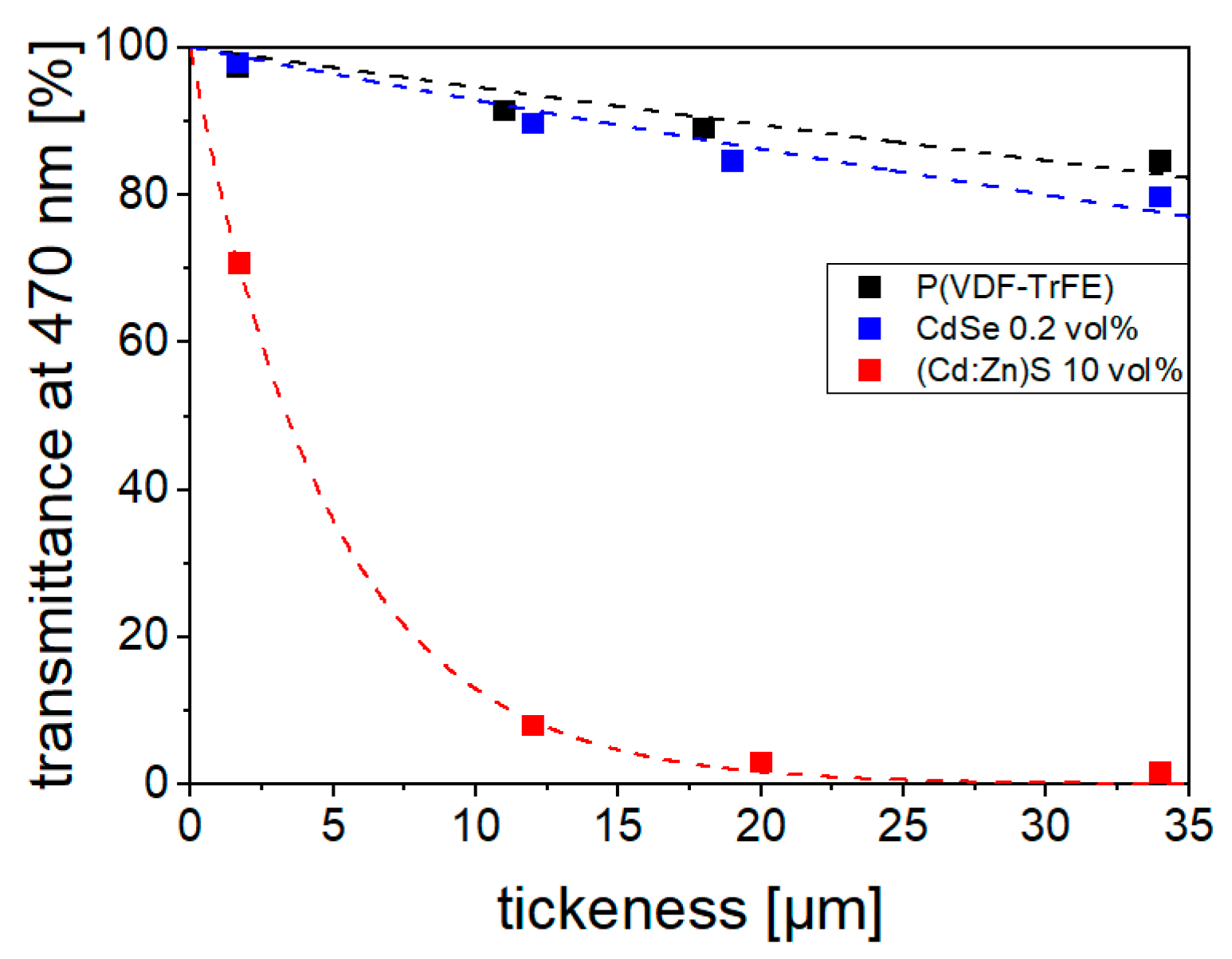
3.3. Optical Properties
3.4. Ferroelectric Properties
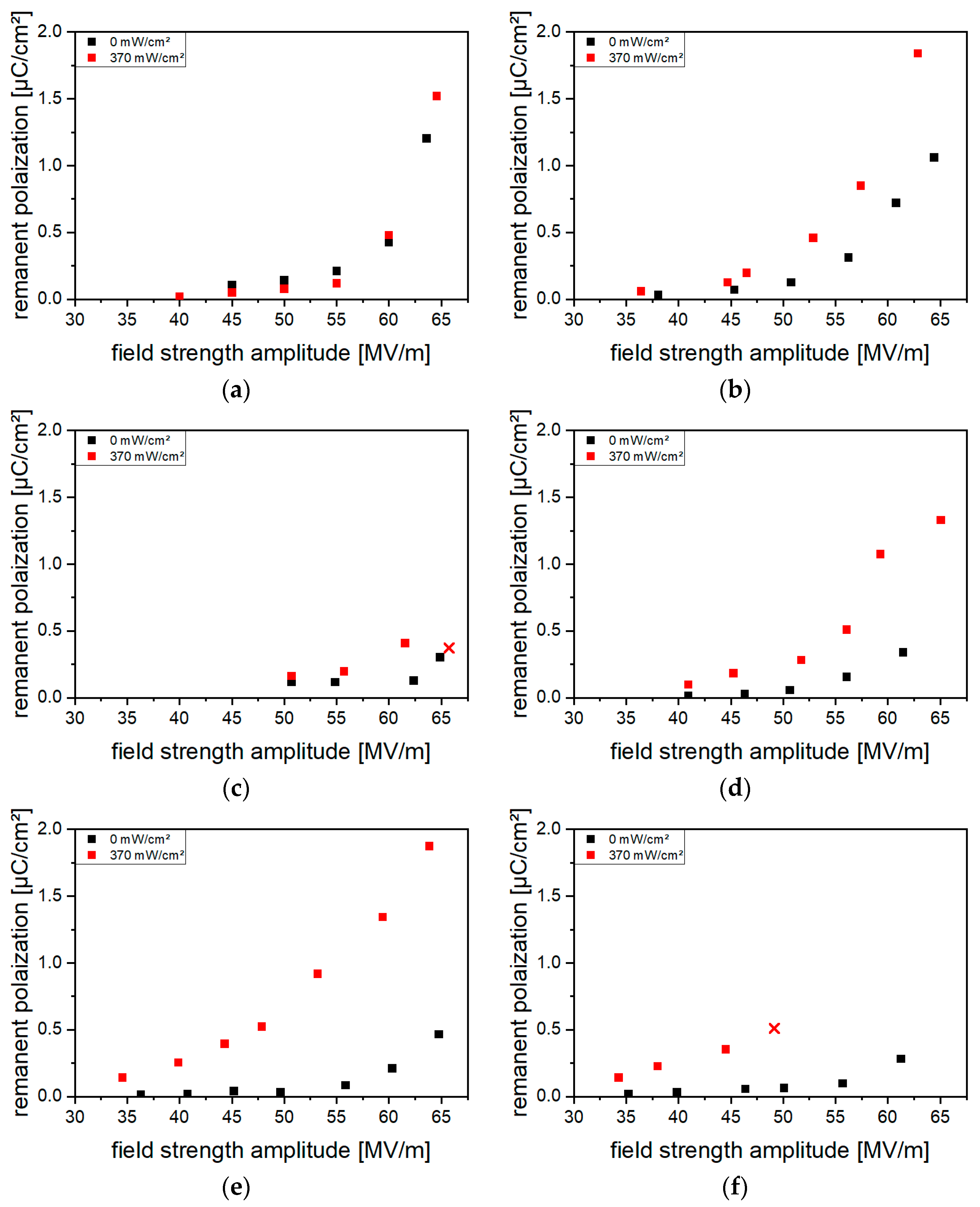
3.4.1. Influence of Particle Concentration
3.4.2. Influence of Poling Frequency
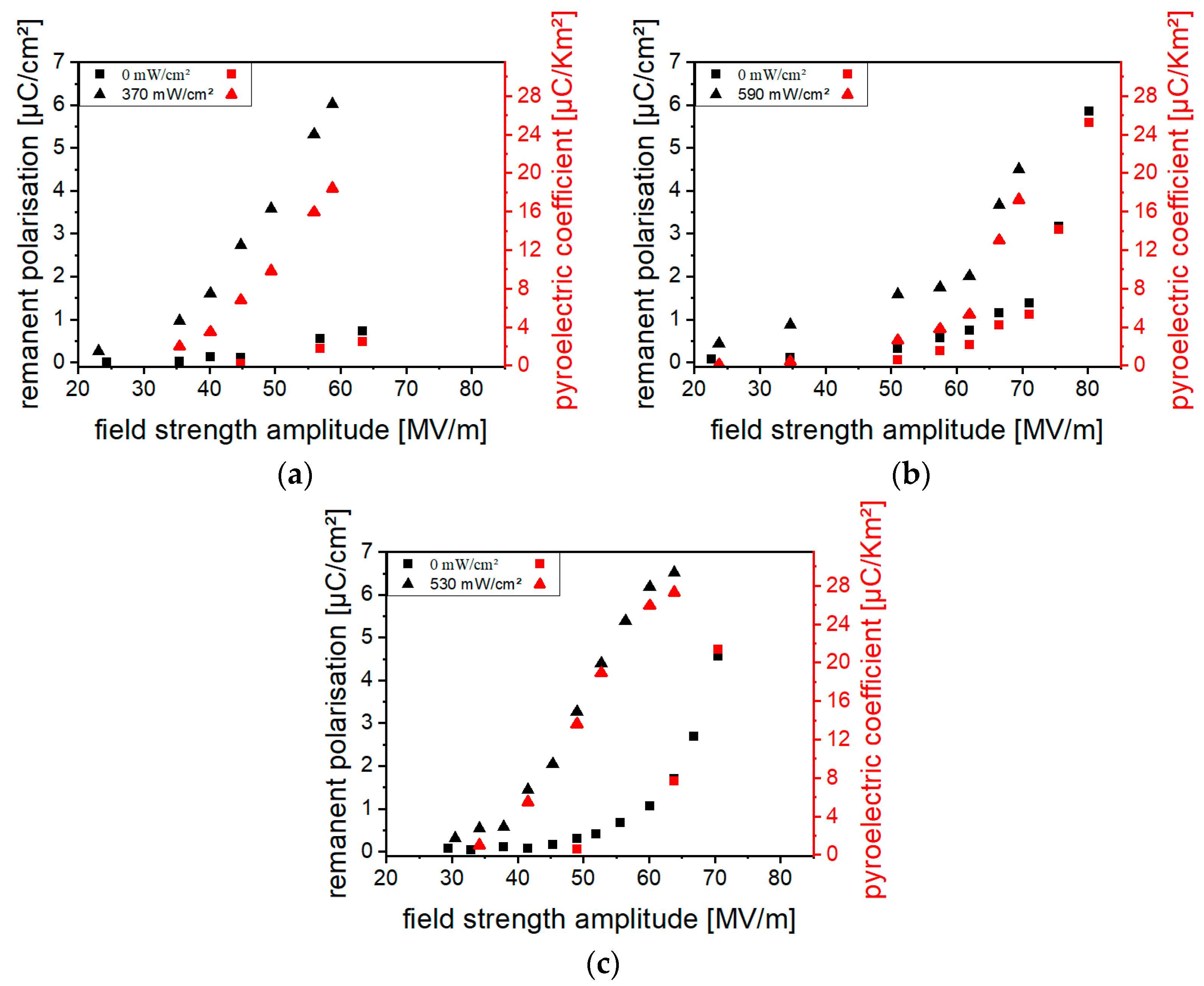
3.4.3. Influence of Poling Field Strength Amplitude
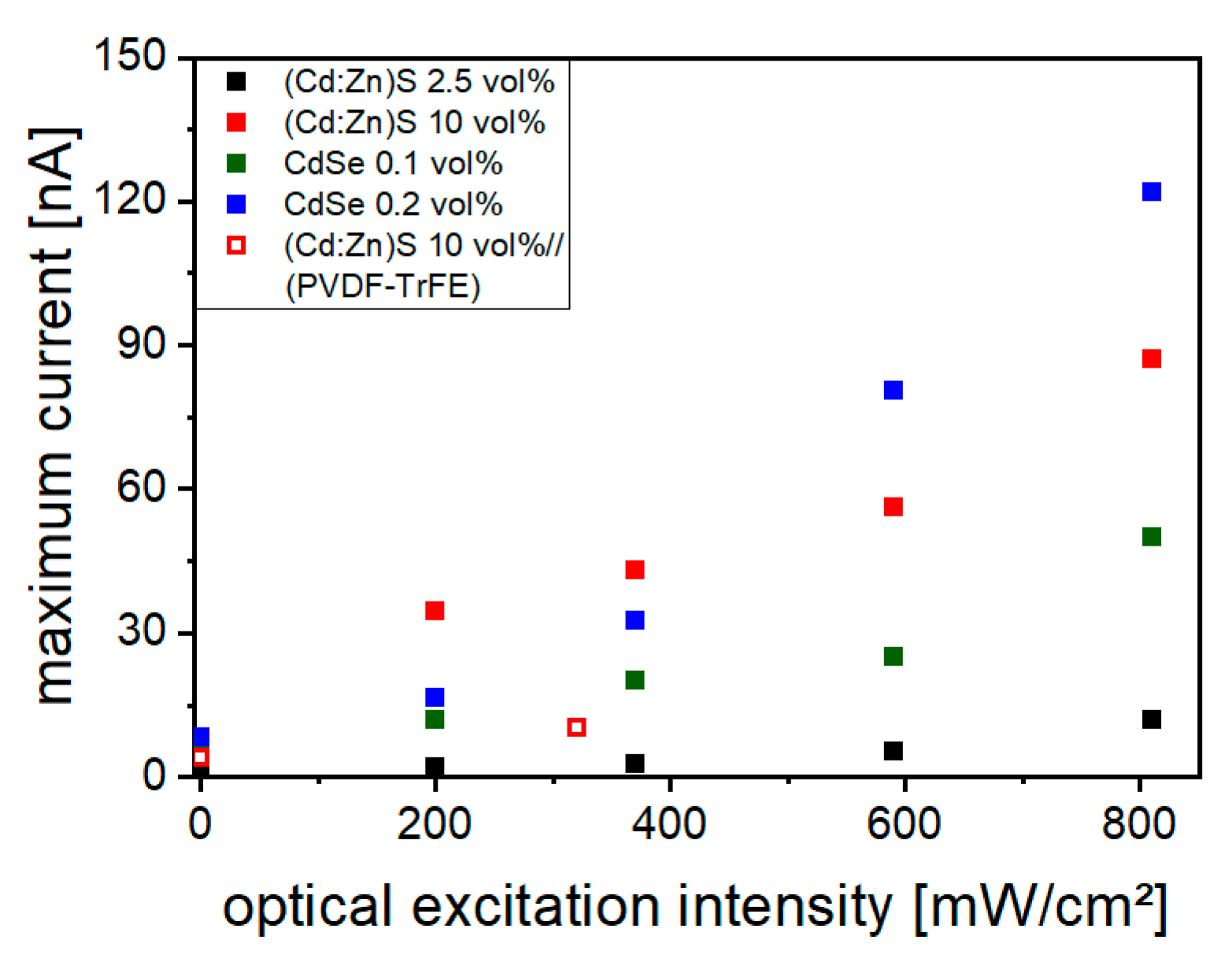
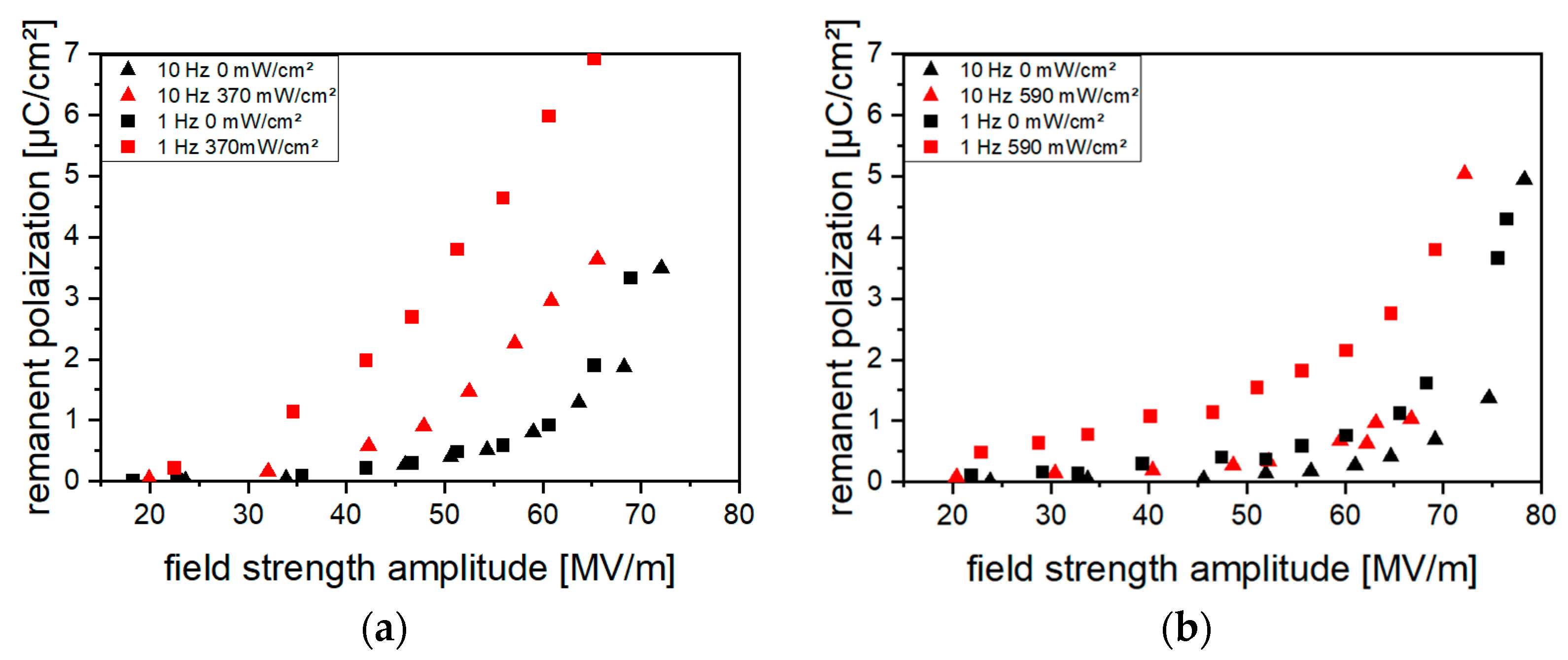
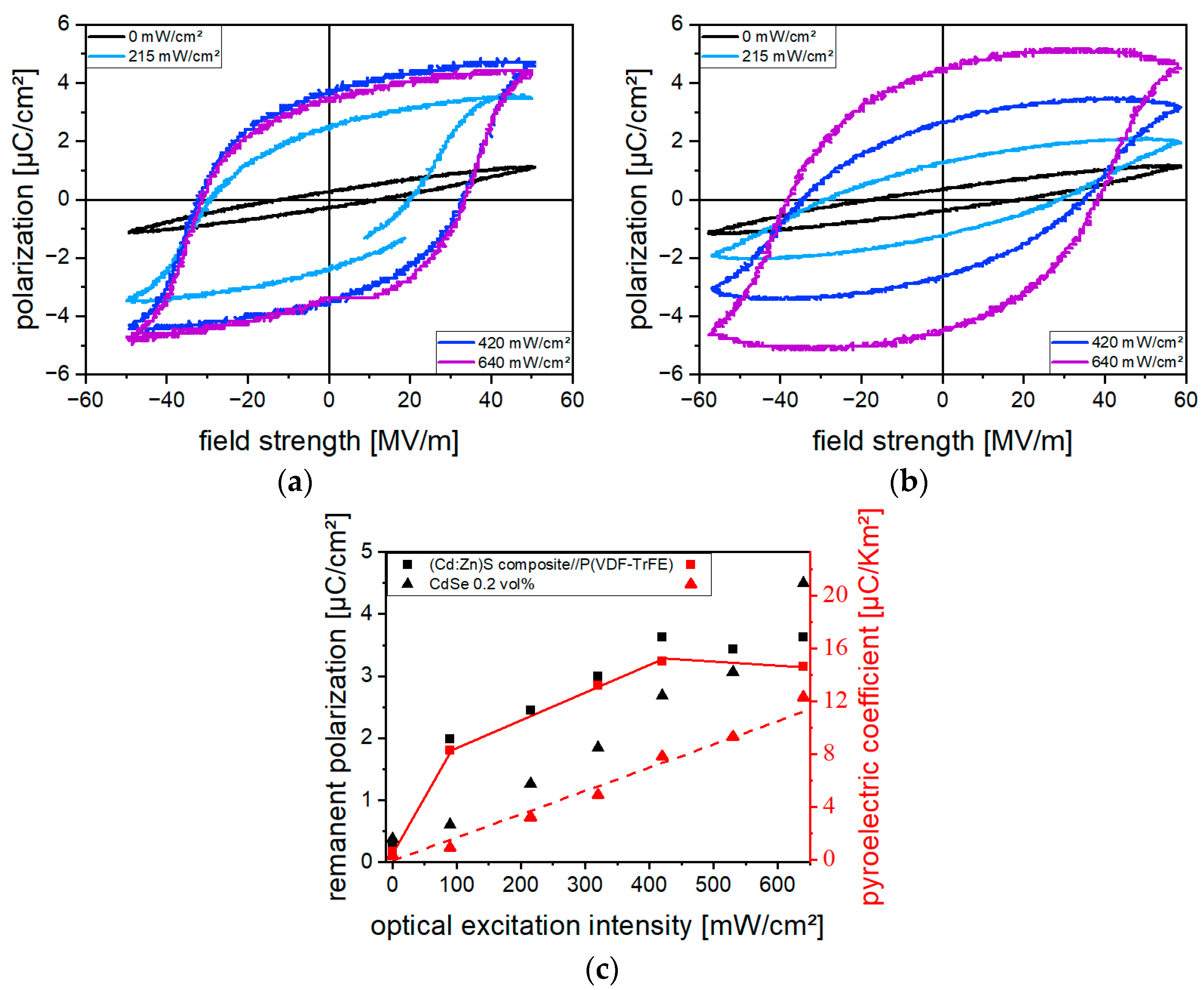
3.4.4. Influence of the Optical Excitation Intensity
4. Discussion
4.1. Electrical Properties
4.2. Ferroelectric Properties
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Saxena, P.; Shukla, P. A comprehensive review on fundamental properties and applications of poly (vinylidene fluoride) (PVDF). Adv. Compos. Hybrid Mater. 2021, 4, 8–26. [Google Scholar] [CrossRef]
- Tsikriteas, Z.M.; Roscow, J.I.; Bowen, C.R.; Khanbareh, H. Flexible ferroelectric wearable devices for medical applications. iScience 2021, 24, 101987. [Google Scholar] [CrossRef] [PubMed]
- Surmenev, R.A.; Chernozem, R.V.; Pariy, I.O.; Surmeneva, M.A. A review on piezo-and pyroelectric responses of flexible nano-and micropatterned polymer surfaces for biomedical sensing and energy harvesting applications. Nano Energy 2021, 79, 105442. [Google Scholar] [CrossRef]
- Thakre, A.; Kumar, A.; Song, H.-C.; Jeong, D.-Y.; Ryu, J. Pyroelectric Energy Conversion and Its Applications—Flexible Energy Harvesters and Sensors. Sensors 2019, 19, 2170. [Google Scholar] [CrossRef]
- Bae, J.-H.; Chang, S.-H. PVDF-based ferroelectric polymers and dielectric elastomers for sensor and actuator applications: A review. Funct. Compos. Struct. 2019, 1, 012003. [Google Scholar] [CrossRef]
- Kanno, I. Piezoelectric MEMS: Ferroelectric thin films for MEMS applications. Jpn. J. Appl. Phys. 2018, 57, 040101. [Google Scholar] [CrossRef]
- Furukawa, T. Ferroelectric properties of vinylidene fluoride copolymers. Phase Transit. 1989, 18, 143–211. [Google Scholar] [CrossRef]
- Furukawa, T. Piezoelectricity and pyroelectricity in polymers. IEEE Trans. Electr. Insul. 1989, 24, 375–394. [Google Scholar] [CrossRef]
- Furukawa, T. Structure and Properties of Ferroelectric Polymers. Key Eng. Mater. 1994, 92–93, 15–30. [Google Scholar] [CrossRef]
- Eisenmenger, W.; Schmidt, H.; Dehlen, B. Space charge and dipoles in polyvinylidenefluoride. Braz. J. Phys. 1999, 29, 295–305. [Google Scholar] [CrossRef]
- Martins, P.; Lopes, A.C.; Lanceros-Mendez, S. Electroactive phases of poly(vinylidene fluoride): Determination, processing and applications. Prog. Polym. Sci. 2014, 39, 683–706. [Google Scholar] [CrossRef]
- Dwivedi, S.; Badole, M.; Gangwar, K.; Kumar, S. Relaxation processes and conduction behaviour in PVDF-TrFE and KNN-based composites. Polymer 2021, 232, 124164. [Google Scholar] [CrossRef]
- Zou, Y.; Han, L.; Yuan, G.; Liu, B.; Zhao, X.; Tian, B.B.; Wang, J.; Sun, S.; Sun, J.; Meng, X.; et al. Enhanced ferroelectric and dielectric properties of the P(VDF-TrFE)/Ag nanoparticles composite thin films. J. Mater. Sci. Mater. Electron. 2014, 25, 3461–3465. [Google Scholar] [CrossRef]
- Paik, H.; Choi, Y.-Y.; Hong, S.; No, K. Effect of Ag nanoparticle concentration on the electrical and ferroelectric properties of Ag/P(VDF-TrFE) composite films. Sci. Rep. 2015, 5, 13209. [Google Scholar] [CrossRef]
- Tsutsumi, N.; Kosugi, R.; Kinashi, K.; Sakai, W. Nature of the Enhancement in Ferroelectric Properties by Gold Nanoparticles in Vinylidene Fluoride and Trifluoroethylene Copolymer. ACS Appl. Mater. Interfaces 2016, 8, 16816–16822. [Google Scholar] [CrossRef] [PubMed]
- Graz, I.; Krause, M.; Bauer-Gogonea, S.; Bauer, S.; Lacour, S.P.; Ploss, B.; Zirkl, M.; Stadlober, B.; Wagner, S. Flexible active-matrix cells with selectively poled bifunctional polymer-ceramic nanocomposite for pressure and temperature sensing skin. J. Appl. Phys. 2009, 106, 034503. [Google Scholar] [CrossRef]
- Ploss, B.; Ploss, B.; Shin, F.G.; Chan, H.L.W.; Choy, C.L. Pyroelectric or piezoelectric compensated ferroelectric composites. Appl. Phys. Lett. 2000, 76, 2776–2778. [Google Scholar] [CrossRef]
- Can-Ortiz, A.; Laudebat, L.; Valdez-Nava, Z.; Diaham, S. Nonlinear electrical conduction in polymer composites for field grading in high-voltage applications: A review. Polymers 2021, 13, 1370. [Google Scholar] [CrossRef]
- Hong, J.; Schadler, L.; Siegel, R.; Mårtensson, E. Electrical behavior of low density polyethylene containing an inhomogeneous distribution of ZnO nanoparticles. J. Mater. Sci. 2006, 41, 5810–5814. [Google Scholar] [CrossRef]
- Engel, S.; Smykalla, D.; Ploss, B.; Gräf, S.; Müller, F.A. Effect of (Cd:Zn)S particle concentration and photoexcitation on the electrical and ferroelectric properties of (Cd:Zn)S/P (VDF-TrFE) composite films. Polymers 2017, 9, 650. [Google Scholar] [CrossRef]
- Engel, S.; Smykalla, D.; Ploss, B.; Gräf, S.; Müller, F.A. Polarization Properties and Polarization Depth Profiles of (Cd:Zn)S/P (VDF-TrFE) Composite Films in Dependence of Optical Excitation. Polymers 2018, 10, 1205. [Google Scholar] [CrossRef]
- Wong, Y.W.; Hui, N.M.; Ong, E.L.; Chan, H.L.W.; Choy, C.L. Specific heat and thermal diffusivity of vinylidene fluoride/trifluoroethylene copolymers. J. Appl. Polym. Sci. 2003, 89, 3160–3166. [Google Scholar] [CrossRef]
- Abrikosov, N.K. Semiconducting II–VI, IV–VI, and V–VI Compounds; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Ploss, B.; Shin, F.G.; Chan, H.L.W.; Choy, C.-L. Pyroelectric activity of ferroelectric PT/PVDF-TRFE. IEEE Trans. Dielectr. Electr. Insul. 2000, 7, 517–522. [Google Scholar] [CrossRef]
- Tajitsu, Y.; Ogura, H.; Chiba, A.; Furukawa, T. Investigation of Switching Characteristics of Vinylidene Fluoride/Trifluoroethylene Copolymers in Relation to Their Structures. Jpn. J. Appl. Phys. 1987, 26, 554–560. [Google Scholar] [CrossRef]
- Gregorio, R., Jr.; Botta, M.M. Effect of crystallization temperature on the phase transitions of P(VDF/TrFE) copolymers. J. Polym. Sci. Part B Polym. Phys. 1998, 36, 403–414. [Google Scholar] [CrossRef]
- Li, Y.; Feng, W.; Meng, L.; Tse, K.M.; Li, Z.; Huang, L.; Su, Z.; Guo, S. Investigation on in-situ sprayed, annealed and corona poled PVDF-TrFE coatings for guided wave-based structural health monitoring: From crystallization to piezoelectricity. Mater. Des. 2021, 199, 109415. [Google Scholar] [CrossRef]
- Choi, J.; Borca, C.N.; Dowben, P.A.; Bune, A.; Poulsen, M.; Pebley, S.; Adenwalla, S.; Ducharme, S.; Robertson, L.; Fridkin, V.M.; et al. Phase transition in the surface structure in copolymer films of vinylidene fluoride (70%) with trifluoroethylene (30%). Phys. Rev. B 2000, 61, 5760–5770. [Google Scholar] [CrossRef]
- Bellet-Amalric, E.; Legrand, J.F. Crystalline structures and phase transition of the ferroelectric P(VDF-TrFE) copolymers, a neutron diffraction study. Eur. Phys. J. B-Condens. Matter Complex Syst. 1998, 3, 225–236. [Google Scholar] [CrossRef]
- Wang, L.; Wang, W.; Shang, M.; Yin, W.; Sun, S.; Zhang, L. Enhanced photocatalytic hydrogen evolution under visible light over Cd1−xZnxS solid solution with cubic zinc blend phase. Int. J. Hydrogen Energy 2010, 35, 19–25. [Google Scholar] [CrossRef]
- Kholmicheva, N.; Yang, M.; Moroz, P.; Eckard, H.; Vore, A.; Cassidy, J.; Pushina, M.; Boddy, A.; Porotnikov, D.; Anzenbacher, P.; et al. Ion-Mediated Ligand Exchange and Size Focusing of Semiconductor Nanocrystals in Ligand-Saturated Solutions. J. Phys. Chem. C 2018, 122, 23623–23630. [Google Scholar] [CrossRef]
- Knauf, R.R.; Lennox, J.C.; Dempsey, J.L. Quantifying Ligand Exchange Reactions at CdSe Nanocrystal Surfaces. Chem. Mater. 2016, 28, 4762–4770. [Google Scholar] [CrossRef]
- Mai, M.; Leschhorn, A.; Kliem, H. The field and temperature dependence of hysteresis loops in P(VDF–TrFE) copolymer films. Phys. B Condens. Matter 2015, 456, 306–311. [Google Scholar] [CrossRef]
- Lee, C.-W.; Renaud, C.; Hsu, C.-S.; Nguyen, T.-P. Traps and performance of MEH-PPV/CdSe(ZnS) nanocomposite-based organic light-emitting diodes. Nanotechnology 2008, 19, 455202. [Google Scholar] [CrossRef] [PubMed]
- Greenham, N.C.; Peng, X.; Alivisatos, A.P. Charge separation and transport in conjugated-polymer/semiconductor-nanocrystal composites studied by photoluminescence quenching and photoconductivity. Phys. Rev. B 1996, 54, 17628–17637. [Google Scholar] [CrossRef] [PubMed]
- Jiang, D.; Ding, L.; Huang, J.; Gu, E.; Liu, L.; Chai, Z. Synthesis and characterization of photorefractive polymer based on chemically hybridized CdS–PVK nanocomposite with a new azo chromophore. Polymer 2007, 48, 7156–7162. [Google Scholar] [CrossRef]
- Yang, C.L.; Wang, J.N.; Ge, W.K.; Wang, S.H.; Cheng, J.X.; Li, X.Y.; Yan, Y.J.; Yang, S.H. Significant enhancement of photoconductivity in truly two-component and chemically hybridized CdS-poly(N-vinylcarbazole) nanocomposites. Appl. Phys. Lett. 2001, 78, 760–762. [Google Scholar] [CrossRef]
- Li, Y.C.; Tjong, S.C. 26—Nonlinear current–voltage characteristics in polymer nanocomposites. In Physical Properties and Applications of Polymer Nanocomposites; Tjong, S.C., Mai, Y.W., Eds.; Woodhead Publishing: Sawston, UK, 2010; pp. 862–890. [Google Scholar] [CrossRef]
- Boles, M.A.; Ling, D.; Hyeon, T.; Talapin, D.V. The surface science of nanocrystals. Nat. Mater. 2016, 15, 141–153. [Google Scholar] [CrossRef]
- Yazdani, N.; Andermatt, S.; Yarema, M.; Farto, V.; Bani-Hashemian, M.H.; Volk, S.; Lin, W.M.M.; Yarema, O.; Luisier, M.; Wood, V. Charge transport in semiconductors assembled from nanocrystal quantum dots. Nat. Commun. 2020, 11, 2852. [Google Scholar] [CrossRef]
- Fedosov, S.N.; Sergeeva, A.E.; Eberle, G.; Eisenmenger, W. Polarization profiles in corona poled P(VDF-TFE) copolymer studied by piezoelectrically induced pressure step method. J. Phys. D Appl. Phys. 1996, 29, 3122–3128. [Google Scholar] [CrossRef]
- Holdik, K.; Eisenmenger, W. Charge and polarization dynamics in polymer films. In Proceedings of the 1985 5th International Symposium on Electrets (ISE 5), Heidelberg, West Germany, 4–6 September 1985; pp. 553–558. [Google Scholar]
- Schilling, D.; Glatz-Reichenbach, J.; Dransfeld, K.; Bihler, E.; Eisenmenger, W. Polarization Profiles of Electron-Beam Polarized VDF-TrFE Copolymer Films. In Proceedings of theAnnual Conference on Electrical Insulation and Dielectric Phenomena, IEEE, Pocono Manor, PA, USA, 28–31 October 1990. [Google Scholar]
- Ploss, B.; Ploss, B. Poling of P(VDF-TrFE) with ferroelectrically applied dielectric displacement. Ferroelectrics 1996, 184, 107–116. [Google Scholar] [CrossRef]
- Bihler, E.; Neumann, G.; Eberle, G.; Eisenmenger, W. Influence of charge injection on the formation of remanent polarization in P(VDF-TrFE) copolymers. In Proceedings of the Annual Conference on Electrical Insulation and Dielectric Phenomena, Pocono Manor, PA, USA, 28–31 October 1990; pp. 140–145. [Google Scholar]
- Eberle, G.; Bihler, E.; Eisenmenger, W. Polarization dynamics of VDF-TrFE copolymers. IEEE Trans. Electr. Insul. 1991, 26, 69–77. [Google Scholar] [CrossRef]
- Garcia de Arquer, F.P.; Talapin, D.V.; Klimov, V.I.; Arakawa, Y.; Bayer, M.; Sargent, E.H. Semiconductor quantum dots: Technological progress and future challenges. Science 2021, 373, 6555. [Google Scholar] [CrossRef] [PubMed]
- Lei, D.; Shen, Y.; Feng, Y.; Feng, W. Recent progress in the fields of tuning the band gap of quantum dots. Sci. China Technol. Sci. 2012, 55, 903–912. [Google Scholar] [CrossRef]
- Kroupa, D.M.; Vörös, M.; Brawand, N.P.; McNichols, B.W.; Miller, E.M.; Gu, J.; Nozik, A.J.; Sellinger, A.; Galli, G.; Beard, M.C. Tuning colloidal quantum dot band edge positions through solution-phase surface chemistry modification. Nat. Commun. 2017, 8, 15257. [Google Scholar] [CrossRef] [PubMed]
- Tal’Roze, R.; Shandryuk, G.; Merekalov, A.; Shatalova, A.; Otmakhova, O. Alignment of nanoparticles in polymer matrices. Polym. Sci. Ser. A 2009, 51, 1194–1203. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kober, M.; Smykalla, D.; Ploss, B.; Wächtler, M.; Kumar, K.; Stelter, M.; Engel, S. Ferroelectric Properties of Polymer–Semiconductor Hybrid Material or Composite under Optical Excitation. Polymers 2024, 16, 929. https://doi.org/10.3390/polym16070929
Kober M, Smykalla D, Ploss B, Wächtler M, Kumar K, Stelter M, Engel S. Ferroelectric Properties of Polymer–Semiconductor Hybrid Material or Composite under Optical Excitation. Polymers. 2024; 16(7):929. https://doi.org/10.3390/polym16070929
Chicago/Turabian StyleKober, Michael, David Smykalla, Bernd Ploss, Maria Wächtler, Krishan Kumar, Michael Stelter, and Sebastian Engel. 2024. "Ferroelectric Properties of Polymer–Semiconductor Hybrid Material or Composite under Optical Excitation" Polymers 16, no. 7: 929. https://doi.org/10.3390/polym16070929
APA StyleKober, M., Smykalla, D., Ploss, B., Wächtler, M., Kumar, K., Stelter, M., & Engel, S. (2024). Ferroelectric Properties of Polymer–Semiconductor Hybrid Material or Composite under Optical Excitation. Polymers, 16(7), 929. https://doi.org/10.3390/polym16070929





