Modeling the Impact of Dye Concentration on Polymer Optical Properties via the Complex Refractive Index: A Pathway to Optical Engineering
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Measurement Methods
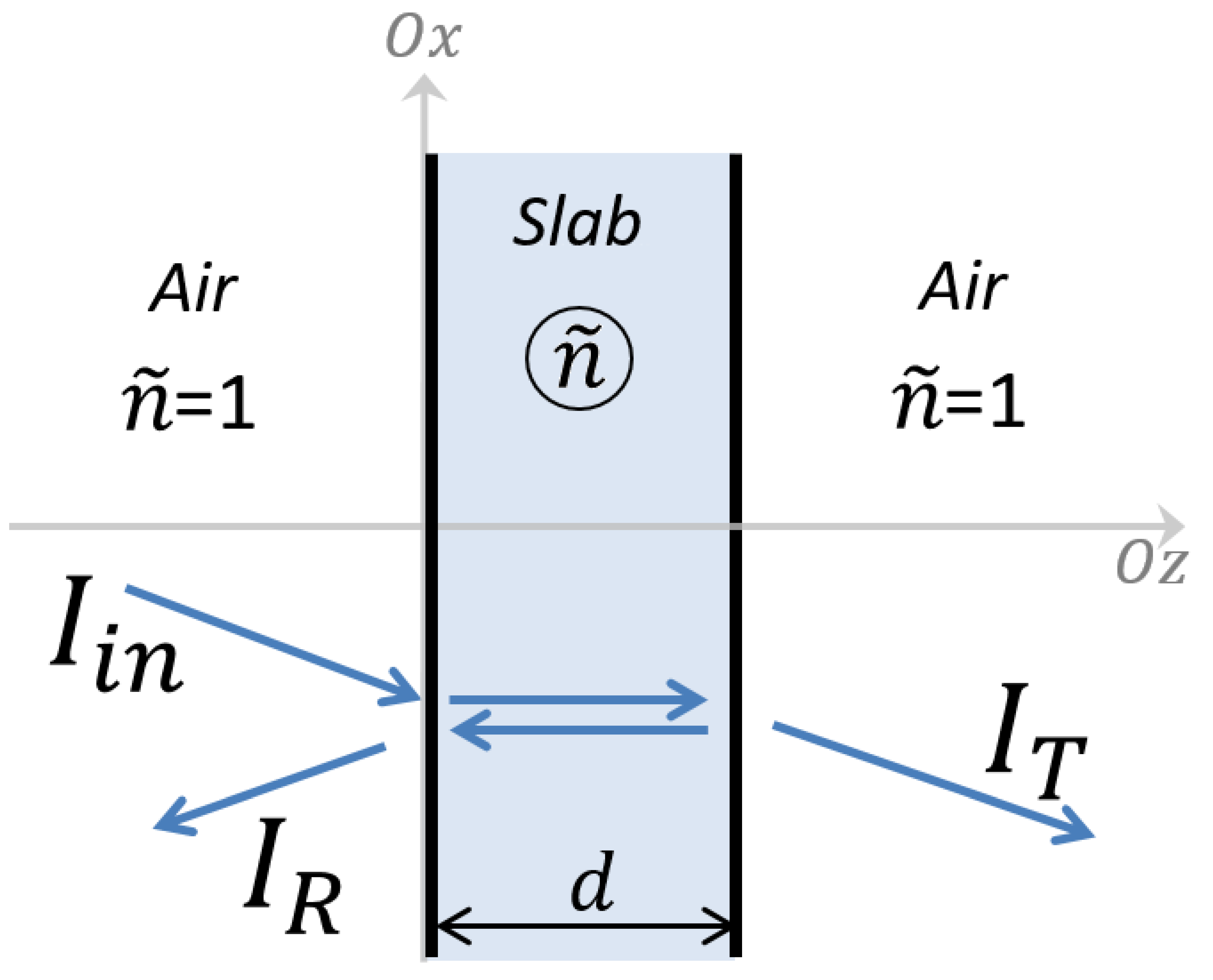
2.3. Theoretical Background
2.4. Complex Refractive Index Calculation from Reflectance and Transmittance
3. Results and Discussion
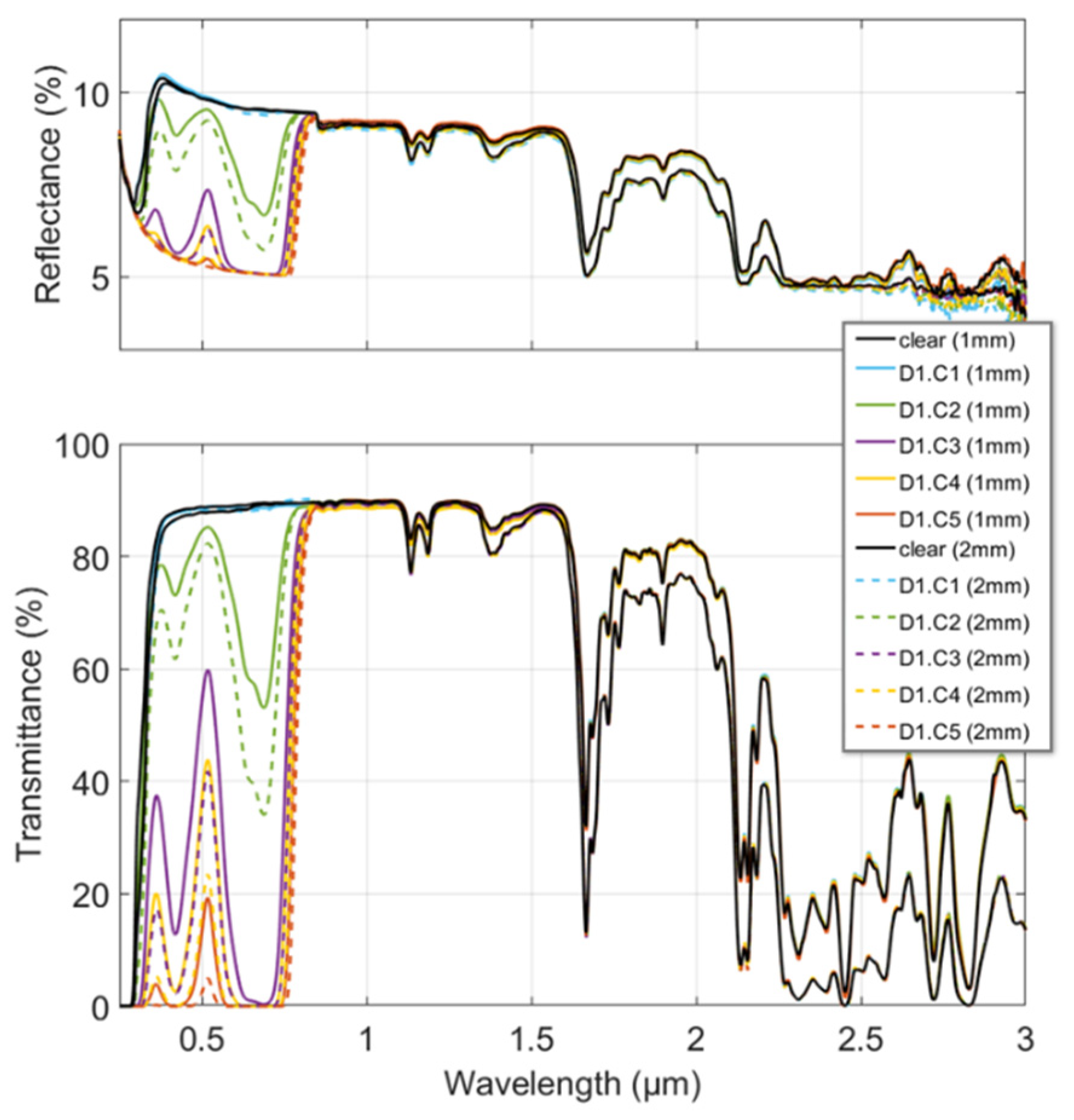
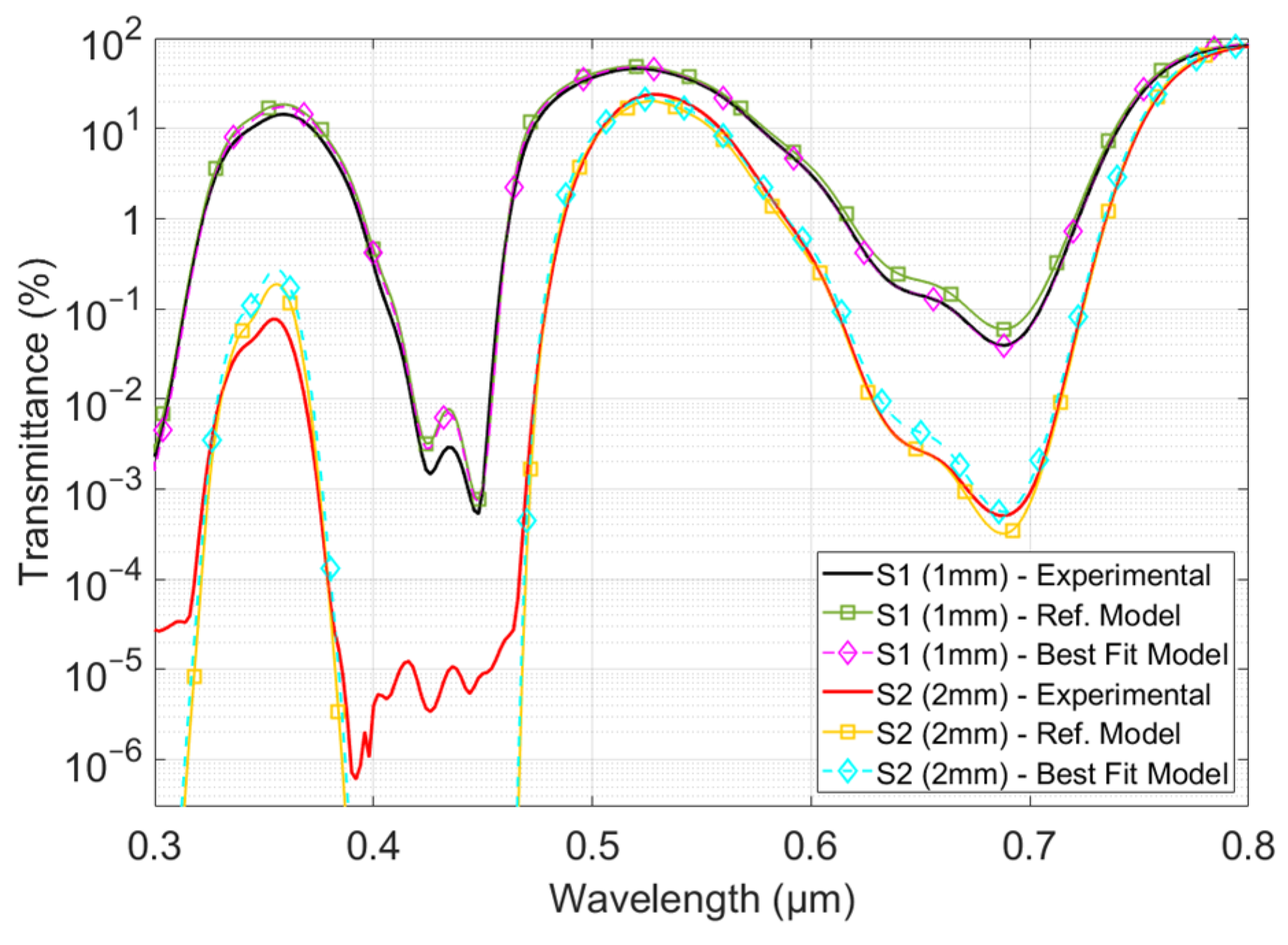
3.1. Reflection and Transmission Measurement
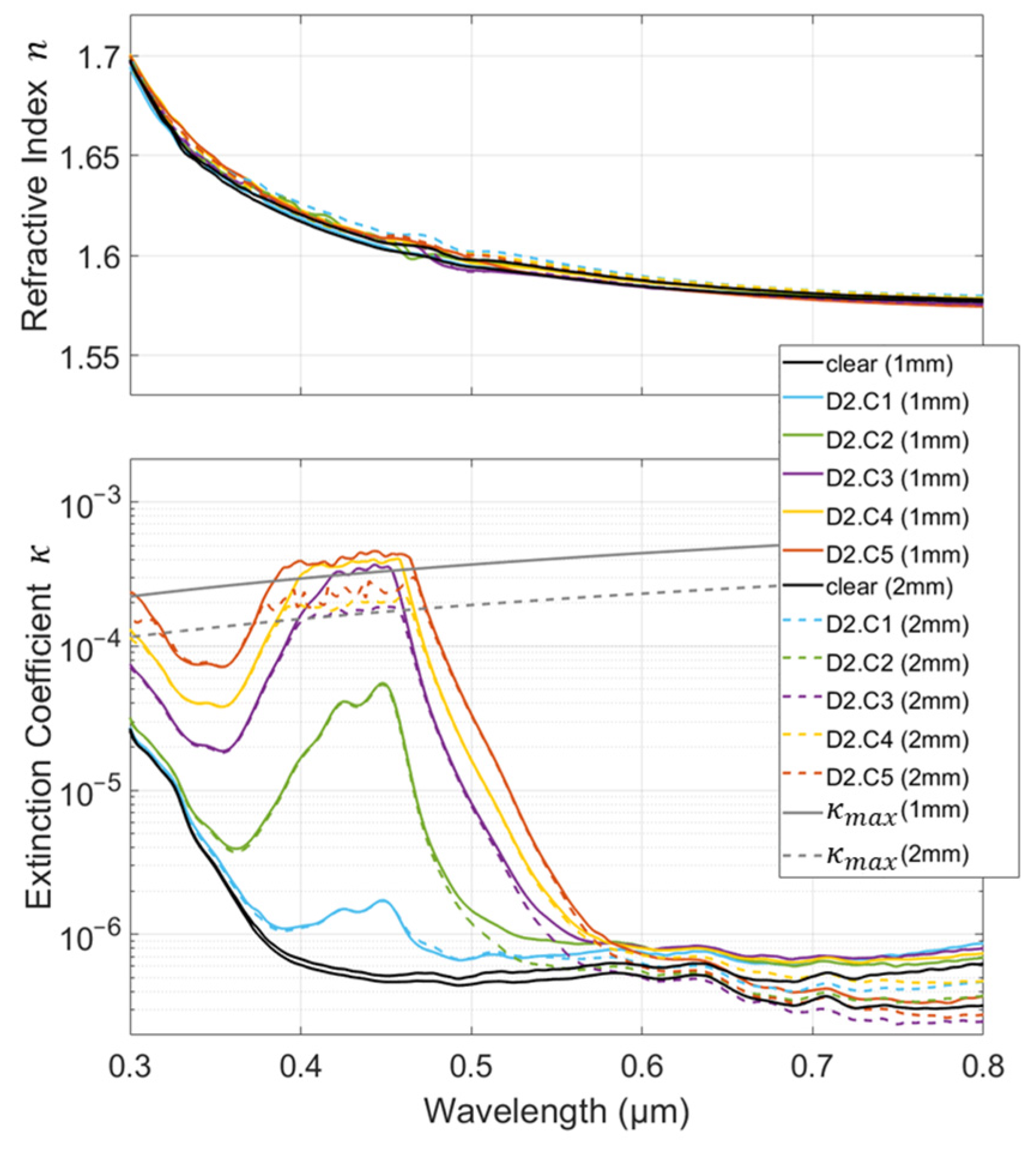
3.2. Complex Refractive Index Calculation
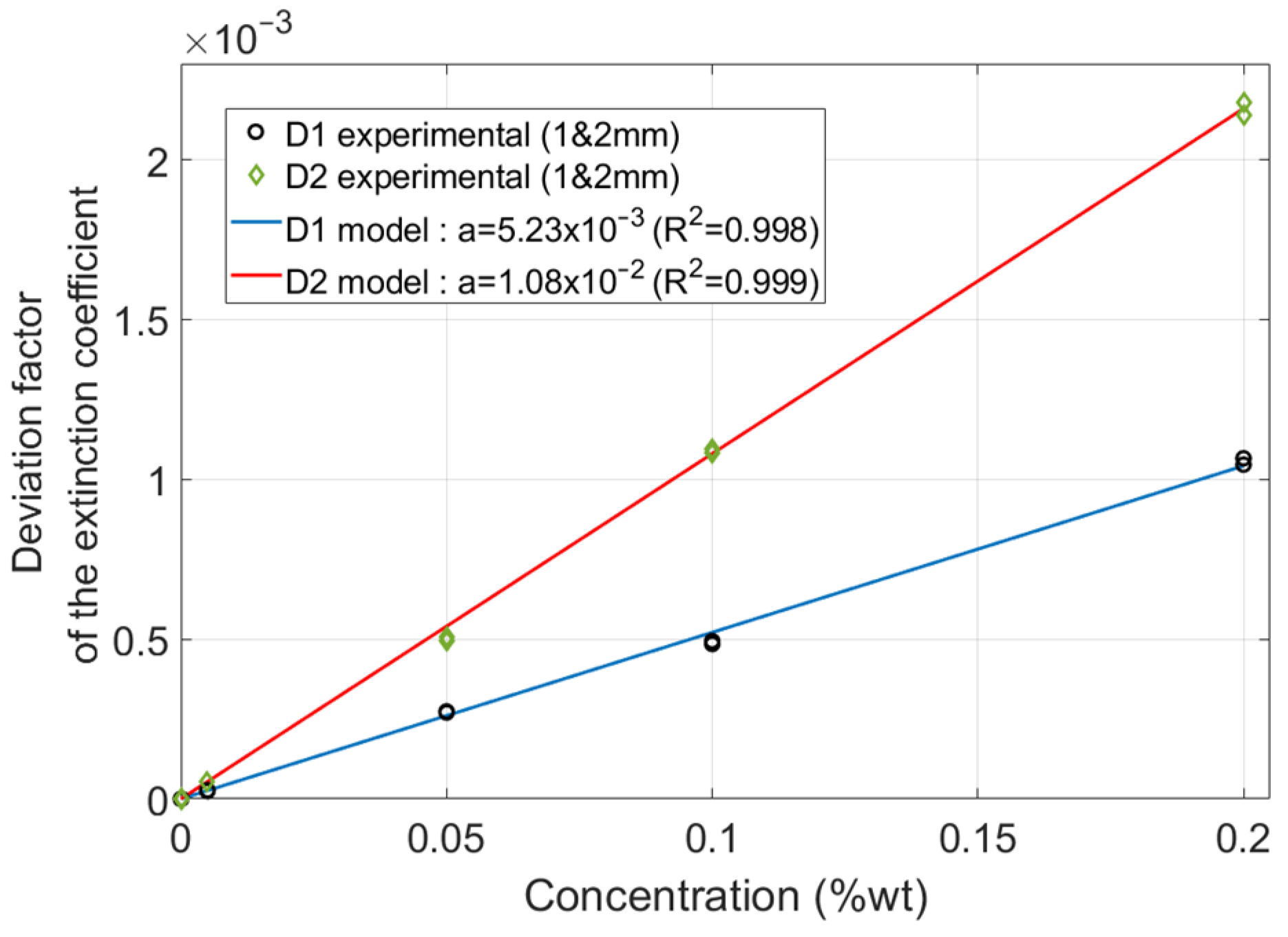
3.3. Modeling the Complex Refractive Index as a Function of the Dye Concentration
3.4. Model Validation and Robustness
4. Conclusions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fleischmann, C.; Lievenbrück, M.; Ritter, H. Polymers and Dyes: Developments and Applications. Polymers 2015, 7, 717–746. [Google Scholar] [CrossRef]
- Ambrogi, A.; Carfagna, C.; Cerruti, P.; Marturano, V. Additives in Polymers. In Modification of Polymer Properties; Elsevier Inc.: Amsterdam, The Netherlands, 2017; Chapter 4; pp. 87–108. [Google Scholar] [CrossRef]
- Plouzeau, M.; Piogé, S.; Peilleron, F.; Fontaine, L.; Pascual, S. Polymer/dye blends: Preparation and optical performance: A short review. J. Appl. Polym. Sci. 2022, 139, e52861. [Google Scholar] [CrossRef]
- Charvat, R.A. Coloring of Plastics: Fundamentals, 2nd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2004. [Google Scholar] [CrossRef]
- Pfaff, G. Colorants in plastic applications. Phys. Sci. Rev. 2021, 6, 20190104. [Google Scholar] [CrossRef]
- Peredo-Guzmán, P.; Trigo-López, M.; Vallejos, S.; García, F.; García, J.M. Intrinsically Coloured Red Aromatic Polyamides. Chem. Proc. 2021, 5, 91. [Google Scholar] [CrossRef]
- Kudrevicius, L.; Adliene, D.; Puiso, J.; Plaga, A. Investigation of Colored Film Indicators for the Assessment of the Occasional Radiation Exposure. Gels 2023, 9, 189. [Google Scholar] [CrossRef] [PubMed]
- Blok, A.J.; Gurnani, P.; Xenopoulos, A.; Burroughs, L.; Duncan, J.; Urbanowicz, R.A.; Tsoleridis, T.; Müller-Kräuter, H.; Strecker, T.; Ball, J.K.; et al. Polymer microarrays rapidly identify competitive adsorbents of virus-like particles. Biointerphases 2020, 15, 061005. [Google Scholar] [CrossRef] [PubMed]
- Blythe, A.R.; Vinson, J.R. Polymeric Materials for Devices in Optical Fibre Systems. Polym. Adv. Technol. 2000, 11, 601–611. [Google Scholar] [CrossRef]
- Villringer, C.; Steglich, P.; Pulwer, S.; Schrader, S.; Laufer, J. Electro-optical properties of doped polymers with high transparency in the visible wavelength range. Opt. Mater. Express 2021, 11, 3801–3811. [Google Scholar] [CrossRef]
- Szukalski, A.; Korbut, A.; Zieniewicz, K.; Zielińska, S. Compatible Photochromic Systems for Opto-Electronic Applications. J. Phys. Chem. B 2021, 125, 13565–13574. [Google Scholar] [CrossRef]
- Koliyoor, J.; Ismayil; Ammathnad Babashankar, D.; Kannenahalli Anandkumar, J.; Thimmaiah, S. Novel approach to enhance the optical and electrical properties of polymer electrolytes using phenol red dye. Polym. Eng. Sci. 2024, 64, 577–593. [Google Scholar] [CrossRef]
- Nogueira, A.F.; Longo, C.; Paoli, M.A.D. Polymers in dye sensitized solar cells: Overview and perspectives. Coord. Chem. Rev. 2004, 248, 1455–1468. [Google Scholar] [CrossRef]
- Papakonstantinou, I.; Portnoi, M.; Debije, M.G. The Hidden Potential of Luminescent Solar Concentrators. Adv. Energy Mater. 2021, 11, 2002883. [Google Scholar] [CrossRef]
- Gnida, P.; Amin, M.F.; Pająk, A.K.; Jarząbek, B. Polymers in High-Efficiency Solar Cells: The Latest Reports. Polymers 2022, 14, 1946. [Google Scholar] [CrossRef] [PubMed]
- Velasco Davoise, L.; Peña Capilla, R.; Díez-Pascual, A.M. Assessment of the Refractive Index and Extinction Coefficient of Graphene-Poly(3-hexylthiophene) Nanocomposites. Polymers 2022, 14, 1828. [Google Scholar] [CrossRef]
- Castelletto, S.; Boretti, A. Luminescence solar concentrators: A technology update. Nano Energy 2023, 109, 108269. [Google Scholar] [CrossRef]
- Chandrahalim, H.; Fan, X. Reconfigurable Solid-state Dye-doped Polymer Ring Resonator Lasers. Sci. Rep. 2015, 5, 18310. [Google Scholar] [CrossRef]
- Ayesta, I.; Zubia, J.; Arrue, J.; Illarramendi, M.A.; Azkune, M. Characterization of Chromatic Dispersion and Refractive Index of Polymer Optical Fibers. Polymers 2017, 9, 730. [Google Scholar] [CrossRef] [PubMed]
- Paz, L.F.; Caño-García, M.; Geday, M.A.; Otón, J.M.; Quintana, X. Identification of dyes and matrices for dye-doped polymer waveguide emitters covering the visible spectrum. Sci. Rep. 2022, 12, 6142. [Google Scholar] [CrossRef]
- Kuriki, K.; Kobayashi, T.; Imai, N.; Tamura, T.; Koike, Y.; Okamoto, Y. Organic dye-doped polymer optical fiber laser. Polym. Adv. Technol. 2000, 11, 612–616. [Google Scholar] [CrossRef]
- Heavens, O.S. Optical properties of thin films. Rep. Prog. Phys. 1960, 23, 1. [Google Scholar] [CrossRef]
- Yeh, P. Optical Waves in Layered Media; John Wiley and Sons: Somerset, NJ, USA, 2005. [Google Scholar]
- Nichelatti, E. Complex refractive index of a slab from reflectance and transmittance: Analytical solution. J. Opt. A Pure Appl. Opt. 2002, 4, 306. [Google Scholar] [CrossRef]
- Brissinger, D. Complex refractive index of polycarbonate over the UV-Vis-IR region from 0.2 to 3 μm. Appl. Opt. 2019, 58, 1341. [Google Scholar] [CrossRef] [PubMed]
- Lamprecht, K.; Papousek, W.; Leising, G. Problem of ambiguity in the determination of optical constants of thin absorbing films from spectroscopic reflectance and transmittance measurements. Appl. Opt. 1997, 36, 6364–6371. [Google Scholar] [CrossRef] [PubMed]
- Lévêque, G.; Villachon-Renard, Y. Determination of optical constants of thin film from reflectance spectra. Appl. Opt. 1990, 29, 3207–3212. [Google Scholar] [CrossRef] [PubMed]
- Poelman, D.; Smet, P.J. Methods for the determination of the optical constants of thin films from single transmission measurements: A critical review. J. Phys. D 2003, 36, 1850–1857. [Google Scholar] [CrossRef]
- Makhlouka, Y.; Sanaâ, F.; Gharbia, M. Ordinary and Extraordinary Complex Refractive Indices Extraction of a Mylar Film by Transmission Spectrophotometry. Polymers 2022, 14, 1805. [Google Scholar] [CrossRef]
- Philipp, H.R.; Legrand, D.G.; Cole, H.S.; Liu, Y.S. The optical properties of bisphenol-A polycarbonate. Polym. Eng. Sci. 1987, 27, 1148–1155. [Google Scholar] [CrossRef]
- Khashan, M.; El-Naggar, A. A new method of finding the optical constants of a solid from the reflectance and transmittance spectrograms of its slab. Opt. Commun. 2000, 174, 445–453. [Google Scholar] [CrossRef]
- Zolotarev, V.M.; Volchek, B.Z.; Vlasova, E.N. Optical constants of industrial polymers in the IR region. Opt. Spectrosc. 2006, 101, 716–723. [Google Scholar] [CrossRef]
- El-Zaiat, S.Y. Determination of the complex refractive index of a thick slab material from its spectral reflectance and transmittance at normal incidence. Optik-Int. J. Light Electron Opt. 2013, 124, 157–161. [Google Scholar] [CrossRef]
- Hofmeister, A.M. Carryover of Sampling Errors and Other Problems in Far-Infrared to Far-Ultraviolet Spectra to Associated Applications. Rev. Mineral. Geochem. 2014, 78, 481–508. [Google Scholar] [CrossRef]
- Mayerhöfer, T.G.; Mutschke, H.; Popp, J. Employing theories far beyond their limits—The case of the (Boguer-) beer–lambert law. Chem. Phys. Chem. 2016, 17, 1948–1955. [Google Scholar] [CrossRef] [PubMed]
- Mayerhöfer, T.G.; Pahlow, S.; Popp, J. The Bouguer-Beer-Lambert Law: Shining Light on the Obscure. Chem. Phys. Chem. 2020, 21, 2029–2046. [Google Scholar] [CrossRef] [PubMed]
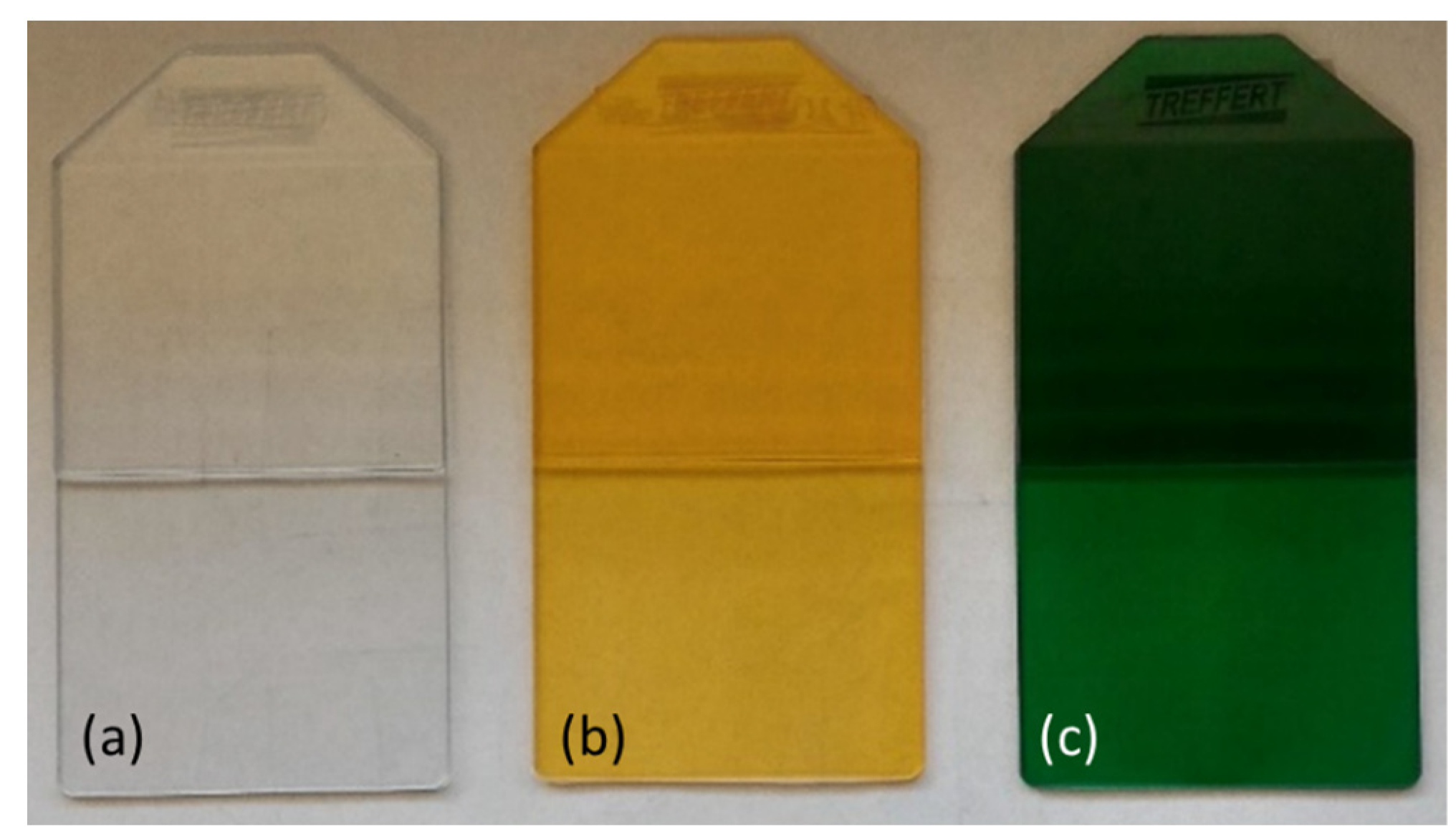

| Learning Group | Test Group | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Clear | Set 1 | Set 2 | Set 3 | ||||||||||
| Concentration (%wt) | C0 | C1 | C2 | C3 | C4 | C5 | C1 | C2 | C3 | C4 | C5 | S1 | S2 |
| Dye 1 (D1) | 0 | 1 × 10−4 | 5 × 10−3 | 5 × 10−2 | 1 × 10−1 | 2 × 10−1 | 0 | 0 | 0 | 0 | 0 | ? > 0 | ? > 0 |
| Dye 2 (D2) | 0 | 0 | 0 | 0 | 0 | 0 | 1 × 10−4 | 5 × 10−3 | 5 × 10−2 | 1 × 10−1 | 2 × 10−1 | ? > 0 | ? > 0 |
| Dye 1 (D1) | Dye 2 (D2) | |||||
|---|---|---|---|---|---|---|
| Reference Concentration (%wt) | Calculated Concentration (%wt) | Deviation (%) | Reference Concentration (%wt) | Calculated Concentration (%wt) | Deviation (%) | |
| Sample Test 1 | 7.0 × 10−2 | 7.42 × 10−2 | 6.0 | 3.0 × 10−2 | 2.99 × 10−2 | 0.3 |
| Sample Test 2 | 6.0 × 10−2 | 6.28 × 10−2 | 4.7 | 1.5 × 10−1 | 1.61 × 10−1 | 7.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brissinger, D. Modeling the Impact of Dye Concentration on Polymer Optical Properties via the Complex Refractive Index: A Pathway to Optical Engineering. Polymers 2024, 16, 660. https://doi.org/10.3390/polym16050660
Brissinger D. Modeling the Impact of Dye Concentration on Polymer Optical Properties via the Complex Refractive Index: A Pathway to Optical Engineering. Polymers. 2024; 16(5):660. https://doi.org/10.3390/polym16050660
Chicago/Turabian StyleBrissinger, Damien. 2024. "Modeling the Impact of Dye Concentration on Polymer Optical Properties via the Complex Refractive Index: A Pathway to Optical Engineering" Polymers 16, no. 5: 660. https://doi.org/10.3390/polym16050660
APA StyleBrissinger, D. (2024). Modeling the Impact of Dye Concentration on Polymer Optical Properties via the Complex Refractive Index: A Pathway to Optical Engineering. Polymers, 16(5), 660. https://doi.org/10.3390/polym16050660





