Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields
Abstract
1. Introduction
2. Electrical Conduction in Polymers
3. Materials and Methods
4. Results and Discussion
4.1. Dynamic Mechanical Analysis
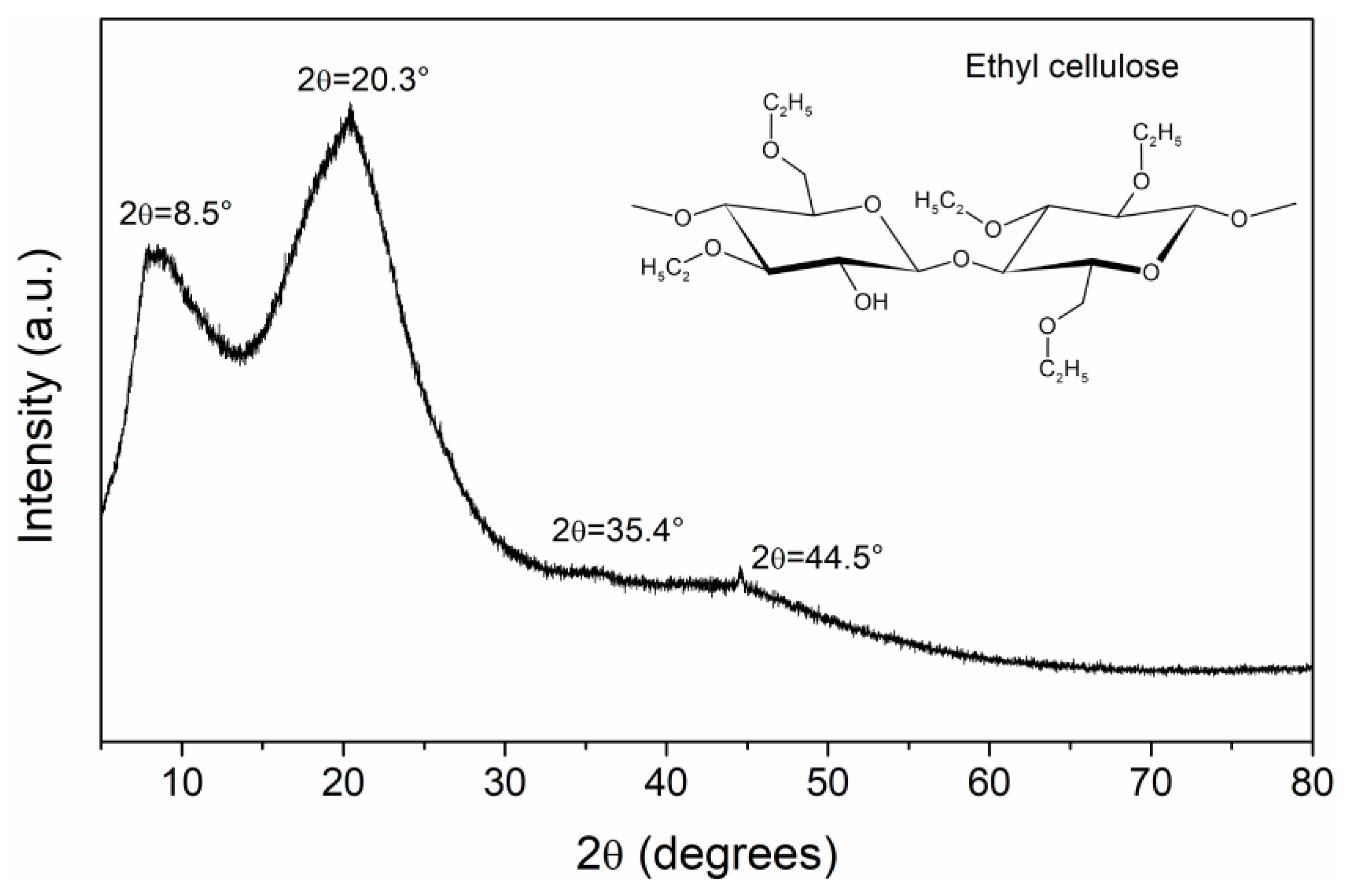
4.2. X-ray Difraction Analysis
4.3. Transient Currents
4.4. Analysis of Conduction Mechanisms
4.5. AC Electrical Conductivity
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Scarpelli, F.; Crispini, A.; Giorno, E.; Marchetti, F.; Pettinari, R.; Di Nicola, C.; De Santo, M.P.; Fuoco, E.; Berardi, R.; Alfano, P.; et al. Preparation and Characterization of Silver(I) Ethylcellulose Thin Films as Potential Food Packaging Materials. Chempluschem 2020, 85, 426–440. [Google Scholar] [CrossRef]
- Reyes-Melo, M.E.; Miranda-Valdez, I.Y.; Puente-Córdova, J.G.; Camarillo-Hernández, C.A.; López-Walle, B. Fabrication and Characterization of a Biocompatible Hybrid Film Based on Silver Nanoparticle/Ethyl Cellulose Polymer. Cellulose 2021, 28, 9227–9240. [Google Scholar] [CrossRef]
- Huang, W.D.; Xu, X.; Wang, H.L.; Huang, J.X.; Zuo, X.H.; Lu, X.J.; Liu, X.L.; Yu, D.G. Electrosprayed Ultra-Thin Coating of Ethyl Cellulose on Drug Nanoparticles for Improved Sustained Release. Nanomaterials 2020, 10, 1758. [Google Scholar] [CrossRef]
- Wasilewska, K.; Winnicka, K. Ethylcellulose-a Pharmaceutical Excipient with Multidirectional Application in Drug Dosage Forms Development. Materials 2019, 12, 3386. [Google Scholar] [CrossRef] [PubMed]
- Kumanek, B.; Stando, G.; Wróbel, P.S.; Krzywiecki, M.; Janas, D. Thermoelectric Properties of Composite Films from Multi-Walled Carbon Nanotubes and Ethyl Cellulose Doped with Heteroatoms. Synth. Met. 2019, 257, 116190. [Google Scholar] [CrossRef]
- Pang, C.; Wang, H.; Lin, X. Ultralight Ethyl Cellulose-Based Electret Fiber Membrane for Low-Resistance and High-Efficient Capture of PM2.5. Colloids Surfaces A Physicochem. Eng. Asp. 2021, 630, 127643. [Google Scholar] [CrossRef]
- Mardi, S.; Risi Ambrogioni, M.; Reale, A. Developing Printable Thermoelectric Materials Based on Graphene Nanoplatelet/Ethyl Cellulose Nanocomposites. Mater. Res. Express 2020, 7, 085101. [Google Scholar] [CrossRef]
- Puente-Córdova, J.G.; Reyes-Melo, M.E.; López-Walle, B.; Miranda-Valdez, I.Y.; Torres-Castro, A. Characterization of a Magnetic Hybrid Film Fabricated by the In-Situ Synthesis of Iron Oxide Nanoparticles into Ethyl Cellulose Polymer. Cellulose 2022, 29, 3845–3857. [Google Scholar] [CrossRef]
- Khare, P.K.; Verma, A.; Paliwal, S.K. Thermally Stimulated Current and Electrical Conduction in Metal (1)-Ethyl Cellulose-Metal (1)/(2) Systems. Bull. Mater. Sci. 1998, 21, 207–212. [Google Scholar] [CrossRef]
- Khare, P.K.; Pandey, R.K.; Jain, P.L. Electrical Transport in Ethyl Cellulose-Chloranil System. Bull. Mater. Sci. 2000, 23, 325–330. [Google Scholar] [CrossRef]
- Bidault, O.; Assifaoui, A.; Champion, D.; Le Meste, M. Dielectric Spectroscopy Measurements of the Sub-Tg Relaxations in Amorphous Ethyl Cellulose: A Relaxation Magnitude Study. J. Non-Cryst. Solids 2005, 351, 1167–1178. [Google Scholar] [CrossRef]
- Chiu, F.-C. A Review on Conduction Mechanisms in Dielectric Films. Adv. Mater. Sci. Eng. 2014, 2014, 578168. [Google Scholar] [CrossRef]
- Laurent, C.; Teyssedre, G.; Le Roy, S.; Baudoin, F. Charge Dynamics and Its Energetic Features in Polymeric Materials. IEEE Trans. Dielectr. Electr. Insul. 2013, 20, 357–381. [Google Scholar] [CrossRef]
- Teyssedre, G.; Laurent, C. Charge Transport Modeling in Insulating Polymers: From Molecular to Macroscopic Scale. IEEE Trans. Dielectr. Electr. Insul. 2005, 12, 857–875. [Google Scholar] [CrossRef]
- Hadri, B.; Mamy, P.R.; Martinez, J.; Mostefa, M. Electrical Conduction in a Semicrystalline Polyethylene Terephthalate in High Electric Field. Solid State Commun. 2006, 139, 35–39. [Google Scholar] [CrossRef]
- Cambareri, P.; de Falco, C.; Di Rienzo, L.; Seri, P.; Montanari, G.C. Simulation and Modelling of Transient Electric Fields in HVDC Insulation Systems Based on Polarization Current Measurements. Energies 2021, 14, 8323. [Google Scholar] [CrossRef]
- Akram, S.; Haq, I.U.; Castellon, J.; Nazir, M.T. Examining the Mechanism of Current Conduction at Varying Temperatures in Polyimide Nanocomposite Films. Energies 2023, 16, 7796. [Google Scholar] [CrossRef]
- Joy Singh, A. Conduction Mechanism in (ZnO/PVC) Polymer Nanocomposite. J. Phys. Conf. Ser. 2021, 2070, 012007. [Google Scholar] [CrossRef]
- Li, H.; Zhou, Y.; Liu, Y.; Li, L.; Liu, Y.; Wang, Q. Dielectric Polymers for High-Temperature Capacitive Energy Storage. Chem. Soc. Rev. 2021, 50, 6369–6400. [Google Scholar] [CrossRef]
- Jensen, K.L. Electron Emission Theory and Its Application: Fowler–Nordheim Equation and Beyond. J. Vac. Sci. Technol. B Microelectron. Nanom. Struct. Process. Meas. Phenom. 2003, 21, 1528–1544. [Google Scholar] [CrossRef]
- Namouchi, F.; Guermazi, H.; Notingher, P.; Agnel, S. Effect of Space Charges on the Local Field and Mechanisms of Conduction in Aged PMMA. IOP Conf. Ser. Mater. Sci. Eng. 2010, 13, 012006. [Google Scholar] [CrossRef]
- Kosaki, M.; Sugiyama, K.; Ieda, M. Ionic Jump Distance and Glass Transition of Polyvinyl Chloride. J. Appl. Phys. 1971, 42, 3388–3392. [Google Scholar] [CrossRef]
- Davidovich-Pinhas, M.; Barbut, S.; Marangoni, A.G. Physical Structure and Thermal Behavior of Ethylcellulose. Cellulose 2014, 21, 3243–3255. [Google Scholar] [CrossRef]
- Luo, Q.; Shen, H.; Zhou, G.; Xu, X. A Mini-Review on the Dielectric Properties of Cellulose and Nanocellulose-Based Materials as Electronic Components. Carbohydr. Polym. 2023, 303, 120449. [Google Scholar] [CrossRef]
- Morsalin, S.; Phung, B.T. Dielectric Response Study of Service-Aged XLPE Cable Based on Polarisation and Depolarisation Current Method. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 58–66. [Google Scholar] [CrossRef]
- Zaengl, W.S. Dielectric Spectroscopy in Time and Frequency Domain for HV Power Equipment. I. Theoretical Considerations. IEEE Electr. Insul. Mag. 2003, 19, 5–19. [Google Scholar] [CrossRef]
- Ryapolov, P.A.; Postnikov, E.B. Mittag–Leffler Function as an Approximant to the Concentrated Ferrofluid’s Magnetization Curve. Fractal Fract. 2021, 5, 147. [Google Scholar] [CrossRef]
- Mainardi, F. Why the Mittag-Leffler Function Can Be Considered the Queen Function of the Fractional Calculus? Entropy 2020, 22, 1359. [Google Scholar] [CrossRef] [PubMed]
- Puente-Córdova, J.G.; Rentería-Baltiérrez, F.Y.; Reyes-Melo, M.E. La Derivada Conformable y Sus Aplicaciones en Ingeniería. Ingenierias 2020, 23, 20–31. [Google Scholar] [CrossRef]
- Górska, K.; Horzela, A.; Penson, K.A. Non-Debye Relaxations: The Ups and Downs of the Stretched Exponential vs. Mittag–Leffler’s Matchings. Fractal Fract. 2021, 5, 265. [Google Scholar] [CrossRef]
- Podlubny, I.; Petras, I.; Skovranek, T. Fitting of Experimental Data Using Mittag-Leffler Function. In Proceedings of the 13th International Carpathian Control Conference (ICCC), High Tatras, Slovakia, 28–31 May 2012; pp. 578–581. [Google Scholar]
- Guillermin, C.; Rain, P.; Rowe, S.W. Transient and Steady-State Currents in Epoxy Resin. J. Phys. D Appl. Phys. 2006, 39, 515–524. [Google Scholar] [CrossRef]
- Einfeldt, J.; Meißner, D.; Kwasniewski, A. Contributions to the Molecular Origin of the Dielectric Relaxation Processes in Polysaccharides–the High Temperature Range. J. Non-Cryst. Solids 2003, 320, 40–55. [Google Scholar] [CrossRef]
- Miranda-Valdez, I.Y.; Camarillo-Hernández, C.A.; Reyes-Melo, M.E.; Puente-Córdova, J.G.; López-Walle, B. Aspectos Estructurales, Reológicos y Dieléctricos de La Etil Celulosa. Ingenierías 2019, 22, 40–53. [Google Scholar]
- Mouchache, C.; Saidi-Amroun, N.; Griseri, V.; Saidi, M.; Teyssedre, G. Electrical Conduction and Space Charge in Gamma-Irradiated XLPE. IEEE Trans. Dielectr. Electr. Insul. 2023, 30, 2099–2106. [Google Scholar] [CrossRef]
- Greenhoe, B.M.; Hassan, M.K.; Wiggins, J.S.; Mauritz, K.A. Universal Power Law Behavior of the AC Conductivity versus Frequency of Agglomerate Morphologies in Conductive Carbon Nanotube-reinforced Epoxy Networks. J. Polym. Sci. Part B Polym. Phys. 2016, 54, 1918–1923. [Google Scholar] [CrossRef]
- Roy, A.; Ponnam, A.; Varade, V.; Honnavar, G.V.; Menon, R. Evidence of Lampert Triangle and Jonscher’s Double Power Law in Doped Poly(3,4-Ethylenedioxythiophene) Devices. J. Phys. Chem. C 2023, 127, 5502–5512. [Google Scholar] [CrossRef]
- Mohamed, H.F.M.; Abdel-Hady, E.E.; Mohammed, W.M. Investigation of Transport Mechanism and Nanostructure of Nylon-6,6/PVA Blend Polymers. Polymers 2022, 15, 107. [Google Scholar] [CrossRef] [PubMed]
- Samet, M.; Kallel, A.; Serghei, A. Maxwell-Wagner-Sillars Interfacial Polarization in Dielectric Spectra of Composite Materials: Scaling Laws and Applications. J. Compos. Mater. 2022, 56, 3197–3217. [Google Scholar] [CrossRef]
- Shukla, P.; Gaur, M.S. Investigation of Electrical Conduction Mechanism in Double-layered Polymeric System. J. Appl. Polym. Sci. 2009, 114, 222–230. [Google Scholar] [CrossRef]

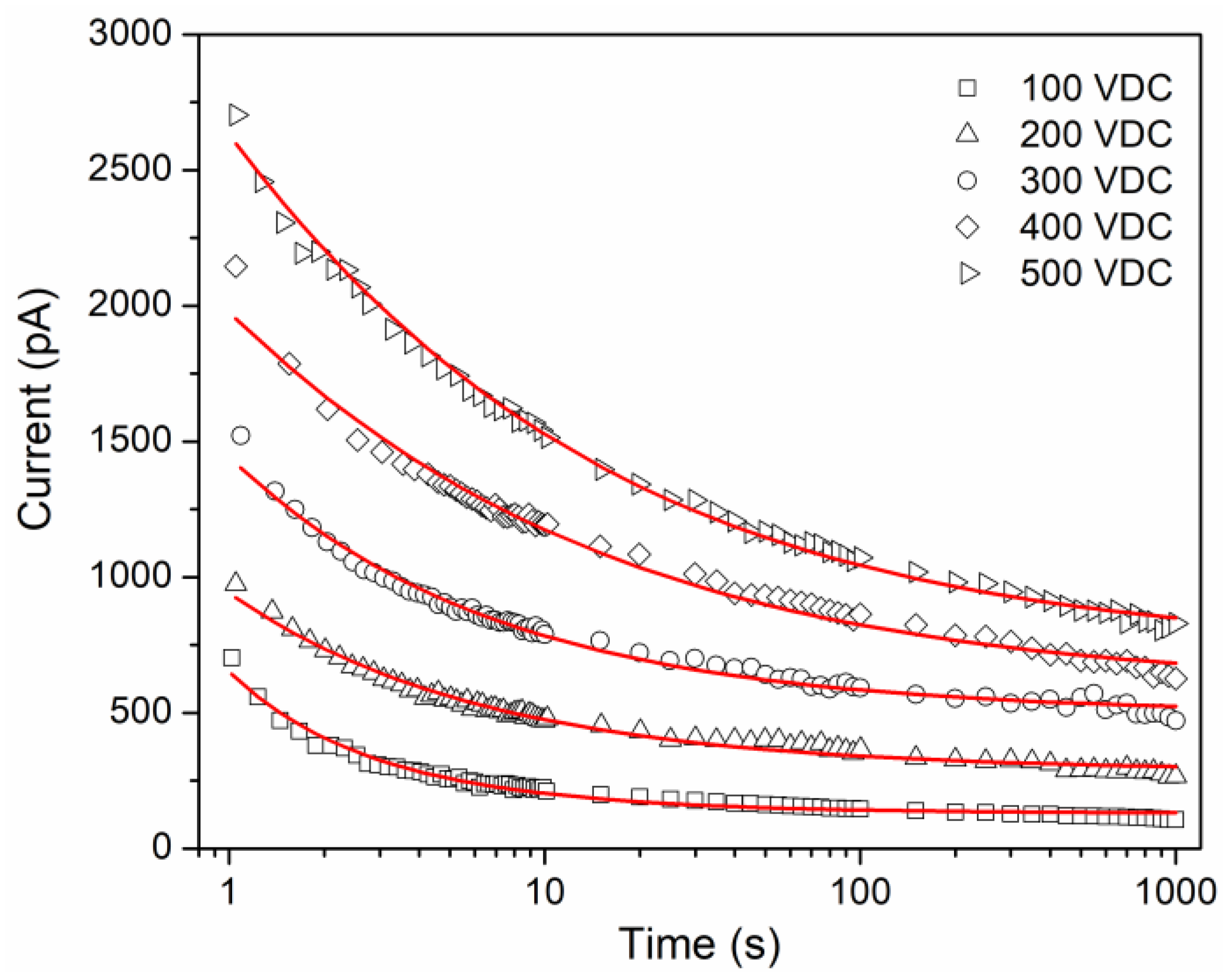
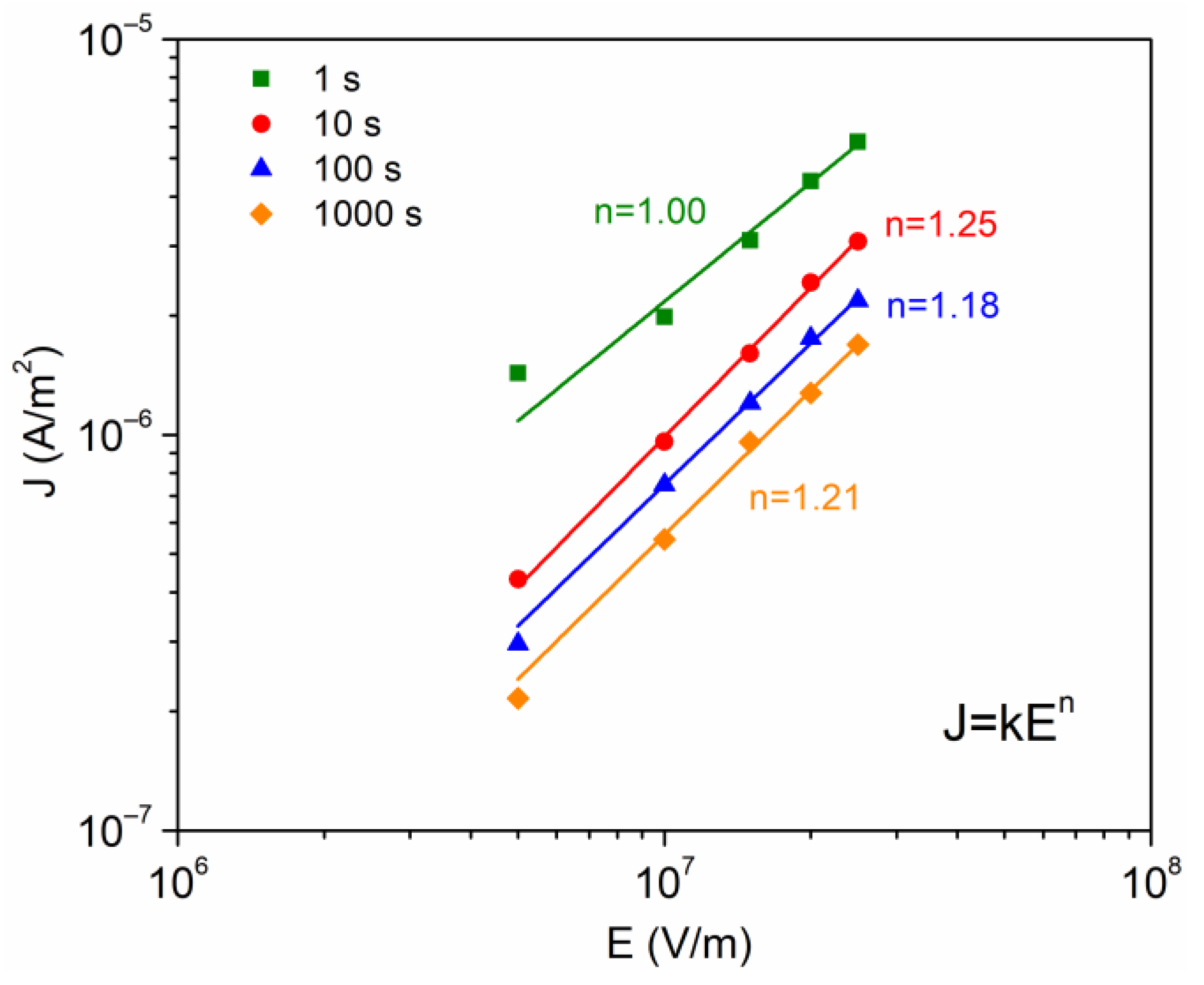
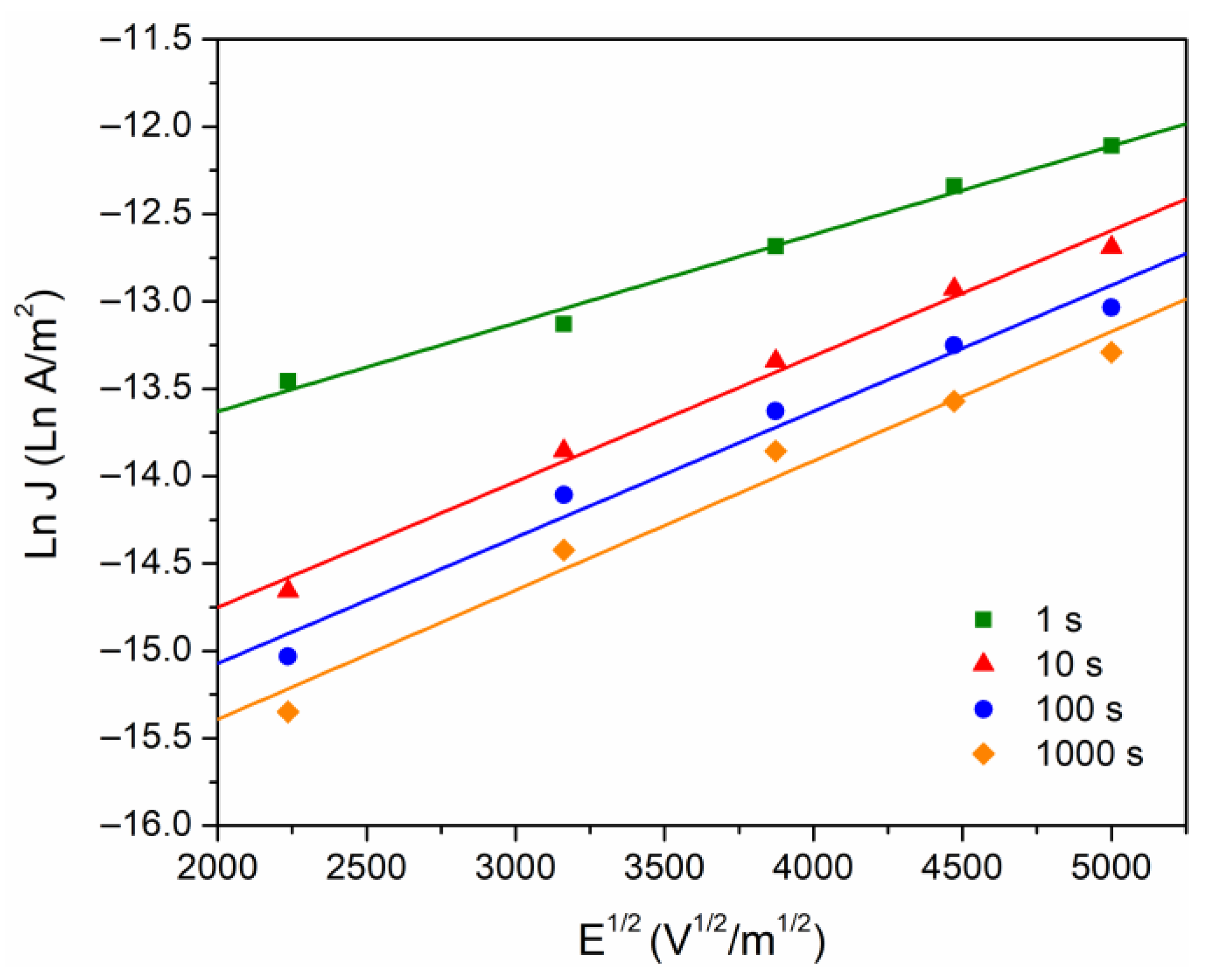
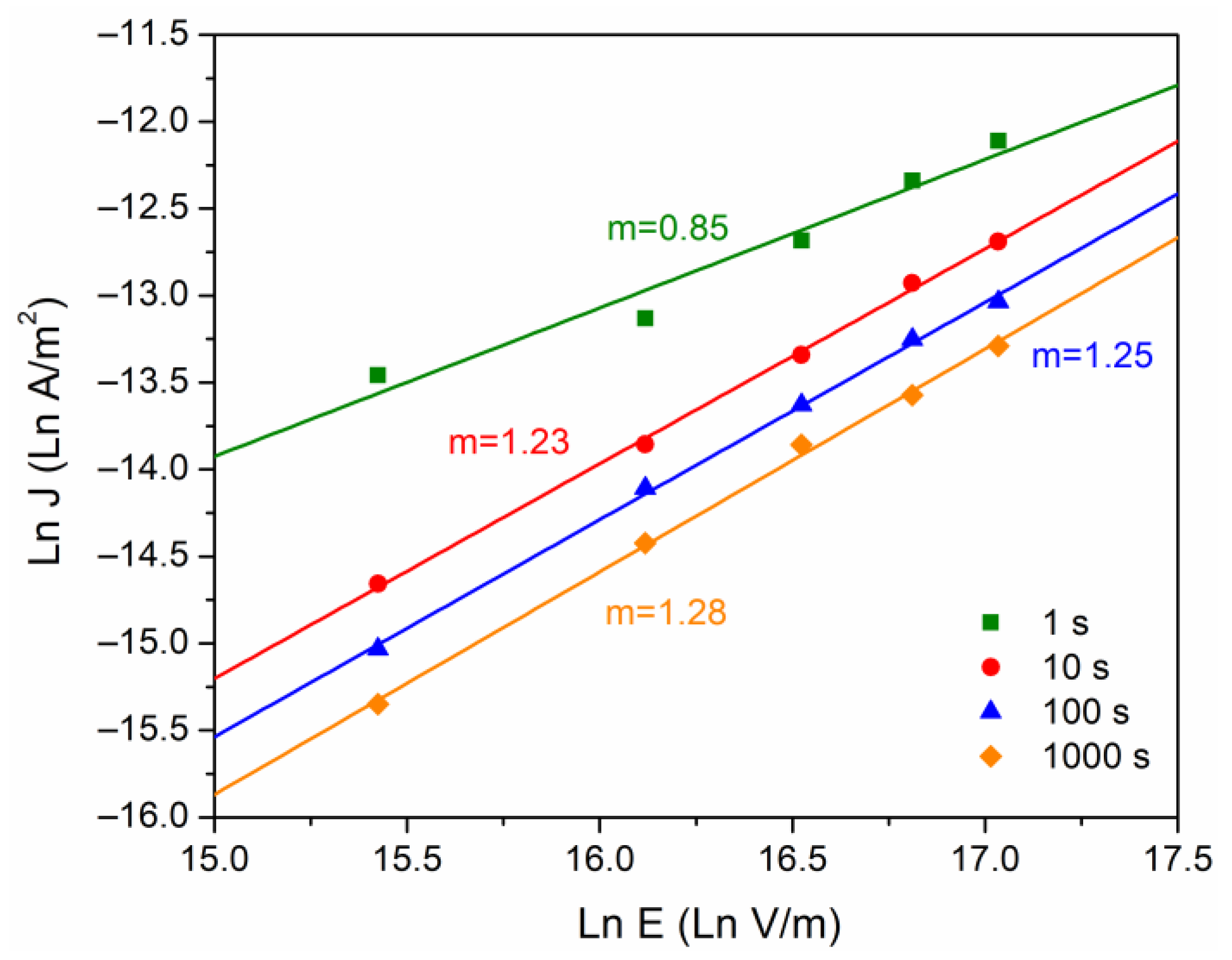
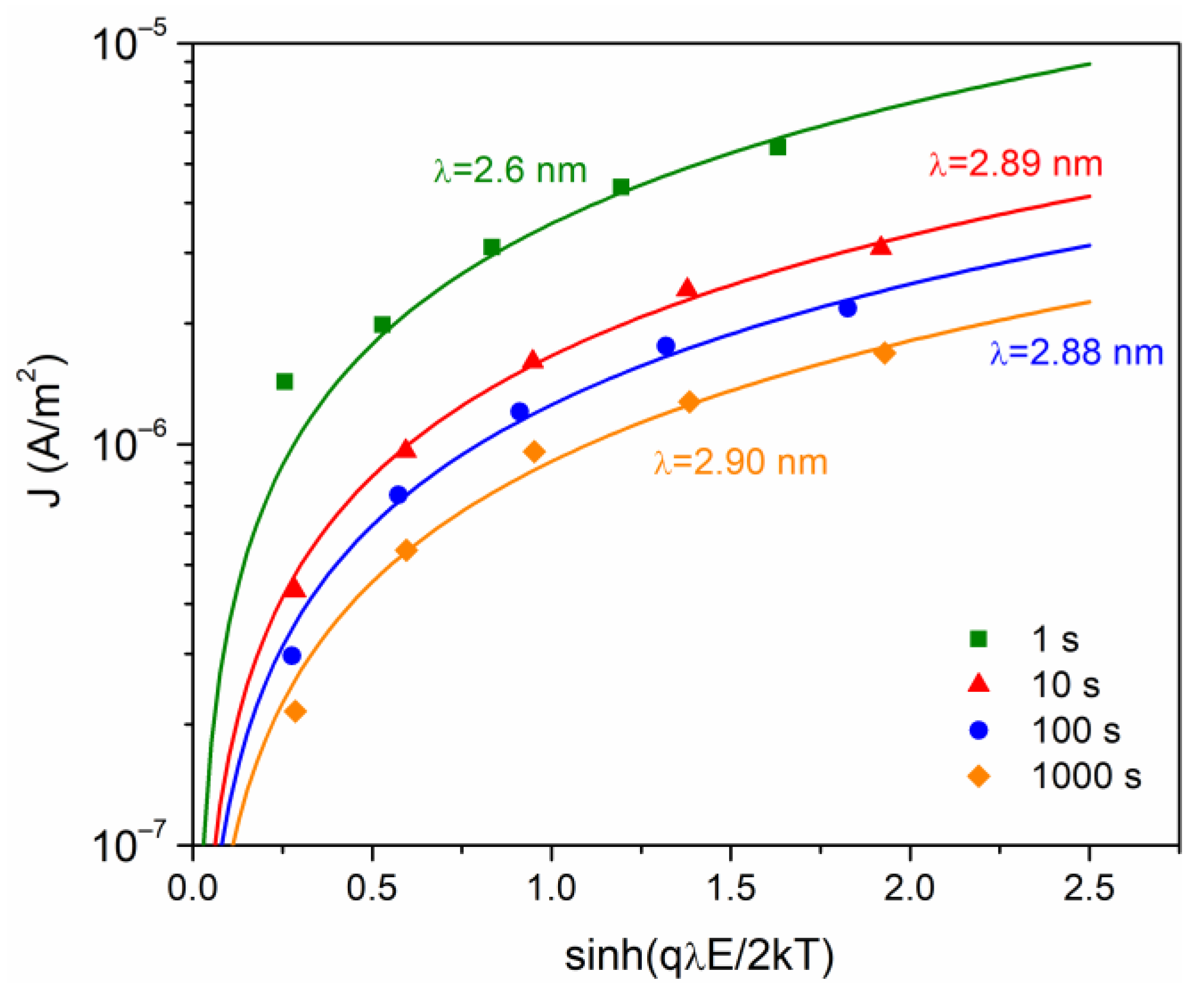

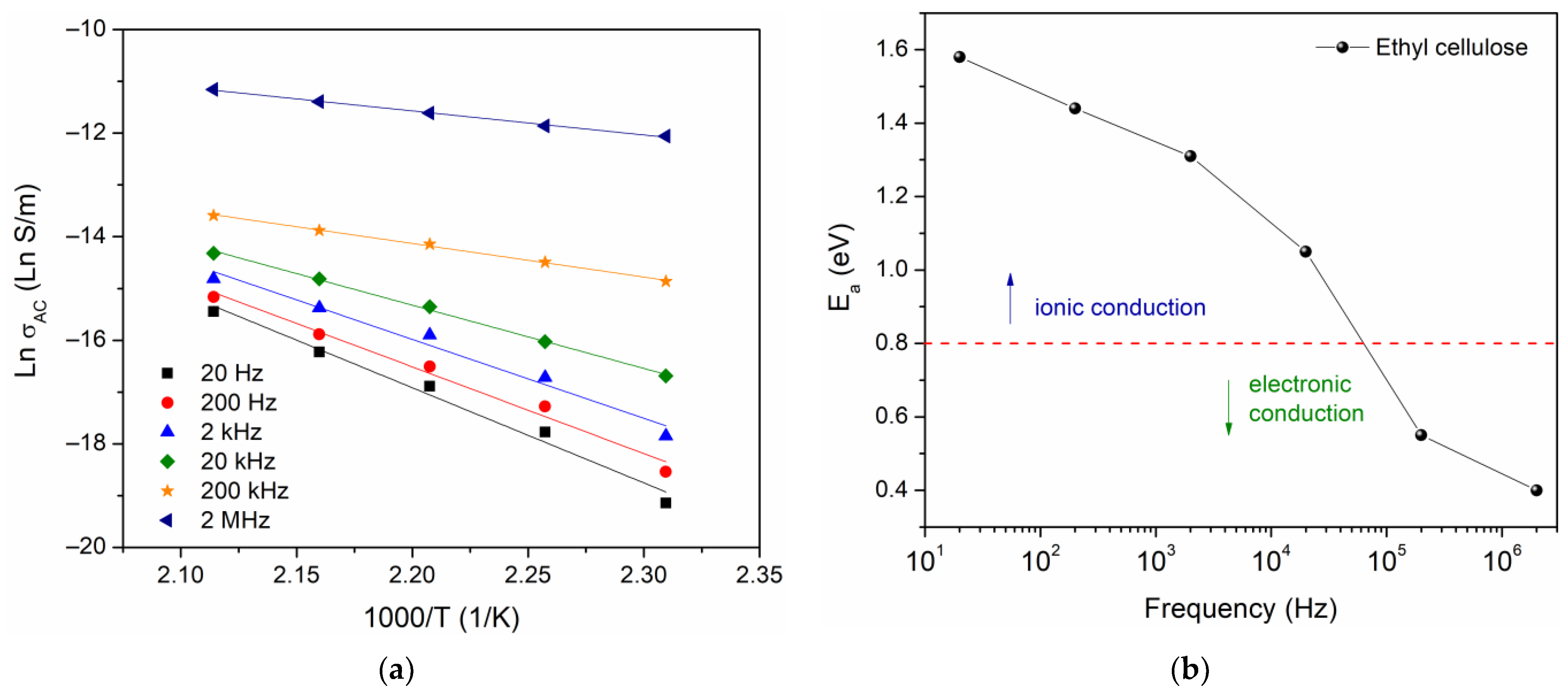
| Parameter | 100 V | 200 V | 300 V | 400 V | 500 V |
|---|---|---|---|---|---|
| Ic (pA) | 129.61 | 286.06 | 497.81 | 591.51 | 726.03 |
| I0 (pA) | 6289 | 11,532 | 16,290 | 7055 | 9120 |
| τ (s) | 0.1519 | 0.0159 | 0.0135 | 0.0707 | 0.0866 |
| α (-) | 0.75 | 0.53 | 0.52 | 0.41 | 0.41 |
| AAD (%) | 6.41 | 3.63 | 2.49 | 2.67 | 1.88 |
| Parameter | 1 s | 10 s | 100 s | 1000 s |
|---|---|---|---|---|
| (J m1/2/V1/2) | 2.08 × 10−24 | 2.96 × 10−24 | 2.97 × 10−24 | 3.04 × 10−24 |
| (J m1/2/V1/2) | 4.17 × 10−24 | 5.91 × 10−24 | 5.94 × 10−24 | 6.09 × 10−24 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Puente-Córdova, J.G.; Luna-Martínez, J.F.; Mohamed-Noriega, N.; Miranda-Valdez, I.Y. Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields. Polymers 2024, 16, 628. https://doi.org/10.3390/polym16050628
Puente-Córdova JG, Luna-Martínez JF, Mohamed-Noriega N, Miranda-Valdez IY. Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields. Polymers. 2024; 16(5):628. https://doi.org/10.3390/polym16050628
Chicago/Turabian StylePuente-Córdova, Jesús G., Juan F. Luna-Martínez, Nasser Mohamed-Noriega, and Isaac Y. Miranda-Valdez. 2024. "Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields" Polymers 16, no. 5: 628. https://doi.org/10.3390/polym16050628
APA StylePuente-Córdova, J. G., Luna-Martínez, J. F., Mohamed-Noriega, N., & Miranda-Valdez, I. Y. (2024). Electrical Conduction Mechanisms in Ethyl Cellulose Films under DC and AC Electric Fields. Polymers, 16(5), 628. https://doi.org/10.3390/polym16050628









