Flexural Behavior of Innovative Glass Fiber-Reinforced Composite Beams Reinforced with Gypsum-Based Composites
Abstract
1. Introduction
2. Materials and Methodology
2.1. Pultruded GFRP Beams’ Properties
2.2. Desulfurization Gypsum
2.3. PVA
2.4. Steel Bars
2.5. Coagulation Test
- (a)
- Weigh 300 g of gypsum, 210 g of water, and the specified ratio of retarder. Thoroughly mix the gypsum and retarder, and within 5 s, pour the sample into water to achieve a uniform slurry, as shown in Figure 3a.
- (b)
- Pour the evenly stirred slurry into the ring mold, ensuring the slurry flushes to the upper end of the ring mold, as depicted in Figure 3b.
- (c)
- Record the initial setting time, defined as the period from the contact of the sample with water to the first instance when the steel needle cannot touch the glass-bottom plate, as demonstrated in Figure 3c.
- (d)
- Record the final setting time, defined as the period from the contact of the sample with water to the time when the depth of the steel needle inserted into the slurry is below 1 mm for the first time, as shown in Figure 3d.
2.6. Fabrication of Gypsum Blocks for Mechanical Test
- (a)
- Weighing: Measure 864 g of gypsum, 605 g of water, 1.04 g of retarder, and PVAs with dosages ranging from 0% to 1.5%.
- (b)
- Mixing and stirring: Pour the aforementioned materials into the blender and stir until a homogeneous mixture is achieved.
- (c)
- Brush oil onto the inner surfaces of the molds.
- (d)
- Pour the mixture into the molds, allowing the blocks to take shape over the course of a day.
- (e)
- Demold the blocks and cure them in a curing box for a duration of 3 days.
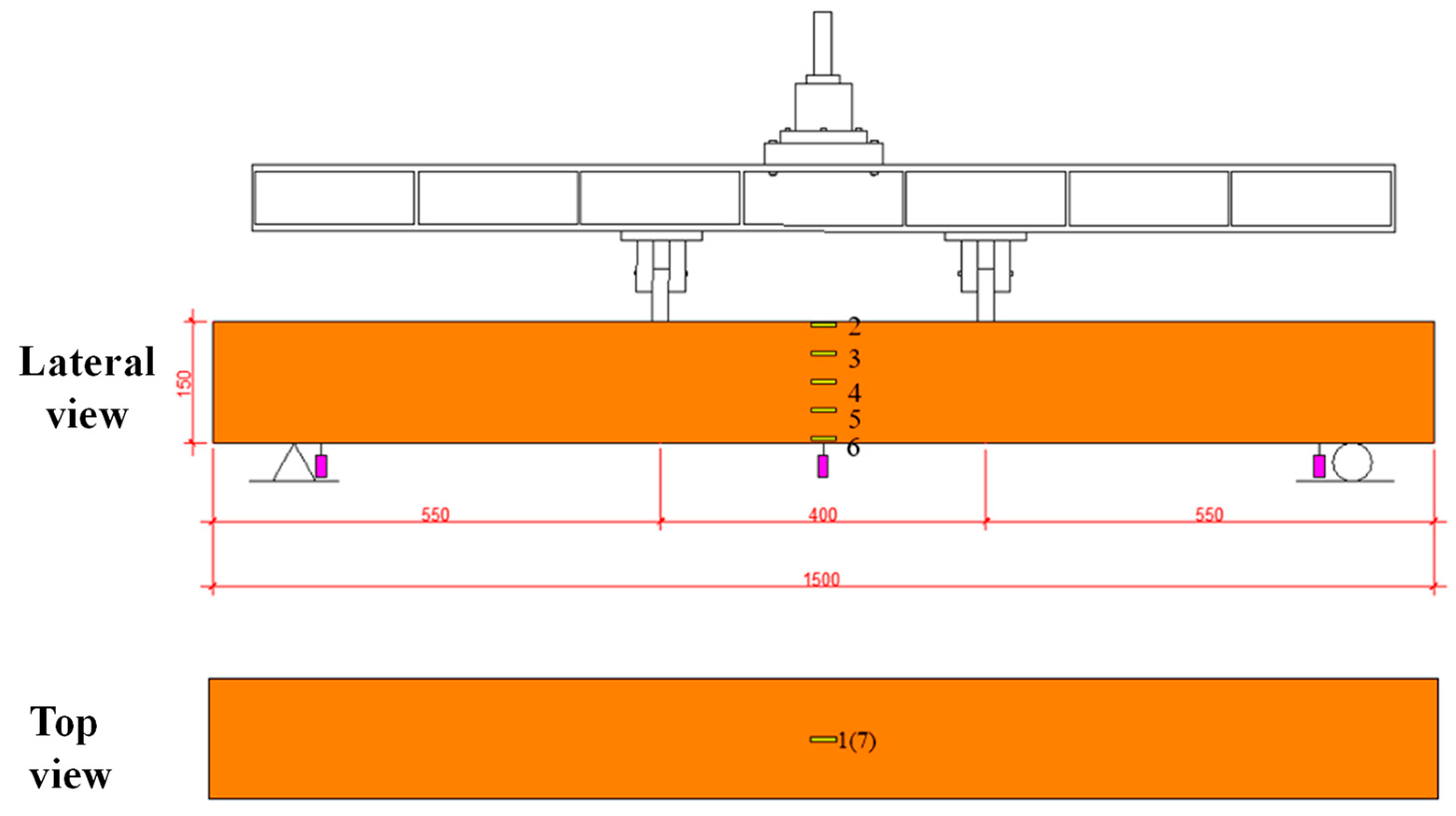
2.7. Four-Point Bending Tests
- (a)
- Prepare square GFRP beams filled with gypsum-based composites and affix the strain gauges using silicone rubber.
- (b)
- Install the GFRP beams in the vertical loading reaction system device, adjusting the position of supports and their height.
- (c)
- Connect the static resistance strain gauges with wires to form a quarter bridge and install displacement indicators.
- (d)
- Preload the specimen with 2.5 kN to ensure full contact between the loading device and the test beam, then unload it back to zero.
- (e)
- Apply a continuous load on the specimen with loading steps of 2.5 kN until failure occurs. Record load levels, strain, and displacement values throughout the entire loading process.
3. Theoretical Study
4. Results and Discussion
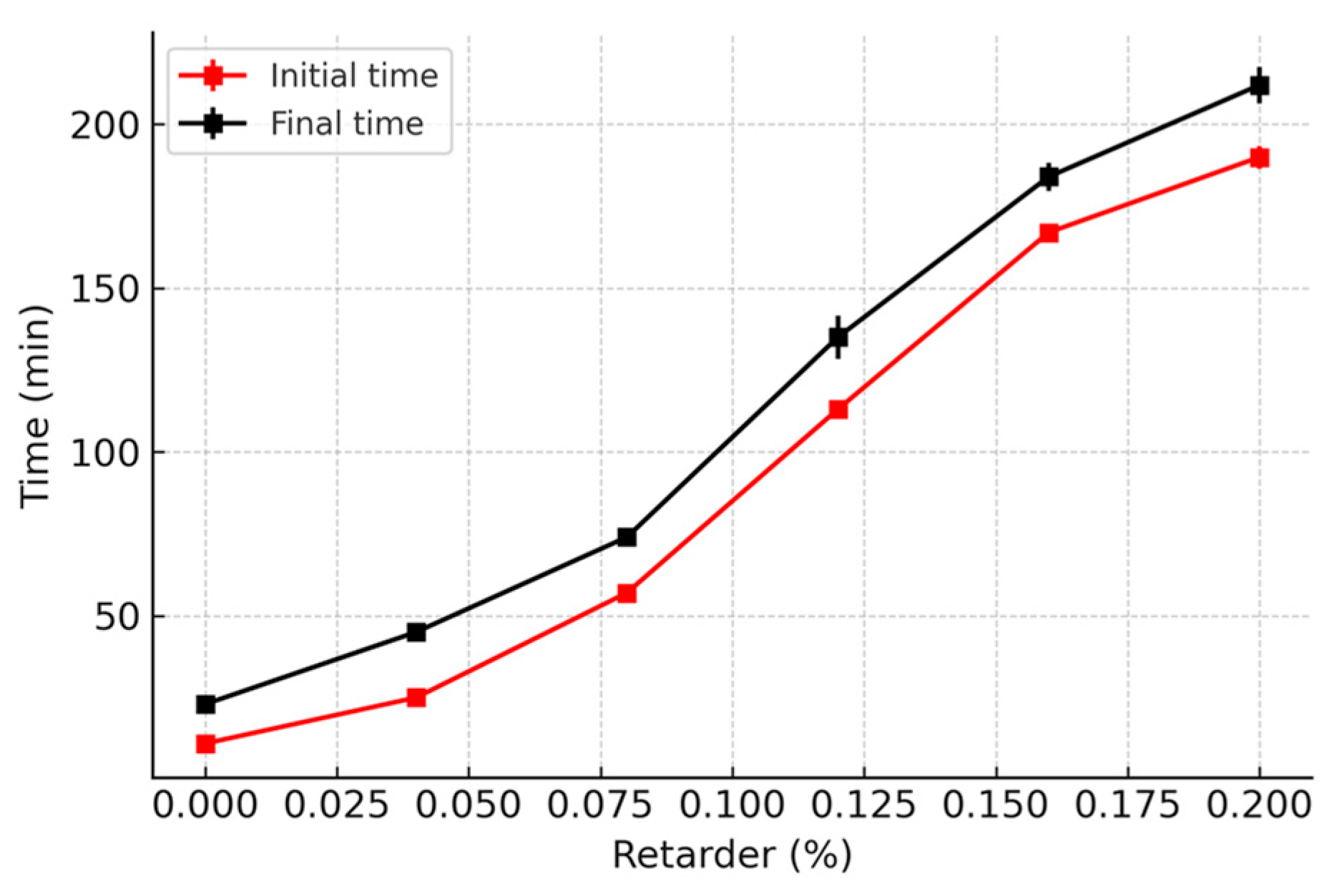
4.1. Coagulation Test Results
4.2. Results of the Flexural and Compressive Strength Tests on Gypsum Blocks
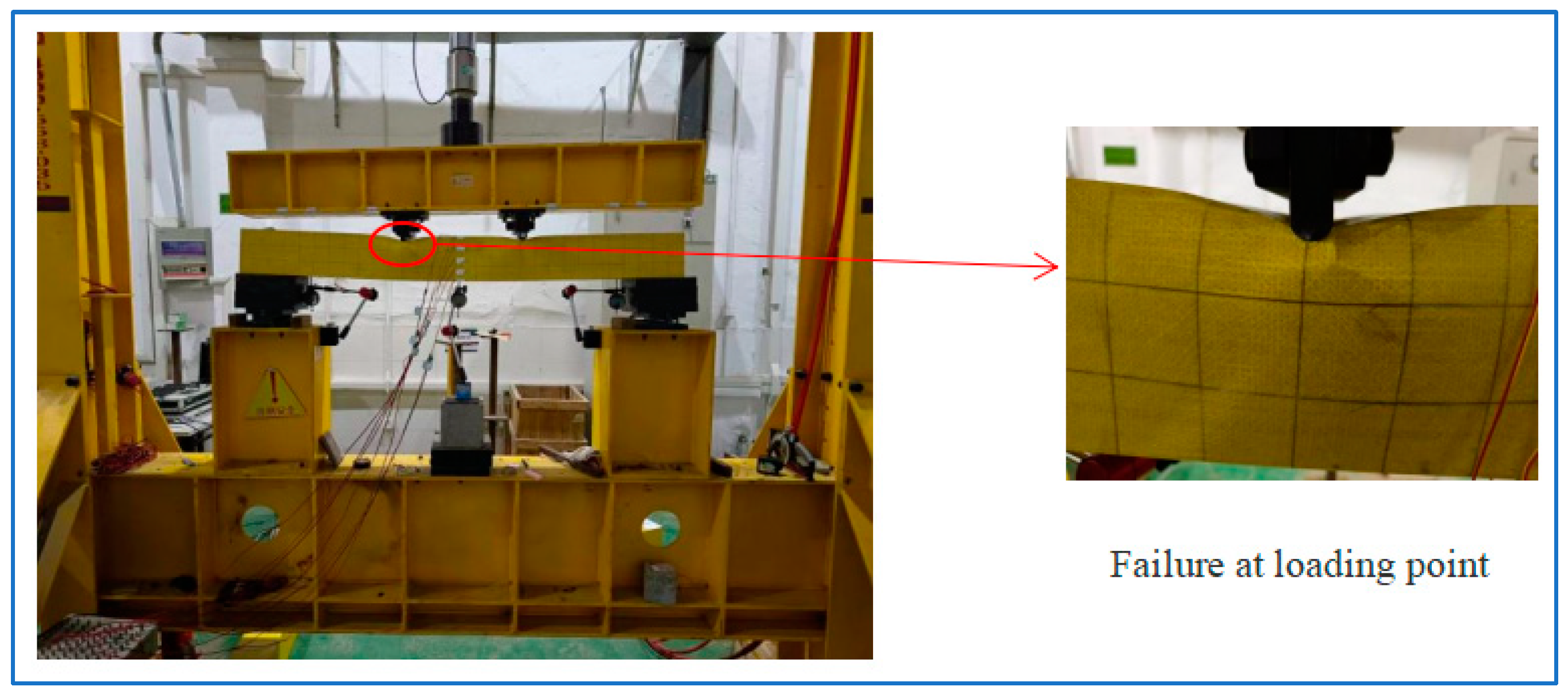
4.3. Results of the Four-Point Bending Tests
4.3.1. Ultimate Limit State Analysis
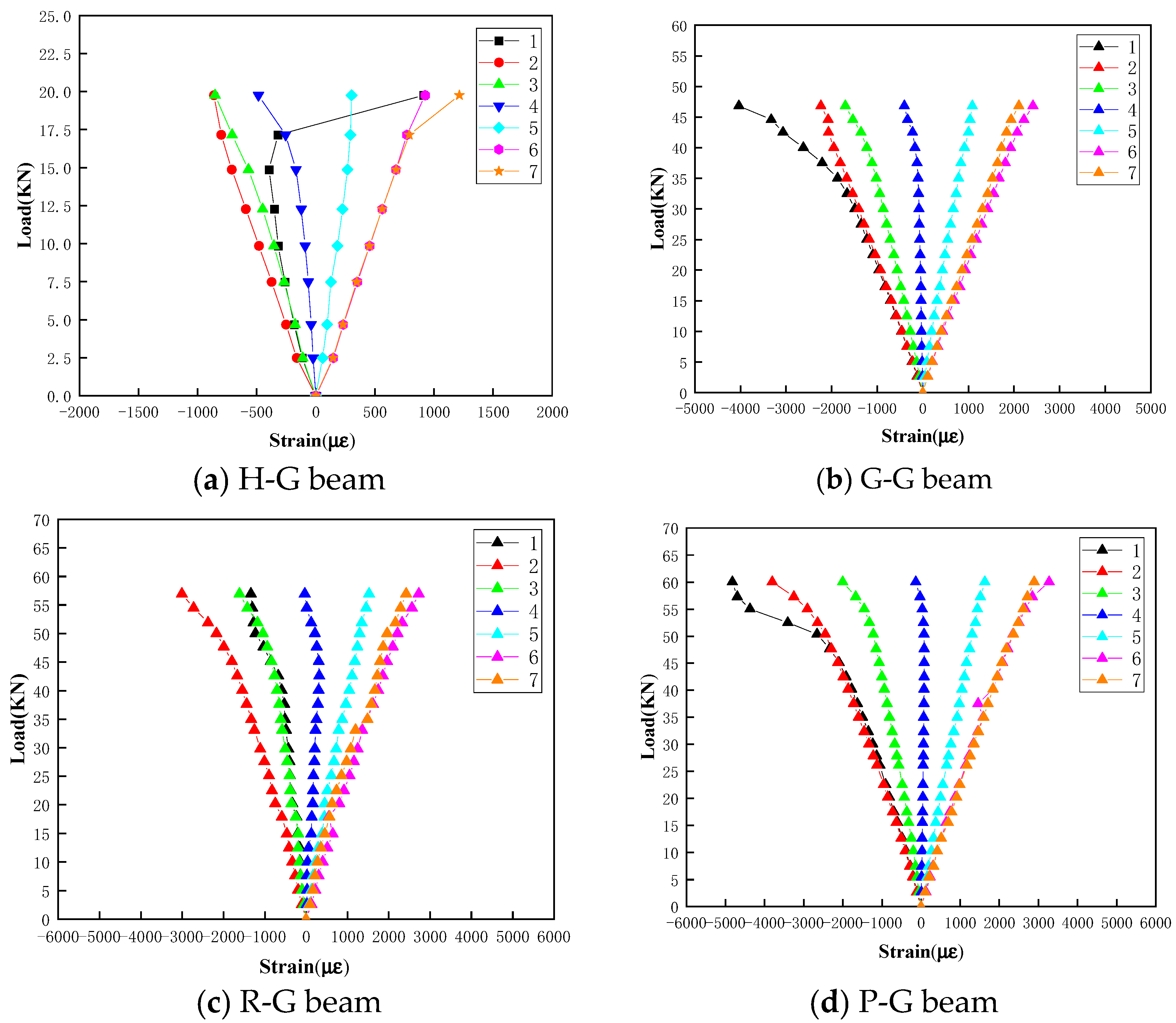
4.3.2. Strain Distribution Analysis
4.4. Discussion
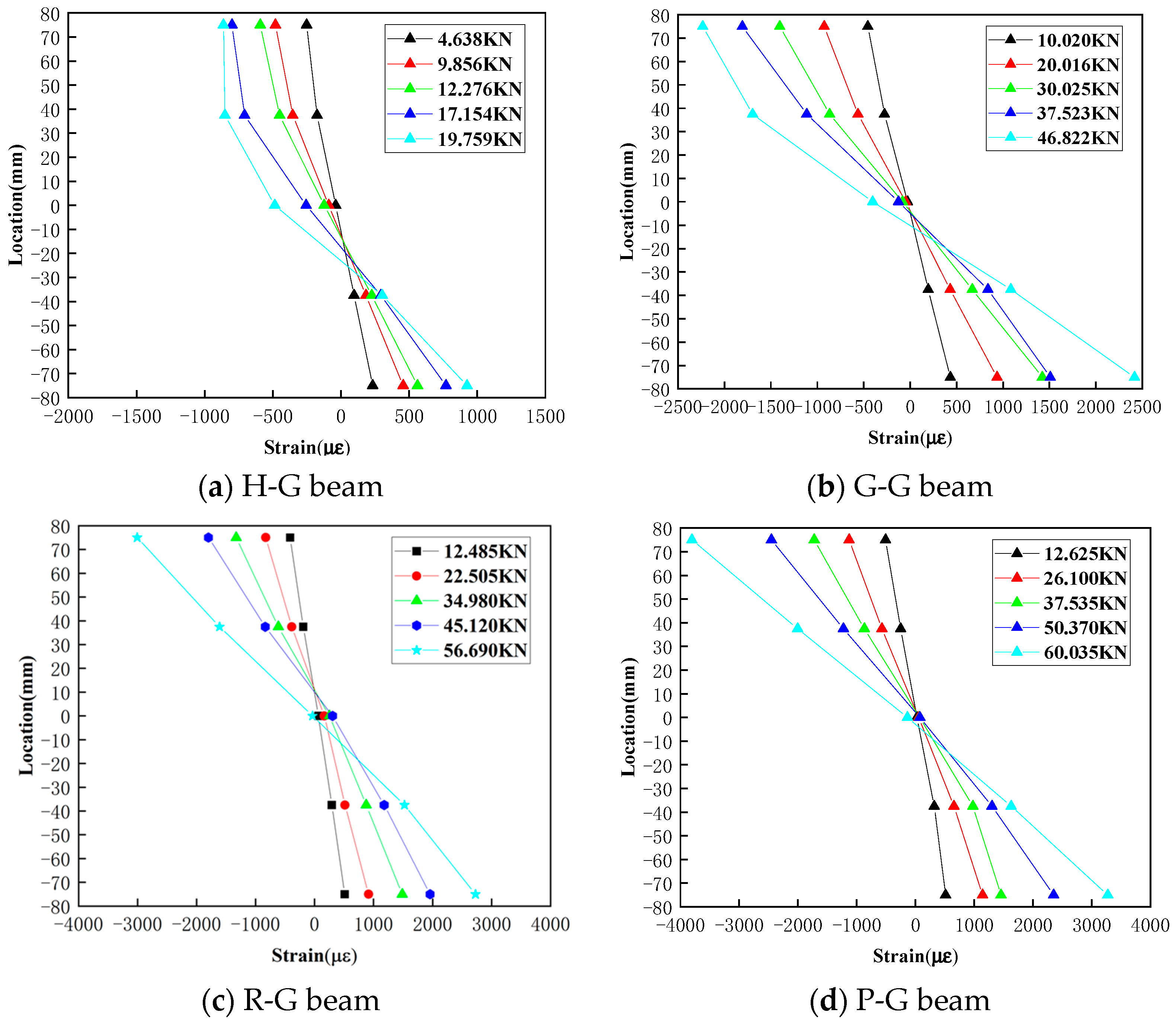
4.4.1. Height-Wise Strain Distribution Analysis
4.4.2. Theoretical Analysis
5. Conclusions
- Protein retarder sensitivity: The setting time of gypsum was found to be sensitive to protein retarder. An optimal dosage of 0.12% protein retarder effectively prolongs the initial and final setting time from 5 min and 10 min to 113 min and 135 min, respectively, making it suitable for specimen preparation.
- PVA composite gypsum strength: The flexural and compressive test results of gypsum blocks indicate that as the PVA content increases from 0% to 1.2%, the strength of PVA gypsum continuously increases. However, a downward trend is observed as the PVA dosage changes from 1.2% to 1.5%. The optimal dosage of PVA is determined to be 1.2%, enhancing the flexural and compressive strength of PVA composite gypsum from 1.17 MPa and 2.50 MPa to 2.97 MPa and 3.4 MPa, respectively.
- Ultimate load values of GRFP square beams: In the four-point bending tests, the ultimate load values of pure gypsum, reinforced gypsum, and PVA gypsum infilled GFRP square beams are 46.822 kN, 56.690 kN, and 60.035 kN, respectively. Compared with the ultimate load value of hollow GFRP square beams (19.759 kN), the ultimate bending capacity of GFRP square beams infilled with gypsum, reinforced gypsum, and PVA gypsum increases by 136.97%, 186.91%, and 203.84%, respectively. Among them, PVA gypsum GFRP square beams exhibit the best mechanical performance.
- Strain distribution and theoretical equations: The strain distribution along the height at the middle span section generally meets the plane section assumption under different load levels. Theoretical equations are established to calculate the ultimate load values, whose results align well with experimental values.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Alnahhal, W.; Aref, A. Structural performance of hybrid fiber reinforced polymer–concrete bridge superstructure systems. Compos. Struct. 2008, 84, 319–336. [Google Scholar] [CrossRef]
- Chakrabortty, A.; Khennane, A.; Kayali, O.; Morozov, E. Performance of outside filament-wound hybrid FRP-concrete beams. Compos. Part B Eng. 2011, 42, 907–915. [Google Scholar] [CrossRef]
- Hadi, M.N.; Wang, W.; Sheikh, M.N. Axial compressive behaviour of GFRP beams reinforced concrete columns. Constr. Build. Mater. 2015, 81, 198–207. [Google Scholar] [CrossRef]
- Keller, T.; Schollmayer, M. Plate bending behavior of a pultruded GFRP bridge deck system. Compos. Struct. 2004, 64, 285–295. [Google Scholar] [CrossRef]
- Evernden, M.C.; Mottram, J.T. A case for houses to be constructed of fibre reinforced polymer components. Proc. Inst. Civ. Eng.-Constr. Mater. 2012, 165, 3–13. [Google Scholar] [CrossRef]
- Yanes-Armas, S.; Keller, T. Structural concept and design of a GFRP-polyurethane sandwich roof structure. In Proceedings of the Eighth International Conference on Fibre-Reinforced Polymer (FRP) Composites in Civil Engineering, Hong Kong, China, 14–16 December 2016. [Google Scholar]
- Wang, J.; Song, Y.; Wang, W.; Tu, L. Evaluation of composite crashworthy device for pier protection against barge impact. Ocean Eng. 2018, 169, 144–158. [Google Scholar] [CrossRef]
- Alagusundaramoorthy, P.; Reddy, R.V.S. Testing and evaluation of GFRP composite deck panels. Ocean. Eng. 2008, 35, 287–293. [Google Scholar] [CrossRef]
- Abdolpour, H.; Garzón-Roca, J.; Escusa, G.; Sena-Cruz, J.M.; Barros, J.A.; Valente, I.B. Development of a composite prototype with GFRP profiles and sandwich panels used as a floor module of an emergency house. Compos. Struct. 2016, 153, 81–95. [Google Scholar] [CrossRef]
- Ndukwe, C.O.; Ezurike, B.O.; Okpala, P.C. Comparative studies of experimental and numerical evaluation of tensile properties of Glass Fibre Reinforced Polyester (GFRP) matrix. Heliyon 2021, 7, e06887. [Google Scholar] [CrossRef]
- Otoom, O.F.; Lokuge, W.; Karunasena, W.; Manalo, A.C.; Ozbakkaloglu, T.; Thambiratnam, D. Experimental and numerical evaluation of the compression behaviour of GFRP-wrapped infill materials. Case Stud. Constr. Mater. 2021, 15, e00654. [Google Scholar] [CrossRef]
- Shekarchi, M.; Yekrangnia, M.; Biniaz, A.; Raftery, G.M. Effect of elevated temperatures on the compressive behavior of timber filled steel and pultruded GFRP Beams. Compos. Struct. 2021, 271, 114135. [Google Scholar] [CrossRef]
- Al-Saadi, A.U.; Aravinthan, T.; Lokuge, W. Structural applications of fibre reinforced polymer (FRP) composite tubes: A review of columns members. Compos. Struct. 2018, 204, 513–524. [Google Scholar] [CrossRef]
- Zhang, F.; Liu, W.; Fang, H.; Xie, G. Experimental study on flexural behavior of GFRP panel-cold-formed thin-walled steel composite beams. J. Build. Struct. 2018, 39, 104–111. [Google Scholar]
- Robinson, M.J.; Melby, I.H. Effects of bonding in short-span rectangular concrete filled GFRP Beams. Compos. Struct. 2015, 133, 131–139. [Google Scholar] [CrossRef]
- Abouzied, A.; Masmoudi, R. Flexural behavior of rectangular FRP-Beams filled with reinforced concrete: Experimental and theoretical studies. Eng. Struct. 2017, 133, 59–73. [Google Scholar] [CrossRef]
- Ahmed, A.A.; Hassan, M.; Masmoudi, R. Effect of concrete strength and beams thickness on the flexural behavior of prestressed rectangular concrete-filled FRP Beams beams. Eng. Struct. 2020, 205, 110112. [Google Scholar] [CrossRef]
- Muttashar, M.; Manalo, A.; Karunasena, W.; Lokuge, W. Influence of infill concrete strength on the flexural behaviour of pultruded GFRP square beams. Compos. Struct. 2016, 145, 58–67. [Google Scholar] [CrossRef]
- Huang, P.; Yu, H.; Feng, J.; Hao, G.; Li, Z. Experimental study on flexural behavior of GFRP profile-wood composite beam. J. Hebei Univ. Sci. Technol. 2022, 43, 560–570. [Google Scholar]
- Yoganantham, C.; Joanna, P. Flexural behaviour of pultruded GFRP beams infilled with HVFA ECC. Mater. Today Proc. 2021, 45, 5978–5981. [Google Scholar] [CrossRef]
- Zhang, F.; Lu, Z.; Wang, D.; Fang, H. Mechanical properties of the composite sandwich structures with cold formed profiled steel plate and balsa wood core. Eng. Struct. 2024, 300, 117256. [Google Scholar] [CrossRef]
- Palomo, I.R.I.; Frappa, G.; de Almeida, L.C.; Trautwein, L.M.; Pauletta, M. Analytical and numerical models to determine the strength of RC exterior beam–column joints retrofitted with UHPFRC. Eng. Struct. 2024, 312, 118244. [Google Scholar] [CrossRef]
- Alyousef, R.; Abbass, W.; Aslam, F.; Shah, M.I. Potential of waste woven polypropylene fiber and textile mesh for production of gypsum-based composite. Case Stud. Constr. Mater. 2023, 18, e02099. [Google Scholar] [CrossRef]
- Balti, S.; Boudenne, A.; Dammak, L.; Hamdi, N. Mechanical and thermophysical characterization of gypsum composites reinforced by different wastes for green building applications. Constr. Build. Mater. 2023, 372, 130840. [Google Scholar] [CrossRef]
- Chen, C.; Fang, L.; Wang, Y.; Jiu, S.; Chen, Y. Mechanical, thermal and microscopic properties of gypsum matrix composites containing capric acid-palmitic acid/urea-formaldehyde microcapsules. Case Stud. Constr. Mater. 2023, 18, e02084. [Google Scholar] [CrossRef]
- Ding, X.; Huang, W.; Li, Y.; Hu, Z.; Shan, Z. Study on retarding feature and retardation mechanism of various retarding materials on gypsum as a construction material: A review. J. Build. Eng. 2023, 72, 106569. [Google Scholar] [CrossRef]
- Wu, C.; He, J.; Wang, K.; Yang, L.; Wang, F. Enhance the mechanical and water resistance performance of flue gas desulfurization gypsum by quaternary phase. Constr. Build. Mater. 2023, 387, 131565. [Google Scholar] [CrossRef]
- Babu, K.S.; Ratnam, C. Mechanical and thermophysical behavior of hemp fiber reinforced gypsum composites. Mater. Today Proc. 2021, 44, 2245–2249. [Google Scholar] [CrossRef]
- Alcaraz, J.S.; Belda, I.M.; Sanchis, E.J.; Borrell, J.M.G. Mechanical properties of plaster reinforced with yute fabrics. Compos. Part B Eng. 2019, 178, 107390. [Google Scholar] [CrossRef]
- Gou, M.; Zhao, M.; Zhou, L.; Zhao, J.; Hou, W.; Ma, W.; Hou, Z. Hydration and mechanical properties of FGD gypsum-cement-mineral powder composites. J. Build. Eng. 2023, 69, 106288. [Google Scholar] [CrossRef]
- Ma, X.; Tan, L.; Lu, Y.; Yao, W.; Wei, Y. Upcycling of waste plasterboard for the synthesis of high-quality gypsum-based 3D printing powder. Constr. Build. Mater. 2023, 373, 130846. [Google Scholar] [CrossRef]
- Zhu, C.; Zhang, J.; Peng, J.; Cao, W.; Liu, J. Physical and mechanical properties of gypsum-based composites reinforced with PVA and PP fibers. Constr. Build. Mater. 2018, 163, 695–705. [Google Scholar] [CrossRef]
- Kuqo, A.; Mai, C. Mechanical properties of lightweight gypsum composites comprised of seagrass Posidonia oceanica and pine (Pinus sylvestris) wood fibers. Constr. Build. Mater. 2021, 282, 122714. [Google Scholar] [CrossRef]
- Li, Z.; Wang, X.; Yan, W.; Ding, L.; Liu, J.; Wu, Z.; Huang, H. Physical and mechanical properties of gypsum-based composites reinforced with basalt, glass, and PVA fibers. J. Build. Eng. 2023, 64, 105640. [Google Scholar] [CrossRef]
- Elkin, A.; Konev, S.; Safonov, A.; Gusev, S.; Sergeichev, I. Compressive residual strength of the pultruded glass-fiber composite after tension-compression fatigue. Compos. Part C Open Access 2024, 14, 100456. [Google Scholar] [CrossRef]
- Guo, R.; Xian, G.; Li, F.; Li, C.; Hong, B. Hygrothermal resistance of pultruded carbon, glass and carbon/glass hybrid fiber reinforced epoxy composites. Constr. Build. Mater. 2021, 315, 125710. [Google Scholar] [CrossRef]
- Shaowei, L.U.; Xie, H.; Chen, P. Simulation and optimization of GFRP pultrusion process. J. Compos. Mater. 2008, 1, 46–51. [Google Scholar]
- ASTM D5379-19; Standard Test Method for Shear Properties of Composite Materials by the V-Notched Beam Method. ASTM International: West Conshohocken, PA, USA, 2019.
- ASTM D3039-07; Standard Test Method for Tensile Properties of Polymer Matrix Composite Materials. ASTM International: West Conshohocken, PA, USA, 2007.
- ASTM D3410-16; Standard Test Method for Compressive Properties of Polymer Matrix Composite Materials with Unsupported Gage Section by Shear Loading. ASTM International: West Conshohocken, PA, USA, 2016.
- GB/T 1499.2-2018; Steel for Reinforced Concrete Part 2, Hot Rolled Ribbed Bars. China Iron and Steel Industry Association: Beijing, China, 2018.
- GB 50081; Standard for Test Methods of Physical and Mechanical Properties of Concrete. China Academy of Building Research Co., Ltd.: Beijing, China, 2019.
- GB/T 1449-2005; Standard for Test Methods of Flexural Properties of Fiber-Reinforced Plastics. China Building Materials Industry Association: Beijing, China, 2005.
- Lejia, L. Theoretical Bifurcation and Numerical Simulation of Flexural Behavior of FRP-Concrete Composite Beams. Master’s Thesis, Hunan University, Changsha, China, 2014. [Google Scholar]
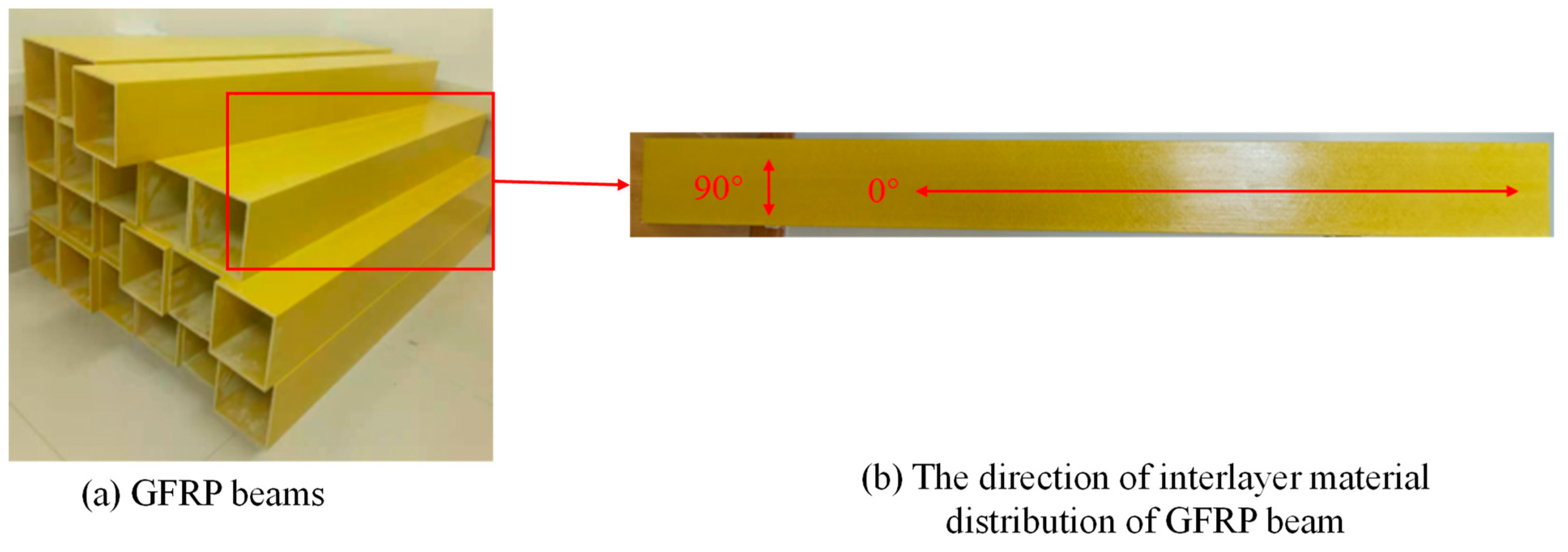
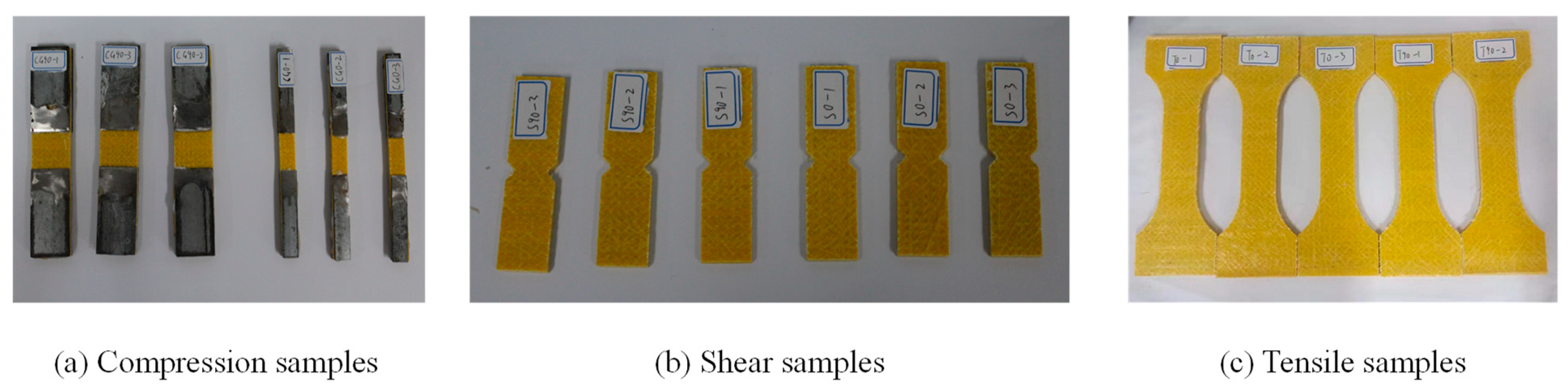





| Direction | Tensile Strength/MPa | Modulus of Elasticity/GPa | Compressive Strength/MPa | Modulus of Elasticity/GPa | Shear Strength/MPa | Modulus of Elasticity/GPa |
|---|---|---|---|---|---|---|
| 0° | 321.7 | 22.00 | 266.7 | 21.28 | 71.7 | 9.87 |
| 90° | 28.8 | 2.65 | 57.8 | 4.45 | 52.4 | 9.76 |
| Material | Water Requirement for Normal Consistency | Setting Times | Powdery Degree/200 Mesh | Whiteness/% | |
|---|---|---|---|---|---|
| Initial | Final | ||||
| Desulfurized gypsum | 70% | 5 min | 10 min | 0.01 | 50 |
| Name | Diameter/ μm | Length/mm | Density/ g/cm3 | Tensile Strength/MPa | Modulus of Elasticity/GPa | Elongation at Break |
|---|---|---|---|---|---|---|
| PVA | 15.09 | 12 | 1.29 | 1830 | 40 | 6.9% |
| Grade | Modulus of Elasticity/MPa | Poisson’s Ratio | Yield Stress/MPa |
|---|---|---|---|
| HRB400 steel bars | 200,000 | 0.3 | 400 |
| Types | Length/mm | Width/mm | Height/mm | End Face Style |
|---|---|---|---|---|
| K-G beam | 1500 | 150 | 150 |  |
| S-G beam | 1500 | 150 | 150 |  |
| G-G beam | 1500 | 150 | 150 |  |
| P-G beam | 1500 | 150 | 150 |  |
| Sample | Ultimate Load/kN | Average Value/kN | Mid-Span Deflection/mm | Average Value/mm |
|---|---|---|---|---|
| H-G-1 | 19.537 | 19.759 | 3.51 | 3.610 |
| H-G-2 | 19.980 | 3.71 | ||
| G-G-1 | 45.130 | 46.822 | 7.62 | 7.928 |
| G-G-2 | 48.514 | 8.235 | ||
| R-G-1 | 53.880 | 56.690 | 8.635 | 8.513 |
| R-G-2 | 59.500 | 8.39 | ||
| P-G-1 | 60.070 | 60.035 | 9.495 | 10.013 |
| P-G-2 | 60.000 | 10.53 |
| Type | /mm | Experimental Ultimate Load /kN | Theoretical Ultimate Load /kN | Relative Error |
|---|---|---|---|---|
| H-G beam | 3.610 | 19.759 | 19.983 | 1.12% |
| G-G beam | 7.928 | 46.822 | 50.428 | 7.15% |
| R-G beam | 8.513 | 56.690 | 53.935 | 5.11% |
| P-G beam | 10.013 | 60.035 | 65.027 | 7.68% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Su, B.; Zhang, T. Flexural Behavior of Innovative Glass Fiber-Reinforced Composite Beams Reinforced with Gypsum-Based Composites. Polymers 2024, 16, 3327. https://doi.org/10.3390/polym16233327
Liu Y, Su B, Zhang T. Flexural Behavior of Innovative Glass Fiber-Reinforced Composite Beams Reinforced with Gypsum-Based Composites. Polymers. 2024; 16(23):3327. https://doi.org/10.3390/polym16233327
Chicago/Turabian StyleLiu, Yiwen, Bo Su, and Tianyu Zhang. 2024. "Flexural Behavior of Innovative Glass Fiber-Reinforced Composite Beams Reinforced with Gypsum-Based Composites" Polymers 16, no. 23: 3327. https://doi.org/10.3390/polym16233327
APA StyleLiu, Y., Su, B., & Zhang, T. (2024). Flexural Behavior of Innovative Glass Fiber-Reinforced Composite Beams Reinforced with Gypsum-Based Composites. Polymers, 16(23), 3327. https://doi.org/10.3390/polym16233327





