Scaling Relationships of the Structural and Rheological Behavior of Tadpole Polymer Chains in Dilute Solution Systems Using Brownian Dynamics Simulations
Abstract
1. Introduction
2. Materials and Methods
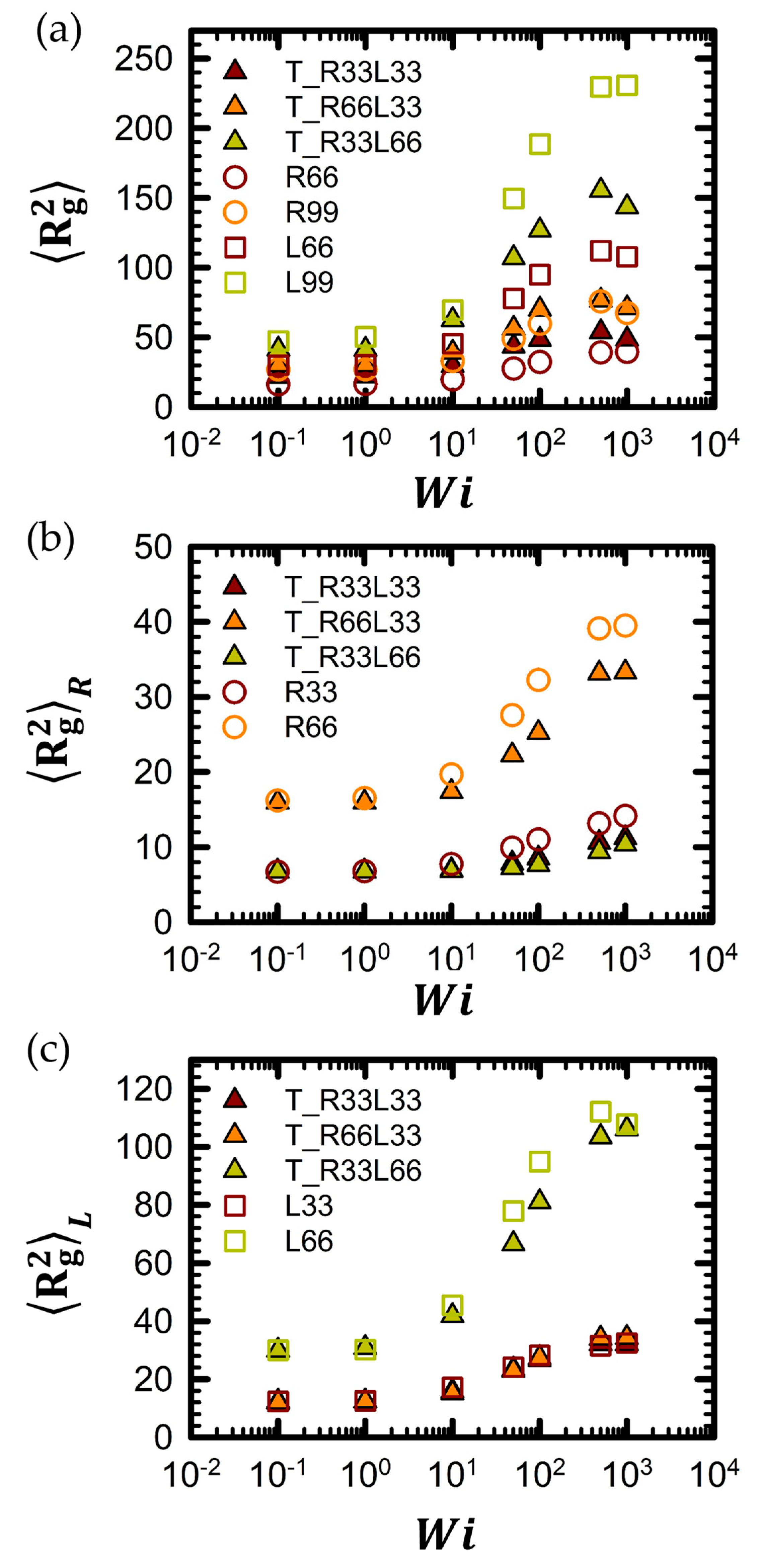
3. Results and Discussion
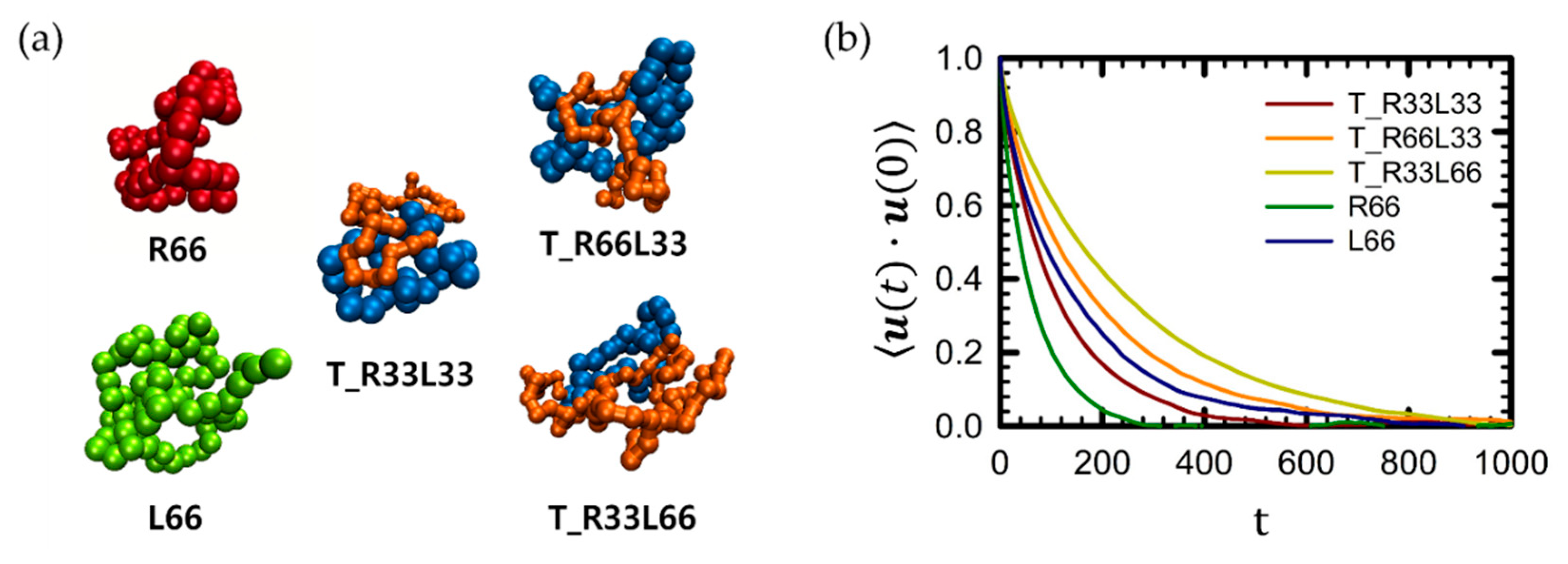
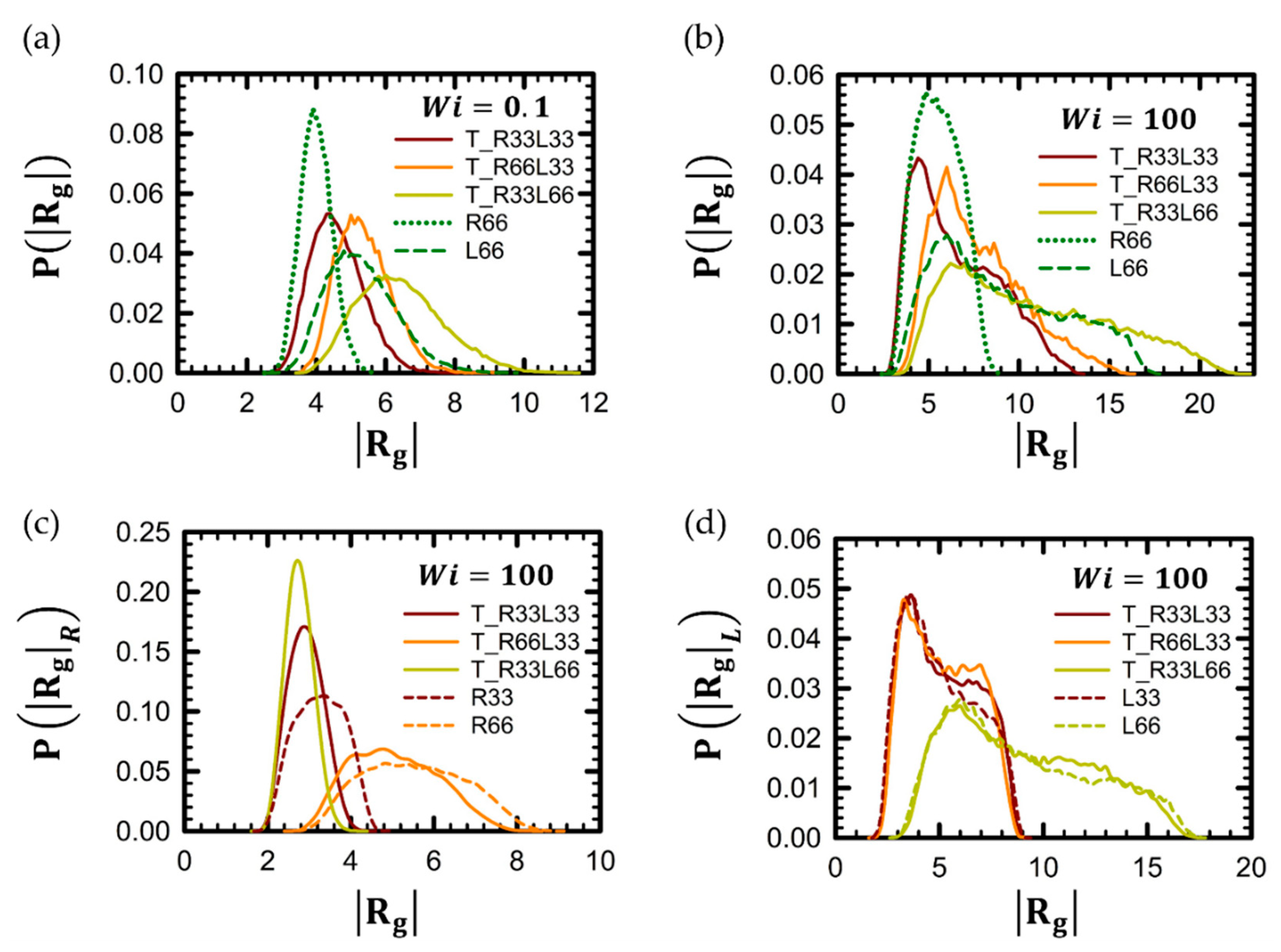
3.1. Equilibrium
3.2. Shear Flow
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Macosko, C.W. Rheology: Principles, Measurements, and Applications; VCH Publisher: New York, NY, USA, 1994. [Google Scholar]
- Morrison, F.A. Understanding Rheology; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Bird, R.B.; Armstrong, R.C.; Hassager, O. Dynamics of Polymeric Liquids; John Wiley and Sons: New York, NY, USA, 1987; Volume 1. [Google Scholar]
- Doi, M.; Edward, S.F. The Theory of Polymer Dynamics; Oxford University Press: New York, NY, USA, 1986. [Google Scholar]
- De Gennes, P.G. Scaling Concepts in Polymer Physics; Cornell University Press: Ithaca, NY, USA, 1979. [Google Scholar]
- Rubinstein, M.; Colby, R.H. Polymer Physics; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Flory, P.J. Principles of Polymer Chemistry; Cornell University Press: Ithaca, NY, USA, 1953. [Google Scholar]
- Hild, G.; Kohler, A.; Rempp, P. Synthesis of Ring-shaped Macromolecules. Eur. Polym. J. 1980, 16, 525. [Google Scholar] [CrossRef]
- Hild, G.; Strazielle, C.; Rempp, P. Cyclic Macromolecules—Synthesis and Characterization of Ring-Shaped Polystyrenes. Eur. Polym. J. 1983, 19, 721–727. [Google Scholar] [CrossRef]
- Semlyen, J.A. Cyclic Polymers, 2nd ed.; Springer: Dordrecht, The Netherlands, 2002. [Google Scholar]
- Roovers, J.; Toporowski, P.M. Synthesis of High Molecular-Weight Ring Polystyrenes. Macromolecules 1983, 16, 843–849. [Google Scholar] [CrossRef]
- Roovers, J.; Toporowski, P.M. Synthesis and characterization of ring polybutadienes. J. Polym. Sci. B 1988, 26, 1251–1259. [Google Scholar] [CrossRef]
- Kruteva, M.; Allgaier, J.; Richter, D. Topology Matters: Conformation and Microscopic Dynamics of Ring Polymers. Macromolecules 2023, 56, 7203–7229. [Google Scholar] [CrossRef]
- Micheletti, C.; Chubak, I.; Orlandini, E.; Smrek, J. Topology-Based Detection and Tracking of Deadlocks Reveal Aging of Active Ring Melts. ACS Macro Lett. 2024, 13, 124–129. [Google Scholar] [CrossRef]
- Ha, T.Y.; Jeong, S.H.; Baig, C. Interfacial Polymer Rheology of Entangled Short-Chain Branched Ring Melts in Shear Flow. Macromolecules 2024, 57, 6177–6189. [Google Scholar] [CrossRef]
- Peponaki, K.; Tsalikis, D.G.; Patelis, N.; Sakellariou, G.; Chang, T.; Vlassopoulos, D. Revisiting the Viscosity of Moderately Entangled Ring Polymer Melts. Macromolecules 2024, 57, 7263–7269. [Google Scholar] [CrossRef]
- Ubertini, M.A.; Rosa, A. Topological Analysis and Recovery of Entanglements in Polymer Melts. Macromolecules 2023, 56, 3354–3362. [Google Scholar] [CrossRef]
- Dehaghani, Z.A.; Chiarantoni, P.; Micheletti, C. Topological Entanglement of Linear Catenanes: Knots and Threadings. ACS Macro Lett. 2023, 12, 1231–1236. [Google Scholar] [CrossRef]
- Kruteva, M.; Monkenbusch, M.; Allgaier, J.; Pyckhout-Hintzen, W.; Porcar, L.; Richter, D. Structure of Polymer Rings in Linear Matrices: SANS Investigation. Macromolecules 2023, 56, 4835–4844. [Google Scholar] [CrossRef]
- Doi, Y. A Review of Rheology for Ring Polymers and Polymers with Internal Loops: Comparison of Recent Experimental and Theoretical Studies. Korea-Aust. Rheol. J. 2024, 4, 1–12. [Google Scholar] [CrossRef]
- Vigil, D.L.; Ge, T.; Rubinstein, M.; O’Connor, T.C.; Grest, G.S. Measuring Topological Constraint Relaxation in Ring-Linear Polymer Blends. Phys. Rev. Lett. 2024, 133, 118101. [Google Scholar] [CrossRef] [PubMed]
- Staňo, R.; Likos, C.N.; Smrek, J. To Thread or Not to Thread? Effective Potentials and Threading Interactions between Asymmetric Ring Polymers. Soft Matter 2022, 19, 17–30. [Google Scholar] [CrossRef] [PubMed]
- Laurent, B.A.; Grayson, S.M. Synthetic Approaches for the Preparation of Cyclic Polymers. Chem. Soc. Rev. 2009, 38, 2202–2213. [Google Scholar] [CrossRef]
- Wang, T.W.; Golder, M.R. Advancing Macromolecular Hoop Construction: Recent Developments in Synthetic Cyclic Polymer Chemistry. Polym. Chem. 2021, 12, 958–969. [Google Scholar] [CrossRef]
- Haque, F.M.; Grayson, S.M. The Synthesis, Properties and Potential Applications of Cyclic Polymers. Nat. Chem. 2020, 12, 433–444. [Google Scholar] [CrossRef]
- Yamamoto, T. Synthesis of Cyclic Polymers and Topology Effects on Their Diffusion and Thermal Properties. Polym. J. 2013, 45, 711–717. [Google Scholar] [CrossRef]
- Ogawa, T.; Nakazono, K.; Aoki, D.; Uchida, S.; Takata, T. Effective Approach to Cyclic Polymer from Linear Polymer: Synthesis and Transformation of Macromolecular [1]Rotaxane. ACS Macro Lett. 2015, 4, 343–347. [Google Scholar] [CrossRef]
- Halverson, J.D.; Lee, W.B.; Grest, G.S.; Grosberg, A.Y.; Kremer, K. Molecular Dynamics Simulation Study of Nonconcatenated Ring Polymers in a Melt. I. Statics. J. Chem. Phys. 2011, 134, 204904. [Google Scholar] [CrossRef]
- Tsamopoulos, A.J.; Katsarou, A.F.; Tsalikis, D.G.; Mavrantzas, V.G. Shear Rheology of Unentangled and Marginally Entangled Ring Polymer Melts from Large-Scale Nonequilibrium Molecular Dynamics Simulations. Polymers 2019, 11, 1194. [Google Scholar] [CrossRef] [PubMed]
- Hsiao, K.W.; Schroeder, C.M.; Sing, C.E. Ring Polymer Dynamics Are Governed by a Coupling between Architecture and Hydrodynamic Interactions. Macromolecules 2016, 49, 1961–1971. [Google Scholar] [CrossRef]
- Young, C.D.; Qian, J.R.; Marvin, M.; Sing, C.E. Ring Polymer Dynamics and Tumbling-Stretch Transitions in Planar Mixed Flows. Phys. Rev. E 2019, 99, 062502. [Google Scholar] [CrossRef] [PubMed]
- Tsolou, G.; Stratikis, N.; Baig, C.; Stephanou, P.S.; Mavrantzas, V.G. Melt Structure and Dynamics of Unentangled Polyethylene Rings: Rouse Theory, Atomistic Molecular Dynamics Simulation, and Comparison with the Linear Analogues. Macromolecules 2010, 43, 10692–10713. [Google Scholar] [CrossRef]
- Wiest, J.M.; Burdette, S.R.; Liu, T.W.; Bird, R.B. Effect of Ring Closure on Rheological Behavior. J. Non-Newton. Fluid Mech. 1987, 24, 279–295. [Google Scholar] [CrossRef]
- Watanabe, H.; Inoue, T.; Matsumiya, Y. Transient Conformational Change of Bead-Spring Ring Chain during Creep Process. Macromolecules 2006, 39, 5419–5426. [Google Scholar] [CrossRef]
- Vettorel, T.; Grosberg, A.Y.; Kremer, K. Statistics of Polymer Rings in the Melt: A Numerical Simulation Study. Phys. Biol. 2009, 6, 062502. [Google Scholar] [CrossRef]
- Arrighi, V.; Gagliardi, S.; Dagger, A.C.; Semlyen, J.A.; Higgins, J.S.; Shenton, M.J. Conformation of Cyclics and Linear Chain Polymers in Bulk by SANS. Macromolecules 2004, 37, 8057–8065. [Google Scholar] [CrossRef]
- Sakaue, T. Statistics and Geometrical Picture of Ring Polymer Melts and Solutions. Phys. Rev. E 2012, 85, 021806. [Google Scholar] [CrossRef]
- Brown, S.; Lenczycki, T.; Szamel, G. Influence of Topological Constraints on the Statics and Dynamics of Ring Polymers. Phys. Rev. E 2001, 63, 528011–528014. [Google Scholar] [CrossRef]
- Pasquino, R.; Vasilakopoulos, T.C.; Jeong, Y.C.; Lee, H.; Rogers, S.; Sakellariou, G.; Allgaier, J.; Takano, A.; Brás, A.R.; Chang, T.; et al. Viscosity of Ring Polymer Melts. ACS Macro Lett. 2013, 2, 874–878. [Google Scholar] [CrossRef] [PubMed]
- Huang, Q.; Ahn, J.; Parisi, D.; Chang, T.; Hassager, O.; Panyukov, S.; Rubinstein, M.; Vlassopoulos, D. Unexpected Stretching of Entangled Ring Macromolecules. Phys. Rev. Lett. 2019, 122, 208001. [Google Scholar] [CrossRef] [PubMed]
- Liebetreu, M.; Likos, C.N. Hydrodynamic Inflation of Ring Polymers under Shear. Commun. Mater. 2020, 1, 4. [Google Scholar] [CrossRef]
- Milner, S.T.; Newhall, J.D. Stress Relaxation in Entangled Melts of Unlinked Ring Polymers. Phys. Rev. Lett. 2010, 105, 208302. [Google Scholar] [CrossRef] [PubMed]
- Kapnistos, M.; Lang, M.; Vlassopoulos, D.; Pyckhout-Hintzen, W.; Richter, D.; Cho, D.; Chang, T.; Rubinstein, M. Unexpected Power-Law Stress Relaxation of Entangled Ring Polymers. Nat. Mater. 2008, 7, 997–1002. [Google Scholar] [CrossRef] [PubMed]
- Rosa, A.; Everaers, R. Ring Polymers in the Melt State: The Physics of Crumpling. Phys. Rev. Lett. 2014, 112, 118302. [Google Scholar] [CrossRef]
- O’Connor, T.C.; Ge, T.; Rubinstein, M.; Grest, G.S. Topological Linking Drives Anomalous Thickening of Ring Polymers in Weak Extensional Flows. Phys. Rev. Lett. 2020, 124, 027801. [Google Scholar] [CrossRef]
- Chen, W.; Chen, J.; An, L. Tumbling and Tank-Treading Dynamics of Individual Ring Polymers in Shear Flow. Soft Matter 2013, 9, 4312–4318. [Google Scholar] [CrossRef]
- Jeong, S.H.; Cho, S.; Roh, E.J.; Ha, T.Y.; Kim, J.M.; Baig, C. Intrinsic Surface Characteristics and Dynamic Mechanisms of Ring Polymers in Solution and Melt under Shear Flow. Macromolecules 2020, 53, 10051–10060. [Google Scholar] [CrossRef]
- Yoon, J.; Kim, J.; Baig, C. Nonequilibrium Molecular Dynamics Study of Ring Polymer Melts under Shear and Elongation Flows: A Comparison with Their Linear Analogs. J. Rheol. 2016, 60, 673–685. [Google Scholar] [CrossRef]
- Roovers, J. Melt Properties of Ring Polystyrenes. Macromolecule 1984, 18, 1359–1361. [Google Scholar] [CrossRef]
- Tsalikis, D.G.; Mavrantzas, V.G.; Vlassopoulos, D. Analysis of Slow Modes in Ring Polymers: Threading of Rings Controls Long-Time Relaxation. ACS Macro Lett. 2016, 5, 755–760. [Google Scholar] [CrossRef] [PubMed]
- Obukhov, S.P.; Rubinstein, M.; Duke, T. Dynamics of a Ring Polymer in a Gel. Phys. Rev. Lett. 1994, 73, 1263. [Google Scholar] [CrossRef] [PubMed]
- Smrek, J.; Kremer, K.; Rosa, A. Threading of Unconcatenated Ring Polymers at High Concentrations: Double-Folded vs. Time-Equilibrated Structures. ACS Macro Lett. 2019, 8, 155–160. [Google Scholar] [CrossRef] [PubMed]
- Takano, A.; Kushida, Y.; Aoki, K.; Masuoka, K.; Hayashida, K.; Cho, D.; Kawaguchi, D.; Matsushita, Y. HPLC Characterization of Cyclization Reaction Product Obtained by End-to-End Ring Closure Reaction of a Telechelic Polystyrene. Macromolecules 2007, 40, 679–681. [Google Scholar] [CrossRef]
- Singla, S.; Zhao, T.; Beckham, H.W. Purification of Cyclic Polymers Prepared from Linear Precursors by Inclusion Complexation of Linear Byproducts with Cyclodextrins. Macromolecules 2003, 36, 6945–6948. [Google Scholar] [CrossRef]
- Noda, T.; Doi, Y.; Ohta, Y.; Takata, S.-I.; Takano, A.; Matsushita, Y. Preparation, Characterization, and Dilute Solution Properties of Four-Branched Cage-Shaped Poly(Ethylene Oxide). J. Polym. Sci. 2020, 58, 2098–2107. [Google Scholar] [CrossRef]
- Hövelmann, C.H.; Gooßen, S.; Allgaier, J. Scale-Up Procedure for the Efficient Synthesis of Highly Pure Cyclic Poly(Ethylene Glycol). Macromolecules 2017, 50, 4169–4179. [Google Scholar] [CrossRef]
- Sawayama, T.; Wang, Y.; Watanabe, T.; Takayanagi, M.; Yamamoto, T.; Hosono, N.; Uemura, T. Metal-Organic Frameworks for Practical Separation of Cyclic and Linear Polymers. Angew. Chem. Int. Ed. 2021, 60, 11830–11834. [Google Scholar] [CrossRef]
- Hadjichristidis, N.; Hirao, A.; Tezuka, Y.; Du Prez, F. Complex Macromolecular Architectures: Synthesis, Characterization, and Self-Assembly; John Wiley & Son: Hoboken, NJ, USA, 2011. [Google Scholar]
- Doi, Y.; Ohta, Y.; Nakamura, M.; Takano, A.; Takahashi, Y.; Matsushita, Y. Precise Synthesis and Characterization of Tadpole-Shaped Polystyrenes with High Purity. Macromolecules 2013, 46, 1075–1081. [Google Scholar] [CrossRef]
- Doi, Y.; Iwasa, Y.; Watanabe, K.; Nakamura, M.; Takano, A.; Takahashi, Y.; Matsushita, Y. Synthesis and Characterization of Comb-Shaped Ring Polystyrenes. Macromolecules 2016, 49, 3109–3115. [Google Scholar] [CrossRef]
- Tezuka, Y.; Tsuchitani, A.; Yoshioka, Y.; Oike, H. Synthesis of θ-Shaped Poly(THF) by Electrostatic Self-Assembly and Covalent Fixation with Three-Armed Star Telechelics Having Cyclic Ammonium Salt Groups. Macromolecules 2003, 36, 65–70. [Google Scholar] [CrossRef]
- Polymeropoulos, G.; Zapsas, G.; Ntetsikas, K.; Bilalis, P.; Gnanou, Y.; Hadjichristidis, N. 50th Anniversary Perspective: Polymers with Complex Architectures. Macromolecules 2017, 50, 1253–1290. [Google Scholar] [CrossRef]
- Kusuyama, N.; Daito, Y.; Kubota, H.; Kametani, Y.; Ouchi, M. Construction of Ring-Based Architectures: Via Ring-Expansion Cationic Polymerization and Post-Polymerization Modification: Design of Cyclic Initiators from Divinyl Ether and Dicarboxylic Acid. Polym. Chem. 2021, 12, 2532–2541. [Google Scholar] [CrossRef]
- Alkayal, N.; Zhang, Z.; Bilalis, P.; Gnanou, Y.; Hadjichristidis, N. Polyethylene-Based Tadpole Copolymers. Macromol. Chem. Phys. 2017, 218, 062502. [Google Scholar] [CrossRef]
- Segawa, Y.; Kuwayama, M.; Itami, K. Synthesis and Structure of [9]Cycloparaphenylene Catenane: An All-Benzene Catenane Consisting of Small Rings. Org. Lett. 2020, 22, 1067–1070. [Google Scholar] [CrossRef]
- Uehara, E.; Deguchi, T. Statistical Properties of Multi-Theta Polymer Chains. J. Phys. A Math. Theor. 2018, 51, 134001. [Google Scholar] [CrossRef]
- Abreu, C.R.A.; Escobedo, F.A. A Novel Configurational-Bias Monte Carlo Method for Lattice Polymers: Application to Molecules with Multicyclic Architectures. Macromolecules 2005, 38, 8532–8545. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, X.; Li, J.; Wang, Y. Radius of Gyration, Mean Span, and Geometric Shrinking Factors of Bridged Polycyclic Ring Polymers. Macromol. Theory. Simul. 2016, 25, 482–496. [Google Scholar] [CrossRef]
- Pipertzis, A.; Hossain, M.D.; Monteiro, M.J.; Floudas, G. Segmental Dynamics in Multicyclic Polystyrenes. Macromolecules 2018, 51, 1488–1497. [Google Scholar] [CrossRef]
- Doi, Y.; Takano, A.; Takahashi, Y.; Matsushita, Y. Viscoelastic Properties of Dumbbell-Shaped Polystyrenes in Bulk and Solution. Macromolecules 2021, 54, 1366–1374. [Google Scholar] [CrossRef]
- Haydukivska, K.; Blavatska, V.; Paturej, J. Molecular Conformations of Dumbbell-Shaped Polymers in Good Solvent. Phys. Rev. E 2023, 108, 034502. [Google Scholar] [CrossRef] [PubMed]
- Doi, Y.; Takano, A.; Takahashi, Y.; Matsushita, Y. Terminal Relaxation Behavior of Entangled Linear Polymers Blended with Ring and Dumbbell-Shaped Polymers in Melts. Rheol. Acta 2022, 61, 681–688. [Google Scholar] [CrossRef]
- Murashima, T.; Hagita, K.; Kawakatsu, T. Topological Transition in Multicyclic Chains with Structural Symmetry Inducing Stress-Overshoot Phenomena in Multicyclic/Linear Blends under Biaxial Elongational Flow. Macromolecules 2022, 55, 9358–9372. [Google Scholar] [CrossRef]
- Doi, Y.; Takano, A.; Takahashi, Y.; Matsushita, Y. Melt Rheology of Tadpole-Shaped Polystyrenes. Macromolecules 2015, 48, 8667–8674. [Google Scholar] [CrossRef]
- Rosa, A.; Smrek, J.; Turner, M.S.; Michieletto, D. Threading-Induced Dynamical Transition in Tadpole-Shaped Polymers. ACS Macro Lett. 2020, 9, 743–748. [Google Scholar] [CrossRef]
- Doi, Y.; Takano, A.; Takahashi, Y.; Matsushita, Y. Melt Rheology of Tadpole-Shaped Polystyrenes with Different Ring Sizes. Soft Matter 2020, 16, 8720–8724. [Google Scholar] [CrossRef]
- Golba, B.; Benetti, E.M.; De Geest, B.G. Biomaterials Applications of Cyclic Polymers. Biomaterials 2021, 267, 120468. [Google Scholar] [CrossRef]
- Niemyska, W.; Dabrowski-Tumanski, P.; Kadlof, M.; Haglund, E.; Sułkowski, P.; Sulkowska, J.I. Complex Lasso: New Entangled Motifs in Proteins. Sci. Rep. 2016, 6, 36895. [Google Scholar] [CrossRef]
- Wilson, K.A.; Kalkum, M.; Ottesen, J.; Yuzenkova, J.; Chait, B.T.; Landick, R.; Muir, T.; Severinov, K.; Darst, S.A. Structure of Microcin J25, a Peptide Inhibitor of Bacterial RNA Polymerase, Is a Lassoed Tail. J. Am. Chem. Soc. 2003, 125, 12475–12483. [Google Scholar] [CrossRef]
- Zong, C.; Maksimov, M.O.; Link, A.J. Construction of Lasso Peptide Fusion Proteins. ACS Chem. Biol. 2016, 11, 61–68. [Google Scholar] [CrossRef] [PubMed]
- Katahira, R.; Yamasaki, M.; Matsuda, Y.; Yoshida, M. MS-271, A Novel Inhibitor of Calmodulin-activated Myosin Light Chain Kinase from Streptomyces sp.—II. Solution Structure of MS-271: Characteristic Features of the ‘Lasso’ Structure. Bioorg. Med. Chem. 2016, 4, 121–129. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.M.; Edwards, B.J.; Keffer, D.J.; Khomami, B. Dynamics of Individual Molecules of Linear Polyethylene Liquids under Shear: Atomistic Simulation and Comparison with a Free-Draining Bead-Rod Chain. J. Rheol. 2010, 54, 283–310. [Google Scholar] [CrossRef]
- Öttinger, H.C. Stochastic Processes in Polymeric Fluids; Springer: Berlin, Germany, 1996. [Google Scholar]
- Liu, T.W. Flexible Polymer Chain Dynamics and Rheological Properties in Steady Flows. J. Chem. Phys. 1989, 90, 5826–5842. [Google Scholar] [CrossRef]
- Rotne, J.; Prager, S. Variational Treatment of Hydrodynamic Interaction in Polymers. J. Chem. Phys. 1969, 50, 4831–4837. [Google Scholar] [CrossRef]
- Yamakawa, H. Transport Properties of Polymer Chains in Dilute Solution: Hydrodynamic Interaction. J. Chem. Phys. 1970, 53, 436–443. [Google Scholar] [CrossRef]
- Weeks, J.D.; Chandler, D.; Andersen, H.C. Role of Repulsive Forces in Determining the Equilibrium Structure of Simple Liquids. J. Chem. Phys. 1971, 54, 5237–5247. [Google Scholar] [CrossRef]
- Schroeder, C.M.; Teixeira, R.E.; Shaqfeh, E.S.G.; Chu, S. Characteristic Periodic Motion of Polymers in Shear Flow. Phys. Rev. Lett. 2005, 95, 018301. [Google Scholar] [CrossRef]
- Teixeira, R.E.; Babcock, H.P.; Shaqfeh, E.S.G.; Chu, S. Shear Thinning and Tumbling Dynamics of Single Polymers in the Flow-Gradient Plane. Macromolecules 2005, 38, 581–592. [Google Scholar] [CrossRef]
- Teixeira, R.E.; Dambal, A.K.; Richter, D.H.; Shaqfeh, E.S.G.; Chu, S. The Individualistic Dynamics of Entangled DNA in Solution. Macromolecules 2007, 40, 2461–2476. [Google Scholar] [CrossRef]
- Cho, S.; Kim, J.M.; Baig, C. Scaling Characteristics of Rotational Dynamics and Rheology of Linear Polymer Melts in Shear Flow. Macromolecules 2020, 53, 3030–3041. [Google Scholar] [CrossRef]
- Jeong, S.H.; Cho, S.; Baig, C. Chain Rotational Dynamics in Dilute Polymer Solutions and Melts under Shear Flow. Polymer 2023, 281, 126101. [Google Scholar] [CrossRef]
- Moore, J.D.; Cui, S.T.; Cochran, H.D.; Cummings, P.T. A Molecular Dynamics Study of a Short-Chain Polyethylene Melt. I. Steady-State Shear. J. Non-Newton. Fluid Mech. 2000, 93, 83–99. [Google Scholar] [CrossRef]
- Kim, J.M.; Keffer, D.J.; Kröger, M.; Edwards, B.J. Rheological and Entanglement Characteristics of Linear-Chain Polyethylene Liquids in Planar Couette and Planar Elongational Flows. J. Non-Newton. Fluid Mech. 2008, 152, 168–183. [Google Scholar] [CrossRef]
- Flyvbjerg, H.; Petersen, H.G. Error Estimates on Averages of Correlated Data. J. Chem. Phys. 1989, 91, 461–466. [Google Scholar] [CrossRef]
- Colby, R.H.; Boris, D.C.; Krause, W.E.; Dou, S. Shear thinning of unentangled flexible polymer liquids. Rheol. Acta 2007, 46, 569–575. [Google Scholar] [CrossRef]
- Stratton, R.A. Non-Newtonian Flow in Polymer Systems with No Macromolecules Entanglement Coupling. Macromolecules 1954, 5, 304–310. [Google Scholar] [CrossRef]
- Yan, Z.C.; Costanzo, S.; Jeong, Y.; Chang, T.; Vlassopoulos, D. Linear and Nonlinear Shear Rheology of a Marginally Entangled Ring Polymer. Macromolecules 2016, 49, 1444–1453. [Google Scholar] [CrossRef]
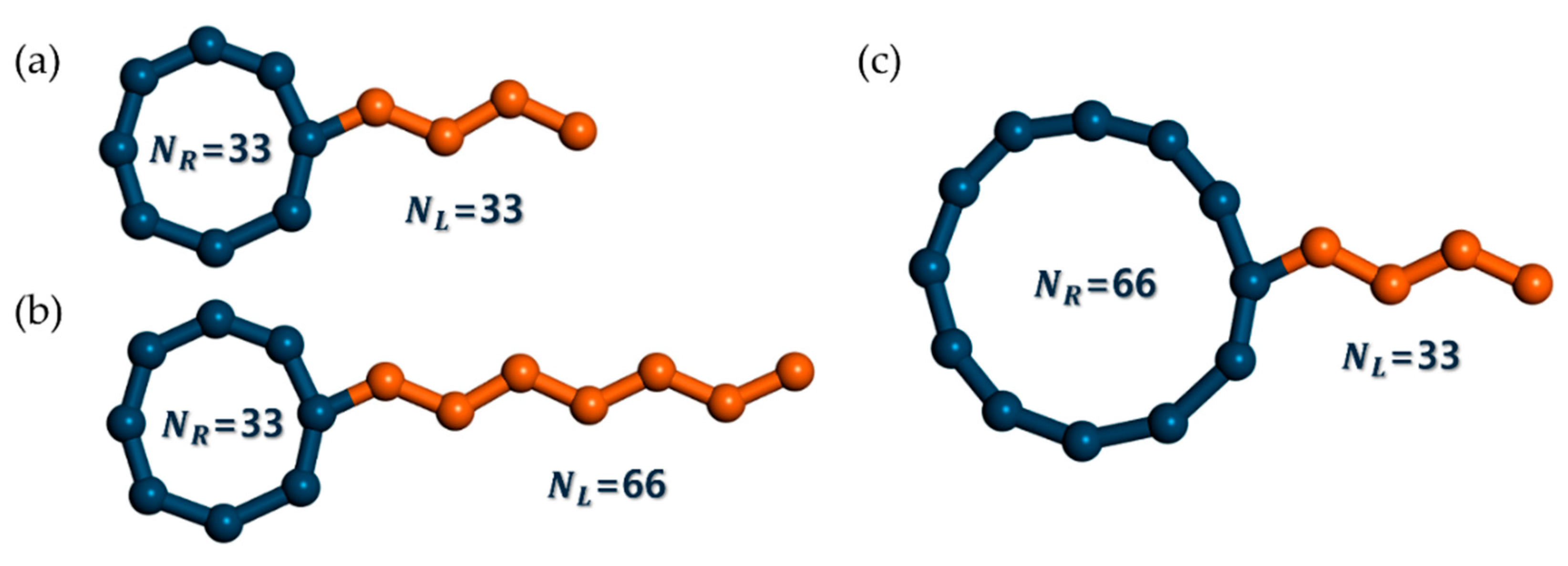
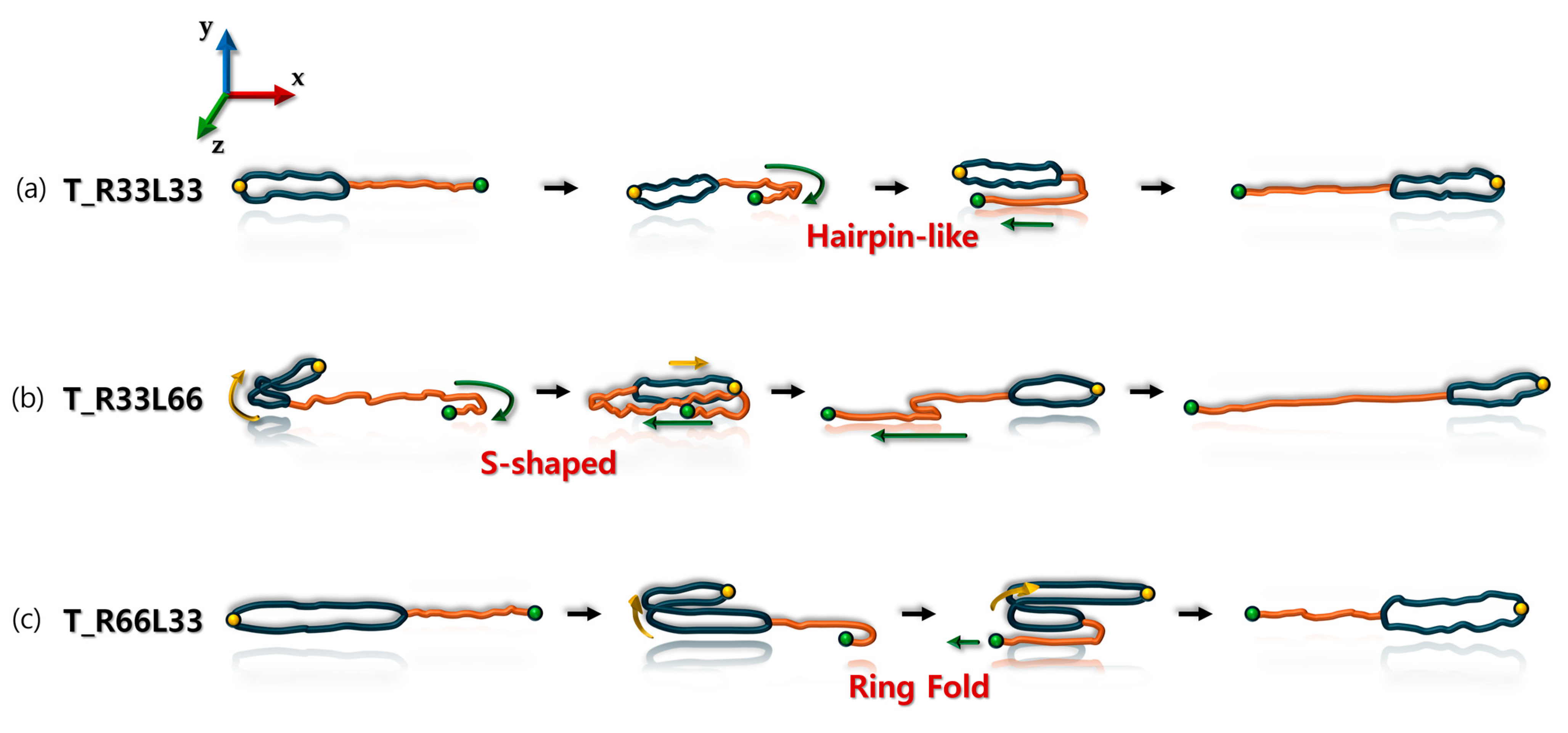
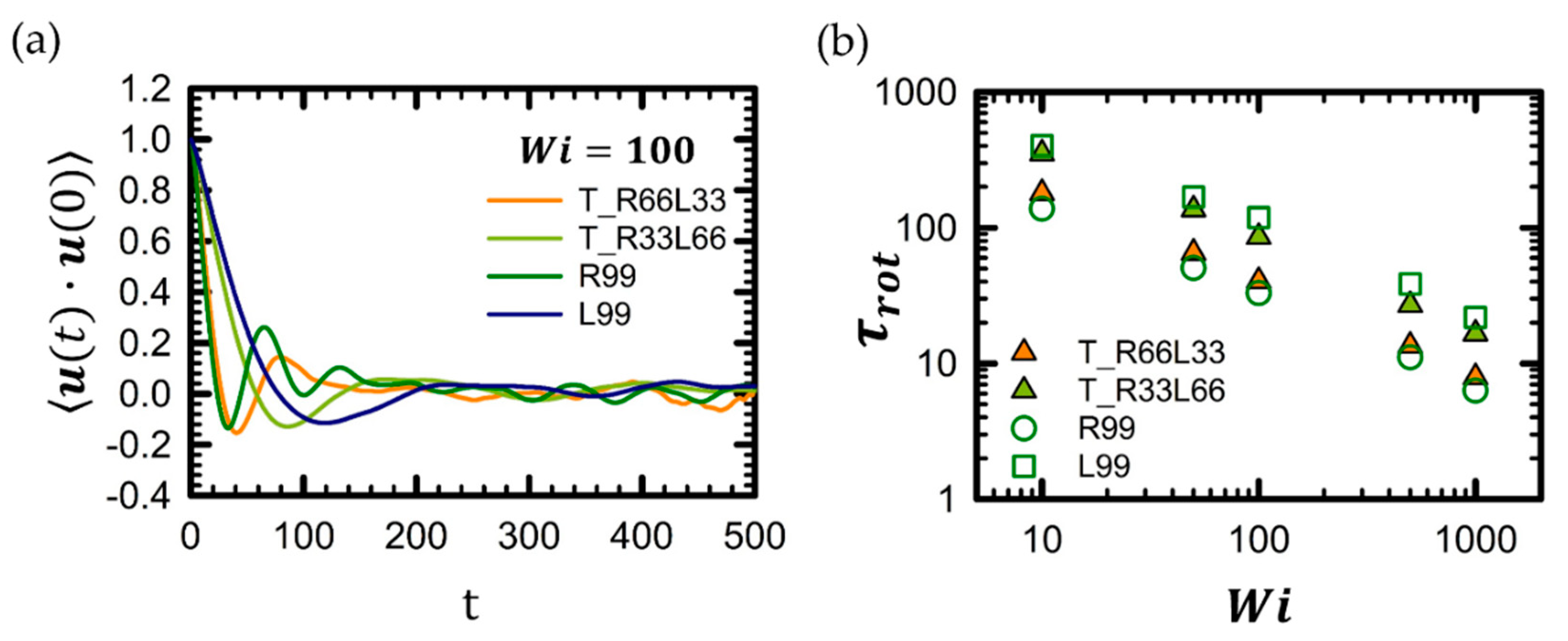

| Chain Molecules | Pure L99 | Pure R99 | T_R33L33 | T_R66L33 | T_R33L66 |
|---|---|---|---|---|---|
| No. of Beads | 99 | 99 | 66 | 99 | 99 |
| No. of Rods | 98 | 99 | 65 | 98 | 98 |
| No. of Molecules | 100 | 100 | 100 | 100 | 100 |
| Timestep | 0.0005 | 0.0005 | 0.0005 | 0.0005 | 0.0005 |
| Relaxation Time | 321.75 | 152.03 | 110.84 | 168.52 | 286.13 |
| Simulation Time | 1500 | 1000 | 500 | 1000 | 1000 |
| Chain Length | Pure Ring | Pure Linear | Tadpole | |||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 33 | 6.73 | 6.58 | 12.23 | 12.25 | ||
| 66 | 16.27 | 15.65 | 29.83 | 29.69 | 21.74 (T_R33L33) | 21.64 (T_R33L33) |
| 99 | 26.75 | 25.97 | 49.70 | 49.82 | 29.40 (T_R66L33) | 32.07 (T_R66L33) |
| 42.94 (T_R33L66) | 41.68 (T_R33L66) | |||||
| Chain Length | Pure Ring | Pure Linear | Tadpole | |||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 33 | 21.12 | 20.92 | 48.81 | 47.59 | ||
| 66 | 71.02 | 72.41 | 144.14 | 154.61 | 110.84 (T_R33L33) | 110.51 (T_R33L33) |
| 99 | 152.02 | 149.69 | 321.75 | 308.02 | 168.52 (T_R66L33) | 193.55 (T_R66L33) |
| 286.13 (T_R33L66) | 283.13 (T_R33L66) | |||||
| Wi | T_R33L33 | T_R66L33 | T_R33L66 | |||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 10 | 29.78 | 29.89 | 39.28 | 42.97 | 66.24 | 58.97 |
| 50 | 43.55 | 40.99 | 56.40 | 57.74 | 109.65 | 82.03 |
| 100 | 48.43 | 46.98 | 70.12 | 65.62 | 131.84 | 94.60 |
| 500 | 53.87 | 64.60 | 76.46 | 88.87 | 157.68 | 131.82 |
| T_R33L33 | T_R66L33 | T_R33L66 | ||||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 10 | 130 | 139.60 | 180 | 220.00 | 352.5 | 380.10 |
| 50 | 44.5 | 44.29 | 65.5 | 70.01 | 136.5 | 120.38 |
| 100 | 28.5 | 27.01 | 40.5 | 42.76 | 85.5 | 73.37 |
| 500 | 9 | 8.57 | 13.5 | 13.61 | 27 | 23.24 |
| 1000 | 4.9 | 5.23 | 7.9 | 8.31 | 16.6 | 14.16 |
| T_R33L33 | T_R66L33 | T_R33L66 | ||||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 10 | 135.19 | 139.03 | 344.26 | 318.89 | 342.66 | 483.67 |
| 50 | 64.63 | 63.75 | 127.89 | 147.14 | 170.52 | 220.52 |
| 100 | 45.74 | 45.57 | 88.66 | 105.46 | 116.62 | 157.24 |
| 500 | 21.35 | 20.90 | 39.47 | 48.66 | 55.45 | 71.69 |
| 1000 | 14.72 | 14.94 | 29.58 | 34.88 | 39.19 | 51.12 |
| T_R33L33 | T_R66L33 | T_R33L66 | ||||
|---|---|---|---|---|---|---|
Simulated | Estimated | Simulated | Estimated | Simulated | Estimated | |
| 10 | 3087.92 | 2997.25 | 9225.14 | 11,605.31 | 23,713.02 | 38,072.64 |
| 50 | 386.85 | 361.01 | 1254.75 | 1452.79 | 3226.67 | 4539.31 |
| 100 | 154.75 | 145.12 | 517.43 | 593.77 | 1227.97 | 1816.41 |
| 500 | 17.60 | 17.49 | 53.69 | 74.40 | 135.67 | 216.58 |
| 1000 | 6.68 | 7.03 | 20.11 | 30.42 | 53.09 | 86.67 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cho, C.; Kim, J.M. Scaling Relationships of the Structural and Rheological Behavior of Tadpole Polymer Chains in Dilute Solution Systems Using Brownian Dynamics Simulations. Polymers 2024, 16, 2871. https://doi.org/10.3390/polym16202871
Cho C, Kim JM. Scaling Relationships of the Structural and Rheological Behavior of Tadpole Polymer Chains in Dilute Solution Systems Using Brownian Dynamics Simulations. Polymers. 2024; 16(20):2871. https://doi.org/10.3390/polym16202871
Chicago/Turabian StyleCho, Chaehyun, and Jun Mo Kim. 2024. "Scaling Relationships of the Structural and Rheological Behavior of Tadpole Polymer Chains in Dilute Solution Systems Using Brownian Dynamics Simulations" Polymers 16, no. 20: 2871. https://doi.org/10.3390/polym16202871
APA StyleCho, C., & Kim, J. M. (2024). Scaling Relationships of the Structural and Rheological Behavior of Tadpole Polymer Chains in Dilute Solution Systems Using Brownian Dynamics Simulations. Polymers, 16(20), 2871. https://doi.org/10.3390/polym16202871






