The Domain and Structural Characteristics of Ferroelectric Copolymers Based on Vinylidene Fluoride Copolymer with Tetrafluoroethylene Composition (94/6)
Abstract
1. Introduction
2. Materials and Methods
2.1. Nuclear Magnetic Resonance (NMR)
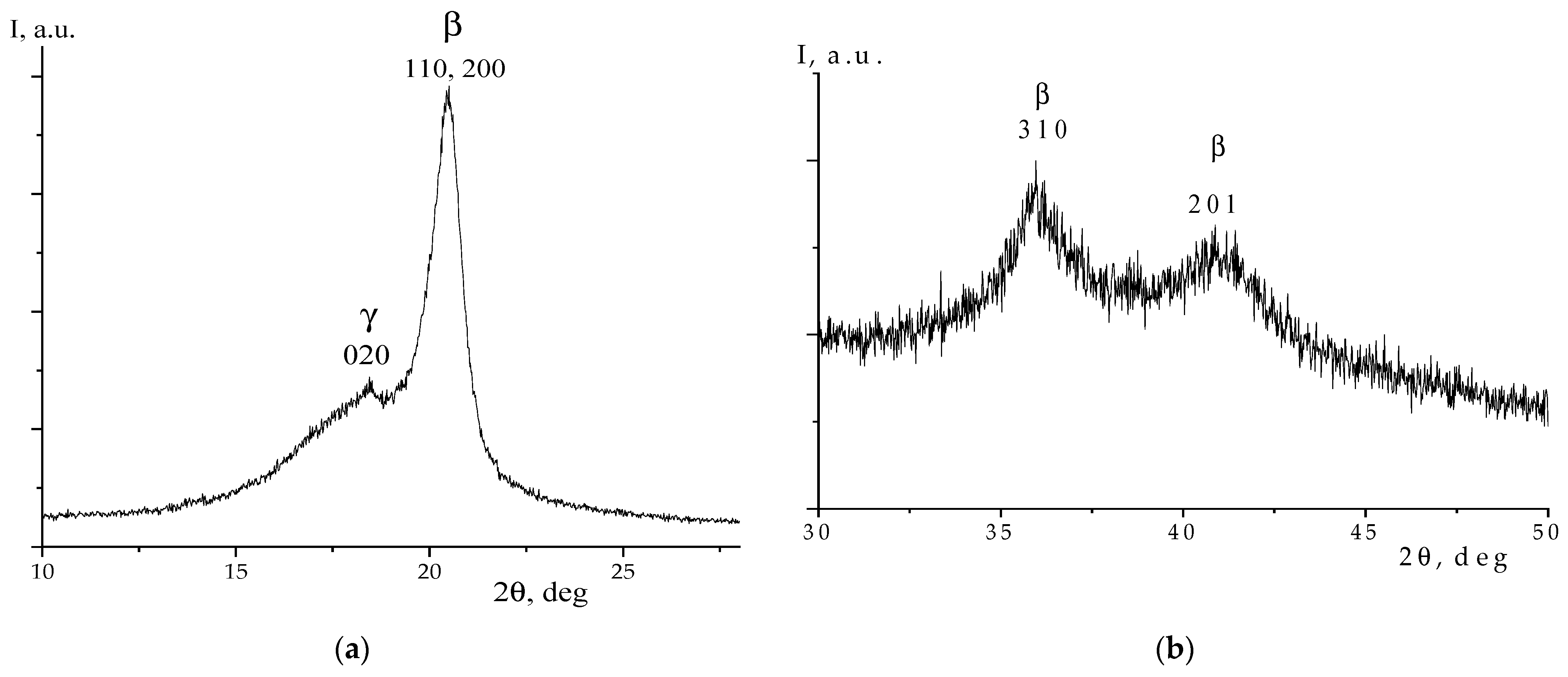
2.2. X-ray Diffraction
2.3. Fourier-Transform Infrared Spectroscopy
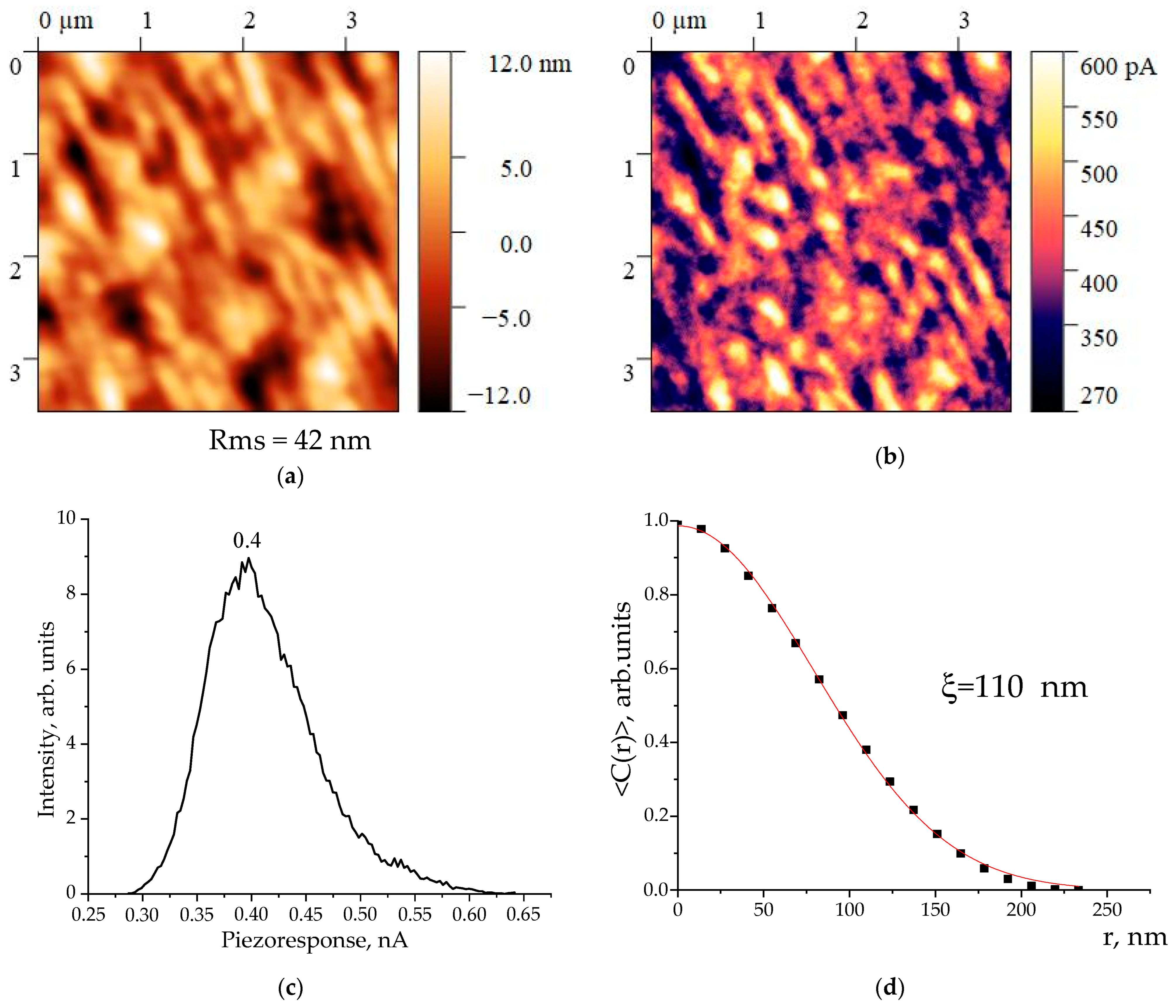
2.4. Scanning Probe Microscopy
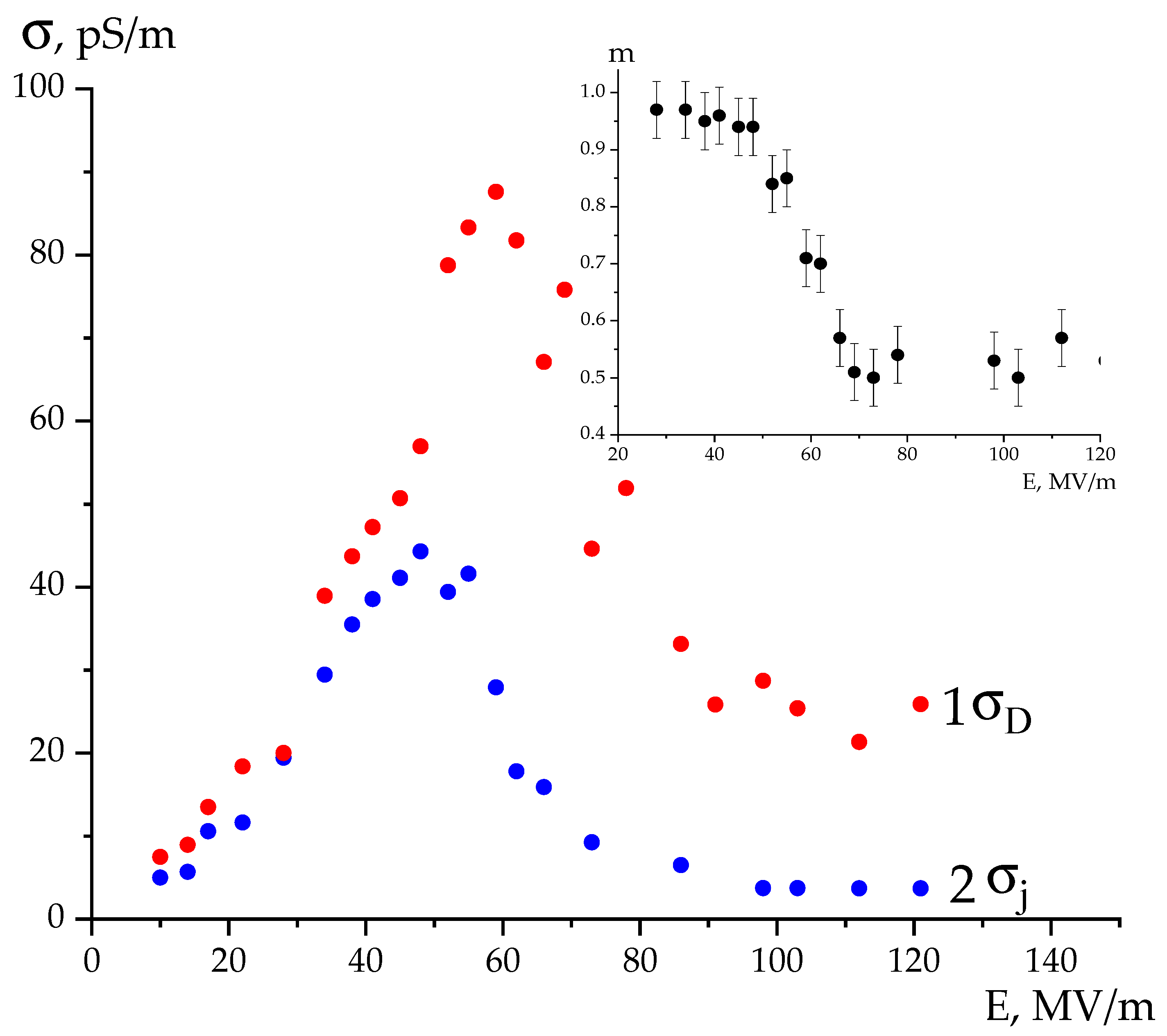
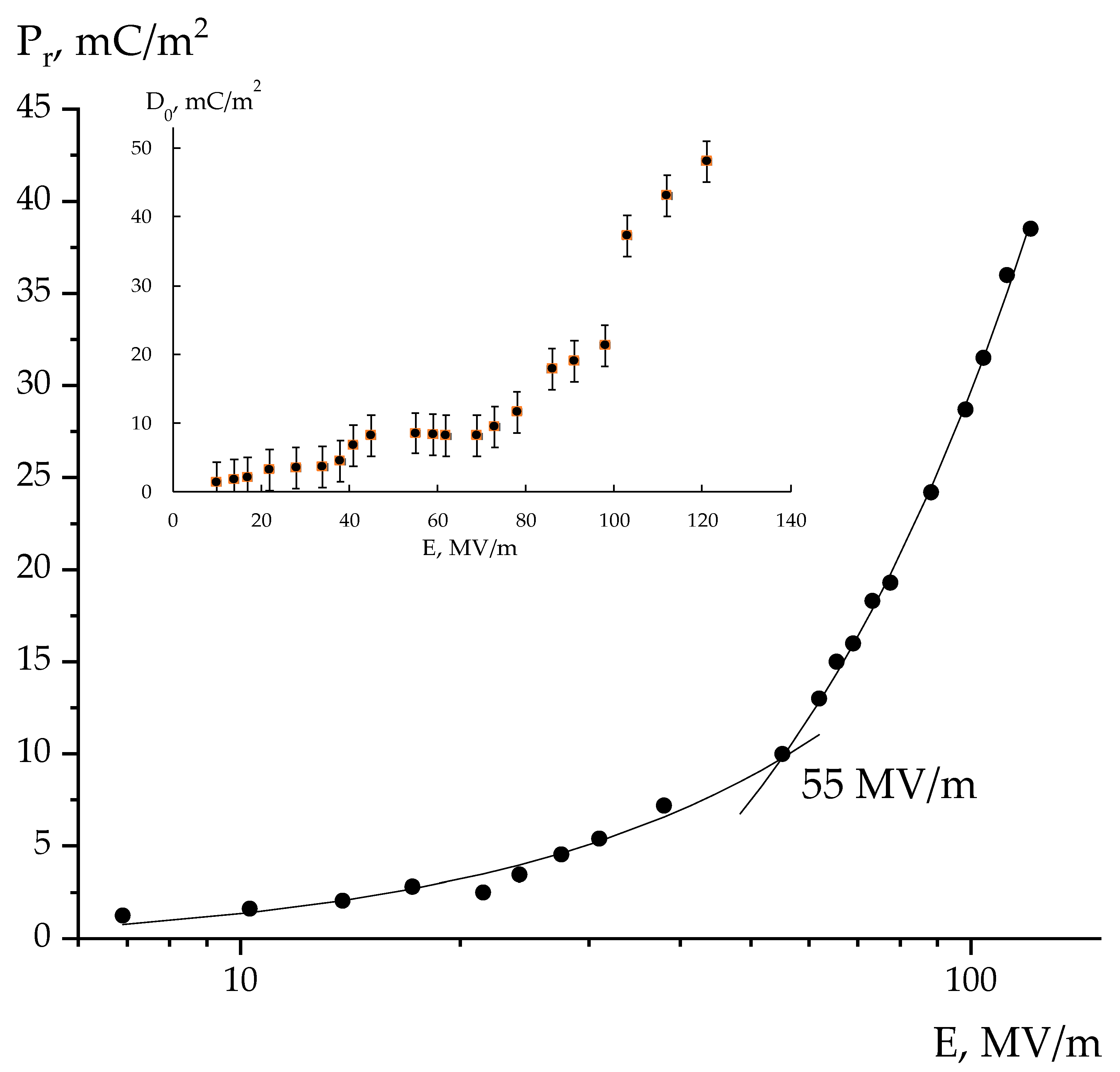
3. Results and Discussion
p(R) = 0.05 × (mol % VF2/100) = 0.05 × 0.936 = 0.047
p(T) = 0.064
p(FFFF) = p(F) × p(F|F) × p(F|F) × p(F|F) = 0.894 = 0.63 [02]
p(FRFF) = p(F) × p(F|R) × p(R|F) × p(F|F) = 0.89 × 0.047 × 0.89 × 0.89 = 0.033 [02,20]
p(FTFF) = p(F) × p(F|T) p(T|F) × p(F|F) = 0.89 × 0.064 × 0.89 × 0.89 = 0.045 [02,22]
p(FRFR) = p(F) × p(F|R) × p(R|F) × p(F|R)= 0.89 × 0.047 × 0.89 × 0.047 = 0.0017 [02,20]
p(FRTF) = p(F) × p(F|R) × p(R|T) × p(T|F) = 0.89 × 0.047 × 0.064 × 0.89 = 0.0024 [02,20,22]
p(FTFT) = p(F) × p(F|T) × p(T|F) × p(F|T) = 0.89 × 0.064 × 0.89 × 0.064 = 0.0032 [02,22]
etc.
- The present amorphous phase is in a liquid-like state, which implies that the chains of the amorphous phase are co-dynamical. The oscillating dipoles (electric field sources) will reduce the observed contrast.
- Furthermore, the structure of the crystallizing polymers is not strictly biphasic, given the thermodynamic point of view, since the amorphous and crystalline phases do not have clear boundary interfaces. Here, one can observe tensegrity chains (constricting-tight chains) passing between them. This circumstance also does not contribute to high contrast of the piezoresponse signal.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, T.T.; Herbert, J.M.; Glass, A.M. The Applications of Ferroelectric Polymers; Blackie and Son: Bishopbriggs, UK; Glasgow, UK, 1988. [Google Scholar]
- Nalwa, H.S. Ferroelectric Polymers: Chemistry: Physics, and Applications; CRC Press: Boca Raton, FL, USA, 1995. [Google Scholar]
- Asadi, K. (Ed.) Organic Ferroelectric Materials and Applications; Woodhead Publishing: Sawston, UK; Elsevier: Amsterdam, The Netherlands, 2021. [Google Scholar]
- Solodilov, V.; Kochervinskii, V.; Osipkov, A.; Makeev, M.; Maltsev, A.; Yurkov, G.; Lokshin, B.; Bedin, S.; Shapetina, M.; Tretyakov, I. Structure and thermomechanical properties of polyvinylidene fluoride film with transparent indium tin oxide electrodes. Polymers 2023, 15, 1483. [Google Scholar] [CrossRef]
- Ohigashi, H.; Kagami, N.; Li, G.R. Formation of ferroelectric domains in a copolymer P (VDF–TrFE). J. Appl. Phys. 1992, 71, 506–508. [Google Scholar] [CrossRef]
- Zhu, Y.; Jiang, P.; Zhang, Z.; Huang, X. Dielectric phenomena and electrical energy storage of poly(vinylidene fluoride) based high-k polymers. Chin. Chem. Lett. 2017, 28, 2027–2035. [Google Scholar] [CrossRef]
- Kolb, R.; Wutz, C.; Stribeck, N.; von Krosigk, G.; Riekel, C. Investigation of secondary crystallization of polymers by means of microbeam X-ray scattering. Polymer 2001, 42, 5257–5266. [Google Scholar] [CrossRef]
- Govinna, N.D.; Sadeghi, I.; Schick, C.; Asatekin, A.; Cebe, P. Crystallization kinetics, polymorphism fine tuning, and rigid amorphous fraction of poly(vinylidene fluoride) blends. Polym. Cryst. 2021, 4, e10205. [Google Scholar] [CrossRef]
- Ando, S.; Harris, R.K.; Holstein, P.; Reinsberg, S.A.; Yamauchi, K. Solid-state 1H-static, 1H-MAS, and 1H→ 19F/19F→ 1H CP/MAS NMR study of poly(vinyl fluoride). Polymer 2001, 42, 8137–8151. [Google Scholar] [CrossRef]
- Mandelkern, L. Crystallization of Polymers: Volume 2, Kinetics and Mechanisms; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Wunderlich, B. Macromolecular Physics: Volume 2, Crystal Nucleation, Growth, Annealing; Rensselaer Polytechnic Institute Troy: New York, NY, USA, 1976. [Google Scholar]
- Kochervinskii, V.V.; Malyshkina, I.A. Dielectric spectroscopy of ferroelectric polymers. In Woodhead Publishing Series in Electronic and Optical Materials. Organic Ferroelectric Materials and Applications; Asadi, K., Ed.; Elsevier: Amsterdam, The Netherlands, 2022; pp. 263–355. [Google Scholar]
- Fukada, E.; Furukawa, T. Piezoelectricity and ferroelectricity in polyvinylidene fluoride. Ultrasonics 1981, 19, 31–39. [Google Scholar] [CrossRef]
- Kochervinskii, V.V. New electrostriction materials based on organic polymers: A review. Crystallogr. Rep. Suppl. 2009, 54, 1146–1171. [Google Scholar] [CrossRef]
- Cais, R.E.; Kometani, J.M. Structural studies of vinylidene fluoride-tetrafluoroethylene copolymers by nuclear magnetic resonance spectroscopy. Anal. Chim. Acta 1986, 189, 101–116. [Google Scholar] [CrossRef]
- Kochervinskii, V.V. Structure and properties of bulk polyvinylidenefluoride and systems based on it. Russ. Chem. Rev. 1996, 65, 865–913. [Google Scholar] [CrossRef]
- Legrand, J.F.; Schuele, P.J.; Schmidt, V.H.; Minier, M. Nmr study of the ferroelectric phase transition in a 7030mol% copolymer of vinylidene fluoride (VF2) and trifluoroethylene (TrFE). Polymer 1985, 26, 1683–1688. [Google Scholar] [CrossRef]
- Shvartsman, V.V.; Kholkin, A.L.; Orlova, A.; Kiselev, D.A.; Bogomolov, A.A.; Sternberg, A. Polar nanodomains and local ferroelectric phenomena in relaxor lead lanthanum zirconate titanate ceramics. Appl. Phys. Lett. 2005, 86, 202907. [Google Scholar] [CrossRef]
- Shvartsman, V.V.; Kholkin, A.L. Evolution of nanodomains in 0.9 PbMg1/3Nb2/3O3-0.1 PbTiO3 single crystals. J. Appl. Phys. 2007, 101, 064108. [Google Scholar] [CrossRef]
- Shvartsman, V.V.; Dkhil, B.; Kholkin, A.L. Mesoscale domains and nature of the relaxor state by piezoresponse force microscopy. Annu. Rev. Mater. Res. 2013, 43, 423–449. [Google Scholar] [CrossRef]
- Furukawa, T.; Naoya, S. Electrostriction as the origin of piezoelectricity in ferroelectric polymers. Jpn. J. Appl. Phys. 1990, 29, 675–680. [Google Scholar] [CrossRef]
- Kwon, Y.K.; Boller, A.; Pyda, M.; Wunderlich, B. Melting and heat capacity of gel-spun, ultra-high molar mass polyethylene fibers. Polymer 2000, 4, 6237–6249. [Google Scholar] [CrossRef]
- De Rosa, C.; Auriemma, F.; Galotto, N.; Di Girolamo, R. Mesomorphic form of isotactic polypropylene in stereodefective polypropylene: Solid mesophase or liquid-crystal like structure. Polymer 2012, 53, 2422–2428. [Google Scholar] [CrossRef]
- Androsch, R. Melting and crystallization of poly(ethylene-co-octene) measured by modulated d.s.c. and temperature-resolved X-ray diffraction. Polymer 1999, 40, 2805–2812. [Google Scholar] [CrossRef]
- Nam, J.Y.; Kadomatsu, S.; Saito, H.; Inoue, T. Thermal reversibility in crystalline morphology of LLDPE crystallites. Polymer 2002, 43, 2101–2107. [Google Scholar] [CrossRef]
- Naga, N.; Arai, R.; Kikuchi, G.; Toyota, A.; Noguchi, K.; Sone, M.; Shirae, F.; Gotoh, T.; Kurosu, H. Crystalline structure of polyethylene containing vinylene units in the main chain. Polymer 2011, 52, 4857–4866. [Google Scholar] [CrossRef]
- Colombe, G.; Gree, S.; Lhost, O.; Dupire, M.; Rosenthal, M.; Ivanov, D. Corequation between mechanical properties and orientation of the crystalline and mesomorphic phases in isotactic polypropylene fibers. Polymer 2011, 52, 5630–5643. [Google Scholar] [CrossRef]
- Kochervinskii, V.V.; Gradova, M.A.; Gradov, O.V.; Kirakosyan, G.A.; Kiselev, D.A.; Buzin, M.I.; Lokshin, B.V.; Korlyukov, A.A.; Maltsev, A.A.; Malyshkina, I.A. Influence of Physical Aging of Ferroelectric Vinylidene Fluoride Copolymer Films on Their Structural and Electrophysical Characteristics. J. Appl. Polym. Sci. 2022, 139, 53025. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kochervinskii, V.V.; Buryanskaya, E.L.; Osipkov, A.S.; Ryzhenko, D.S.; Kiselev, D.A.; Lokshin, B.V.; Zvyagina, A.I.; Kirakosyan, G.A. The Domain and Structural Characteristics of Ferroelectric Copolymers Based on Vinylidene Fluoride Copolymer with Tetrafluoroethylene Composition (94/6). Polymers 2024, 16, 233. https://doi.org/10.3390/polym16020233
Kochervinskii VV, Buryanskaya EL, Osipkov AS, Ryzhenko DS, Kiselev DA, Lokshin BV, Zvyagina AI, Kirakosyan GA. The Domain and Structural Characteristics of Ferroelectric Copolymers Based on Vinylidene Fluoride Copolymer with Tetrafluoroethylene Composition (94/6). Polymers. 2024; 16(2):233. https://doi.org/10.3390/polym16020233
Chicago/Turabian StyleKochervinskii, Valentin V., Evgeniya L. Buryanskaya, Alexey S. Osipkov, Dmitriy S. Ryzhenko, Dmitry A. Kiselev, Boris V. Lokshin, Aleksandra I. Zvyagina, and Gayane A. Kirakosyan. 2024. "The Domain and Structural Characteristics of Ferroelectric Copolymers Based on Vinylidene Fluoride Copolymer with Tetrafluoroethylene Composition (94/6)" Polymers 16, no. 2: 233. https://doi.org/10.3390/polym16020233
APA StyleKochervinskii, V. V., Buryanskaya, E. L., Osipkov, A. S., Ryzhenko, D. S., Kiselev, D. A., Lokshin, B. V., Zvyagina, A. I., & Kirakosyan, G. A. (2024). The Domain and Structural Characteristics of Ferroelectric Copolymers Based on Vinylidene Fluoride Copolymer with Tetrafluoroethylene Composition (94/6). Polymers, 16(2), 233. https://doi.org/10.3390/polym16020233






