Experimental Study on Warpage Phenomenon of Wax Parts Manufactured by Fused Filament Fabrication
Abstract
1. Introduction
2. Methodology
2.1. Materials and Characterization
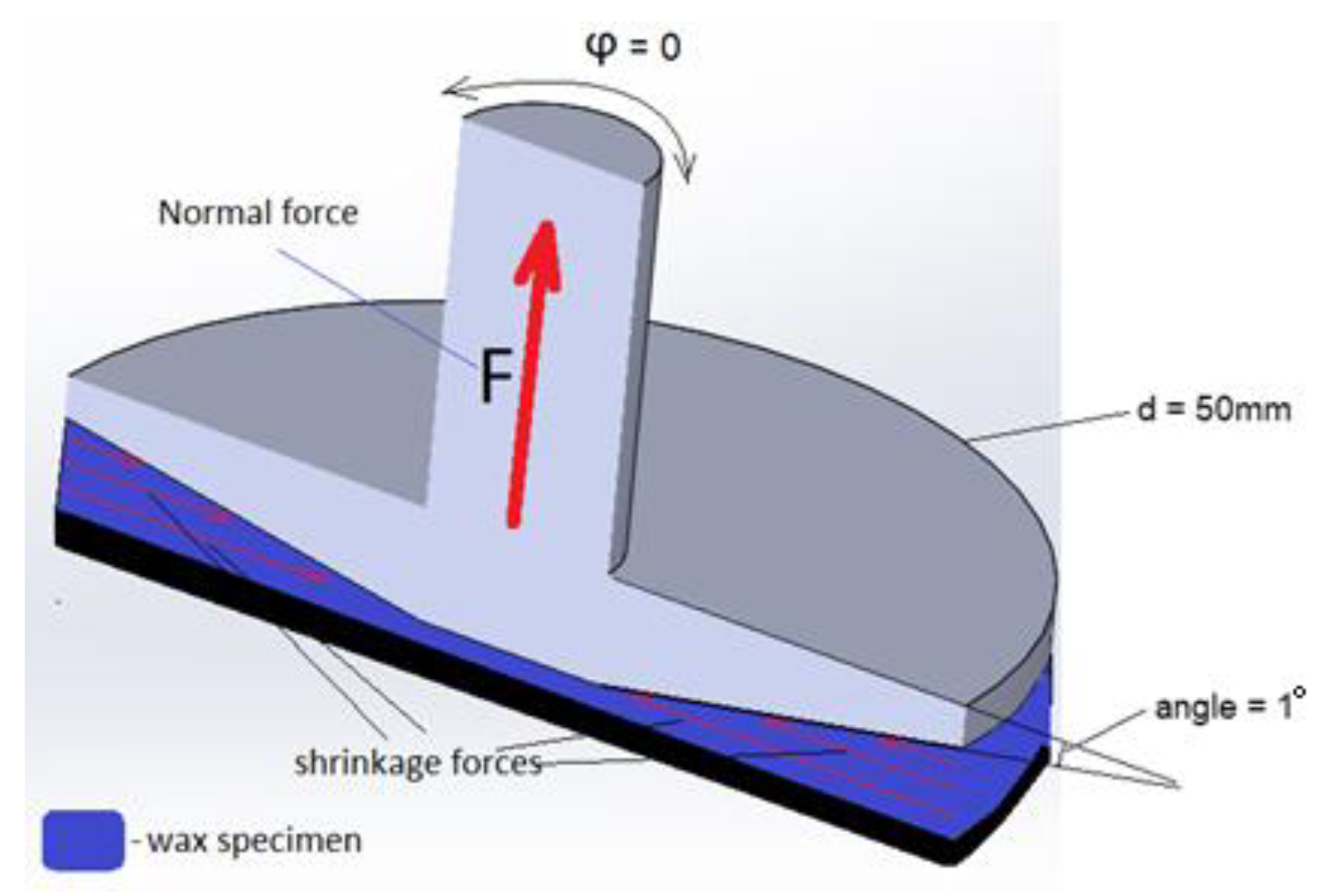
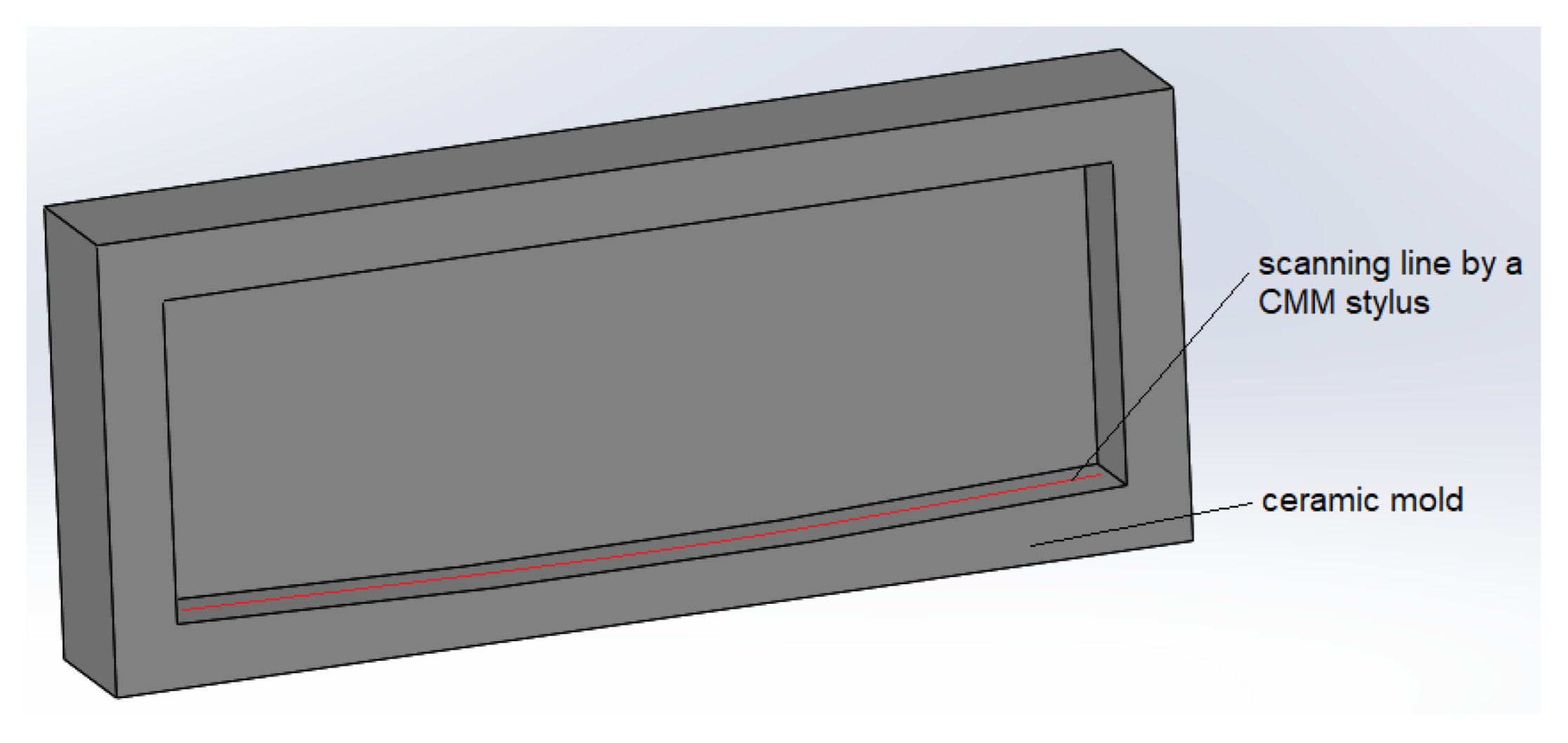
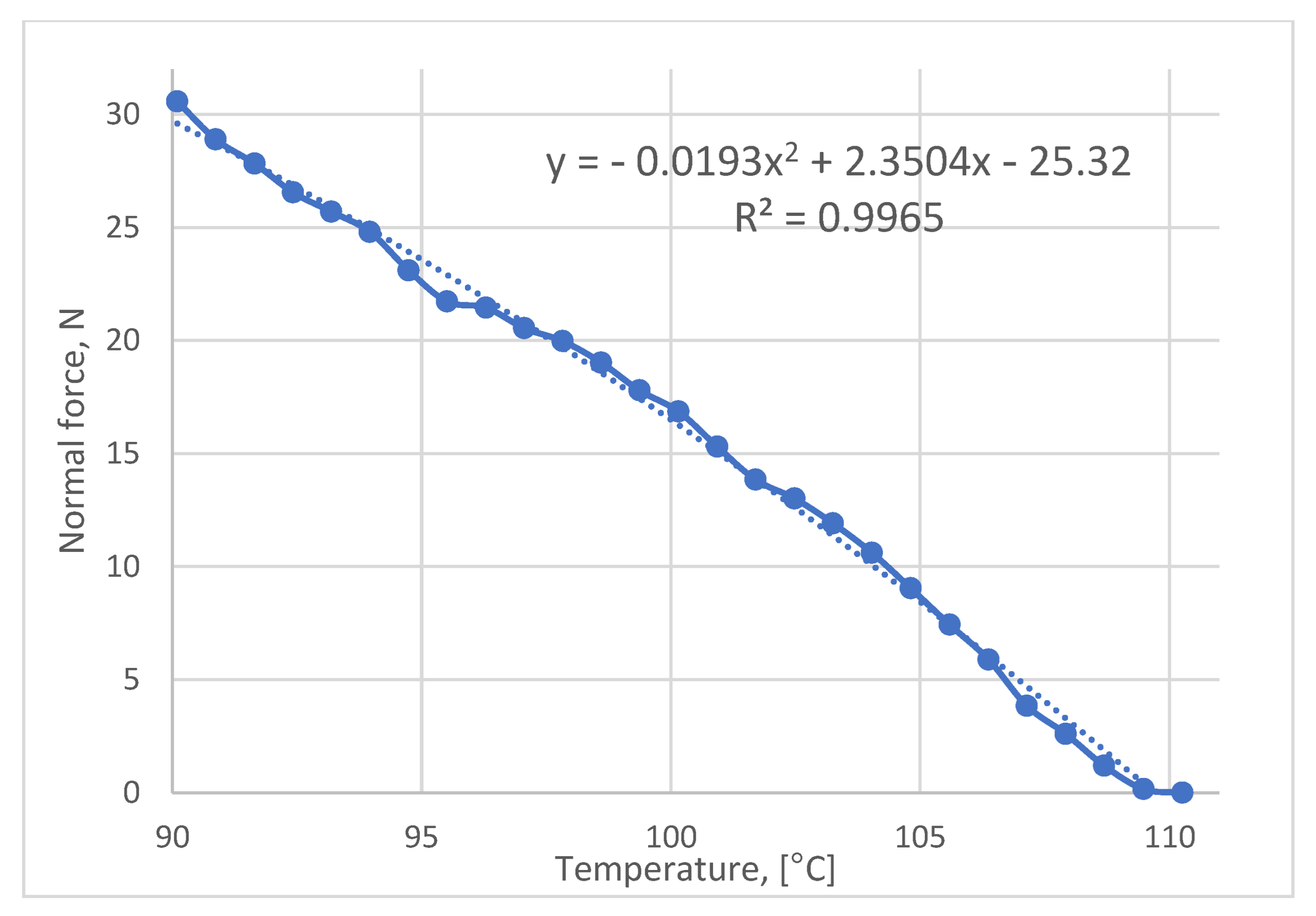
2.2. Assessment of Shrinkage Forces Due to Rapid Cooling Using a Rheometer
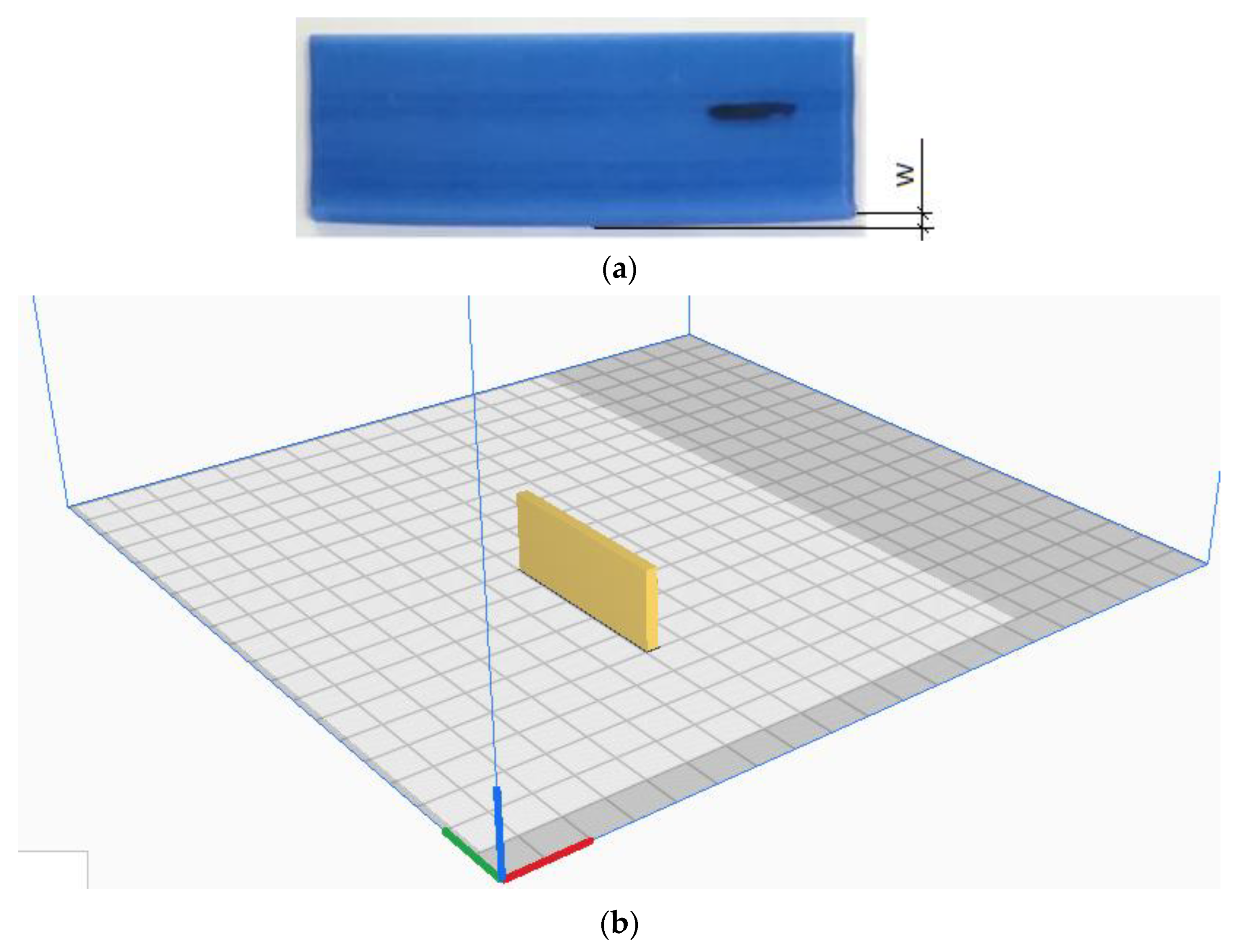
2.3. Design of Experiments for Identification of FFF Parameters Causing Warpage
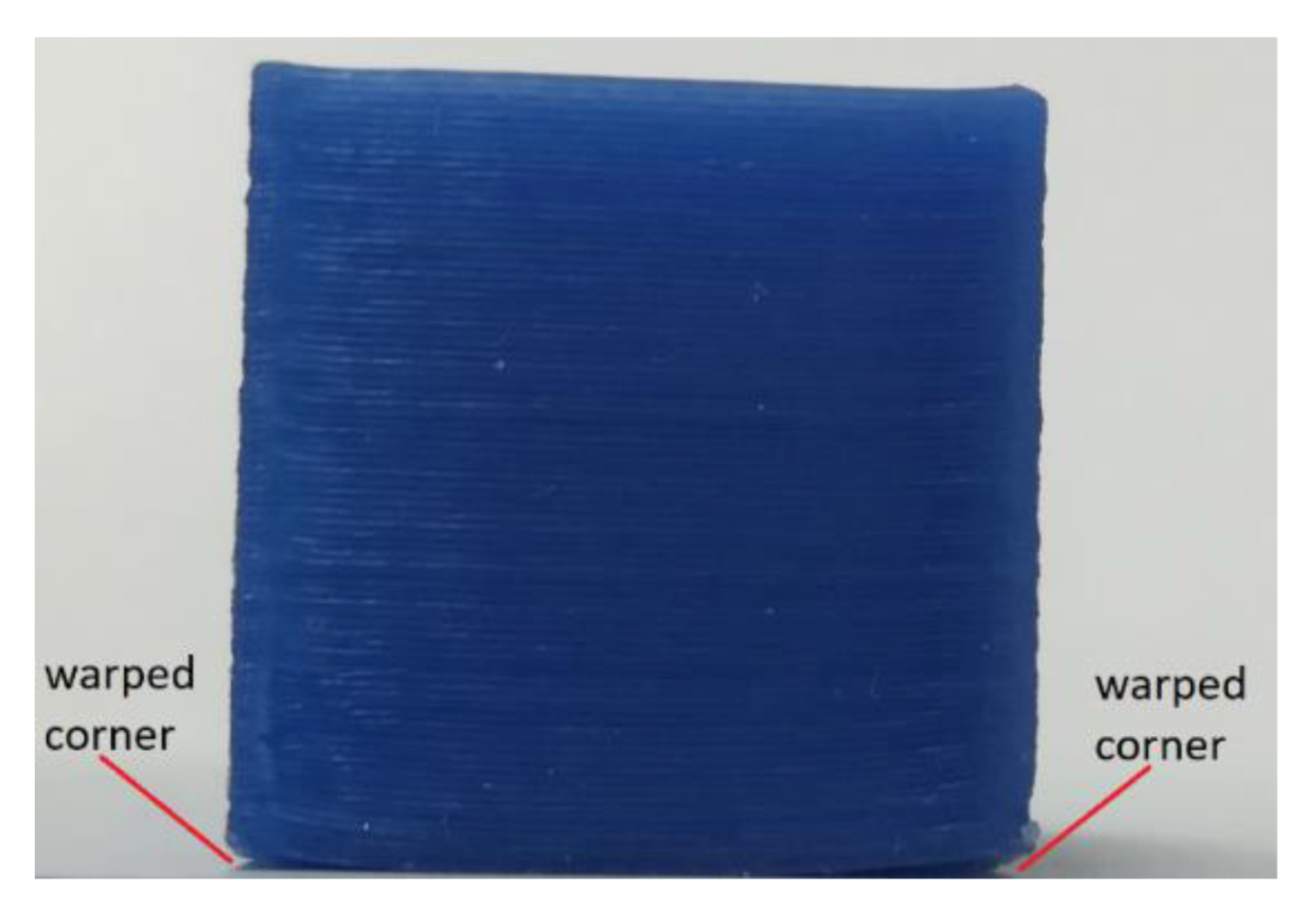
3. Results and Discussion
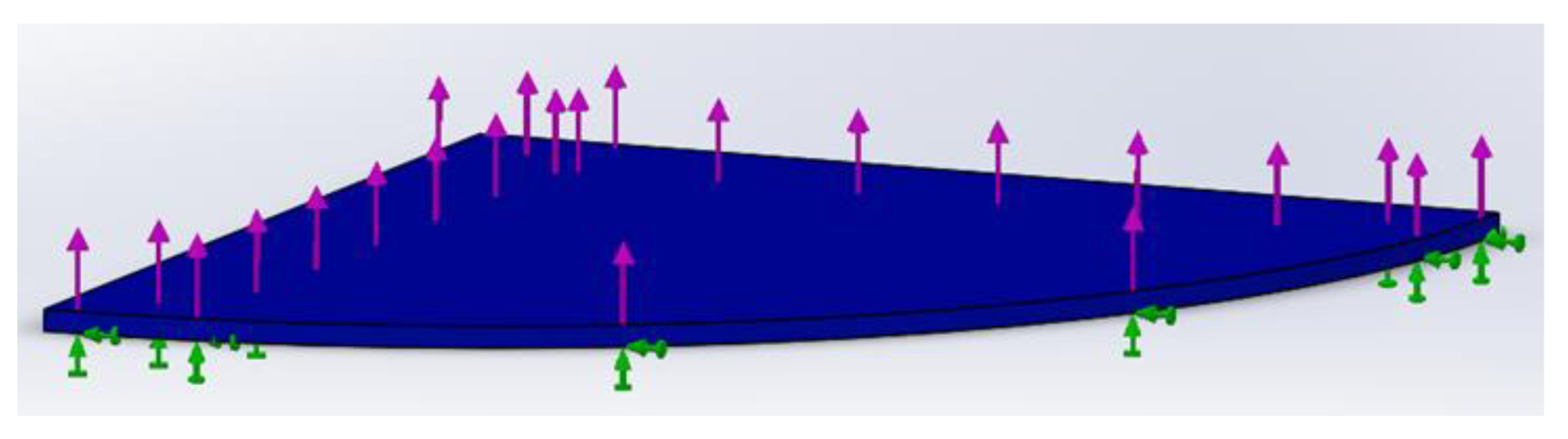
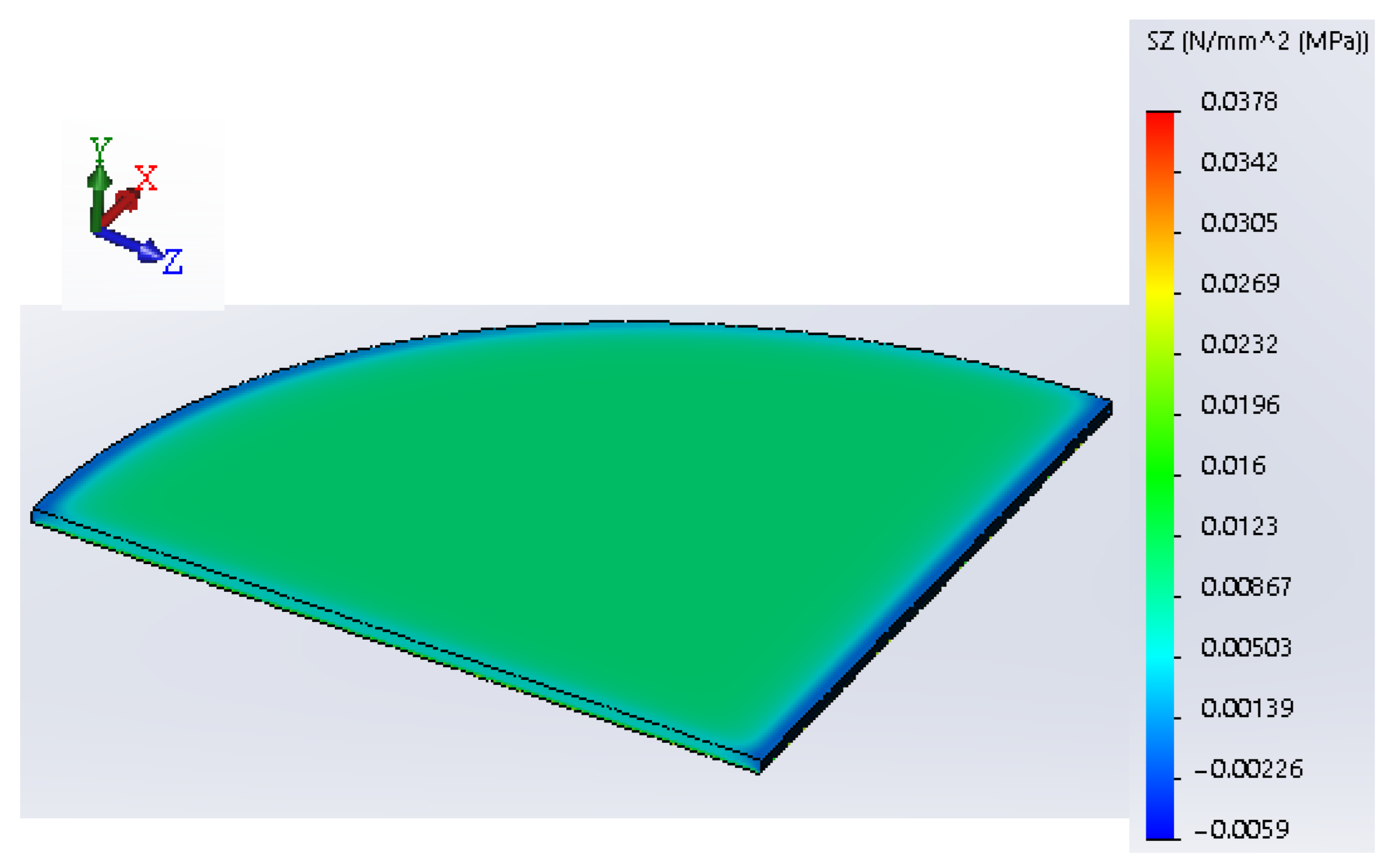
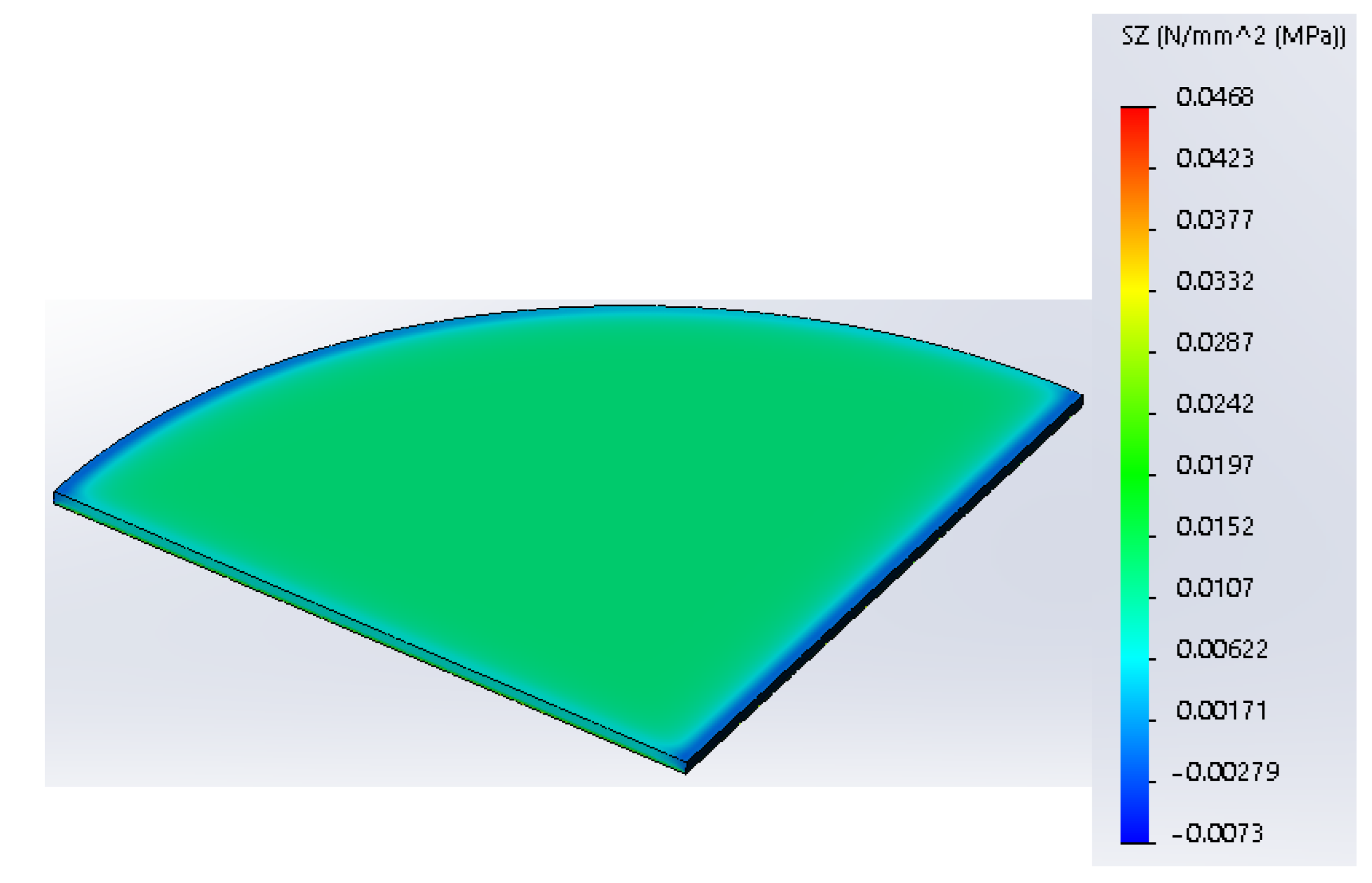
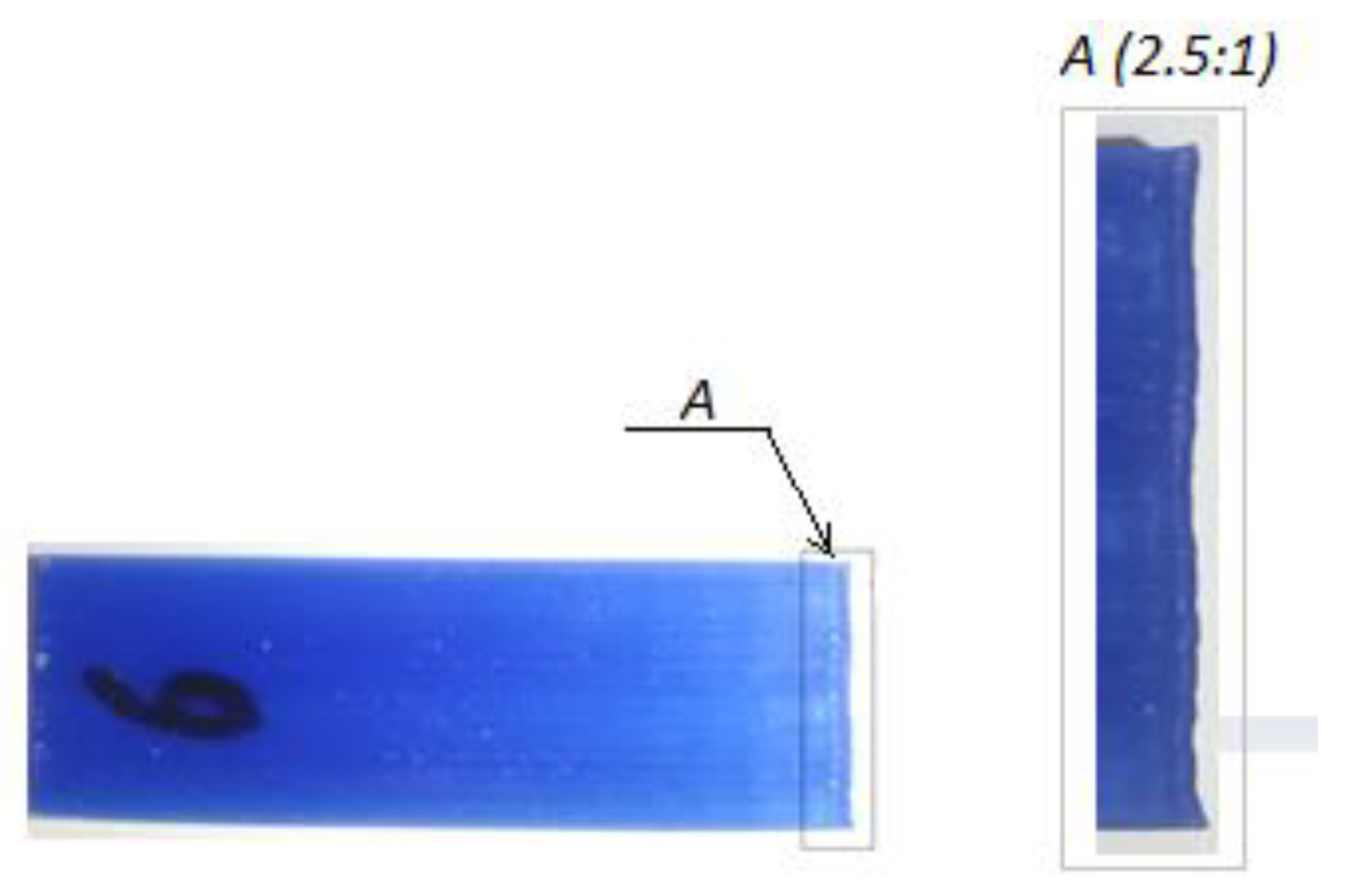
3.1. Identified Stresses Due to Thermal Shrinkage
- (a)
- The thermal effects including polymer relaxation were neglected. During solidification of the polymer, the mechanical properties change significantly. Although this type of transformation is neglected, it is assumed that all the stresses due to the shrinkage of the material accumulate as residual stresses in the solidified body.
- (b)
- The polymer is assumed to have isotropic properties.
- (c)
- During the FFF, the material is deposited in a layer-by-layer fashion. However, in FEA the entire body of thin film is assumed to be shrinking at once. Since it is observed that the warpage manifests with some delay in time for 3D printed parts, the stress accumulation within the entire body is likely the major factor for warpage occurrence.
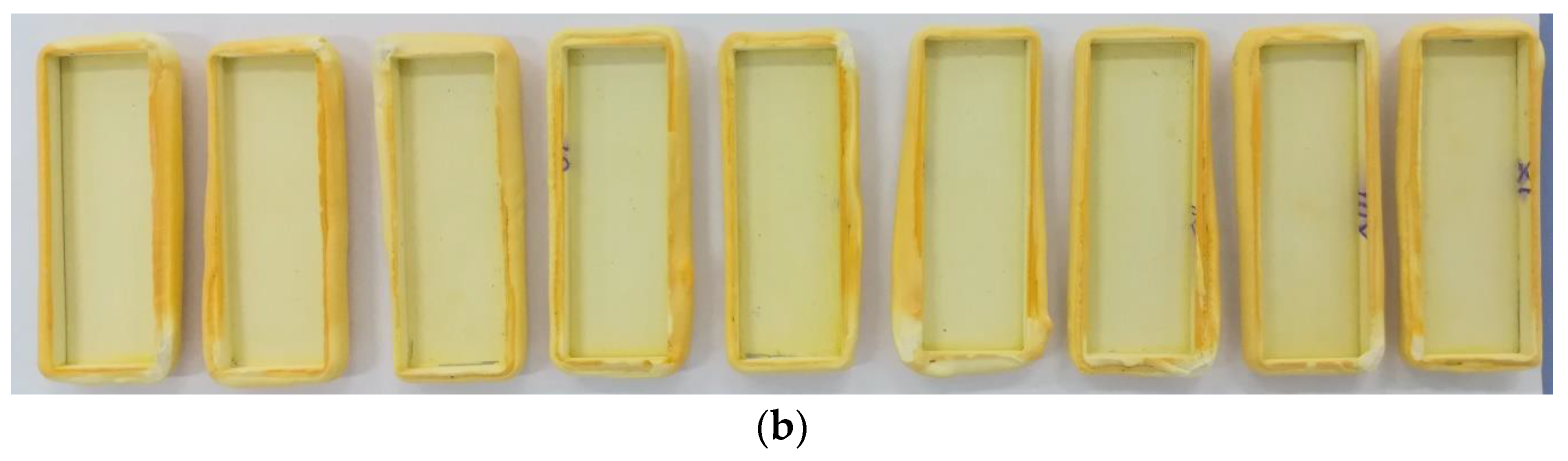
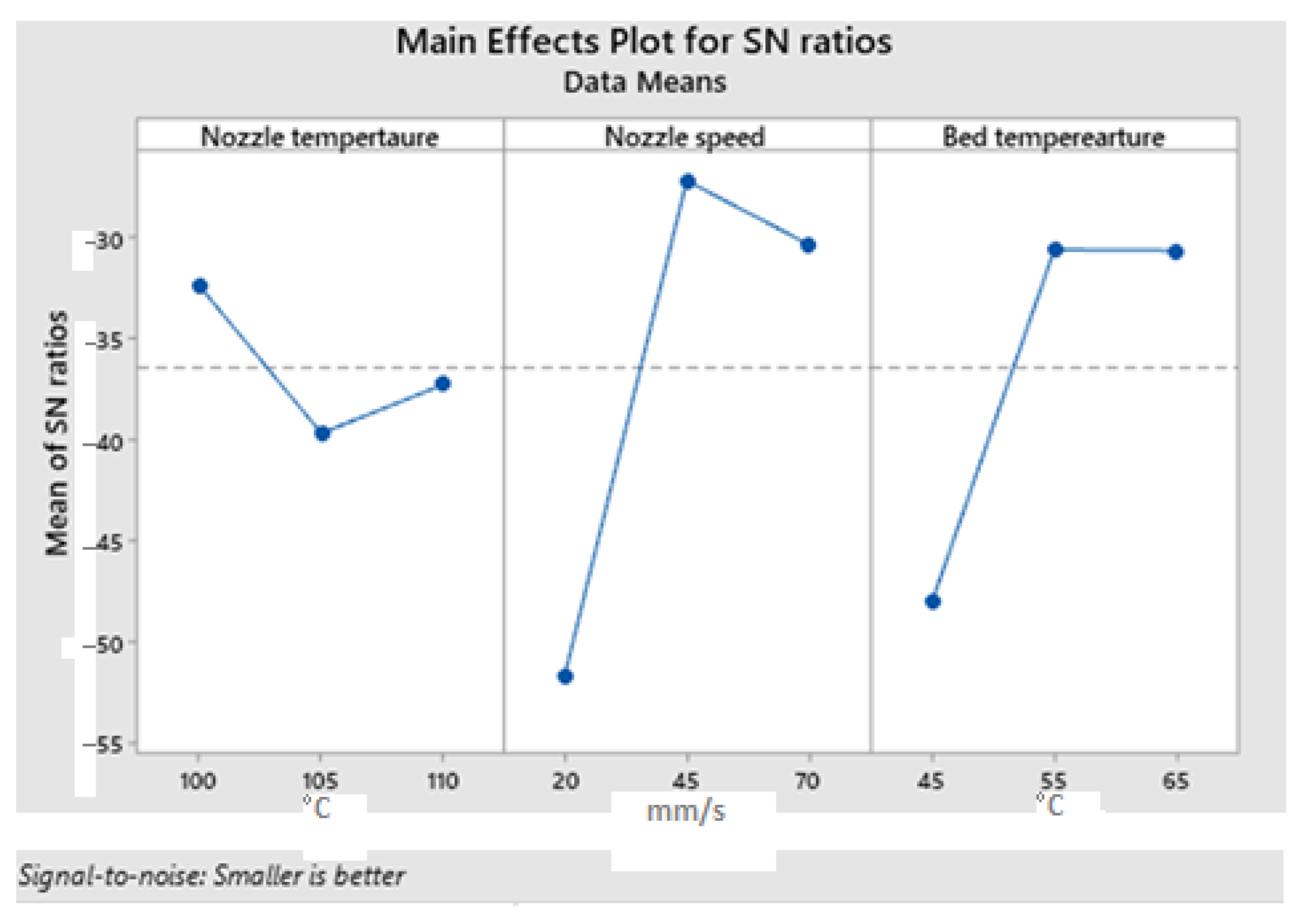
3.2. Results of Taguchi Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Chhabra, M.; Singh, R. Rapid casting solutions: A review. Rapid Prototyp. J. 2011, 17, 328–350. [Google Scholar] [CrossRef]
- Raoufi, M.A.; Bazaz, S.R.; Niazmand, H.; Rouhi, O.; Asadnia, M.; Razmjou, A.; Warkiani, M.E. Fabrication of unconventional inertial microfluidic channels using wax 3D printing. Soft Matter 2020, 16, 2448–2459. [Google Scholar] [CrossRef] [PubMed]
- Kuo, C.-C.; Tasi, Y.-R.; Chen, M.-Y.; Yan, Z.-Y. Development of a cost-effective technique for batch production of precision wax patterns using 3D optical inspection and rapid tooling technologies. Int. J. Adv. Manuf. Technol. 2021, 117, 3211–3227. [Google Scholar] [CrossRef]
- Mandolini, M.; Sartini, M.; Favi, C. Techno-Economic Analysis for Comparing Stereolithography and Wax Injection for Pattern Manufacturing in Investment Casting. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition, Columbus, OH, USA, 30 October 2022. [Google Scholar]
- Mukhtarkhanov, M.; Perveen, A.; Talamona, D. Application of Stereolithography Based 3D Printing Technology in Investment Casting. Micromachines 2020, 11, 946. [Google Scholar] [CrossRef] [PubMed]
- Badanova, N.; Perveen, A. Study of SLA Printing Parameters Affecting the Dimensional Accuracy of the Pattern and Casting in Rapid Investment Casting. J. Manuf. Mater. Process. 2022, 6, 109. [Google Scholar] [CrossRef]
- Wine, L.N. Applications of Investment Casting as a Manufacturing Process for Injection Mold Tooling. Bachelor’s Thesis, Massachusetts Institute of Technology, Boston, MA, USA, 2022. [Google Scholar]
- Mukhtarkhanov, M.; Shehab, E.; Ali, M. Process Parameter Optimization for 3D Printed Investment Casting Wax Pattern and Its Post-Processing Technique. Appl. Sci. 2022, 12, 6847. [Google Scholar] [CrossRef]
- Szabó, L.; Deák, G.; Nyul, D.; Kéki, S. Flexible Investment Casting Wax Patterns for 3D-Printing: Their Rheological and Mechanical Characterizations. Polymers 2022, 14, 4744. [Google Scholar] [CrossRef] [PubMed]
- Samy, A.A.; Golbang, A.; Harkin-Jones, E.; Archer, E.; Tormey, D.; McIlhagger, A. Finite element analysis of residual stress and warpage in a 3D printed semi-crystalline polymer: Effect of ambient temperature and nozzle speed. J. Manuf. Process. 2021, 70, 389–399. [Google Scholar] [CrossRef]
- Armillotta, A.; Bellotti, M.; Cavallaro, M. Warpage of FFF parts: Experimental tests and analytic model. Robot. Comput. Integr. Manuf. 2018, 50, 140–152. [Google Scholar] [CrossRef]
- Zhang, Y.; Chou, K. A parametric study of part distortions in fused deposition modelling using three-dimensional finite element analysis. Proc. Inst. Mech. Eng. B J. Eng. Manuf. 2008, 222, 959–968. [Google Scholar] [CrossRef]
- Syrlybayev, D.; Zharylkassyn, B.; Seisekulova, A.; Perveen, A.; Talamona, D. Optimization of the warpage of fused deposition modeling parts using finite element method. Polymers 2021, 13, 3849. [Google Scholar] [CrossRef] [PubMed]
- Kuo, C.-C.; Wu, Y.-R.; Li, M.-H.; Wu, H.-W. Minimizing warpage of ABS prototypes built with low-cost fused deposition modeling machine using developed closed-chamber and optimal process parameters. Int. J. Adv. Manuf. Technol. 2019, 101, 593–602. [Google Scholar] [CrossRef]
- Liu, X.; Li, S.; Liu, Z.; Zheng, X.; Chen, X.; Wang, Z. An investigation on distortion of PLA thin-plate part in the FFF process. Int. J. Adv. Manuf. Technol. 2015, 79, 1117–1126. [Google Scholar]
- Alsoufi, M.S.; Alhazmi, M.W.; Suker, D.K.; Alghamdi, T.A.; Sabbagh, R.A.; Felemban, M.A.; Bazuhair, F.K. Experimental characterization of the influence of nozzle temperature in FFF 3D printed pure PLA and advanced PLA+. Am. J. Mech. Eng. 2019, 7, 45–60. [Google Scholar] [CrossRef]
- Fitzharris, E.R.; Watanabe, N.; Rosen, D.W.; Shofner, M.L. Effects of material properties on warpage in fused deposition modeling parts. Int. J. Adv. Manuf. Technol. 2018, 95, 2059–2070. [Google Scholar] [CrossRef]
- Akbar, I.; El Hadrouz, M.; El Mansori, M.; Lagoudas, D. Continuum and subcontinuum simulation of FFF process for 4D printed shape memory polymers. J. Manuf. Process. 2022, 76, 335–348. [Google Scholar] [CrossRef]
- Verma, N.; Awasthi, P.; Gupta, A.; Banerjee, S.S. Fused Deposition Modeling of Polyolefins: Challenges and Opportunities. Macromol. Mater. Eng. 2023, 308, 2200421. [Google Scholar] [CrossRef]
- Das, A.; Gilmer, E.L.; Biria, S.; Bortner, J. Importance of Polymer Rheology on Material Extrusion Additive Manufacturing: Correlating Process Physics to Print Properties. ACS Appl. Polym. Mater. 2021, 3, 1218–1249. [Google Scholar] [CrossRef]
- Li, Z.; Liu, Y.; Liang, Z.; Liu, Y. The Influence of Fused Deposition Modeling Parameters on the Properties of PA6/PA66 Composite Specimens by the Taguchi Method and Analysis of Variance. 3D Print. Addit. Manuf. 2023. ahead of print. [Google Scholar] [CrossRef]
- Mukhtarkanov, M.; Shehab, E.; Tanveer, M.H.; Araby, S.; Ali, M.H. Investigation of Multi-material Composite Parts Manufactured by Multi-extrusion FFF Printer. In Flexible Automation and Intelligent Manufacturing: The Human-Data-Technology Nexus, Proceedings of FAIM 2022, Detroit, MI, USA, 19–23 June 2022; Lecture Notes in Mechanical Engineering; Kim, K.Y., Monplaisir, L., Rickli, J., Eds.; Springer: Cham, Switzerland, 2022. [Google Scholar]
- Yin, J.; Lu, C.; Fu, J.; Huang, Y.; Zheng, Y. Interfacial bonding during multi-material fused deposition modeling (FFF) process due to inter-molecular diffusion. Mater. Des. 2018, 150, 104–112. [Google Scholar] [CrossRef]
- Kwon, N.; Deshpande, H.; Hasan, M.K.; Darnal, A.; Kim, J. Multi-Ttach: Techniques to Enhance Multi-Material Attachments in Low-cost FFF 3D Printing. In Proceedings of the SCF 2021: ACM Symposium on Computational Fabrication, Virtual Event, 28 October 2021. [Google Scholar]
- Gao, X.; Zhang, D.; Qi, S.; Wen, X.; Su, Y. Mechanical properties of 3D parts fabricated by fused deposition modeling: Effect of various fillers in polylactide. J. Appl. Polym. Sci. 2019, 136, 47824. [Google Scholar] [CrossRef]
- Dawoud, M.; Taha, I.; Ebeid, S.J. Mechanical behavior of ABS: An experimental study using FFF and injection molding techniques. J. Manuf. Process. 2016, 21, 39–45. [Google Scholar] [CrossRef]
- Ansari, A.A.; Kamil, M. Effect of print speed and extrusion temperature on properties of 3D printed PLA using fused deposition modeling process. Mater. Today Proc. 2021, 45, 5462–5468. [Google Scholar] [CrossRef]


| Material | Part Geometry | Experimental | Analytical/Numerical | Significant Factors Affecting Warpage | Ref. |
|---|---|---|---|---|---|
| Acrylonitrile Butadiene Styrene (ABS) | Rectangular plate | + | + | Length of the part, thickness Layer thickness | [11] |
| Thin and thickplates | + | + | Print speed, layer thickness | [12] | |
| Tensile specimen | + | + | Layer thickness | [13] | |
| Rectangular plate | + | Bed and chamber temperature | [14] | ||
| Polylactic acid (PLA) | Thin plate | + | Layer thickness | [15] | |
| Rectangular block | + | Nozzle temperature | [16] | ||
| Polyphenylene sulfide (PPS) | Thin plate | + | + | Coefficient of thermal expansion | [17] |
| Polyurethane-based shape memory polymer | Thin plate | + | + | Bed temperature, printing speed, layer thickness | [18] |
| Input Parameters | Symbol | Level 1 | Level 2 | Level 3 |
|---|---|---|---|---|
| Nozzle temperature, °C | I | 100 | 105 | 110 |
| Nozzle speed, mm/s | II | 20 | 45 | 70 |
| Bed temperature, °C | III | 45 | 55 | 65 |
| Temperature Range, [C] | Thermal Stress, σw [MPa] | Max Stress Magnitude Found by the FEA, [MPa] |
|---|---|---|
| 110–90 | 0.94 | 0.037 |
| 110–40 | 1.17 | 0.046 |
| Level | Nozzle Temperature | Nozzle Speed | Bed Temperature |
|---|---|---|---|
| 1 | −32.36 | −51.78 | −48.07 |
| 2 | −39.69 | −27.19 | −30.61 |
| 3 | −37.27 | −30.36 | −30.65 |
| Delta | 7.33 | 24.59 | 17.47 |
| Rank | 3 | 1 | 2 |
| Source | DF | Adj SS | Adj MS | F | p | Significance | Contribution |
|---|---|---|---|---|---|---|---|
| Nozzle temperature | 2 | 83.76 | 41.88 | 1.65 | 0.378 | Nonsignificant | 4 |
| Nozzle speed | 2 | 1073.55 | 536.78 | 21.10 | 0.045 | Significant | 59 |
| Bed temperature | 2 | 608.53 | 304.27 | 11.96 | 0.077 | Nonsignificant | 32 |
| Residual Error | 2 | 50.89 | 25.44 | ||||
| Total | 8 | 1816.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mukhtarkhanov, M.; Shehab, E.; Ali, M.H. Experimental Study on Warpage Phenomenon of Wax Parts Manufactured by Fused Filament Fabrication. Polymers 2024, 16, 208. https://doi.org/10.3390/polym16020208
Mukhtarkhanov M, Shehab E, Ali MH. Experimental Study on Warpage Phenomenon of Wax Parts Manufactured by Fused Filament Fabrication. Polymers. 2024; 16(2):208. https://doi.org/10.3390/polym16020208
Chicago/Turabian StyleMukhtarkhanov, Muslim, Essam Shehab, and Md. Hazrat Ali. 2024. "Experimental Study on Warpage Phenomenon of Wax Parts Manufactured by Fused Filament Fabrication" Polymers 16, no. 2: 208. https://doi.org/10.3390/polym16020208
APA StyleMukhtarkhanov, M., Shehab, E., & Ali, M. H. (2024). Experimental Study on Warpage Phenomenon of Wax Parts Manufactured by Fused Filament Fabrication. Polymers, 16(2), 208. https://doi.org/10.3390/polym16020208







