An Insight into the Mechanical Properties of Unidirectional C/C Composites Considering the Effect of Pore Microstructures via Numerical Calculation
Abstract
1. Introduction
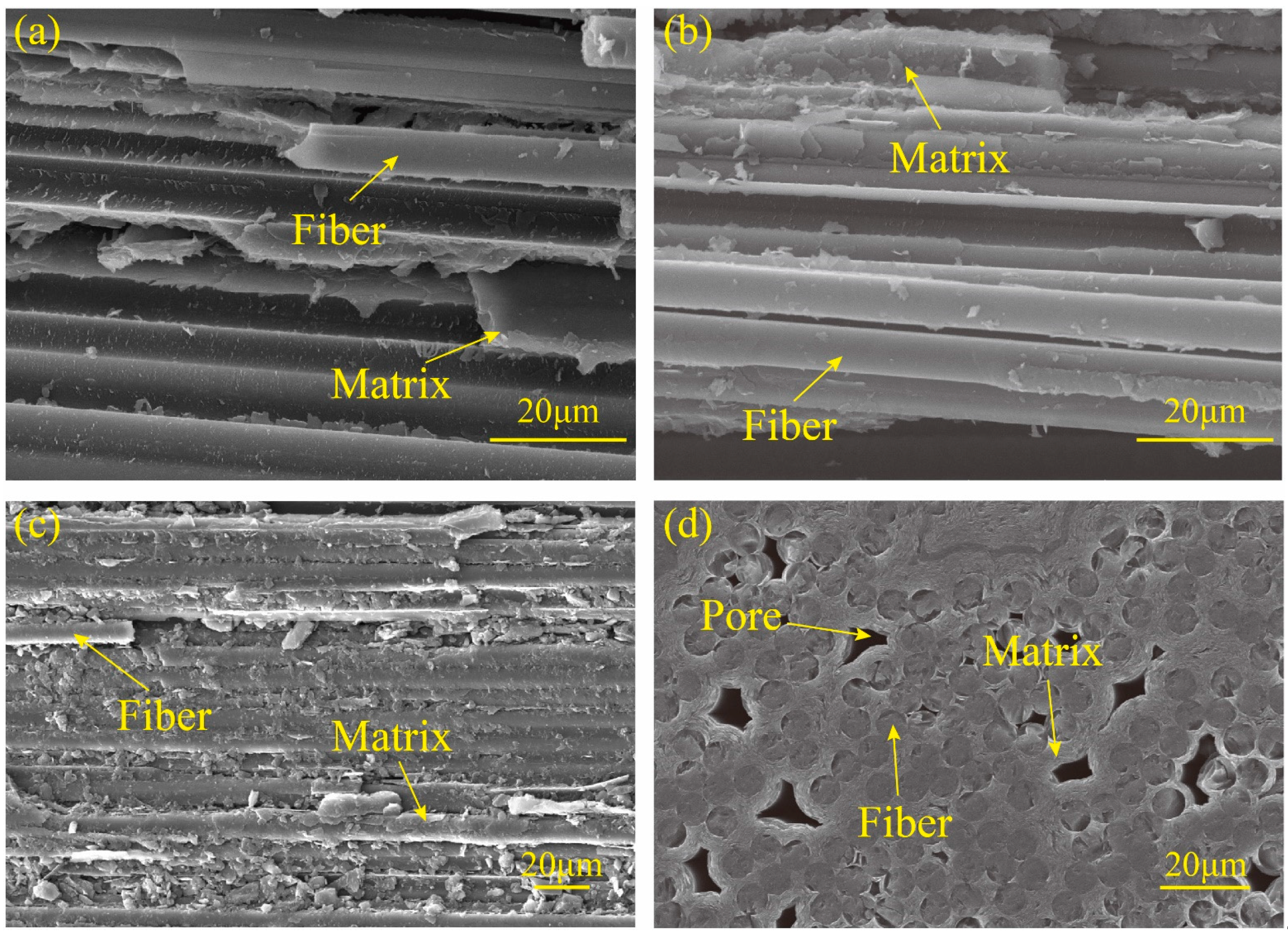
2. Experimental Section
3. Simulation Models and Methods
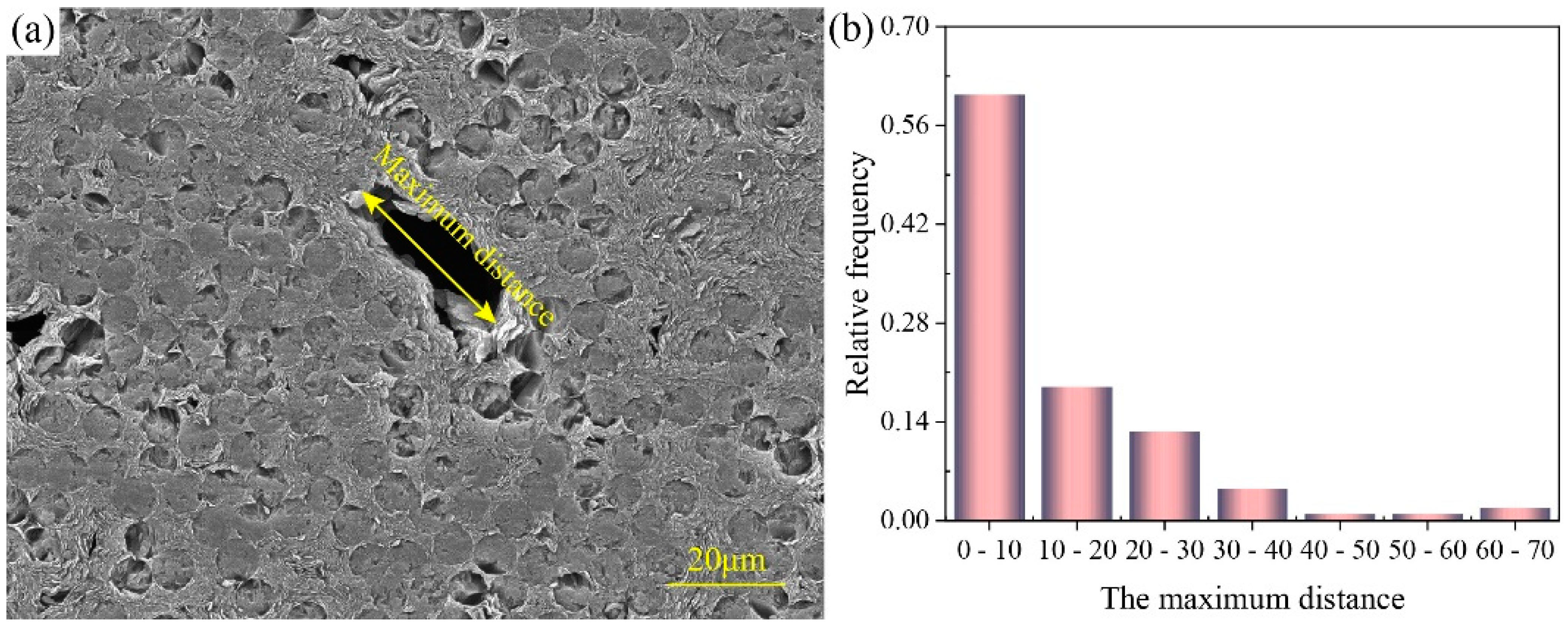
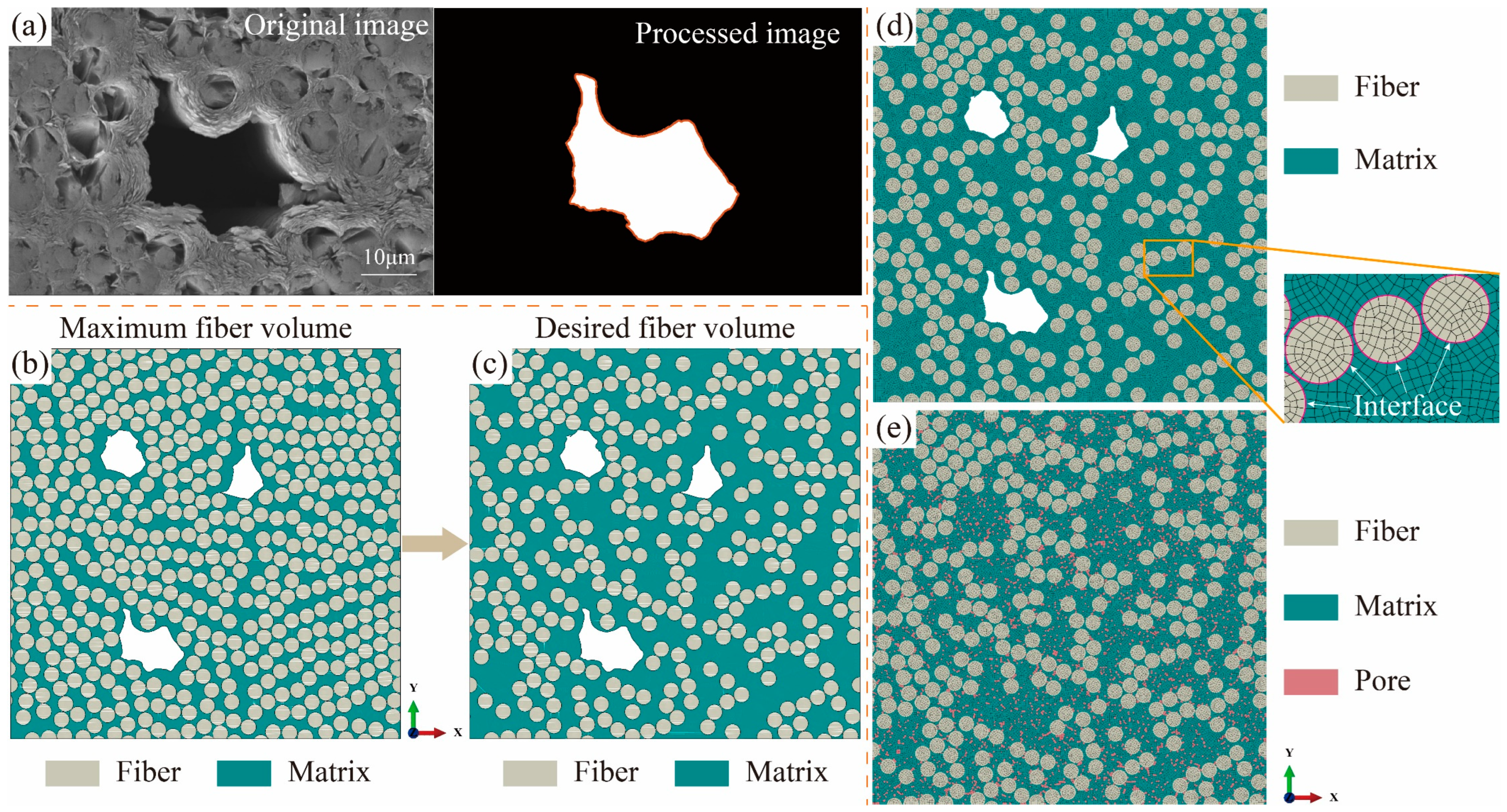
3.1. Generation of the Geometric Model
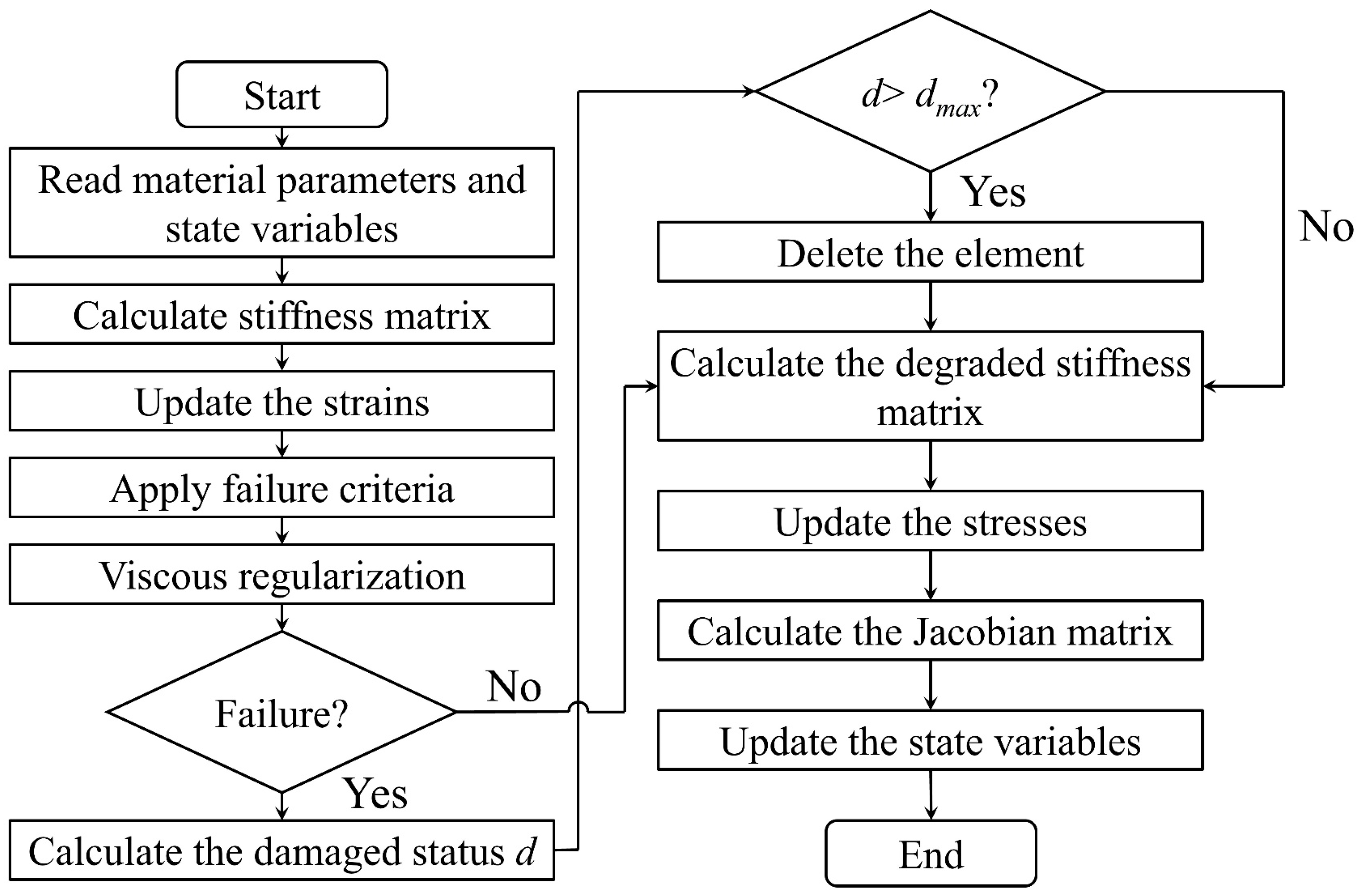
3.2. Constitutive Material
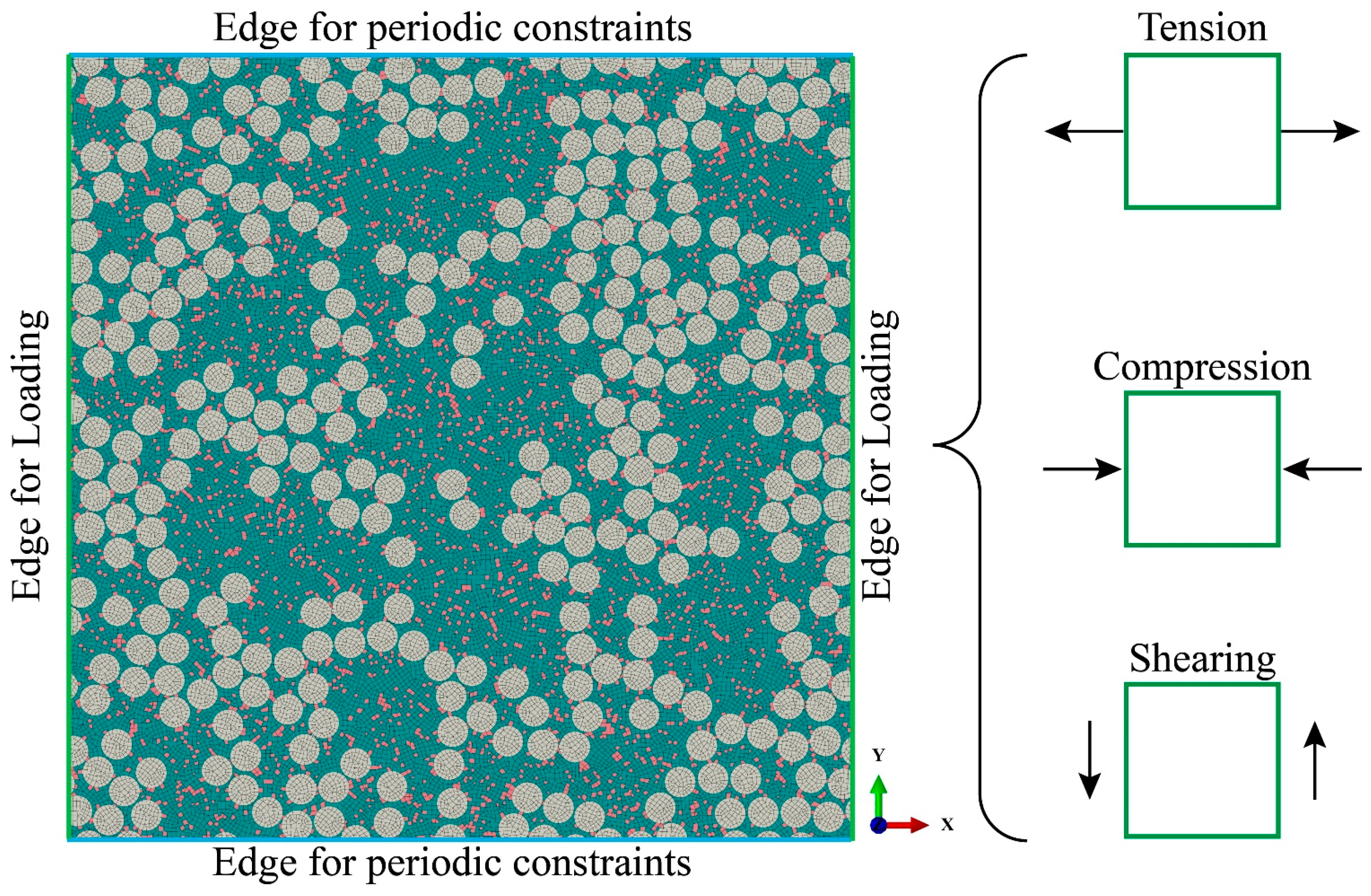
3.3. Boundary Conditions
4. Mesh Sensitivity
5. Model Validation
6. Results and Discussion
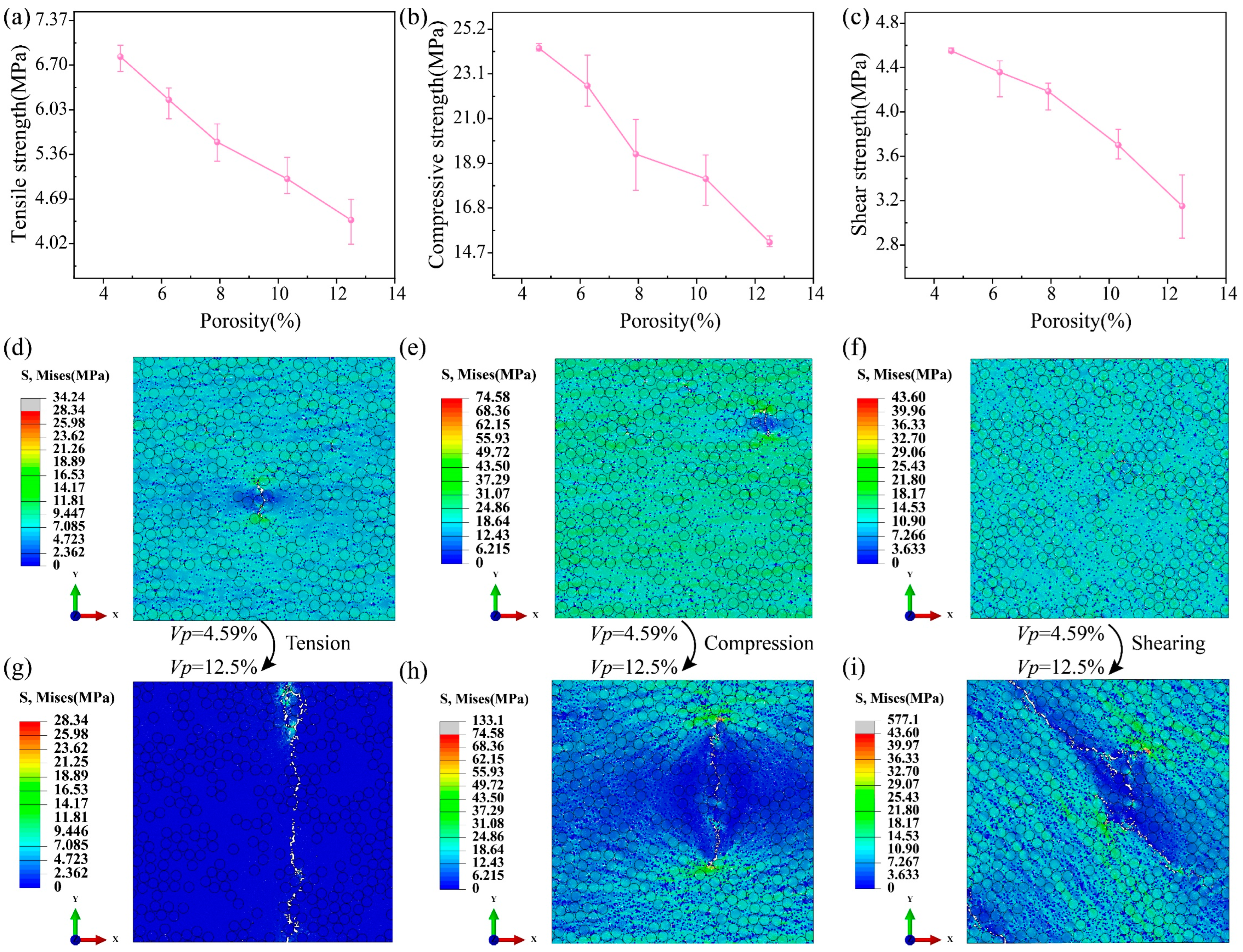
6.1. Effects of Porosities
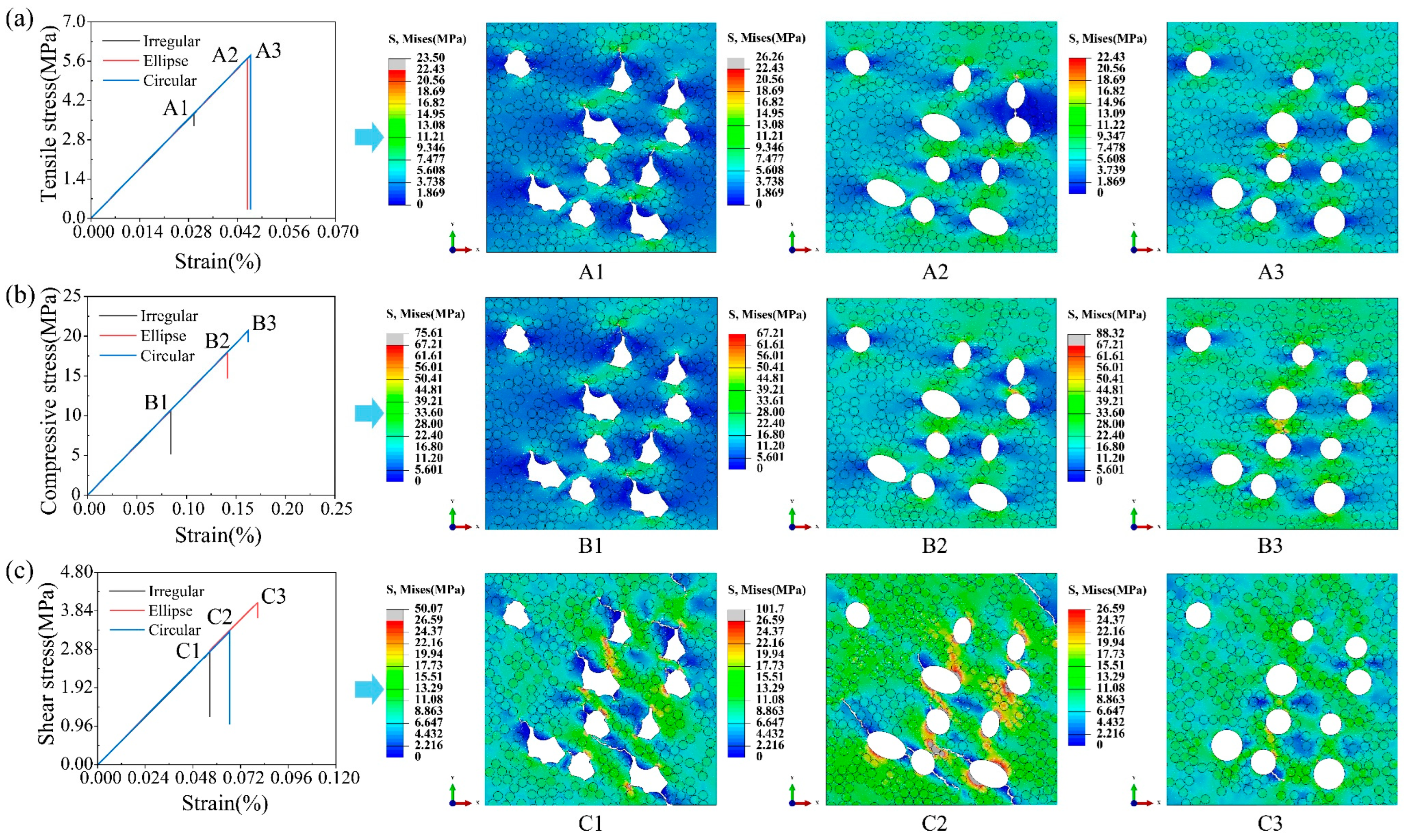
6.2. Effects of Pore Shapes
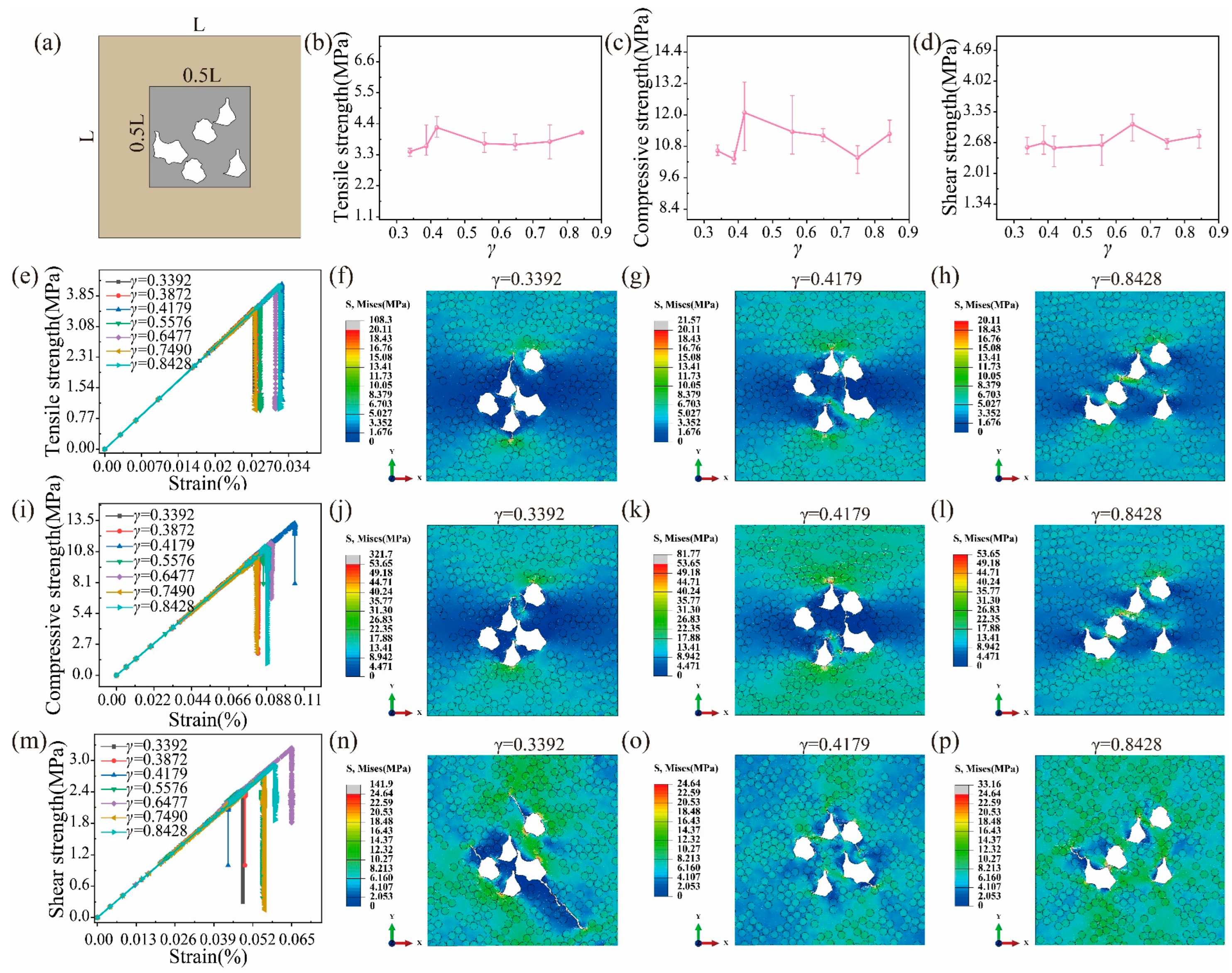
6.3. Effects of Pore Locations
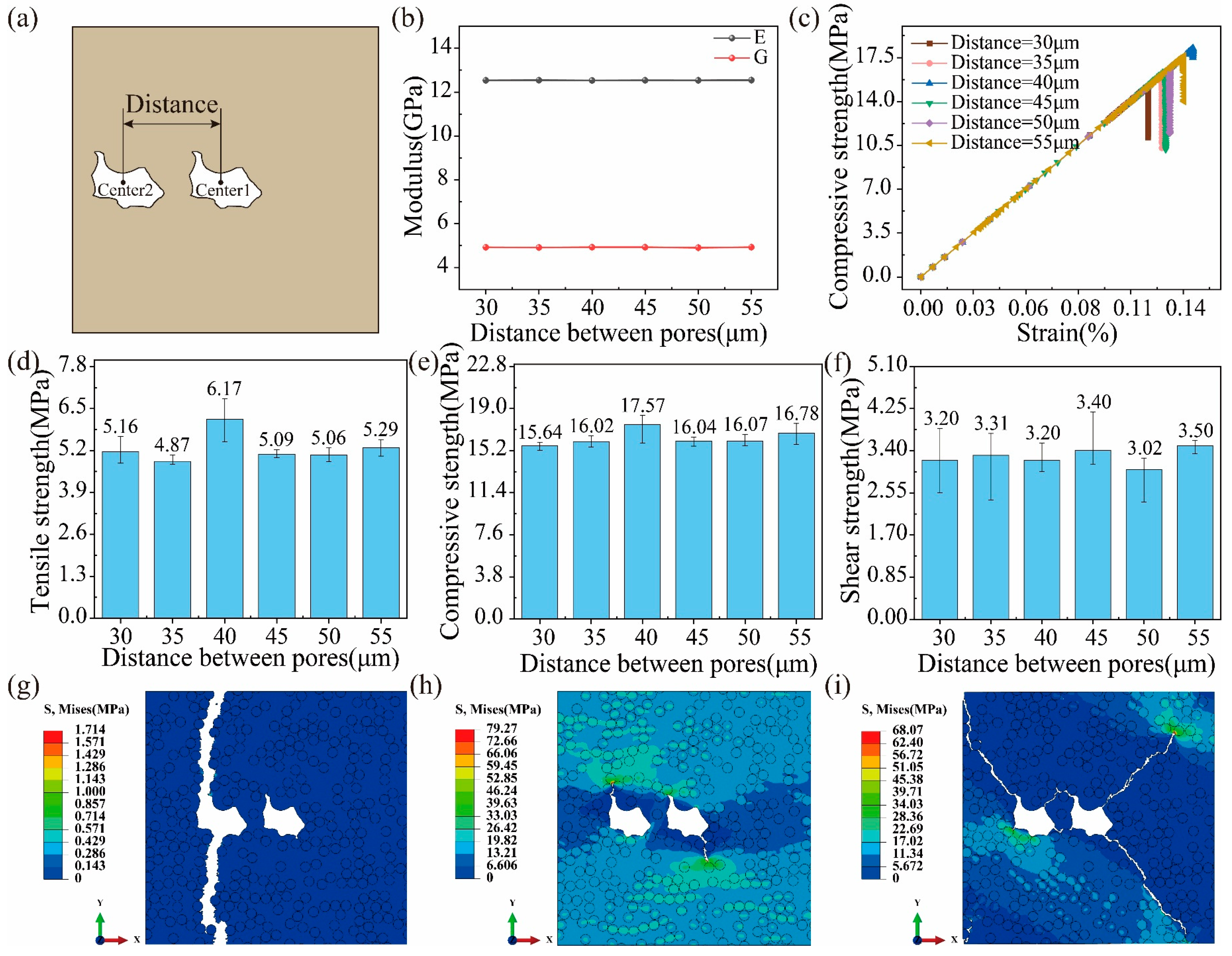
6.4. Effects of the Distance between Pores
6.5. Effects of Pore Clustering
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, P.; Zhang, S.; Li, H.; Kong, J.; Li, W.; Zaman, W. Variation of thermal expansion of carbon/carbon composites from 850 to 2500 °C. Ceram. Int. 2014, 40, 1273–1276. [Google Scholar] [CrossRef]
- Aly-Hassan, M.S.; Hatta, H.; Wakayama, S.; Watanabe, M.; Miyagawa, K. Comparison of 2D and 3D carbon/carbon composites with respect to damage and fracture resistance. Carbon 2003, 41, 1069–1078. [Google Scholar] [CrossRef]
- Gillard, A.P.; Couégnat, G.; Chupin, S.; Vignoles, G.L. Modeling of the non-linear mechanical and thermomechanical behavior of 3D carbon/carbon composites based on internal interfaces. Carbon 2019, 154, 178–191. [Google Scholar] [CrossRef]
- Rodríguez-Mirasol, J.; Thrower, P.A.; Radovic, L.R. On the oxidation resistance of C/C composites obtained by liquid-phase impregnation/carbonization of different carbon cloths. Carbon 1993, 31, 789–799. [Google Scholar] [CrossRef]
- Zhang, W.G.; Hüttinger, K.J. Densification of a 2D carbon fiber preform by isothermal, isobaric CVI: Kinetics and carbon microstructure. Carbon 2003, 41, 2325–2337. [Google Scholar] [CrossRef]
- Li, K.; Li, H.; Li, N.; Song, Q.; Qi, L. Role of hydrogen in the dissociation of CH4 on different graphene by DFT study. Chin. J. Aeronaut. 2022, 35, 407–419. [Google Scholar] [CrossRef]
- Benzinger, W.; Hüttinger, K.J. Chemistry and kinetics of chemical vapor infiltration of pyrocarbon—VI. Mechanical and structural properties of infiltrated carbon fiber felt. Carbon 1999, 37, 1311–1322. [Google Scholar] [CrossRef]
- Ahmad, W.; Zaka, U.; Sonil, N.I.; Khan, K. Introduction, production, characterization and applications of defects in graphene. J. Mater. Sci. Mater. Electron. 2021, 32, 19991–20030. [Google Scholar] [CrossRef]
- Yoshikawa, N. Modeling of Chemical Vapor Infiltration Rate Considering a Pore Size Distribution. J. Am. Ceram. Soc. 2002, 85, 1485–1491. [Google Scholar] [CrossRef]
- Hatta, H.; Suzuki, K.; Shigei, T.; Somiya, S.; Sawada, Y. Strength improvement by densification of C/C composites. Carbon 2001, 39, 83–90. [Google Scholar] [CrossRef]
- Yan, K.; Zhang, C.; Qiao, S.; Han, D.; Li, M. Charpy Impact Properties of C/C and C/SiC Composties. J. Aeronaut. Mater. 2011, 31, 95–98. [Google Scholar]
- Li, Z.; Liu, Z.; Lei, Z.; Zhu, P. An innovative computational framework for the analysis of complex mechanical behaviors of short fiber reinforced polymer composites. Compos. Struct. 2021, 277, 114594. [Google Scholar] [CrossRef]
- Tian, Z.; Yan, Y.; Ye, J.; Hong, Y.; Li, X. An analytical constitutive model for progressive damage analysis of three-dimensional braided composites. Polym. Compos. 2018, 39, 4188–4204. [Google Scholar] [CrossRef]
- Heidari-Rarani, M.; Bashandeh-Khodaei-Naeini, K.; Mirkhalaf, S.M. Micromechanical modeling of the mechanical behavior of unidirectional composites—A comparative study. J. Reinf. Plast. Compos. 2018, 37, 1051–1071. [Google Scholar] [CrossRef]
- Ye, J.; Hong, Y.; Liu, L.; Cai, H.; He, W.; Huang, B.; Saafi, M.; Wang, Y.; Ye, J. Microscale damage evolutions in fiber-reinforced composites with different initial defects. Compos. Struct. 2022, 279, 114856. [Google Scholar] [CrossRef]
- Zhao, S.; Xue, P. Continuum description of damage and failure of composite laminates based on viscous regularization. Multidiscip. Model. Mater. Struct. 2014, 10, 525–536. [Google Scholar] [CrossRef]
- Lin, S.; Yang, L.; Xu, H.; Jia, X.; Yang, X.; Zu, L. Progressive damage analysis for multiscale modelling of composite pressure vessels based on Puck failure criterion. Compos. Struct. 2021, 255, 113046. [Google Scholar] [CrossRef]
- Hyde, A.; He, J.; Cui, X.; Lua, J.; Liu, L. Effects of microvoids on strength of unidirectional fiber-reinforced composite materials. Compos. Part B Eng. 2020, 187, 107844. [Google Scholar] [CrossRef]
- Vajari, D.A.; González, C.; Llorca, J.; Legarth, B.N. A numerical study of the influence of microvoids in the transverse mechanical response of unidirectional composites. Compos. Sci. Technol. 2014, 97, 46–54. [Google Scholar] [CrossRef]
- He, C.; Ge, J.; Cao, X.; Chen, Y.; Chen, H.; Fang, D. The effects of fiber radius and fiber shape deviations and of matrix void content on the strengths and failure mechanisms of UD composites by computational micromechanics. Compos. Sci. Technol. 2022, 218, 109139. [Google Scholar] [CrossRef]
- Qi, L.; Chao, X.; Tian, W.; Ma, W.; Li, H. Numerical study of the effects of irregular pores on transverse mechanical properties of unidirectional composites. Compos. Sci. Technol. 2018, 159, 142–151. [Google Scholar] [CrossRef]
- Ali, J.; Berre, C.; Mummery, P.M. Image based modelling of stress–strain behaviour in carbon/carbon composites. Energy Mater. 2006, 1, 179–186. [Google Scholar] [CrossRef]
- Ai, S.; Fang, D.; He, R.; Pei, Y. Effect of manufacturing defects on mechanical properties and failure features of 3D orthogonal woven C/C composites. Compos. Part B Eng. 2015, 71, 113–121. [Google Scholar]
- Xu, J.; Lu, X.; Zhu, X. Effect of Random Void Defects on the Mechanical Behavior of C/C Braided Composites. Adv. Eng. Mater. 2018, 20, 1701164. [Google Scholar] [CrossRef]
- Chao, X.; Qi, L.; Cheng, J.; Tian, W.; Zhang, S.; Li, H. Numerical evaluation of the effect of pores on effective elastic properties of carbon/carbon composites. Compos. Struct. 2018, 196, 108–116. [Google Scholar] [CrossRef]
- ASTM-C830; Standard Test Methods for Apparent Porosity, Liquid Absorption, Apparent Specific Gravity, and Bulk Density of Refractory Shapes By Vacuum Pressure. ASTM International: West Conshohocken, PA, USA, 2011.
- ASTM-C1275; Standard Test Method for Monotonic Tensile Behavior of Continuous Fiber-Reinforced Advanced Ceramics with Solid Rectangular Cross-Section Test Specimens at Ambient Temperature. ASTM International: West Conshohocken, PA, USA, 2018.
- DIN EN 658-2; Advanced Technical Ceramics—Mechanical Properties of Ceramic Composites at Room Temperature—Part 2: Determination of Compression Properties. Deutsches Institut für Normung: Berlin, Germany, 2003.
- ASTM-D3846; Standard Test Method for In-Plane Shear Strength of Reinforced Plastics. ASTM International: West Conshohocken, PA, USA, 2015.
- Drach, B.; Tsukrov, I.; Gross, T.; Dietrich, S.; Weidenmann, K.; Piat, R.; Böhlke, T. Numerical modeling of carbon/carbon composites with nanotextured matrix and 3D pores of irregular shapes. Int. J. Solids Struct. 2011, 48, 2447–2457. [Google Scholar] [CrossRef]
- Herráez, M.; González, C.; Lopes, C.S.; de Villoria, R.G.; LLorca, J.; Varela, T.; Sánchez, J. Computational micromechanics evaluation of the effect of fibre shape on the transverse strength of unidirectional composites: An approach to virtual materials design. Compos. Part A Appl. Sci. Manuf. 2016, 91, 484–492. [Google Scholar] [CrossRef]
- Ge, J.; Qi, L.; Chao, X.; Xue, Y.; Hou, X.; Li, H. The effects of interphase parameters on transverse elastic properties of Carbon–Carbon composites based on FE model. Compos. Struct. 2021, 268, 113961. [Google Scholar] [CrossRef]
- Li, X.; Jia, Y.; Hong, R. Comparison Between the Stress Form and Strain Form of Hashin Criteria in Progressive Failure Analysis of Composite Materials. J. Mech. Eng. 2022, 58, 284–293. [Google Scholar]
- Kan, J. Investigation on Characterization of Micro and Meso Structures and Their Influence on Effective Properties of Carbon/carbon Composites; Harbin Institute of Technology: Harbin, China, 2010. (In Chinese) [Google Scholar]
- He, X. Mechanical Properties of Kinds of Carbon Materials at Elevated Temperature; Northwestern Polytechnical University: Xi’an, China, 2009. (In Chinese) [Google Scholar]
- Warren, K.C.; Lopez-Anido, R.A.; Vel, S.S.; Bayraktar, H.H. Progressive failure analysis of three-dimensional woven carbon composites in single-bolt, double-shear bearing. Compos. Part B Eng. 2016, 84, 266–276. [Google Scholar] [CrossRef]
- Benzeggagh, M.L.; Kenane, M. Measurement of mixed-mode delamination fracture toughness of unidirectional glass/epoxy composites with mixed-mode bending apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]
- Alabbad, M.; Vel, S.S.; Lopez-Anido, R.A. Computational model for predicting the low-velocity impact resistance and tolerance of composite laminates. Compos. Part B Eng. 2022, 244, 110187. [Google Scholar] [CrossRef]
- Chao, X.; Qi, L.; Tian, W.; Yang, K.; Li, H. Evaluation for interfacial fracture of fiber-reinforced pyrocarbon matrix composites by using a zero-thickness cohesive approach. J. Alloys Compd. 2020, 820, 153378. [Google Scholar] [CrossRef]
- Kogo, Y.; Hatta, H.; Kawada, H.; Machida, T. Effect of Stress Concentration on Tensile Fracture Behavior of Carbon-Carbon Composites. J. Compos. Mater. 1998, 32, 1273–1294. [Google Scholar] [CrossRef]
- Wang, J.; Qian, J.; Jin, Z.; Qiao, G. Microstructure of C/C composites prepared by chemical vapor infiltration method with vaporized kerosene as a precursor. Mater. Sci. Eng. A 2006, 419, 162–167. [Google Scholar] [CrossRef]


| Predicted Result | Experimental Result | Deviation | |
|---|---|---|---|
| Tensile strength | 5.14 MPa | 3.94 ± 0.21 MPa | 1.2 MPa |
| Compressive strength | 19.94 MPa | 21.0 ± 0.84 MPa | 1.06 MPa |
| Shear strength | 4.58 MPa | 6.78 ± 0.7 MPa | 2.2 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ge, J.; Chao, X.; Tian, W.; Li, W.; Qi, L. An Insight into the Mechanical Properties of Unidirectional C/C Composites Considering the Effect of Pore Microstructures via Numerical Calculation. Polymers 2024, 16, 2577. https://doi.org/10.3390/polym16182577
Ge J, Chao X, Tian W, Li W, Qi L. An Insight into the Mechanical Properties of Unidirectional C/C Composites Considering the Effect of Pore Microstructures via Numerical Calculation. Polymers. 2024; 16(18):2577. https://doi.org/10.3390/polym16182577
Chicago/Turabian StyleGe, Jian, Xujiang Chao, Wenlong Tian, Weiqi Li, and Lehua Qi. 2024. "An Insight into the Mechanical Properties of Unidirectional C/C Composites Considering the Effect of Pore Microstructures via Numerical Calculation" Polymers 16, no. 18: 2577. https://doi.org/10.3390/polym16182577
APA StyleGe, J., Chao, X., Tian, W., Li, W., & Qi, L. (2024). An Insight into the Mechanical Properties of Unidirectional C/C Composites Considering the Effect of Pore Microstructures via Numerical Calculation. Polymers, 16(18), 2577. https://doi.org/10.3390/polym16182577







