Mechanical Properties of Latex-Modified Cement Stone under Uniaxial and Triaxial Cyclic Loading
Abstract
1. Introduction
2. Experimental Program
2.1. Sample Preparation
2.2. Testing Methods
3. Experimental Results and Discussion
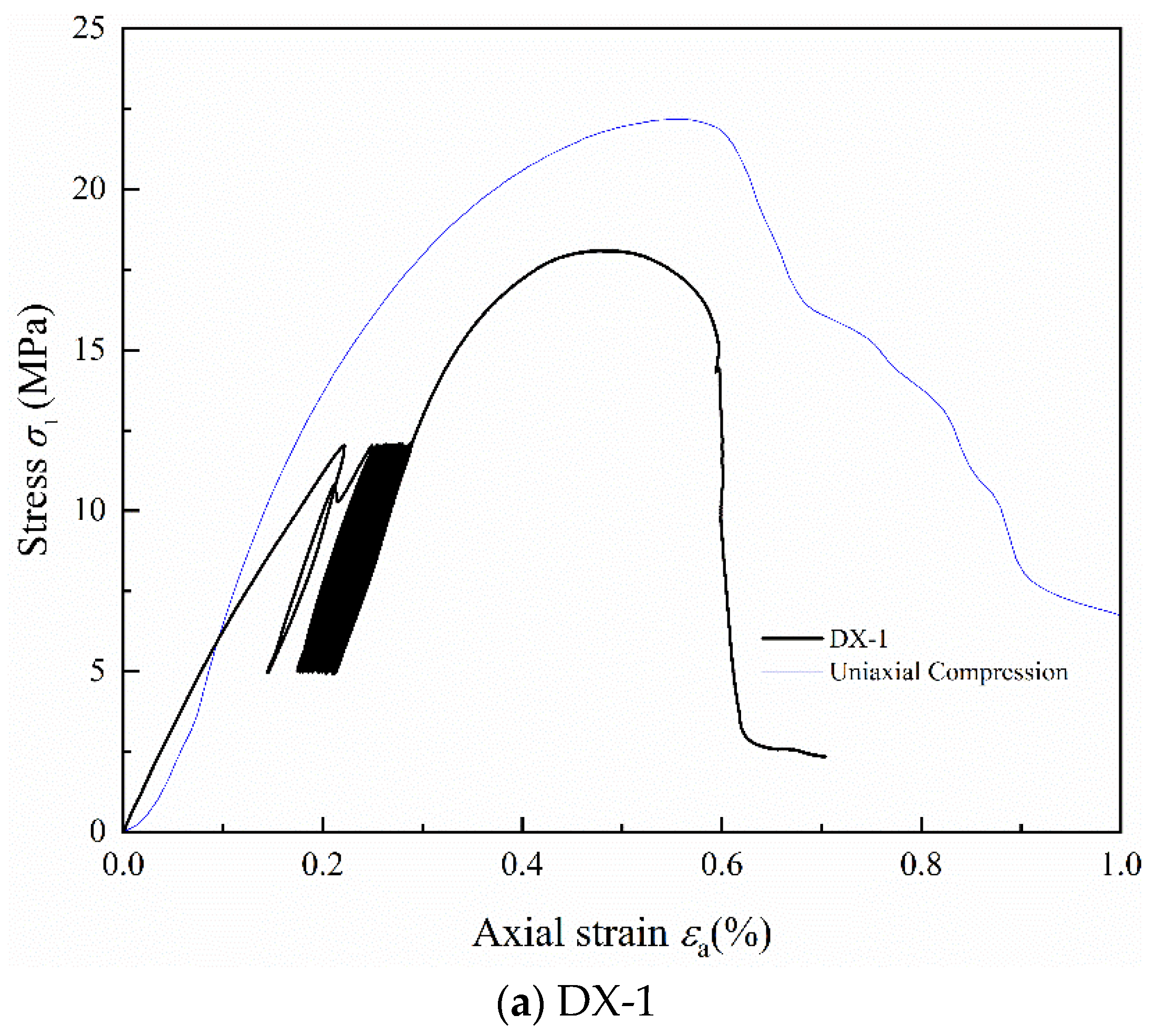
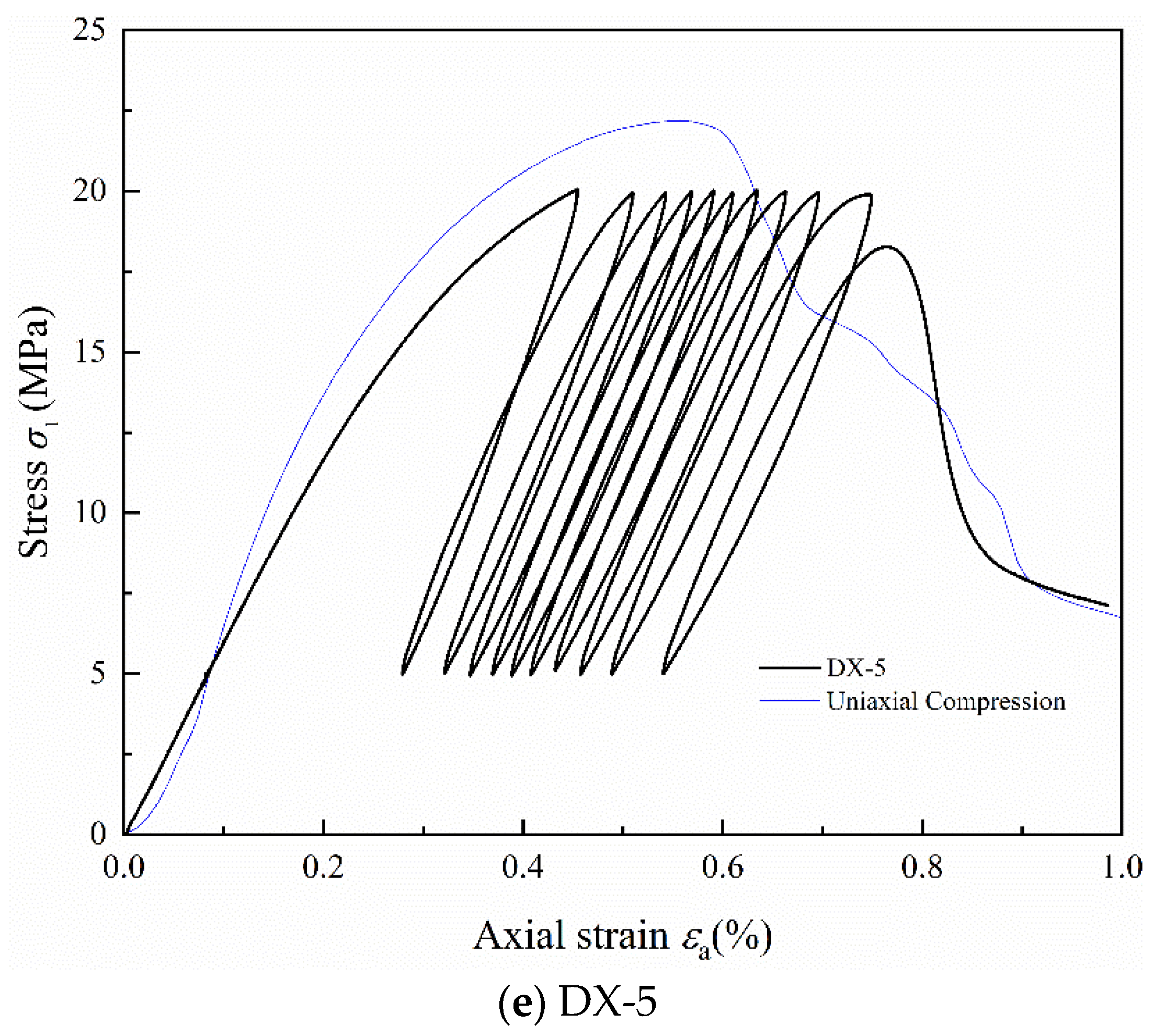
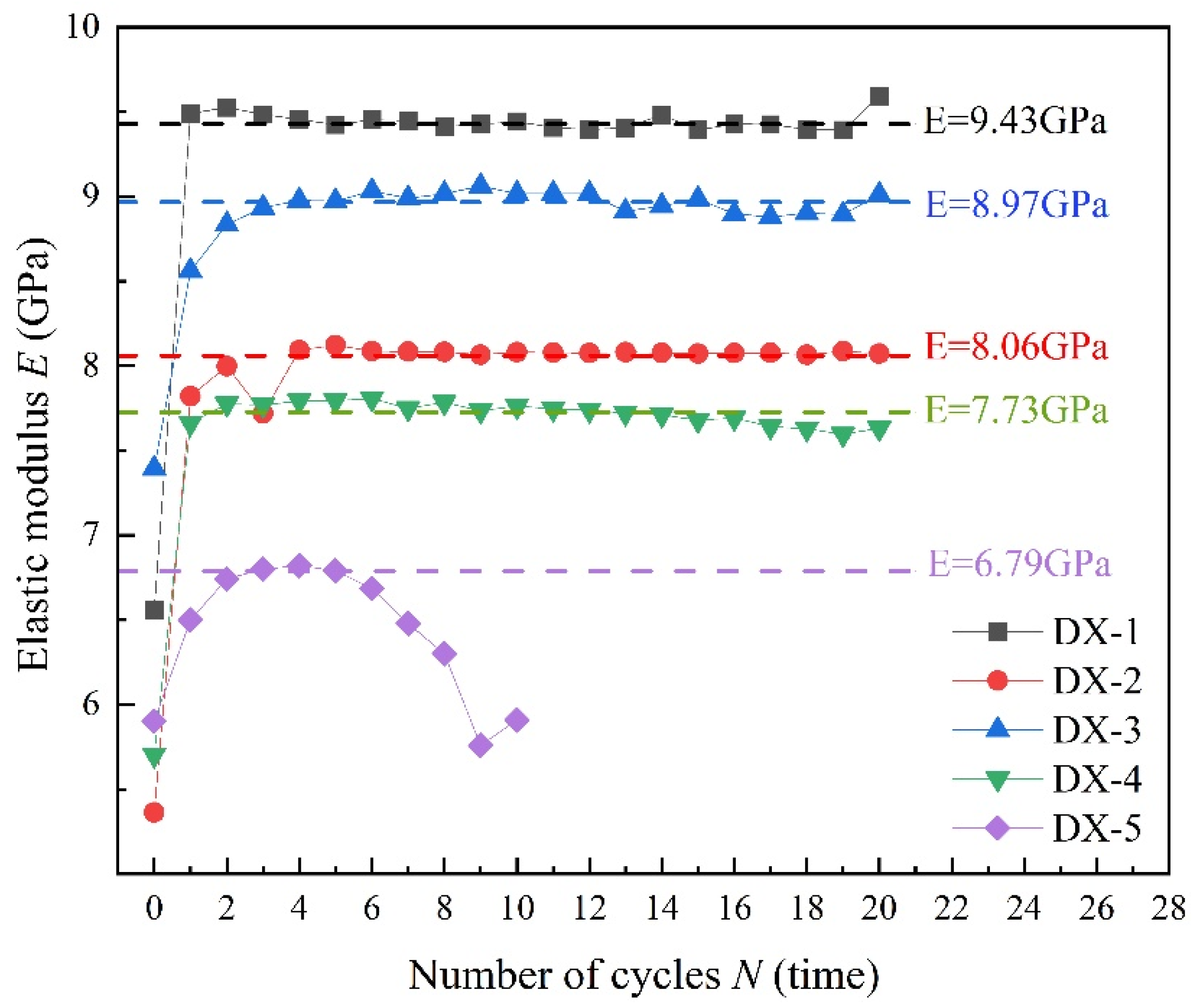
3.1. Uniaxial Cyclic Compression Tests
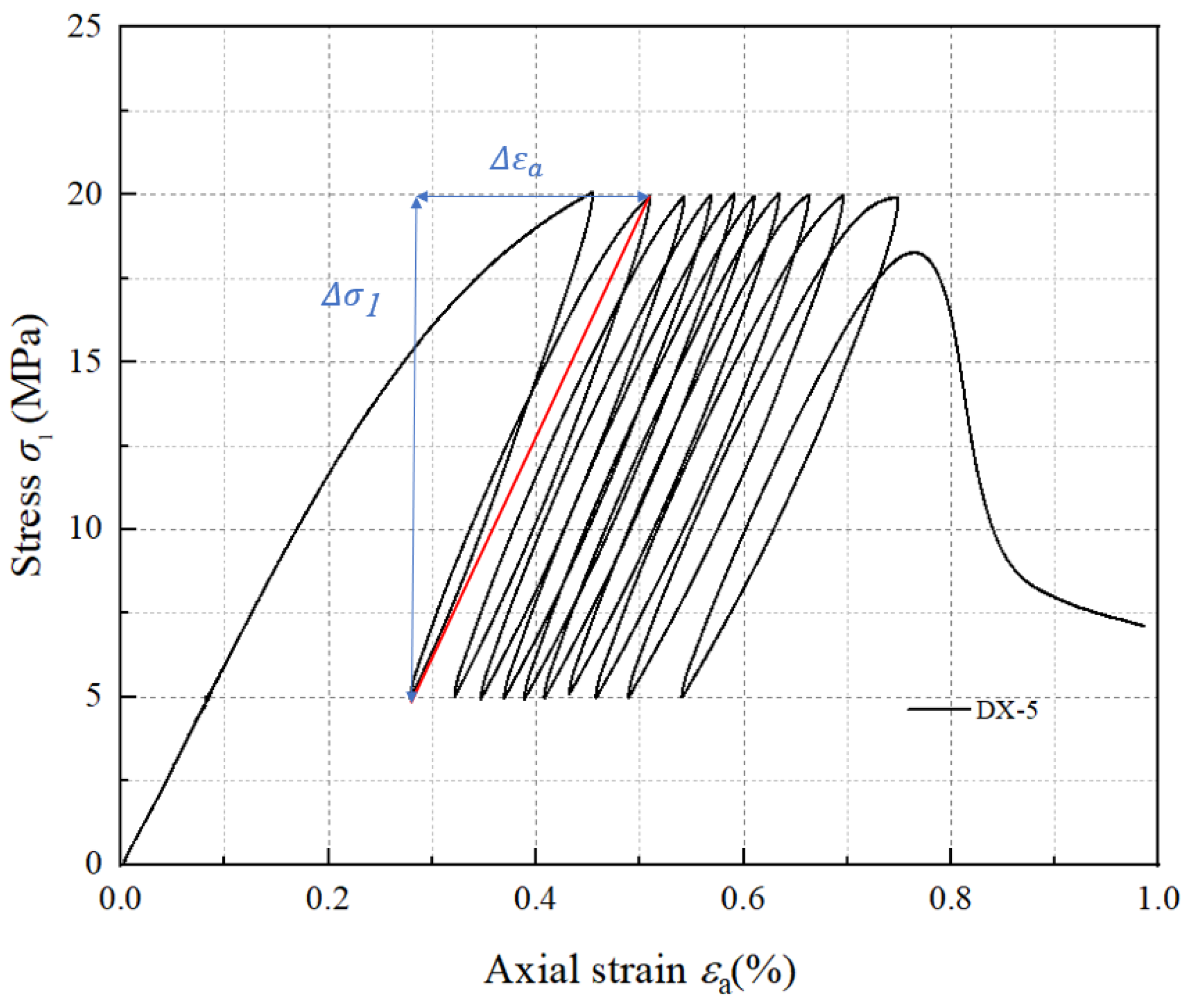
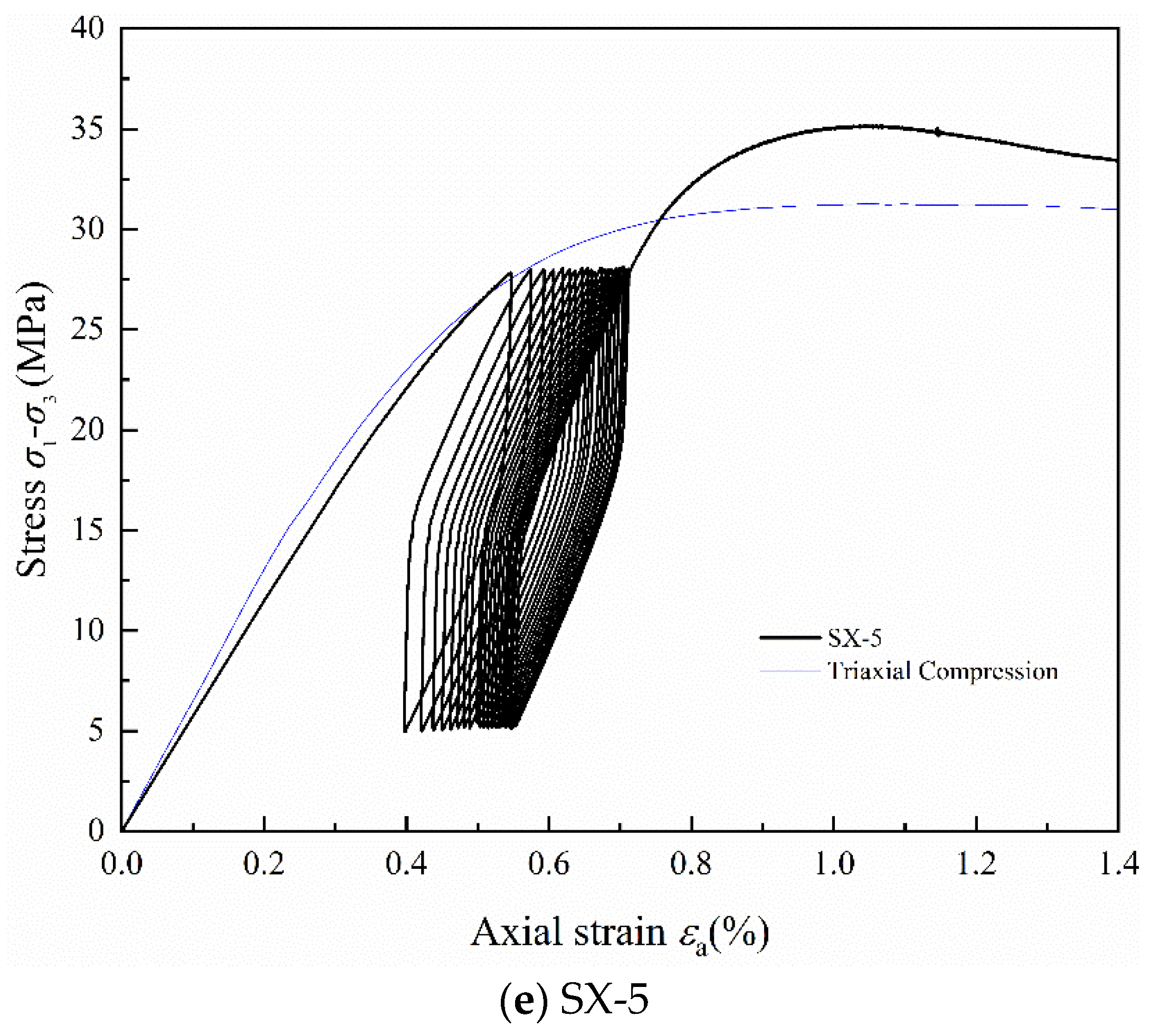
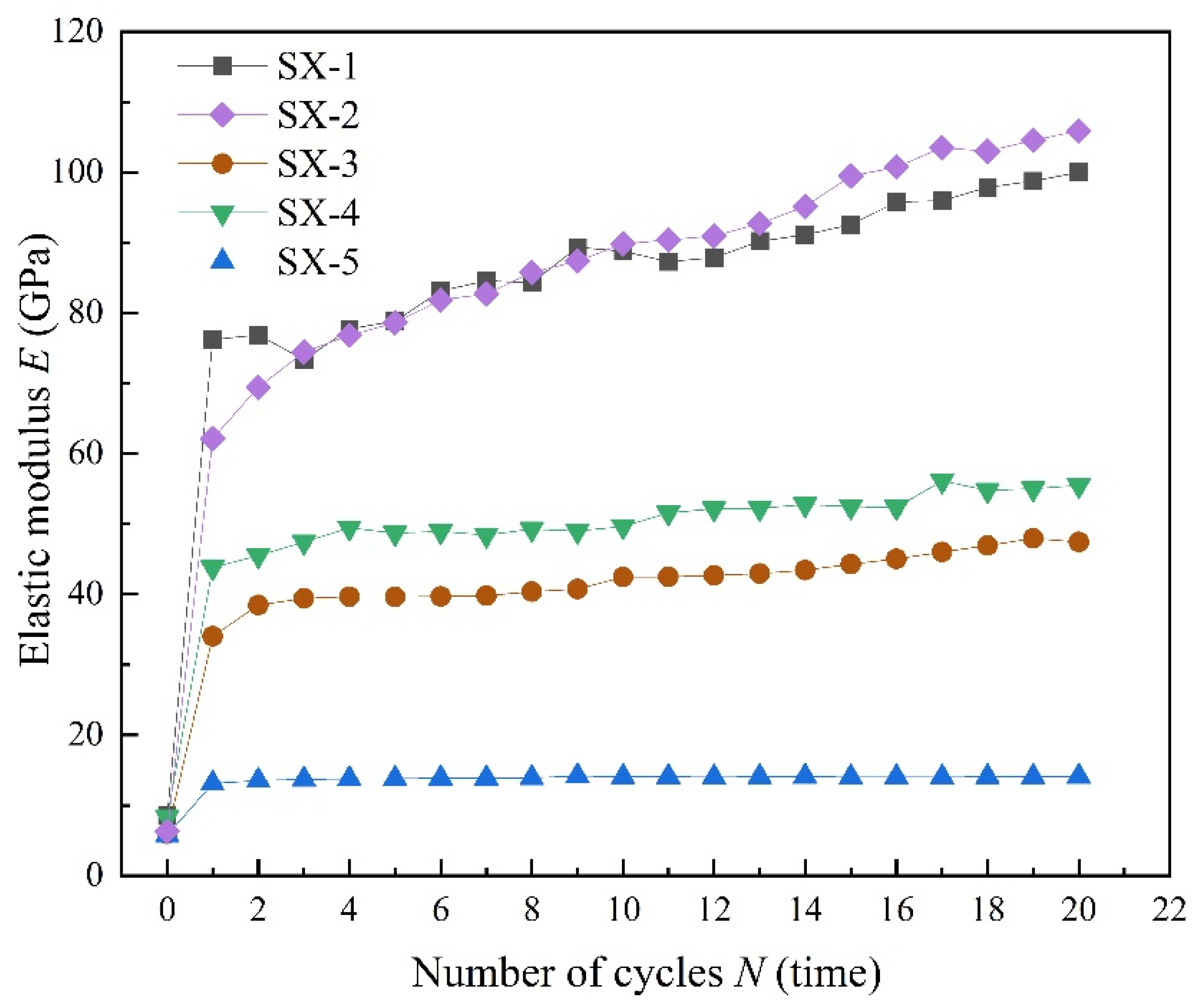
3.2. Triaxial Cyclic Compression Tests
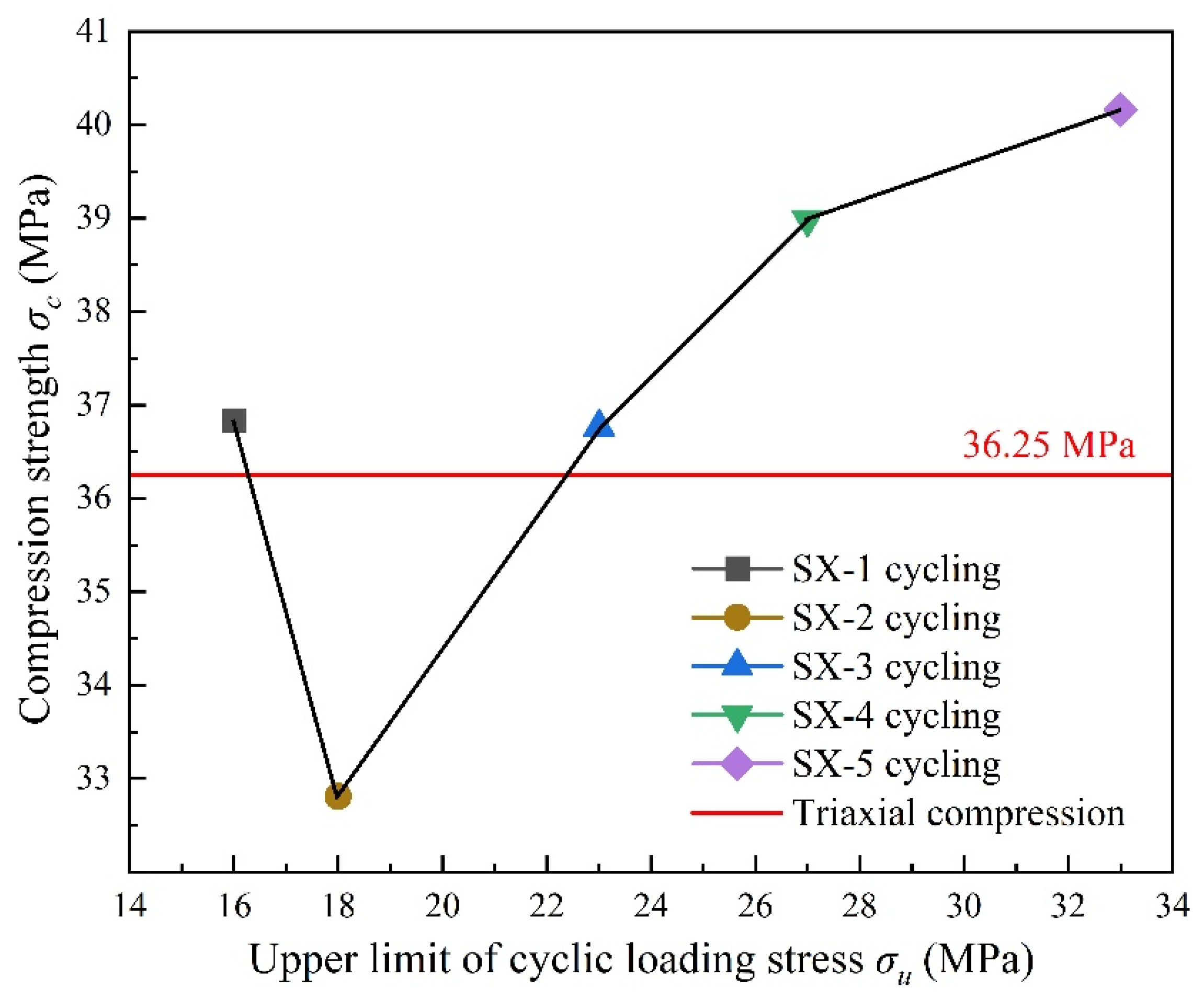
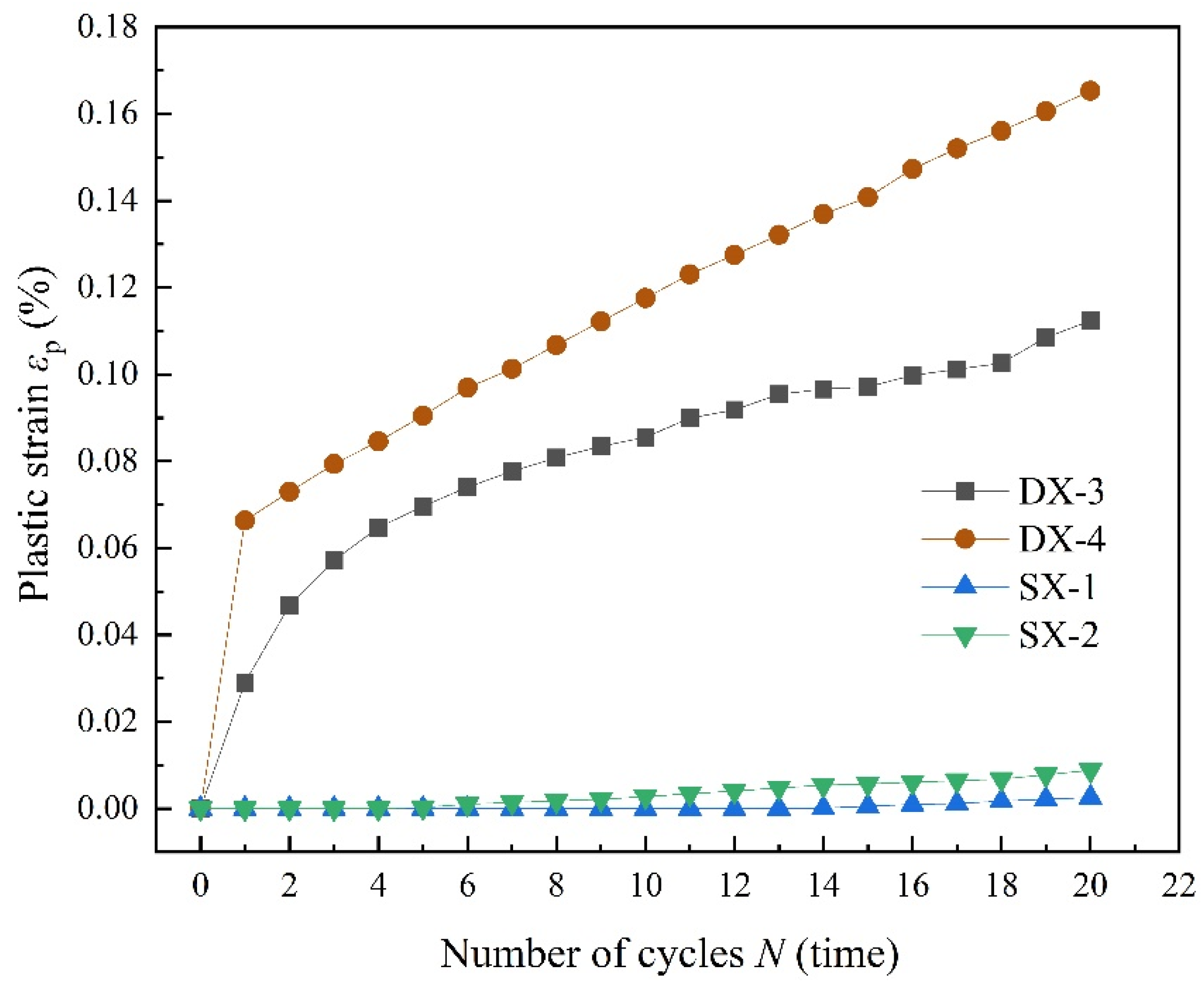
3.3. Discussion on the Difference between Uniaxial and Triaxial Cyclic Compressions
4. Conclusions
- (1)
- The peak strength of LMCS is generally lower under uniaxial cyclic loading compared to monotonic loading, whereas it is typically higher under triaxial cyclic loading. This increase may be attributed to the triaxial cyclic load, which raises the damage threshold of LMCS, thereby increasing the compactness and strength of the samples.
- (2)
- Under cyclic loading and unloading conditions, the hysteresis loop interval of the stress–strain curve of LMCS gradually decreases. The stress level is negatively correlated with the density of the hysteresis curve.
- (3)
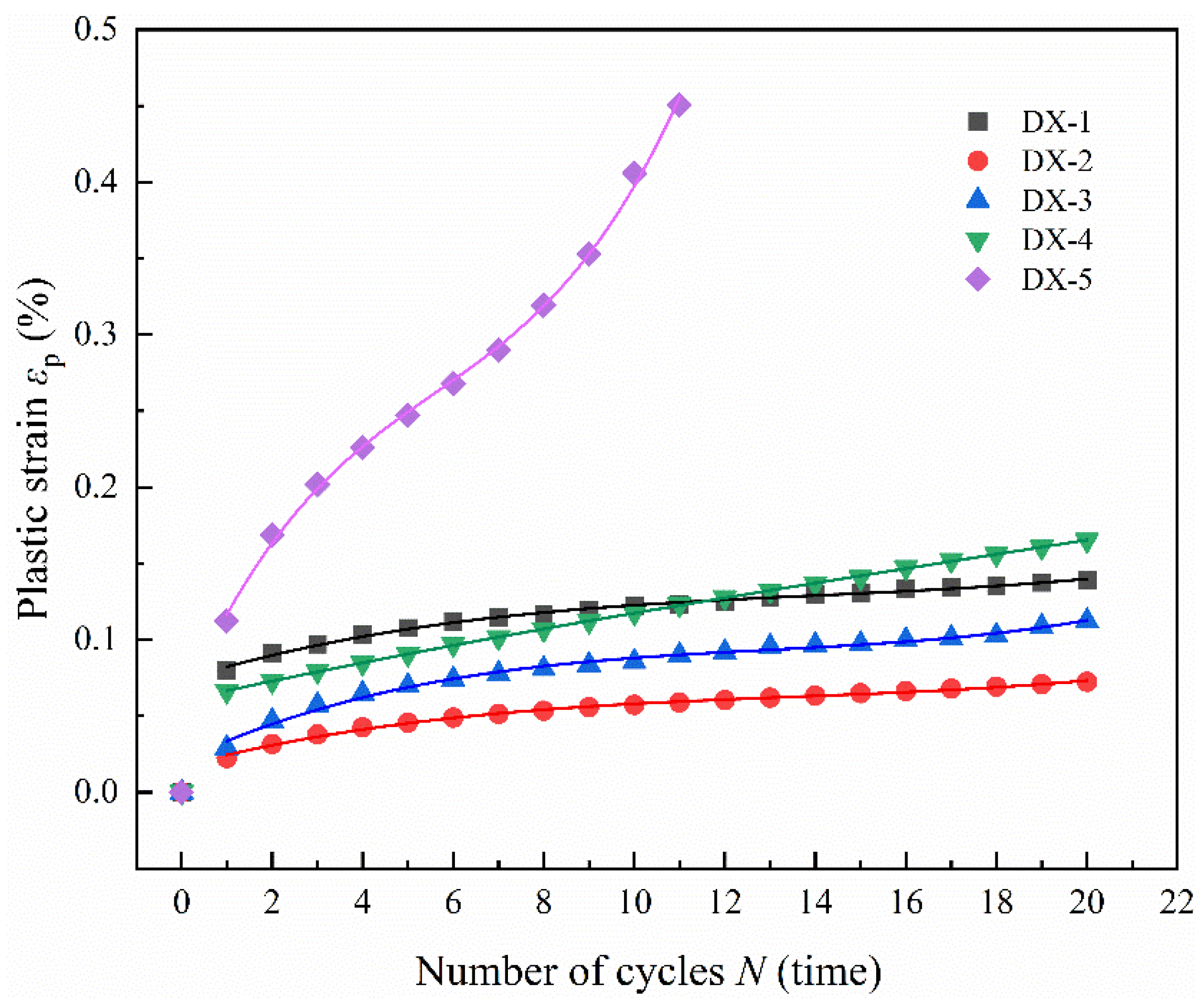
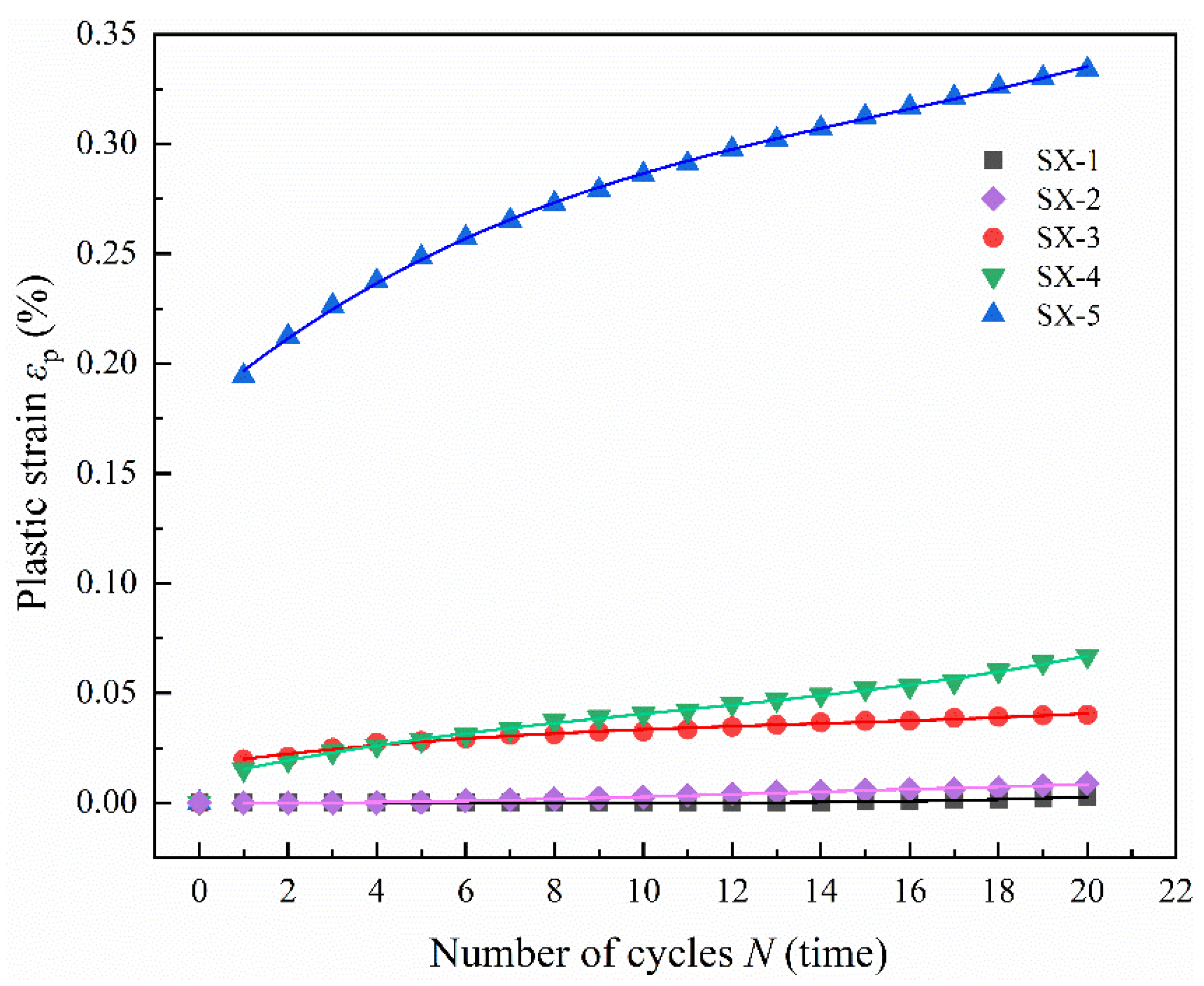
- As the number of cycles increases, the accumulated plastic strain in LMCS increases linearly.
- (4)
- The cyclic loading and unloading process fundamentally acts as a hardening mechanism for LMCS. Notably, after the initial cycle, the elastic modulus of LMCS increases significantly but tends to stabilize when subjected to uniaxial cyclic loading and unloading. Conversely, under triaxial cyclic loading and unloading, the elastic modulus does not stabilize; it continues to increase with each successive cycle.
- (5)
- A comparison of uniaxial and triaxial cyclic loading and unloading of LMCS shows that cumulative plastic strain develops rapidly under uniaxial conditions, while the elastic modulus is larger under triaxial conditions. This result can provide reference for engineering life prediction and numerical modeling analysis.
- (6)
- The findings of this study can provide a reference and guidance for the cyclic injection and extraction engineering of underground storage wellbores.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, Y.; Wen, Z.; Xu, M.; Kosajan, V. The carbon-energy-water nexus of the carbon capture, utilization, and storage technology deployment schemes: A case study in China’s cement industry. Appl. Energy 2024, 362, 122991. [Google Scholar] [CrossRef]
- Wang, Z.; Lyu, X.; Shi, W.; Feng, X.T.; Qiao, L.; Kong, R. Assessing the effect of intermediate principal geostress on the caprock integrity for underground gas storage. Gas Sci. Eng. 2024, 123, 205252. [Google Scholar] [CrossRef]
- Shoushtari, S.; Namdar, H.; Jafari, A. Utilization of CO2 and N2 as cushion gas in underground gas storage process: A review. J. Energy Storage 2023, 67, 107596. [Google Scholar] [CrossRef]
- Moradi, P.; Chahardowli, M.; Simjoo, M. Insights into underground gas storage in water-wet carbonate saline aquifers: The use of fluorinated surfactants to change the wettability. Fuel 2023, 364, 130843. [Google Scholar] [CrossRef]
- Long, K.; Tang, Y.; He, Y.; Luo, Y.; Hong, Y.; Sun, Y.; Rui, Z. Full-cycle enhancing condensate recovery-underground gas storage by integrating cyclic gas flooding and storage from gas condensate reservoirs. Energy 2024, 293, 130724. [Google Scholar] [CrossRef]
- Yang, H.; Bu, Y.; Guo, S.; Liu, H.; Du, J.; Cao, X. Effects of in-situ stress and elastic parameters of cement sheath in salt rock formation of underground gas storage on seal integrity of cement sheath. Eng. Fail. Anal. 2021, 123, 105258. [Google Scholar] [CrossRef]
- Patel, H.; Salehi, S. Structural integrity of liner cement in oil & gas wells: Parametric study, sensitivity analysis, and risk assessment. Eng. Fail. Anal. 2021, 122, 105203. [Google Scholar] [CrossRef]
- Zhou, S.; Liu, R.; Zeng, H.; Zeng, Y.; Zhang, L.; Zhang, J.; Li, X. Mechanical characteristics of well cement under cyclic loading and its influence on the integrity of shale gas wellbores. Fuel 2019, 250, 132–143. [Google Scholar] [CrossRef]
- Wang, X.; Xu, M.; Qin, Y.; Song, J.; Chen, R.; Yin, Z. The Effect of Polymer Elastic Particles Modified with Nano-Silica on the Mechanical Properties of Oil Well Cement-Based Composite Materials. Polymers 2023, 15, 3130. [Google Scholar] [CrossRef]
- Aluah, R.; Oni, O.; Fadairo, A.; Pothana, P. Enhancing the performance of class G cement for subsurface gas storage and well completion: Synergistic impact of North Dakota’s fly ash and eggshell powder. Powder Technol. 2024, 440, 119773. [Google Scholar] [CrossRef]
- Yan, R.; Wang, L.; Ni, Y.; Zhang, S.; He, Z.; Guan, B. A Study on the Properties of Composite Modified Mortar with Styrene–Butadiene Rubber Latex and Silica Fume. Polymers 2024, 16, 697. [Google Scholar] [CrossRef] [PubMed]
- Xie, Z.; Yuan, Q.; Yao, H.; Zhong, F.; Jiang, M. Hardened properties and microstructure change of sulphoaluminate cement modified with different doses of styrene-butadiene rubber latex. Constr. Build. Mater. 2023, 400, 132618. [Google Scholar] [CrossRef]
- Lu, Z.; Kong, X.; Zhang, Q.; Cai, Y.; Zhang, Y.; Wang, Z.; Dong, B.; Xing, F. Influences of styrene-acrylate latexes on cement hydration in oil well cement system at different temperatures. Colloids Surf. A Physicochem. Eng. Asp. 2016, 507, 46–57. [Google Scholar] [CrossRef]
- Hoy, M.; Tran, N.Q.; Suddeepong, A.; Horpibulsuk, S.; Mobkrathok, M.; Chinkulkijniwat, A.; Arulrajah, A. Improved fatigue properties of cement-stabilized recycled materials–Lateritic soil using natural rubber latex for sustainable pavement applications. Transp. Geotech. 2023, 40, 100959. [Google Scholar] [CrossRef]
- Zhang, T.; Xu, W.; Wang, R.; Yan, L.; He, M. Deformation characteristics of cement mortar under triaxial cyclic loading: An experimental investigation. Int. J. Fatigue 2021, 150, 106305. [Google Scholar] [CrossRef]
- Cheng, X.; Chen, Z.; Gu, T.; Zeng, L.; Yao, L.; Chen, Z.; Huang, K.; Zhang, Z.; Zhang, C.; Liu, K.; et al. Study on the dynamic and static mechanical properties of microsphere rubber powder reinforced oil well cement composites. Constr. Build. Mater. 2021, 309, 125145. [Google Scholar] [CrossRef]
- Xu, Y.; Yang, R.; Chen, P.; Ge, J.; Liu, J.; Xie, H. Experimental study on energy and failure characteristics of rubber-cement composite short-column under cyclic loading. Case Stud. Constr. Mater. 2022, 16, e00885. [Google Scholar] [CrossRef]
- Yuan, Z.; Teodoriu, C.; Schubert, J. Low cycle cement fatigue experimental study and the effect on HPHT well integrity. J. Pet. Sci. Eng. 2013, 105, 84–90. [Google Scholar] [CrossRef]
- Zhang, S.; Yan, Y.; Sheng, Z.; Yan, X. Uncertainty failure risk quantitative assessments for underground gas storage near-wellbore area. J. Energy Storage 2021, 36, 102393. [Google Scholar] [CrossRef]
- He, T.; Wang, T.; Xie, D.; Daemen, J.J.K. The mechanism of pores enhancing the deformation of completion cement under confining pressure. Cem. Concr. Compos. 2022, 125, 104322. [Google Scholar] [CrossRef]
- GB/T 19139; Test Method for Oil Well Cement. General Administration of Quality Supervision ISO: Beijing, China, 2012.
- API RP 10B-2; Recommended Practice for Testing Well Cements. API Publishing Services: Washington, DC, USA, 2013.
- Miao, S.; Pan, P.Z.; Hou, W.; He, B.; Yu, P. Stress intensity factor evolution considering fracture process zone development of granite under monotonic and stepwise cyclic loading. Eng. Fract. Mech. 2022, 273, 108727. [Google Scholar] [CrossRef]
- Shu, R.; Kong, L.; Li, T.; Jian, T.; Zhou, Z. Accumulated plastic deformation behavior of granite residual soil under cyclic loading considering the influence of initial unloading. Transp. Geotech. 2023, 41, 101004. [Google Scholar] [CrossRef]
- Peng, K.; Zhou, J.; Zou, Q.; Yan, F. Deformation characteristics of sandstones during cyclic loading and unloading with varying lower limits of stress under different confining pressures. Int. J. Fatigue 2019, 127, 82–100. [Google Scholar] [CrossRef]
- Su, C.; Zhu, H.; Cai, W.; Wu, W.; Zhang, Q. Elastic perfect plastic rock mass analysis and equivalent GSI determination considering strain-softening behavior and three-dimensional strength. Tunn. Undergr. Space Technol. 2023, 142, 105422. [Google Scholar] [CrossRef]
- Zhong, J.; Xu, T.; Wang, W.; Guan, K.; Song, M.; Huang, S.; Zhang, S. Use of database and small punch test to estimate true stress-plastic strain curve of steels. Int. J. Press. Vessel. Pip. 2021, 191, 104370. [Google Scholar] [CrossRef]
- Xi, Y.; Li, J.; Tao, Q.; Guo, B.; Liu, G. Experimental and numerical investigations of accumulated plastic deformation in cement sheath during multistage fracturing in shale gas wells. J. Pet. Sci. Eng. 2020, 187, 106790. [Google Scholar] [CrossRef]
- Liu, H.; Pei, J.; Liu, J.; Xiao, M.; Zhuo, L.; Xie, H. Influence of volume compression on the unloading deformation behavior of red sandstone under damage-controlled cyclic triaxial loading. J. Rock Mech. Geotech. Eng. 2023, 15, 1200–1212. [Google Scholar] [CrossRef]
- Peellage, W.H.; Fatahi, B.; Rasekh, H. Stiffness and damping characteristics of jointed rocks under cyclic triaxial loading subjected to prolonged cyclic loading. Int. J. Fatigue 2024, 181, 108121. [Google Scholar] [CrossRef]
- Zhou, Z.; Bai, R.; Shen, M.; Wang, Q. The effect of overconsolidation on monotonic and cyclic behaviours of frozen subgrade soil. Transp. Geotech. 2022, 32, 100710. [Google Scholar] [CrossRef]
- Li, P.; Wu, Y.F. Stress–strain behavior of actively and passively confined concrete under cyclic axial load. Compos. Struct. 2016, 149, 369–384. [Google Scholar] [CrossRef]
- Shi, D.; Chen, X.; Ning, Y.; Ji, T. Deformation responses and strength criteria of different shotcrete-rock composites under triaxial cyclic compression. Constr. Build. Mater. 2023, 374, 130935. [Google Scholar] [CrossRef]
- Fu, Q.; Bu, M.; Su, L.; Guo, B.; Chen, L.; Song, H.; Yuan, Q.; Niu, D. Dynamic triaxial compressive response and failure mechanism of basalt fibre-reinforced coral concrete. Int. J. Impact Eng. 2021, 156, 103930. [Google Scholar] [CrossRef]
- Abood, A.S.; Fattah, M.Y.; Al-Adili, A. Effect of saturation on dynamic characteristics of collapsible gypseous soil using cyclic triaxial testing. Case Stud. Constr. Mater. 2023, 19, e02502. [Google Scholar] [CrossRef]






| Raw Material | Cement | Water | KCM028 (Latex) | KCM018A (Stabilizer) | KCM043 (Latex Defoamer) | KCM003 (Defoamer) |
|---|---|---|---|---|---|---|
| Proportion (%) | 70.18 | 22.69 | 6.33 | 0.25 | 0.38 | 0.17 |
| Specimen Number | Length L (mm) | Diameter D (mm) | Density ρ (g/cm3) | Compressive Strength σc (MPa) |
|---|---|---|---|---|
| D-1 | 50.54 | 24.62 | 1.90 | 22.21 |
| D-2 | 49.58 | 24.30 | 1.90 | 22.09 |
| D-3 | 50.80 | 24.32 | 1.89 | 19.28 |
| Mean value | 50.31 | 24.41 | 1.90 | 21.19 |
| Specimen Number | Length L (mm) | Diameter D (mm) | Density ρ (g/cm3) | Upper Limit of Stress σu (MPa) | Lower Limit of Stress σL (MPa) |
|---|---|---|---|---|---|
| DX-1 | 50.61 | 24.44 | 1.85 | 12.0 | 5.0 |
| DX-2 | 50.33 | 24.33 | 1.88 | 14.0 | 5.0 |
| DX-3 | 51.01 | 24.71 | 1.90 | 16.0 | 5.0 |
| DX-4 | 49.24 | 24.49 | 1.89 | 18.0 | 5.0 |
| DX-5 | 49.72 | 24.60 | 1.89 | 20.0 | 5.0 |
| Mean value | 50.18 | 24.51 | 1.88 | 16.0 | 5.0 |
| Specimen Number | Number of Cycles N (Time) | Compressive Strength σc (MPa) |
|---|---|---|
| DX-1 | 20 | 18.10 |
| DX-2 | 20 | 20.67 |
| DX-3 | 20 | 23.75 |
| DX-4 | 20 | 21.01 |
| DX-5 (Damaged) | 11 | 20.06 |
| Mean value | 18.2 | 20.72 |
| Specimen Number | Plastic Strain Formula | R2 |
|---|---|---|
| DX-1 | 0.99 | |
| DX-2 | 0.99 | |
| DX-3 | 0.99 | |
| DX-4 | 0.99 | |
| DX-5 | 0.99 |
| Specimen Number | Length L (mm) | Diameter D (mm) | Density ρ (g/cm3) | Confining Pressure σ3 (MPa) | Upper Limit of Stress σu (MPa) | Lower Limit of Stress σL (MPa) |
|---|---|---|---|---|---|---|
| SX-1 | 49.36 | 24.51 | 1.89 | 5.0 | 17.0 | 5.0 |
| SX-2 | 50.18 | 24.54 | 1.84 | 5.0 | 18.0 | 5.0 |
| SX-3 | 51.03 | 24.26 | 1.89 | 5.0 | 23.0 | 5.0 |
| SX-4 | 50.72 | 24.30 | 1.88 | 5.0 | 27.0 | 5.0 |
| SX-5 | 49.60 | 24.60 | 1.90 | 5.0 | 33.0 | 5.0 |
| Mean value | 50.18 | 24.44 | 1.88 | 5.0 | 23.8 | 5.0 |
| Specimen Number | Number of Cycles N (Time) | Compressive Strength σc (MPa) |
|---|---|---|
| SX-1 | 20 | 36.83 |
| SX-2 | 20 | 32.81 |
| SX-3 | 20 | 36.76 |
| SX-4 | 20 | 38.98 |
| SX-5 | 20 | 40.16 |
| Mean value | 20 | 37.53 |
| Specimen Number | Plastic Strain Formula | R2 |
|---|---|---|
| SX-1 | 0.98 | |
| SX-2 | 0.99 | |
| SX-3 | 0.99 | |
| SX-4 | 0.99 | |
| SX-5 | 0.99 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Q.; Yang, L.; Zhang, J.; Xing, Z. Mechanical Properties of Latex-Modified Cement Stone under Uniaxial and Triaxial Cyclic Loading. Polymers 2024, 16, 2543. https://doi.org/10.3390/polym16172543
Tian Q, Yang L, Zhang J, Xing Z. Mechanical Properties of Latex-Modified Cement Stone under Uniaxial and Triaxial Cyclic Loading. Polymers. 2024; 16(17):2543. https://doi.org/10.3390/polym16172543
Chicago/Turabian StyleTian, Qizhong, Lianzhi Yang, Jie Zhang, and Zhenzhong Xing. 2024. "Mechanical Properties of Latex-Modified Cement Stone under Uniaxial and Triaxial Cyclic Loading" Polymers 16, no. 17: 2543. https://doi.org/10.3390/polym16172543
APA StyleTian, Q., Yang, L., Zhang, J., & Xing, Z. (2024). Mechanical Properties of Latex-Modified Cement Stone under Uniaxial and Triaxial Cyclic Loading. Polymers, 16(17), 2543. https://doi.org/10.3390/polym16172543






