Correlation of Dielectric Properties and Vibrational Spectra of Composite PVDF/Salt Fibers
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Preparation
2.2. Sample Experimental Characterization
2.3. Computational Methods
2.4. Electrical Characterization
3. Results
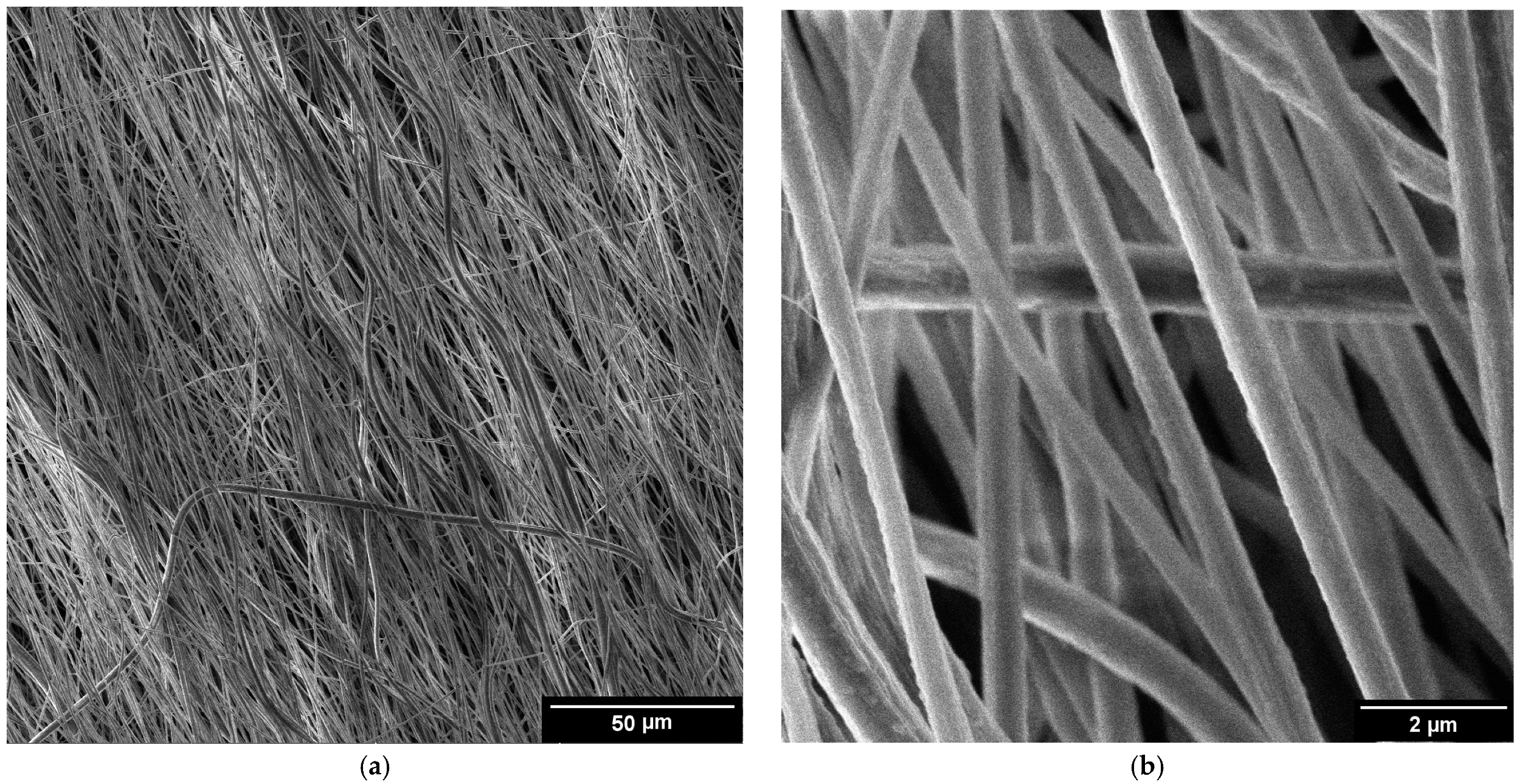
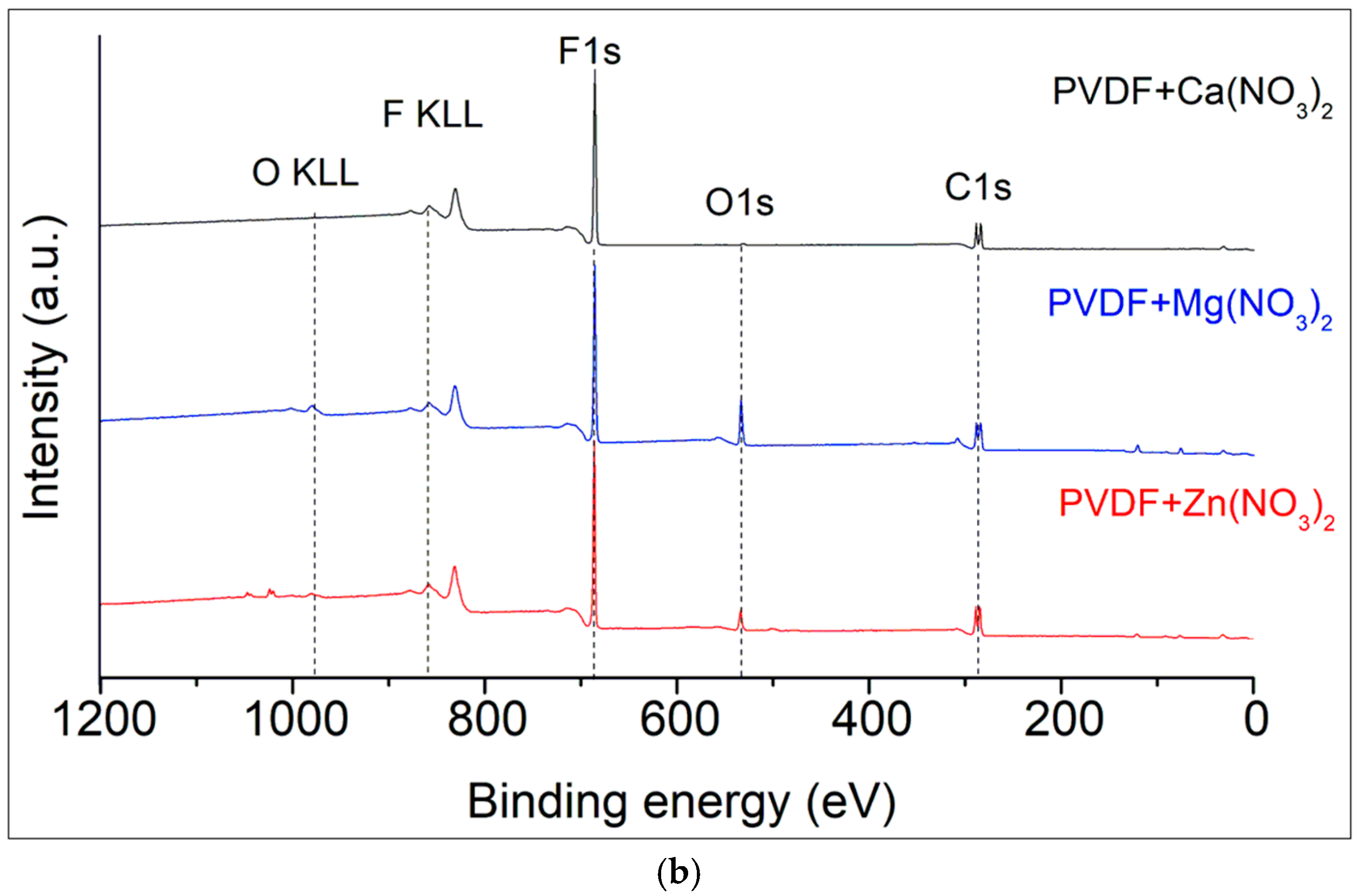
3.1. Sample Morphology
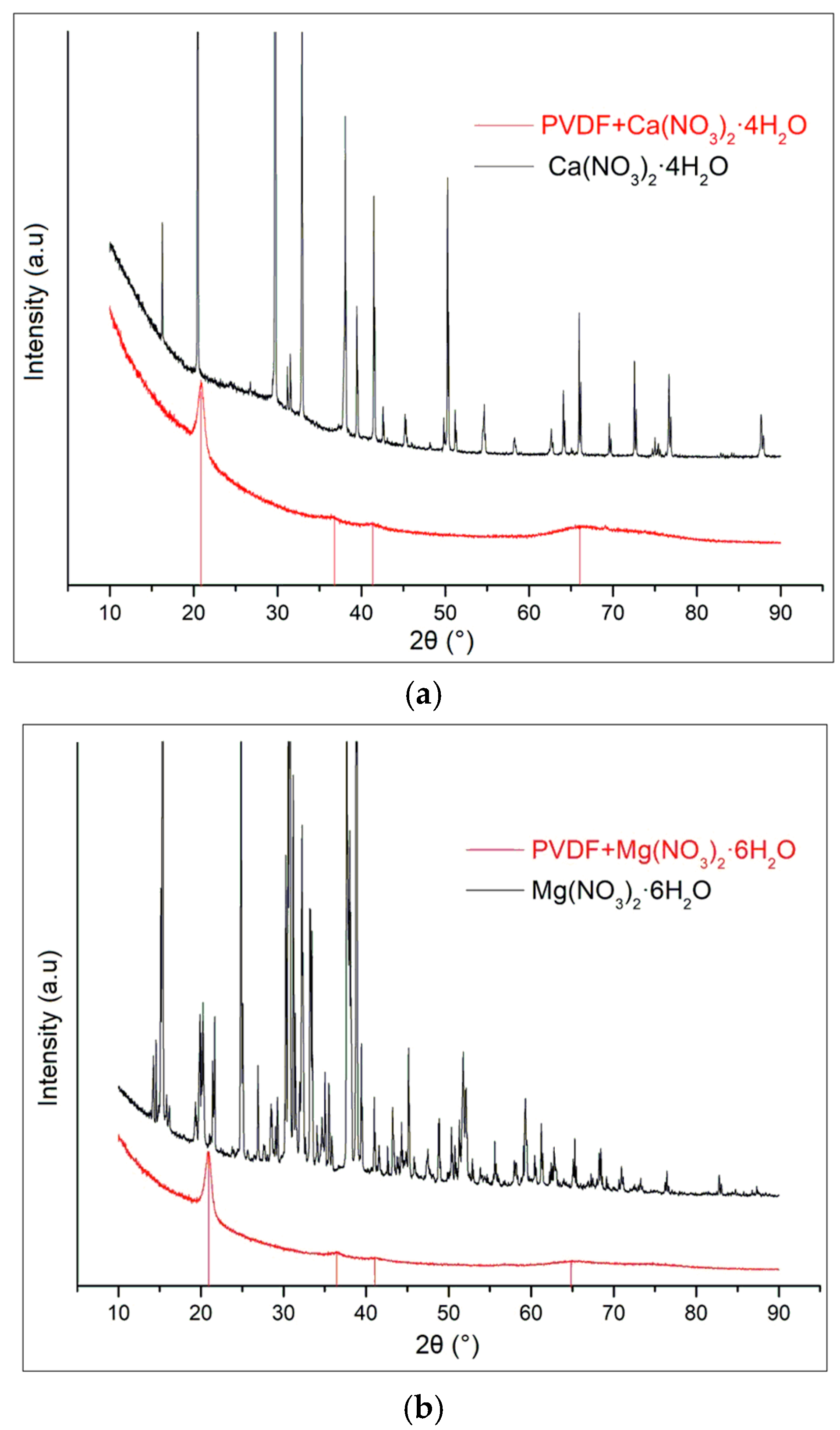
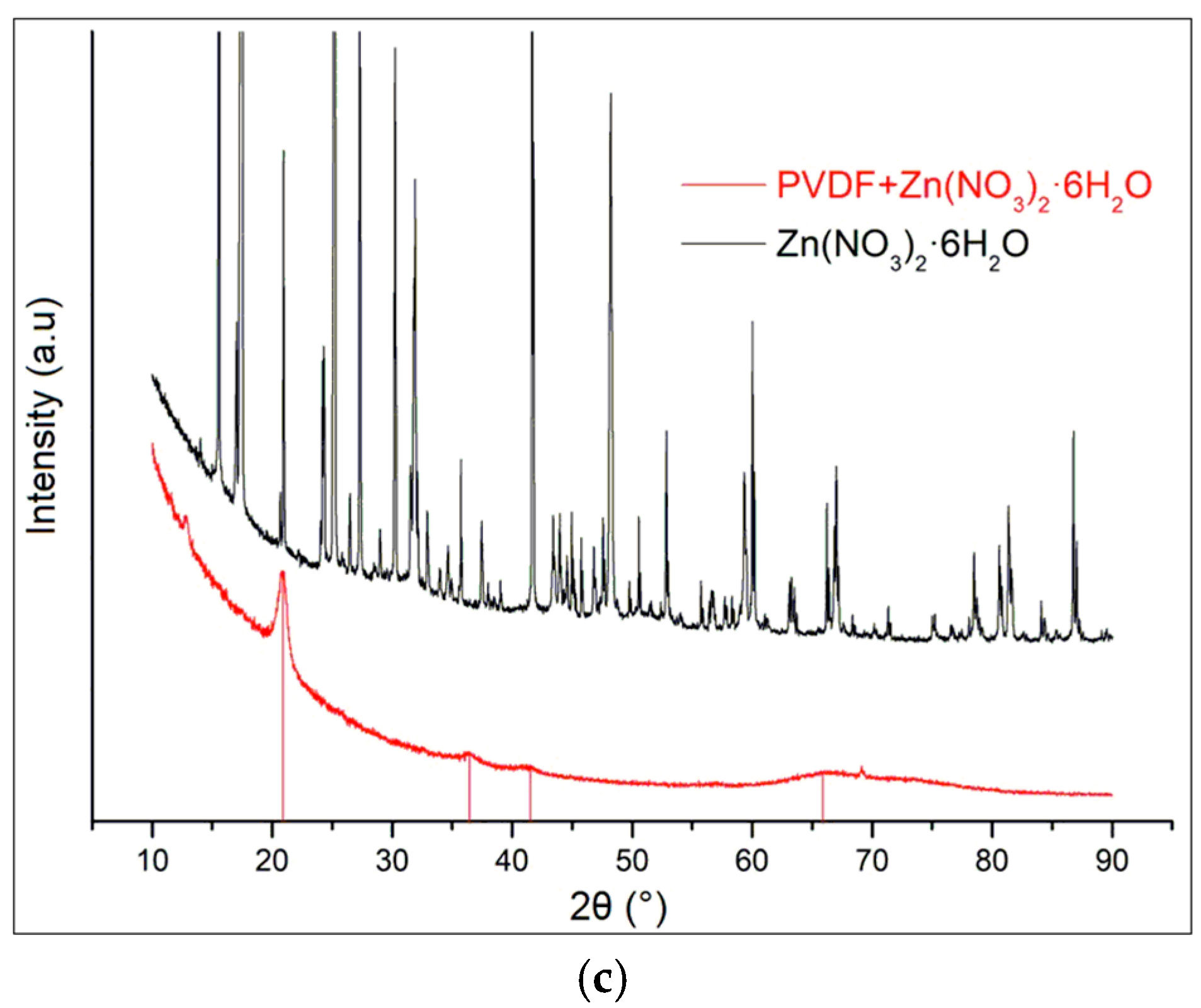
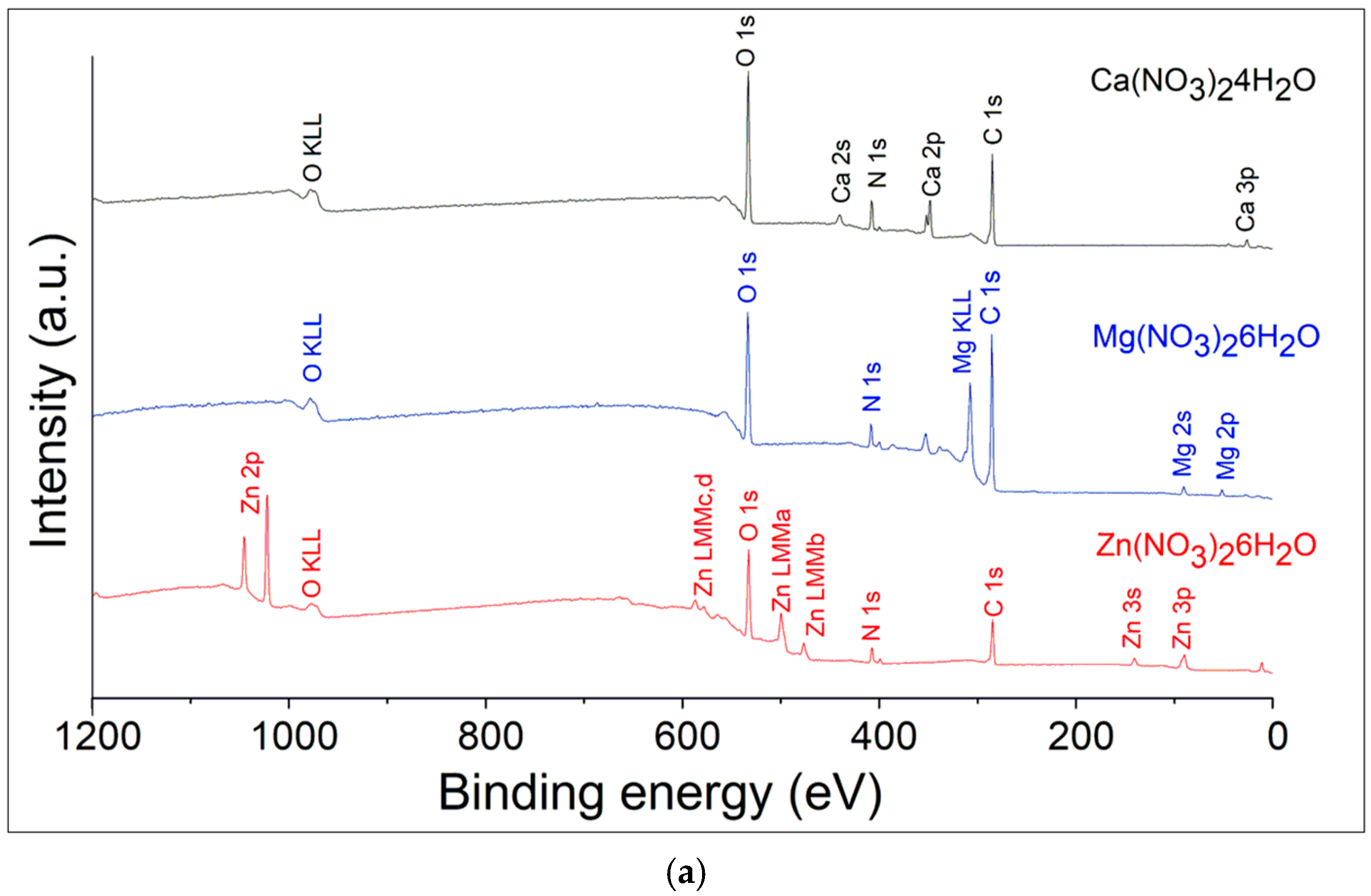
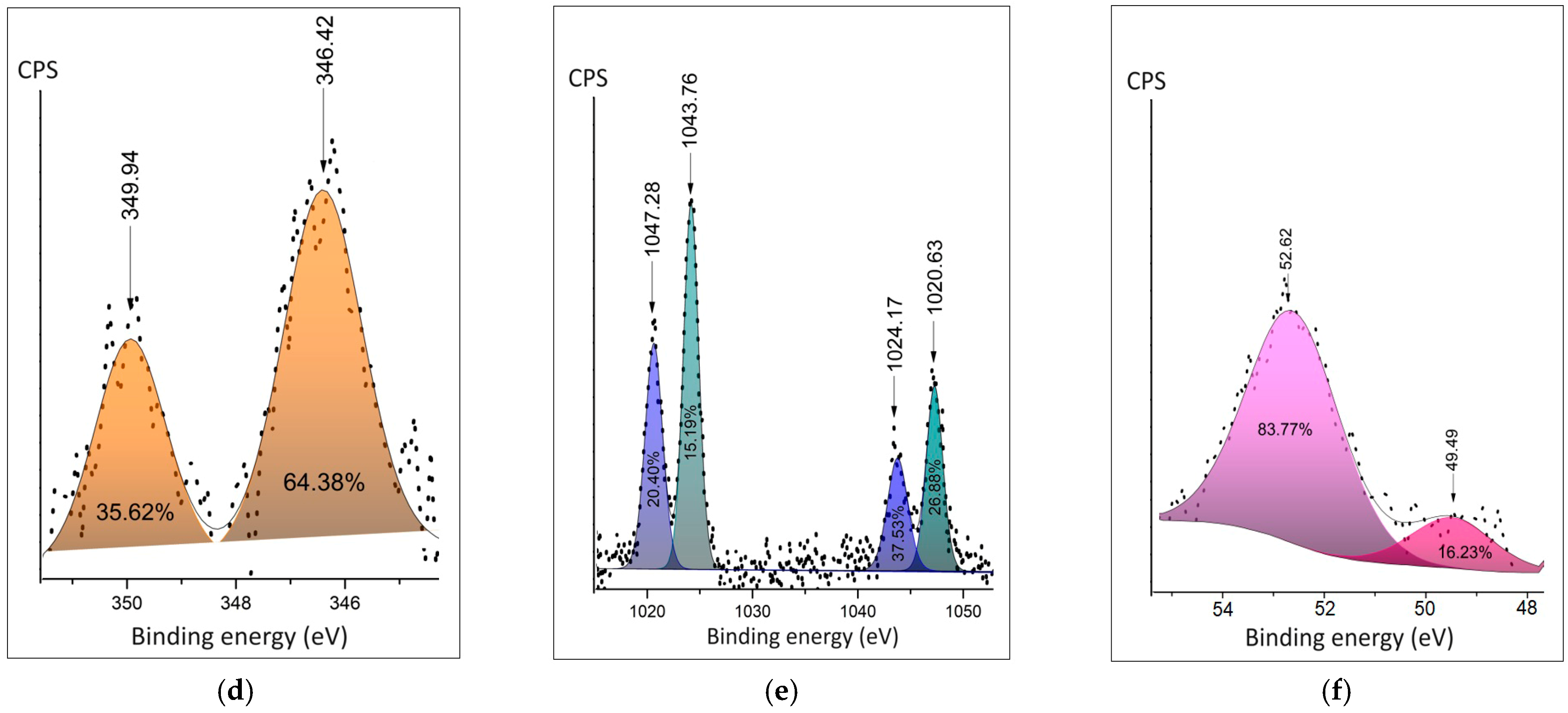
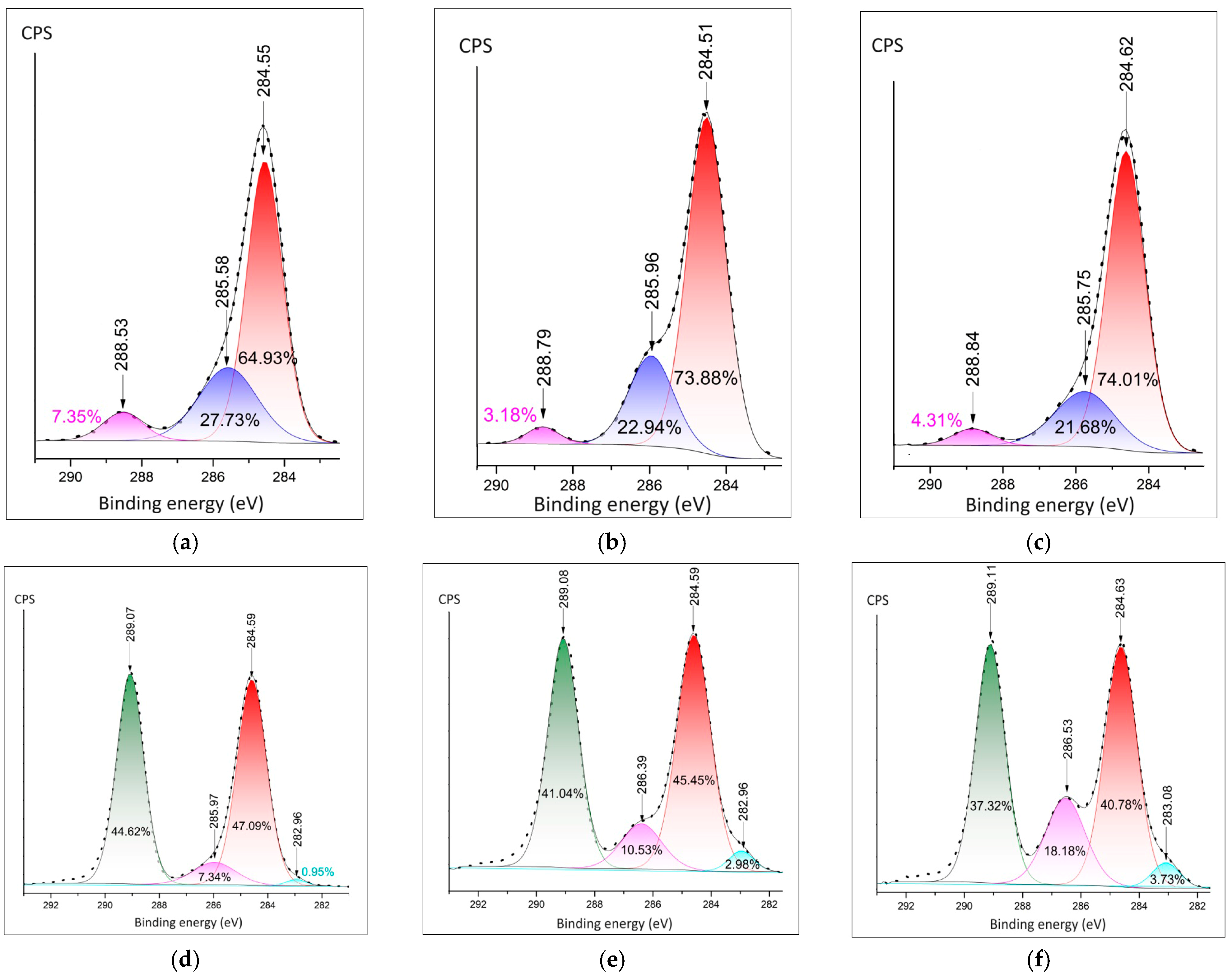
3.2. Sample Composition
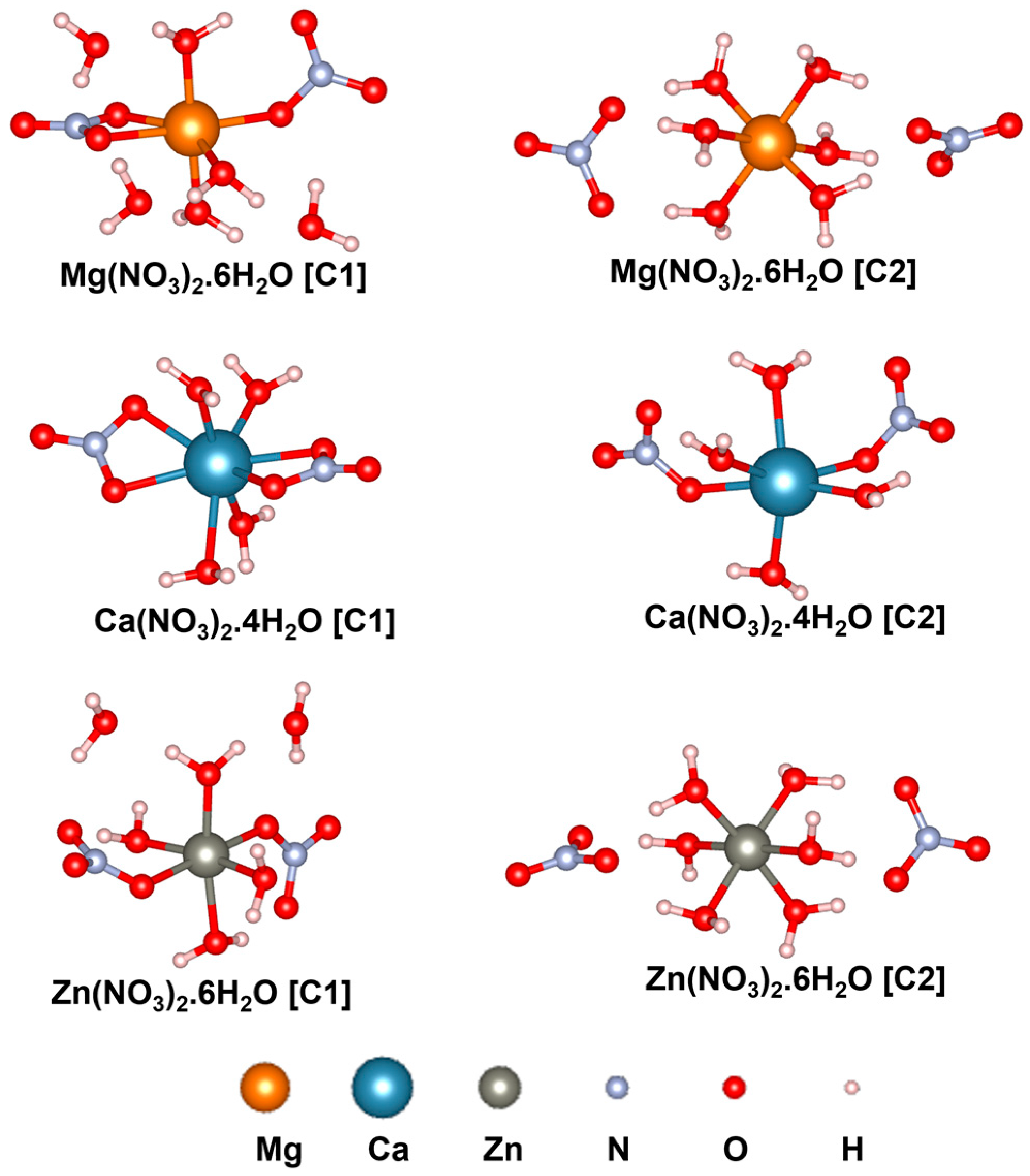
3.3. DFT Analyses
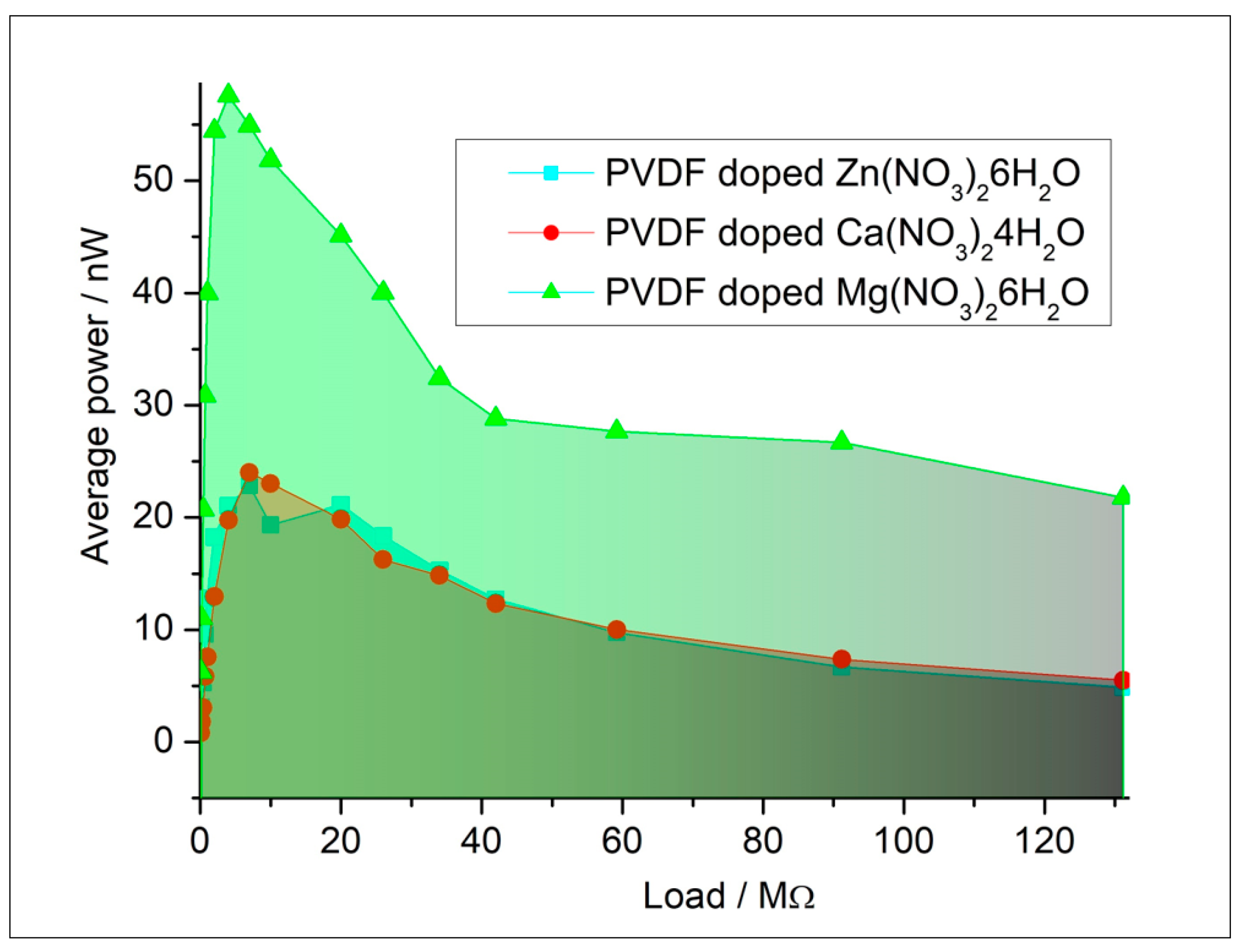
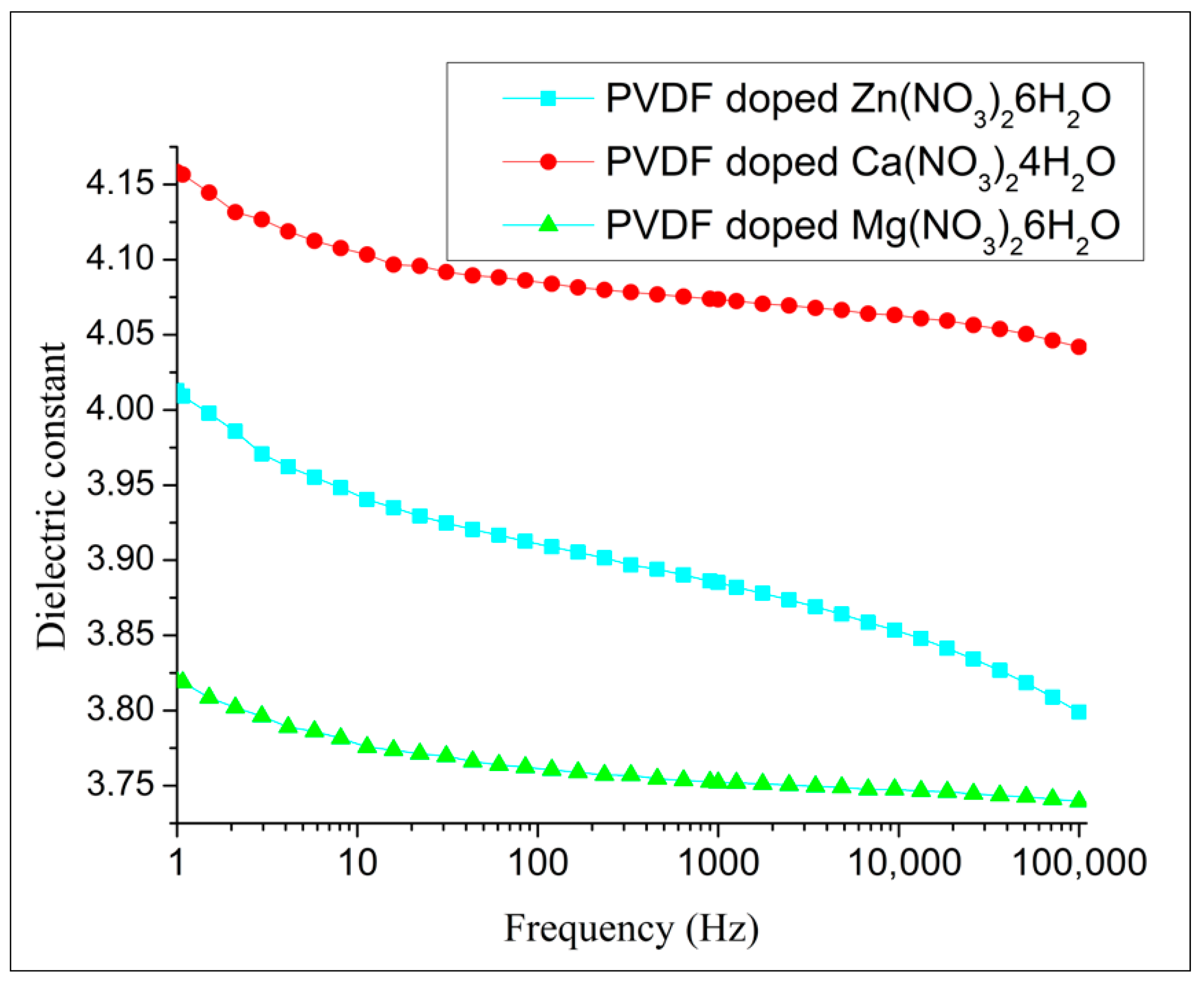
3.4. Dielectric Properties
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Orudzhev, F.; Ramazanov, S.; Sobola, D.; Kaspar, P.; Trčka, T.; Částková, K.; Kastyl, J.; Zvereva, I.; Wang, C.; Selimov, D.; et al. Ultrasound and Water Flow Driven Piezophototronic Effect in Self-Polarized Flexible α-Fe2O3 Containing PVDF Nanofibers Film for Enhanced Catalytic Oxidation. Nano Energy 2021, 90, 106586. [Google Scholar] [CrossRef]
- Luo, W.; Wang, Y.; Hitz, E.; Lin, Y.; Yang, B.; Hu, L.; Luo, W.; Wang, Y.; Hitz, E.; Hu, L.; et al. Article Type: Feature Article Solution Processed Boron Nitride Nanosheets: Synthesis, Assemblies and Emerging Applications. Adv. Funct. Mater. 2017, 27, 1701450. [Google Scholar] [CrossRef]
- Hu, Y.; Kang, W.; Fang, Y.; Xie, L.; Qiu, L.; Jin, T. Piezoelectric Poly(Vinylidene Fluoride) (PVDF) Polymer-Based Sensor for Wrist Motion Signal Detection. Appl. Sci. 2018, 8, 836. [Google Scholar] [CrossRef]
- Mokhtari, F.; Cheng, Z.; Raad, R.; Xi, J.; Foroughi, J. Piezofibers to Smart Textiles: A Review on Recent Advances and Future Outlook for Wearable Technology. J. Mater. Chem. A Mater. 2020, 8, 9496–9522. [Google Scholar] [CrossRef]
- Abednejad, A.; Ghaee, A.; Morais, E.S.; Sharma, M.; Neves, B.M.; Freire, M.G.; Nourmohammadi, J.; Mehrizi, A.A. Polyvinylidene Fluoride–Hyaluronic Acid Wound Dressing Comprised of Ionic Liquids for Controlled Drug Delivery and Dual Therapeutic Behavior. Acta Biomater. 2019, 100, 142–157. [Google Scholar] [CrossRef]
- Manh, L.N.; Li, J.; Kweon, H.; Chae, Y. Simultaneous Measurement of Two Biological Signals Using a Multi-Layered Polyvinylidene Fluoride Sensor. Sci. Rep. 2022, 12, 1507. [Google Scholar] [CrossRef]
- Bauer, F. PVDF Shock Sensors: Applications to Polar Materials and High Explosives. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2000, 47, 1448–1454. [Google Scholar] [CrossRef]
- Salem, E.F.; Na, A.; Ka, E. Studying of Thermal and Shielding Properties of PVDF (PolyVinyliDene Fluoride) Pipes Used in Aqueous Solution Sector of the Nuclear Fuel Fabrication Facility Corresponding Authors. J. Mater. Sci. Manuf. Res. 2022, 3, 1–6. [Google Scholar] [CrossRef]
- Orudzhev, F.F.; Sobola, D.S.; Ramazanov, S.M.; Častková, K.; Selimov, D.A.; Rabadanova, A.A.; Shuaibov, A.O.; Gulakhmedov, R.R.; Abdurakhmanov, M.G.; Giraev, K.M. Hydrogen Bond-Induced Activation of Photocatalytic and Piezophotocatalytic Properties in Calcium Nitrate Doped Electrospun PVDF Fibers. Polymers 2023, 15, 3252. [Google Scholar] [CrossRef]
- Sobola, D.; Kaspar, P.; Částková, K.; Dallaev, R.; Papež, N.; Sedlák, P.; Trčka, T.; Orudzhev, F.; Kaštyl, J.; Weiser, A.; et al. PVDF Fibers Modification by Nitrate Salts Doping. Polymers 2021, 13, 2439. [Google Scholar] [CrossRef]
- Frisch, M.; Trucks, G.; Schlegel, H.; Scuseria, G.; Robb, M.; Cheeseman, J.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.J.; et al. Gaussian 09, Revision a.01; Gaussian Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Becke, A.D. A New Mixing of Hartree–Fock and Local Density-functional Theories. J. Chem. Phys. 1993, 98, 1372–1377. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron Density. Phys. Rev. B 1988, 37, 785. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A Consistent and Accurate Ab Initio Parametrization of Density Functional Dispersion Correction (DFT-D) for the 94 Elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- Andersson, M.P.; Uvdal, P. New Scale Factors for Harmonic Vibrational Frequencies Using the B3LYP Density Functional Method with the Triple-ζ Basis Set 6-311+G(d,p). J. Phys. Chem. A 2005, 109, 2937–2941. [Google Scholar] [CrossRef]
- Sarkar, R.; Kundu, T.K. Hydrogen Bond Interactions of Hydrated Aluminum Nitrate with PVDF, PVDF-TrFE, and PVDF-HFP: A Density Functional Theory-Based Illustration. Int. J. Quantum Chem. 2020, 120, e26328. [Google Scholar] [CrossRef]
- Sarkar, R.; Kumari, S.; Kundu, T.K. Density Functional Theory Based Studies on the Adsorption of Rare-Earth Ions from Hydrated Nitrate Salt Solutions on g-C3N4 Monolayer Surface. J. Mol. Graph. Model. 2020, 97, 107577. [Google Scholar] [CrossRef]
- Tofel, P.; Částková, K.; Říha, D.; Sobola, D.; Papež, N.; Kaštyl, J.; Ţălu, Ş.; Hadaš, Z. Triboelectric Response of Electrospun Stratified PVDF and PA Structures. Nanomaterials 2022, 12, 349. [Google Scholar] [CrossRef]
- Galazutdinova, Y.; Vega, M.; Grágeda, M.; Cabeza, L.F.; Ushak, S. Preparation and Characterization of an Inorganic Magnesium Chloride/Nitrate/Graphite Composite for Low Temperature Energy Storage. Sol. Energy Mater. Sol. Cells 2018, 175, 60–70. [Google Scholar] [CrossRef]
- Zhong, Y.; Yuan, J.; Wang, M.; Li, J. Phase Diagrams of Binary Systems Mg(NO3)2-KNO3, Mg(NO3)2-LiNO3 and Ternary System Mg(NO3)2-LiNO3-NaNO3. J. Chem. Eng. Data 2020, 65, 3420–3427. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, G.; Bowron, D.T.; Zhu, F.; Hannon, A.C.; Zhou, Y.; Liu, X.; Shi, G. Unveiling the Structure of Aqueous Magnesium Nitrate Solutions by Combining X-ray Diffraction and Theoretical Calculations. Phys. Chem. Chem. Phys. 2022, 24, 22939–22949. [Google Scholar] [CrossRef]
- Castkova, K.; Kastyl, J.; Sobola, D.; Petrus, J.; Stastna, E.; Riha, D.; Tofel, P. Structure–Properties Relationship of Electrospun PVDF Fibers. Nanomaterials 2020, 10, 1221. [Google Scholar] [CrossRef]
- Chang, G.; Pan, X.; Hao, Y.; Du, W.; Wang, S.; Zhou, Y.; Yang, J.; He, Y. PVDF/ZnO Piezoelectric Nanofibers Designed for Monitoring of Internal Micro-Pressure. RSC Adv. 2024, 14, 11775–11783. [Google Scholar] [CrossRef]
- Zhang, J.; Yang, T.; Tian, G.; Lan, B.; Deng, W.; Tang, L.; Ao, Y.; Sun, Y.; Zeng, W.; Ren, X.; et al. Spatially Confined MXene/PVDF Nanofiber Piezoelectric Electronics. Adv. Fiber Mater. 2024, 6, 133–144. [Google Scholar] [CrossRef]
- Amrutha, B.; Anand Prabu, A.; Pathak, M. Enhancing Piezoelectric Effect of PVDF Electrospun Fiber through NiO Nanoparticles for Wearable Applications. Heliyon 2024, 10, e29192. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, M.; Hu, Y.; Wang, S.; Gu, H.; Xiong, J. Ultrahigh Energy Harvesting Ability of PVDF Incorporated with 2D Halide Perovskite Nanosheets via Interface Effect. Chem. Eng. J. 2024, 497, 154558. [Google Scholar] [CrossRef]
- Yue, T.; Wang, M.; Li, X.; Zheng, M.; Liu, J.; Lin, J.; Liu, Y. Core-Sheath PVDF Hollow Porous Fibers via Coaxial Wet Spinning for Energy Harvesting. Compos. Commun. 2024, 50, 102019. [Google Scholar] [CrossRef]
- Chen, J.; Fan, J.; Livojevic, M.; Gupta, M.; Tang, T.; Ayranci, C. Enhancing Piezoelectric Properties of PVDF-HFP Composite Nanofibers with Cellulose Nanocrystals. Mater. Today Commun. 2024, 39, 108872. [Google Scholar] [CrossRef]





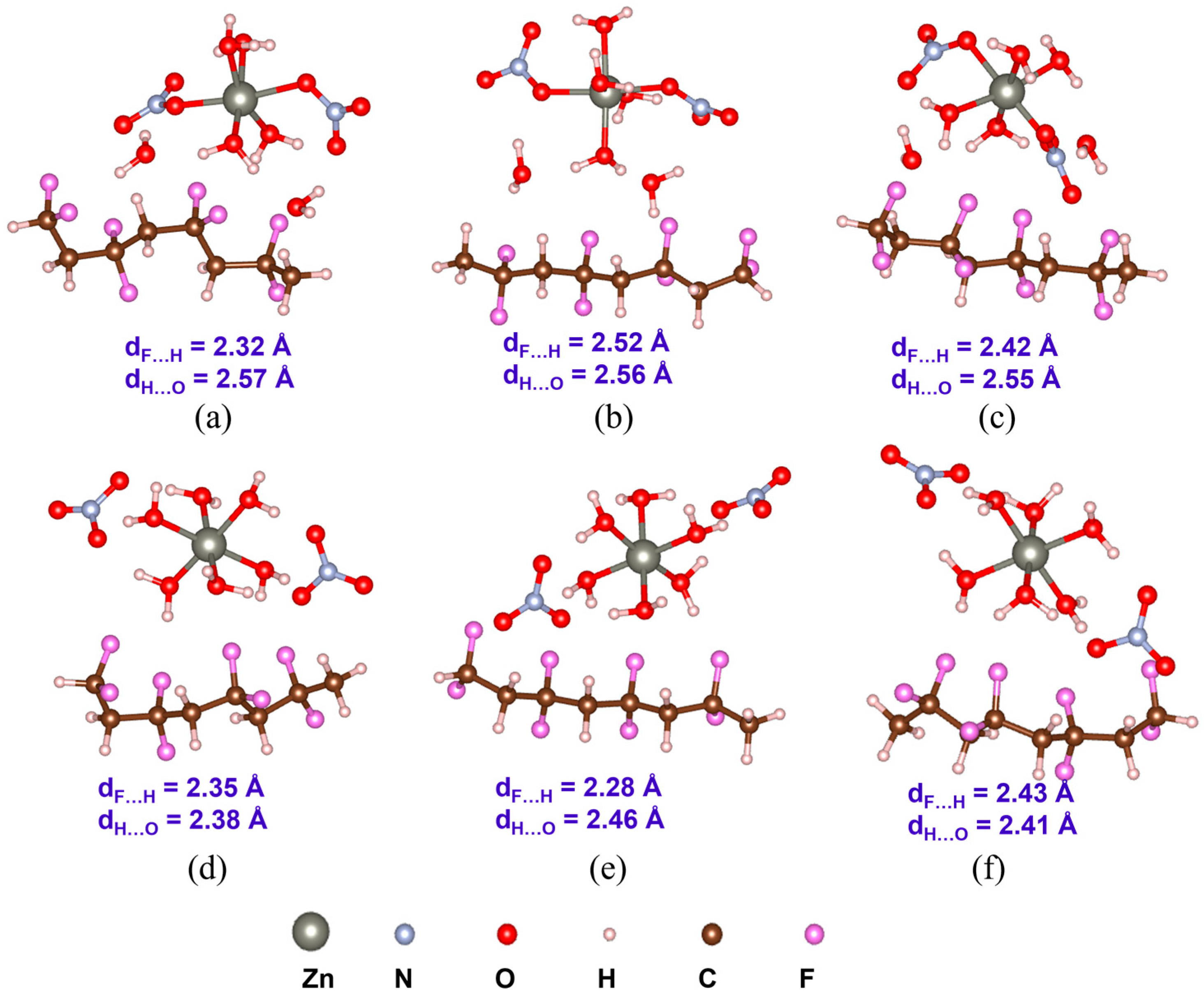
| Hydrated Salts | (kcal/mol) | (kcal/mol) | (kcal/mol) |
|---|---|---|---|
| Mg(NO3)2·6H2O | 1.88 | −96.20 | −312.90 |
| Ca(NO3)2·4H2O | −1.26 | −70.10 | −315.49 |
| Zn(NO3)2·6H2O | −1.88 | −86.88 | −313.085 |
| PVDF/Salt Complexes | (kcal/mol) | (kcal/mol) | (kcal/mol) |
|---|---|---|---|
| α-PVDF/Mg(NO3)2·6H2O | 8.16 | −5.54 | −14.03 |
| α-PVDF/Ca(NO3)2·4H2O | −0.62 | −10.48 | −11.05 |
| α-PVDF/Zn(NO3)2·6H2O | −1.26 | −9.11 | −12.59 |
| β-PVDF/Mg(NO3)2·6H2O | 9.41 | −10.59 | −18.42 |
| β-PVDF/Ca(NO3)2·4H2O | −2.51 | −21.55 | −20.31 |
| β-PVDF/Zn(NO3)2·6H2O | 7.53 | −9.20 | −18.66 |
| γ-PVDF/Mg(NO3)2·6H2O | −2.51 | −15.85 | −11.56 |
| γ-PVDF/Ca(NO3)2·4H2O | 0.62 | −10.46 | −12.48 |
| γ-PVDF/Zn(NO3)2·6H2O | −2.51 | −17.22 | −16.85 |
| Material | Pressure | Voltage | Current | Reference |
|---|---|---|---|---|
| PVDF + (Zn(NO3)2·6H2O) | 22.7 kPa | 0.4 V | 57 nA | This study |
| PVDF + (Ca(NO3)2·4H2O) | 22.7 kPa | 0.41 V | 58 nA | This study |
| PVDF + (Mg(NO3)2·6H2O) | 22.7 kPa | 0.48 V | 120 nA | This study |
| PVDF + ZnO | ∼1.13 kPa | 0.51 ± 0.12 V | ∼190 nA | [23] |
| PVDF + MXene | 200 kPa | 3.15 V | 134 nA | [24] |
| PVDF + NiO | 9.81 Pa | 5.5 V | 1830 nA | [25] |
| PVDF + CsPbBr3 | 100 MPa | 120 V | 35 µA | [26] |
| PVDF + Cu@AgNP | 7.3 MPa | 0.147 V | 0.42 nA | [27] |
| PVDF + cellulose nanocrystal | 15 kPa | 0.146 mV | 0.009 nA | [28] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dallaev, R.; Sarkar, R.; Selimov, D.; Papež, N.; Kočková, P.; Schubert, R.; Častková, K.; Orudzhev, F.; Ramazanov, S.; Holcman, V. Correlation of Dielectric Properties and Vibrational Spectra of Composite PVDF/Salt Fibers. Polymers 2024, 16, 2412. https://doi.org/10.3390/polym16172412
Dallaev R, Sarkar R, Selimov D, Papež N, Kočková P, Schubert R, Častková K, Orudzhev F, Ramazanov S, Holcman V. Correlation of Dielectric Properties and Vibrational Spectra of Composite PVDF/Salt Fibers. Polymers. 2024; 16(17):2412. https://doi.org/10.3390/polym16172412
Chicago/Turabian StyleDallaev, Rashid, Ranjini Sarkar, Daud Selimov, Nikola Papež, Pavla Kočková, Richard Schubert, Klara Častková, Farid Orudzhev, Shikhgasan Ramazanov, and Vladimír Holcman. 2024. "Correlation of Dielectric Properties and Vibrational Spectra of Composite PVDF/Salt Fibers" Polymers 16, no. 17: 2412. https://doi.org/10.3390/polym16172412
APA StyleDallaev, R., Sarkar, R., Selimov, D., Papež, N., Kočková, P., Schubert, R., Častková, K., Orudzhev, F., Ramazanov, S., & Holcman, V. (2024). Correlation of Dielectric Properties and Vibrational Spectra of Composite PVDF/Salt Fibers. Polymers, 16(17), 2412. https://doi.org/10.3390/polym16172412









