Investigations of the Laser Ablation Mechanism of PMMA Microchannels Using Single-Pass and Multi-Pass Laser Scans
Abstract
1. Introduction
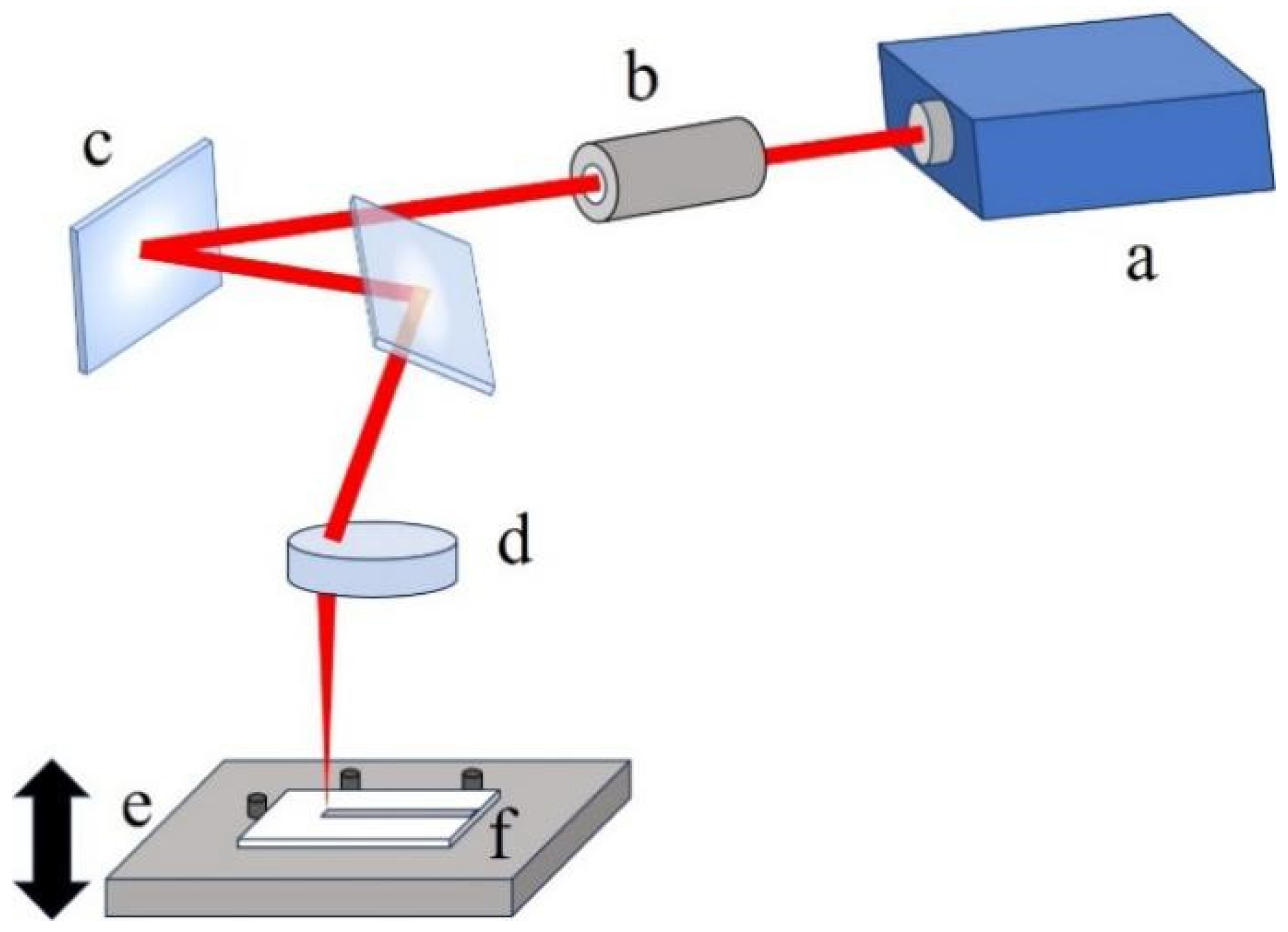
2. Experimental Set-Up and Procedure
3. Experimental Results and Discussion
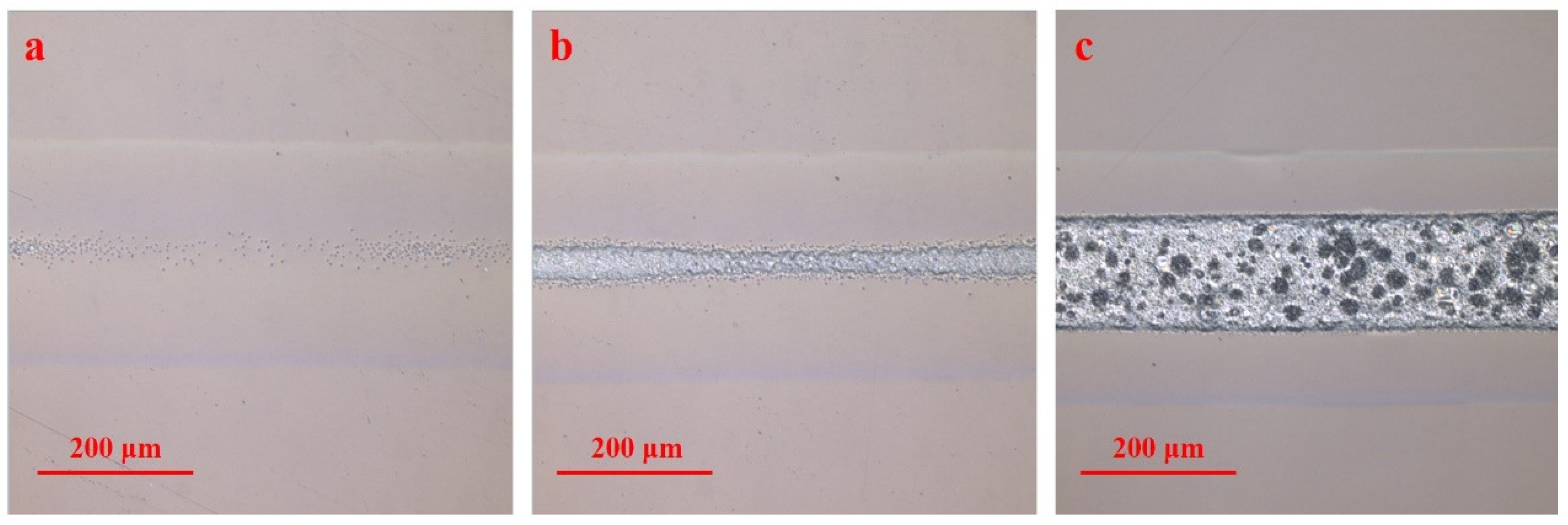
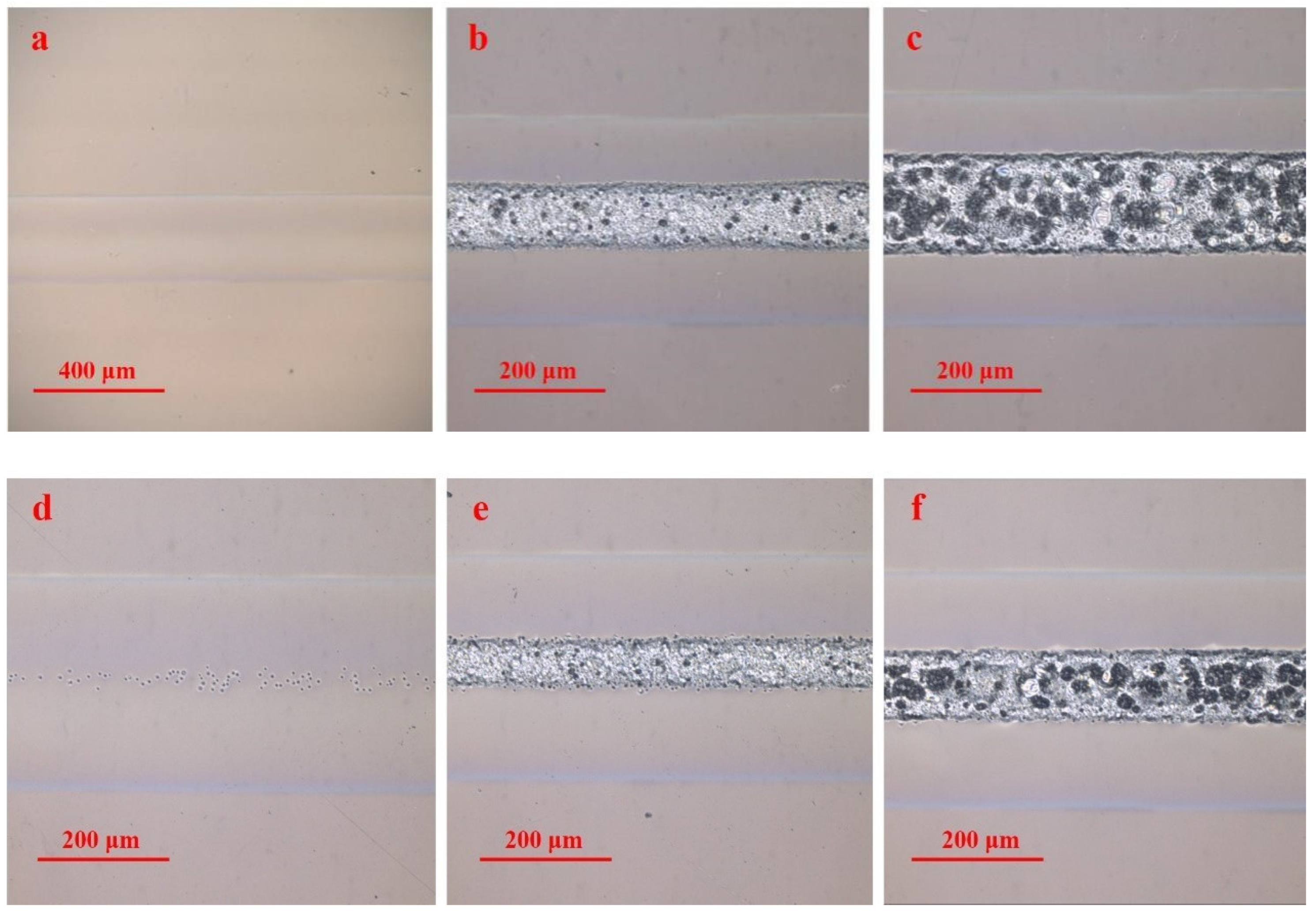
3.1. Laser Ablation Threshold
3.2. Thermal Decomposition Behavior of PMMA
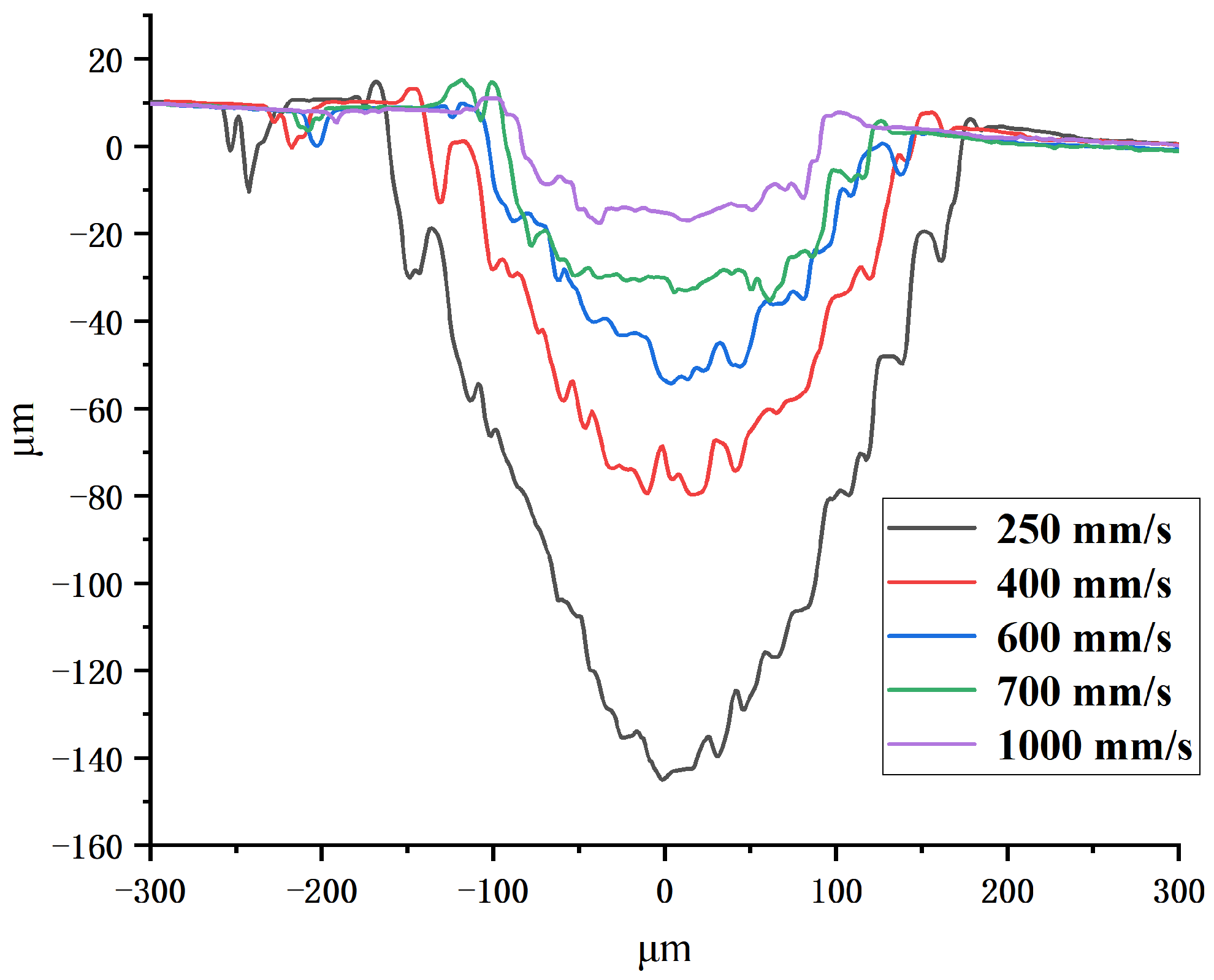
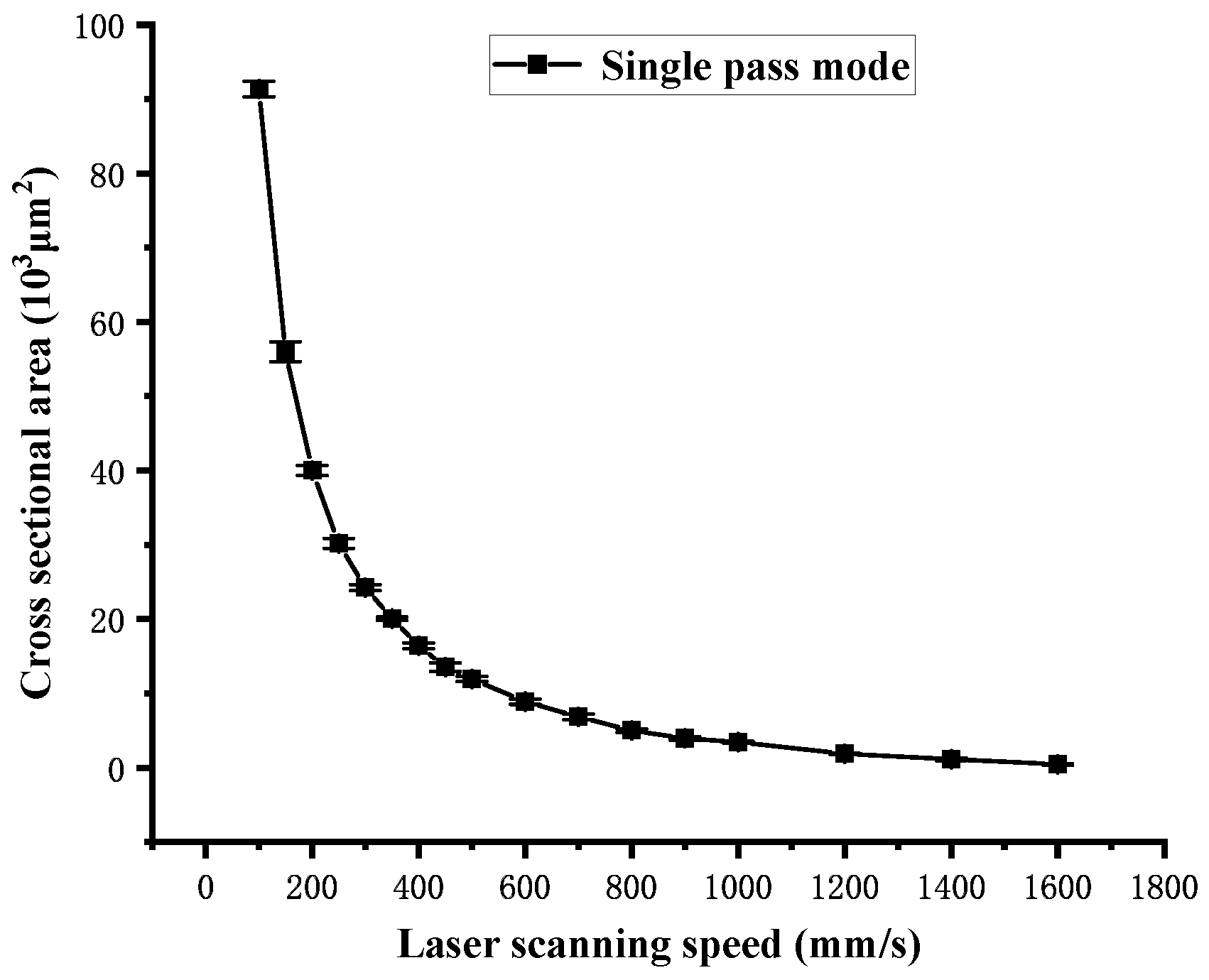
3.3. The Profile, Width, and Depth of the Channel
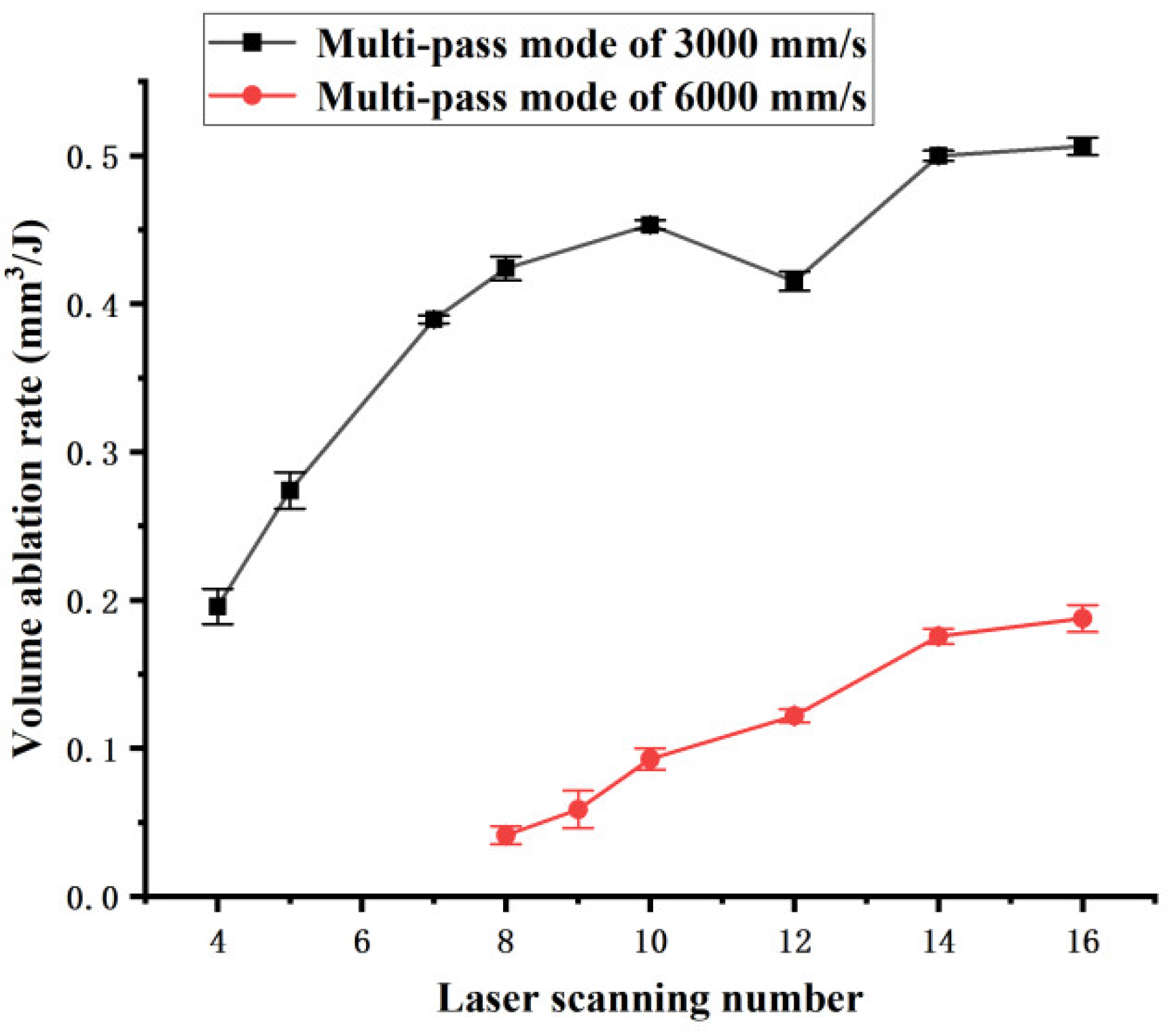
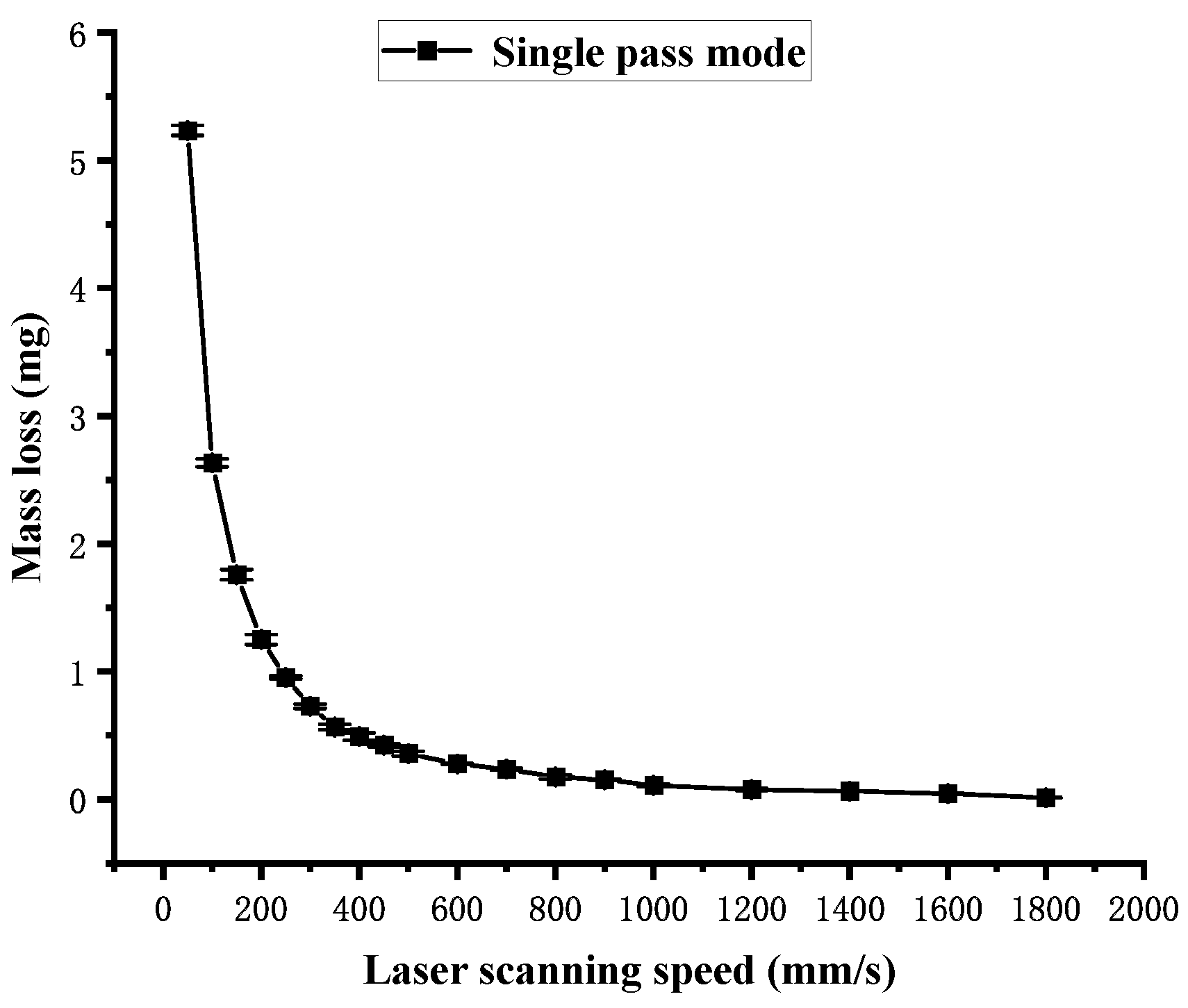
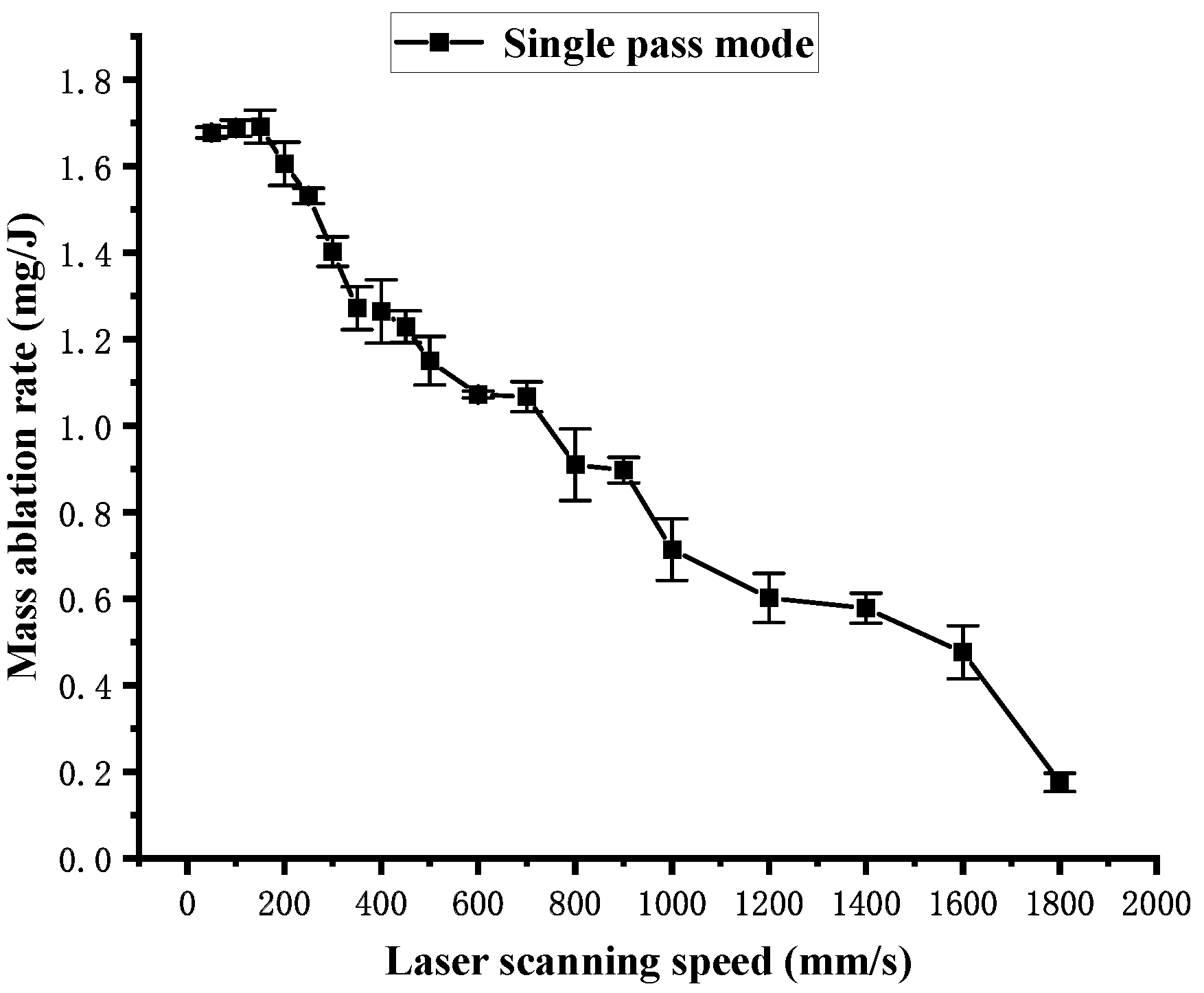
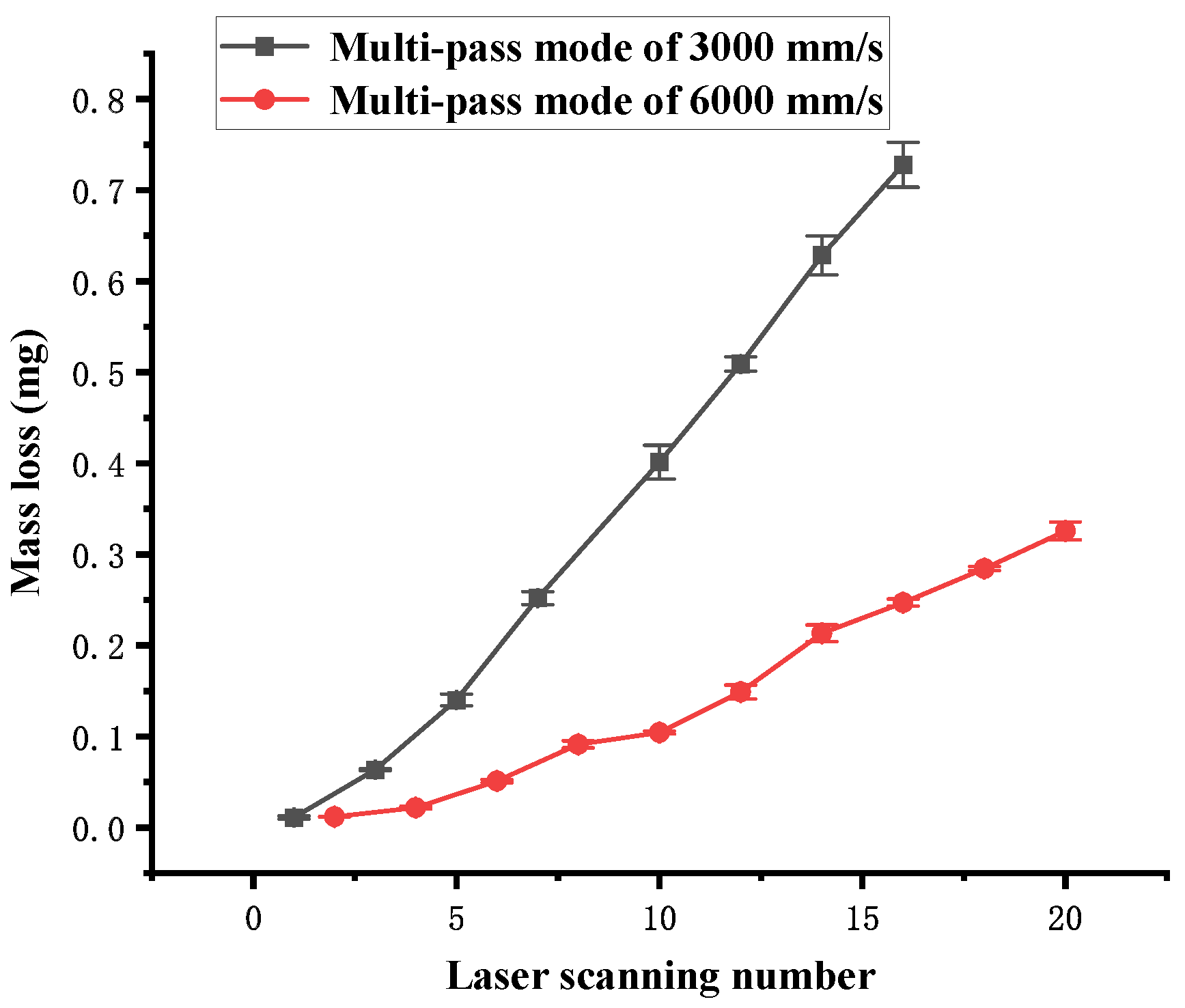
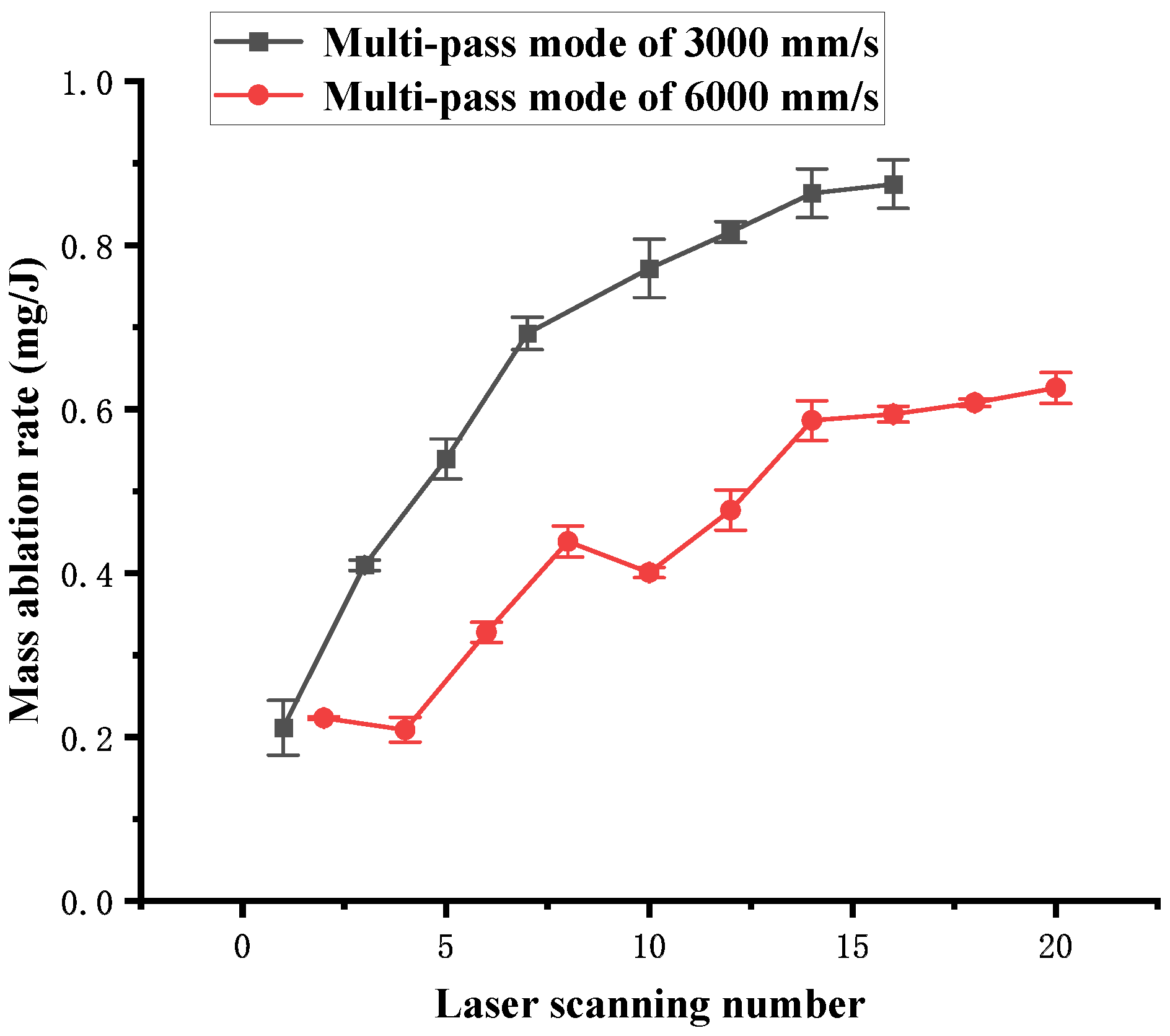
3.4. Volume Ablation Rate and Mass Ablation Rate
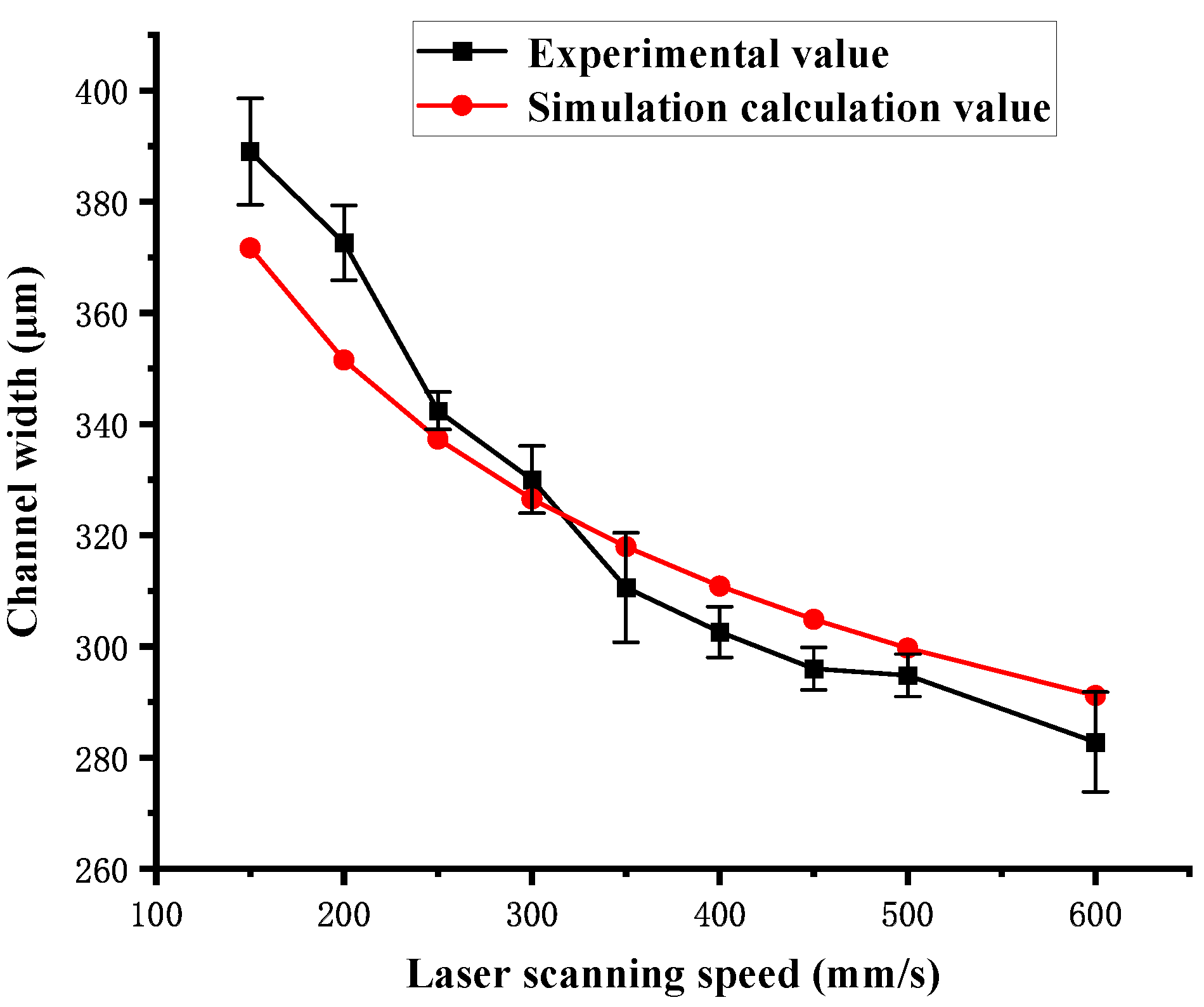
4. Simulation Calculation
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Abendroth, J.M.; Bushuyev, O.S.; Weiss, P.S.; Barrett, C.J. Controlling motion at the nanoscale: Rise of the molecular machines. ACS Nano 2015, 9, 7746–7768. [Google Scholar]
- Xia, Y.; Si, J.; Li, Z. Fabrication techniques for microfluidic paper-based analytical devices and their applications for biological testing: A review. Biosens. Bioelectron. 2016, 77, 774–789. [Google Scholar]
- Kam, W.; Ong, Y.; Lim, W.; Zakaria, R. Laser ablation and waveguide fabrication using CR39 polymer. Opt. Lasers Eng. 2014, 55, 1–4. [Google Scholar]
- Ma, X.; Li, R.; Jin, Z.; Fan, Y.; Zhou, X.; Zhang, Y. Injection molding and characterization of PMMA-based microfluidic devices. Microsyst. Technol. 2020, 26, 1317–1324. [Google Scholar]
- Tanveer, M.; Ambreen, T.; Khan, H.; Kim, G.M.; Park, C.W. Paper-based microfluidic fuel cells and their applications: A prospective review. Energy Convers. Manag. 2022, 264, 115732. [Google Scholar]
- Bruijns, B.; Van Asten, A.; Tiggelaar, R.; Gardeniers, H. Microfluidic devices for forensic DNA analysis: A review. Biosensors 2016, 6, 41. [Google Scholar]
- Al-Aqbi, Z.T.; Yap, Y.C.; Li, F.; Breadmore, M.C. Integrated microfluidic devices fabricated in poly (methyl methacrylate)(PMMA) for on-site therapeutic drug monitoring of aminoglycosides in whole blood. Biosensors 2019, 9, 19. [Google Scholar]
- Goya, K.; Yamachoshi, Y.; Fuchiwaki, Y.; Tanaka, M.; Ooie, T.; Abe, K.; Kataoka, M. Femtosecond laser direct fabrication of micro-grooved textures on a capillary flow immunoassay microchip for spatially-selected antibody immobilization. Sens. Actuators B Chem. 2017, 239, 1275–1281. [Google Scholar]
- Su, Y.; Song, Y.; Xiang, L. Continuous-flow microreactors for polymer synthesis: Engineering principles and applications. In Accounts on Sustainable Flow Chemistry; Springer: Cham, Switzerland, 2020; pp. 147–190. [Google Scholar]
- Van Erp, R.; Kampitsis, G.; Matioli, E. A manifold microchannel heat sink for ultra-high power density liquid-cooled converters. In Proceedings of the 2019 IEEE Applied Power Electronics Conference and Exposition (APEC), Anaheim, CA, USA, 17–21 March 2019; pp. 1383–1389. [Google Scholar]
- Chen, P.-C.; Pan, C.-W.; Lee, W.-C.; Li, K.-M. An experimental study of micromilling parameters to manufacture microchannels on a PMMA substrate. Int. J. Adv. Manuf. Technol. 2014, 71, 1623–1630. [Google Scholar]
- Kim, M.; Moon, B.-U.; Hidrovo, C.H. Enhancement of the thermo-mechanical properties of PDMS molds for the hot embossing of PMMA microfluidic devices. J. Micromech. Microeng. 2013, 23, 095024. [Google Scholar]
- Marson, S.; Attia, U.M.; Lucchetta, G.; Wilson, A.; Alcock, J.R.; Allen, D.M. Flatness optimization of micro-injection moulded parts: The case of a PMMA microfluidic component. J. Micromech. Microeng. 2011, 21, 115024. [Google Scholar]
- Kotz, F.; Arnold, K.; Wagner, S.; Bauer, W.; Keller, N.; Nargang, T.M.; Helmer, D.; Rapp, B.E. Liquid PMMA: A high resolution polymethylmethacrylate negative photoresist as enabling material for direct printing of microfluidic chips. Adv. Eng. Mater. 2018, 20, 1700699. [Google Scholar]
- Pan, C.; Chen, K.; Liu, B.; Ren, L.; Wang, J.; Hu, Q.; Liang, L.; Zhou, J.; Jiang, L. Fabrication of micro-texture channel on glass by laser-induced plasma-assisted ablation and chemical corrosion for microfluidic devices. J. Mater. Process. Technol. 2017, 240, 314–323. [Google Scholar]
- Rodríguez, C.F.; Guzmán-Sastoque, P.; Gantiva-Diaz, M.; Gómez, S.C.; Quezada, V.; Muñoz-Camargo, C.; Osma, J.F.; Reyes, L.H.; Cruz, J.C. Low-cost inertial microfluidic device for microparticle separation: A laser-Ablated PMMA lab-on-a-chip approach without a cleanroom. HardwareX 2023, 16, e00493. [Google Scholar] [PubMed]
- Dudala, S.; Rao, L.T.; Dubey, S.K.; Javed, A.; Goel, S. Experimental characterization to fabricate CO2 laser ablated PMMA microchannel with homogeneous surface. Mater. Today Proc. 2020, 28, 804–807. [Google Scholar]
- Hu, Z.; Chen, X.; Ren, Y. A study on the surface qualities of four polymer substrate microchannels using CO2 laser for microfluidic chip. Surf. Rev. Letters. 2019, 26, 1850160. [Google Scholar]
- Rakebrandt, J.-H.; Zheng, Y.; Besser, H.; Scharnweber, T.; Seifert, H.J.; Pfleging, W. Laser-assisted surface processing for functionalization of polymers on micro-and nano-scale. Microsyst. Technol. 2020, 26, 1085–1091. [Google Scholar]
- Go’mez, D.; Goenaga, I.; Lizuain, I.; Ozaita, M. Femtosecond laser ablation for microfluidics. Opt. Eng. 2005, 44, 051105. [Google Scholar]
- Singh, S.S.; Samuel, G. Near-infrared femtosecond laser direct writing of microchannel and controlled surface wettability. Opt. Laser Technol. 2024, 170, 110214. [Google Scholar]
- Agarwal, S.; Saxena, N.S.; Kumar, V. Study on effective thermal conductivity of zinc sulphide/poly (methyl methacrylate) nanocomposites. Appl. Nanosci. 2015, 5, 697–702. [Google Scholar]
- Patti, A.; Acierno, D. Thermal conductivity of polypropylene-based materials. In Polypropylene—Polymerization and Characterization of Mechanical and Thermal Properties; IntechOpen: Rijeka, Croatia, 2020. [Google Scholar]
- Gunel, E.; Basaran, C. Damage characterization in non-isothermal stretching of acrylics. Part II: Experimental validation. Mech. Mater. 2011, 43, 992–1012. [Google Scholar]
- Sojiphan, K. Finite Element Modeling of Residual Stress Formation in Polycarbonate Welds. Ph.D. Thesis, Ohio State University, Columbus, OH, USA, 2008. [Google Scholar]
- Passaglia, E.; Kevorkian, H.K. Specific heat of atactic and isotactic polypropylene and the entropy of the glass. J. Appl. Phys. 1963, 34, 90–97. [Google Scholar]
- Moghadasi, K.; Tamrin, K.F.; Sheikh, N.A.; Jawaid, M. A numerical failure analysis of laser micromachining in various thermoplastics. Int. J. Adv. Manuf. Technol. 2021, 117, 523–538. [Google Scholar]
- Chen, X.; Li, T.; Zhai, K.; Hu, Z.; Zhou, M. Using orthogonal experimental method optimizing surface quality of CO2 laser cutting process for PMMA microchannels. Int. J. Adv. Manuf. Technol. 2017, 88, 2727–2733. [Google Scholar]
- Bilican, I.; Tahsin Guler, M. Assessment of PMMA and polystyrene based microfluidic chips fabricated using CO2 laser machining. Appl. Surf. Science. 2020, 534, 147642. [Google Scholar]
- Yuan, D.; Das, S. Experimental and theoretical analysis of direct-write laser micromachining of polymethyl methacrylate by CO2 laser ablation. J. Appl. Phys. 2007, 101. [Google Scholar] [CrossRef]
- Prakash, S.; Kumar, S. Profile and depth prediction in single-pass and two-pass CO2 laser microchanneling processes. J. Micromech. Microeng. 2015, 25, 035010. [Google Scholar]
- Hedieh, P. Theoretical and experimental investigations of the influence of overlap between the laser beam tracks on channel profile and morphology in pulsed laser machining of polymers. Optik. 2018, 171, 431–436. [Google Scholar]
- Snakenborg, D.; Klank, H.; Kutter, J.P. Microstructure fabrication with a CO2 laser system. J. Micromech. Microeng. 2003, 14, 182. [Google Scholar]
- Romoli, L.; Tantussi, G.; Dini, G. Experimental approach to the laser machining of PMMA substrates for the fabrication of microfluidic devices. Opt. Lasers Eng. 2011, 49, 419–427. [Google Scholar]
- Anjum, A.; Shaikh, A. Experimental and analytical modeling for channel profile using CO2 laser considering gaussian beam distribution. J. Eng. Res. 2023, 11, 100035. [Google Scholar]
- Anjum, A.; Shaikh, A.; Tiwari, N. Experimental investigations and modeling for multi-pass laser micro-milling by soft computing-physics informed machine learning on PMMA sheet using CO2 laser. Opt. Laser Technol. 2023, 158, 108922. [Google Scholar]
- Gómez, D.; Goenaga, I. On the incubation effect on two thermoplastics when irradiated with ultrashort laser pulses: Broadening effects when machining microchannels. Appl. Surf. Sci. 2006, 253, 2230–2236. [Google Scholar]
- Xiao, S.; Gurevich, E.L.; Ostendorf, A. Incubation effect and its influence on laser patterning of ITO thin film. Appl. Phys. A 2012, 107, 333–338. [Google Scholar]
- Singh, S.S.; Khare, A.; Joshi, S.N. Fabrication of microchannel on polycarbonate below the laser ablation threshold by repeated scan via the second harmonic of Q-switched Nd: YAG laser. J. Manuf. Process. 2020, 55, 359–372. [Google Scholar]
- Romoli, L.; Fischer, F.; Kling, R. A study on UV laser drilling of PEEK reinforced with carbon fibers. Opt. Lasers Eng. 2012, 50, 449–457. [Google Scholar]
- Ali, U.; Karim, K.J.B.A.; Buang, N.A. A review of the properties and applications of poly (methyl methacrylate) (PMMA). Polym. Rev. 2015, 55, 678–705. [Google Scholar]
- Xiang, H.; Fu, J.; Chen, Z. 3D finite element modeling of laser machining PMMA. In Proceedings of the 2006 1st IEEE International Conference on Nano/Micro Engineered and Molecular Systems, Zhuhai, China, 18–21 January 2006; pp. 942–946. [Google Scholar]









| Laser Scan Mode | Ablation Threshold (J/cm2) | Surface Retreat Threshold (J/cm2) |
|---|---|---|
| Single pass | 2.14 | 3.10 |
| Multi-pass at 3000 mm/s | 3.71 | 5.57 |
| Multi-pass at 6000 mm/s | 5.57 | 6.50 |
| Laser Scanning Speed (mm/s) | Error Percentage of Depth (%) | Error Percentage of Width (%) |
|---|---|---|
| 150 | 0.44 | 4.5 |
| 200 | 4.2 | 5.7 |
| 250 | 0.77 | 1.5 |
| 300 | 2.4 | 1.1 |
| 350 | 0.52 | 2.4 |
| 400 | 3.8 | 2.7 |
| 450 | 1.3 | 3.0 |
| 500 | 5.9 | 1.7 |
| 600 | 3.3 | 2.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Tang, R.; Li, D.; Li, F.; Chen, L.; Zhu, D.; Feng, G.; Zhang, K.; Han, B. Investigations of the Laser Ablation Mechanism of PMMA Microchannels Using Single-Pass and Multi-Pass Laser Scans. Polymers 2024, 16, 2361. https://doi.org/10.3390/polym16162361
Li X, Tang R, Li D, Li F, Chen L, Zhu D, Feng G, Zhang K, Han B. Investigations of the Laser Ablation Mechanism of PMMA Microchannels Using Single-Pass and Multi-Pass Laser Scans. Polymers. 2024; 16(16):2361. https://doi.org/10.3390/polym16162361
Chicago/Turabian StyleLi, Xiao, Rujun Tang, Ding Li, Fengping Li, Leiqing Chen, Dehua Zhu, Guang Feng, Kunpeng Zhang, and Bing Han. 2024. "Investigations of the Laser Ablation Mechanism of PMMA Microchannels Using Single-Pass and Multi-Pass Laser Scans" Polymers 16, no. 16: 2361. https://doi.org/10.3390/polym16162361
APA StyleLi, X., Tang, R., Li, D., Li, F., Chen, L., Zhu, D., Feng, G., Zhang, K., & Han, B. (2024). Investigations of the Laser Ablation Mechanism of PMMA Microchannels Using Single-Pass and Multi-Pass Laser Scans. Polymers, 16(16), 2361. https://doi.org/10.3390/polym16162361






