Study on Bonding Characteristics of Polymer Grouted Concrete-Soil Interface
Abstract
1. Introduction
2. Materials and Methods
2.1. Test Soil
2.2. Polymer Grouting Materials
2.3. Concrete Materials
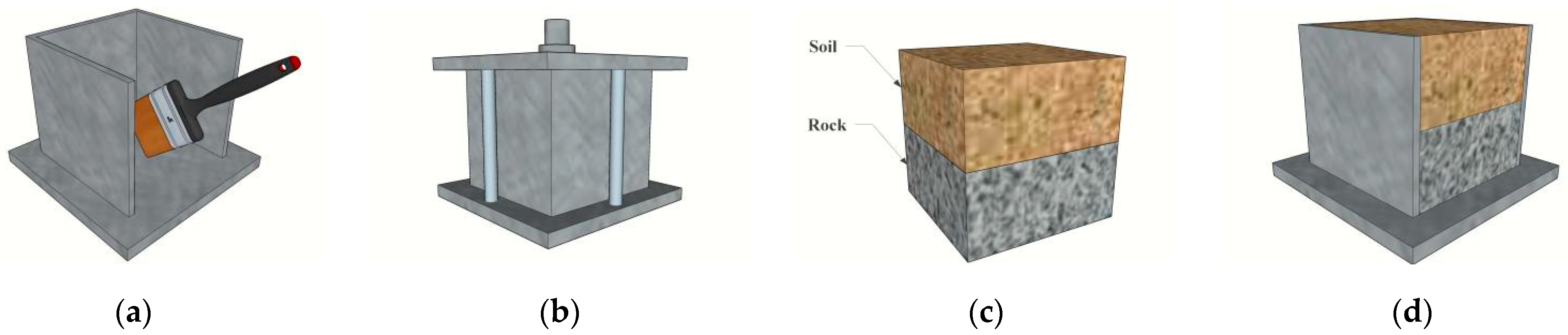
2.4. Grouting Test and Specimen Preparation Methods
- Apply a layer of mold-release oil on the inner wall of the grouting mold, affix a layer of tinfoil to facilitate the late demolding, and ensure that there are no air bubbles and wrinkles in the process of tinfoil affixing so as to prevent the influence on the outer shape of the test piece;
- The test specifications of the concrete specimen are placed on the bottom of the mold, to ensure that the concrete cutting surface faces upward and makes soil contact, and then according to the test set, the dry density of the measured test soil is poured into the mold; using the layered compaction method, each layer of the specimen height should be equal to the junction of the soil layer and should be shaved to prevent the soil stratification;
- Tighten the mold’s fixed screw, open the grouting valve above the mold, and then connect to the constant pressure grouting equipment; grouting is completed after closing the grouting valve above the mold. In this test, pressure grouting was used to bond the polymer to the soil-rock interface.
- Grouting the completed mold is performed at a constant temperature of 20 °C for the solidification reaction and hardened 3 h after the slurry; remove the model to take out the molding specimen and mark and place it in a constant temperature room for natural maintenance for 7 days.
3. Results
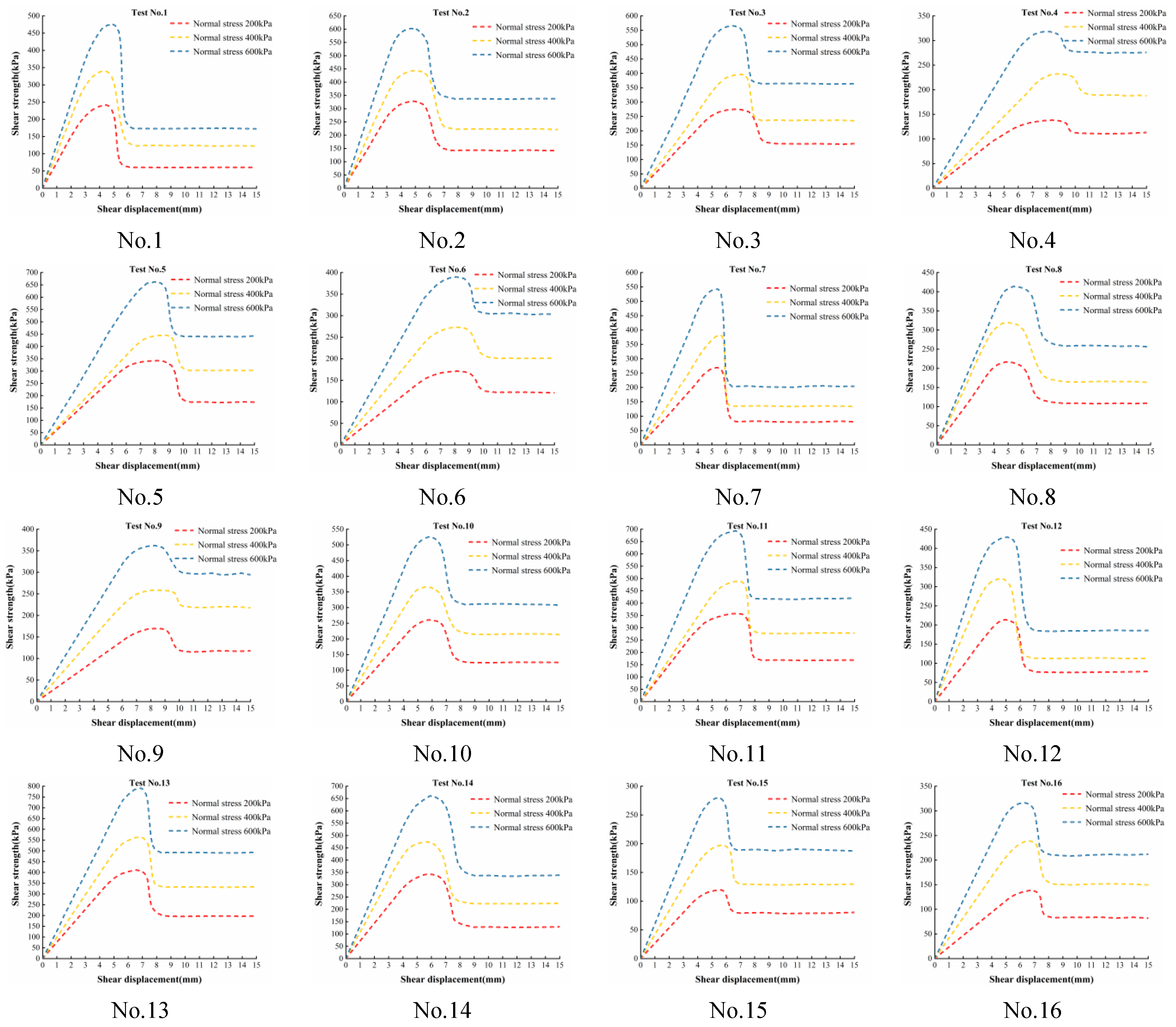
3.1. Interface Shear Strength and Shear Displacement Curves
3.2. Shear Failure Model
3.3. Study of the Whole Process of Interfacial Shear Damage
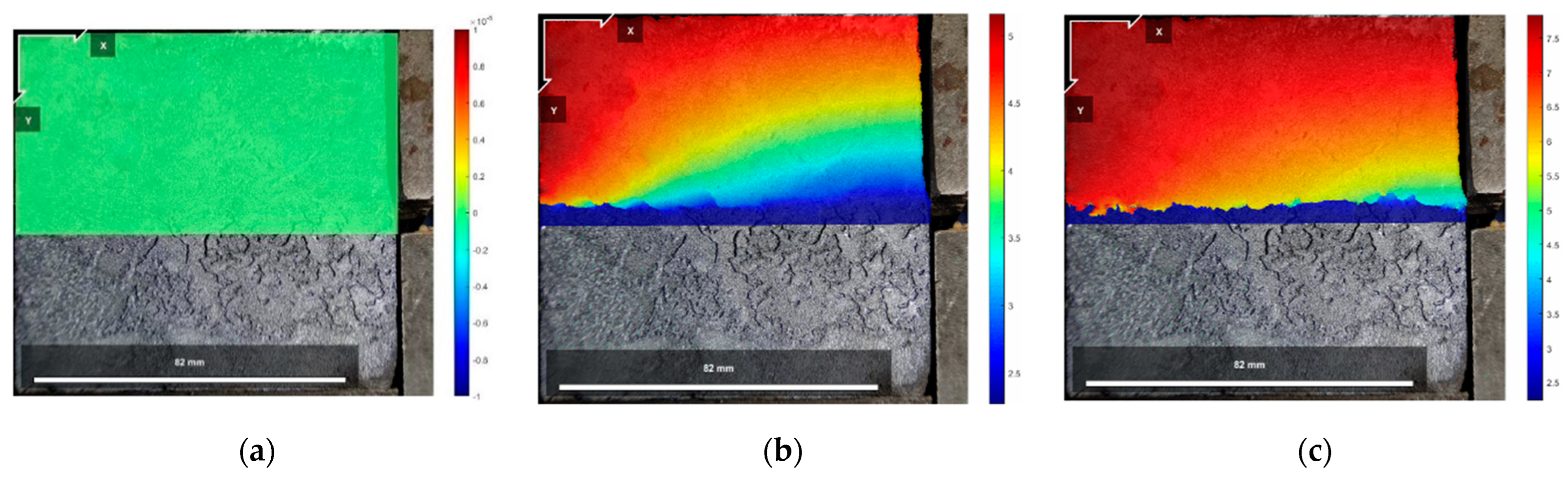
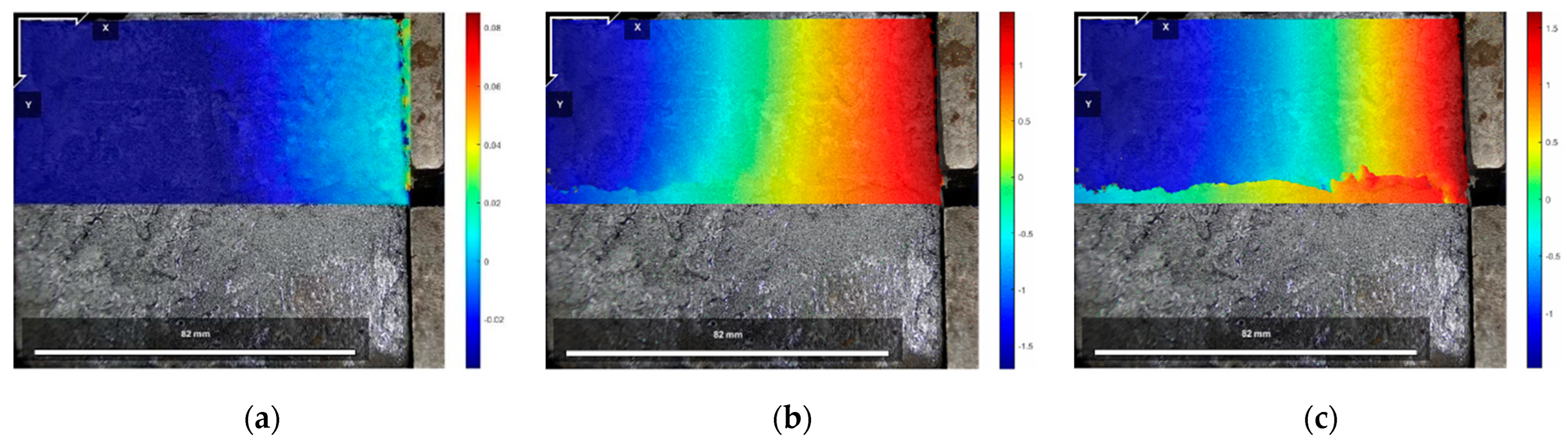
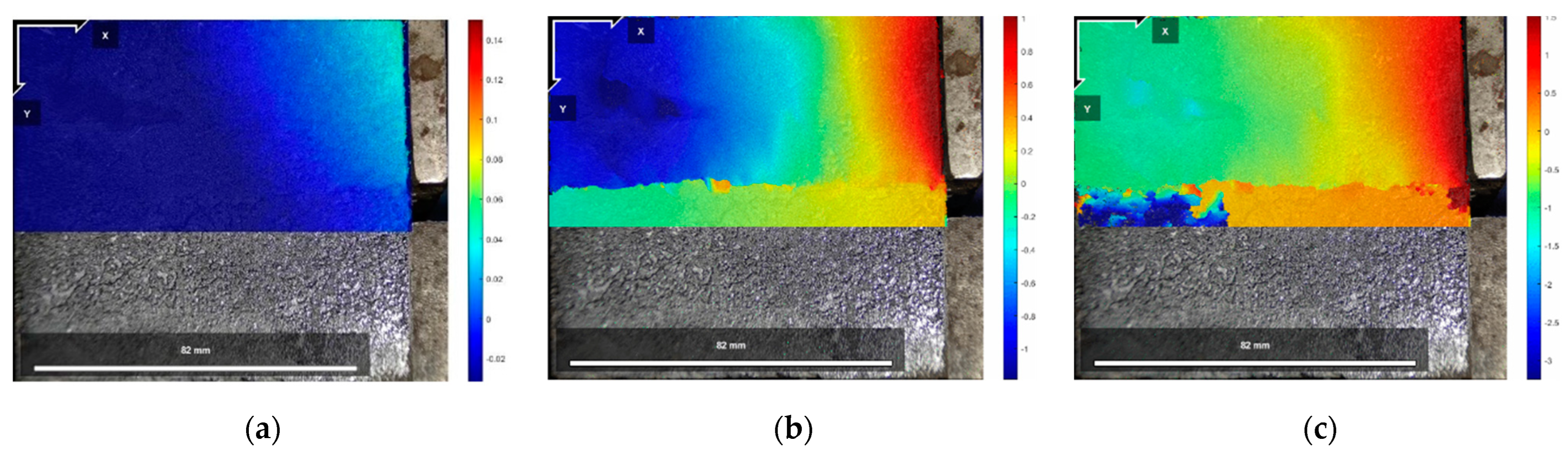
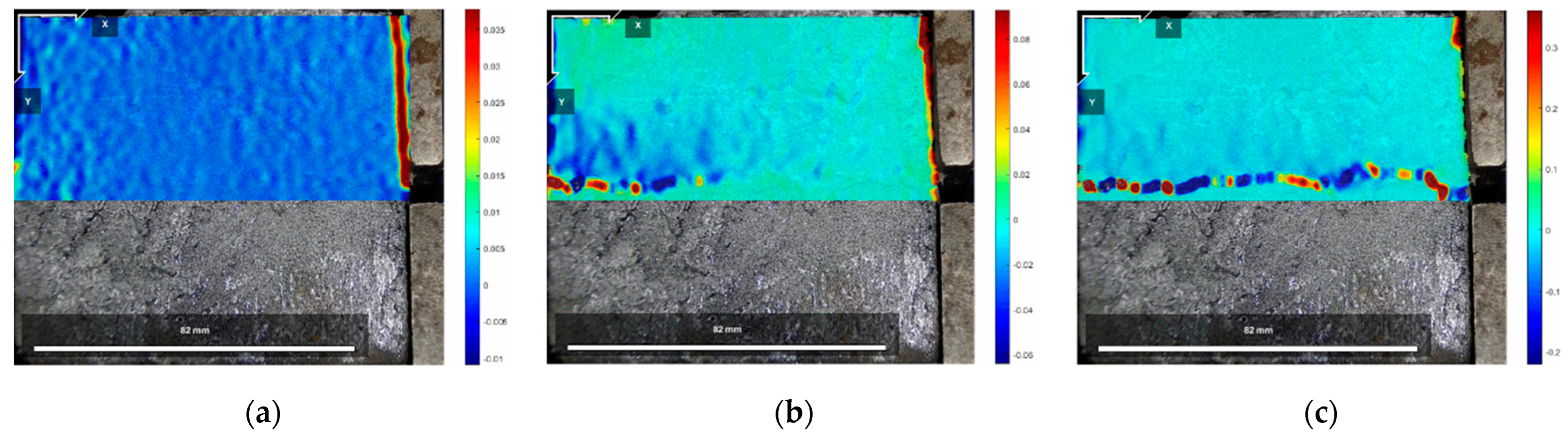
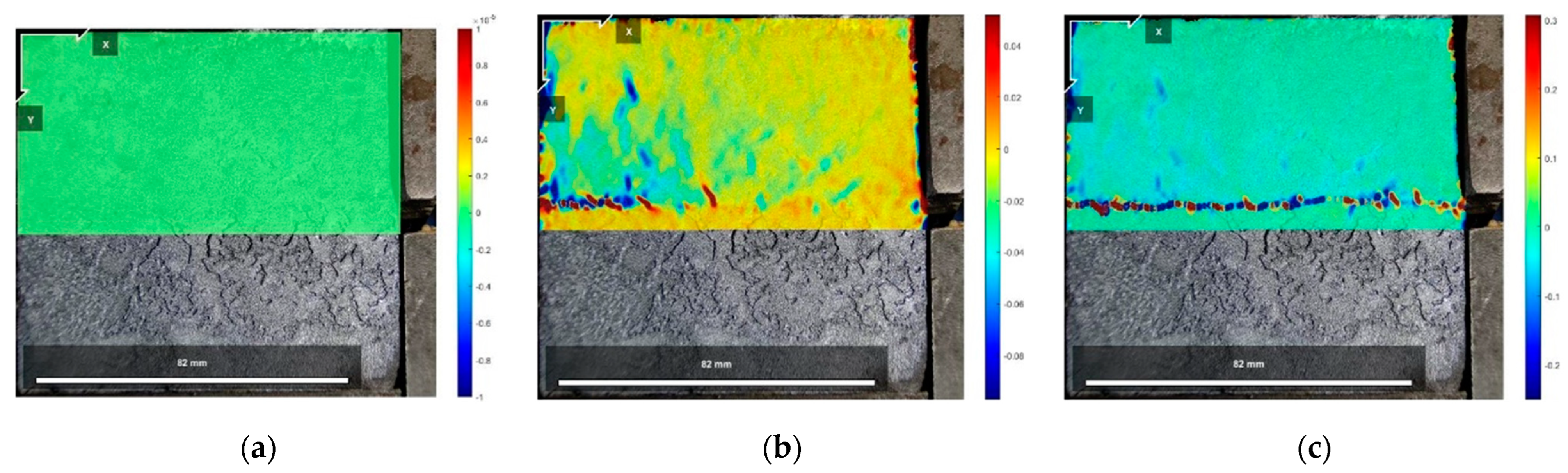
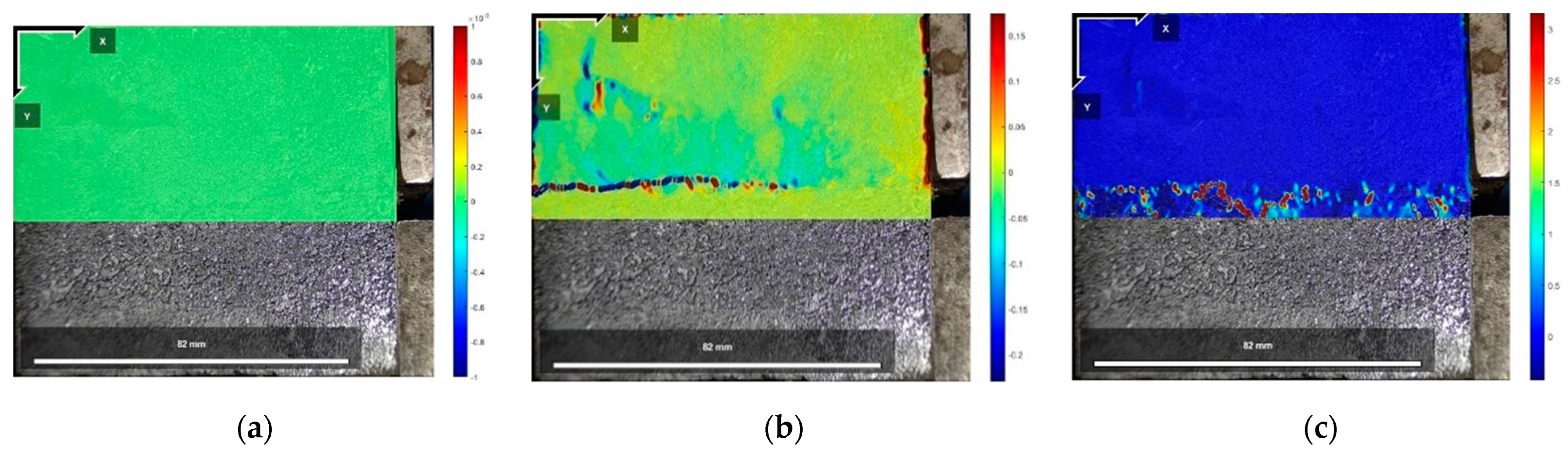
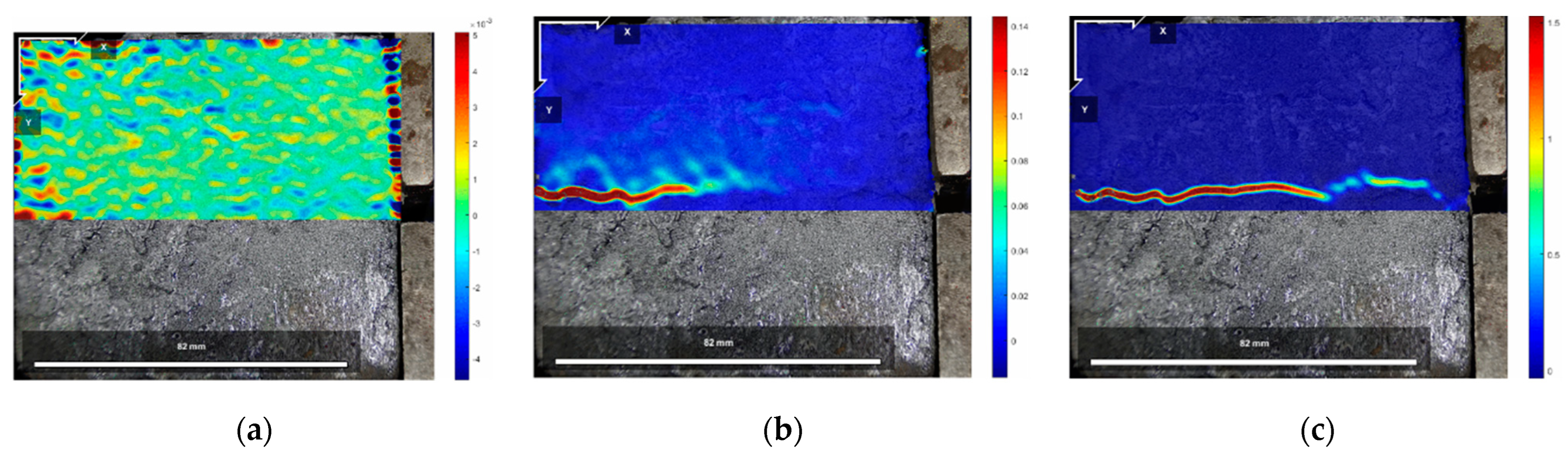
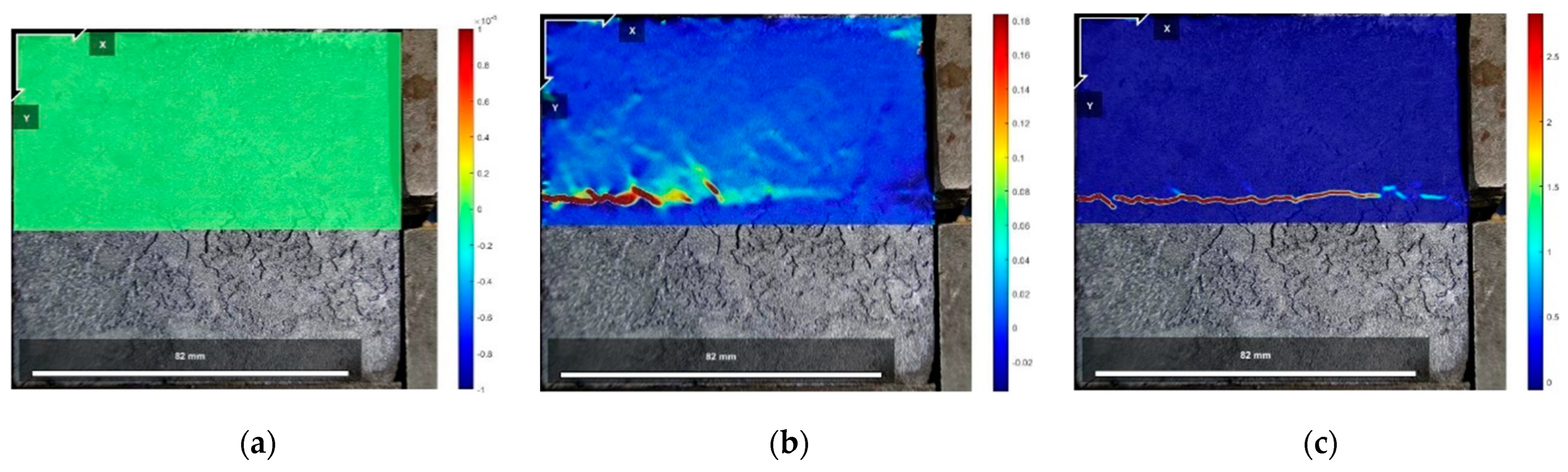
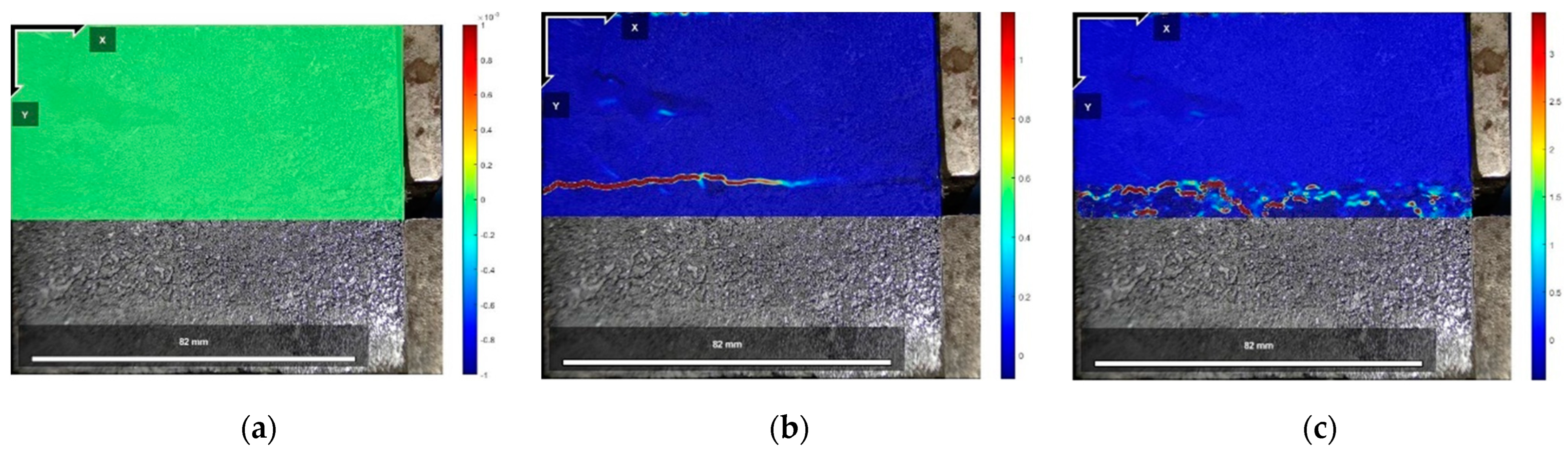
3.3.1. Displacement Analysis of the Whole Process of Interfacial Shear Damage
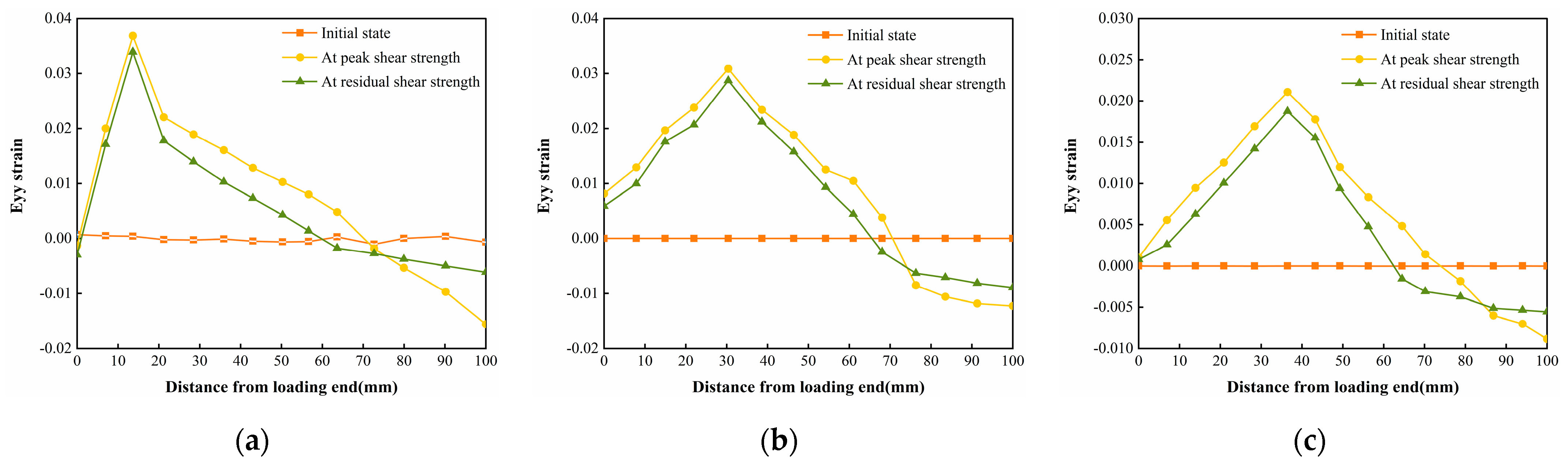
3.3.2. Strain Analysis of the Whole Process of Interfacial Shear Damage
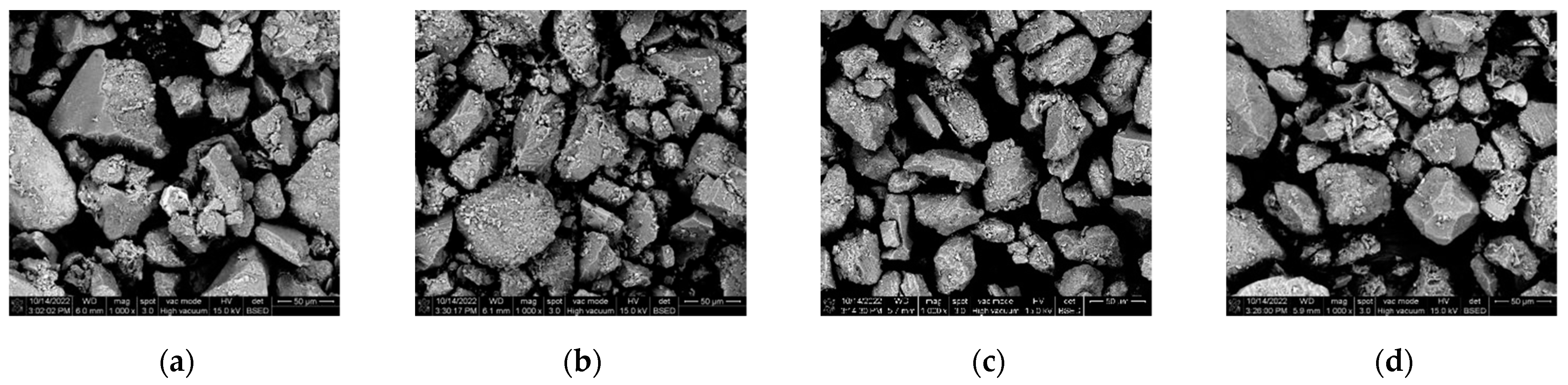
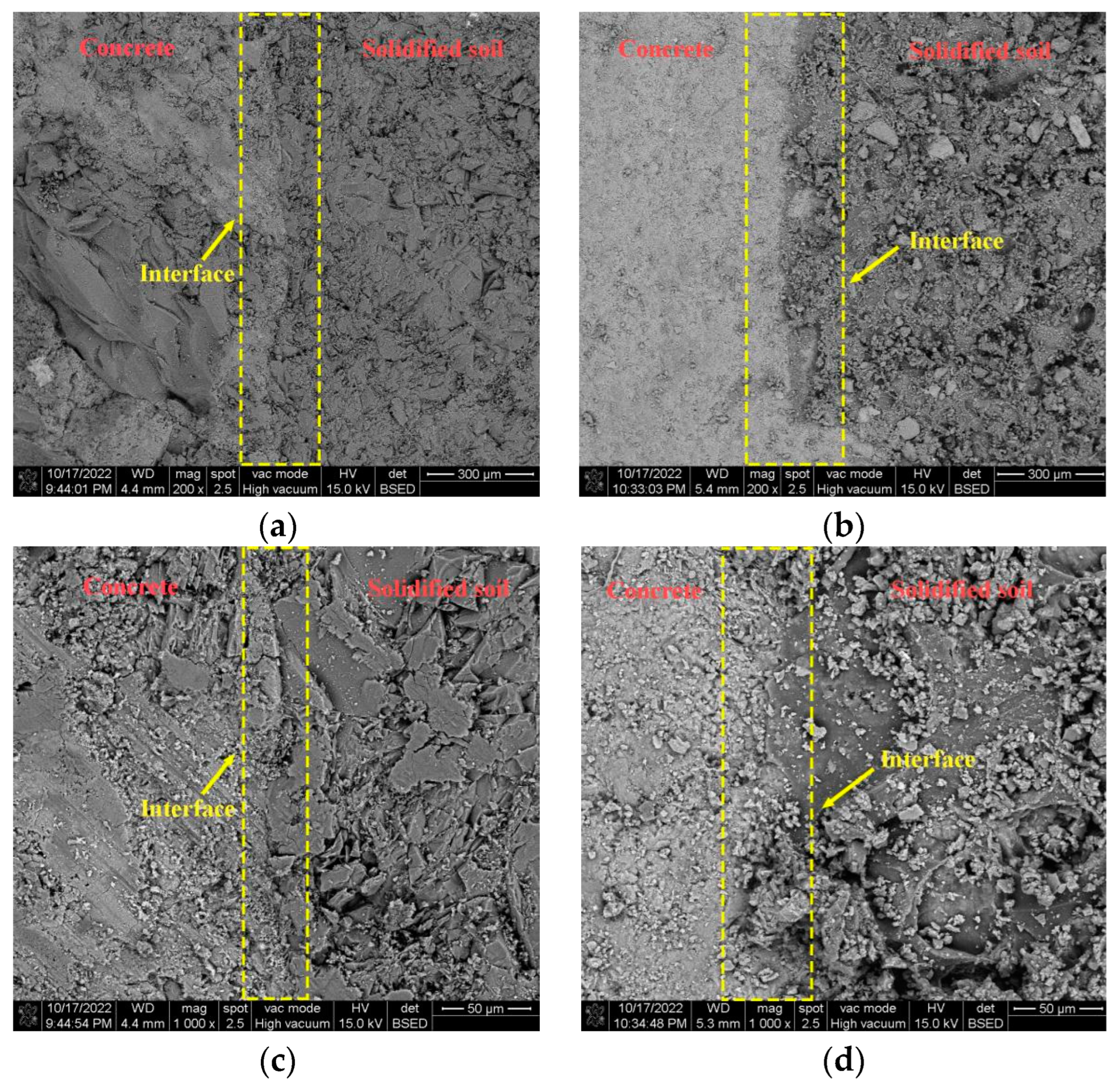
3.4. Analysis of Microtest Results
4. Conclusions
- By applying grouting pressure, the permeable polymer effectively fills the soil particle pores and infiltrates the interface between the soil and rock. The chemical reaction leads to the creation of interfacial cohesion.
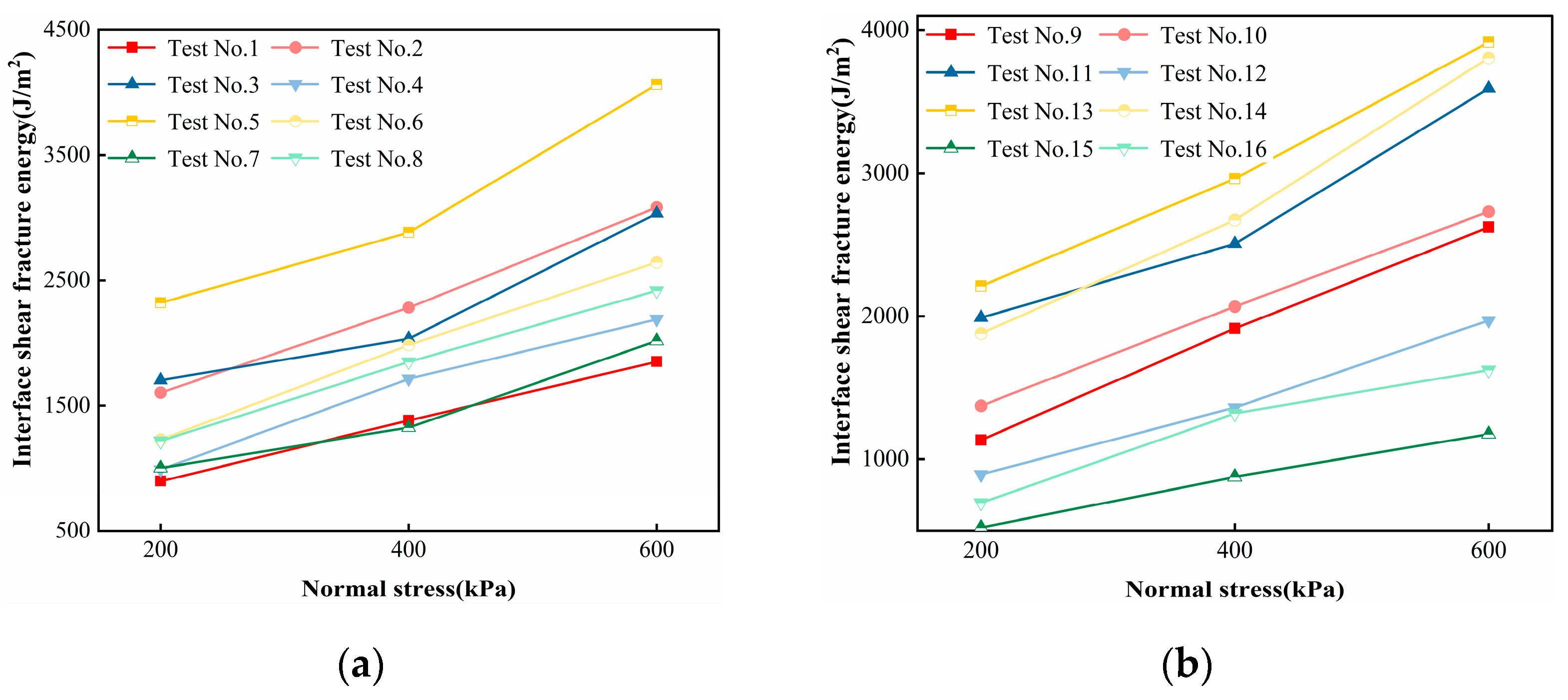
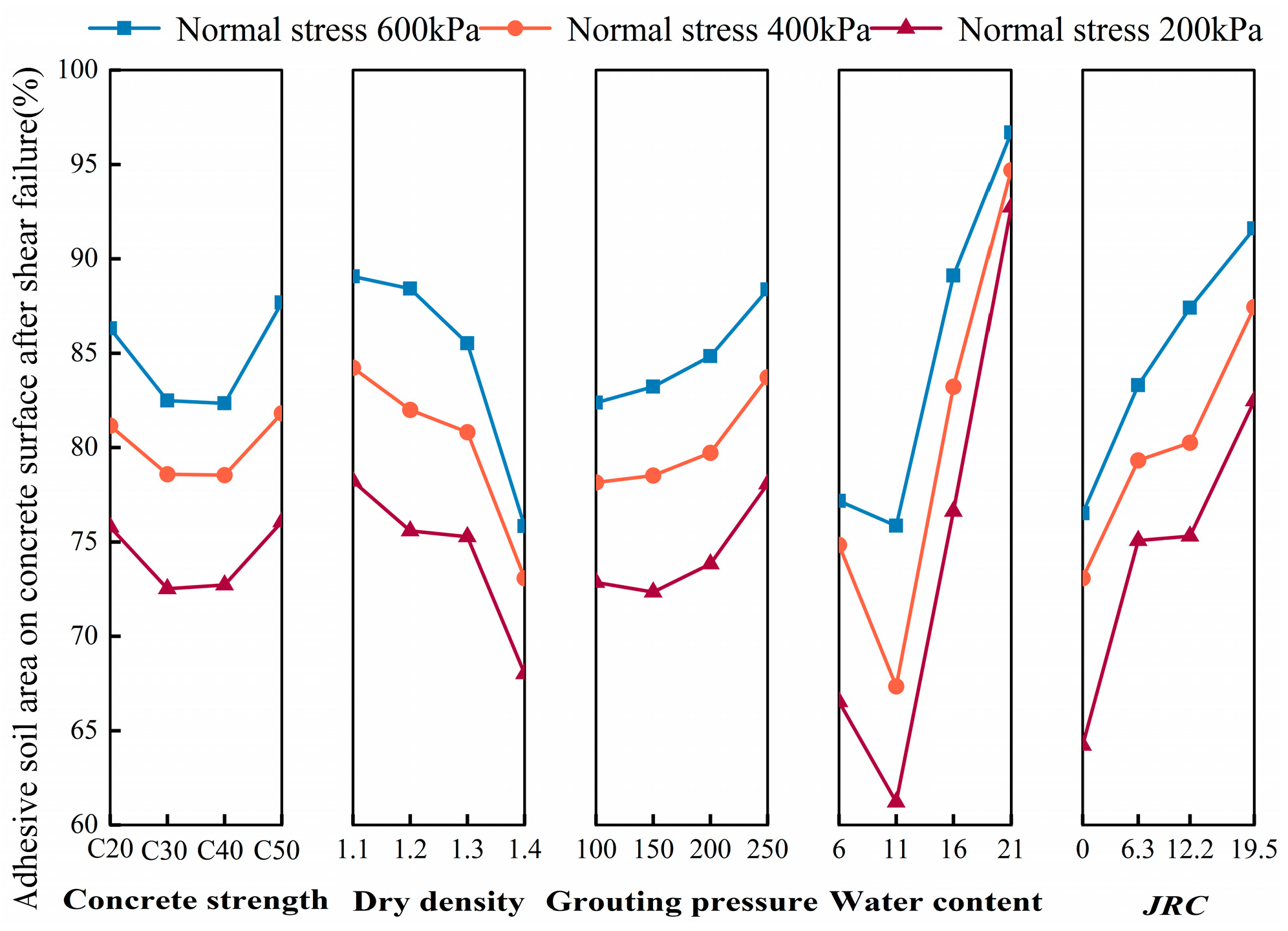
- The interfacial shear fracture energy is calculated from the interfacial shear strength-shear displacement curve, and the factors affecting the interfacial shear fracture energy are in the following order of priority: interfacial roughness > water content > dry density > grouting pressure > concrete strength, and at the same time, with the increase in the normal stress, the interfacial shear fracture energy is also increased.
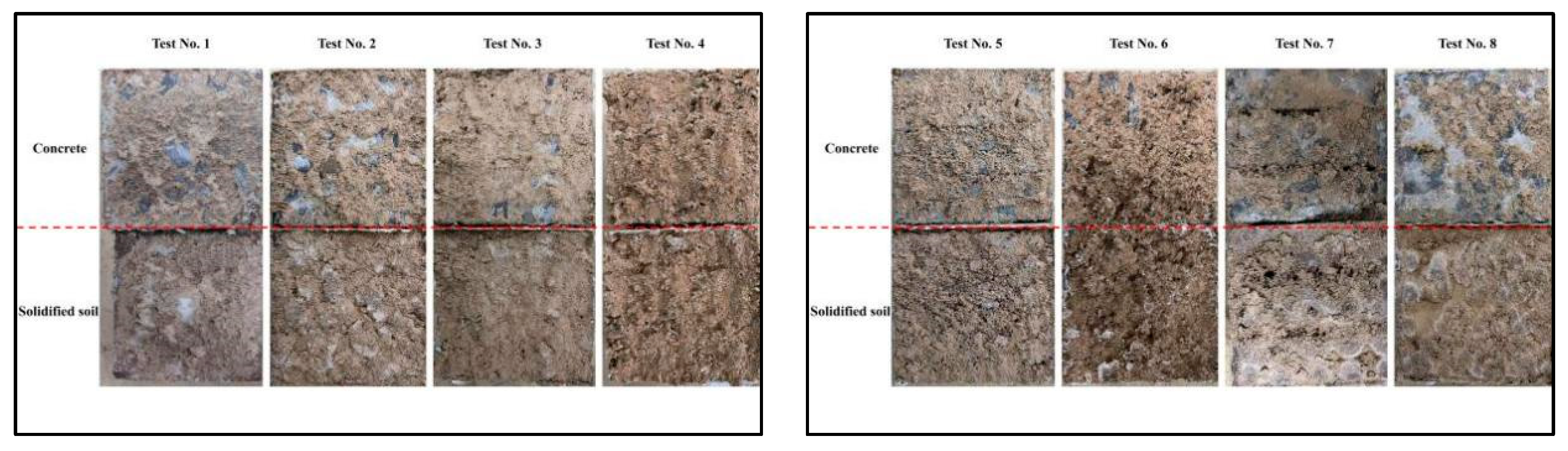
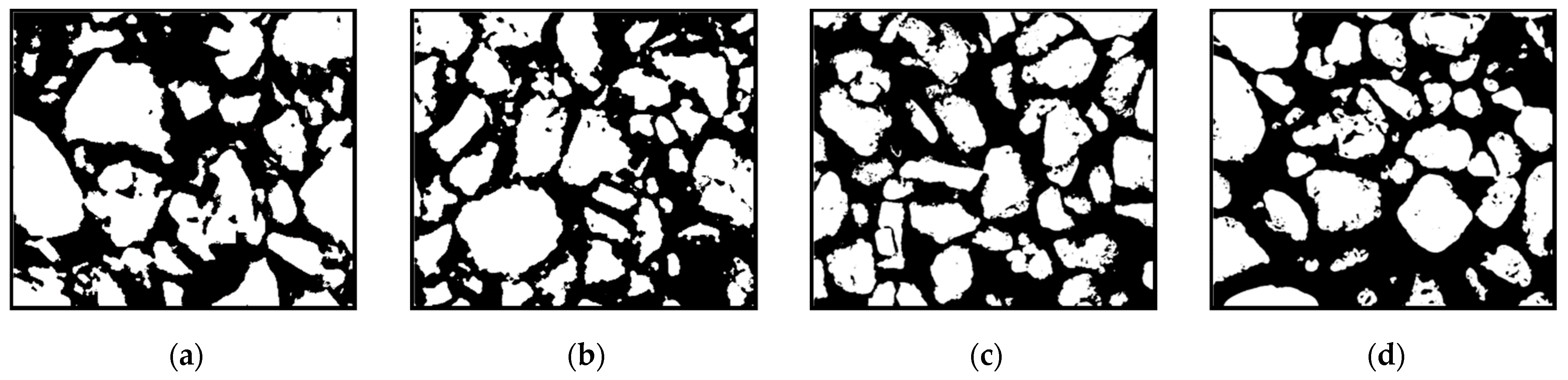
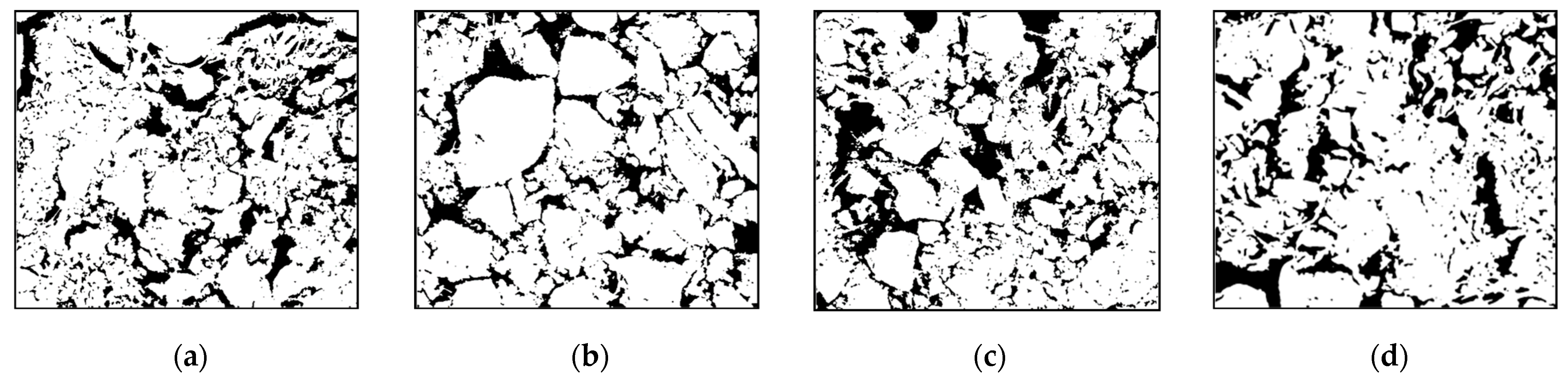
- We quantitatively analyzed the change in interface damage pattern by ImageJ, and concluded that the factors affecting the interface damage pattern are in the following order: water content > interface roughness > dry density > grouting pressure > concrete strength, and with the increase in normal stress, the percentage of the area of adherent soil on the surface of the concrete after shear damage basically shows a tendency to increase.
- Using the DIC technique in the process of the direct shear test, it can be seen through the analysis of the displacement and strain data during the whole process of interface shear damage that the horizontal displacement and horizontal compressive strain of the cured soil at the distal end of the loading end decreases gradually with the increase in normal stress in the process of shear, and the maximum vertical uplift displacement and maximum vertical tensile strain of the cured soil at the proximal end of the loading end.
- Some test numbers in the orthogonal test were selected for microtests to investigate the micro-mechanism of the concrete-soil interface after grouting. From the SEM images, it can be seen that the polymer penetrates into the concrete-soil interface and forms a cementing layer, which makes the concrete-soil interface have a strong bond, and the bonding form is in the mode of concrete-cohesive layer-cured soil.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yin, K.; Fauchille, A.-L.; Vasilescu, R.; Dano, C.; Kotronis, P.; Sciarra, G. Influence of clay fraction on the shear behavior of an interface between sand-clay mixture and concrete. Géoméch. Energy Environ. 2024, 38, 100543. [Google Scholar] [CrossRef]
- Fei, Y.; Yasufuku, N.; Zhao, C.; Zhao, C.; Xu, K. Shear behaviour of sand-concrete interface with side post-grouting considering the unloading effect. Soils Found. 2020, 60, 1440–1449. [Google Scholar] [CrossRef]
- Pan, J.; Wang, B.; Wang, Q.; Ling, X.; Fang, R.; Liu, J.; Wang, Z. Thickness of the shear band of silty clay–concrete interface based on the particle image velocimetry technique. Constr. Build. Mater. 2023, 388, 131712. [Google Scholar] [CrossRef]
- Li, J.; Li, J.; Zhang, J.; Liu, G. Static and Dynamic Properties Study on Interface between New Polymer Materials and Silty Clay Based on Ring Shear Tests. Polymers 2023, 15, 634. [Google Scholar] [CrossRef]
- Zhao, W.; Guo, C.; Wang, C.; Wang, Y.; Wang, L. Study on Mechanical Properties of Permeable Polymer Treated Loess. Materials 2022, 15, 6647. [Google Scholar] [CrossRef]
- Liang, J.; Du, X.; Fang, H.; Du, M.; Shi, M.; Gao, X.; Han, Y. Numerical and experimental study of diffusion law of foamed polymer grout in fracture considering viscosity variation of slurry. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2022, 128, 104674. [Google Scholar] [CrossRef]
- Xia, Y.; Lu, B.; Wang, J.; Wu, Y.; Gao, Y.; Zhang, C.; Wang, C.; Zhao, P.; Fang, H.; Zhang, J.; et al. Splitting tensile properties and damage characterization of polymer grouting materials: Density, geometry and size effects. Polym. Test. 2024, 132, 108358. [Google Scholar] [CrossRef]
- Li, G.; Zhou, Q.; Wang, W.; Lu, C.; Chen, C.; Guo, Z.; Lu, C. Chloride diffusion along the interface between concrete matrix and repair materials under flexural loading. Constr. Build. Mater. 2023, 372, 130829. [Google Scholar] [CrossRef]
- Wu, Y.; Zhao, C.; Zhao, C.; Wang, Y.; Fei, Y. Effect of grout conditions on the mechanical behaviors of unloading sand-concrete interface for reinforcing bored pile foundation. Constr. Build. Mater. 2020, 243, 118218. [Google Scholar] [CrossRef]
- Zhou, D.; Zhao, Z.; Li, B.; Chen, Y.; Ding, W. Permeability evolution of grout infilled fractures subjected to triaxial compression with low confining pressure. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2020, 104, 103539. [Google Scholar] [CrossRef]
- Sharghi, M.; Chakeri, H.; Ozcelik, Y. Investigation into the effects of two component grout properties on surface settlements. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2017, 63, 205–216. [Google Scholar] [CrossRef]
- Li, S.; Sha, F.; Liu, R.; Zhang, Q.; Li, Z. Investigation on fundamental properties of microfine cement and cement-slag grouts. Constr. Build. Mater. 2017, 153, 965–974. [Google Scholar] [CrossRef]
- Zheng, K.; Yang, X.; Chen, R.; Xu, L. Application of a capillary crystalline material to enhance cement grout for sealing tunnel leakage. Constr. Build. Mater. 2019, 214, 497–505. [Google Scholar] [CrossRef]
- Diagne, M.; Ndiaye, M.C.; Sow, L. Reinforcement of the ground by injection of cement grout for the installation of piles in karstified limestone zone. Case Stud. Constr. Mater. 2020, 12, e00310. [Google Scholar] [CrossRef]
- El Mohtar, C.; Jaffal, H.; Miller, A.K.; Ward, K. Implementing Digital Imaging for Improved Understanding of Microfine Cement Grout Permeation and Filtration. Geotech. Geol. Eng. 2022, 40, 4473–4485. [Google Scholar] [CrossRef]
- Guo, C.; Hu, D.; Wang, F. Diffusion Behavior of Polymer Grouting Materials in Sand and Gravel. Soil Mech. Found. Eng. 2021, 57, 440–444. [Google Scholar] [CrossRef]
- Wang, Z.; Du, M.; Fang, H.; Zhang, C.; Li, M.; Shi, M. Influence of different corrosion environments on mechanical properties of a roadbed rehabilitation polyurethane grouting material under uniaxial compression. Constr. Build. Mater. 2021, 301, 124092. [Google Scholar] [CrossRef]
- Fang, H.; Su, Y.; Du, X.; Wang, F.; Li, B. Experimental and Numerical Investigation on Repairing Effect of Polymer Grouting for Settlement of High-Speed Railway Unballasted Track. Appl. Sci. 2019, 9, 4496. [Google Scholar] [CrossRef]
- Wang, Y.; Han, M.; Cao, T.; Yu, X.; Song, Y. Cyclic interface behavior of non-water reactive polymer and concrete during dam restoration. Structures 2021, 34, 748–757. [Google Scholar] [CrossRef]
- Zhang, B.; Zhang, X.; Zhong, Y.; Li, X.; Hao, M.; Sang, X.; Wang, X.; Liu, J. Research on Fatigue Model of Semi-Rigid Base Asphalt Pavement before and after Polymer Grouting. Adv. Civ. Eng. 2021, 2021, 1–16. [Google Scholar] [CrossRef]
- Wang, C.; Zhao, W.; Guo, C.; Du, X.; Pan, Y.; Gao, J.; Gao, X. Surface Free Energy Theory for Evaluating Moisture Damage in Expandable Polyurethane Grouting Materials. Adv. Eng. Mater. 2022, 24, 2200409. [Google Scholar] [CrossRef]
- Hossain, M.A.; Yin, J.H. Behavior of a Pressure-Grouted Soil-Cement Interface in Direct Shear Tests. Int. J. Geomech. 2014, 14, 101–109. [Google Scholar] [CrossRef]
- Fang, H.; Su, Z.; Li, X.; Wang, F.; Fu, Y. Interfacial bond performance between self-expansion polymer and concrete. Constr. Build. Mater. 2021, 270, 121459. [Google Scholar] [CrossRef]
- Qin, L.; Guo, C.; Sun, W.; Guan, H.; Yan, W.; Wang, F. Experimental investigation on the interfacial shear bond performance of non-water reacting polymer and concrete. Constr. Build. Mater. 2022, 331, 127351. [Google Scholar] [CrossRef]
- Papastergiou, D.; Lebet, J.-P. Experimental investigation and modelling of the structural behaviour of confined grouted interfaces for a new steel–concrete connection. Eng. Struct. 2014, 74, 180–192. [Google Scholar] [CrossRef]
- Zhang, Q.; Deng, H.; Yi, W.; Dai, G.; Li, H.; Guo, X. Study on uplift mechanism of grouted implantation steel pipe pile by direct shear and model tests. Soils Found. 2024, 64, 101459. [Google Scholar] [CrossRef]
- Zhang, C.; Li, Z.; Wu, Y.; Wang, C.; Fang, H.; He, C.; Duan, C. Micromechanical properties of polymer-bentonite interface: A molecular dynamics study. Constr. Build. Mater. 2024, 420, 135638. [Google Scholar] [CrossRef]
- Li, P.; Shi, M.; Xia, Y.; Xue, B.; Zhang, C.; Chen, J.; Wang, J.; Pan, Y.; Wang, C.; Han, B. Study on bonding performance and load transfer model between polyurethane anchor bolts and silty soil. Constr. Build. Mater. 2023, 384, 131335. [Google Scholar] [CrossRef]
- Guo, C.; Pei, L.; Guan, H.; Chu, X.; Wang, H.; Shi, F.; An, Z.; Qin, L. Experimental study on interfacial damage mechanisms of polymer-concrete composite structure. J. Build. Eng. 2023, 75, 106968. [Google Scholar] [CrossRef]
- Wang, Y.; Wan, Y.; Guo, C.; Zeng, C.; Shao, J.; Wang, F. Experimental investigation on the monotonic, cyclic and post cyclic interfacial behavior of non-water reacted polymer and concrete. Constr. Build. Mater. 2021, 292, 123323. [Google Scholar] [CrossRef]
- Barton, N.; Choubey, V. The shear strength of rock joints in theory and practice. Rock Mech. Felsmech. Mécanique Des Roches 1978, 10, 1–54. [Google Scholar] [CrossRef]
- Du, S.; Hu, Y.; Hu, X. Measurement of joint roughness coefficient by using profilograph and roughness ruler. J. Earth Sci. 2009, 20, 890–896. [Google Scholar] [CrossRef]
- Du, S.; Hu, Y.; Hu, X.; Guo, X. Comparison between empirical estimation by JRC-JCS model and direct shear test for joint shear strength. J. Earth Sci. 2011, 22, 411–420. [Google Scholar] [CrossRef]
- Guo, C.; Yang, X.; Zhao, W. Experimental Study on Compressibility and Microstructure of Loess Solidified by Permeable Polymer. KSCE J. Civ. Eng. 2024, 28, 1739–1752. [Google Scholar] [CrossRef]
- Zhao, L.; Wang, C.; Guo, C.; Ma, X.; Li, Z.; Du, X.; Jiang, W. Properties of Low-Exothermic polymer grouting materials and its application on highway. Constr. Build. Mater. 2023, 408, 133771. [Google Scholar] [CrossRef]
- Yang, S.; Yang, T.; Sun, Z.; Ren, Z. A predictive model for determining shear strength and shear fracture energy of FRP bars in alkali-activated slag seawater coral aggregate concrete. J. Build. Eng. 2022, 59, 105085. [Google Scholar] [CrossRef]
- Dong, M.; Hao, Y.; Zhang, C.; Li, L.; Sun, W.; Jin, C. Shear fracture energy of asphalt-aggregate systems and its application to predicting shear fatigue in asphalt mixtures. Int. J. Pavement Eng. 2020, 21, 246–256. [Google Scholar] [CrossRef]
- Choo, J.; Sohail, A.; Fei, F.; Wong, T.F. Shear fracture energies of stiff clays and shales. Acta Geotech. 2021, 16, 1–9. [Google Scholar] [CrossRef]
- An, F.C.; Zhang, F.Y.; Hou, C.C. Influence of mechanical properties of concrete on the failure behaviour of FRP-to-concrete interface. Constr. Build. Mater. 2020, 264, 120572. [Google Scholar] [CrossRef]
- Soda PR, K.; Chakravarthi, K.; Mini, K.M. Experimental and statistical investigation on strength and microcracks remediation in cement mortar using expanded vermiculite as a bacterial carrier. J. Build. Eng. 2023, 63, 105567. [Google Scholar] [CrossRef]
- Soda, P.R.K.; Mini, K.M. Performance Enhancement and Remediation of Microcracks in Cement Mortar by Doping Calcite-Precipitating Microorganisms. J. Mater. Civ. Eng. 2022, 34, 04022035. [Google Scholar] [CrossRef]
- Thiam, M.; Fall, M. Engineering properties of a building material with melted plastic waste as the only binder. J. Build. Eng. 2021, 44, 102684. [Google Scholar] [CrossRef]
- Al-Tahan, M.A.; Shatat, M.R.; Abdallah, M.H.; Taher, M.A. Influence of the thermally and hot water treatment of cement kiln dust on the Physico-chemical features of Portland cement. J. Build. Eng. 2021, 38, 102170. [Google Scholar] [CrossRef]
- Wang, Y.; Surahman, R.; Nagai, K. Shear cracking behavior of pre-damaged PVA-aggregate mixed ECC beams: Direct observation using DIC. Eng. Fract. Mech. 2024, 295, 109757. [Google Scholar] [CrossRef]
- Ribeiro, B.; Asaue, H.; Hattori, A.; Shiotani, T.; Kobayashi, T.; Sato, J.; Uchida, M.; Ishida, M. Load carrying behavior of concrete reinforced with bundled BFRP bars by using DIC. Case Stud. Constr. Mater. 2022, 17, e01538. [Google Scholar] [CrossRef]
- Li, X.; Zhang, X.; Wang, Z.; Zheng, D.; Li, P. Experimental study with DIC technique on the bond failure of structural concrete under repeated reversed loading with different amplitude. Adv. Struct. Eng. 2023, 26, 3089–3111. [Google Scholar] [CrossRef]
- Dehghani, A.; Aslani, F. Advanced shape memory alloy fibers designed to enhance crack closure and re-centring performance in cement-based composites. Constr. Build. Mater. 2024, 415, 135095. [Google Scholar] [CrossRef]



















| Property | Value | The Standards Used |
|---|---|---|
| Specific gravity GS | 2.70 | Geotechnical engineering test method and criterion (GB/T50123-2019) [5] |
| Percent moisture content of liquid limit wL/% | 24.00 | |
| Percent moisture content of plastic limit wP/% | 16.90 | |
| Plasticity index IP | 7.10 | |
| Natural moisture content/% | 11.80–19.37 | |
| Maximum dry density ρdmax/g·cm−3 | 1.44 |
| Viscosity 23 ± 2 °C (mPa·s) | Density (g/cm3) | Compressive Strength (MPa) | Components | Viscosity 23 ± 2 °C (mPa·s) | Density (g/cm3) |
|---|---|---|---|---|---|
| 10 | 1.1 | 2.5 | A | 4 | 1.05 |
| B | 23 | 1.20 |
| Roughness of Surface | Surface Grooving Method | JCR |
|---|---|---|
| Smoothness | Not dealt with | 0 |
| Roughness 1 | Depth 3 mm, width 2 mm, length 100 mm, slot spacing 20 mm | 6.3 |
| Roughness 2 | Depth 6 mm, width 2 mm, length 100 mm, slot spacing 10 mm | 12.2 |
| Roughness 3 | Depth 6 mm, width 2 mm, length 100 mm, slot spacing 50 mm | 19.5 |
| Test Number | Normal Stress 200 kPa | Normal Stress 400 kPa | Normal Stress 600 kPa | |||
|---|---|---|---|---|---|---|
| Sm (mm) | Sr (mm) | Sm (mm) | Sr (mm) | Sm (mm) | Sr (mm) | |
| 1 | 4.43 | 6.13 | 4.26 | 6.58 | 4.92 | 6.32 |
| 2 | 4.88 | 7.56 | 5.01 | 7.79 | 4.71 | 7.78 |
| 3 | 6.65 | 9.49 | 7.07 | 8.46 | 6.48 | 8.51 |
| 4 | 8.37 | 10.55 | 8.72 | 11.64 | 7.88 | 10.45 |
| 5 | 8.32 | 10.52 | 8.74 | 10.47 | 8.10 | 10.02 |
| 6 | 8.18 | 10.87 | 8.07 | 11.12 | 8.08 | 10.46 |
| 7 | 5.34 | 6.69 | 5.59 | 6.30 | 5.40 | 6.41 |
| 8 | 4.89 | 8.92 | 4.88 | 8.98 | 5.18 | 8.98 |
| 9 | 8.46 | 10.73 | 8.63 | 11.08 | 8.06 | 10.96 |
| 10 | 5.77 | 8.59 | 5.55 | 8.90 | 5.79 | 8.27 |
| 11 | 6.84 | 8.47 | 6.86 | 8.35 | 6.64 | 8.11 |
| 12 | 4.93 | 7.14 | 4.63 | 6.92 | 5.06 | 7.12 |
| 13 | 6.56 | 8.78 | 6.79 | 8.54 | 6.77 | 8.29 |
| 14 | 5.77 | 8.93 | 5.61 | 8.84 | 5.90 | 9.06 |
| 15 | 5.51 | 6.89 | 5.75 | 7.07 | 5.44 | 6.76 |
| 16 | 6.72 | 8.34 | 6.49 | 8.42 | 6.30 | 8.16 |
| Test Number | Interface Shear Fracture Energy (J/m2) | ||
|---|---|---|---|
| Normal Stress 200 kPa | Normal Stress 400 kPa | Normal Stress 600 kPa | |
| 1 | 896.39 | 1382.23 | 1850.05 |
| 2 | 1604.05 | 2281.08 | 3083.28 |
| 3 | 1701.91 | 2035.24 | 3034.06 |
| 4 | 989.97 | 1713.79 | 2189.13 |
| 5 | 2320.66 | 2883.01 | 4062.51 |
| 6 | 1226.8 | 1984.31 | 2644.26 |
| 7 | 1002.63 | 1323.93 | 2017.27 |
| 8 | 1217.01 | 1848.98 | 2418.85 |
| 9 | 1134.94 | 1913.85 | 2622.73 |
| 10 | 1373.34 | 2068.5 | 2730.92 |
| 11 | 1989.94 | 2506.85 | 3592.27 |
| 12 | 893.31 | 1361.68 | 1970.3 |
| 13 | 2210.15 | 2962.23 | 3916.35 |
| 14 | 1877.87 | 2672.69 | 3806.92 |
| 15 | 521.23 | 876.8 | 1176.72 |
| 16 | 695.52 | 1320.09 | 1624.57 |
| Test Number | Percentage of Area of Soil Adhering to the Concrete Surface After Shear Damage (%) | ||
|---|---|---|---|
| Normal Stress 200 kPa | Normal Stress 400 kPa | Normal Stress 600 kPa | |
| 1 | 60.51 | 71.35 | 72.61 |
| 2 | 62.9 | 68.23 | 78.28 |
| 3 | 79.74 | 85.05 | 94.36 |
| 4 | 100 | 100 | 100 |
| 5 | 85.08 | 91.88 | 96.67 |
| 6 | 91.91 | 93.57 | 98.56 |
| 7 | 70.36 | 77.17 | 78.04 |
| 8 | 42.73 | 51.72 | 56.72 |
| 9 | 95.49 | 96.39 | 97.41 |
| 10 | 70.13 | 80.44 | 85.92 |
| 11 | 67.43 | 72.19 | 78.88 |
| 12 | 57.81 | 65.13 | 67.13 |
| 13 | 71.79 | 77.28 | 89.54 |
| 14 | 77.41 | 85.72 | 90.9 |
| 15 | 83.56 | 88.82 | 90.8 |
| 16 | 71.53 | 75.48 | 79.48 |
| Distance from Loaded End (mm) | Normal Stress 200 kPa | Normal Stress 400 kPa | Normal Stress 600 kPa | |||
|---|---|---|---|---|---|---|
| Peak Shear Strength Phase | Residual Shear Strength Stage | Peak Shear Strength Phase | Residual Shear Strength Stage | Peak Shear Strength Phase | Residual Shear Strength Stage | |
| 100 | 3.23 | 5.98 | 2.73 | 5.06 | 1.24 | 3.34 |
| 0 | 4.44 | 7.6 | 5.02 | 7.68 | 4.8 | 7.8 |
| Specific value | 72.75% | 78.68% | 54.38% | 65.89% | 25.83% | 42.82% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Yang, X.; Diao, Y.; Guo, C. Study on Bonding Characteristics of Polymer Grouted Concrete-Soil Interface. Polymers 2024, 16, 2207. https://doi.org/10.3390/polym16152207
Wang L, Yang X, Diao Y, Guo C. Study on Bonding Characteristics of Polymer Grouted Concrete-Soil Interface. Polymers. 2024; 16(15):2207. https://doi.org/10.3390/polym16152207
Chicago/Turabian StyleWang, Lina, Xiaodong Yang, Yueliang Diao, and Chengchao Guo. 2024. "Study on Bonding Characteristics of Polymer Grouted Concrete-Soil Interface" Polymers 16, no. 15: 2207. https://doi.org/10.3390/polym16152207
APA StyleWang, L., Yang, X., Diao, Y., & Guo, C. (2024). Study on Bonding Characteristics of Polymer Grouted Concrete-Soil Interface. Polymers, 16(15), 2207. https://doi.org/10.3390/polym16152207






