Experimental Study on Fracture Behavior of Adhesive-Bonded Structure with V-notch Based on Digital Gradient Sensing Method
Abstract
1. Introduction
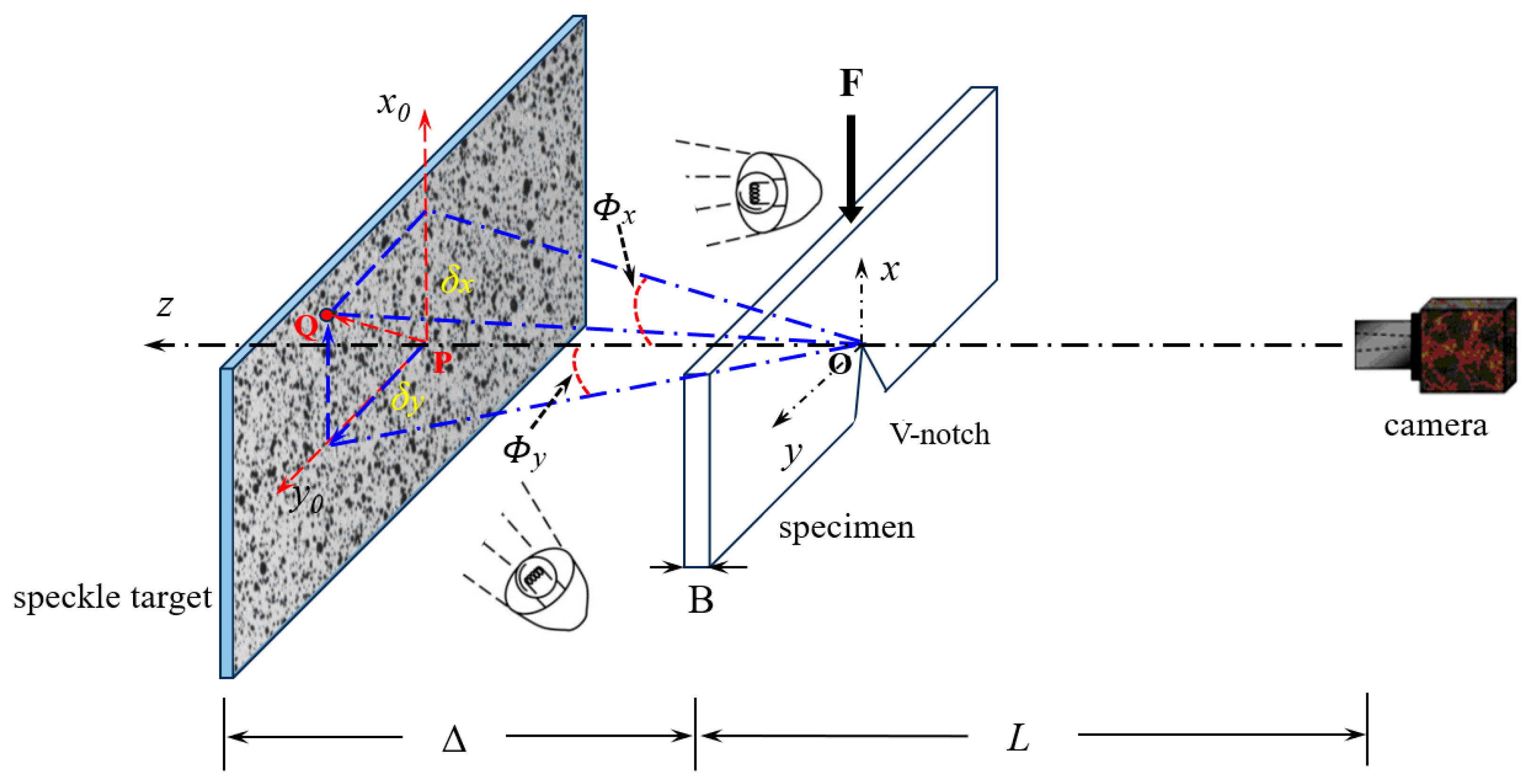
2. Basic Principles and Methods
2.1. Basic Principles of DGS Method
2.2. Elastic Stress Field around the V-notch Tip
2.3. Solving Equation of
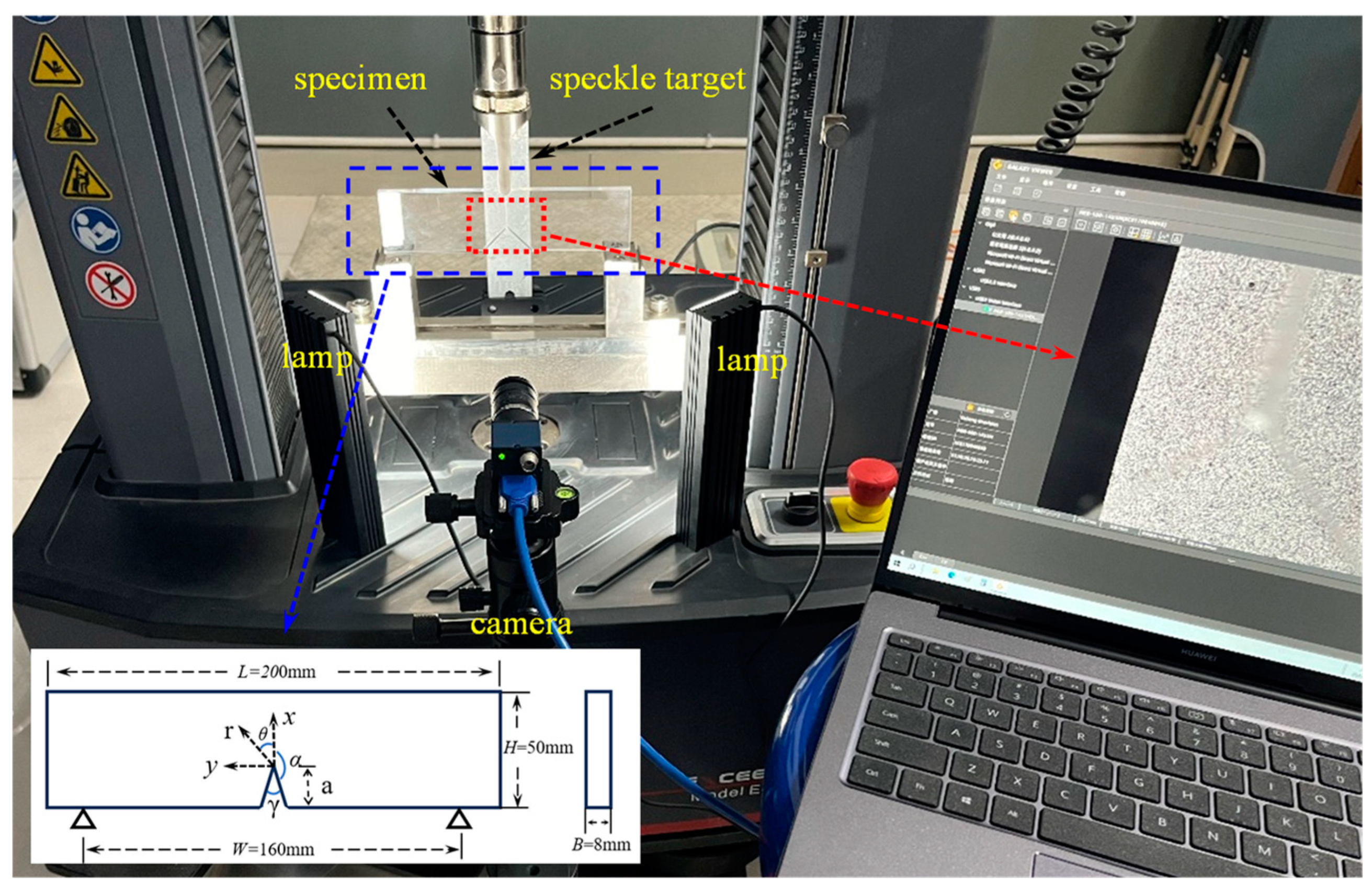
3. Experiment Process
3.1. Specimen Preparation
3.2. Experimental Setup
4. Results and Discussion
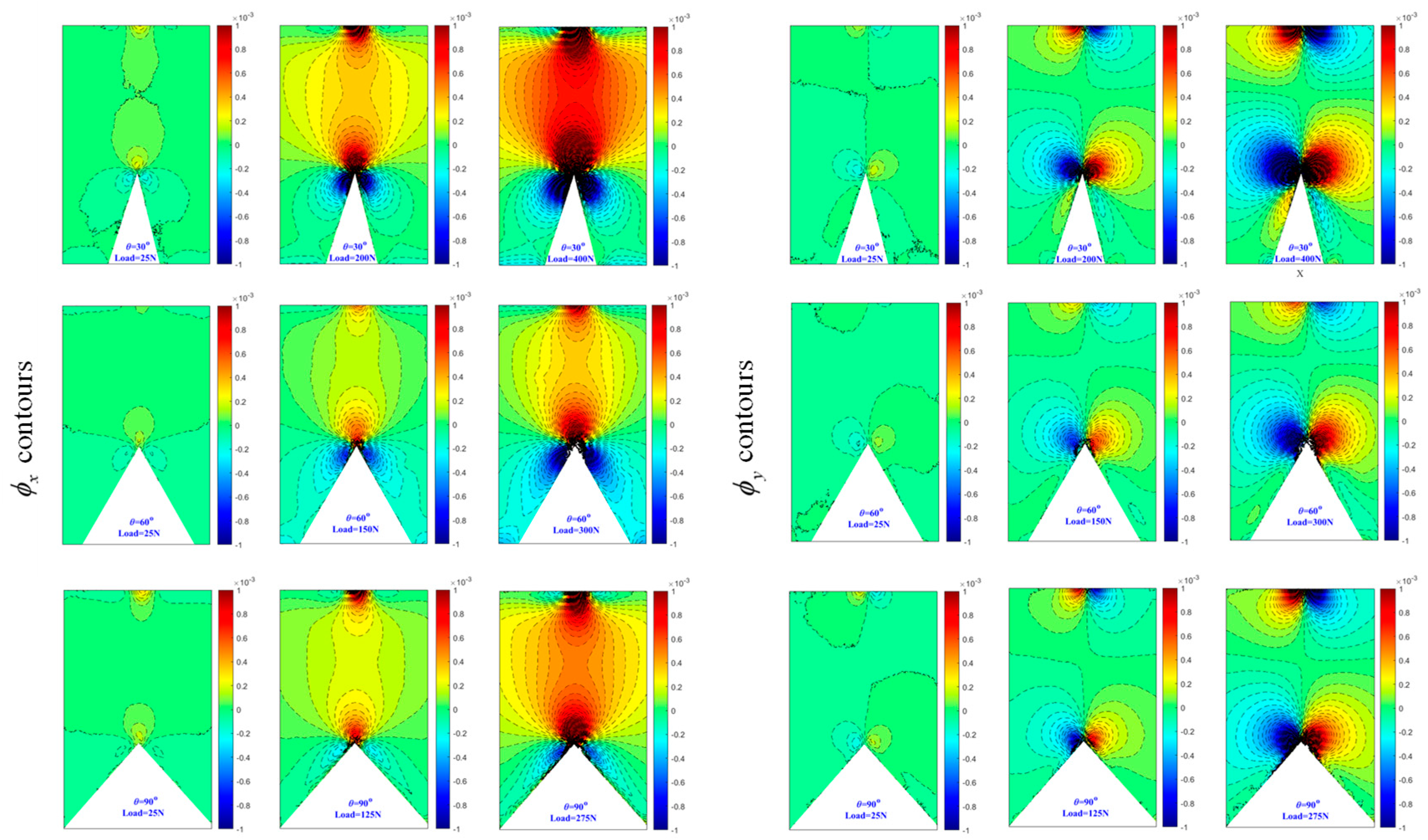
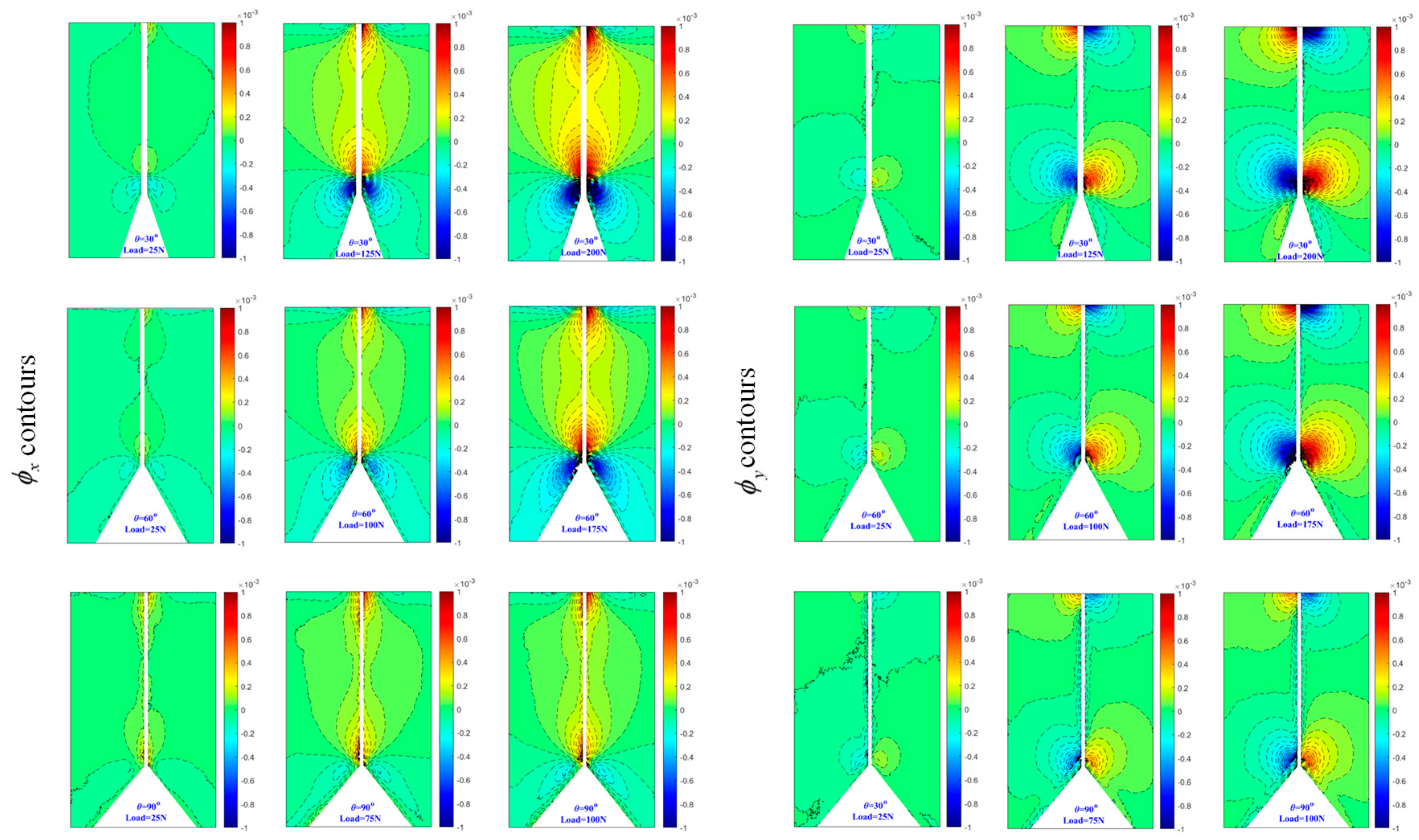
4.1. Analysis of Angular Deflection Fields
4.2. Extraction of SIFs
4.3. Fracture Toughness
5. Conclusions
- By carrying out DGS experiments on PMMA bonded and one-piece specimens with different V-notch defects, the stress field near the V-notch of the two types of specimens under the plane stress state was characterized, and the fracture behaviors of the two types of specimens were compared. The effectiveness of the DGS method in the study of the stress concentration of two types of specimens under plane stress is proved.
- In this study, the adhesive used resulted in a lower fracture load for bonded specimens compared to one-piece specimens in three-point bending tests. The fracture loads of bonded specimens were 49%, 61%, and 48% of the fracture load of one-piece specimens at three different V-notch angles. Additionally, the fracture loads of both types of specimens decreased as the V-notch angle increased.
- The fracture toughness of the two types of specimens in the three-point bending test showed significant differences. The fracture toughness of the bonded specimen was 52%, 67%, and 46% of that of the one-piece specimen at the three V-notch angles, respectively. The fracture toughness of both types of specimens was found to be dependent on the size of the V-notch angle, with a more pronounced effect observed in the bonded specimen. This variation highlights the distinct fracture behaviors exhibited by the two types of specimens.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DIC | Digital image correlation |
| DGS | Digital gradient sensing |
| SIFs | Stress intensity factors |
References
- Chen, Y.; Li, M.; Yang, X.; Luo, W. Damage and failure characteristics of CFRP/aluminum single lap joints designed for lightweight applications. Thin-Walled Struct. 2020, 153, 106802. [Google Scholar] [CrossRef]
- Xie, Y.; Yang, B.; Lu, L.; Wan, Z.; Liu, X. Shear strength of bonded joints of carbon fiber reinforced plastic (CFRP) laminates enhanced by a two-step laser surface treatment. Compos. Struct. 2020, 232, 111559. [Google Scholar] [CrossRef]
- Liang, J.; Jiang, H.; Zhang, J.; Wu, X.; Zhang, X.; Li, G.; Cui, J. Investigations on mechanical properties and microtopography of electromagnetic self-piercing riveted joints with carbon fiber reinforced plastics/aluminum alloy 5052. Arch. Civ. Mech. Eng. 2019, 19, 240–250. [Google Scholar] [CrossRef]
- Li, M.; Li, S.; Yang, X. The influence of machining processes on strain distribution and progressive failure characteristics when producing holes in CFRP. Compos. Struct. 2020, 238, 111994. [Google Scholar] [CrossRef]
- Moutrille, M.P.; Derrien, K.; Baptiste, D.; Balandraud, X.; Grédiac, M. Through-thickness strain field measurement in a composite/aluminum adhesive joint. Compos. Part B-Eng. 2009, 40, 985–996. [Google Scholar] [CrossRef]
- Camanho, P.P.; Matthews, F.L. Stress analysis and strength prediction of mechanically fastened joints in FRP: A review. Compos. Compos. Part A-Eng. 1997, 28, 529–547. [Google Scholar] [CrossRef]
- Kweon, J.; Jung, J.; Kim, T.; Choi, J.; Kim, D. Failure of carbon composite-to-aluminum joints with combined mechanical fastening and adhesive bonding. Compos. Struct. 2006, 75, 192–198. [Google Scholar] [CrossRef]
- Dano, M.; Gendron, G.; Picard, A. Stress and failure analysis of mechanically fastened joints in composite laminates. Compos. Struct. 2000, 50, 287–296. [Google Scholar] [CrossRef]
- Ribas, M.J.P.; Akhavan-Safar, A.; Pigray, N. From high strain rates to elevated temperatures: Investigating mixed-mode fracture behaviour in a polyurethane adhesive. Polymers 2023, 15, 2675. [Google Scholar] [CrossRef]
- He, X. A review of finite element analysis of adhesively bonded joints. Int. J. Adhes. Adhes. 2011, 31, 248–264. [Google Scholar] [CrossRef]
- Shang, X.; Marques, E.A.; Machado, J.J.; Carbas, R.J.; Jiang, D.; Da Silva, L.F. Review on techniques to improve the strength of adhesive joints with composite adherends. Compos. Part B-Eng. 2019, 177, 107363. [Google Scholar] [CrossRef]
- Guo, L.; Liu, J.; Xia, H.; Li, X.; Zhang, X.; Yang, H.; Yang, Y. Effects of loading rate, temperature, and thickness on the tensile strength of precision adhesive joints. Polym. Test. 2022, 109, 107528. [Google Scholar] [CrossRef]
- Liu, J.; Sawa, T. Stress analysis and strength evaluation of single-lap band adhesive joints of dissimilar adherends subjected to external bending moments. J. Adhes. Sci. Technol. 2000, 14, 67–92. [Google Scholar] [CrossRef]
- Stein, N.; Weißgraeber, P.; Becker, W. Stress solution for functionally graded adhesive joints. Int. J. Solids Struct. 2016, 97, 300–311. [Google Scholar] [CrossRef]
- Stuparu, F.A.; Apostol, D.A.; Constantinescu, D.M.; Picu, C.R.; Sandu, M.; Sorohan, S. Local evaluation of adhesive failure in similar and dissimilar single-lap joints. Eng. Fract. Mech. 2017, 183, 39–52. [Google Scholar] [CrossRef]
- Wang, W.; Hosseini, A.; Ghafoori, E. Experimental study on Fe-SMA-to-steel adhesively bonded interfaces using DIC. Eng. Fract. Mech. 2021, 244, 107553. [Google Scholar] [CrossRef]
- Heidarpour, F.; Farahani, M.; Ghabezi, P. Experimental investigation of the effects of adhesive defects on the single lap joint strength. Int. J. Adhes. Adhes. 2018, 80, 128–132. [Google Scholar] [CrossRef]
- He, Z.; Luo, Q.; Li, Q.; Zheng, G.; Sun, G. Fatigue behavior of CFRP/Al adhesive joints-Failure mechanisms study using digital image correlation (DIC) technique. Thin-Walled Struct. 2022, 174, 109075. [Google Scholar] [CrossRef]
- Sutton, M.A.; Hild, F. Recent advances and perspectives in digital image correlation. Exp. Mech. 2015, 55, 1–8. [Google Scholar] [CrossRef]
- Oats, R.C.; Dai, Q.; Head, M. Digital image correlation advances in structural evaluation applications: A review. Pract. Period. Struct. Des. Constr. 2022, 27, 03122007. [Google Scholar] [CrossRef]
- Periasamy, C.; Tippur, H.V. Measurement of crack-tip and punch-tip transient deformations and stress intensity factors using Digital Gradient Sensing technique. Eng. Fract. Mech. 2013, 98, 185–199. [Google Scholar] [CrossRef]
- Periasamy, C.; Tippur, H.V. Full-field digital gradient sensing method for evaluating stress gradients in transparent solids. Appl. Opt. 2012, 51, 2088–2097. [Google Scholar] [CrossRef] [PubMed]
- Dai, X.; Liu, Y.; Li, W.; Qi, J.; Xu, M.; Zhou, J.; Yang, F. Intrinsic stress determination based on the combination of photoelasticity and digital gradient sensing. Opt. Lasers Eng. 2022, 159, 107201. [Google Scholar] [CrossRef]
- Yuan, Y.; Hao, W.; Ma, Y. Stress field at V-notch tip in polymer materials using digital gradient sensing. Mech. Adv. Mater. Struct. 2017, 24, 350–356. [Google Scholar] [CrossRef]
- Liu, W.; Li, L.; Qiao, Y. Local stress analysis of blunt V-notches using the digital gradient sensing method. Appl. Opt. 2021, 60, 1489–1499. [Google Scholar] [CrossRef] [PubMed]
- Williams, M.L. Stress singularities resulting from various boundary conditions in angular corners of plates in extension. J. Appl. Mech. 1952, 19, 526–528. [Google Scholar] [CrossRef]
- Ju, S.H.; Chung, H.Y. Accuracy and limit of a least-squares method to calculate 3D notch SIFs. Int. J. Fract. 2017, 148, 169–183. [Google Scholar] [CrossRef]





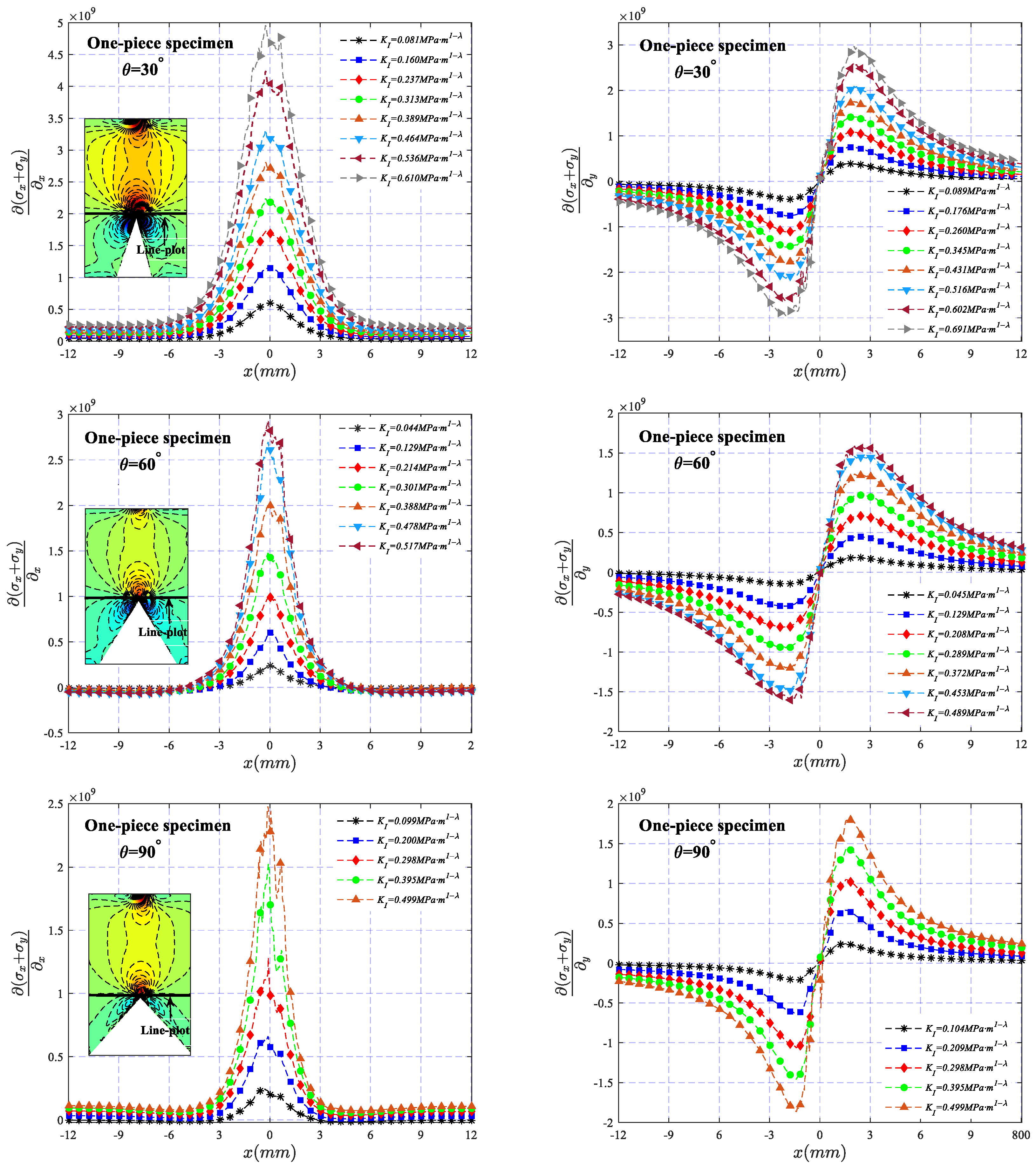
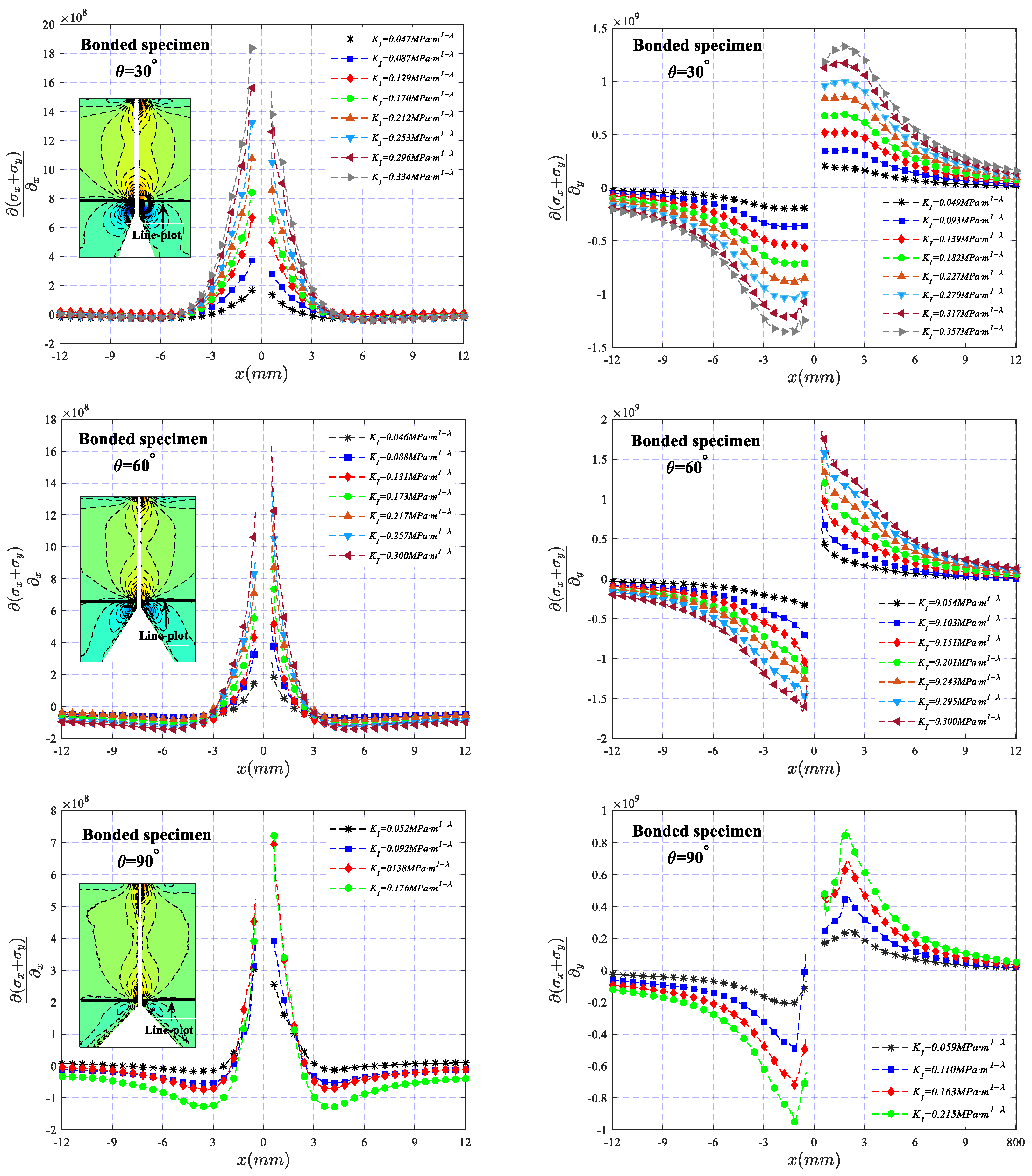
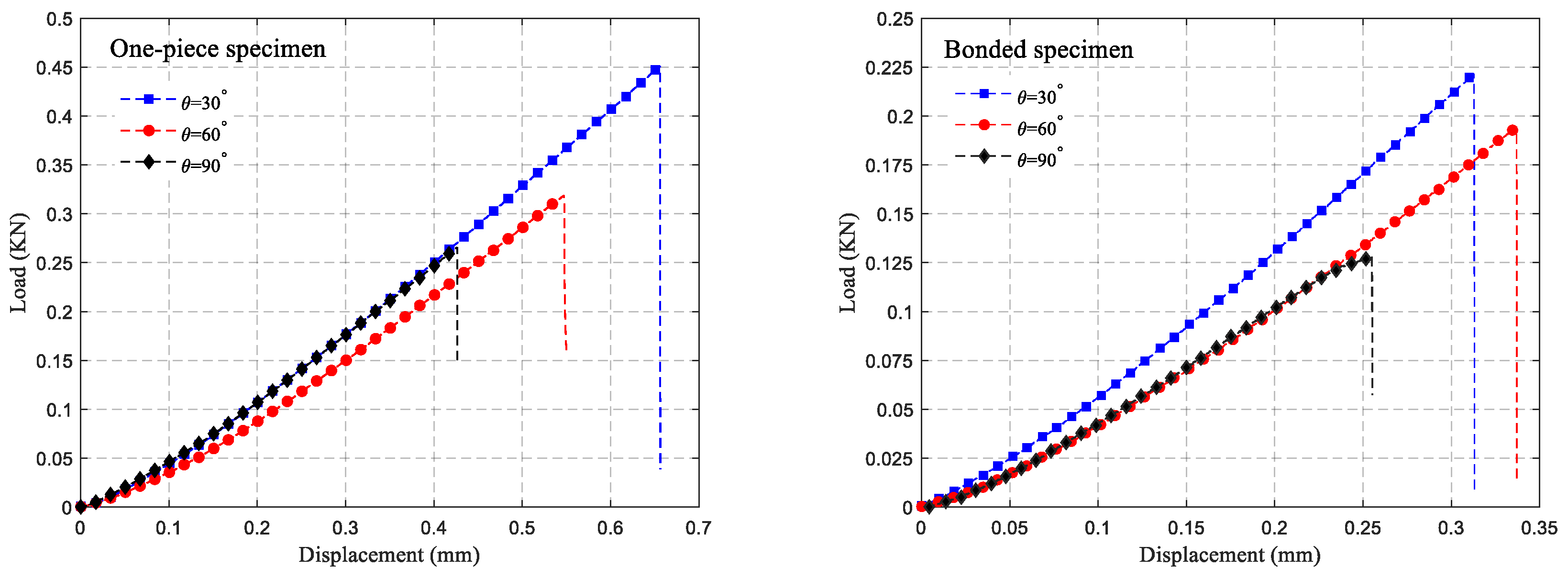
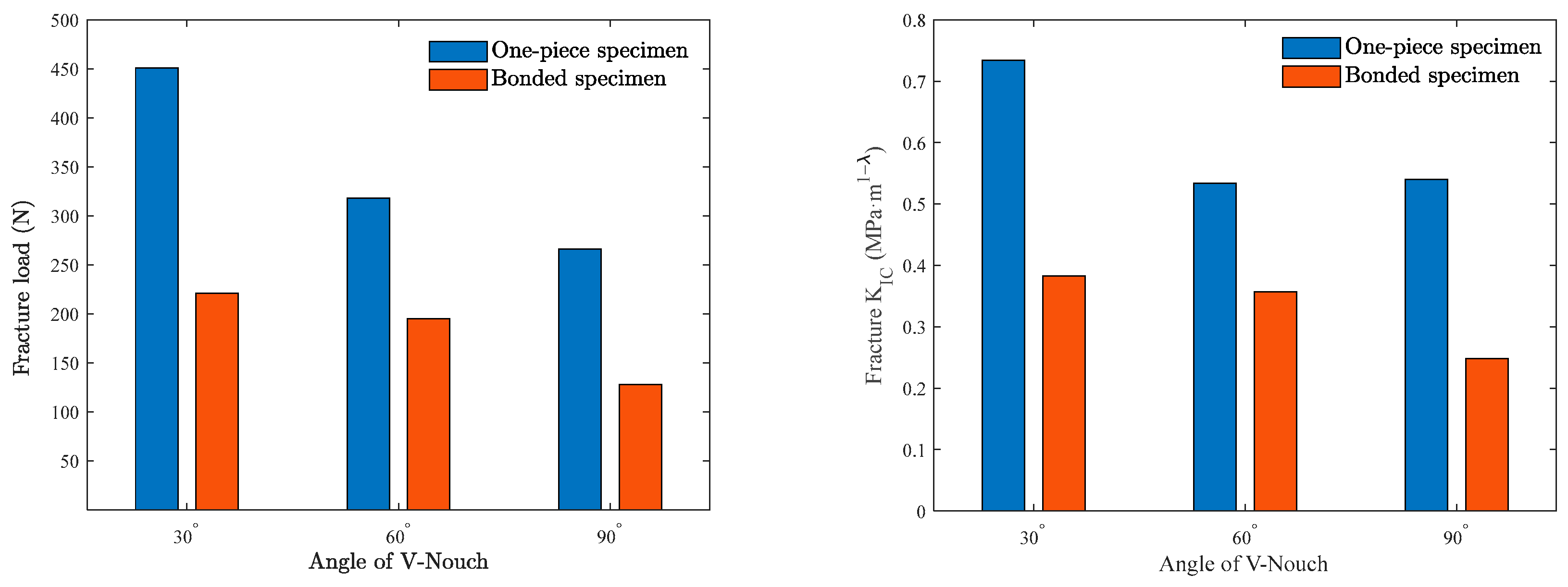
| γ | 30° | 60° | 90° |
| 165° | 150° | 135° | |
| 0.5014 | 0.5123 | 0.5445 |
| Material | PMMA |
|---|---|
| Elastic modulus E(GPa) | 3.33 |
| Poisson’s ratio υ | 0.35 |
| Stress optical constants |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, H.; An, Y.; Liu, Y. Experimental Study on Fracture Behavior of Adhesive-Bonded Structure with V-notch Based on Digital Gradient Sensing Method. Polymers 2024, 16, 2011. https://doi.org/10.3390/polym16142011
Yu H, An Y, Liu Y. Experimental Study on Fracture Behavior of Adhesive-Bonded Structure with V-notch Based on Digital Gradient Sensing Method. Polymers. 2024; 16(14):2011. https://doi.org/10.3390/polym16142011
Chicago/Turabian StyleYu, Hai, Yangzhuang An, and Yunpeng Liu. 2024. "Experimental Study on Fracture Behavior of Adhesive-Bonded Structure with V-notch Based on Digital Gradient Sensing Method" Polymers 16, no. 14: 2011. https://doi.org/10.3390/polym16142011
APA StyleYu, H., An, Y., & Liu, Y. (2024). Experimental Study on Fracture Behavior of Adhesive-Bonded Structure with V-notch Based on Digital Gradient Sensing Method. Polymers, 16(14), 2011. https://doi.org/10.3390/polym16142011






