Double Percolation of Poly(lactic acid)/Low-Density Polyethylene/Carbon Nanotube (PLA/LDPE/CNT) Composites for Force-Sensor Application: Impact of Preferential Localization and Mixing Sequence
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Sample Preparations
2.3. Characterizations
3. Results and Discussion
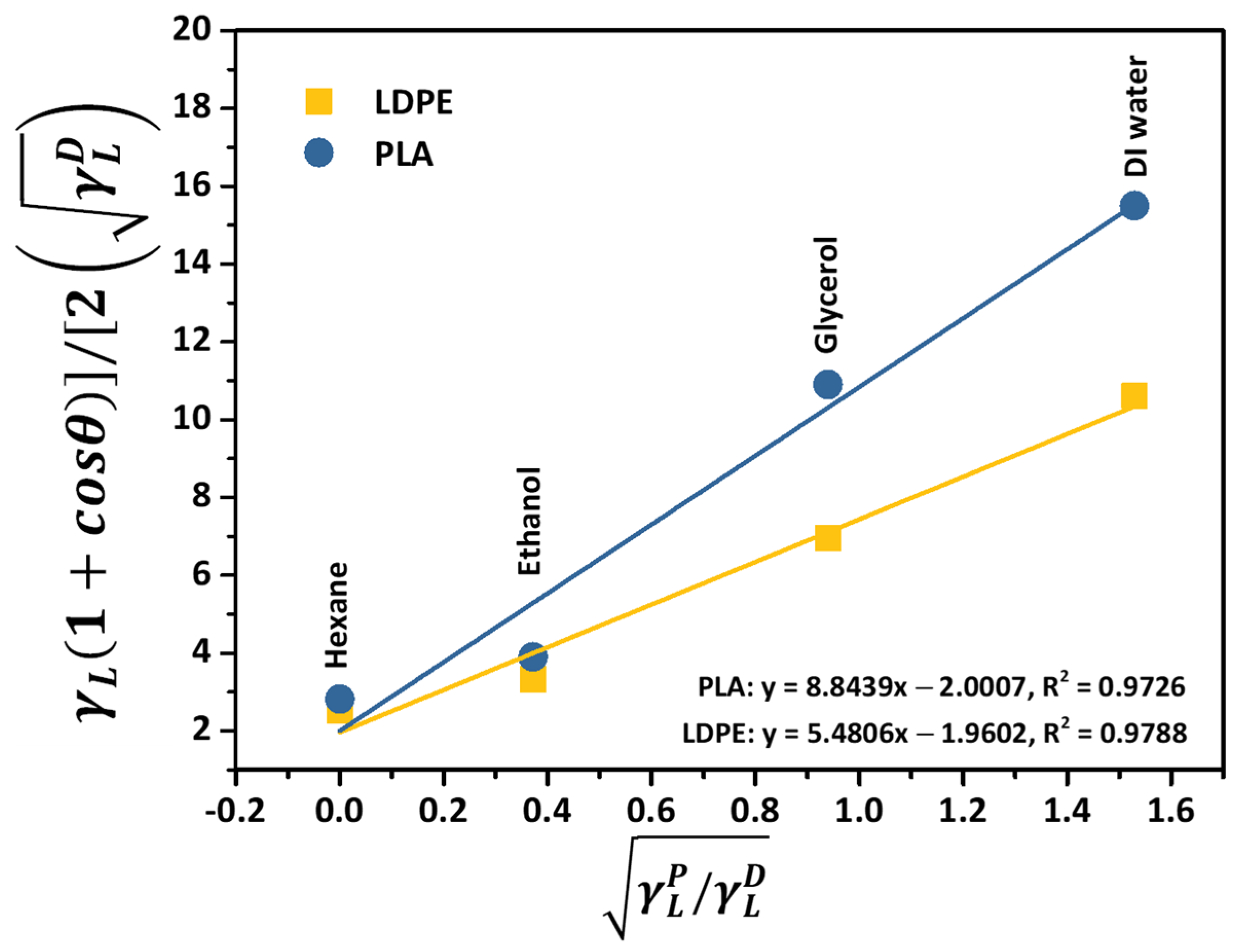
3.1. Thermodynamic Prediction on the Preferential Localization of CNTs
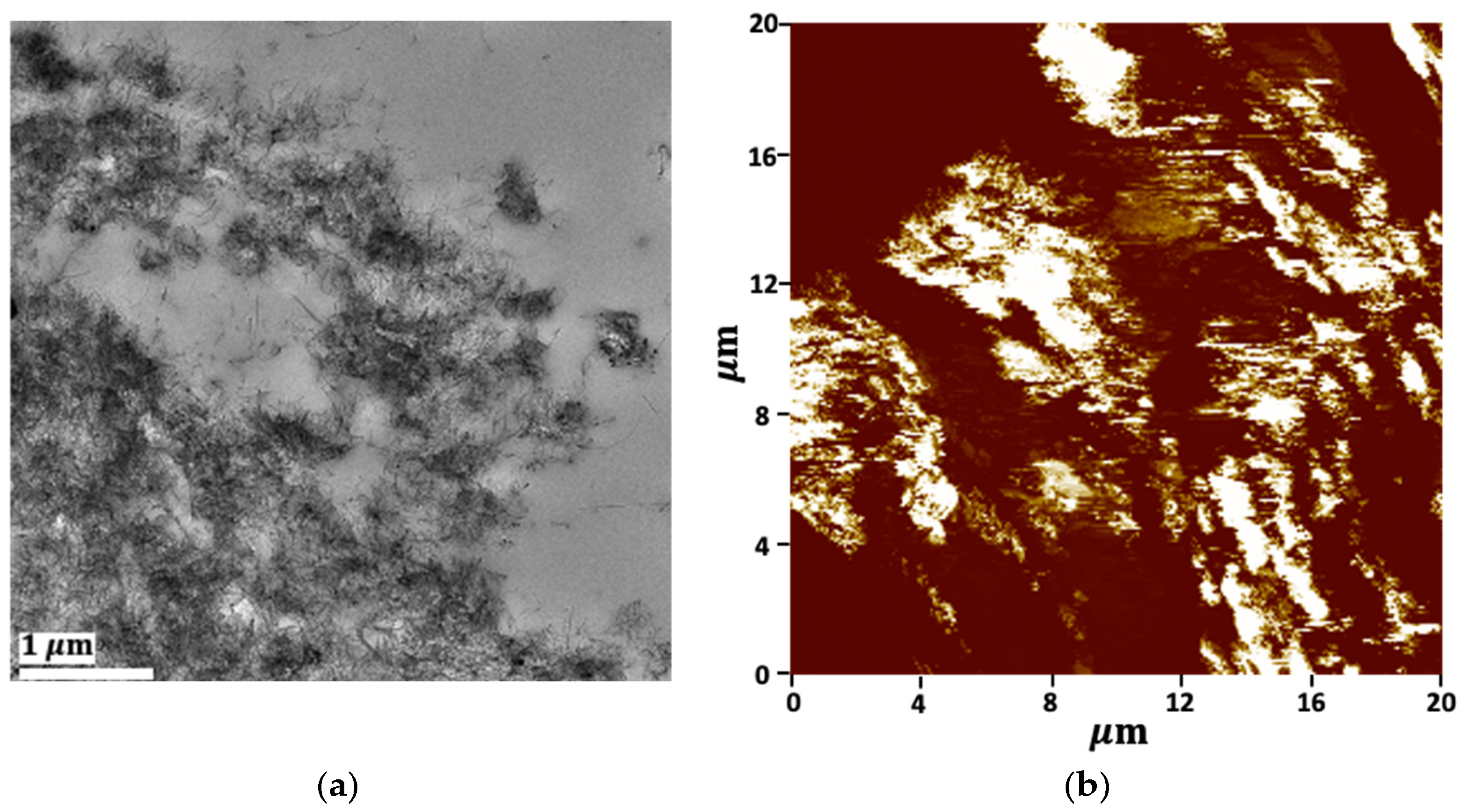
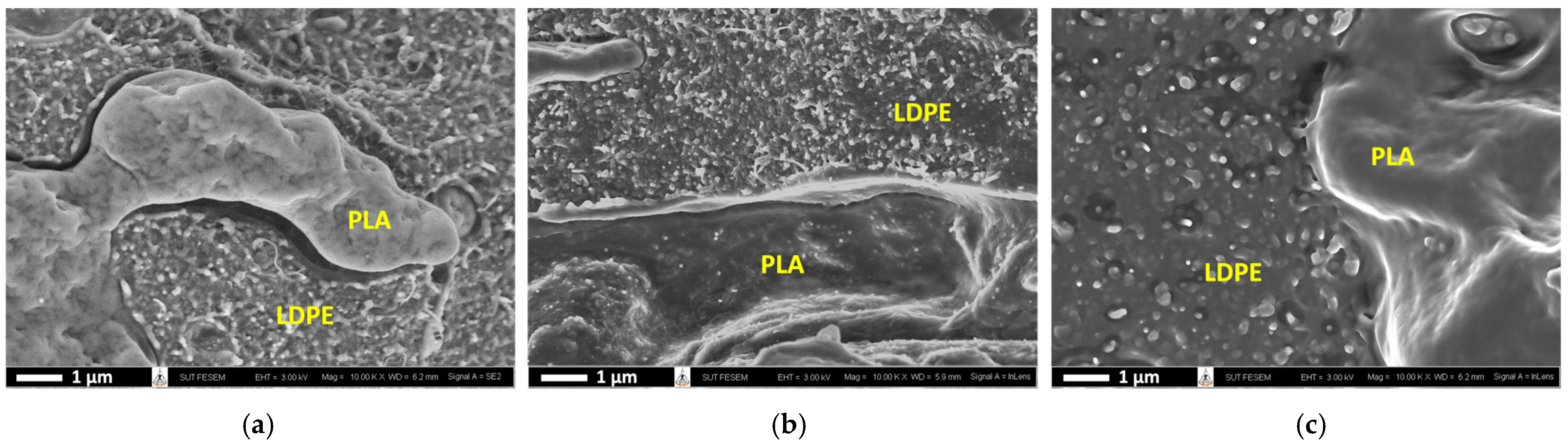
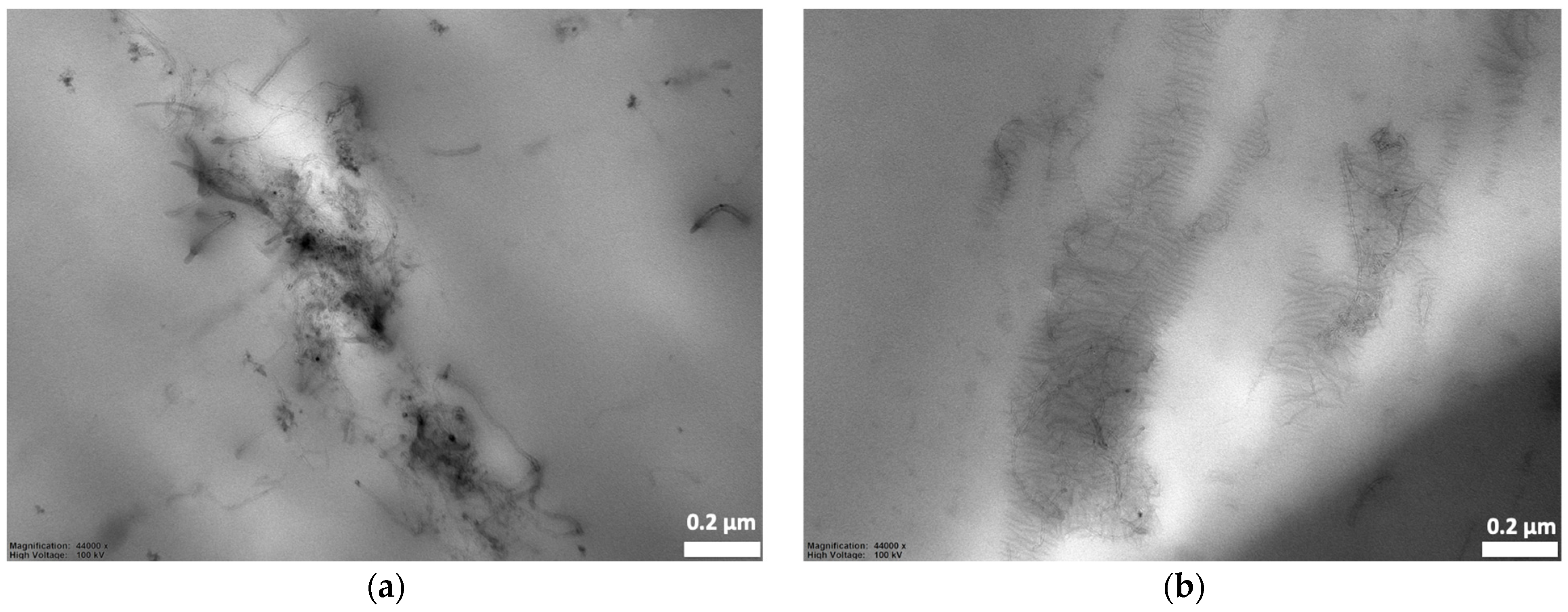
3.2. Preferential Localization of CNTs in PLA/LDPE/CNT Composites
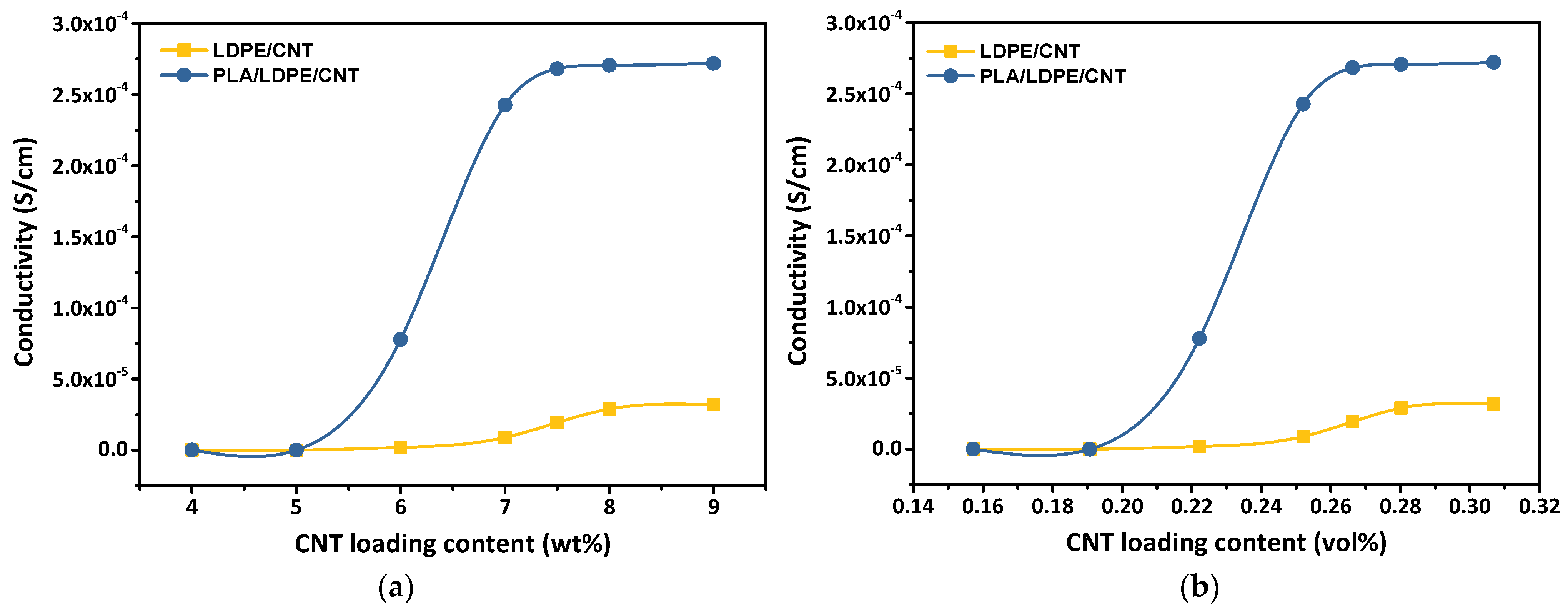
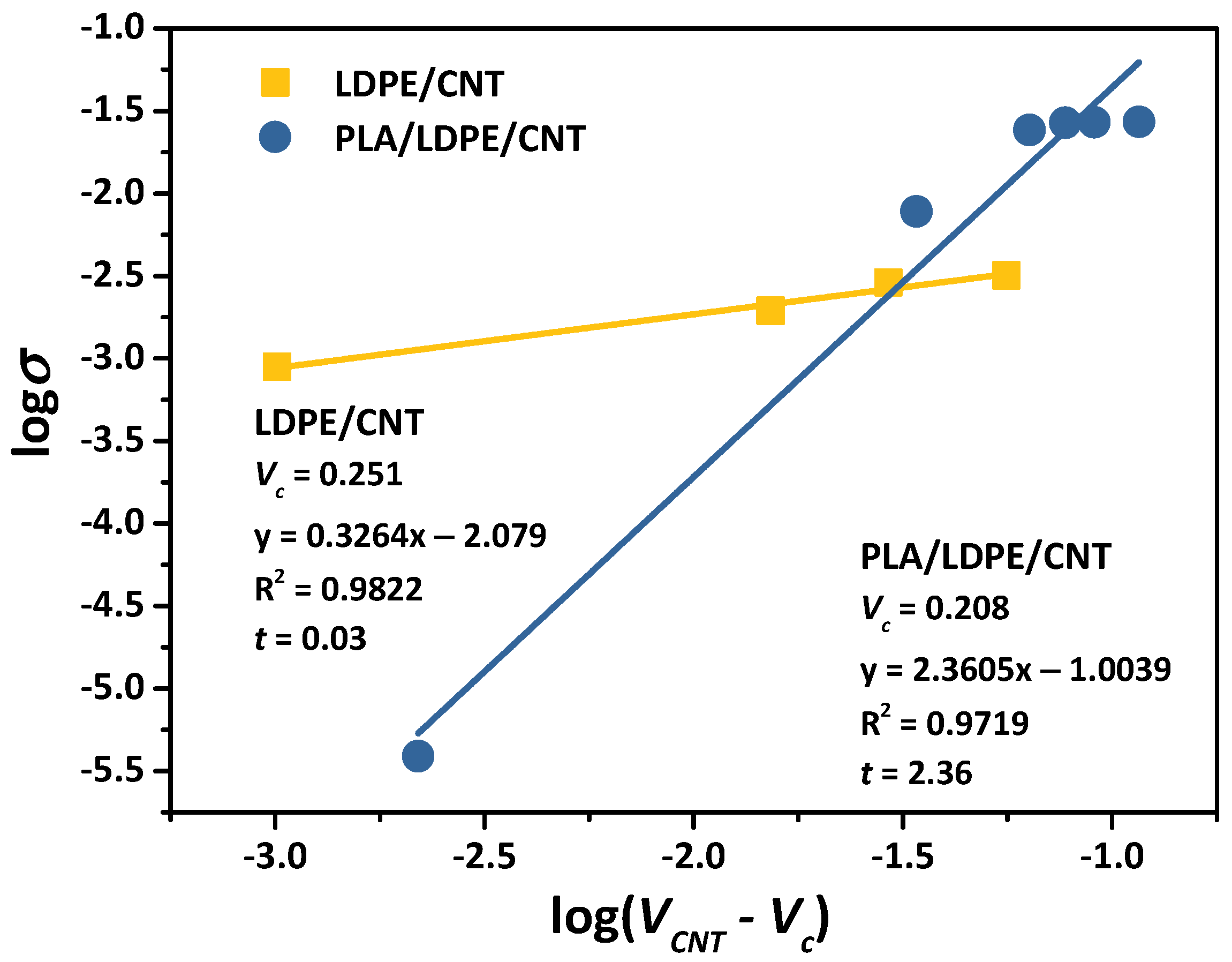
3.3. Electrical Conductivity and Percolation Threshold
3.4. Mixing Sequences
3.5. Discussion on the Competition between Thermodynamic and Kinetic Factors on the Final Localization of CNTs
3.6. Discussion on the Competition between Thermodynamic and Rheological Effects on the Final Localization of CNTs
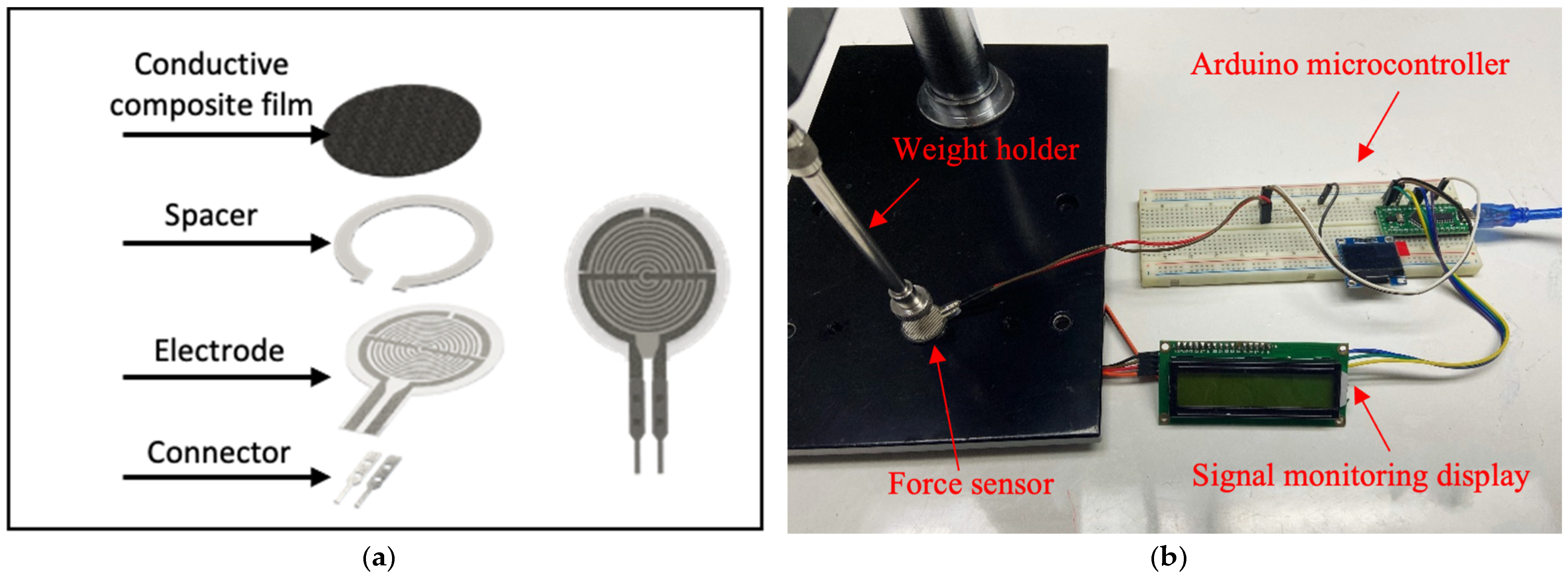
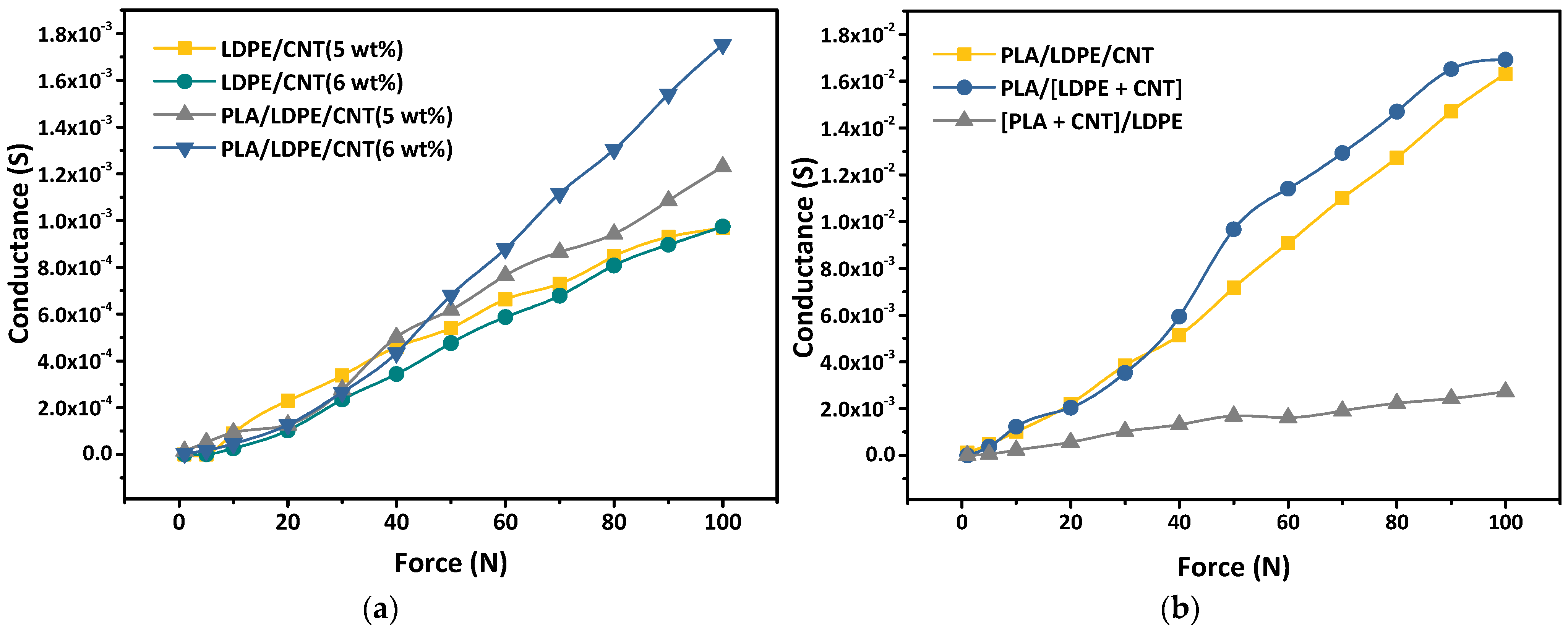
3.7. Force Sensor Application
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sharma, S.; Verma, A.; Rangappa, S.M.; Siengchin, S.; Ogata, S. Recent progressive developments in conductive-fillers based polymer nanocomposites (CFPNC’s) and conducting polymeric nanocomposites (CPNC’s) for multifaceted sensing applications. J. Mater. Res. Technol. 2023, 26, 5921–5974. [Google Scholar] [CrossRef]
- Loos, M. Chapter 5—Fundamentals of Polymer Matrix Composites Containing CNTs. In Carbon Nanotube Reinforced Composites; Loos, M., Ed.; William Andrew Publishing: Oxford, UK, 2015; pp. 125–170. [Google Scholar]
- Zeng, X.; Xu, X.; Shenai, P.M.; Kovalev, E.; Baudot, C.; Mathews, N.; Zhao, Y. Characteristics of the Electrical Percolation in Carbon Nanotubes/Polymer Nanocomposites. J. Phys. Chem. C 2011, 115, 21685–21690. [Google Scholar] [CrossRef]
- Zare, Y.; Rhee, K.Y. Study on the Effects of the Interphase Region on the Network Properties in Polymer Carbon Nanotube Nanocomposites. Polymers 2020, 12, 182. [Google Scholar] [CrossRef]
- Bauhofer, W.; Kovacs, J.Z. A review and analysis of electrical percolation in carbon nanotube polymer composites. Compos. Sci. Technol. 2009, 69, 1486–1498. [Google Scholar] [CrossRef]
- Buatip, N.; Munthala, D.; Amonpattaratkit, P.; Pakawanit, P.; Hu, X.; Jongpinit, W.; Janphuang, P.; Wan, C.; Bowen, C.; Pojprapai, S. Piezo-tribo-electric nanogenerator based on BCZT/MCNTs/PDMS piezoelectric composite for compressive energy harvesting. Mater. Res. Bull. 2024, 173, 112686. [Google Scholar] [CrossRef]
- Nath, K.; Ghosh, S.K.; Das, P.; Katheria, A.; Das, N.C. Synthesis of ionic liquid modified 1-D nanomaterial and its strategical distribution into the biodegradable binary polymer matrix to get reduced electrical percolation threshold and electromagnetic interference shielding effectiveness. J. Appl. Polym. Sci. 2023, 141, e54897. [Google Scholar] [CrossRef]
- He, L.; Tjong, S.C. Low percolation threshold of graphene/polymer composites prepared by solvothermal reduction of graphene oxide in the polymer solution. Nanoscale Res. Lett. 2013, 8, 132. [Google Scholar] [CrossRef]
- Cheraghi, M.S.; Pircheraghi, G. Simultaneous enhancement of electrical conductivity and shape memory behavior of polystyrene/ethylene octene copolymer blend nanocomposites by CNTs through double percolation. Mater. Today Commun. 2023, 37, 107209. [Google Scholar] [CrossRef]
- Zhou, L.; Tian, Y.; Xu, P.; Wei, H.; Li, Y.; Peng, H.-X.; Qin, F. Effect of the selective localization of carbon nanotubes and phase domain in immiscible blends on tunable microwave dielectric properties. Compos. Sci. Technol. 2021, 213, 108919. [Google Scholar] [CrossRef]
- Chen, J.; Shi, Y.-Y.; Yang, J.-H.; Zhang, N.; Huang, T.; Chen, C.; Wang, Y.; Zhou, Z.-W. A simple strategy to achieve very low percolation threshold via the selective distribution of carbon nanotubes at the interface of polymer blends. J. Mater. Chem. 2012, 22, 22398–22404. [Google Scholar] [CrossRef]
- Liu, Y.; He, H.; Tian, G.; Wang, Y.; Gao, J.; Wang, C.; Xu, L.; Zhang, H. Morphology evolution to form double percolation polylactide/polycaprolactone/MWCNTs nanocomposites with ultralow percolation threshold and excellent EMI shielding. Compos. Sci. Technol. 2021, 214, 108956. [Google Scholar] [CrossRef]
- Gubbels, F.; Jerome, R.; Teyssie, P.; Vanlathem, E.; Deltour, R.; Calderone, A.; Parente, V.; Bredas, J.L. Selective Localization of Carbon Black in Immiscible Polymer Blends: A Useful Tool to Design Electrical Conductive Composites. Macromolecules 1994, 27, 1972–1974. [Google Scholar] [CrossRef]
- Sumita, M.; Sakata, K.; Asai, S.; Miyasaka, K.; Nakagawa, H. Dispersion of fillers and the electrical conductivity of polymer blends filled with carbon black. Polym. Bull. 1991, 25, 265–271. [Google Scholar] [CrossRef]
- Pötschke, P.; Bhattacharyya, A.R.; Janke, A. Carbon nanotube-filled polycarbonate composites produced by melt mixing and their use in blends with polyethylene. Carbon 2004, 42, 965–969. [Google Scholar] [CrossRef]
- Al-Saleh, M.H.; Al-Anid, H.K.; Hussain, Y.A. Electrical double percolation and carbon nanotubes distribution in solution processed immiscible polymer blend. Synth. Met. 2013, 175, 75–80. [Google Scholar] [CrossRef]
- De Aguiar, J.; Decol, M.; Pachekoski, W.M.; Becker, D. Mixing-sequence controlled selective localization of carbon nanoparticles in PLA/PCL blends. Polym. Eng. Sci. 2018, 59, 323–329. [Google Scholar] [CrossRef]
- Pisitsak, P.; Magaraphan, R.; Jana, S.C. Electrically Conductive Compounds of Polycarbonate, Liquid Crystalline Polymer, and Multiwalled Carbon Nanotubes. J. Nanomater. 2012, 2012, 642080. [Google Scholar] [CrossRef]
- Yang, J.; Qi, X.; Zhang, N.; Huang, T.; Wang, Y. Carbon nanotubes toughened immiscible polymer blends. Compos. Commun. 2018, 7, 51–64. [Google Scholar] [CrossRef]
- Huang, J.; Mao, C.; Zhu, Y.; Jiang, W.; Yang, X. Control of carbon nanotubes at the interface of a co-continuous immiscible polymer blend to fabricate conductive composites with ultralow percolation thresholds. Carbon 2014, 73, 267–274. [Google Scholar] [CrossRef]
- Salehiyan, R.; Choi, W.J.; Lee, J.H.; Hyun, K. Effects of mixing protocol and mixing time on viscoelasticity of compatibilized PP/PS blends. Korea-Australia Rheol. J. 2014, 26, 311–318. [Google Scholar] [CrossRef]
- Nofar, M.; Salehiyan, R.; Ray, S.S. Influence of nanoparticles and their selective localization on the structure and properties of polylactide-based blend nanocomposites. Compos. Part B Eng. 2021, 215, 108845. [Google Scholar] [CrossRef]
- Mural, P.K.S.; Madras, G.; Bose, S. Positive temperature coefficient and structural relaxations in selectively localized MWNTs in PE/PEO blends. RSC Adv. 2013, 4, 4943–4954. [Google Scholar] [CrossRef]
- Cardinaud, R.; McNally, T. Localization of MWCNTs in PET/LDPE blends. Eur. Polym. J. 2013, 49, 1287–1297. [Google Scholar] [CrossRef]
- Zhang, K.; Yu, H.-O.; Shi, Y.-D.; Chen, Y.-F.; Zeng, J.-B.; Guo, J.; Wang, B.; Guo, Z.; Wang, M. Morphological regulation improved electrical conductivity and electromagnetic interference shielding in poly(l-lactide)/poly(ε-caprolactone)/carbon nanotube nanocomposites via constructing stereocomplex crystallites. J. Mater. Chem. C 2017, 5, 2807–2817. [Google Scholar] [CrossRef]
- Hamad, K.; Kaseem, M.; Deri, F. Poly(lactic acid)/low density polyethylene polymer blends: Preparation and characterization. Asia-Pacific J. Chem. Eng. 2012, 7, S310–S316. [Google Scholar] [CrossRef]
- Djellali, S.; Haddaoui, N.; Sadoun, T.; Bergeret, A.; Grohens, Y. Structural, morphological and mechanical characteristics of polyethylene, poly(lactic acid) and poly(ethylene-co-glycidyl methacrylate) blends. Iran. Polym. J. 2013, 22, 245–257. [Google Scholar] [CrossRef]
- Trongsatitkul, T.; Chaiwong, S. In situ fibre-reinforced composite films of poly(lactic acid)/low-density polyethylene blends: Effects of composition on morphology, transport and mechanical properties. Polym. Int. 2017, 66, 1456–1462. [Google Scholar] [CrossRef]
- Zolali, A.M.; Favis, B.D. Toughening of Cocontinuous Polylactide/Polyethylene Blends via an Interfacially Percolated Intermediate Phase. Macromolecules 2018, 51, 3572–3581. [Google Scholar] [CrossRef]
- Lu, X.; Kang, B.; Shi, S. Selective Localization of Carbon Black in Bio-Based Poly (Lactic Acid)/Recycled High-Density Polyethylene Co-Continuous Blends to Design Electrical Conductive Composites with a Low Percolation Threshold. Polymers 2019, 11, 1583. [Google Scholar] [CrossRef]
- Roman, C.; García-Morales, M.; Gupta, J.; McNally, T. On the phase affinity of multi-walled carbon nanotubes in PMMA:LDPE immiscible polymer blends. Polymer 2017, 118, 1–11. [Google Scholar] [CrossRef]
- Eutionnat-Diffo, P.A.; Cayla, A.; Chen, Y.; Guan, J.; Nierstrasz, V.; Campagne, C. Development of Flexible and Conductive Immiscible Thermoplastic/Elastomer Monofilament for Smart Textiles Applications Using 3D Printing. Polymers 2020, 12, 2300. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Shaw, L. Electrical and mechanical behaviors of carbon nanotube-filled polymer blends. J. Appl. Polym. Sci. 2005, 99, 477–488. [Google Scholar] [CrossRef]
- Brigandi, P.J.; Cogen, J.M.; Pearson, R.A. Electrically conductive multiphase polymer blend carbon-based composites. Polym. Eng. Sci. 2013, 54, 1–16. [Google Scholar] [CrossRef]
- Gao, J.-F.; Yan, D.-X.; Yuan, B.; Huang, H.-D.; Li, Z.-M. Large-scale fabrication and electrical properties of an anisotropic conductive polymer composite utilizing preferable location of carbon nanotubes in a polymer blend. Compos. Sci. Technol. 2010, 70, 1973–1979. [Google Scholar] [CrossRef]
- Owens, D.K.; Wendt, R.C. Estimation of the surface free energy of polymers. J. Appl. Polym. Sci. 1969, 13, 1741–1747. [Google Scholar] [CrossRef]
- Fowkes, F.M. Attractive Forces at Interfaces. Ind. Eng. Chem. 1964, 56, 40–52. [Google Scholar] [CrossRef]
- Kaelble, D.H. Dispersion-polar surface tension properties of organic solids. J. Adhes. 1970, 2, 66–81. [Google Scholar] [CrossRef]
- Annamalai, M.; Gopinadhan, K.; Han, S.A.; Saha, S.; Park, H.J.; Cho, E.B.; Kumar, B.; Patra, A.; Kim, S.-W.; Venkatesan, T. Surface energy and wettability of van der Waals structures. Nanoscale 2016, 8, 5764–5770. [Google Scholar] [CrossRef]
- Sobolewski, S.; Lodes, M.A.; Rosiwal, S.M.; Singer, R.F. Surface energy of growth and seeding side of free standing nanocrystalline diamond foils. Surf. Coat. Technol. 2013, 232, 640–644. [Google Scholar] [CrossRef]
- Barshilia, H.C.; Mohan, D.K.; Selvakumar, N.; Rajam, K.S. Effect of substrate roughness on the apparent surface free energy of sputter deposited superhydrophobic polytetrafluoroethylene thin films. Appl. Phys. Lett. 2009, 95, 033116. [Google Scholar] [CrossRef]
- Barber, A.H.; Cohen, S.R.; Wagner, H.D. Static and Dynamic Wetting Measurements of Single Carbon Nanotubes. Phys. Rev. Lett. 2004, 92, 186103. [Google Scholar] [CrossRef] [PubMed]
- Babinec, S.; Mussell, R.D.; Lundgard, R.; Cieslinski, R. Electroactive thermoplastics. Adv. Mater. 2000, 12, 1823–1834. [Google Scholar] [CrossRef]
- Hu, G.; Zhao, C.; Zhang, S.; Yang, M.; Wang, Z. Low percolation thresholds of electrical conductivity and rheology in poly(ethylene terephthalate) through the networks of multi-walled carbon nanotubes. Polymer 2005, 47, 480–488. [Google Scholar] [CrossRef]
- Al-Saleh, M.H.; Sundararaj, U. An innovative method to reduce percolation threshold of carbon black filled immiscible polymer blends. Compos. Part A Appl. Sci. Manuf. 2008, 39, 284–293. [Google Scholar] [CrossRef]
- McNally, T.; Pötschke, P.; Halley, P.; Murphy, M.; Martin, D.; Bell, S.E.; Brennan, G.P.; Bein, D.; Lemoine, P.; Quinn, J.P. Polyethylene multiwalled carbon nanotube composites. Polymer 2005, 46, 8222–8232. [Google Scholar] [CrossRef]
- Wang, Y.; Yang, C.; Xin, Z.; Luo, Y.; Wang, B.; Feng, X.; Mao, Z.; Sui, X. Poly(lactic acid)/carbon nanotube composites with enhanced electrical conductivity via a two-step dispersion strategy. Compos. Commun. 2022, 30, 101087. [Google Scholar] [CrossRef]
- Koehler, M.; Canestraro, C.D.; Schnitzler, M.C.; Oliveira, M.M.; Zarbin, A.J.G.; Roman, L.S.; da Luz, M.G.E. Evidence of fractal structure for charge transport in carbon-nanotube/conjugated-polymer composites. EPL Europhys. Lett. 2007, 79, 47011. [Google Scholar] [CrossRef]
- Zhang, R.; Baxendale, M.; Peijs, T. Universal resistivity–strain dependence of carbon nanotube/polymer composites. Phys. Rev. B 2007, 76, 195433. [Google Scholar] [CrossRef]
- Zare, Y.; Rhee, K.Y. The effective conductivity of polymer carbon nanotubes (CNT) nanocomposites. J. Phys. Chem. Solids 2019, 131, 15–21. [Google Scholar] [CrossRef]
- Liu, Z.; Peng, W.; Zare, Y.; Hui, D.; Rhee, K.Y. Predicting the electrical conductivity in polymer carbon nanotube nanocomposites based on the volume fractions and resistances of the nanoparticle, interphase, and tunneling regions in conductive networks. RSC Adv. 2018, 8, 19001–19010. [Google Scholar] [CrossRef] [PubMed]
- Min, C.; Shen, X.; Shi, Z.; Chen, L.; Xu, Z. The Electrical Properties and Conducting Mechanisms of Carbon Nanotube/Polymer Nanocomposites: A Review. Polym. Technol. Eng. 2010, 49, 1172–1181. [Google Scholar] [CrossRef]
- Feng, C.; Jiang, L. Micromechanics modeling of the electrical conductivity of carbon nanotube (CNT)–polymer nanocomposites. Compos. Part A Appl. Sci. Manuf. 2013, 47, 143–149. [Google Scholar] [CrossRef]
- Gao, J.; Wang, H.; Huang, X.; Hu, M.; Xue, H.; Li, R.K. Electrically conductive polymer nanofiber composite with an ultralow percolation threshold for chemical vapour sensing. Compos. Sci. Technol. 2018, 161, 135–142. [Google Scholar] [CrossRef]
- Fu, M.; Yu, Y.; Xie, J.J.; Wang, L.P.; Fan, M.Y.; Jiang, S.L.; Zeng, Y.K. Significant influence of film thickness on the percolation threshold of multiwall carbon nanotube/low density polyethylene composite films. Appl. Phys. Lett. 2009, 94, 012904. [Google Scholar] [CrossRef]
- Zeng, Y.; Liu, P.; Du, J.; Zhao, L.; Ajayan, P.M.; Cheng, H.-M. Increasing the electrical conductivity of carbon nanotube/polymer composites by using weak nanotube–polymer interactions. Carbon 2010, 48, 3551–3558. [Google Scholar] [CrossRef]
- Mamunya, Y.; Levchenko, V.; Boiteux, G.; Seytre, G.; Zanoaga, M.; Tanasa, F.; Lebedev, E. Controlling morphology, electrical, and mechanical properties of polymer blends by heterogeneous distribution of carbon nanotubes. Polym. Compos. 2015, 37, 2467–2477. [Google Scholar] [CrossRef]
- Salehiyan, R.; Ray, S.S. Tuning the Conductivity of Nanocomposites through Nanoparticle Migration and Interface Crossing in Immiscible Polymer Blends: A Review on Fundamental Understanding. Macromol. Mater. Eng. 2018, 304, 1800431. [Google Scholar] [CrossRef]
- Poothanari, M.A.; Xavier, P.; Bose, S.; Kalarikkal, N.; Komalan, C.; Thomas, S. Compatibilising action of multiwalled carbon nanotubes in polycarbonate/polypropylene (PC/PP) blends: Phase morphology, viscoelastic phase separation, rheology and percolation. J. Polym. Res. 2019, 26, 178. [Google Scholar] [CrossRef]
- Arif, P.M.; Sarathchandran, C.; Narayanan, A.; Saiter, A.; Terzano, R.; Allegretta, I.; Porfido, C.; Kalarikkal, N.; Thomas, S. Multiwalled carbon nanotube promotes crystallisation while preserving co-continuous phase morphology of polycarbonate/polypropylene blend. Polym. Test. 2017, 64, 1–11. [Google Scholar] [CrossRef]
- Sharika, T.; Abraham, J.; Arif, P.M.; George, S.C.; Kalarikkal, N.; Thomas, S. Excellent electromagnetic shield derived from MWCNT reinforced NR/PP blend nanocomposites with tailored microstructural properties. Compos. Part B Eng. 2019, 173, 106798. [Google Scholar] [CrossRef]
- Luo, Y.; Xiong, S.; Zhang, F.; He, X.; Lu, X.; Peng, R. Preparation of conductive polylactic acid/high density polyethylene/carbon black composites with low percolation threshold by locating the carbon black at the Interface of co-continuous blends. J. Appl. Polym. Sci. 2020, 138, 50291. [Google Scholar] [CrossRef]
- Al-Saleh, M.H.; El-Methaly, H.M. Influence of PLA/HDPE Ratio and CNT content on the morphology, electrical, and EMI shielding of CNT-filled PLA/HDPE blends. Synth. Met. 2024, 304, 117592. [Google Scholar] [CrossRef]
- Hamad, K.; Kaseem, M.; Deri, F. Melt Rheology of Poly(Lactic Acid)/Low Density Polyethylene Polymer Blends. Adv. Chem. Eng. Sci. 2011, 1, 208–214. [Google Scholar] [CrossRef]
- Djellali, S.; Sadoun, T.; Haddaoui, N.; Bergeret, A. Viscosity and viscoelasticity measurements of low density polyethylene/poly(lactic acid) blends. Polym. Bull. 2015, 72, 1177–1195. [Google Scholar] [CrossRef]
- Göldel, A.; Marmur, A.; Kasaliwal, G.R.; Pötschke, P.; Heinrich, G. Shape-Dependent Localization of Carbon Nanotubes and Carbon Black in an Immiscible Polymer Blend during Melt Mixing. Macromolecules 2011, 44, 6094–6102. [Google Scholar] [CrossRef]
- Li, Q.; Basavarajaiah, S.; Kim, N.H.; Heo, S.-B.; Lee, J.H. Synergy effect of hybrid fillers on the positive temperature coefficient behavior of polypropylene/ultra-high molecular weight polyethylene composites. J. Appl. Polym. Sci. 2009, 116, 116–124. [Google Scholar] [CrossRef]
- Shi, S.; Xu, W.; Zhou, B.; Qin, S.; Liu, X.; Li, H. Low-density polyethylene-multi-walled carbon nanotube nanocomposite membranes with enhanced conductivity for highly sensitive vapor sensing. Adv. Compos. Hybrid Mater. 2023, 6, 168. [Google Scholar] [CrossRef]
- Ma, C.; Zhu, B.; Qian, Z.; Ren, L.; Yuan, H.; Meng, Y. 3D-printing of conductive inks based flexible tactile sensor for monitoring of temperature, strain and pressure. J. Manuf. Process. 2023, 87, 1–10. [Google Scholar] [CrossRef]
- Lee, J.; So, H. 3D-printing-assisted flexible pressure sensor with a concentric circle pattern and high sensitivity for health monitoring. Microsystems Nanoeng. 2023, 9, 44. [Google Scholar] [CrossRef]
- Tang, Z.-H.; Xue, S.-S.; Wang, D.-Y.; Huang, P.; Li, Y.-Q.; Fu, S.-Y. 3D printing of soft and porous composite pressure sensor with monotonic and positive resistance response. Compos. Sci. Technol. 2023, 241, 110126. [Google Scholar] [CrossRef]



| Nomenclature | Components on 1st Mixing Step | Components on 2nd Mixing Step |
|---|---|---|
| PLA/LDPE/CNT | PLA, LDPE, and CNT | - |
| PLA/[LDPE + CNT] | LDPE and CNT; [LDPE + CNT] | Added PLA after 5 min melt-mixing |
| [PLA + CNT]/LDPE | PLA and CNT; [PLA + CNT] | Added LDPE after 5 min melt-mixing |
| LDPE/CNT | LDPE and CNT | - |
| Solvent | Surface Tension (mJ/m2) | ||
|---|---|---|---|
| ) | |||
| DI water | 21.8 | 51.0 | 72.8 |
| Hexane | 18.4 | 0 | 18.4 |
| Glycerol | 34.0 | 30.0 | 64.0 |
| Ethanol | 18.8 | 2.6 | 21.4 |
| Components | (mJ/m2) | (mJ/m2) | (mJ/m2) | Polarity (%) | |||
|---|---|---|---|---|---|---|---|
| 25 °C | 170 °C | 25 °C | 170 °C | 25 °C | 170 °C | ||
| PLA | 4.0 | 1.57 | 78.2 | 30.62 | 82.2 | 32.19 | 95 |
| LDPE | 3.8 | 1.50 | 30.0 | 11.76 | 33.9 | 13.26 | 89 |
| CNT [42] | 17.6 | 10.2 | 27.8 | 37 | |||
| Parameters | Harmonic-Mean Equation | Geometric-Mean Equation |
|---|---|---|
| (mJ/m2) | 23.629 | 14.139 |
| (mJ/m2) | 13.671 | 8.869 |
| (mJ/m2) | 8.396 | 4.430 |
| Wetting coefficient (ω) | –1.186 | –1.190 |
| Theoretical prediction of CNTs’ localization | LDPE phase | LDPE phase |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kajornprai, T.; Jarapanyacheep, R.; Saikaeo, J.; Pojprapai, S.; Jarukumjorn, K.; Trongsatitkul, T. Double Percolation of Poly(lactic acid)/Low-Density Polyethylene/Carbon Nanotube (PLA/LDPE/CNT) Composites for Force-Sensor Application: Impact of Preferential Localization and Mixing Sequence. Polymers 2024, 16, 1906. https://doi.org/10.3390/polym16131906
Kajornprai T, Jarapanyacheep R, Saikaeo J, Pojprapai S, Jarukumjorn K, Trongsatitkul T. Double Percolation of Poly(lactic acid)/Low-Density Polyethylene/Carbon Nanotube (PLA/LDPE/CNT) Composites for Force-Sensor Application: Impact of Preferential Localization and Mixing Sequence. Polymers. 2024; 16(13):1906. https://doi.org/10.3390/polym16131906
Chicago/Turabian StyleKajornprai, Todsapol, Rapisa Jarapanyacheep, Jatupat Saikaeo, Soodkhet Pojprapai, Kasama Jarukumjorn, and Tatiya Trongsatitkul. 2024. "Double Percolation of Poly(lactic acid)/Low-Density Polyethylene/Carbon Nanotube (PLA/LDPE/CNT) Composites for Force-Sensor Application: Impact of Preferential Localization and Mixing Sequence" Polymers 16, no. 13: 1906. https://doi.org/10.3390/polym16131906
APA StyleKajornprai, T., Jarapanyacheep, R., Saikaeo, J., Pojprapai, S., Jarukumjorn, K., & Trongsatitkul, T. (2024). Double Percolation of Poly(lactic acid)/Low-Density Polyethylene/Carbon Nanotube (PLA/LDPE/CNT) Composites for Force-Sensor Application: Impact of Preferential Localization and Mixing Sequence. Polymers, 16(13), 1906. https://doi.org/10.3390/polym16131906






