Biocomposite Material Based on Lactococcus lactis sp. Immobilized in Natural Polymer Matrix for Pharmaceutical Removal from Aqueous Media
Abstract
1. Introduction
2. Materials and Methods
2.1. Reagents and Analytical Procedure
2.2. Biosorbent Synthesis and Characterization
2.2.1. Synthesis of Biosorbent Using Biomass of Lactococcus lactis
2.2.2. Biosorbent Characterization (SEM, FTIR, Point of Zero Charge)
2.3. Batch Biosorption Methodology
2.4. Modeling the Biosorption Experimental Data
2.4.1. Kinetic Models
2.4.2. Equilibrium Isotherms
2.5. Regeneration Studies
3. Results and Discussion
3.1. Biosorbent Synthesis and Characterization
3.2. Influence of Main Parameters on the Biosorption Process
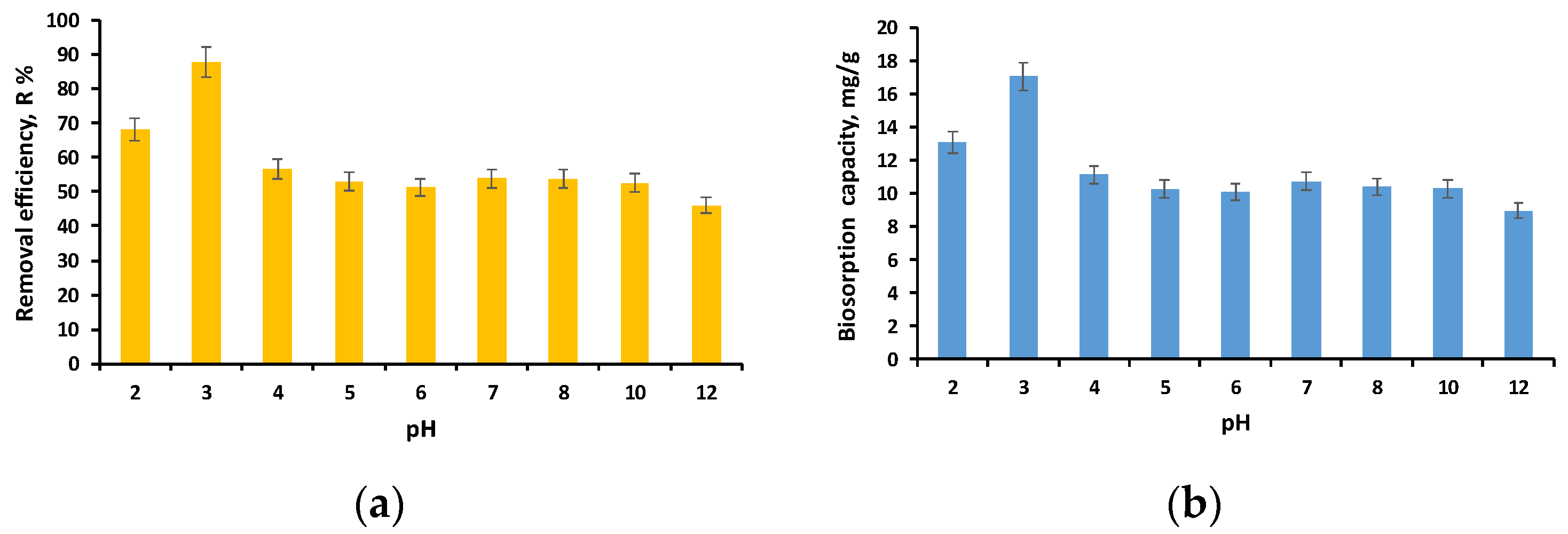
3.2.1. Influence of pH on the Biosorption Process
3.2.2. Influence of Biosorbent Dose on the Biosorption Process
3.2.3. Influence of EL Initial Concentration on the Biosorption Process
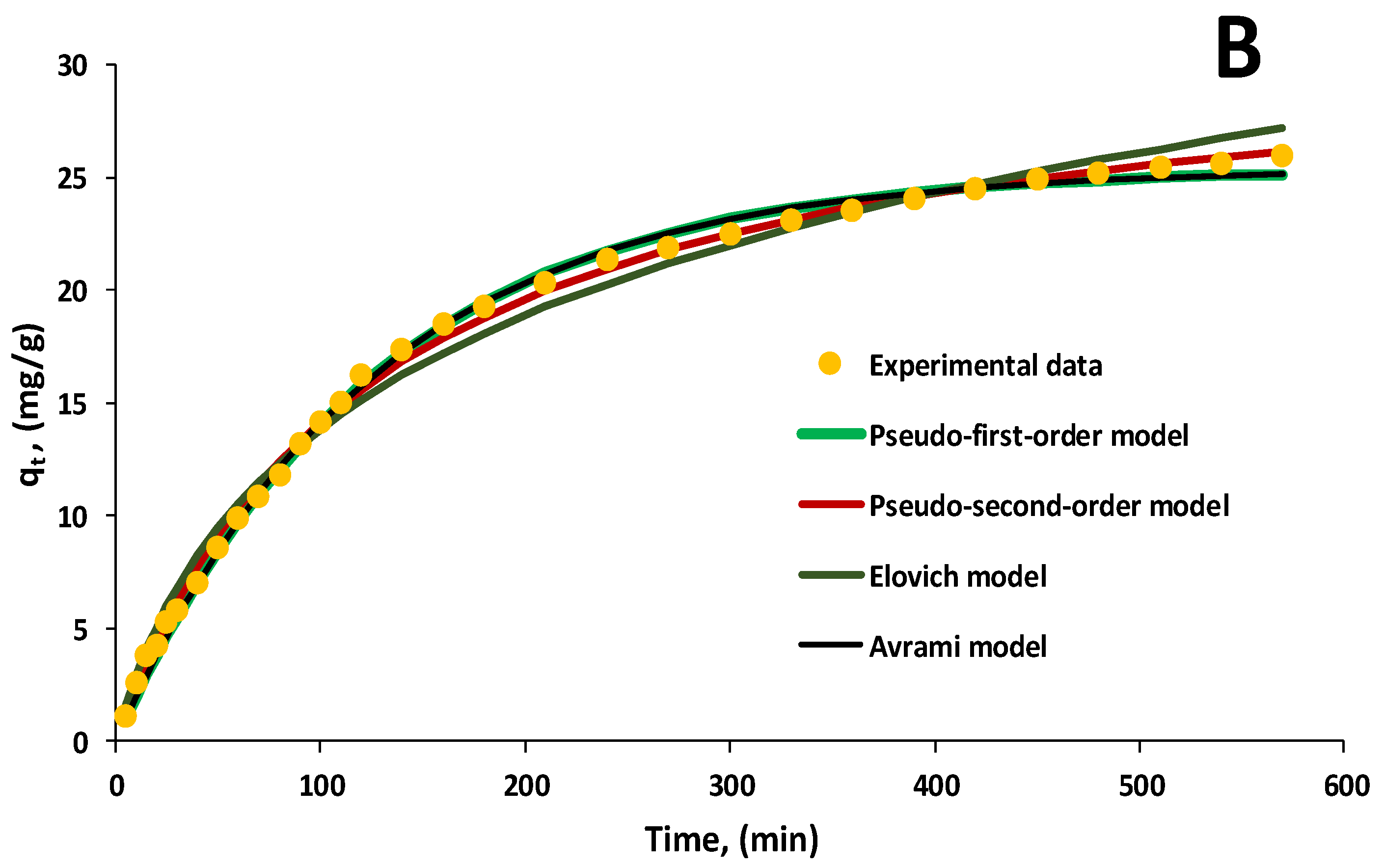
3.3. Kinetic Evaluation of the Biosorption Process
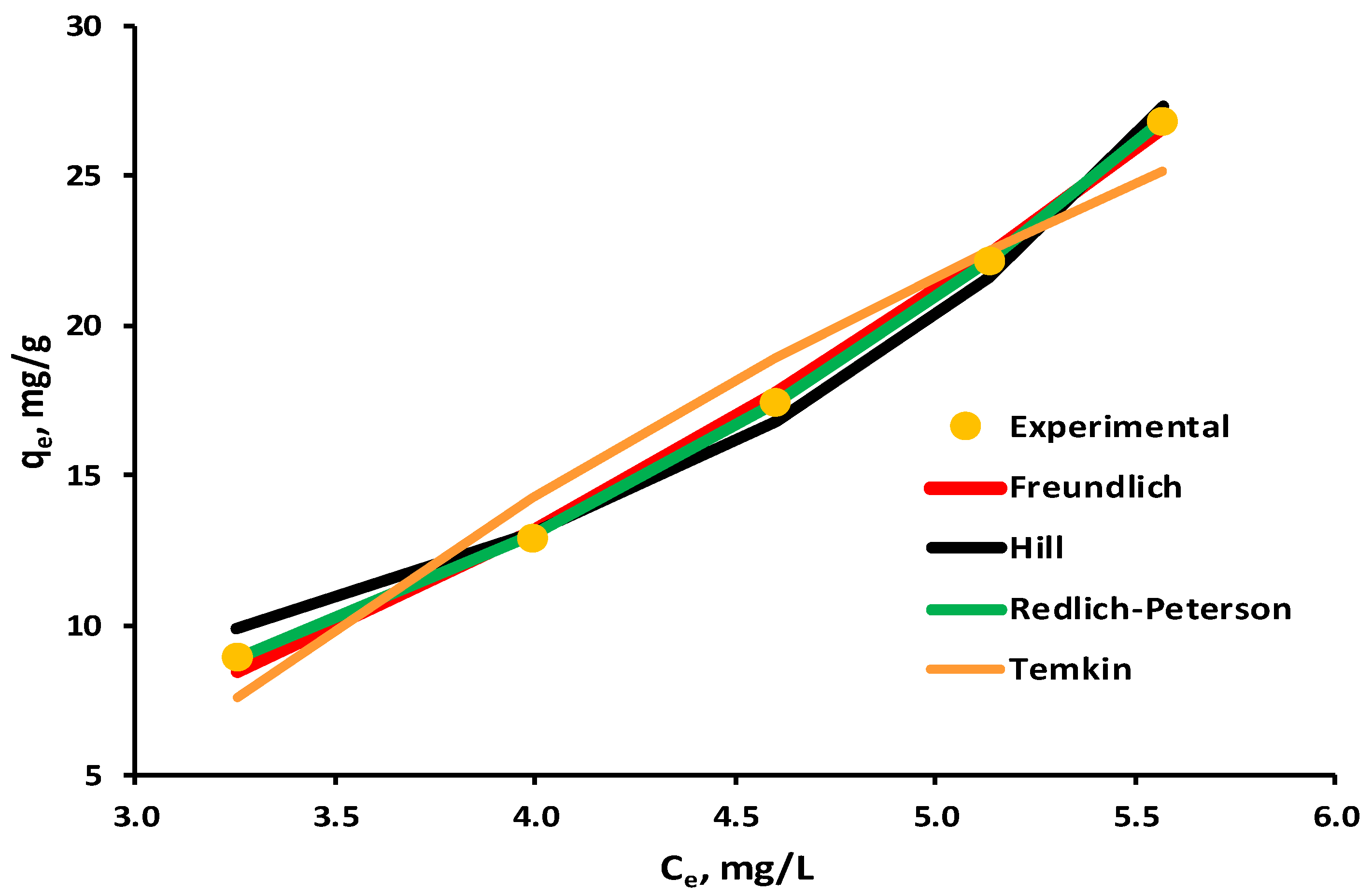
3.4. Equilibrium Isotherms
3.5. Regeneration and Reusability of Spent 5% LLA Biosorbent
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Ternes, T.; Joss, A. Human Pharmaceuticals, Hormones and Fragrances; IWA Publishing: London, UK, 2007. [Google Scholar]
- Fernandes, J.P.; Almeida, C.M.R.; Salgado, M.A.; Carvalho, M.F.; Mucha, A.P. Pharmaceutical compounds in aquatic environments—Occurrence, fate and bioremediation prospective. Toxics 2021, 9, 257. [Google Scholar] [CrossRef] [PubMed]
- Caracciolo, A.B.; Topp, E.; Grenni, P. Pharmaceuticals in the environment: Biodegradation and effects on natural microbial communities. A review. J. Pharm. Biomed. Anal. 2015, 106, 25–36. [Google Scholar] [CrossRef] [PubMed]
- Bendz, D.; Paxéus, N.A.; Ginn, T.R.; Loge, F.J. Occurrence and fate of pharmaceutically active compounds in the environment, a case study: Höje River in Sweden. J. Hazard. Mater. 2005, 122, 195–204. [Google Scholar] [CrossRef] [PubMed]
- Patel, M.; Kumar, R.; Kishor, K.; Mlsna, T.; Pittman, C.U., Jr.; Mohan, D. Pharmaceuticals of emerging concern in aquatic systems: Chemistry, occurrence, effects, and removal methods. Chem. Rev. 2019, 119, 3510–3673. [Google Scholar] [CrossRef] [PubMed]
- Kümmerer, K. The presence of pharmaceuticals in the environment due to human use—Present knowledge and future challenges. J. Environ. Manag. 2009, 90, 2354–2366. [Google Scholar] [CrossRef] [PubMed]
- Li, W.C. Occurrence, sources, and fate of pharmaceuticals in aquatic environment and soil. Environ. Pollut. 2014, 187, 193–201. [Google Scholar] [CrossRef] [PubMed]
- Verlicchi, P.; Al Aukidy, M.; Zambello, E. Occurrence of pharmaceutical compounds in urban wastewater: Removal, mass load and environmental risk after a secondary treatment—A review. Sci. Total Environ. 2012, 429, 123–155. [Google Scholar] [CrossRef] [PubMed]
- Rivera-Utrilla, J.; Sánchez-Polo, M.; Ferro-García, M.Á.; Prados-Joya, G.; Ocampo-Pérez, R. Pharmaceuticals as emerging contaminants and their removal from water. A review. Chemosphere 2013, 93, 1268–1287. [Google Scholar] [CrossRef] [PubMed]
- Wilkinson, J.; Hooda, P.S.; Barker, J.; Barton, S.; Swinden, J. Occurrence, fate and transformation of emerging contaminants in water: An overarching review of the field. Environ. Pollut. 2017, 231, 954–970. [Google Scholar] [CrossRef]
- Miller, T.H.; Bury, N.R.; Owen, S.F.; MacRae, J.I.; Barron, L.P. A review of the pharmaceutical exposome in aquatic fauna. Environ. Pollut. 2018, 239, 129–146. [Google Scholar] [CrossRef]
- Brown, K.D.; Kulis, J.; Thomson, B.; Chapman, T.H.; Mawhinney, D.B. Occurrence of antibiotics in hospital, residential, and dairy effluent, municipal wastewater, and the Rio Grande in New Mexico. Sci. Total Environ. 2006, 366, 772–783. [Google Scholar] [CrossRef]
- Feitosa-Felizzola, J.; Chiron, S. Occurrence and distribution of selected antibiotics in a small Mediterranean stream (Arc River, Southern France). J. Hydrol. 2009, 364, 50–57. [Google Scholar] [CrossRef]
- Watkinson, A.; Murby, E.; Kolpin, D.W.; Costanzo, S. The occurrence of antibiotics in an urban watershed: From wastewater to drinking water. Sci. Total Environ. 2009, 407, 2711–2723. [Google Scholar] [CrossRef] [PubMed]
- Da Silva, B.F.; Jelic, A.; López-Serna, R.; Mozeto, A.A.; Petrovic, M.; Barceló, D. Occurrence and distribution of pharmaceuticals in surface water, suspended solids and sediments of the Ebro river basin, Spain. Chemosphere 2011, 85, 1331–1339. [Google Scholar] [CrossRef] [PubMed]
- Verlicchi, P.; Zambello, E. Pharmaceuticals and personal care products in untreated and treated sewage sludge: Occurrence and environmental risk in the case of application on soil—A critical review. Sci. Total Environ. 2015, 538, 750–767. [Google Scholar] [CrossRef] [PubMed]
- Grenni, P.; Ancona, V.; Caracciolo, A.B. Ecological effects of antibiotics on natural ecosystems: A review. Microchem. J. 2018, 136, 25–39. [Google Scholar] [CrossRef]
- Bartrons, M.; Peñuelas, J. Pharmaceuticals and personal-care products in plants. Trends Plant Sci. 2017, 22, 194–203. [Google Scholar] [CrossRef] [PubMed]
- González-Alonso, S.; Merino, L.M.; Esteban, S.; de Alda, M.L.; Barceló, D.; Durán, J.J.; López-Martínez, J.; Aceña, J.; Pérez, S.; Mastroianni, N. Occurrence of pharmaceutical, recreational and psychotropic drug residues in surface water on the northern Antarctic Peninsula region. Environ. Pollut. 2017, 229, 241–254. [Google Scholar] [CrossRef] [PubMed]
- Felis, E.; Kalka, J.; Sochacki, A.; Kowalska, K.; Bajkacz, S.; Harnisz, M.; Korzeniewska, E. Antimicrobial pharmaceuticals in the aquatic environment—Occurrence and environmental implications. Eur. J. Pharmacol. 2020, 866, 172813. [Google Scholar] [CrossRef]
- Rusu, L.; Suceveanu, E.-M.; Blaga, A.-C.; Nedeff, F.M.; Șuteu, D. Insights into recent advances of biomaterials based on microbial biomass and natural polymers for sustainable removal of pharmaceuticals residues. Polymers 2023, 15, 2923. [Google Scholar] [CrossRef]
- Quesada, H.B.; Baptista, A.T.A.; Cusioli, L.F.; Seibert, D.; de Oliveira Bezerra, C.; Bergamasco, R. Surface water pollution by pharmaceuticals and an alternative of removal by low-cost adsorbents: A review. Chemosphere 2019, 222, 766–780. [Google Scholar] [CrossRef] [PubMed]
- U.S. Food and Drug Administration. Orange Book: Approved Drug Products with Therapeutic Equivalence Evaluations; FDA: Silver Spring, MD, USA, 2020. [Google Scholar]
- Puckowski, A.; Mioduszewska, K.; Łukaszewicz, P.; Borecka, M.; Caban, M.; Maszkowska, J.; Stepnowski, P. Bioaccumulation and analytics of pharmaceutical residues in the environment: A review. J. Pharm. Biomed. Anal. 2016, 127, 232–255. [Google Scholar] [CrossRef] [PubMed]
- Ebele, A.J.; Abdallah, M.A.-E.; Harrad, S. Pharmaceuticals and personal care products (PPCPs) in the freshwater aquatic environment. Emerg. Contam. 2017, 3, 1–16. [Google Scholar] [CrossRef]
- Ding, C.; He, J. Effect of antibiotics in the environment on microbial populations. Appl. Microbiol. Biotechnol. 2010, 87, 925–941. [Google Scholar] [CrossRef]
- Marti, E.; Variatza, E.; Balcazar, J.L. The role of aquatic ecosystems as reservoirs of antibiotic resistance. Trends Microbiol. 2014, 22, 36–41. [Google Scholar] [CrossRef]
- Le-Minh, N.; Khan, S.; Drewes, J.; Stuetz, R. Fate of antibiotics during municipal water recycling treatment processes. Water Res. 2010, 44, 4295–4323. [Google Scholar] [CrossRef]
- Erşan, M. Removal of tetracycline using new biocomposites from aqueous solutions. Desalination Water Treat. 2016, 57, 9982–9992. [Google Scholar] [CrossRef]
- Rusu, L.; Grigoraș, C.-G.; Simion, A.-I.; Suceveanu, E.-M.; Blaga, A.-C.; Harja, M. Encapsulation of Saccharomyces pastorianus residual biomass in calcium alginate matrix with insights in ethacridine lactate biosorption. Polymers 2022, 14, 170. [Google Scholar] [CrossRef]
- Ahmad, A.; Priyadarshini, M.; Das, S.; Ghangrekar, M.M. Electrocoagulation as an efficacious technology for the treatment of wastewater containing active pharmaceutical compounds: A review. Sep. Sci. Technol. 2022, 57, 1234–1256. [Google Scholar] [CrossRef]
- Vrinceanu, N.; Hlihor, R.M.; Simion, A.I.; Rusu, L.; Fekete-Kertész, I.; Barka, N.; Favier, L. New evidence of the enhanced elimination of a persistent drug used as a lipid absorption inhibitor by advanced oxidation with UV-A and nanosized catalysts. Catalysts 2019, 9, 761. [Google Scholar] [CrossRef]
- Akhtar, J.; Amin, N.A.S.; Shahzad, K. A review on removal of pharmaceuticals from water by adsorption. Desalination Water Treat. 2016, 57, 12842–12860. [Google Scholar] [CrossRef]
- Silva, C.P.; Jaria, G.; Otero, M.; Esteves, V.I.; Calisto, V. Waste-based alternative adsorbents for the remediation of pharmaceutical contaminated waters: Has a step forward already been taken? Bioresour. Technol. 2018, 250, 888–901. [Google Scholar] [CrossRef] [PubMed]
- Torres, E. Biosorption: A review of the latest advances. Processes 2020, 8, 1584. [Google Scholar] [CrossRef]
- Wang, L.; Xiao, H.; He, N.; Sun, D.; Duan, S. Biosorption and biodegradation of the environmental hormone nonylphenol by four marine microalgae. Sci. Rep. 2019, 9, 5277. [Google Scholar] [CrossRef] [PubMed]
- Rusu, L.; Grigoraș, C.-G.; Suceveanu, E.M.; Simion, A.-I.; Dediu Botezatu, A.V.; Istrate, B.; Doroftei, I. Eco-friendly biosorbents based on microbial biomass and natural polymers: Synthesis, characterization and application for the removal of drugs and dyes from aqueous solutions. Materials 2021, 14, 4810. [Google Scholar] [CrossRef] [PubMed]
- Moghazy, R.M.; Labena, A.; Husien, S. Eco-friendly complementary biosorption process of methylene blue using micro-sized dried biosorbents of two macro-algal species (Ulva fasciata and Sargassum dentifolium): Full factorial design, equilibrium, and kinetic studies. Int. J. Biol. Macromol. 2019, 134, 330–343. [Google Scholar] [CrossRef] [PubMed]
- Kazou, M. Lactic acid bacteria: Lactococcus lactis. In Encyclopedia of Dairy Sciences, 3rd ed.; McSweeney, P.L.H., McNamara, J.P., Eds.; Academic Press: Cambridge, MA, USA, 2022; pp. 218–225. [Google Scholar] [CrossRef]
- Monachese, M.; Burton, J.P.; Reid, G. Advances in ureteral stent technology. Appl. Environ. Microbiol. 2012, 78, 6397. [Google Scholar]
- Mrvčić, J.; Stanzer, D.; Šolić, E.; Stehlik-Tomas, V. Interaction of lactic acid bacteria with metal ions: Opportunities for improving food safety and quality. World J. Microbiol. Biotechnol. 2012, 28, 2771–2782. [Google Scholar] [CrossRef]
- Bhakta, J.; Ohnishi, K.; Munekage, Y.; Iwasaki, K.; Wei, M. Characterization of lactic acid bacteria-based probiotics as potential heavy metal sorbents. J. Appl. Microbiol. 2012, 112, 1193–1206. [Google Scholar] [CrossRef]
- Singh, A.L.; Sarma, P. Removal of arsenic (III) from waste water using Lactobacillus acidophilus. Bioremediation J. 2010, 14, 92–97. [Google Scholar] [CrossRef]
- Halttunen, T.; Salminen, S.; Tahvonen, R. Rapid removal of lead and cadmium from water by specific lactic acid bacteria. Int. J. Food Microbiol. 2007, 114, 30–35. [Google Scholar] [CrossRef] [PubMed]
- Mishra, R.; Sinha, V.; Kannan, A.; Upreti, R.K. Reduction of chromium-VI by chromium resistant lactobacilli: A prospective bacterium for bioremediation. Toxicol. Int. 2012, 19, 25. [Google Scholar] [CrossRef] [PubMed]
- Mosier, A.P.; Behnke, J.; Jin, E.T.; Cady, N.C. Microbial biofilms for the removal of Cu2+ from CMP wastewater. J. Environ. Manag. 2015, 160, 67–72. [Google Scholar] [CrossRef] [PubMed]
- Lin, Z.; Zhou, C.; Wu, J.; Zhou, J.; Wang, L. A further insight into the mechanism of Ag+ biosorption by Lactobacillus sp. strain A09. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2005, 61, 1195–1200. [Google Scholar] [CrossRef] [PubMed]
- Milanowski, M.; Pomastowski, P.; Railean-Plugaru, V.; Rafińska, K.; Ligor, T.; Buszewski, B. Biosorption of silver cations onto Lactococcus lactis and Lactobacillus casei isolated from dairy products. PLoS ONE 2017, 12, e0174521. [Google Scholar] [CrossRef] [PubMed]
- Aronson, J. Methylendioxy-metamfetamine. In Meyler’s Side Effects of Drugs, 10th ed.; Elsevier: Barcelona, Spain, 2016. [Google Scholar]
- Wainwright, M. Acridine—A neglected antibacterial chromophore. J. Antimicrob. Chemother. 2001, 47, 1–13. [Google Scholar] [CrossRef] [PubMed]
- Talman, R.; Salihi, E.; Göktürk, S.; Baștuğ, A. Removal of ethacridine lactate from aqueous solutions onto bentonite and activated carbon. Fresenius Environ. Bull. 2015, 24, 3603–3608. [Google Scholar]
- Rusu, L.; Grigoraș, C.-G.; Simion, A.-I.; Suceveanu, E.-M.; Istrate, B.; Harja, M. Biosorption Potential of Microbial and Residual Biomass of Saccharomyces pastorianus Immobilized in Calcium Alginate Matrix for Pharmaceuticals Removal from Aqueous Solutions. Polymers 2022, 14, 2855. [Google Scholar] [CrossRef] [PubMed]
- Sahoo, T.R.; Prelot, B. Adsorption processes for the removal of contaminants from wastewater: The perspective role of nanomaterials and nanotechnology. In Nanomaterials for the Detection and Removal of Wastewater Pollutants; Elsevier: Amsterdam, The Netherlands, 2020; pp. 161–222. [Google Scholar]
- Ebelegi, A.N.; Ayawei, N.; Wankasi, D. Interpretation of adsorption thermodynamics and kinetics. Open J. Phys. Chem. 2020, 10, 166–182. [Google Scholar] [CrossRef]
- Lagergren, S. Zur Theorie der sogenannten Adsorption geloster Stoffe. Kolloid-Z. Z. Polym. 1898, 2, 15. [Google Scholar]
- Ho, Y.; Wase, D.A.J.; Forster, C.F. Removal of lead ions from aqueous solution using sphagnum moss peat as adsorbent. Water SA 1996, 22, 219–224. [Google Scholar]
- Elovich, S.Y.; Larinov, O.G. Theory of adsorption from solutions of non electrolytes on solid (I) equation adsorption from solutions and the analysis of its simplest form, (II) verification of the equation of adsorption isotherm from solutions. Izv. Akad. Nauk. SSSR Otd. Khim. Nauk 1962, 2, 209–216. [Google Scholar]
- Avrami, M. Kinetics of phase change. I General theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Weber, W.J., Jr.; Morris, J.C. Kinetics of adsorption on carbon from solution. J. Sanit. Eng. Div. 1963, 89, 31–59. [Google Scholar] [CrossRef]
- Musah, M.; Azeh, Y.; Mathew, J.T.; Umar, M.T.; Abdulhamid, Z.; Muhammad, A.I. Adsorption kinetics and isotherm models: A review. CaJoST 2022, 4, 20–26. [Google Scholar] [CrossRef]
- Freundlich, H.; Helle, W. Flubber die adsorption in lusungen. J. Am. Chem. Soc. 1939, 61, 2–28. [Google Scholar]
- Redlich, O.; Peterson, D.L. A useful adsorption isotherm. J. Phys. Chem. 1959, 63, 1024. [Google Scholar] [CrossRef]
- Temkin, M. Kinetics of ammonia synthesis on promoted iron catalysts. Acta Physiochim. URSS 1940, 12, 327–356. [Google Scholar]
- Tóth, J. Uniform interpretation of gas/solid adsorption. Adv. Colloid Interface Sci. 1995, 55, 1–239. [Google Scholar] [CrossRef]
- Pierce, C. The Frenkel-Halsey-Hill adsorption isotherm and capillary condensation. J. Phys. Chem. 1960, 64, 1184–1187. [Google Scholar] [CrossRef]
- Ayawei, N.; Ebelegi, A.N.; Wankasi, D. Modelling and interpretation of adsorption isotherms. J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Wang, J.; Guo, X. Adsorption isotherm models: Classification, physical meaning, application and solving method. Chemosphere 2020, 258, 127279. [Google Scholar] [CrossRef] [PubMed]
- Jing, H.; Huang, X.; Du, X.; Mo, L.; Ma, C.; Wang, H. Facile synthesis of pH-responsive sodium alginate/carboxymethyl chitosan hydrogel beads promoted by hydrogen bond. Carbohydr. Polym. 2022, 278, 118993. [Google Scholar] [CrossRef] [PubMed]
- Al-sareji, O.J.; Abdulzahra, M.A.; Hussein, T.S.; Shlakaa, A.S.; Karhib, M.M.; Meiczinger, M.; Grmasha, R.A.; Al-Juboori, R.A.; Somogyi, V.; Domokos, E. Removal of pharmaceuticals from water using laccase immobilized on orange peels waste-derived activated carbon. Water 2023, 15, 3437. [Google Scholar] [CrossRef]
- Bhattarai, S.; Kim, J.S.; Yun, Y.-S.; Lee, Y.-S. Thiourea-Immobilized Polymer Beads for Sorption of Cr (VI) Ions in Acidic Aqueous Media. Macromol. Res. 2019, 27, 515–521. [Google Scholar] [CrossRef]
- Pereira, R.; Mendes, A.; Bártolo, P. Alginate/Aloe vera hydrogel films for biomedical applications. Procedia CIRP 2013, 5, 210–215. [Google Scholar] [CrossRef]
- Van der Meulen, R.; Grosu-Tudor, S.; Mozzi, F.; Vaningelgem, F.; Zamfir, M.; de Valdez, G.F.; De Vuyst, L. Screening of lactic acid bacteria isolates from dairy and cereal products for exopolysaccharide production and genes involved. Int. J. Food Microbiol. 2007, 118, 250–258. [Google Scholar] [CrossRef] [PubMed]
- Dabour, N.; LaPointe, G. Identification and molecular characterization of the chromosomal exopolysaccharide biosynthesis gene cluster from Lactococcus lactis subsp. cremoris SMQ-461. Appl. Environ. Microbiol. 2005, 71, 7414–7425. [Google Scholar] [CrossRef] [PubMed]
- Bajpai, V.K.; Rather, I.A.; Majumder, R.; Shukla, S.; Aeron, A.; Kim, K.; Kang, S.C.; Dubey, R.; Maheshwari, D.; Lim, J. Exopolysaccharide and lactic acid bacteria: Perception, functionality and prospects. Bangladesh J. Pharmacol. 2016, 11, 1–23. [Google Scholar] [CrossRef]
- Wang, W.; Ju, Y.; Liu, N.; Shi, S.; Hao, L. Structural characteristics of microbial exopolysaccharides in association with their biological activities: A review. Chem. Biol. Technol. Agric. 2023, 10, 137. [Google Scholar] [CrossRef]
- Kowalczuk, D.; Pitucha, M. Application of FTIR method for the assessment of immobilization of active substances in the matrix of biomedical materials. Materials 2019, 12, 2972. [Google Scholar] [CrossRef] [PubMed]
- Jang, M.-H.; Kim, M.-S.; Han, M.; Kwak, D.-H. Handling Editor: Derek Muir Keywords: Aggregation Attachment efficiency Isoelectric point Microplastic Particle Zero-point charge. Chemosphere 2022, 299, 134388. [Google Scholar] [CrossRef] [PubMed]
- Largitte, L.; Pasquier, R. A review of the kinetics adsorption models and their application to the adsorption of lead by an activated carbon. Chem. Eng. Res. Des. 2016, 109, 495–504. [Google Scholar] [CrossRef]
- Silva, E.C.; Soares, V.R.; Fajardo, A.R. Removal of pharmaceuticals from aqueous medium by alginate/polypyrrole/ZnFe2O4 beads via magnetic field enhanced adsorption. Chemosphere 2023, 316, 137734. [Google Scholar] [CrossRef]
- Amor, A.B.; Arenas, M.; Martín, J.; Ouakouak, A.; Santos, J.L.; Aparicio, I.; Alonso, E.; Hamdi, N. Alginate/geopolymer hybrid beads as an innovative adsorbent applied to the removal of 5-fluorouracil from contaminated environmental water. Chemosphere 2023, 335, 139092. [Google Scholar] [CrossRef]
- Correa-Navarro, Y.M.; Giraldo, L.; Moreno-Piraján, J.C. Biochar from fique bagasse for remotion of caffeine and diclofenac from aqueous solution. Molecules 2020, 25, 1849. [Google Scholar] [CrossRef]
- Tagyan, A.I.; Yasser, M.M.; Mousa, A.M.; Alkhalifah, D.H.M.; Hozzein, W.N.; Marzouk, M.A. Potential application of innovative Aspergillus terreus/sodium alginate composite beads as eco-friendly and sustainable adsorbents for alizarin red S dye: Isotherms and kinetics models. Microorganisms 2023, 11, 1135. [Google Scholar] [CrossRef] [PubMed]
- Mokeddem, A.; Benykhlef, S.; Bendaoudi, A.A.; Boudouaia, N.; Mahmoudi, H.; Bengharez, Z.; Topel, S.D.; Topel, Ö. Sodium alginate-based composite films for effective removal of congo red and coralene dark red 2B dyes: Kinetic, isotherm and thermodynamic analysis. Water 2023, 15, 1709. [Google Scholar] [CrossRef]
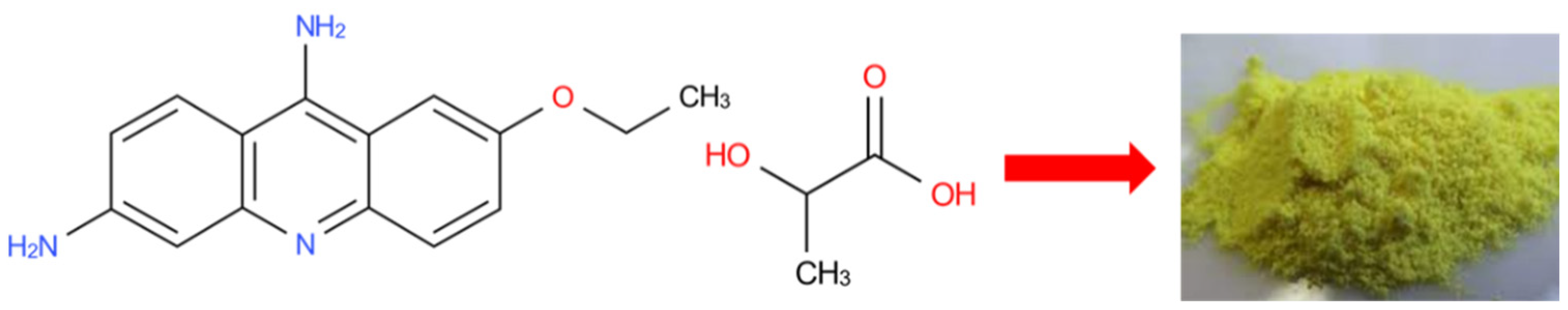







| Kinetic Model | Equation | Parameter Significance | Ref. * |
|---|---|---|---|
| Pseudo-first-order | t—time (min), qt—concentration on the solid phase at time t (mg/g), qe—adsorbent capacity at equilibrium (mg/g), k1—constant rate (L/min) | [55] | |
| Pseudo-second-order | t—time (min), qt—concentration on the solid phase at time t (mg/g), qe—adsorbent capacity at equilibrium (mg/g), k2—constant rate (L/min) | [56] | |
| Elovich | t—time (min), qt—concentration on the solid phase at time t (mg/g), α—initial adsorption rate (mg/(gmin)), β—extent of surface coverage and activation energy for chemisorption (g/mg) | [57] | |
| Avrami | t—time (min), qt—concentration on the solid phase at time t (mg/g), qe—adsorbent capacity at equilibrium (mg/g), k_avr—the overall rate constant (L/min), n_avr– (dimensionless) is parameter related to adsorption | [58] | |
| Weber–Morris | t—time (min), qt—concentration on the solid phase at time t (mg/g), Kwm—(mg/gmin0.5), B –provides information about the thickness of the boundary layer (mg/g) | [59] |
| Equilibrium Isotherm | Equation | Constant Significance | Ref. * |
|---|---|---|---|
| Freundlich | qe—adsorbate concentration on the solid phase at equilibrium (mg/g), Ce—adsorbate concentration on the fluid phase at equilibrium (mg/L), Kf—Freundlich constant ((mg/g) n—Freundlich constant (dimensionless) | [61] | |
| Redlich–Peterson | qe—adsorbate concentration on the solid phase at equilibrium (mg/g), Ce—adsorbate concentration on the fluid phase at equilibrium (mg/L), Kr1,, Kr2—Redlich–Peterson constants, Cen—Redlich–Peterson exponent (dimensionless) | [62] | |
| Temkin | qe—adsorbate concentration on the solid phase at equilibrium (mg/g), Ce—adsorbate concentration on the fluid phase at equilibrium (mg/L), R—gas constant (R = 8.314 J/(mol K)), T—temperature (K), Kt—Temkin constant (L/mg), b—Temkin constant (J/mg) | [63] | |
| Toth | qe—adsorbate concentration on the solid phase at equilibrium (mg/g), Ce—adsorbate concentration on the fluid phase at equilibrium (mg/L), qt—Toth maximum uptake (mg/g), bt—Toth constant (L/mg), nt—Toth constant (dimensionless) | [64] | |
| Hill | qe—adsorbate concentration on the solid phase at equilibrium (mg/g), Ce—adsorbate concentration on the fluid phase at equilibrium (mg/L), qh—Hill maximum uptake (mg/g), Kh—Hill constant (L/mg), nh—cooperativity coefficient of the binding interaction (dimensionless) | [65] |
| Kinetic Model | EL Initial Concentration, mg/L | qe | k1 | k2 | α | β | kAv | nAv | k | B | R2 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Pseudo-first- order | 20 | 8.1175 | 0.0101 | - | - | - | - | - | - | - | 0.9939 |
| 30 | 12.2177 | 0.0082 | - | - | - | - | - | - | - | 0.9980 | |
| 40 | 16.3806 | 0.0109 | - | - | - | - | - | - | - | 0.9908 | |
| 50 | 20.8372 | 0.0090 | - | - | - | - | - | - | - | 0.9949 | |
| 60 | 25.3984 | 0.0081 | - | - | - | - | - | - | - | 0.9972 | |
| Pseudo-second-order | 20 | 9.8225 | - | 0.0011 | - | - | - | - | - | - | 0.9976 |
| 30 | 15.4417 | - | 0.0005 | - | - | - | - | - | - | 0.9942 | |
| 40 | 19.4463 | - | 0.0006 | - | - | - | - | - | - | 0.9911 | |
| 50 | 25.7185 | - | 0.0004 | - | - | - | - | - | - | 0.9977 | |
| 60 | 31.9761 | - | 0.0002 | - | - | - | - | - | - | 0.9981 | |
| Elovich | 20 | - | - | - | 0.1648 | 0.4180 | - | - | - | - | 0.9873 |
| 30 | - | - | - | 0.1618 | 0.2386 | - | - | - | - | 0.9818 | |
| 40 | - | - | - | 0.4205 | 0.2246 | - | - | - | - | 0.9897 | |
| 50 | - | - | - | 0.3430 | 0.1524 | - | - | - | - | 0.9896 | |
| 60 | - | - | - | 0.3458 | 0.1170 | - | - | - | - | 0.9904 | |
| Avrami | 20 | 8.1175 | - | - | - | - | 0.1481 | 0.6793 | - | - | 0.9939 |
| 30 | 12.2177 | - | - | - | - | 0.0093 | 0.8753 | - | - | 0.9979 | |
| 40 | 16.3629 | - | - | - | - | 0.0117 | 0.9365 | - | - | 0.9875 | |
| 50 | 20.8372 | - | - | - | - | 0.0115 | 0.7751 | - | - | 0.9949 | |
| 60 | 25.3984 | - | - | - | - | 0.0088 | 0.9217 | - | - | 0.9972 | |
| Weber–Morris | 20 | - | - | - | - | - | - | - | 0.3622 | 0.8553 | 0.9284 |
| 30 | - | - | - | - | - | - | - | 0.5835 | 0.1741 | 0.9351 | |
| 40 | - | - | - | - | - | - | - | 0.6954 | 2.5525 | 0.9392 | |
| 50 | - | - | - | - | - | - | - | 0.9507 | 1.4244 | 0.9438 | |
| 60 | - | - | - | - | - | - | - | 1.1861 | 0.7804 | 0.9517 |
| Parameter | Freundlich | Temkin | Hill | Redlich–Peterson |
|---|---|---|---|---|
| Kfr | 0.6929 | - | - | - |
| nfr | 0.4709 | - | - | - |
| Kt | - | 0.3871 | - | - |
| bt | - | 75.0691 | - | - |
| qhi | - | - | 0.0020 | - |
| Khi | - | - | −0.9995 | - |
| nhi | - | - | −0.0002 | - |
| Krd1 | - | - | - | 0.3349 |
| Krd2 | - | - | - | −0.7713 |
| nrd | - | - | - | 0.1094 |
| R2 | 0.9978 | 0.9563 | 0.9907 | 0.9999 |
| Desorbtion Reagent, Concentration | qads * (mg/g) | qdes (mg/g) | Rdes (%) |
|---|---|---|---|
| Distilled H2O | 17.3235 | - | - |
| 0.1 M HCl | 17.3235 | 7.9913 | 46.9624 |
| 0.05 M HCl | 17.3235 | 9.7217 | 56.9913 |
| 0.1 M CH3COOH | 17.3235 | 1.5956 | 9.3539 |
| 0.1 M HNO3 | 17.3235 | 3.0103 | 17.6471 |
| 0.1 M NaOH | 17.3235 | Desorption in strongly basic environments causes irreversible damage to the polymer matrix and distortion of the granule structure due to swelling or rupture. | |
| 0.05 M NaOH | 17.3235 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niță, N.-T.; Suceveanu, E.-M.; Nedeff, F.M.; Tița, O.; Rusu, L. Biocomposite Material Based on Lactococcus lactis sp. Immobilized in Natural Polymer Matrix for Pharmaceutical Removal from Aqueous Media. Polymers 2024, 16, 1804. https://doi.org/10.3390/polym16131804
Niță N-T, Suceveanu E-M, Nedeff FM, Tița O, Rusu L. Biocomposite Material Based on Lactococcus lactis sp. Immobilized in Natural Polymer Matrix for Pharmaceutical Removal from Aqueous Media. Polymers. 2024; 16(13):1804. https://doi.org/10.3390/polym16131804
Chicago/Turabian StyleNiță, Narcis-Teodor, Elena-Mirela Suceveanu, Florin Marian Nedeff, Ovidiu Tița, and Lăcrămioara Rusu. 2024. "Biocomposite Material Based on Lactococcus lactis sp. Immobilized in Natural Polymer Matrix for Pharmaceutical Removal from Aqueous Media" Polymers 16, no. 13: 1804. https://doi.org/10.3390/polym16131804
APA StyleNiță, N.-T., Suceveanu, E.-M., Nedeff, F. M., Tița, O., & Rusu, L. (2024). Biocomposite Material Based on Lactococcus lactis sp. Immobilized in Natural Polymer Matrix for Pharmaceutical Removal from Aqueous Media. Polymers, 16(13), 1804. https://doi.org/10.3390/polym16131804









