Analysis of Fatigue Crack Nucleation in Double-Network Hydrogels
Abstract
1. Introduction
2. Formulation of the Configurational Stress Tensor
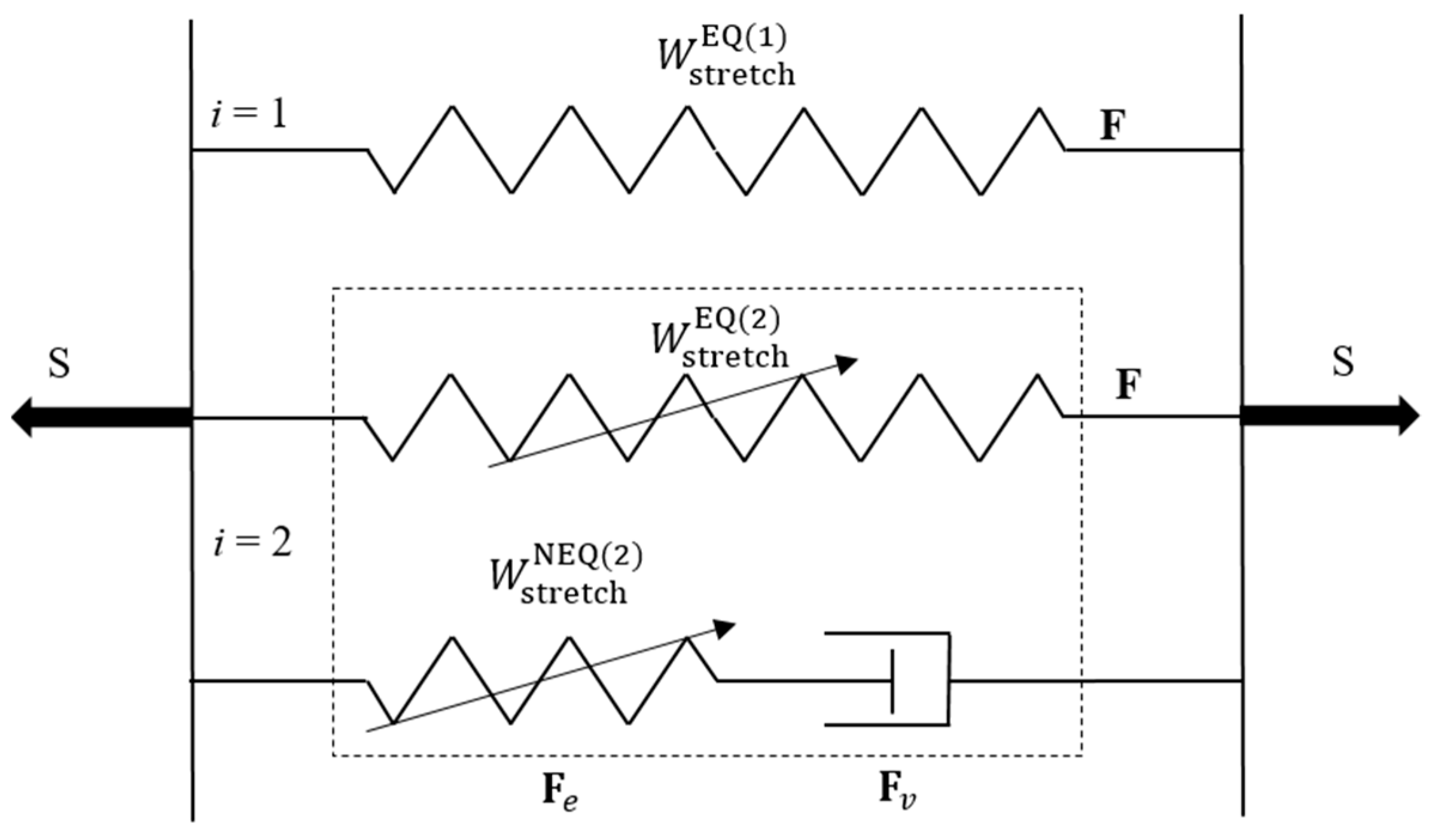
2.1. Constitutive Theory for DN Hydrogels
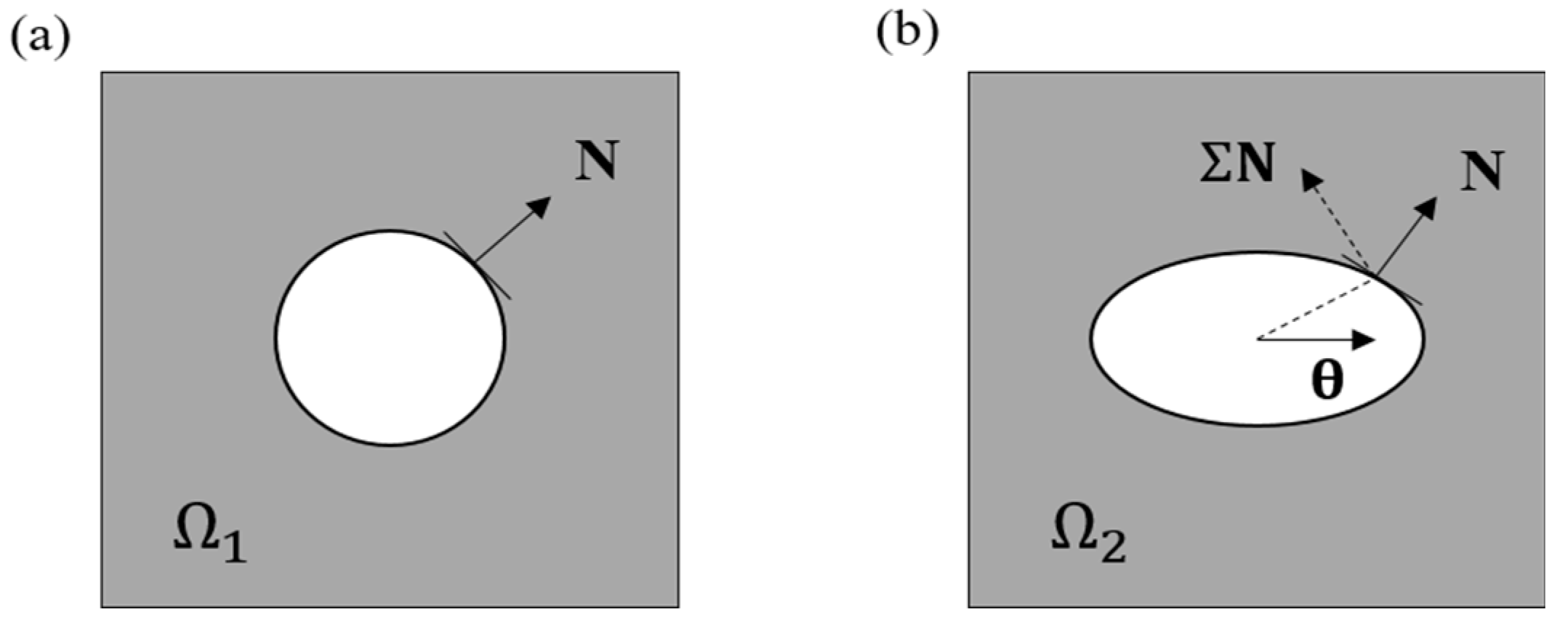
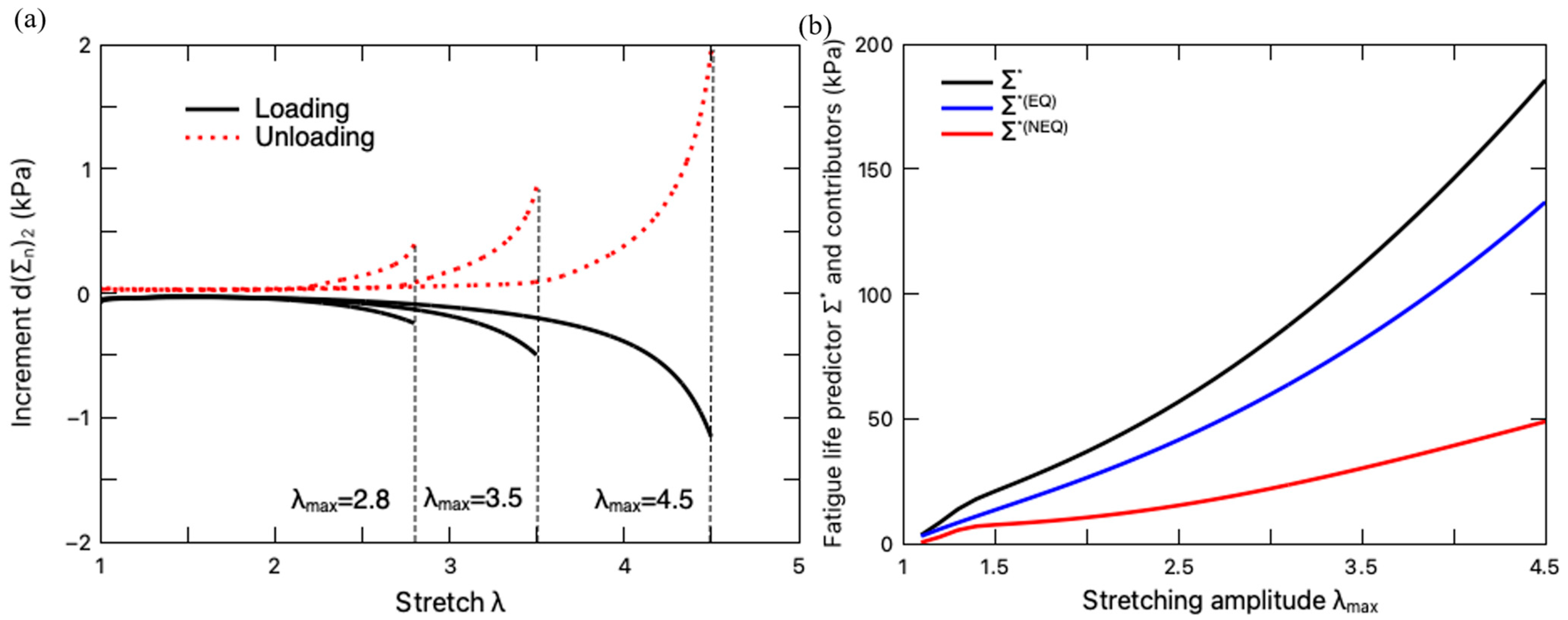
2.2. Fatigue Life Predictor for Crack Nucleation
3. Representative Case Study
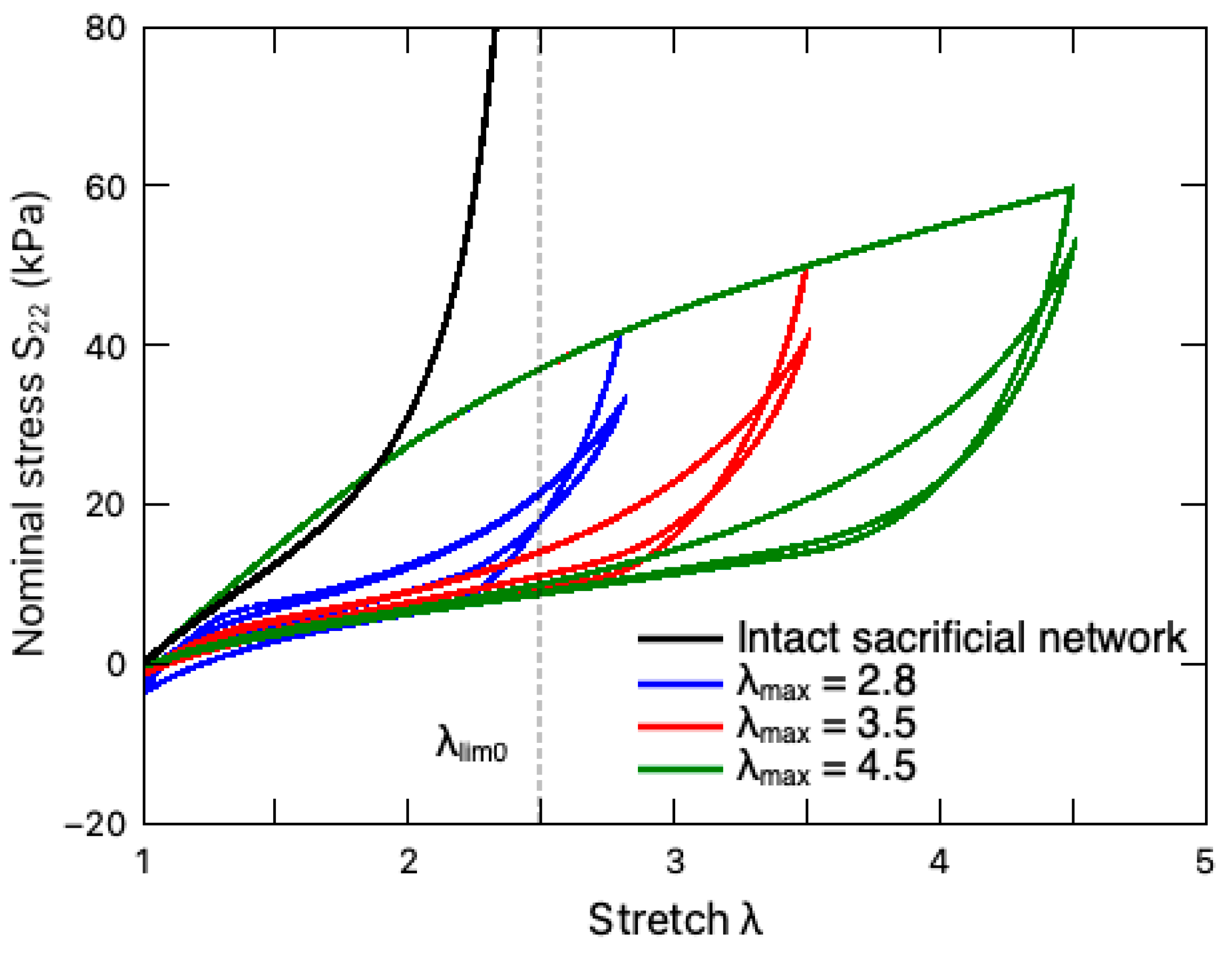
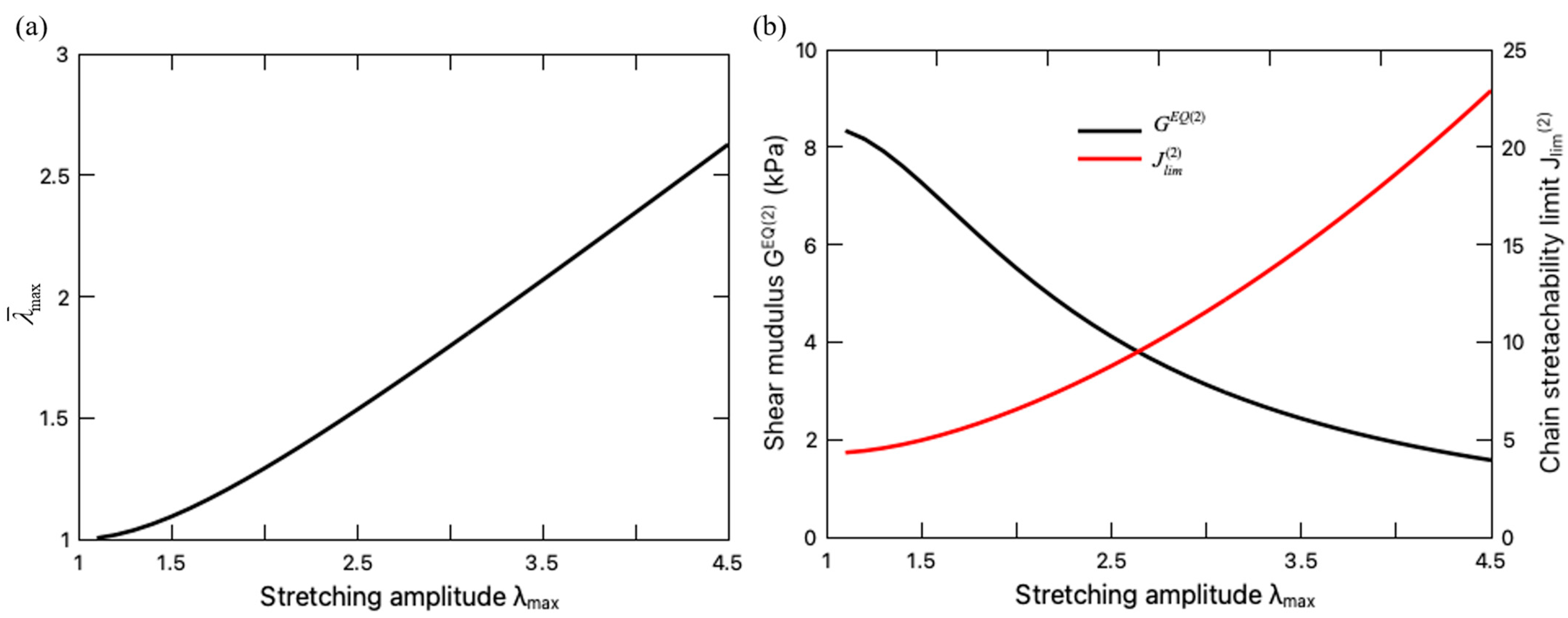
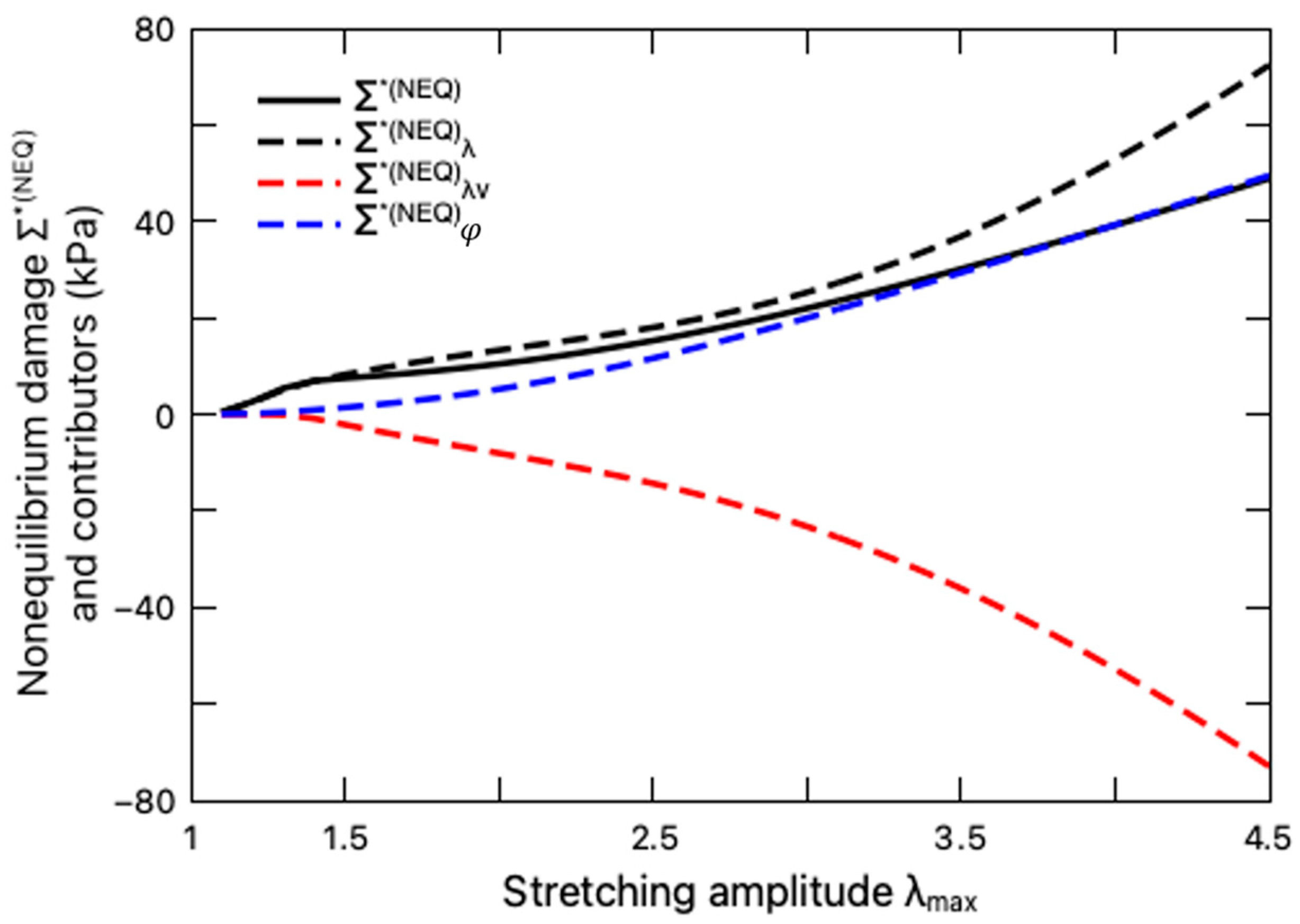
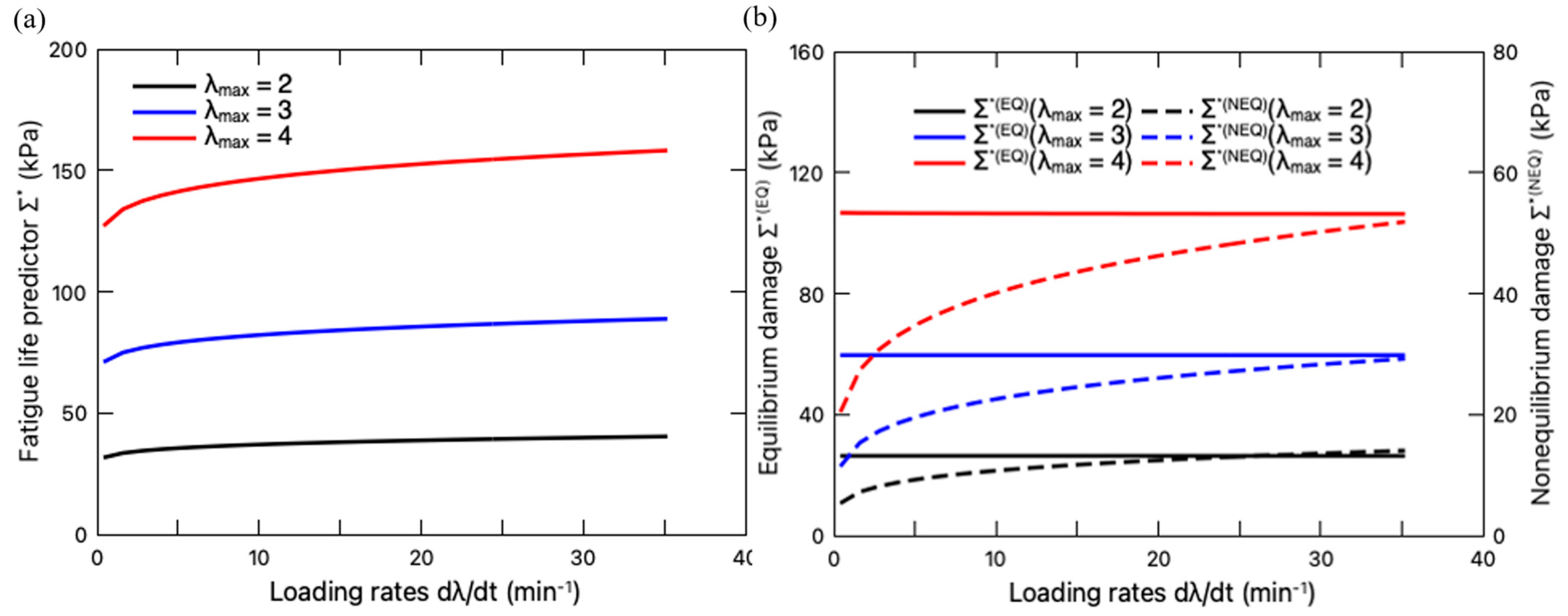
3.1. Microdamage and Viscoelastic Effects
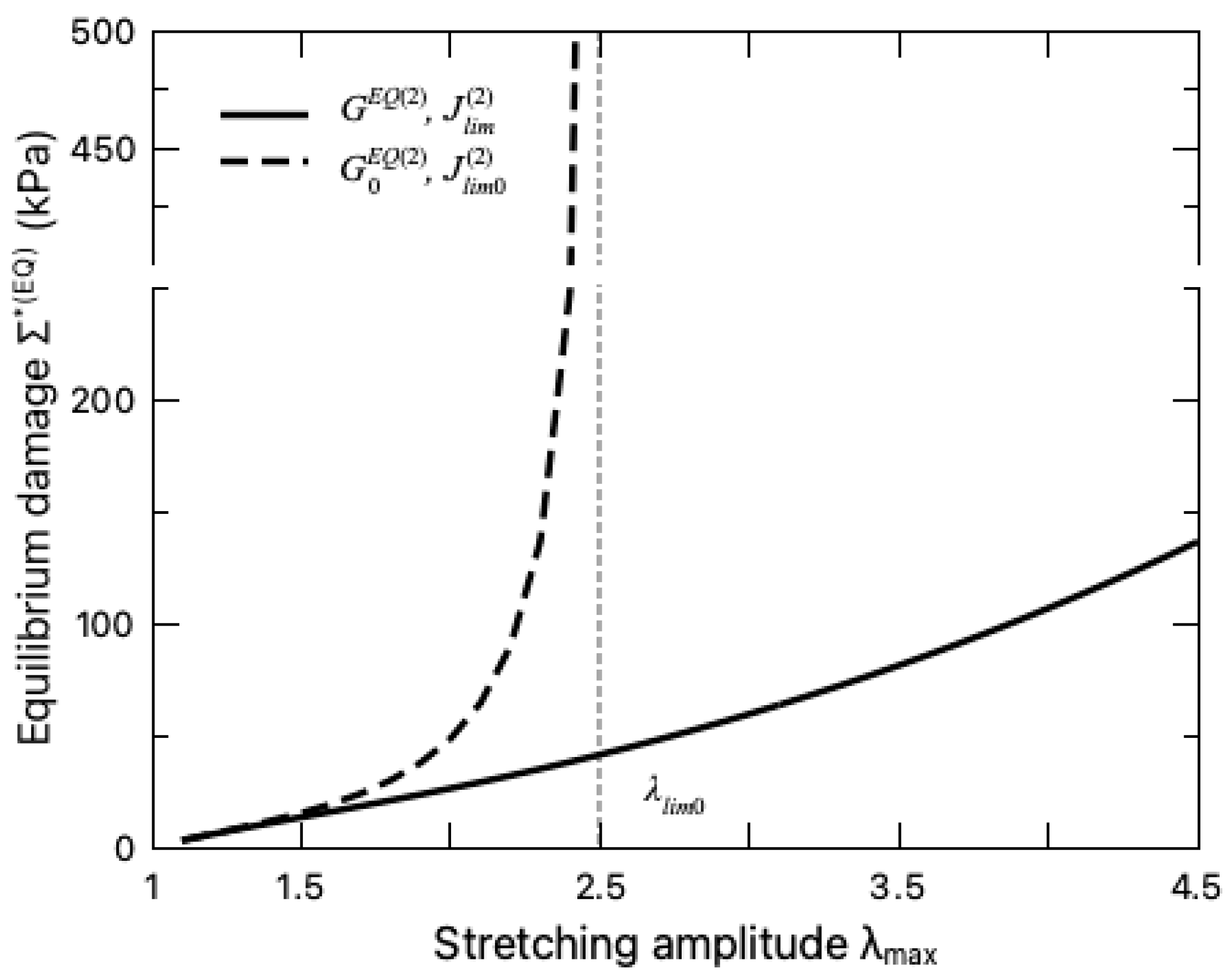
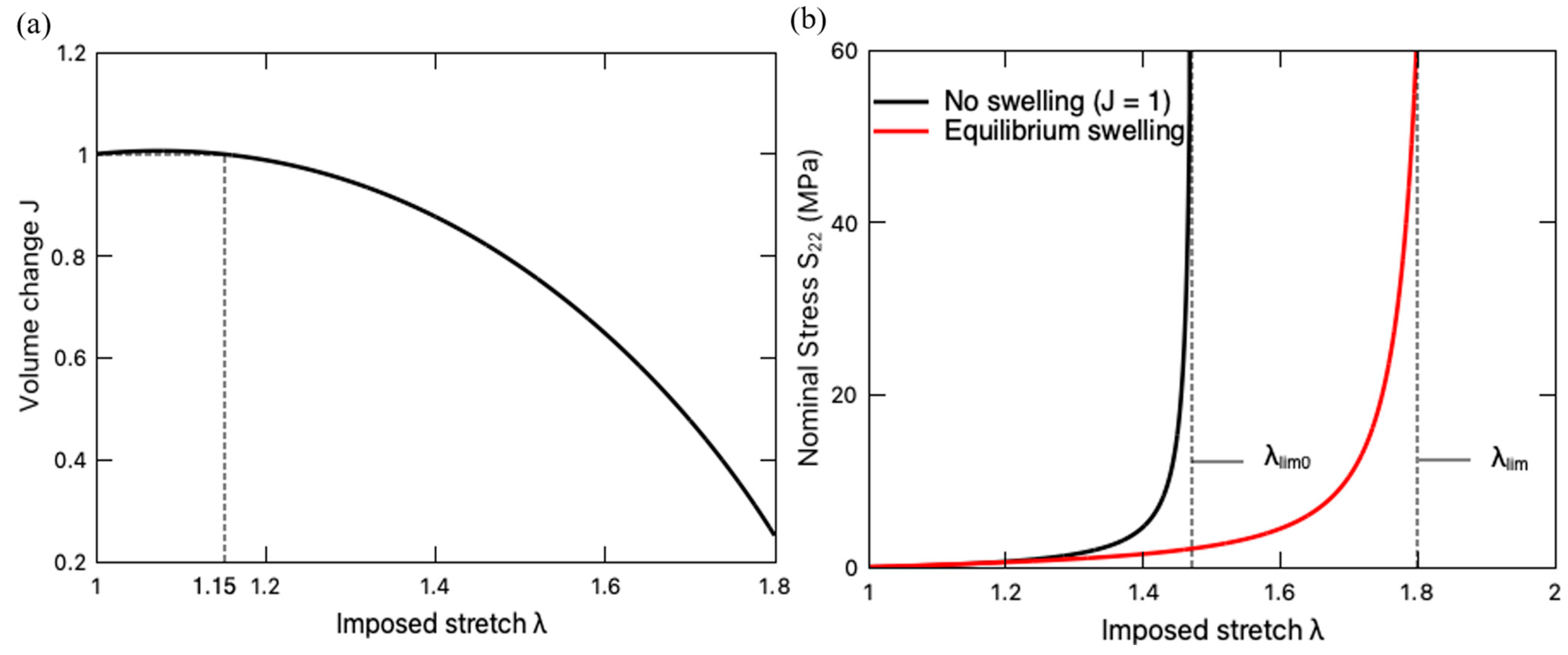
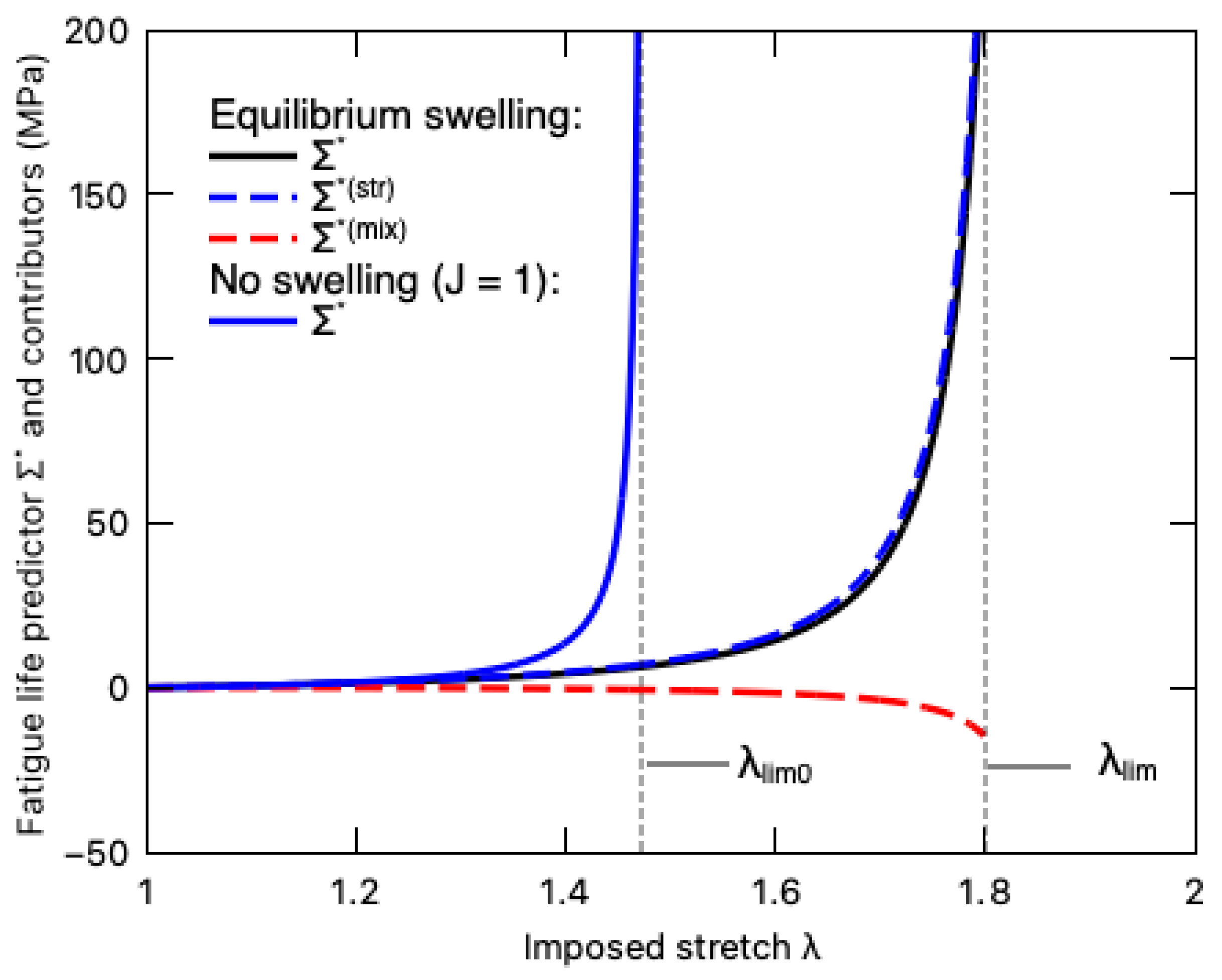
3.2. Ultimate Swelling Effect
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Acome, E.; Mitchell, S.K.; Morrissey, T.G.; Emmett, M.B.; Benjamin, C.; King, M.; Radakovitz, M.; Keplinger, C. Hydraulically amplified self-healing electrostatic actuators with muscle-like performance. Science 2018, 359, 61–65. [Google Scholar] [CrossRef] [PubMed]
- Sackett, S.D.; Tremmel, D.M.; Ma, F.; Feeney, A.K.; Maguire, R.M.; Brown, M.E.; Zhou, Y.; Li, X.; O’Brien, C.; Li, L.; et al. Extracellular matrix scaffold and hydrogel derived from decellularized and delipidized human pancreas. Sci. Rep. 2018, 8, 10452. [Google Scholar] [CrossRef] [PubMed]
- Song, Y.; Nagai, N.; Saijo, S.; Kaji, H.; Nishizawa, M.; Abe, T. In situ formation of injectable chitosan-gelatin hydrogels through double crosslinking for sustained intraocular drug delivery. Mater. Sci. Eng. C Mater. Biol. Appl. 2018, 88, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.; Song, W.J.; Sun, J.Y. Hydrogel soft robotics. Mater. Today Phys. 2020, 15, 100258. [Google Scholar] [CrossRef]
- Sun, J.Y.; Zhao, X.; Illeperuma, W.; Chaudhuri, O.; Oh, K.H.; Mooney, D.J.; Vlassak, J.J.; Suo, Z. Highly stretchable and tough hydrogels. Nature 2012, 489, 133–136. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.; Wang, Z.; Suo, Z. Flaw sensitivity of highly stretchable materials. Extrem. Mech. Lett. 2017, 10, 50–57. [Google Scholar] [CrossRef]
- Bai, R.; Yang, Q.; Tang, J.; Morelle, X.P.; Vlassak, J.; Suo, Z. Fatigue fracture of tough hydrogels. Extrem. Mech. Lett. 2017, 15, 91–96. [Google Scholar] [CrossRef]
- Bai, R.; Yang, J.; Morelle, X.P.; Yang, C.; Suo, Z. Fatigue fracture of self-recovery hydrogels. ACS Macro Lett. 2018, 7, 312–317. [Google Scholar] [CrossRef]
- Zhang, W.; Liu, X.; Wang, J.; Tang, J.; Hu, J.; Lu, T.; Suo, Z. Fatigue of double-network hydrogels. Eng. Fract. Mech. 2018, 187, 74–93. [Google Scholar] [CrossRef]
- Lin, S.; Liu, J.; Liu, X.; Zhao, X. Muscle-like fatigue-resistant hydrogels by mechanical training. Proc. Natl. Acad. Sci. USA 2019, 116, 10244–10249. [Google Scholar] [CrossRef]
- Lin, S.; Liu, X.; Liu, J.; Yuk, H.; Loh, H.-C.; Parada, G.A.; Settens, C.; Song, J.; Masic, A.; McKinley, G.H.; et al. Anti-fatigue-fracture hydrogels. Sci. Adv. 2019, 5, eaau8528. [Google Scholar] [CrossRef] [PubMed]
- Xiang, C.; Wang, Z.; Yang, C.; Yao, X.; Wang, Y.; Suo, Z. Stretchable and fatigue-resistant materials. Mater. Today 2020, 34, 7–16. [Google Scholar] [CrossRef]
- Lei, H.; Dong, L.; Li, Y.; Zhang, J.; Chen, H.; Wu, J.; Zhang, Y.; Fan, Q.; Xue, B.; Qin, M.; et al. Stretchable hydrogels with low hysteresis and anti-fatigue fracture based on polyprotein cross-linkers. Nat. Commun. 2020, 11, 4032. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.; Wu, J.; Zhou, Y.; Pan, Y.; Lu, T.; Song, X.; Hu, J. Fatigue behaviors of physical hydrogels based on hydrogen bonds. Extrem. Mech. Lett. 2021, 46, 101320. [Google Scholar] [CrossRef]
- Hua, M.; Wu, S.; Ma, Y.; Zhao, Y.; Chen, Z.; Frenkel, I.; Strzalka, J.; Zhou, H.; Zhu, X.; He, X. Strong tough hydrogels via the synergy of freeze-casting and salting out. Nature 2021, 590, 594–599. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Hu, J.; Tang, J.; Wang, Z.; Wang, J.; Lu, T.; Suo, Z. Fracture toughness and fatigue threshold of tough hydrogels. ACS Macro Lett. 2019, 8, 17–23. [Google Scholar] [CrossRef] [PubMed]
- Mao, Y.; Lin, S.; Zhao, X.; Anand, L. A large deformation viscoelastic model for double-network hydrogels. J. Mech. Phys. Solids 2017, 100, 103–130. [Google Scholar] [CrossRef]
- Lu, T.; Wang, J.; Yang, R.; Wang, T.J. A constitutive model for soft materials incorporating viscoelasticity and Mullins effect. J. Appl. Mech. 2017, 84, 021010. [Google Scholar] [CrossRef]
- Nakajima, T.; Kurokawa, T.; Ahmed, S.; Wu, W.L.; Gong, J.P. Characterization of internal fracture process of double network hydrogels under uniaxial elongation. Soft Matter 2013, 9, 1955–1966. [Google Scholar] [CrossRef]
- Mai, T.T.; Matsuda, T.; Nakajima, T.; Gong, J.P.; Urayama, K. Damage cross-effect and anisotropy in tough double network hydrogels revealed by biaxial stretching. Soft Matter 2019, 15, 3719–3732. [Google Scholar] [CrossRef]
- Imaoka, C.; Nakajima, T.; Indei, T.; Iwata, M.; Hong, W.; Marcellan, A.; Gong, J.P. Inverse mechanical-swelling coupling of a highly deformed double-network gel. Sci. Adv. 2023, 9, eabp8351. [Google Scholar] [CrossRef]
- Wang, X.; Hong, W. Pseudo-elasticity of a double network gel. Soft Matter 2011, 7, 8576–8581. [Google Scholar] [CrossRef]
- Gent, A.N. A new constitutive relation for rubber. Rubber Chem. Technol. 1996, 69, 59–61. [Google Scholar] [CrossRef]
- Zhao, X. A theory for large deformation and damage of interpenetrating polymer networks. J. Mech. Phys. Solids 2012, 60, 319–332. [Google Scholar] [CrossRef]
- Marckmann, G.; Verron, E.; Gornet, L.; Chagnon, G.; Charrier, P.; Fort, P. A theory of network alteration for the Mullins effect. J. Mech. Phys. Solids 2002, 50, 2011–2028. [Google Scholar] [CrossRef]
- Chagnon, G.; Verron, E.; Marckmann, G. Development of new constitutive equations for the Mullins effect in rubber using the network alteration theory. Int. J. Solids Struct. 2006, 43, 6817–6831. [Google Scholar] [CrossRef]
- Boyce, M.C.; Arruda, E.M. Swelling and mechanical stretching of elastomeric materials. Math. Mech. Solids 2001, 6, 641–659. [Google Scholar] [CrossRef]
- Ogden, R.; Roxburgh, D. A pseudo–elastic model for the Mullins effect in filled rubber. Proc. R. Soc. Lond. A 1999, 455, 2861–2877. [Google Scholar] [CrossRef]
- Lin, J.; Zheng, S.Y.; Xiao, R.; Yin, J.; Wu, Z.L.; Zheng, Q.; Qian, J. Constitutive behaviors of tough physical hydrogels with dynamic metal-coordinated bonds. J. Mech. Phys. Solids 2020, 139, 103935. [Google Scholar] [CrossRef]
- Morovati, V.; Dargazany, R. Modelling damage accumulation during cyclic loading in elastomeric gels with interpenetrating networks. In Proceedings of the ASME 2019 International Mechanical Engineering Congress and Exposition. Volume 9: Mechanics of Solids, Structures, and Fluids, Salt Lake City, UT, USA, 11–14 November 2019. [Google Scholar] [CrossRef]
- Morovati, V.; Bahrololoumi, A.; Dargazany, R. Fatigue-induced stress-softening in cross-linked multi-network elastomers: Effect of damage accumulation. Int. J. Plast. 2021, 142, 102993. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, H.; Zheng, Y. A micromechanically based constitutive model for the inelastic and swelling behaviors in double network hydrogels. J. Appl. Mech. 2016, 83, 021008. [Google Scholar] [CrossRef]
- Mars, W.V.; Fatemi, A. A Literature Survey on Fatigue Analysis Approaches for Rubber. Int. J. Fatigue 2002, 24, 949–961. [Google Scholar] [CrossRef]
- Verron, E.; Andriyana, A. Definition of a new predictor for multiaxial fatigue crack nucleation in rubber. J. Mech. Phys. Solids 2008, 56, 417–443. [Google Scholar] [CrossRef]
- Yu, Q.M.; Tanaka, Y.; Furukawa, H.; Kurokawa, T.; Gong, J.P. Direct observation of damage zone around crack tips in double-network gels. Macromolecules 2009, 42, 3852–3855. [Google Scholar] [CrossRef]
- Bai, R.; Chen, B.; Yang, J.; Suo, Z. Tearing a hydrogel of complex rheology. J. Mech. Phys. Solids 2019, 125, 749–761. [Google Scholar] [CrossRef]
- Bai, R.; Yang, J.; Suo, Z. Fatigue of hydrogels. Eur. J. Mech. A Solids 2019, 74, 337–370. [Google Scholar] [CrossRef]
- Jia, Y.; Zhou, Z.; Jiang, H.; Liu, Z. Characterization of fracture toughness and damage zone of double network hydrogels. J. Mech. Phys. Solids 2022, 169, 105090. [Google Scholar] [CrossRef]
- Dekker, R.; van der Meer, F.; Maljaars, J.; Sluys, L.J. A cohesive XFEM model for simulating fatigue crack growth under mixed-mode loading and overloading. Int. J. Numer. Methods Eng. 2019, 118, 561–577. [Google Scholar] [CrossRef]
- Pandey, V.B.; Samant, S.S.; Singh, I.V.; Mishra, B.K. An improved methodology based on continuum damage mechanics and stress triaxiality to capture the constraint effect during fatigue crack propagation. Int. J. Fatigue 2020, 140, 105823. [Google Scholar] [CrossRef]
- Liu, D.; Ma, S.; Yuan, H.; Markert, B. Computational modelling of poro-visco-hyperelastic effects on time-dependent fatigue crack growth of hydrogels. Int. J. Plast. 2022, 155, 103307. [Google Scholar] [CrossRef]
- Zhou, J.; Jiang, L. Development of a predictor for fatigue crack nucleation of dielectric viscoelastomers under electromechanical loads. J. Mech. Phys. Solids 2018, 119, 400–416. [Google Scholar] [CrossRef]
- Gao, S.; Feng, H.; Jiang, L. A predictor for fatigue crack nucleation of single-network hydrogels considering water diffusion effect. Int. J. Fract. 2023, 246, 245–264. [Google Scholar] [CrossRef]
- Maugin, G.A. Non-equilibrium thermodynamics of electromagnetic solids. In Non-Equilibrium Thermodynamics with Application to Solids: Dedicated to the Memory of Professor Theodor Lehmann; Springer: Vienna, Austria, 1993; pp. 205–258. [Google Scholar]
- Maugin, G.A. The Thermomechanics of Nonlinear Irreversible Behaviours; World Scientific: Singapore, 1999. [Google Scholar]
- Nguyen, T.D.; Govindjee, S.; Klein, P.A.; Gao, H. A material force method for inelastic fracture mechanics. J. Mech. Phys. Solids 2005, 53, 91–121. [Google Scholar] [CrossRef]
- Gross, D.; Kolling, S.; Mueller, R.; Schmidt, I. Configurational forces and their application in solid mechanics. Eur. J. Mech. A/Solids 2003, 22, 669–692. [Google Scholar] [CrossRef]
- Duda, F.P.; Souza, A.C.; Fried, E. A theory for species migration in a finitely strained solid with application to polymer network swelling. J. Mech. Phys. Solids 2010, 58, 515–529. [Google Scholar] [CrossRef]
- Chen, S.; Ravi-Chandar, K. Nonlinear poroviscoelastic behavior of gelatin-based hydrogel. J. Mech. Phys. Solids 2022, 158, 104650. [Google Scholar] [CrossRef]
- Flory, P.J. Thermodynamics of high polymer solutions. J. Chem. Phys. 1942, 10, 51–61. [Google Scholar] [CrossRef]
- Flory, P.J.; Rehner, J. Statistical mechanics of cross-linked polymer networks II. Swelling. J. Chem. Phys. 1943, 11, 521–526. [Google Scholar] [CrossRef]
- Flory, P.J. Principles of Polymer Chemistry; Cornell University Press: Ithaca, NY, USA, 1953. [Google Scholar]
- Hong, W.; Zhao, X.; Zhou, J.; Suo, Z. A theory of coupled diffusion and large deformation in polymeric gels. J. Mech. Phys. Solids 2008, 56, 1779–1793. [Google Scholar] [CrossRef]
- Duda, F.P.; Souza, A.C.; Fried, E. Fluid flow and interface motion in gels: A finite-strain theory and its application to a channel flow problem. J. Mech. Phys. Solids 2021, 155, 104566. [Google Scholar] [CrossRef]
- Coleman, B.D.; Noll, W. The thermodynamics of elastic materials with heat conduction and viscosity. Arch. Ration. Mech. Anal. 1963, 13, 167–178. [Google Scholar] [CrossRef]
- Chester, S.A. A constitutive model for coupled fluid permeation and large viscoelastic deformation in polymeric gels. Soft Matter 2012, 8, 8223–8233. [Google Scholar] [CrossRef]
- Gurtin, M.E.; Fried, E.; Anand, L. The Mechanics and Thermodynamics of Continua; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Gent, A.N.; Lindley, P.B.; Thomas, A.G. Cut growth and fatigue of rubbers. I. The relationship between cut growth and fatigue. J. Appl. Polym. Sci. 1964, 8, 455–466. [Google Scholar] [CrossRef]
- Eshelby, J.D. The force on an elastic singularity. Philos. Trans. R. Soc. A 1951, 244, 87–112. [Google Scholar] [CrossRef]
- Eshelby, J.D. The elastic energy-momentum tensor. J. Elast. 1975, 5, 321–335. [Google Scholar] [CrossRef]
- Maugin, G.A. Material mechanics of materials. Theor. Appl. Mech. 2002, 27, 1–12. [Google Scholar] [CrossRef]
- Zhou, J.; Jiang, L. Electromechanical fatigue of dielectric viscoelastomers. Extrem. Mech. Lett. 2020, 36, 100666. [Google Scholar] [CrossRef]
- Wang, X.; Hong, W. Delayed fracture in gels. Soft Matter 2012, 8, 8171–8178. [Google Scholar] [CrossRef]
- Anand, L.; On, H. Hencky’s approximate strain-energy function for moderate deformations. ASME J. Appl. Mech. 1979, 46, 78–82. [Google Scholar] [CrossRef]
- Anand, L. Moderate deformations in extension-torsion of incompressible isotropic elastic materials. J. Mech. Phys. Solids 1986, 34, 293–304. [Google Scholar] [CrossRef]
- Lin, S.; Zhou, Y.; Zhao, X. Designing extremely resilient and tough hydrogels via delayed dissipation. Extrem. Mech. Lett. 2014, 1, 70–75. [Google Scholar] [CrossRef]
- Nakajima, T.; Chida, T.; Mito, K.; Kurokawa, T.; Gong, J.P. Double-network gels as polyelectrolyte gels with salt-insensitive swelling properties. Soft Matter 2020, 16, 5487–5496. [Google Scholar] [CrossRef] [PubMed]
- Marcombe, R.; Cai, S.; Hong, W.; Zhao, X.; Lapusta, Y.; Suo, Z. A theory of constrained swelling of a pH-sensitive hydrogel. Soft Matter 2010, 6, 784–793. [Google Scholar] [CrossRef]
- Zheng, S.; Liu, Z. Constitutive model of salt concentration-sensitive hydrogel. Mech. Mater. 2019, 136, 103092. [Google Scholar] [CrossRef]
- Okumura, D.; Chester, S.A. Ultimate swelling described by limiting chain extensibility of swollen elastomers. Int. J. Mech. Sci. 2018, 144, 531–539. [Google Scholar] [CrossRef]
- Okumura, D.; Kawabata, H.; Chester, S.A. A general expression for linearized properties of swollen elastomers undergoing large deformations. J. Mech. Phys. Solids 2020, 135, 103805. [Google Scholar] [CrossRef]



| i | Parameter | Value |
|---|---|---|
| 1 | 2.03 kPa | |
| 330 | ||
| 2 | 8.4 kPa | |
| 4.3 | ||
| a | 0.196 | |
| b | 2 | |
| 0.2 kPa | ||
| Hg | 70.48 kPa | |
| r | 1.3 | |
| 1 | ||
| m | 0.22 | |
| s0 | 0.2 kPa | |
| Hs | 25.88 kPa | |
| q | 1.34 | |
| A | 3 |
| Parameter | Value |
|---|---|
| 2.2 | |
| 45 kPa | |
| −1.8 kPa | |
| 14.1 | |
| 0.03 nm3 | |
| 0.2 nm3 | |
| 0.015 | |
| 0.3 | |
| 0.45 | |
| 0.0152 | |
| 0.0723 | |
| k | 1.370649 × 10−23 J/K |
| T | 293 K |
| 0.237 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, S.; Jiang, L. Analysis of Fatigue Crack Nucleation in Double-Network Hydrogels. Polymers 2024, 16, 1700. https://doi.org/10.3390/polym16121700
Gao S, Jiang L. Analysis of Fatigue Crack Nucleation in Double-Network Hydrogels. Polymers. 2024; 16(12):1700. https://doi.org/10.3390/polym16121700
Chicago/Turabian StyleGao, Shan, and Liying Jiang. 2024. "Analysis of Fatigue Crack Nucleation in Double-Network Hydrogels" Polymers 16, no. 12: 1700. https://doi.org/10.3390/polym16121700
APA StyleGao, S., & Jiang, L. (2024). Analysis of Fatigue Crack Nucleation in Double-Network Hydrogels. Polymers, 16(12), 1700. https://doi.org/10.3390/polym16121700





