Damage Resistance of Kevlar® Fabric, UHMWPE, PVB Multilayers Subjected to Concentrated Drop-Weight Impact
Abstract
1. Introduction
2. Materials and Methods
2.1. Components and Chemicals
2.2. Fabrication of Multilayers Structures
2.3. Tensile Measurements
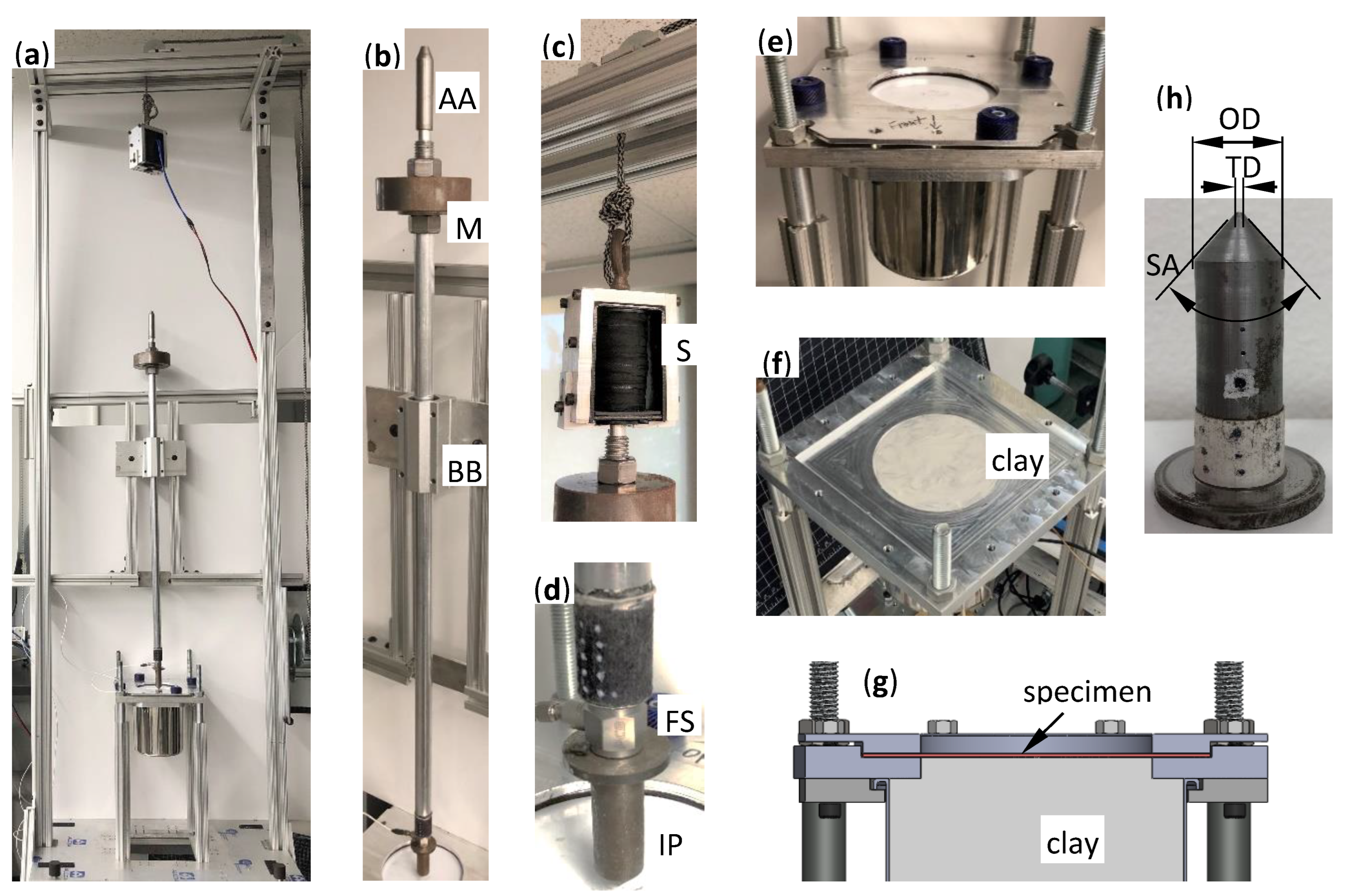
2.4. Drop-Weight Impact Device
3. Results
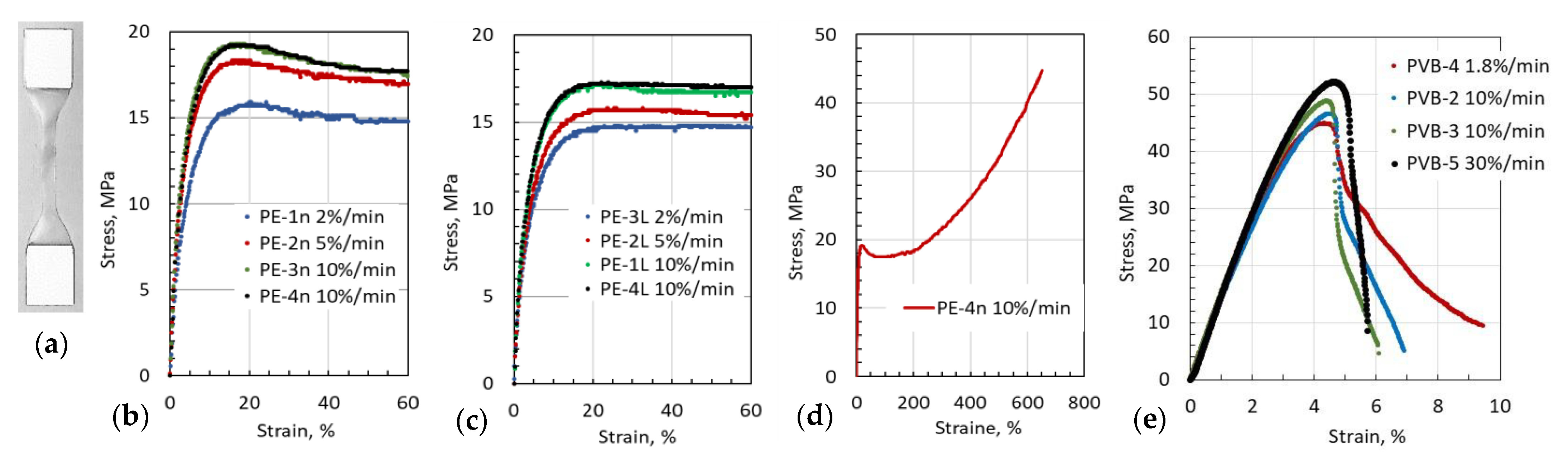
3.1. Unidirectional Tensile Measurements
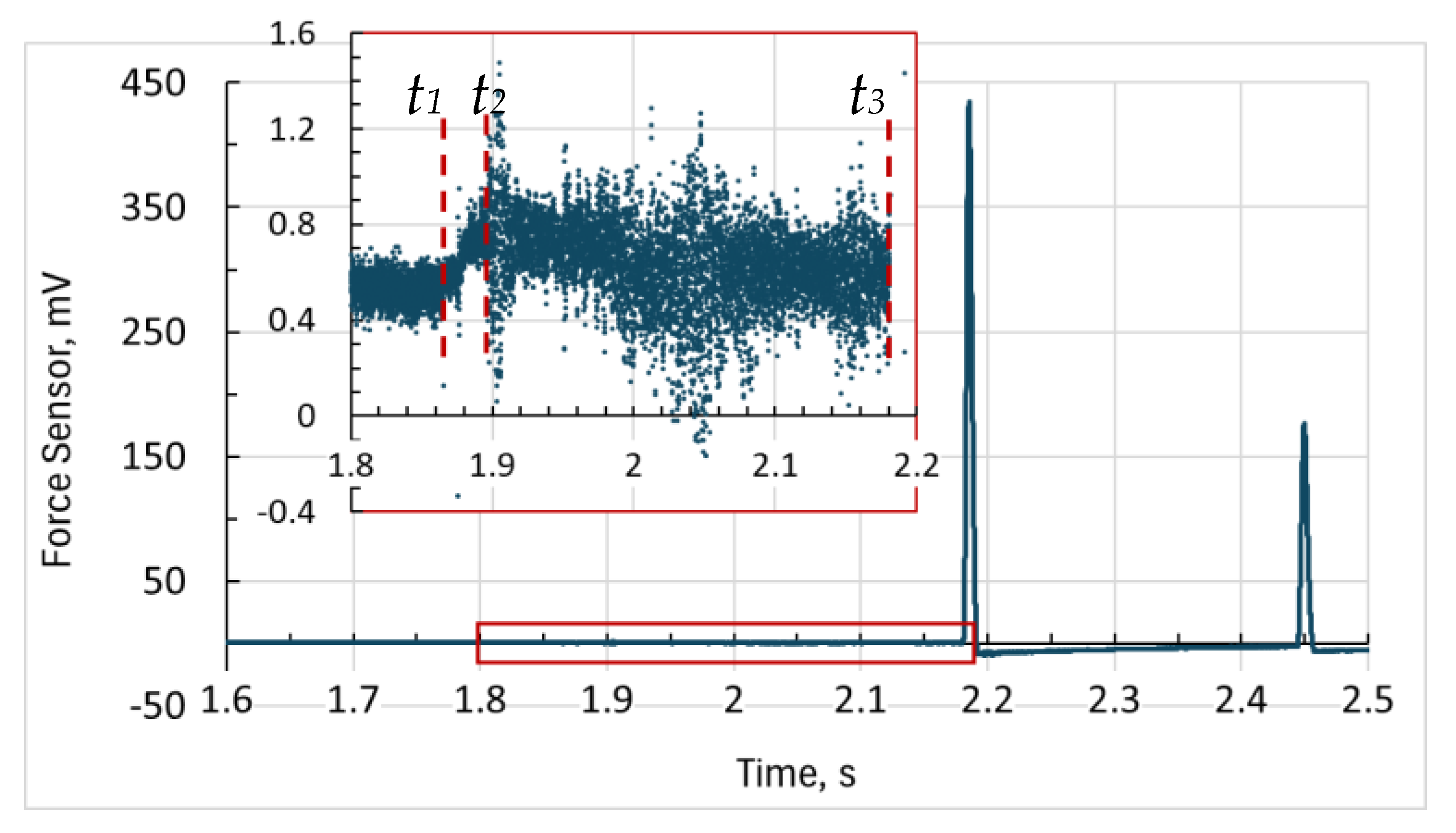
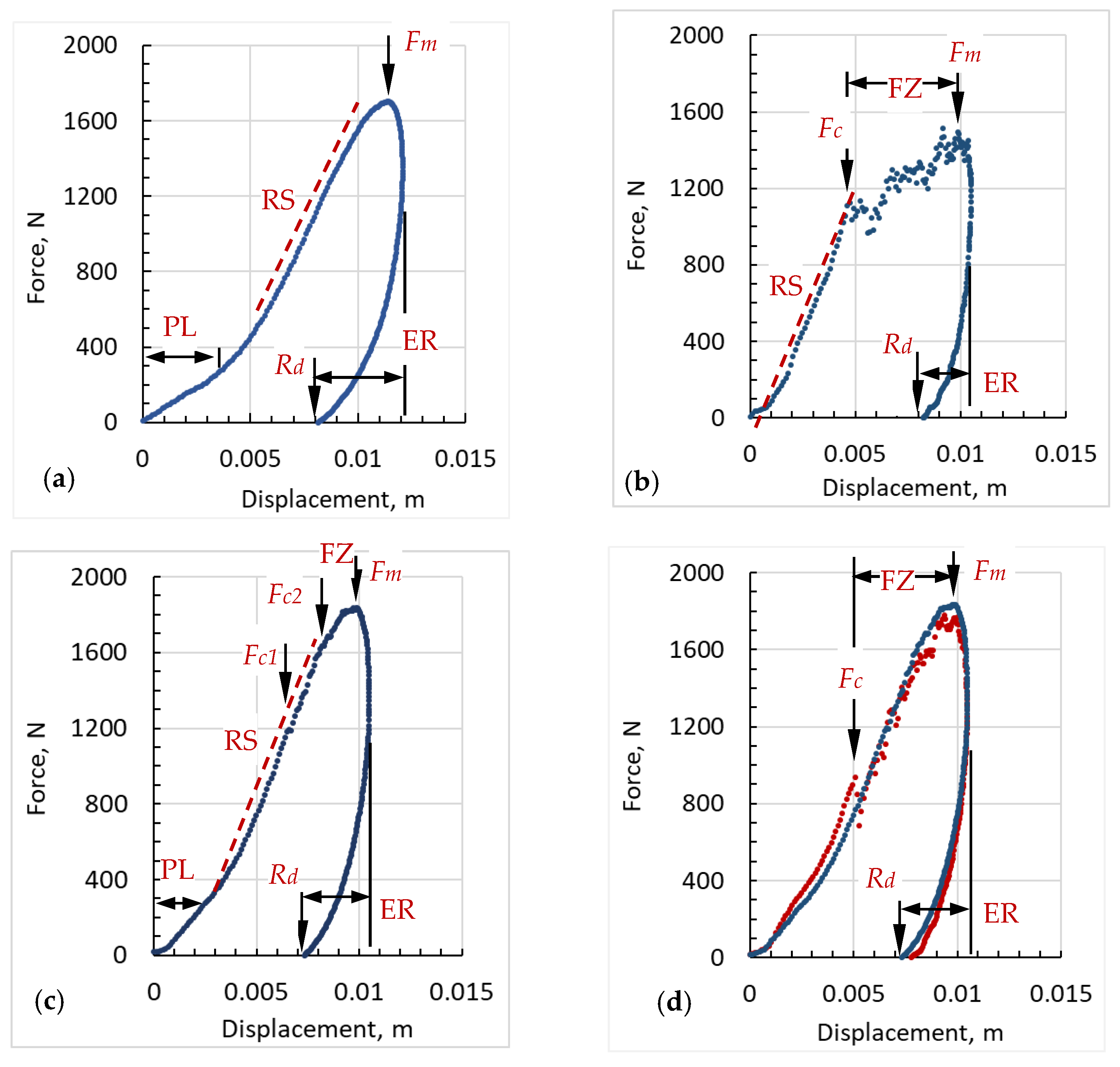
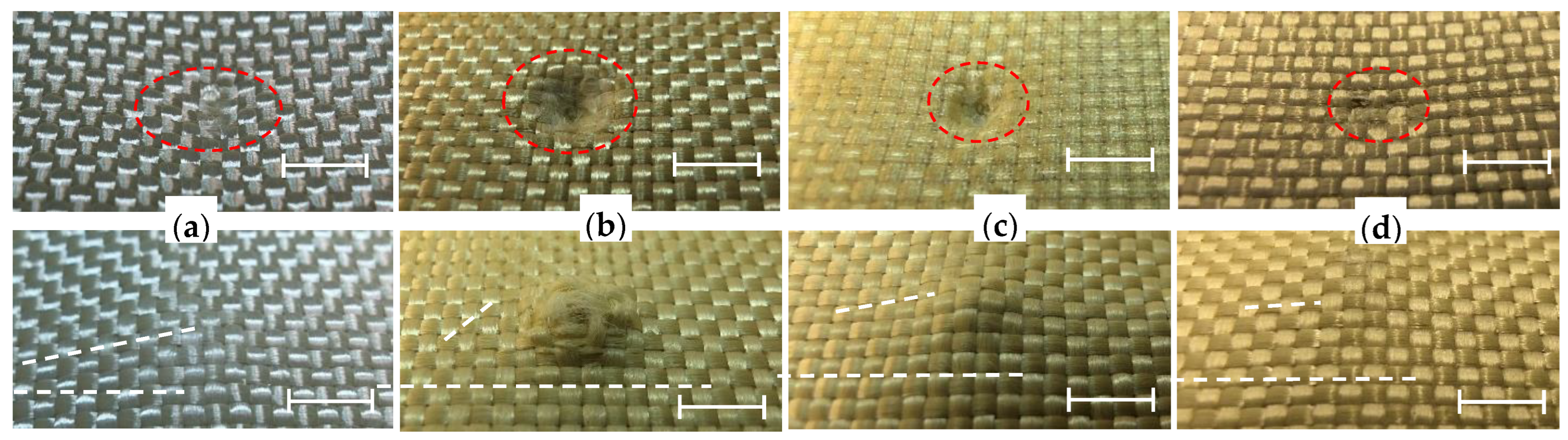
3.2. Drop-Weight Impact Test
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ranaweera, P.; Weerasinghe, D.; Fernando, P.L.N.; Raman, S.N.; Mohotti, D. Ballistic Performance of Multi-Metal Systems. Int. J. Prot. Struct. 2020, 11, 379–410. [Google Scholar] [CrossRef]
- Fras, T.; Roth, C.C.; Mohr, D. Fracture of High-Strength Armor Steel under Impact Loading. Int. J. Impact Eng. 2018, 111, 147–164. [Google Scholar] [CrossRef]
- Yu, Z.; Yang, B.; Chen, X.; Zhang, C.; Guo, F.; Wang, Q.; Cao, W.; Huang, C. Mechanical Anisotropy of Ultra Strong-and-Ductile Lamellar Dual-Phase Steel. J. Mater. Res. Technol. 2024, 28, 3025–3036. [Google Scholar]
- Shaikeea, A.J.D.; Cui, H.; O’masta, M.; Zheng, X.R.; Deshpande, V.S. The toughness of mechanical metamaterials. Nat. Mater. 2022, 21, 297–304. [Google Scholar] [CrossRef] [PubMed]
- SPatel, K.; Swain, B.K.; Behera, A.; Mohapatra, S.S. Metallic Glasses: A Revolution in Material Science. In Metallic Glasses, Minić, Dragica, Vasić, Milica; Intechopen: Rijeka, Croatia, 2020. [Google Scholar] [CrossRef]
- NAndraskar, N.D.; Tiwari, G.; Goel, M.D. Impact response of ceramic structures—A review. Ceram. Int. 2022, 48, 27262–27279. [Google Scholar] [CrossRef]
- Zhong, F.; Schwabe, J.; Hofmann, D.; Meier, J.; Thomann, R.; Enders, M.; Mülhaupt, R. All-polyethylene composites reinforced via extended-chain UHMWPE nanostructure formation during melt processing. Polymer 2018, 140, 107–116. [Google Scholar] [CrossRef]
- Benzait, Z.; Trabzon, L. A review of recent research on materials used in polymer-matrix composites for body armor application. J. Compos. Mater. 2018, 52, 3241–3263. [Google Scholar] [CrossRef]
- Pundhir, N.; Pathak, H.; Zafar, S. Ballistic Impact Performance of Ultra-High Molecular Weight Polyethylene (Uhmwpe) Composite Armour. Sādhanā 2021, 46, 194. [Google Scholar] [CrossRef]
- Liang, Y.; Chen, X.; Soutis, C. Review on Manufacture of Military Composite Helmet. Appl. Compos. Mater. 2021, 29, 305–323. [Google Scholar] [CrossRef]
- Ballistic Resistance of Body Armor. Nij Standard-0101.06. 2008. Available online: https://nij.ojp.gov/library/publications/ballistic-resistance-body-armor-nij-standard-010106 (accessed on 18 April 2024).
- Kevlar® Technical Guide. DuPont. Available online: https://www.dupont.com/content/dam/dupont/amer/us/en/safety/public/documents/en/Kevlar_Technical_Guide_0319.pdf (accessed on 19 February 2024).
- Naik, S.; Dandagwhal, R.; Loharkar, P.K. A review on various aspects of Kevlar composites used in ballistic applications. Mater. Today: Proc. 2020, 21, 1366–1374. [Google Scholar] [CrossRef]
- Carlsson, D.J.; Gan, L.H.; Wiles, D.M. Photodegradation of Aramids. II. Irradiation in Air. J. Polym. Sci. Polym. Chem. Ed. 1978, 16, 2365–2376. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, J.; Chen, J.; Hao, X.; Wang, S.; Feng, X.; Guo, Y. Effects of solar UV irradiation on the tensile properties and structure of PPTA fiber. Polym. Degrad. Stab. 2006, 91, 2761–2767. [Google Scholar] [CrossRef]
- Wei, C.; Qian, X.; He, X.; Liu, J. Enhanced Ultraviolet Resistance of Kevlar Fibers with Tio 2 Films. In Proceedings of the 2011 9th International Conference on Reliability, Maintainability and Safety, Guiyang, China, 12–15 June 2011. [Google Scholar]
- Zhang, Z.; Lee, N.; Patel, K.; Young, M.; Zhang, J.; Percec, S.; Ren, S. Poly(P-Phenylene Terephthalamide) Fibers Reinforced with Ultrathin Ceramic Coatings. Adv. Eng. Mater. 2018, 20, 1800095. [Google Scholar] [CrossRef]
- Biradar, A.; Arulvel, S.; Kandasamy, J. Significance of ballistic parameters and nanohybridization in the development of textile-based body armor: A review. Int. J. Impact Eng. 2023, 180, 104700. [Google Scholar] [CrossRef]
- Wu, S.; Sikdar, P.; Bhat, G.S. Recent progress in developing ballistic and anti-impact materials: Nanotechnology and main approaches. Def. Technol. 2023, 21, 33–61. [Google Scholar] [CrossRef]
- Barhoumi, H.; Bhouri, N.; Feki, I.; Baffoun, A.; Hamdaoui, M.; Ben Abdessalem, S. Review of ballistic protection materials: Properties and performances. J. Reinf. Plast. Compos. 2022, 42, 685–699. [Google Scholar] [CrossRef]
- Abtew, M.A.; Boussu, F.; Bruniaux, P.; Loghin, C.; Cristian, I. Ballistic impact mechanisms—A review on textiles and fibre-reinforced composites impact responses. Compos. Struct. 2019, 223, 110966. [Google Scholar] [CrossRef]
- Hooper, P.A.; Blackman, B.R.K.; Dear, J.P. The mechanical behaviour of poly(vinyl butyral) at different strain magnitudes and strain rates. J. Mater. Sci. 2011, 47, 3564–3576. [Google Scholar] [CrossRef]
- Zhang, X.; Hao, H.; Shi, Y.; Cui, J. The mechanical properties of Polyvinyl Butyral (PVB) at high strain rates. Constr. Build. Mater. 2015, 93, 404–415. [Google Scholar] [CrossRef]
- Samolov, A.D.; Simić, D.M.; Fidanovski, B.Z.; Obradović, V.M.; Tomić, L.D.; Knežević, D.M. Improvement of VIS and IR camouflage properties by impregnating cotton fabric with PVB/IF-WS2. Def. Technol. 2020, 17, 2050–2056. [Google Scholar] [CrossRef]
- Salman, S.D.; Leman, Z.; Sultan, M.T.; Ishak, M.R.; Cardona, F. Ballistic Impact Resistance of Plain Woven Kenaf/Aramid Reinforced Polyvinyl Butyral Laminated Hybrid Composite. BioResources 2016, 11, 7282–7295. [Google Scholar] [CrossRef]
- Salman, S.D.; Leman, Z.; Ishak, M.R.; Sultan MT, H.; Cardona, F. Quasi-Static Penetration Behavior of Plain Woven Kenaf/Aramid Reinforced Polyvinyl Butyral Hybrid Laminates. J. Ind. Text. 2018, 47, 1427–1446. [Google Scholar] [CrossRef]
- Lee, B.L.; Walsh, T.F.; Won, S.T.; Patts, H.M.; Song, J.W.; Mayer, A.H. Penetration Failure Mechanisms of Armor-Grade Fiber Composites under Impact. J. Compos. Mater. 2001, 35, 1605–1633. [Google Scholar] [CrossRef]
- Khan, M.I.; Umair, M.; Hussain, R.; Karahan, M.; Nawab, Y. Investigation of impact properties of para-aramid composites made with a thermoplastic-thermoset blend. J. Thermoplast. Compos. Mater. 2021, 36, 866. [Google Scholar] [CrossRef]
- Carpenter, A.J.; Chocron, S.; Scott, N.L.; Anderson, C.E., Jr. Characterization, Modeling, and Ballistic Impact of Kevlar/Phenolic Pvb Composite. J. Dyn. Behav. Mater. 2023, 9, 225–239. [Google Scholar] [CrossRef]
- Soykasap, O.; Colakoglu, M. Ballistic performance of a Kevlar-29 woven fibre composite under varied temperatures. Mech. Compos. Mater. 2010, 46, 35–42. [Google Scholar] [CrossRef]
- de Ruijter, C.; van der Zwaag, S.; Stolze, R.; Dingemans, T. Liquid crystalline matrix polymers for aramid ballistic composites. Polym. Compos. 2009, 31, 612–619. [Google Scholar] [CrossRef]
- Ali, M.F.; Hussain, M.; Tariq, A.; Ahmed, H.I.; Shahid, S.; Saouab, A.; Nawab, Y. Damage-Tolerant Woven Glass Fiber Composites Developed Using Polyvinyl Butyral (PVB) Unsaturated Polyester (UP) Blends. Adv. Mater. Sci. Eng. 2022, 2022, 9077788. [Google Scholar] [CrossRef]
- ASTM D7136m-20; Standard Test Method for Measuring the Damage Resistance of a Fiber-Reinforced Polymer Matrix Composite to a Drop-Weight Impact Event. ASTM International: West Conshohocken, PA, USA, 2020.
- Mowital® (Polyvinyl Butyral) is a Building Block for Specialty Coatings and Films, Including Trosifol®, Polyvinyl Butyral Film. Kuraray Produces Polyvinyl Butyral (PVB) Resins with Properties That Are Characterized by the Presence of Butyral Units, Hydroxyl, and Acetyl Groups. Kuraray America, Inc. 2024. Available online: https://kuraray.us.com/products/polymers/mowital-piolofor/ (accessed on 3 June 2024).
- ASTM D638-10; Standard Test Method for Tensile Properties of Plastics. ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM D3763-18; Standard Test Method for High Speed Puncture Properties of Plastics Using Load and Displacement Sensors. ASTM International: West Conshohocken, PA, USA, 2018.
- Bai, R.; Ma, Y.; Lei, Z.; Feng, Y.; Liu, D.; Yan, C. Impact resistance analysis of flexible fabric by 3D shape of impact basin in low-speed impact test. Polym. Test. 2020, 81, 106215. [Google Scholar] [CrossRef]
- Boumbimba, R.M.; Coulibaly, M.; Khabouchi, A.; Kinvi-Dossou, A.; Bonfoh, N.; Gerard, P. Glass fibres reinforced acrylic thermoplastic resin-based tri-block copolymers composites: Low velocity impact response at various temperatures. Compos. Struct. 2017, 160, 939–951. [Google Scholar] [CrossRef]
- Ngan, A.H.W.; Tang, B. Viscoelastic effects during unloading in depth-sensing indentation. J. Mater. Res. 2002, 17, 2604–2610. [Google Scholar] [CrossRef]
- Furmanski, J.; Cady, C.M.; Brown, E.N. Time–temperature equivalence and adiabatic heating at large strains in high density polyethylene and ultrahigh molecular weight polyethylene. Polymer 2013, 54, 381–390. [Google Scholar] [CrossRef]
- Yakovlev, S.; Fiscus, D.; Brant, P.; Butler, J.; Bucknall, D.G.; Downing, K.H. Mechanism of stress induced crystallization of polyethylene. Polymer 2019, 175, 25–31. [Google Scholar] [CrossRef]
- Moreno, C.A.; Piroird, K.; Lorenceau, E. Extended time–temperature rheology of polyvinyl butyral (PVB). Rheol. Acta 2022, 61, 539–547. [Google Scholar] [CrossRef]
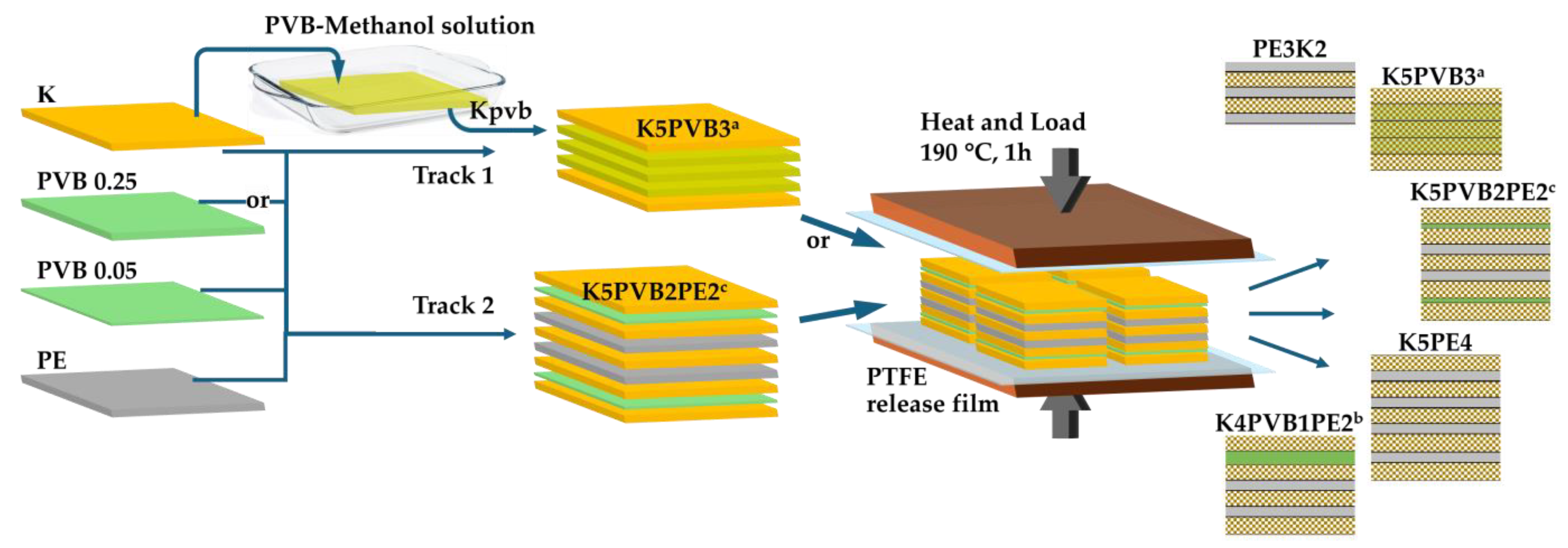


| Index | Stacking | Number of Layers/Kevlar Layers | Thickness *, mm | Areal Density **, kg/m2 | Heat Press T, °C |
|---|---|---|---|---|---|
| PE3K2 | PE–K–PE–K–PE | 5/2 | 1.34 ± 0.05 | 1.00 | 190 |
| K5PE4 | K–PE–K–PE–K–PE–K–PE–K | 9/5 | 2.62 ± 0.05 | 1.84 | 190 |
| K5PVB3 a | K–Kpvb–Kpvb–Kpvb–K | 8/5 | 1.39 ± 0.05 | 1.37 | 190 |
| K4PVB1PE2 b | K–PVB–K–PE–K–PE–K | 7/4 | 1.6 ± 0.1 | 1.52 | 190 |
| K5PVB2PE2 c | K–PVB–K–PE–K–PE–K–PVB–K | 9/5 | 1.75 ± 0.05 | 1.57 | 190 |
| PE3K2 | K5PE4 | K5PVB3 a | K4PVB1PE2 b | K5PVB2PE2 c |
|---|---|---|---|---|
 |  |  |  |  |
 ), Kevlar® fabric (
), Kevlar® fabric ( ), a Kevlar® fabric impregnated with PVB (
), a Kevlar® fabric impregnated with PVB ( ), b Mowital® PVB 0.25 mm (
), b Mowital® PVB 0.25 mm ( ) and c 0.05 mm (
) and c 0.05 mm ( ) film thickness.
) film thickness.| UHMWPE | Strain Rate, %/min | ||
| 2 | 5 | 10 | |
| E along, MPa | 250 ± 3 | 268 ± 3 | 319 ± 3 |
| E normal, MPa | 286 ± 3 | 348 ± 3 | 380 ± 3 |
| UTS along, MPa | 14.8 ± 0.2 | 15.8 ± 0.2 | 17.1 ± 0.2 |
| UTS normal, MPa | 15.9 ± 0.2 | 18.3 ± 0.2 | 19.3 ± 0.2 |
| PVB—Mowital® | Strain Rate, %/min | ||
| 2 | 10 | 30 | |
| E, GPa | 1.30 ± 0.01 | 1.28 ± 0.01 | 1.42 ± 0.01 |
| UTS, MPa | 44.9 ± 0.2 | 47.5 ± 0.2 | 52.2 ± 0.2 |
| Samples | Peak Force, N | Peak Stress, GPa | Time to Peak, ms | Fracture Initiation Force, N | Maximum Penetration, mm | Residual Deformation, mm |
| PE3K2 | 1330 | 1.24 | 5.68 | - | 13.92 | 11.03 |
| K5PE4 | 1770 | 1.65 | 5.00 | - | 12.07 | 8.14 |
| K5PVB3 | 1520 | 1.42 | 4.04 | 1100 | 10.50 | 8.23 |
| K4PVB1PE2 | 1780 | 1.66 | 4.00 | 900 | 10.47 | 7.79 |
| K5PVB2PE2 | 1840 | 1.71 | 4.24 | 1190 | 10.49 | 7.33 |
| Samples | Fracture Initiation Energy (Total and Per Areal Density) | Elastic Energy (Total and Per Areal Density) | Absorbed Energy (Total and Per Areal Density) | |||
| J | J/(kg/m2) | J | J/(kg/m2) | J | J/(kg/m2) | |
| PE3K2 | - | - | 0.62 | 0.617 | 8.69 | 8.69 |
| K5PE4 | - | - | 1.41 | 0.77 | 7.90 | 4.29 |
| K5PVB3 | 2.31 | 1.68 | 0.65 | 0.47 | 8.66 | 6.31 |
| K4PVB1PE2 | 1.90 | 1.25 | 0.93 | 0.61 | 8.38 | 5.52 |
| K5PVB2PE2 | 3.14 | 1.99 | 1.17 | 0.75 | 8.14 | 5.17 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nael, M.A.; Dikin, D.A.; Admassu, N.; Elfishi, O.B.; Percec, S. Damage Resistance of Kevlar® Fabric, UHMWPE, PVB Multilayers Subjected to Concentrated Drop-Weight Impact. Polymers 2024, 16, 1693. https://doi.org/10.3390/polym16121693
Nael MA, Dikin DA, Admassu N, Elfishi OB, Percec S. Damage Resistance of Kevlar® Fabric, UHMWPE, PVB Multilayers Subjected to Concentrated Drop-Weight Impact. Polymers. 2024; 16(12):1693. https://doi.org/10.3390/polym16121693
Chicago/Turabian StyleNael, Manal A., Dmitriy A. Dikin, Natnael Admassu, Omar Bahgat Elfishi, and Simona Percec. 2024. "Damage Resistance of Kevlar® Fabric, UHMWPE, PVB Multilayers Subjected to Concentrated Drop-Weight Impact" Polymers 16, no. 12: 1693. https://doi.org/10.3390/polym16121693
APA StyleNael, M. A., Dikin, D. A., Admassu, N., Elfishi, O. B., & Percec, S. (2024). Damage Resistance of Kevlar® Fabric, UHMWPE, PVB Multilayers Subjected to Concentrated Drop-Weight Impact. Polymers, 16(12), 1693. https://doi.org/10.3390/polym16121693








