Mechanical Properties and Stress–Strain Relationship of PVA-Fiber-Reinforced Engineered Geopolymer Composite
Abstract
1. Introduction
2. Materials and Experiments
2.1. Materials
2.1.1. Binders
2.1.2. Alkali Activator
2.1.3. Fiber and Superplasticizer
2.1.4. Quartz Sand
2.2. Mix Proportion and Specimen Preparation
2.3. Test Methods
3. Experimental Results
3.1. Rheological Property
3.2. Mechanical Strength
3.2.1. Compressive Strength
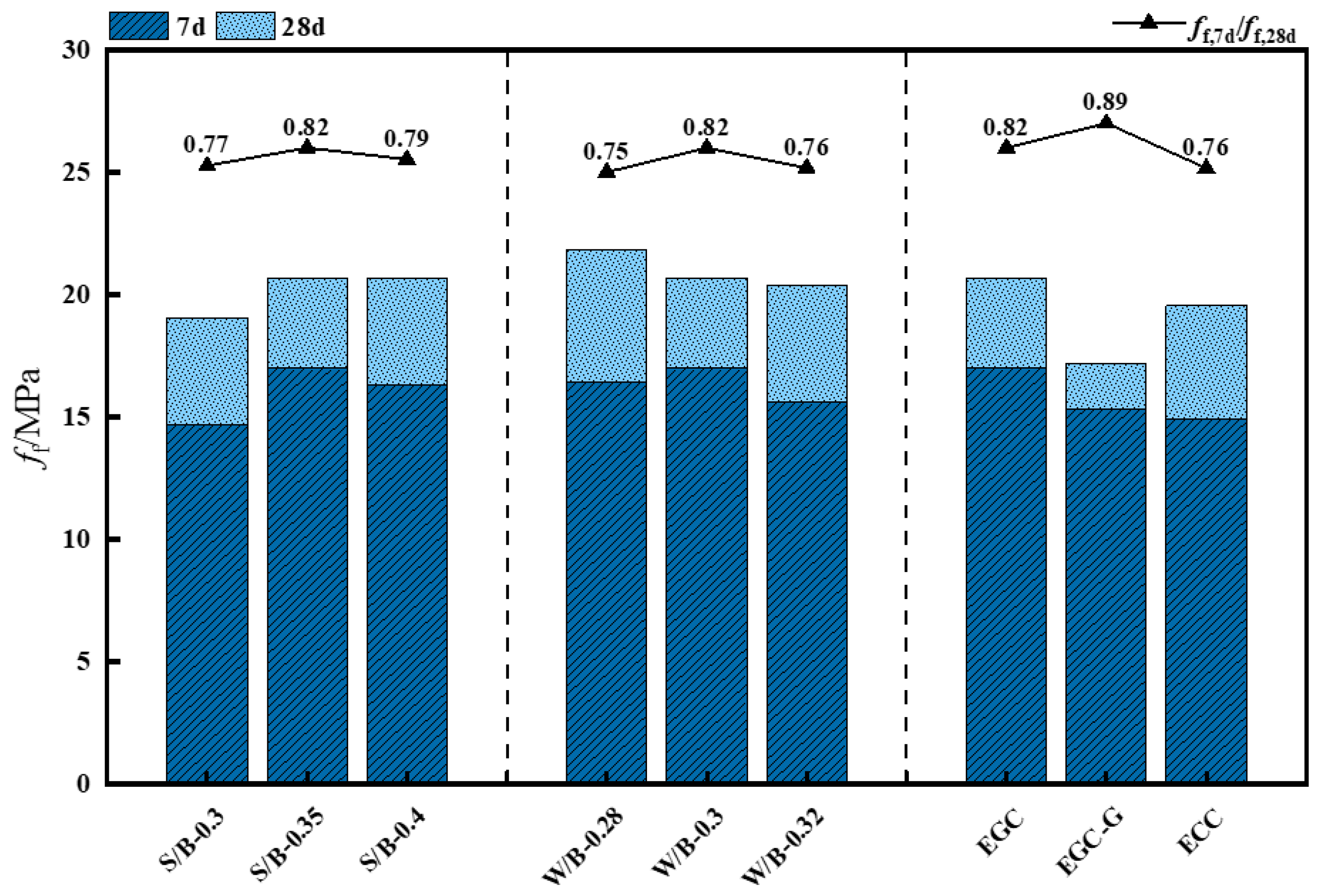
3.2.2. Flexural Strength
3.3. Uniaxial Tensile Behaviors
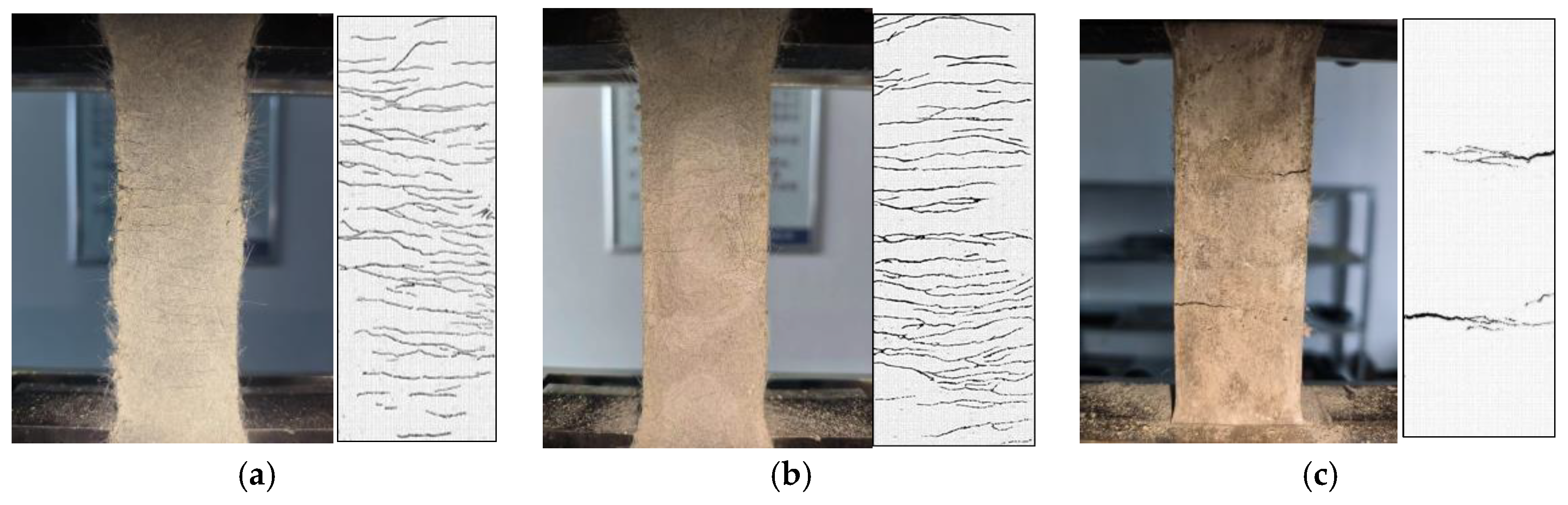
3.3.1. Failure Patterns
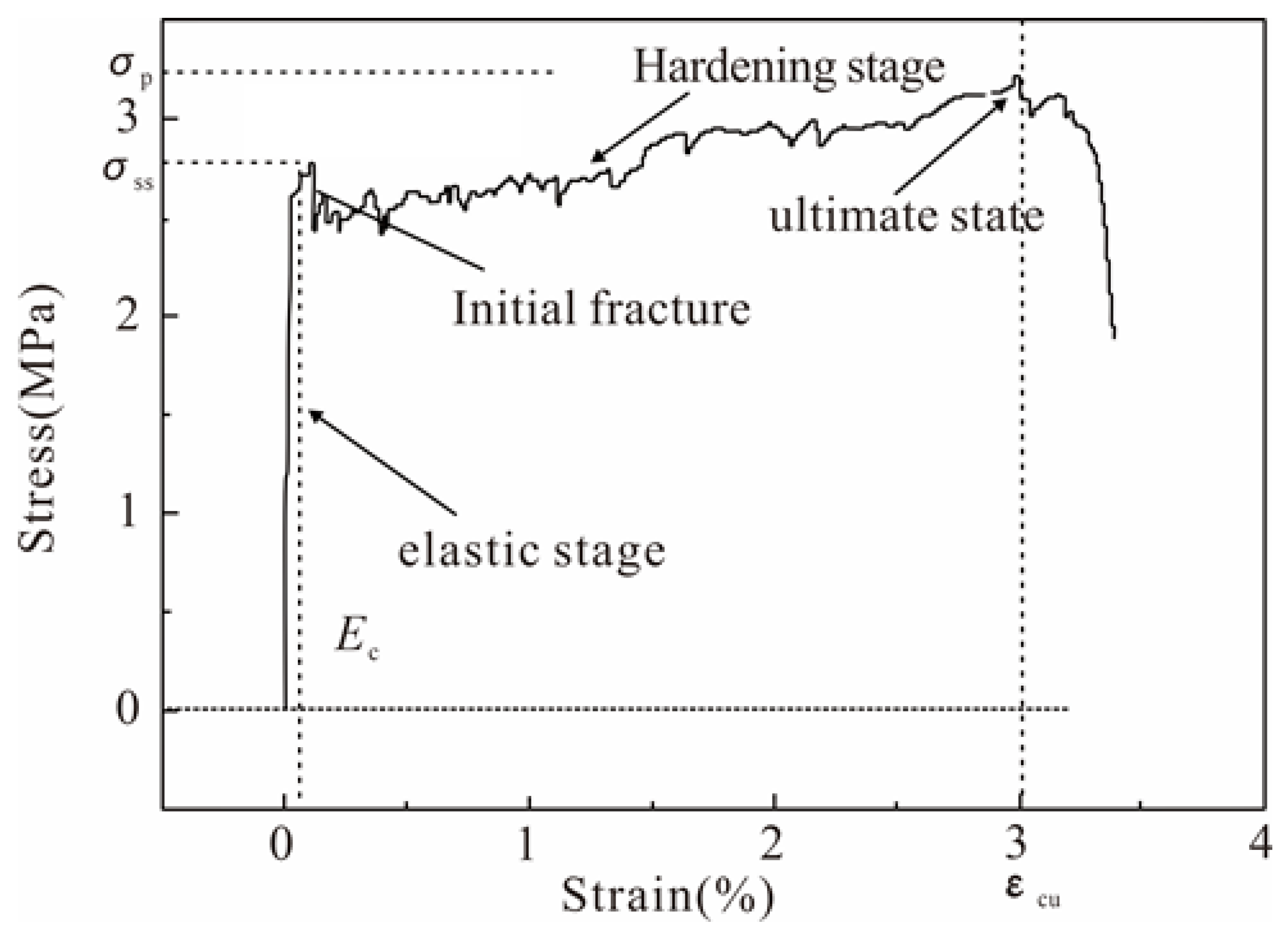
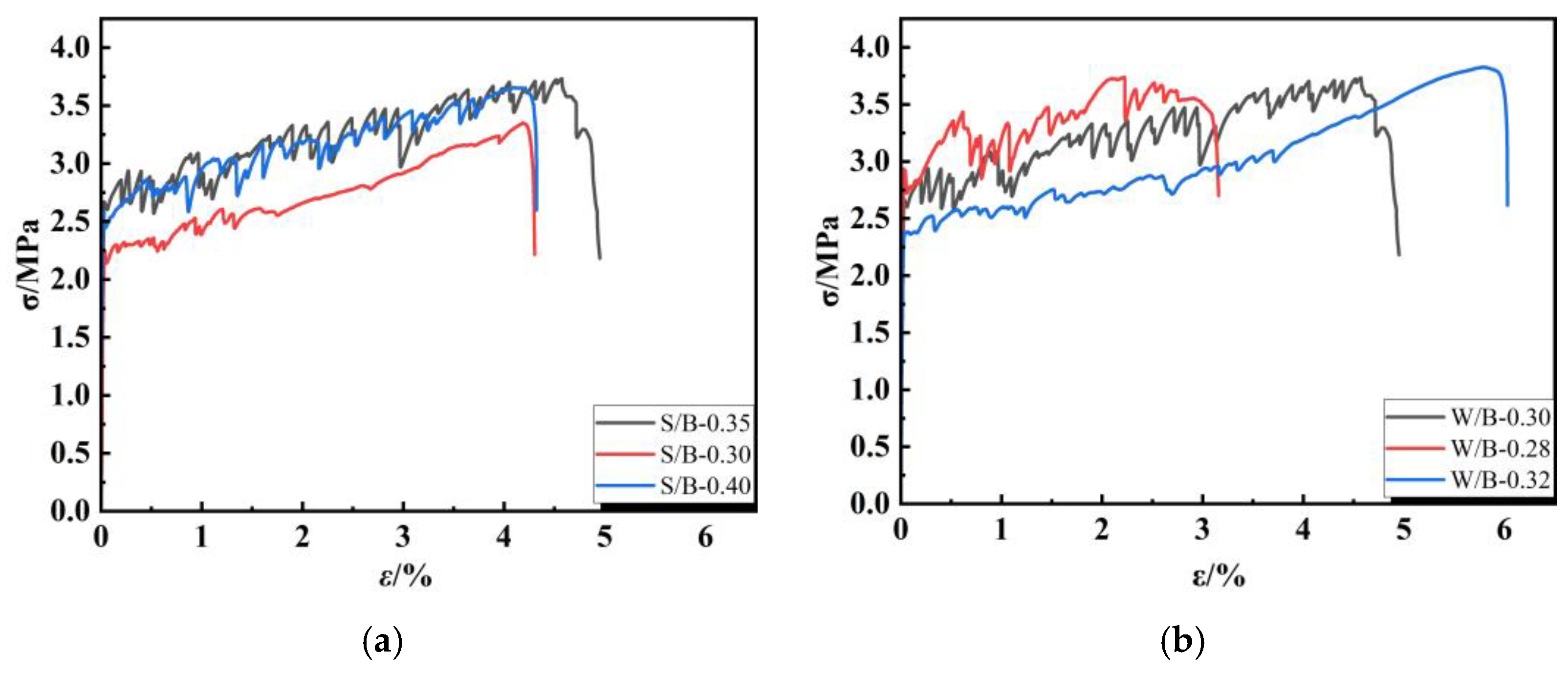
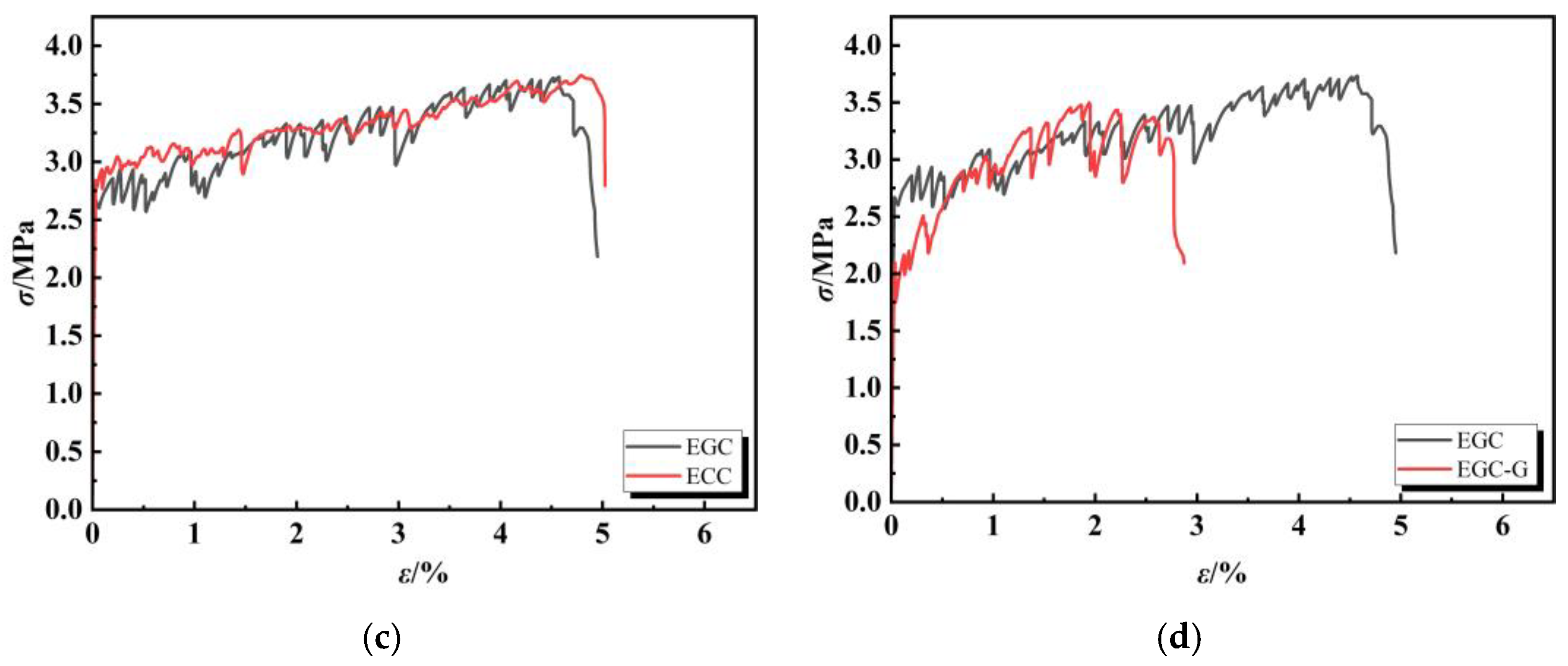
3.3.2. Stress–Strain Curves
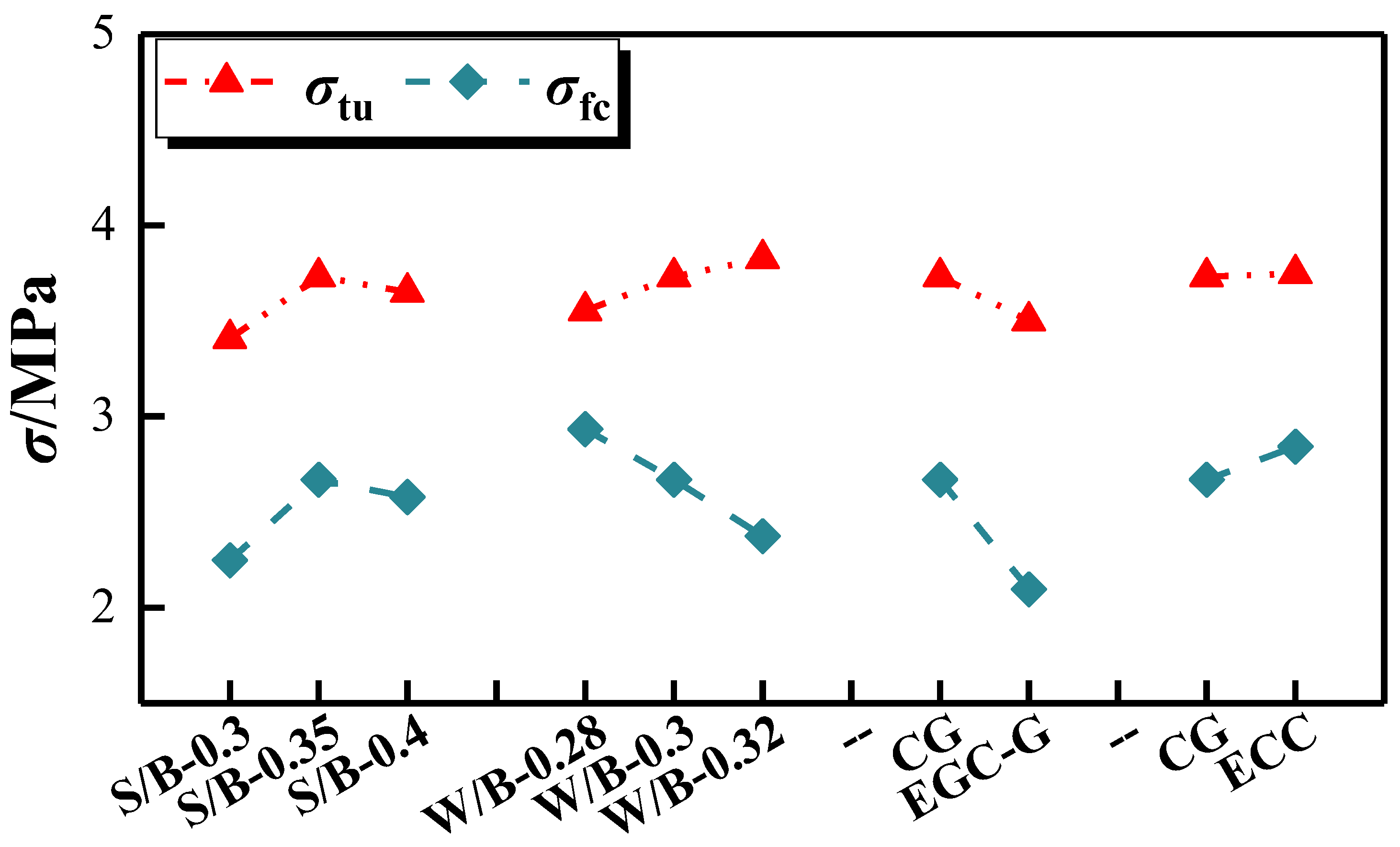
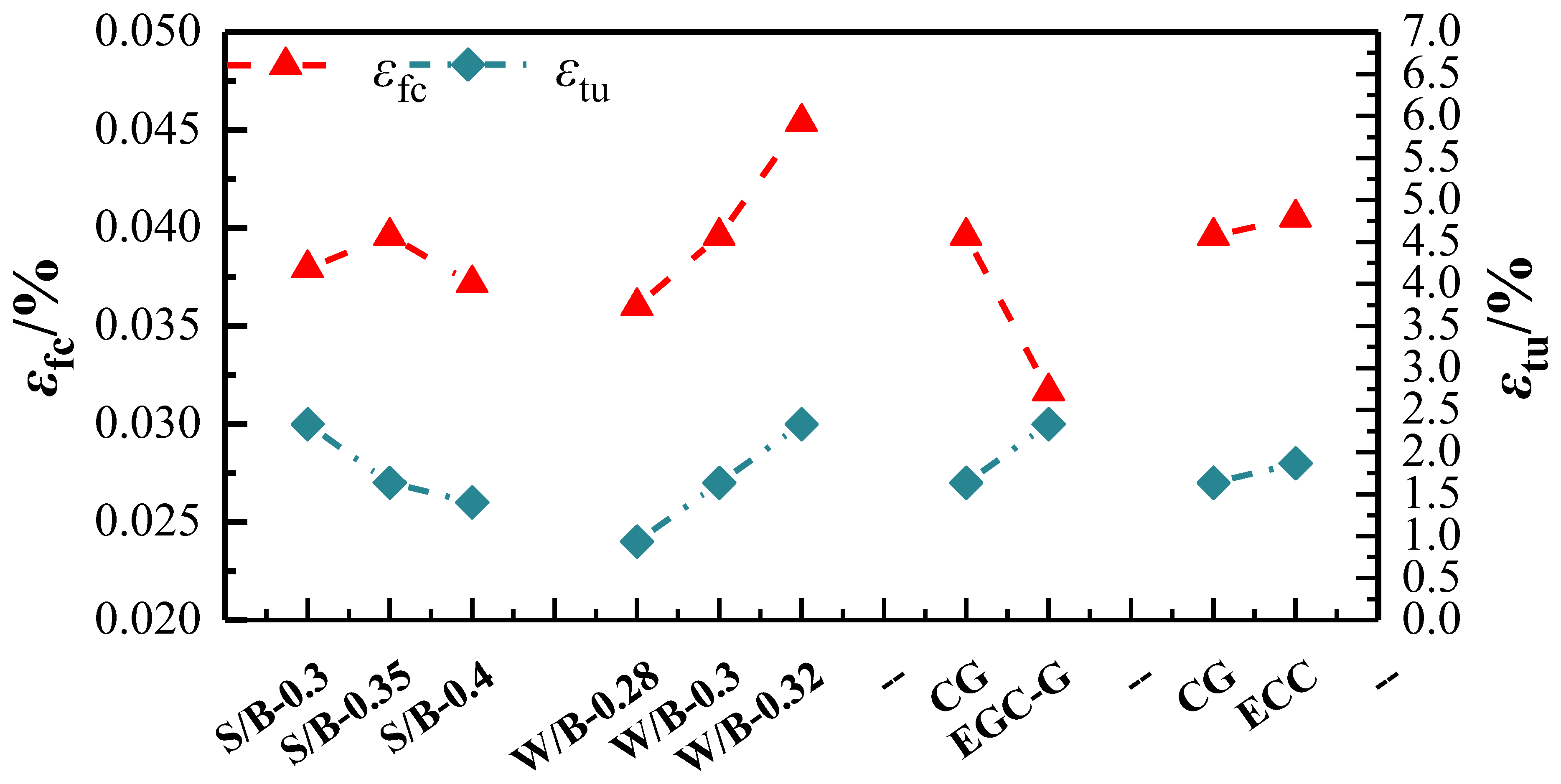
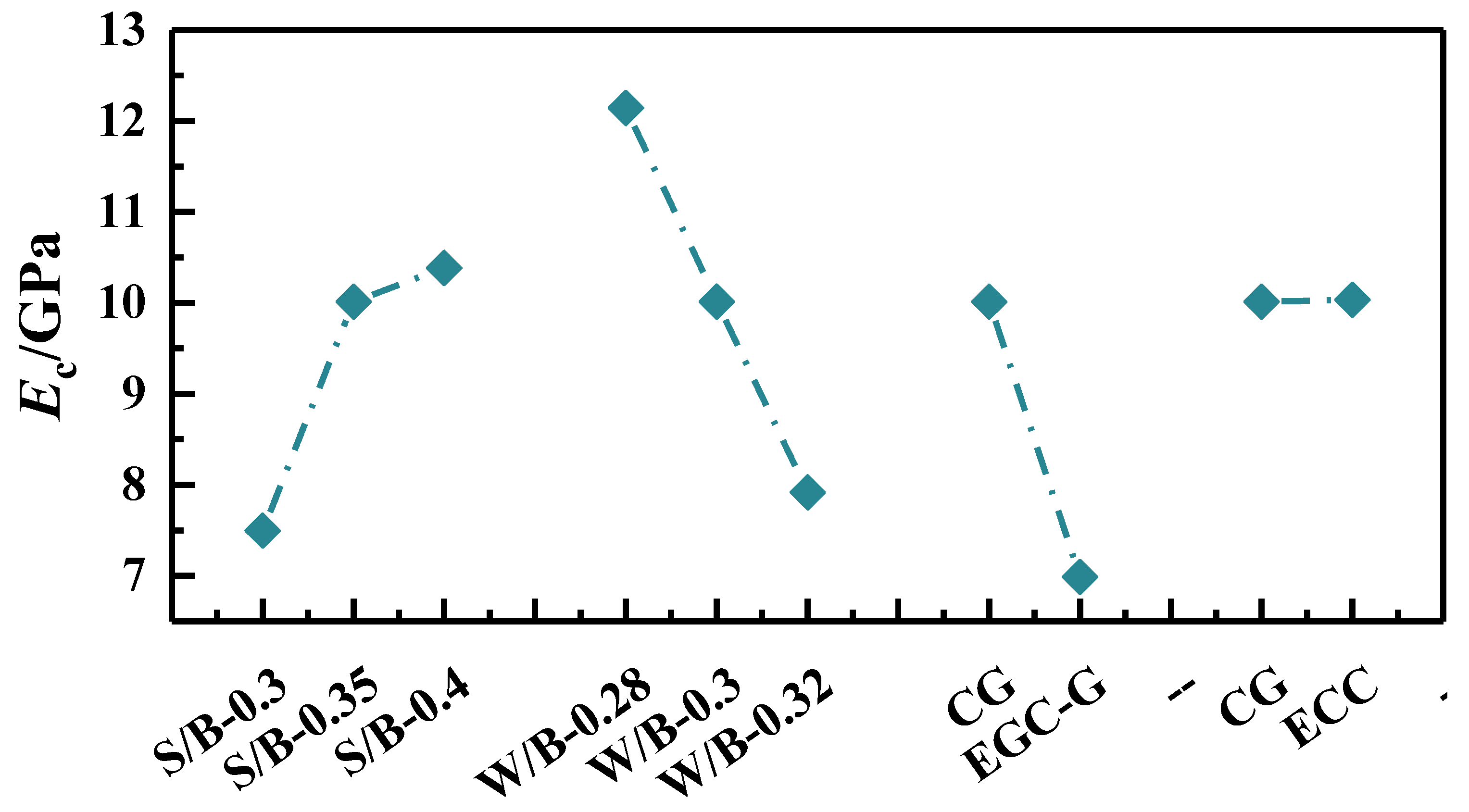
3.3.3. Characteristic Parameters
- (1)
- Uniaxial tensile strength
- (2)
- Uniaxial tensile strain
- (3)
- Elastic modulus
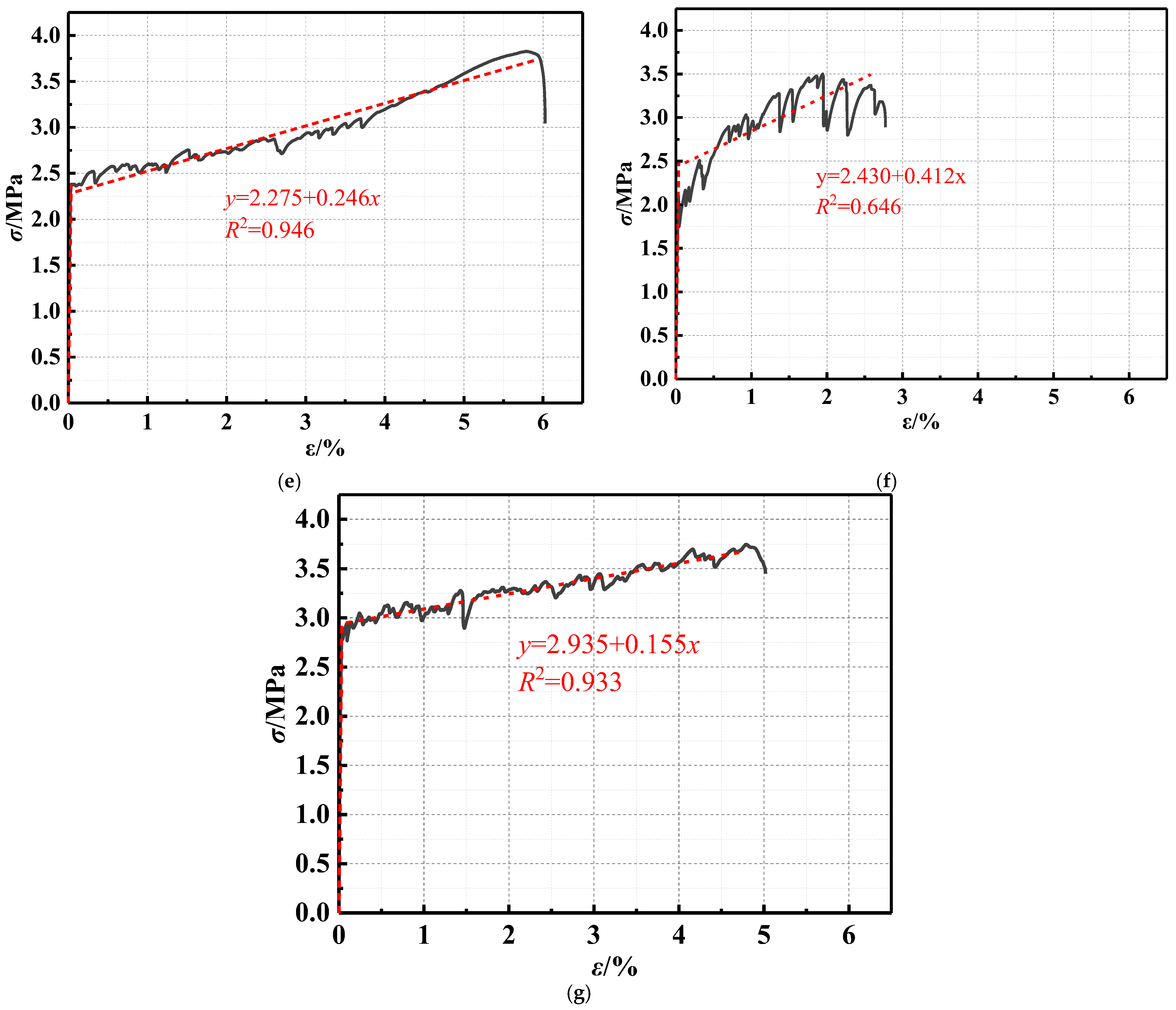
3.3.4. Stress–Strain Model
4. Fractal Characteristics of Cracks
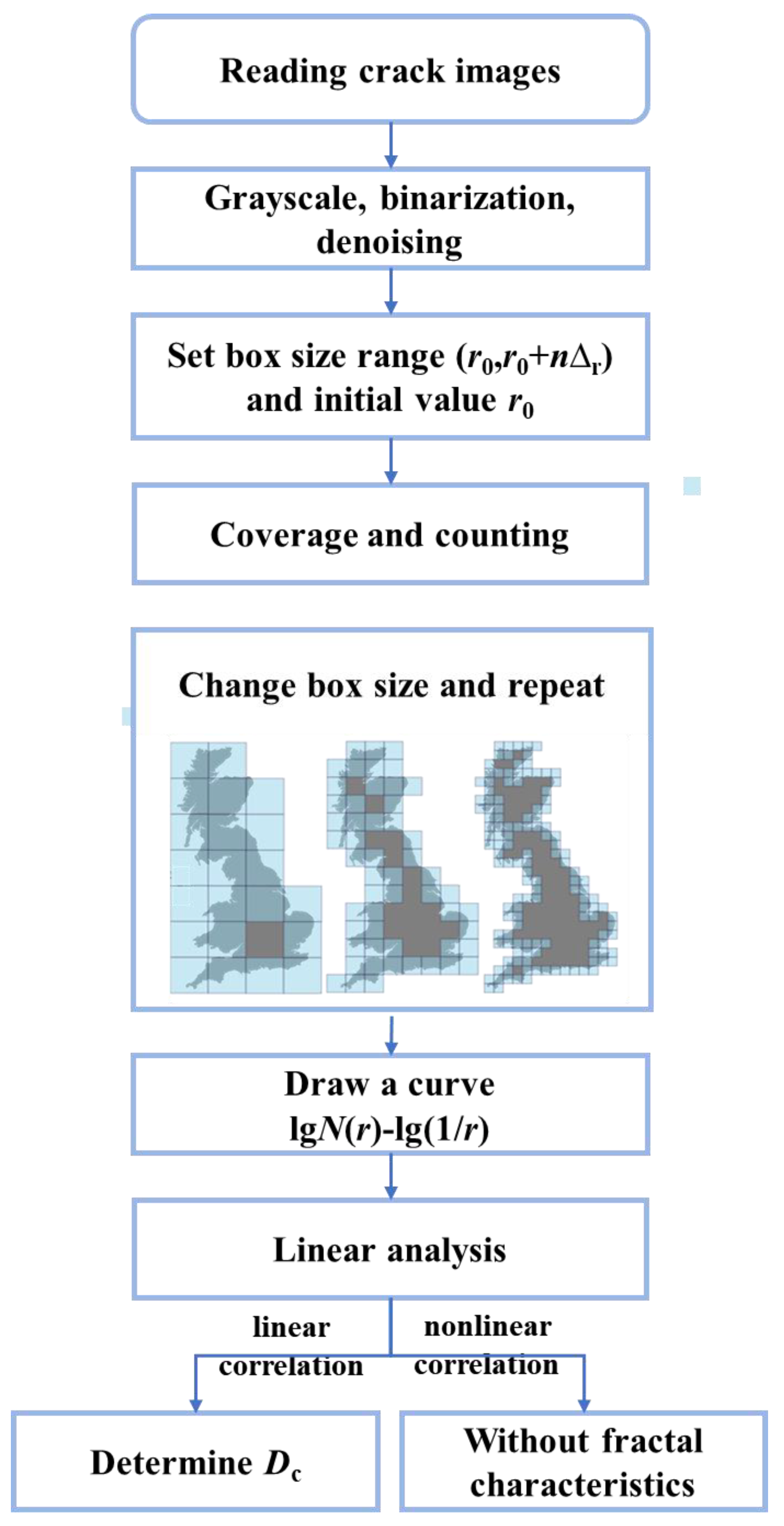
4.1. Fractal Theory
4.2. Experimental Data Collection
4.3. Fractal Dimension
5. Conclusions
- (1)
- The sand-to-binder ratio significantly influences the rheological properties of the EGC; an increase in this ratio enhances rheology, whereas a decrease tends to diminish it. Additionally, a lower water-to-binder ratio was found to substantially reduce rheology, indicating that fluidity and workability are critically dependent on the precise balance of these ratios, affecting the ease of application and quality of the final product.
- (2)
- The compressive strength of the EGC specimens at seven days reached 80% to 92% of their 28-day strength, indicating a rapid early strength gain. The hierarchy of factors influencing compressive strength was identified as the fiber type having the greatest impact, followed by the water-to-binder ratio and then the sand-to-binder ratio.
- (3)
- The stress–strain curves for both the EGC and Engineered Cementitious Composite (ECC) displayed similar characteristics, predominantly in the pre-cracking elastic stage where the matrix material sustains most of the applied stress, maintaining a consistent and predictable deformation pattern. This similarity underscores the potential of the EGC as a viable alternative to the ECC with comparable mechanical resilience.
- (4)
- An increase in the water/binder ratio leads to higher ultimate strain capacities in the composite materials, albeit at the expense of reduced strength, demonstrating a trade-off between ductility and strength. This study has established a quantifiable stress–strain relationship for EGC, tailored to different mix ratios.
- (5)
- Future research should address the long-term durability and economic viability of PVA-EGCs, exploring their performance across a broader range of environmental conditions and material compositions to enhance their practical application in the construction industry.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, V.C. Engineered Cementitious Composites (ECC): Bendable Concrete for Sustainable and Resilient Infrastructure; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar]
- Li, V.C. From micromechanics to structural engineering the design of cementitious composites for civil engineering applications. Doboku Gakkai Ronbunshu 1993, 471, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Li, V.C. Engineered cementitious composites with high-volume fly ash. ACI Struct. J. 2007, 104, 233–241. [Google Scholar]
- Celik, K. Development and Characterization of Sustainable Self-Consolidating Concrete Containing High Volume of Limestone Powder and Natural or Calcined Pozzolanic Materials. Master’s Thesis, University of California, Berkeley, CA, USA, 2015. [Google Scholar]
- Bosoaga, A.; Masek, O.; Oakey, J.E. CO2 capture technologies for cement industry. Energy Procedia 2009, 1, 133–140. [Google Scholar] [CrossRef]
- Celik, K.; Meral, C.; Petek Gursel, A.; Mehta, P.K.; Horvath, A. Mechanical properties, durability, and life-cycle assessment of self-consolidating concrete mixtures made with blended portland cements containing fly ash and limestone powder. Cem. Concr. Compos. 2015, 56, 59–72. [Google Scholar] [CrossRef]
- Celik, K.; Hay, R.; Hargis, C.W.; Moon, J. Effect of volcanic ash pozzolan or limestone replacement on hydration of portland cement. Constr. Build. Mater. 2019, 197, 803–812. [Google Scholar] [CrossRef]
- Celik, K.; Jackson, M.D.; Mancio, M.; Meral, C.; Emwas, A.H.; Mehta, P.K.; Monteiro, P.J.M. High-volume natural volcanic pozzolan and limestone powder as partial replacements for portland cement in self-compacting and sustainable concrete. Cem. Concr. Compos. 2014, 45, 136–147. [Google Scholar] [CrossRef]
- Scrivener, K.L.; Lothenbach, B.; De Belie, N.; Gruyaert, E.; Skibsted, J.; Snellings, R.; Vollpracht, A. TC 238-SCM: Hydration and microstructure of concrete with SCMs: State of the art on methods to determine degree of reaction of SCMs. Mater. Struct. 2015, 48, 835–862. [Google Scholar] [CrossRef]
- Li, J.; Zhang, W.; Garbev, K.; Monteiro, P.J.M. Coordination environment of si in calcium silicate hydrates, silicate minerals, and blast furnace slags: A xanes database. Cem. Concr. Res. 2021, 143, 106376. [Google Scholar] [CrossRef]
- Ren, R.; Li, L. Impact of polyethylene fiber reinforcing index on the flexural toughness of geopolymer mortar. J. Build. Eng. 2022, 57, 104943. [Google Scholar] [CrossRef]
- Kumar, S.; Das, C.S.; Lao, J.C.; Alrefaei, Y.; Dai, J.G. Effect of sand content on bond performance of engineered geopolymer composites (EGC) repair material. Constr. Build. Mater. 2022, 328, 127080. [Google Scholar] [CrossRef]
- Deng, B.Y.; Tan, D.; Li, L.Z.; Zhang, Z.; Cai, Z.W.; Yu, K.Q. Flexural behavior of precast ultra-lightweight ECC-concrete composite slab with lattice girders. Eng. Struct. 2023, 279, 115553. [Google Scholar] [CrossRef]
- Shaikh, F.U.A. Deflection hardening behaviour of short fibre reinforced fly ash based geopolymer composites. Mater. Des. 2013, 50, 674–682. [Google Scholar] [CrossRef]
- Nematollahi, B.; Sanjayan, J.; Shaikh, F.U.A. Comparative deflection hardening behavior of short fiber reinforced geopolymer composites. Constr. Build. Mater. 2014, 70, 54–64. [Google Scholar] [CrossRef]
- Nematollahi, B.; Sanjayan, J.; Shaikh, F.U.A. Influence of the type of activator combinations on the deflection hardening behavior of geopolymer composites. In Proceedings of the SHCC3-3rd International RILEM Conference on Strain Hardening Cementitious Composites, Dordrecht, The Netherlands, 3 November 2014. [Google Scholar]
- Lee, B.Y.; Cho, C.G.; Lim, H.J.; Song, J.K.; Yang, K.H.; Li, V.C. Strain hardening fiber reinforced alkali-activated mortar—A feasibility study. Constr. Build. Mater. 2012, 37, 15–20. [Google Scholar] [CrossRef]
- Ohno, M.; Li, V.C. A feasibility study of strain hardening fiber reinforced fly ash-based geopolymer composites. Constr. Build. Mater. 2014, 57, 163–168. [Google Scholar] [CrossRef]
- Fernández-Jiménez, A.; Palomo, A. Composition and microstructure of alkali activated fly ash binder: Effect of the activator. Cem. Concr. Res. 2005, 35, 1984–1992. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, Y.; Zhang, M. Effect of sand content on engineering properties of fly ash-slag based strain hardening geopolymer composites. J. Build. Eng. 2021, 34, 101951. [Google Scholar] [CrossRef]
- Lao, J.-C.; Ma, R.-Y.; Xu, L.-Y.; Li, Y.; Shen, Y.-N.; Yao, J.; Wang, Y.-S.; Xie, T.-Y.; Huang, B.-T. Fly ash-dominated high-strength engineered/strain-hardening geopolymer composites (HS-EGC/SHGC): Influence of alkalinity and environmental assessment. J. Clean. Prod. 2024, 447, 141182. [Google Scholar] [CrossRef]
- Wang, F.; Ma, J.; Ding, Y.; Yu, J.; Yu, K. Engineered geopolymer composite (EGC) with ultra-low fiber content of 0.2%. Constr. Build. Mater. 2024, 411, 134626. [Google Scholar] [CrossRef]
- Krevaikas, T.D. Experimental study on carbon fiber textile reinforced mortar system as a means for confinement of masonry columns. Constr. Build. Mater. 2019, 208, 723–733. [Google Scholar] [CrossRef]
- Ombres, L.; Mazzuca, P. Residual Flexural Behavior of PBO FRCM-Strengthened Reinforced Concrete Beams after Exposure to Elevated Temperatures. J. Compos. Constr. 2024, 28, 04023063. [Google Scholar] [CrossRef]
- GB/T 2419-2005; Test Method for Fluidity of Cement Mortar. Standardization Administration of China: Beijing, China, 2005.
- Peng, Y.-Q.; Zheng, D.-P.; Pan, H.-S.; Yang, J.-L.; Lin, J.-X.; Lai, H.-M.; Wu, P.-Z.; Zhu, H.-Y. Strain hardening geopolymer composites with hybrid POM and UHMWPE fibers: Analysis of static mechanical properties, economic benefits, and environmental impact. J. Build. Eng. 2023, 76, 107315. [Google Scholar] [CrossRef]
- Ling, Y.; Wang, K.; Li, W.; Shi, G.; Lu, P. Effect of slag on the mechanical properties and bond strength of fly ash-based engineered geopolymer composites. Compos. Part B Eng. 2019, 164, 747–757. [Google Scholar] [CrossRef]
- Nguyễn, H.H.; Lương, Q.-H.; Choi, J.-I.; Ranade, R.; Li, V.C.; Lee, B.Y. Ultra-ductile behavior of fly ash-based engineered geopolymer composites with a tensile strain capacity up to 13.7%. Cem. Concr. Compos. 2021, 122, 104133. [Google Scholar] [CrossRef]
- Nguyễn, H.H.; Nguyễn, P.H.; Lương, Q.-H.; Meng, W.; Lee, B.Y. Mechanical and autogenous healing properties of high-strength and ultra-ductility engineered geopolymer composites reinforced by PE-PVA hybrid fibers. Cem. Concr. Compos. 2023, 142, 105155. [Google Scholar] [CrossRef]
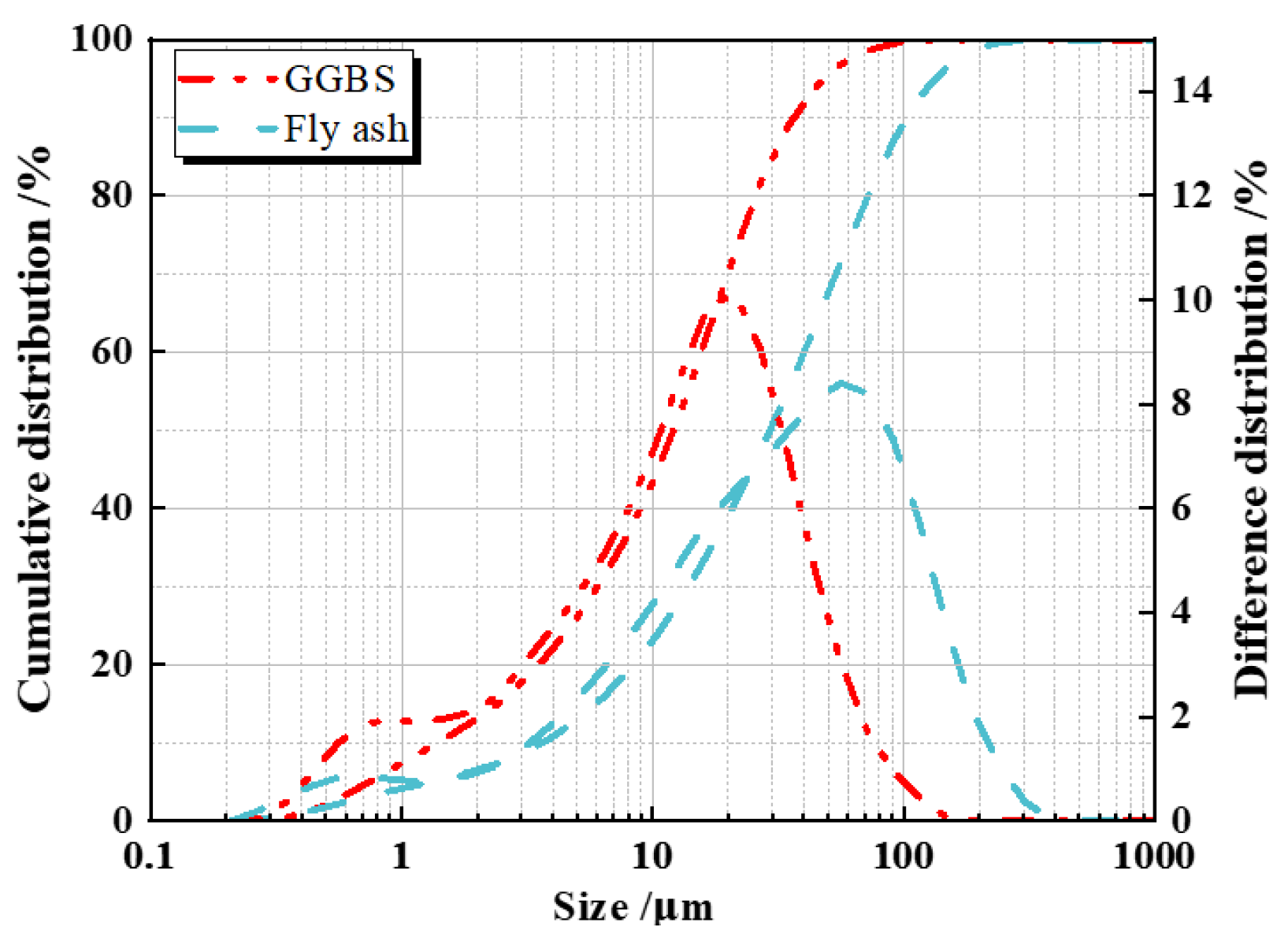

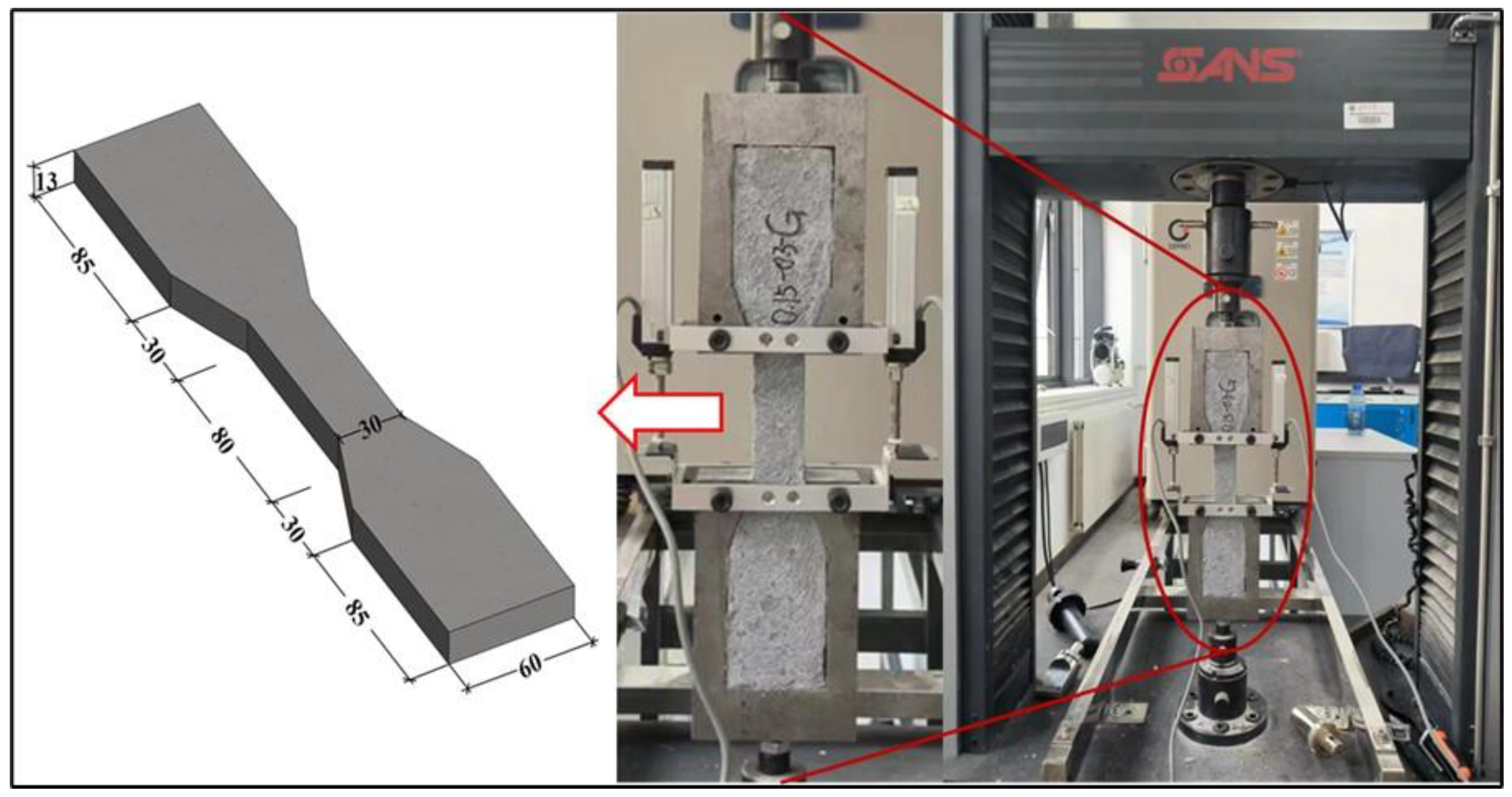








| Material | SiO2 | Al2O3 | Fe2O3 | CaO | K2O | MgO | Na2O | D50 Size/μm |
|---|---|---|---|---|---|---|---|---|
| Fly ash | 54.06 | 28.26 | 4.52 | 6.27 | 1.84 | 1.29 | 0.91 | 23.2 |
| GGBS | 32.08 | 15.13 | 0.47 | 38.61 | 0.43 | 8.45 | 0.49 | 24.0 |
| Material | Length/mm | Diameter/μm | Ultimate Strength/MPa | Elasticity Modulus/GPa | Elongation/% | Oil Coverage Rate/% |
|---|---|---|---|---|---|---|
| REC15-type PVA | 12 | 39 | 1620 | 42.8 | 6.0 | 0.8 |
| Yingjia PVA | 12 | 38 | 1600 | 40.0 | 6.5 | - |
| Material | Particle Size/μm | Packing Density/(g∙cm−3) | Appearance Density/(g∙cm−3) | Breakage Rate/% | Wear Rate/% | Porosity/% | Hardness/% |
|---|---|---|---|---|---|---|---|
| Quartz sand | 100–200 | 2.66 | 1.75 | 0.51 | 0.35 | 43 | 7.5 |
| Specimens | FA /(kg∙m−3) | GGBS /(kg∙m−3) | Concrete /(kg∙m−3) | Quartz Sand /(kg∙m−3) | Water /(kg∙m−3) | Alkali Activator /(kg∙m−3) | Fiber Volume Content /(kg∙m−3) | Fiber Type |
|---|---|---|---|---|---|---|---|---|
| CG | 923.2 | 230.8 | - | 403.9 | 86.5 | 432.7 | 26.0 | K |
| S/B-0.3 | 943.6 | 235.9 | - | 353.9 | 88.5 | 442.3 | 26.0 | K |
| S/B-0.4 | 903.6 | 225.9 | - | 451.8 | 84.7 | 423.5 | 26.0 | K |
| W/B-0.28 | 945.0 | 236.2 | - | 413.4 | 65.0 | 443.0 | 26.0 | K |
| W/B-0.32 | 902.3 | 225.6 | - | 394.8 | 107.2 | 423.0 | 26.0 | K |
| EGC-G | 923.2 | 230.8 | - | 403.9 | 86.5 | 432.7 | 26.0 | G |
| ECC | - | - | 1236.2 | 432.7 | 370.9 | - | 26.0 | K |
| Specimens | D1 | D2 | Average Value | SE |
|---|---|---|---|---|
| CG | 16.7 | 16.9 | 16.80 | 0.07 |
| S/B-0.3 | 17.2 | 17.5 | 17.35 | 0.11 |
| S/B-0.4 | 15.3 | 15.4 | 15.35 | 0.04 |
| W/B-0.28 | 14.9 | 14.7 | 14.80 | 0.07 |
| W/B-0.32 | 16.9 | 17.3 | 17.10 | 0.14 |
| EGC-G | 16.5 | 16.2 | 16.35 | 0.11 |
| ECC | 17.2 | 17.3 | 17.25 | 0.04 |
| Specimens | fcu,3d | SE | fcu,7d | SE | fcu,28d | SE |
|---|---|---|---|---|---|---|
| CG | 72.37 | 0.85 | 74.98 | 1.71 | 76.53 | 1.64 |
| S/B-0.3 | 68.12 | 1.61 | 70.59 | 1.61 | 74.92 | 1.77 |
| S/B-0.4 | 73.29 | 1.73 | 76.08 | 1.73 | 79.08 | 1.81 |
| W/B-0.28 | 80.95 | 1.91 | 82.86 | 1.91 | 86.50 | 1.84 |
| W/B-0.32 | 66.71 | 1.57 | 67.80 | 1.57 | 71.57 | 1.69 |
| EGC-G | 67.98 | 1.60 | 72.81 | 1.60 | 80.91 | 1.91 |
| ECC | 31.82 | 0.75 | 45.29 | 0.75 | 88.14 | 2.08 |
| Mixtures | ff,7d/MPa | SE | ff,28d/MPa | SE |
|---|---|---|---|---|
| CG | 17.00 | 0.40 | 20.70 | 0.49 |
| S/B-0.3 | 14.70 | 0.35 | 19.08 | 0.45 |
| S/B-0.4 | 16.32 | 0.38 | 20.70 | 0.49 |
| W/B-0.28 | 16.44 | 0.39 | 21.86 | 0.52 |
| W/B-0.32 | 15.61 | 0.37 | 20.42 | 0.48 |
| EGC-G | 15.34 | 0.36 | 17.20 | 0.41 |
| ECC | 14.94 | 0.35 | 19.58 | 0.46 |
| Mixtures | σfc/MPa | εfc/% | σtu/MPa | εtu/% | Ec/GPa |
|---|---|---|---|---|---|
| CG | 2.670 | 0.027 | 3.732 | 4.574 | 10.013 |
| S/B-0.3 | 2.249 | 0.030 | 3.409 | 4.190 | 7.497 |
| S/B-0.4 | 2.579 | 0.025 | 3.652 | 4.008 | 10.385 |
| W/B-0.28 | 2.934 | 0.024 | 3.554 | 3.736 | 12.141 |
| W/B-0.32 | 2.375 | 0.030 | 3.826 | 5.929 | 7.917 |
| EGC-G | 2.096 | 0.030 | 3.501 | 2.724 | 6.987 |
| ECC | 2.843 | 0.028 | 3.747 | 4.792 | 10.034 |
| Mixtures | σnt/MPa | εnt/% | Ec/GPa | Etu/GPa | R2 |
|---|---|---|---|---|---|
| CG | 2.726 | 0.0272 | 10.013 | 0.216 | 0.869 |
| S/B-0.3 | 2.191 | 0.0292 | 7.497 | 0.257 | 0.976 |
| S/B-0.4 | 2.666 | 0.0257 | 10.385 | 0.235 | 0.925 |
| W/B-0.28 | 2.929 | 0.0241 | 12.141 | 0.301 | 0.746 |
| W/B-0.32 | 2.282 | 0.0288 | 7.917 | 0.246 | 0.946 |
| EGC-G | 2.444 | 0.0350 | 6.987 | 0.412 | 0.646 |
| ECC | 2.939 | 0.0293 | 10.034 | 0.155 | 0.933 |
| Specimen | σu/MPa | εtu/% | |
|---|---|---|---|
| Peng | R-0 | 6.66 | 4.28 |
| R25 | 6.63 | 4.34 | |
| R-50 | 5.62 | 4.54 | |
| R-75 | 4.49 | 2.19 | |
| R-100 | 2.31 | 0.0196 | |
| Ling | I | 4.7 | 3.14 |
| II | 5.1 | 1.62 | |
| III | 6.8 | 1.04 | |
| IV | 6.1 | 0.74 | |
| Nguyễn | F-PVA | 6.64 | 10.51 |
| F-PE | 6.04 | 6.79 | |
| S-PVA | 5.18 | 11.99 | |
| S-PE | 4.60 | 10.35 | |
| Nguyễn | UD-10 | 7.11 | 12.49 |
| UD-15 | 6.79 | 13.68 | |
| UD-20 | 8.10 | 12.26 | |
| UD-25 | 7.18 | 10.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, J.; Li, Z.; Liu, X.; Yang, X.; Lv, J. Mechanical Properties and Stress–Strain Relationship of PVA-Fiber-Reinforced Engineered Geopolymer Composite. Polymers 2024, 16, 1685. https://doi.org/10.3390/polym16121685
Zhou J, Li Z, Liu X, Yang X, Lv J. Mechanical Properties and Stress–Strain Relationship of PVA-Fiber-Reinforced Engineered Geopolymer Composite. Polymers. 2024; 16(12):1685. https://doi.org/10.3390/polym16121685
Chicago/Turabian StyleZhou, Jian, Zhenjun Li, Xi Liu, Xinzhuo Yang, and Jiaojiao Lv. 2024. "Mechanical Properties and Stress–Strain Relationship of PVA-Fiber-Reinforced Engineered Geopolymer Composite" Polymers 16, no. 12: 1685. https://doi.org/10.3390/polym16121685
APA StyleZhou, J., Li, Z., Liu, X., Yang, X., & Lv, J. (2024). Mechanical Properties and Stress–Strain Relationship of PVA-Fiber-Reinforced Engineered Geopolymer Composite. Polymers, 16(12), 1685. https://doi.org/10.3390/polym16121685







