Optimizing Transport Carrier Free All-Polymer Solar Cells for Indoor Applications: TCAD Simulation under White LED Illumination
Abstract
1. Introduction
2. Methods and Materials
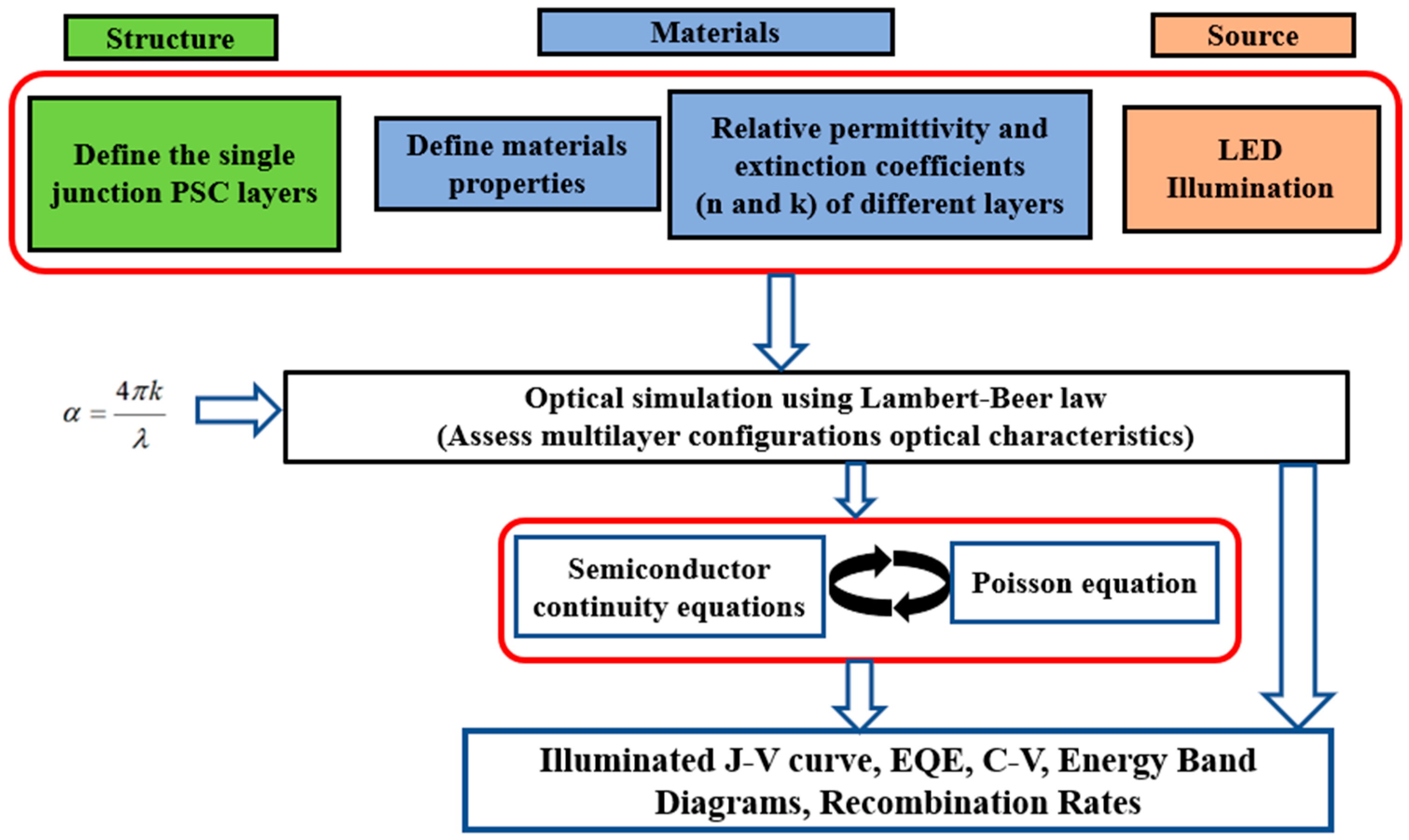
2.1. SCAPS Simulation Procedure
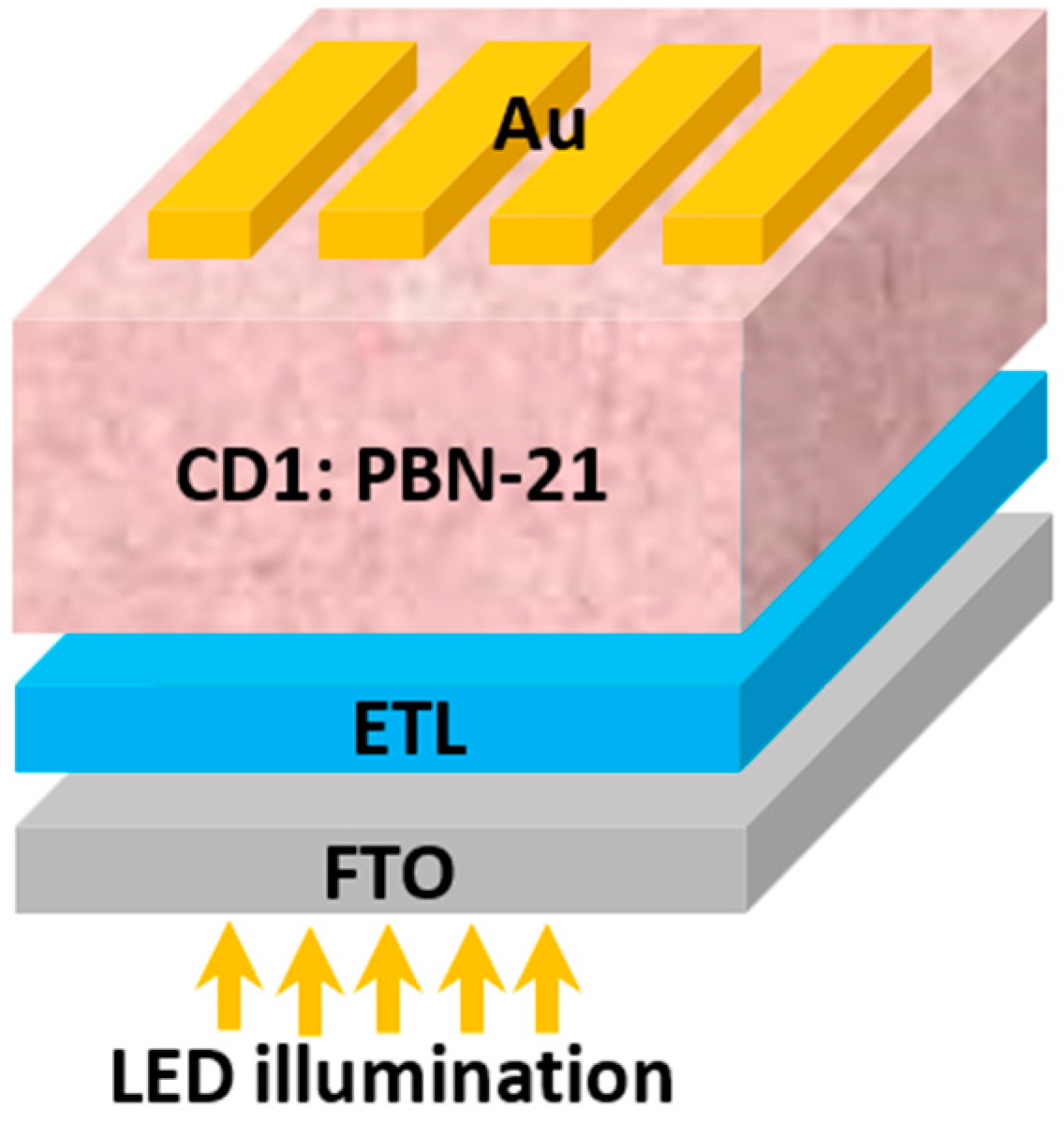
2.2. Basic Structure and Layer Materials
2.3. Calibration of APSC
2.4. Design Rules for Enhancing Cell Performance
3. Results and Discussion
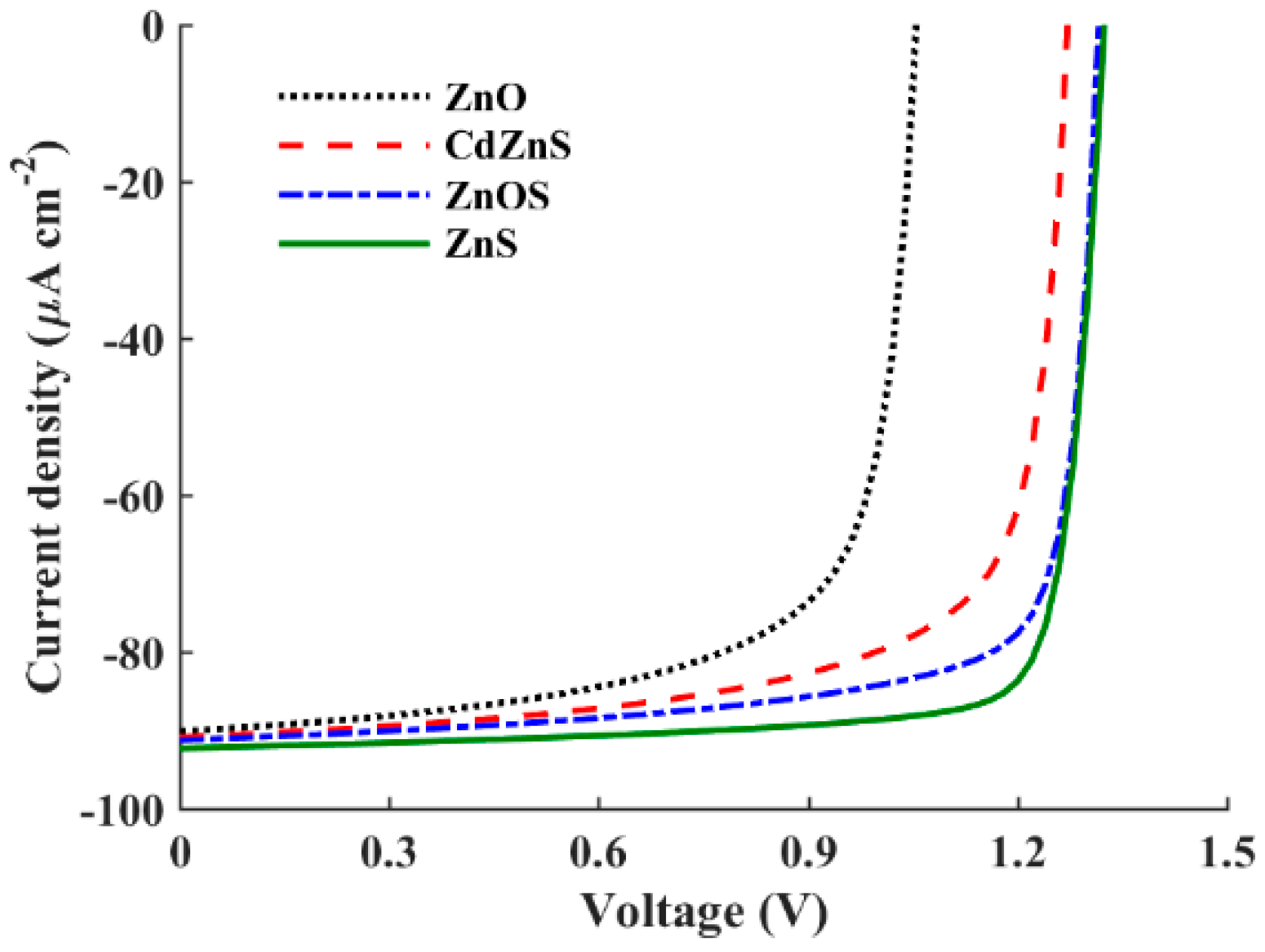
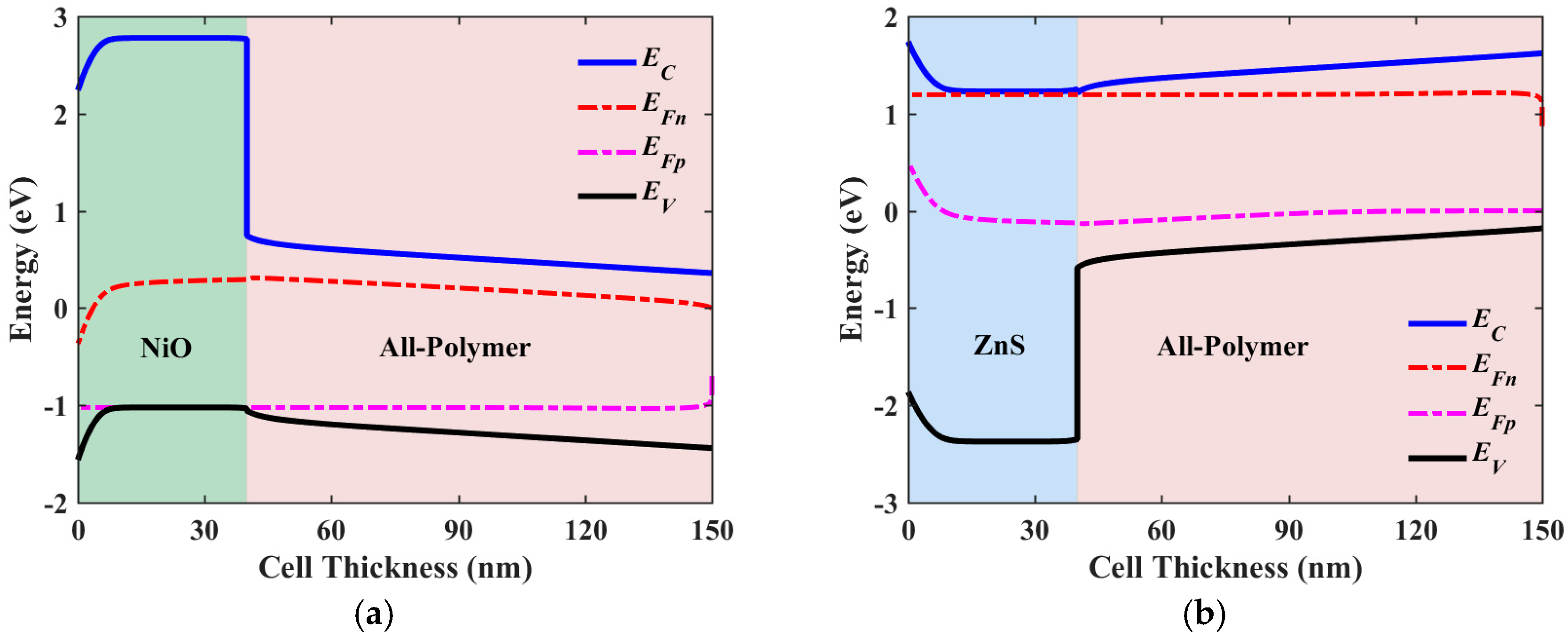
3.1. Impact of Band Alignment in ETL-Free APSC
3.2. Impact of Band Alignment in Inverted HTL-Free APSC
3.3. Comparison between ETL-Free and HTL-Free Structures
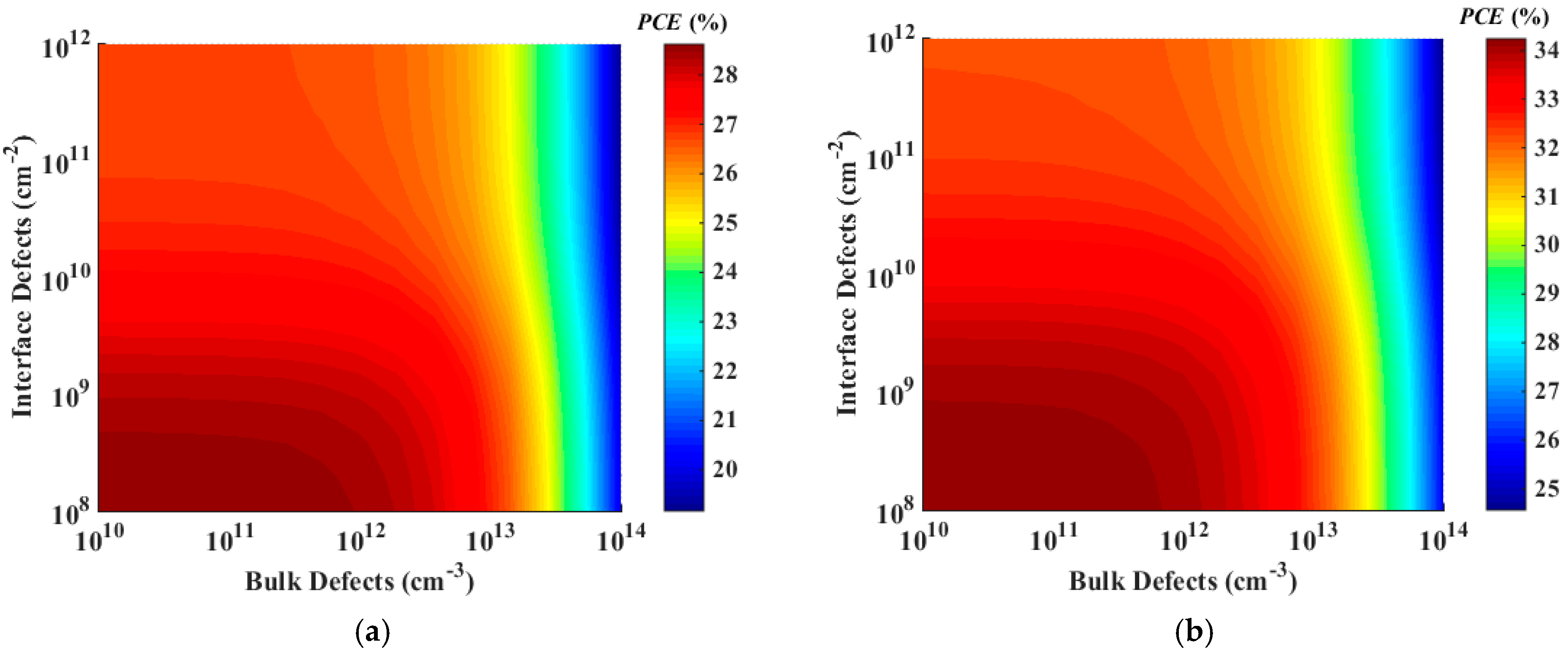
3.4. Impact of Bulk and Interface Defects on Optimized APSCs
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shaheen, M.A.M.; Hasanien, H.M.; Turky, R.A.; Ćalasan, M.; Zobaa, A.F.; Aleem, S.H.E.A. OPF of Modern Power Systems Comprising Renewable Energy Sources Using Improved CHGS Optimization Algorithm. Energies 2021, 14, 6962. [Google Scholar] [CrossRef]
- Li, B.; Hou, B.; Amaratunga, G.A.J. Indoor photovoltaics, The Next Big Trend in solution-processed solar cells. InfoMat 2021, 3, 445–459. [Google Scholar] [CrossRef]
- Goje, A.A.; Ludin, N.A.; Fahsyar, P.N.A.; Syafiq, U.; Chelvanathan, P.; Syakirin, A.D.A.-G.; Teridi, M.A.; Ibrahim, M.A.; Su’ait, M.S.; Sepeai, S.; et al. Review of flexible perovskite solar cells for indoor and outdoor applications. Mater. Renew. Sustain. Energy 2024, 13, 155–179. [Google Scholar] [CrossRef]
- Pirc, M.; Ajdič, D.; Uršič, D.; Jošt, M.; Topič, M. Indoor Energy Harvesting with Perovskite Solar Cells for IoT Applications—A Full Year Monitoring Study. ACS Appl. Energy Mater. 2024, 7, 565–575. [Google Scholar] [CrossRef]
- Jahandar, M.; Kim, S.; Lim, D.C. Indoor Organic Photovoltaics for Self-Sustaining IoT Devices: Progress, Challenges and Practicalization. ChemSusChem 2021, 14, 3449–3474. [Google Scholar] [CrossRef] [PubMed]
- Michaels, H.; Rinderle, M.; Benesperi, I.; Freitag, R.; Gagliardi, A.; Freitag, M. Emerging indoor photovoltaics for self-powered and self-aware IoT towards sustainable energy management. Chem. Sci. 2023, 14, 5350–5360. [Google Scholar] [CrossRef] [PubMed]
- Shcherbachenko, S.; Astakhov, O.; Liu, Z.; Kin, L.; Zahren, C.; Rau, U.; Kirchartz, T.; Merdzhanova, T. High-Bandgap Perovskites for Efficient Indoor Light Harvesting. Adv. Energy Sustain. Res. 2024, 5, 2400032. [Google Scholar] [CrossRef]
- Kumar, G.; Chen, F.-C. A review on recent progress in organic photovoltaic devices for indoor applications. J. Phys. D Appl. Phys. 2023, 56, 353001. [Google Scholar] [CrossRef]
- Srivishnu, K.; Rajesh, M.N.; Prasanthkumar, S.; Giribabu, L. Photovoltaics for indoor applications: Progress, challenges and perspectives. Sol. Energy 2023, 264, 112057. [Google Scholar] [CrossRef]
- Wang, Z.; Guo, Y.; Liu, X.; Shu, W.; Han, G.; Ding, K.; Mukherjee, S.; Zhang, N.; Yip, H.-L.; Yi, Y.; et al. The role of interfacial donor–acceptor percolation in efficient and stable all-polymer solar cells. Nat. Commun. 2024, 15, 1212. [Google Scholar] [CrossRef]
- Chen, M.; Wang, S.; Sun, R.; Yang, X.; Wu, X.; Gao, Y.; Xiao, B.; Xu, L.-Y.; Shao, Y.; Xiao, B.; et al. High-performance binary all-polymer solar cells enabled by a Y-derivative pendant random-copolymerized polymer acceptor with a broad donor–acceptor matching tolerance. J. Mater. Chem. A 2024, 12, 7754–7764. [Google Scholar] [CrossRef]
- Wang, J.; Wang, Y.; Xian, K.; Qiao, J.; Chen, Z.; Bi, P.; Zhang, T.; Zheng, Z.; Hao, X.; Ye, L.; et al. Regulating Phase Separation Kinetics for High-Efficiency and Mechanically Robust All-Polymer Solar Cells. Adv. Mater. 2023, 36, e2305424. [Google Scholar] [CrossRef] [PubMed]
- Okil, M.; Shaker, A.; Salah, M.M.; Abdolkader, T.M.; Ahmed, I.S. Investigation of Polymer/Si Thin Film Tandem Solar Cell Using TCAD Numerical Simulation. Polymers 2023, 15, 2049. [Google Scholar] [CrossRef] [PubMed]
- Salem, M.S.; Shaker, A.; Salah, M.M. Device Modeling of Efficient PBDB-T:PZT-Based All-Polymer Solar Cell: Role of Band Alignment. Polymers 2023, 15, 869. [Google Scholar] [CrossRef] [PubMed]
- Alanazi, T.I.; El Sabbagh, M. Proposal and Design of Flexible All-Polymer/CIGS Tandem Solar Cell. Polymers 2023, 15, 1823. [Google Scholar] [CrossRef] [PubMed]
- Hwang, S.; Yasuda, T. Indoor photovoltaic energy harvesting based on semiconducting π-conjugated polymers and oligomeric materials toward future IoT applications. Polym. J. 2022, 55, 297–316. [Google Scholar] [CrossRef]
- Ding, Z.; Zhao, R.; Yu, Y.; Liu, J. All-polymer indoor photovoltaics with high open-circuit voltage. J. Mater. Chem. A 2019, 7, 26533–26539. [Google Scholar] [CrossRef]
- Chau, H.D.; Park, S.H.; Park, J.Y.; Kwak, H.; Park, C.Y.; Son, J.H.; Woo, H.Y.; Chae, W.; Cho, M.J.; Choi, D.H. Complementary Absorption Pseudo-Ternary Blend Containing a Y-Series Block Copolymer Acceptor for Indoor and Outdoor All-Polymer Photovoltaics. Sol. RRL 2023, 8, 2300871. [Google Scholar] [CrossRef]
- Wang, B.; Lai, W.; Liang, S.; Wang, Y.; Wang, C.; Xiao, C.; Li, W. All-polymer solar cells based on wide bandgap polymerized non-fused electron acceptors for indoor photovoltaics. J. Mater. Chem. C 2023, 11, 16049–16055. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, N.; Wang, Y.; Zhang, J.; Liu, J.; Wang, L. All-polymer indoor photovoltaic modules. iScience 2021, 24, 103104. [Google Scholar] [CrossRef]
- Qaid, S.M.H.; Shaker, A.; Okil, M.; Alkadi, M.; Ahmed, A.A.A.; Zein, W. Optimizing CBTSSe solar cells for indoor applications through numerical simulation. Opt. Quantum Electron. 2023, 55, 1245. [Google Scholar] [CrossRef]
- Biswas, S.; You, Y.; Vincent, P.; Bae, J.; Shim, J.W.; Kim, H. Organic tandem solar cells under indoor light illumination. Prog. Photovolt. Res. Appl. 2020, 28, 946–955. [Google Scholar] [CrossRef]
- Qaid, S.M.H.; Shaker, A.; Okil, M.; Gontrand, C.; Alkadi, M.; Ghaithan, H.M.; Salah, M.M. Optoelectronic Device Modeling and Simulation of Selenium-Based Solar Cells under LED Illumination. Crystals 2023, 13, 1668. [Google Scholar] [CrossRef]
- Alanazi, T.I. TCAD Device Simulation of All-Polymer Solar Cells for Indoor Applications: Potential for Tandem vs. Single Junction Cells. Polymers 2023, 15, 2217. [Google Scholar] [CrossRef] [PubMed]
- Xie, L.; Song, W.; Ge, J.; Tang, B.; Zhang, X.; Wu, T.; Ge, Z. Recent progress of organic photovoltaics for indoor energy harvesting. Nano Energy 2021, 82, 105770. [Google Scholar] [CrossRef]
- Cui, Y.; Wang, Y.; Bergqvist, J.; Yao, H.; Xu, Y.; Gao, B.; Yang, C.; Zhang, S.; Inganäs, O.; Gao, F.; et al. Wide-gap non-fullerene acceptor enabling high-performance organic photovoltaic cells for indoor applications. Nat. Energy 2019, 4, 768–775. [Google Scholar] [CrossRef]
- Vandewal, K.; Benduhn, J.; Nikolis, V.C. How to determine optical gaps and voltage losses in organic photovoltaic materials. Sustain. Energy Fuels 2018, 2, 538–544. [Google Scholar] [CrossRef]
- Lee, H.K.H.; Li, Z.; Durrant, J.R.; Tsoi, W.C. Is organic photovoltaics promising for indoor applications? Appl. Phys. Lett. 2016, 108, 253301. [Google Scholar] [CrossRef]
- Burgelman, M.; Decock, K.; Khelifi, S.; Abass, A. Advanced electrical simulation of thin film solar cells. Thin Solid Films 2013, 535, 296–301. [Google Scholar] [CrossRef]
- Salem, M.S.; Okil, M.; Shaker, A.; Albaker, A.; Alturki, M. Design of n-i-p and p-i-n Sb2Se3 solar cells: Role of band alignment. J. Phys. Energy 2023, 5, 045007. [Google Scholar] [CrossRef]
- Burgelman, M.; Decock, K.; Niemegeers, A.; Verschraegen, J.; Degrave, S. SCAPS Manual; University of Ghent: Ghent, Belgium, 2016. [Google Scholar]
- Quintero-Bermudez, R.; Kirman, J.; Ma, D.; Sargent, E.H.; Quintero-Torres, R. Mechanisms of LiF Interlayer Enhancements of Perovskite Light-Emitting Diodes. J. Phys. Chem. Lett. 2020, 11, 4213–4220. [Google Scholar] [CrossRef] [PubMed]
- Jin, Y.; Ding, X.; Reynaert, J.; Arkhipov, V.; Borghs, G.; Heremans, P.; Van der Auweraer, M. Role of LiF in polymer light-emitting diodes with LiF-modified cathodes. Org. Electron. 2004, 5, 271–281. [Google Scholar] [CrossRef]
- Salem, M.S.; Shaker, A.; Al-Bagawia, A.H.; Aleid, G.M.; Othman, M.S.; Alshammari, M.T.; Fedawy, M. Narrowband Near-Infrared Perovskite/Organic Photodetector: TCAD Numerical Simulation. Crystals 2022, 12, 1033. [Google Scholar] [CrossRef]
- Deswal, V.; Kaushik, S.; Kundara, R.; Baghel, S. Numerical simulation of highly efficient Cs2AgInBr6-based double perovskite solar cell using SCAPS 1-D. Mater. Sci. Eng. B 2024, 299, 117041. [Google Scholar] [CrossRef]
- Anrango-Camacho, C.; Pavón-Ipiales, K.; Frontana-Uribe, B.A.; Palma-Cando, A. Recent Advances in Hole-Transporting Layers for Organic Solar Cells. Nanomaterials 2022, 12, 443. [Google Scholar] [CrossRef] [PubMed]
- Wang, Q.; Lin, Z.; Su, J.; Hu, Z.; Chang, J.; Hao, Y. Recent progress of inorganic hole transport materials for efficient and stable perovskite solar cells. Nano Sel. 2021, 2, 1055–1080. [Google Scholar] [CrossRef]
- Bakr, Z.H.; Wali, Q.; Fakharuddin, A.; Schmidt-Mende, L.; Brown, T.M.; Jose, R. Advances in hole transport materials engineering for stable and efficient perovskite solar cells. Nano Energy 2017, 34, 271–305. [Google Scholar] [CrossRef]
- Hossain, M.K.; Rubel, M.H.K.; Toki, G.F.I.; Alam, I.; Rahman, F.; Bencherif, H. Effect of Various Electron and Hole Transport Layers on the Performance of CsPbI3-Based Perovskite Solar Cells: A Numerical Investigation in DFT, SCAPS-1 D, and wxAMPS Frameworks. ACS Omega 2022, 7, 43210–43230. [Google Scholar] [CrossRef]
- Salah, M.M.; Abouelatta, M.; Shaker, A.; Hassan, K.M.; Saeed, A. A comprehensive simulation study of hybrid halide perovskite solar cell with copper oxide as HTM. Semicond. Sci. Technol. 2019, 34, 115009. [Google Scholar] [CrossRef]
- Noman, M.; Khan, A.H.H.; Jan, S.T. Interface engineering and defect passivation for enhanced hole extraction, ion migration, and optimal charge dynamics in both lead-based and lead-free perovskite solar cells. Sci. Rep. 2024, 14, 5449. [Google Scholar] [CrossRef]
- Roy, P.; Khare, A.; Tiwari, S.; Mahapatra, A. Revealing the Role of Band Offsets on the Charge Carrier Dynamics of CsSn0.5Ge0.5I3-Based Perovskite Solar Cell: A Theoretical Study. Energy Technol. 2023, 11, 2300253. [Google Scholar] [CrossRef]
- Okil, M.; Shaker, A.; Ahmed, I.S.; Abdolkader, T.M.; Salem, M.S. Design and analysis of Sb2S3/Si thin film tandem solar cell. Sol. Energy Mater. Sol. Cells 2023, 253, 112210. [Google Scholar] [CrossRef]
- Song, Z.; McElvany, C.L.; Phillips, A.B.; Celik, I.; Krantz, P.W.; Watthage, S.C.; Liyanage, G.K.; Apul, D.; Heben, M.J. A technoeconomic analysis of perovskite solar module manufacturing with low-cost materials and techniques. Energy Environ. Sci. 2017, 10, 1297–1305. [Google Scholar] [CrossRef]
- Khattak, Y.H.; Baig, F.; Soucase, B.M.; Beg, S.; Gillani, S.R.; Ahmed, S. Efficiency enhancement of novel CNTS/ZnS/Zn (O, S) thin film solar cell. Optik 2018, 171, 453–462. [Google Scholar] [CrossRef]
- Ahmed, A.; Riaz, K.; Mehmood, H.; Tauqeer, T.; Ahmad, Z. Performance optimization of CH3NH3Pb(I1-xBrx)3 based perovskite solar cells by comparing different ETL materials through conduction band offset engineering. Opt. Mater. 2020, 105, 109897. [Google Scholar] [CrossRef]
- Rai, N.; Rai, S.; Singh, P.K.; Lohia, P.; Dwivedi, D.K. Analysis of various ETL materials for an efficient perovskite solar cell by numerical simulation. J. Mater. Sci. Mater. Electron. 2020, 31, 16269–16280. [Google Scholar] [CrossRef]
- Chen, C.; Liu, X.; Li, K.; Lu, S.; Wang, S.; Li, S.; Lu, Y.; He, J.; Zheng, J.; Lin, X.; et al. High-efficient Sb2Se3 solar cell using ZnxCd1-xS n-type layer. Appl. Phys. Lett. 2021, 118, 172103. [Google Scholar] [CrossRef]
- Minemoto, T.; Murata, M. Theoretical analysis on effect of band offsets in perovskite solar cells. Sol. Energy Mater. Sol. Cells 2015, 133, 8–14. [Google Scholar] [CrossRef]








| Parameters | PEDOT:PSS | CD1:PBN-21 |
|---|---|---|
| Thickness, t (nm) | 40 | 110 |
| ELUMO (eV) | −3.40 | −3.48 |
| EHOMO (eV) | −5.00 | −5.28 |
| Relative permittivity, εr | 3.0 | 3.0 |
| Electron/Hole mobility, (μn/μp) (cm2/Vs) | 5.0 × 10−4 /5.0 × 10−4 | 2.77 × 10−4 /4.46 × 10−4 |
| Effective DOS in CB, Nc (cm−3) | 2.2 × 1018 | 1.0 × 1019 |
| Effective DOS in VB, Nv (cm−3) | 1.8 × 1019 | 1.0 × 1019 |
| Donor level, ND (cm−3) | - | - |
| Acceptor level, NA (cm−3) | 1.0 × 1019 | - |
| Reference | [34,35] | [20] |
| Parameters | Bulk Defects | Interface Defects | |
|---|---|---|---|
| CD1:PBN-21 | PEDOT:PSS | HTL/Polymer | |
| Defect type | Single Acceptor | Single Acceptor | Acceptor |
| Electron/Hole capture cross-section (cm2) | 1 × 10−15 /1 × 10−15 | 1 × 10−15 /1 × 10−15 | 1 × 10−15 /1 × 10−15 |
| Trap energy level above Ev (eV) | 0.6 | 0.6 | 0.6 |
| Concentration (cm−3) | 1 × 1012 | 1 × 1014 | 1 × 1010 |
| Contact | Material | WF (eV) | SRVn (cm/s) | SRVp (cm/s) |
|---|---|---|---|---|
| Front | ITO | 4.70 | 1 × 107 | 1 × 106 |
| Rear | LiF/Al | 3.84 | 1 × 106 | 1 × 107 |
| PV Parameters | VOC (V) | JSC (µA/cm2) | FF (%) | PCE (%) |
|---|---|---|---|---|
| Experimental [20] | 1.10 | 73.90 | 62.80 | 16.75 |
| This work | 1.11 | 73.67 | 62.03 | 16.75 |
| Parameters | NiO | CuI | CuO | CBTS |
|---|---|---|---|---|
| t (nm) | 40 | 40 | 40 | 40 |
| Eg (eV) | 3.8 | 3.1 | 2.1 | 1.9 |
| χ (eV) | 1.46 | 2.1 | 3.2 | 3.6 |
| VBO (eV) | −0.02 | −0.08 | 0.02 | 0.22 |
| φp (eV) | 0.56 | 0.5 | 0.6 | 0.8 |
| εr | 10.7 | 6.5 | 7.11 | 5.4 |
| μn/μp (cm2/Vs) | 12/2.8 | 100/43.9 | 3.4/3.4 | 30/10 |
| Nc/Nv (cm−3) | 2.8 × 1019 /1 × 1019 | 2.8 × 1019 /1 × 1019 | 2.2 × 1018 /1.8 × 1018 | 2.2 × 1018 /1.8 × 1019 |
| Reference | [30,39] | [30,39] | [13,40] | [13] |
| HTL | φp (eV) | VBO (eV) | VOC (V) | JSC (µA/cm2) | FF (%) | PCE (%) |
|---|---|---|---|---|---|---|
| PEDOT:PSS | 0.30 | −0.28 | 1.11 | 73.67 | 62.03 | 16.75 |
| CuI | 0.50 | −0.08 | 1.13 | 90.65 | 77.72 | 26.22 |
| NiO | 0.56 | −0.02 | 1.14 | 91.53 | 79.73 | 27.19 |
| CuO | 0.60 | 0.02 | 1.10 | 86.80 | 77.72 | 24.38 |
| CBTS | 0.80 | 0.22 | 0.94 | 78.18 | 77.37 | 18.64 |
| Parameters | ZnS | ZnOS | CdZnS | ZnO |
|---|---|---|---|---|
| t (nm) | 40 | 40 | 40 | 40 |
| Eg (eV) | 3.6 | 2.83 | 3.18 | 3.3 |
| χ (eV) | 3.44 | 3.6 | 3.71 | 3.9 |
| εr | 9 | 9 | 10 | 9 |
| μn/μp (cm2/Vs) | 100/25 | 100/25 | 340/50 | 50/5 |
| Nc/Nv (cm−3) | 1.8 × 1019 /2.4 × 1019 | 2.2 × 1018 /1.8 × 1018 | 2.5 × 1018 /2.5 × 1019 | 1 × 1019 /1 × 1019 |
| Reference | [45] | [40] | [46] | [47] |
| ETL | φn (eV) | CBO (eV) | VOC (V) | JSC (µA/cm2) | FF (%) | PCE (%) |
|---|---|---|---|---|---|---|
| ZnS | 0.56 | 0.04 | 1.32 | 92.32 | 82.34 | 33.02 |
| ZnOS | 0.40 | −0.12 | 1.31 | 91.27 | 77.72 | 30.64 |
| CdZnS | 0.29 | −0.23 | 1.27 | 90.91 | 71.62 | 27.18 |
| ZnO | 0.10 | −0.42 | 1.05 | 90.16 | 69.68 | 21.75 |
| Cell Configuration | VOC (V) | JSC (µA/cm2) | FF (%) | PCE (%) | Enhancement Ratio (%) |
|---|---|---|---|---|---|
| PEDOT:PSS/absorber/ETL-free | 1.11 | 73.67 | 62.03 | 16.75 | - |
| NiO/absorber/ETL-free | 1.14 | 91.53 | 79.73 | 27.19 | 62.33 |
| ZnS/absorber/HTL-free | 1.32 | 92.32 | 82.34 | 33.02 | 97.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salem, M.S.; Okil, M.; Shaker, A.; Abouelatta, M.; Aledaily, A.N.; Al-Dhlan, K.A.; Alshammari, M.T.; Salah, M.M.; El Sabbagh, M. Optimizing Transport Carrier Free All-Polymer Solar Cells for Indoor Applications: TCAD Simulation under White LED Illumination. Polymers 2024, 16, 1412. https://doi.org/10.3390/polym16101412
Salem MS, Okil M, Shaker A, Abouelatta M, Aledaily AN, Al-Dhlan KA, Alshammari MT, Salah MM, El Sabbagh M. Optimizing Transport Carrier Free All-Polymer Solar Cells for Indoor Applications: TCAD Simulation under White LED Illumination. Polymers. 2024; 16(10):1412. https://doi.org/10.3390/polym16101412
Chicago/Turabian StyleSalem, Marwa S., Mohamed Okil, Ahmed Shaker, Mohamed Abouelatta, Arwa N. Aledaily, Kawther A. Al-Dhlan, Mohammad T. Alshammari, Mostafa M. Salah, and Mona El Sabbagh. 2024. "Optimizing Transport Carrier Free All-Polymer Solar Cells for Indoor Applications: TCAD Simulation under White LED Illumination" Polymers 16, no. 10: 1412. https://doi.org/10.3390/polym16101412
APA StyleSalem, M. S., Okil, M., Shaker, A., Abouelatta, M., Aledaily, A. N., Al-Dhlan, K. A., Alshammari, M. T., Salah, M. M., & El Sabbagh, M. (2024). Optimizing Transport Carrier Free All-Polymer Solar Cells for Indoor Applications: TCAD Simulation under White LED Illumination. Polymers, 16(10), 1412. https://doi.org/10.3390/polym16101412








