Non-Stick Length of Polymer–Polymer Interfaces under Small-Amplitude Oscillatory Shear Measurement
Abstract
1. Introduction
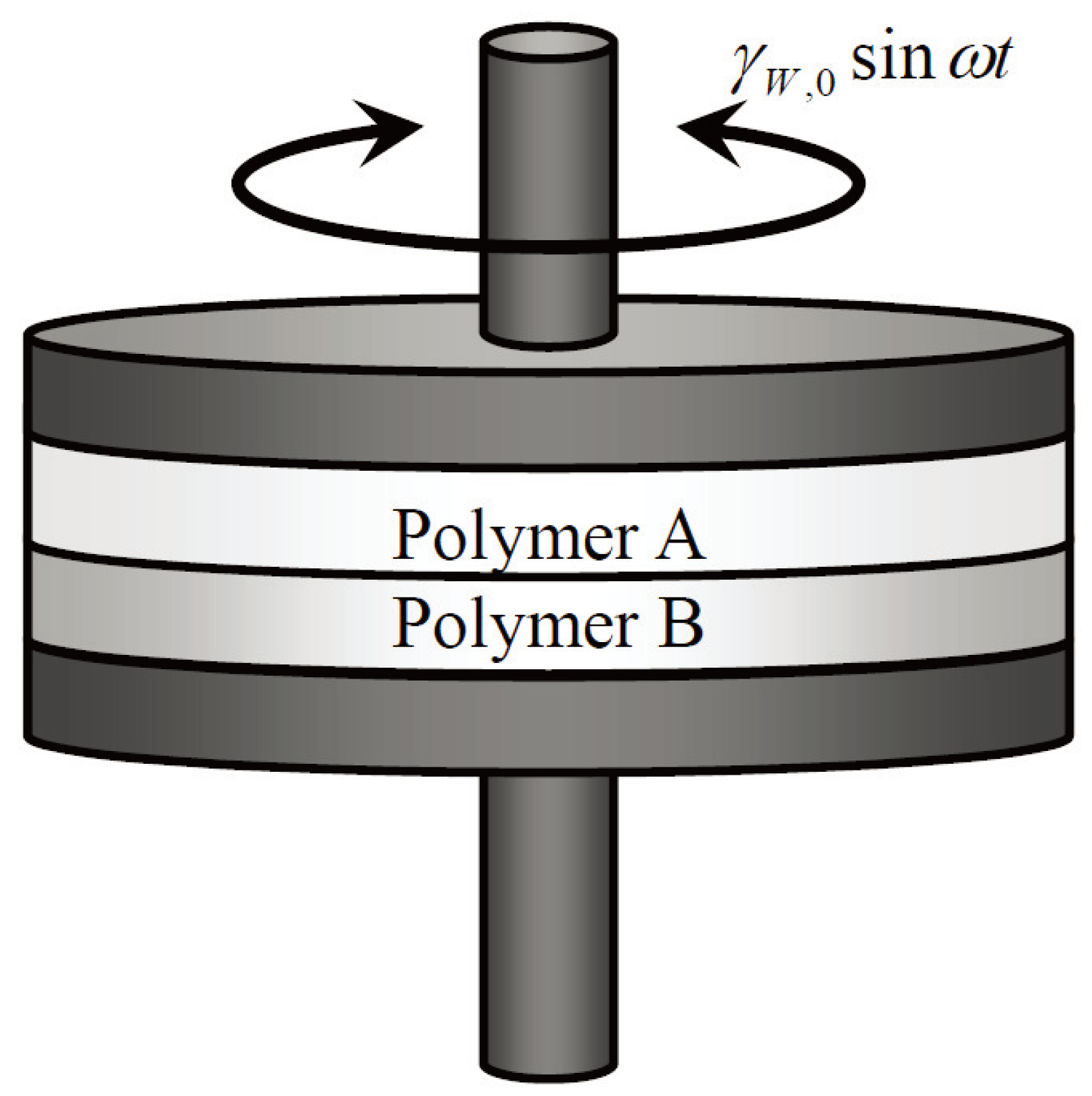
2. Experimental Section
2.1. Materials
2.2. Sample Preparation
2.3. Rheological Measurements
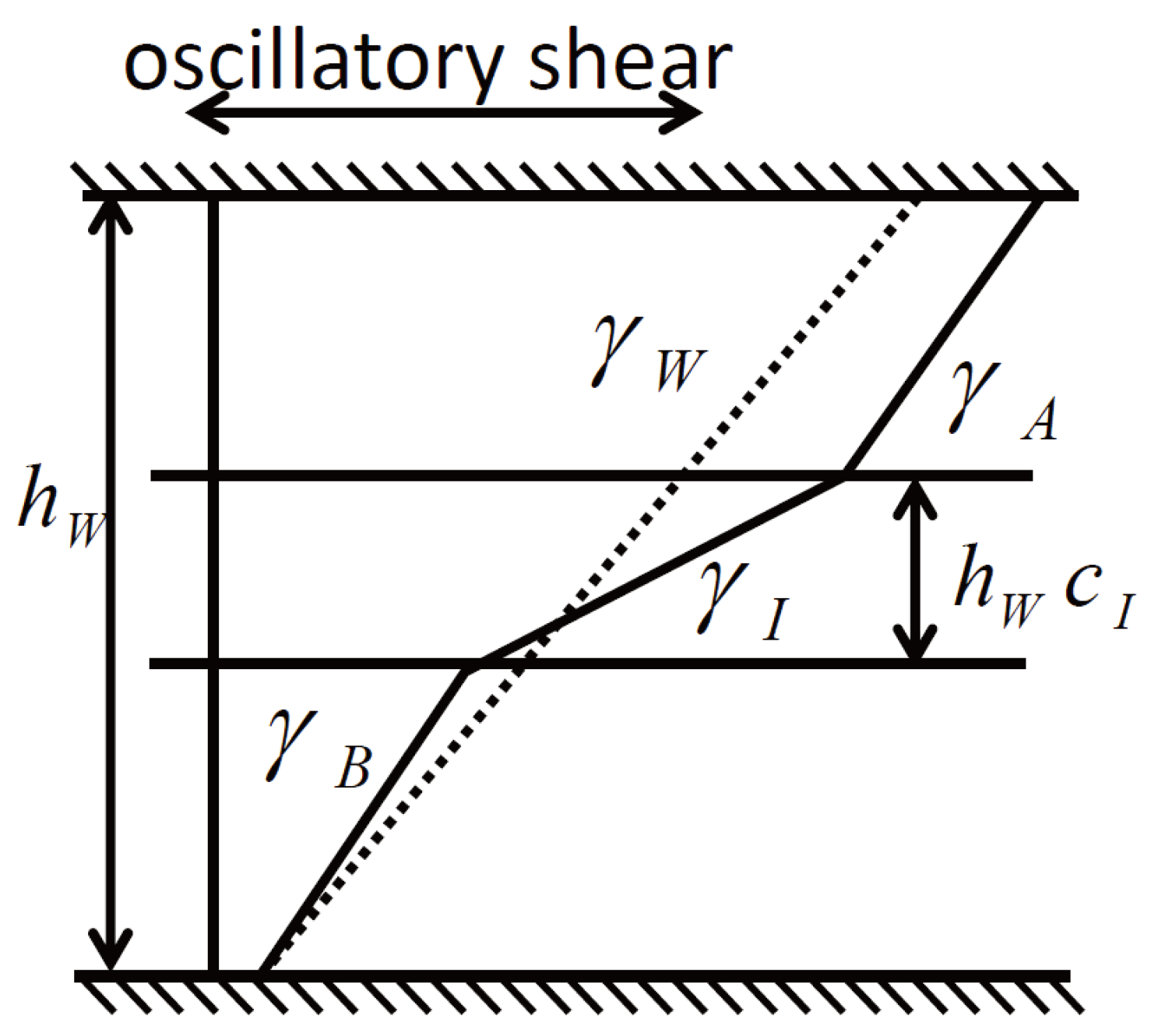
3. Non-Stick Length in Small-Amplitude Oscillatory Shear Response of an Interface
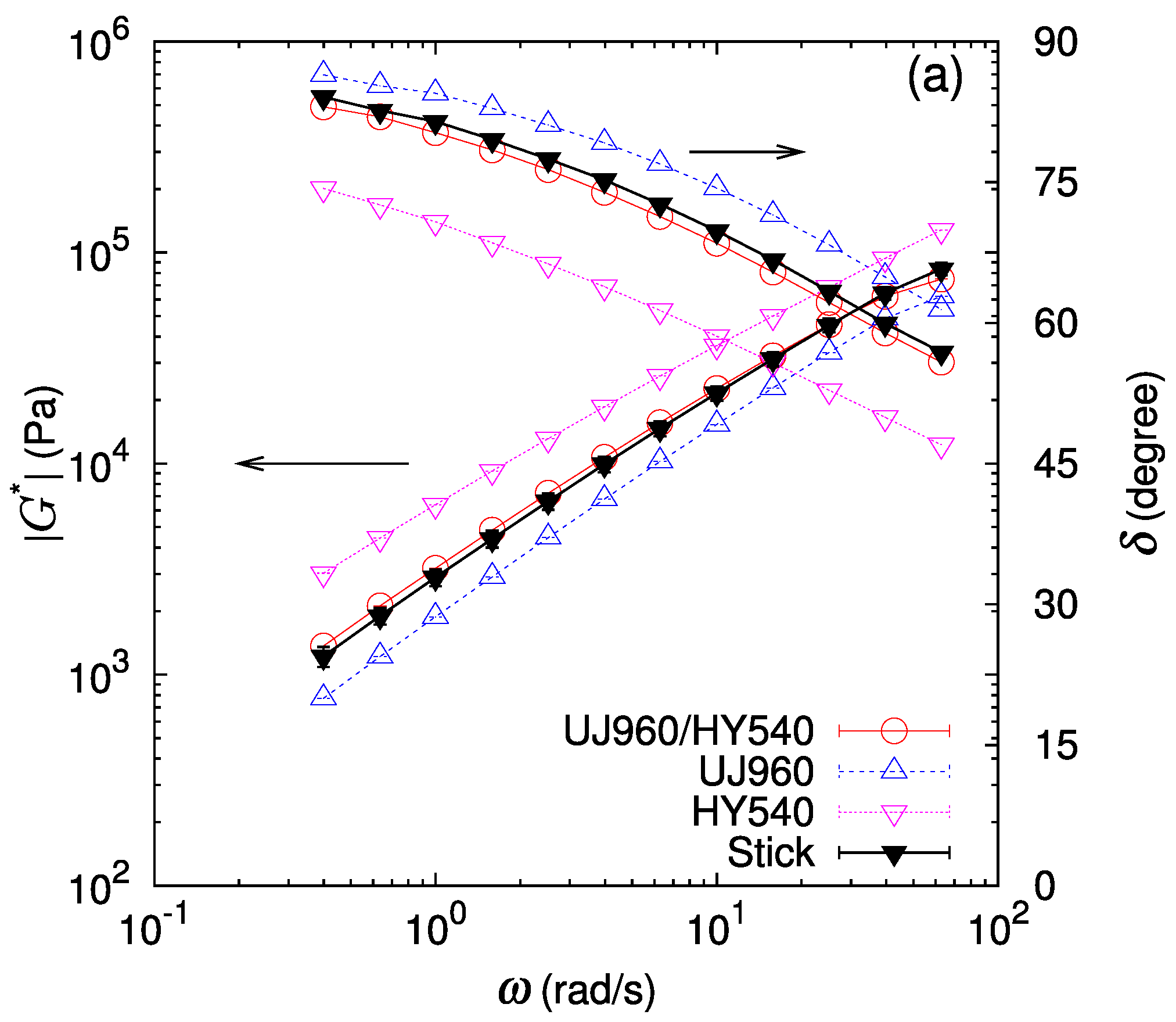
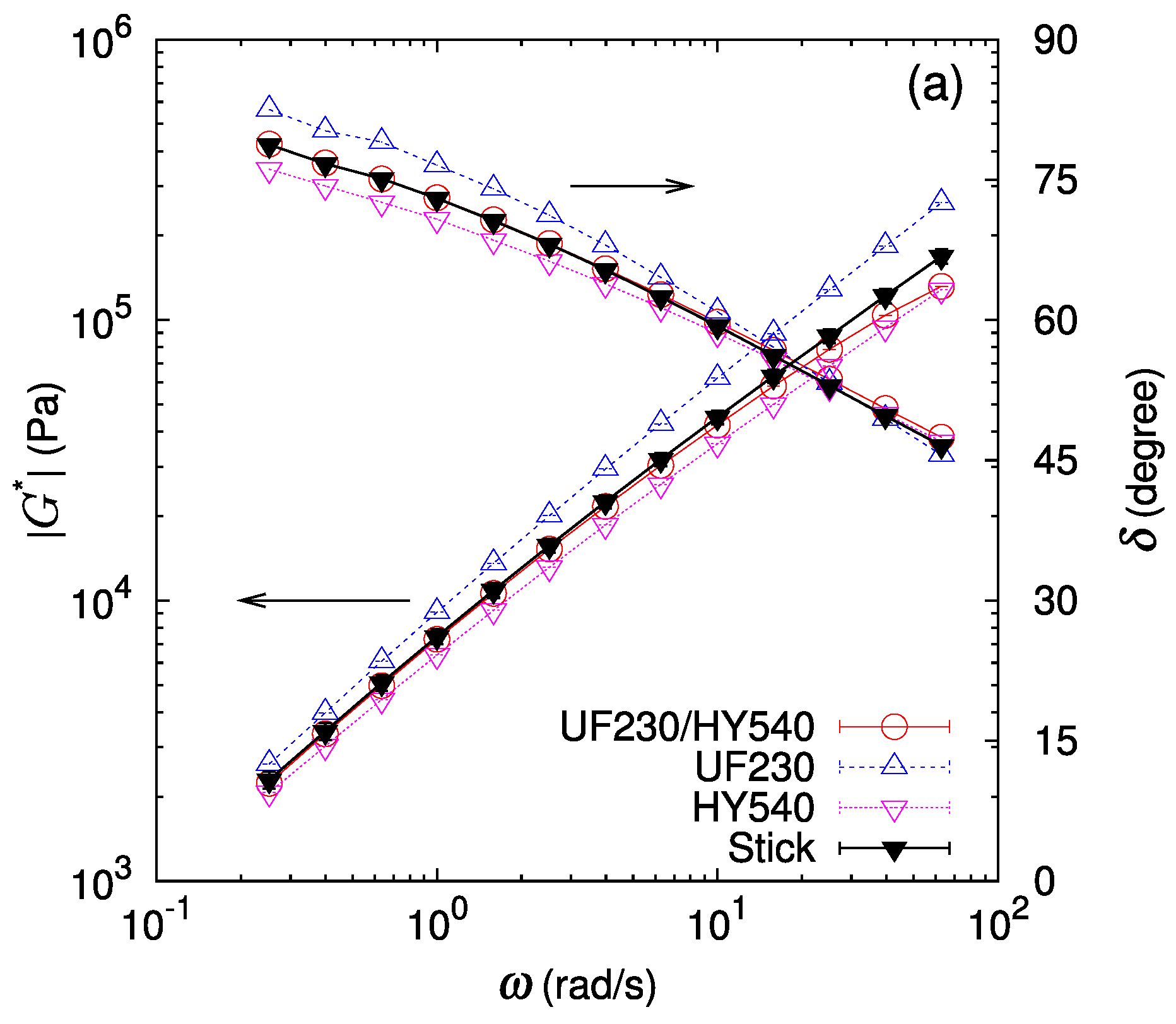
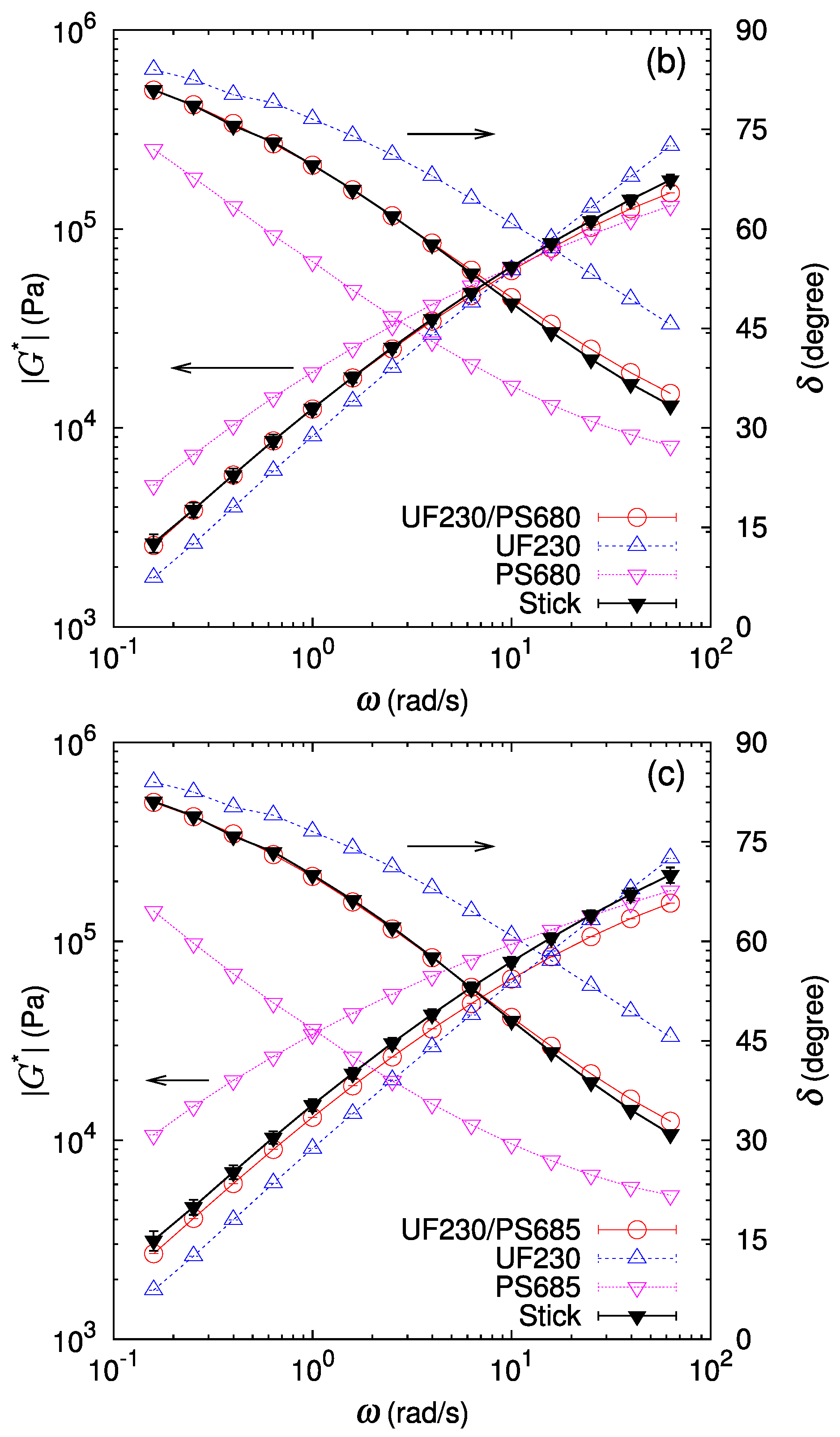
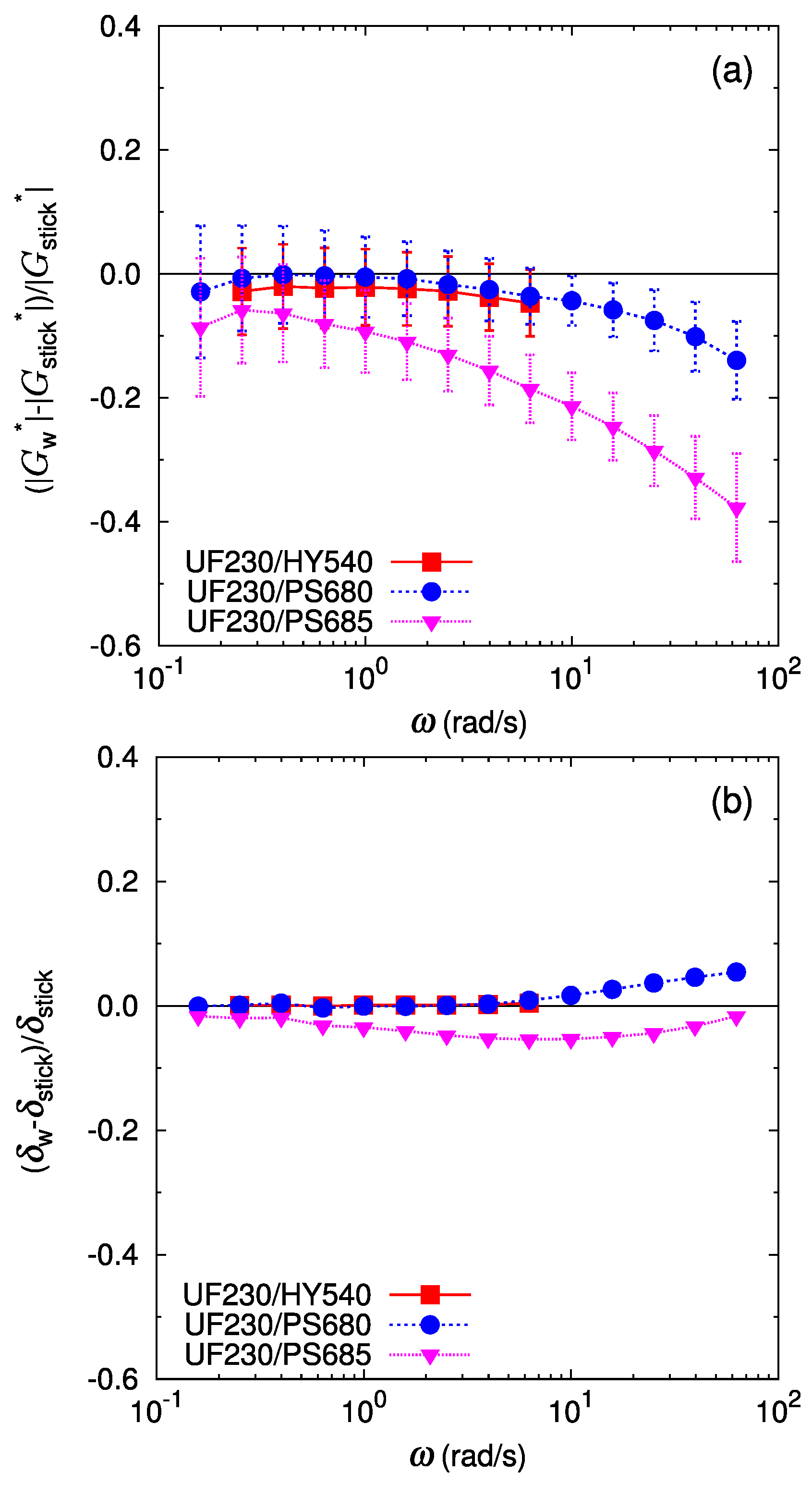
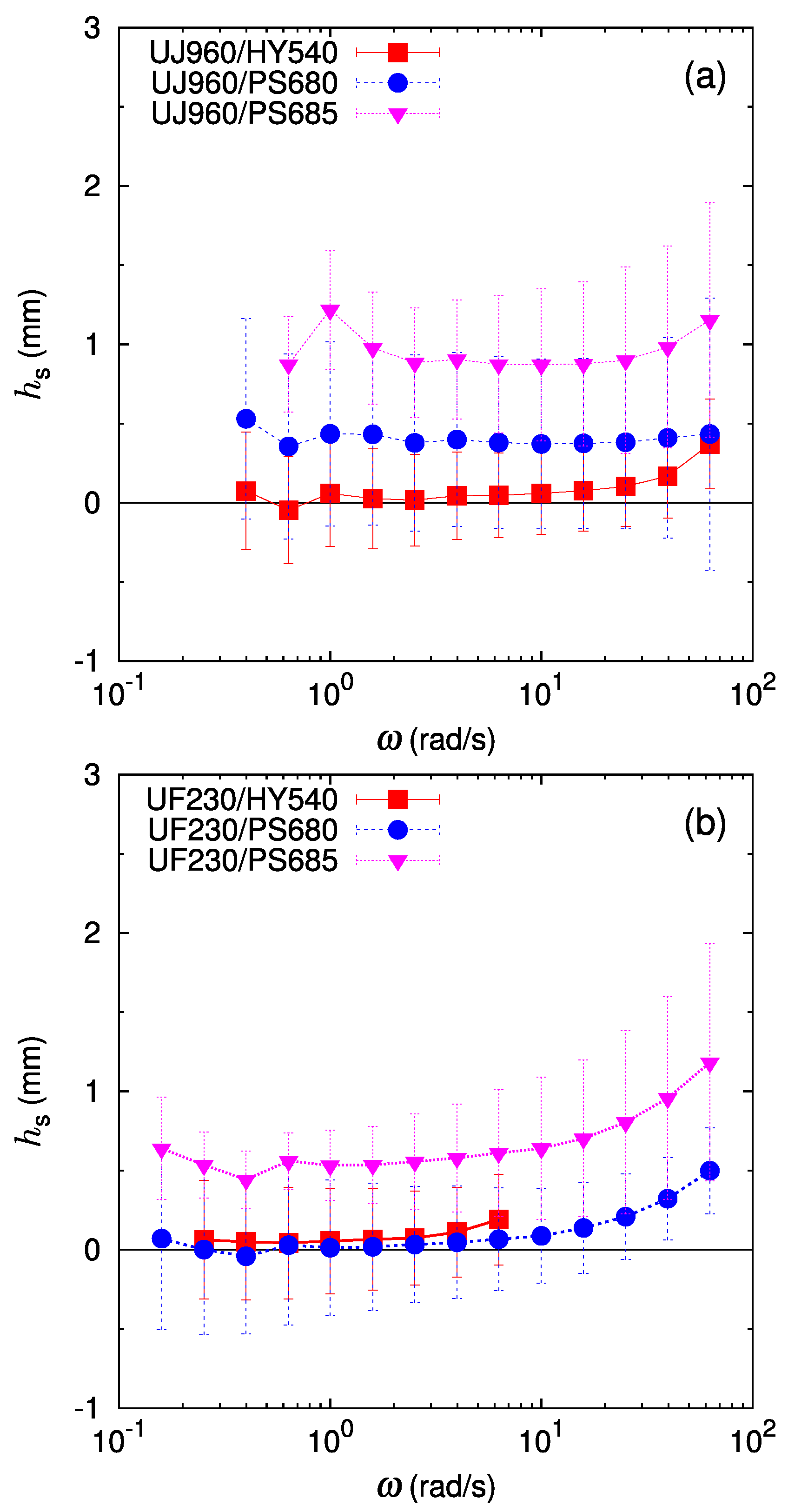
4. Results and Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Boussinesq, J. Existence of a superficial viscosity in the thin transition layer separating one liquid from another contiguous fluid. CR Hehbd. Seanc. Acad. Sci. 1913, 156, 983–989. [Google Scholar]
- Happel, J.; Brenner, H. Low Reynolds Number Hydrodynamics: With Special Applications to Particulate Media (Mechanics of Fluids and Transport Processes); Kluwer: Dordrecht, The Netherlads, 1973. [Google Scholar]
- Yu, W.; Zhou, C. The effect of interfacial viscosity on the droplet dynamics under flow field. J. Polym. Sci. Part B Polym. Phys. 2008, 46, 1505–1514. [Google Scholar] [CrossRef]
- Tanaka, Y.; Sako, T.; Hiraoka, T.; Yamaguchi, M.; Yamaguchi, M. Effect of morphology on shear viscosity for binary blends of polycarbonate and polystyrene. J. Appl. Polym. Sci. 2020, 137, 49516. [Google Scholar] [CrossRef]
- Moonprasith, N.; Nasri, M.S.; Saari, R.A.; Phulkerd, P.; Yamaguchi, M. Viscosity decrease by interfacial slippage between immiscible polymers. Polym. Eng. Sci. 2021, 61, 1096–1103. [Google Scholar] [CrossRef]
- Das, S.; Bhattacharjee, A.; Chakraborty, S. Influence of interfacial slip on the suspension rheology of a dilute emulsion of surfactant-laden deformable drops in linear flows. Phys. Fluids 2018, 30, 032005. [Google Scholar] [CrossRef]
- Wilson, H.J. Instabilities and constitutive modelling. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2006, 364, 3267–3283. [Google Scholar] [CrossRef]
- Zhang, J.; Lodge, T.P.; Macosko, C.W. Interfacial slip reduces polymer–polymer adhesion during coextrusion. J. Rheol. 2006, 50, 41–57. [Google Scholar] [CrossRef]
- Lopes, L.; Silva, A.; Carneiro, O. Multi-material 3D printing: The relevance of materials affinity on the boundary interface performance. Addit. Manuf. 2018, 23, 45–52. [Google Scholar] [CrossRef]
- Fuller, G.G.; Vermant, J. Complex Fluid–Fluid Interfaces: Rheology and Structure. Annu. Rev. Chem. Biomol. Eng. 2012, 3, 519–543. [Google Scholar] [CrossRef]
- Pelipenko, J.; Kristl, J.; Rošic, R.; Baumgartner, S.; Kocbek, P. Interfacial rheology: An overview of measuring techniques and its role in dispersions and electrospinning. Acta Pharm. 2012, 62, 123–140. [Google Scholar] [CrossRef]
- Prasad, V.; Koehler, S.A.; Weeks, E.R. Two-Particle Microrheology of Quasi-2D Viscous Systems. Phys. Rev. Lett. 2006, 97, 176001. [Google Scholar] [CrossRef] [PubMed]
- Song, Y.; Dai, L.L. Two-Particle Interfacial Microrheology at Polymer–Polymer Interfaces. Langmuir 2010, 26, 13044–13047. [Google Scholar] [CrossRef] [PubMed]
- Moghaddam, R.K.; Roy, T.; Natale, G. Interfacial microrheology: Characteristics of homogeneous and heterogeneous interfaces. Rheol. Acta 2022, 61, 733–744. [Google Scholar] [CrossRef]
- Tanaka, K.; Takahara, A.; Kajiyama, T. Rheological Analysis of Surface Relaxation Process of Monodisperse Polystyrene Films. Macromolecules 2000, 33, 7588–7593. [Google Scholar] [CrossRef]
- Wang, D.; Fujinami, S.; Nakajima, K.; Nishi, T. True Surface Topography and Nanomechanical Mapping Measurements on Block Copolymers with Atomic Force Microscopy. Macromolecules 2010, 43, 3169–3172. [Google Scholar] [CrossRef]
- Zhao, R.; Macosko, C.W. Slip at polymer–polymer interfaces: Rheological measurements on coextruded multilayers. J. Rheol. 2002, 46, 145–167. [Google Scholar] [CrossRef]
- Lam, Y.C.; Jiang, L.; Yue, C.Y.; Tam, K.C.; Li, L.; Hu, X. Interfacial slip between polymer melts studied by confocal microscopy and rheological measurements. J. Rheol. 2003, 47, 795–807. [Google Scholar] [CrossRef]
- Jiang, L.; Lam, Y.C.; Zhang, J. Rheological properties and interfacial slip of a multilayer structure under dynamic shear. J. Polym. Sci. B Polym. Phys. 2005, 43, 2683–2693. [Google Scholar] [CrossRef]
- Lee, P.C.; Park, H.E.; Morse, D.C.; Macosko, C.W. Polymer–polymer interfacial slip in multilayered films. J. Rheol. 2009, 53, 893–915. [Google Scholar] [CrossRef]
- Park, H.E.; Lee, P.C.; Macosko, C.W. Polymer–polymer interfacial slip by direct visualization and by stress reduction. J. Rheol. 2010, 54, 1207–1218. [Google Scholar]
- Nakayama, Y.; Kataoka, K.; Kajiwara, T. Dynamic shear responses of polymer–polymer interfaces. Nihon Reoroji Gakkaishi (J. Soc. Rheol. Jpn.) 2013, 40, 245–252. [Google Scholar] [CrossRef][Green Version]
- Beuguel, Q.; Guinault, A.; Léger, L.; Restagno, F.; Sollogoub, C.; Miquelard-Garnier, G. Nanorheology with a conventional rheometer: Probing the interfacial properties in compatibilized multinanolayer polymer films. ACS Macro Lett. 2019, 8, 1309–1315. [Google Scholar] [CrossRef] [PubMed]
- Beuguel, Q.; Guinault, A.; Chinesta, F.; Sollogoub, C.; Miquelard-Garnier, G. Modeling of the rheological properties of multinanolayer films in the presence of compatibilized interphase. J. Rheol. 2020, 64, 981–989. [Google Scholar] [CrossRef]
- Zhao, R.; Macosko, C.W. Polymer–polymer mutual diffusion via rheology of coextruded multilayers. AIChE J. 2007, 53, 978–985. [Google Scholar] [CrossRef]
- Silva, J.; Maia, J.a.M.; Huang, R.; Meltzer, D.; Cox, M.; Andrade, R. Interfacial rheology of coextruded elastomeric and amorphous glass thermoplastic polyurethanes. Rheol. Acta 2012, 51, 947–957. [Google Scholar] [CrossRef]
- Qiu, H.; Bousmina, M. New technique allowing the quantification of diffusion at polymer/polymer interfaces using rheological analysis: Theoretical and experimental results. J. Rheol. 1999, 43, 551–568. [Google Scholar] [CrossRef]
- Levitt, L.; Macosko, C.W.; Schweizer, T.; Meissner, J. Extensional rheometry of polymer multilayers: A sensitive probe of interfaces. J. Rheol. 1997, 41, 671–685. [Google Scholar] [CrossRef]
- Jordan, A.M.; Lee, B.; Kim, K.; Ludtke, E.; Lhost, O.; Jaffer, S.A.; Bates, F.S.; Macosko, C.W. Rheology of polymer multilayers: Slip in shear, hardening in extension. J. Rheol. 2019, 63, 751–761. [Google Scholar] [CrossRef]
- Gholami, F.; Pakzad, L.; Behzadfar, E. Morphological, interfacial and rheological properties in multilayer polymers: A review. Polymer 2020, 208, 122950. [Google Scholar] [CrossRef]
- Dmochowska, A.; Peixinho, J.; Sollogoub, C.; Miquelard-Garnier, G. Extensional Viscosity of Immiscible Polymer Multi-Nanolayer Films: Signature of the Interphase. Macromolecules 2023, 56, 6222–6231. [Google Scholar] [CrossRef]
- Komuro, R.; Sukumaran, S.K.; Sugimoto, M.; Koyama, K. Slip at the interface between immiscible polymer melts I: Method to measure slip. Rheol. Acta 2014, 53, 23–30. [Google Scholar] [CrossRef]
- Lu, B.; Zhang, H.; Maazouz, A.; Lamnawar, K. Interfacial phenomena in multi-micro-/nanolayered polymer coextrusion: A review of fundamental and engineering aspects. Polymers 2021, 13, 417. [Google Scholar] [CrossRef] [PubMed]
- Dziadowiec, D.; Matykiewicz, D.; Szostak, M.; Andrzejewski, J. Overview of the Cast Polyolefin Film Extrusion Technology for Multi-Layer Packaging Applications. Materials 2023, 16, 1071. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Lamnawar, K.; Maazouz, A. Understanding of Transient Rheology in Step Shear and Its Implication to Explore Nonlinear Relaxation Dynamics of Interphase in Compatible Polymer Multi-microlayered Systems. Ind. Eng. Chem. Res. 2018, 57, 8093–8104. [Google Scholar] [CrossRef]
- Lu, B.; Bondon, A.; Touil, I.; Zhang, H.; Alcouffe, P.; Pruvost, S.; Liu, C.; Maazouz, A.; Lamnawar, K. Role of the Macromolecular Architecture of Copolymers at Layer–Layer Interfaces of Multilayered Polymer Films: A Combined Morphological and Rheological Investigation. Ind. Eng. Chem. Res. 2020, 59, 22144–22154. [Google Scholar] [CrossRef]
- Li, Y.; Guo, H. Nonequilibrium Behaviors of Entangled Diblock Copolymers at the Entangled Polymer–Polymer Interface under Steady Shear Flow. J. Phys. Chem. B 2023, 127, 9642–9655. [Google Scholar] [CrossRef] [PubMed]
- Paiva, F.L.; Secchi, A.R.; Calado, V.; Maia, J.; Khani, S. Slip and momentum transfer mechanisms mediated by Janus rods at polymer interfaces. Soft Matter 2020, 16, 6662–6672. [Google Scholar] [CrossRef]
- Qiao, H.; Zheng, B.; Zhong, G.; Li, Z.; Cardinaels, R.; Moldenaers, P.; Lamnawar, K.; Maazouz, A.; Liu, C.; Zhang, H. Understanding the rheology of polymer–polymer interfaces covered with Janus nanoparticles: Polymer blends versus particle sandwiched multilayers. Macromolecules 2023, 56, 647–663. [Google Scholar] [CrossRef]
- Saha, S.; Xu, D.; Gersappe, D. Effect of compatibilizers on the structure and dynamics at polymer blend interfaces. Tribol. Lett. 2021, 69, 61. [Google Scholar] [CrossRef]
- Mark, J.E. (Ed.) Physical Properties of Polymers Handbook, 2nd ed.; Springer: New York, NY, USA, 2006. [Google Scholar]
- Brandrup, J.; Immergut, E.H.; Grulke, E.A. (Eds.) Polymer Handbook, 4th ed.; Wiley-Interscience: New York, NY, USA, 1999. [Google Scholar]
- Young, R.J.; Lovell, P.A. Introduction to Polymers, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Kotera, M.; Urushihara, Y.; Izumo, D.; Nishino, T. Interfacial structure of poly-α-olefin laminate by using scanning thermal microscope. Thermochim. Acta 2012, 531, 1–5. [Google Scholar] [CrossRef]
- Kotera, M.; Urushihara, Y.; Izumo, D.; Nishino, T. Interfacial structure of all-polyethylene laminate using scanning thermal microscope and nano-Raman spectroscope. Polymer 2012, 53, 1966–1971. [Google Scholar] [CrossRef]
- Helfand, E.; Tagami, Y. Theory of the interface between immiscible polymers. J. Polym. Sci. B Polym. Lett. 1971, 9, 741–746. [Google Scholar] [CrossRef]
- Helfand, E.; Tagami, Y. Theory of the Interface between Immiscible Polymers. II. J. Chem. Phys. 1972, 56, 3592–3601. [Google Scholar] [CrossRef]
- Jiang, L.; Lam, Y.C.; Yue, C.Y.; Tam, K.C.; Li, L.; Hu, X. Energy model of the interfacial slip of polymer blends under steady shear. J. Appl. Polym. Sci. 2003, 89, 1464–1470. [Google Scholar] [CrossRef]
- Lam, Y.C.; Yue, C.Y.; Yang, Y.X.; Tam, K.C.; Hu, X. Interfacial properties of polycarbonate/liquid-crystal polymer and polystyrene/high-impact polystyrene polymer pairs under shear deformation. J. Appl. Polym. Sci. 2003, 87, 258–269. [Google Scholar] [CrossRef]
- Lam, Y.C.; Jiang, L.; Li, L.; Yue, C.Y.; Tam, K.C.; Hu, X. Interfacial slip at the thermotropic liquid-crystalline polymer/poly (ethylene naphthalate) interface. J. Polym. Sci. B Polym. Phys. 2004, 42, 302–315. [Google Scholar] [CrossRef]
- de Gennes, P.G. Viscometric flows of tangled polymers. Comptes Rendus Hebd. Des Seances Acad. Des Sci. Ser. B 1979, 288, 219–220. [Google Scholar]


| Polymer | (kg/mol) | |
|---|---|---|
| polystyrene (PS) | ||
| PS680 | 187 | 2.5 |
| PS685 | 247 | 2.4 |
| linear low-density polyethylene (LLDPE) | ||
| UJ960 | 78 | 3.2 |
| UF230 | 265 | 3.7 |
| high-density polyethylene (HDPE) | ||
| HY540 | 301 | 7.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nakayama, Y. Non-Stick Length of Polymer–Polymer Interfaces under Small-Amplitude Oscillatory Shear Measurement. Polymers 2024, 16, 77. https://doi.org/10.3390/polym16010077
Nakayama Y. Non-Stick Length of Polymer–Polymer Interfaces under Small-Amplitude Oscillatory Shear Measurement. Polymers. 2024; 16(1):77. https://doi.org/10.3390/polym16010077
Chicago/Turabian StyleNakayama, Yasuya. 2024. "Non-Stick Length of Polymer–Polymer Interfaces under Small-Amplitude Oscillatory Shear Measurement" Polymers 16, no. 1: 77. https://doi.org/10.3390/polym16010077
APA StyleNakayama, Y. (2024). Non-Stick Length of Polymer–Polymer Interfaces under Small-Amplitude Oscillatory Shear Measurement. Polymers, 16(1), 77. https://doi.org/10.3390/polym16010077






