Gas Permeability through Polyimides: Unraveling the Influence of Free Volume, Intersegmental Distance and Glass Transition Temperature
Abstract
:1. Introduction
2. Materials and Methods
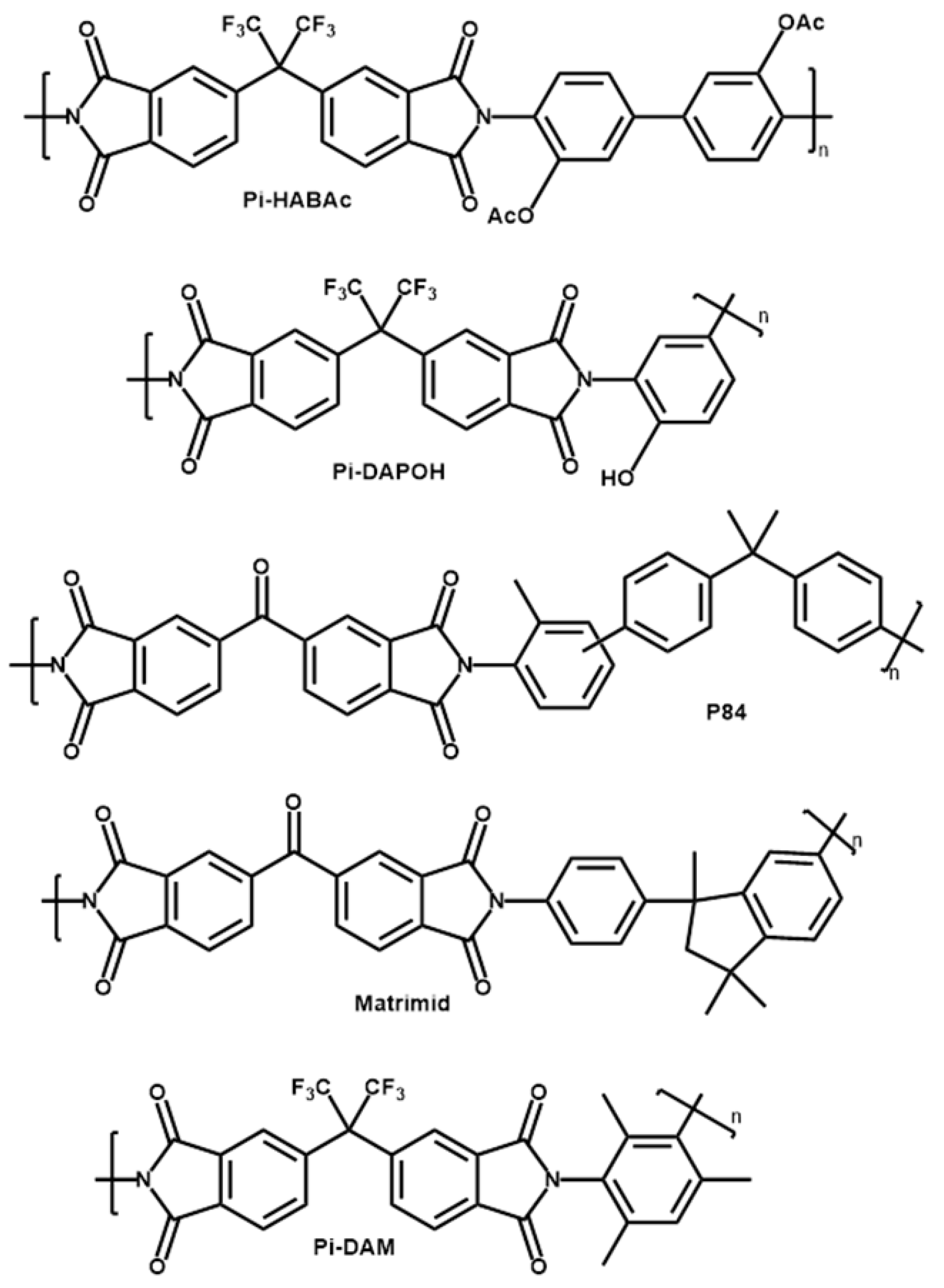
2.1. Synthesis of Matrix Polyimides
2.2. Gas Separation Transport Properties
3. Theory
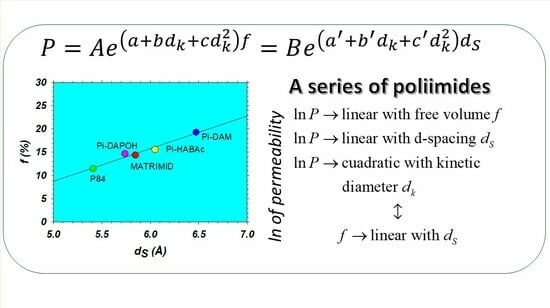
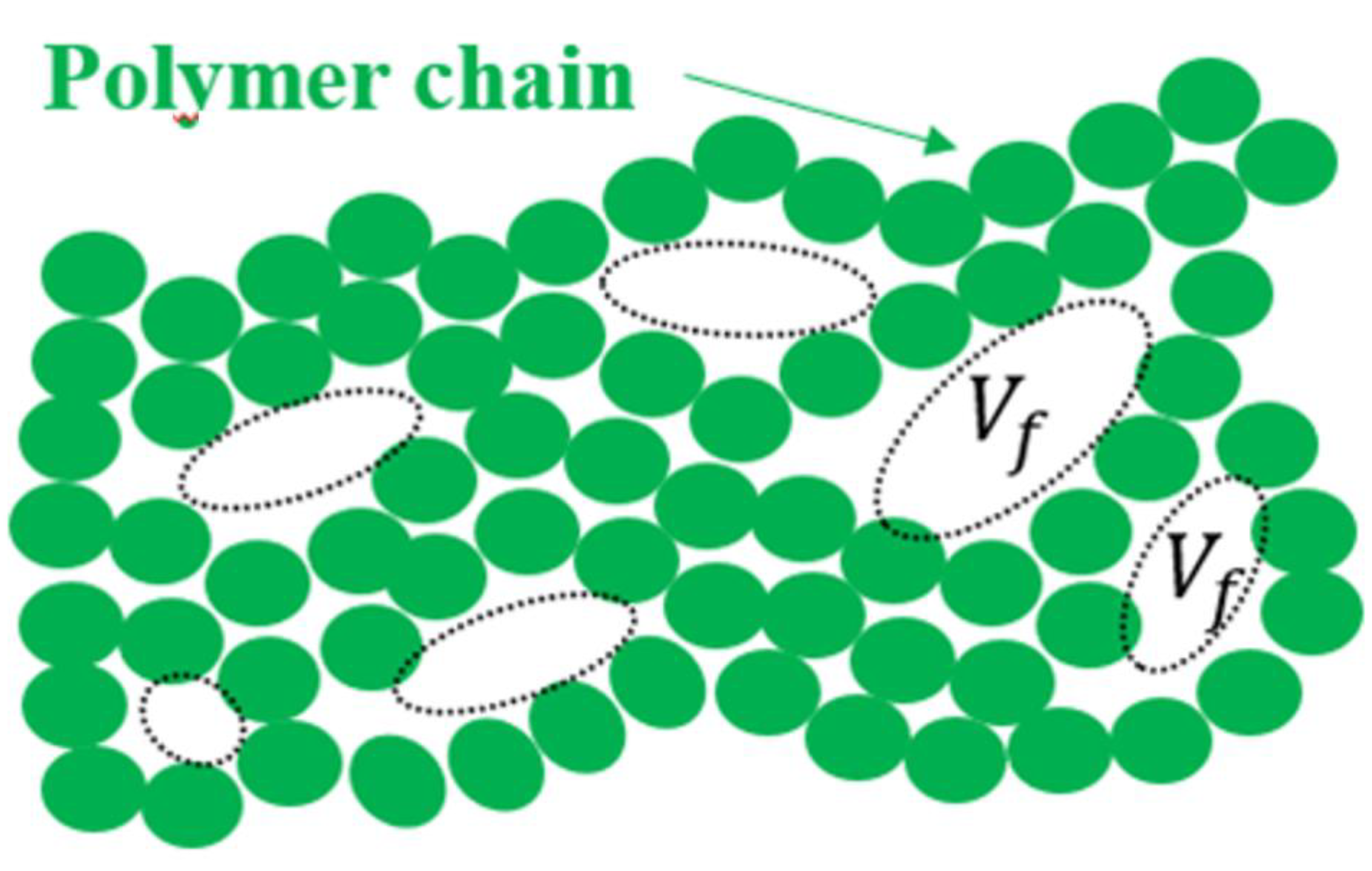
3.1. Free Volume Fraction
3.2. Glass Transition Temperature
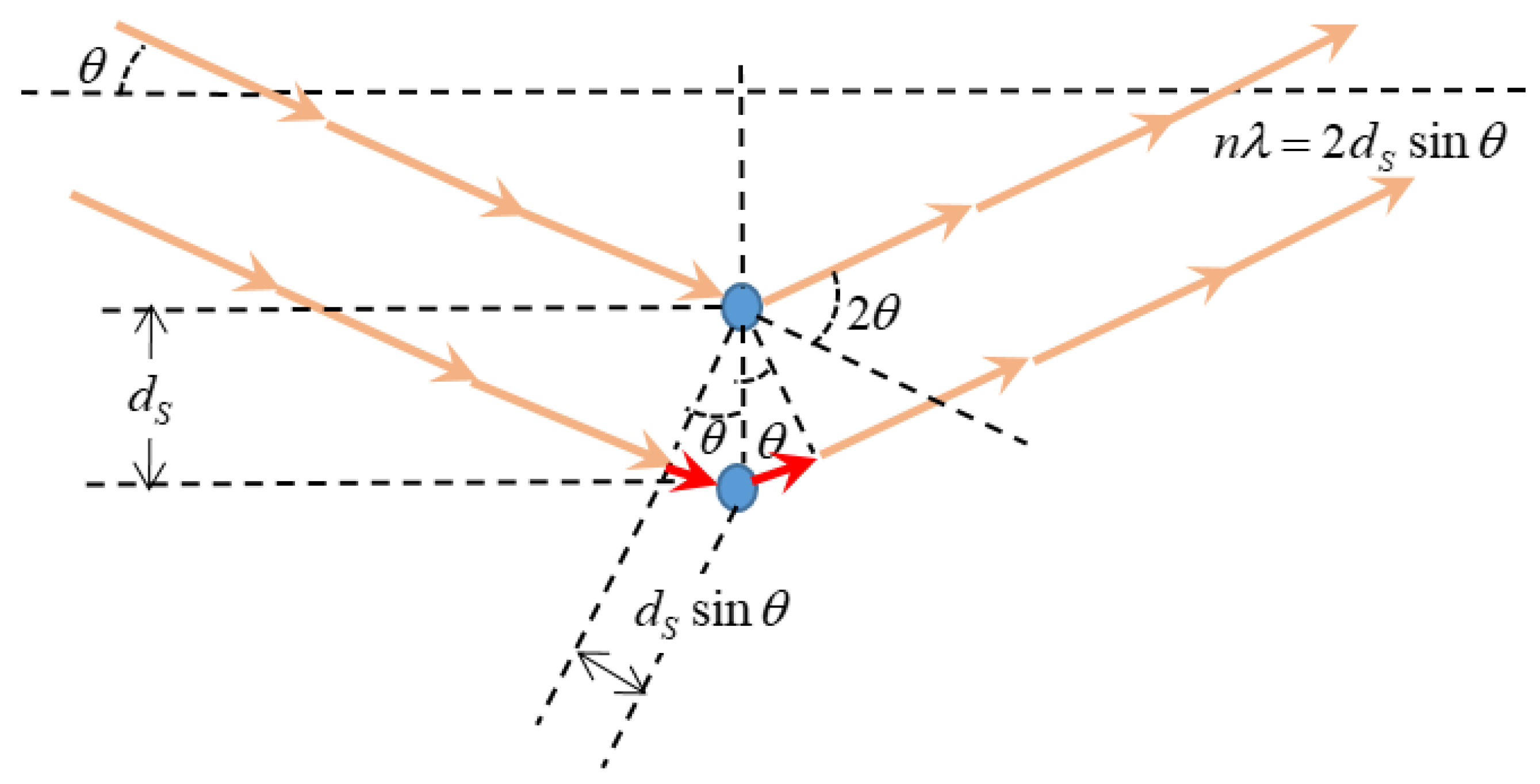
3.3. Intersegmental Distance between Polymer Chains
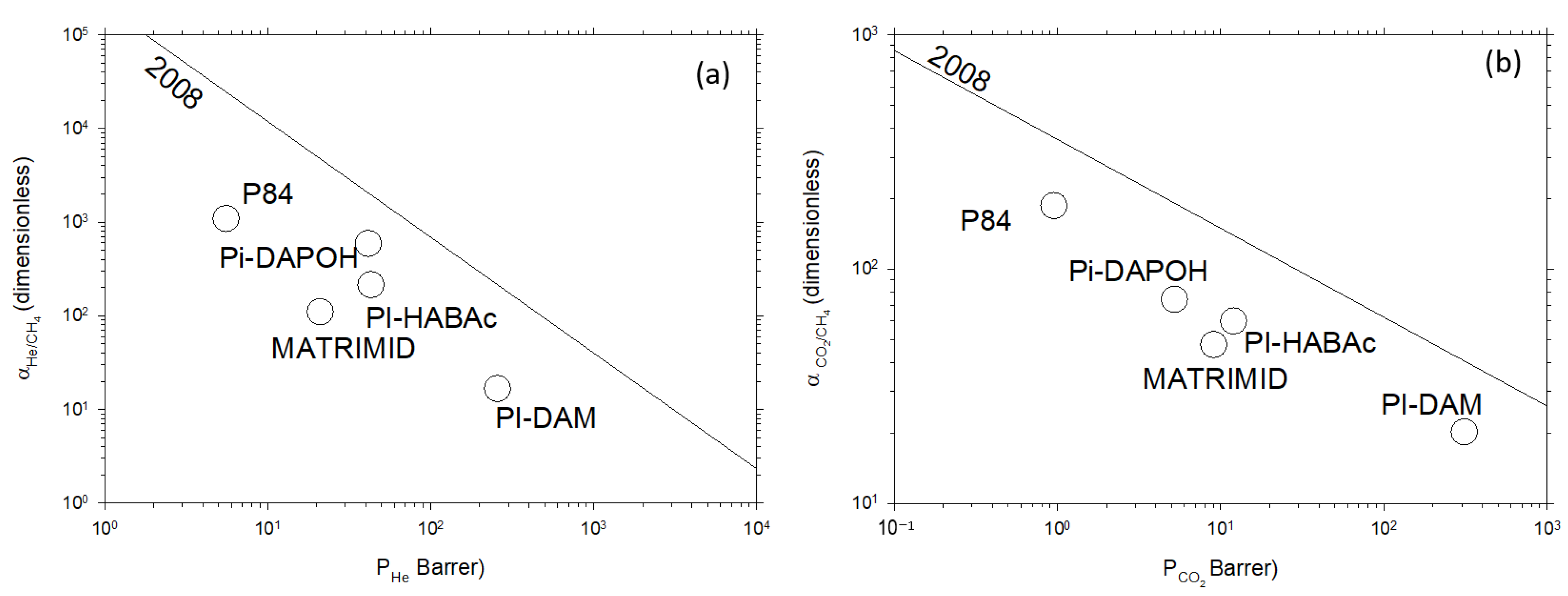
4. Results
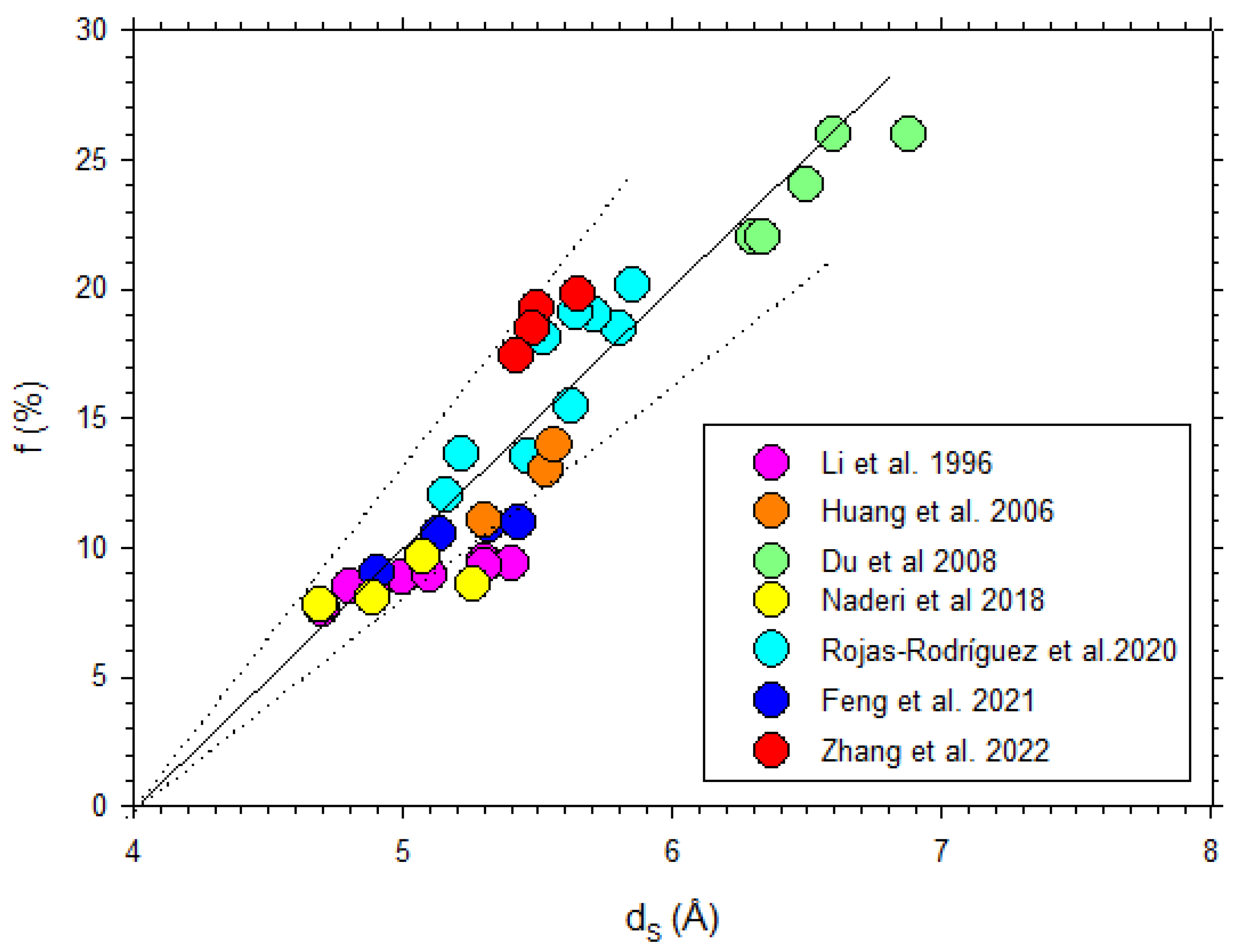
4.1. Free Volume
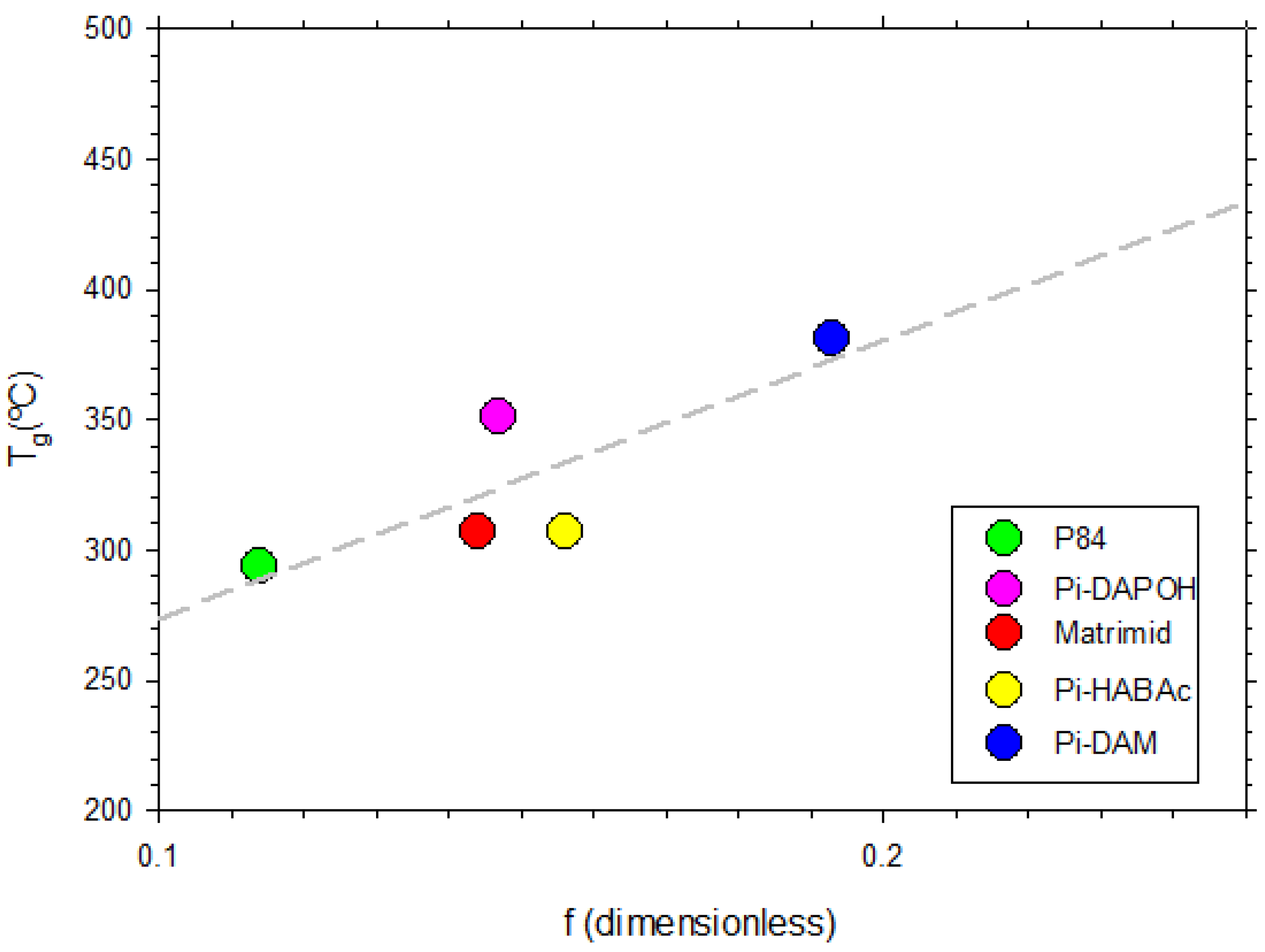
4.2. Free Volume Fraction and Glass Transition Temperature
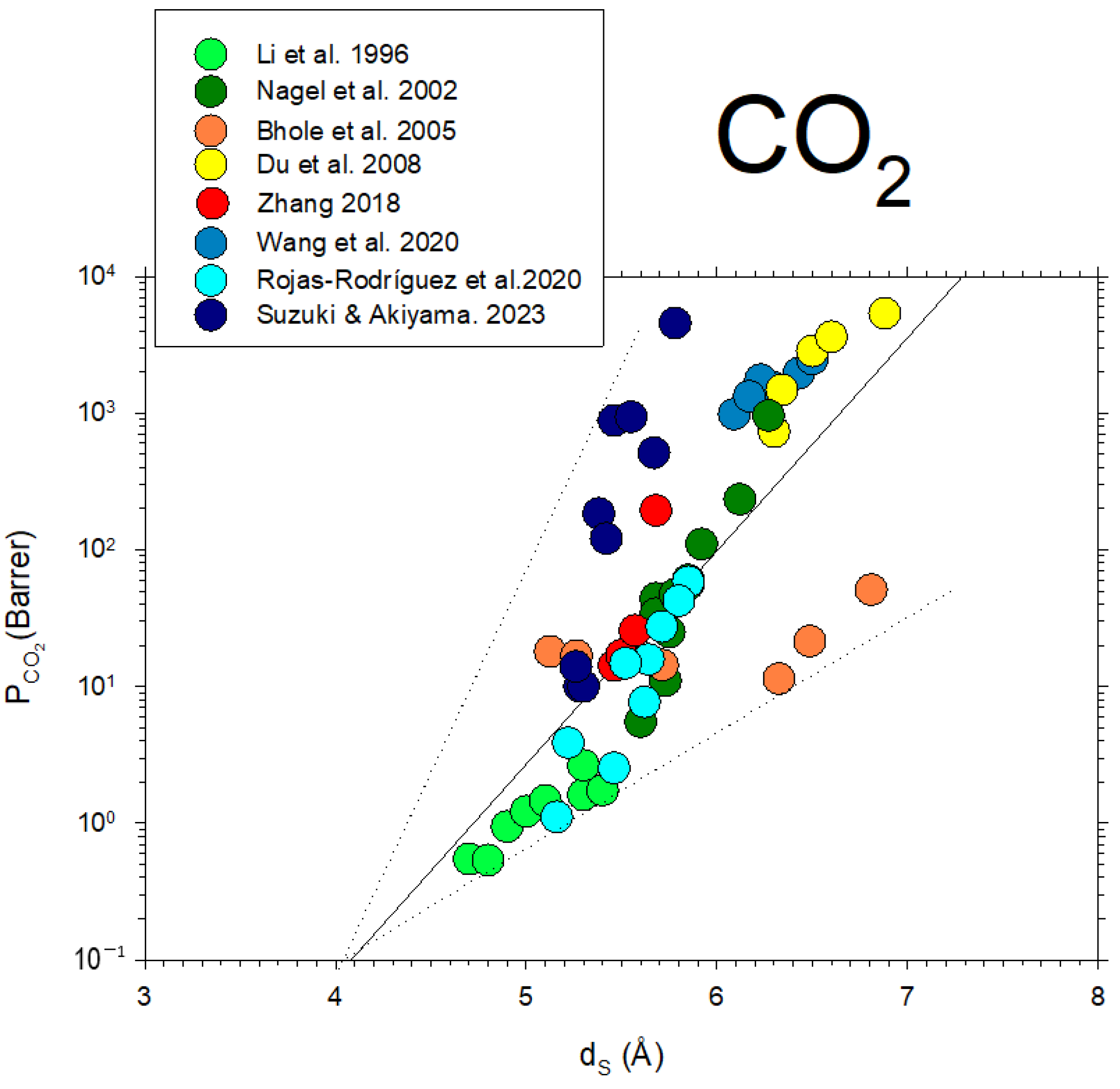
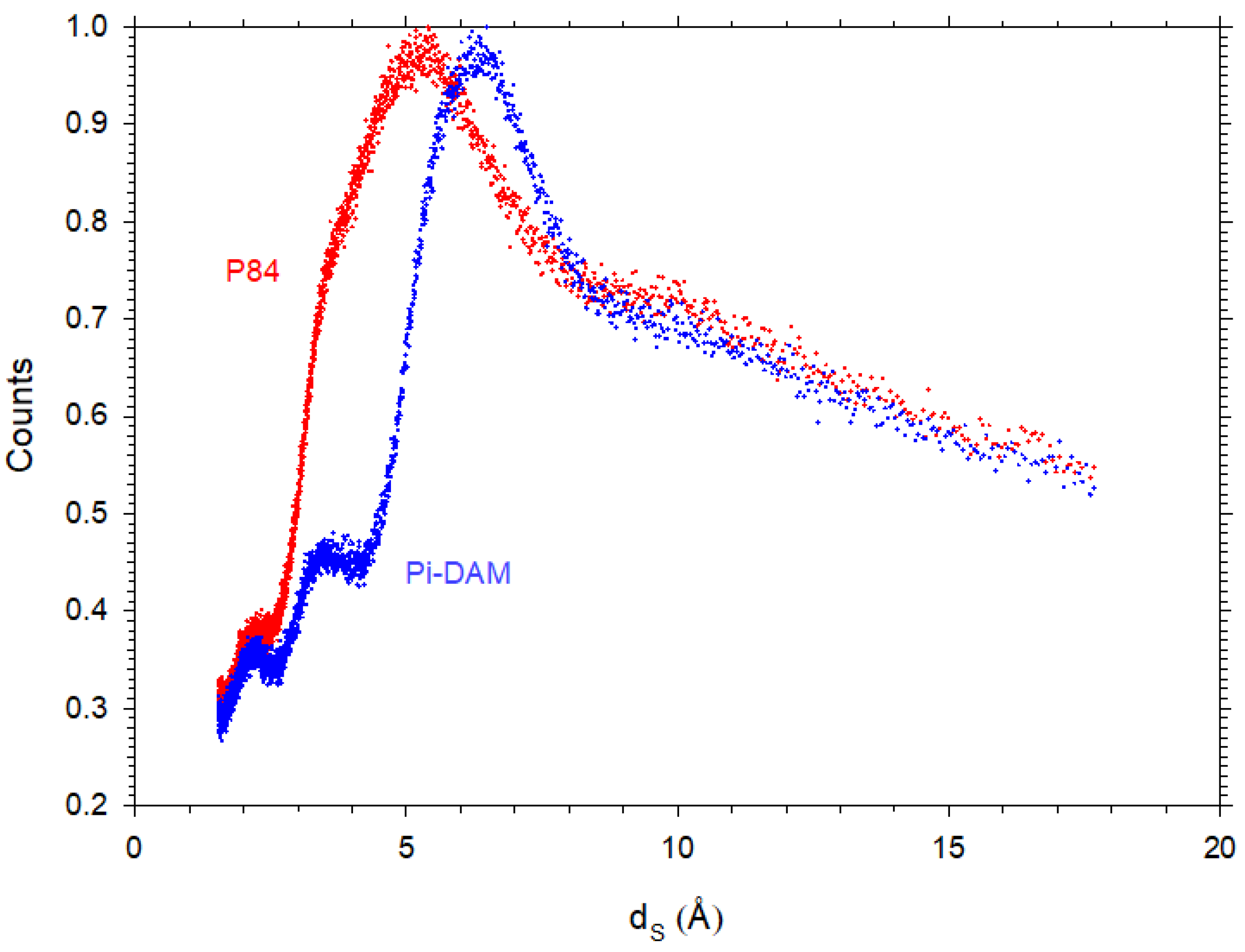
4.3. Intersegmental Distance between Polymer Chains
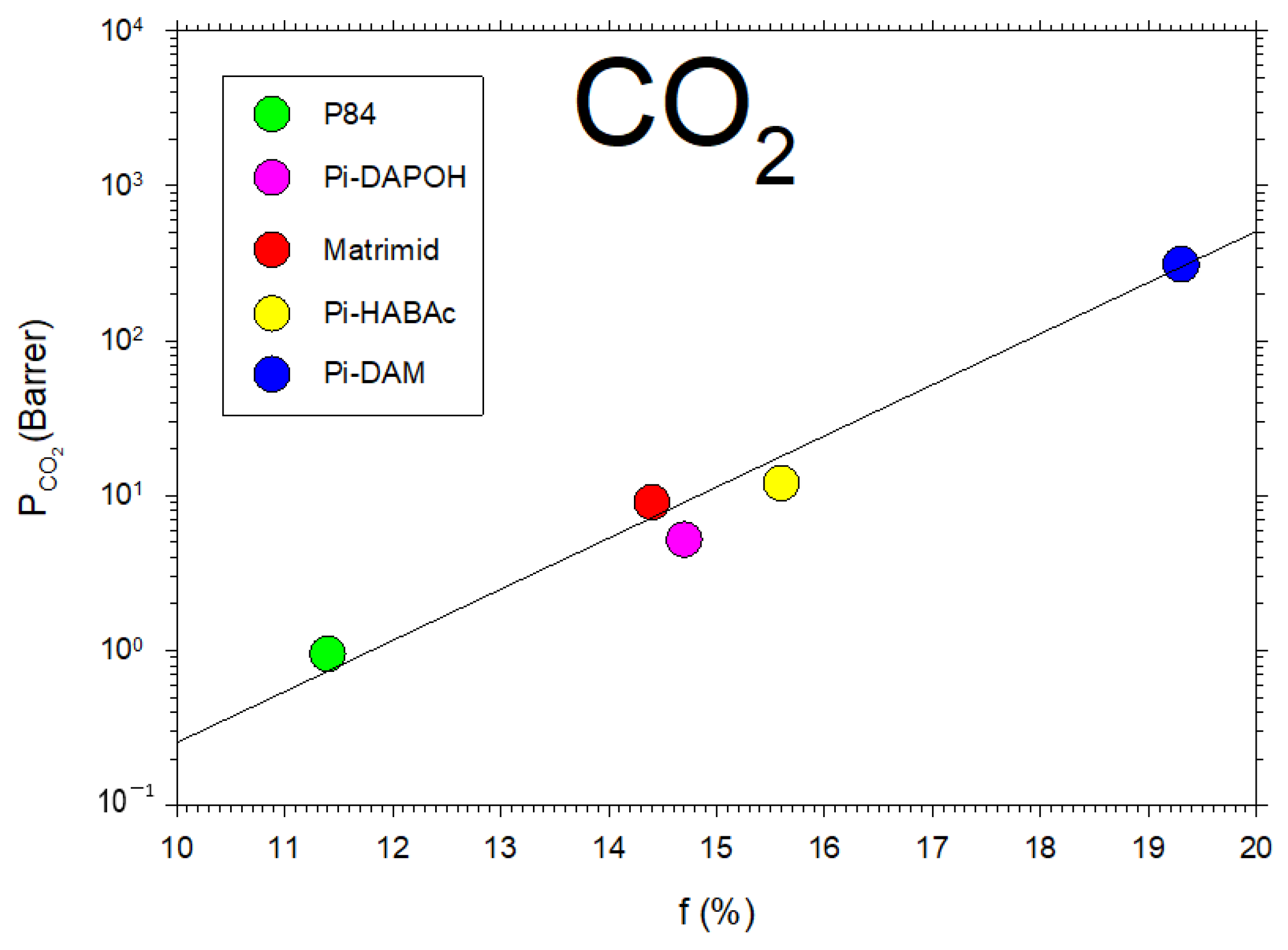
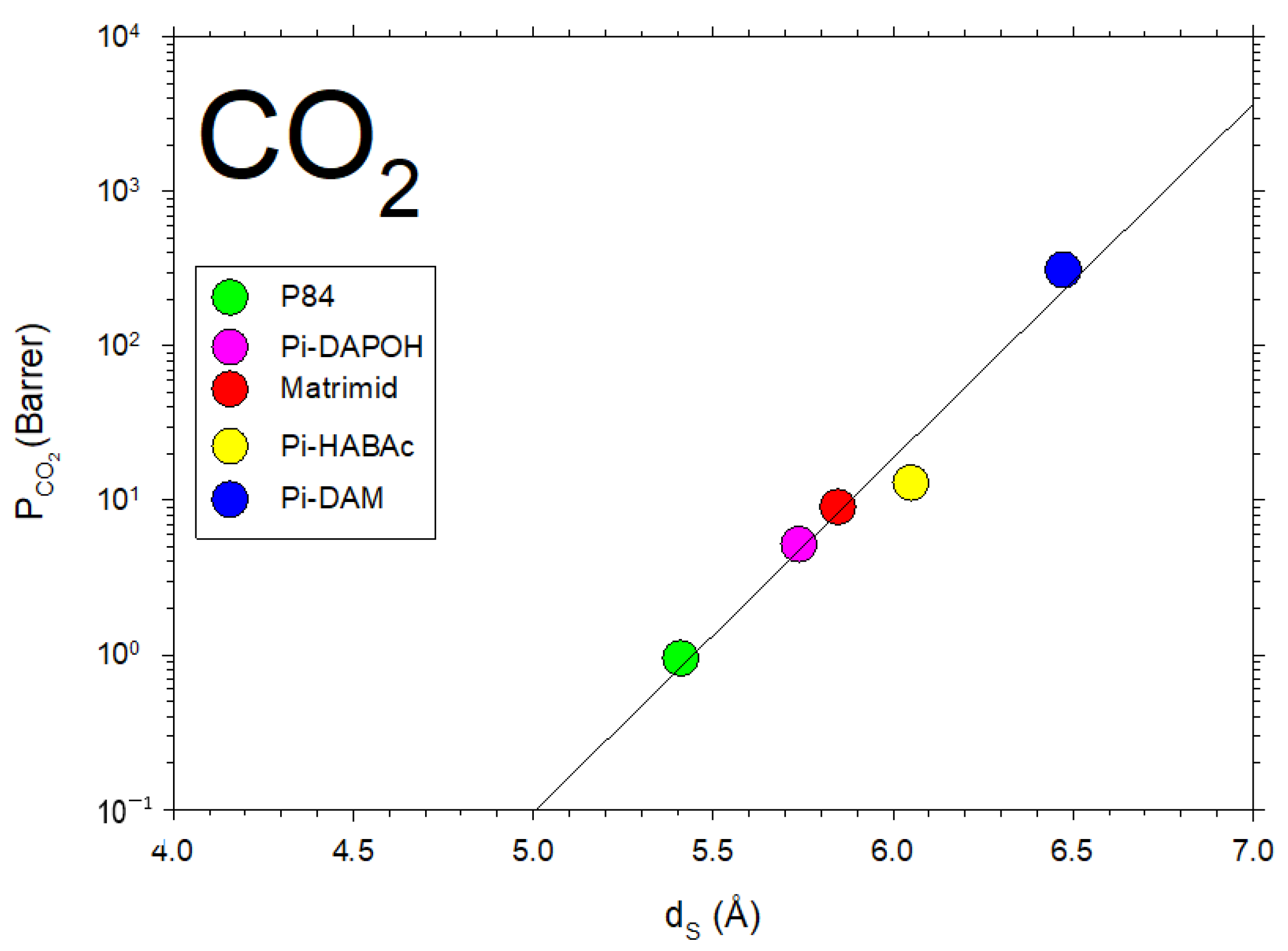
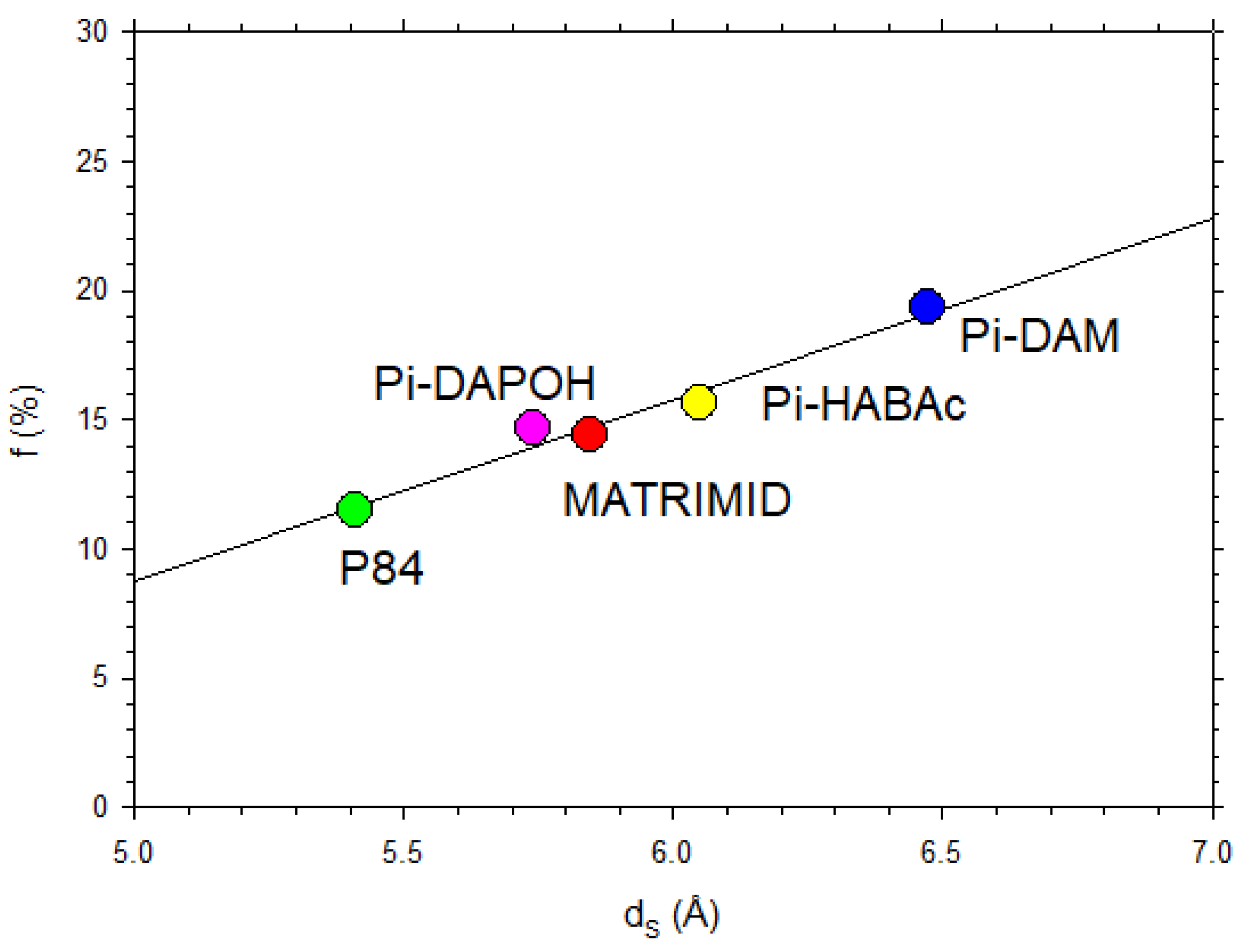
4.4. Fractional Free Volume and d-Spacing
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Chowdhury, G.; Kruczek, B.; Matsuura, T. Polyphenylene Oxide and Modified Polyphenylene Oxide Membranes. Gas, Vapor and Liquid Separation, 1st ed.; Springer: New York, NY, USA, 2001; ISBN 978-1-4615-1483-1. [Google Scholar]
- Stadler, F.J.; Takahashi, T.; Yonetake, K. Lattice Sizes, Crystallinities, and Spacing between Amorphous Chains—Characterization of Ethene-α-Olefin Copolymers with Various Comonomers and Comonomer Contents Measured by Wide Angle X-ray Scattering. e-Polymers 2009, 9, 1–19. [Google Scholar] [CrossRef]
- Lasseuguette, E.; Malpass-Evans, R.; Carta, M.; McKeown, N.B.; Ferrari, M.-C. Temperature and Pressure Dependence of Gas Permeation in a Microporous Tröger’s Base Polymer. Membranes 2018, 8, 132. [Google Scholar] [CrossRef] [PubMed]
- Sandhya, P.K.; Lakshmipriya, R.; Sreekala, M. Gas Permeability through Thermosets. In Transport Properties of Polymeric Membranes; Thomas, S., Wilson, R., Kumar, A., Goerge, S., Eds.; Elservier: Amsterdam, The Netherlands, 2018; pp. 475–516. ISBN 978-0-12-809884-4. [Google Scholar]
- Bas, C.; Mercier, R.; Dauwe, C.; Albérola, N.D. Microstructural Parameters Controlling Gas Permeability and Permselectivity in Polyimide Membranes. J. Memb. Sci. 2010, 349, 25–34. [Google Scholar] [CrossRef]
- Park, J.Y.; Paul, D.R. Correlation and Prediction of Gas Permeability in Glassy Polymer Membrane Materials via a Modified Free Volume Based Group Contribution Method. J. Memb. Sci. 1997, 125, 23–39. [Google Scholar] [CrossRef]
- Soto, C.; Torres-Cuevas, E.S.; Palacio, L.; Prádanos, P.; Freeman, B.D.; Lozano, Á.E.; Hernández, A.; Comesaña-Gándara, B. Gas Permeability, Fractional Free Volume and Molecular Kinetic Diameters: The Effect of Thermal Rearrangement on Ortho-Hydroxy Polyamide Membranes Loaded with a Porous Polymer Network. Membranes 2022, 12, 200. [Google Scholar] [CrossRef] [PubMed]
- Soto, C.; Carmona, J.; Freeman, B.D.; Palacio, L.; González-Ortega, A.; Prádanos, P.; Lozano, Á.E.; Hernandez, A. Free Volume and Permeability of Mixed Matrix Membranes Made from a Terbutil-M-Terphenyl Polyamide and a Porous Polymer Network. Polymers 2022, 14, 3176. [Google Scholar] [CrossRef]
- Soto, C.; Comesaña-Gandara, B.; Marcos, Á.; Cuadrado, P.; Palacio, L.; Lozano, Á.E.; Álvarez, C.; Prádanos, P.; Hernandez, A. Thermally Rearranged Mixed Matrix Membranes from Copoly(o-Hydroxyamide)s and Copoly(o-Hydroxyamide-Amide)s with a Porous Polymer Network as a Filler—A Comparison of Their Gas Separation Performances. Membranes 2022, 12, 998. [Google Scholar] [CrossRef]
- Soto, C.; Cicuttin, N.; Carmona, F.J.; de la Viuda, M.; Tena, A.; Lozano, E.; Hernández, A.; Palacio, L.; Prádanos, P. Gas Adsorption Isotherm, Pore Size Distribution, and Free Volume Fraction of Polymer-Polymer Mixed Matrix Membranes before and after Thermal Rearrangement. J. Memb. Sci. 2023, 683, 1841. [Google Scholar] [CrossRef]
- Van Krevelen, D.W.; Te Nijenhuis, K. Properties of Polymers. Their Correlation with Chemical Structure. In Their Numerical Estimation and Prediction from Additive Group Contributions, 4th ed.; Elservier: Amsterdam, The Netherlands, 2009; ISBN 978-0-08-054819-7. [Google Scholar]
- Hensema, E.R.; Mulder, M.H.V.; Smolders, C.A. On the Mechanism of Gas Transport in Rigid Polymer Membranes. J. Appl. Polym. Sci. 1993, 49, 2081–2090. [Google Scholar] [CrossRef]
- White, R.P.; Lipson, J.E.G. Polymer Free Volume and Its Connection to the Glass Transition. Macromolecules 2016, 49, 3987–4007. [Google Scholar] [CrossRef]
- Comesaña-Gándara, B.; De La Campa, J.G.; Hernández, A.; Jo, H.J.; Lee, Y.M.; De Abajo, J.; Lozano, A.E. Gas Separation Membranes Made through Thermal Rearrangement of Ortho-Methoxypolyimides. RSC Adv. 2015, 5, 102261–102276. [Google Scholar] [CrossRef]
- Comesaña-Gándara, B.; Hernández, A.; de la Campa, J.G.; de Abajo, J.; Lozano, A.E.; Lee, Y.M. Thermally Rearranged Polybenzoxazoles and Poly(Benzoxazole-Co-Imide)s from Ortho-Hydroxyamine Monomers for High Performance Gas Separation Membranes. J. Memb. Sci. 2015, 493, 329–339. [Google Scholar] [CrossRef]
- Robeson, L.M. The Upper Bound Revisited. J. Memb. Sci. 2008, 320, 390–400. [Google Scholar] [CrossRef]
- Bondi, A. Van Der Waals Volumes and Radii. J. Phys. Chem. 1964, 68, 441–451. [Google Scholar] [CrossRef]
- Bondi, A. Physical Properties of Molecular Crystals, Liquids and Glasses; John Wiley & Sons: New York, NY, USA, 1968. [Google Scholar]
- Horn, N.R. A Critical Review of Free Volume and Occupied Volume Calculation Methods. J. Memb. Sci. 2016, 518, 289–294. [Google Scholar] [CrossRef]
- Hypercube Inc. HyperChem Professional. Available online: http://www.hypercubeusa.com/ (accessed on 13 December 2023).
- Díez, B.; Cuadrado, P.; Marcos-Fernández, Á.; de la Campa, J.G.; Tena, A.; Prádanos, P.; Palacio, L.; Lee, Y.M.; Alvarez, C.; Lozano, Á.E.; et al. Thermally Rearranged Polybenzoxazoles Made from Poly(Ortho-Hydroxyamide)s. Characterization and Evaluation as Gas Separation Membranes. React. Funct. Polym. 2018, 127, 38–47. [Google Scholar] [CrossRef]
- Dassault Systèmes BIOVIA Materials Studio. Available online: https://www.3ds.com/es/productos-y-servicios/biovia/ (accessed on 13 December 2023).
- Thornton, A.W.; Nairn, K.M.; Hill, A.J.; Hill, J.M. New Relation between Diffusion and Free Volume: I. Predicting Gas Diffusion. J. Memb. Sci. 2009, 338, 29–37. [Google Scholar] [CrossRef]
- Fujita, H. Diffusion in Polymer-Diluent Systems. In Fortschritte der Hochpolymeren-Forschung; Springer: Berlin/Heidelberg, Germany, 1961; pp. 1–47. [Google Scholar]
- Lee, W.M. Selection of Barrier Materials from Molecular Structure. Polym. Eng. Sci. 1980, 20, 65–69. [Google Scholar] [CrossRef]
- Cohen, M.H.; Turnbull, D. Molecular Transport in Liquids and Glasses. J. Chem. Phys. 1959, 31, 1164–1169. [Google Scholar] [CrossRef]
- Soto, C.; Torres-Cuevas, E.S.; González-Ortega, A.; Palacio, L.; Lozano, Á.E.; Freeman, B.D.; Prádanos, P.; Hernández, A. Gas Separation by Mixed Matrix Membranes with Porous Organic Polymer Inclusions within O-Hydroxypolyamides Containing m-Terphenyl Moieties. Polymers 2021, 13, 931. [Google Scholar] [CrossRef]
- Breck, D.W. Zeolite Molecular Sieves: Structure, Chemistry and Use. Anal. Chim. Acta 1975, 75, 493. [Google Scholar] [CrossRef]
- Matteucci, S.; Yampolskii, Y.; Freeman, B.D.; Pinnau, I. Transport of Gases and Vapors in Glassy and Rubbery Polymers. In Materials Science of Membranes for Gas and Vapor Separation; Yampolskii, Y., Pinnau, I., Freeman, B., Eds.; John Wiley & Sons: Berlin, Germany, 2006; pp. 1–47. [Google Scholar]
- Teplyakov, V.V.; Durgar’yan, S.G. Correlation Analysis of the Gas Permeability Parameters of Polymers. Polym. Sci. USSR 1984, 26, 1498–1505. [Google Scholar] [CrossRef]
- Teplyakov, V.; Meares, P. Correlation Aspects of the Selective Gas Permeabilities of Polymeric Materials and Membranes. Gas Sep. Purif. 1990, 4, 66–74. [Google Scholar] [CrossRef]
- Reynier, A.; Dole, P.; Humbel, S.; Feigenbaum, A. Diffusion Coefficients of Additives in Polymers. I. Correlation with Geometric Parameters. J. Appl. Polym. Sci. 2001, 82, 2422–2433. [Google Scholar] [CrossRef]
- McCormick, H.W.; Brower, F.M.; Kin, L. The Effect of Molecular Weight Distribution on the Physical Properties of Polystyrene. J. Polym. Sci. 1959, 39, 87–100. [Google Scholar] [CrossRef]
- Ismail, A.F.; Khulbe, K.C.; Matsuura, T. Gas Separation Membranes: Polymeric and Inorganic, 1st ed.; Springer Cham: New York, NY, USA, 2015; ISBN 978-3-319-01095-3. [Google Scholar]
- Shimazu, A.; Miyazaki, T.; Ikeda, K. Interpretation of D-Spacing Determined by Wide Angle X-ray Scattering in 6FDA-Based Polyimide by Molecular Modeling. J. Memb. Sci. 2000, 166, 113–118. [Google Scholar] [CrossRef]
- Tin, P.S.; Chung, T.-S.; Liu, Y.; Wang, R. Separation of CO2/CH4 through Carbon Molecular Sieve Membranes Derived from P84 Polyimide. Carbon N. Y. 2004, 42, 3123–3131. [Google Scholar] [CrossRef]
- Xu, S.; Ren, X.; Zhao, N.; Wu, L.; Zhang, Z.; Fan, Y.; Li, N. Self-Crosslinking of Bromomethylated 6FDA-DAM Polyimide for Gas Separations. J. Memb. Sci. 2021, 636, 119534. [Google Scholar] [CrossRef]
- Mazinani, S.; Ramezani, R.; Molelekwa, G.F.; Darvishmanesh, S.; Di Felice, R.; Van der Bruggen, B. Plasticization Suppression and CO2 Separation Enhancement of Matrimid through Homogeneous Blending with a New High Performance Polymer. J. Memb. Sci. 2019, 574, 318–324. [Google Scholar] [CrossRef]
- Abdulhamid, M.A.; Genduso, G.; Wang, Y.; Ma, X.; Pinnau, I. Plasticization-Resistant Carboxyl-Functionalized 6FDA-Polyimide of Intrinsic Microporosity (PIM-PI) for Membrane-Based Gas Separation. Ind. Eng. Chem. Res. 2020, 59, 5247–5256. [Google Scholar] [CrossRef]
- Wu, A.X.; Lin, S.; Rodriguez, K.M.; Benedetti, F.M.; Joo, T.; Grosz, A.F.; Storme, K.R.; Roy, N.; Syar, D.; Smith, Z.P. Revisiting Group Contribution Theory for Estimating Fractional Free Volume of Microporous Polymer Membranes. J. Memb. Sci. 2021, 636, 119526. [Google Scholar] [CrossRef]
- Yampolskii, Y. Polymeric Gas Separation Membranes. Macromolecules 2012, 45, 3298–3311. [Google Scholar] [CrossRef]
- Du, N.; Robertson, G.P.; Song, J.; Pinnau, I.; Thomas-Droz, S.; Guiver, M.D. Polymers of Intrinsic Microporosity Containing Trifluoromethyl and Phenylsulfone Groups as Materials for Membrane Gas Separation. Macromolecules 2008, 41, 9656–9662. [Google Scholar] [CrossRef]
- Zhang, C.; Cao, B.; Li, P. Thermal Oxidative Crosslinking of Phenolphthalein-Based Cardo Polyimides with Enhanced Gas Permeability and Selectivity. J. Memb. Sci. 2018, 546, 90–99. [Google Scholar] [CrossRef]
- Rojas-Rodríguez, M.; Aguilas-Lugo, C.; Lozano, Á.E.; Hernández, A.; Mancilla-Cetina, E.; Alexandrova, L. Synthesis and Properties of Highly Processable Asymmetric Polyimides with Bulky Phenoxy Groups. High Perform. Polym. 2019, 32, 455–468. [Google Scholar] [CrossRef]
- Li, Y.; Ding, M.; Xu, J. Structure/Permeability and Permselectivity Relathionship of Polyetherimides from 1,4-Bis(3,4-Dicarboxyphenoxy)Benzene Dianhydride. Eur. Polym. J. 1996, 32, 1313–1317. [Google Scholar] [CrossRef]
- Nagel, C.; Günther-Schade, K.; Fritsch, D.; Strunskus, T.; Faupel, F. Free Volume and Transport Properties in Highly Selective Polymer Membranes. Macromolecules 2002, 35, 2071–2077. [Google Scholar] [CrossRef]
- Bhole, Y.S.; Kharul, U.K.; Somani, S.P.; Kumbharkar, S.C. Benzoylation of Polyphenylene Oxide: Characterization and Gas Permeability Investigations. Eur. Polym. J. 2005, 41, 2461–2471. [Google Scholar] [CrossRef]
- Wang, Z.; Shen, Q.; Liang, J.; Zhang, Y.; Jin, J. Adamantane-Grafted Polymer of Intrinsic Microporosity with Finely Tuned Interchain Spacing for Improved CO2 Separation Performance. Sep. Purif. Technol. 2020, 233, 116008. [Google Scholar] [CrossRef]
- Suzuki, T.; Akiyama, R. Unexpected Permeability Enhancement of Thermally Rearranged (TR) Copolybenzoxazole Membranes. Mater. Today Commun. 2023, 35, 106120. [Google Scholar] [CrossRef]
- Feng, F.; Liang, C.-Z.; Wu, L.; Weber, M.; Maletzko, C.; Zhang, S.; Chung, T. Polyphenylsulfone (PPSU)-Based Copolymeric Membranes: Effects of Chemical Structure and Content on Gas Permeation and Separation. Polymers 2021, 13, 2745. [Google Scholar] [CrossRef] [PubMed]
- Huang, S.H.; Hu, C.C.; Lee, K.R.; Liaw, D.J.; Lai, J.Y. Gas Separation Properties of Aromatic Poly(Amide-Imide) Membranes. Eur. Polym. J. 2006, 42, 140–148. [Google Scholar] [CrossRef]
- Zhang, B.; Qiao, J.; Wu, D.; He, X.; Liu, J.; Yi, C.; Qi, S. Enhanced Gas Separation by Free Volume Tuning in a Crown Ether-Containing Polyimide Membrane. Sep. Purif. Technol. 2022, 293, 121116. [Google Scholar] [CrossRef]
- Naderi, A.; Yong, W.F.; Xiao, Y.; Chung, T.S.; Weber, M.; Maletzko, C. Effects of Chemical Structure on Gas Transport Properties of Polyethersulfone Polymers. Polymer 2018, 135, 76–84. [Google Scholar] [CrossRef]



| Polymer | Solvent | Drying |
|---|---|---|
| Matrimid® | THF | Room temperature until dry and 120 °C for 12 h under vacuum to complete solvent evaporation |
| P84®, Pi-HABAc, Pi-DAPOH and Pi-DAM | NMP | At 60 °C for 12 h and 100 °C for 1 h. Finally, until 300 °C under N2 atmosphere a |
| a (Dimensionless) | b (1/Å) | c (1/Å2) |
|---|---|---|
| 146.61 | −92.66 | 21.11 |
| a′ (1/Å) | b′ (1/Å2) | c′ (1/Å3) |
|---|---|---|
| 1939.47 | −1255.83 | 245.68 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Torres, A.; Soto, C.; Carmona, J.; Comesaña-Gandara, B.; de la Viuda, M.; Palacio, L.; Prádanos, P.; Simorte, M.T.; Sanz, I.; Muñoz, R.; et al. Gas Permeability through Polyimides: Unraveling the Influence of Free Volume, Intersegmental Distance and Glass Transition Temperature. Polymers 2024, 16, 13. https://doi.org/10.3390/polym16010013
Torres A, Soto C, Carmona J, Comesaña-Gandara B, de la Viuda M, Palacio L, Prádanos P, Simorte MT, Sanz I, Muñoz R, et al. Gas Permeability through Polyimides: Unraveling the Influence of Free Volume, Intersegmental Distance and Glass Transition Temperature. Polymers. 2024; 16(1):13. https://doi.org/10.3390/polym16010013
Chicago/Turabian StyleTorres, Alba, Cenit Soto, Javier Carmona, Bibiana Comesaña-Gandara, Mónica de la Viuda, Laura Palacio, Pedro Prádanos, María Teresa Simorte, Inmaculada Sanz, Raúl Muñoz, and et al. 2024. "Gas Permeability through Polyimides: Unraveling the Influence of Free Volume, Intersegmental Distance and Glass Transition Temperature" Polymers 16, no. 1: 13. https://doi.org/10.3390/polym16010013
APA StyleTorres, A., Soto, C., Carmona, J., Comesaña-Gandara, B., de la Viuda, M., Palacio, L., Prádanos, P., Simorte, M. T., Sanz, I., Muñoz, R., Tena, A., & Hernández, A. (2024). Gas Permeability through Polyimides: Unraveling the Influence of Free Volume, Intersegmental Distance and Glass Transition Temperature. Polymers, 16(1), 13. https://doi.org/10.3390/polym16010013