Buckling Analysis on Resin Base Laminated Plate Reinforced with Uniform and Functional Gradient Distribution of Carbon Fiber in Thermal Environment
Abstract
1. Introduction
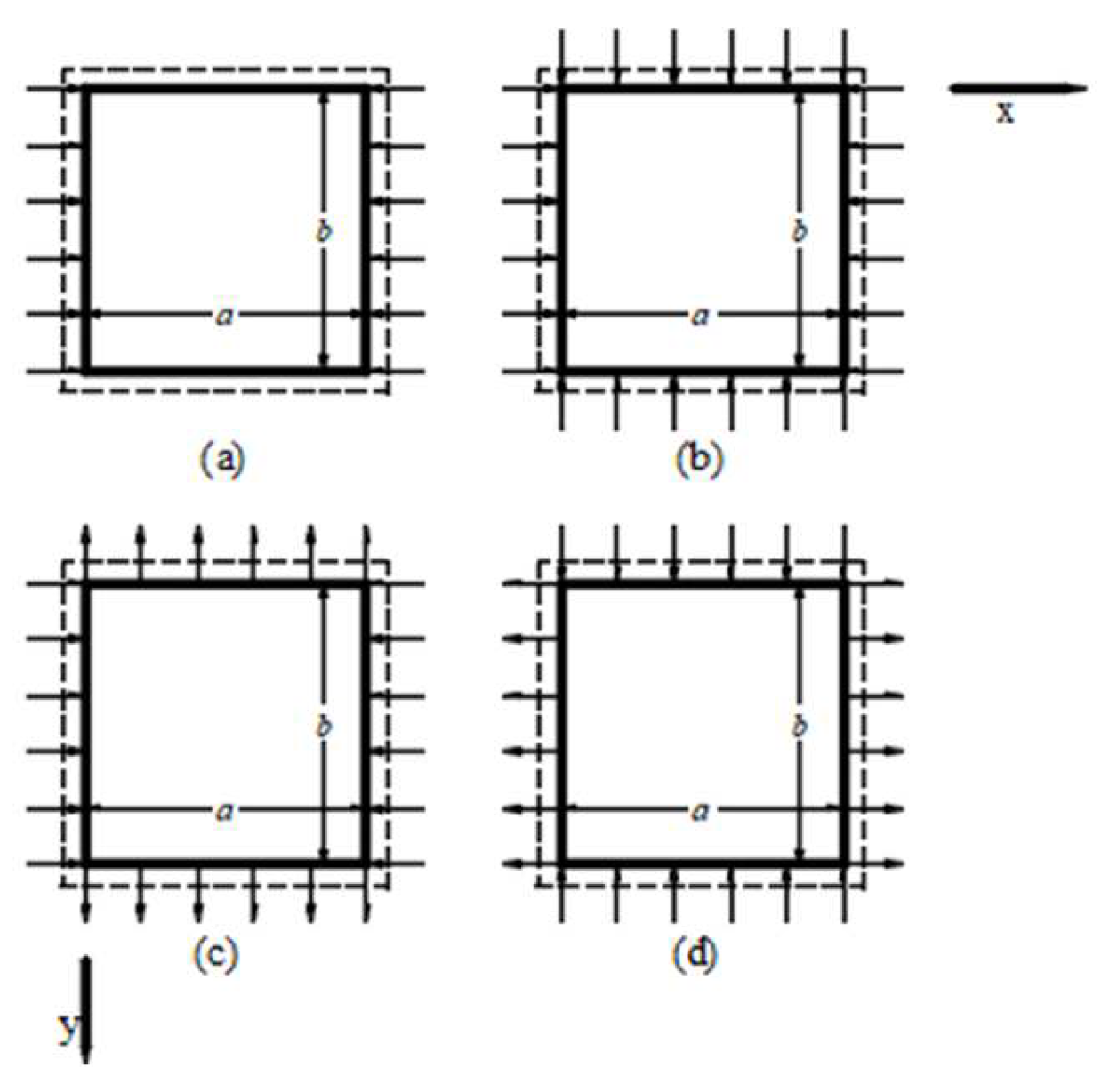
2. Problem Formulation
3. Theoretical Formulation
3.1. Displacement Field Model
3.2. Buckling Equations
4. Result and Discussion
4.1. Validation
4.2. Parametric Studies
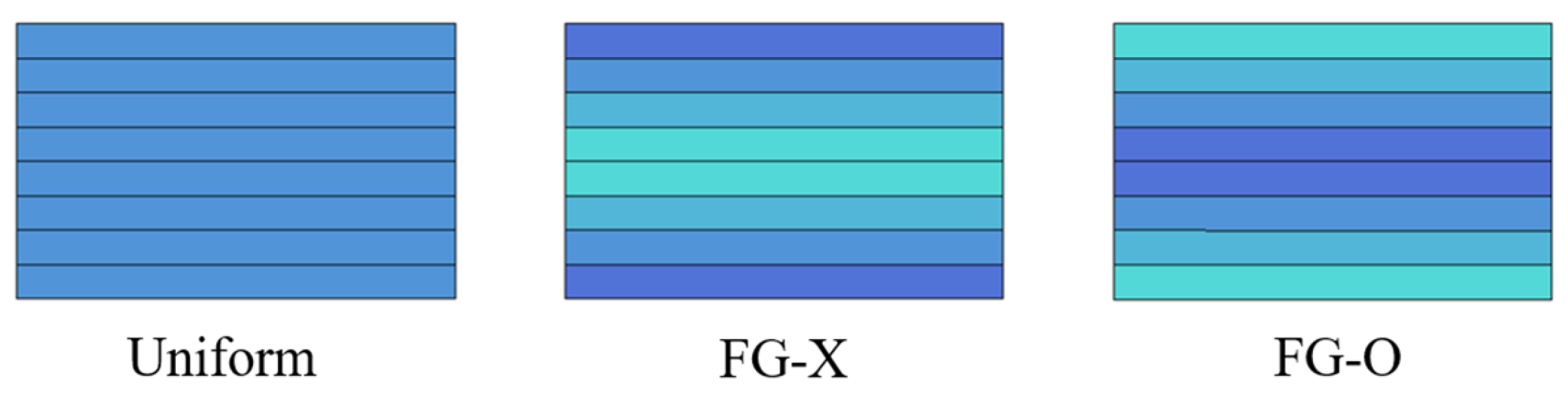
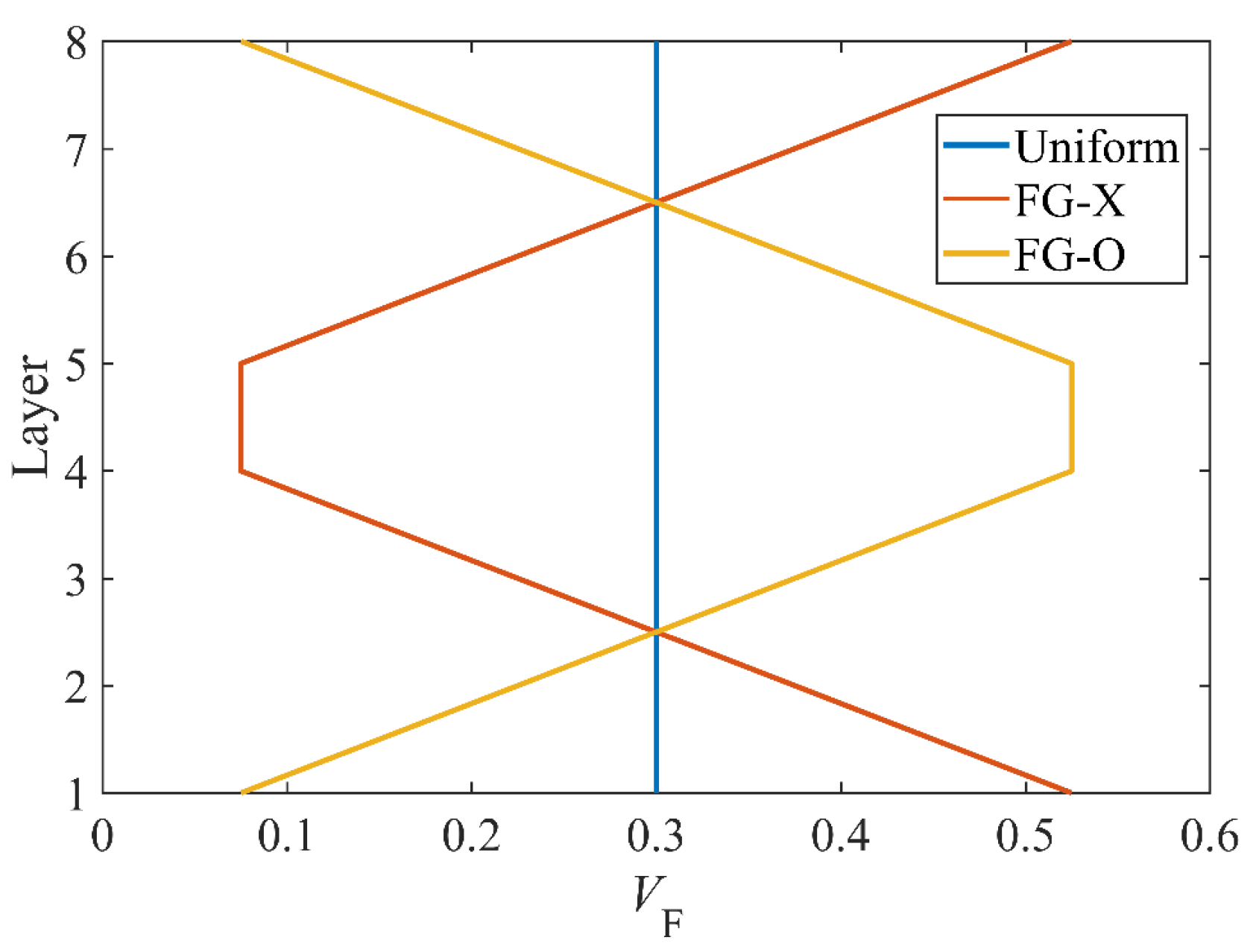
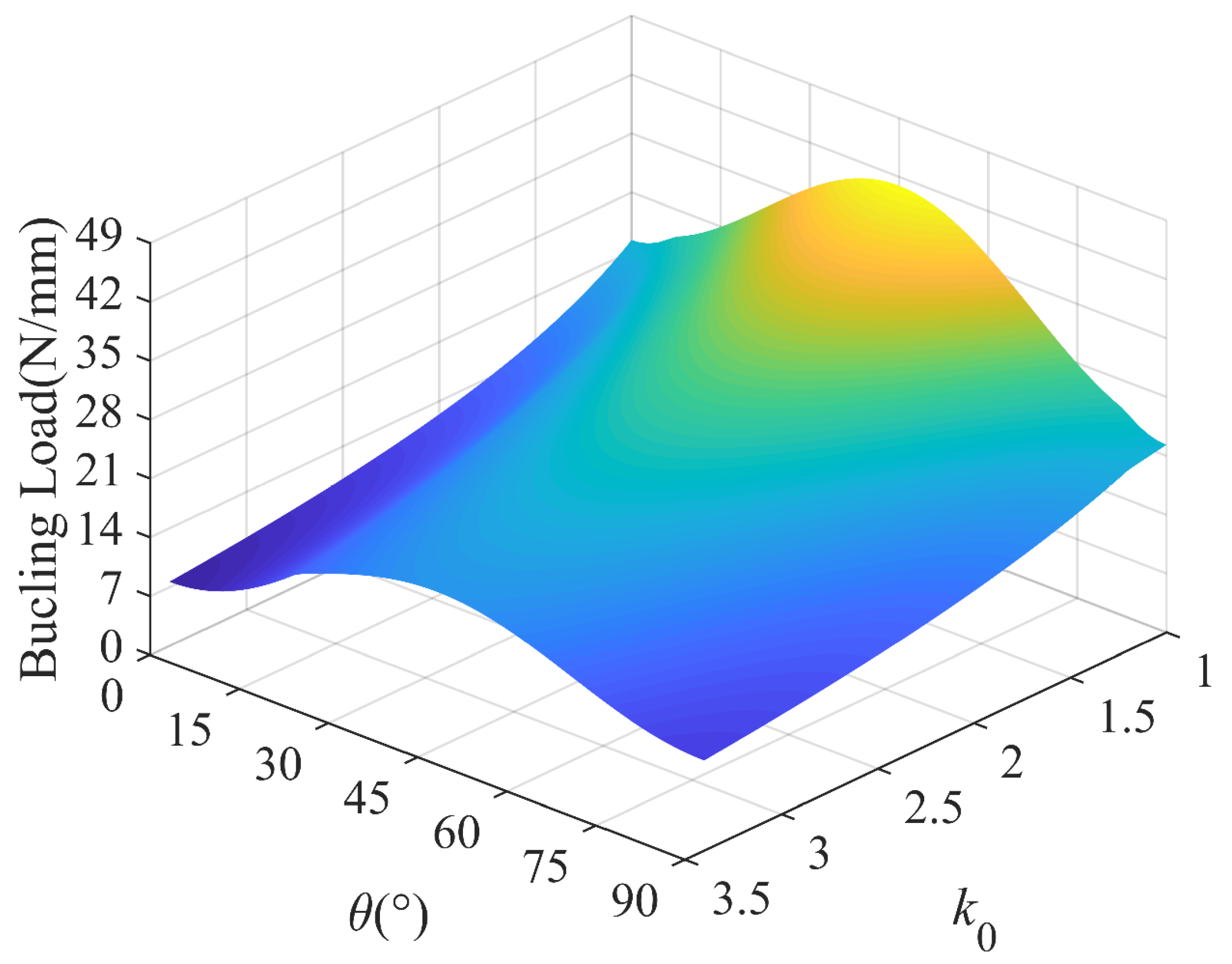
4.2.1. Effect of Carbon Fiber Volume Fraction
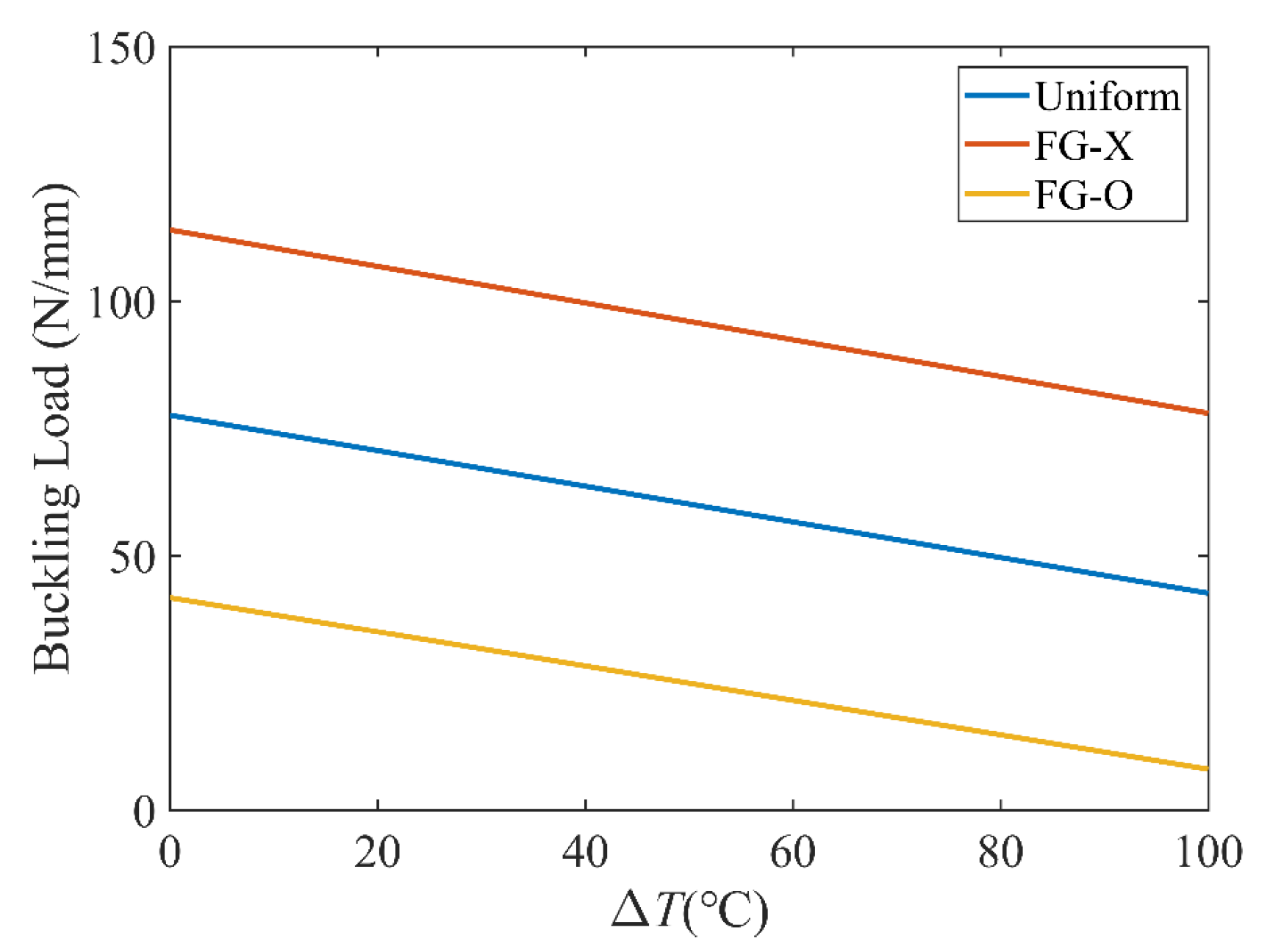
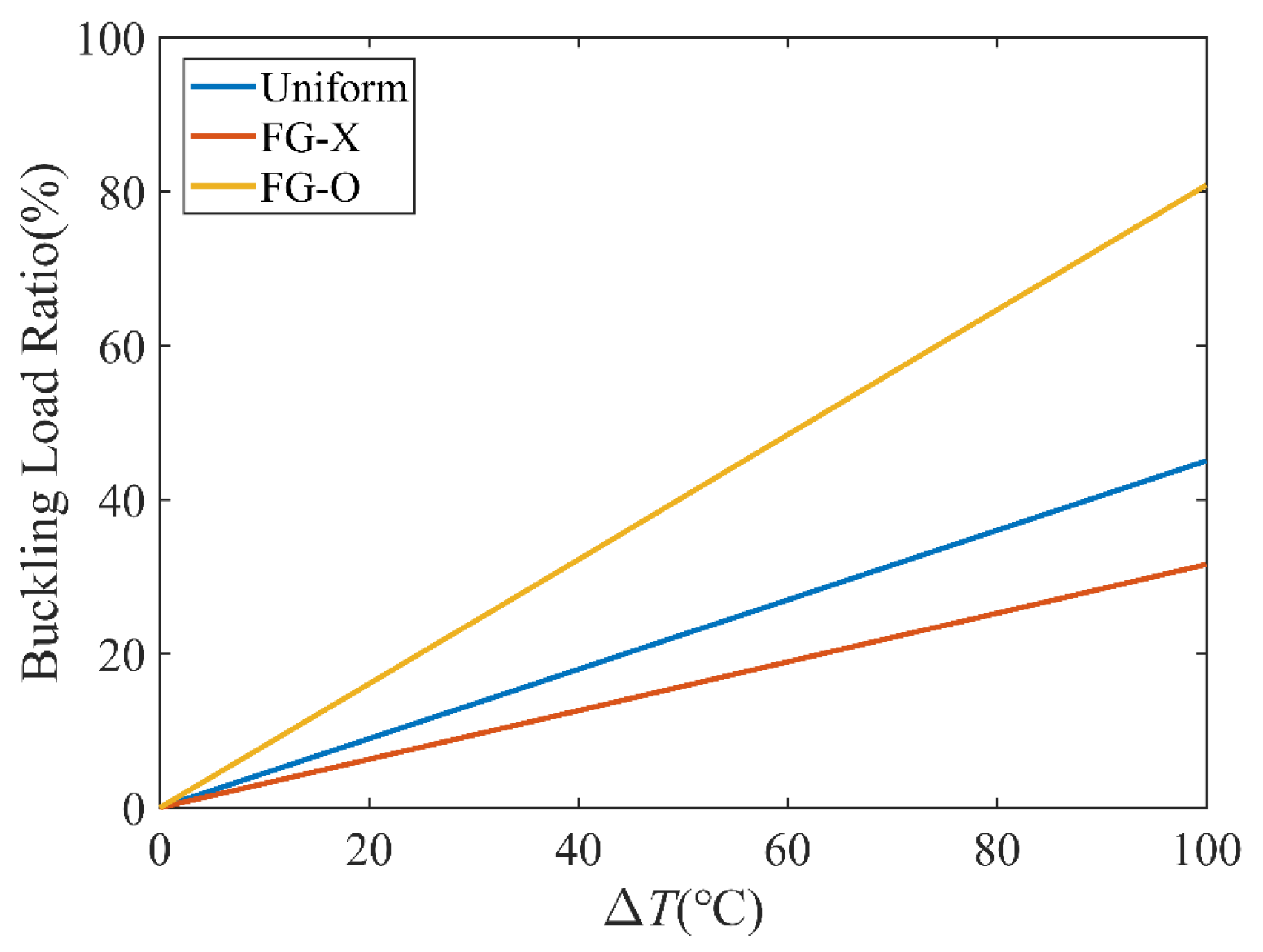
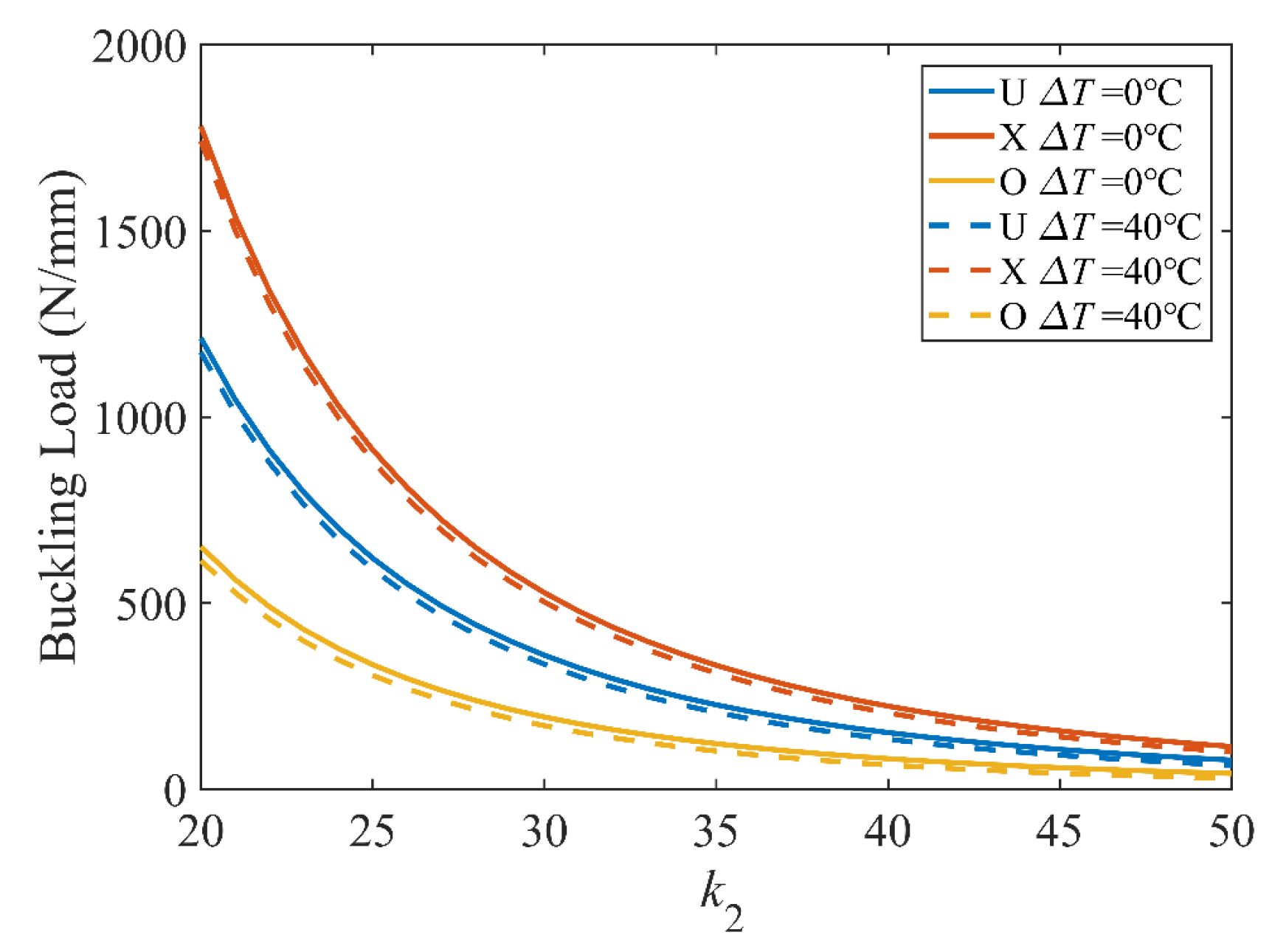
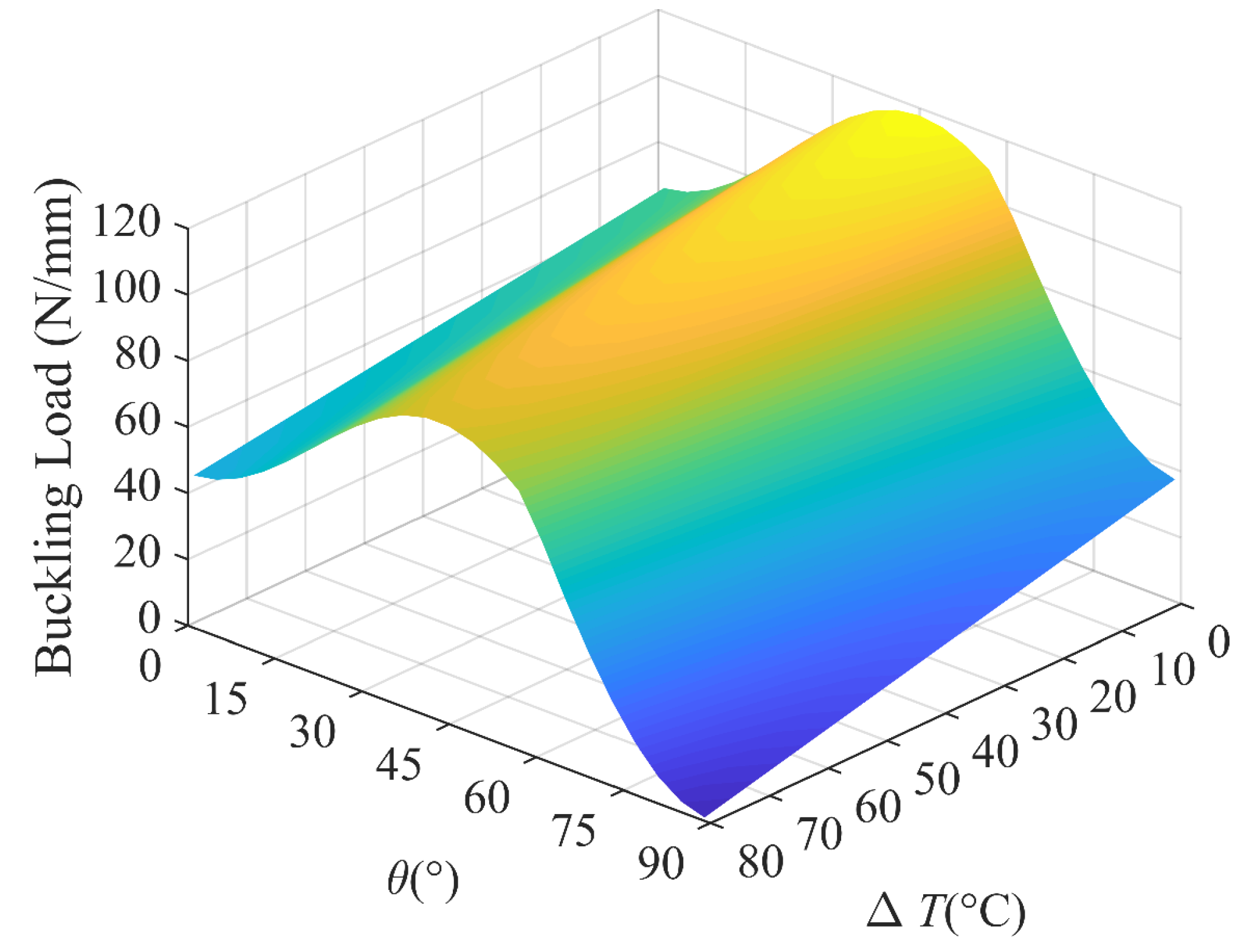
4.2.2. Effect of Thermal Environment
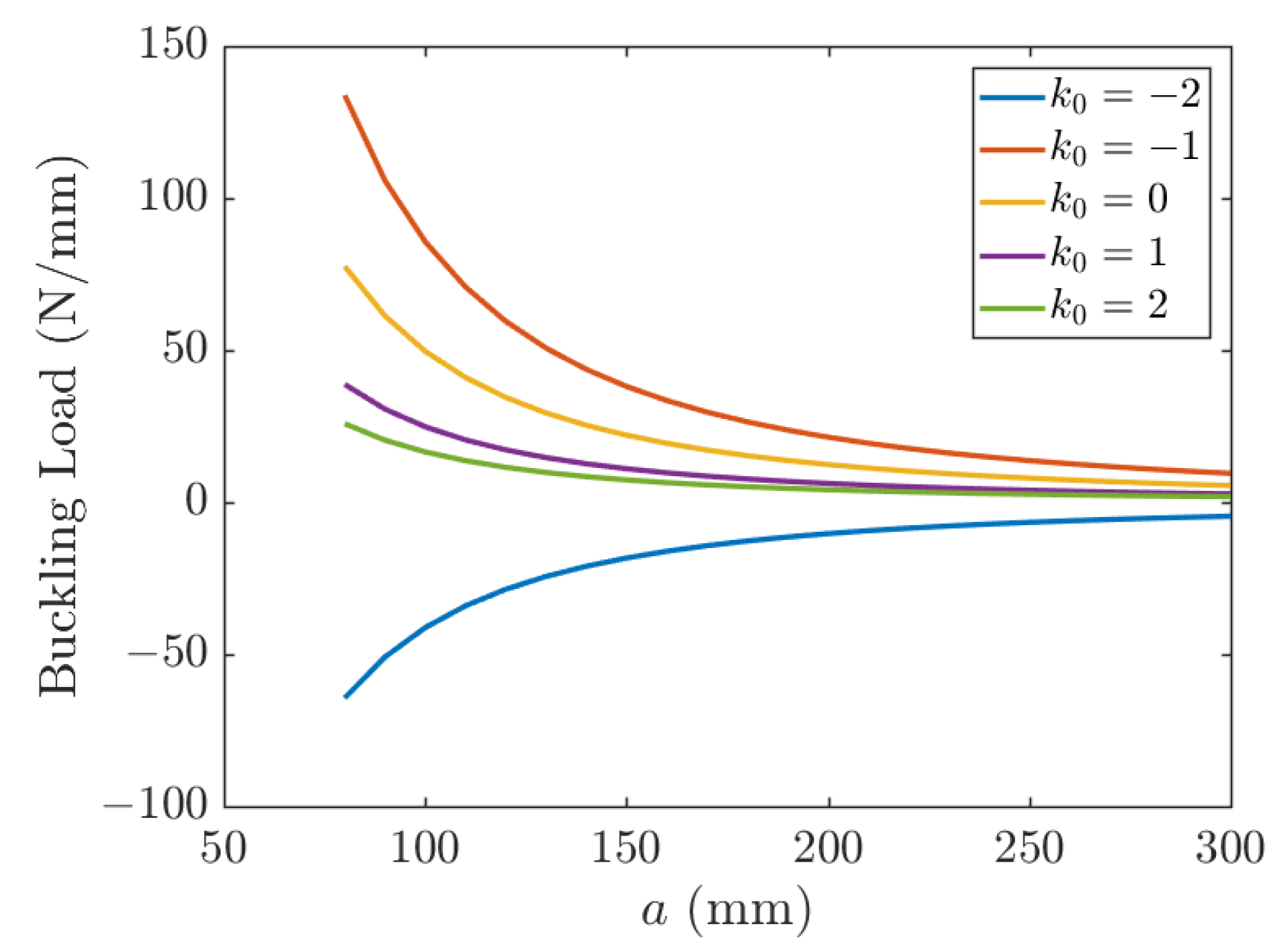
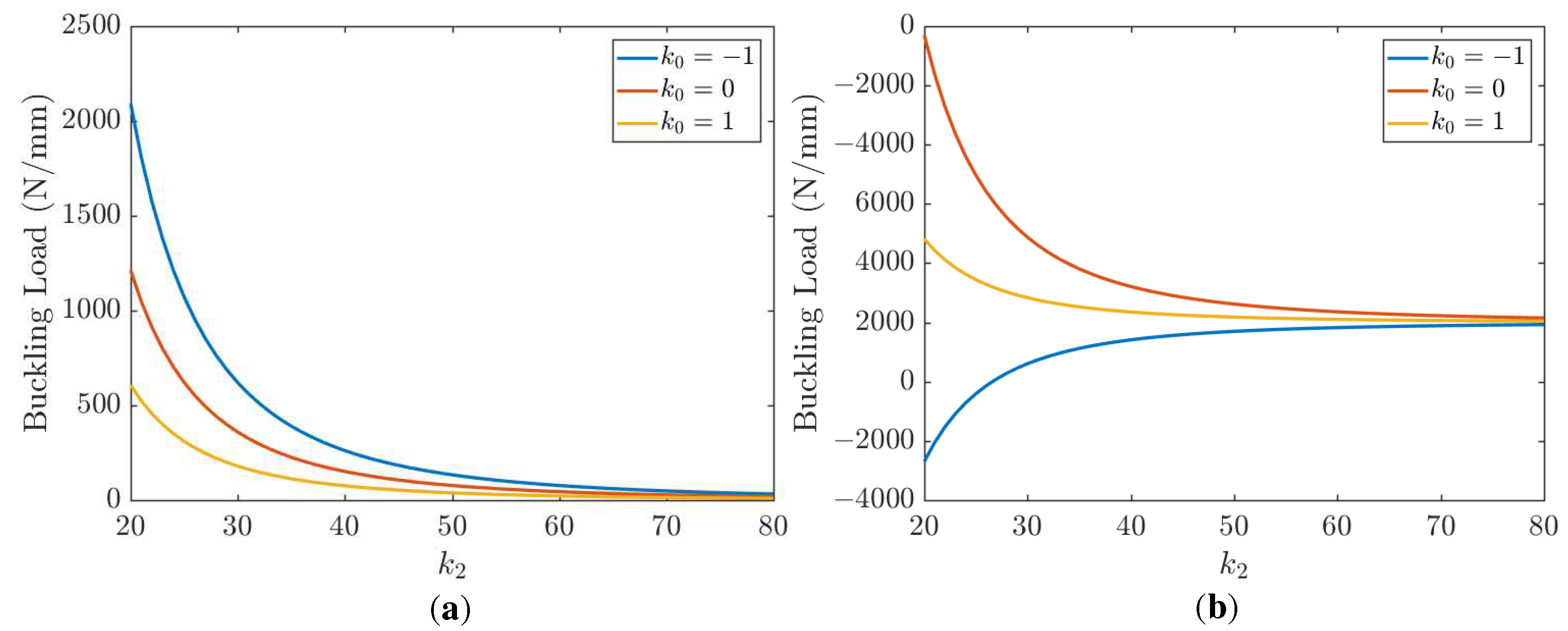
4.2.3. Effect of Geometrical Dimension of Plate
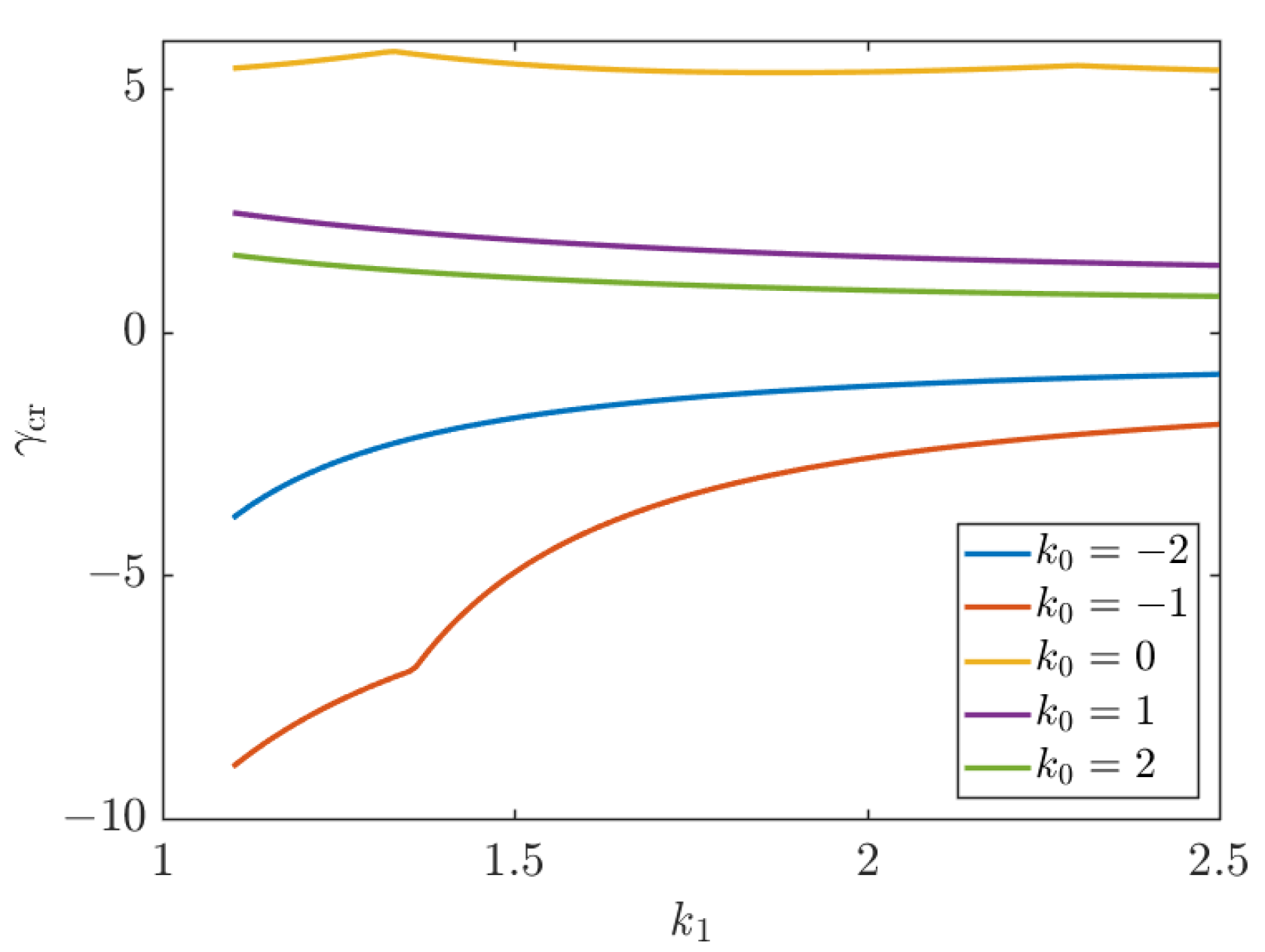
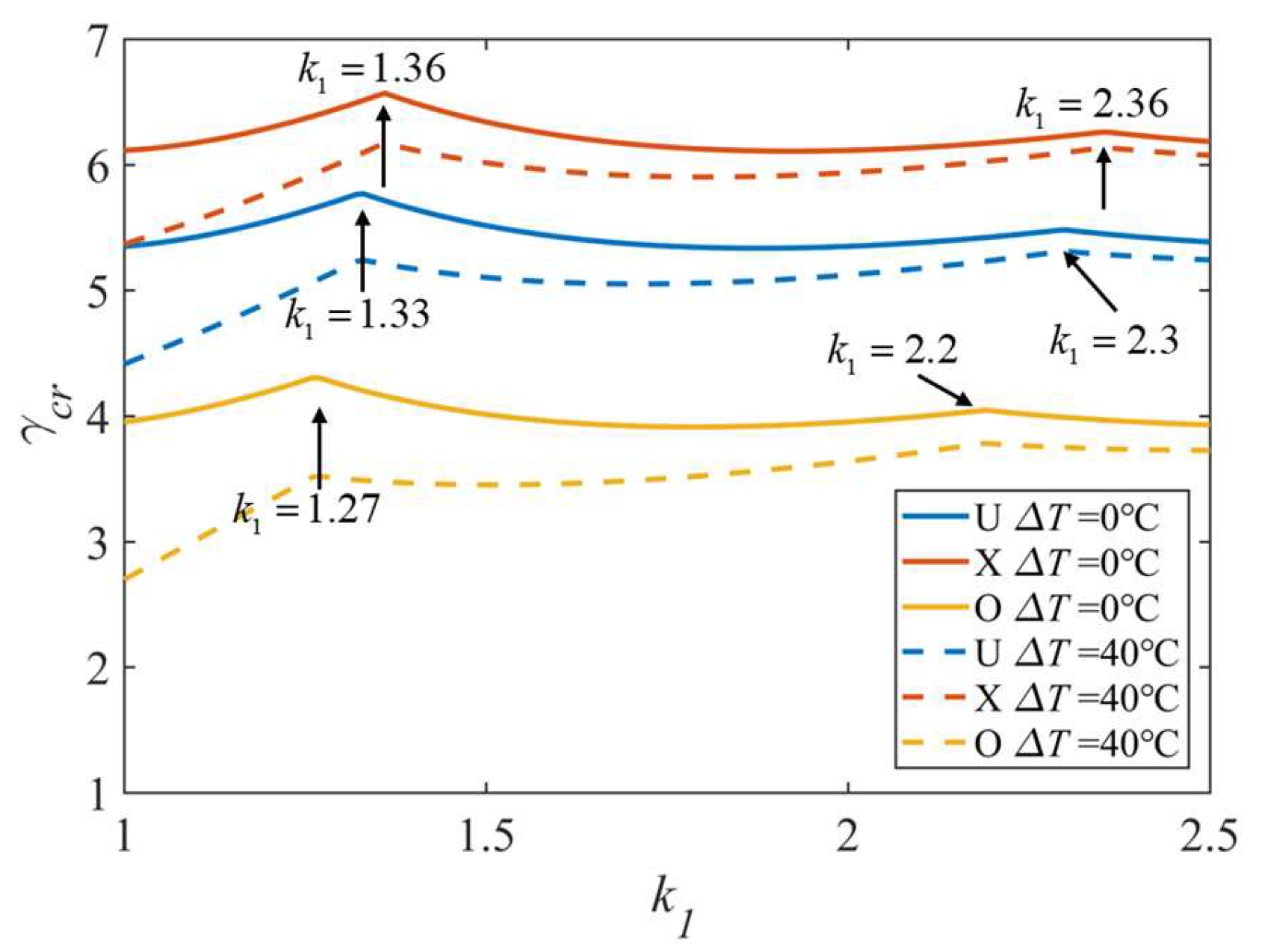
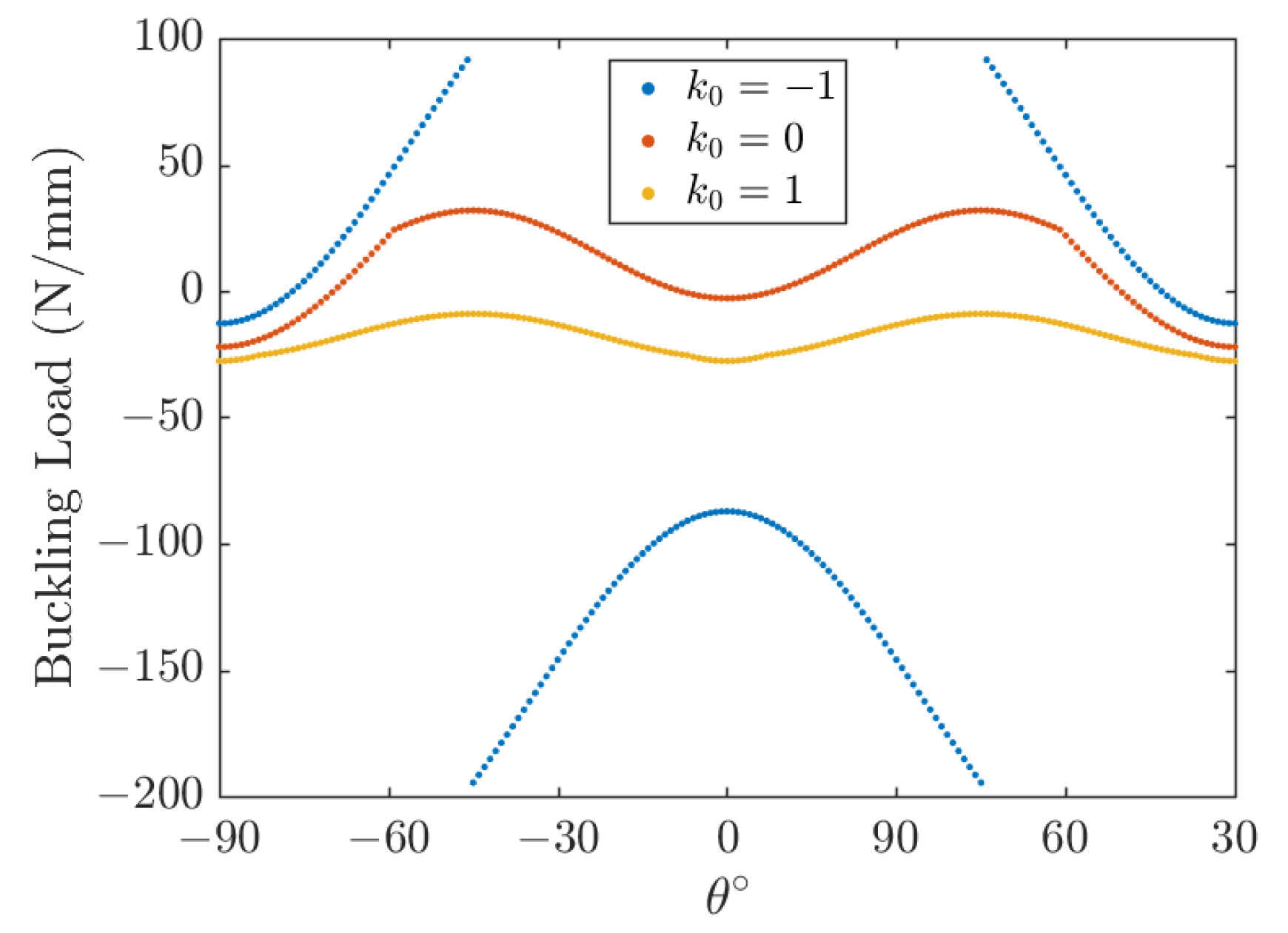
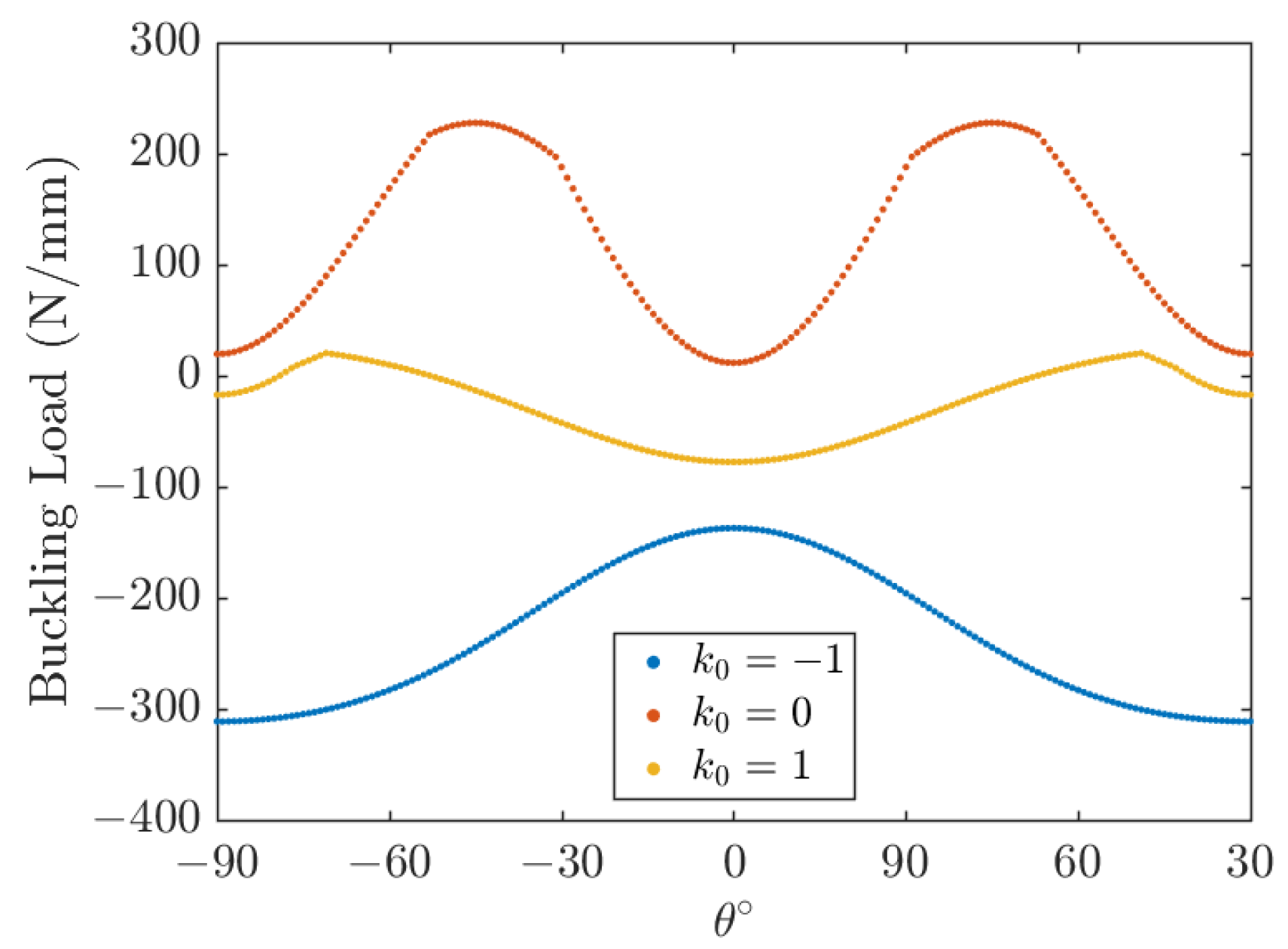
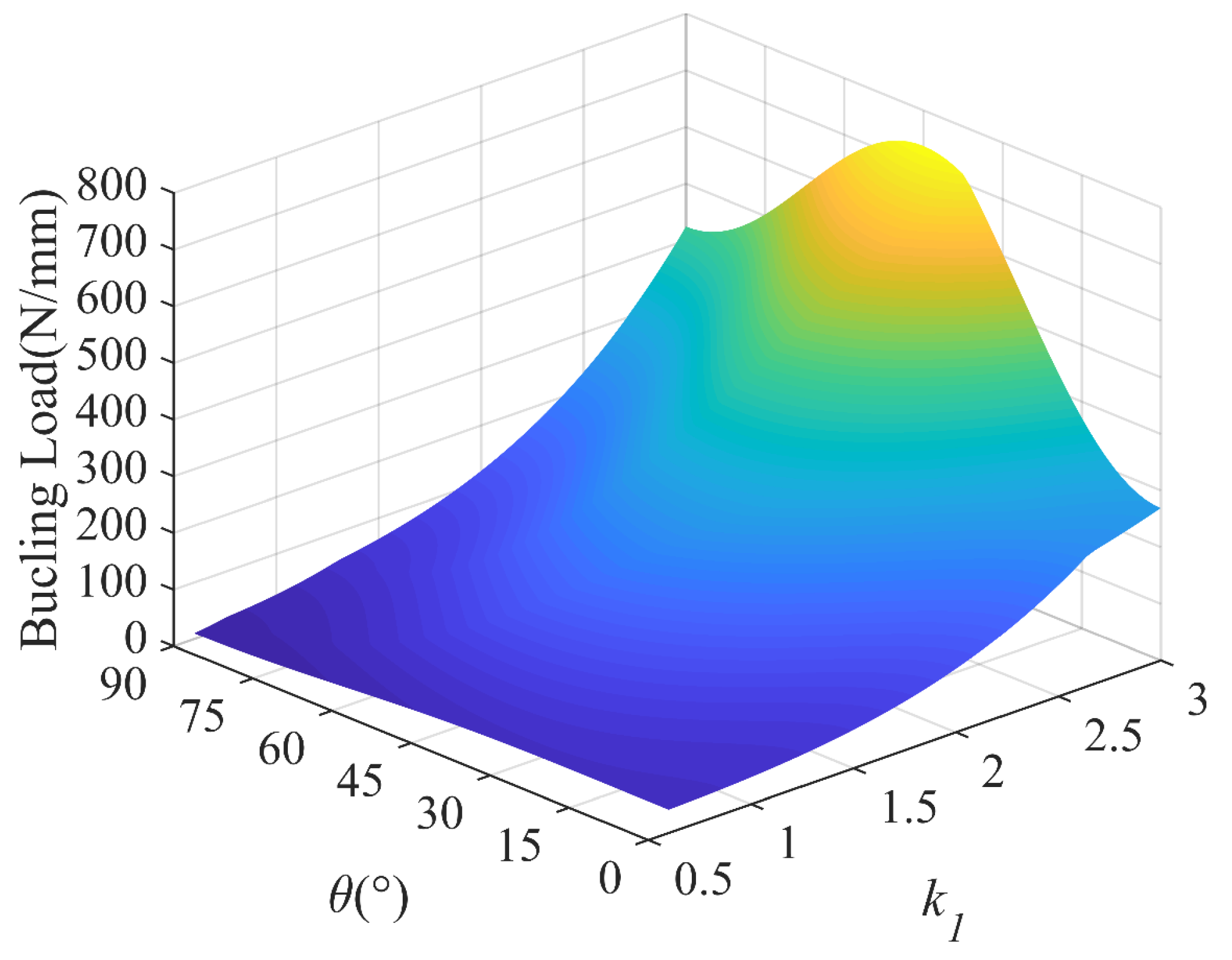
4.2.4. Effect of Lamination Angle
5. Conclusions
- (1)
- A larger corresponds to higher critical buckling loads; the buckling load decreases rapidly with the increase of the length-to-thickness ratio k2, then tends to become zero. The buckling loads are also significantly influenced by the lamination angle.
- (2)
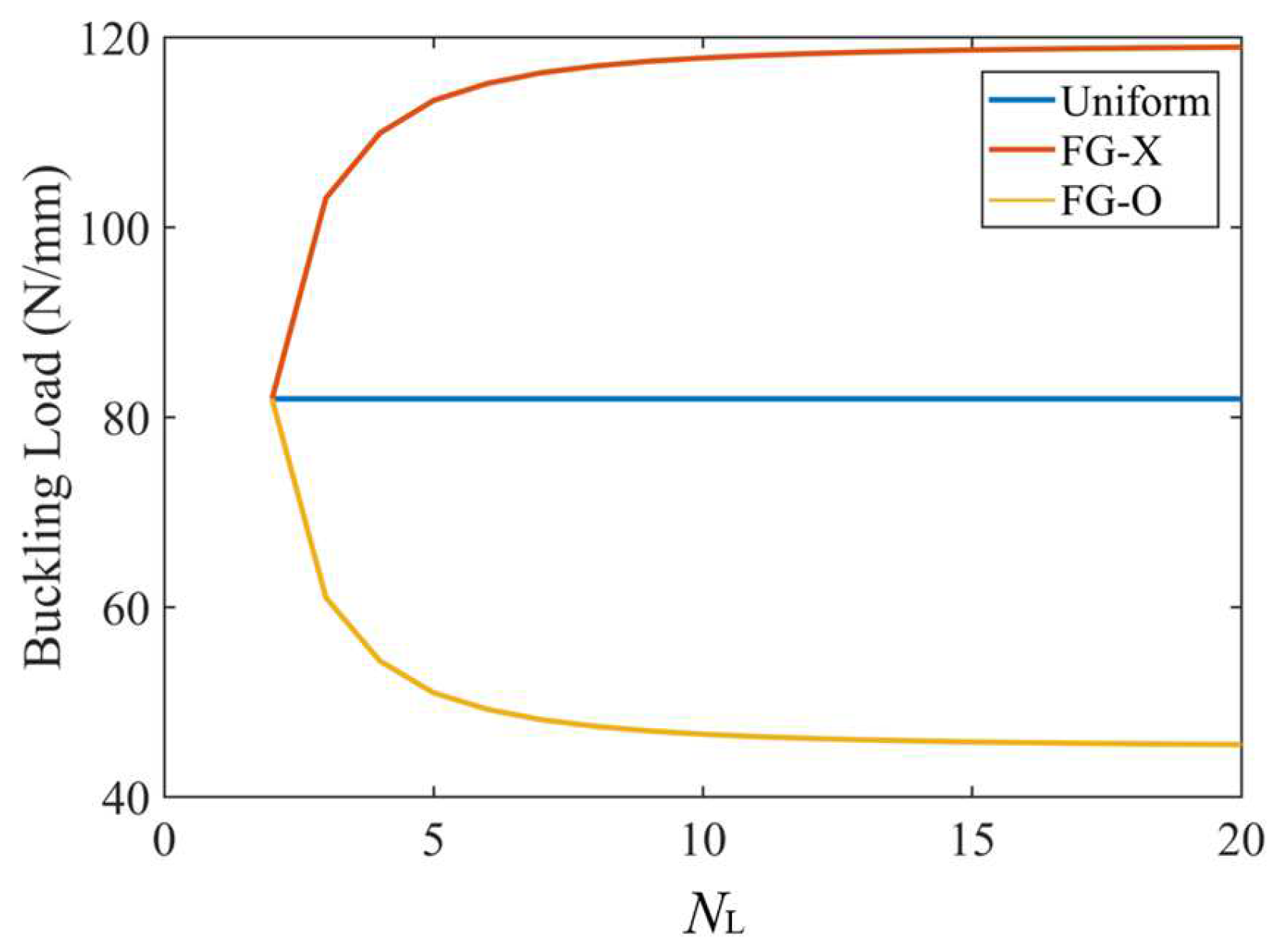
- X-shaped FG distribution is more effective than the other two distributions for reinforcing the plate for a higher buckling load, and compared to uniform distribution, the buckling load increased by 47%.
- (3)
- A functionally graded composited plate with 10~15 individual layers stacked up can achieve a sufficient in-plane load.
- (4)
- Critical buckling loads decrease with a temperature increase ranging from 0 °C to 80 °C.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Altin Karataş, M.; Gökkaya, H. A review on machinability of carbon fiber reinforced polymer (CFRP) and glass fiber reinforced polymer (GFRP) composite materials. Def. Technol. 2018, 14, 318–326. [Google Scholar] [CrossRef]
- Ogawa, H. Architectural application of carbon fibers-Development of new carbon fiber reinforced glulam. Carbon 2000, 38, 211–226. [Google Scholar] [CrossRef]
- Hashish, M. Trimming of CFRP Aircraft Components. In Proceedings of the American WJTA-IMCA Conference & Expo., Houston, TX, USA, 9–11 September 2013. [Google Scholar]
- Saleem, M.; Toubal, L.; Zitoune, R.; Bougherara, H. Investigating the effect of machining processes on the mechanical behavior of composite plates with circular holes. Compos. Part A Appl. Sci. Manuf. 2013, 55, 169–177. [Google Scholar] [CrossRef]
- Wang, C.; Liu, G.; An, Q.; Chen, M. Occurrence and formation mechanism of surface cavity defects during orthogonal milling of CFRP laminates. Compos. Part B Eng. 2017, 109, 10–22. [Google Scholar] [CrossRef]
- Soutis, C. Fibre reinforced composites in aircraft construction. Prog. Aerosp. Sci. 2005, 41, 143–151. [Google Scholar] [CrossRef]
- Byklum, E.; Steen, E.; Amdahl, L. A semi-analytical model for global buckling and postbuckling analysis of stiffened panels. Thin Walled Struct. 2004, 42, 701–717. [Google Scholar] [CrossRef]
- Fazzolari, F.A.; Carrera, E. Advanced variable kinematics Ritz and Galerkin formulations for accurate buckling and vibration analysis of anisotropic laminated composite plates. Compos. Struct. 2011, 94, 50–67. [Google Scholar] [CrossRef]
- Jaberzadeh, E.; Azhari, M. Elastic and inelastic local buckling of stiffened plates subjected to non-uniform compression using the Galerkin method. Appl. Math. Modell. 2009, 33, 1874–1885. [Google Scholar] [CrossRef]
- Ovesy, H.R.; Ghannadpour, S.A.M.; Zia-Dehkordi, E. Buckling analysis of moderately thick composite plates and plate structures using an exact finite strip. Compos. Struct. 2013, 95, 697–704. [Google Scholar] [CrossRef]
- Ovesy, H.R.; GhannadPour, S.A.M.; Morada, G. Geometric non-linear analysis of composite laminated plates with initial imperfection under end shortening, using two versions of finite strip method. Compos. Struct. 2005, 71, 307–314. [Google Scholar] [CrossRef]
- Ovesy, H.; Assaee, H. Buckling characteristics of some composite stiffened boxes under longitudinal compression and bending using finite strip approach. In Proceedings of the 44th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Norfolk, VA, USA, 7–10 April 2003; p. 1791. [Google Scholar]
- Ovesy, H.R.; Assaee, H. An investigation on the post-buckling behavior of symmetric cross-ply laminated plates using a semi-energy finite strip approach. Compos. Struct. 2005, 71, 365–370. [Google Scholar] [CrossRef]
- Vescovini, R.; Dozio, L.; D’Ottavio, M.; Polit, O. On the application of the Ritz method to free vibration and buckling analysis of highly anisotropic plates. Compos. Struct. 2018, 192, 460–474. [Google Scholar] [CrossRef]
- Feng, L.F.; Sun, T.S.; Ou, J.P. Elastic buckling analysis of horizontally-placed trapezoidally-corrugated steel shear walls stiffened with vertical steel strips. Thin Walled Struct. 2022, 180, 109894. [Google Scholar] [CrossRef]
- Wang, M.; Li, Z.M.; Qiao, P.Z. Semi-analytical solutions to buckling and free vibration analysis of carbon nanotube-reinforced composite thin plates. Compos. Struct. 2016, 144, 33–43. [Google Scholar] [CrossRef]
- Dawe, D.J.; Yuan, W.X. Overall and local buckling of sandwich plates with laminated faceplates, Part I: Analysis. Comput. Methods Appl. Mech. Eng. 2001, 190, 5197–5213. [Google Scholar] [CrossRef]
- Pandit, M.K.; Singh, B.N.; Sheikh, A.H. Buckling of laminated sandwich plates with soft core based on an improved higher order zigzag theory. Thin Walled Struct. 2008, 46, 1183–1191. [Google Scholar] [CrossRef]
- Falkowicz, K. Numerical Investigations of Perforated CFRP Z-Cross-Section Profiles, under Axial Compression. Materials 2022, 15, 6874. [Google Scholar] [CrossRef]
- Debski, H.; Samborski, S.; Rozylo, P.; Wysmulski, P. Stability and Load-Carrying Capacity of Thin-Walled FRP Composite Z-Profiles under Eccentric Compression. Materials 2020, 13, 2956. [Google Scholar] [CrossRef]
- Wysmulski, P. Non-linear analysis of the postbuckling behaviour of eccentrically compressed composite channel-section columns. Compos. Struct. 2023, 305, 116446. [Google Scholar] [CrossRef]
- Akbas, S.D. Wave propagation of a functionally graded beam in thermal environments. Steel Compos. Struct. 2015, 19, 1421–1447. [Google Scholar] [CrossRef]
- Avcar, M. Free vibration of imperfect sigmoid and power law functionally graded beams. Steel Compos. Struct. 2019, 30, 603–615. [Google Scholar] [CrossRef]
- Madenci, E. A refined functional and mixed formulation to static analyses of fgm beams. Struct. Eng. Mech. 2019, 69, 427–437. [Google Scholar] [CrossRef]
- Birman, V.; Kardomateas, G.A. Review of current trends in research and applications of sandwich structures. Compos. Part B Eng. 2018, 142, 221–240. [Google Scholar] [CrossRef]
- Ghaffari Mosanenzadeh, S.; Naguib, H.E.; Park, C.B.; Atalla, N. Design and development of novel bio-based functionally graded foams for enhanced acoustic capabilities. J. Mater. Sci. 2014, 50, 1248–1256. [Google Scholar] [CrossRef]
- Li, Y.; Feng, Z.Y.; Hao, L.; Huang, L.J.; Xin, C.X.; Wang, Y.S.; Bilotti, E.; Essa, K.; Zhang, H.; Li, Z.; et al. A Review on Functionally Graded Materials and Structures via Additive Manufacturing: From Multi-Scale Design to Versatile Functional Properties. Adv. Mater. Technol. 2020, 5, 1900981. [Google Scholar] [CrossRef]
- Hu, Z.; Zhou, C.; Ni, Z.; Lin, X.; Li, R. New symplectic analytic solutions for buckling of CNT reinforced composite rectangular plates. Compos. Struct. 2023, 303, 116361. [Google Scholar] [CrossRef]
- Lei, Z.X.; Liew, K.M.; Yu, J.L. Buckling analysis of functionally graded carbon nanotube-reinforced composite plates using the element-free kp-Ritz method. Compos. Struct. 2013, 98, 160–168. [Google Scholar] [CrossRef]
- Lei, Z.X.; Zhang, L.W.; Liew, K.M. Buckling analysis of CNT reinforced functionally graded laminated composite plates. Compos. Struct. 2016, 152, 62–73. [Google Scholar] [CrossRef]
- Luo, X.B.; Li, D.; Liu, C.L.; Pan, J.H. Buckling analysis of variable stiffness composite plates with elliptical cutouts using an efficient RPIM based on naturally stabilized nodal integration scheme. Compos. Struct. 2022, 302, 116243. [Google Scholar] [CrossRef]
- Wang, Z.X.; Shen, H.S. Nonlinear dynamic response of nanotube-reinforced composite plates resting on elastic foundations in thermal environments. Nonlinear Dyn. 2012, 70, 735–754. [Google Scholar] [CrossRef]
- Shen, H.S.; Xiang, Y.; Lin, F. Nonlinear bending of functionally graded graphene-reinforced composite laminated plates resting on elastic foundations in thermal environments. Compos. Struct. 2017, 170, 80–90. [Google Scholar] [CrossRef]
- Shen, H.S. Hygrothermal effects on the postbuckling of shear deformable laminated plates. Int. J. Mech. Sci. 2001, 43, 1259–1281. [Google Scholar] [CrossRef]
- Whitney, J.M.; Ashton, J.E. Effect of Environment on the Elastic Response of Layered Composite Plates. AIAA J. 1971, 9, 1708–1713. [Google Scholar] [CrossRef]
- Shen, H.S.; Zhang, C.L. Thermal buckling and postbuckling behavior of functionally graded carbon nanotube-reinforced composite plates. Mater. Des. 2010, 31, 3403–3411. [Google Scholar] [CrossRef]
- Shen, H.S.; Xiang, Y.; Lin, F.; Hui, D. Buckling and postbuckling of functionally graded graphene-reinforced composite laminated plates in thermal environments. Compos. Part B Eng. 2017, 119, 67–78. [Google Scholar] [CrossRef]
- Song, M.T.; Yang, J.; Kitipornchai, S. Bending and buckling analyses of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Part B Eng. 2018, 134, 106–113. [Google Scholar] [CrossRef]
- Thai, C.H.; Ferreira, A.J.M.; Tran, T.D.; Phung-Van, P. Free vibration, buckling and bending analyses of multilayer functionally graded graphene nanoplatelets reinforced composite plates using the NURBS formulation. Compos. Struct. 2019, 220, 749–759. [Google Scholar] [CrossRef]
- Wu, T.L.; Shukla, K.K.; Huang, J.H. Post-buckling analysis of functionally graded rectangular plates. Compos. Struct. 2007, 81, 1–10. [Google Scholar] [CrossRef]
- Zaitoun, M.W.; Chikh, A.; Tounsi, A.; Al-Osta, M.A.; Sharif, A.; Al-Dulaijan, S.U.; Al-Zahrani, M.M. Influence of the visco-Pasternak foundation parameters on the buckling behavior of a sandwich functional graded ceramic–metal plate in a hygrothermal environment. Thin Walled Struct. 2022, 170, 108549. [Google Scholar] [CrossRef]
- Abaimov, S.G.; Khudyakova, A.A.; Lomov, S.V. On the closed form expression of the Mori-Tanaka theory prediction for the engineering constants of a unidirectional fiber-reinforced ply. Compos. Struct. 2016, 142, 1–6. [Google Scholar] [CrossRef]
- Reddy, J.N. Mechanics of Laminated Composite Plates and Shells: Theory and Analysis; CRC Press: Boca Raton, FL, USA, 2003; pp. 320–321. [Google Scholar]
- Kassapoglou, C.; Kassapoglou, C. Design and Analysis of Composite Structures: With Applications to Aerospace Structures; John Wiley & Sons, Incorporated: New York, NY, USA, 2010; pp. 126–128. [Google Scholar]
- Li, D.D.; Deng, Z.B.; Chen, G.P.; Ma, T. Mechanical and thermal buckling of exponentially graded sandwich plates. J. Therm. Stresses 2018, 41, 883–902. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Habibi, S. Nonlinear eccentric low-velocity impact response of a polymer-carbon nanotube-fiber multiscale nanocomposite plate resting on elastic foundations in hygrothermal environments. Mech. Adv. Mater. Struct. 2018, 25, 425–438. [Google Scholar] [CrossRef]




| k | a/b | Theory | |||||
|---|---|---|---|---|---|---|---|
| 5 | 10 | 20 | 25 | 40 | |||
| 0 | 0.5 | Reddy [43] | 13.9000 | 18.1260 | 21.8780 | 22.8740 | 24.5900 |
| Present | 13.9000 | 18.1265 | 21.8778 | 22.8738 | 24.5899 | ||
| Ansys | 13.8666 | 17.6971 | 20.4871 | 20.9996 | 21.3372 | ||
| 1.0 | Reddy [43] | 5.6500 | 6.3470 | 6.9610 | 7.1240 | 7.4040 | |
| Present | 5.6500 | 6.3470 | 6.9611 | 7.1238 | 7.4037 | ||
| Ansys | 6.0568 | 6.6009 | 7.0363 | 7.1347 | 7.2598 | ||
| 1.5 | Reddy [43] | 5.2330 | 5.2770 | 5.3100 | 5.3180 | 5.3320 | |
| Present | 5.2333 | 5.2768 | 5.3099 | 5.3182 | 5.3322 | ||
| Ansys | 6.1796 | 5.8636 | 5.6220 | 5.5597 | 5.4452 | ||
| 1 | 0.5 | Reddy [43] | 11.1200 | 12.6940 | 13.9220 | 14.2480 | 14.7660 |
| Present | 11.1200 | 12.6941 | 13.9222 | 14.2475 | 14.7661 | ||
| Ansys | 11.6391 | 13.3583 | 13.7016 | 13.6693 | 13.3272 | ||
| 1.0 | Reddy [43] | 2.8250 | 3.1740 | 3.4840 | 3.5620 | 3.7020 | |
| Present | 2.8250 | 3.1735 | 3.4806 | 3.5619 | 3.7019 | ||
| Ansys | 3.0285 | 3.3005 | 3.5183 | 3.5675 | 3.6300 | ||
| 1.5 | Reddy [43] | 1.6100 | 1.6240 | 1.6340 | 1.6360 | 1.6410 | |
| Present | 1.6103 | 1.6236 | 1.6338 | 1.6364 | 1.6407 | ||
| Ansys | 1.7208 | 1.6935 | 1.6671 | 1.6590 | 1.6414 | ||
| Plate | Tsung-Lin Wu [40] | Present |
|---|---|---|
| Alumina | 3.6498 | 3.6152 |
| Aluminum | 0.67 | 0.6660 |
| Material properties of fiber(carbon): | |||
| = 230 GPa | = 23 GPa | = 9 GPa | |
| = 0.2 | |||
| Material properties of epoxy matrix: | |||
| = (3.51 − 0.003 ΔT) GPa | |||
| Lay-Up | UD | FG_X | FG_O | |
|---|---|---|---|---|
| Ansys | Present | |||
| [90/0/−45/45]s, [90/0/45/−45]s, [0/90/−45/45]s, [0/90/45/−45]s | 50.78 | 51.46 | 69.11 ( + 34%) | 34.61 (−33%) |
| [90/−45/45/0]s, [90/45/−45/0]s | 59.41 | 61.25 | 73.48 (+20%) | 41.43 (−32%) |
| [90/−45/0/45]s, [90/45/0/−45]s | 54.63 | 57.99 | 77.40 (+33%) | 37.62 (−35%) |
| [0/−45/90/45]s, [0/45/90/−45]s | 54.63 | 57.99 | 79.14 (+36%) | 37.62 (−35%) |
| [0/−45/45/90]s, [0/45/−45/90]s | 59.41 | 61.25 | 81.87 (+34%) | 41.43 (−32%) |
| [−45/90/45/0]s, [−45/0/45/90]s, [45/90/−45/0]s, [45/0/−45/90]s | 63.26 | 71.04 | 103.94 (+46%) | 38.72 (−45%) |
| [−45/45/90/0]s, [−45/45/0/90]s, [45/−45/90/0]s, [45/−45/0/90]s | 74.24 | 77.57 | 113.97 (+47%) | 41.73 (−46%) |
| 0.05 | 0.1 | 0.15 | 0.2 | 0.25 | 0.3 | |
|---|---|---|---|---|---|---|
| Uniaxial compression | ||||||
| Uniform | 14.78 | 21.17(+43%) | 27.59(+30%) | 34.05(+23%) | 40.55(+19%) | 47.11(+16%) |
| FG-X | 17.84 | 27.34(+53%) | 36.93(+35%) | 46.65(+26%) | 56.51(+21%) | 66.58(+18%) |
| FG-O | 11.73 | 15.04(+28%) | 18.37(+22%) | 21.72(+18%) | 25.09(+16%) | 28.48(+14%) |
| Biaxial compression | ||||||
| Uniform | 7.39 | 10.58(+43%) | 13.79(+30%) | 17.02(+23%) | 20.28(+19%) | 23.56(+16%) |
| FG-X | 8.92 | 13.67(+53%) | 18.47(+35%) | 23.32(+26%) | 28.26(+21%) | 33.29(+18%) |
| FG-O | 5.86 | 7.52(+28%) | 9.19(+22%) | 10.86(+18%) | 12.54(+15%) | 14.24(+14%) |
| Distribution Pattern | Temperature Difference | k1 = 1 | k1 = 2.5 | ||
|---|---|---|---|---|---|
| γcr | Difference | γcr | Difference | ||
| Uniform | ΔT = 0 ℃ | 5.35 | −17.57% | 5.39 | −2.60% |
| ΔT = 40 ℃ | 4.41 | 5.24 | |||
| FG-X | ΔT = 0 ℃ | 6.11 | −12.11% | 6.18 | −1.78% |
| ΔT = 40 ℃ | 5.37 | 6.07 | |||
| FG-O | ΔT = 0 ℃ | 3.95 | −31.65% | 3.93 | −5.09% |
| ΔT = 40 ℃ | 2.70 | 3.73 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; You, Q.; Gao, Y.; Hua, F.; Fu, W.; Huang, Q.; Wang, Y. Buckling Analysis on Resin Base Laminated Plate Reinforced with Uniform and Functional Gradient Distribution of Carbon Fiber in Thermal Environment. Polymers 2023, 15, 2086. https://doi.org/10.3390/polym15092086
Zhou X, You Q, Gao Y, Hua F, Fu W, Huang Q, Wang Y. Buckling Analysis on Resin Base Laminated Plate Reinforced with Uniform and Functional Gradient Distribution of Carbon Fiber in Thermal Environment. Polymers. 2023; 15(9):2086. https://doi.org/10.3390/polym15092086
Chicago/Turabian StyleZhou, Xiaoqiang, Qingquan You, Yuan Gao, Fenfei Hua, Wanbiao Fu, Qingyang Huang, and Yuanfang Wang. 2023. "Buckling Analysis on Resin Base Laminated Plate Reinforced with Uniform and Functional Gradient Distribution of Carbon Fiber in Thermal Environment" Polymers 15, no. 9: 2086. https://doi.org/10.3390/polym15092086
APA StyleZhou, X., You, Q., Gao, Y., Hua, F., Fu, W., Huang, Q., & Wang, Y. (2023). Buckling Analysis on Resin Base Laminated Plate Reinforced with Uniform and Functional Gradient Distribution of Carbon Fiber in Thermal Environment. Polymers, 15(9), 2086. https://doi.org/10.3390/polym15092086






