Nonlinear Mechanical Property of 3D Braided Composites with Multi-Types Micro-Distortion: A Quantitative Evaluation
Abstract
1. Introduction
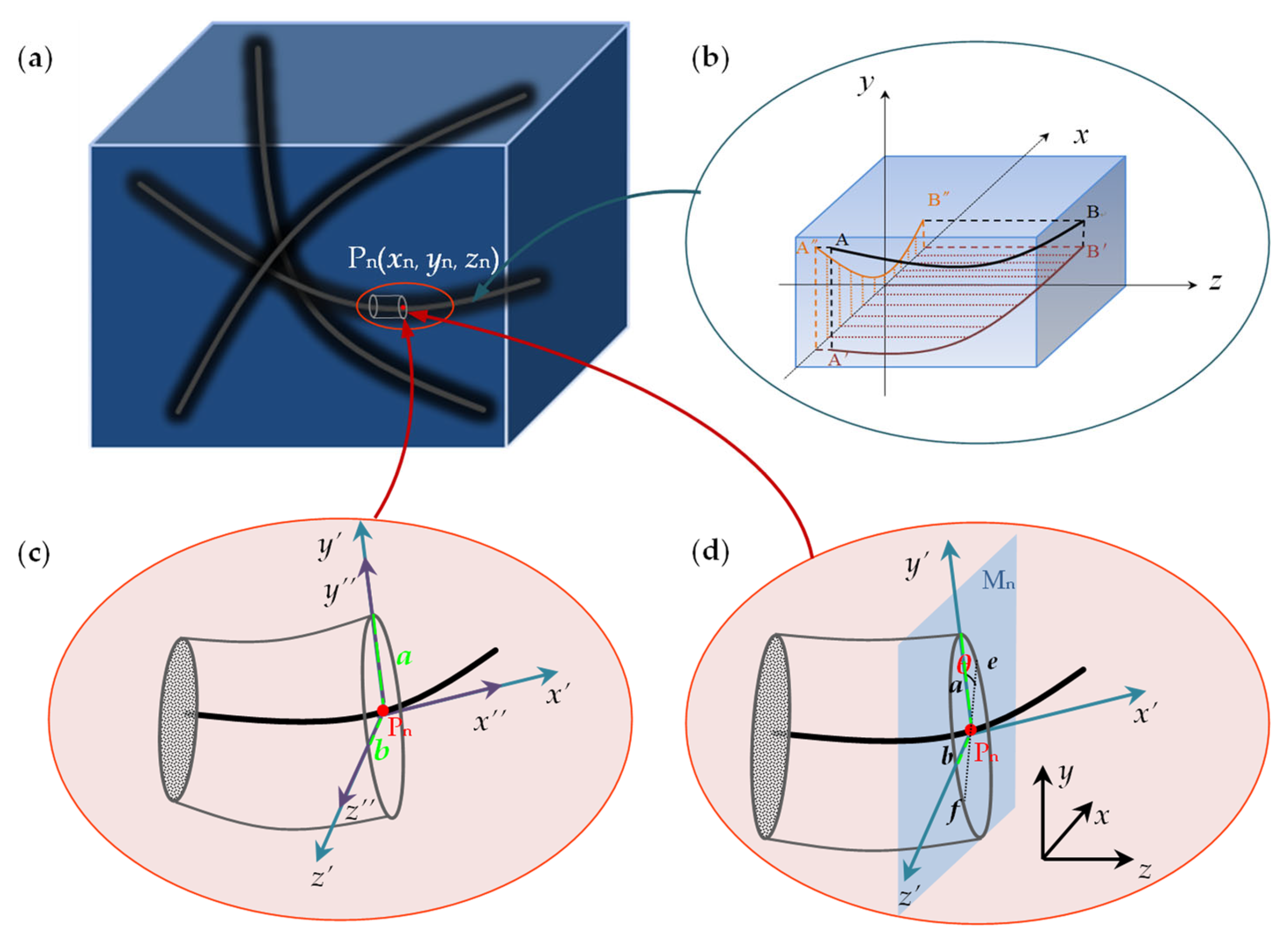
2. Characteristic Parameters and Properties of Distortion Yarn
2.1. Introduction of Characteristic Parameters
2.2. Expansion of Characteristic Parameters
2.3. Theoretical Derivation
3. Results and Discussion
3.1. Mechanical Properties’ Response of Distortion Yarn
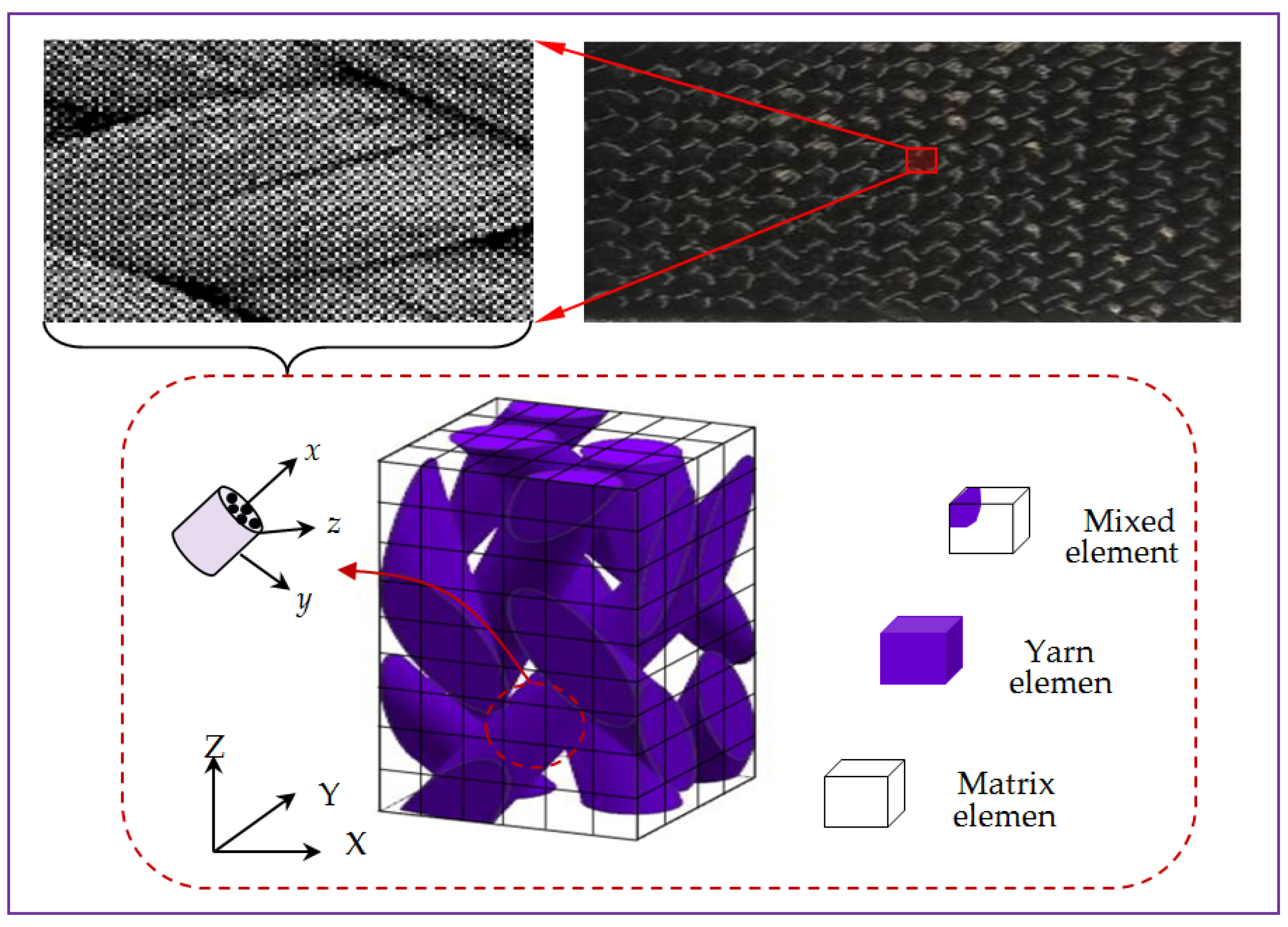
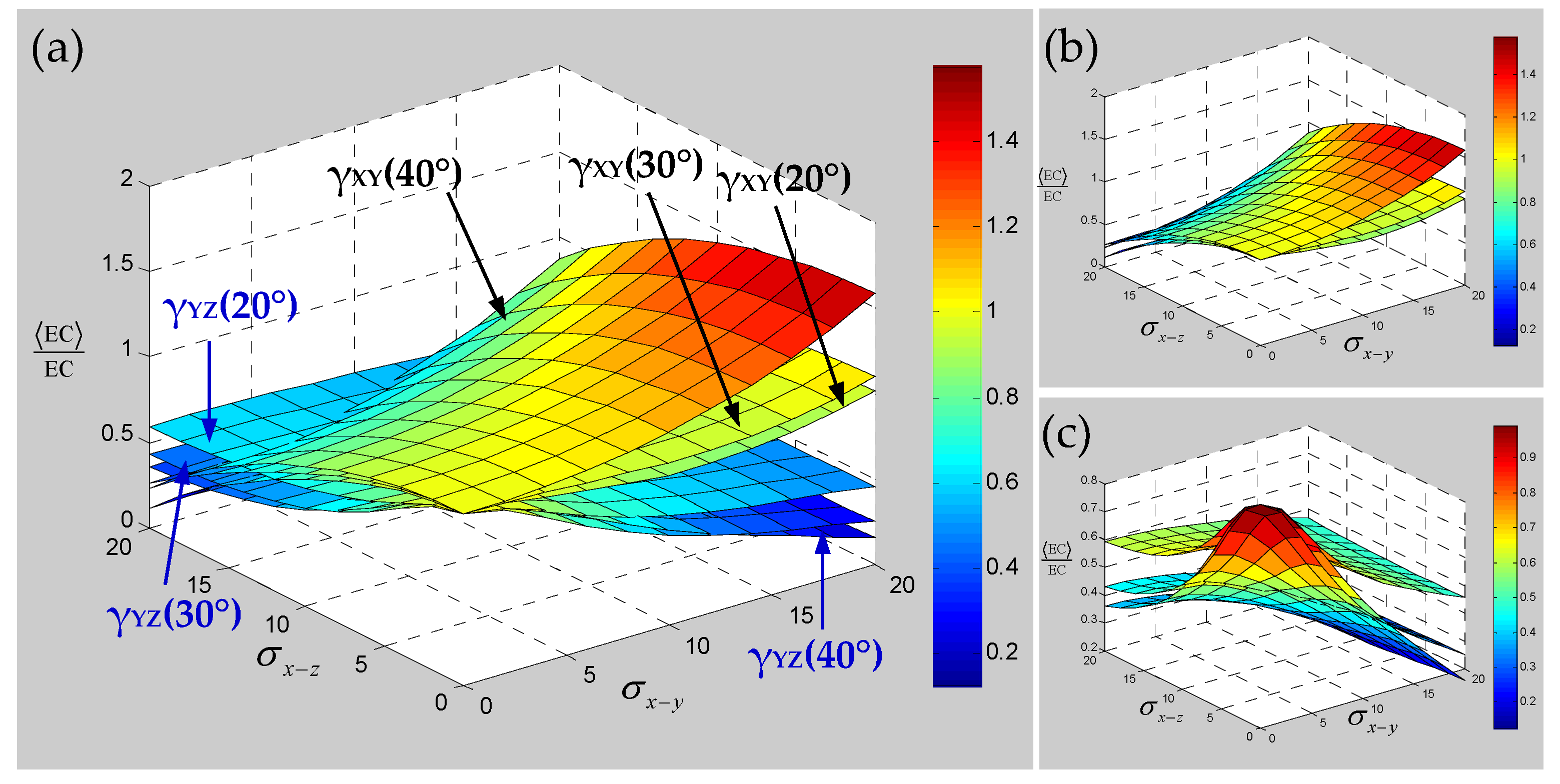
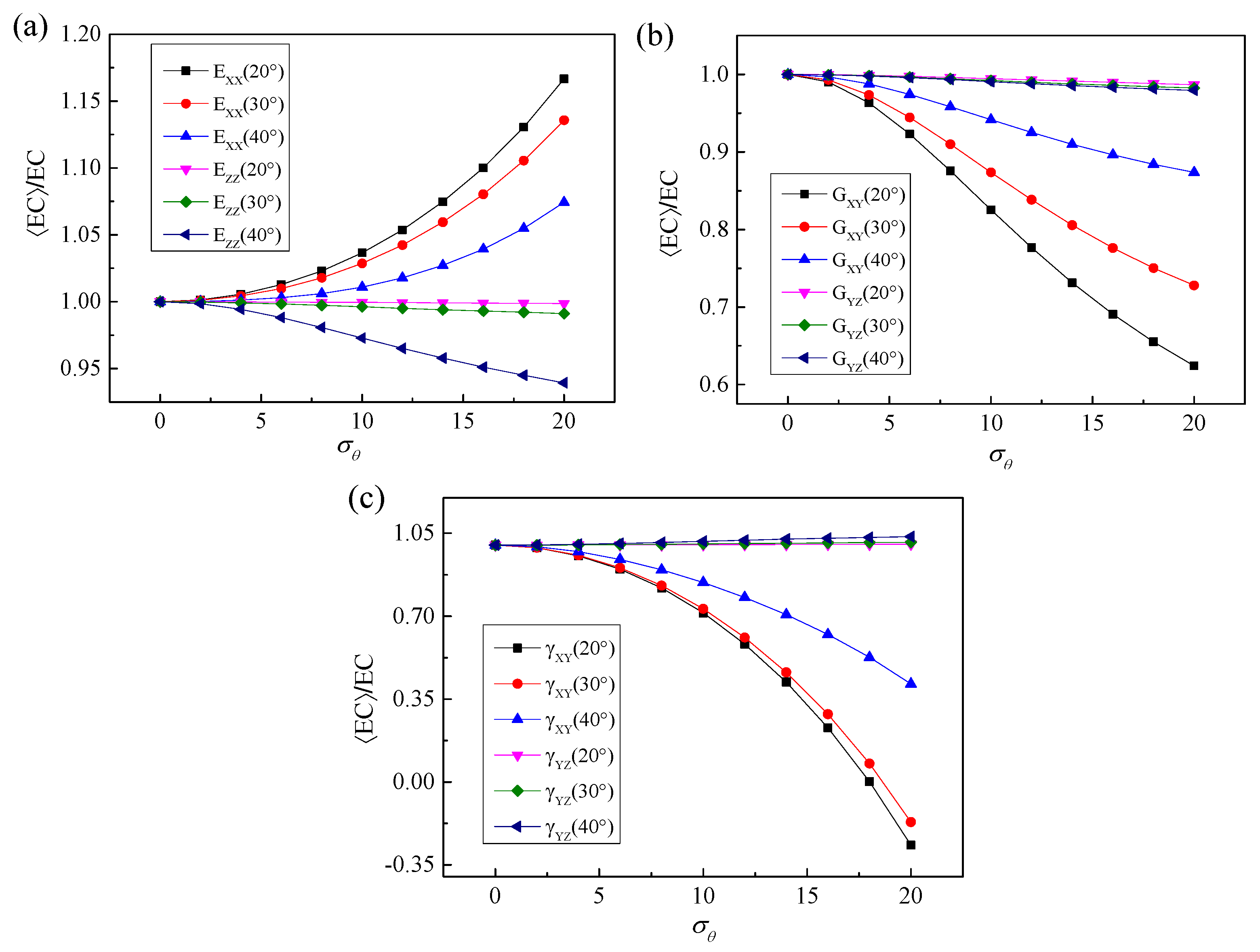
3.2. Mechanical Properties’ Response of 3D Braided Carbon/Resin Composites
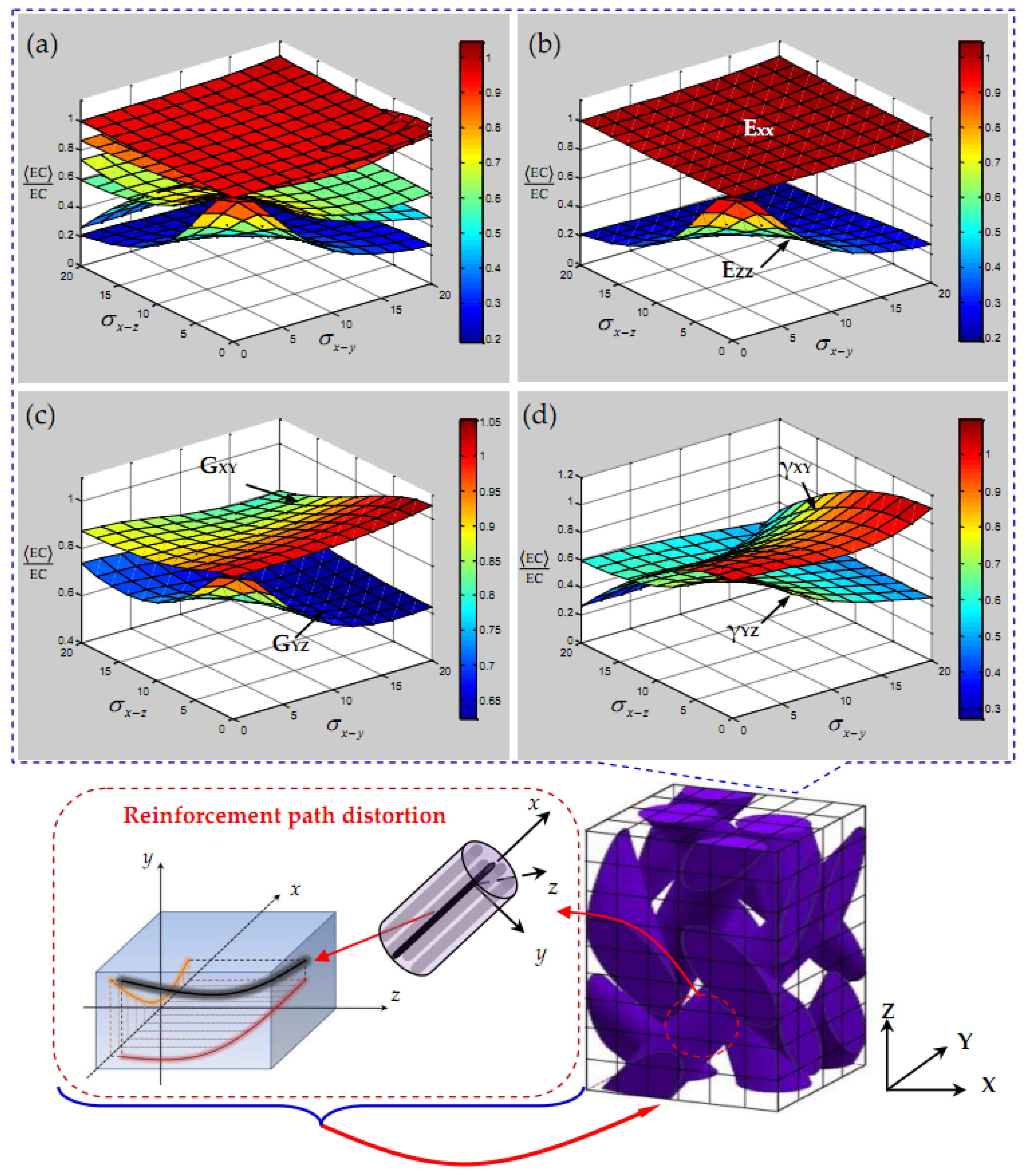
3.2.1. Influence of Random Parameters
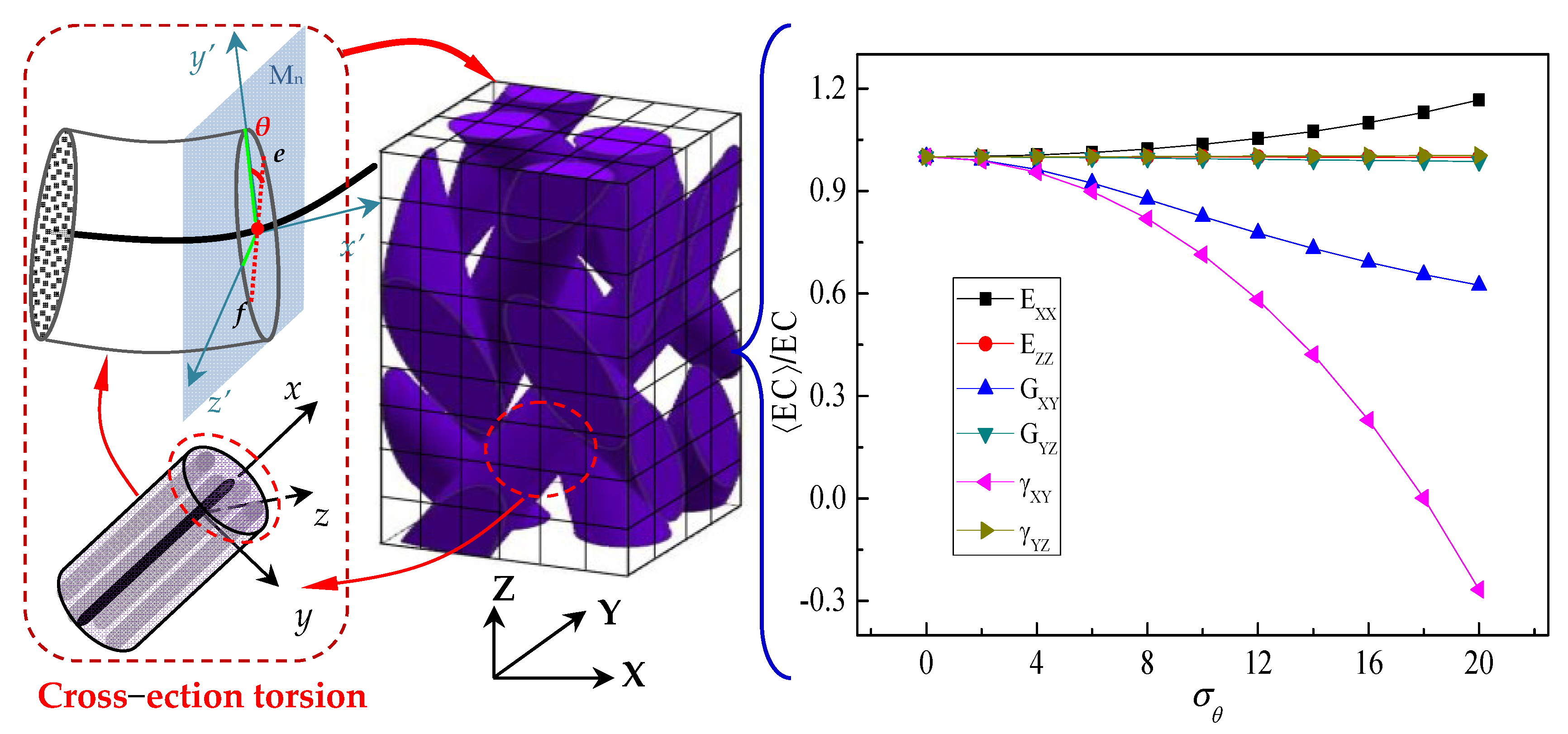
3.2.2. Influence of Braiding Angle
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, J.L.; L’Hostis, G.; Hamila, N.; Wang, P. Analysis of thermomechanical behavior of the tubular braided fabrics with flax/polyamide commingled yarns. Polymers 2023, 15, 637. [Google Scholar] [CrossRef]
- Zhai, J.J.; Zeng, T.; Xu, G.D.; Wang, Z.H.; Cheng, S.; Fang, D.N. A multi-scale finite element method for failure analysis of three-dimensional braided composite structures. Compos. Part B Eng. 2017, 110, 476–486. [Google Scholar] [CrossRef]
- Zhou, W.; Wang, H.; Chen, Y.; Wang, Y. A methodology to obtain the accurate RVEs by a multiscale numerical simulation of the 3D braiding process. Polymers 2022, 14, 4210. [Google Scholar] [CrossRef]
- Zhang, D.; Zheng, X.T.; Zhou, J.; Song, X.Y.; Jia, P.; Liu, H.B.; Liu, X.C. Effect of braiding architectures on the mechanical and failure behavior of 3D braided composites: Experimental investigation. Polymers 2022, 14, 1916. [Google Scholar] [CrossRef]
- Li, Z.G.; Li, D.S.; Zhu, H.; Guo, Z.X.; Jiang, L. Mechanical properties prediction of 3D angle-interlock woven composites by finite element modeling method. Mater. Today Commun. 2020, 22, 100769. [Google Scholar] [CrossRef]
- Ko, F. Three-Dimensional Fabrics for Composites an Introduction to the Magna Weave Structure. In Proceedings of the ICCM-4 Conference, Tokyo, Japan, 25–28 October 1982; pp. 1609–1619. [Google Scholar]
- Wang, Y.; Wang, A. On the topological yarn structure in 3D rectangular and tubular braided perform. Compos. Sci. Technol. 1994, 51, 575–586. [Google Scholar] [CrossRef]
- Wu, D. Three-Cell model and 5D braided structural composites. Compos. Sci. Technol. 1996, 56, 225–233. [Google Scholar] [CrossRef]
- Chen, L.; Tao, X.; Choy, C. On the microstructure of three-dimensional braided preforms. Compos. Sci. Technol. 1999, 59, 391–404. [Google Scholar] [CrossRef]
- Zheng, X.; Ye, T.Q. Microstructure analysis of 4-step three-dimensional braided composite. Chin. J. Aeronaut 2003, 16, 142–150. [Google Scholar] [CrossRef]
- Li, D.; Chen, L.; Li, J. Microstructure and unit-cell geometry of four-step three-dimensional rectangular braided composites. J. Reinf. Plast. Comp. 2010, 29, 3353–3363. [Google Scholar] [CrossRef]
- Jiang, L.; Zeng, T.; Yan, S. Predicting mechanical properties of 3D braided composites using a helix geometry model. Polym. Polym. Compos. 2011, 100, 511–516. [Google Scholar] [CrossRef]
- Han, Q.R.; Li, J.L.; Li, X.M. Unit cell geometry of 3-D braided structure of composites. Acta Mater. Compos. Sin. 1996, 13, 76–80. [Google Scholar]
- Zhang, C.; Xu, X. Finite element analysis of 3D braided composites based on three unit-cell models. Compos. Struct. 2013, 98, 130–142. [Google Scholar] [CrossRef]
- Li, D.; Li, J.; Chen, L. Finite element analysis of mechanical properties of 3D four directional rectangular braided composites part 1: Microgeometry and 3D finite element model. Appl. Compos. Mater. 2010, 17, 373–387. [Google Scholar] [CrossRef]
- Xu, K.; Qian, X. Microstructure analysis and multi-unit cell model of three dimensionally four-directional braided composites. Appl. Compos. Mater. 2015, 22, 29–50. [Google Scholar] [CrossRef]
- Zhang, D.; Sun, Y.; Wang, X. Meso-scale finite element analyses of three-dimensional five-directional braided composites subjected to uniaxial and biaxial loading. J. Reinf. Plast. Comp. 2015, 34, 1989–2005. [Google Scholar] [CrossRef]
- Zhu, Y.; Cui, H.; Wen, W. Microstructure model and stiffness prediction of 3D braided composites considering yarns′ cross-section variation. Acta Mater. Compos. Sin. 2012, 29, 187–196. [Google Scholar]
- Wang, R.; Liu, X.; Hu, D. Improved unit cell model and elastic constant prediction method of 3D four-directional braided composites. Acta Mater. Compos. Sin. 2017, 34, 1973–1981. [Google Scholar]
- Fang, G.; Liang, J. A review of numerical modeling of three-dimensional braided textile composites. J. Compos. Mater. 2011, 45, 2415–2436. [Google Scholar] [CrossRef]
- Gu, Q.; Quan, Z.; Yu, J. Structural modeling and mechanical characterizing of three-dimensional four-step braided composites: A review. Compos. Struct. 2019, 207, 119–128. [Google Scholar] [CrossRef]
- Yushanov, S.P.; Bogdanovich, A.E. Stochastic theory of composite materials with random waviness of the reinforcements. Int. J. Solids Struct. 1998, 35, 2901–2930. [Google Scholar] [CrossRef]
- Yushanov, S.P.; Bogdanovich, A.E. Fiber waviness in textile composites and its stochastic modeling. Mech. Compos. Mater. 2000, 36, 297–318. [Google Scholar] [CrossRef]
- Amato, E. Nonlinearities in mechanical behavior of textile composites. Compos. Struct. 2005, 71, 61–67. [Google Scholar]
- Kang, H.; Shan, Z.; Zang, Y.; Liu, F. Effect of yarn distortion on the mechanical properties of fiber-bar composites reinforced by three-dimensional weaving. Appl. Compos. Mater. 2016, 23, 119–138. [Google Scholar] [CrossRef]
- Adumitroaie, A.; Barbero, E. Beyond plain weave fabrics-II. mechanical properties. Compos. Struct. 2011, 93, 1449–1462. [Google Scholar] [CrossRef]
- Wang, H.; Wang, Z.W. A variable metric stochastic theory of textile composites with random geometric parameters of yarn cross-section. Compos. Struct. 2015, 126, 78–88. [Google Scholar] [CrossRef]
- Fang, G.D.; Liang, J.; Wang, Y.; Wang, B.L. The effect of yarn distortion on the mechanical properties of 3D four-directional braided composites. Compos. Part A 2009, 40, 343–350. [Google Scholar]
- Pugachev, V.S. Theory of Random Functiom and Its Application to Control Problems; Pergamon Press: London, UK, 1965. [Google Scholar]










| Component | Modulus (GPa) | γ12 | |||
|---|---|---|---|---|---|
| E11 | E22 | E12 | E23 | ||
| TDE-86 resin | 3.45 | 3.45 | — | — | 0.35 |
| T700-12K fiber | 215.6 | 17.21 | 12.92 | 9.3 | 0.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhai, J.; Kong, X.; Wang, L.; Yan, S.; Jiang, L.; Cai, Z. Nonlinear Mechanical Property of 3D Braided Composites with Multi-Types Micro-Distortion: A Quantitative Evaluation. Polymers 2023, 15, 1428. https://doi.org/10.3390/polym15061428
Zhai J, Kong X, Wang L, Yan S, Jiang L, Cai Z. Nonlinear Mechanical Property of 3D Braided Composites with Multi-Types Micro-Distortion: A Quantitative Evaluation. Polymers. 2023; 15(6):1428. https://doi.org/10.3390/polym15061428
Chicago/Turabian StyleZhai, Junjun, Xiangxia Kong, Luchen Wang, Shi Yan, Lili Jiang, and Zhiwei Cai. 2023. "Nonlinear Mechanical Property of 3D Braided Composites with Multi-Types Micro-Distortion: A Quantitative Evaluation" Polymers 15, no. 6: 1428. https://doi.org/10.3390/polym15061428
APA StyleZhai, J., Kong, X., Wang, L., Yan, S., Jiang, L., & Cai, Z. (2023). Nonlinear Mechanical Property of 3D Braided Composites with Multi-Types Micro-Distortion: A Quantitative Evaluation. Polymers, 15(6), 1428. https://doi.org/10.3390/polym15061428






