Variation in the Optical Properties of PEO-Based Composites via a Green Metal Complex: Macroscopic Measurements to Explain Microscopic Quantum Transport from the Valence Band to the Conduction Band
Abstract
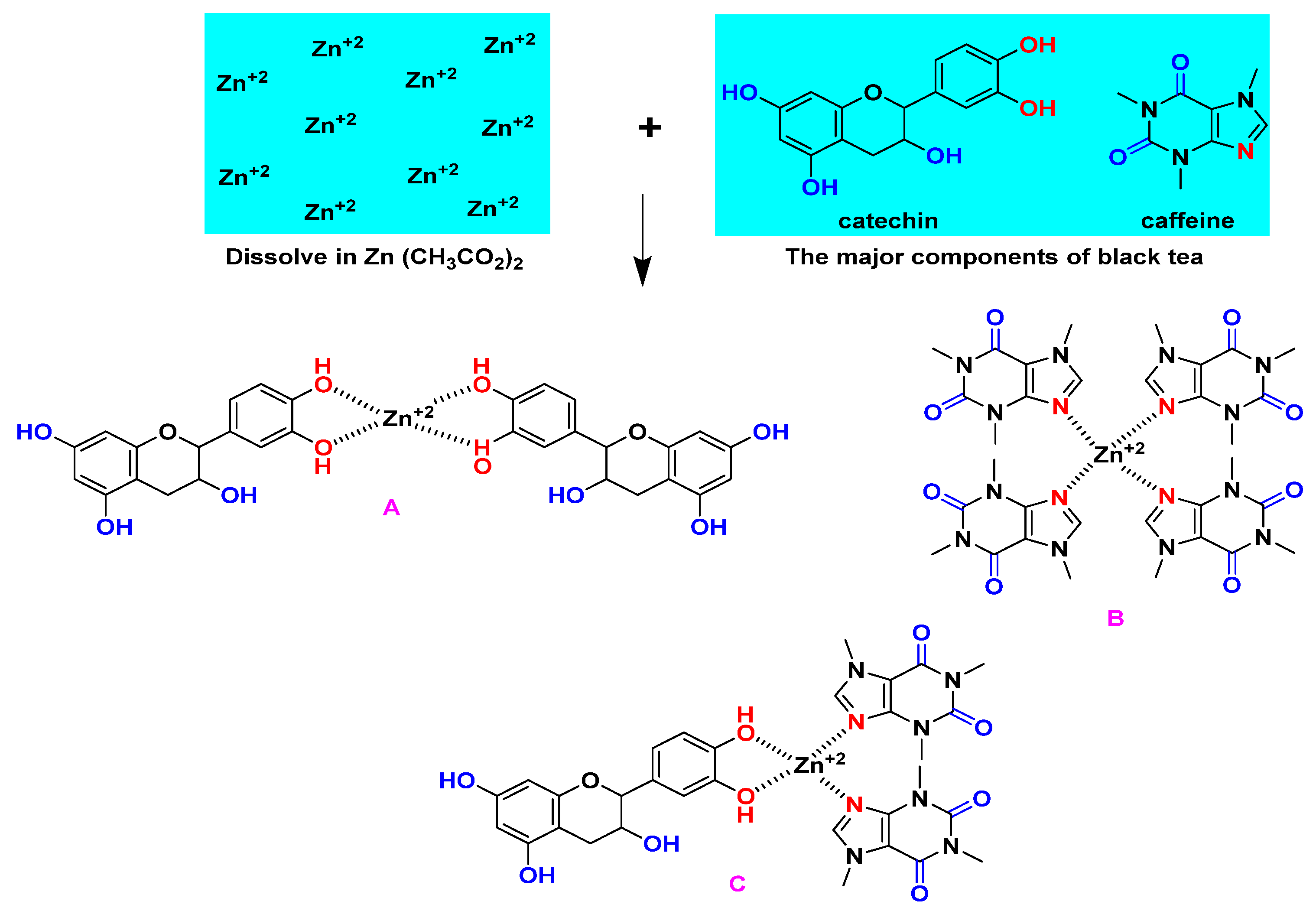
1. Introduction
2. Methodology
2.1. Materials and Sample Preparation
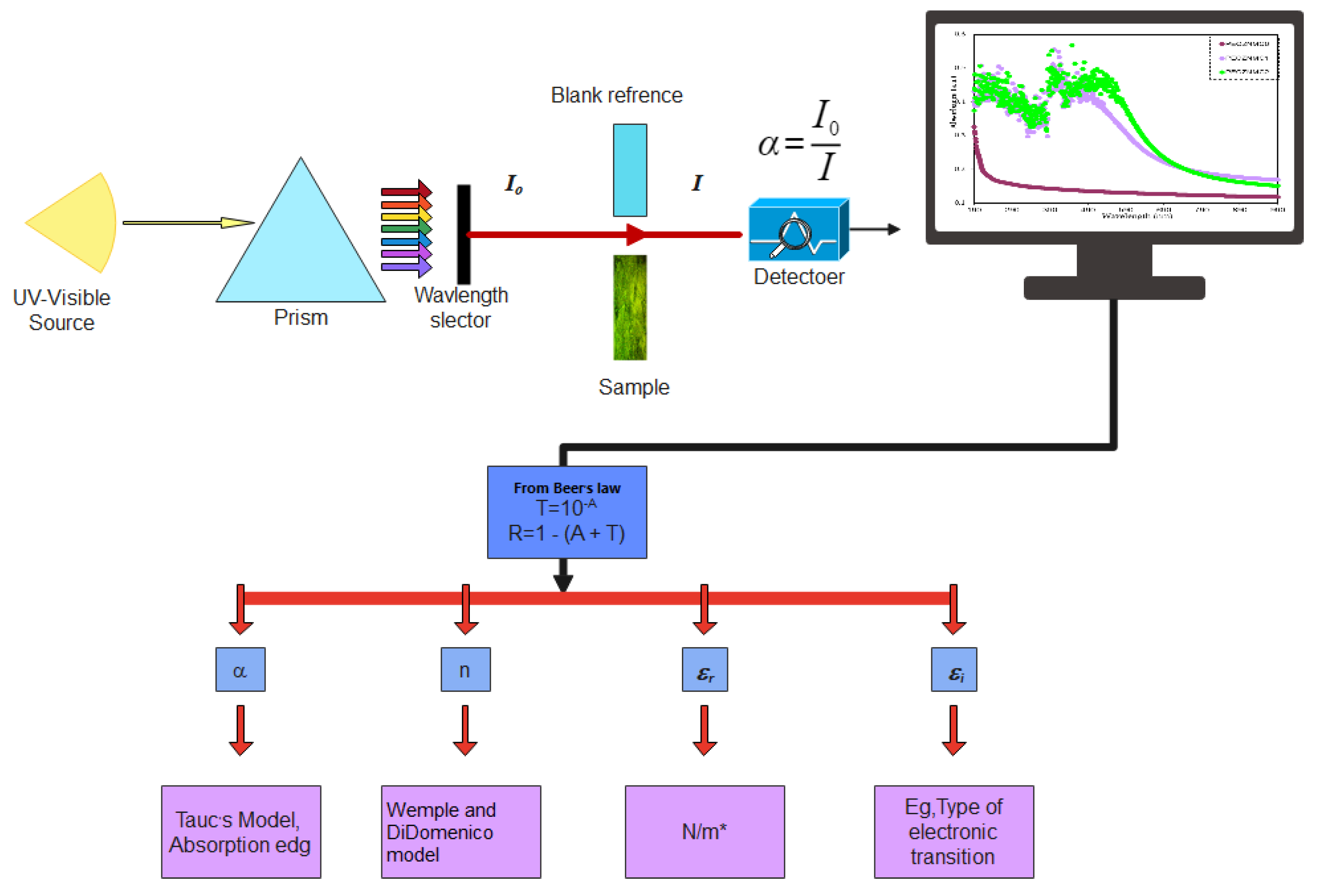
2.2. Spectroscopic Studies
3. Results and Discussion
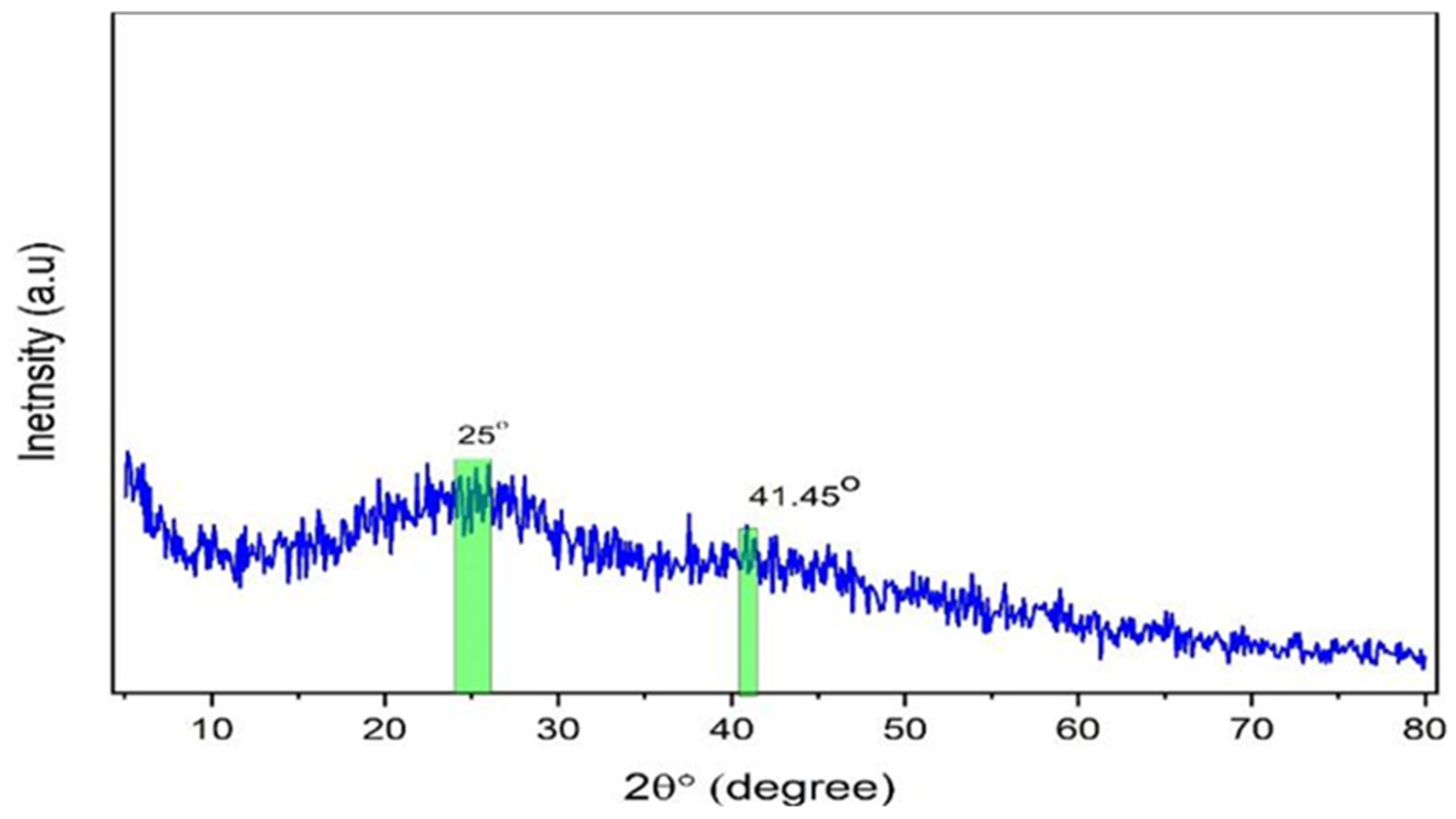
3.1. XRD Analysis
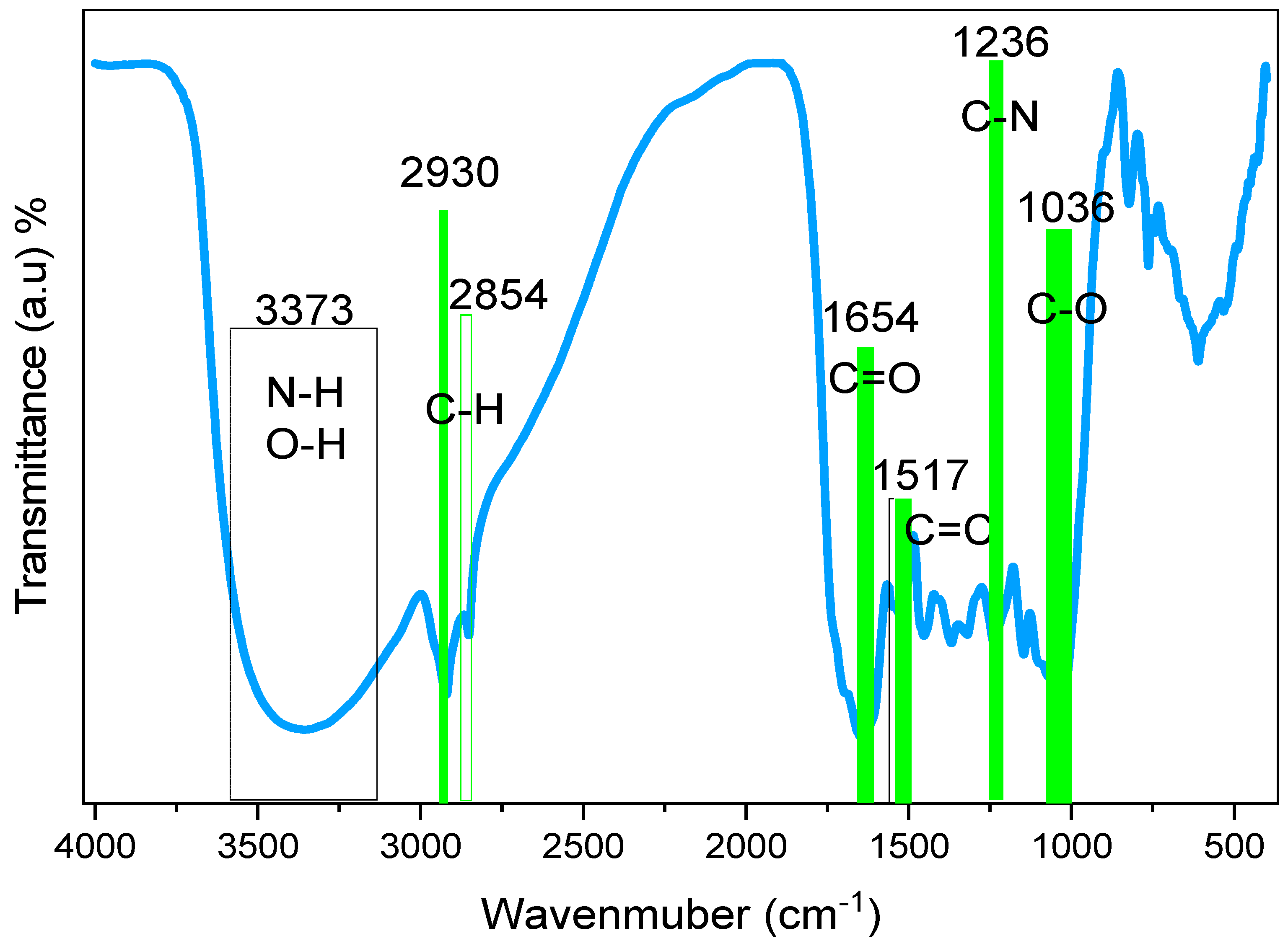
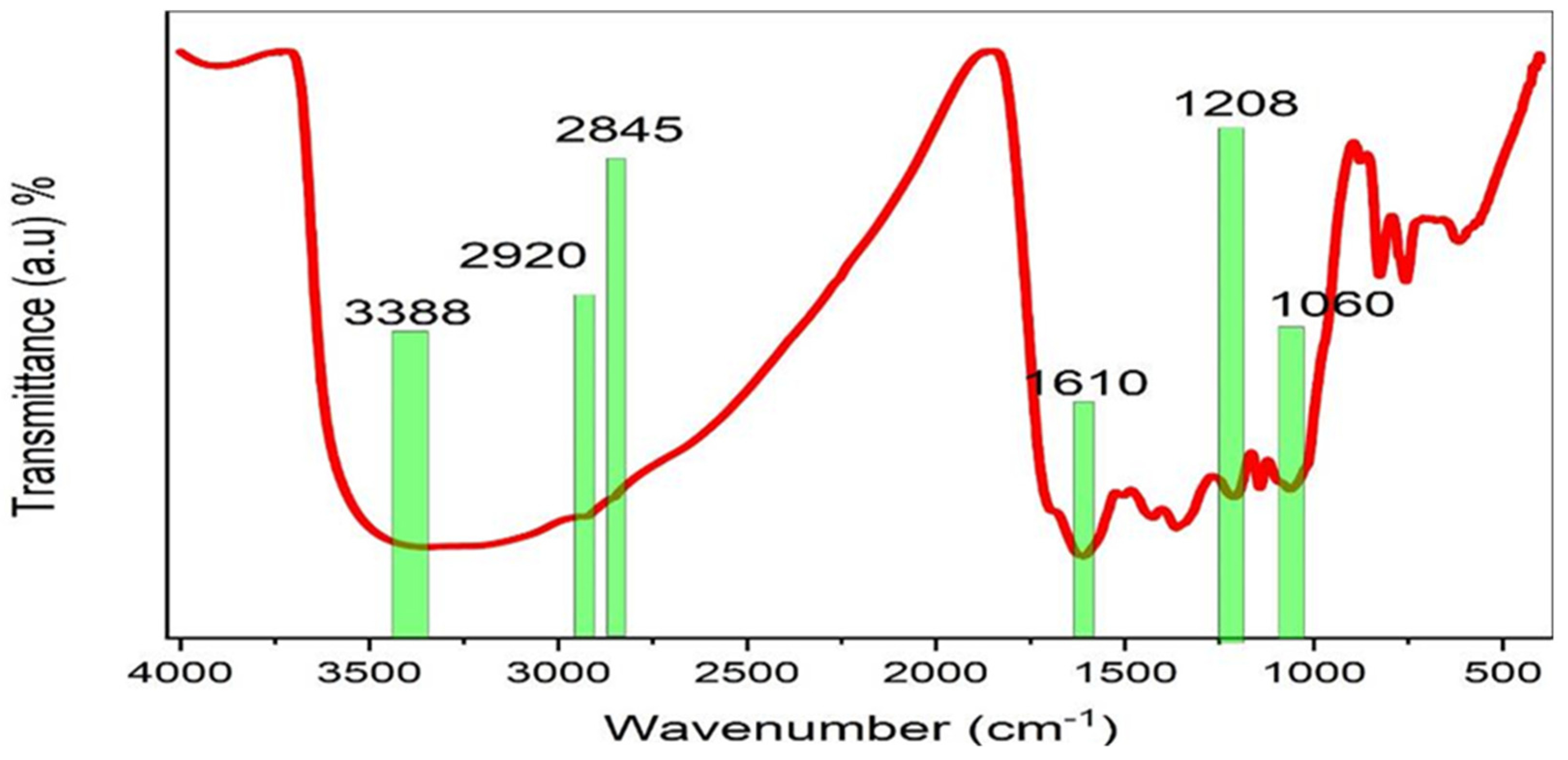
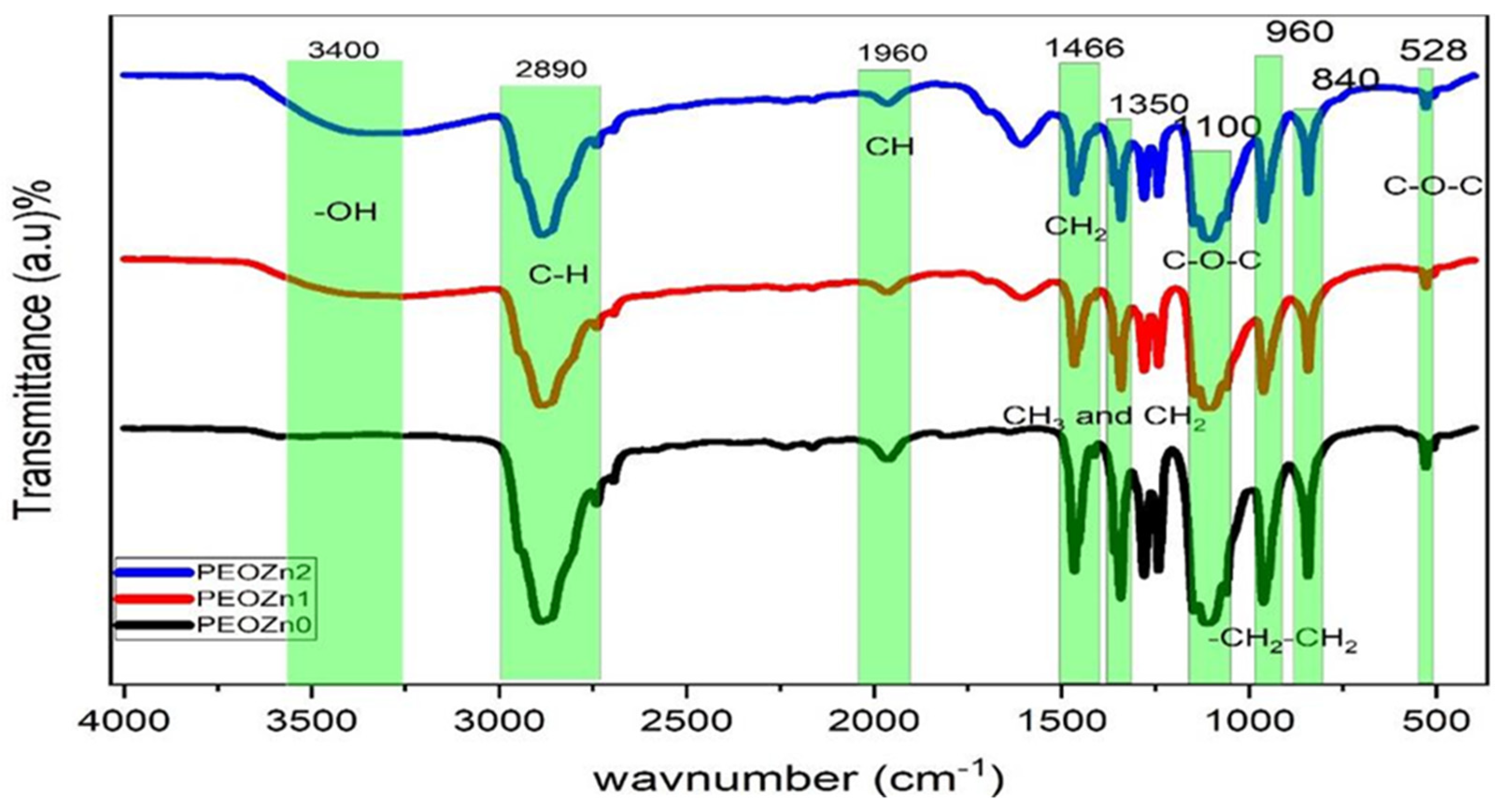
3.2. FTIR Analysis
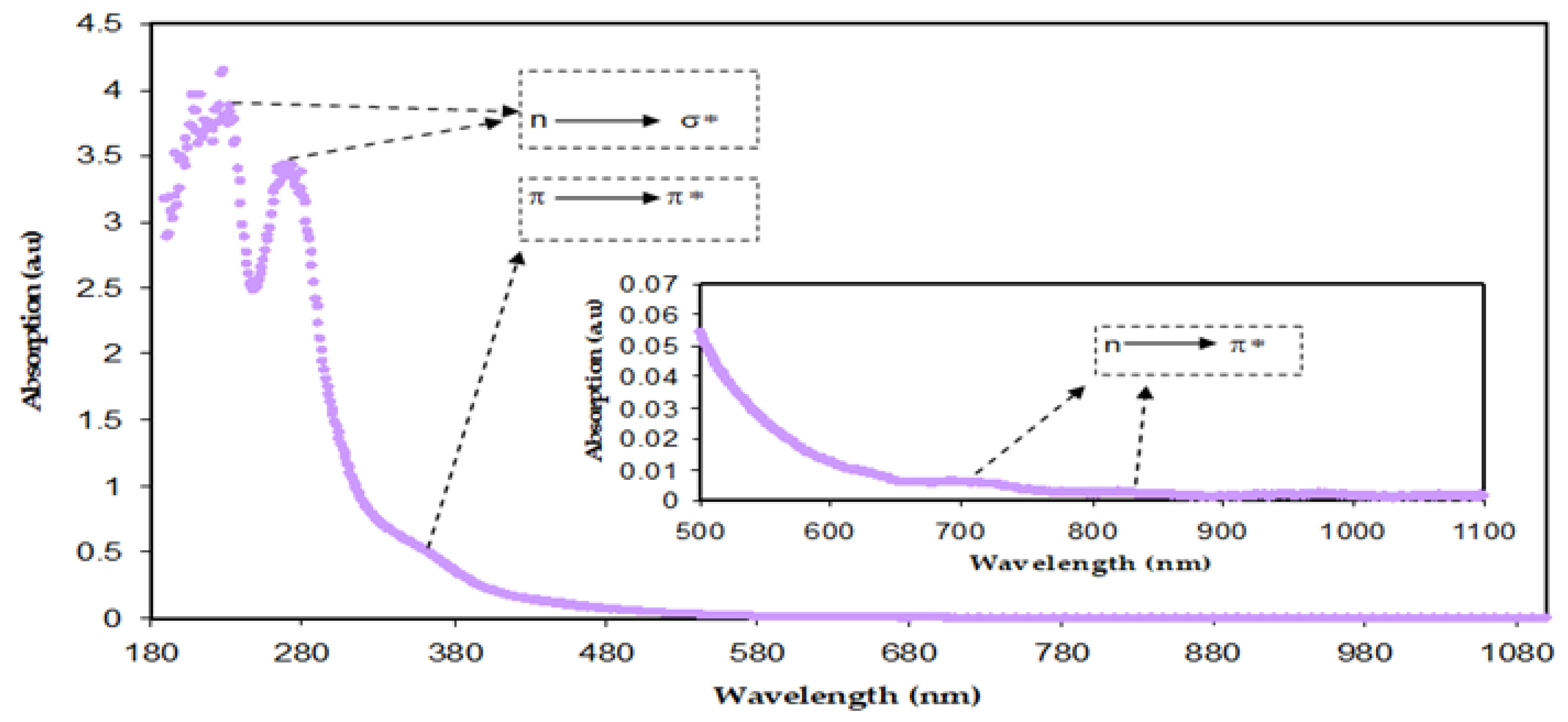
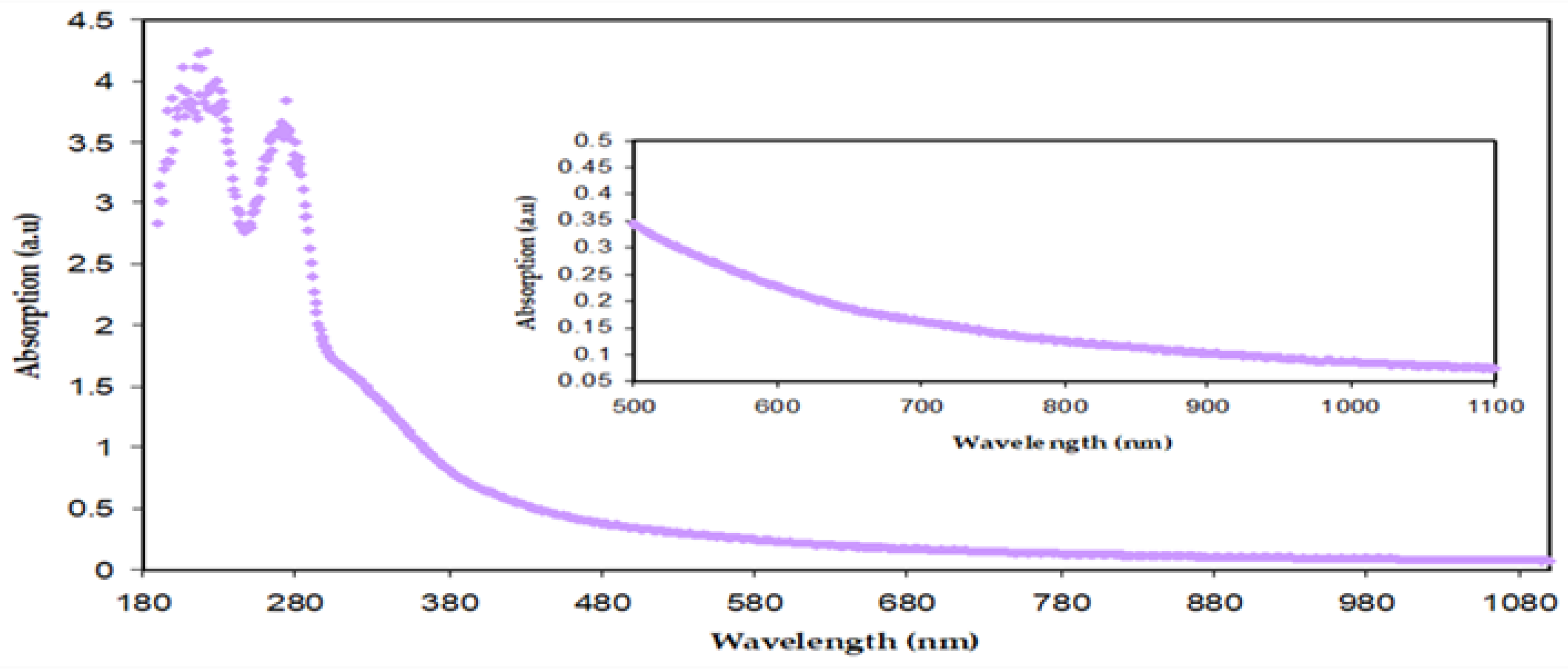
3.3. Absorption Study
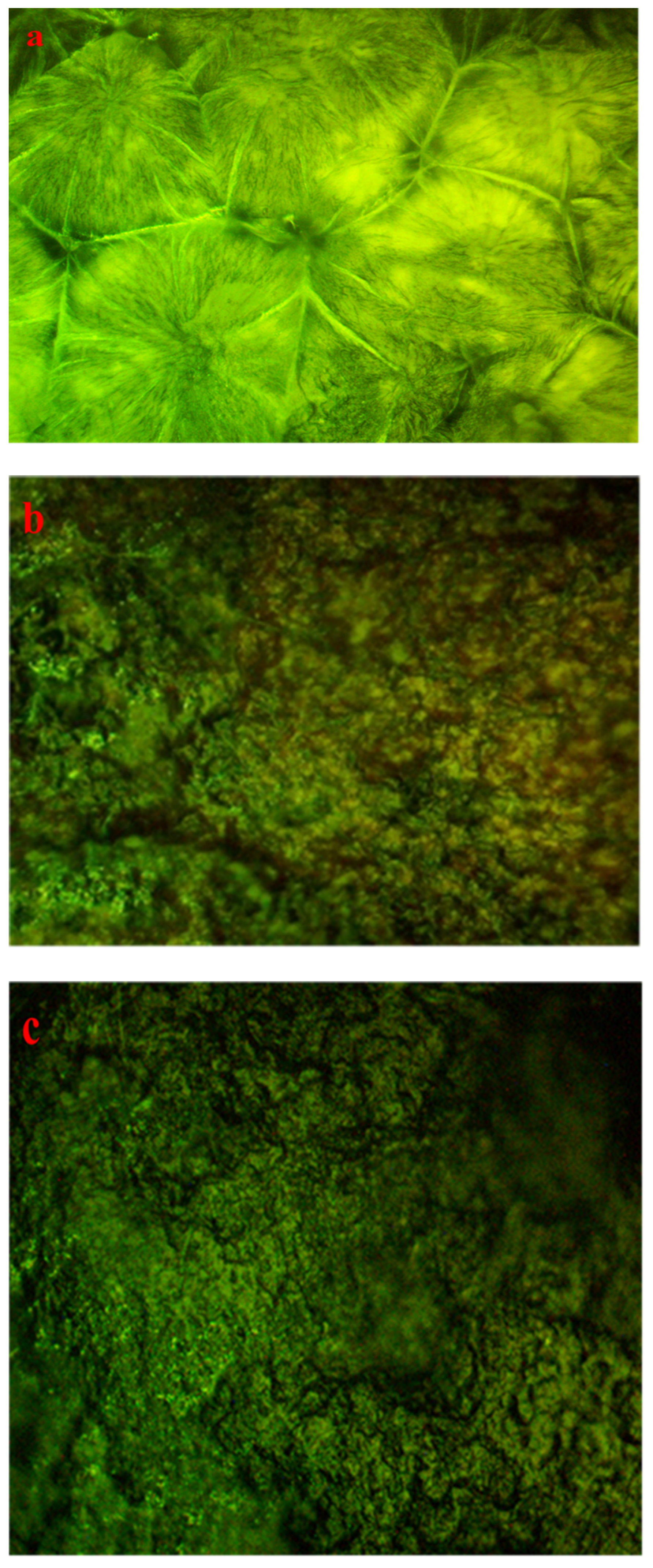
3.4. Morphological Study of PEO Composites
4. Absorption Coefficient Study
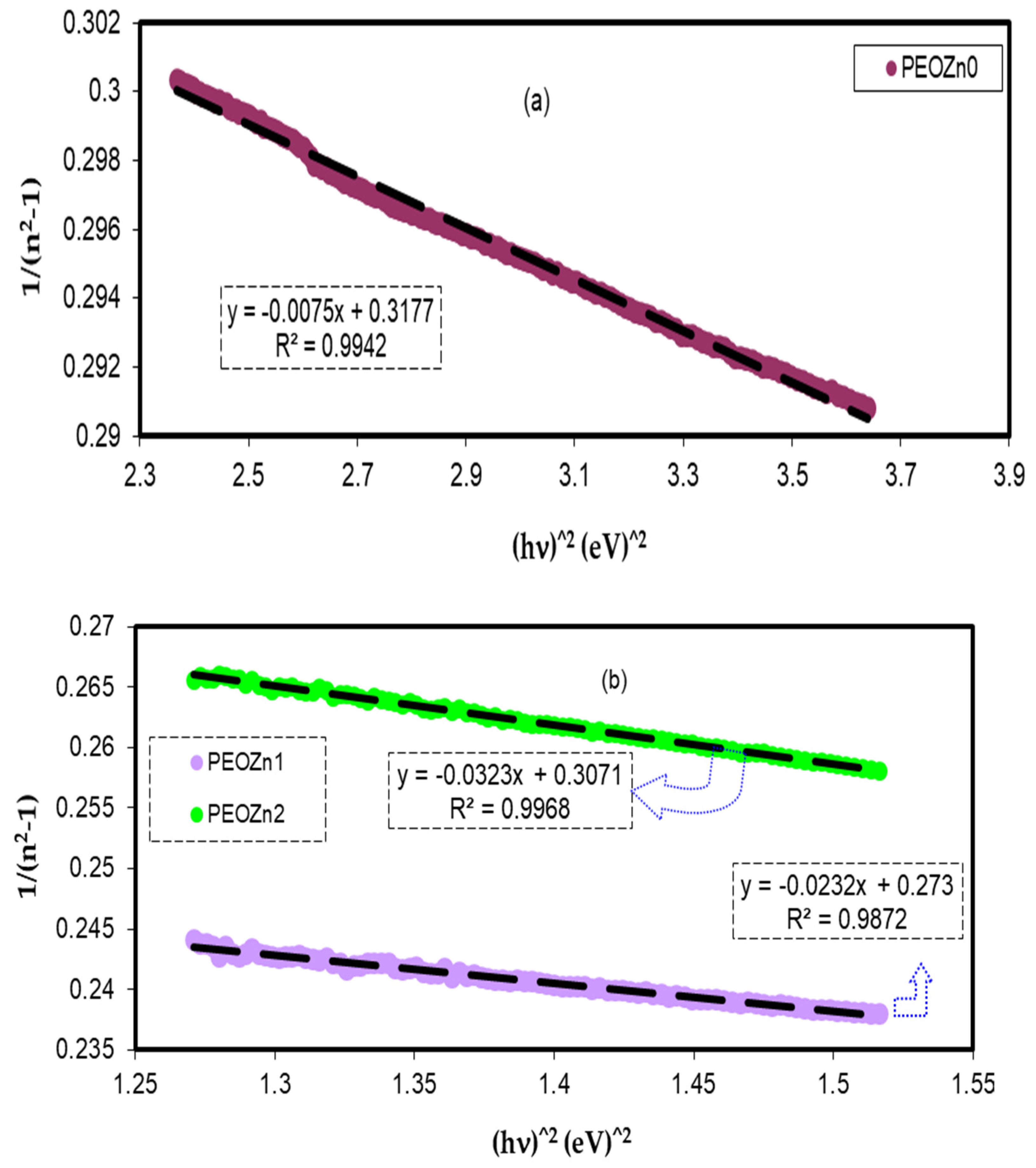
4.1. Refractive Index Study
4.2. Wemple and DiDomenico (W–D) Model
4.3. Optical Dielectric Constant Study
4.4. Tauc’s Approach for Band Gap Study
4.5. Dielectric Function Study
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Karman, S.B.; Diah, S.Z.M.; Gebeshuber, I.C. Raw Materials Synthesis from Heavy Metal Industry Effluents with Bioremediation and Phytomining: A Biomimetic Resource Management Approach. Adv. Mater. Sci. Eng. 2014, 2014, 185071. [Google Scholar] [CrossRef]
- Fu, F.; Wang, Q. Removal of heavy metal ions from wastewaters: A review. J. Environ. Manag. 2011, 92, 407–418. [Google Scholar] [CrossRef]
- Mohamed, E.L.A.; Hicham, E.L.H. Synthesis and Characterization of caffeine Complexes [M (caf) 4X2] M = Ni (II), Cu (II), Zn (II), Cd (II) X = SCN-, CN-; caf: Caffeine. Res. J. Chem. Sci. 2014, 4, 42–48. [Google Scholar]
- Khalid, S.; Shahid, M.; Niazi, N.K.; Murtaza, B.; Bibi, I.; Dumat, C. A comparison of technologies for remediation of heavy metal contaminated soils. J. Geochem. Explor. 2017, 182, 247–268. [Google Scholar] [CrossRef]
- Zhai, X.; Li, Z.; Huang, B.; Luo, N.; Huang, M.; Zhang, Q.; Zeng, G. Remediation of multiple heavy metal-contaminated soil through the combination of soil washing and in situ immobilization. Sci. Total Environ. 2018, 635, 92–99. [Google Scholar] [CrossRef] [PubMed]
- Drynan, J.W.; Clifford, M.N.; Obuchowicz, J.; Kuhnert, N. The chemistry of low molecular weight black tea polyphenols. Nat. Prod. Rep. 2010, 27, 417–462. [Google Scholar] [CrossRef]
- van der Hooft, J.J.; Akermi, M.; Unlu, F.Y.; Mihaleva, V.; Roldan, V.G.; Bino, R.J.; de Vos, R.C.H.; Vervoort, J. Structural annotation and elucidation of conjugated phenolic compounds in black, green, and white tea extracts. J. Agric. Food Chem. 2012, 60, 8841–8850. [Google Scholar] [CrossRef] [PubMed]
- Lee, L.S.; Kim, S.H.; Kim, Y.B.; Kim, Y.C. Quantitative analysis of major constituents in green tea with different plucking periods and their antioxidant activity. Molecules 2014, 19, 9173–9186. [Google Scholar] [CrossRef] [PubMed]
- Reto, M.; Figueira, M.E.; Filipe, H.M.; Almeida, C.M.M. Chemical composition of green tea (Camellia sinensis) infusions commercialized in Portugal. Plant Foods Hum. Nutr. 2007, 62, 139–144. [Google Scholar] [CrossRef]
- Aziz, S.B. Modifying Poly(Vinyl Alcohol) (PVA) from Insulator to Small-Bandgap Polymer: A Novel Approach for Organic Solar Cells and Optoelectronic Devices. J. Electron. Mater. 2016, 45, 736–745. [Google Scholar] [CrossRef]
- Aziz, S.B.; Abdullah, O.G.; Hussein, A.M.; Ahmed, H.M. From insulating PMMA polymer to conjugated double bond behavior: Green chemistry as a novel approach to fabricate small band gap polymers. Polymers 2017, 9, 626. [Google Scholar] [CrossRef]
- Kotrba, P.; Mackova, M.; Macek, T. Microbial Biosorption of Metals; no. January 1970; Springer: Dordrecht, The Netherlands, 2011. [Google Scholar]
- MBrza, A.; Aziz, S.B.; Anuar, H.; Al Hazza, M.H.F. From green remediation to polymer hybrid fabrication with improved optical band gaps. Int. J. Mol. Sci. 2019, 20, 3910. [Google Scholar] [CrossRef]
- Bauer, R.E.; Grimsdale, A.C.; Müllen, K. Optical Properties of Hybrid Organic-Inorganic Materials and their Applications. Anion. Sens. 2005, 15, 253–286. [Google Scholar]
- Huang, J.; Yin, Z.; Zheng, Q. Applications of ZnO in organic and hybrid solar cells. Energy Environ. Sci. 2011, 4, 3861–3877. [Google Scholar] [CrossRef]
- Aziz, S.B.; Abdulwahid, R.T.; Rsaul, H.A.; Ahmed, H.M. In situ synthesis of CuS nanoparticle with a distinguishable SPR peak in NIR region. J. Mater. Sci. Mater. Electron. 2016, 27, 4163–4171. [Google Scholar] [CrossRef]
- Saeed, C.O.; Qader, A.A.; Aziz, S.B. Low cost novel PEO based nano-composite for semiconductor and He–Ne lasers beam attenuation: Structural and optical properties. Opt. Mater. 2022, 129, 112502. [Google Scholar] [CrossRef]
- Yakuphanoglu, F.; Barim, G.; Erol, I. The effect of FeCl3 on the optical constants and optical band gap of MBZMA-co-MMA polymer thin films. Phys. B Condens. Matter. 2007, 391, 136–140. [Google Scholar] [CrossRef]
- Abdullah, O.G.; Aziz, S.B.; Omer, K.M.; Salih, Y.M. Reducing the optical band gap of polyvinyl alcohol (PVA) based nanocomposite. J. Mater. Sci. Mater. Electron. 2015, 26, 5303–5309. [Google Scholar] [CrossRef]
- Aziz, S.B.; Hussein, S.; Hussein, A.M.; Saeed, S.R. Optical Characteristics of Polystyrene Based Solid Polymer Composites: Effect of Metallic Copper Powder. Int. J. Met. 2013, 2013, 1–6. [Google Scholar] [CrossRef]
- Kumar, R.; Ali, S.A.; Mahur, A.K.; Virk, H.S.; Singh, F.; Khan, S.A.; Avasthi, D.; Prasad, R. Study of optical band gap and carbonaceous clusters in swift heavy ion irradiated polymers with UV-Vis spectroscopy. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. At. 2008, 266, 1788–1792. [Google Scholar] [CrossRef]
- Han, C.C.; Shi, W.; Jin, J. Morphology and Crystallization of Crystalline/Amorphous Polymer Blends. Encycl. Polym. Compos. 2013, 1–19. [Google Scholar] [CrossRef]
- Koduru, H.K.; Iliev, M.T.; Kondamareddy, K.K.; Karashanova, D.; Vlakhov, T.; Zhao, X.Z.; Scaramuzza, N. Investigations on Poly (ethylene oxide) (PEO)-Blend based solid polymer electrolytes for sodium ion batteries. In Journal of Physics: Conference Series; IOP Publishing: Velingrad, Bulgaria, 2016; Volume 764. [Google Scholar] [CrossRef]
- Cao, Y.C.; Xu, C.; Wu, X.; Wang, X.; Xing, L.; Scott, K. A poly (ethylene oxide)/graphene oxide electrolyte membrane for low temperature polymer fuel cells. J. Power Source 2011, 196, 8377–8382. [Google Scholar] [CrossRef]
- Aziz, S.B.; Abdullah, R.M. Crystalline and amorphous phase identification from the tanδ relaxation peaks and impedance plots in polymer blend electrolytes based on [CS:AgNt]x:PEO(x-1) (10 ≤ x ≤ 50). Electrochim. Acta 2018, 285, 30–46. [Google Scholar] [CrossRef]
- Huang, C.I.; Chen, J.R. Crystallization and chain conformation of semicrystalline and amorphous polymer blends studied by wide-angle and small-angle scattering. J. Polym. Sci. Part B Polym. Phys. 2001, 39, 2705–2715. [Google Scholar] [CrossRef]
- Yang, R.; Zhang, S.; Zhang, L.; Liu, W. Electrical properties of composite polymer electrolytes based on PEO-SN-LiCF3SO3. Int. J. Electrochem. Sci. 2013, 8, 10163–10169. [Google Scholar]
- Kim, M.; Lee, L.; Jung, Y.; Kim, S. Study on ion conductivity and crystallinity of composite polymer electrolytes based on poly(ethylene oxide)/poly(acrylonitrile) containing nano-sized Al2O3 Fillers. J. Nanosci. Nanotechnol. 2013, 13, 7865–7869. [Google Scholar] [CrossRef] [PubMed]
- Rajeh, A.; Morsi, M.A.; Elashmawi, I.S. Enhancement of spectroscopic, thermal, electrical and morphological properties of polyethylene oxide/carboxymethyl cellulose blends: Combined FT-IR/DFT. Vacuum 2019, 159, 430–440. [Google Scholar] [CrossRef]
- Abdelrazek, E.M.; Abdelghany, A.M.; Badr, S.I.; Morsi, M.A. Structural, optical, morphological and thermal properties of PEO/PVP blend containing different concentrations of biosynthesized Au nanoparticles. J. Mater. Res. Technol. 2018, 7, 419–431. [Google Scholar] [CrossRef]
- Wen, S.J.; Richardson, T.J.; Ghantous, D.I.; Striebel, K.A.; Ross, P.N.; Cairns, E.J. FTIR characterization of PEO + LiN(CF3SO2)2 electrolytes. J. Electroanal. Chem. 1996, 408, 113–118. [Google Scholar] [CrossRef]
- Bandara, T.M.W.J.; Karunathilaka, D.G.N.; Ratnasekera, J.L.; De Silva, L.A.; Herath, A.C.; Mellander, B.E. Electrical and complex dielectric behaviour of composite polymer electrolyte based on PEO, alumina and tetrapropylammonium iodide. Ionics 2017, 23, 1711–1719. [Google Scholar] [CrossRef]
- Zhou, H.; Fu, H.; Wu, X.; Wu, B.; Dai, C. Discrimination of tea varieties based on FTIR spectroscopy and an adaptive improved possibilistic c-means clustering. J. Food Process. Preserv. 2020, 44, e14795. [Google Scholar] [CrossRef]
- Verma, D.; Khan, F. Corrosion Inhibition of High Carbon Steel in Phosphoric Acid Solution by Extract of Black Tea. Adv. Res. 2015, 5, 1–9. [Google Scholar] [CrossRef]
- Loo, Y.Y.; Chieng, B.W.; Nishibuchi, M.; Radu, S. Synthesis of silver nanoparticles by using tea leaf extract from Camellia sinensis. Int. J. Nanomed. 2012, 7, 4263–4267. [Google Scholar] [CrossRef]
- Szymczycha-Madeja, A.; Welna, M.; Zyrnicki, W. Multi-element analysis, bioavailability and fractionation of herbal tea products. J. Braz. Chem. Soc. 2013, 24, 777–787. [Google Scholar] [CrossRef]
- Begum, N.A.; Mondal, S.; Basu, S.; Laskar, R.A.; Mandal, D. Biogenic synthesis of Au and Ag nanoparticles using aqueous solutions of Black Tea leaf extracts. Colloids Surf. B Biointerfaces 2009, 71, 113–118. [Google Scholar] [CrossRef] [PubMed]
- Tao, P.; Li, Y.; Rungta, A.; Viswanath, A.; Gao, J.; Benicewicz, B.C.; Siegela, R.W.; Schadler, L.S. TiO2 nanocomposites with high refractive index and transparency. J. Mater. Chem. 2011, 21, 18623–18629. [Google Scholar] [CrossRef]
- Chaudhuri, B.; Uddin, M.J.; Chaudhuri, B.; Pramanik, K.; Middya, T.R. Black tea leaf extract derived Ag nanoparticle-PVA composite film: Structural and dielectric properties. Mater. Sci. Eng. B Solid-State Mater. Adv. Technol. 2012, 177, 1741–1747. [Google Scholar] [CrossRef]
- Senthilkumar, S.R.; Sivakumar, T. Green tea (Camellia sinensis) mediated synthesis of zinc oxide (ZnO) nanoparticles and studies on their antimicrobial activities. Int. J. Pharm. Pharm. Sci. 2014, 6, 461–465. [Google Scholar]
- Hao, R.; Li, D.; Zhang, J.; Jiao, T. Green synthesis of iron nanoparticles using green tea and its removal of hexavalent chromium. Nanomaterials 2021, 11, 650. [Google Scholar] [CrossRef]
- Dubey, S.P.; Sillanpaa, M.; Varma, R.S. Reduction of hexavalent chromium using Sorbaria sorbifolia aqueous leaf extract. Appl. Sci. 2017, 7, 715. [Google Scholar] [CrossRef]
- Ó’Coinceanainn, M.; Astill, C.; Schumm, S. Potentiometric, FTIR and NMR studies of the complexation of metals with theaflavin. Dalt. Trans. 2003, 5, 801–807. [Google Scholar] [CrossRef]
- Huang, L.; Weng, X.; Chen, Z.; Megharaj, M.; Naidu, R. Synthesis of iron-based nanoparticles using oolong tea extract for the degradation of malachite green. Spectrochim. Acta-Part A Mol. Biomol. Spectrosc. 2014, 117, 801–804. [Google Scholar] [CrossRef]
- Li, X.; Zhang, Y.; He, Y. Rapid detection of talcum powder in tea using FT-IR spectroscopy coupled with chemometrics. Sci. Rep. 2016, 6, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Ucun, F.; Sa, A.; Güçlü, V. Molecular structures and vibrational frequencies of xanthine and its methyl derivatives (caffeine and theobromine) by ab initio Hartree-Fock and density functional theory calculations. Spectrochim. Acta-Part A Mol. Biomol. Spectrosc. 2007, 67, 342–349. [Google Scholar] [CrossRef]
- BGoodman, A.; Severino, J.F.; Pirker, K.F. Reactions of green and black teas with Cu(ii). Food Funct. 2012, 3, 399–409. [Google Scholar] [CrossRef] [PubMed]
- Zielinski, A.A.F.; Haminiuk, C.W.I.; Alberti, A.; Nogueira, A.; Demiate, I.M.; Granato, D. A comparative study of the phenolic compounds and the in vitro antioxidant activity of different Brazilian teas using multivariate statistical techniques. Food Res. Int. 2014, 60, 246–254. [Google Scholar] [CrossRef]
- Kolayli, S.; Ocak, M.; Küçük, M.; Abbaso, R. Does caffeine bind to metal ions? Food Chem. 2004, 84, 383–388. [Google Scholar] [CrossRef]
- Brza, M.A.; Aziz, S.B.; Anuar, H.; Ali, F.; Dannoun, E.M.; Saeed, S.R.; Mohammed, S.J.; Abdulwahid, R.T. Green coordination chemistry as a novel approach to fabricate polymer:Cd(II)-complex composites: Structural and optical properties. Opt. Mater. 2021, 116, 111062. [Google Scholar] [CrossRef]
- Brza, M.A.; Aziz, S.B.; Anuar, H.; Ali, F.; Dannoun, E.; Mohammed, S.J.; Abdulwahid, R.T.; Al, S. Tea from the drinking to the synthesis of metal complexes and fabrication of PVA based polymer composites with controlled optical band gap. Sci. Rep. 2020, 10, 1–17. [Google Scholar] [CrossRef]
- Aziz, S.B.; Nofal, M.M.; Brza, M.A.; Sadiq, N.M.; Dannoun, E.M.A.; Ahmed, K.K.; Al-Saeedi, S.I.; Hussen, S.A.; Hussein, A.M. Innovative Green Chemistry Approach to Synthesis of Sn2+-Metal Complex and Design of Polymer Composites with Small Optical Band Gaps. Molecules 2022, 27, 1965. [Google Scholar] [CrossRef] [PubMed]
- Nofal, M.M.; Aziz, S.B.; Hadi, J.M.; Karim, W.O.; Dannoun, E.M.; Hussein, A.M.; Hussen, S.A. Polymer composites with 0.98 transparencies and small optical energy band gap using a promising green methodology: Structural and optical properties. Polymers 2021, 13, 1648. [Google Scholar] [CrossRef] [PubMed]
- Aziz, S.B.; Nofal, M.M.; Ghareeb, H.O.; Dannoun, E.M.A.; Hussen, S.A.; Hadi, J.M.; Ahmed, K.K.; Hussein, A.M. Characteristics of poly(Vinyl alcohol) (PVA) based composites integrated with green synthesized Al3+-metal complex: Structural, optical, and localized density of state analysis. Polymers 2021, 13, 1316. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Peng, H.; Wang, W.; Liu, T. Crystallization behavior of poly(ε-caprolactone)/layered double hydroxide nanocomposites. J. Appl. Polym. Sci. 2010, 116, 2658–2667. [Google Scholar] [CrossRef]
- Ramesh, S.; Yuen, T.F.; Shen, C.J. Conductivity and FTIR studies on PEO-LiX [X: CF3SO3-, SO42-] polymer electrolytes. Spectrochim. Acta-Part A Mol. Biomol. Spectrosc. 2008, 69, 670–675. [Google Scholar] [CrossRef]
- Chu, P.P.; Reddy, M.J.; Tsai, J. Structural and transport characteristics of polyethylene oxide/phenolic resin blend solid polymer electrolytes. J. Polym. Sci. Part B Polym. Phys. 2004, 42, 3866–3875. [Google Scholar] [CrossRef]
- Patil, S.U.; Yawale, S.S.; Yawale, S.P. Conductivity study of PEO-LiClO4 polymer electrolyte doped with ZnO nanocomposite ceramic filler. Bull. Mater. Sci. 2014, 37, 1403–1409. [Google Scholar] [CrossRef]
- Senak, L.; Davies, M.A.; Mendelsohn, R. A quantitative IR study of hydrocarbon chain conformation in alkanes and phospholipids: CH2 wagging modes in disordered bilayer and HII phases. J. Phys. Chem. 1991, 95, 2565–2571. [Google Scholar] [CrossRef]
- Rao, B.N.N.; Suvarna, R.P. A study on optical properties of poly (ethylene oxide) based polymer electrolyte with different alkali metal iodides. In AIP Conference Proceedings; AIP Publishing: Nandyal, India, 2016; Volume 1728. [Google Scholar] [CrossRef]
- Sim, L.H.; Gan, S.N.; Chan, C.H.; Yahya, R. ATR-FTIR studies on ion interaction of lithium perchlorate in polyacrylate/poly(ethylene oxide) blends. Spectrochim. Acta-Part A Mol. Biomol. Spectrosc. 2010, 76, 287–292. [Google Scholar] [CrossRef] [PubMed]
- Soman, V.V.; Kelkar, D.S. FTIR studies of doped PMMA-PVC blend system. Macromol. Symp. 2009, 277, 152–161. [Google Scholar] [CrossRef]
- APalacios-Morillo, A.; Alcázar, Á.; De Pablos, F.; Jurado, J.M. Differentiation of tea varieties using UV-Vis spectra and pattern recognition techniques. Spectrochim. Acta-Part A Mol. Biomol. Spectrosc. 2013, 103, 79–83. [Google Scholar] [CrossRef]
- Srivastavaab, A.; Singha, V.; Aggarwat, P.; Schneeweiss, F.; Scherer, U.W.; Friedrichc, W. Optical studies of insulating polymers for radiation dose monitoring. Indian J. Pure Appl. Phys. 2010, 48, 782–786. [Google Scholar]
- Marzuki, A.; Suryanti, V.; Virgynia, A. Spectroscopic Study of Green Tea (Camellia sinensis) Leaves Extraction. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Surakarta, Indonesia, 2017; Volume 193. [Google Scholar] [CrossRef]
- Kumar, K.R.P.; Murali, M.G.; Udayakumar, D. Synthesis and study of optical properties of linear and hyperbranched conjugated polymers containing thiophene and triphenylamine units. Des. Monomers Polym. 2014, 17, 7–18. [Google Scholar] [CrossRef]
- Koyuncu, F.B.; Sefer, E.; Koyuncu, S.; Ozdemir, E. A new low band gap electrochromic polymer containing 2,5-bis-dithienyl-1H-pyrrole and 2,1,3-benzoselenadiazole moiety with high contrast ratio. Polymer 2011, 52, 5772–5779. [Google Scholar] [CrossRef]
- López-Gutiérrez, N.; Romero-González, R.; Plaza-Bolaños, P.; Vidal, J.L.M.; Frenich, A.G. Identification and quantification of phytochemicals in nutraceutical products from green tea by UHPLC-Orbitrap-MS. Food Chem. 2015, 173, 607–618. [Google Scholar] [CrossRef]
- Pasrija, D.; Anandharamakrishnan, C. Techniques for Extraction of Green Tea Polyphenols: A Review. Food Bioprocess Technol. 2015, 8, 935–950. [Google Scholar] [CrossRef]
- Wang, X.; Huang, J.; Fan, W.; Lu, H. Identification of green tea varieties and fast quantification of total polyphenols by near-infrared spectroscopy and ultraviolet-visible spectroscopy with chemometric algorithms. Anal. Methods 2015, 7, 787–792. [Google Scholar] [CrossRef]
- Hoag, G.E.; Collins, J.B.; Holcomb, J.L.; Hoag, J.R.; Nadagouda, M.N.; Varma, R.S. Degradation of bromothymol blue by ‘greener’ nano-scale zero-valent iron synthesized using tea polyphenols. J. Mater. Chem. 2009, 19, 8671–8677. [Google Scholar] [CrossRef]
- Aziz, S.B.; Marif, R.B.; Brza, M.A.; Hassan, A.N.; Ahmad, H.A.; Faidhalla, Y.A.; Kadir, M.F.Z. Structural, thermal, morphological and optical properties of PEO filled with biosynthesized Ag nanoparticles: New insights to band gap study. Results Phys. 2019, 13, 102220. [Google Scholar] [CrossRef]
- Aziz, S.B.; Faraj, M.G.; Abdullah, O.G. Impedance Spectroscopy as a Novel Approach to Probe the Phase Transition and Microstructures Existing in CS:PEO Based Blend Electrolytes. Sci. Rep. 2018, 8, 1–14. [Google Scholar] [CrossRef]
- Saeed, K.; Ishaq, M.; Ilyas, M. Preparation, morphology, and thermomechanical properties of coal ash/polyethylene oxide composites. Turk. J. Chem. 2011, 35, 237–243. [Google Scholar] [CrossRef]
- Abdullah, M.; Lenggoro, W.; Okuyama, K. Polymer Electrolyte Nanocomposites; American Scientific Publishers: Stevenson Ranch, CA, USA, 2004; Volume 8. [Google Scholar]
- Surov, O.V.; Voronova, M.I.; Afineevskii, A.V.; Zakharov, A.G. Polyethylene oxide films reinforced by cellulose nanocrystals: Microstructure-properties relationship. Carbohydr. Polym. 2018, 181, 489–498. [Google Scholar] [CrossRef]
- Aziz, S.B.; Hassan, A.Q.; Mohammed, S.J.; Karim, W.O.; FZKadir, M.; ATajuddin, H.; NMY Chan, N. Structural and optical characteristics of pva:C-dot composites: Tuning the absorption of ultra violet (uv) region. Nanomaterials 2019, 9, 216. [Google Scholar] [CrossRef] [PubMed]
- Yin, X.; Liu, J.; Jäkle, F. Electron-Deficient Conjugated Materials via p–π* Conjugation with Boron: Extending Monomers to Oligomers, Macrocycles, and Polymers. Chem.-A Eur. J. 2021, 27, 2973–2986. [Google Scholar] [CrossRef]
- Hasegawa, T.; Ashizawa, M.; Hiyoshi, J.; Kawauchi, S.; Mei, J.; Bao, Z.; Matsumoto, H. An ultra-narrow bandgap derived from thienoisoindigo polymers: Structural influence on reducing the bandgap and self-organization. Polym. Chem. 2016, 7, 1181–1190. [Google Scholar] [CrossRef]
- Hareesh, K.; Sanjeev, G.; Pandey, A.K.; Rao, V. Characterization of UV-irradiated Lexan polycarbonate films. Iran. Polym. J. 2013, 22, 341–349. [Google Scholar] [CrossRef]
- Singh, V.; Mohan, S.; Singh, G.; Pandey, P.C.; Prakash, R. Synthesis and characterization of polyaniline-carboxylated PVC composites: Application in development of ammonia sensor. Sens. Actuators B Chem. 2008, 132, 99–106. [Google Scholar] [CrossRef]
- Elimat, Z.M.; Zihlif, A.M.; Avella, M. Thermal and optical properties of poly(methyl methacrylate)/calcium carbonate nanocomposite. J. Exp. Nanosci. 2008, 3, 259–269. [Google Scholar] [CrossRef]
- Aziz, S.B.; Ahmed, H.M.; Hussein, A.M.; Fathulla, A.B.; Wsw, R.M.; Hussein, R.T. Tuning the absorption of ultraviolet spectra and optical parameters of aluminum doped PVA based solid polymer composites. J. Mater. Sci. Mater. Electron. 2015, 26, 8022–8028. [Google Scholar] [CrossRef]
- Abdullah, R.M.; Aziz, S.B.; Mamand, S.M.; Hassan, A.Q.; Hussein, S.A.; Kadir, M.F.Z. Reducing the crystallite size of spherulites in PEO-based polymer nanocomposites mediated by carbon nanodots and Ag nanoparticles. Nanomaterials 2019, 9, 874. [Google Scholar] [CrossRef] [PubMed]
- Aziz, S.B. Morphological and optical characteristics of chitosan(1−x):Cuox (4 ≤ x ≤ 12) based polymer nano-composites: Optical dielectric loss as an alternative method for tauc’s model. Nanomaterials 2017, 7, 444. [Google Scholar] [CrossRef]
- Tippins, H.H. Charge-transfer spectra of transition-metal ions in corundum. Phys. Rev. B 1970, 1, 126–135. [Google Scholar] [CrossRef]
- Sharma, H.; Sharma, S.N.; Singh, G.; Shivaprasad, S.M. Studies of optical and structural properties of CdSe/polymer nanocomposites: Evidence of charge transfer and photostability. Colloid Polym. Sci. 2007, 285, 1213–1227. [Google Scholar] [CrossRef]
- Yakuphanoglu, F.; Sekerci, M.; Balaban, A. The effect of film thickness on the optical absorption edge and optical constants of the Cr(III) organic thin films. Opt. Mater. 2005, 27, 1369–1372. [Google Scholar] [CrossRef]
- Aziz, S.B.; Rasheed, M.A.; Ahmed, H.M. Synthesis of polymer nanocomposites based on [methyl cellulose] (1−x):(CuS)x (0.02 M ≤ x ≤ 0.08 M) with desired optical band gaps. Polymers 2017, 9, 194. [Google Scholar] [CrossRef] [PubMed]
- Yetisen, A.K.; Montelongo, Y.; Butt, H. Rewritable three-dimensional holographic data storage via optical forces. Appl. Phys. Lett. 2016, 109, 6–11. [Google Scholar] [CrossRef]
- Muhammad, F.F.; Sulaiman, K. Photovoltaic performance of organic solar cells based on DH6T/PCBM thin film active layers. Thin Solid Film. 2011, 519, 5230–5233. [Google Scholar] [CrossRef]
- Taha, T.A. Optical properties of PVC/Al2O3 nanocomposite films. Polym. Bull. 2019, 76, 903–918. [Google Scholar] [CrossRef]
- Wemple, S.H.; DiDomenico, M. Behavior of the electronic dielectric constant in covalent and ionic materials. Phys. Rev. B 1971, 3, 1338–1351. [Google Scholar] [CrossRef]
- Ammar, A.H. Studies on some structural and optical properties of Zn x Cd 1-x Te thin films. Appl. Surf. Sci. 2002, 201, 9–19. [Google Scholar] [CrossRef]
- Benchaabane, A.; Ben Hamed, Z.; Kouki, F.; Abderrahmane Sanhoury, M.; Zellama, K.; Zeinert, A.; Bouchriha, H. Performances of effective medium model in interpreting optical properties of polyvinylcarbazole:ZnSe nanocomposites. J. Appl. Phys. 2014, 115, 134313. [Google Scholar] [CrossRef]
- Aziz, S.B.; Brza, M.A.; Nofal, M.M.; Abdulwahid, R.T.; Hussen, S.A.; Hussein, A.M.; Karim, W.O. A comprehensive review on optical properties of polymer electrolytes and composites. Materials 2020, 13, 3675. [Google Scholar] [CrossRef]
- Ebnalwaled, A.A.; Thabet, A. Controlling the optical constants of PVC nanocomposite films for optoelectronic applications. Synth. Met. 2016, 220, 374–383. [Google Scholar] [CrossRef]
- Ali, F.M.; Kershi, R.M.; Sayed, M.A.; AbouDeif, Y.M. Evaluation of structural and optical properties of Ce3+ ions doped (PVA/PVP) composite films for new organic semiconductors. Phys. B Condens. Matter. 2018, 538, 160–166. [Google Scholar] [CrossRef]
- Spitzer, W.G.; Fan, H.Y. Determination of optical constants and carrier effective mass of semiconductors. Phys. Rev. 1957, 106, 882–890. [Google Scholar] [CrossRef]
- Kiselev, A.I.; Akashev, L.A.; Kononenko, V.I. Effective electron mass in melts of aluminum, cerium, and Al-3 at.% Ce binary system. Tech. Phys. 2004, 49, 302–305. [Google Scholar] [CrossRef]
- Alsaad, A.M.; Al-Bataineh, Q.M.; Ahmad, A.A.; Albataineh, Z.; Telfah, A. Optical band gap and refractive index dispersion parameters of boron-doped ZnO thin films: A novel derived mathematical model from the experimental transmission spectra. Optik 2020, 211, 164641. [Google Scholar] [CrossRef]
- Benchaabane, A.; Hajlaoui, M.E.; Hnainia, N.; Al-Tabbakh, A.; Zeinert, A.; Bouchriha, H. Optical properties enhancement of hybrid nanocomposites thin films based on P3HT matrix and ZnO@SiO2 core-shell nanoparticles. Opt. Mater. 2020, 102, 109829. [Google Scholar] [CrossRef]
- Yakuphanoglu, F.; Sekerci, M.; Ozturk, O.F. The determination of the optical constants of cu(ii) compound having 1-chloro-2,3-o-cyclohexylidinepropane thin film. Opt. Commun. 2004, 239, 275–280. [Google Scholar] [CrossRef]
- Ahmed, N.M.; Sauli, Z.; Hashim, U.; Al-Douri, Y. Investigation of the absorption coefficient, refractive index, energy band gap, and film thickness for Al0.11Ga0.89N by optical transmission method. Int. J. Nanoelectron. Mater. 2009, 2, 189–195. [Google Scholar]
- Vergara, M.E.S.; Rebollo, A.O.; Alvarez, J.R.; Rivera, M. Molecular materials derived from MPc (M = Fe, Pb, Co) and 1,8-dihydroxiantraquinone thin films: Formation, electrical and optical properties. J. Phys. Chem. Solids 2008, 69, 1–7. [Google Scholar] [CrossRef]
- El-Nahass, M.M.; Farid, A.M.; Atta, A.A. Structural and optical properties of Tris(8-hydroxyquinoline) aluminum (III) (Alq3) thermal evaporated thin films. J. Alloys Compd. 2010, 507, 112–119. [Google Scholar] [CrossRef]
- Edukondalu, A.; Rahman, S.; Ahmmad, S.K.; Gupta, A.; Kumar, K.S. Optical properties of amorphous Li2O–WO3–B2O3 thin films deposited by electron beam evaporation. J. Taibah Univ. Sci. 2016, 10, 363–368. [Google Scholar] [CrossRef]
- Rodríguez, A.; Vergara, M.E.S.; Montalvo, V.G.; Ortiz, A.; Alvarez, J.R. Thin films of molecular materials synthesized from C32H20N10M (M = Co, Pb, Fe): Film formation, electrical and optical properties. Appl. Surf. Sci. 2010, 256, 3374–3379. [Google Scholar] [CrossRef]
- Rozra, J.; Saini, I.; Sharma, A.; Chandak, N.; Aggarwal, S.; Dhiman, R.; Sharma, P.K. Cu nanoparticles induced structural, optical and electrical modification in PVA. Mater. Chem. Phys. 2012, 134, 1121–1126. [Google Scholar] [CrossRef]
- Abdelaziz, M. Cerium (III) doping effects on optical and thermal properties of PVA films. Phys. B Condens. Matter. 2011, 406, 1300–1307. [Google Scholar] [CrossRef]
- SAziz, B.; Mamand, S.M.; Saed, S.R.; Abdullah, R.M.; Hussein, S.A. New Method for the Development of Plasmonic Metal-Semiconductor Interface Layer: Polymer Composites with Reduced Energy Band Gap. J. Nanomater. 2017, 2017, 8140693. [Google Scholar] [CrossRef]
- Ezat, G.S.; Hussen, S.A.; Aziz, S.B. Structure and optical properties of nanocomposites based on polystyrene (PS) and calcium titanate (CaTiO3) perovskite nanoparticles. Optik 2021, 241, 166963. [Google Scholar] [CrossRef]
- Ahmed, K.K.; Hussen, S.A.; Aziz, S.B. Transferring the wide band gap chitosan: POZ-based polymer blends to small optical energy band gap polymer composites through the inclusion of green synthesized Zn2+-PPL metal complex. Arab. J. Chem. 2022, 15, 103913. [Google Scholar] [CrossRef]
- Aziz, S.B.; Dannoun, E.M.A.; Tahir, D.A.; Hussen, S.A.; Abdulwahid, R.T.; Nofal, M.M.M.; Abdullah, R.M.; Hussein, A.; Brevik, I. Synthesis of pva/ceo2 based nanocomposites with tuned refractive index and reduced absorption edge: Structural and optical studies. Materials 2021, 14, 1570. [Google Scholar] [CrossRef]
- Hussein, A.M.; Dannoun, E.M.A.; Aziz, S.B.; Brza, M.A.; Abdulwahid, R.T.; Hussen, S.A.; Rostam, S.; Mustafa, D.M.T.; Muhammad, D.S. Steps toward the band gap identification in polystyrene based solid polymer nanocomposites integrated with tin titanate nanoparticles. Polymers 2020, 12, 2320. [Google Scholar] [CrossRef]
- Aziz, S.B.; Nofal, M.M.; Brza, M.A.; Hussein, S.A.; Mahmoud, K.H.; El-Bahy, Z.M.; Dannoun, E.M.A.; Kareem, W.O.; Hussein, A.M. Characteristics of peo incorporated with CaTiO3 nanoparticles: Structural and optical properties. Polymers 2021, 13, 3484. [Google Scholar] [CrossRef] [PubMed]
- Muhammad, F.F.; Aziz, S.B.; Hussein, S.A. Effect of the dopant salt on the optical parameters of PVA:NaNO3 solid polymer electrolyte. J. Mater. Sci. Mater. Electron. 2015, 26, 521–529. [Google Scholar] [CrossRef]
- Abdulwahid, R.T.; Abdullah, O.G.; Aziz, S.B.; Hussein, S.A.; Muhammad, F.F.; Yahya, M.Y. The study of structural and optical properties of PVA:PbO2 based solid polymer nanocomposites. J. Mater. Sci. Mater. Electron. 2016, 27, 12112–12118. [Google Scholar] [CrossRef]
- Soni, A.; Dashora, A.; Gupta, V.; Arora, C.M.; Rérat, M.; Ahuja, B.L.; Pandey, R. Electronic and optical modeling of solar cell compounds CuGaSe 2 and CuInSe 2. J. Electron. Mater. 2011, 40, 2197–2208. [Google Scholar] [CrossRef]
- Mahmood, Q.; Haq, B.U.; Yaseen, M.; Ramay, S.M.; Ashiq, M.G.B.; Mahmood, A. The first-principle study of mechanical, optical and thermoelectric properties of SnZrO 3 and SnHfO 3 for renewable energy applications. Solid State Commun. 2019, 292, 17–23. [Google Scholar] [CrossRef]
- Kreher, K. Fundamentals of Semiconductors–Physics and Materials Properties. Z. Phys. Chem. 1997, 198, 275. [Google Scholar] [CrossRef]
- Bouzidi, C.; Horchani-Naifer, K.; Khadraoui, Z.; Elhouichet, H.; Ferid, M. Synthesis, characterization and DFT calculations of electronic and optical properties of CaMoO4. Phys. B Condens. Matter. 2016, 497, 34–38. [Google Scholar] [CrossRef]
- Ravindra, N.; Ganapathy, P.; Choi, J. Energy gap-refractive index relations in semiconductors-An overview (in press version with book). Infrared Phys. Technol. 2007, 50, 21–29. Available online: http://linkinghub.elsevier.com/retrieve/pii/S135044950600048X (accessed on 25 November 2022).
- Rasul, S.M.; Saber, D.R.; Aziz, S.B. Role of Titanium replacement with Pd atom on band gap reduction in the anatase Titanium Dioxide: First-Principles calculation approach. Results Phys. 2022, 38, 105688. [Google Scholar] [CrossRef]
- Qadr, R.A.; Saber, D.R.; Aziz, S.B. Theoretical Investigations of Electronic and Optical Properties of Vanadium Doped Wurtzite Zinc Oxide from First Principle Calculation Method. Iraqi J. Phys. 2022, 20, 38–52. [Google Scholar] [CrossRef]
- Hossain, F.M.; Sheppard, L.; Nowotny, J.; Murch, G.E. Optical properties of anatase and rutile titanium dioxide: Ab initio calculations for pure and anion-doped material. J. Phys. Chem. Solids 2008, 69, 1820–1828. [Google Scholar] [CrossRef]
- Feng, J.; Xiao, B.; Chen, J.C.; Zhou, C.T.; Du, Y.P.; Zhou, R. Optical properties of new photovoltaic materials: AgCuO2 and Ag2Cu2O3. Solid State Commun. 2009, 149, 1569–1573. [Google Scholar] [CrossRef]
- Logothetidis, S. Optical and electronic properties of amorphous carbon materials. Diam. Relat. Mater. 2003, 12, 141–150. [Google Scholar] [CrossRef]
- Su, B.; Zhou, Y.-G. Improvement of transparencies and mechanical properties of poly (cyclohexylene dimethylene cyclohexanedicarboxylate) parts using a compounding nucleating agent to control crystallization. Materials 2019, 12, 563. [Google Scholar] [CrossRef] [PubMed]


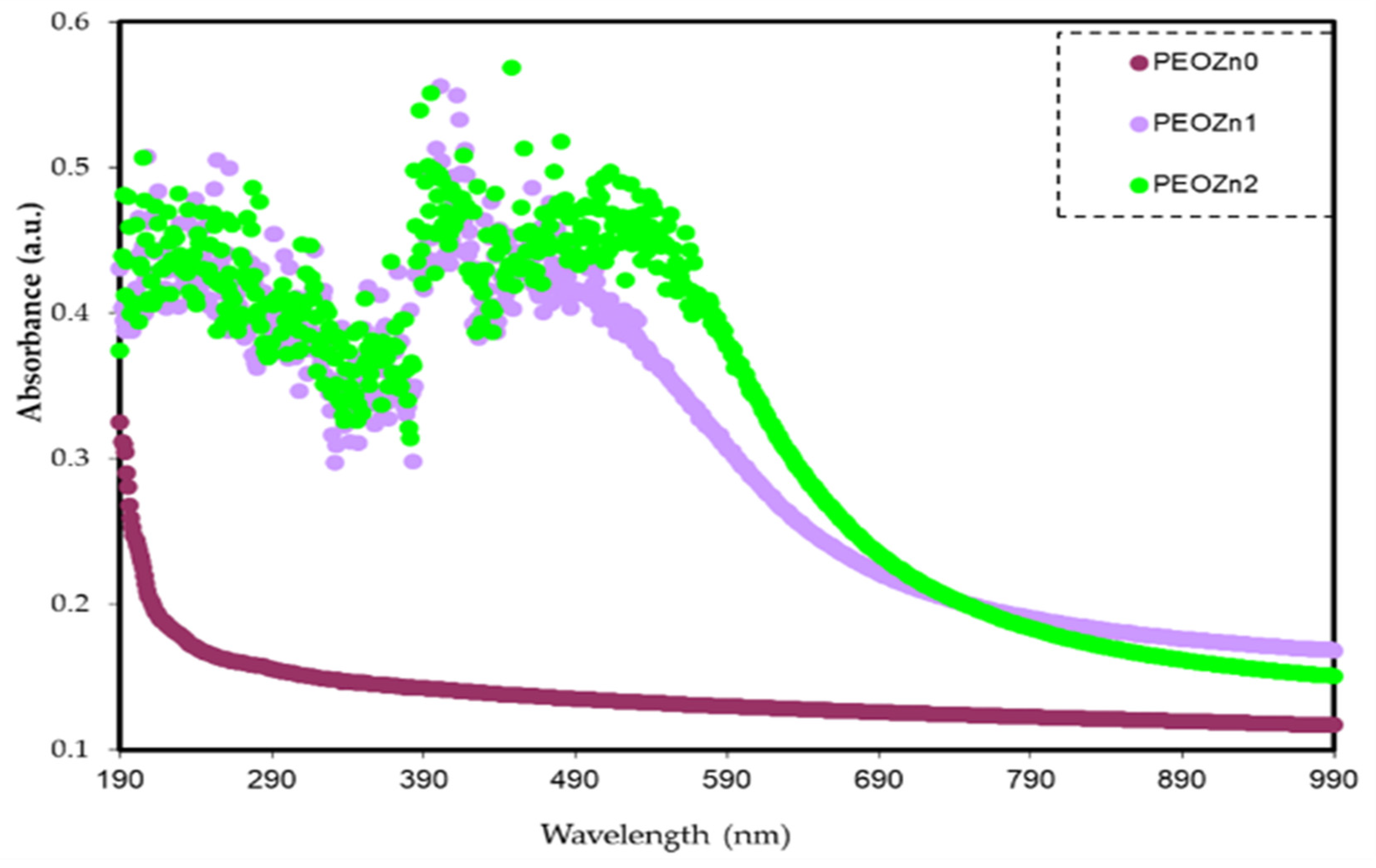
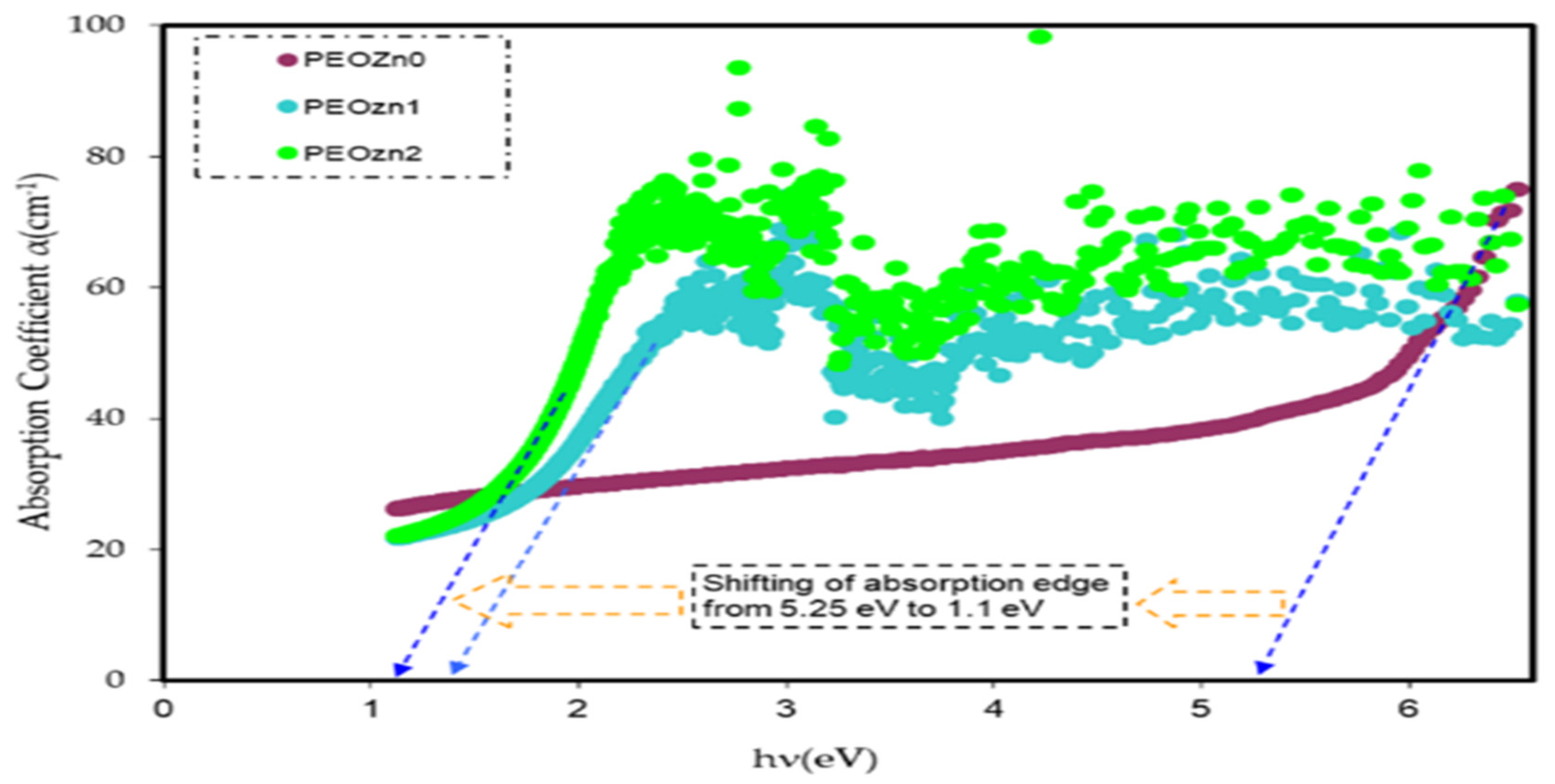

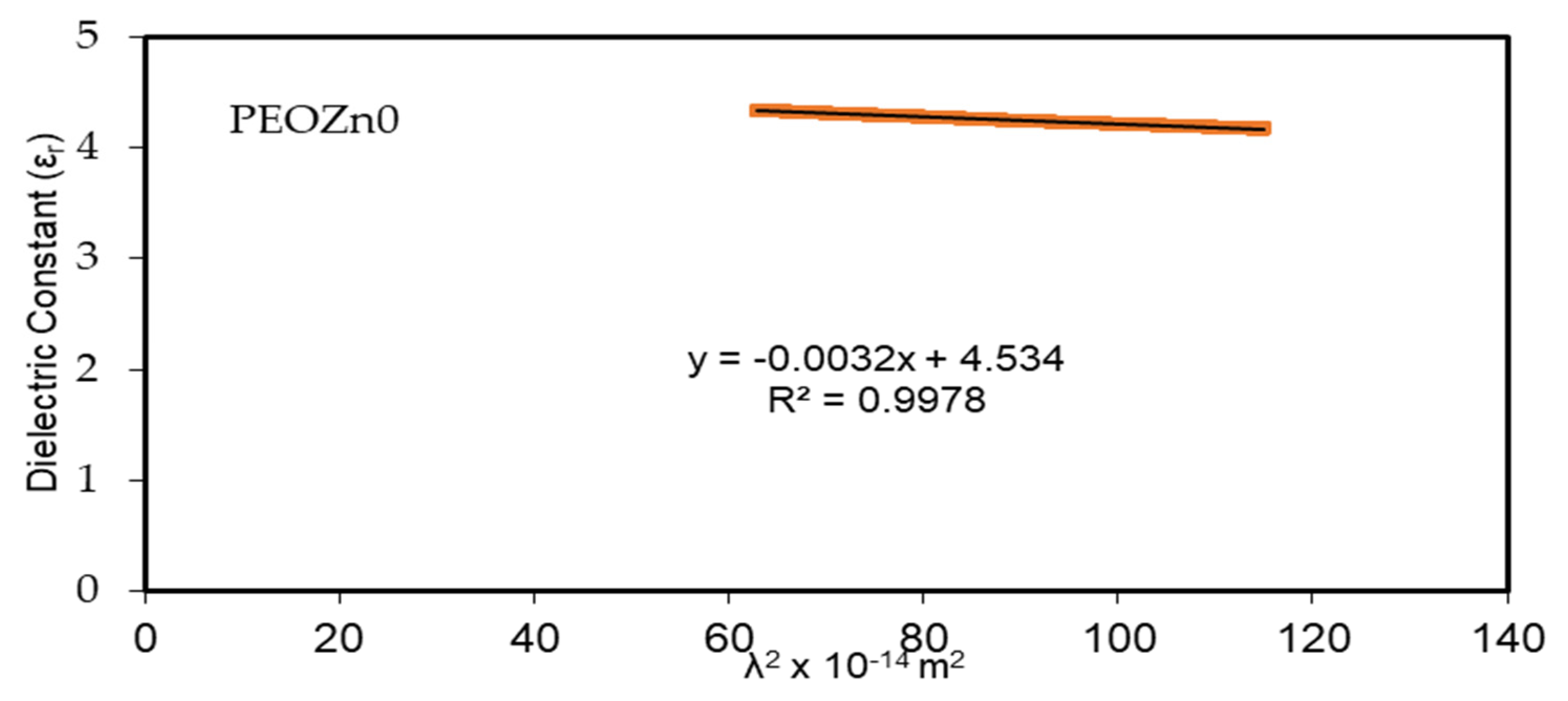


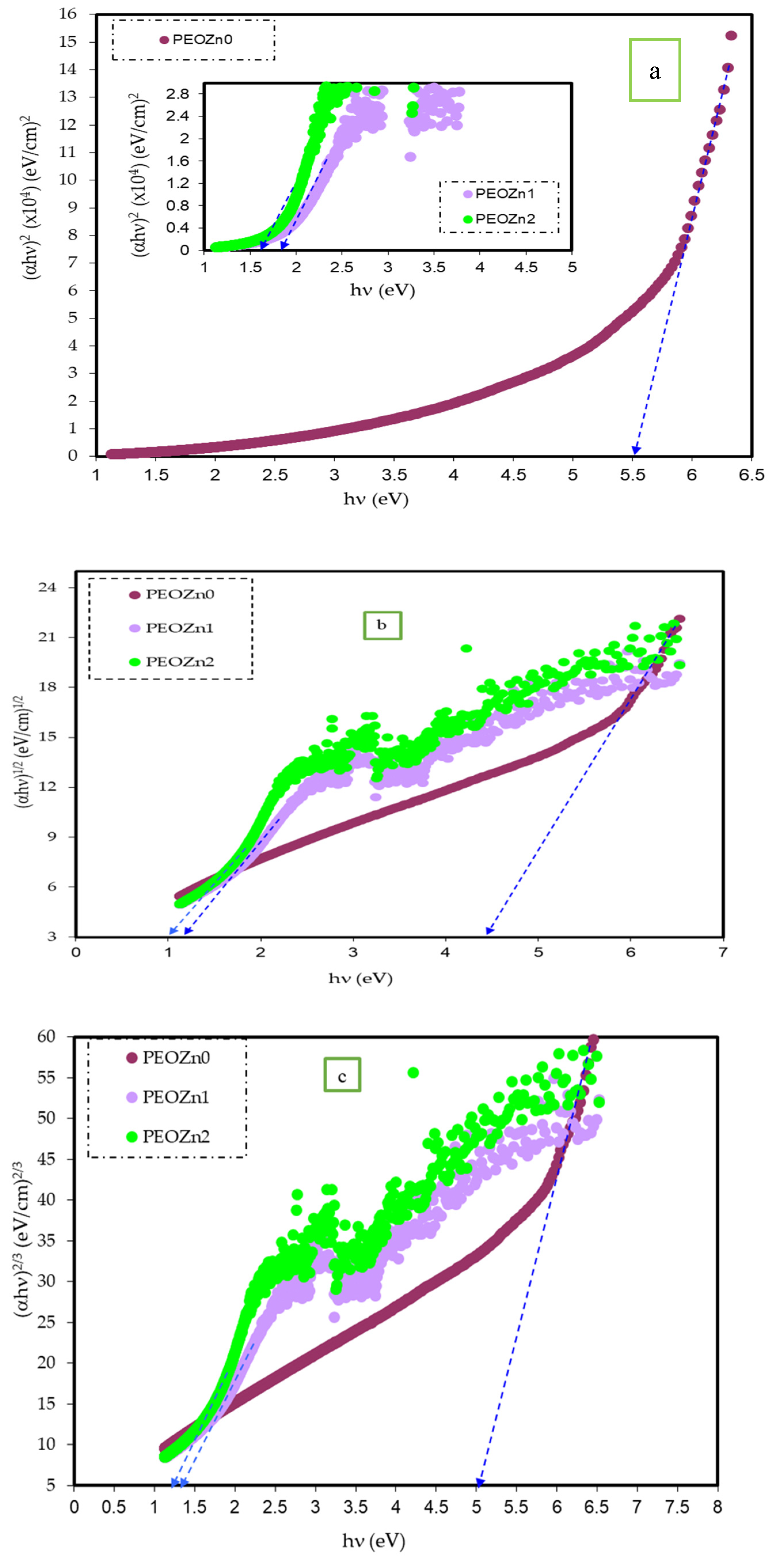
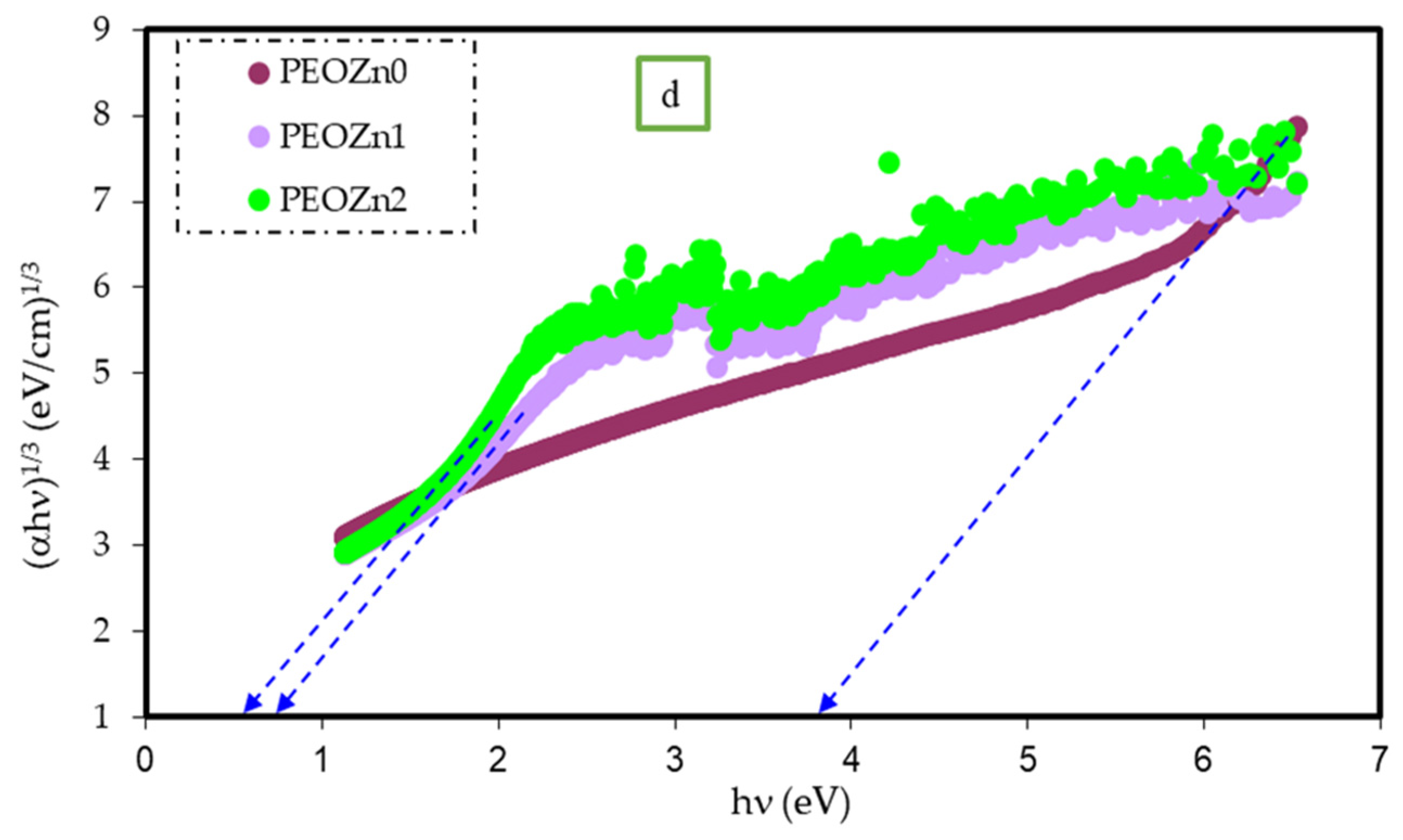

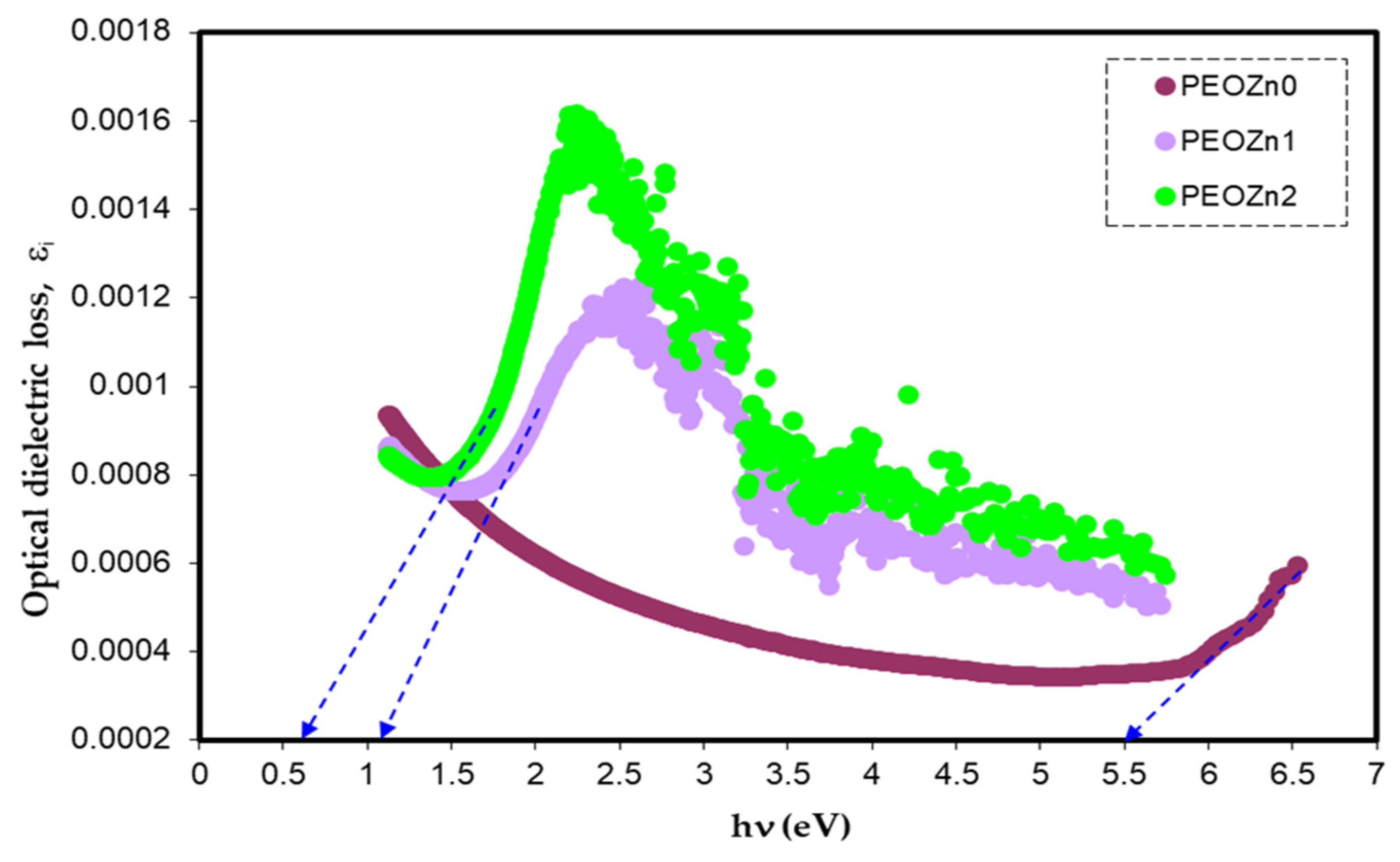
| Sample Code | Absorption Edge (eV) |
|---|---|
| PEOZn0 | 5.25 |
| PEOZn1 | 1.35 |
| PEOZn2 | 1.1 |
| Films | Ed | Eo | no |
|---|---|---|---|
| PEOZn0 | 20.48618 | 6.508456 | 4.147624 |
| PEOZn1 | 12.56535 | 3.430341 | 4.663004 |
| PEOZn2 | 10.04058 | 3.083462 | 4.256268 |
| Physical Parameter | Value |
|---|---|
| Mass of electron (me) | 9.109 × 10−31 Kg |
| Charge of electron (e) | 1.602 × 10−19 coulombs |
| Permittivity of free space (ԑo) | 8.85 × 10−12 F/m |
| π | 3.14 |
| Speed of light (c) | 2.99 × 108 m/s |
| Effective mass (m*) | 10.566 × 10−31 Kg |
| Film | N/m* × 1055 (m3/kg) | ɛ∞ |
|---|---|---|
| PEOZn0 | 3.89 | 4.54 |
| PEOZn1 | 5.96 | 5.6973 |
| PEOZn2 | 6.93 | 5.4503 |
| Sample Code | Eg for (αhv)2 | Eg for (αhv)2/3 | Eg for (αhv)1/2 | Eg for (αhv)1/3 | Eg from ɛi |
|---|---|---|---|---|---|
| PEOZn0 | 5.5 | 5 | 4.42 | 3.8 | 5.5 |
| PEOZn1 | 1.9 | 1.28 | 1.13 | 0.71 | 1.13 |
| PEOZn2 | 1.67 | 1.23 | 1 | 0.6 | 0.61 |
| Polymer Composite | Band Gap Energy Eg (eV) | Reference |
|---|---|---|
| CS: silver nanoparticles | 2.8–1.4 | [111] |
| PS: (CaTiO3) | 4.42–4.26 | [112] |
| (CS: POZ): Zn2+-PPL complex | 4.8–1.6 | [113] |
| PVA: CeO2 | 6.34–6.09 | [114] |
| PS:tin titanate nanoparticles | 4.42–3.25 | [115] |
| PVA: Al3+-metal complex | 6.39–1.68 | [54] |
| PVA: Co2+-polyphenol complex | 5.8–1.82 | [53] |
| PEO: CaTiO3nanoparticles | 4.90–4.19 | [116] |
| PVA: NaNO3 | 5.71–5.05 | [117] |
| PVA: PbO2 | 6.32–4.33 | [118] |
| PEO: Zn metal complex | 5.5–0.61 | Present work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Muheddin, D.Q.; Aziz, S.B.; Mohammed, P.A. Variation in the Optical Properties of PEO-Based Composites via a Green Metal Complex: Macroscopic Measurements to Explain Microscopic Quantum Transport from the Valence Band to the Conduction Band. Polymers 2023, 15, 771. https://doi.org/10.3390/polym15030771
Muheddin DQ, Aziz SB, Mohammed PA. Variation in the Optical Properties of PEO-Based Composites via a Green Metal Complex: Macroscopic Measurements to Explain Microscopic Quantum Transport from the Valence Band to the Conduction Band. Polymers. 2023; 15(3):771. https://doi.org/10.3390/polym15030771
Chicago/Turabian StyleMuheddin, Daron Q., Shujahadeen B. Aziz, and Pshko A. Mohammed. 2023. "Variation in the Optical Properties of PEO-Based Composites via a Green Metal Complex: Macroscopic Measurements to Explain Microscopic Quantum Transport from the Valence Band to the Conduction Band" Polymers 15, no. 3: 771. https://doi.org/10.3390/polym15030771
APA StyleMuheddin, D. Q., Aziz, S. B., & Mohammed, P. A. (2023). Variation in the Optical Properties of PEO-Based Composites via a Green Metal Complex: Macroscopic Measurements to Explain Microscopic Quantum Transport from the Valence Band to the Conduction Band. Polymers, 15(3), 771. https://doi.org/10.3390/polym15030771







