Using Standstill Time to Evaluate the Startup in Polymer Pair Systems
Abstract
:1. Introduction
2. Materials and Methods
2.1. Samples
2.2. Method of Measuring Friction
2.3. Evaluation of Rubbing Surface Conditions
3. Results and Discussion
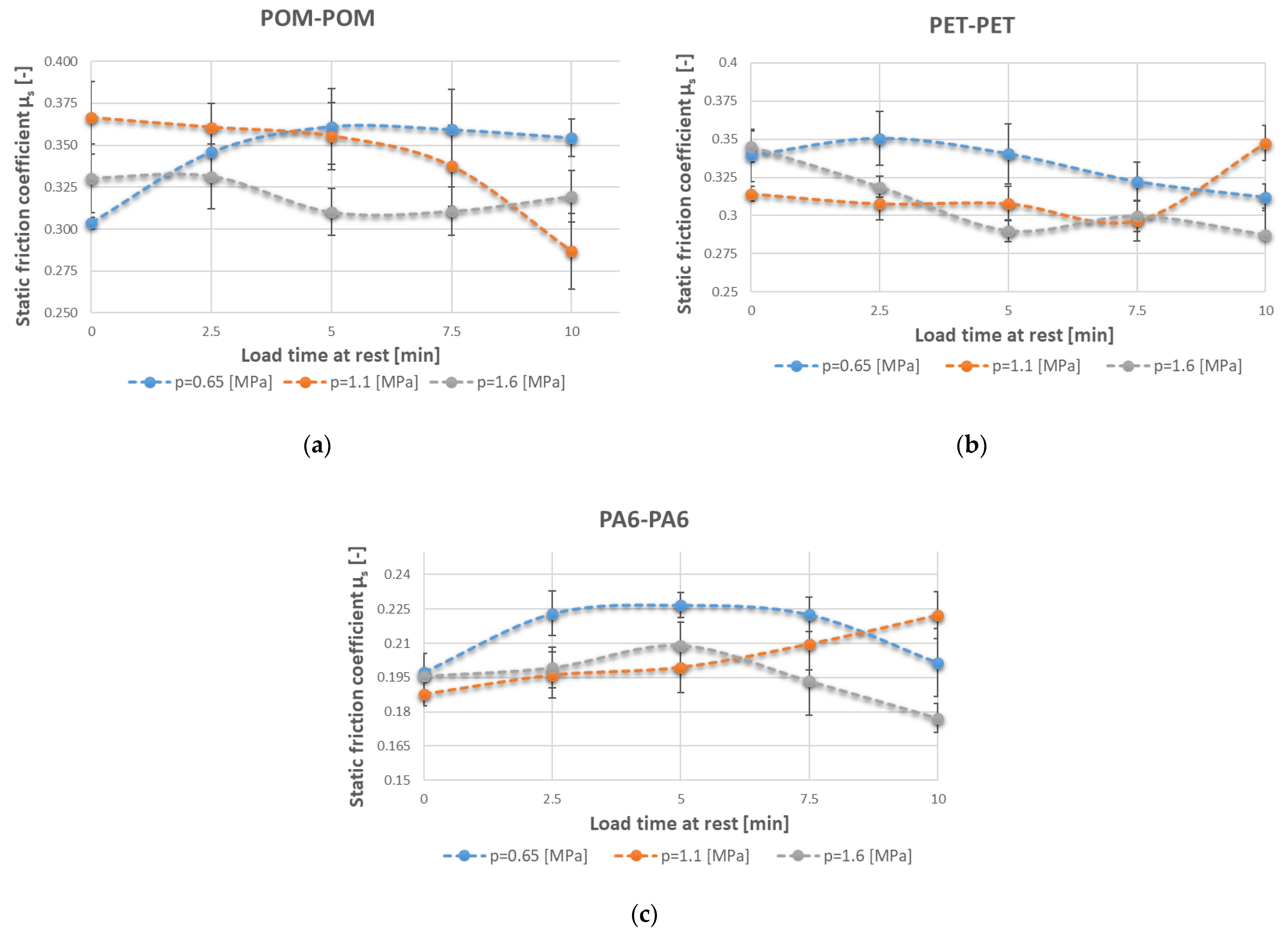
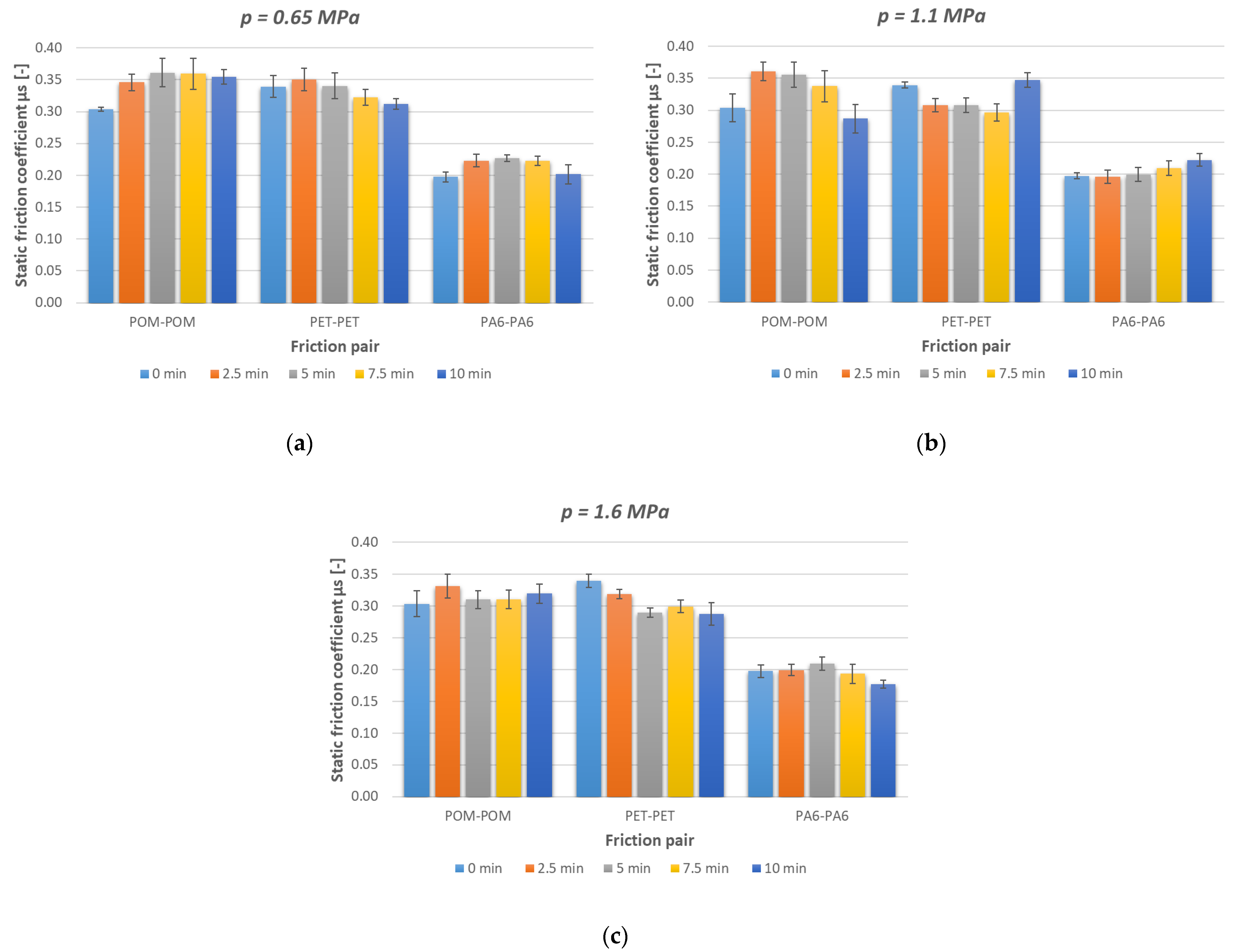
3.1. Analysis of the Effects of Standstill Time and Unit Pressure on the Static Friction Coefficient
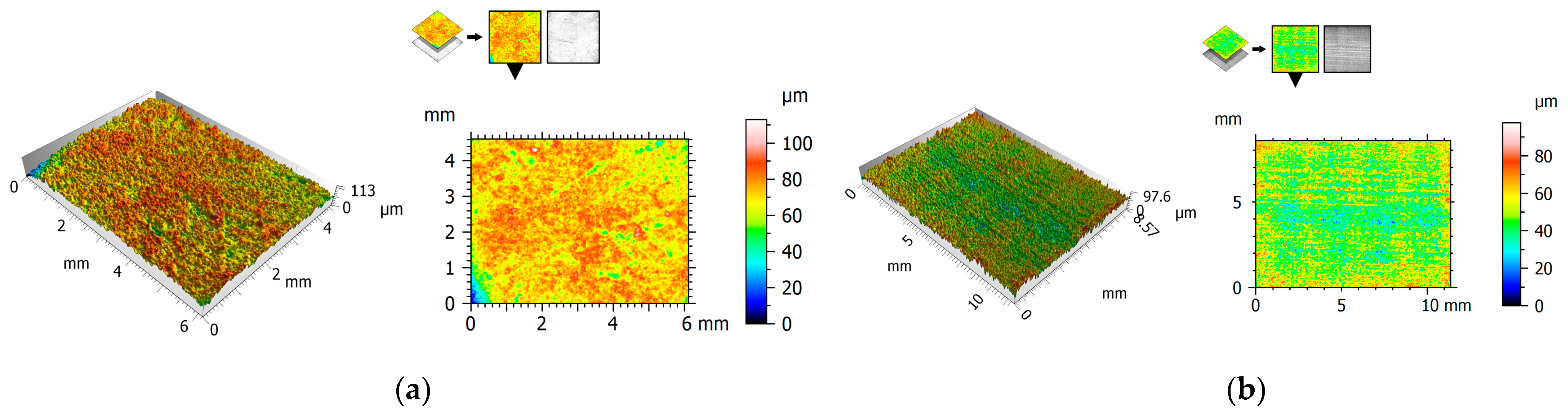
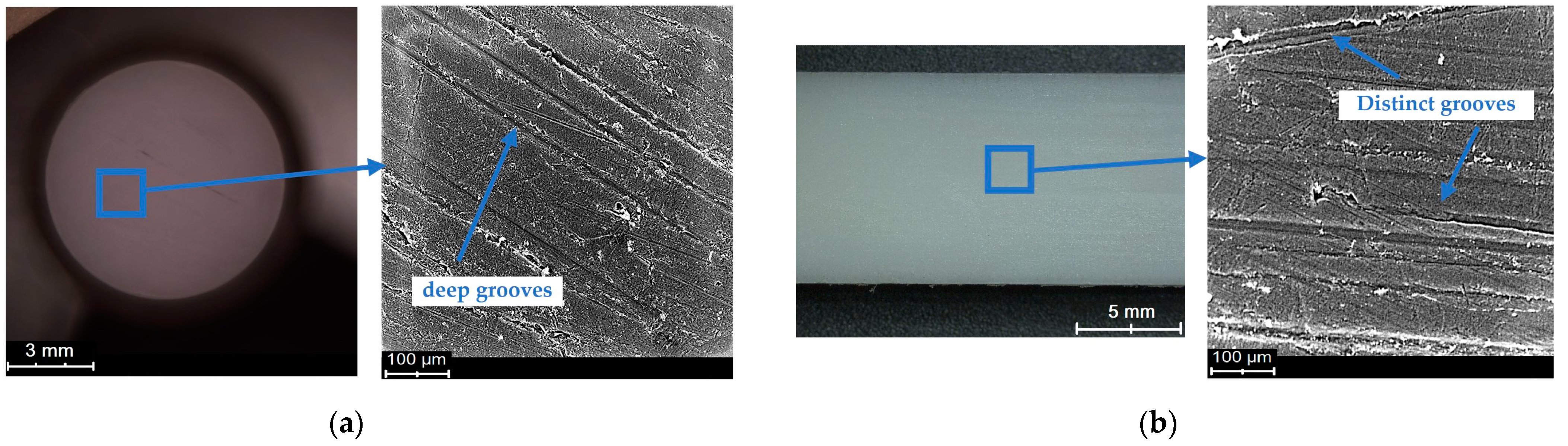
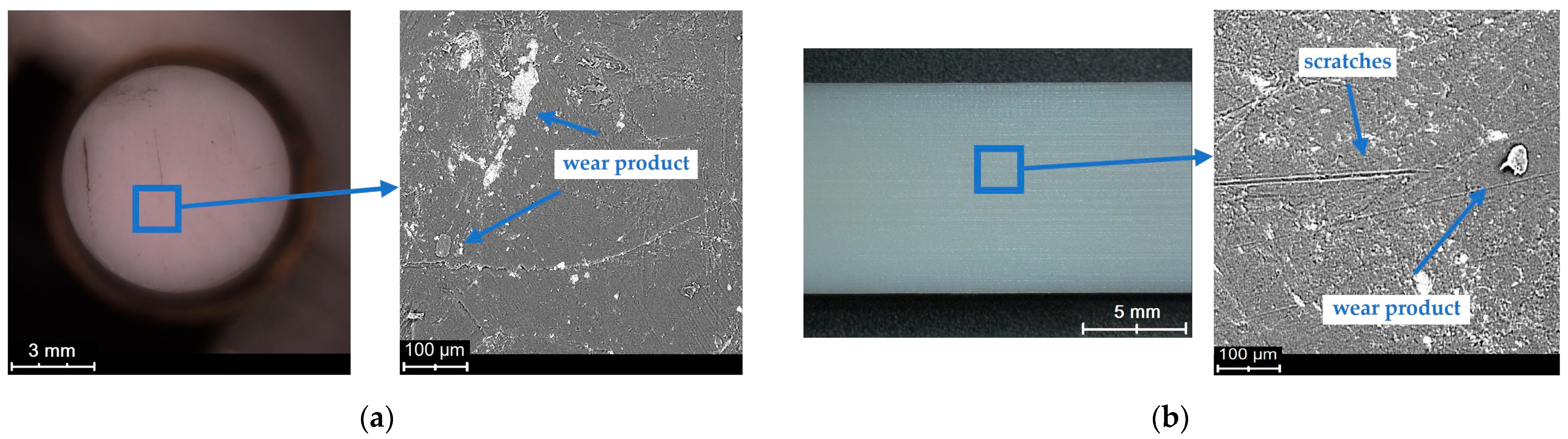
3.2. Qualitative Analysis of the Rubbing Surfaces
3.3. Evaluation of the Phenomena Taking Place on the Rubbing Surfaces
3.4. Summary of Results
4. Conclusions
- The test results clearly show that changing the process parameters for the tested polymer–polymer sliding pairs significantly impacts both the unit pressure and the standstill time under static load on the startup parameters of the friction system. A general downward tendency is visible for the static friction coefficient depending on the load time at rest.
- For the particular loads, an increase in stationary contact time affects the static friction coefficient value, but it does not reveal unequivocal relationships between the materials. As regards sliding properties, the PA6–PA6 pair has the most advantageous tribological characteristics. The relatively low coefficient of static friction was due to an increase in the actual contact area and a decrease in the actual unit pressure.
- A marked abrupt change in the static friction coefficient value for the longest adopted load time of t = 10 min was visible (at a downward tendency it began to rise and vice versa). The literature reports quite different findings on the static friction coefficient, but investigated for the metal–polymer pair [11,28]. According to reports, the greatest tribological changes take place during motion initiation after a very short load time—up to a maximum of t = 10 min. At longer times, these characteristics are already more stable and constant. Hence, one can conclude that the general characteristics ascribed to various relationships have not been corroborated for the tested polymer–polymer sliding pairs.
- On the basis of the identified scratches, grooves, and wear products, one can conclude that, unlike the elastic response, the transition from the partial to full slip of the viscoelastic materials exhibits a dynamic tendency. When identical contact parameters are determined for different viscoelastic materials, one can expect a quicker transition from the partial slip to the full slip at the different microhardness and thermal conductivity of the tested materials [28,50].
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Popova, E.; Popov, V.L. The research works of Coulomb and Amontons and generalized laws of friction. Friction 2015, 3, 183–190. [Google Scholar] [CrossRef]
- Hu, J.; Liu, X.; Wei, Y. Effect of plasticity and adhesion on the stick-slip transition at nanoscale friction. Tribol. Int. 2021, 164, 107230. [Google Scholar] [CrossRef]
- Persson, B.N.J.; Albohr, O.; Mancosu, F.; Peveri, V.; Samoilov, V.N.; Sivebaek, I.M. On the nature of the static friction, kinetic friction and creep. Wear 2003, 254, 835–851. [Google Scholar] [CrossRef]
- Ptak, A.; Taciak, P.; Wieleba, W. Effect of Temperature on the Tribological Properties of Selected Thermoplastic Materials Cooperating with Aluminium Alloy. Materials 2021, 14, 7318. [Google Scholar] [CrossRef] [PubMed]
- Rill, G.; Schuderer, M. A Second-Order Dynamic Friction Model Compared to Commercial Stick–Slip Models. Modeling 2023, 4, 366–381. [Google Scholar]
- Hwang, D.H.; Zum Gahr, K.H. Transition from static to kinetic friction of unlubricated or oil lubricated steel/steel, steel/ceramic and ceramic/ceramic pairs. Wear 2003, 255, 365–375. [Google Scholar] [CrossRef]
- Hausberger, A.; Pecora, M.; Favier, D.; Rossegger, E.; Tockner, M.; Ules, T.; Haselmann, M.; Schlögl, S.; Gauthier, C. In Situ Observation of Micro-Patterned Elastomeric Surfaces: The Formation of the Area of Real Contact and the Influence on Its Friction and Deformation Behaviour. Materials 2023, 16, 6489. [Google Scholar] [CrossRef]
- Benabdallah, H.S. Static friction coefficient of some plastics against steel and aluminum under different contact conditions. Tribol. Int. 2007, 40, 64–73. [Google Scholar] [CrossRef]
- Opałka, M.; Wieleba, W.; Radzińska, A. Influence of the Dynamics of Forced Motion on the Static Friction in Metal-Polymer Sliding Pairs. Tribologia 2021, 295, 21–26. [Google Scholar] [CrossRef]
- Deladi, E.L.; de Rooij, M.B.; Schipper, D.J. Modelling of static friction in rubber-metal contact. Tribol. Int. 2007, 40, 588–594. [Google Scholar] [CrossRef]
- Capanidis, D.; Sokolska, J. the Effect of Pressure and Time of Stationary Contact Under Load on the Static Friction Coefficient of Polyurethane Elastomers (Epur) during Friction on Steel in Various Lubricating Conditions. Tribologia 2020, 289, 21–29. [Google Scholar] [CrossRef]
- Chen, L.; Ruan, X.; Guo, X.; Chen, W.; Fu, L. A new quasi-static friction model for the spherical hinge bearing in structural engineering. Eng. Struct. 2023, 291, 116414. [Google Scholar] [CrossRef]
- Liang, Q.; Liang, P.; Guo, F.; Li, S.; Zhang, X.; Jiang, F. The Influence of Scratches on the Tribological Performance of Friction Pairs Made of Different Materials under Water-Lubrication Conditions. Lubricants 2023, 11, 449. [Google Scholar] [CrossRef]
- Heß, M.; Li, Q.; Chen, J.; Dong, N.; Min, J. Study on the Coupling Relationship between Wear and Dynamics in Planetary Gear Systems. Machines 2023, 11, 986. [Google Scholar]
- Evangelista, I.; Wencel, D.; Beguin, S.; Zhang, N.; Gilchrist, M.D. Influence of Surface Texturing on the Dry Tribological Properties of Polymers in Medical Devices. Polymers 2023, 15, 2858. [Google Scholar] [CrossRef] [PubMed]
- Gadelmoula, A.; Aldahash, S.A. Dry Friction and Wear Behavior of Laser-Sintered Graphite/Carbon Fiber/Polyamide 12 Composite. Polymers 2023, 15, 3916. [Google Scholar] [CrossRef] [PubMed]
- Yilmaz, M.; Mirza, M.; Lohner, T.; Stahl, K. Superlubricity in EHL Contacts with Water-Containing Gear Fluids. Lubricants 2019, 7, 46. [Google Scholar] [CrossRef]
- Bin Jaber, S.; Xu, Y.; Kartal, M.E.; Gadegaard, N.; Mulvihill, D.M. The static friction peak in reciprocating sliding. Tribol. Int. 2023, 180, 108240. [Google Scholar] [CrossRef]
- Erhard, G. Sliding friction behaviour of polymer-polymer material combinations. Wear 1983, 84, 167–181. [Google Scholar] [CrossRef]
- Maeda, N.; Chen, N.; Tirrell, M.; Israelachvili, J.N. Adhesion and friction mechanisms of polymer-on-polymer surfaces. Science 2002, 297, 379–382. [Google Scholar] [CrossRef]
- Jia, B.B.; Li, T.S.; Liu, X.J.; Cong, P.H. Tribological behaviors of several polymer–polymer sliding combinations under dry friction and oil-lubricated conditions. Wear 2007, 262, 1353–1359. [Google Scholar] [CrossRef]
- Rymuza, Z.; Kusznierewicz, Z.; Solarski, T.; Kwacz, M.; Chizhik, S.A.; Goldade, A. V Static friction and adhesion in polymer-polymer microbearings. Wear 2000, 238, 56–69. [Google Scholar] [CrossRef]
- Rymuza, Z.; Kusznierewicz, Z.; Mańturzyk, G. Testing miniature, in particular polymer-polymer, journal bearings. Wear 1994, 174, 39–46. [Google Scholar] [CrossRef]
- Rymuza, Z. Tribology of Polymers. Arch. Civ. Mech. Eng. 2007, 7, 177–184. [Google Scholar] [CrossRef]
- Bahadur, S. The development of transfer layers and their role in polymer tribology. Wear 2000, 245, 92–99. [Google Scholar] [CrossRef]
- Bowden, F.; Tabor, D. The Friction and Lubrication of Solids; Oxford University Press: Oxford, UK, 1950. [Google Scholar]
- Sahli, R.; Pallares, G.; Ducottet, C.; Ben Ali, I.E.; Al Akhrass, S.; Guibert, M.; Scheibert, J. Evolution of real contact area under shear and the value of static friction of soft materials. Proc. Natl. Acad. Sci. USA 2018, 115, 471–476. [Google Scholar] [CrossRef] [PubMed]
- Albertini, G.; Karrer, S.; Grigoriu, M.D.; Kammer, D.S. Stochastic properties of static friction. J. Mech. Phys. Solids 2021, 147, 104242. [Google Scholar] [CrossRef]
- Pouzada, A.S.; Ferreira, E.C.; Pontes, A.J. Friction properties of moulding thermoplastics. Polym. Test. 2006, 25, 1017–1023. [Google Scholar] [CrossRef]
- Bazrafshan, M.; de Rooij, M.B.; Schipper, D.J. On the role of adhesion and roughness in stick-slip transition at the contact of two bodies: A numerical study. Tribol. Int. 2018, 121, 381–388. [Google Scholar] [CrossRef]
- Li, S.; Zhang, S.; Chen, Z.; Feng, X.Q.; Li, Q. Length Scale Effect in Frictional Aging of Silica Contacts. Phys. Rev. Lett. 2020, 125, 215502. [Google Scholar] [CrossRef]
- Filippov, A.E.; Klafter, J.; Urbakh, M. Friction through dynamical formation and rupture of molecular bonds. Phys. Rev. Lett. 2004, 92, 135503. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Yu, Q.; Zhang, J.; Jiang, Y. Microstructure and deformation mechanism of Mg6Al1ZnA alloy experienced tension–compression cyclic loading. Scr. Mater. 2011, 64, 233–236. [Google Scholar] [CrossRef]
- Sahli, R.; Pallares, G.; Papangelo, A.; Ciavarella, M.; Ducottet, C.; Ponthus, N.; Scheibert, J. Shear-Induced Anisotropy in Rough Elastomer Contact. Phys. Rev. Lett. 2019, 122, 214301. [Google Scholar] [CrossRef] [PubMed]
- Weber, B.; Suhina, T.; Brouwer, A.M.; Bonn, D. Frictional weakening of slip interfaces. Sci. Adv. 2019, 5, eaav7603. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Scheibert, J.; Gadegaard, N.; Mulvihill, D.M. An asperity-based statistical model for the adhesive friction of elastic nominally flat rough contact interfaces. J. Mech. Phys. Solids 2022, 164, 104878. [Google Scholar] [CrossRef]
- Wayne, W.; Wang, C.Q.J.; Huan, Z.; Luo, X. Semi-Analytical Viscoelastic Contact Modeling of Polymer-Based Materials. J. Tribol. 2011, 133, 041404. [Google Scholar] [CrossRef]
- Hualong, Y.; Li, Z.; Wang, Q.J. Viscoelastic-adhesive contact modeling: Application to the characterization of the viscoelastic behavior of materials. Mech. Mater. 2013, 60, 55–65. [Google Scholar]
- Jerrams, S.J. Friction and adhesion in rigid surface indentation of nitrile rubber. Mater. Des. 2005, 26, 251–258. [Google Scholar] [CrossRef]
- Spencer, N.D.; Tysoe, W.T. The Cutting Edge of Tribology. Cut. Edge Tribol. 2015, 111, 74. [Google Scholar]
- Vijaya Bhaskar, K.; Sundarrajan, S.; Subba Rao, B.; Ravindra, K. Effect of reinforcement and wear parameters on dry sliding wear of aluminum composites—A review. Mater. Today Proc. 2018, 5, 5891–5900. [Google Scholar] [CrossRef]
- Tung, S.C.; McMillan, M.L. Automotive tribology overview of current advances and challenges for the future. Tribol. Int. 2004, 37, 517–536. [Google Scholar] [CrossRef]
- Ptak, A. The influence of the motion parameters on the surface layer of metal-polimer sliding pairs at low temperature. Tribologia 2016, 265, 79–87. [Google Scholar] [CrossRef]
- Niemczewska-Wójcik, M.; Wójcik, A. The multi-scale analysis of ceramic surface topography created in abrasive machining process. Measurement 2020, 166, 108217. [Google Scholar] [CrossRef]
- Pogačnik, A.; Kupec, A.; Kalin, M. Tribological properties of polyamide (PA6) in self-mated contacts and against steel as a stationary and moving body. Wear 2017, 378, 17–26. [Google Scholar] [CrossRef]
- Moeskops, E.; Kamperman, N.; van de Vorst, B.; Knoppers, R. Creep Behaviour of Polyamide in Selective Laser Sintering. In Proceedings of the 2004 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 2–4 August 2004. [Google Scholar]
- Fiorenza De Lima, H.; Augusto Vaz, M.; Ferreira Da Costa, M.; Ariza Gomez, A.; Lima De Oliveira, G. Creep behavior of in-service flexible flowline polyamide 11. Polym. Test. 2020, 81, 106205. [Google Scholar] [CrossRef]
- Kowalewski, P.; Wieleba, W.; Ptak, A. The influence of friction kinematic parameters on microhardness of sliding polymers. Tribologia 2011, 5, 109–116. [Google Scholar]
- Erhard, G.; Thompson, M. Designing with Plastics; Hanser Publications: Cincinnati, OH, USA, 2006. [Google Scholar]
- Wang, D.; de Boer, G.; Ghanbarzadeh, A. A Numerical Model for Investigating the Effect of Viscoelasticity on the Partial Slip Solution. Materials 2022, 15, 5182. [Google Scholar] [CrossRef]
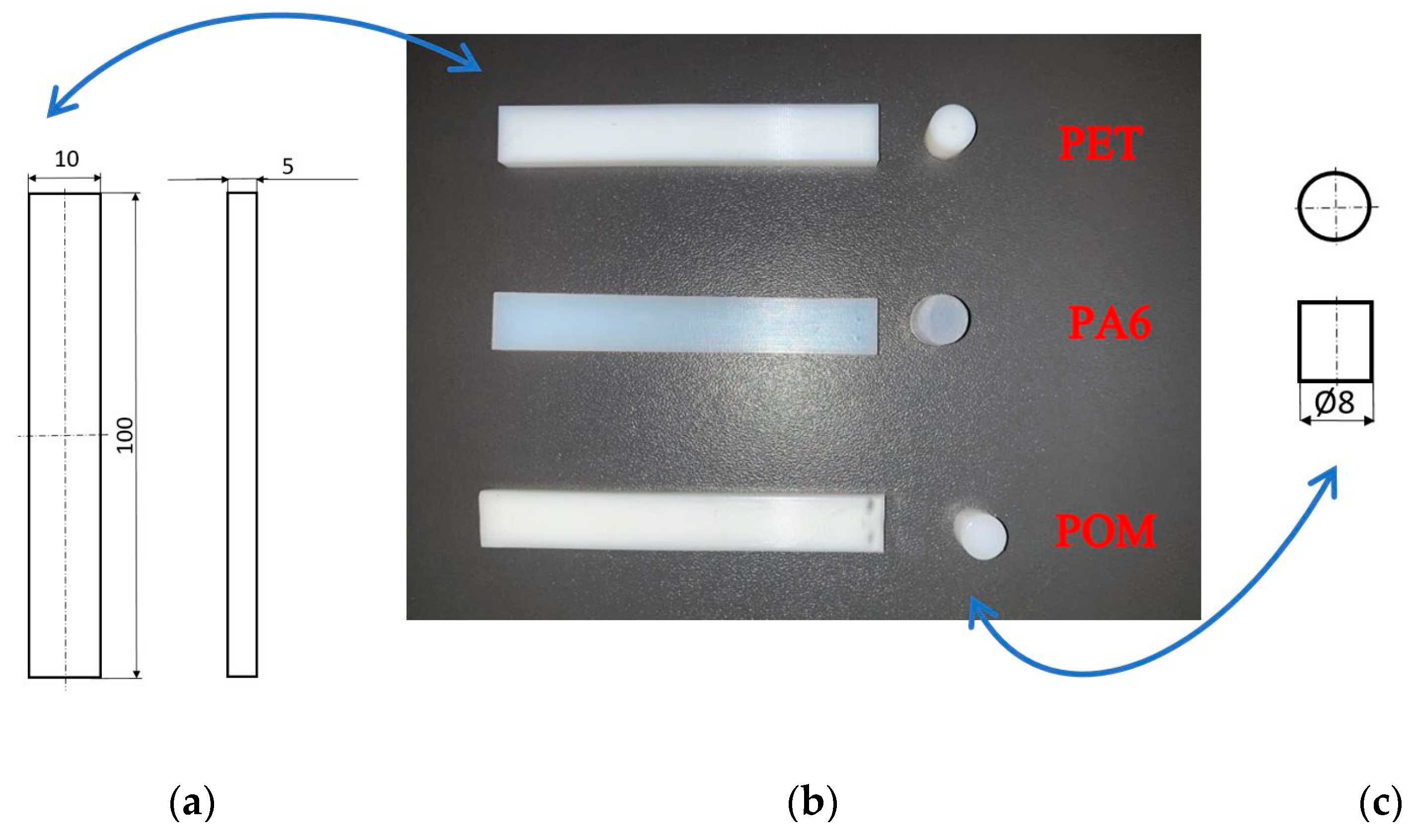
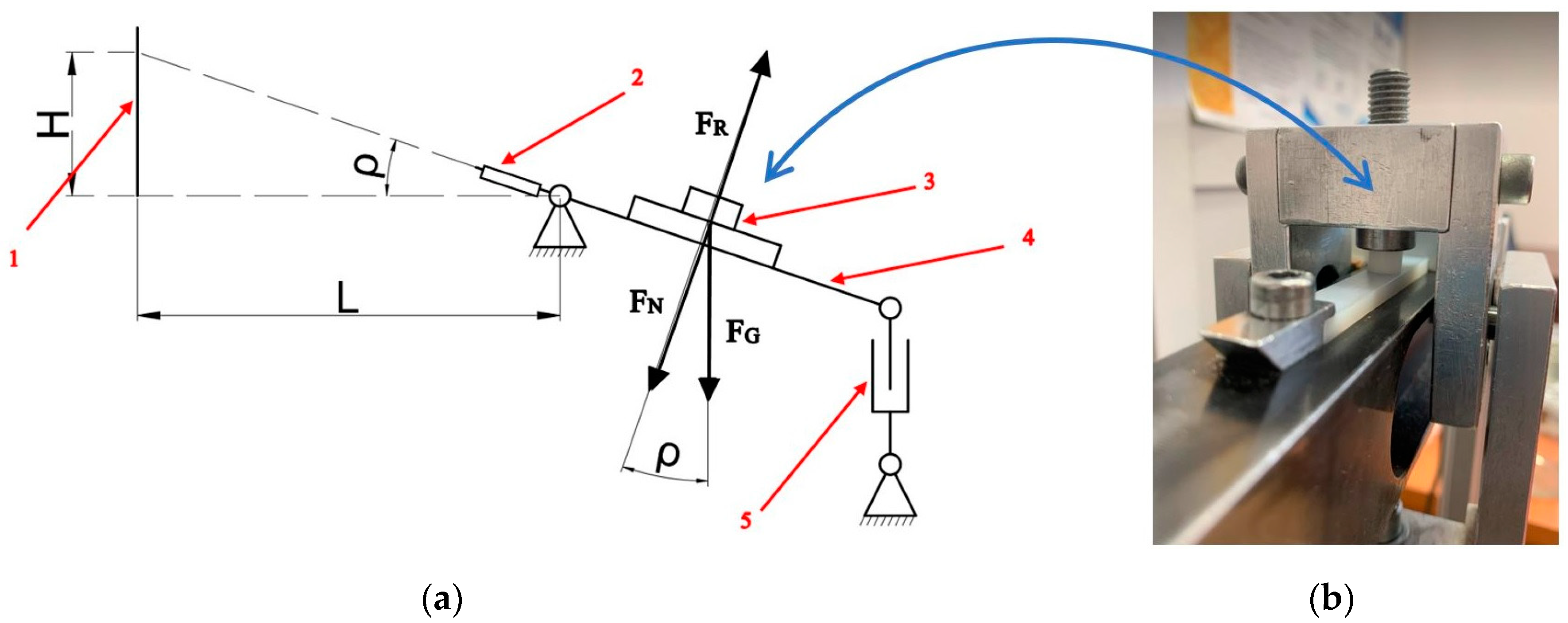



| Property | POM | PA6 | PET |
|---|---|---|---|
| Composition | Non-modified | Non-modified | Non-modified |
| Yield point, Re | 62 MPa | 60 MPa | 80 MPa |
| Modulus of tensile elasticity, Er | 2.7 GPa | 1.80 GPa | 2.8 GPa |
| Long-term use temperature | 100 °C | 100 °C | 110 °C |
| Glass transition temperature, Tg | −60 °C | +60 °C | 70 °C |
| Density, ρ | 1.41 g/cm3 | 1.13 g/cm3 | 1.37 g/cm3 |
| Water absorption, Ws | 0.5% | 9.5% | 0.5% |
| Experimental Parameters | |
|---|---|
| Polymer sliding pair pin-on-plate | POM–POM |
| PA6–PA6 | |
| PET–PET | |
| Weight of pan | ~9.40 N |
| Load force | 25 N, 50 N, 75 N |
| Standstill time | 0–10 min |
| Environment | Technically dry friction |
| Number of repetitions | 10 |
| Standstill | POM–POM | PET–PET | PA6–PA6 | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Time | 0.65 MPa | 1.1 MPa | 1.6 MPa | 0.65 MPa | 1.1 MPa | 1.6 MPa | 0.65 MPa | 1.1 MPa | 1.6 MPa | |||||||||
| (min) | µav. | α | µav. | α | µav. | α | µav. | α | µav. | α | µav. | α | µav. | α | µav. | α | µav. | α |
| 0 | 0.30 | 0.00 | 0.37 | 0.02 | 0.33 | 0.02 | 0.34 | 0.02 | 0.31 | 0.00 | 0.35 | 0.01 | 0.20 | 0.01 | 0.19 | 0.01 | 0.20 | 0.01 |
| 2.5 | 0.35 | 0.01 | 0.36 | 0.01 | 0.33 | 0.02 | 0.35 | 0.02 | 0.31 | 0.01 | 0.32 | 0.01 | 0.22 | 0.01 | 0.20 | 0.01 | 0.20 | 0.01 |
| 5 | 0.36 | 0.02 | 0.36 | 0.02 | 0.31 | 0.01 | 0.34 | 0.02 | 0.31 | 0.01 | 0.29 | 0.01 | 0.23 | 0.01 | 0.20 | 0.01 | 0.21 | 0.01 |
| 7.5 | 0.36 | 0.02 | 0.34 | 0.02 | 0.31 | 0.01 | 0.32 | 0.01 | 0.30 | 0.01 | 0.30 | 0.01 | 0.22 | 0.01 | 0.21 | 0.01 | 0.19 | 0.02 |
| 10 | 0.35 | 0.01 | 0.29 | 0.02 | 0.32 | 0.02 | 0.31 | 0.01 | 0.35 | 0.01 | 0.29 | 0.02 | 0.20 | 0.01 | 0.22 | 0.01 | 0.18 | 0.01 |
| Roughness Parameters | POM | PET | PA6 | |||
|---|---|---|---|---|---|---|
| Pin | Plate | Pin | Plate | Pin | Plate | |
| Sq (µm) | 5.725 | 13.411 | 8.069 | 9.948 | 8.430 | 11.965 |
| Ssk (–) | 0.421 | −0.879 | −0.301 | 0.041 | −0.849 | −0.299 |
| Sku (–) | 2.444 | 4.005 | 3.512 | 3.114 | 5.879 | 3.300 |
| Sp (µm) | 20.306 | 37.743 | 39.548 | 50.159 | 29.652 | 51.929 |
| Sv (µm) | 14.919 | 69.155 | 41.054 | 47.472 | 58.114 | 58.186 |
| Sz (µm) | 35.225 | 106.898 | 80.602 | 97.631 | 87.766 | 110.115 |
| Sa (µm) | 4.806 | 10.487 | 6.322 | 7.928 | 6.338 | 9.441 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ptak, A.; Łuksza, Z. Using Standstill Time to Evaluate the Startup in Polymer Pair Systems. Polymers 2023, 15, 4696. https://doi.org/10.3390/polym15244696
Ptak A, Łuksza Z. Using Standstill Time to Evaluate the Startup in Polymer Pair Systems. Polymers. 2023; 15(24):4696. https://doi.org/10.3390/polym15244696
Chicago/Turabian StylePtak, Anita, and Zuzanna Łuksza. 2023. "Using Standstill Time to Evaluate the Startup in Polymer Pair Systems" Polymers 15, no. 24: 4696. https://doi.org/10.3390/polym15244696
APA StylePtak, A., & Łuksza, Z. (2023). Using Standstill Time to Evaluate the Startup in Polymer Pair Systems. Polymers, 15(24), 4696. https://doi.org/10.3390/polym15244696





