Investigation of Mechanical Properties of Ultra-High-Performance Polyethylene-Fiber-Reinforced Recycled-Brick-Aggregate Concrete
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
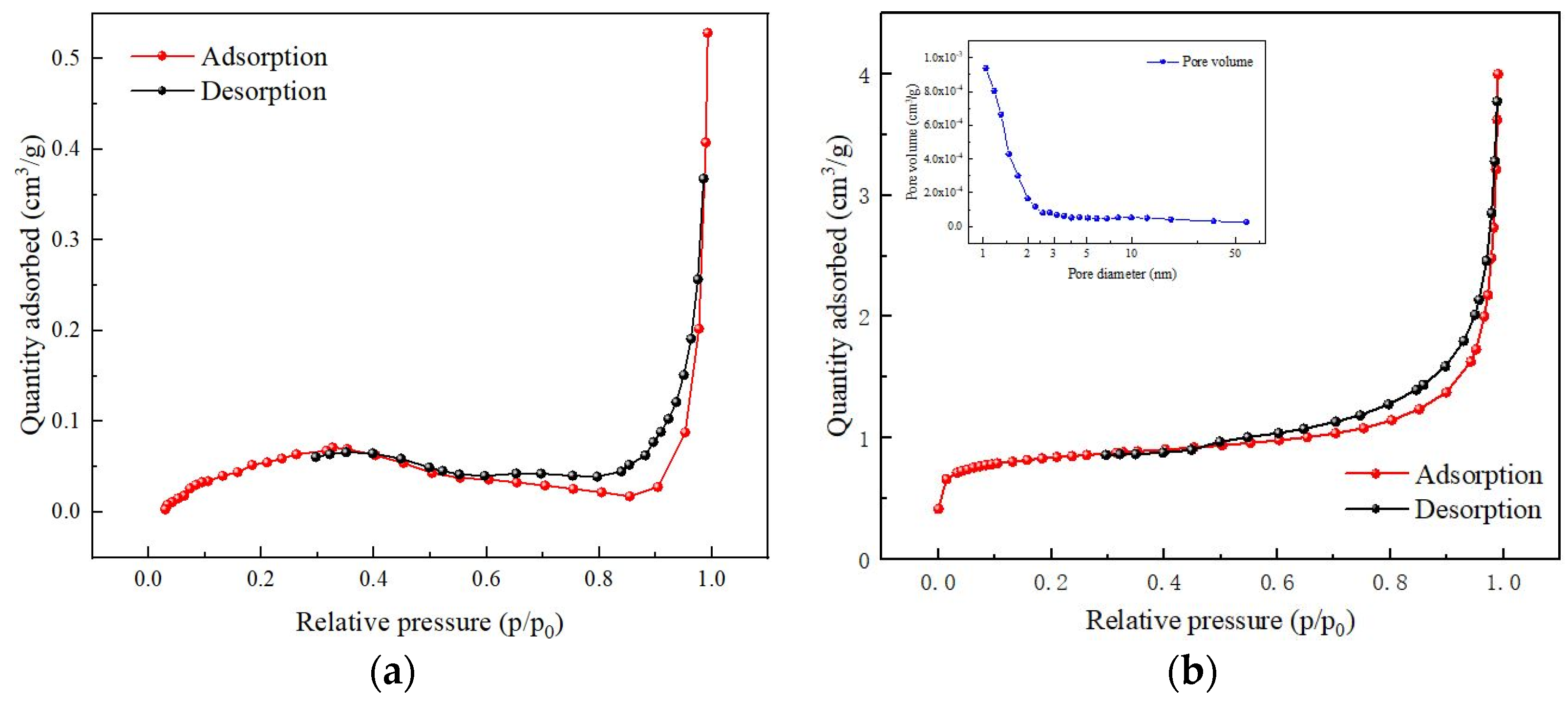
2.1.1. Aggregate
2.1.2. Ultra-High Molecular Weight Polyethylene Fiber
2.1.3. Concrete Matrix Material
2.2. Experimental Methods
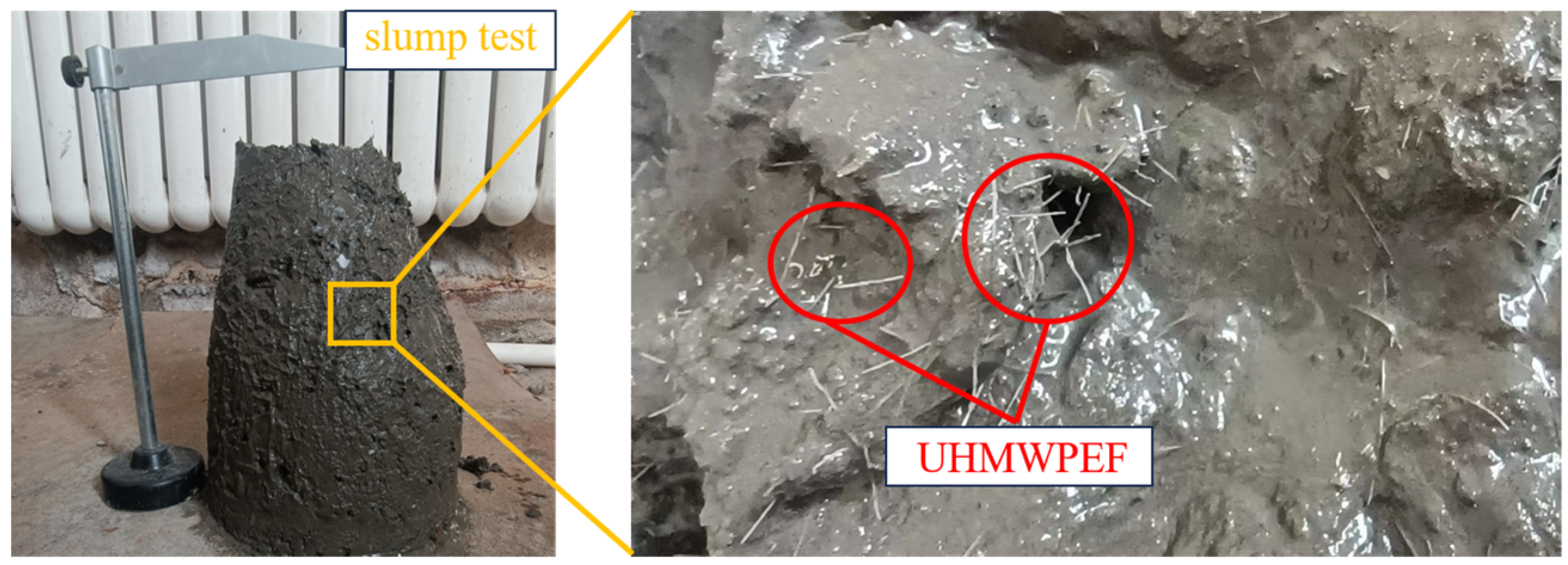
2.2.1. Slump Test
2.2.2. Shrinkage Test
2.2.3. Flexural Strength Test
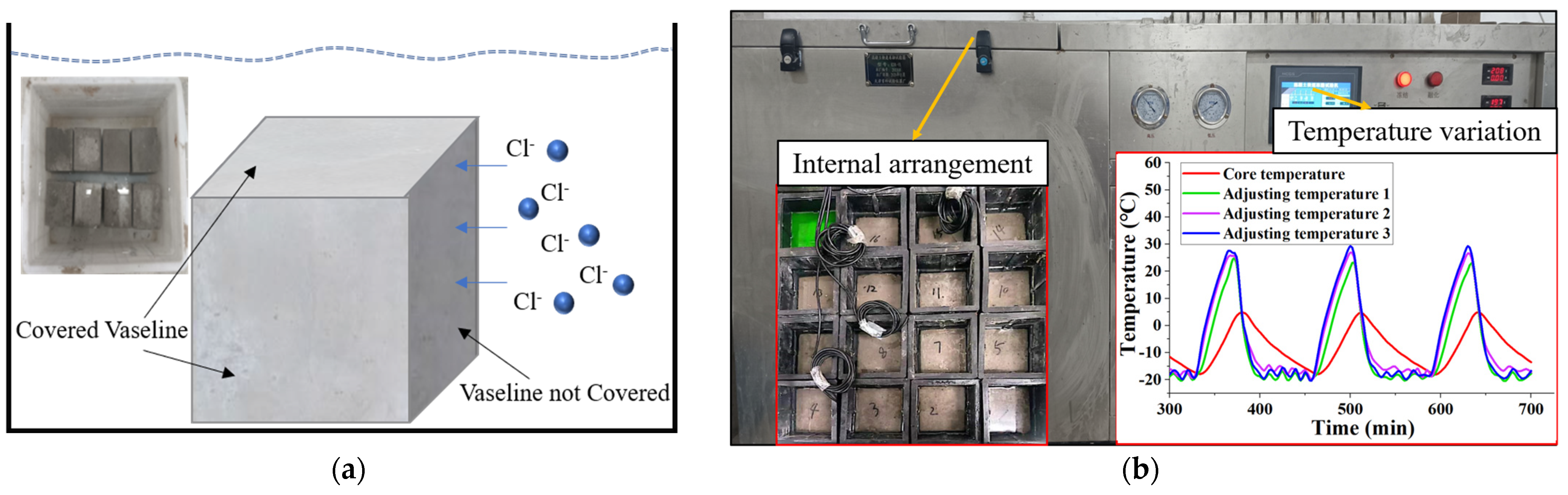
2.2.4. Chloride Ion Penetration Test
2.2.5. Freeze–Thaw Cycling Test
2.3. Experimental Mix Proportions
3. Results and Discussion
3.1. Slump
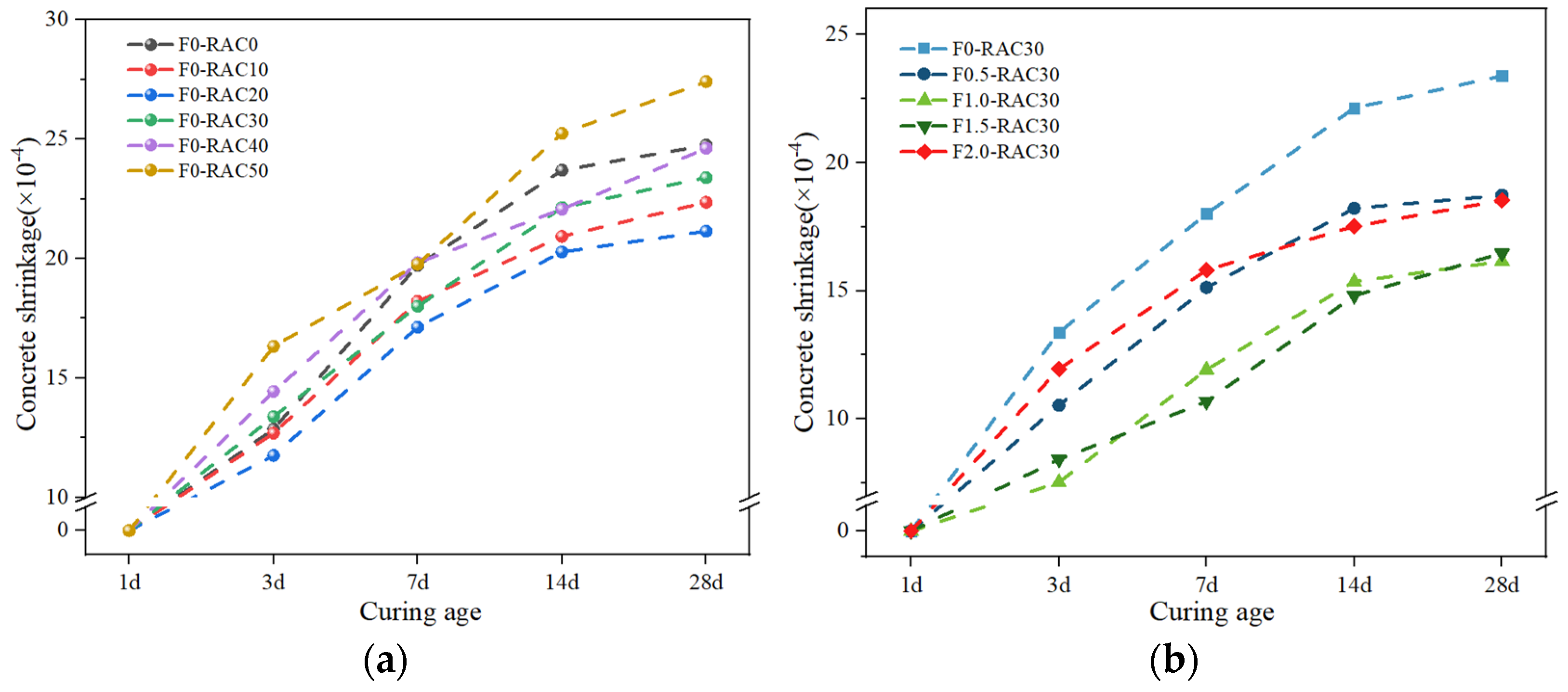
3.2. Shrinkage
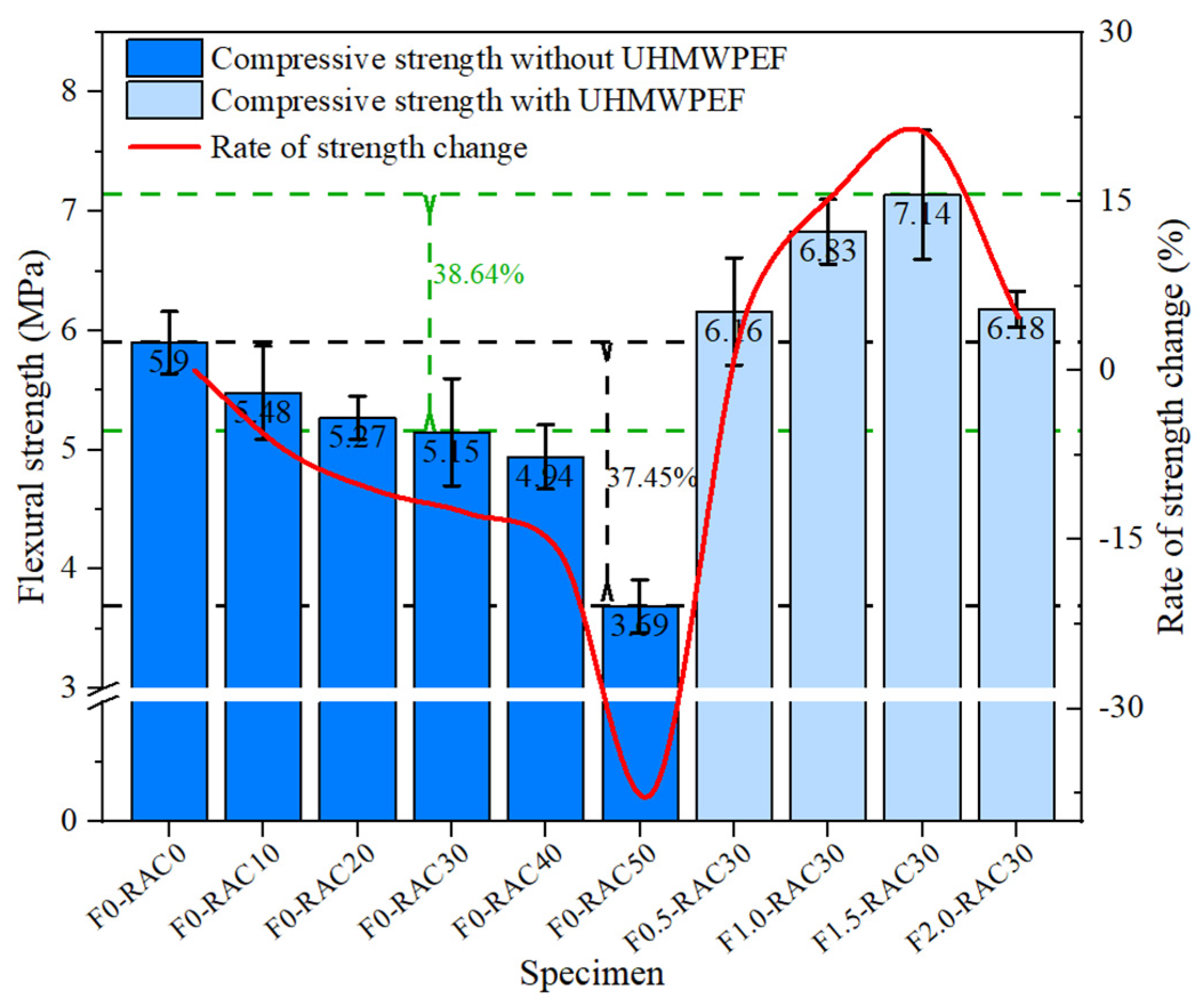
3.3. Flexural Strength
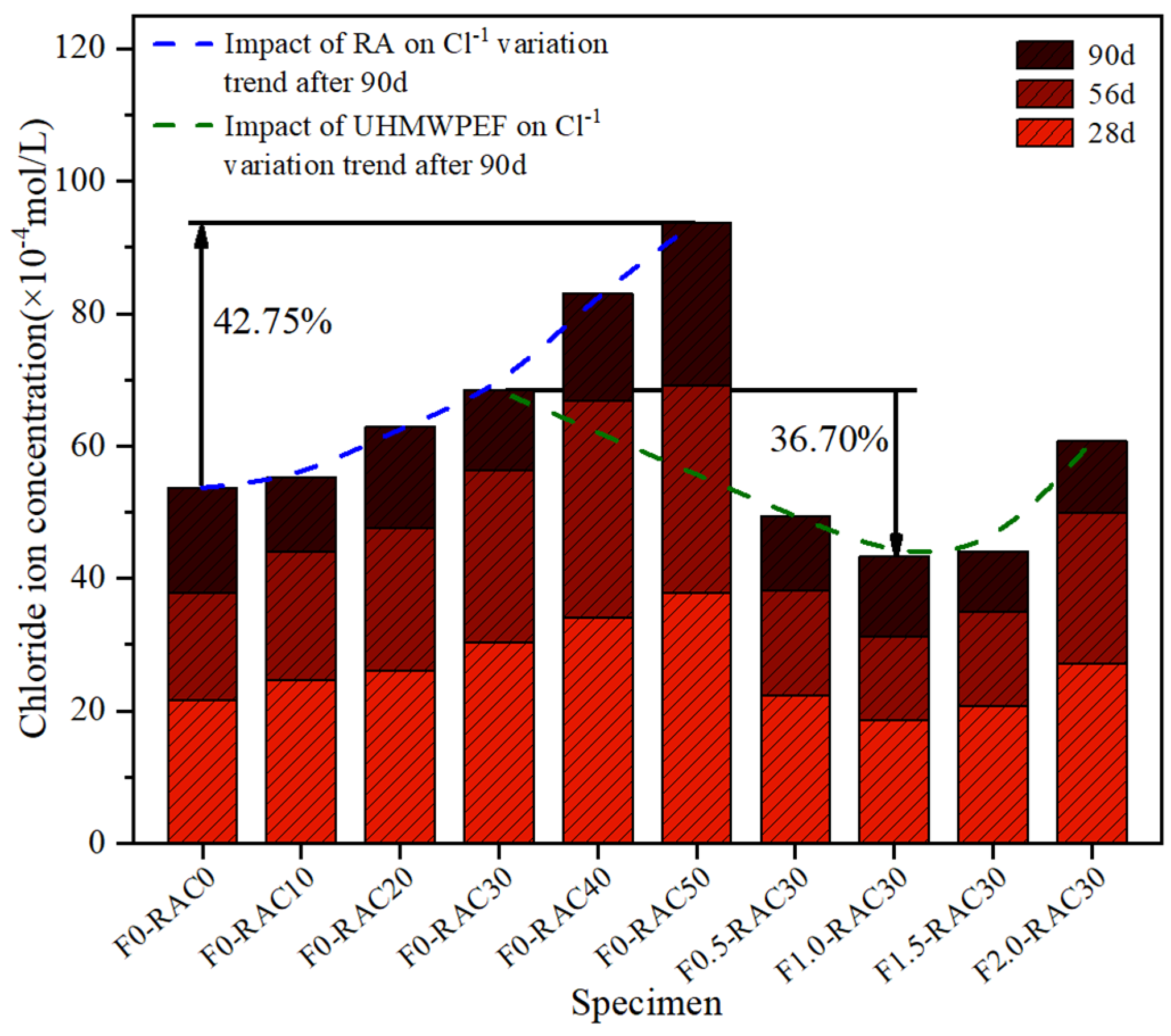
3.4. Resistance to Chloride Ion Erosion
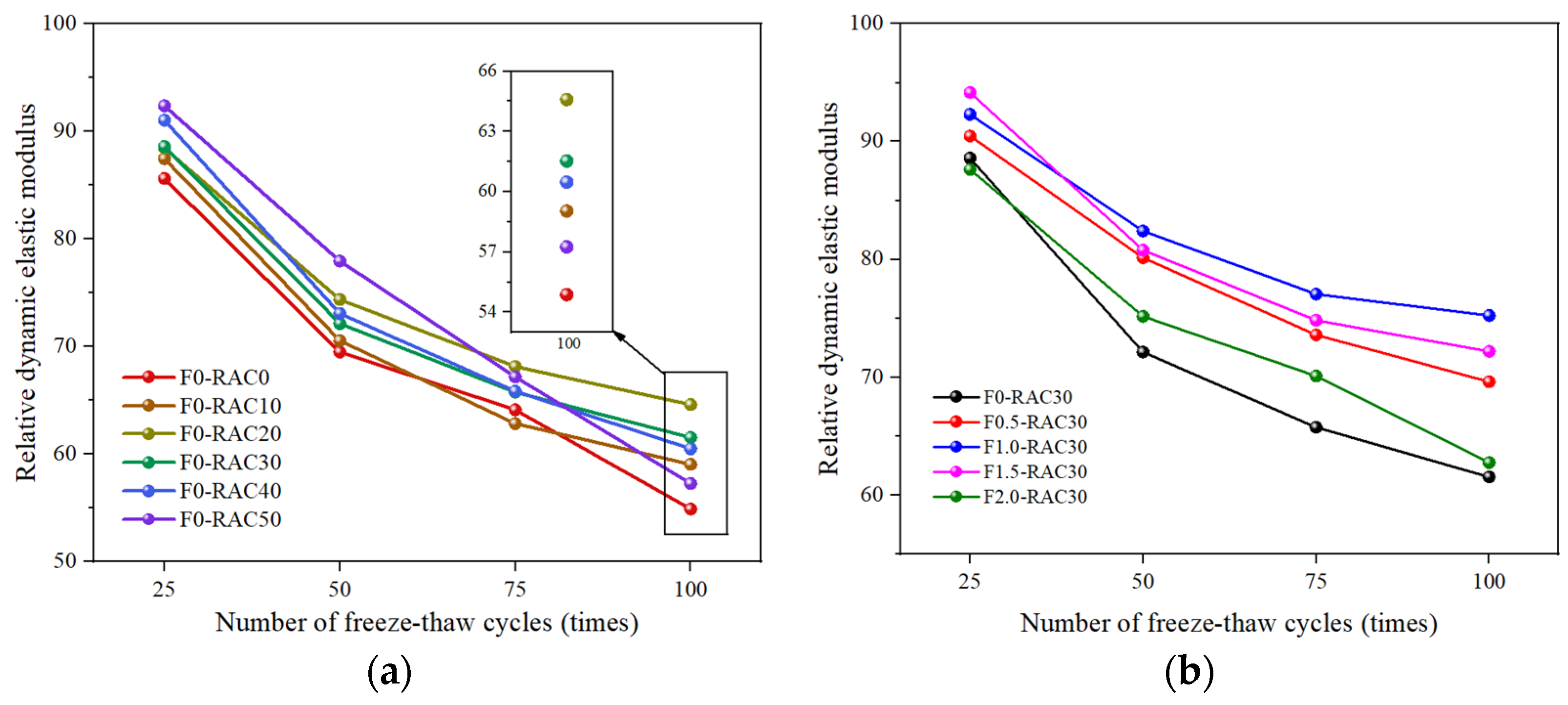
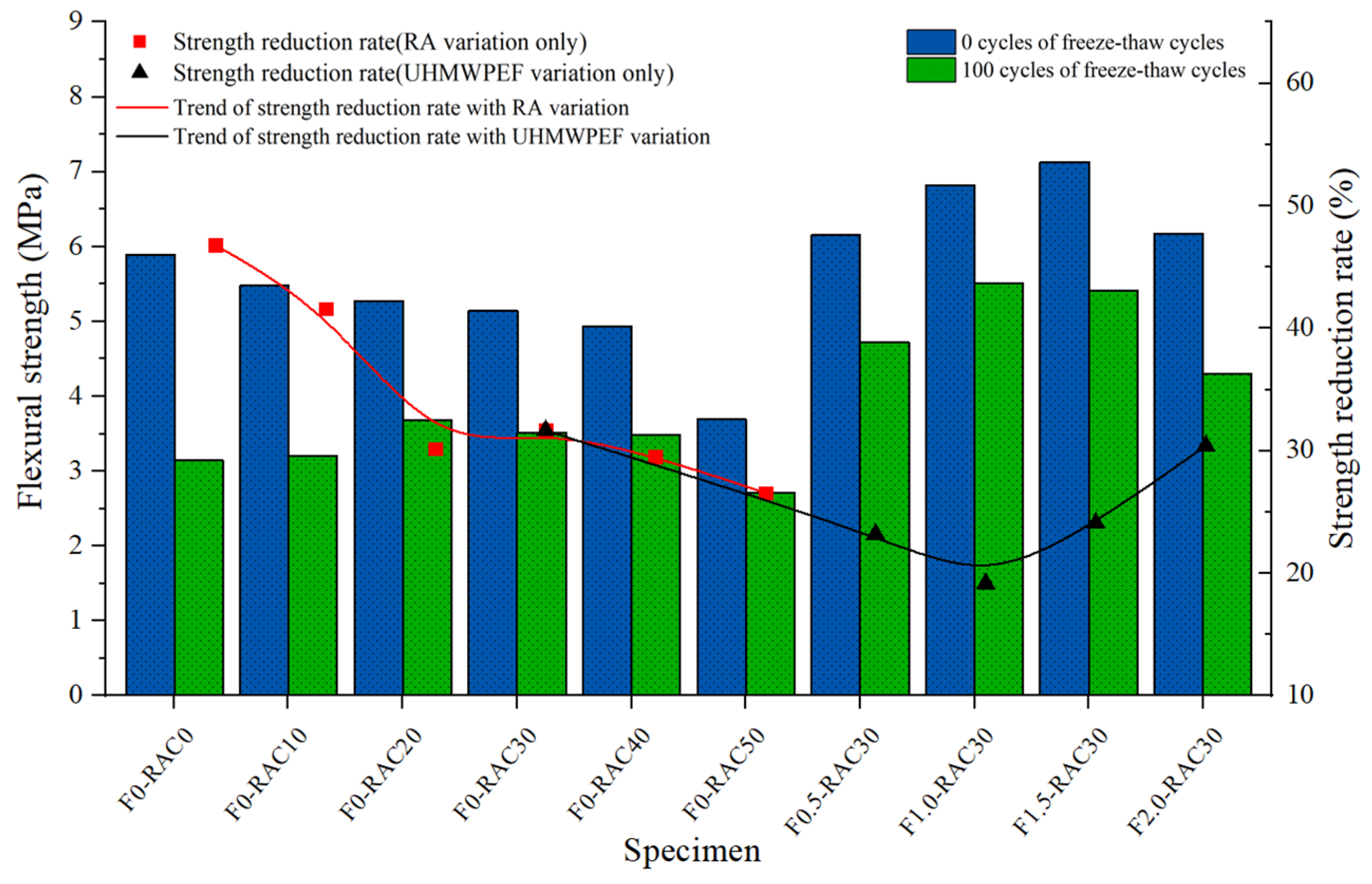
3.5. Freeze–Thaw Cycling
4. Response-Surface Optimization
4.1. Response-Surface Design
4.2. Variance Analysis of the Regression Model
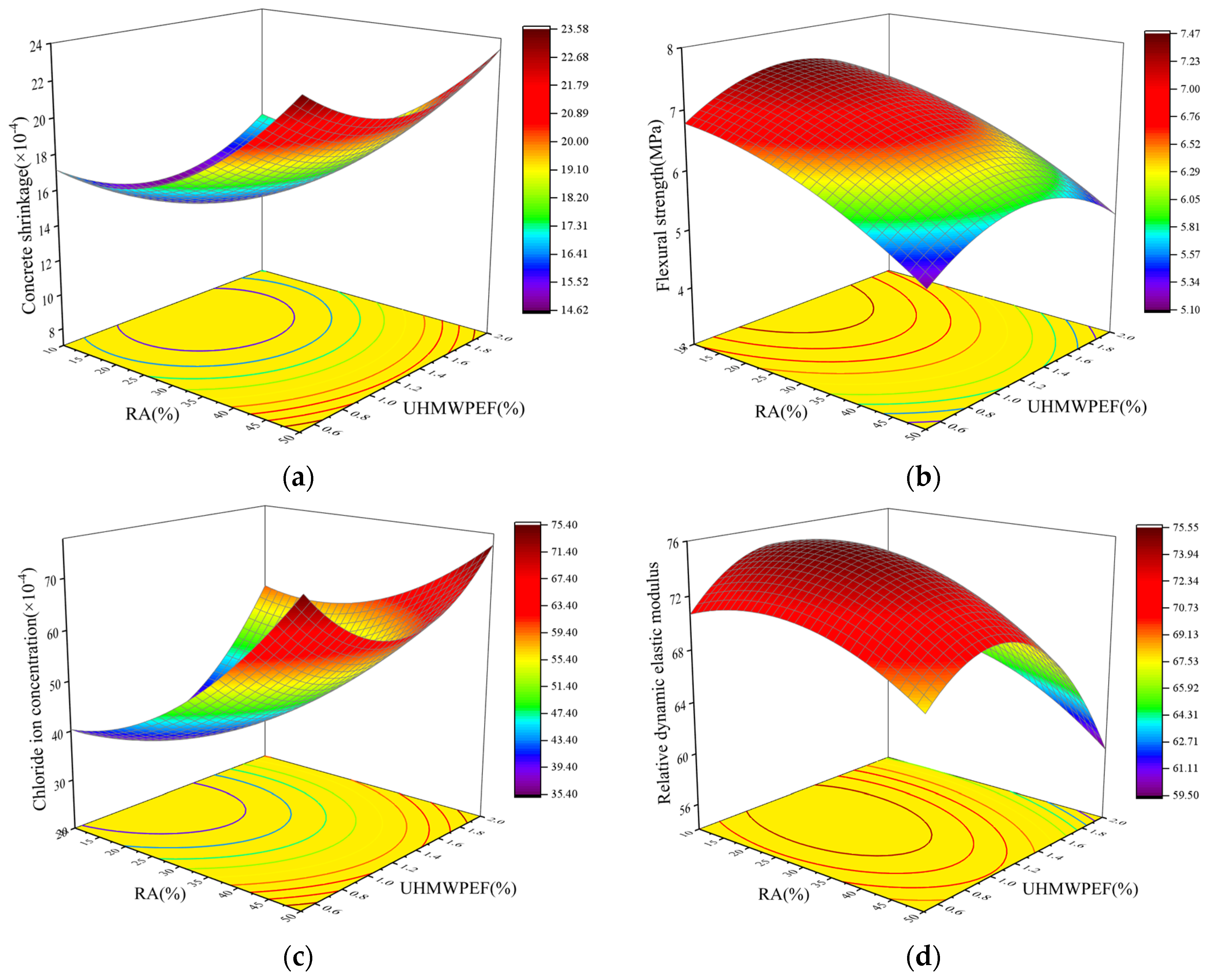
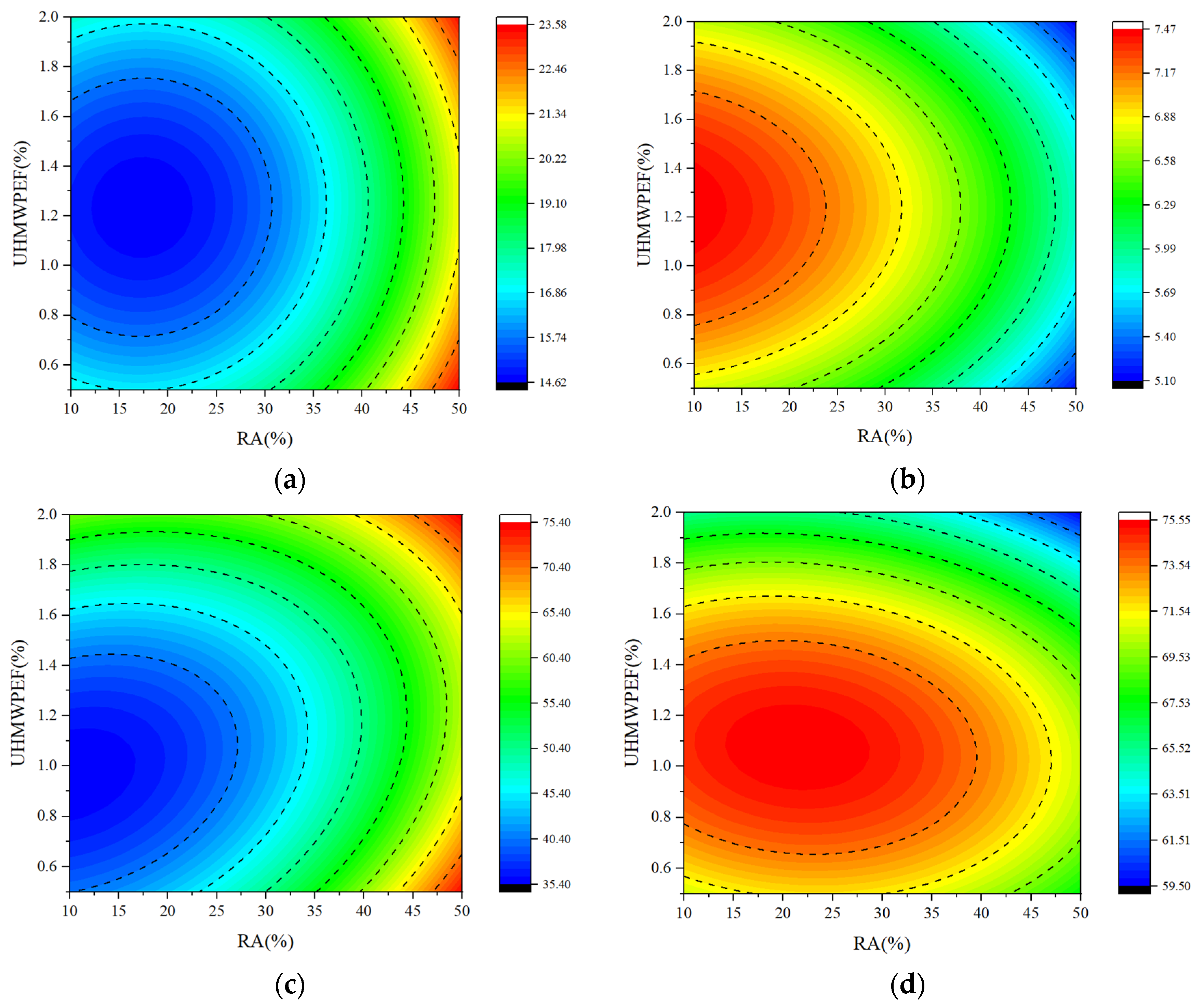
4.3. Response-Surface and Contour Analysis
4.4. Multi-Objective Optimization
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, J.; Liu, Z.; Liu, H. Experimental study on bond strength of fiber recycled concrete and BFRP bar under freeze-thaw recycles. Bull. Chin. Ceram. Soc. 2018, 37, 3355–3360. [Google Scholar]
- Cachim, P.B. Mechanical properties of brick aggregate concrete. Constr. Build. Mater. 2009, 23, 1292–1297. [Google Scholar] [CrossRef]
- Meng, T.; Wei, H.; Dai, D.; Liao, J.; Ahmed, S. Effect of brick aggregate on failure process of mixed recycled aggregate concrete via X-CT. Constr. Build. Mater. 2022, 327, 126934. [Google Scholar] [CrossRef]
- Nguyen, M.H.; Trinh, S.H.; Ly, H.B. Toward improved prediction of recycled brick aggregate concrete compressive strength by designing ensemble machine learning models. Constr. Build. Mater. 2023, 369, 130613. [Google Scholar]
- Rashid, M.A.; Hossain, T.; Islam, M.A. Properties of higher strength concrete made with crushed brick as coarse aggregate. J. Civ. Eng. (IEB) 2009, 37, 43–52. [Google Scholar]
- Akhtaruzzaman, A.A.; Hasnat, A. Properties of concrete using crushed brick as aggregate. Concr. Int. 1983, 5, 58–63. [Google Scholar]
- Debieb, F.; Kenai, S. The use of coarse and fine crushed bricks as aggregate in concrete. Constr. Build. Mater. 2008, 22, 886–893. [Google Scholar] [CrossRef]
- Mohammed, T.U.; Hasnat, A.; Awal, M.A.; Bosunia, S.Z. Recycling of brick aggregate concrete as coarse aggregate. J. Mater. Civ. Eng. 2015, 27, B4014005. [Google Scholar] [CrossRef]
- Islam, M.S.; Siddique, M.A.A. Behavior of low grade steel fiber reinforced concrete made with fresh and recycled brick aggregates. Adv. Civ. Eng. 2017, 2017, 1812363. [Google Scholar] [CrossRef]
- Zhang, W.; Zheng, M.; Zhu, L.; Ren, Y.; Lv, Y. Investigation of steel fiber reinforced high-performance concrete with full aeolian sand: Mix design, characteristics and microstructure. Constr. Build. Mater. 2022, 342, 128065. [Google Scholar] [CrossRef]
- Elshazli, M.T.; Ramirez, K.; Ibrahim, A.; Badran, M. Mechanical, durability and corrosion properties of basalt fiber concrete. Fibers 2022, 10, 10. [Google Scholar] [CrossRef]
- Małek, M.; Jackowski, M.; Łasica, W.; Kadela, M. Characteristics of recycled polypropylene fibers as an addition to concrete fabrication based on portland cement. Materials 2020, 13, 1827. [Google Scholar] [CrossRef] [PubMed]
- Tomlal, E.J.; Thomas, P.C.; George, K.C.; Jayanarayanan, K.; Joseph, K. Impact, tear, and dielectric properties of cotton/polypropylene commingled composites. J. Reinf. Plast. Compos. 2010, 29, 1861–1874. [Google Scholar] [CrossRef]
- Cho, D.; Nnadi, O.; Netravali, A.; Joo, Y.L. Electrospun hybrid soy protein/PVA fibers. Macromol. Mater. Eng. 2010, 295, 763–773. [Google Scholar] [CrossRef]
- Kurtz, S.M.; Muratoglu, O.K.; Evans, M.; Edidin, A.A. Advances in the processing, sterilization, and crosslinking of ultra-high molecular weight polyethylene for total joint arthroplasty. Biomaterials 1999, 20, 1659–1688. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Li, M.; Fan, C.; Ren, M.; Wu, P.; Luo, L.; Liu, X. The wear-resistance of composite depending on the interfacial interaction between thermoplastic polyurethane and fluorinated UHMWPE particles with or without oxygen. Compos. Sci. Technol. 2015, 106, 68–75. [Google Scholar] [CrossRef]
- Osman, B.H.; Sun, X.; Tian, Z.; Lu, H.; Jiang, G. Dynamic compressive and tensile characteristics of a new type of ultra-high-molecular weight polyethylene (UHMWPE) and polyvinyl alcohol (PVA) fibers reinforced concrete. Shock Vib. 2019, 2019, 6382934. [Google Scholar] [CrossRef]
- Gong, T.; Curosu, I.; Liebold, F.; Vo, D.M.; Zierold, K.; Maas, H.G.; Mechtcherine, V. Tensile behavior of high-strength, strain-hardening cement-based composites (HS-SHCC) reinforced with continuous textile made of ultra-high-molecular-weight polyethylene. Materials 2020, 13, 5628. [Google Scholar] [CrossRef]
- Li, J.; Wu, C.; Liu, Z.X. Comparative evaluation of steel wire mesh, steel fibre and high performance polyethylene fibre reinforced concrete slabs in blast tests. Thin-Walled Struct. 2018, 126, 117–126. [Google Scholar] [CrossRef]
- Zhang, Y.W.; Yan, L.H.; Zhu, L.; Zhang, S.; Li, L.F. Experiment research on mechanical properties and penetration performance of UHMWPE fiber concrete. Adv. Mater. Res. 2014, 989, 961–965. [Google Scholar] [CrossRef]
- Game, D.; Giwa, I.; Ahmed, H.; Noorvand, H.; Arce, G.; Hassan, M.M.; Kazemian, A. Development of Ultra-High-Performance Engineered Cementitious Composites for 3D Printing Applications. In Tran-SET 2022; American Society of Civil Engineers: Reston, VA, USA, 2022; pp. 35–44. [Google Scholar]
- Adesina, A.Y.; Khan, M.F.; Azam, M.U.; Abdul Samad, M.; Sorour, A.A. Characterization and corrosion resistance of ultra-high molecular weight polyethylene composite coatings reinforced with tungsten carbide particles in hydrochloric acid medium. J. Polym. Eng. 2019, 39, 861–873. [Google Scholar] [CrossRef]
- GB/T 14685-2011; Pebbie and Crushed Stone for Building. China Standard Press: Beijing, China, 2011.
- John, V.J.; Dharmar, B. Influence of basalt fibers on the mechanical behavior of concrete—A review. Struct. Concr. 2021, 22, 491–502. [Google Scholar] [CrossRef]
- Yan, H.; Gao, D.; Guo, A.; Gu, Z.; Ji, D.; Zhang, Y. Monotonic and cyclic bond responses of steel bar with steel-polypropylene hybrid fiber reinforced recycled aggregate concrete. Constr. Build. Mater. 2022, 327, 127031. [Google Scholar] [CrossRef]
- Ramujee, K. Strength properties of polypropylene fiber reinforced concrete. Int. J. Innov. Res. Sci. Eng. Technol. 2013, 2, 3409–3413. [Google Scholar]
- Patel, K.; Chikkali, S.H.; Sivaram, S. Ultrahigh molecular weight polyethylene: Catalysis, structure, properties, processing and applications. Prog. Polym. Sci. 2022, 109, 101290. [Google Scholar] [CrossRef]
- JGJ 52-2006; Standard for Technical Requirements and Test Method of Sand and Crushed Stone (or Gravel) for Ordinary Concrete. China Building Materials Industry Press: Beijing, China, 2006.
- GB/T 50080-2002; Standard for Test Method of Performance on Ordinary Fresh Concrete. China Construction Industry Press: Beijing, China, 2002.
- GB/T 50082-2009; Standard for Test Methods of Long-Term Performance and Durability of Ordinary Concrete. China Construction Industry Press: Beijing, China, 2009.
- GB/T 50081-2019; Standard for Test Methods of Concrete Physical and Mechanical Properties. China Construction Industry Press: Beijing, China, 2019.
- Ma, Z.; Liu, M.; Tang, Q.; Liang, C.; Duan, Z. Chloride permeability of recycled aggregate concrete under the coupling effect of freezing-thawing, elevated temperature or mechanical damage. Constr. Build. Mater. 2020, 237, 117648. [Google Scholar] [CrossRef]
- JGJ/T 322-2013; Technical Specification for Test of Chloride Ion Content in Concrete. China Construction Industry Press: Beijing, China, 2013.
- Zhu, C.; Liu, X.; Liu, C.; Yu, W.; Bai, G. Study on the chloride ion transport mechanism of recycled mixed aggregate concrete based on evolution characteristics of pore structure. Constr. Build. Mater. 2022, 353, 129101. [Google Scholar] [CrossRef]
- Hachemi, S.; Khattab, M.; Benzetta, H. The effects of recycled brick and water/cement ratios on the physical and mechanical performance of recycled aggregates concrete. Innov. Infrastruct. Solut. 2022, 7, 270. [Google Scholar] [CrossRef]
- Brooshan, E.; Kauppila, T.; Szlachta, M.; Jooshaki, M.; Leveinen, J. Utilizing Recycled concrete aggregate for treating Acid mine drainage. Clean. Mater. 2023, 9, 100205. [Google Scholar] [CrossRef]
- Seifi, S.; Levacher, D.; Razakamanantsoa, A.; Sebaibi, N. Microstructure of Dry Mortars without Cement: Specific Surface Area, Pore Size and Volume Distribution Analysis. Appl. Sci. 2023, 13, 5616. [Google Scholar] [CrossRef]
- Dang, J.; Zhao, J. Influence of waste clay bricks as fine aggregate on the mechanical and microstructural properties of concrete. Constr. Build. Mater. 2019, 228, 116757. [Google Scholar] [CrossRef]
- Song, Q.; Yu, R.; Shui, Z.; Chen, L.; Liu, Z.; Gao, X.; He, Y. Intrinsic effect of hybrid fibres 3D network on the electrochemical characteristics of Ultra-High Performance Fibre Reinforced Composites (UHPFRC). Cem. Concr. Compos. 2020, 114, 103818. [Google Scholar] [CrossRef]
- Liu, J.; Tian, Q.; Wang, Y.; Li, H.; Xu, W. Evaluation method and mitigation strategies for shrinkage cracking of modern concrete. Engineering 2021, 7, 348–357. [Google Scholar] [CrossRef]
- Boshoff, W.P.; Combrinck, R. Modelling the severity of plastic shrinkage cracking in concrete. Cem. Concr. Res. 2013, 48, 34–39. [Google Scholar] [CrossRef]
- Slowik, V.; Schmidt, M.; Fritzsch, R. Capillary pressure in fresh cement-based materials and identification of the air entry value. Cem. Concr. Compos. 2008, 30, 557–565. [Google Scholar] [CrossRef]
- Lura, P.; Pease, B.; Mazzotta, G.B.; Rajabipour, F.; Weiss, J. Influence of shrinkage-reducing admixtures on development of plastic shrinkage cracks. ACI Mater. J. 2007, 104, 187–194. [Google Scholar]
- Du, X.; Li, Z.; Han, J.; Tan, C. Effect of different humidity-controlling modes on microstructure and compressive behavior of ordinary concrete. J. Mater. Civ. Eng. 2020, 32, 04019337. [Google Scholar] [CrossRef]
- Fonseca, P.C.; Jennings, H.M. The effect of drying on early-age morphology of C–S–H as observed in environmental SEM. Cem. Concr. Res. 2010, 40, 1673–1680. [Google Scholar] [CrossRef]
- Bielak, J.; Schöneberg, J.; Classen, M.; Hegger, J. Shear capacity of continuous concrete slabs with CFRP reinforcement. Constr. Build. Mater. 2022, 320, 126117. [Google Scholar] [CrossRef]
- Li, Z.; Lara, M.A.P.; Bolander, J.E. Restraining effects of fibers during non-uniform drying of cement composites. Cem. Concr. Res. 2006, 36, 1643–1652. [Google Scholar] [CrossRef]
- Wei, J.; Meyer, C. Degradation mechanisms of natural fiber in the matrix of cement composites. Cem. Concr. Res. 2015, 73, 1–16. [Google Scholar] [CrossRef]
- Gao, S.; Guo, J.; Gong, Y.; Ban, S.; Liu, A. Study on the penetration and diffusion of chloride ions in interface transition zone of recycled concrete prepared by modified recycled coarse aggregates. Case Stud. Constr. Mater. 2022, 16, e01034. [Google Scholar] [CrossRef]
- Xiao, Q.; Liu, X.; Qiu, J.; Li, Y. Capillary water absorption characteristics of recycled concrete in Freeze-Thaw environment. Adv. Mater. Sci. Eng. 2020, 2020, 1620914. [Google Scholar] [CrossRef]
- Wei, Z.; Yang, W.; Zhai, C.; Sun, Y.; Tang, W.; Chen, A.; Wang, Y. Freezing characteristics and microstructural damage evolution of granular materials in cold regions under freezing–thawing cycles. Environ. Earth Sci. 2023, 82, 191. [Google Scholar] [CrossRef]
- Xia, P.; Yang, L.; Wang, S.; Gong, F.; Cao, W.; Zhao, Y. Improved freeze-thaw modification of recycled concrete aggregate originally from frost resistive concrete. Cem. Concr. Compos. 2023, 144, 105302. [Google Scholar] [CrossRef]
- Gong, F.; Wang, Y.; Zhang, D.; Ueda, T. Mesoscale simulation of deformation for mortar and concrete under cyclic freezing and thawing stress. J. Adv. Concr. Technol. 2015, 13, 291–304. [Google Scholar] [CrossRef]
- Xu, Y.; Yuan, Q.; Dai, X.; Xiang, G. Improving the freeze-thaw resistance of mortar by a combined use of superabsorbent polymer and air entraining agent. J. Build. Eng. 2022, 52, 104471. [Google Scholar] [CrossRef]
- Tian, Z.; Abou-Chakra, A.; Geoffroy, S.; Kondo, D. The effective elastic properties and thermal conductivity of porous fired clay bricks. Eur. J. Environ. Civ. Eng. 2022, 26, 547–569. [Google Scholar] [CrossRef]
- Sweety Poornima Rau, M.; Manjunath, Y.M. An experimental study on mechanical behaviour of concrete by partial replacement of sand with brick fines. Aust. J. Struct. Eng. 2023, 1–18. [Google Scholar] [CrossRef]
- Allaix, D.L.; Carbone, V.I. An improvement of the response surface method. Struct. Saf. 2011, 33, 165–172. [Google Scholar] [CrossRef]
- Tanyildizi, H. Variance analysis of crack characteristics of structural lightweight concrete containing silica fume exposed to high temperature. Constr. Build. Mater. 2013, 47, 1154–1159. [Google Scholar] [CrossRef]
- Atahan, H.N.; Oktar, O.N.; Taşdemir, M.A. Factors determining the correlations between high strength concrete properties. Constr. Build. Mater. 2011, 25, 2214–2222. [Google Scholar] [CrossRef]
- Bektas, F.; Bektas, B.A. Analyzing mix parameters in ASR concrete using response surface methodology. Constr. Build. Mater. 2014, 66, 299–305. [Google Scholar] [CrossRef]
- Shahmansouri, A.A.; Nematzadeh, M.; Behnood, A. Mechanical properties of GGBFS-based geopolymer concrete incorporating natural zeolite and silica fume with an optimum design using response surface method. J. Build. Eng. 2021, 36, 102138. [Google Scholar] [CrossRef]
- Nafees, A.; Amin, M.N.; Khan, K.; Nazir, K.; Ali, M.; Javed, M.F.; Vatin, N.I. Modeling of mechanical properties of silica fume-based green concrete using machine learning techniques. Polymers 2021, 14, 30. [Google Scholar] [CrossRef] [PubMed]








| Chemical Compositions | SiO2 (%) | Al2O3 (%) | Fe2O3 (%) | K2O (%) | MgO (%) | Others (%) |
|---|---|---|---|---|---|---|
| Content | 62.72 | 19.83 | 6.62 | 2.49 | 1.95 | 6.39 |
| Aggregate Types | Apparent Density/Kg·m−3 | Bulk Density/Kg·m−3 | Water Absorption/% | Crush Value/% |
|---|---|---|---|---|
| Recycled-brick aggregate | 2130 | 1206 | 18.3 | 24.3 |
| Natural aggregate | 2794 | 1542 | 1.2 | 4.8 |
| Fiber | Appearance | Density /(g/cm3) | Diameter /μm | Tensile Strength /MPa | Elastic Modulus /GPa | Elongation /% |
|---|---|---|---|---|---|---|
| UHMWPEF |  | 0.97 | 40 | 2000–3400 | 100 | 2.8 |
| Polypropylene fiber |  | 0.91 | 100 | 300–700 | 5.8 | 19.9 |
| Basalt fiber |  | 2.6 | 17 | 2000 | 85 | 3.1 |
| Steel fiber |  | 7.8 | 300–800 | 1270 | 200 | 3.4–4 |
| Test Items | Fineness/% | Setting Time/(min) | Compressive Strength/(MPa) | Flexural Strength/(MPa) | |||
|---|---|---|---|---|---|---|---|
| Initial Setting Time | Final Setting Time | 3 Day | 28 Day | 3 Day | 28 Day | ||
| Standard value | ≤10.0 | ≥45 | ≤600 | ≥17 | ≥42.5 | ≥3.5 | ≥6.5 |
| Detection value | 3.9 | 155 | 370 | 28.5 | 51.4 | 5.1 | 8.1 |
| Label | Cement | 5–10 mmNA | 10–20 mmNA | 5–10 mmRA | 10–20 mmRA | Sand | Water | Fiber |
|---|---|---|---|---|---|---|---|---|
| F0-RAC0 | 487 | 341.4 | 796.6 | 0.0 | 0.0 | 613 | 233 | 0 |
| F0-RAC10 | 487 | 307.3 | 716.9 | 26.0 | 60.7 | 613 | 233 | 0 |
| F0-RAC20 | 487 | 273.1 | 637.3 | 52.1 | 121.5 | 613 | 233 | 0 |
| F0-RAC30 | 487 | 239.0 | 557.6 | 78.1 | 182.2 | 613 | 233 | 0 |
| F0-RAC40 | 487 | 204.8 | 478.0 | 104.1 | 242.9 | 613 | 233 | 0 |
| F0-RAC50 | 487 | 170.7 | 398.3 | 130.1 | 303.6 | 613 | 233 | 0 |
| F0.5-RAC30 | 487 | 239.0 | 557.6 | 78.1 | 182.2 | 613 | 233 | 4.9 |
| F1-RAC30 | 487 | 239.0 | 557.6 | 78.1 | 182.2 | 613 | 233 | 9.7 |
| F1.5-RAC30 | 487 | 239.0 | 557.6 | 78.1 | 182.2 | 613 | 233 | 14.6 |
| F2-RAC30 | 487 | 239.0 | 557.6 | 78.1 | 182.2 | 613 | 233 | 19.4 |
| Serial Number | RA (%) | UHMWPEF (%) | Shrinkage (×10−4) | Flexural Strength (MPa) | Chloride Ion Concentration (×10−4 mol/L) | Relative Dynamic Elastic Modulus |
|---|---|---|---|---|---|---|
| 1 | 10 | 0.5 | 17.19 | 6.74 | 42 | 70.34 |
| 2 | 50 | 0.5 | 24.51 | 5.23 | 77 | 66.25 |
| 3 | 10 | 2 | 17.82 | 6.45 | 65 | 63.61 |
| 4 | 50 | 2 | 24.75 | 4.95 | 81 | 56.69 |
| 5 | 30 | 1.25 | 15.84 | 7.02 | 43 | 75.66 |
| 6 | 30 | 1.25 | 15.48 | 6.93 | 41 | 71.06 |
| 7 | 30 | 1.25 | 15.57 | 6.85 | 47 | 74.05 |
| 8 | 1.72 | 1.25 | 16.05 | 7.69 | 36 | 73.88 |
| 9 | 58.28 | 1.25 | 23.61 | 5.22 | 71 | 68.77 |
| 10 | 30 | 0.19 | 19.74 | 5.48 | 60 | 66.97 |
| 11 | 30 | 2.31 | 19.26 | 5.76 | 69 | 59.94 |
| 12 | 30 | 1.25 | 15.75 | 7.11 | 40 | 74.73 |
| 13 | 30 | 1.25 | 15.39 | 6.95 | 39 | 75.14 |
| 14 | 30 | 1.25 | 15.66 | 6.84 | 46 | 77.39 |
| Source | F-Value | p-Value | R2 | Pre-R2 | Statistical Significance |
|---|---|---|---|---|---|
| Equation (5) | 50.55 | <0.0001 | 0.9774 | 0.8154 | Significant |
| Equation (6) | 50.70 | <0.0001 | 0.9775 | 0.8159 | Significant |
| Equation (7) | 37.69 | <0.0001 | 0.9700 | 0.8466 | Significant |
| Equation (8) | 25.05 | <0.0001 | 0.9555 | 0.7945 | Significant |
| Target | Factor | Predicted Values | ||||
|---|---|---|---|---|---|---|
| RA (%) | UHMWPEF (%) | Shrinkage (×10−4) | Flexural Strength (MPa) | Chloride Ion Concentration (×10−4 mol/L) | Relative Dynamic Elastic Modulus | |
| Min. S; Max. F. | 12.57 | 1.24 | 14.76 | 7.43 | — | — |
| Min. S and C; Max. F. | 12.00 | 1.15 | 14.82 | 7.43 | 36.24 | — |
| Min. S and C; Max. F and R. | 14.02 | 1.13 | 14.73 | 7.40 | 36.18 | 75.19 |
| Response | Average Actual Value | Fitting Value | Residual | 95% Confidence Interval |
|---|---|---|---|---|
| Shrinkage (×10−4) | 15.38 | 14.73 | 0.65 | (13.974, 15.494) |
| Flexural strength (MPa) | 7.26 | 7.40 | −0.14 | (7.2099, 7.5831) |
| Chloride ion concentration (×10−4 mol/L) | 38.58 | 36.18 | 2.4 | (32.27, 40.10) |
| Relative dynamic elastic modulus | 71.61 | 75.19 | −3.58 | (70.527, 79.848) |
| Item | Three Experimental Values | Variance (S2) | Root Mean Square Error (RMSE) | RMSE/Fitting Value | ||
|---|---|---|---|---|---|---|
| Shrinkage (×10−4) | 14.35 | 15.77 | 16.02 | 0.96 | 0.98 | 0.0665 |
| Flexural strength (MPa) | 7.57 | 6.84 | 7.37 | 0.11 | 0.34 | 0.0459 |
| Chloride ion concentration (×10−4 mol/L) | 40.36 | 36.24 | 39.14 | 8.75 | 2.96 | 0.0818 |
| Relative dynamic elastic modulus | 70.94 | 76.42 | 67.47 | 26.39 | 5.14 | 0.0684 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ji, Y.; Pei, Z. Investigation of Mechanical Properties of Ultra-High-Performance Polyethylene-Fiber-Reinforced Recycled-Brick-Aggregate Concrete. Polymers 2023, 15, 4573. https://doi.org/10.3390/polym15234573
Ji Y, Pei Z. Investigation of Mechanical Properties of Ultra-High-Performance Polyethylene-Fiber-Reinforced Recycled-Brick-Aggregate Concrete. Polymers. 2023; 15(23):4573. https://doi.org/10.3390/polym15234573
Chicago/Turabian StyleJi, Yongcheng, and Zhiyang Pei. 2023. "Investigation of Mechanical Properties of Ultra-High-Performance Polyethylene-Fiber-Reinforced Recycled-Brick-Aggregate Concrete" Polymers 15, no. 23: 4573. https://doi.org/10.3390/polym15234573
APA StyleJi, Y., & Pei, Z. (2023). Investigation of Mechanical Properties of Ultra-High-Performance Polyethylene-Fiber-Reinforced Recycled-Brick-Aggregate Concrete. Polymers, 15(23), 4573. https://doi.org/10.3390/polym15234573






