Petal-like Patterning of Polylactide/Poly (Butylene Succinate) Thin Films Induced by Phase Separation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Fabrication of PLA/PBS Blend
2.3. Fabrication of PLA/PBS Blend Thin Films
2.4. Process and Conditions of Crystallization
2.5. Characterization of PLA/PBS Blend Films
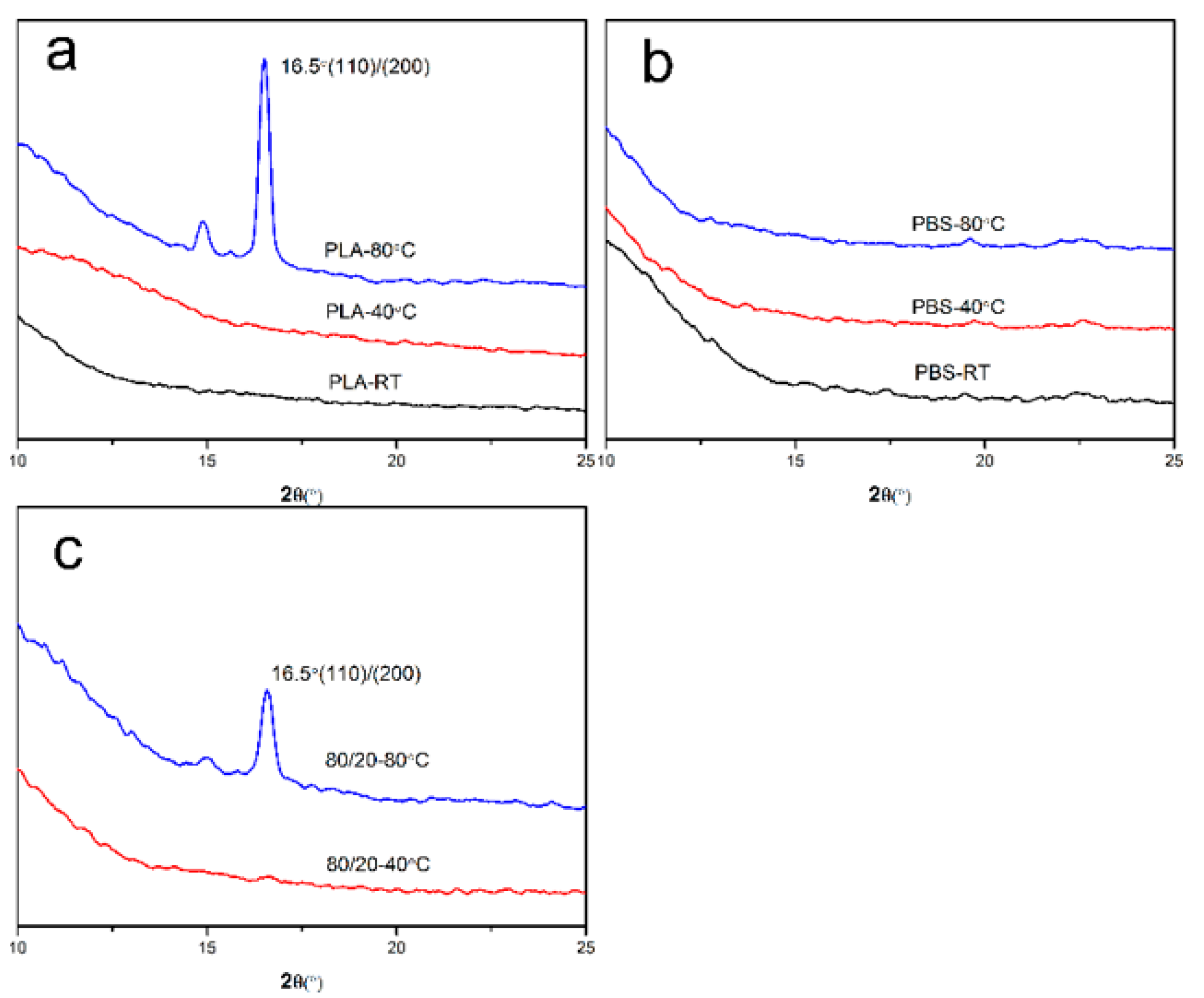
2.5.1. X-ray Diffraction (XRD)
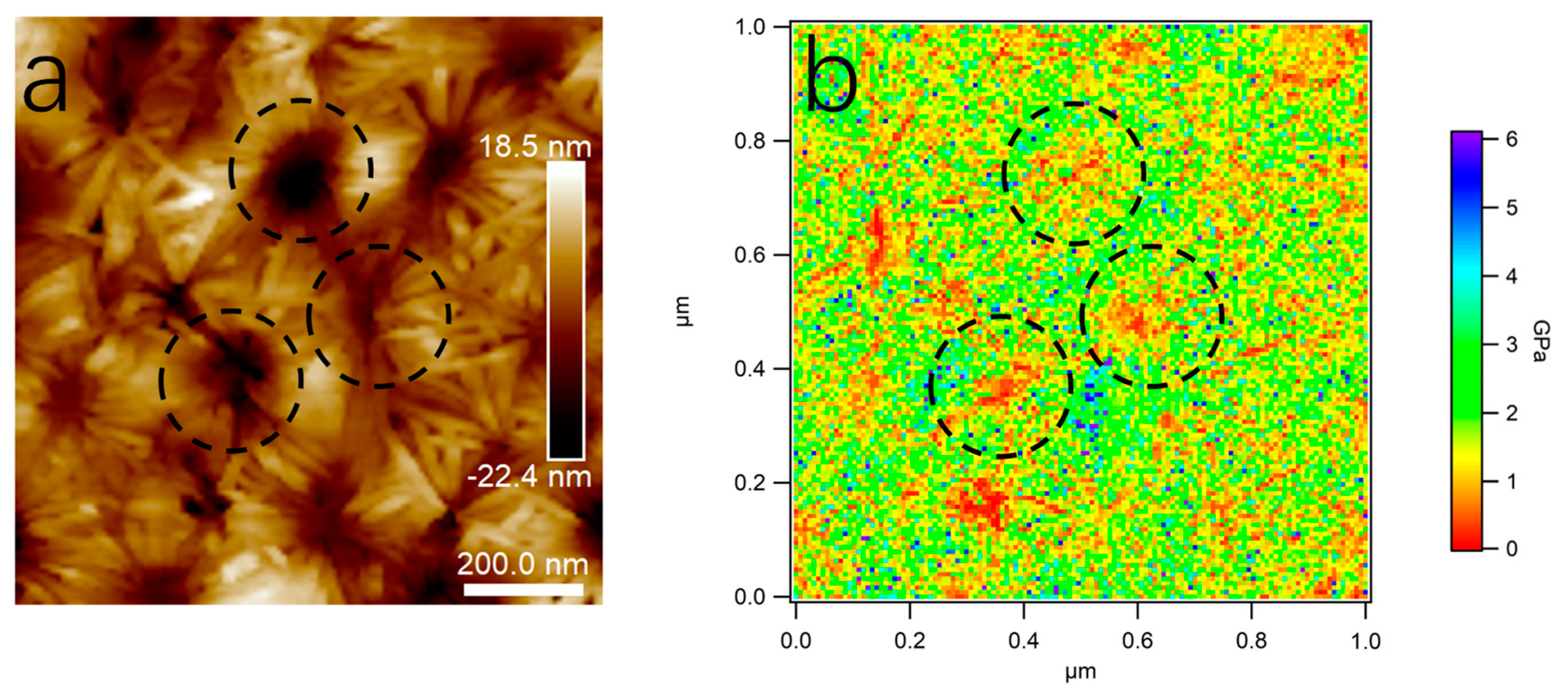
2.5.2. Micromorphology
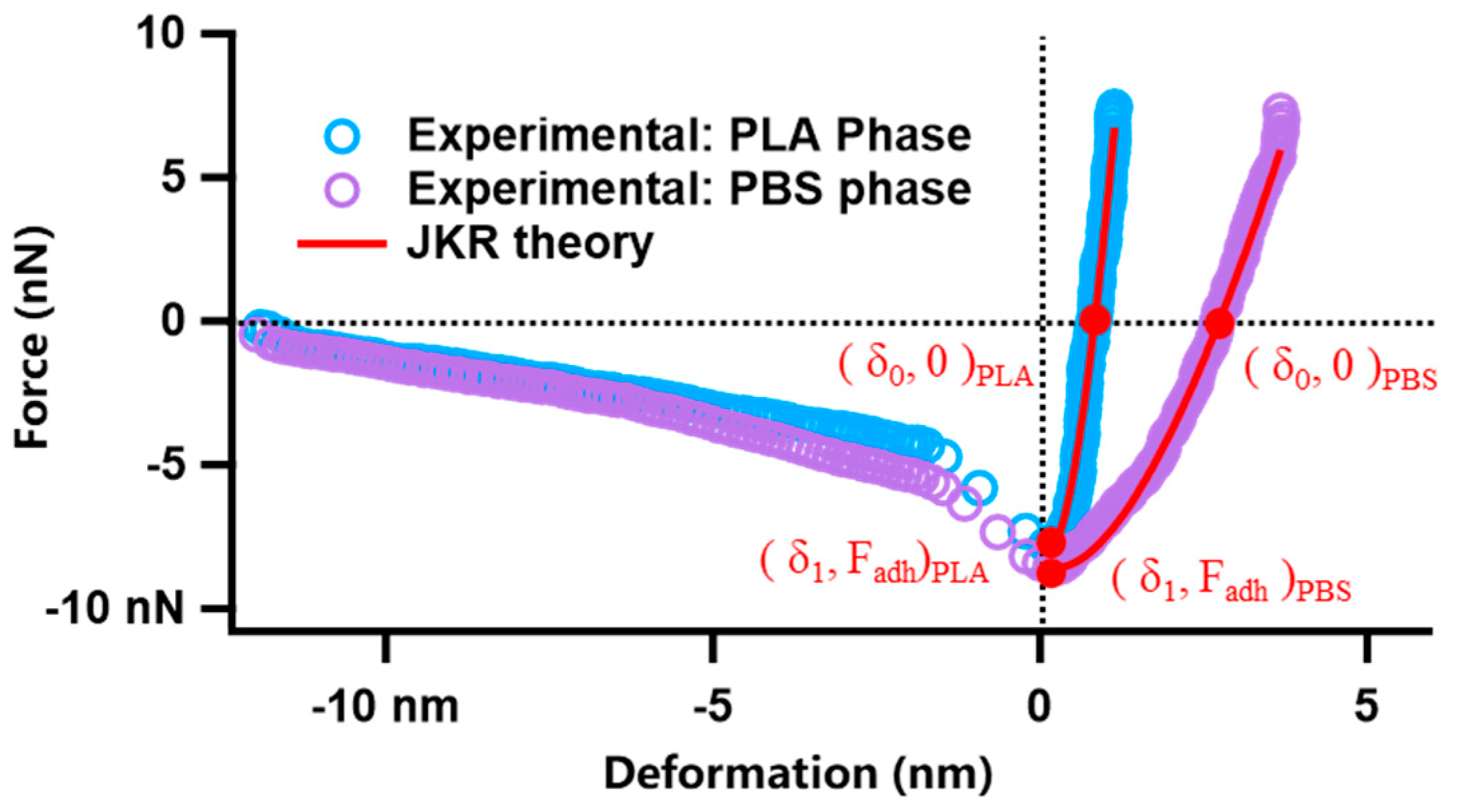
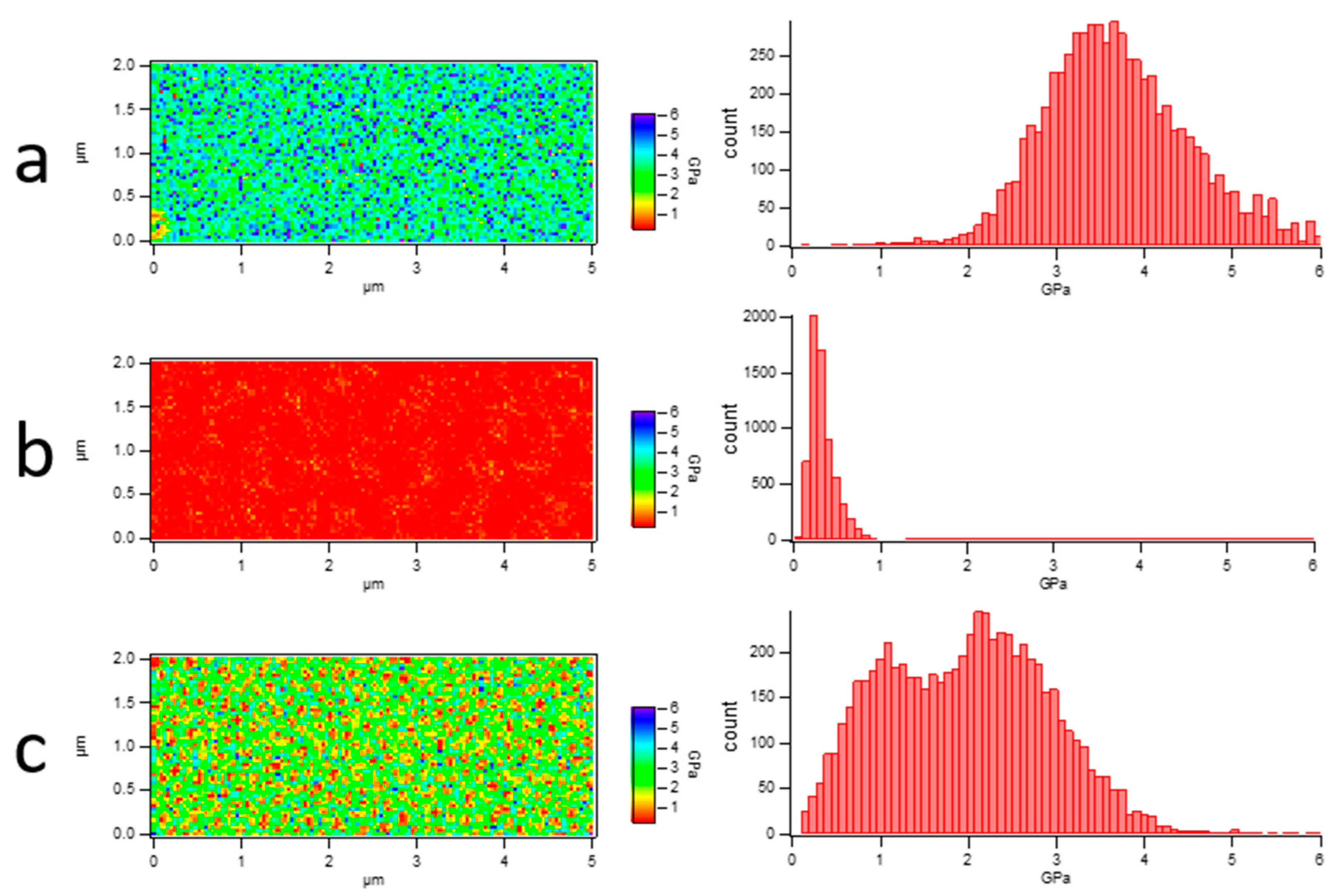
2.5.3. Nanomechanical Mapping
3. Results and Discussion
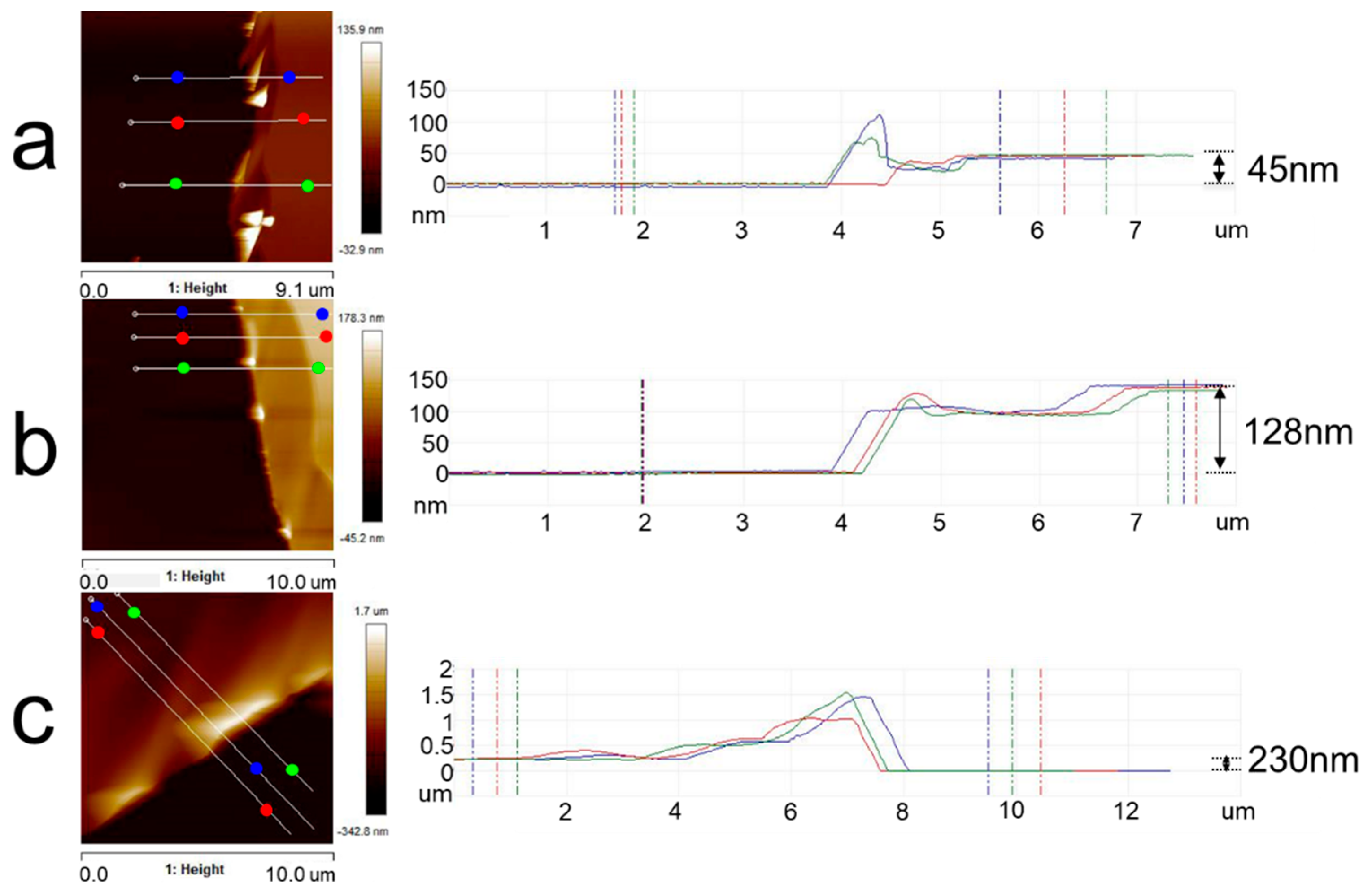
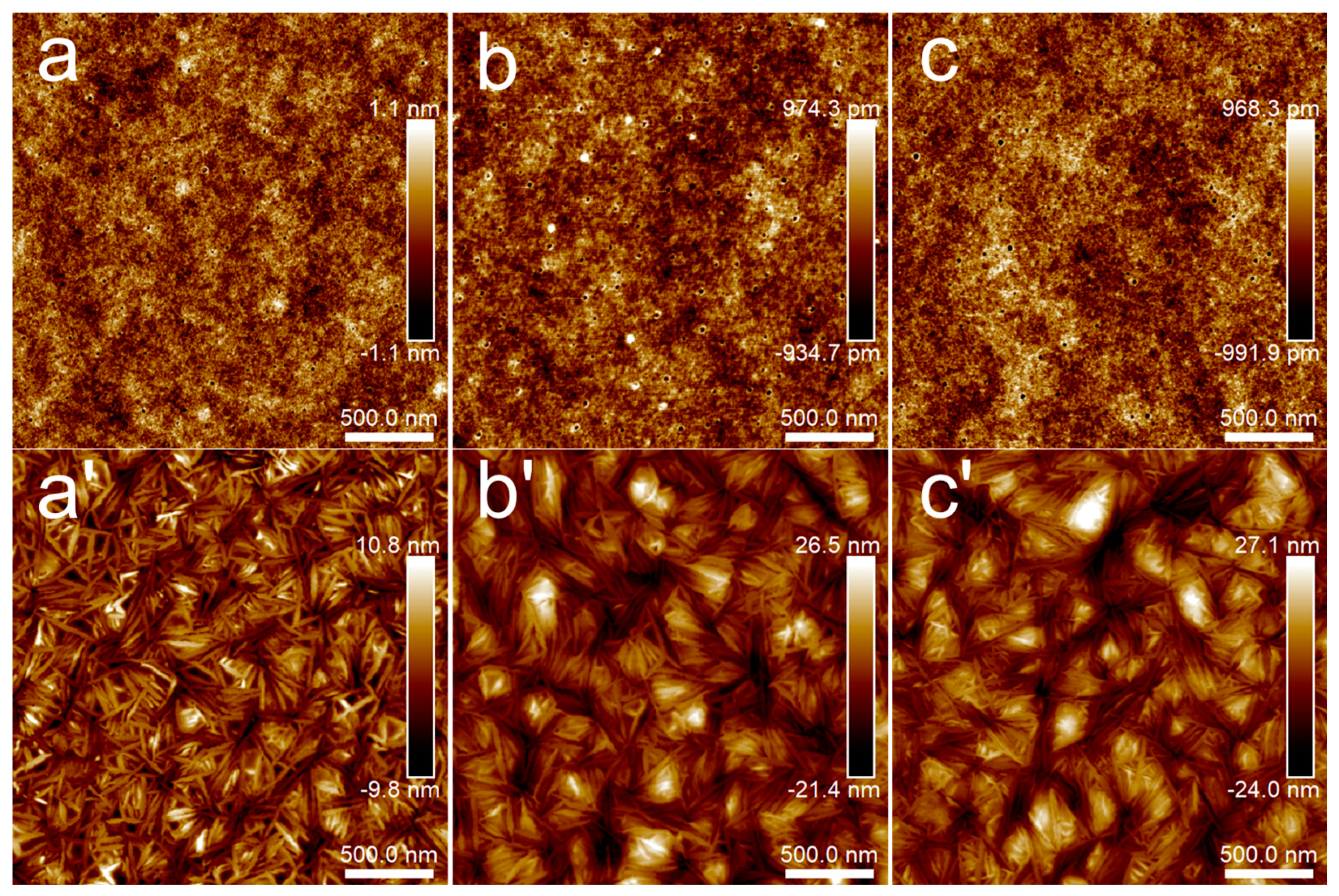
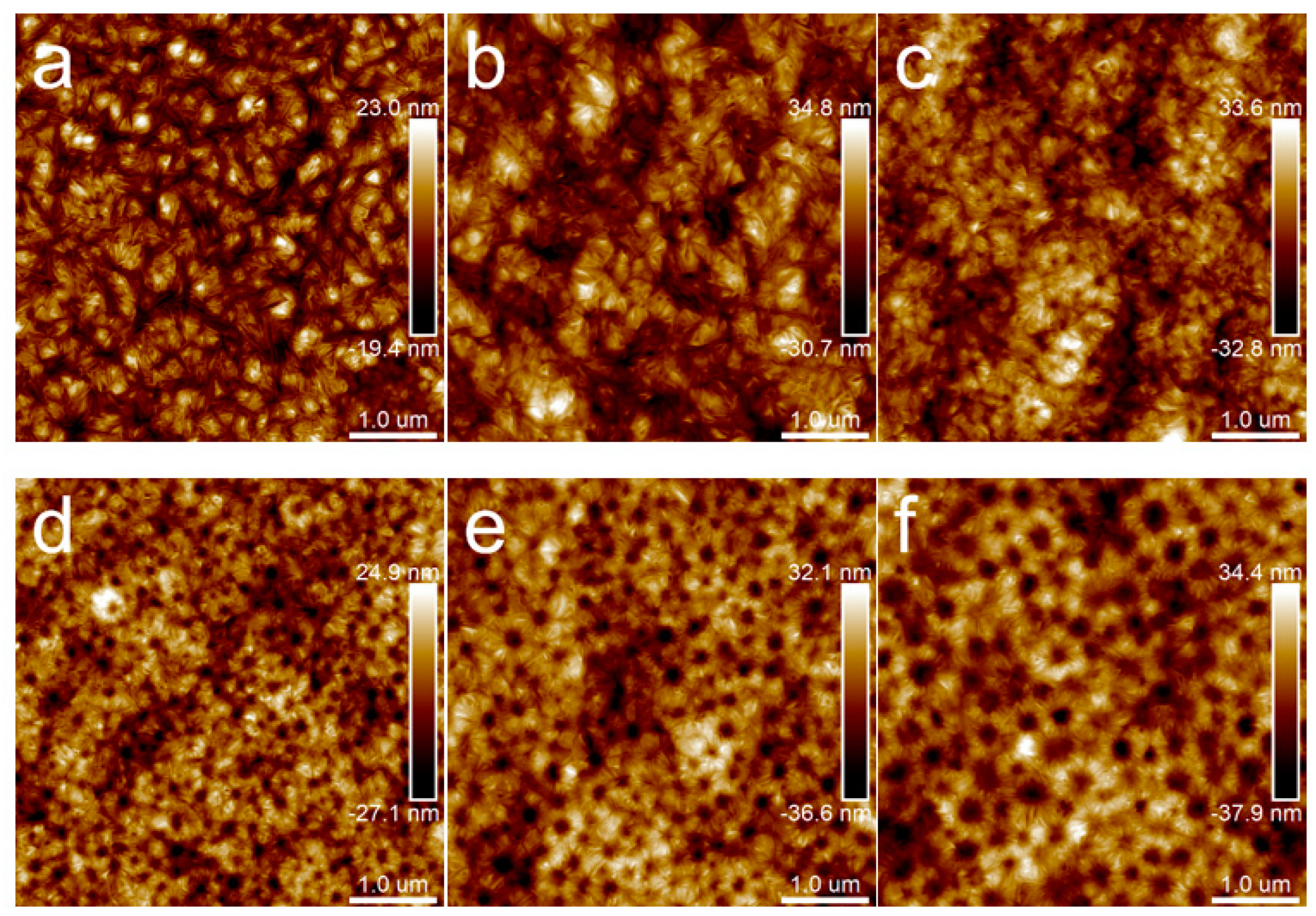
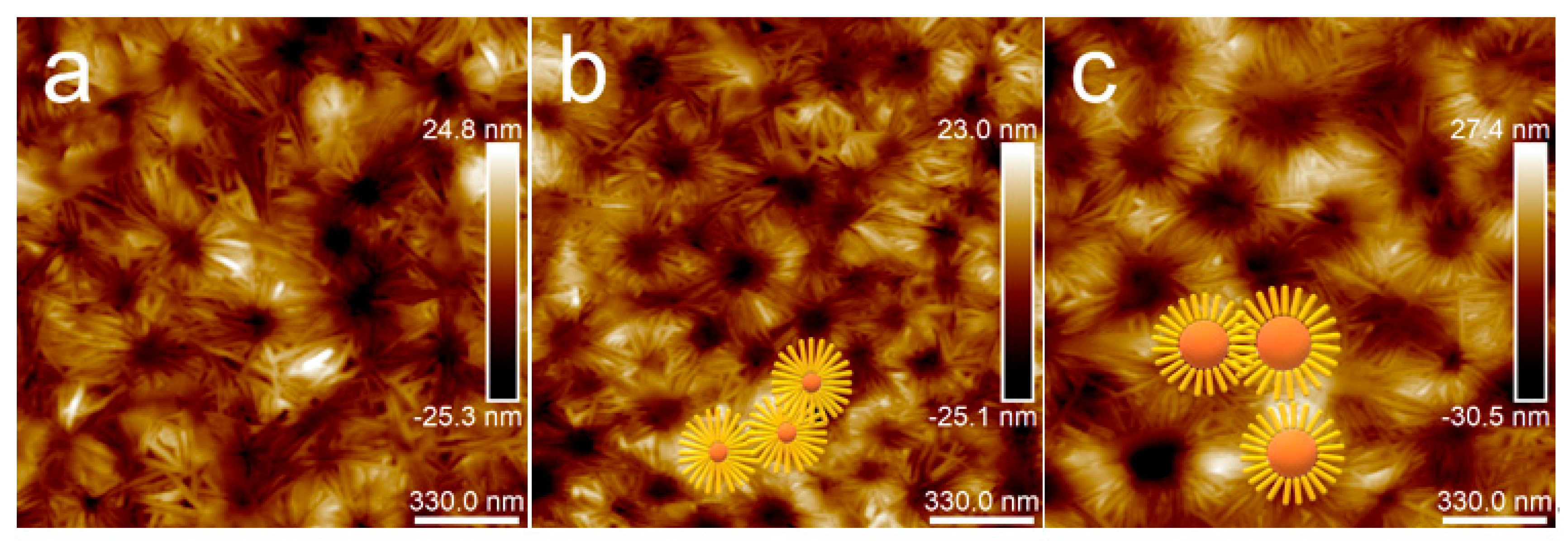
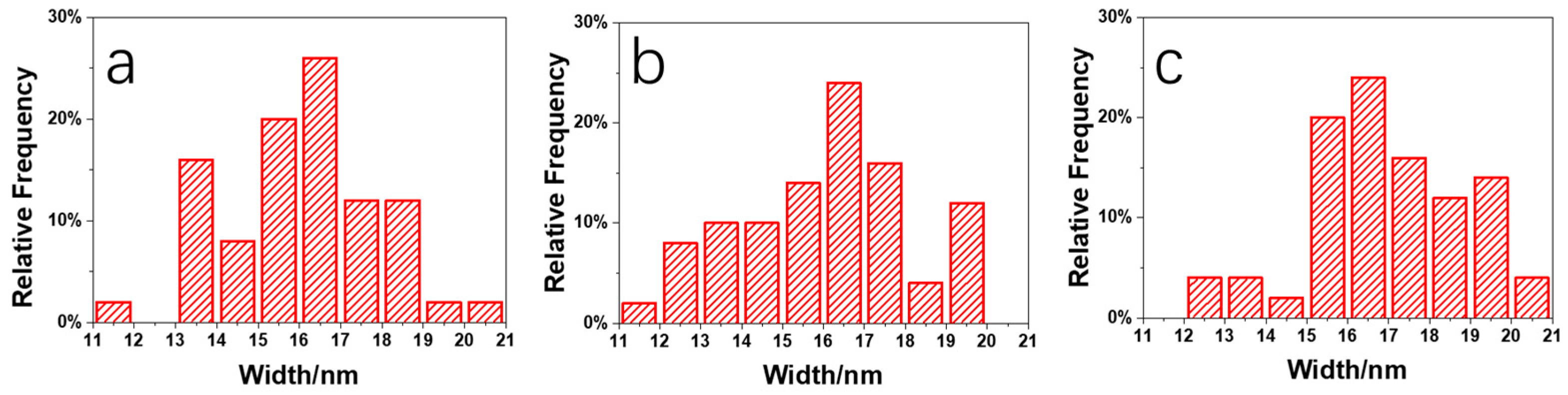
3.1. Microstructure of PLA Film
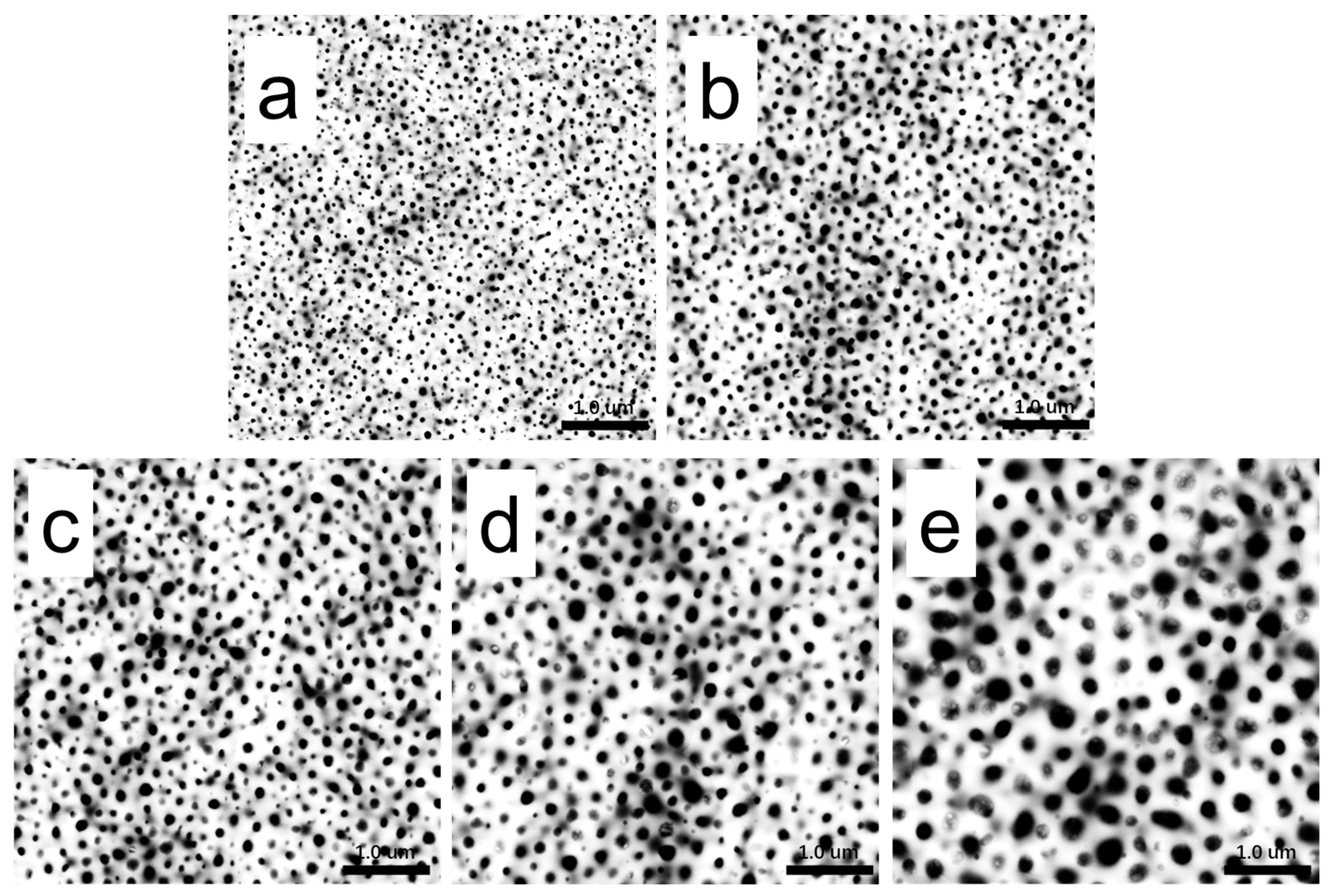
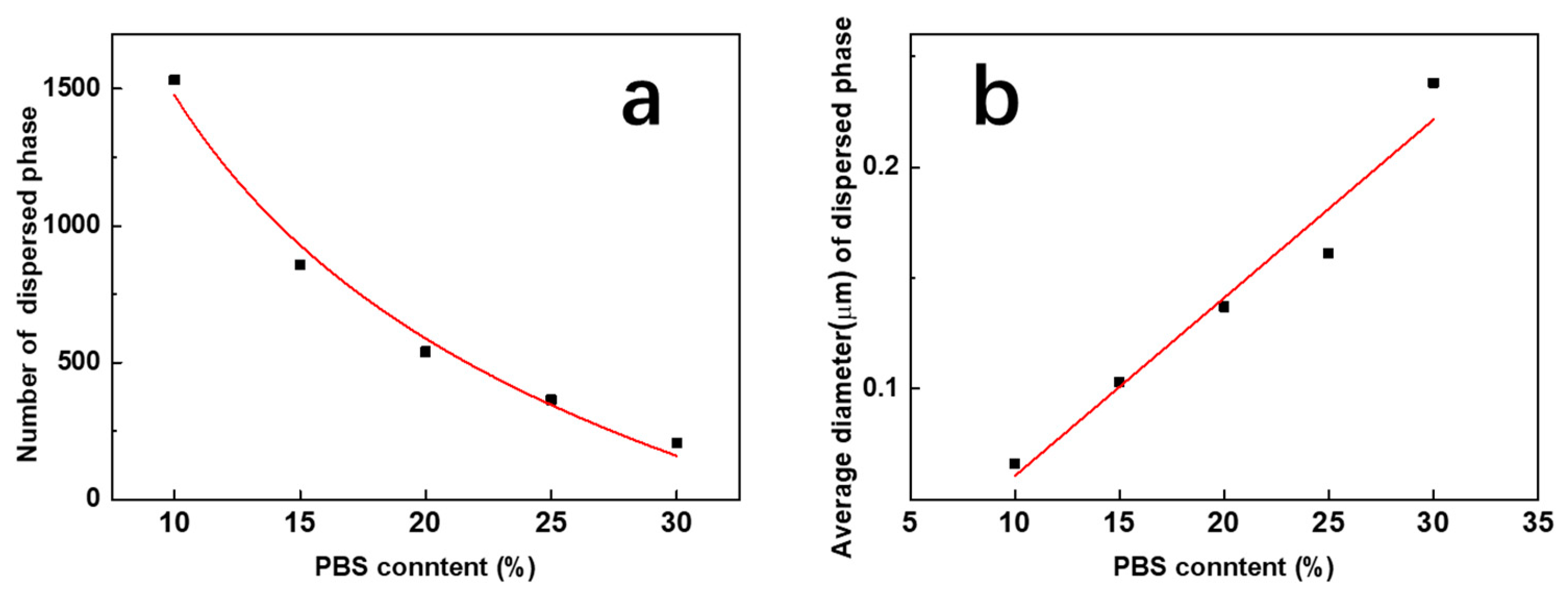
3.2. Microstructure of PLA/PBS Blend Film
3.3. Crystallization of PLA/PBS Blend Film
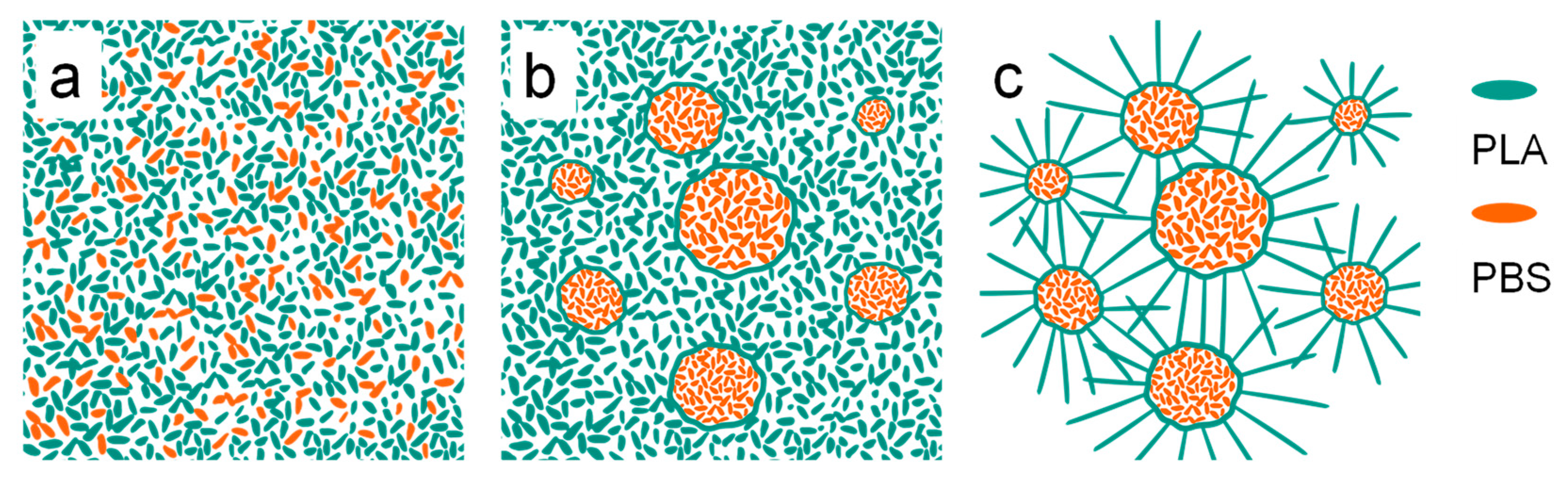
3.4. Mechanism of Crystallization
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Mtibe, A.; Motloung, M.P.; Bandyopadhyay, J.; Ray, S.S. Synthetic Biopolymers and Their Composites: Advantages and Limitations-An Overview. Macromol. Rapid Commun. 2021, 42, 2100130. [Google Scholar] [CrossRef]
- Lim, L.T.; Auras, R.; Rubino, M. Processing technologies for poly(lactic acid). Prog. Polym. Sci. 2008, 33, 820–852. [Google Scholar] [CrossRef]
- Hamad, K.; Kaseem, M.; Ayyoob, M.; Joo, J.; Deri, F. Polylactic acid blends: The future of green, light and tough. Prog. Polym. Sci. 2018, 85, 83–127. [Google Scholar] [CrossRef]
- Andrzejewski, J.; Cheng, J.; Anstey, A.; Mohanty, A.K.; Misra, M. Development of Toughened Blends of Poly(lactic acid) and Poly(butylene adipate-co-terephthalate) for 3D Printing Applications: Compatibilization Methods and Material Performance Evaluation. ACS Sustain. Chem. Eng. 2020, 8, 6576–6589. [Google Scholar] [CrossRef]
- Xue, B.; He, H.-Z.; Huang, Z.-X.; Zhu, Z.; Xue, F.; Liu, S.; Liu, B. Fabrication of super-tough ternary blends by melt compounding of poly(lactic acid) with poly(butylene succinate) and ethylene-methyl acrylate-glycidyl methacrylate. Compos. Eng. 2019, 172, 743–749. [Google Scholar] [CrossRef]
- Chen, J.; Rong, C.; Lin, T.; Chen, Y.; Wu, J.; You, J.; Wang, H.; Li, Y. Stable Co-Continuous PLA/PBAT Blends Compatibilized by Interfacial Stereocomplex Crystallites: Toward Full Biodegradable Polymer Blends with Simultaneously Enhanced Mechanical Properties and Crystallization Rates. Macromolecules 2021, 54, 2852–2861. [Google Scholar] [CrossRef]
- Maroufkhani, M.; Katbab, A.; Liu, W.; Zhang, J. Polylactide (PLA) and acrylonitrile butadiene rubber (NBR) blends: The effect of ACN content on morphology, compatibility and mechanical properties. Polymer 2017, 115, 37–44. [Google Scholar] [CrossRef]
- Srimalanon, P.; Prapagdee, B.; Markpin, T.; Sombatsompop, N. Effects of DCP as a free radical producer and HPQM as a biocide on the mechanical properties and antibacterial performance of in situ compatibilized PBS/PLA blends. Polym. Test. 2018, 67, 331–341. [Google Scholar] [CrossRef]
- Rasal, R.M.; Janorkar, A.V.; Hirt, D.E. Poly(lactic acid) modifications. Prog. Polym. Sci. 2010, 35, 338–356. [Google Scholar] [CrossRef]
- Low, Z.-X.; Chua, Y.T.; Ray, B.M.; Mattia, D.; Metcalfe, I.S.; Patterson, D.A. Perspective on 3D printing of separation membranes and comparison to related unconventional fabrication techniques. J. Membr. Sci. 2017, 523, 596–613. [Google Scholar] [CrossRef]
- Alvarez, S.; Marcasuzaa, P.; Billon, L. Bio-Inspired Silica Films Combining Block Copolymers Self-Assembly and Soft Chemistry: Paving the Way toward Artificial Exosqueleton of Seawater Diatoms. Macromol. Rapid Commun. 2021, 42, 2000582. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Wang, L.; Liu, H.; He, S.; Liu, X.; Liu, W.; Huang, M.; Zhu, C. Polyurethane as smart biocoatings: Effects of hard segments on phase structures and properties. Prog. Org. Coat. 2021, 150, 106000. [Google Scholar] [CrossRef]
- Zhang, C.X.; Li, H.W.; Wu, Y. Polyvinyl Alcohol-Supported AuAgNCs-CDs Film as a Selective Sensor for Gas Hydrogen Sulfide Detection in Air. Macromol. Rapid Commun. 2020, 41, 2000120. [Google Scholar] [CrossRef]
- Li, J.; Yu, X.; Herberg, A.; Kuckling, D. Biomolecule Sensor Based on Azlactone-Modified Hydrogel Films. Macromol. Rapid Commun. 2018, 40, 1800674. [Google Scholar] [CrossRef]
- Liu, S.S.Y.; Ludwigs, S. Electrochemical Manipulation of Aligned Block Copolymer Templates. Macromol. Rapid Commun. 2020, 41, 1900485. [Google Scholar] [CrossRef]
- Turgut, H.; Varadharajan, D.; Dingenouts, N.; Delaittre, G. Nanostructured Thin Films of Moderately Functionalized PMMA-b-PS. Macromol. Rapid Commun. 2018, 39, 1800231. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.; Chen, J.; Freyberg, P.; Reiter, R.; Mülhaupt, R.; Xu, J.; Reiter, G. High-Temperature Stability of Dewetting-Induced Thin Polyethylene Filaments. Macromolecules 2015, 48, 1518–1523. [Google Scholar] [CrossRef]
- Wu, X.; Zhou, J.; Huang, J. Integration of Biomaterials into Sensors Based on Organic Thin-Film Transistors. Macromol. Rapid Commun. 2018, 39, 1800084. [Google Scholar] [CrossRef]
- Xue, L.; Han, Y. Inhibition of dewetting of thin polymer films. Prog. Mater. Sci. 2012, 57, 947–979. [Google Scholar] [CrossRef]
- Xue, L.; Zhang, J.; Han, Y. Phase separation induced ordered patterns in thin polymer blend films. Prog. Polym. Sci. 2012, 37, 564–594. [Google Scholar] [CrossRef]
- Guo, S.; Lu, Y.; Wang, B.; Shen, C.; Chen, J.; Reiter, G.; Zhang, B. Controlling the pore size in conjugated polymer films via crystallization-driven phase separation. Soft Matter 2019, 15, 2981–2989. [Google Scholar] [CrossRef] [PubMed]
- Zhang, B.; Wang, B.; Chen, J.; Shen, C.; Reiter, R.; Chen, J.; Reiter, G. Flow-Induced Dendritic β-Form Isotactic Polypropylene Crystals in Thin Films. Macromolecules 2016, 49, 5145–5151. [Google Scholar] [CrossRef]
- Guan, G.; Zhang, J.; Sun, X.; Li, H.; Yan, S.; Lotz, B. Oriented Overgrowths of Poly(l-Lactide) on Oriented Isotactic Polypropylene: A Sequence of Soft and Hard Epitaxies. Macromol. Rapid Commun. 2018, 39, 1800353. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.H.; Fang, D.F.; Lei, J.; Li, L.B.; Hsiao, B.S.; Li, Z.M. Shear-Induced Precursor Relaxation-Dependent Growth Dynamics and Lamellar Orientation of beta-Crystals in beta-Nucleated Isotactic Polypropylene. J. Phys. Chem. B 2015, 119, 5716–5727. [Google Scholar] [CrossRef] [PubMed]
- Su, F.; Zhou, W.; Li, X.; Ji, Y.; Cui, K.; Qi, Z.; Li, L. Flow-Induced Precursors of Isotactic Polypropylene: An in Situ Time and Space Resolved Study with Synchrotron Radiation Scanning X-ray Microdiffraction. Macromolecules 2014, 47, 4408–4416. [Google Scholar] [CrossRef]
- Liu, Q.; Sun, X.; Li, H.; Yan, S. Orientation-induced crystallization of isotactic polypropylene. Polymer 2013, 54, 4404–4421. [Google Scholar] [CrossRef]
- Prud’homme, R.E. Crystallization and morphology of ultrathin films of homopolymers and polymer blends. Prog. Polym. Sci. 2016, 54–55, 214–231. [Google Scholar] [CrossRef]
- Li, L.; Hu, J.; Li, Y.; Huang, Q.; Sun, X.; Yan, S. Evidence for the Soft and Hard Epitaxies of Poly (l-lactic acid) on an Oriented Polyethylene Substrate and Their Dependence on the Crystallization Temperature. Macromolecules 2020, 53, 1745–1751. [Google Scholar] [CrossRef]
- Aliotta, L.; Seggiani, M.; Lazzeri, A.; Gigante, V.; Cinelli, P. A Brief Review of Poly (Butylene Succinate) (PBS) and Its Main Copolymers: Synthesis, Blends, Composites, Biodegradability, and Applications. Polymers 2022, 14, 844. [Google Scholar] [CrossRef]
- Binti Ali, F.; Mohan, R. Thermal, Mechanical, and Rheological Properties of Biodegradable Polybutylene Succinate/Carbon Nanotubes. Polym. Compos. 2010, 31, 1309–1314. [Google Scholar]
- Aziman, N.; Kian, L.K.; Jawaid, M.; Sanny, M.; Alamery, S. Morphological, Structural, Thermal, Permeability, and Antimicrobial Activity of PBS and PBS/TPS Films Incorporated with Biomaster-Silver for Food Packaging Application. Polymers 2021, 13, 391. [Google Scholar] [CrossRef]
- Chaiwutthinan, P.; Pimpan, V.; Chuayjuljit, S.; Leejarkpai, T. Biodegradable Plastics Prepared from Poly (lactic acid), Poly (butylene succinate) and Microcrystalline Cellulose Extracted from Waste-Cotton Fabric with a Chain Extender. J. Polym. Environ. 2015, 23, 114–125. [Google Scholar] [CrossRef]
- Chen, G.; Yoon, J. Thermal stability of poly(l-lactide)/poly (butylene succinate)/clay nanocomposites. Polym. Degrad. Stab. 2005, 88, 206–212. [Google Scholar] [CrossRef]
- Gigante, V.; Coltelli, M.-B.; Vannozzi, A.; Panariello, L.; Fusco, A.; Trombi, L.; Donnarumma, G.; Danti, S.; Lazzeri, A. Flat Die Extruded Biocompatible Poly (Lactic Acid) (PLA)/Poly (Butylene Succinate) (PBS) Based Films. Polymers 2019, 11, 1857. [Google Scholar] [CrossRef] [PubMed]
- Xu, J.; Guo, B. Poly(butylene succinate) and its copolymers: Research, development and industrialization. Biotechnol. J. 2010, 5, 1149–1163. [Google Scholar] [CrossRef]
- Sun, Y.; Akhremitchev, B.; Walker, G.C. Using the adhesive interaction between atomic force microscopy tips and polymer surfaces to measure the elastic modulus of compliant samples. Langmuir 2004, 20, 5837–5845. [Google Scholar] [CrossRef]
- Liu, H.; Chen, N.; Fujinami, S.; Louzguine-Luzgin, D.; Nakajima, K.; Nishi, T. Quantitative Nanomechanical Investigation on Deformation of Poly(lactic acid). Macromolecules 2012, 45, 8770–8779. [Google Scholar] [CrossRef]
- Rose, M.M.; Alejandro, J.M. Confined crystallization of polymeric materials. Prog. Polym. Sci. 2016, 54–55, 183–213. [Google Scholar]
- Fujinami, S.; Ueda, E.; Nakajima, K.; Nishi, T. Analytical methods to derive the elastic modulus of soft and adhesive materials from atomic force microcopy force measurements. J. Polym. Sci. Polym. Phys. 2019, 57, 1279–1286. [Google Scholar] [CrossRef]
- Johnson, K.L.; Kendall, K.; Roberts, A.D. Surface energy and the contact of elastic solids. Proc. R. Soc. Lond. A 1971, 324, 301–313. [Google Scholar]
- Zhu, B.; Wang, Y.; Liu, H.; Ying, J.; Liu, C.; Shen, C. Effects of interface interaction and microphase dispersion on the mechanical properties of PCL/PLA/MMT nanocomposites visualized by nanomechanical mapping. Compos. Sci. Technol. 2020, 190, 108048. [Google Scholar] [CrossRef]
- Zhang, X.; Sun, S.; Ning, N.; Yan, S.; Wu, X.; Lu, Y.; Zhang, L. Visualization and Quantification of the Microstructure Evolution of Isoprene Rubber during Uniaxial Stretching Using AFM Nanomechanical Mapping. Macromolecules 2020, 53, 3082–3089. [Google Scholar] [CrossRef]
- Abràmoff, M.D.; Magalhães, P.J.; Ram, S.J. Image Processing with ImageJ.pdf. Biophotonics Int. 2004, 11, 36–42. [Google Scholar]
- Hsieh, Y.-T.; Nozaki, S.; Kido, M.; Kamitani, K.; Kojio, K.; Takahara, A. Crystal polymorphism of polylactide and its composites by X-ray diffraction study. Polym. J. 2020, 52, 755–763. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Wang, Y.; Mou, C.; Wang, W.; Zhu, C.; He, S.; Liu, H.; Liu, W. Petal-like Patterning of Polylactide/Poly (Butylene Succinate) Thin Films Induced by Phase Separation. Polymers 2023, 15, 4463. https://doi.org/10.3390/polym15224463
Wang L, Wang Y, Mou C, Wang W, Zhu C, He S, Liu H, Liu W. Petal-like Patterning of Polylactide/Poly (Butylene Succinate) Thin Films Induced by Phase Separation. Polymers. 2023; 15(22):4463. https://doi.org/10.3390/polym15224463
Chicago/Turabian StyleWang, Lili, Yujie Wang, Chudi Mou, Wanjie Wang, Chengshen Zhu, Suqin He, Hao Liu, and Wentao Liu. 2023. "Petal-like Patterning of Polylactide/Poly (Butylene Succinate) Thin Films Induced by Phase Separation" Polymers 15, no. 22: 4463. https://doi.org/10.3390/polym15224463
APA StyleWang, L., Wang, Y., Mou, C., Wang, W., Zhu, C., He, S., Liu, H., & Liu, W. (2023). Petal-like Patterning of Polylactide/Poly (Butylene Succinate) Thin Films Induced by Phase Separation. Polymers, 15(22), 4463. https://doi.org/10.3390/polym15224463






