Investigation of the Gas Permeation Properties Using the Volumetric Analysis Technique for Polyethylene Materials Enriched with Pure Gases under High Pressure: H2, He, N2, O2 and Ar
Abstract
:1. Introduction
2. Materials and Methods
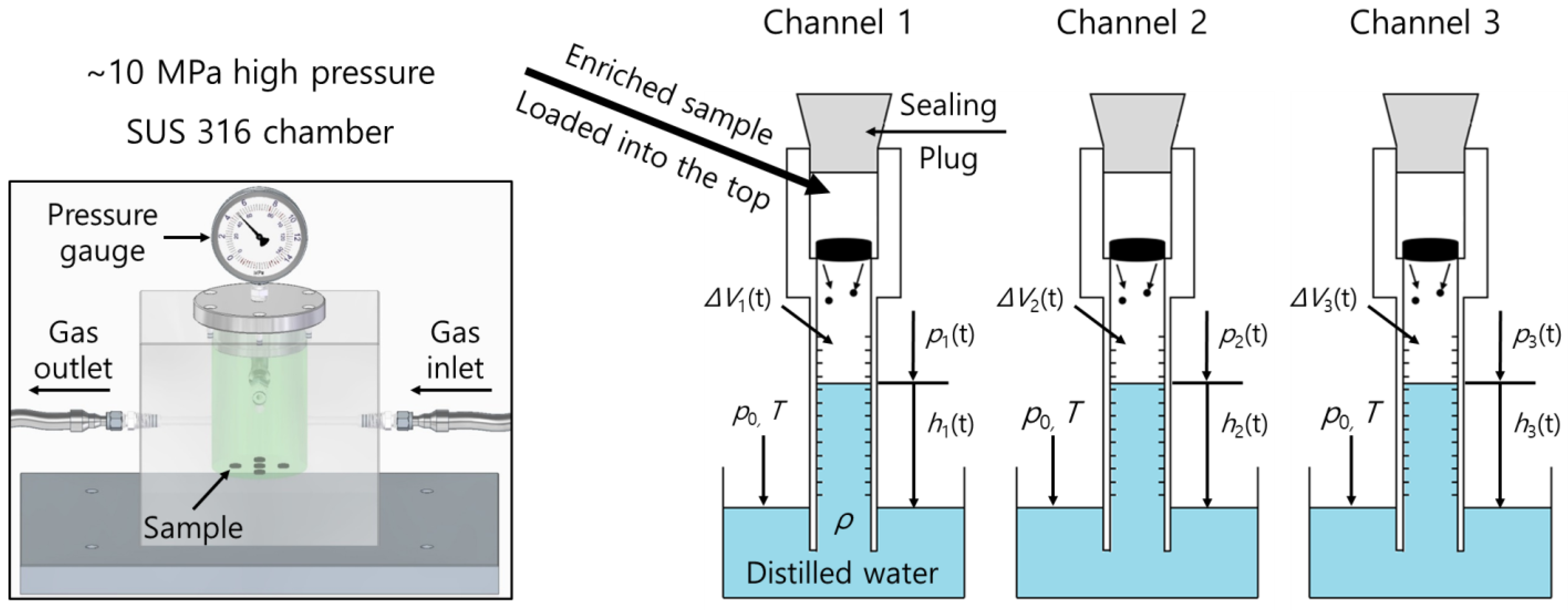
2.1. Sample Preparation and Gas Exposure Conditions
- Cylindrical-shaped LDPE, UHMWPE and HDPE with radii of 9.5 mm and thicknesses of 1.64, 3.24 and 4.93 mm, respectively.
2.2. Measurement of Density and Crystallinity
2.3. Measurement of Emitted Gas Concentration
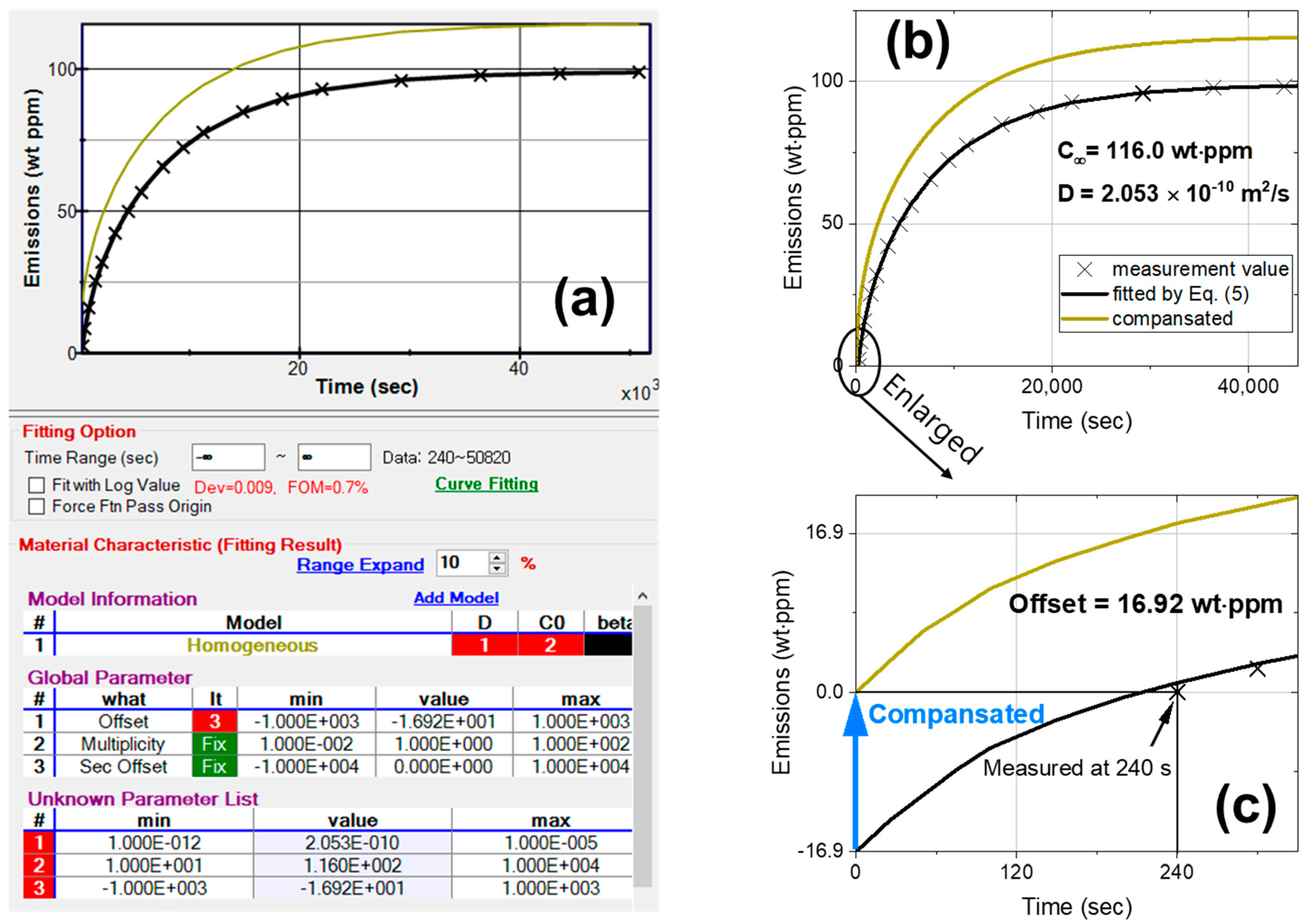
2.4. Diffusion Analysis Program for Determining Gas Uptake and Diffusivity
3. Results and Discussion
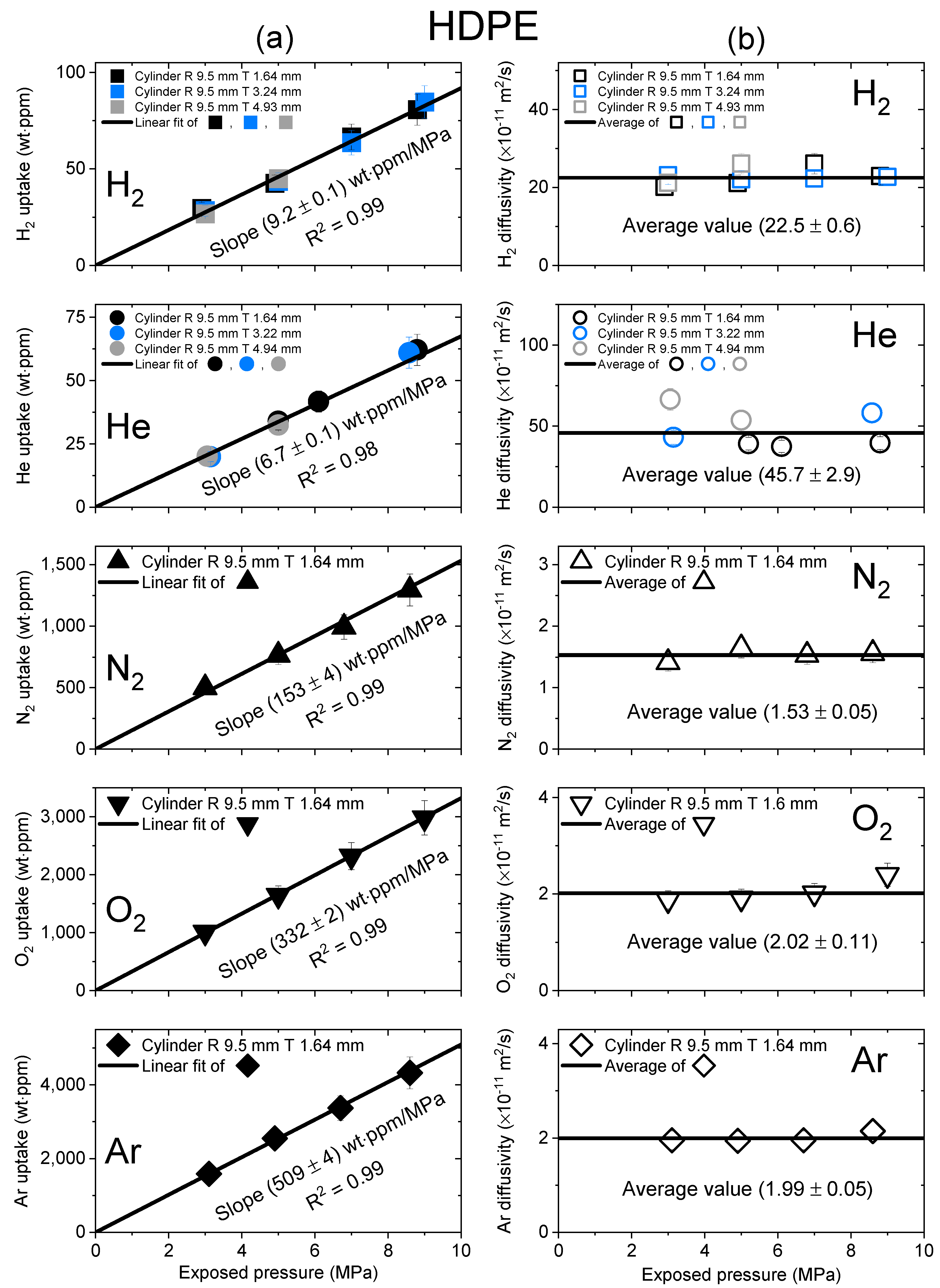
3.1. Effect of Pressure and Gases on Gas Uptake/Diffusivity
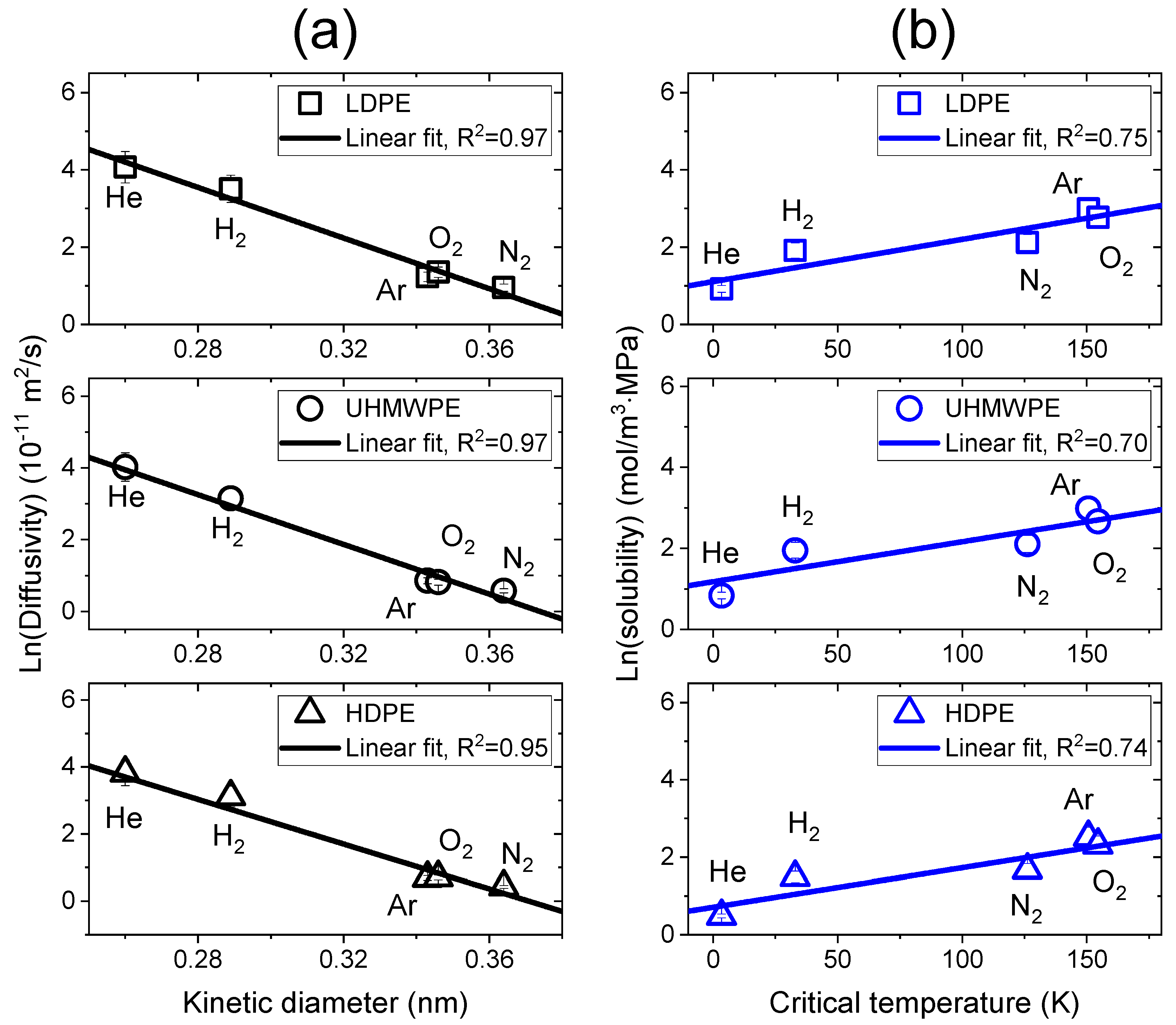
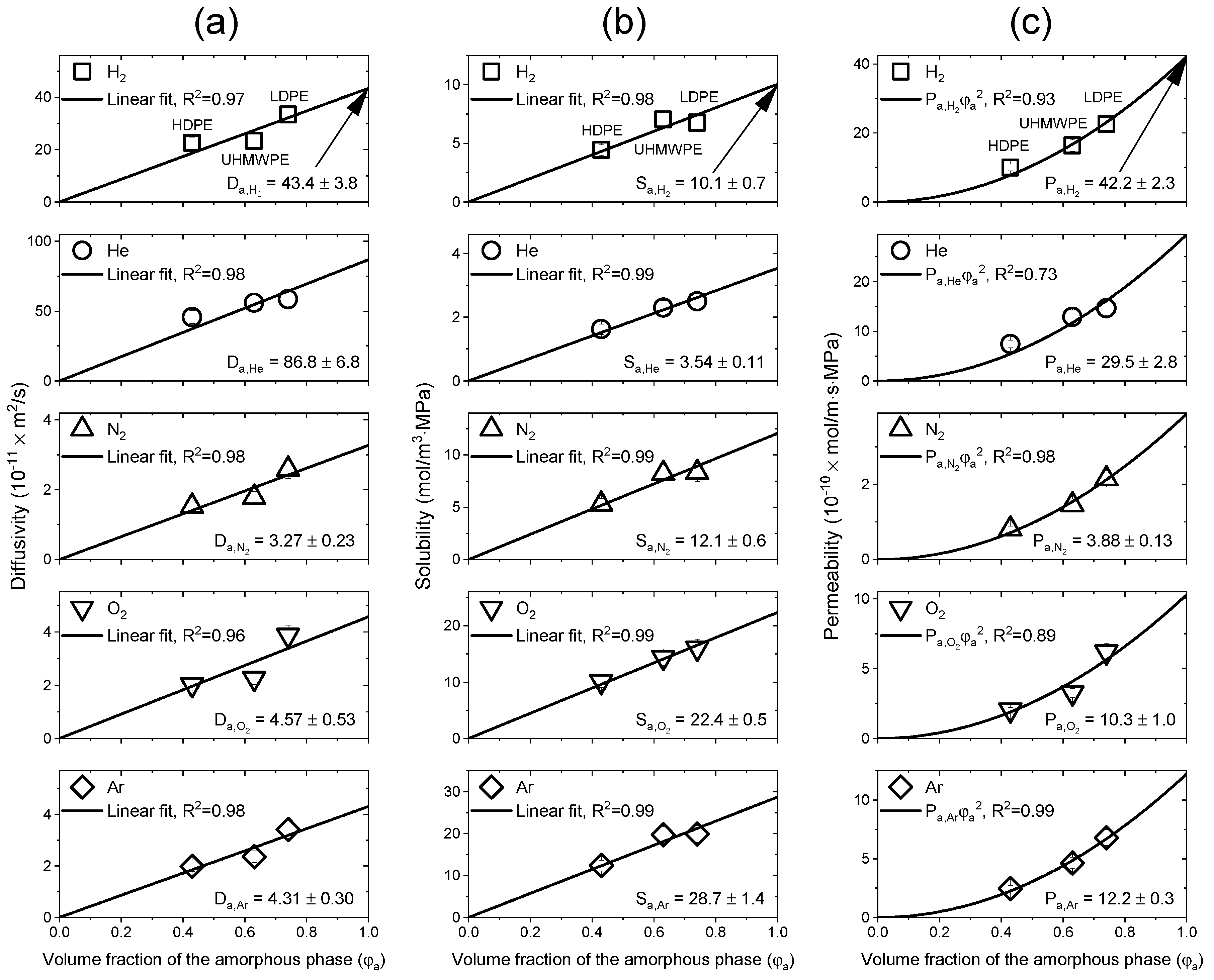
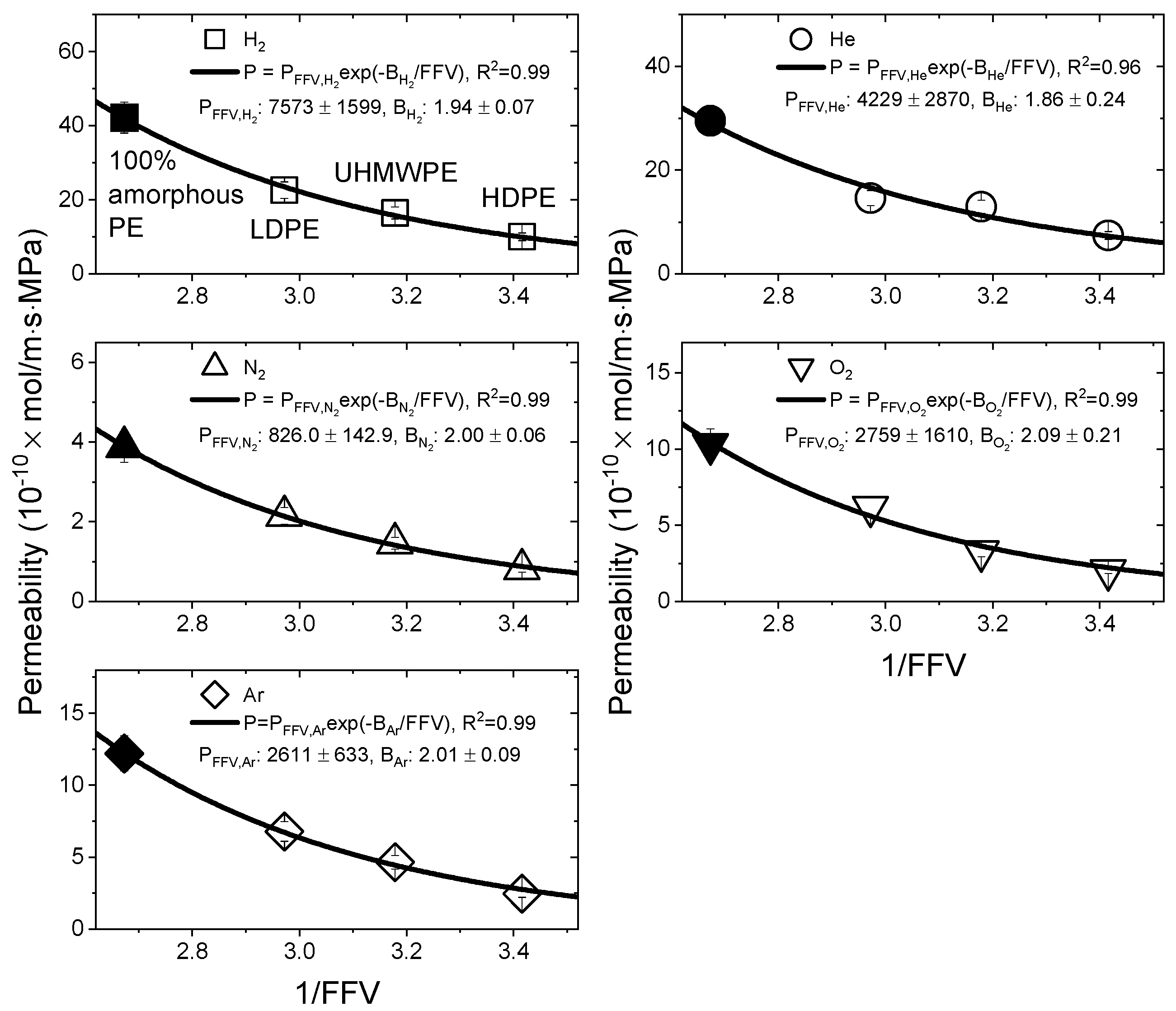
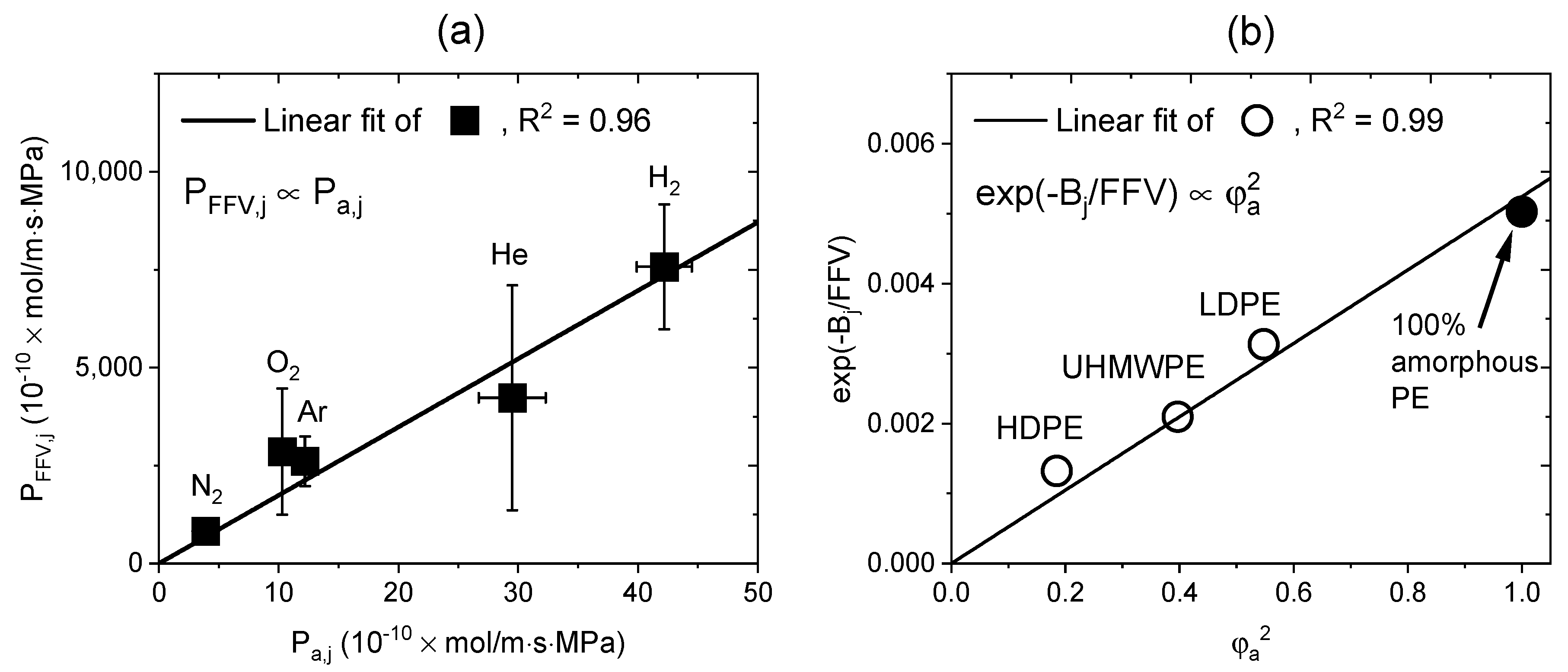
3.2. Effects of the Amorphous Phase and Free Volume on the Gas Permeation Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Patel, R.M. Types and basics of polyethylene. In Handbook of Industrial Polyethylene and Technology; Spalding, M.A., Chatterjee, A.M., Eds.; Wiley: New York, NY, USA, 2017; pp. 105–138. [Google Scholar]
- Peacock, A. Handbook of Polyethylene: Structures: Properties, and Applications; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Vasile, C. Handbook of Polyolefins; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Lustiger, A.; Ishikawa, N. An analytical technique for measuring relative tie-molecule concentration in polyethylene. J. Polym. Sci. B Polym. Phys. 1991, 29, 1047–1055. [Google Scholar] [CrossRef]
- Patel, R.M.; Sehanobish, K.; Jain, P.; Chum, S.P.; Knight, G.W. Theoretical prediction of tie-chain concentration and its characterization using postyield response. J. Appl. Polym. Sci. 1996, 60, 749–758. [Google Scholar] [CrossRef]
- Gaur, U.; Wunderlich, B. Heat capacity and other thermodynamic properties of linear macromolecules. II. Polyethylene. J. Phys. Chem. Ref. Data 1981, 10, 119–152. [Google Scholar] [CrossRef]
- Visco, A.; Scolaro, C.; Torrisi, A.; Torrisi, L. Diffusion of nitrogen gas through polyethylene based films. Polym. Cryst. 2021, 4, e10207. [Google Scholar] [CrossRef]
- Siracusa, V. Food packaging permeability behaviour: A report. Int. J. Polym. Sci. 2012, 2012, 302029. [Google Scholar] [CrossRef]
- Pleşa, I.; Noţingher, P.V.; Stancu, C.; Wiesbrock, F.; Schlögl, S. Polyethylene nanocomposites for power cable insulations. Polymers 2018, 11, 24. [Google Scholar]
- Dodds, P.E.; Demoullin, S. Conversion of the UK gas system to transport hydrogen. Int. J. Hydrog. Energy 2013, 38, 7189–7200. [Google Scholar] [CrossRef]
- Gąsior, P.; Wachtarczyk, K.; Błachut, A.; Kaleta, J.; Yadav, N.; Ozga, M.; Baron, A. Validation of selected optical methods for assessing polyethylene (PE) liners used in high pressure vessels for hydrogen storage. Appl. Sci. 2021, 11, 5667. [Google Scholar] [CrossRef]
- Alter, H. A critical investigation of polyethylene gas permeability. J. Polym. Sci. 1962, 57, 925–935. [Google Scholar] [CrossRef]
- Michaels, A.S.; Bixler, H.J. Flow of gases through polyethylene. J. Polym. Sci. 1961, 50, 413–439. [Google Scholar] [CrossRef]
- Michaels, A.S.; Vieth, W.R.; Barrie, J.A. Solution of gases in polyethylene terephthalate. J. Appl. Phys. 1963, 34, 1–12. [Google Scholar] [CrossRef]
- Shrestha, R.; Ronevich, J.A.; Fring, L.; Simmons, K.; Meeks, N.D.; Lowe, Z.E.; Harris, T.J., Jr.; San Marchi, C. Compatibility of medium density polyethylene (MDPE) for distribution of gaseous hydrogen. In Proceedings of the Pressure Vessels and Piping Conference, Las Vegas, Nevada, USA, July 2022; American Society of Mechanical Engineers: New York, NY, USA, 2022; Volume 86182, p. V04BT06A041. [Google Scholar]
- Fujiwara, H.; Ono, H.; Ohyama, K.; Kasai, M.; Kaneko, F.; Nishimura, S. Hydrogen permeation under high pressure conditions and the destruction of exposed polyethylene-property of polymeric materials for high-pressure hydrogen devices (2)-. Int. J. Hydrog. Energy 2021, 46, 11832–11848. [Google Scholar] [CrossRef]
- Kanesugi, H.; Ohyama, K.; Fujiwara, H.; Nishimura, S. High-pressure hydrogen permeability model for crystalline polymers. Int. J. Hydrog. Energy 2023, 48, 723–739. [Google Scholar] [CrossRef]
- Pixton, M.R.; Paul, D.R. Gas transport properties of polyarylates: Substituent size and symmetry effects. Macromolecules 1995, 28, 8277–8286. [Google Scholar] [CrossRef]
- Aitken, C.L.; Koros, W.J.; Paul, D.R. Gas transport properties of biphenol polysulfones. Macromolecules 1992, 25, 3651–3658. [Google Scholar] [CrossRef]
- Yampolskii, Y. Polymeric gas separation membranes. Macromolecules 2012, 45, 3298–3311. [Google Scholar] [CrossRef]
- Kim, T.H.; Koros, W.J.; Husk, G.R.; O’brien, K.C. Relationship between gas separation properties and chemical structure in a series of aromatic polyimides. J. Membr. Sci. 1988, 37, 45–62. [Google Scholar] [CrossRef]
- Thran, A.; Kroll, G.; Faupel, F. Correlation between fractional free volume and diffusivity of gas molecules in glassy polymers. J. Polym. Sci. B Polym. Phys. 1999, 37, 3344–3358. [Google Scholar] [CrossRef]
- Mousavi, S.A.; Gholizadeh, M.; Sedghi, S.; Pourafshari-Chenar, M.; Barmala, M.; Soltani, A. Effects of preparation conditions on the morphology and gas permeation properties of polyethylene (PE) and ethylene vinyl acetate (EVA) films. Chem. Eng. Res. Des. 2010, 88, 1593–1598. [Google Scholar] [CrossRef]
- Flaconnèche, B.; Martin, J.; Klopffer, M.-H. Permeability, diffusion and solubility of gases in polyethylene, polyamide 11 and poly (vinylidene fluoride). Oil Gas Sci. Technol. 2001, 56, 261–278. [Google Scholar] [CrossRef]
- Khoshkam, M.; Sadeghi, M.; Chenar, M.P.; Naghsh, M.; Shafiei, M. Synthesis, characterization and gas separation properties of novel copolyimide membranes based on flexible etheric–aliphatic moieties. RSC Adv. 2016, 6, 35751–35763. [Google Scholar] [CrossRef]
- Mehio, N.; Dai, S.; Jiang, D.E. Quantum mechanical basis for kinetic diameters of small gaseous molecules. J. Phys. Chem. A 2014, 118, 1150–1154. [Google Scholar] [CrossRef] [PubMed]
- Jung, J.K.; Lee, J.H.; Jang, J.S.; Chung, N.K.; Park, C.Y.; Baek, U.B.; Nahm, S.H. Characterization technique of gases permeation properties in polymers: H2, He, N2 and Ar gas. Sci. Rep. 2022, 12, 3328. [Google Scholar] [CrossRef]
- Olivoni, E.; Vertechy, R.; Parenti-Castelli, V. Power skiving manufacturing process: A review. Mech. Mach. Theory 2022, 175, 104955. [Google Scholar] [CrossRef]
- Webb, J.A.; Bower, D.I.; Ward, I.M.; Cardew, P.T. The effect of drawing on the transport of gases through polyethylene. J. Polym. Sci. B Polym. Phys. 1993, 31, 743–757. [Google Scholar] [CrossRef]
- Mathot, V.B.F.; Pijpers, M.F.J. Heat capacity, enthalpy and crystallinity for a linear polyethylene obtained by DSC. J. Therm. Anal. Calorim. 1983, 28, 349–358. [Google Scholar] [CrossRef]
- Zahariea, D. Dynamic response of the U-tube liquid manometer with equal diameter columns. IOP Conf. Ser. Earth Environ. Sci. 2010, 12, 012114. [Google Scholar] [CrossRef]
- Crank, J. The Mathematics of Diffusion; Oxford University Press: Oxford, UK, 1979. [Google Scholar]
- Yang, Y.; Liu, S. Estimation and modeling of pressure-dependent gas diffusion coefficient for coal: A fractal theory-based approach. Fuel 2019, 253, 588–606. [Google Scholar] [CrossRef]
- Bowman, F. Introduction to Bessel Functions; Courier Corporation: Chelmsford, MA, USA, 2012. [Google Scholar]
- Jung, J.K.; Kim, I.G.; Jeon, S.K.; Kim, K.-T.; Baek, U.B.; Nahm, S.H. Volumetric analysis technique for analyzing the transport properties of hydrogen gas in cylindrical-shaped rubbery polymers. Polym. Test. 2021, 99, 107147. [Google Scholar]
- Sander, R. Compilation of Henry’s law constants (version 4.0) for water as solvent. Atmos. Chem. Phys. 2015, 15, 4399–4981. [Google Scholar] [CrossRef]
- Ghosal, K.; Freeman, B.D. Gas separation using polymer membranes: An overview. Polym. Adv. Technol. 1994, 5, 673–697. [Google Scholar] [CrossRef]
- Pabby, A.K.; Rizvi, S.S.; Requena, A.M.S. Handbook of Membrane Separations: Chemical, Pharmaceutical, Food, and Biotechnological Applications; CRC Press: Boca Raton, FL, USA, 2008. [Google Scholar]
- Mohagheghian, M.; Sadeghi, M.; Chenar, M.P.; Naghsh, M. Gas separation properties of polyvinylchloride (PVC)-silica nanocomposite membrane. Korean J. Chem. Eng. 2014, 31, 2041–2050. [Google Scholar] [CrossRef]
- Kucukpinar, E.; Doruker, P. Molecular simulations of small gas diffusion and solubility in copolymers of styrene. Polymer 2003, 44, 3607–3620. [Google Scholar] [CrossRef]
- Robeson, L.M.; Smith, Z.P.; Freeman, B.D.; Paul, D.R. Contributions of diffusion and solubility selectivity to the upper bound analysis for glassy gas separation membranes. J. Membr. Sci. 2014, 453, 71–83. [Google Scholar] [CrossRef]
- Koros, W.J.; Fleming, G.K.; Jordan, S.M.; Kim, T.H.; Hoehn, H.H. Polymeric membrane materials for solution-diffusion based permeation separations. Prog. Polym. Sci. 1988, 13, 339–401. [Google Scholar] [CrossRef]
- Wijmans, J.G.; Baker, R.W. The solution-diffusion model: A review. J. Membr. Sci. 1995, 107, 1–21. [Google Scholar] [CrossRef]
- Paul, D.R. Reformulation of the solution-diffusion theory of reverse osmosis. J. Membr. Sci. 2004, 241, 371–386. [Google Scholar] [CrossRef]
- Runt, J.; Kanchanasopa, M. Crystallinity determination. In Encyclopedia of Polymer Science and Technology; Wiley: New York, NY, USA, 2002. [Google Scholar]
- Mohr, J.M.; Paul, D.R. Comparison of gas permeation in vinyl and vinylidene polymers. J. Appl. Polym. Sci. 1991, 42, 1711–1720. [Google Scholar] [CrossRef]
- Michaels, A.S.; Bixler, H.J. Solubility of gases in polyethylene. J. Polym. Sci. 1961, 50, 393–412. [Google Scholar] [CrossRef]
- Michaels, A.S.; Parker, R.B., Jr. Sorption and flow of gases in polyethylene. J. Polym. Sci. 1959, 41, 53–71. [Google Scholar] [CrossRef]
- Michaels, A.S.; Vieth, W.R.; Barrie, J.A. Diffusion of gases in polyethylene terephthalate. J. Appl. Phys. 1963, 34, 13–20. [Google Scholar] [CrossRef]
- Lasoski, S.W.; Cobbs, W.H. Moisture permeability of polymers. I. Role of crystallinity and orientation. J. Polym. Sci. 1959, 36, 21–33. [Google Scholar] [CrossRef]
- Park, J.Y.; Paul, D.R. Correlation and prediction of gas permeability in glassy polymer membrane materials via a modified free volume based group contribution method. J. Membr. Sci. 1997, 125, 23–39. [Google Scholar] [CrossRef]
- Wu, A.X.; Lin, S.; Rodriguez, K.M.; Benedetti, F.M.; Joo, T.; Grosz, A.F.; Storme, K.R.; Roy, N.; Syar, D.; Smith, Z.P. Revisiting group contribution theory for estimating fractional free volume of microporous polymer membranes. J. Membr. Sci. 2021, 636, 119526. [Google Scholar] [CrossRef]
- Choudalakis, G.; Gotsis, A.D. Free volume and mass transport in polymer nanocomposites. Curr. Opin. Colloid Interface Sci. 2012, 17, 132–140. [Google Scholar] [CrossRef]



| PE Material | Thickness (mm) | Density (g/cm3) | Crystallinity (%) |
|---|---|---|---|
| LDPE | 1.64 ± 0.03 | 0.91 ± 0.04 | 30 ± 3 |
| 3.24 ± 0.06 | |||
| 4.93 ± 0.10 | |||
| UHMWPE | 1.64 ± 0.08 | 0.94 ± 0.06 | 42 ± 3 |
| 3.24 ± 0.16 | |||
| 4.93 ± 0.25 | |||
| HDPE | 1.64 ± 0.03 | 0.97 ± 0.04 | 62 ± 6 |
| 3.24 ± 0.06 | |||
| 4.93 ± 0.10 |
| Sample | Diffusivity [×10−11 m2/s] | Solubility [mol/m3∙MPa] | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| H2 | He | N2 | O2 | Ar | H2 | He | N2 | O2 | Ar | |
| LDPE | 33.4 | 58.4 | 2.59 | 3.87 | 3.42 | 6.77 | 2.50 | 8.32 | 16.0 | 19.9 |
| UHMWPE | 23.3 | 55.9 | 1.78 | 2.26 | 2.36 | 7.04 | 2.30 | 8.22 | 14.4 | 19.7 |
| HDPE | 22.5 | 45.7 | 1.53 | 2.02 | 1.99 | 4.43 | 1.62 | 5.30 | 10.1 | 12.4 |
| Gas | H2 | He | N2 | O2 | Ar |
|---|---|---|---|---|---|
| Kinetic diameter [nm] | 0.289 | 0.260 | 0.364 | 0.346 | 0.343 |
| Critical temperature [K] | 32.98 | 3.35 | 126.19 | 154.58 | 150.70 |
| Volume Fraction of Amorphous Phase | Fractional Free Volume | ||||
|---|---|---|---|---|---|
| Gas(j) | Da,j [×10−11 m2/s] | Sa,j [mol/m3∙MPa] | Pa,j [×10−10 mol/m∙s∙MPa] | PFFV,j [×10−10 mol/m∙s∙MPa] | Bj |
| H2 | 43.4 ± 3.8 | 10.1 ± 0.7 | 42.2 ± 2.3 | 7573 ± 1599 | 1.94 ± 0.07 |
| He | 86.8 ± 6.8 | 3.54 ± 0.11 | 29.5 ± 2.8 | 4229 ± 2870 | 1.86 ± 0.24 |
| N2 | 3.27 ± 0.23 | 12.1 ± 0.23 | 3.88 ± 0.13 | 826.0 ± 142.9 | 2.00 ± 0.06 |
| O2 | 4.57 ± 0.53 | 22.4 ± 0.5 | 10.3 ± 1.0 | 2859 ± 1610 | 2.09 ± 0.21 |
| Ar | 4.31 ± 0.30 | 28.7 ± 1.4 | 12.2 ± 0.3 | 2611 ± 633 | 2.01 ± 0.09 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, J.-H.; Kim, Y.-W.; Jung, J.-K. Investigation of the Gas Permeation Properties Using the Volumetric Analysis Technique for Polyethylene Materials Enriched with Pure Gases under High Pressure: H2, He, N2, O2 and Ar. Polymers 2023, 15, 4019. https://doi.org/10.3390/polym15194019
Lee J-H, Kim Y-W, Jung J-K. Investigation of the Gas Permeation Properties Using the Volumetric Analysis Technique for Polyethylene Materials Enriched with Pure Gases under High Pressure: H2, He, N2, O2 and Ar. Polymers. 2023; 15(19):4019. https://doi.org/10.3390/polym15194019
Chicago/Turabian StyleLee, Ji-Hun, Ye-Won Kim, and Jae-Kap Jung. 2023. "Investigation of the Gas Permeation Properties Using the Volumetric Analysis Technique for Polyethylene Materials Enriched with Pure Gases under High Pressure: H2, He, N2, O2 and Ar" Polymers 15, no. 19: 4019. https://doi.org/10.3390/polym15194019
APA StyleLee, J.-H., Kim, Y.-W., & Jung, J.-K. (2023). Investigation of the Gas Permeation Properties Using the Volumetric Analysis Technique for Polyethylene Materials Enriched with Pure Gases under High Pressure: H2, He, N2, O2 and Ar. Polymers, 15(19), 4019. https://doi.org/10.3390/polym15194019






