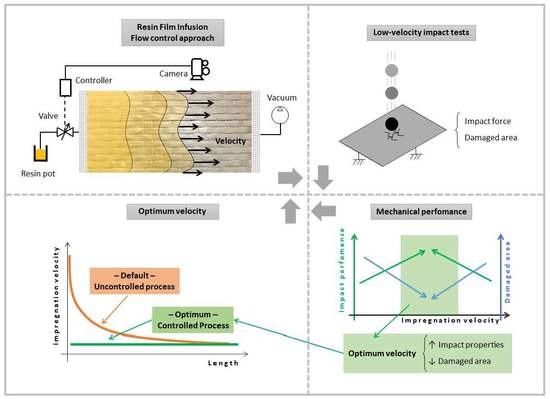
Enhanced Low-Velocity Impact Properties for Resin Film Infusion-Manufactured Composites by Flow-Control Approach
Abstract
1. Introduction
2. Materials and Methods
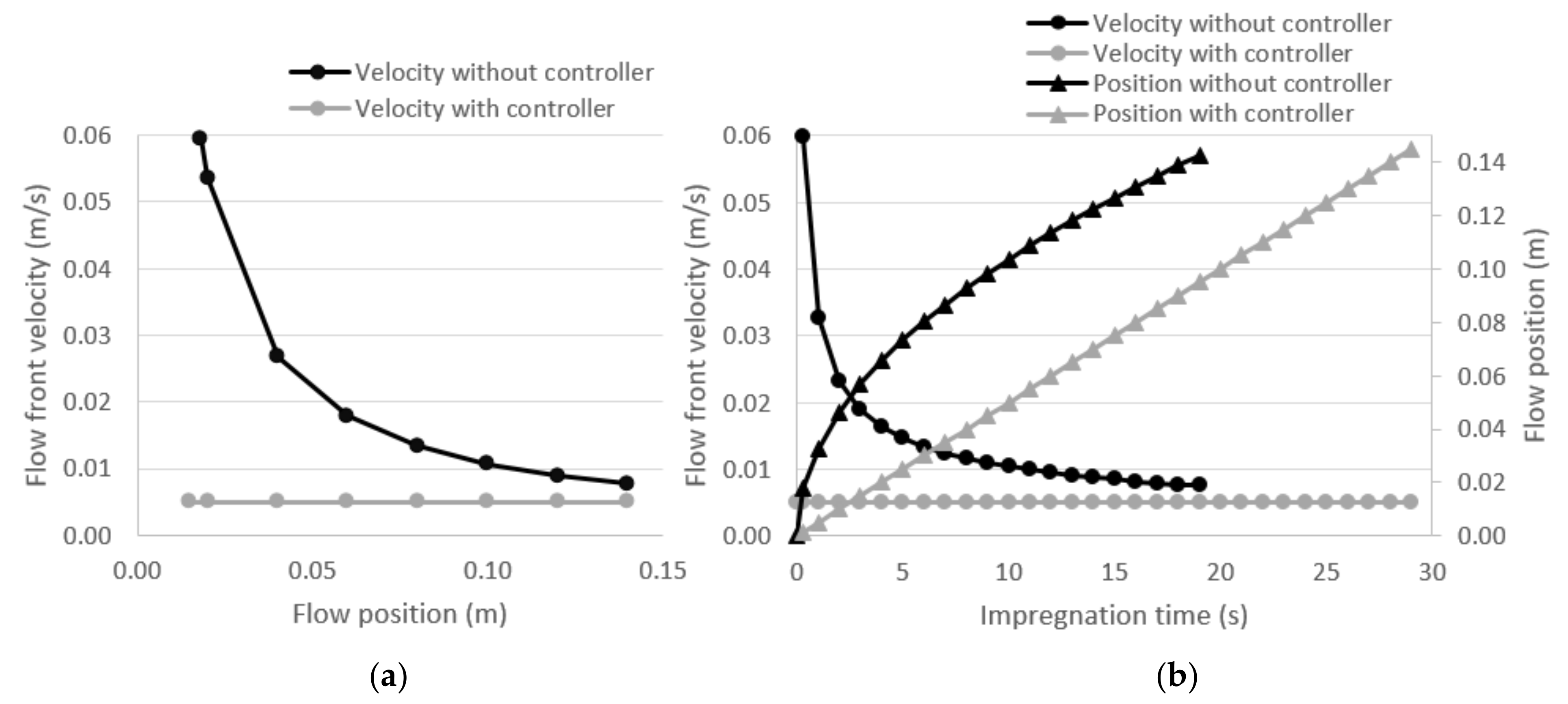
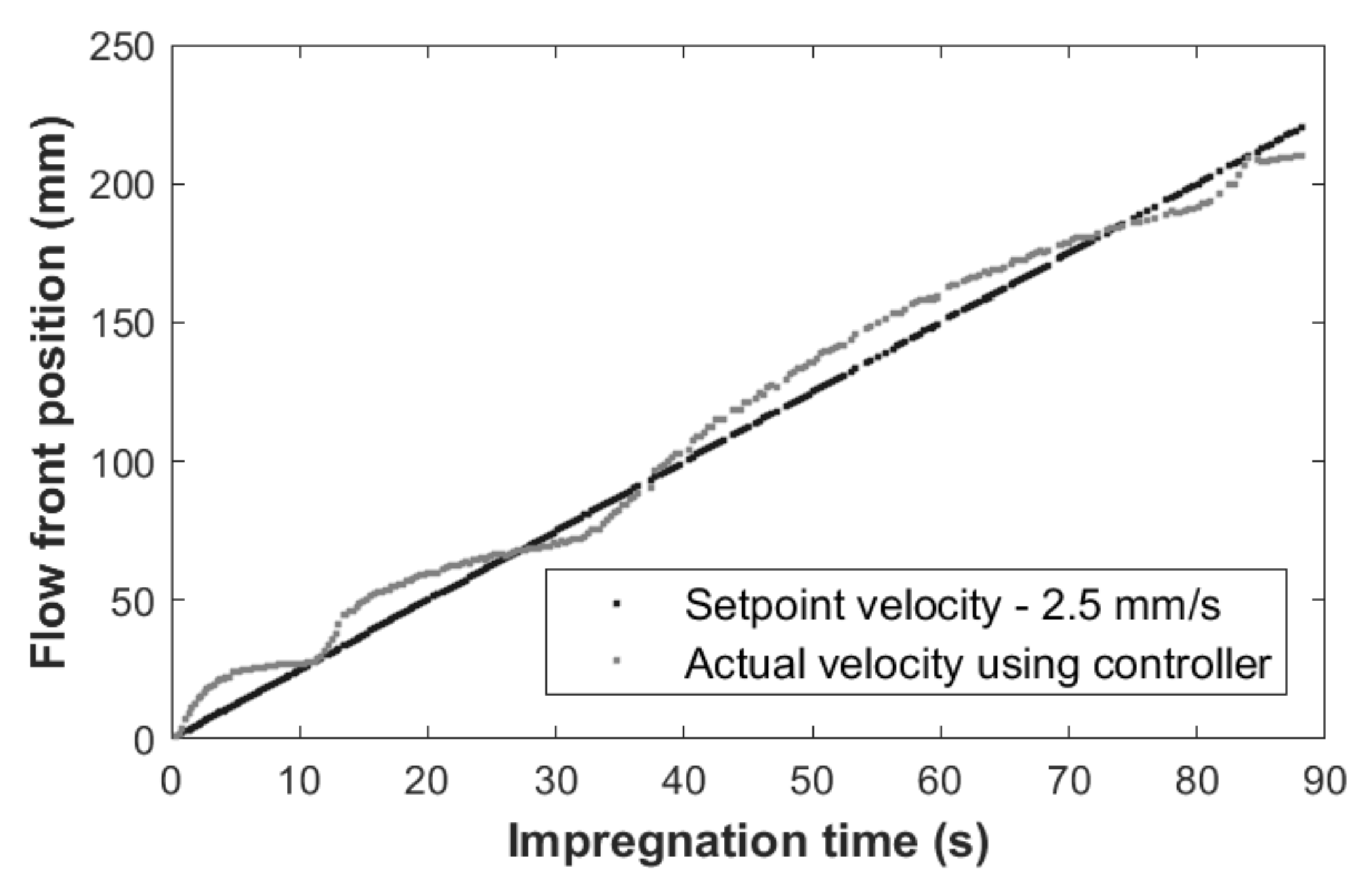
2.1. Flow Front Controller
2.2. Specimen Manufacturing
- -
- Four groups of four specimens [02, 90]S without stitching at constant impregnation velocity;
- -
- Four groups of four specimens [902, 0]S with stitching at constant impregnation velocity;
- -
- Four group of four specimens [902, 0]S without stitching and manufactured at constant and optimum impregnation velocity, obtained from the previous specimen analysis.
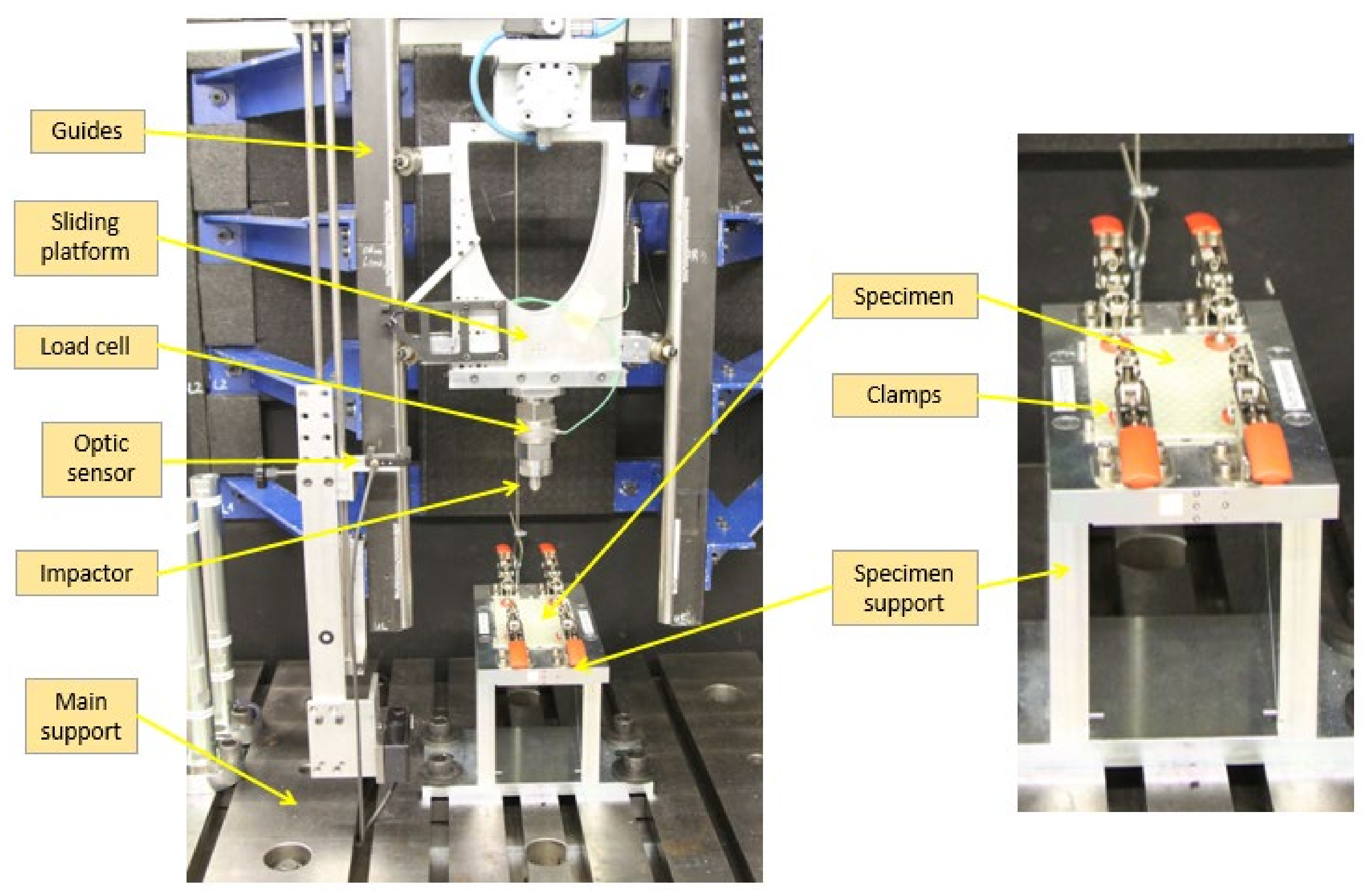
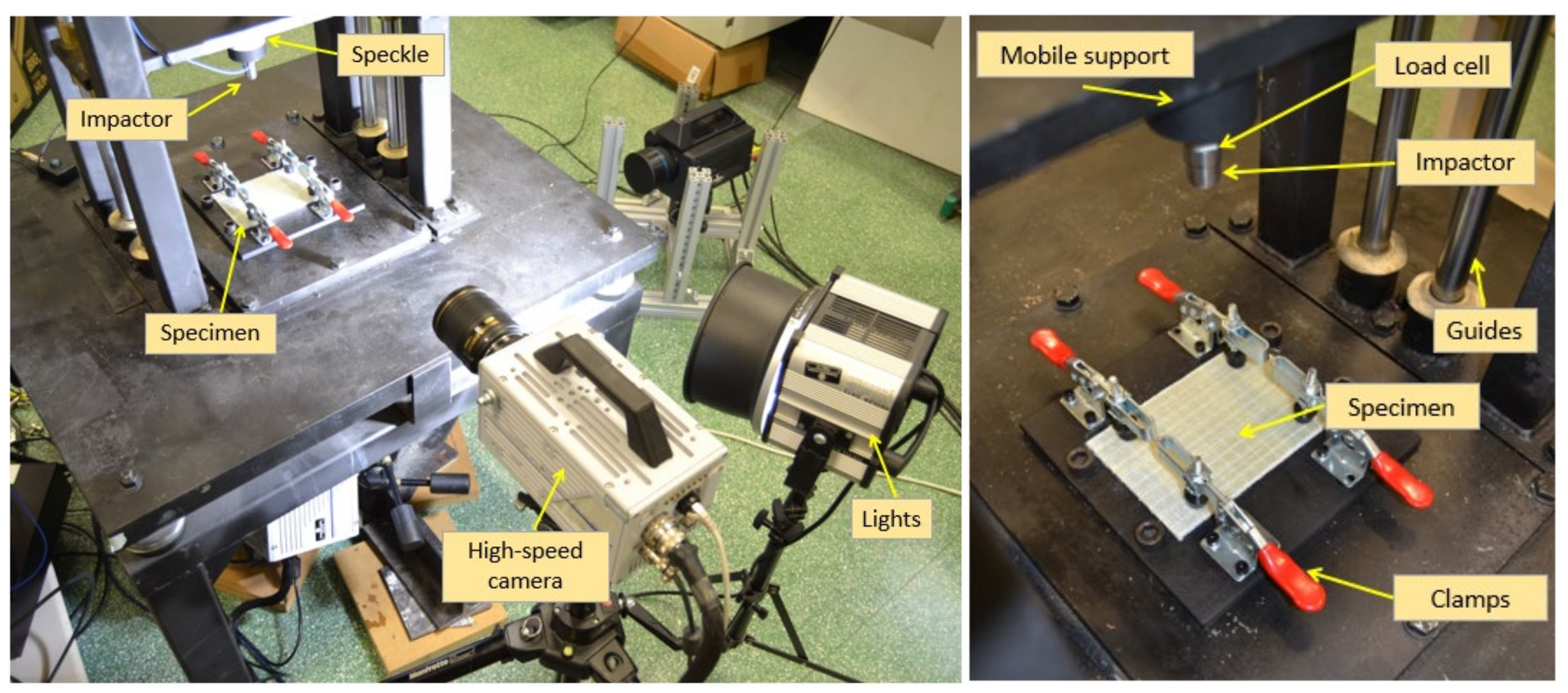
2.3. Testing Procedure
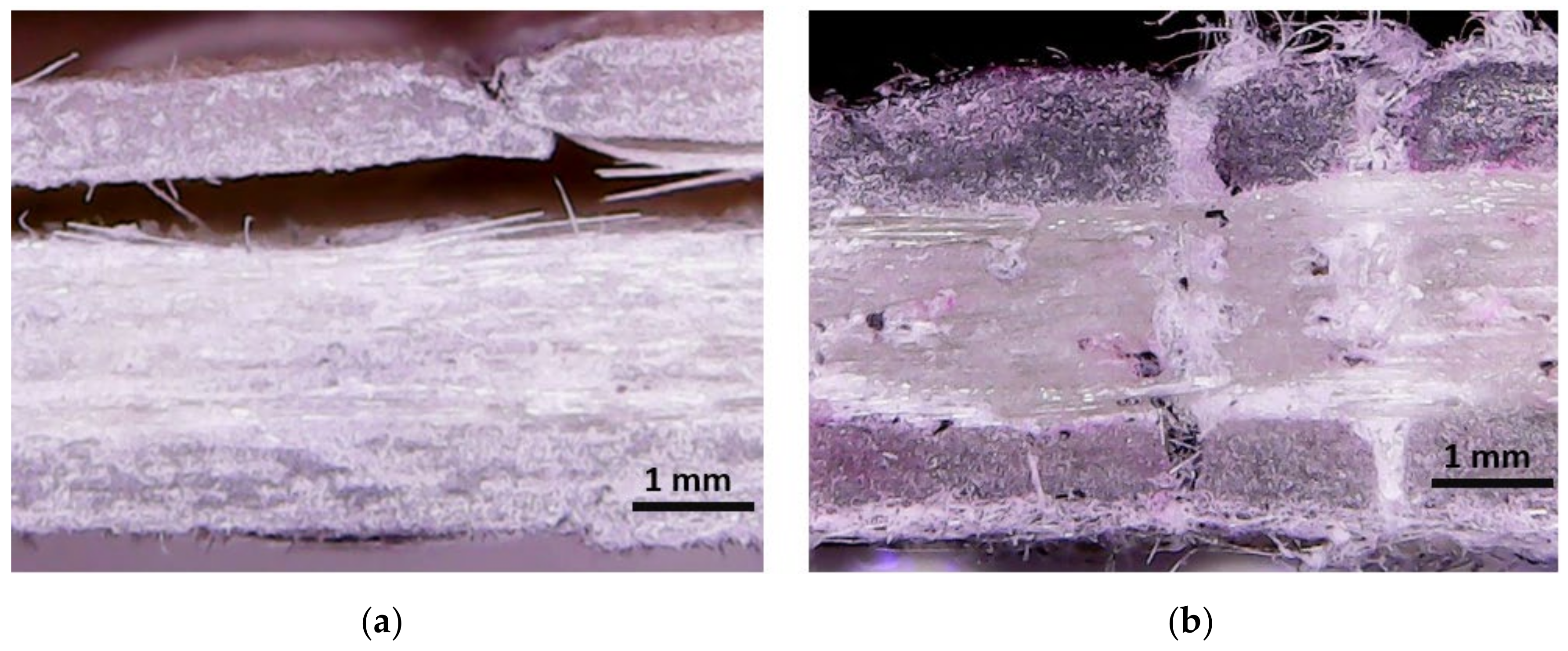
3. Results and Discussion
4. Conclusions
- Impact properties were improved in both stitched and unstitched laminates by optimizing the flow front velocity. The peak force during the impact event and the damaged area were analyzed at two energy levels;
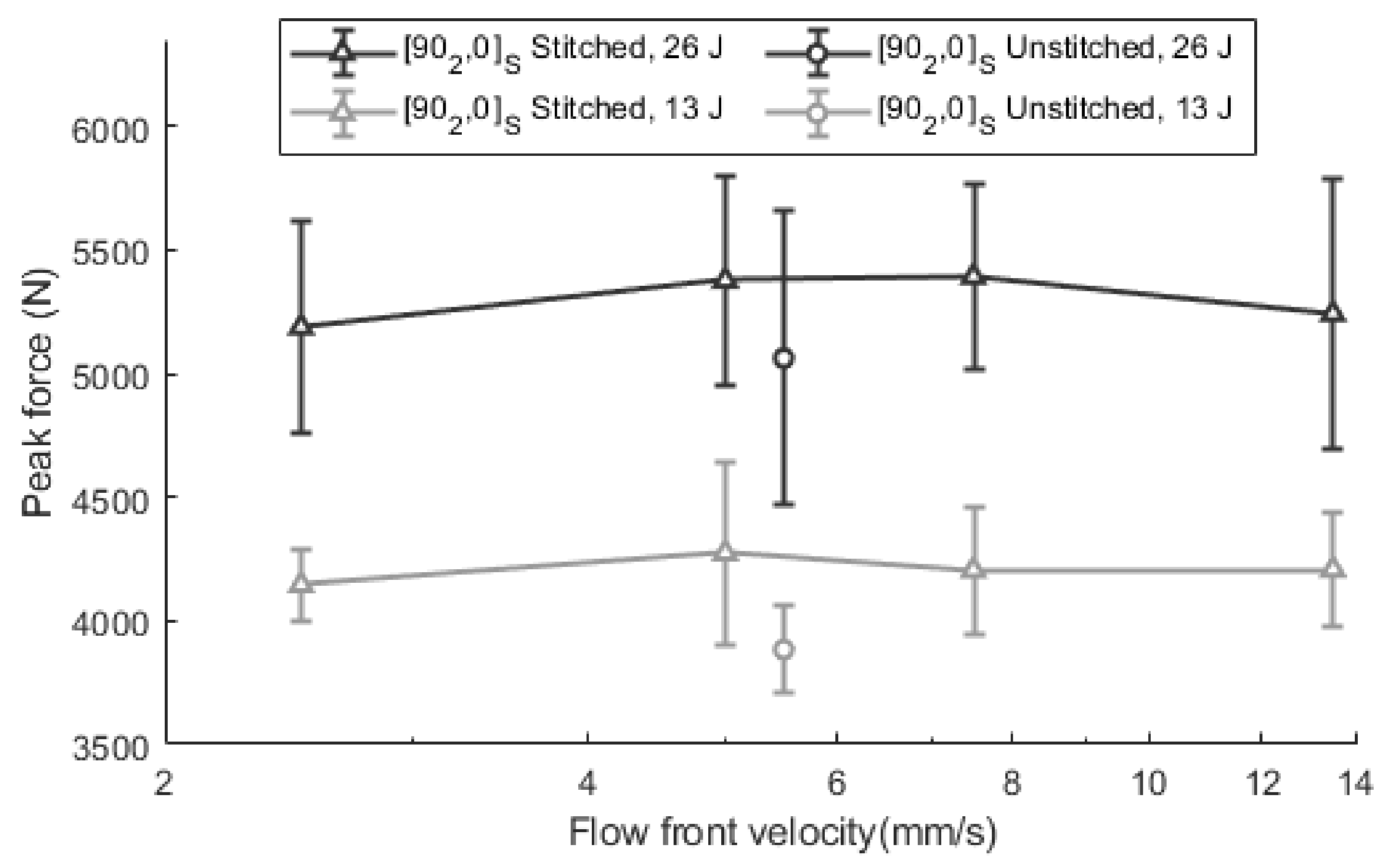
- When the peak forces are analyzed, the same optimum range of impregnation velocities is concluded for different fiber orientations. No significant differences were found in the optimum velocity for unstitched and stitched laminates. For high energies, significant increases in the peak force up to 14.9% were observed. Minor improvements were reported for low impact energies, because the effect of voids during the crack propagation was also reduced. In all cases, the range for optimum velocities was set between 5 mm/s and 7.5 mm/s;
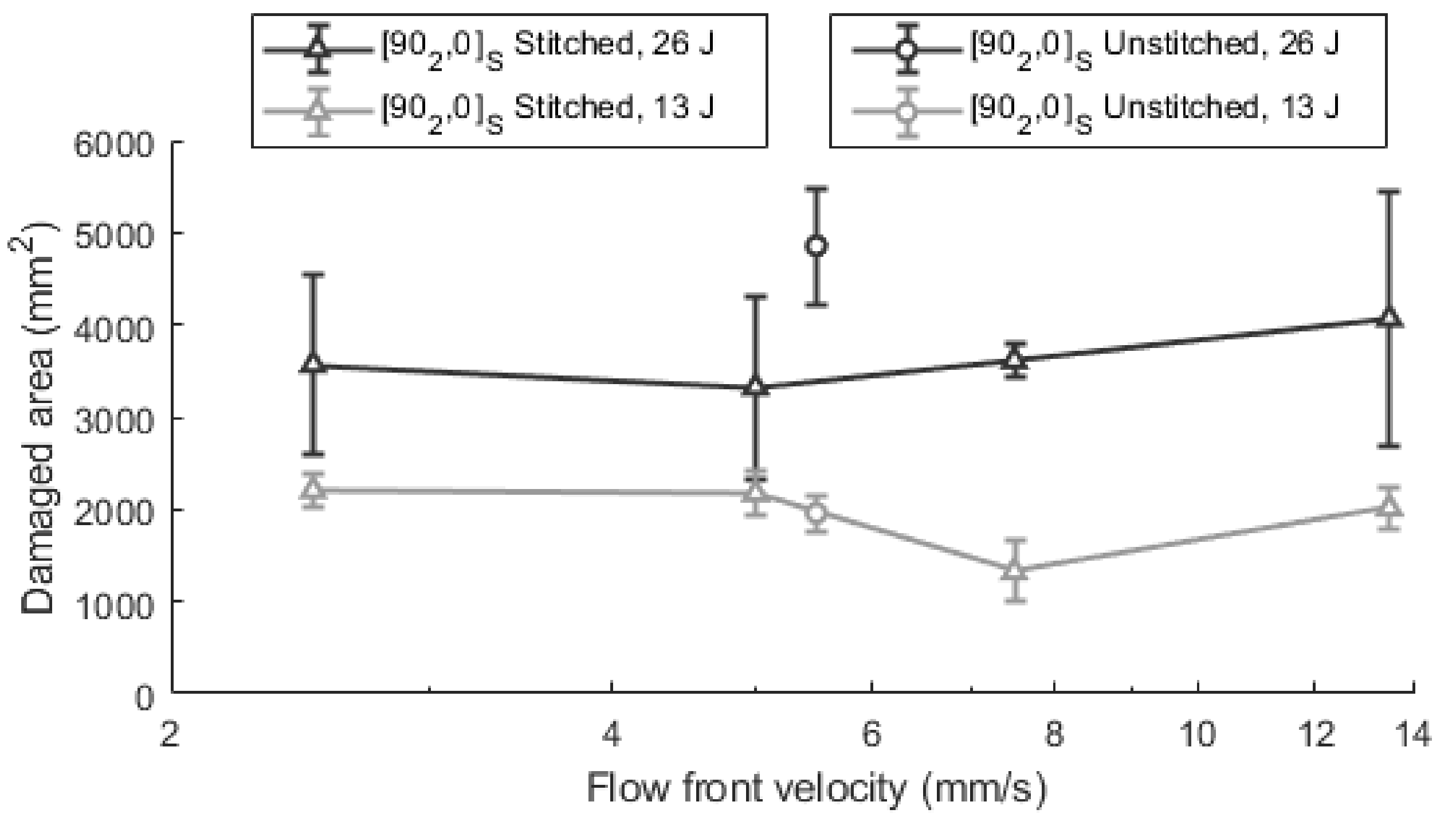
- Damaged area analysis supports the peak force analysis conclusions, and the same optimum range was deduced and statistically significant. The relative reduction in the damaged area can reach 40.0%. Then, the “process window” for these materials can be set between 5 mm/s and 7.5 mm/s;
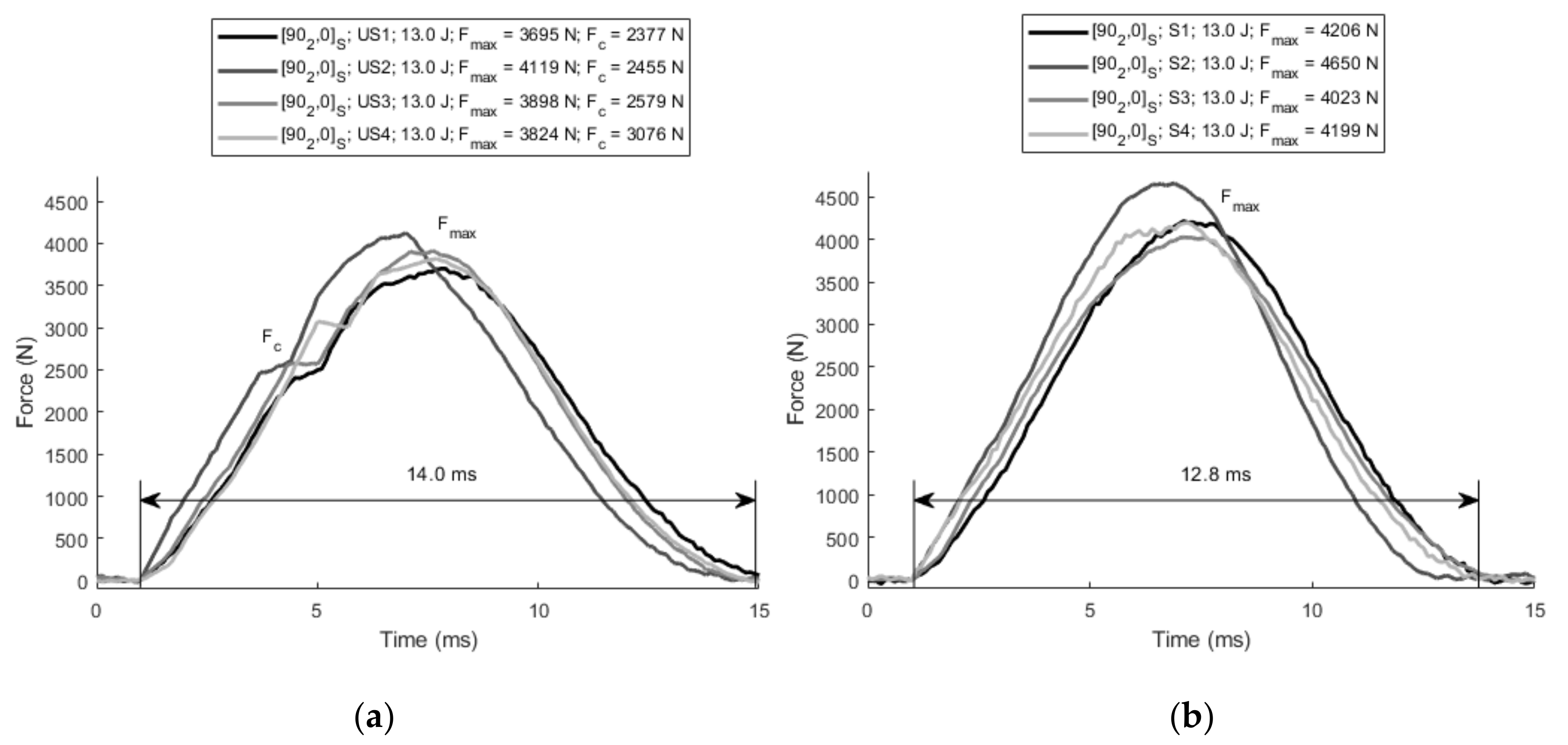
- At optimum values, stitched laminates reported the highest impact properties as well as the minimum damaged area. While unstitched specimens showed critical impacts, subcritical impacts were observed in stitched cases;
- During the impregnation of stitched laminates, several voids and empty areas were observed around the stitch point. These may be associated with the use of high vacuum pressures and further analysis is required;
- Stitching does not affect the delaminations for low impact energies. For high impact energies, the average delaminated area can be reduced by 31.6% if stitching is added. In this sense, stitching is highly effective for high energy levels. In these cases, stitch points prevent the propagation of delamination.
Author Contributions
Funding
Institutional Review Board Statement
Acknowledgments
Conflicts of Interest
References
- Slayton, R.; Spinardi, G. Radical innovation in scaling up: Boeing’s Dreamliner and the challenge of socio-technical transitions. Technovation 2016, 47, 47–58. [Google Scholar] [CrossRef]
- Roth, Y.C.; Weinholdt, M.; Winkelmann, L. Liquid Composite Moulding—Enabler for the Automated Production or CFRP Aircraft Components. In Proceedings of the ECCM16—16th European Conference on Composite Materials, Seville, Spain, 22–26 June 2014; pp. 1–8. [Google Scholar]
- Poorzeinolabedin, M.; Parnas, L.; Dashatan, S.H. Resin infusion under flexible tooling process and structural design optimization of the complex composite part. Mater. Des. 2014, 64, 450–455. [Google Scholar] [CrossRef]
- Alampalli, S.; O’Connor, J.; Yannotti, A.P. Fiber reinforced polymer composites for the superstructure of a short-span rural bridge. Compos. Struct. 2002, 58, 21–27. [Google Scholar] [CrossRef]
- Pierce, R.S.; Falzon, B.G. Injection repair of composites for automotive and aerospace applications. In Proceedings of the 21st International Conference on Composite Materials, Xi’an, China, 20–25 August 2017; pp. 20–25. [Google Scholar]
- Hadigheh, S.A.; Gravina, R.J.; Setunge, S.; Kim, S.J. Bond characterization of adhesively bonded joints made with the resin infusion (RI) process. Int. J. Adhes. Adhes. 2014, 57, 13–21. [Google Scholar] [CrossRef]
- Almazán-Lázaro, J.-A.; López-Alba, E.; Díaz-Garrido, F.-A. Improving Composite Tensile Properties during Resin Infusion Based on a Computer Vision Flow-Control Approach. Materials 2018, 11, 2469. [Google Scholar] [CrossRef] [PubMed]
- Ravey, C.; LeBel, F.; Ruiz, E.; Trochu, F. Void minimization and optimization of injection velocity in RTM processing. In Proceedings of the 19th International Conference on Composite Materials, Montreal, QC, Canada, 28 July–2 August 2013; pp. 1–10. [Google Scholar]
- Rimmel, O.; May, D.; Mitschang, P. Impact of Stitching on Permeability and Mechanical Properties of Preforms Manufactured by Dry Fiber Placement. Polym. Compos. 2019, 40, 1631–1642. [Google Scholar] [CrossRef]
- LeBel, F.; Fanaei, A.E.; Ruiz, E.; Trochu, F. Prediction of optimal flow front velocity to minimize void formation in dual scale fibrous reinforcements. Int. J. Mater. Form. 2014, 7, 93–116. [Google Scholar] [CrossRef]
- LeBel, F.; Ruiz, É.; Trochu, F. Void content analysis and processing issues to minimize defects in liquid composite molding. Polym. Compos. 2019, 40, 109–120. [Google Scholar] [CrossRef]
- Gourichon, B.; Binétruy, C.; Krawczak, P. A new numerical procedure to predict dynamic void content in liquid composite molding. Compos. Part A Appl. Sci. Manuf. 2006, 37, 1961–1969. [Google Scholar] [CrossRef]
- Park, C.H.; Lebel, A.; Saouab, A.; Bréard, J.; Lee, W. Il Modeling and simulation of voids and saturation in liquid composite molding processes. Compos. Part A Appl. Sci. Manuf. 2011, 42, 658–668. [Google Scholar] [CrossRef]
- Ghiorse, S.R. Effect of void content on the mechanical properties of carbon/epoxy laminates. SAMPE Q. 1993, 24, 54–59. [Google Scholar]
- Leclerc, J.S.; Ruiz, E. Porosity reduction using optimized flow velocity in Resin Transfer Molding. Compos. Part A Appl. Sci. Manuf. 2008, 39, 1859–1868. [Google Scholar] [CrossRef]
- Andraju, L.B.; Ramji, M.; Raju, G. Snap-buckling and failure studies on CFRP laminate with an embedded circular delamination under flexural loading. Compos. Part B Eng. 2021, 214, 108739. [Google Scholar] [CrossRef]
- Gemi, D.S.; Şahin, Ö.S.; Gemi, L. Experimental investigation of the effect of diameter upon low velocity impact response of glass fiber reinforced composite pipes. Compos. Struct. 2021, 275, 114428. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, J.; Fang, G.; Zhang, J.; Zhou, Z.; Wang, S. Influence of temperature on the impact behavior of woven-ply carbon fiber reinforced thermoplastic composites. Compos. Struct. 2018, 185, 435–445. [Google Scholar] [CrossRef]
- Andrew, J.J.; Srinivasan, S.M.; Arockiarajan, A.; Dhakal, H.N. Parameters influencing the impact response of fiber-reinforced polymer matrix composite materials: A critical review. Compos. Struct. 2019, 224, 111007. [Google Scholar] [CrossRef]
- Alam, S.; Yandek, G.; Lee, R.C.; Mabry, J. A study of residual burst strength of composite over wrapped pressure vessel due to low velocity impact. Int. J. Press. Vessel. Pip. 2021, 194, 104511. [Google Scholar] [CrossRef]
- Atas, C.; Icten, B.M.; Küçük, M. Thickness effect on repeated impact response of woven fabric composite plates. Compos. Part B Eng. 2013, 49, 80–85. [Google Scholar] [CrossRef]
- Vitiello, L.; Russo, P.; Papa, I.; Lopresto, V.; Mocerino, D.; Filippone, G. Flexural Properties and Low-Velocity Impact Behavior of Polyamide 11/Basalt Fiber Fabric Laminates. Polymers 2021, 13, 1055. [Google Scholar] [CrossRef] [PubMed]
- Sreekantha Reddy, T.; Rama Subba Reddy, P.; Madhu, V. Low velocity impact studies of E-glass/epoxy composite laminates at different thicknesses and temperatures. Def. Technol. 2019, 15, 897–904. [Google Scholar] [CrossRef]
- Shyr, T.; Pan, Y. Impact resistance and damage characteristics of composite laminates. Compos. Struct. 2003, 62, 193–203. [Google Scholar] [CrossRef]
- Robinson, P.; Greenhalgh, E.; Silvestre, P. Failure Mechanisms in Polymer Matrix Composites. Criteria, Testing and Industrial Applications; Woodhead Publishing Limited: Cambridge, UK, 2012; ISBN 978-1-84569-750-1. [Google Scholar]
- Kumari, P.; Wang, J. Saahil Residual Tensile Strength of the Multi-Impacted Scarf-Repaired Glass Fiber-Reinforced Polymer (GFRP) Composites. Materials 2018, 11, 2351. [Google Scholar] [CrossRef]
- Tan, K.T.; Watanabe, N.; Iwahori, Y.; Ishikawa, T. Understanding effectiveness of stitching in suppression of impact damage: An empirical delamination reduction trend for stitched composites. Compos. Part A Appl. Sci. Manuf. 2012, 43, 823–832. [Google Scholar] [CrossRef]
- Aymerich, F.; Francesconi, L. Damage Mechanisms in Thin Stitched Laminates Subjected to Low-velocity Impact. Procedia Eng. 2014, 88. [Google Scholar] [CrossRef]
- Rieber, G.; Mitschang, P. Full Characterization of a Stitched Twill Weave Textile by Unsaturated 2-D and 3-D Permeability Measurements. In Proceedings of the 17th International Conference on Composite Materials, ICCM-17, Edinburgh, Scotland, 27–31 July 2009. [Google Scholar]
- Reid, S.R.; Zhou, G. Impact Behaviour of Fibre-Reinforced Composite Materials and Structures; Elsevier: Amsterdam, The Netherlands, 2000; ISBN 084930847X. [Google Scholar]
- Feraboli, P.; Kedward, K.T. Enhanced Evaluation of the Low-Velocity Impact Response of Composite Plates. AIAA J. 2015. [Google Scholar] [CrossRef]
- Van Oosterom, S.; Schreier, A.; Battley, M.; Bickerton, S.; Allen, T. Influence of dissolved gasses in epoxy resin on resin infusion part quality. Compos. Part A Appl. Sci. Manuf. 2020, 132, 105818. [Google Scholar] [CrossRef]
- Tong, L.; Mouritz, A.P.; Bannister, M.K. 3D Fibre Reinforced Polymer Composites, 1st ed.; Elsevier: Oxford, UK, 2002; ISBN 0-08-043938-1. [Google Scholar]
- ASTM International ASTM D7136/D7136M. Standard Test Method for Measuring the Damage Resistance of a Fiber-Reinforced Polymer Matrix Composite to a Drop-Weight Impact Event; ASTM International: West Conshohocken, PE, USA, 2012; Volume I. [Google Scholar]
- Sutherland, L.S.; Soares, C.G. Impact characterisation of low fibre-volume glass reinforced polyester circular laminated plates. Int. J. Impact Eng. 2005, 31, 1–23. [Google Scholar] [CrossRef]
- Butterworth, S. On the Theory of Filter Amplifiers. Exp. Wirel. Wirel. Eng. 1930, 7, 536–541. [Google Scholar]
- Palanivelu, S.; De Pauw, S.; Van Paepegem, W.; Degrieck, J.; Ackeren, J.; Kakogiannis, D.; Wastiels, J.; Hemelrijck, D.; Vantomme, J. Validation of digital image correlation technique for impact loading applications. Proc. DYMAT 2009, 373–379. [Google Scholar] [CrossRef]
- Reddy, T.S.; Reddy, P.R.S.; Madhu, V. Response of E-glass/Epoxy and Dyneema Composite Laminates Subjected to low and High Velocity Impact. Procedia Eng. 2017, 173, 278–285. [Google Scholar] [CrossRef]
- Gonzalez, R.C.; Woods, R.; Steven, L.E. Digital Image Processing Using MATLAB; Pearson Prentice Hall: Hoboken, NJ, USA, 2003; ISBN 978-0130085191. [Google Scholar]
- Yang, G.; Xu, F. Research and analysis of Image edge detection algorithm Based on the MATLAB. Procedia Eng. 2011, 15, 1313–1318. [Google Scholar] [CrossRef][Green Version]
- Muruganadhan, R. Velamurali Low Velocity Impact Analysis on Stitched and Unstitched Laminates. Asian J. Res. Soc. Sci. Humanit. 2016, 6, 752. [Google Scholar] [CrossRef]
- Ricotta, M.; Quaresimin, M.; Talreja, R. Mode I Strain Energy Release Rate in composite laminates in the presence of voids. Compos. Sci. Technol. 2008, 68, 2616–2623. [Google Scholar] [CrossRef][Green Version]
- Kharazan, M.; Sadr, M.H.; Kiani, M. Delamination Growth Analysis in Composite laminates Subjected to Low Velocity Impact. Steel Compos. Struct. 2014, 17, 307–403. [Google Scholar] [CrossRef]
- Tan, K.T.; Watanabe, N.; Iwahori, Y. Effect of stitch density and stitch thread thickness on low-velocity impact damage of stitched composites. Compos. Part A Appl. Sci. Manuf. 2010, 41, 1857–1868. [Google Scholar] [CrossRef]




| Unstitched Laminates [02, 90]S | Stitched Laminates [902, 0]S | ||
|---|---|---|---|
| Group—Velocity | Target Velocity (mm/s) | Group—Velocity | Target Velocity (mm/s) |
| US—V1 | 2.5 | S—V1 | 2.5 |
| US—V2 | 4.0 | S—V2 | 5.0 |
| US—V3 | 6.0 | S—V3 | 7.5 |
| US—V4 | 11.0 | S—V4 | 13.5 |
| US—VO | 5.5 | ||
| Ref. | Target Velocity (mm/s) | Real Velocity (mm/s) |
|---|---|---|
| US—V1 | 2.5 | 2.5 ± 0.7 |
| US—V2 | 4.0 | 4.1 ± 0.5 |
| US—V3 | 6.0 | 5.9 ± 1.8 |
| US—V4 | 11.0 | 11.3 ± 0.5 |
| S—V1 | 2.5 | 2.5 ± 0.7 |
| S—V2 | 5.0 | 5.2 ± 0.7 |
| S—V3 | 7.5 | 7.3 ± 1.6 |
| S—V4 | 13.5 | 13.7 ± 0.7 |
| US—VO | 5.5 | 5.6 ± 0.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almazán-Lázaro, J.-A.; López-Alba, E.; Schmeer, S.; Díaz-Garrido, F.-A. Enhanced Low-Velocity Impact Properties for Resin Film Infusion-Manufactured Composites by Flow-Control Approach. Polymers 2021, 13, 3431. https://doi.org/10.3390/polym13193431
Almazán-Lázaro J-A, López-Alba E, Schmeer S, Díaz-Garrido F-A. Enhanced Low-Velocity Impact Properties for Resin Film Infusion-Manufactured Composites by Flow-Control Approach. Polymers. 2021; 13(19):3431. https://doi.org/10.3390/polym13193431
Chicago/Turabian StyleAlmazán-Lázaro, Juan-Antonio, Elías López-Alba, Sebastian Schmeer, and Francisco-Alberto Díaz-Garrido. 2021. "Enhanced Low-Velocity Impact Properties for Resin Film Infusion-Manufactured Composites by Flow-Control Approach" Polymers 13, no. 19: 3431. https://doi.org/10.3390/polym13193431
APA StyleAlmazán-Lázaro, J.-A., López-Alba, E., Schmeer, S., & Díaz-Garrido, F.-A. (2021). Enhanced Low-Velocity Impact Properties for Resin Film Infusion-Manufactured Composites by Flow-Control Approach. Polymers, 13(19), 3431. https://doi.org/10.3390/polym13193431