A Review on Mechanical Models for Cellular Media: Investigation on Material Characterization and Numerical Simulation
Abstract
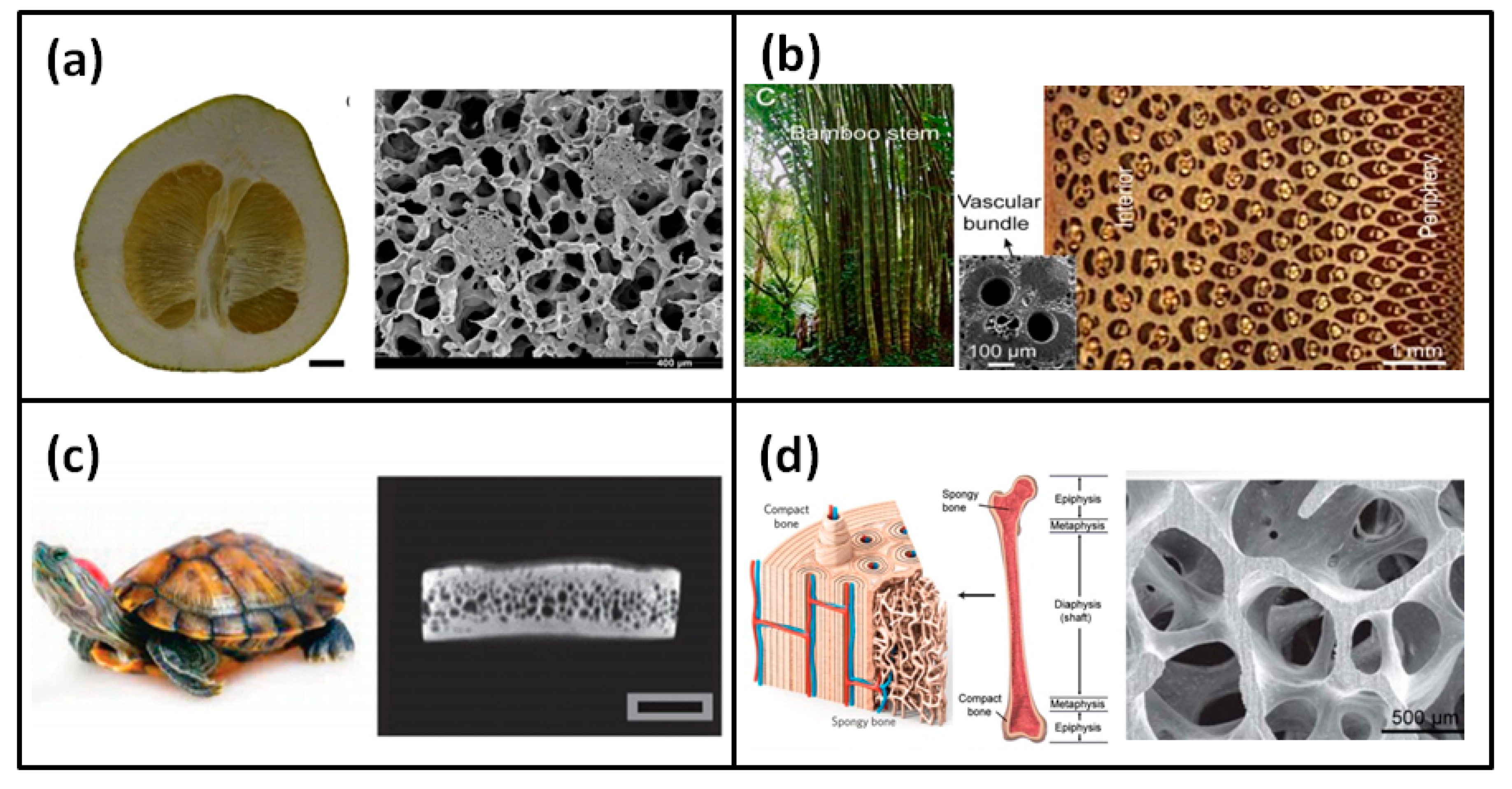
:1. Introduction
2. Characterization of Cellular Medium Mechanical Properties
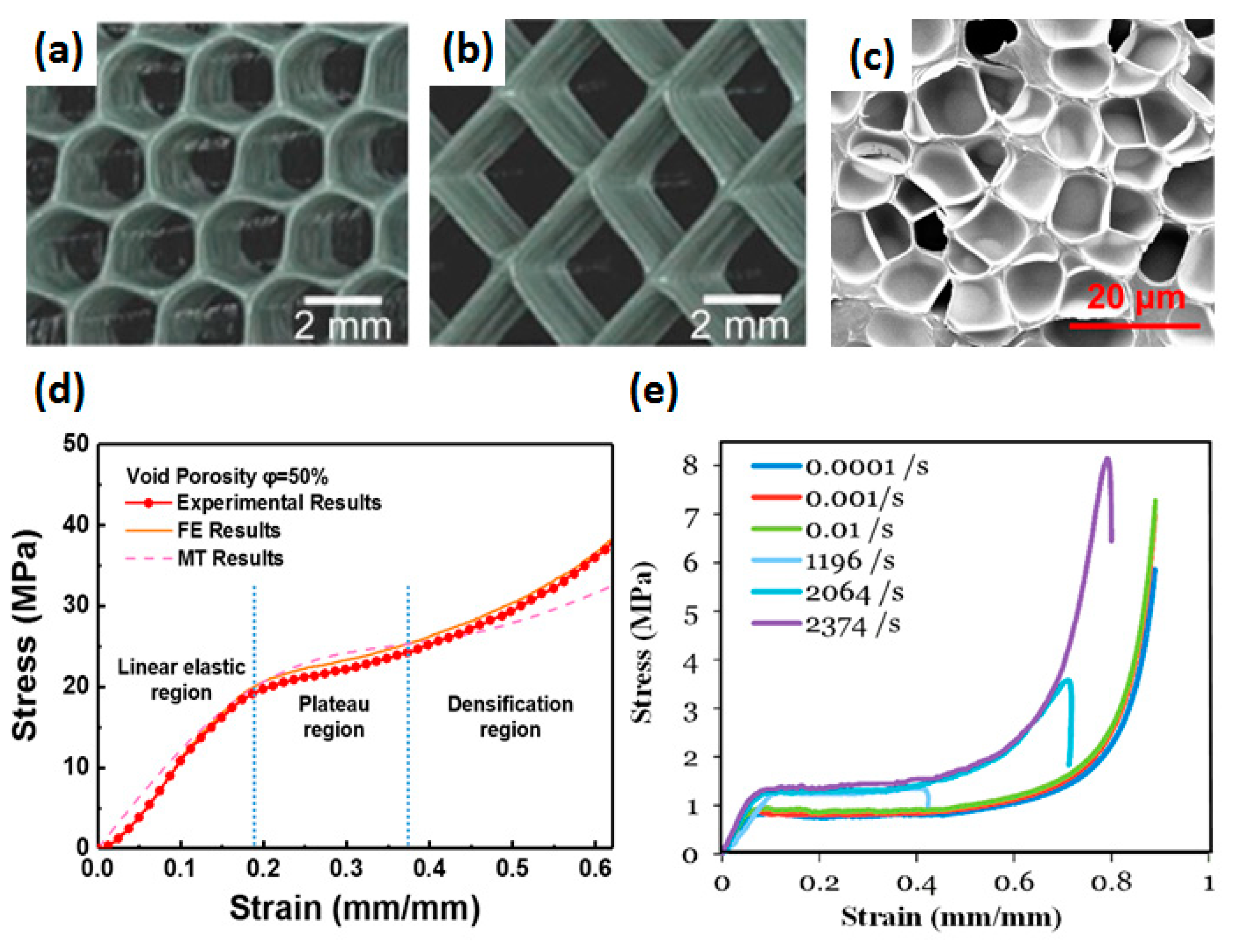
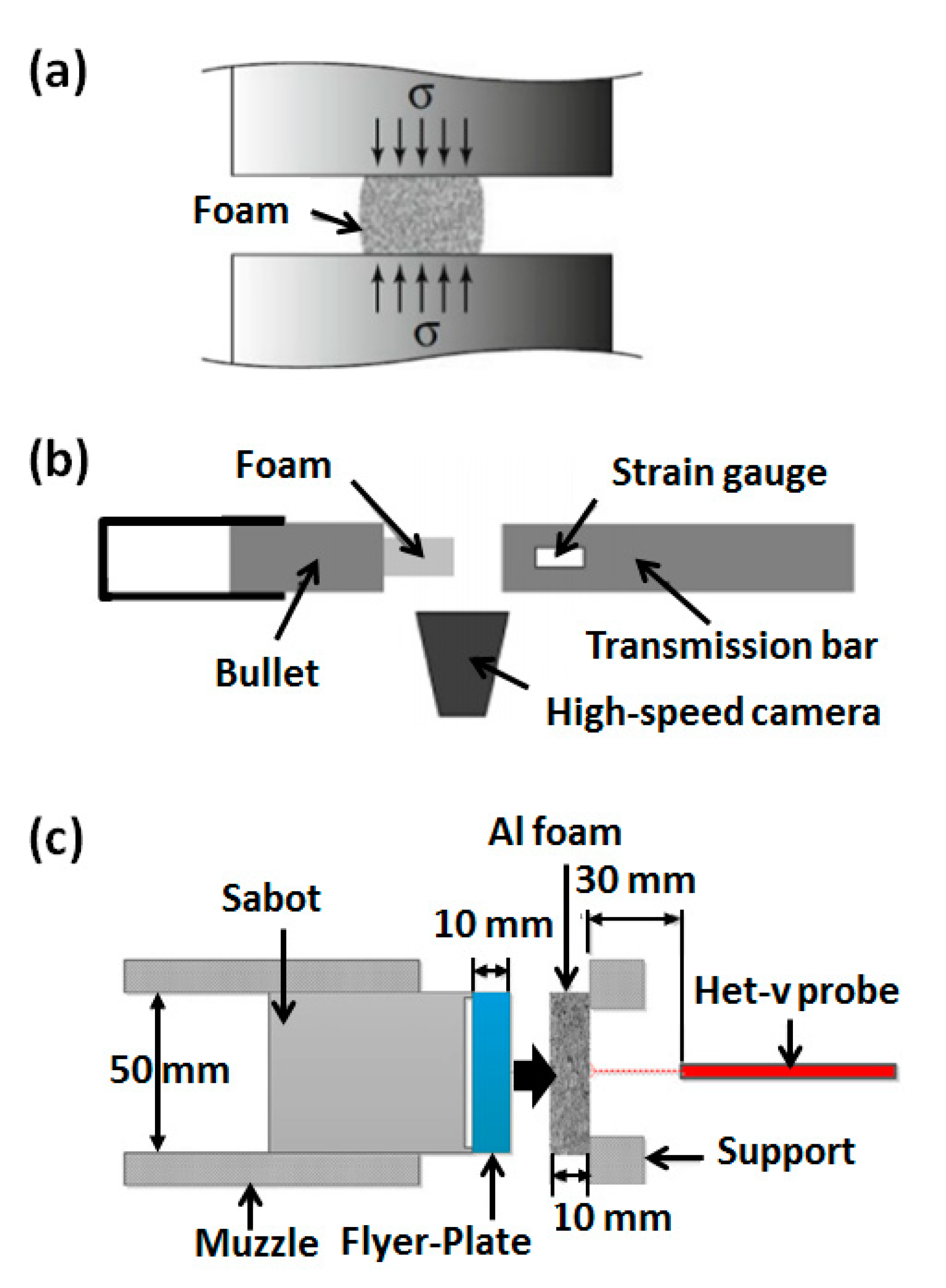
2.1. Quasi-Static Mechanical Properties
2.2. Dynamic Mechanical Properties
3. The Constitutive Relationship of Cellular Medium
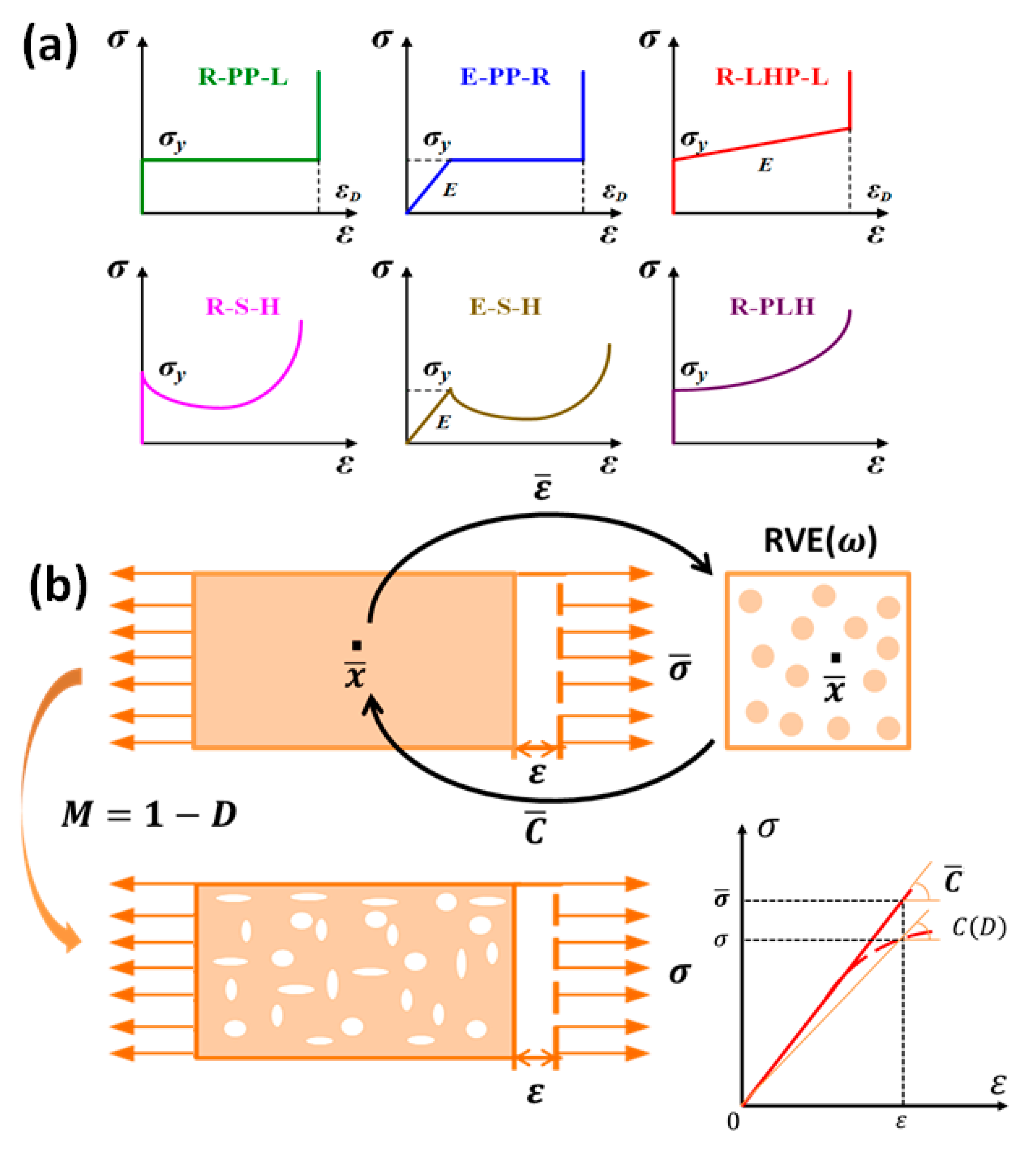
3.1. Phenomenological Constitutive Model
3.2. Homogenization Algorithm Model
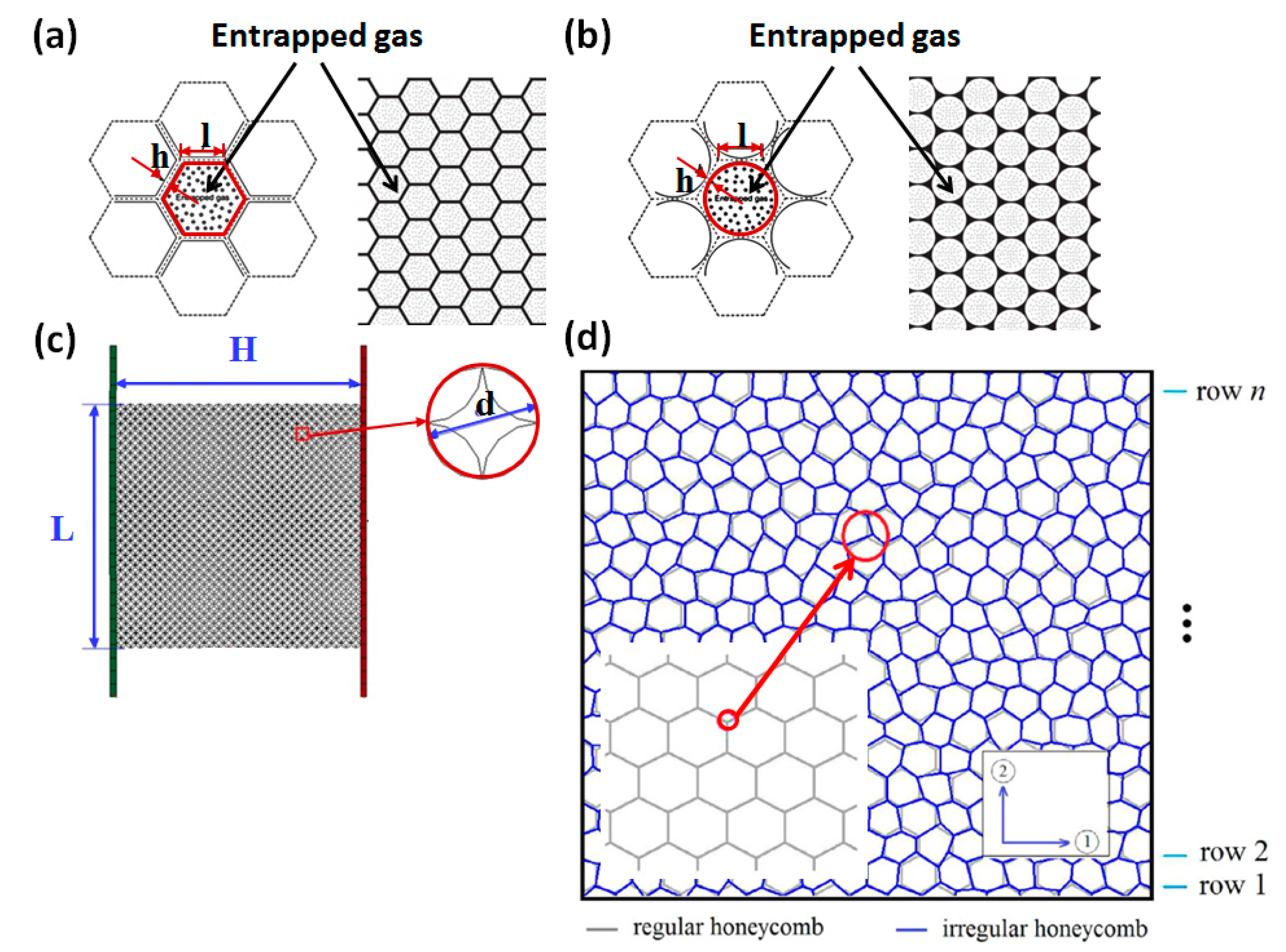
4. Microstructure Mechanical Models of Cellular Medium
4.1. Single Cell Model
4.2. Multi-Cell Model
5. Discussion
- The effects of cell size, matrix, relative density, strain rate and testing methods on the mechanical properties of cellular media were systematically investigated through quasi-static mechanical testing and dynamic mechanical testing. The investigation on mechanical properties of cellular media under explosive loading and ultra-high strain rates involves complex stress waves, and systematic analysis methods and influence laws have not yet been obtained.
- The constitutive model can quickly and accurately describe the stress-strain relationship of a cellular medium, and obtain mechanical parameters (elastic modulus, plateau stress, densification strain, etc.). However, it cannot explain the microstructure deformation mechanism during stretching or compressing. The constitutive model with cell parameters (cell size and cell shape), high strain rate (greater than 104 s−1) and explosive loading needs further study.
- The microstructure model can not only obtain the stress-strain curve, but also explain the microstructure deformation mechanism of cellular media, such as cell deformation, weak parts of the material, stress concentration and local deformation. However, most of the models are based on various algorithms and describe the local microstructure (RVE) of cellular media, which is greatly affected by random factors and is not stable. It is a great challenge to establish a stable, low-cost, real and effective microstructure model of cellular media.
- Molecular dynamics, phase field method and peridynamics can be used to establish nanoscopic models to investigate the structural evolution and properties of molecules and atoms in cellular media. Due to these methods involve nanoscale, simulation results and experimental characterization results are not comparable, and the scale range is small and the calculation amount is large, so they are not introduced in this paper.
- The deformation mode and failure mechanism of cellular media under explosive loading and ultrahigh strain rates, gradient cellular material (gradient mode) structural design and performance optimization [18,57,71,154,155,156,157,158,159], and functional foam composite materials (e.g., doped metal particles, carbon nanotubes, and graphene) [21,160,161,162,163] are the keys to future research on cellular media.
- Expand the mechanical constitutive models of cellular media to suitable for various complex loads, and propose more constitutive models to understand the multifunctional performance of cellular media.
- Multifunctional applications for potential industrial applications, such as: high-performance wave absorption characteristics [175,176,177], electromagnetic interference shielding [162,178,179,180], adsorption thermodynamics and dynamics characteristics [181], and can be used in medical care, satellite communications, electronic equipment, national defense security and other fields in the future [180,182,183,184,185]. And conduct more experiments to verify potential industrial applications.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Naleway, S.E.; Porter, M.; McKittrick, J.; Meyers, M. Structural Design Elements in Biological Materials: Application to Bioinspiration. Adv. Mater. 2015, 27, 5455–5476. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, Z.; Meyers, M.A.; Zhang, Z.; Ritchie, R.O. Functional gradients and heterogeneities in biological materials: Design princi-ples, functions, and bioinspired applications. Prog. Mater. Sci. 2017, 88, 467–498. [Google Scholar] [CrossRef]
- Bührig-Polaczek, A.; Fleck, C.; Speck, T.; Schüler, P.; Fischer, S.F.; Caliaro, M.; Thielen, M. Biomimetic cellular metals—using hierarchical structuring for energy absorption. Bioinspir. Biomim. 2016, 11, 045002. [Google Scholar] [CrossRef] [PubMed]
- Habibi, M.K.; Samaei, A.T.; Gheshlaghi, B.; Lu, J.; Lu, Y. Asymmetric flexural behavior from bamboo’s functionally graded hierarchical structure: Underlying mechanisms. Acta Biomater. 2015, 16, 178–186. [Google Scholar] [CrossRef]
- Wegst, U.G.; Bai, H.; Saiz, E.; Tomsia, A.P.; Ritchie, R.O. Bioinspired structural materials. Nat. Mater. 2015, 14, 23–36. [Google Scholar] [CrossRef] [PubMed]
- Ritchie, R.O.; Buehler, M.J.; Hansma, P. Plasticity and toughness in bone. Phys. Today 2009, 62, 41–47. [Google Scholar] [CrossRef]
- Novitskaya, E.; Chen, P.Y.; Lee, S.; Castro-Cese, A.; Hirata, G.; Lubarda, V.A.; Mckittrick, J. Anisotropy in the compressive mechanical properties of bovine cortical bone and the mineral and protein constituents. Acta Biomater. 2011, 7, 3170–3177. [Google Scholar] [CrossRef]
- Hang, X.; He, S.; Dong, Z.; Minnick, G.; Rosenbohm, J.; Chen, Z.; Yang, R.; Chang, L. Nanosensors for single cell mechanical interrogation. Biosens. Bioelectron. 2021, 179, 113086. [Google Scholar] [CrossRef]
- Julian, B.; Norman, P.; Moon, S.-J.; Robin, H.B.; Peng, G.; Nazeeruddin, M.K.; Michael, G.T. Sequential deposition as a route to high-performance perovskite-sensitized solar cells. Nature 2013, 499, 316–319. [Google Scholar]
- Gérard, F. Hybrid porous solids: Past, present, future. Chem. Soc. Rev. 2008, 37, 191–214. [Google Scholar]
- Huang, Z.; Chen, H.; Yi, H.; Zhen, G.; Chen, Y. Ultra-Broadband Wide-Angle Terahertz Absorption Properties of 3D Graphene Foam. Adv. Funct. Mater. 2017, 28, 1704363. [Google Scholar] [CrossRef]
- Exerowa, D.; Kruglyakov, P.M. (Eds.) Foam and Foam Films: Theory, Experiment, Application; Elsevier: Amsterdam, The Netherlands, 1998. [Google Scholar]
- Huang, P.; Wu, F.; Shen, B.; Zheng, H.; Ren, Q.; Luo, H.; Zheng, W. Biomimetic porous polypropylene foams with special wettability properties. Compos. Part B Eng. 2020, 190, 107927. [Google Scholar] [CrossRef]
- Liu, Q.; Gao, S.; Zhao, Y.; Tao, W.; Yu, X.; Zhi, M. Review of layer-by-layer self-assembly technology for fire protection of flexible polyurethane foam. J. Mater. Sci. 2021, 56, 9605–9643. [Google Scholar] [CrossRef]
- Pierre, J.; Yoel, F.; Olivier, P. A constitutive law for dense granular flows. Nature 2006, 441, 727–730. [Google Scholar]
- Cisse, C.; Zaki, W.; Ben Zineb, T. A review of constitutive models and modeling techniques for shape memory alloys. Int. J. Plast. 2016, 76, 244–284. [Google Scholar] [CrossRef]
- Steinmetz, D.R.; Jäpel, T.; Wietbrock, B.; Eisenlohr, P.; Urrutia, I.G.; Saeed-Akbari, A.; Hickel, T.; Roters, F.; Raabe, D. Revealing the strain-hardening behavior of twinning-induced plasticity steels: Theory, simulations, experiments. Acta Mater. 2013, 61, 494–510. [Google Scholar] [CrossRef]
- Ha, N.S.; Lu, G. A review of recent research on bio-inspired structures and materials for energy absorption applications. Compos. Part B Eng. 2020, 181, 107496. [Google Scholar] [CrossRef]
- Silva, E.C.N.; Walters, M.C.; Paulino, G.H. Modeling bamboo as a functionally graded material: Lessons for the analysis of affordable materials. J. Mater. Sci. 2006, 41, 6991–7004. [Google Scholar] [CrossRef]
- Li, W.; Li, C.; Lin, L.; Wang, Y.; Zhang, J. All-dielectric radar absorbing array metamaterial based on silicon carbide/carbon foam material. J. Alloy. Compd. 2019, 781, 883–891. [Google Scholar] [CrossRef]
- Wang, P.; Deng, G.; Zhu, H.; Zhang, H.; Yin, J.; Xiong, X.; Wu, X. Effect of MWCNT content on conductivity and mechanical and wear properties of copper foam/resin composite. Compos. Part B Eng. 2019, 168, 572–580. [Google Scholar] [CrossRef]
- Ashby, M.F.; Gibson, L.J.; Wegst, U.G.; Olive, R. The mechanical properties of natural materials. I. Material property charts. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1995, 450, 123–140. [Google Scholar]
- Meyers, M.A.; Chen, P.-Y.; Lin, A.Y.-M.; Seki, Y. Biological materials: Structure and mechanical properties. Prog. Mater. Sci. 2008, 53, 1–206. [Google Scholar] [CrossRef] [Green Version]
- Chen, P.-Y.; McKittrick, J.; Meyers, M. Biological materials: Functional adaptations and bioinspired designs. Prog. Mater. Sci. 2012, 57, 1492–1704. [Google Scholar] [CrossRef]
- Yang, W.; Meyers, M.A.; Ritchie, R.O. Structural architectures with toughening mechanisms in Nature: A review of the ma-terials science of Type-I collagenous materials. Prog. Mater. Sci. 2019, 103, 425–483. [Google Scholar] [CrossRef]
- Wang, B.; Yang, W.; McKittrick, J.; Meyers, M.A. Keratin: Structure, mechanical properties, occurrence in biological organisms, and efforts at bioinspiration. Prog. Mater. Sci. 2016, 76, 229–318. [Google Scholar] [CrossRef] [Green Version]
- Matsushita, A.K.; Gonzalez, D.; Wang, M.; Doan, J.; Qiao, Y.; McKittrick, J. Beyond density: Mesostructural features of impact resistant wood. Mater. Today Commun. 2020, 22, 100697. [Google Scholar] [CrossRef]
- Gibson, L.J.; Ashby, M.F. Cellular Solids Structures and Properties; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Compton, B.G.; Lewis, J.A. 3D-Printing of Lightweight Cellular Composites. Adv. Mater. 2014, 26, 5930–5935. [Google Scholar] [CrossRef]
- Zhu, Y.; Luo, G.; Zhang, R.; Cao, P.; Liu, Q.; Zhang, J.; Sun, Y.; Li, J.; Shen, Q.; Zhang, L. Numerical simulation of static me-chanical properties of PMMA microcellular foams. Compos. Sci. Technol. 2020, 192, 108110. [Google Scholar] [CrossRef]
- Luong, D.D.; Pinisetty, D.; Gupta, N. Compressive properties of closed-cell polyvinyl chloride foams at low and high strain rates: Experimental investigation and critical review of state of the art. Compos. Part B Eng. 2013, 44, 403–416. [Google Scholar] [CrossRef]
- Petel, O.E.; Ouellet, S.; Higgins, A.J.; Frost, D.L. The elastic–plastic behaviour of foam under shock loading. Shock. Waves 2013, 23, 55–67. [Google Scholar] [CrossRef]
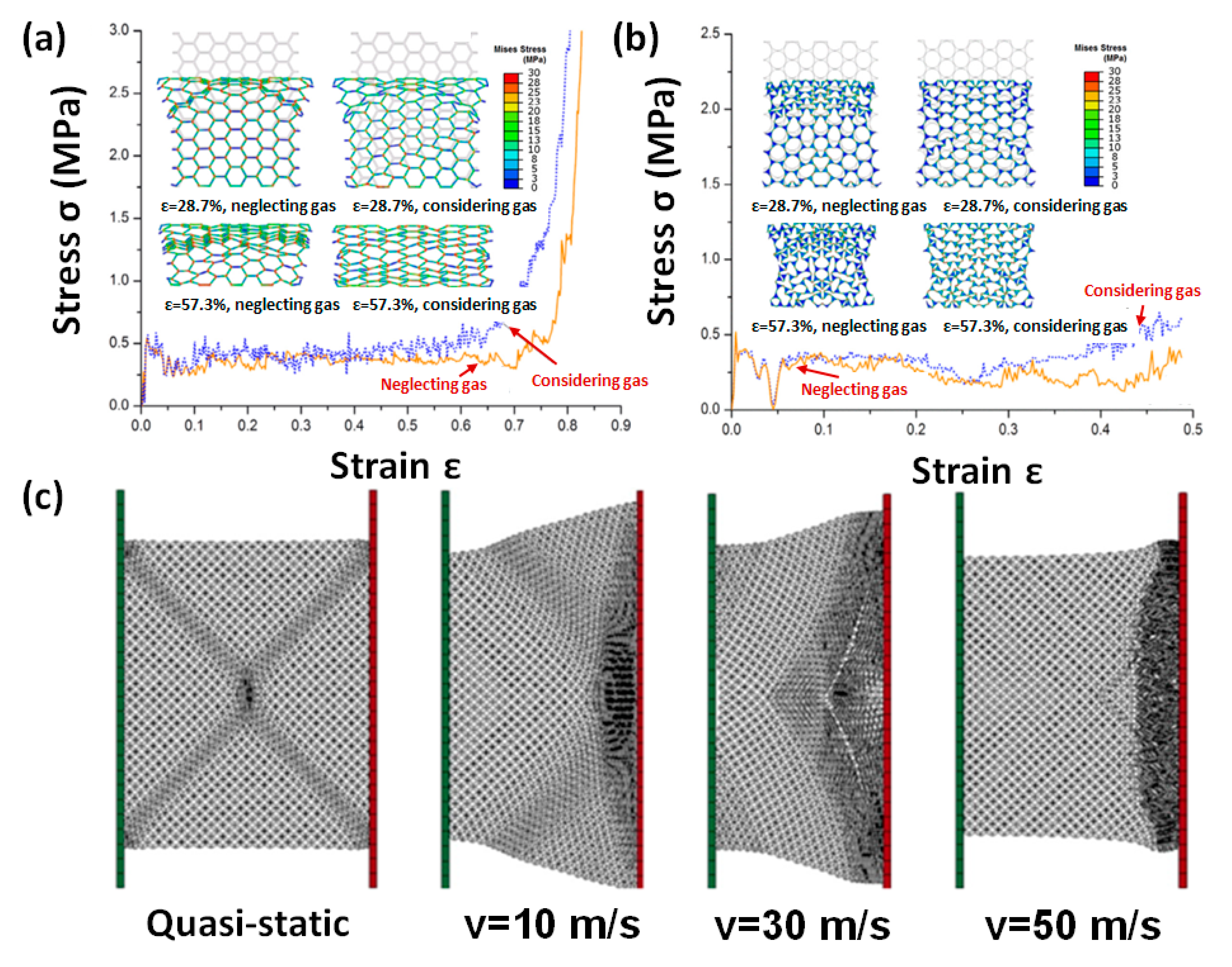
- Li, L.; Xue, P.; Luo, G. A numerical study on deformation mode and strength enhancement of metal foam under dynamic loading. Mater. Des. 2016, 110, 72–79. [Google Scholar] [CrossRef]
- Kader, M.A.; Islam, M.A.; Hazell, P.J.; Escobedo, J.P.; Saadatfar, M.; Brown, A.D.; Appleby-Thomas, G.J. Modelling and characterization of cell collapse in aluminium foams during dynamic loading. Int. J. Impact Eng. 2016, 96, 78–88. [Google Scholar] [CrossRef] [Green Version]
- Brezny, R.; Green, D. The effect of cell size on the mechanical behavior of cellular materials. Acta Met. Mater. 1990, 38, 2517–2526. [Google Scholar] [CrossRef]
- Weller, J.E.; Kumar, V. Solid-state microcellular polycarbonate foams. II. The effect of cell size on tensile properties. Polym. Eng. Sci. 2010, 50, 2170–2175. [Google Scholar] [CrossRef]
- Miller, D.; Kumar, V. Microcellular and nanocellular solid-state polyetherimide (PEI) foams using sub-critical carbon dioxide II. Tensile and impact properties. Polymer 2011, 52, 2910–2919. [Google Scholar] [CrossRef]
- Smits, G. Effect of Cellsize Reduction on Polyurethane Foam Physical Properties. J. Therm. Insul. Build. Envel. 1994, 17, 309–329. [Google Scholar] [CrossRef]
- Chen, Q.; Pugno, N.M. Mechanics of hierarchical 3-D nanofoams. EPL 2012, 97, 26002. [Google Scholar] [CrossRef] [Green Version]
- Notario, B.; Pinto, J.; Perez, M.A.R. Towards a new generation of polymeric foams: PMMA nanocellular foams with enhanced physical properties. Polymer 2015, 63, 116–126. [Google Scholar] [CrossRef]
- Kenny, L. Mechanical Properties of Particle Stabilized Aluminum Foam. Mater. Sci. Forum 1996, 217, 1883–1890. [Google Scholar] [CrossRef]
- Lankford, J.; Dannemann, K.A. Strain Rate Effects in Porous Materials. MRS Proc. 1998, 521, 103. [Google Scholar] [CrossRef]
- Landro, L.D.; Sala, G.; Olivieri, D. Deformation mechanisms and energy absorption of polystyrene foams for protective hel-mets. Polym. Test. 2002, 21, 217–228. [Google Scholar] [CrossRef]
- Yi, F.; Zhu, Z.; Zu, F.; Hu, S.; Yi, P. Strain rate effects on the compressive property and the energy-absorbing capacity of alu-minum alloy foams. Mater. Charact. 2001, 47, 417–422. [Google Scholar] [CrossRef]
- Ramachandra, S.; Kumar, P.S.; Ramamurty, U. Impact energy absorption in an Al foam at low velocities. Scr. Mater. 2003, 49, 741–745. [Google Scholar] [CrossRef]
- Suna, Y.; Li, Q.M. Dynamic compressive behaviour of cellular materials: A review of phenomenon, mechanism and modelling. Int. J. Impact Eng. 2018, 112, 74–115. [Google Scholar] [CrossRef] [Green Version]
- Bouix, R.; Viot, P.; Lataillade, J.-L. Polypropylene foam behaviour under dynamic loadings: Strain rate, density and microstructure effects. Int. J. Impact Eng. 2009, 36, 329–342. [Google Scholar] [CrossRef] [Green Version]
- Chen, W.; Hao, H.; Hughes, D.; Shi, Y.; Cui, J.; Li, Z.-X. Static and dynamic mechanical properties of expanded polystyrene. Mater. Des. 2015, 69, 170–180. [Google Scholar] [CrossRef]
- Doroudiani, S.; Kortschot, M.T. Polystyrene foams. III. Structure-tensile properties relationships. J. Appl. Polym. Sci. 2003, 90, 1427–1434. [Google Scholar] [CrossRef]
- Alvarez, P.; Mendizabal, A.; Petite, M.; Rodríguez-Pérez, M.; Echeverria, A. Finite element modelling of compressive mechanical behaviour of high and low density polymeric foams. Mater. Werkst. 2009, 40, 126–132. [Google Scholar] [CrossRef]
- Tekoglu, C.; Gibson, L.J.; Pardoen, T.; Onck, P.R. Size effects in foams: Experiments and modeling. Prog. Mater. Sci. 2011, 56, 109–138. [Google Scholar] [CrossRef]
- Ramamurty, U.; Paul, A. Variability in mechanical properties of a metal foam. Acta Mater. 2004, 52, 869–876. [Google Scholar] [CrossRef]
- Markaki, A.; Clyne, T. The effect of cell wall microstructure on the deformation and fracture of aluminium-based foams. Acta Mater. 2001, 49, 1677–1686. [Google Scholar] [CrossRef]
- Zhang, R.; Chen, J.; Zhu, Y.; Zhang, J.; Luo, G.; Cao, P.; Shen, Q.; Zhang, L. Correlation Between the Structure and Compressive Property of PMMA Microcellular Foams Fabricated by Supercritical CO2 Foaming Method. Polymers 2020, 12, 315. [Google Scholar] [CrossRef]
- Koohbor, B.; Kidane, A.; Lu, W.-Y. Effect of specimen size, compressibility and inertia on the response of rigid polymer foams subjected to high velocity direct impact loading. Int. J. Impact Eng. 2016, 98, 62–74. [Google Scholar] [CrossRef] [Green Version]
- Gigliotti, L.; Pinho, S. Prediction of the post-crushing compressive response of progressively crushable sandwich foam cores. Compos. Part A Appl. Sci. Manuf. 2016, 80, 148–158. [Google Scholar] [CrossRef] [Green Version]
- Zhou, H.; Wang, X.; Zhao, Z. High velocity impact mitigation with gradient cellular solids. Compos. Part B Eng. 2016, 85, 93–101. [Google Scholar] [CrossRef]
- Montanini, R. Measurement of strain rate sensitivity of aluminium foams for energy dissipation. Int. J. Mech. Sci. 2005, 47, 26–42. [Google Scholar] [CrossRef]
- Caglayan, C.; Osken, I.; Ataalp, A.; Turkmen, H.S.; Cebeci, H. Impact response of shear thickening fluid filled polyurethane foam core sandwich composites. Compos. Struct. 2020, 243, 112171. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Q.; Lowe, T.; McDonald, S.; Withers, P. Investigation of strain-rate effect on the compressive behaviour of closed-cell aluminium foam by 3D image-based modelling. Mater. Des. 2016, 89, 215–224. [Google Scholar] [CrossRef]
- Zheng, Z.; Wang, C.; Yu, J.; Reid, S.R.; Harrigan, J.J. Dynamic stress-strain states for metal foams using a 3D cellular model. J. Mech. Phys. Solids 2014, 72, 93–114. [Google Scholar] [CrossRef]
- Jing, L.; Su, X.; Yang, F.; Ma, H.; Zhao, L. Compressive strain rate dependence and constitutive modeling of closed-cell aluminum foams with various relative densities. J. Mater. Sci. 2018, 53, 14739–14757. [Google Scholar] [CrossRef]
- Langseth, M.; Hopperstad, O.; Hanssen, A. Crash behaviour of thin-walled aluminium members. Thin-Walled Struct. 1998, 32, 127–150. [Google Scholar] [CrossRef]
- Gruttmann, F.; Sauer, R.; Wagner, W. Theory and numerics of three-dimensional beams with elastoplastic material behavior. Int. J. Numer. Methods Eng. 2000, 48, 1675–1702. [Google Scholar] [CrossRef]
- Branch, B.; Ionita, A.; Patterson, B.M.; Schmalzer, A.; Clements, B.; Mueller, A.; Dattelbaum, D.M. A comparison of shock-wave dynamics in stochastic and periodic porous polymer architectures. Polymer 2019, 160, 325–337. [Google Scholar] [CrossRef]
- Branch, B.; Ionita, A.; Clements, B.E.; Montgomery, D.S.; Jensen, B.J.; Patterson, B.; Schmalzer, A.; Mueller, A.; Dattelbaum, D.M. Controlling shockwave dynamics using architecture in periodic porous materials. J. Appl. Phys. 2017, 121, 135102. [Google Scholar] [CrossRef] [Green Version]
- Yin, H.; Liu, Z.; Dai, J.; Wen, G.; Zhang, C. Crushing behavior and optimization of sheet-based 3D periodic cellular structures. Compos. Part B Eng. 2020, 182, 107565. [Google Scholar] [CrossRef]
- Radford, D.; Deshpande, V.; Fleck, N. The use of metal foam projectiles to simulate shock loading on a structure. Int. J. Impact Eng. 2005, 31, 1152–1171. [Google Scholar] [CrossRef]
- Ozturk, U.E.; Anlas, G. Energy absorption calculations in multiple compressive loading of polymeric foams. Mater. Design 2009, 30, 15–22. [Google Scholar] [CrossRef]
- Xin, Y.; Yong, X.; Zhou, Q. Influence of stress softening on energy-absorption capability of polymeric foams. Mater. Design 2011, 32, 1167–1176. [Google Scholar]
- Koohbor, B.; Kidane, A. Design optimization of continuously and discretely graded foam materials for efficient energy ab-sorption. Mater. Design 2016, 102, 151–161. [Google Scholar] [CrossRef]
- Wang, D.-A.; Pan, J. A non-quadratic yield function for polymeric foams. Int. J. Plast. 2006, 22, 434–458. [Google Scholar] [CrossRef]
- Wu, Q.; Yang, C.; Ohrndorf, A.; Christ, H.-J.; Han, J.; Xiong, J. Impact behaviors of human skull sandwich cellular bones: Theoretical models and simulation. J. Mech. Behav. Biomed. Mater. 2020, 104, 103669. [Google Scholar] [CrossRef]
- Xiang, X.; Zou, S.; Ha, N.S.; Lu, G.; Kong, I. Energy absorption of bio-inspired multi-layered graded foam-filled structures under axial crushing. Compos. Part B Eng. 2020, 198, 108216. [Google Scholar] [CrossRef]
- Deshpande, V.S.; Fleck, N. Isotropic constitutive models for metallic foams. J. Mech. Phys. Solids 2000, 48, 1253–1283. [Google Scholar] [CrossRef] [Green Version]
- Carranza, I.; Crocombe, A.D.; Mohagheghian, I.; Smith, P.A.; Sordon, A.; Meeks, G.; Santoni, C. Characterising and modelling the mechanical behaviour of polymeric foams under complex loading. J. Mater. Sci. 2019, 54, 11328–11344. [Google Scholar] [CrossRef] [Green Version]
- Goga, V. New Phenomenological Model for Solid Foams; Springer: Dordrecht, The Netherlands, 2011; pp. 67–82. [Google Scholar]
- Rusch, K.C. Energy-absorbing characteristics of foamed polymers. J. Appl. Polym. Sci. 1970, 14, 1433–1447. [Google Scholar] [CrossRef]
- Liu, Q.; Subhash, G. A phenomenological constitutive model for foams under large deformations. Polym. Eng. Sci. 2004, 44, 463–473. [Google Scholar] [CrossRef]
- Avalle, M.; Belingardi, G.; Ibba, A. Mechanical models of cellular solids: Parameters identification from experimental tests. Int. J. Impact Eng. 2007, 34, 3–27. [Google Scholar] [CrossRef]
- Cousins, R.R. A theory for the impact behavior of rate-dependent padding materials. J. Appl. Polym. Sci. 1976, 20, 2893–2903. [Google Scholar] [CrossRef]
- Sherwood, J.A.; Frost, C.C. Constitutive modeling and simulation of energy absorbing polyurethane foam under impact loading. Polym. Eng. Sci. 1992, 32, 1138–1146. [Google Scholar] [CrossRef]
- Nagy, A.; Ko, W.; Lindholm, U. Mechanical Behavior of Foamed Materials Under Dynamic Compression. J. Cell. Plast. 1974, 10, 127–134. [Google Scholar] [CrossRef]
- Zhang, J.; Kikuchi, N.; Li, V.; Yee, A.; Nusholtz, G. Constitutive modeling of polymeric foam material subjected to dynamic crash loading. Int. J. Impact Eng. 1998, 21, 369–386. [Google Scholar] [CrossRef]
- Jeong, K.Y.; Cheon, S.S.; Munshi, M.B. A constitutive model for polyurethane foam with strain rate sensitivity. J. Mech. Sci. Technol. 2012, 26, 2033–2038. [Google Scholar] [CrossRef]
- Jeong, K.Y. Constitutive modeling of polymeric foams having a four-parameter modulus function with strain rate sensitivity. J. Mech. Sci. Technol. 2016, 30, 683–688. [Google Scholar] [CrossRef]
- Zhu, F.; Chou, C.C.; Yang, K.H. Shock enhancement effect of lightweight composite structures and materials. Compos. Part B Eng. 2011, 42, 1202–1211. [Google Scholar] [CrossRef]
- Reid, S.; Peng, C. Dynamic uniaxial crushing of wood. Int. J. Impact Eng. 1997, 19, 531–570. [Google Scholar] [CrossRef]
- Lopatnikov, S.L.; Gama, B.A.; Haque, J.; Krauthauser, C.; Gillespie, J.W.; Guden, M.; Hall, I.W. Dynamics of metal foam deformation during Taylor cylinder–Hopkinson bar impact experiment. Compos. Struct. 2003, 61, 61–71. [Google Scholar] [CrossRef] [Green Version]
- Lopatnikov, S.L.; Gama, B.A.; Haque, J.; Krauthauser, C.; Gillespie, J.W. High-velocity plate impact of metal foams. Int. J. Impact Eng. 2004, 30, 421–445. [Google Scholar] [CrossRef]
- Lopatnikov, S.L.; Gama, B.A., Jr.; Gillespie, J.W. Modeling the progressive collapse behavior of metal foams. Int. J. Impact Eng. 2007, 34, 587–595. [Google Scholar] [CrossRef]
- Zheng, Z.; Liu, Y.; Yu, J.; Reid, S.R. Dynamic crushing of cellular materials: Continuum-based wave models for the transitional and shock modes. Int. J. Impact Eng. 2012, 42, 66–79. [Google Scholar] [CrossRef]
- Harrigan, J.J.; Reid, S.R.; Tan, P.; Reddy, T.Y. High rate crushing of wood along the grain. Int. J. Mech. Sci. 2005, 47, 521–544. [Google Scholar] [CrossRef]
- Elnasri, I.; Pattofatto, S.; Zhao, H.; Tsitsiris, H.; Hild, F.; Girard, Y. Shock enhancement of cellular structures under impact loading: Part I Experiments. J. Mech. Phys. Solids 2007, 55, 2652–2671. [Google Scholar] [CrossRef] [Green Version]
- Pattofatto, S.; Elnasri, I.; Zhao, H.; Tsitsiris, E.; Hild, F.; Girard, Y. Shock enhancement of cellular structures under impact loading: Part II Analysis. J. Mech. Phys. Solids 2007, 55, 2672–2686. [Google Scholar] [CrossRef] [Green Version]
- Zheng, Z.; Yu, J.; Wang, C.; Liao, S.; Liu, Y. Dynamic crushing of cellular materials: A unified framework of plastic shock wave models. Int. J. Impact Eng. 2013, 53, 29–43. [Google Scholar] [CrossRef]
- Sun, X.; Gao, Z.; Cao, P.; Zhou, C. Mechanical properties tests and multiscale numerical simulations for basalt fiber reinforced concrete. Constr. Build. Mater. 2019, 202, 58–72. [Google Scholar] [CrossRef]
- Nikolov, S.; Doghri, I. A micro/macro constitutive model for the small-deformation behavior of polyethylene. Polymer 2000, 41, 1883–1891. [Google Scholar] [CrossRef]
- Kitazono, K.; Sato, E.; Kuribayashi, K. Application of mean-field approximation to elastic-plastic behavior for closed-cell met-al foams. Acta Mater. 2003, 51, 4823–4836. [Google Scholar] [CrossRef]
- Doghri, I.; Tinel, L. Micromechanical modeling and computation of elasto-plastic materials reinforced with distributed-orientation fibers. Int. J. Plast. 2005, 21, 1919–1940. [Google Scholar] [CrossRef]
- Doghri, I.; Ouaar, A. Homogenization of two-phase elasto-plastic composite materials and structures—Study of tangent operators, cyclic plasticity and numerical algorithms. Int. J. Solids Struct. 2003, 40, 1681–1712. [Google Scholar] [CrossRef]
- Krairi, A.; Doghri, I. A thermodynamically-based constitutive model for thermoplastic polymers coupling viscoelasticity, viscoplasticity and ductile damage. Int. J. Plast. 2014, 60, 163–181. [Google Scholar] [CrossRef]
- Wu, L.; Noels, L.; Adam, L.; Doghri, I. A multiscale mean-field homogenization method for fiber-reinforced composites with gradient-enhanced damage models. Comput. Methods Appl. Mech. Eng. 2012, 233–236, 164–179. [Google Scholar] [CrossRef] [Green Version]
- Wu, L.; Noels, L.; Adam, L.; Doghri, I. An implicit-gradient-enhanced incremental-secant mean-field homogenization scheme for elasto-plastic composites with damage. Int. J. Solids Struct. 2013, 50, 3843–3860. [Google Scholar] [CrossRef] [Green Version]
- Zako, M.; Uetsuji, Y.; Kurashiki, T. Finite element analysis of damaged woven fabric composite materials. Compos. Sci. Technol. 2003, 63, 507–516. [Google Scholar] [CrossRef]
- Zhong, S.; Guo, L.; Liu, G.; Lu, H.; Zeng, T. A continuum damage model for three-dimensional woven composites and finite element implementation. Compos. Struct. 2015, 128, 1–9. [Google Scholar] [CrossRef]
- Delannay, L.; Kammoun, S.; Doghri, I.; Brassart, L. Micromechanical modeling of the progressive failure in short glass-fiber reinforced thermoplastics—First Pseudo-Grain Damage model. Compos. Part A Appl. Sci. Manuf. 2015, 73, 166–175. [Google Scholar]
- Wang, X.; Guan, Z.; Du, S.; Han, G.; Li, Z. An accurate and easy to implement method for predicting matrix crack and plasticity of composites with an efficient search algorithm for LaRC05 criterion. Compos. Part A Appl. Sci. Manuf. 2020, 131, 105808. [Google Scholar] [CrossRef]
- Jo, C.; Naguib, H.E. Constitutive modeling of HDPE polymer/clay nanocomposite foams. Polymer 2007, 48, 3349–3360. [Google Scholar] [CrossRef]
- Chen, L.; Schadler, L.S.; Ozisik, R. An experimental and theoretical investigation of the compressive properties of multi-walled carbon nanotube/poly(methyl methacrylate) nanocomposite foams. Polymer 2011, 52, 2899–2909. [Google Scholar] [CrossRef]
- Triawan, F.; Kishimoto, K.; Adachi, T.; Inaba, K.; Nakamura, T.; Hashimura, T. The elastic behavior of aluminum alloy foam under uniaxial loading and bending conditions. Acta Mater. 2012, 60, 3084–3093. [Google Scholar] [CrossRef]
- Jo, C.; Fu, J.; Naguib, H.E. Constitutive modeling for characterizing the compressive behavior of PMMA open-cell foams. J. Polym. Sci. Part B Polym. Phys. 2007, 45, 436–443. [Google Scholar] [CrossRef]
- De Giorgi, M.; Carofalo, A.; Dattoma, V.; Nobile, R.; Palano, F. Aluminium foams structural modelling. Comput. Struct. 2010, 88, 25–35. [Google Scholar] [CrossRef]
- Zhu, H.; Knott, J.; Mills, N. Analysis of the elastic properties of open-cell foams with tetrakaidecahedral cells. J. Mech. Phys. Solids 1997, 45, 319–343. [Google Scholar] [CrossRef]
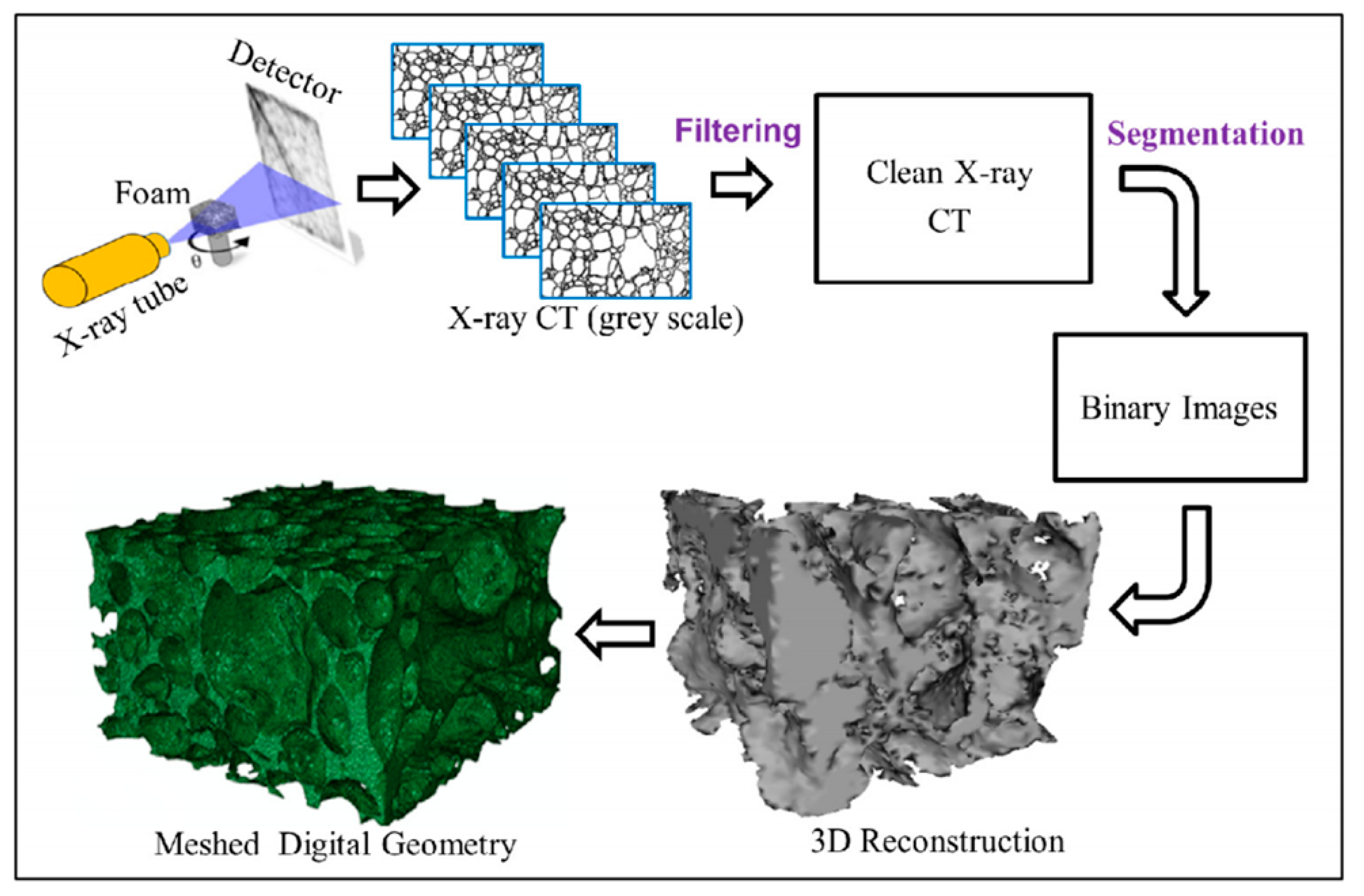
- Chen, Y.; Das, R.; Battley, M. Finite element analysis of the compressive and shear responses of structural foams using com-puted tomography. Compos. Struct. 2017, 159, 784–799. [Google Scholar] [CrossRef]
- Liu, P. Mechanical relation for porous metal foams under complex loads of triaxial tension and compression. Mater. Des. 2010, 31, 2264–2269. [Google Scholar] [CrossRef]
- Liu, P.S.; Chen, G.F. Mechanical relation of foamed metals under uniaxial and biaxial loads of collective tension and compression. Mater. Sci. Eng. A 2009, 507, 190–193. [Google Scholar] [CrossRef]
- Liu, P. Mechanical behaviors of porous metals under biaxial tensile loads. Mater. Sci. Eng. A 2006, 422, 176–183. [Google Scholar] [CrossRef]
- Zhang, J.; Wei, H.; Wang, Z.; Zhao, L. Dynamic crushing of uniform and density graded cellular structures based on the circle arc model. Lat. Am. J. Solids Struct. 2015, 12, 1102–1125. [Google Scholar] [CrossRef] [Green Version]
- Sun, Y.; Li, Q. Effect of entrapped gas on the dynamic compressive behaviour of cellular solids. Int. J. Solids Struct. 2015, 63, 50–67. [Google Scholar] [CrossRef]
- Mitrias, C.; Egelmeers, T.R.N.; Jaensson, N.O.; Hulsen, M.A.; Anderson, P.D. Simulation of bubble growth during the foaming process and mechanics of the solid foam. Rheol. Acta 2019, 58, 131–144. [Google Scholar] [CrossRef]
- Mukhopadhyay, T.; Adhikari, S.; Batou, A. Frequency domain homogenization for the viscoelastic properties of spatially correlated quasi-periodic lattices. Int. J. Mech. Sci. 2019, 150, 784–806. [Google Scholar] [CrossRef] [Green Version]
- Adhikari, S.; Mukhopadhyay, T.; Liu, X. Broadband dynamic elastic moduli of honeycomb lattice materials: A generalized analytical approach. Mech. Mater. 2021, 157, 103796. [Google Scholar] [CrossRef]
- Singh, A.; Mukhopadhyay, T.; Adhikari, S.; Bhattacharya, B. Voltage-dependent modulation of elastic moduli in lattice met-amaterials: Emergence of a programmable state-transition capability. Int. J. Solids Struct. 2021, 208–209, 31–48. [Google Scholar] [CrossRef]
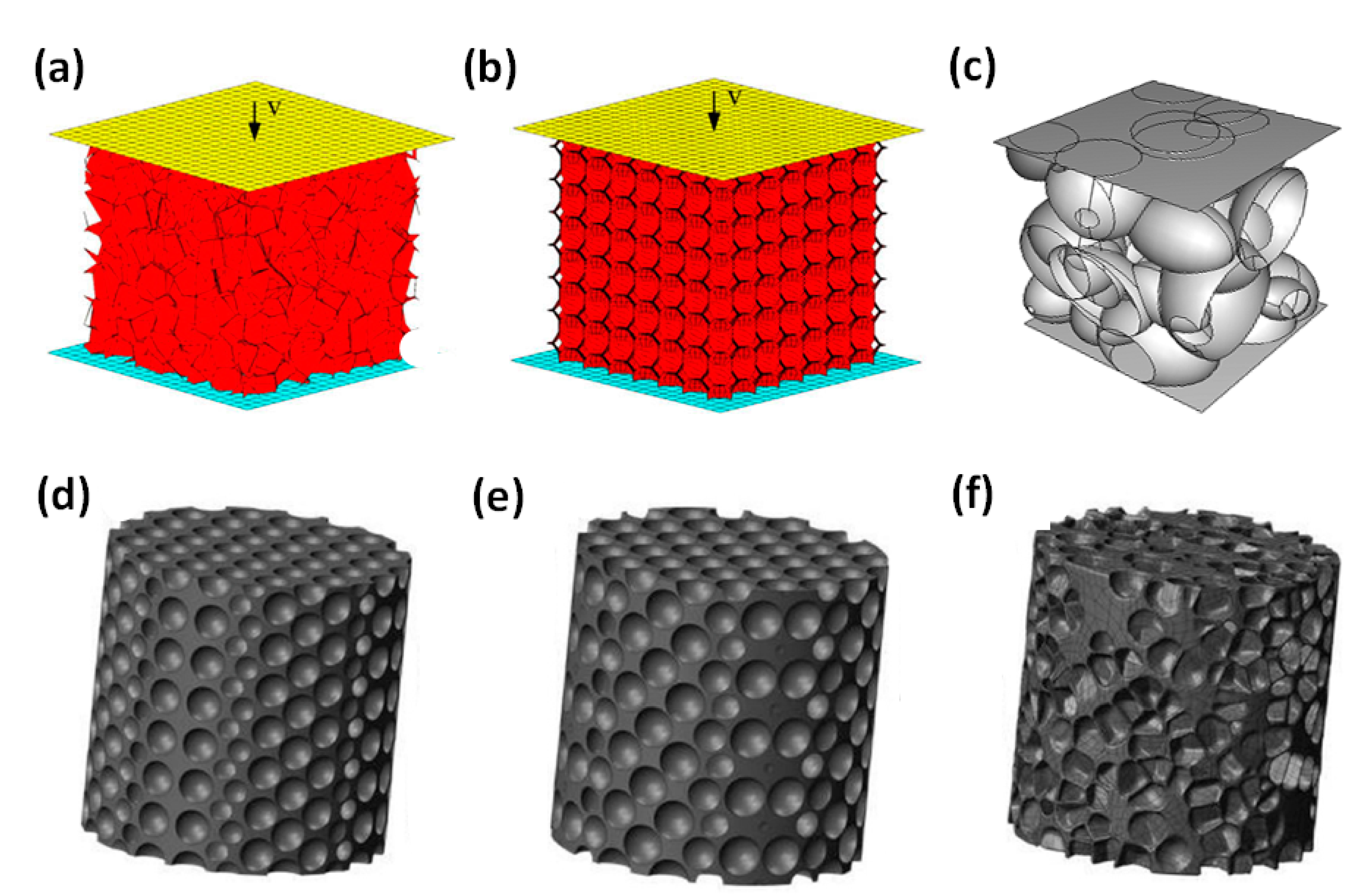
- Song, Y.; Wang, Z.; Zhao, L.; Luo, J. Dynamic crushing behavior of 3D closed-cell foams based on Voronoi random model. Mater. Des. 2010, 31, 4281–4289. [Google Scholar] [CrossRef]
- Ghazlan, A.; Ngo, T.D.; Tran, P. Three-dimensional Voronoi model of a nacre-mimetic composite structure under impulsive loading. Compos. Struct. 2016, 153, 278–296. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, L.; Jiang, Z.; Liu, Z.; Liu, Y.; Fang, D. Effects of Meso Shape Irregularity of Metal Foam on Yield Features under Triaxial Loading. Int. J. Struct. Stab. Dyn. 2015, 15, 1540014. [Google Scholar] [CrossRef]
- Zhang, X.; Tang, L.; Liu, Z.; Jiang, Z.; Liu, Y.; Wu, Y. Yield properties of closed-cell aluminum foam under triaxial loadings by a 3D Voronoi model. Mech. Mater. 2017, 104, 73–84. [Google Scholar] [CrossRef]
- Wu, Y.; Qiao, D.; Tang, L.; Xi, H.; Liu, Y.; Jiang, Z.; Liu, Z.; Zhou, L. Global topology of failure surfaces of metallic foams in principal-stress space and principal-strain space studied by numerical simulations. Int. J. Mech. Sci. 2019, 151, 551–562. [Google Scholar] [CrossRef]
- Tang, L.; Shi, X.; Zhang, L.; Liu, Z.; Jiang, Z.; Liu, Y. Effects of statistics of cell’s size and shape irregularity on mechanical properties of 2D and 3D Voronoi foams. Acta Mech. 2014, 225, 1361–1372. [Google Scholar] [CrossRef]
- Talebi, S.; Sadighi, M.; Aghdam, M.M.; Mirbagheri, S.M.H. Micro–macro analysis of closed-cell aluminum foam with crushing behavior subjected to dynamic loadings. Mater. Today Commun. 2017, 13, 170–177. [Google Scholar] [CrossRef]
- Vengatachalam, B.; Poh, L.H.; Liu, Z.S.; Qin, Q.H.; Swaddiwudhipong, S. Three dimensional modelling of closed-cell aluminium foams with predictive macroscopic behaviour. Mech. Mater. 2019, 136, 103067. [Google Scholar] [CrossRef]
- Roberts, A.; Garboczi, E. Elastic moduli of model random three-dimensional closed-cell cellular solids. Acta Mater. 2001, 49, 189–197. [Google Scholar] [CrossRef] [Green Version]
- Dabo, M.; Roland, T.; Dalongeville, G.; Gauthier, C.; Kékicheff, P. Ad-hoc modeling of closed-cell foam microstructures for structure-properties relationships. Eur. J. Mech.—A/Solids 2019, 75, 128–141. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, J.; Fan, J.; Wang, Z.; Zhao, L. On crushing response of the three-dimensional closed-cell foam based on Voronoi model. Mech. Mater. 2014, 68, 85–94. [Google Scholar] [CrossRef]
- Carofalo, A.; De Giorgi, M.; Morabito, A. Geometric modelling of metallic foams. Eng. Comput. 2013, 30, 924–935. [Google Scholar] [CrossRef]
- Fang, Q.; Zhang, J.; Zhang, Y.; Wu, H.; Gong, Z. A 3D mesoscopic model for the closed-cell metallic foams subjected to static and dynamic loadings. Int. J. Impact Eng. 2015, 82, 103–112. [Google Scholar] [CrossRef]
- Fang, Q.; Zhang, J.; Zhang, Y.; Liu, J.; Gong, Z. Mesoscopic investigation of closed-cell aluminum foams on energy absorption capability under impact. Compos. Struct. 2015, 124, 409–420. [Google Scholar] [CrossRef]
- Carolan, D.; Mayall, A.; Dear, J.; Fergusson, A. Micromechanical modelling of syntactic foam. Compos. Part B Eng. 2020, 183, 107701. [Google Scholar] [CrossRef]
- Cheng, Z.; Fang, Q.; Zhang, J.; Zhang, Y. Mesoscopic methodology for the three-dimensional modelling of closed-cell metallic foam. Eng. Mech. 2017, 34, 212–221. [Google Scholar]
- Huang, R.; Li, P.; Liu, T. X-ray microtomography and finite element modelling of compressive failure mechanism in cenosphere epoxy syntactic foams. Compos. Struct. 2016, 140, 157–165. [Google Scholar] [CrossRef]
- Siegkas, P.; Petrinic, N.; Tagarielli, V. Measurements and micro-mechanical modelling of the response of sintered titanium foams. J. Mech. Behav. Biomed. Mater. 2016, 57, 365–375. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kader, M.; Islam, A.; Saadatfar, M.; Hazell, P.; Brown, A.; Ahmed, S.; Escobedo, J. Macro and micro collapse mechanisms of closed-cell aluminium foams during quasi-static compression. Mater. Des. 2017, 118, 11–21. [Google Scholar] [CrossRef]
- Luo, G.; Xue, P.; Sun, S. Investigations on the yield behavior of metal foam under multiaxial loadings by an imaged-based mesoscopic model. Int. J. Mech. Sci. 2018, 142–143, 153–162. [Google Scholar] [CrossRef]
- Jeon, I.; Asahina, T.; Kang, K.-J.; Im, S.; Lu, T.J. Finite element simulation of the plastic collapse of closed-cell aluminum foams with X-ray computed tomography. Mech. Mater. 2010, 42, 227–236. [Google Scholar] [CrossRef]
- Zhang, C.Y.; Tang, L.Q.; Yang, B.; Zhang, L.; Huang, X.Q.; Fang, D.N. Meso-mechanical study of collapse and fracture behaviors of closed-cell metallic foams. Comput. Mater. Sci. 2013, 79, 45–51. [Google Scholar] [CrossRef]
- Caty, O.; Maire, E.; Youssef, S.; Bouchet, R. Modeling the properties of closed-cell cellular materials from tomography images using finite shell elements. Acta Mater. 2008, 56, 5524–5534. [Google Scholar] [CrossRef]
- Arai, Y.; Daigo, Y.; Kojo, E.; Inoue, R.; Kogo, Y. Relationship between the microstructures and Young’s modulus of 3D-networked porous carbon material. J. Mater. Sci. 2021, 56, 10338–10352. [Google Scholar] [CrossRef]
- Singaravelu, A.S.S.; Williams, J.J.; Ruppert, J.; Henderson, M.; Holmes, C.; Chawla, N. In situ X-ray microtomography of the compression behaviour of eTPU bead foams with a unique graded structure. J. Mater. Sci. 2021, 56, 5082–5099. [Google Scholar] [CrossRef]
- Wang, E.; Sun, G.; Zheng, G.; Li, Q. Characterization of initial and subsequent yield behaviors of closed-cell aluminum foams under multiaxial loadings. Compos. Part B Eng. 2020, 202, 108247. [Google Scholar] [CrossRef]
- Roszkos, C.S.; Bocko, J.; Kula, T.; Šarloši, J. Static and dynamic analyses of aluminum foam geometric models using the homogenization procedure and the FEA. Compos. Part B Eng. 2019, 171, 361–374. [Google Scholar] [CrossRef]
- Doyoyo, M.; Wierzbicki, T. Experimental studies on the yield behavior of ductile and brittle aluminum foams. Int. J. Plast. 2003, 19, 1195–1214. [Google Scholar] [CrossRef]
- Ruan, D.; Lu, G.; Ong, L.; Wang, B. Triaxial compression of aluminium foams. Compos. Sci. Technol. 2007, 67, 1218–1234. [Google Scholar] [CrossRef]
- Sarathchandra, D.; Subbu, S.K.; Venkaiah, N. Functionally graded materials and processing techniques: An art of review. Mater. Today Proc. 2018, 5, 21328–21334. [Google Scholar] [CrossRef]
- Choy, S.Y.; Sun, C.-N.; Leong, K.F.; Wei, J. Compressive properties of functionally graded lattice structures manufactured by selective laser melting. Mater. Des. 2017, 131, 112–120. [Google Scholar] [CrossRef]
- Zhang, C.; Chen, F.; Huang, Z.; Jia, M.; Chen, G.; Ye, Y.; Lin, Y.; Liu, W.; Chen, B.; Shen, Q.; et al. Additive manufacturing of functionally graded materials: A review. Mater. Sci. Eng. A 2019, 764, 138209. [Google Scholar] [CrossRef]
- Duan, Y.; Zhao, X.; Liu, Z.; Hou, N.; Liu, H.; Du, B.; Hou, B.; Li, Y. Dynamic response of additively manufactured graded foams. Compos. Part B Eng. 2020, 183, 107630. [Google Scholar] [CrossRef]
- Liu, H.; Ding, S.; Ng, B.F. Impact response and energy absorption of functionally graded foam under temperature gradient environment. Compos. Part B Eng. 2019, 172, 516–532. [Google Scholar] [CrossRef]
- Xiao, Z.; Fang, J.; Sun, G.; Li, Q. Crashworthiness design for functionally graded foam-filled bumper beam. Adv. Eng. Softw. 2015, 85, 81–95. [Google Scholar] [CrossRef]
- Fei, Y.; Chen, F.; Fang, W.; Xu, L.; Ruan, S.; Liu, X.; Zhong, M.; Kuang, T. High-strength, flexible and cycling-stable piezo-resistive polymeric foams derived from thermoplastic polyurethane and multi-wall carbon nanotubes. Compos. Part B Eng. 2020, 199, 108279. [Google Scholar] [CrossRef]
- Guo, K.-Y.; Wu, Q.; Mao, M.; Chen, H.; Zhang, G.-D.; Zhao, L.; Gao, J.-F.; Song, P.; Tang, L.-C. Water-based hybrid coatings toward mechanically flexible, super-hydrophobic and flame-retardant polyurethane foam nanocomposites with high-efficiency and reliable fire alarm response. Compos. Part B Eng. 2020, 193, 108017. [Google Scholar] [CrossRef]
- Liu, Q.; He, X.; Yi, C.; Sun, D.; Chen, J.; Wang, D.; Liu, K.; Li, M. Fabrication of ultra-light nickel/graphene composite foam with 3D interpenetrating network for high-performance electromagnetic interference shielding. Compos. Part B Eng. 2020, 182, 107614. [Google Scholar] [CrossRef]
- Zhu, Y.; Luo, G.; Zhang, R.; Liu, Q.; Sun, Y.; Zhang, J.; Shen, Q.; Zhang, L. Investigation of the Constitutive Model of W/PMMA Composite Microcellular Foams. Polymers 2019, 11, 1136. [Google Scholar] [CrossRef] [Green Version]
- Schaedler, T.A.; Carter, W.B. Architected Cellular Materials. Annu. Rev. Mater. Res. 2016, 46, 187–210. [Google Scholar] [CrossRef]
- Zheng, X.; Smith, W.; Jackson, J.; Moran, B.; Cui, H.; Chen, D.; Ye, J.; Fang, N.; Rodriguez, N.; Weisgraber, T.; et al. Multiscale metallic metamaterials. Nat. Mater. 2016, 15, 1100–1106. [Google Scholar] [CrossRef] [PubMed]
- Frenzel, T.; Kadic, M.; Wegener, M. Three-dimensional mechanical metamaterials with a twist. Science 2017, 358, 1072–1074. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cheung, K.C.; Gershenfeld, N. Reversibly Assembled Cellular Composite Materials. Science 2013, 341, 1219–1221. [Google Scholar] [CrossRef] [Green Version]
- Ferkl, P.; Toulec, M.; Laurini, E.; Pricl, S.; Fermeglia, M.; Auffarth, S.; Eling, B.; Settels, V.; Kosek, J. Multi-scale modelling of heat transfer in polyurethane foams. Chem. Eng. Sci. 2017, 172, 323–334. [Google Scholar] [CrossRef]
- Lane, J.M.D.; Grest, G.S.; Mattsson, T.R. Hot spot and temperature analysis of shocked hydrocarbon polymer foams using molecular dynamics simulation. Comput. Mater. Sci. 2013, 79, 873–876. [Google Scholar] [CrossRef]
- Karimi, M.; Marchisio, D.; Laurini, E.; Fermeglia, M.; Pricl, S. Bridging the gap across scales: Coupling CFD and MD/GCMC in polyurethane foam simulation. Chem. Eng. Sci. 2018, 178, 39–47. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Zhao, C.; Xu, Z.; Xu, H. Modeling metal foam enhanced phase change heat transfer in thermal energy storage by using phase field method. Int. J. Heat Mass Transf. 2016, 99, 170–181. [Google Scholar] [CrossRef]
- Kim, J.S.; Chung, S.Y.; Han, T.S.; Stephan, D.; Abd Elrahman, M. Modeling of multiple phase solid microstructures and pre-diction of mechanical behaviors of foamed concrete. Constr. Build. Mater. 2020, 248, 118637. [Google Scholar] [CrossRef]
- Wang, F.; Altschuh, P.; Matz, A.M.; Heimann, J.; Matz, B.S.; Nestler, B.; Jost, N. Phase-field study on the growth of magnesium silicide occasioned by reactive diffusion on the surface of Si-foams. Acta Mater. 2019, 170, 138–154. [Google Scholar] [CrossRef]
- Postek, E.; Pecherski, R.B.; Nowak, Z. Peridynamic simulation of crushing processes in copper open-cell foam. Arch. Metall. Mater. 2019, 64, 1603–1610. [Google Scholar]
- Opiela, K.C.; Zieliński, T.G. Microstructural design, manufacturing and dual-scale modelling of an adaptable porous compo-site sound absorber. Compos. Part B Eng. 2020, 187, 107833. [Google Scholar] [CrossRef]
- Oh, J.H.; Kim, J.S.; Nguyen, V.H.; Oh, I.K. Auxetic graphene oxide-porous foam for acoustic wave and shock energy dissipation. Compos. Part B Eng. 2020, 186, 107817. [Google Scholar] [CrossRef]
- Xiao, S.; Mei, H.; Han, D.; Cheng, L. Sandwich-like SiCnw/C/Si3N4 porous layered composite for full X-band electromagnetic wave absorption at elevated temperature. Compos. Part B Eng. 2020, 183, 107629. [Google Scholar] [CrossRef]
- Zhu, S.; Zhou, Q.; Wang, M.; Dale, J.; Qiang, Z.; Fan, Y.; Zhu, M.; Ye, C. Modulating electromagnetic interference shielding performance of ultra-lightweight composite foams through shape memory function. Compos. Part B Eng. 2021, 204, 108497. [Google Scholar] [CrossRef]
- Guo, W.; Zhao, Y.; Wang, X.; Cai, W.; Wang, J.; Song, L.; Hu, Y. Multifunctional epoxy composites with highly flame retardant and effective electromagnetic interference shielding performances. Compos. Part B Eng. 2020, 192, 107990. [Google Scholar] [CrossRef]
- Chen, Z.; Xu, C.; Ma, C.; Ren, W.; Cheng, H.-M. Lightweight and Flexible Graphene Foam Composites for High-Performance Electromagnetic Interference Shielding. Adv. Mater. 2013, 25, 1296–1300. [Google Scholar] [CrossRef] [PubMed]
- Li, M.-E.; Yan, Y.-W.; Zhao, H.-B.; Jian, R.-K.; Wang, Y.-Z. A facile and efficient flame-retardant and smoke-suppressant resin coating for expanded polystyrene foams. Compos. Part B Eng. 2020, 185, 107797. [Google Scholar] [CrossRef]
- Davis, M.E. Ordered porous materials for emerging applications. Nature 2002, 417, 813–821. [Google Scholar] [CrossRef]
- Nugent, P.; Belmabkhout, Y.; Burd, S.D.; Cairns, A.J.; Luebke, R.; Forrest, K.; Pham, T.; Ma, S.; Space, B.; Wojtas, L.; et al. Porous materials with optimal adsorption thermodynamics and kinetics for CO2 separation. Nature 2013, 495, 80–84. [Google Scholar] [CrossRef]
- Seo, J.S.; Whang, D.; Lee, H.; Jun, S.I.; Oh, J.; Jeon, Y.J.; Kim, K. A homochiral metal–organic porous material for enantiose-lective separation and catalysis. Nature 2000, 404, 982–986. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Huang, Y.; Zhang, T.; Chang, H.; Xiao, P.; Chen, H.; Huang, Z.; Chen, Y. Broadband and Tunable High-Performance Microwave Absorption of an Ultralight and Highly Compressible Graphene Foam. Adv. Mater. 2015, 27, 2049–2053. [Google Scholar] [CrossRef] [PubMed]




| Models | Advantages | Disadvantages |
|---|---|---|
| Phenomenological constitutive model |
|
|
| Homogenization algorithm model |
|
|
| Single cell model |
|
|
| Multi-cell model |
|
|
| Cellular Medium | Relative Density | Cell Size | Strain Rate | Modulus | Strength | Toughness | Performance | Ref. |
|---|---|---|---|---|---|---|---|---|
| Brittle carbon foam | 0.029–0.04 | 0.49–4.9 mm | - | 45–62 MPa | - | 0.04–0.05 MPa |
| [35] |
| Polycarbonate (PC) foam | 0.56 | 2.8–37.1 μm | - | 836–978 MPa | 25.6–29.8 MPa | 7.02–10.22 J/cm3 | [36] | |
| Polyetherimide (PEI) foam | 0.75–0.9 | 2–4 μm 30–120 nm | - | 1929–2628 MPa | 64.1–87.7 MPa | 17.6–103.1 J/cm3 | [37] | |
| Polyurethane (PU) foam | 0.98 | 0–800 μm | - | - | 0.06–0.11 MPa | - |
| [38] |
| 3D hierarchical foam | - | 0–55 nm | - | 0.5–1 GPa | 0.8–13 MPa | - |
| [39] |
| Polymethyl methacrylate (PMMA) foam | 0.5 | 0.2–11μm | - | 577–791 MPa | - | 0.13–0.44 J/cm3 | [40] | |
| Aluminum (Al) foam | 0.02–0.2 | 3–25 mm | 10−4–103 s−1 | 1–700 MPa | 1.5 MPa | - |
| [41,42] |
| Expanded polystyrene (EPS) foam | 0.04–0.11 | 130–320 μm | - | 6.5–32.3 MPa | 0.19–0.7 MPa | - | [43] | |
| Al foam | 0.15–0.29 | 0.8 mm | 10−3–2600 s−1 | - | 5–22 MPa | - |
| [44,45,46] |
| Expanded polypropylene (EPP) foam | 0.03–0.17 | 0–0.4 mm | 10−2–1500 s−1 | 0.2–4 MPa | 0.2–2.7 MPa | - | [47] | |
| Polyvinyl chloride (PVC) foam | 0.04–0.18 | 300–450 μm | 10−4–2000 s−1 | 74–400 MPa | 1.08–8.65 MPa | - | [31] | |
| EPS foam | 0.021–0.044 | - | 2.68–280 s−1 | 2.7–4.8 MPa | 0.171–0.478 MPa | - | [48] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, G.; Zhu, Y.; Zhang, R.; Cao, P.; Liu, Q.; Zhang, J.; Sun, Y.; Yuan, H.; Guo, W.; Shen, Q.; et al. A Review on Mechanical Models for Cellular Media: Investigation on Material Characterization and Numerical Simulation. Polymers 2021, 13, 3283. https://doi.org/10.3390/polym13193283
Luo G, Zhu Y, Zhang R, Cao P, Liu Q, Zhang J, Sun Y, Yuan H, Guo W, Shen Q, et al. A Review on Mechanical Models for Cellular Media: Investigation on Material Characterization and Numerical Simulation. Polymers. 2021; 13(19):3283. https://doi.org/10.3390/polym13193283
Chicago/Turabian StyleLuo, Guoqiang, Yuxuan Zhu, Ruizhi Zhang, Peng Cao, Qiwen Liu, Jian Zhang, Yi Sun, Huan Yuan, Wei Guo, Qiang Shen, and et al. 2021. "A Review on Mechanical Models for Cellular Media: Investigation on Material Characterization and Numerical Simulation" Polymers 13, no. 19: 3283. https://doi.org/10.3390/polym13193283
APA StyleLuo, G., Zhu, Y., Zhang, R., Cao, P., Liu, Q., Zhang, J., Sun, Y., Yuan, H., Guo, W., Shen, Q., & Zhang, L. (2021). A Review on Mechanical Models for Cellular Media: Investigation on Material Characterization and Numerical Simulation. Polymers, 13(19), 3283. https://doi.org/10.3390/polym13193283









