Conformation of Flexible and Semiflexible Chains Confined in Nanoposts Array of Various Geometries
Abstract
1. Introduction
2. Method and Model
3. Results and Discussion
3.1. Occupation Number
3.2. Chain Extension Along the Post Axes
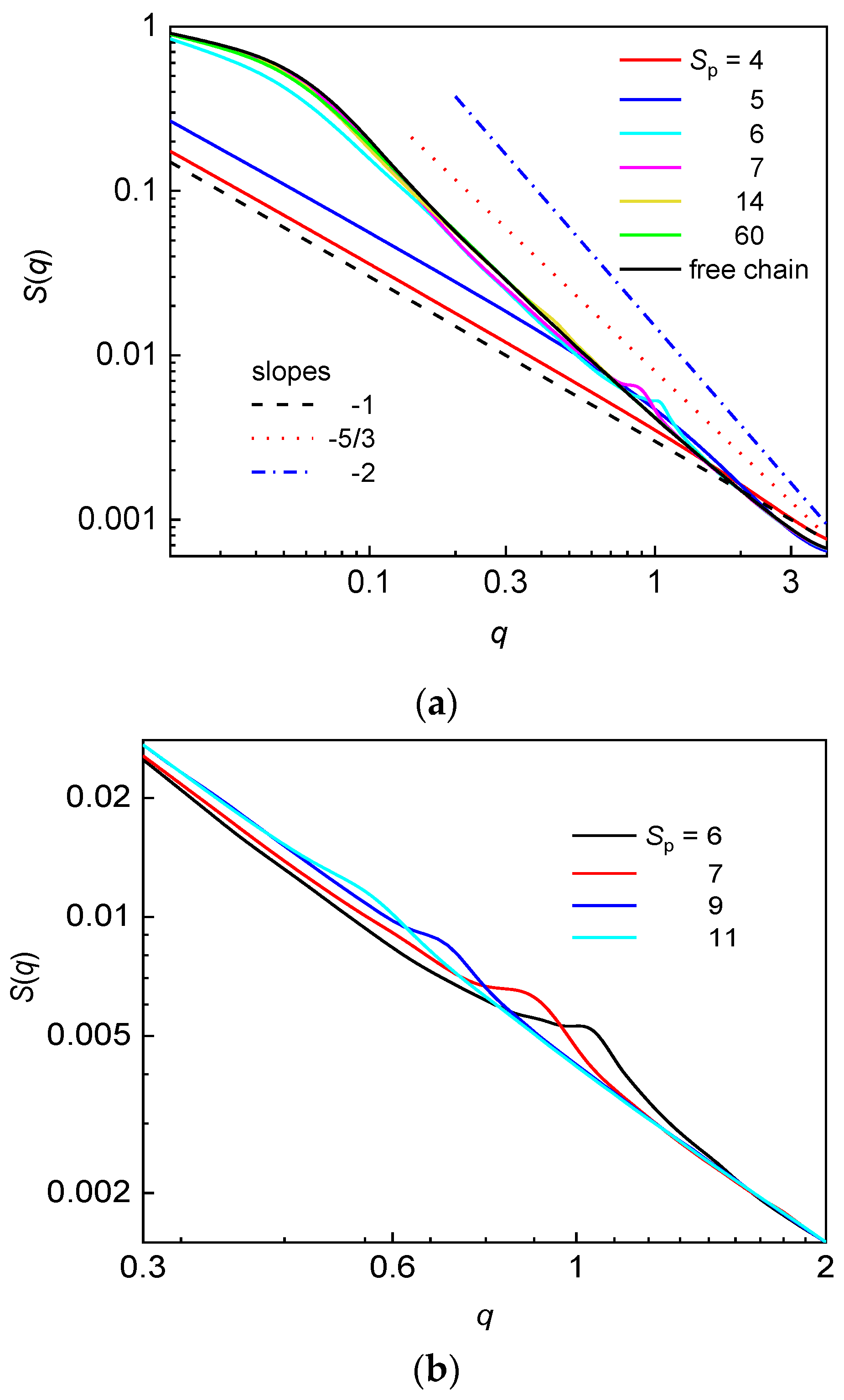
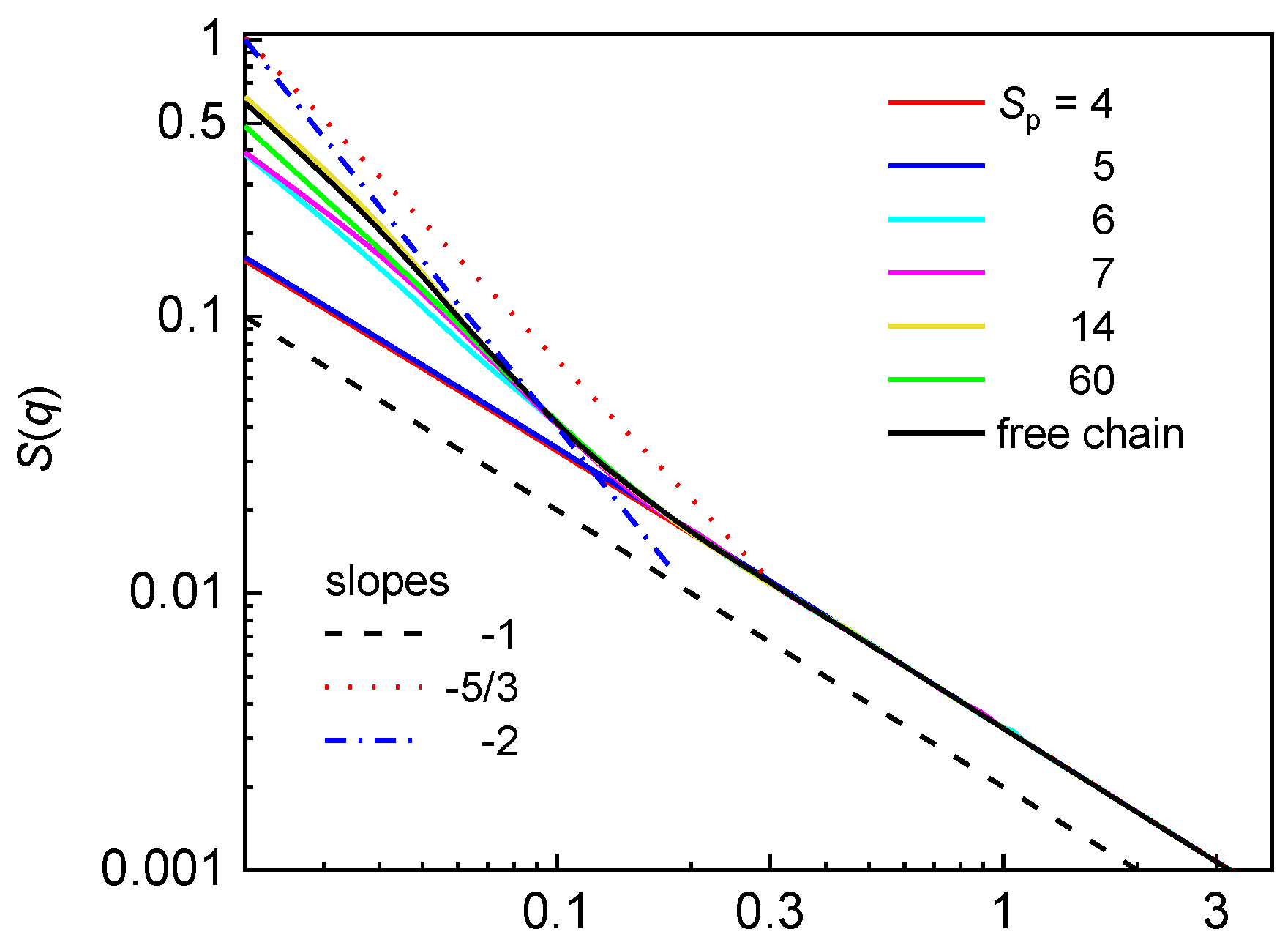
3.3. Structure Factor
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhou, H.; Dill, K.A. New Concepts Stabilization of Proteins in Confined Spaces. Biochemistry 2001, 40, 11289–11293. [Google Scholar] [CrossRef] [PubMed]
- Eggers, D.K.; Valentine, J.S. Molecular confinement influences protein structure and enhances thermal protein stability. Protein Sci. 2001, 10, 250–261. [Google Scholar] [CrossRef] [PubMed]
- Minton, A.P. Macromolecular Crowding and Confinement: Biochemical, Biophysical, and Potential Physiological. Annu. Rev. Biophys. 2008, 37, 375–397. [Google Scholar]
- Frey, E.; Gerland, U. Spontaneous Unknotting of a Polymer Confined in a Nanochannel. Nano Lett. 2008, 8, 4518–4522. [Google Scholar]
- Lam, E.T.; Hastie, A.; Lin, C.; Ehrlich, D.; Das, S.K.; Austin, M.D.; Deshpande, P.; Cao, H.; Nagarajan, N.; Xiao, M.; et al. Genome mapping on nanochannel arrays for structural variation analysis and sequence assembly. Nat. Biotechnol. 2012, 30, 771–776. [Google Scholar] [CrossRef]
- Chan, E.Y.; Goncalves, N.M.; Haeusler, R.A.; Hatch, A.J.; Larson, J.W.; Maletta, A.M.; Yantz, G.R.; Carstea, E.D.; Fuchs, M.; Wong, G.G.; et al. DNA Mapping Using Microfluidic Stretching and Single-Molecule Detection of Fluorescent Site-Specific Tags. Genome Res. 2004, 14, 1137–1146. [Google Scholar] [CrossRef]
- Tegenfeldt, J.O.; Prinz, C.; Cao, H.; Chou, S.; Reisner, W.W.; Riehn, R.; Wang, Y.M.; Cox, E.C.; Sturm, J.C.; Silberzan, P.; et al. The Dynamics of Genomic-Length DNA Molecules in 100-nm Channels. Proc. Natl. Acad. Sci. USA 2004, 101, 10979–10983. [Google Scholar] [CrossRef]
- Reisner, W.; Morton, K.J.; Riehn, R.; Wang, Y.M.; Yu, Z.; Rosen, M.; Sturm, J.C.; Chou, S.Y.; Frey, E.; Austin, R.H. Statics and Dynamics of Single DNA Molecules Confined in Nanochannels. Phys. Rev. Lett. 2005, 94, 196101. [Google Scholar] [CrossRef]
- Reisner, W.; Pedersen, J.N.; Austin, R.H. DNA confinement in nanochannels: Physics and biological applications. Rep. Prog. Phys. 2012, 75, 106601. [Google Scholar] [CrossRef]
- Bakajin, O.; Duke, T.A.J.; Tegenfeldt, J.; Chou, C.; Chan, S.S.; Austin, R.H.; Cox, E.C. Separation of 100-Kilobase DNA Molecules in 10 Seconds. Anal. Chem. 2001, 73, 6053–6056. [Google Scholar] [CrossRef]
- Kaji, N.; Tezuka, Y.; Takamura, Y.; Ueda, M.; Nishimoto, T.; Nakanishi, H.; Horiike, Y.; Baba, Y. Separation of Long DNA Molecules by Quartz Nanopillar Chips under a Direct Current Electric Field. Anal. Chem. 2004, 76, 3496–3503. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.Z.Y.; Sullivan, D.E. Free Energy of a Wormlike Polymer Chain Confined in a Slit: Crossover between Two Scaling Regimes. Macromolecules 2006, 39, 7769–7773. [Google Scholar] [CrossRef]
- Jo, K.; Dhingra, D.M.; Odijk, T.; de Pablo, J.J.; Graham, M.D.; Runnheim, R.; Forrest, D.; Schwartz, D.C. A single-molecule barcoding system using nanoslits for DNA analysis. Proc. Natl. Acad. Sci. USA 2007, 104, 2673–2678. [Google Scholar] [CrossRef] [PubMed]
- Balducci, A.; Hsieh, C.; Doyle, P.S. Relaxation of Stretched DNA in Slitlike Confinement. Phys. Rev. Lett. 2007, 99, 238102. [Google Scholar] [CrossRef]
- Tang, J.; Levy, S.L.; Trahan, D.W.; Jones, J.J.; Craighead, H.G.; Doyle, P.S. Revisiting the Conformation and Dynamics of DNA in Slitlike Confinement. Macromolecules 2010, 43, 7368–7377. [Google Scholar] [CrossRef]
- Dai, L.; Jones, J.J.; Van Der Maarel, J.R.C.; Doyle, P.S. A systematic study of DNA conformation in slitlike confinement. Soft Matter 2012, 8, 2972–2982. [Google Scholar] [CrossRef]
- Tree, D.R.; Reinhart, W.F.; Dorfman, K.D. The Odijk Regime in Slits. Macromolecules 2014, 47, 3672–3684. [Google Scholar] [CrossRef]
- Dai, L.; Ng, S.Y.; Doyle, P.S.; Van der Maarel, J.R.C. Conformation Model of Back-Folding and Looping of a Single DNA Molecule Confined Inside a Nanochannel. ACS Macro Lett. 2012, 1, 1046–1050. [Google Scholar] [CrossRef]
- Chen, J.Z.Y. Free Energy and Extension of a Wormlike Chain in Tube Confinement. Macromolecules 2013, 46, 9837–9844. [Google Scholar] [CrossRef]
- Dai, L.; Van der Maarel, J.; Doyle, P.S. Extended de Gennes Regime of DNA Confined in a Nanochannel. Macromolecules 2014, 47, 2445–2450. [Google Scholar] [CrossRef]
- Muralidhar, A.; Tree, D.R.; Dorfman, K.D. Backfolding of Wormlike Chains Confined in Nanochannels. Macromolecules 2014, 47, 8446–8458. [Google Scholar] [CrossRef]
- Muralidhar, A.; Tree, D.R.; Wang, Y.; Dorfman, K.D. Interplay between chain stiffness and excluded volume of semiflexible polymers confined in nanochannels. J. Chem. Phys. 2014, 140, 084905. [Google Scholar] [CrossRef] [PubMed]
- Werner, E.; Mehlig, B. Scaling regimes of a semiflexible polymer in a rectangular channel. Phys. Rev. E 2015, 91, 050601. [Google Scholar] [CrossRef] [PubMed]
- Gupta, D.; Miller, J.J.; Muralidhar, A.; Mahshid, S.; Reisner, W.; Dorfman, K.D. Experimental Evidence of Weak Excluded Volume Effects for Nanochannel Confined DNA. ACS Macro Lett. 2015, 4, 759–763. [Google Scholar] [CrossRef]
- Reinhart, W.F.; Reifenberger, J.G.; Gupta, D.; Muralidhar, A.; Sheats, J.; Cao, H.; Dorfman, K.D. Distribution of distances between DNA barcode labels in nanochannels close to the persistence length. J. Chem. Phys. 2015, 142, 064902. [Google Scholar] [CrossRef]
- Huang, A.; Hsu, H.-P.; Bhattacharya, A.; Binder, K. Semiflexible macromolecules in quasi-one-dimensional confinement: Discrete versus continuous bond angles. J. Chem. Phys. 2015, 143, 243102. [Google Scholar] [CrossRef]
- Muralidhar, A.; Dorfman, K.D. Backfolding of DNA Confined in Nanotubes: Flory Theory versus the Two-State Cooperativity Model. Macromolecules 2016, 49, 1120–1126. [Google Scholar] [CrossRef]
- Odijk, T. On the Statistics and Dynamics of Confined or Entangled Stiff Polymers. Macromolecules 1983, 16, 1340–1344. [Google Scholar] [CrossRef]
- Reccius, C.H.; Mannion, J.T.; Cross, J.D.; Craighead, H.G. Compression and Free Expansion of Single DNA Molecules in Nanochannels. Phys. Rev. Lett. 2005, 95, 268101. [Google Scholar] [CrossRef]
- Köster, S.; Kierfeld, J.; Pfohl, T. Characterization of single semiflexible filaments under geometric constraints. Eur. Phys. J. E 2008, 25, 439–449. [Google Scholar] [CrossRef]
- Kalb, J.; Chakraborty, B. Single polymer confinement in a tube: Correlation between structure and dynamics. J. Chem. Phys. 2009, 130, 025103. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P.; Benková, Z.; Bleha, T. Chain Extension of DNA Confined in Channels. J. Phys. Chem. B 2009, 113, 1843–1851. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P.; Benková, Z.; Bleha, T. Persistence length of DNA molecules confined in nanochannels. Phys. Chem. Chem. Phys. 2010, 12, 8934–8942. [Google Scholar] [CrossRef] [PubMed]
- Burkhardt, T.W.; Yang, Y.; Gompper, G. Fluctuations of a long, semiflexible polymer in a narrow channel. Phys. Rev. E 2010, 82, 041801. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Tree, D.R.; Dorfman, K.D. Simulation of DNA Extension in Nanochannels. Macromolecules 2011, 44, 6594–6604. [Google Scholar] [CrossRef] [PubMed]
- Cacciuto, A.; Luijten, E. Self-Avoiding Flexible Polymers under Spherical Confinement. Nano Lett. 2006, 6, 901–905. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Chakraborty, B. Shapes of semiflexible polymers in confined spaces. Phys. Biol. 2008, 5, 026004. [Google Scholar] [CrossRef]
- Morrison, G.; Thirumalai, D. Semiflexible chains in confined spaces. Phys. Rev. E 2009, 79, 011924. [Google Scholar] [CrossRef]
- Gao, J.; Tang, P.; Yang, Y.; Chen, J.Z.Y. Free energy of a long semiflexible polymer confined in a spherical cavity. Soft Matter 2014, 10, 4674–4685. [Google Scholar] [CrossRef]
- Sakaue, T.; Raphaël, E. Polymer Chains in Confined Spaces and Flow-Injection Problems: Some Remarks. Macromolecules 2006, 39, 2621–2628. [Google Scholar] [CrossRef]
- Randel, R.; Loebl, H.C.; Matthai, C.C. Molecular Dynamics Simulations of Polymer Translocations. Macromol. Theory Simul. 2004, 13, 387–391. [Google Scholar] [CrossRef]
- Sousa, A.F.; Pais, A.A.C.C.; Linse, P. Polymer distribution in connected spherical domains. J. Chem. Phys. 2005, 122, 214902. [Google Scholar] [CrossRef] [PubMed]
- Matysiak, S.; Montesi, A.; Pasquali, M.; Kolomeisky, A.B.; Clementi, C. Dynamics of Polymer Translocation through Nanopores: Theory Meets Experiment. Phys. Rev. Lett. 2006, 96, 118103. [Google Scholar] [CrossRef] [PubMed]
- Cifra, P. Asymmetric bridging of interconnected pores by encased semiflexible macromolecules. J. Chem. Phys. 2006, 124, 024706. [Google Scholar] [CrossRef] [PubMed]
- Gauthier, M.G.; Slater, G.W. Molecular Dynamics simulation of a polymer chain translocating through a nanoscopic pore - Hydrodynamic interactions versus pore radius. Eur. Phys. J. E 2008, 25, 17–23. [Google Scholar] [CrossRef]
- Magill, M.; Falconer, C.; Waller, E.; Wan de Haan, H. Translocation Time through a Nanopore with an Internal Cavity Is Minimal for Polymers of Intermediate Length. Phys. Rev. Lett. 2016, 117, 247802. [Google Scholar] [CrossRef]
- Joo, H.; Kim, J.S. Confinement and partitioning of a single polymer chain in a dense array of nanoposts. Soft Matter 2015, 11, 8262–8272. [Google Scholar] [CrossRef]
- Joo, H.; Kim, J.S. Confinement-driven organization of a histone-complexed DNA molecule in a dense array of nanoposts. Nanoscale 2017, 9, 6391–6398. [Google Scholar] [CrossRef]
- Benková, Z.; Rišpanová, L.; Cifra, P. Structural behavior of a semiflexible polymer chain in an array of nanoposts. Polymers 2017, 9, 313. [Google Scholar] [CrossRef]
- Benková, Z.; Rišpanová, L.; Cifra, P. Effect of chain stiffness for semiflexible macromolecules in array of cylindrical nanoposts. J. Chem. Phys. 2017, 147, 134907. [Google Scholar] [CrossRef]
- Rišpanová, L.; Benková, Z.; Cifra, P. Block Copolymer of Flexible and Semi-Flexible Block Confined in Nanopost Array. Polymers 2018, 10, 1301. [Google Scholar] [CrossRef] [PubMed]
- Duke, T.A.J.; Austin, R.H.; Cox, E.C.; Chan, S.S. Pulsed-field electrophoresis in microlithographic arrays. Electrophoresis 1996, 17, 1075–1079. [Google Scholar] [CrossRef] [PubMed]
- Bakajin, O.B.; Duke, T.A.J.; Chou, C.F.; Chan, S.S.; Austin, R.H.; Cox, E.C. Electrohydrodynamic Stretching of DNA in Confined Environments. Phys. Rev. Lett. 1998, 80, 2737–2740. [Google Scholar] [CrossRef]
- Doyle, P.S.; Bibette, J.; Bancau, A.; Viovy, J.-L. Self-Assembled Magnetic Matrices for DNA Separation Chips. Science 2002, 295, 2237. [Google Scholar] [CrossRef]
- Kaji, N.; Okamoto, Y.; Tokeshi, M.; Baba, Y. Nanopillar, nanoball, and nanofibers for highly efficient analysis of biomolecules. Chem. Soc. Rev. 2010, 39, 948–956. [Google Scholar] [CrossRef]
- Todorov, I.T.; Smith, W.; Trachenko, K.; Dove, M.T. DL_POLY_3: New dimensions in molecular dynamics simulations via massive parallelism. J. Mater. Chem. 2006, 16, 1911–1918. [Google Scholar] [CrossRef]
- Brochard, F.; de Gennes, P.G. Dynamics of confined polymer chains. J. Chem. Phys. 2006, 67, 52–56. [Google Scholar] [CrossRef]
- Daoud, M.; de Gennes, P.G. Statistics of macromolecular solutions trapped in small pores. J. Phys. France 1977, 38, 85–93. [Google Scholar] [CrossRef]
- Yang, Y.; Burkhardt, T.W.; Gompper, G. Free energy and extension of a semiflexible polymer in cylindrical confining geometries. Phys. Rev. E 2007, 76, 011804. [Google Scholar] [CrossRef]
- Cifra, P.; Teraoka, I. Partitioning of polymer chains in solution with a square channel: Lattice Monte Carlo simulations. Polymer 2002, 43, 2409–2415. [Google Scholar] [CrossRef]
- Brochard-Wyart, F.; Tanaka, T.; Borghi, N.; de Gennes, P.G. Semiflexible Polymers Confined in Soft Tubes. Langmuir 2005, 21, 4144–4148. [Google Scholar] [CrossRef] [PubMed]
- Odijk, T. Scaling theory of DNA confined in nanochannels and nanoslits. Phys. Rev. E 2008, 77, 060901. [Google Scholar] [CrossRef] [PubMed]
- Benková, Z.; Cifra, P. Simulation of Semiflexible Cyclic and Linear Chains Moderately and Strongly Confined in Nanochannels. Macromolecules 2012, 45, 2597–2608. [Google Scholar] [CrossRef]
- Hsu, H.-P.; Paul, W.; Binder, K. Scattering function of semiflexible polymer chains under good solvent conditions. J. Chem. Phys. 2012, 137, 174902. [Google Scholar] [CrossRef] [PubMed]
- Benková, Z.; Námer, P.; Cifra, P. Stripe to slab con fi nement for the linearization of macromolecules in nanochannels. Soft Matter 2015, 11, 2279–2289. [Google Scholar] [CrossRef]
- Benková, Z.; Námer, P.; Cifra, P. Comparison of a stripe and slab confinement for ring and linear macromolecules in nanochannel. Soft Matter 2016, 12, 8425–8439. [Google Scholar] [CrossRef]
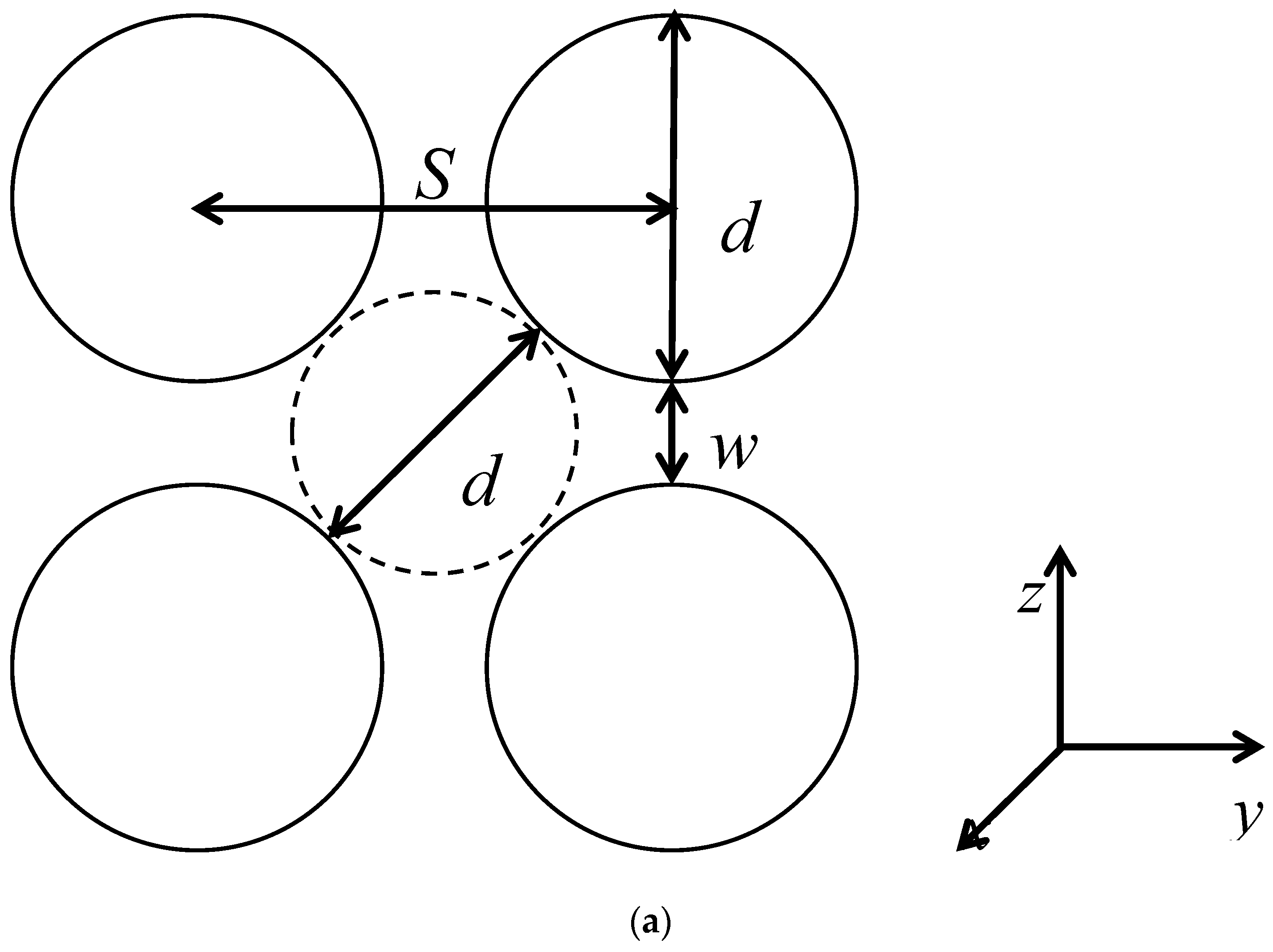

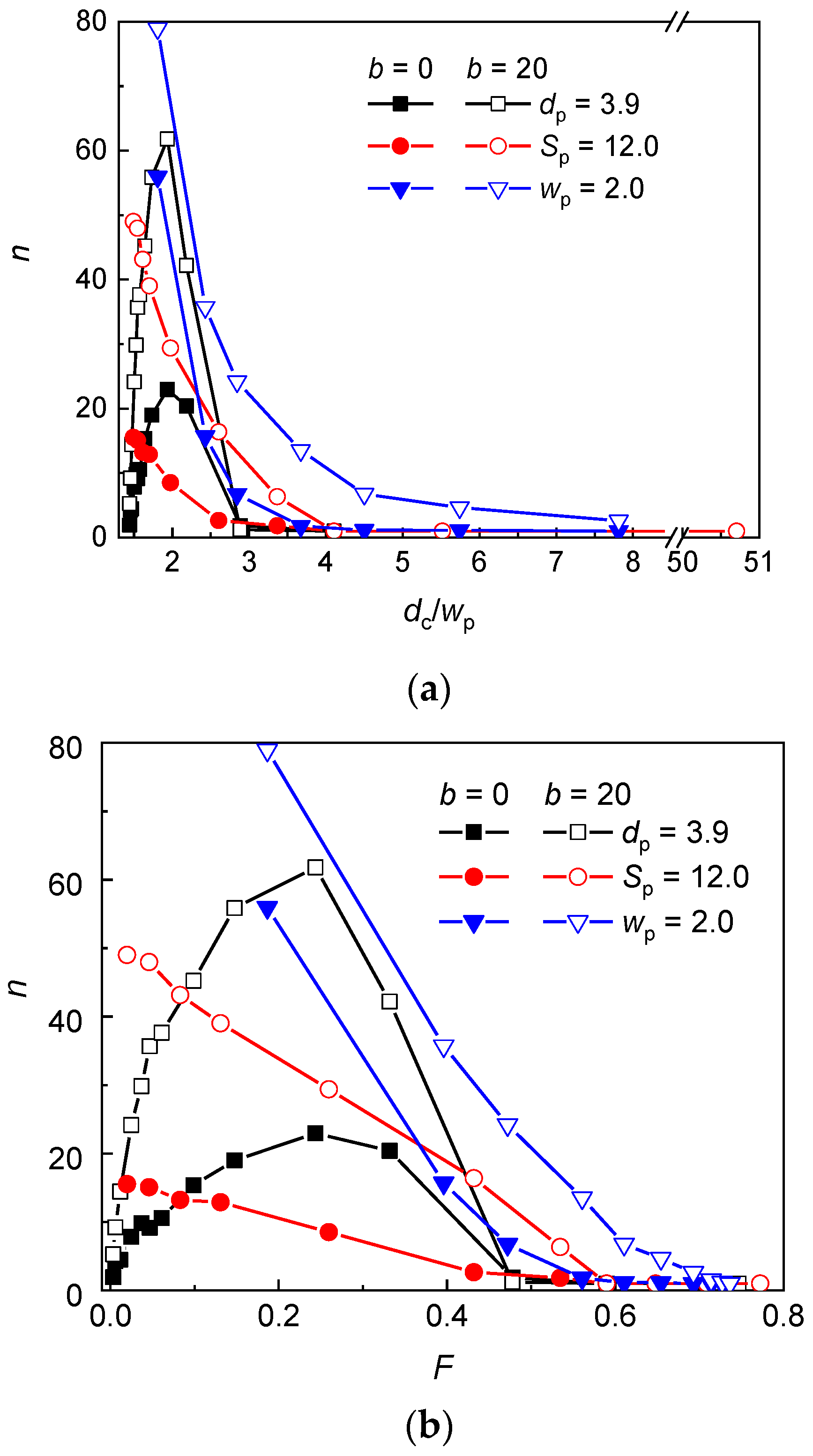
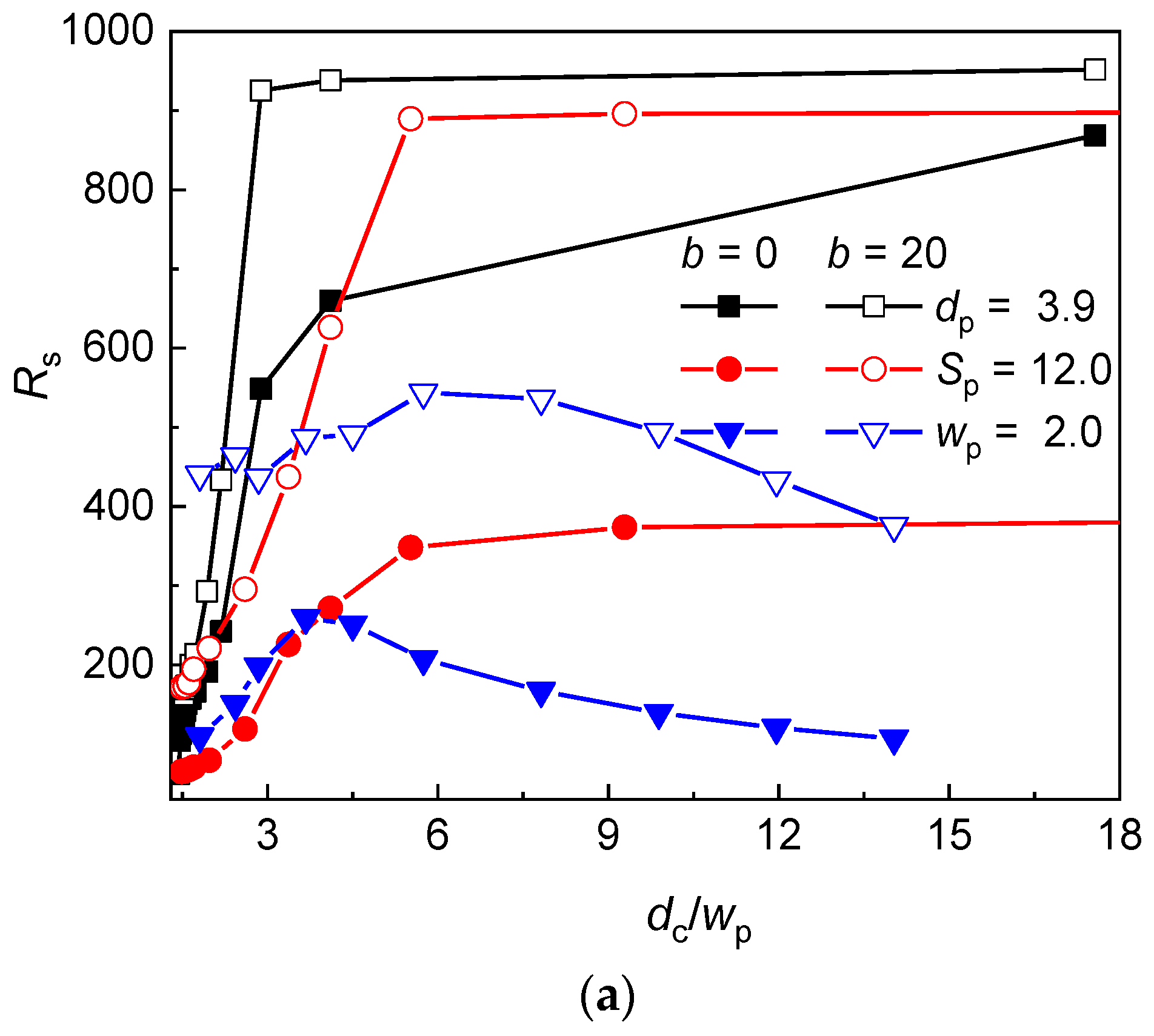
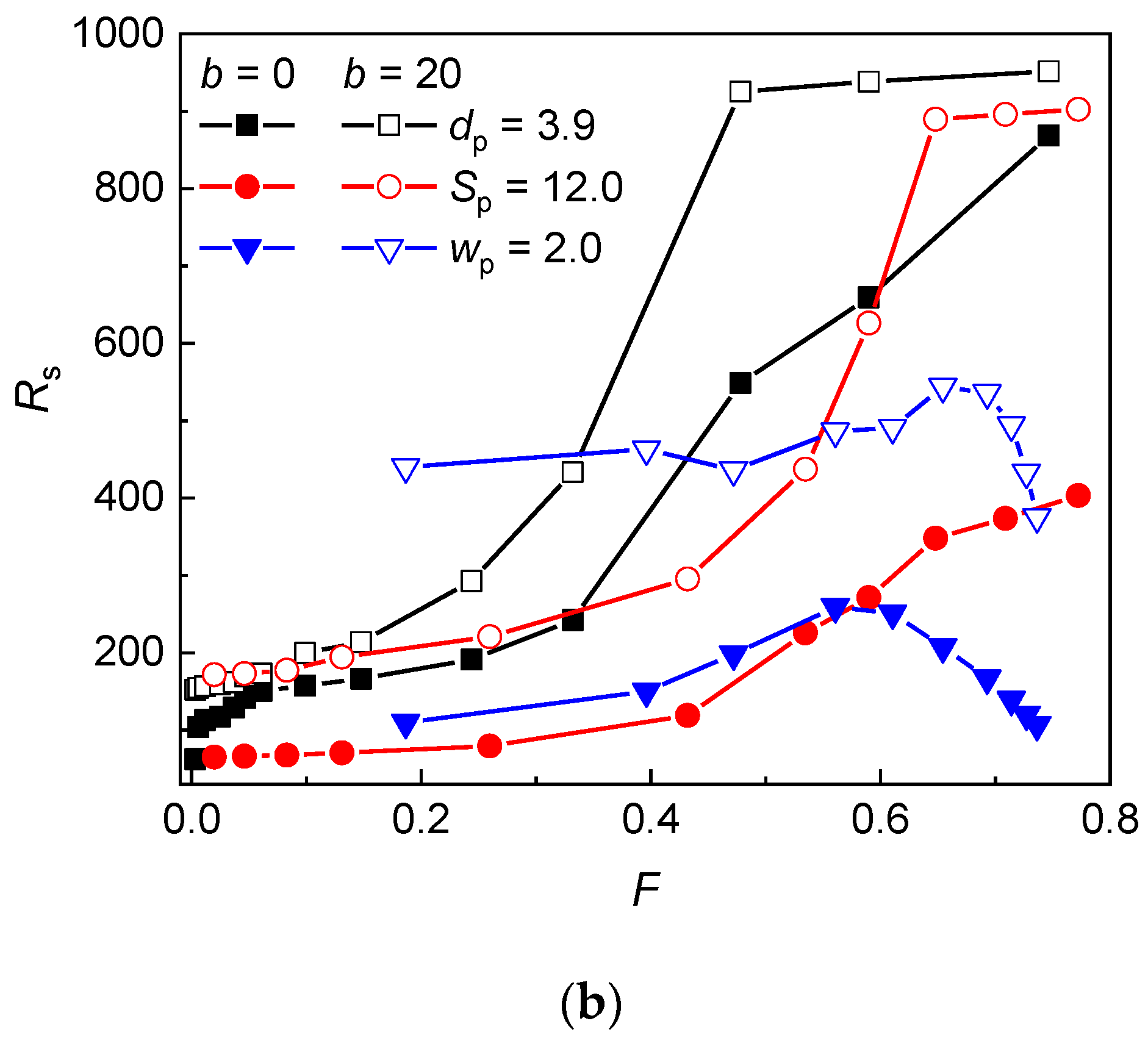

| dp = 3.9 | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Sp | wp | dc | dc/wp | F | Sp | wp | dc | dc/wp | F |
| 4.0 | 0.1 | 1.757 | 17.569 | 0.747 | 14.0 | 10.1 | 15.899 | 1.574 | 0.061 |
| 4.5 | 0.6 | 2.464 | 4.107 | 0.590 | 16.0 | 12.1 | 18.727 | 1.548 | 0.047 |
| 5.0 | 1.1 | 3.171 | 2.883 | 0.478 | 18.0 | 14.1 | 21.556 | 1.529 | 0.037 |
| 6.0 | 2.1 | 4.585 | 2.183 | 0.332 | 22.0 | 18.1 | 27.213 | 1.503 | 0.025 |
| 7.0 | 3.1 | 5.999 | 1.935 | 0.244 | 32.0 | 28.1 | 41.355 | 1.472 | 0.012 |
| 9.0 | 5.1 | 8.828 | 1.731 | 0.147 | 45.0 | 41.1 | 59.740 | 1.454 | 0.006 |
| 11.0 | 7.1 | 11.656 | 1.642 | 0.099 | 60.0 | 56.1 | 80.953 | 1.443 | 0.003 |
| Interstitial Volume | Passage Aperture | Lqc/Lqs |
|---|---|---|
| de Gennes regime | de Gennes regime | |
| de Gennes regime | Odijk regime | |
| Odijk regime | Odijk regime |
| dp = 3.9 | Sp = 12 | wp = 2 | ||||||
|---|---|---|---|---|---|---|---|---|
| dc/wp | wp | dc | dc/wp | wp | dc | dc/wp | wp | dc |
| 1.443 | 56.1 | 80.953 | 1.492 | 10.1 | 15.071 | 1.808 | 2.0 | 3.615 |
| 1.454 | 41.1 | 59.740 | 1.546 | 9.1 | 14.071 | 2.429 | 2.0 | 4.858 |
| 1.472 | 28.1 | 41.355 | 1.614 | 8.1 | 13.071 | 2.843 | 2.0 | 5.687 |
| 1.504 | 18.1 | 27.213 | 1.700 | 7.1 | 12.071 | 3.672 | 2.0 | 7.343 |
| 1.529 | 14.1 | 21.556 | 1.975 | 5.1 | 10.071 | 4.500 | 2.0 | 9.000 |
| 1.548 | 12.1 | 18.727 | 2.603 | 3.1 | 8.071 | 5.743 | 2.0 | 11.485 |
| 1.574 | 10.1 | 15.899 | 3.367 | 2.1 | 7.071 | 7.814 | 2.0 | 15.628 |
| 1.642 | 7.1 | 11.656 | 4.107 | 1.6 | 6.571 | 9.885 | 2.0 | 19.770 |
| 1.731 | 5.1 | 8.828 | 5.519 | 1.1 | 6.071 | 11.956 | 2.0 | 23.912 |
| 1.935 | 3.1 | 5.999 | 9.284 | 0.6 | 5.571 | 14.027 | 2.0 | 28.054 |
| 2.183 | 2.1 | 4.585 | 50.706 | 0.1 | 5.071 | |||
| 2.882 | 1.1 | 3.171 | ||||||
| 4.107 | 0.6 | 2.464 | ||||||
| 17.569 | 0.1 | 1.757 | ||||||
| Sp | 6 | 7 | 9 | 11 |
|---|---|---|---|---|
| q | 1.02 | 0.86 | 0.68 | 0.56 |
| 2π/q | 6.16 | 7.31 | 9.24 | 11.22 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Benková, Z.; Rišpanová, L.; Cifra, P. Conformation of Flexible and Semiflexible Chains Confined in Nanoposts Array of Various Geometries. Polymers 2020, 12, 1064. https://doi.org/10.3390/polym12051064
Benková Z, Rišpanová L, Cifra P. Conformation of Flexible and Semiflexible Chains Confined in Nanoposts Array of Various Geometries. Polymers. 2020; 12(5):1064. https://doi.org/10.3390/polym12051064
Chicago/Turabian StyleBenková, Zuzana, Lucia Rišpanová, and Peter Cifra. 2020. "Conformation of Flexible and Semiflexible Chains Confined in Nanoposts Array of Various Geometries" Polymers 12, no. 5: 1064. https://doi.org/10.3390/polym12051064
APA StyleBenková, Z., Rišpanová, L., & Cifra, P. (2020). Conformation of Flexible and Semiflexible Chains Confined in Nanoposts Array of Various Geometries. Polymers, 12(5), 1064. https://doi.org/10.3390/polym12051064





